Design and Performance Assessment of Multi-Use Offshore Tension Leg Platform Equipped with an Embedded Wave Energy Converter System
Abstract
1. Introduction
2. Methods and Materials
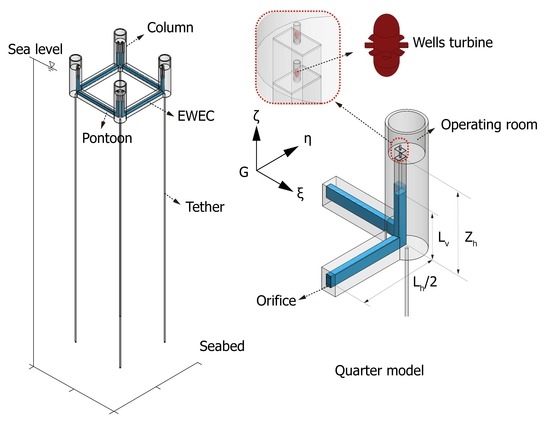
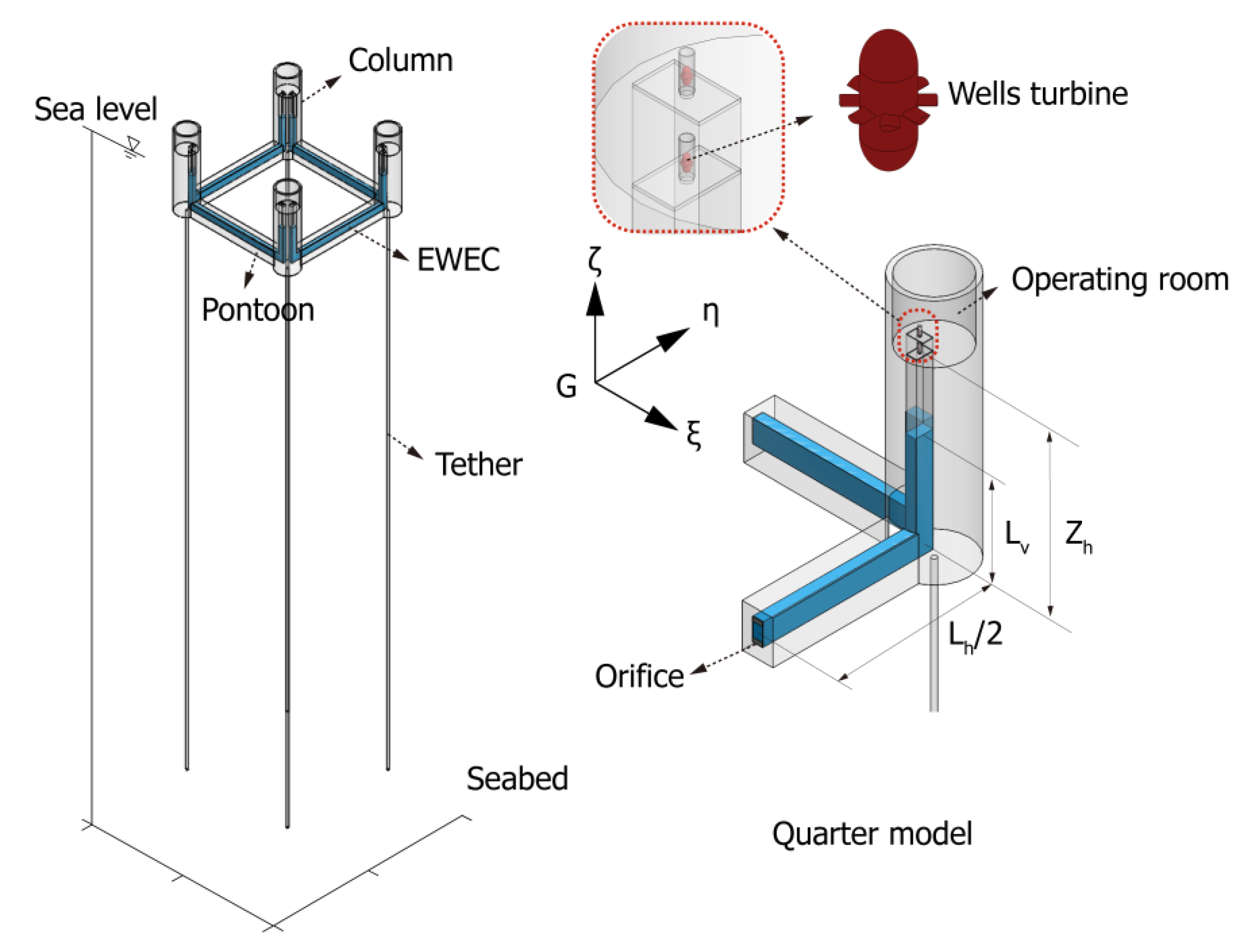
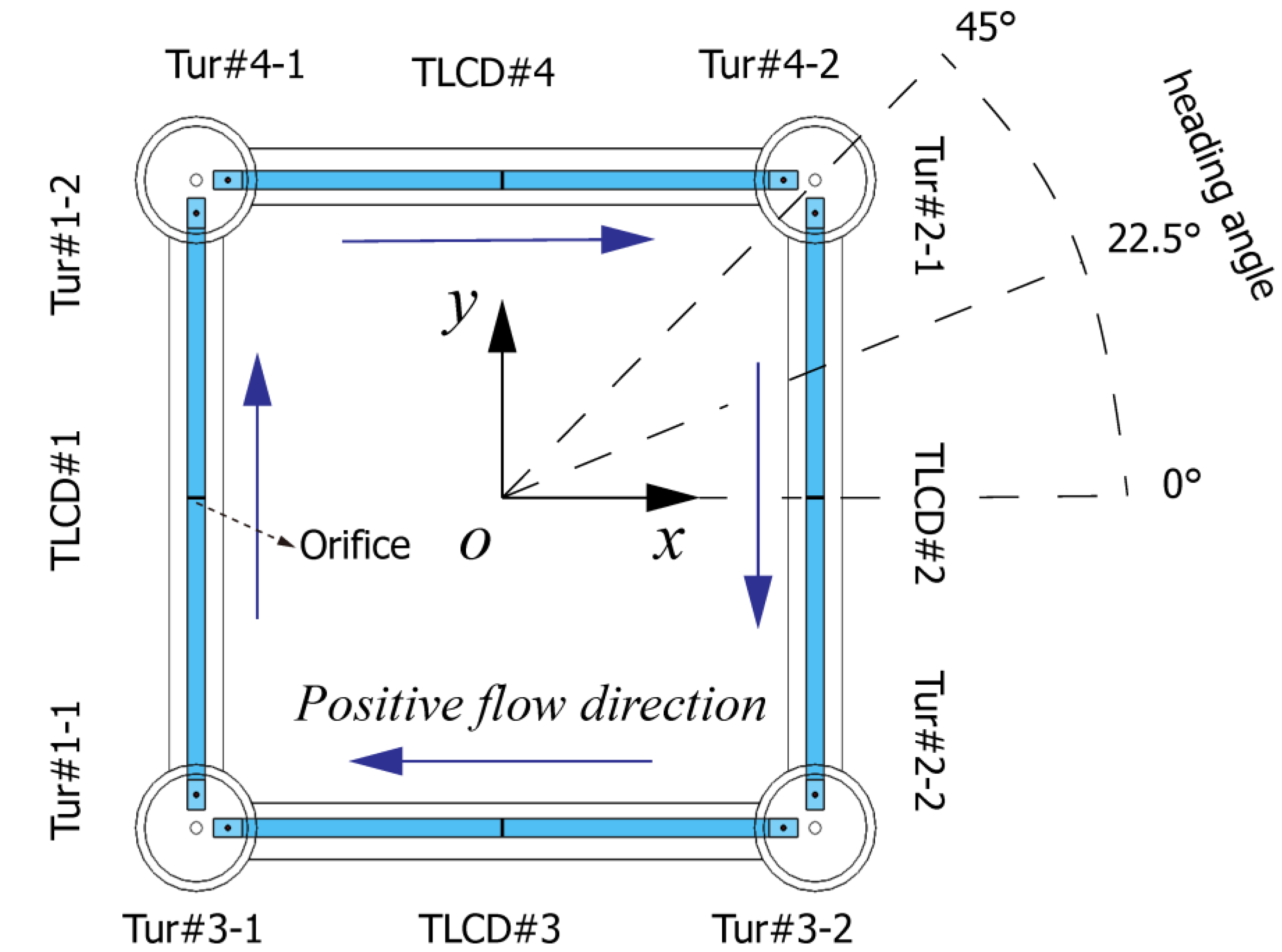
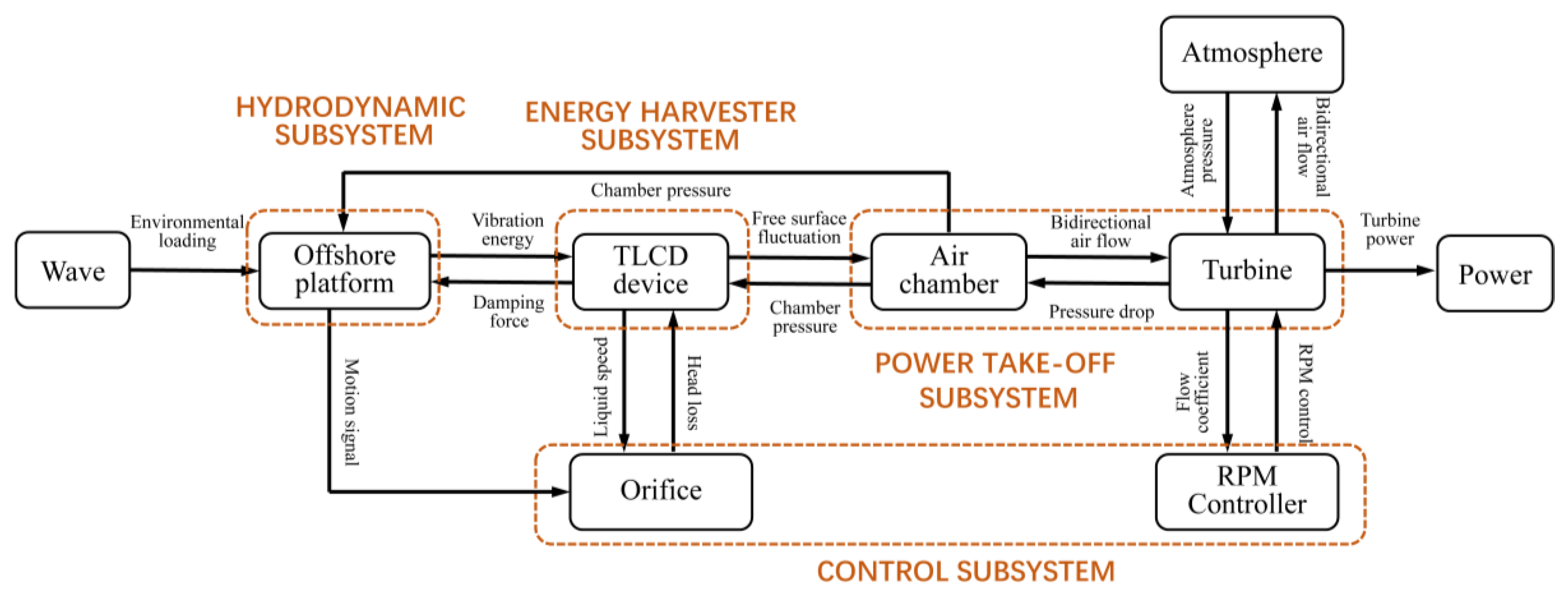
2.1. General Description of TLP-EWEC System
- The hydrodynamic subsystem is the TLP hull, which experiences the environment load and, hence, the vibrations.
- The energy harvester subsystem consists of the TLCDs that absorb the hull motion energy based on the oscillations of the working liquid within them.
- The PTO subsystem consists of the air chamber and self-rectifying turbines installed at the two ends of the TLCDs. This chamber-turbine module is the same as that used in common OWCs (i.e., M-OWCs).
- The control subsystem is the intelligent part of the system, as it is responsible for the control of the TLP-EWEC system and its measurements. It mainly consists of an orifice and turbine controller, the relevant components for the automation and electromechanical processes.
2.2. Governing Equations of Coupled TLP-EWEC System
2.2.1. Equations for Hydrodynamic Subsystem
2.2.2. Equations for Energy Harvester Subsystem
2.2.3. Equations for Power Take-Off (PTO) Subsystem
3. Results
3.1. Hydrodynamic Validation and Target Design
3.2. Hydrodynamic Performance Analysis
3.2.1. Comparison with the ISSC TLP System
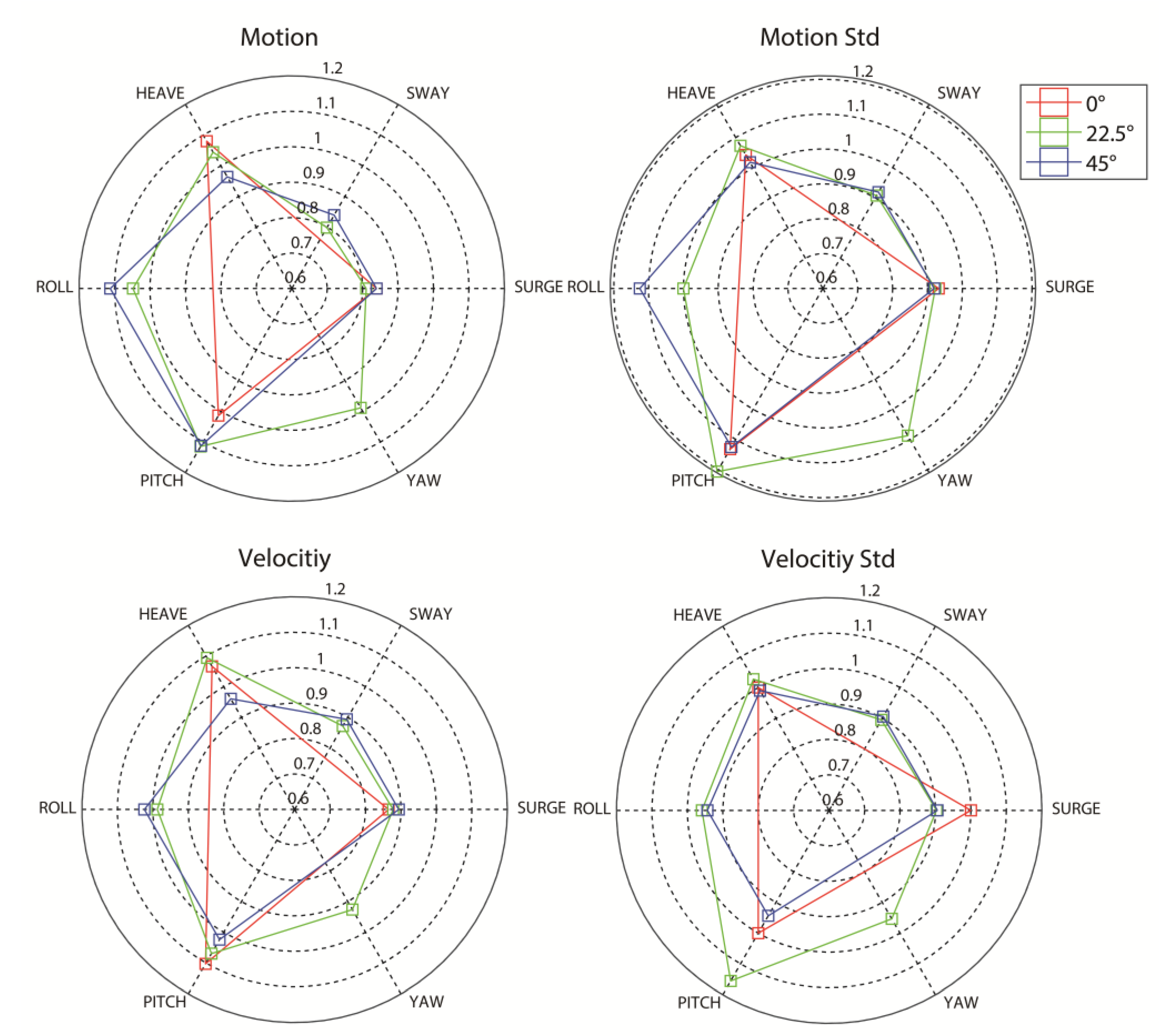
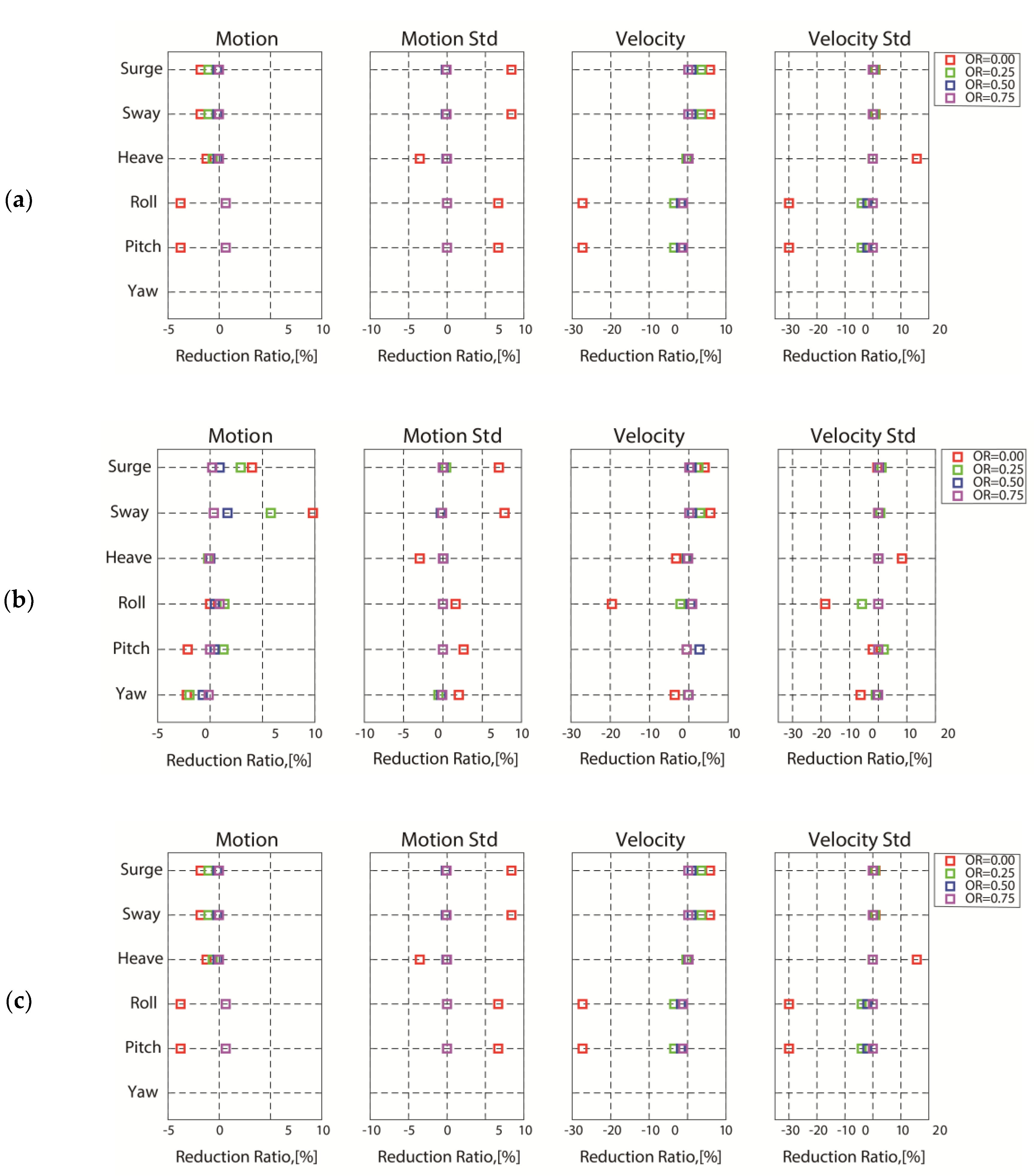
3.2.2. Hydrodynamic Stability for Different Orifice Ratios
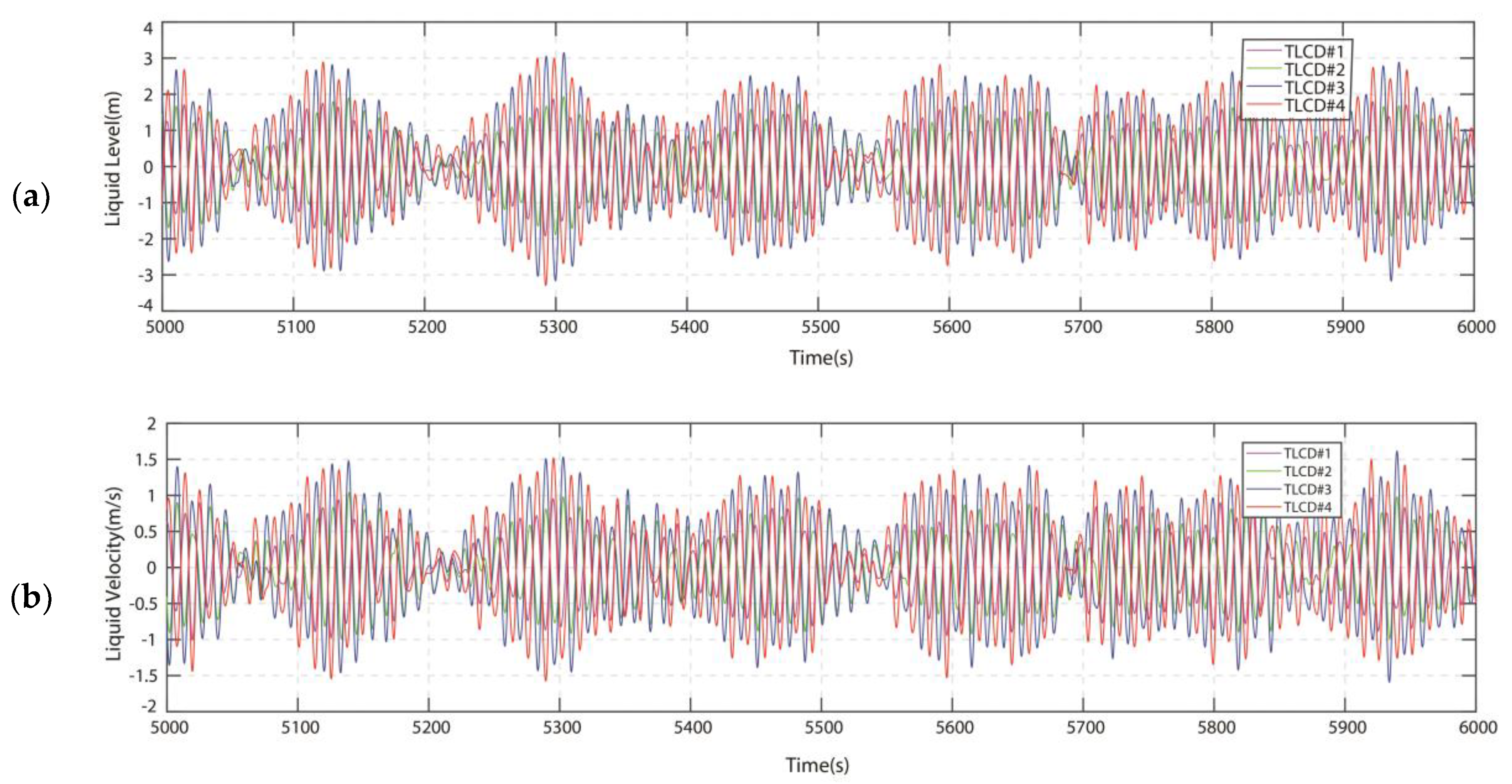
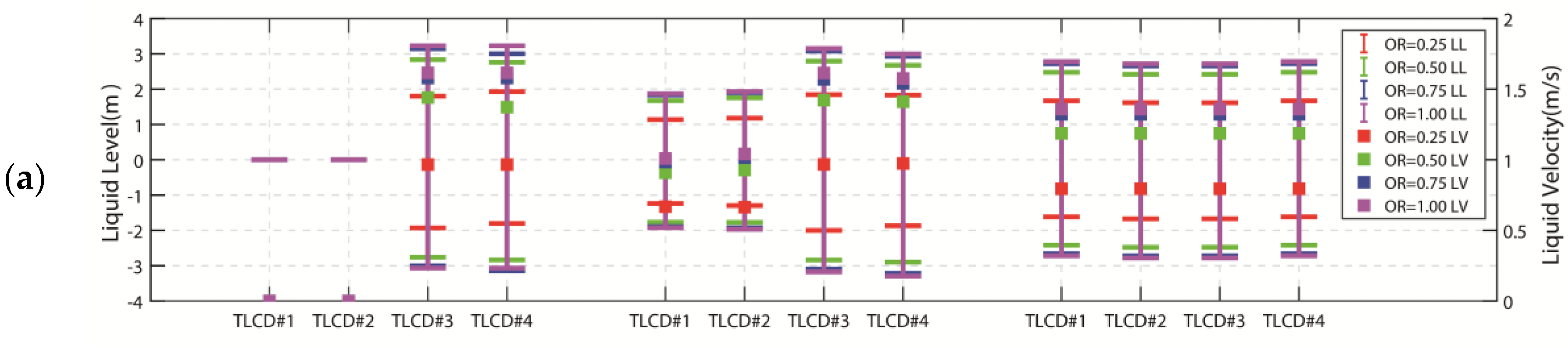
3.3. Generating Capacity of TLP-EWEC System
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| OWC | Oscillating water column | DOF | Degree of freedom |
| PTO | Power take-off | MTLCD | Multi-tuned liquid column damper |
| M-OWCs | Multi-oscillating water columns | OR | Orifice ratio |
| EWEC | Embedded wave energy converter | C.G. | Center of gravity |
| TLP | Tension leg platform | ISSC | The International Ship and Offshore Structures Congress |
| TLCD | Tuned liquid column damper | HA | Heading angle |
Nomenclature
| , | Global fixed coordinate system and local coordinate system |
| , , , , , | Surge, sway, heave, roll, pitch, and yaw motion |
| Relative motion of liquid in TLCD#j | |
| Vector of the TLP-EWEC system coordinates | |
| Vector of external forces | |
| , , | Kinetic energies of TLP-EWEC, TLP, and TLCD#j |
| , , | Potential energies of TLP-EWEC, TLP, and TLCD#j |
| , | Final mass matrix of hull and TLCD#j |
| , , | Final force vector of hull, TLCD#j, and chamber#k |
| Body mass of a TLP | |
| Density of sea water | |
| , , | Moments of inertia of a TLP |
| , | External forces and moments on a TLP |
| (, , ), (, , ) | Axial base vectors of and |
| Transformation matrix | |
| Coordinates of microscale liquid column in | |
| , | Relative motion and absolute velocity of microscale liquid column |
| Angular velocity of hull | |
| Total head resistance of TLCD#j | |
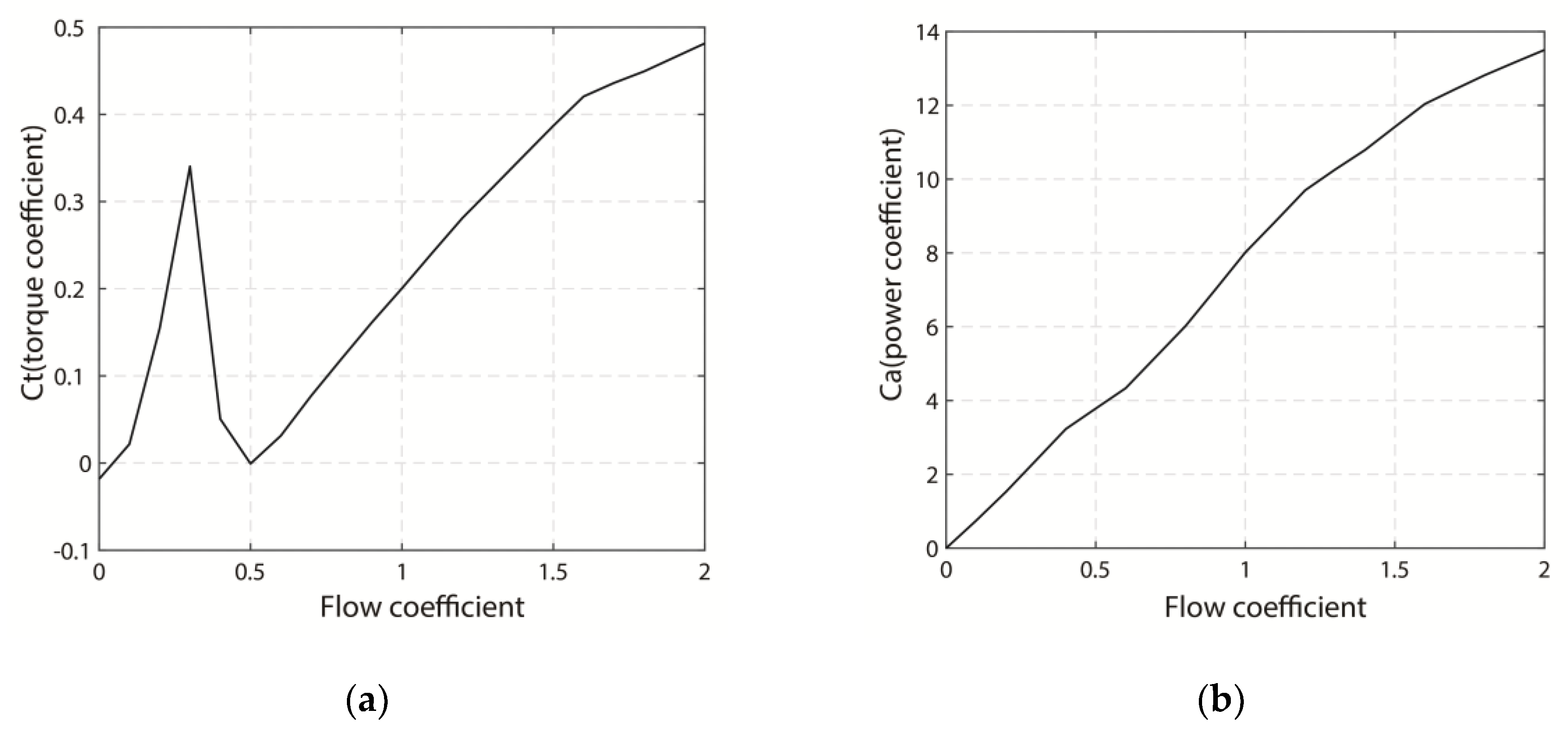
| , , | Pressure drop across the rotor, turbine torque, and turbine power |
| , , | Turbine constant, turbine cross-section area, and turbine mean radius |
| , , | Airflow velocity, turbine’s angular velocity, and flow rate |
| , , , | Resultant forces acting on the TLP and TLCD#j due to air pressure |
| , , , | Resultant moments acting on the TLP and TLCD#j due to air pressure |
| , | Turbine power coefficient and torque coefficient |
| Force on TLCD#j result from right and left chamber pressure difference | |
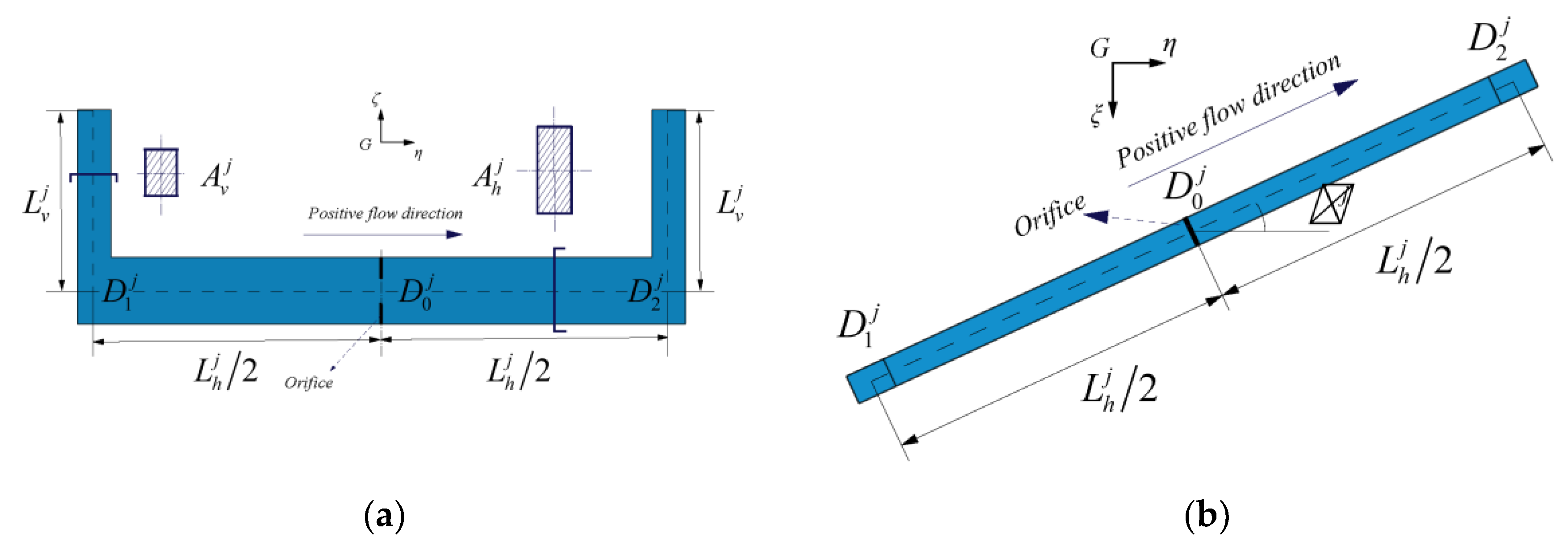
| , | Length and cross area of the horizontal liquid column |
| , | Length and cross area of the vertical liquid column |
| ,, | Peak wave period, significant wave height, and peak enhancement factor |
Appendix A
References
- López, I.; Andreu, J.; Ceballos, S.; De Alegría, I.M.; Kortabarria, I. Review of wave energy technologies and the necessary power-equipment. Renew. Sustain. Energy Rev. 2013, 27, 413–434. [Google Scholar] [CrossRef]
- Salter, S.H. Wave power. Nature 1974, 249, 720–724. [Google Scholar] [CrossRef]
- Pecher, A.; Kofoed, J.P. Handbook of Ocean Wave Energy; Springer International Publishing: Berlin, Germany, 2017; 20p. [Google Scholar]
- Cui, Y.; Liu, Z.; Zhang, X.; Xu, C. Review of CFD studies on axial-flow self-rectifying turbines for OWC wave energy conversion. Ocean Eng. 2019, 175, 80–102. [Google Scholar] [CrossRef]
- Salter, S.H.; Taylor, J.R.M.; Caldwell, N.J. Power conversion mechanisms for wave energy. Proc. Inst. Mech. Eng. Part M J. Eng. Marit. Environ. 2002, 216, 1–27. [Google Scholar] [CrossRef]
- Khan, N.; Kalair, A.; Abas, N.; Haider, A. Review of ocean tidal, wave and thermal energy technologies. Renew. Sustain. Energy Rev. 2017, 72, 590–604. [Google Scholar] [CrossRef]
- Melikoglu, M. Current status and future of ocean energy sources: A global review. Ocean Eng. 2017, 148, 563–573. [Google Scholar] [CrossRef]
- Gouaud, F.; Rey, V.; Piazzola, J.; Van Hooff, R. Experimental study of the hydrodynamic performance of an onshore wave power device in the presence of an underwater mound. Coast. Eng. 2010, 57, 996–1005. [Google Scholar] [CrossRef]
- López, I.; Pereiras, B.; Castro, F.; Iglesias, G. Performance of OWC wave energy converters: Influence of turbine damping and tidal variability. Int. J. Energy Res. 2015, 39, 472–483. [Google Scholar] [CrossRef]
- Ning, D.Z.; Wang, R.Q.; Zou, Q.P.; Teng, B. An experimental investigation of hydrodynamics of a fixed OWC Wave Energy Converter. Appl. Energy 2016, 168, 636–648. [Google Scholar] [CrossRef]
- Falcão, A.F.O.; Henriques, J.C.C. Oscillating-water-column wave energy converters and air turbines: A review. Renew Energy 2016, 85, 1391–1424. [Google Scholar] [CrossRef]
- Elhanafi, A.; Kim, C.J. Experimental and numerical investigation on wave height and power take–off damping effects on the hydrodynamic performance of an offshore–stationary OWC wave energy converter. Renew. Energy 2018, 125, 518–528. [Google Scholar] [CrossRef]
- Sheng, W. Motion and performance of BBDB OWC wave energy converters: I, hydrodynamics. Renew. Energy 2019, 138, 106–120. [Google Scholar] [CrossRef]
- Doyle, S.; Aggidis, G.A. Development of multi-oscillating water columns as wave energy converters. Renew. Sustain. Energy Rev. 2019, 107, 75–86. [Google Scholar] [CrossRef]
- Dhanak, M.R.; Xiros, N.I. Springer Handbook of Ocean Engineering; Springer: New York, NY, USA, 2016; 747p. [Google Scholar]
- Zeng, X.H.; Shen, X.P.; Wu, Y.X. Governing equations and numerical solutions of tension leg platform with finite amplitude motion. Appl. Math. Mech. 2007, 28, 37–49. [Google Scholar] [CrossRef]
- Zeng, X.; Liu, J.; Liu, Y.; Wu, Y. Parametric studies of tension leg platform with large amplitude motions. In Proceedings of the International Offshore and Polar Engineering Conference, Lisbon, Portugal, 1–6 July 2007; pp. 202–209. [Google Scholar]
- Koundouri, P.; Osiel, G. Investment Assessment of Multi-Use Offshore Platforms: Methodology and Applications. In The Ocean of Tomorrow; Springer International Publishing: Berlin, Germany, 2017; Volume 1. [Google Scholar]
- The Maribe H2020 Project. Available online: https://ec.europa.eu/research/participants/portal/desktop/en/opportunities/h2020/topics/bg-05-2014.html (accessed on 13 May 2020).
- Space@Sea. Available online: https://spaceatsea-project.eu/ (accessed on 13 May 2020).
- The Blue Growth Farm. Available online: http://www.thebluegrowthfarm.eu/ (accessed on 13 May 2020).
- Dalton, G.; Bardócz, T.; Blanch, M.; Campbell, D.; Johnson, K.; Lawrence, G.; Lilas, T.; Friis-Madsen, E.; Neumann, F.; Nikitas, N.; et al. Feasibility of investment in Blue Growth multiple-use of space and multi-use platform projects; results of a novel assessment approach and case studies. Renew. Sustain. Energy Rev. 2019, 107, 338–359. [Google Scholar] [CrossRef]
- Oliveira-Pinto, S.; Rosa-Santos, P.; Taveira-Pinto, F. Electricity supply to offshore oil and gas platforms from renewable ocean wave energy: Overview and case study analysis. Energy Convers. Manag. 2019, 186, 556–569. [Google Scholar] [CrossRef]
- El-Khoury, O.; Adeli, H. Recent Advances on Vibration Control of Structures under Dynamic Loading. Arch. Comput. Methods Eng. 2013, 20, 353–360. [Google Scholar] [CrossRef]
- Coudurier, C.; Lepreux, O.; Petit, N. Passive and semi-active control of an offshore floating wind turbine using a tuned liquid column damper. IFAC-PapersOnLine 2015, 48, 241–247. [Google Scholar] [CrossRef]
- Alkmim, M.H.; De Morais, M.V.G.; Fabro, A.T. Vibration Reduction of Wind Turbines Using Tuned Liquid Column Damper Using Stochastic Analysis. J. Phys. Conf. Ser. 2016, 744, 12178. [Google Scholar] [CrossRef]
- O’Donnell, D.; Murphy, J.; Desmond, C.; Jaksic, V.; Pakrashi, V. Tuned Liquid Column Damper based Reduction of Dynamic Responses of Scaled Offshore Platforms in Different Ocean Wave Basins. J. Phys. Conf. Ser. 2017, 842, 012–043. [Google Scholar] [CrossRef]
- Kandasamy, R.; Cui, F.; Townsend, N.; Foo, C.C.; Guo, J.; Shenoi, A.; Xiong, Y. A review of vibration control methods for marine offshore structures. Ocean Eng. 2016, 127, 279–297. [Google Scholar] [CrossRef]
- Zhang, B.L.; Han, Q.L.; Zhang, X.M. Recent advances in vibration control of offshore platforms. Nonlinear Dyn. 2017, 89, 755–771. [Google Scholar] [CrossRef]
- Lee, H.H.; Wong, S.H.; Lee, R.S. Response mitigation on the offshore floating platform system with tuned liquid column damper. Ocean Eng. 2006, 33, 1118–1142. [Google Scholar] [CrossRef]
- Lee, H.H.; Juang, H.H. Experimental study on the vibration mitigation of offshore tension leg platform system with UWTLCD. Smart Struct. Syst. 2012, 9, 71–104. [Google Scholar] [CrossRef]
- Shabana Ahmed, A. Dynamics of Multibody Systems, 3rd ed.; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Kim, D.I.; Min, K.W.; Park, J.H.; Lee, H.R.; Kim, J.K.; Hwang, K.S.; Gil, Y.S. Tunable tuned liquid column dampers with multi-cells for wind vibration control of tall buildings. Proc. SPIE Int. Soc. Opt. Eng. 2012, 8345, 67. [Google Scholar]
- Dormand, J.R.; Prince, P.J. A family of embedded Runge-Kutta formulae. J. Comput. Appl. Math. 1980, 6, 19–26. [Google Scholar] [CrossRef]
- Chandrasekaran, S. Dynamic Analysis and Design of Offshore Structures; Springer: New Delhi, India, 2015; p. 5. [Google Scholar]
- Yalla, S.K.; Kareem, A. Optimum absorber parameters for tuned liquid column dampers. J. Struct. Eng. 2000, 126, 906–915. [Google Scholar] [CrossRef]
- El Marjani, A.; Castro Ruiz, F.; Rodriguez, M.A. Numerical modelling in wave energy conversion systems. Energy 2008, 33, 1246–1253. [Google Scholar] [CrossRef]
- Henriques, J.C.C.; Portillo, J.C.C.; Sheng, W. Dynamics and control of air turbines in oscillating-water-column wave energy converters: Analyses and case study. Renew. Sustain. Energy Rev. 2019, 112, 571–589. [Google Scholar] [CrossRef]
- Spanos, P.D.; Strati, F.M.; Malara, G. An approach for non-linear stochastic analysis of U-shaped OWC wave energy converters. Prob. Eng. Mech. 2018, 54, 44–52. [Google Scholar] [CrossRef]
- Liu, C. A tunable resonant oscillating water column wave energy converter. Ocean Eng. 2016, 116, 82–89. [Google Scholar] [CrossRef]
- Bailey, H.; Robertson, B.R.; Buckham, B.J. Wave-to-wire simulation of a floating oscillating water column wave energy converter. Ocean Eng. 2016, 125, 248–260. [Google Scholar] [CrossRef]
- Amundarain, M.; Alberdi, M.; Garrido, A.J. Neural rotational speed control for wave energy converters. Int. J. Control. 2011, 84, 293–309. [Google Scholar] [CrossRef]
- Amundarain, M.; Alberdi, M.; Garrido, A.J. Modeling and simulation of wave energy generation plants: Output power control. IEEE Trans. Ind. Electron. 2011, 58, 105–117. [Google Scholar] [CrossRef]
- Kim, M.H. Hydrodynamics of Offshore Structures. Dev. Offshore Eng. 1999, 336–381. [Google Scholar]
- Finnegan, W.; Goggins, J. Numerical simulation of linear water waves and wavestructure interaction. Ocean Eng. 2012, 43, 23–31. [Google Scholar] [CrossRef]
- Zeng, X.; Yu, Y.; Zhang, L. A New Energy-Absorbing Device for Motion Suppression in Deep-Sea Floating Platforms. Energies 2014, 8, 111–132. [Google Scholar] [CrossRef]




| Parameter | ISSC TLP | TLP-EWEC |
|---|---|---|
| Column spacing between centers, (m) | 86.25 | 86.25 |
| Column diameter (m) | 16.88 | 16.88 |
| Pontoon width, (m) | 7.50 | 7.50 |
| Pontoon height, (m) | 10.50 | 10.50 |
| Draft, (m) | 35.00 | 40.84 |
| Displacement (m) | 5.346 × 105 | 5.346 × 105 |
| Total tether pretension (m) | 1.373 × 105 | 1.373 × 105 |
| Platform mass, (m) | 40.5 × 106 | 40.5 × 106 |
| Roll mass moment of Inertia, (m) | 82.37 × 109 | 82.37 × 109 |
| Pitch mass moment of Inertia, (m) | 82.37 × 109 | 82.37 × 109 |
| Yaw mass moment of Inertia, (m) | 98.07 × 109 | 98.07 × 109 |
| Vertical position of COG above Keel, (m) | 38.0 | 38.0 |
| Length of Mooring tethers, L (m) | 415.0 | 409.16 |
| Vertical stiffness of combined tethers, ES/L (kN/m) | 0.813 × 106 | 0.813 × 106 |
| Spectrum | |||
|---|---|---|---|
| JONSWAP | 2.4 | 6.5 m | 12.3 s |
| Parameter | Value |
|---|---|
| Length of the horizontal liquid column, | 75 |
| Height of vertical liquid column, | 7.6 |
| Cross area of the horizontal liquid column, (m) | 15 |
| Cross area of the vertical liquid column, (m) | 12 |
| -coordinate of those TLCDs, (m) | {−43.125, 43.125, 0, 0} |
| -coordinate of those TLCDs, (m) | {0, 0, −43.125, 43.125} |
| -coordinate of those TLCDs, (m) | {−32.75, −32.75, −32.75, −32.75} |
| -laying angle of those TLCDs, | {π/2, −π/2, π, 0} |
| Turbine Constant, K | Cross-Sectional Area, a | Turbine Radius, r | |
|---|---|---|---|
| 0.7079 | 1.1763 m2 | 0.7285 m | 60 m |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, J.; Li, Z.; Yu, Y.; Hao, S.; Fu, Y.; Cui, Y.; Xu, L.; Wu, H. Design and Performance Assessment of Multi-Use Offshore Tension Leg Platform Equipped with an Embedded Wave Energy Converter System. Energies 2020, 13, 3991. https://doi.org/10.3390/en13153991
Yu J, Li Z, Yu Y, Hao S, Fu Y, Cui Y, Xu L, Wu H. Design and Performance Assessment of Multi-Use Offshore Tension Leg Platform Equipped with an Embedded Wave Energy Converter System. Energies. 2020; 13(15):3991. https://doi.org/10.3390/en13153991
Chicago/Turabian StyleYu, Jianxing, Zhenmian Li, Yang Yu, Shuai Hao, Yiqin Fu, Yupeng Cui, Lixin Xu, and Han Wu. 2020. "Design and Performance Assessment of Multi-Use Offshore Tension Leg Platform Equipped with an Embedded Wave Energy Converter System" Energies 13, no. 15: 3991. https://doi.org/10.3390/en13153991
APA StyleYu, J., Li, Z., Yu, Y., Hao, S., Fu, Y., Cui, Y., Xu, L., & Wu, H. (2020). Design and Performance Assessment of Multi-Use Offshore Tension Leg Platform Equipped with an Embedded Wave Energy Converter System. Energies, 13(15), 3991. https://doi.org/10.3390/en13153991