Three-Dimensional Geomechanical Modeling and Analysis of Refracturing and “Frac-Hits” in Unconventional Reservoirs
Abstract
:1. Introduction
2. Theory and Governing Equations
2.1. Poroelastic Deformation of Rock Matrix and Pore Fluid Diffusion
2.1.1. Constitutive Equations
2.1.2. Transport Equation
2.1.3. Conservation Laws
2.1.4. Field Equations
2.2. Fluid Flow Inside a Fracture
3. Numerical Methodology
3.1. Coupled Solution of Solid Rock Deformation, Pore Fluid Diffusion, and Fluid Flow in a Fracture
3.2. Fracture Propagation
4. Stress Reorientation and “Frac-Hits”
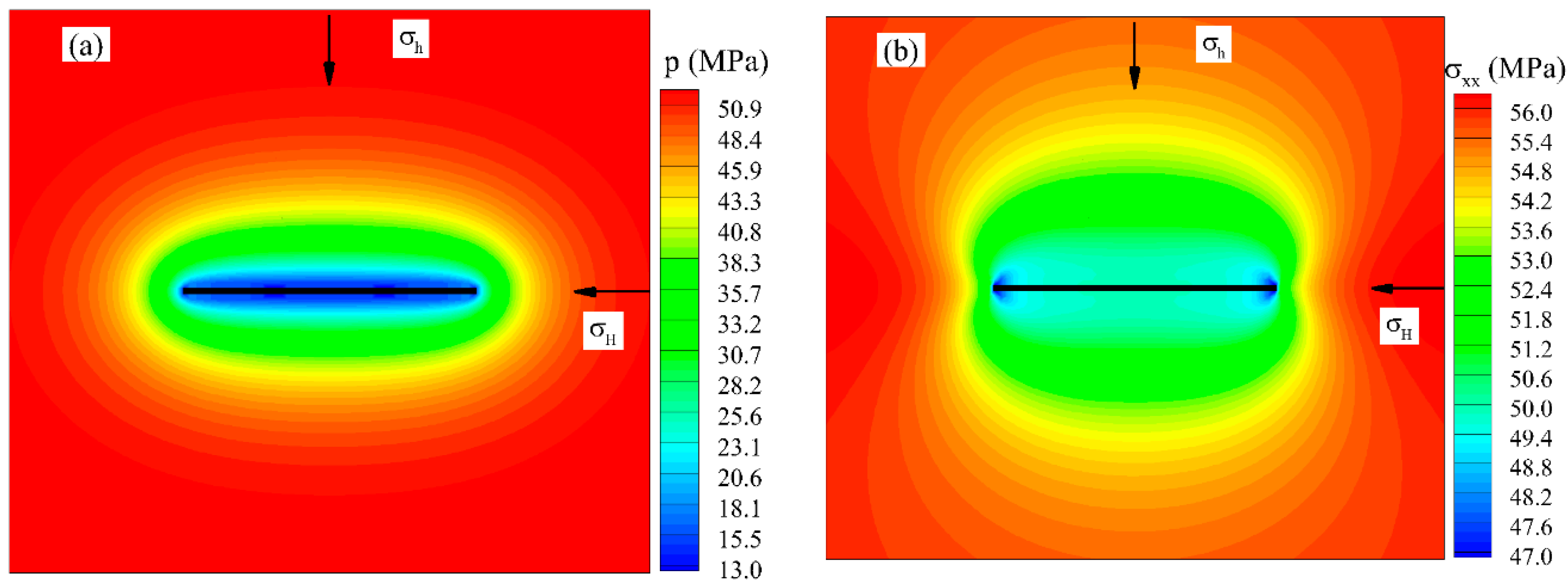
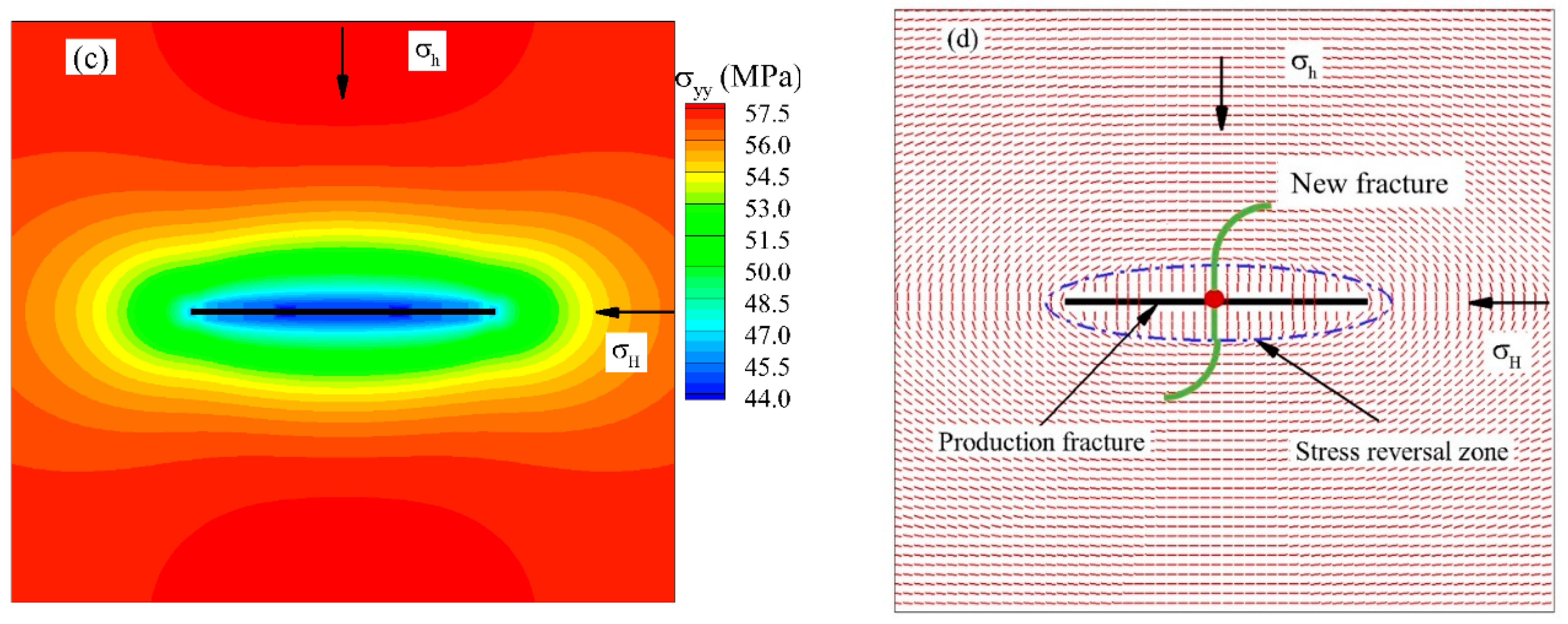
4.1. The Concept of Stress Reorientation/Reversal
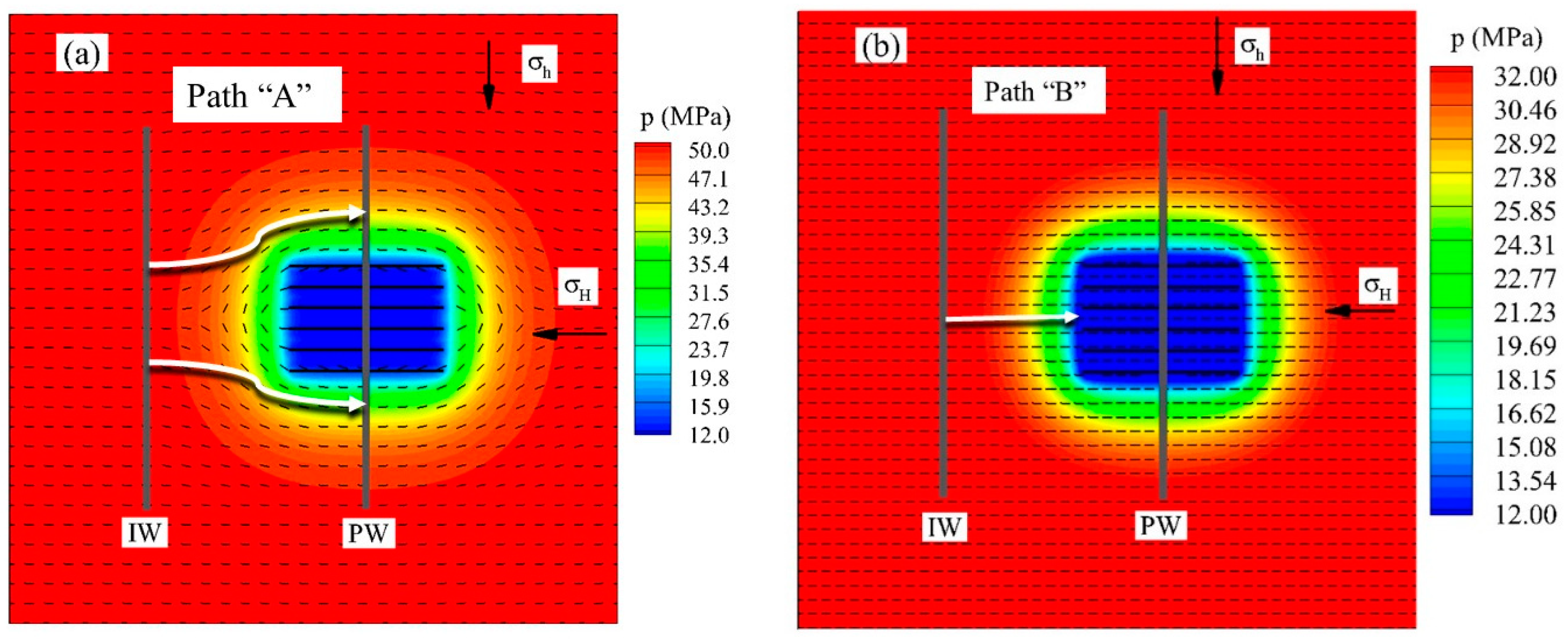
4.2. The “Frac-Hits” in Horizontal Well Refracturing
5. Model Verification
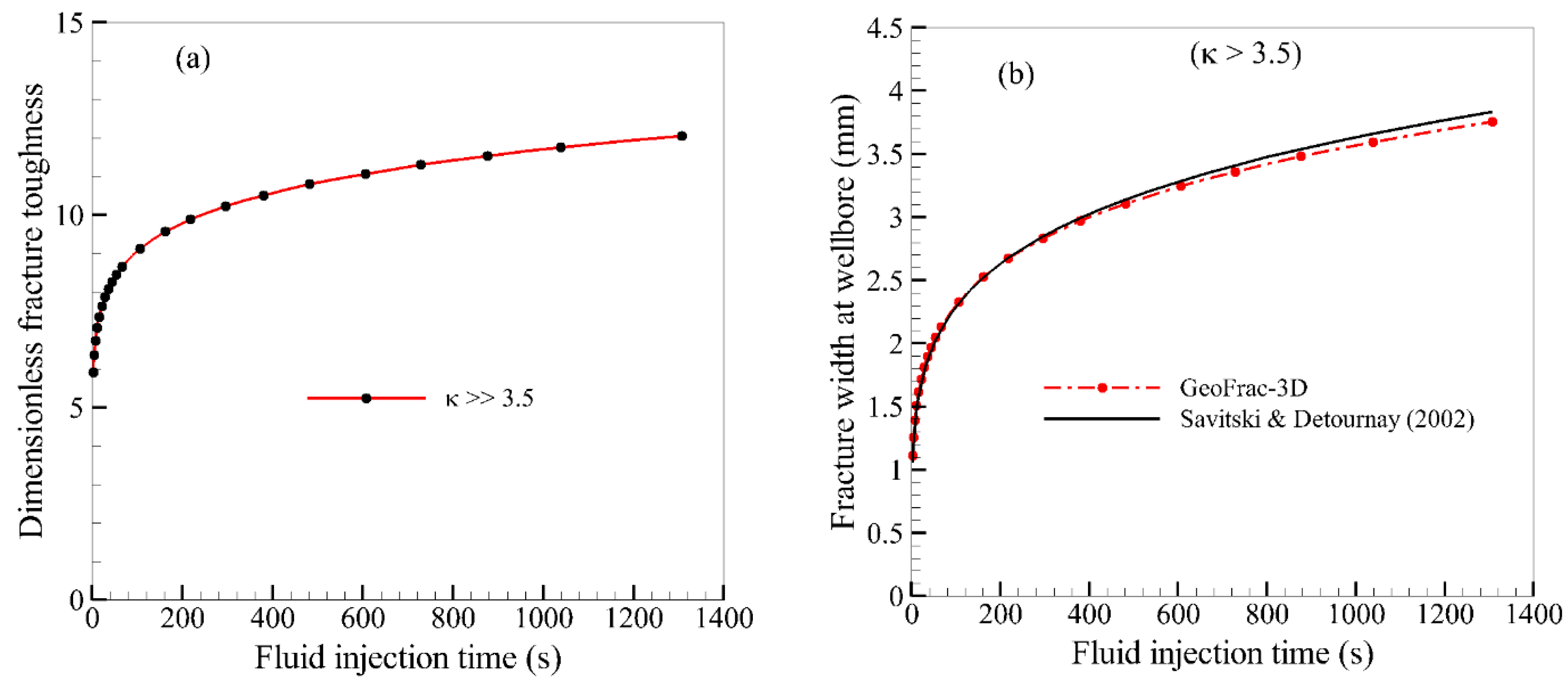
5.1. Penny-Shaped Fracture Propagation in Toughness Dominated Regime
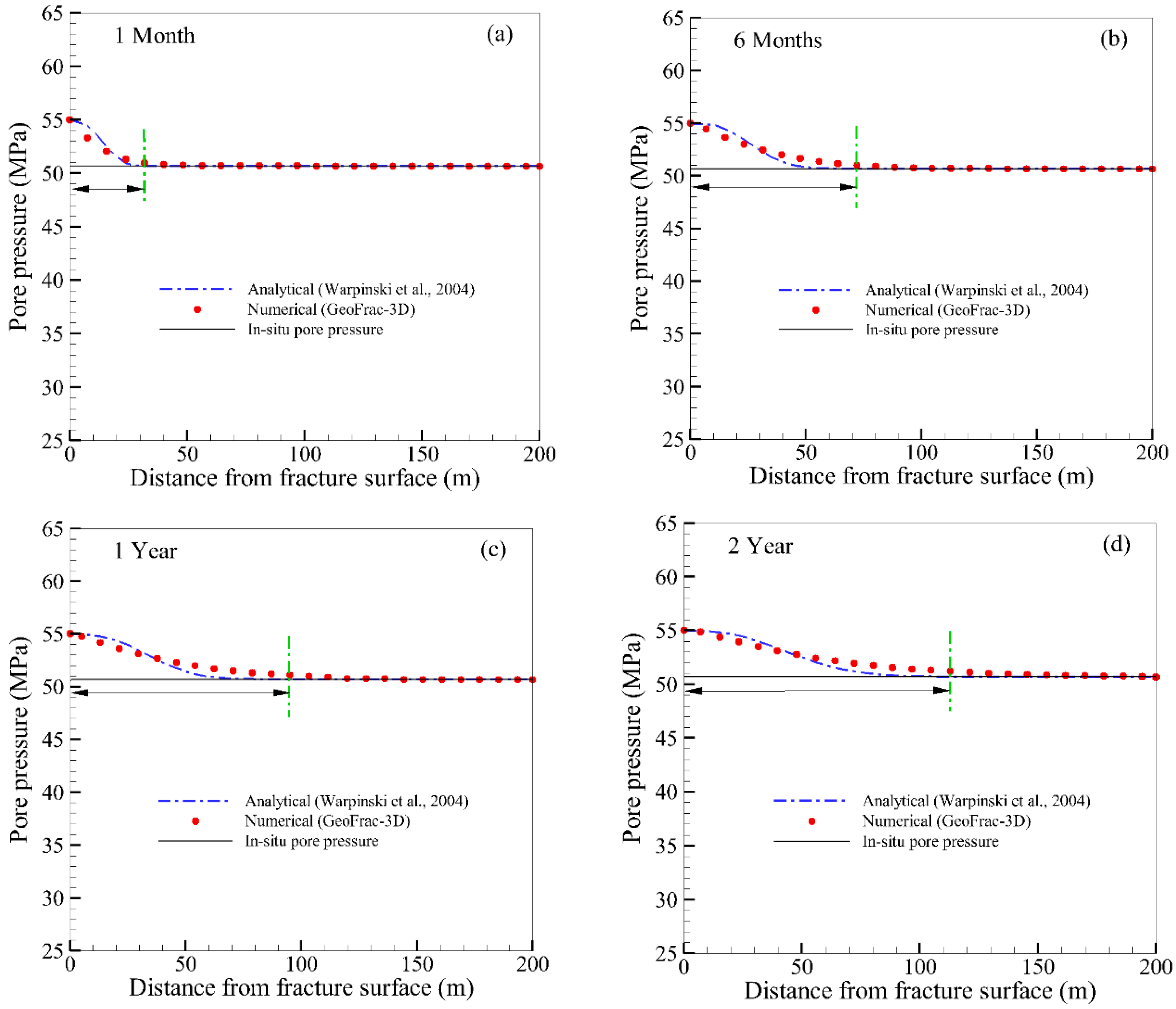
5.2. Injection Induced-Pore Pressure Change around a Fracture
6. Reservoir Rock Properties and In-Situ Stresses
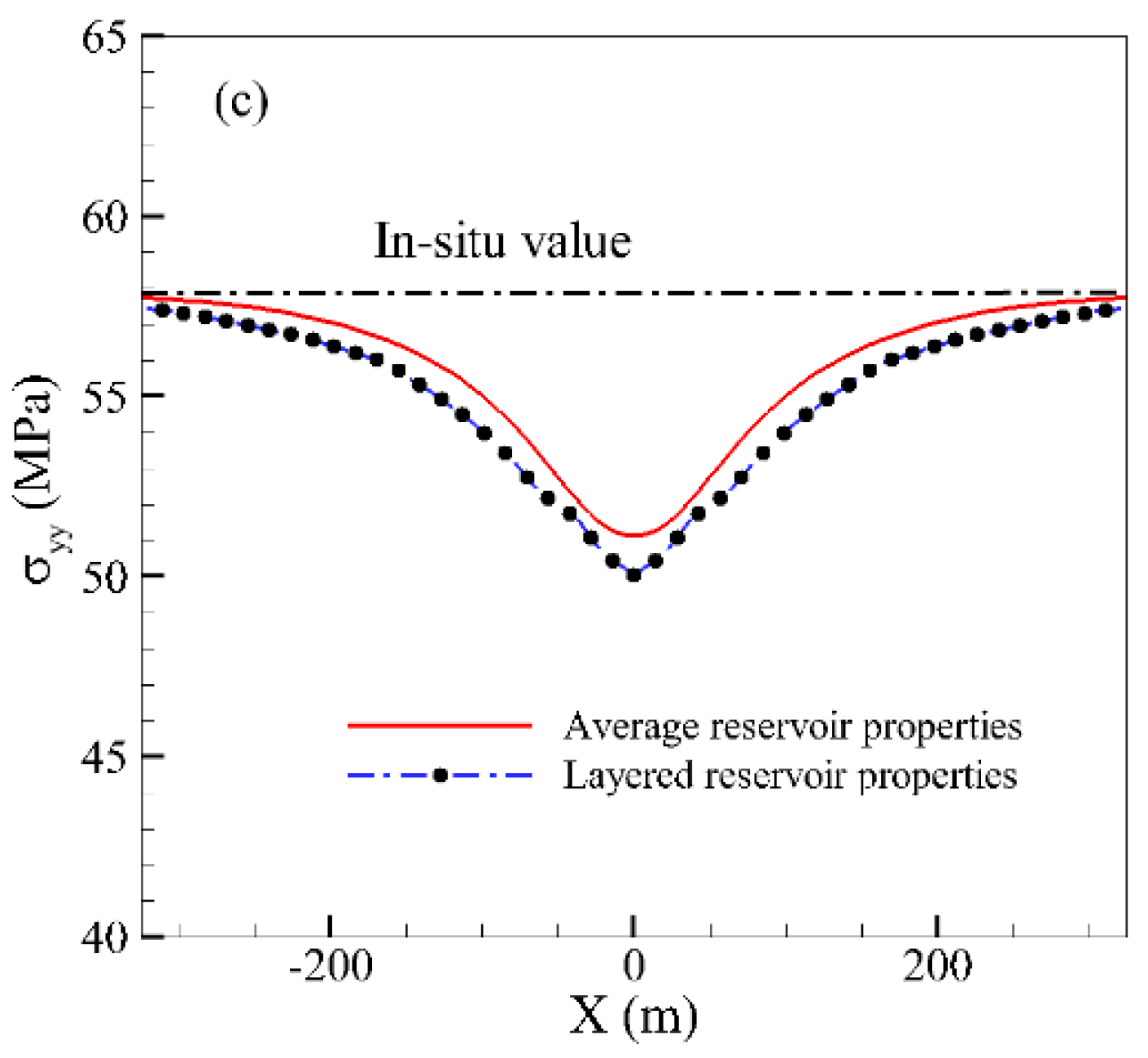
7. Impact of Reservoir Layers Properties
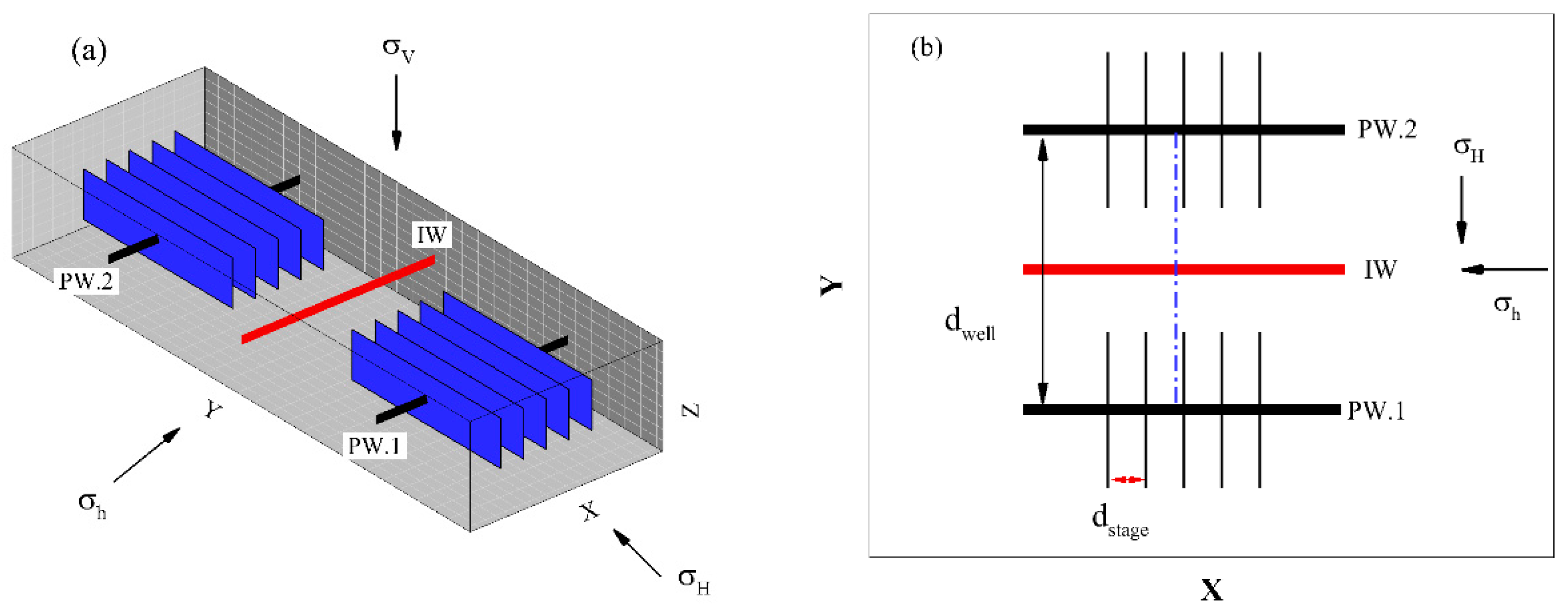
8. Numerical Examples
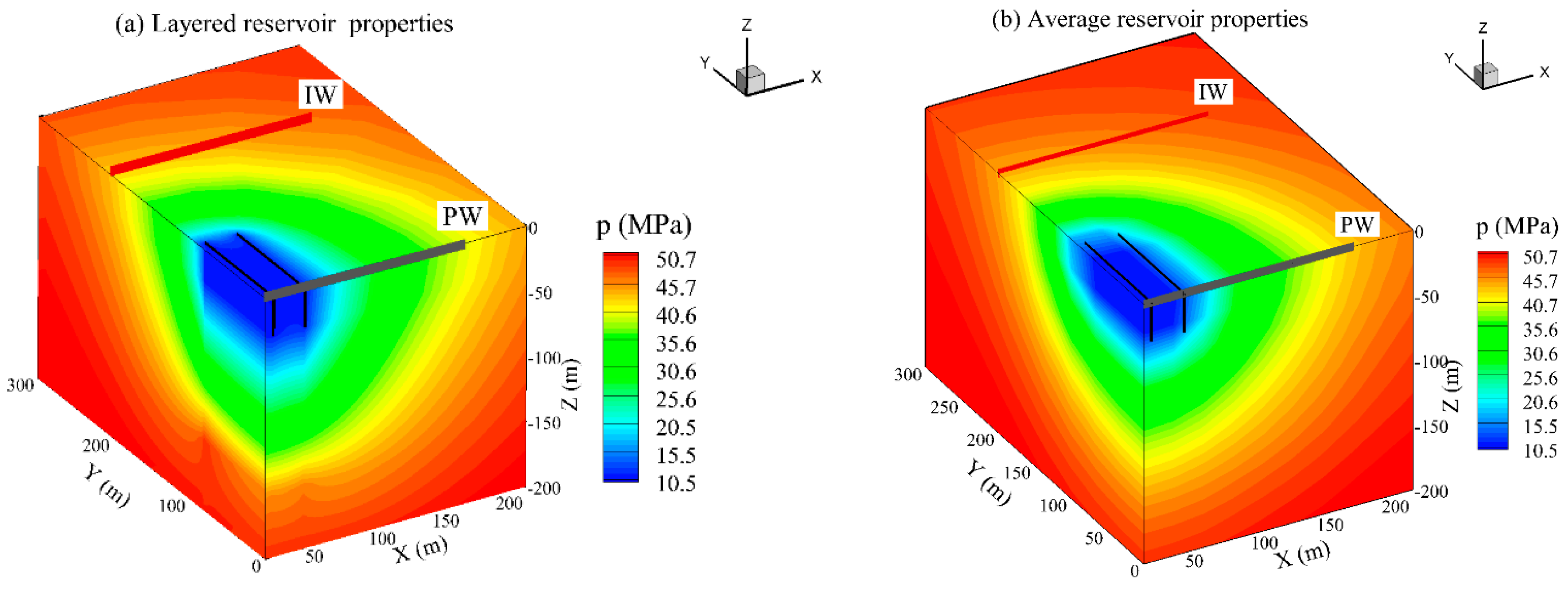
8.1. Reservoir Depletion Due to Production from Parent Wells
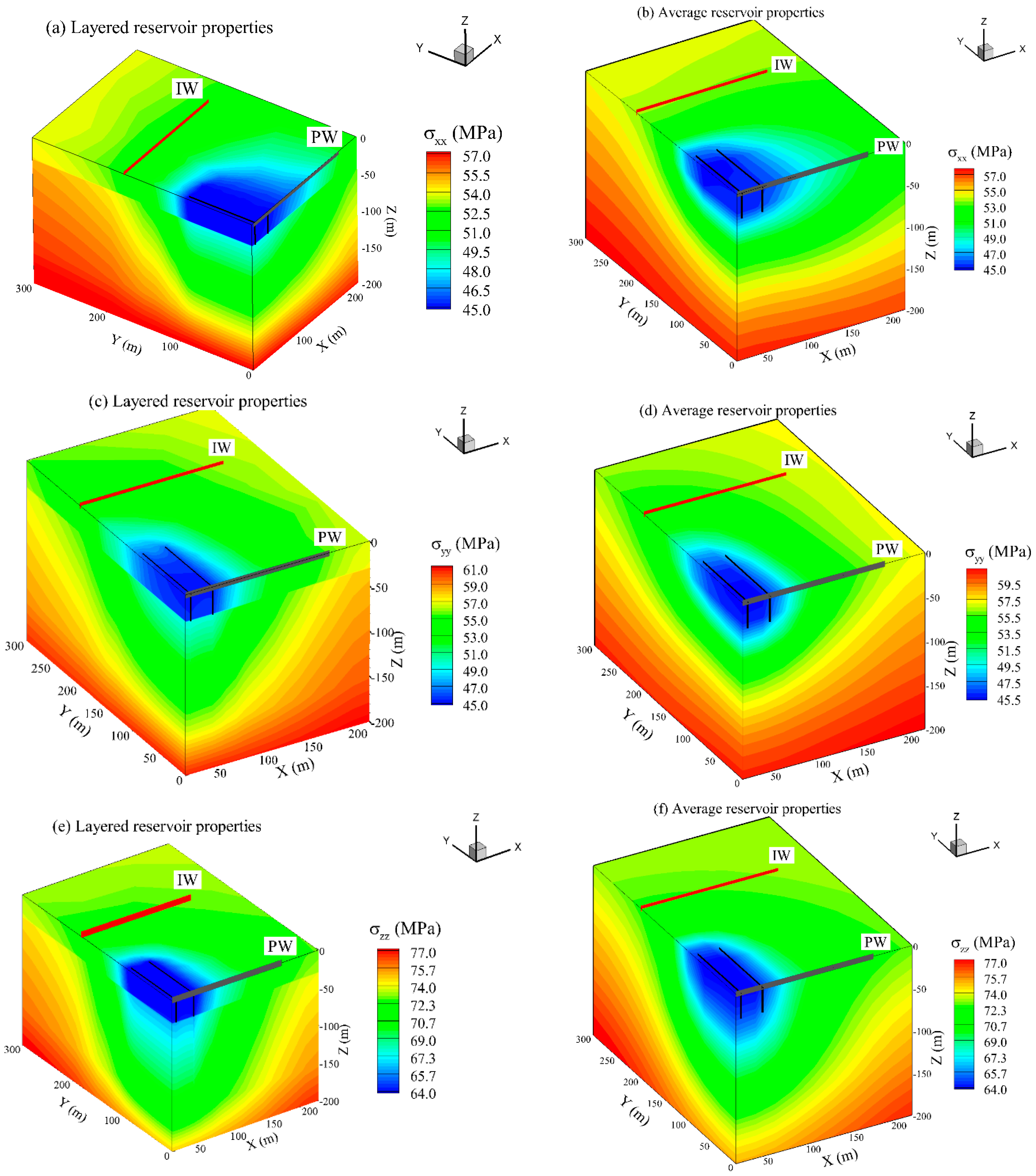
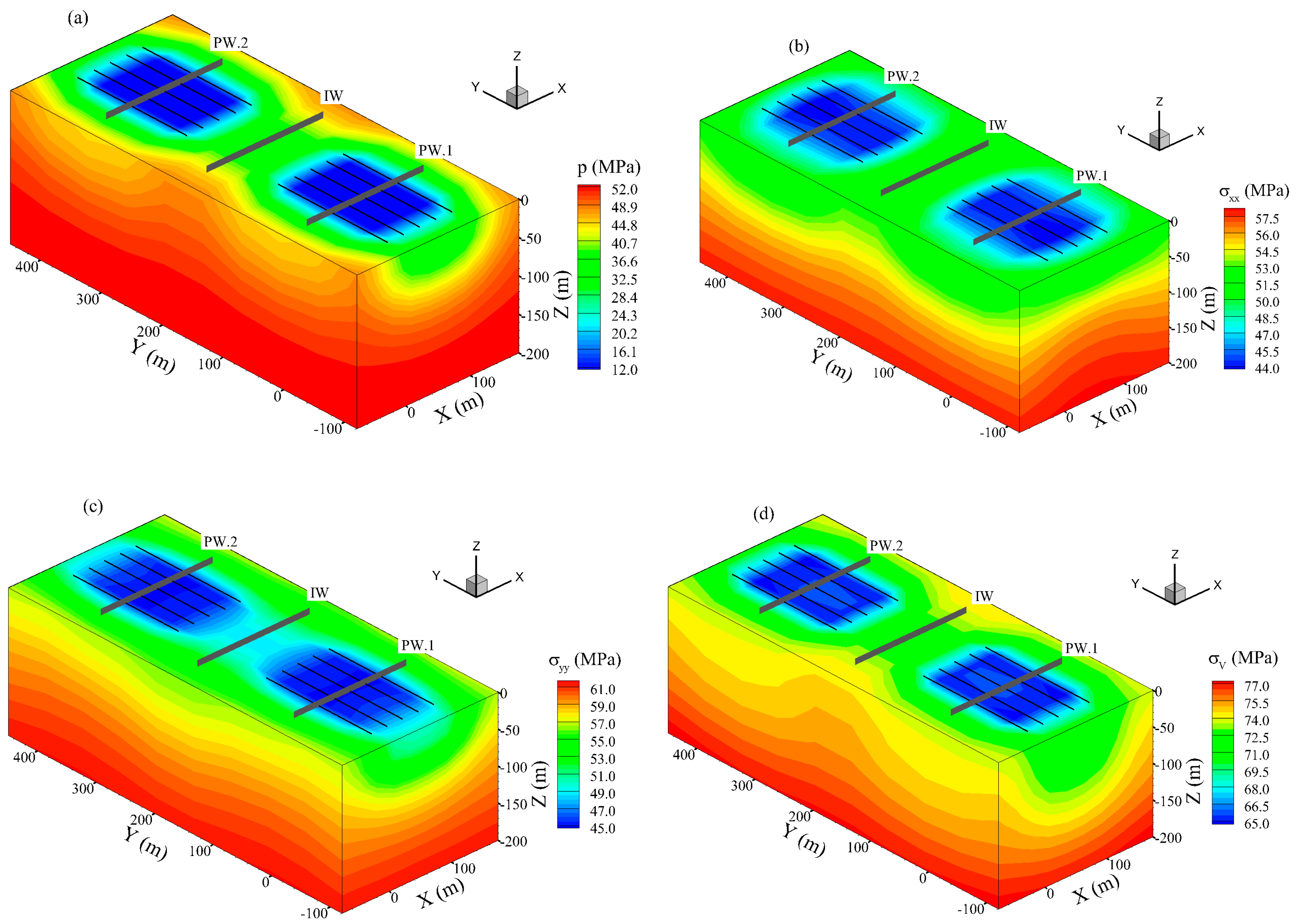
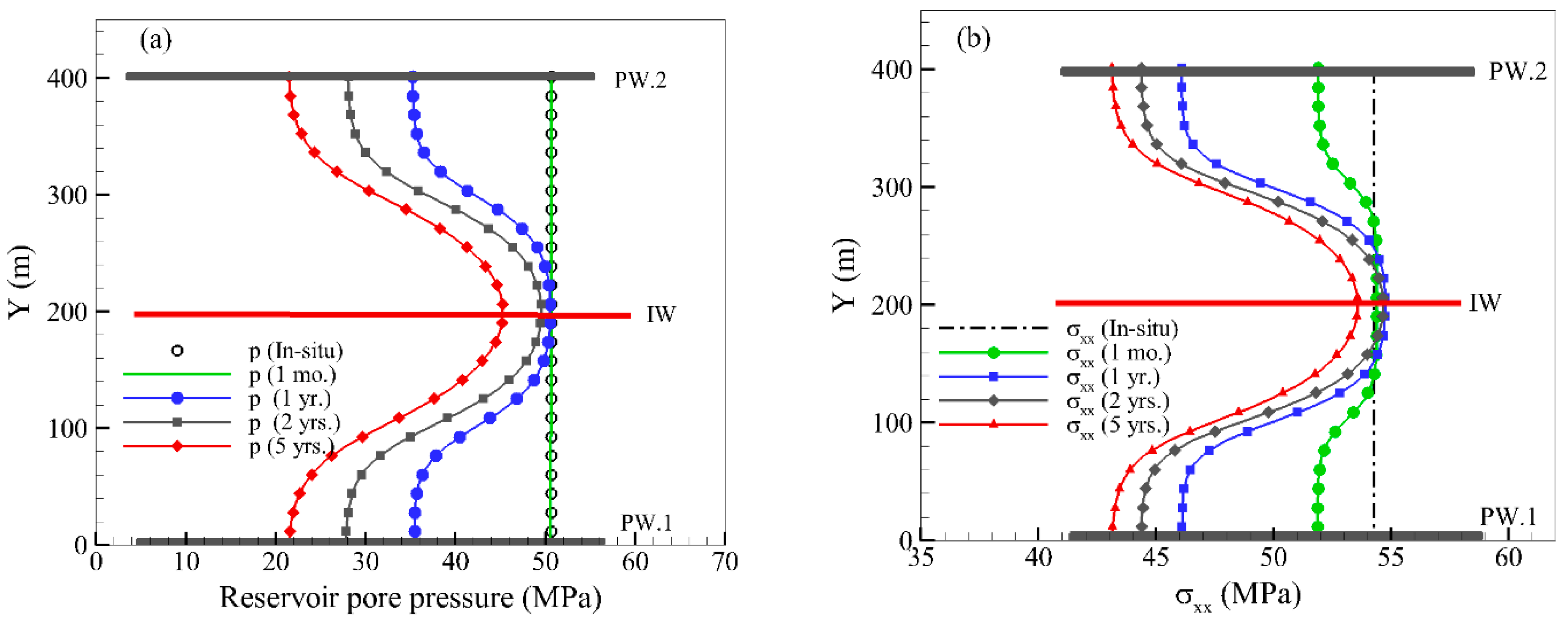
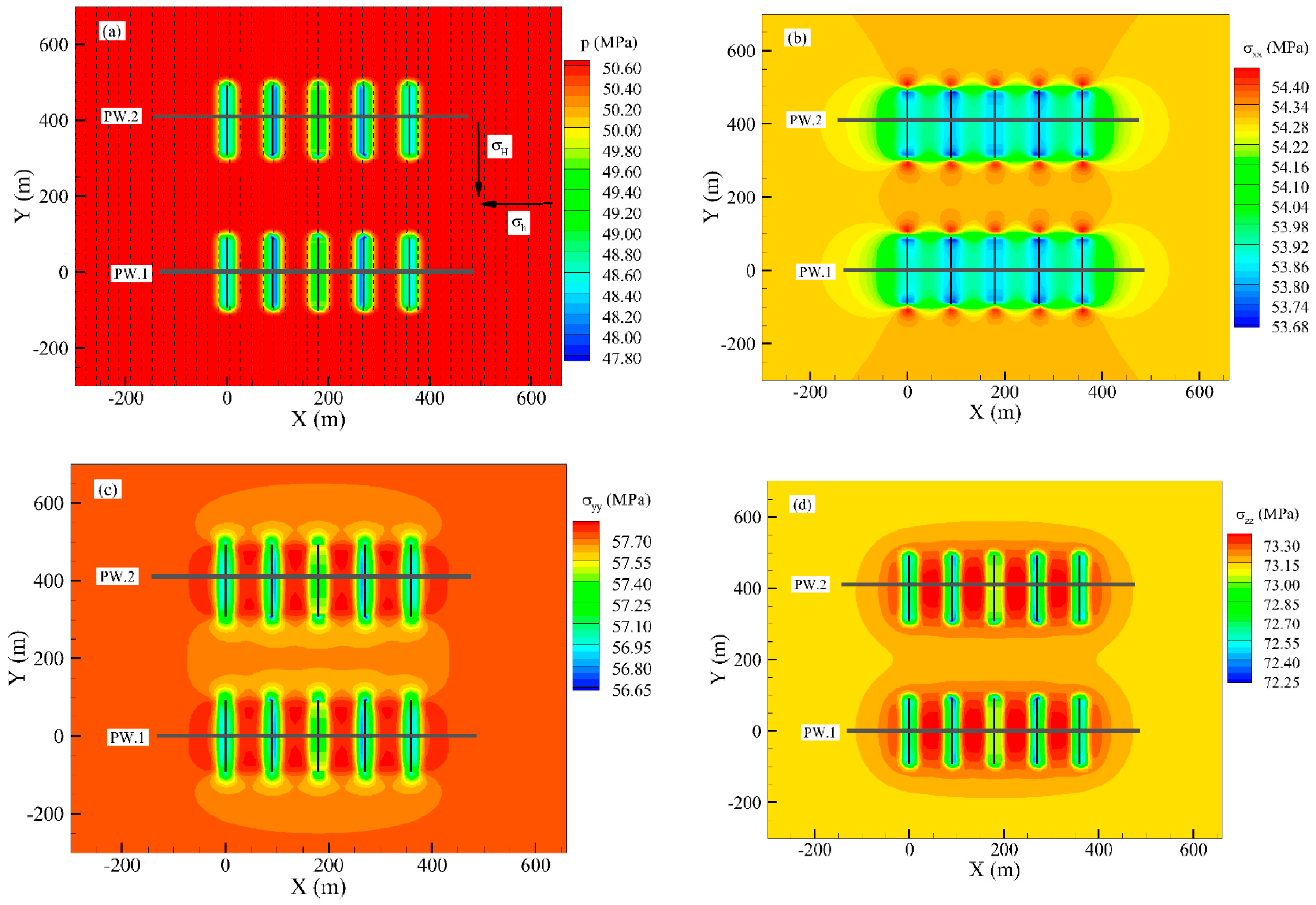
8.1.1. Impact of Reservoir Depletion on the Total Stresses
8.1.2. Impact of Reservoir Depletion on the Effective Stresses
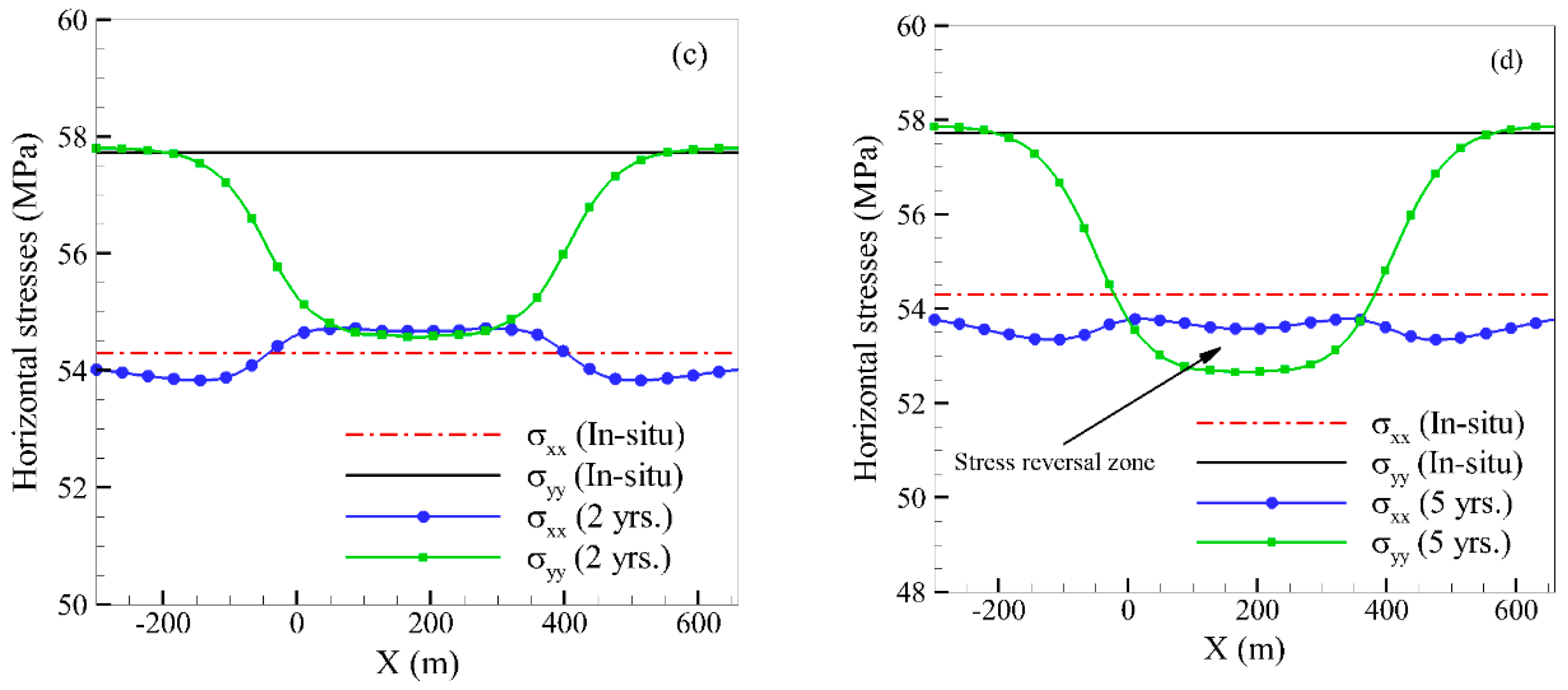
8.1.3. Impact of Production Time on the Reservoir Pore pressure and Total Stresses
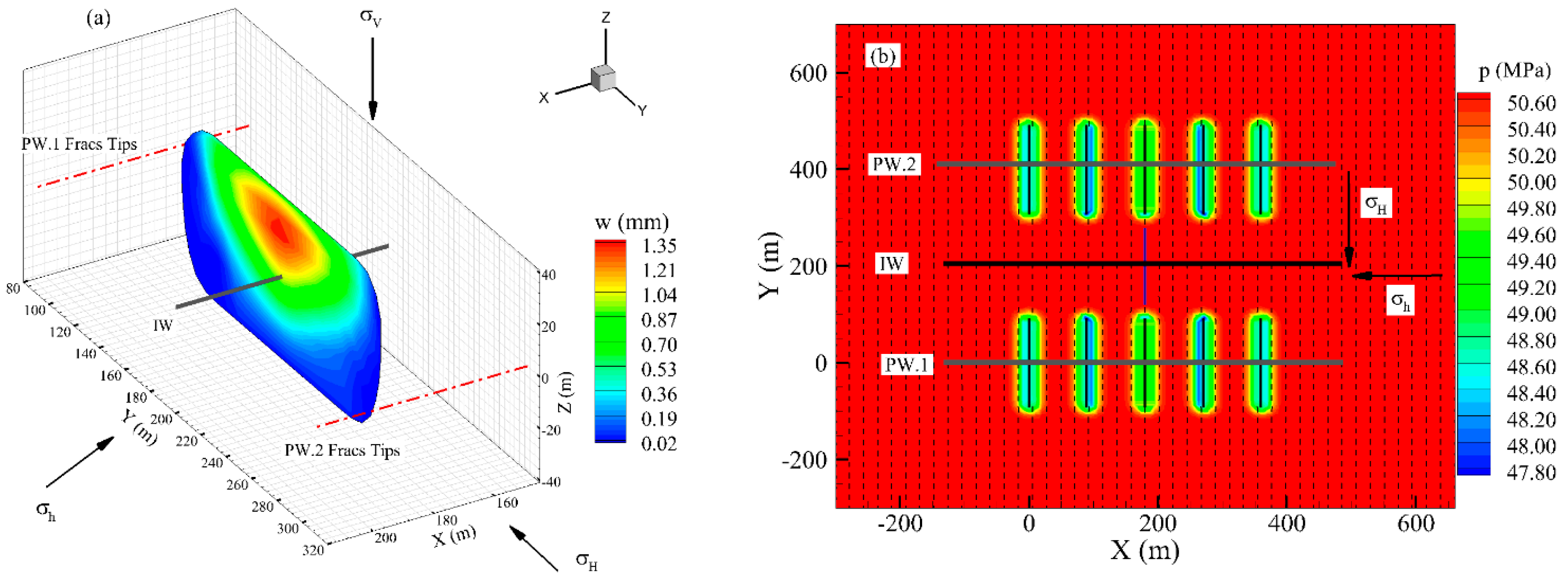
8.2. Fracture Propagation from the Infill Well
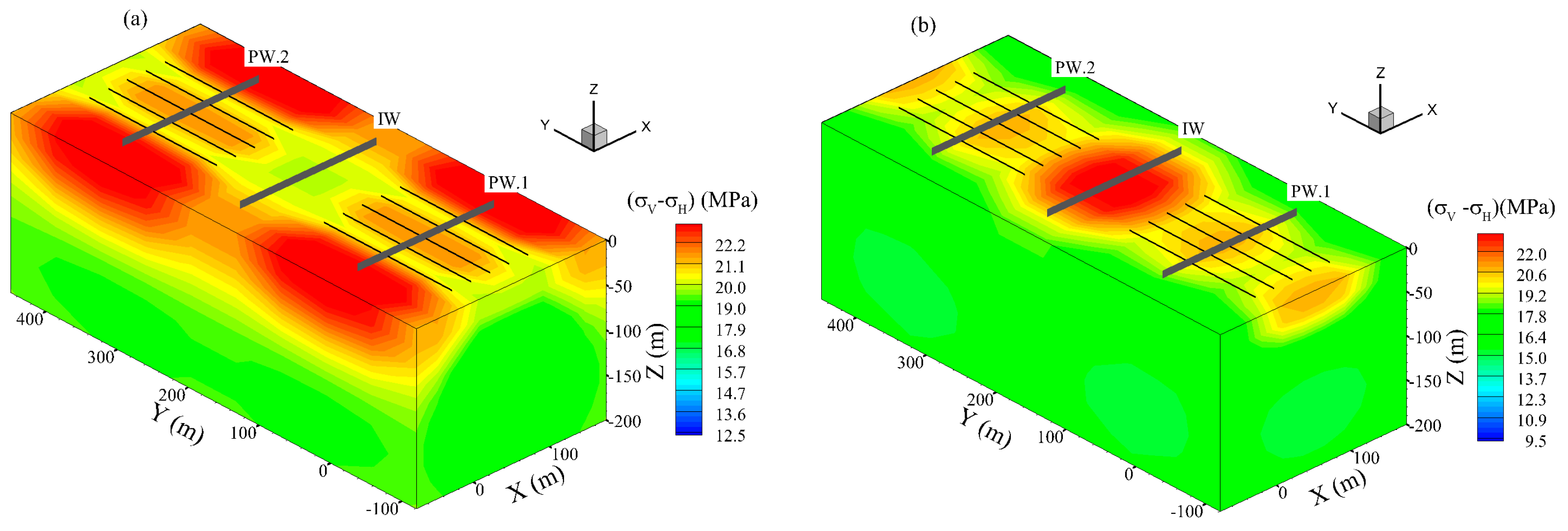
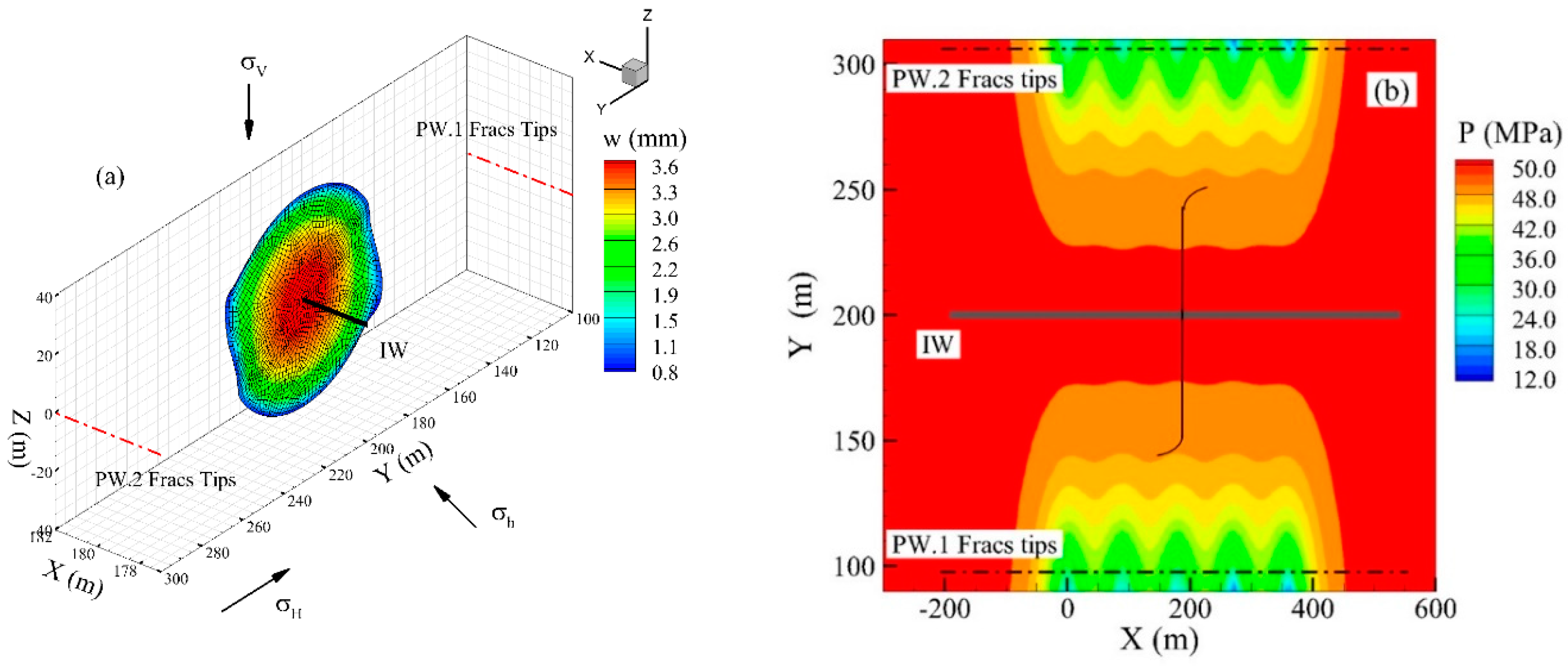
8.2.1. Infill Well Fracture Propagation in the Normal Faulting Stress Regime
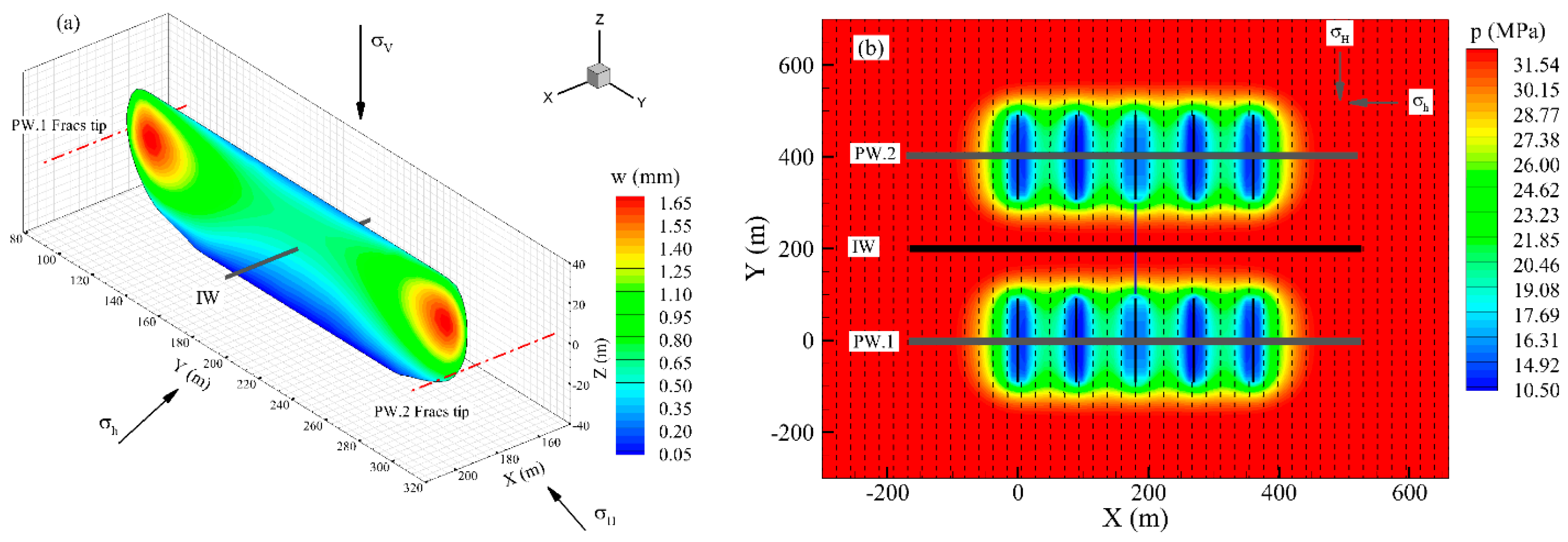
8.2.2. Infill Well Fracture Propagation in the Strike-Slip Stress Regime
8.2.3. Infill Well Fracture Propagation in the Strike-Slip Stress Regime
9. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| α | Biot’s coefficient |
| M | Biot’s modulus |
| BHP | bottomhole pressure |
| SRV | Stimulated reservoir volume |
| body force per unit volume in direction “i” | |
| bulk modulus of the rock mass | |
| bulk modulus of the solid grains | |
| displacement in the direction “i” | |
| ν | Poisson’s ratio |
| fluid flux or fluid volume per unit area | |
| fluid injection rate | |
| fluid extraction rate | |
| injection pressure | |
| production pressure | |
| µ | fluid viscosity |
| ζ | fluid content per unit volume |
| fluid source intensity per unit volume | |
| gravity vector | |
| fluid density | |
| fluid bulk modulus | |
| fluid leak-off | |
| ϕ | formation porosity |
| w | fracture width |
| A | fracture area |
| x | local coordinates of the fracture plane |
| γ | intensity of fluid source or sink |
| k | intrinsic permeability |
| κ | dimensionless fracture toughness |
| Kronecker delta function | |
| p | reservoir pore pressure |
| fluid pressure inside the fracture | |
| G | shear modulus |
| total stress tensor | |
| total strain tensor | |
| vertical stress | |
| maximum horizontal stress | |
| minimum horizontal stress | |
| horizontal stress anisotropy | |
| normal stress | |
| in-plane shear stress | |
| out-of-plane shear stress | |
| normal stiffness of joint filling material | |
| shear stiffness of joint filling material | |
| normal displacement discontinuity | |
| change in the normal displacement discontinuity | |
| in-plane shear displacement discontinuity | |
| out-of-plane shear displacement discontinuity |
References
- Martinez, R.; Rosinski, J.; Dreher, D. Horizontal pressure sink mitigation completion design: A case study in the Haynesville Shale. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 8–10 October 2012. [Google Scholar]
- Miller, G.; Linsay, G.; Baihly, J.; Xu, T. Parent well refracturing: Economic safety nets in an uneconomic market. In Proceedings of the SPE Low Perm Symposium, Denver, CO, USA, 5–6 May 2016. [Google Scholar]
- Morales, A.; Zhang, K.; Gakhar, K.; Porcu, M.M.; Lee, D.; Shan, D.; Malpani, R.; Sobernheim, D.; Acock, A. Advanced modeling of interwell fracturing interference: An Eagle Ford Shale oil study-refracturing. In Proceedings of the SPE Hydraulic Fracturing Technology Conference, Woodlands, TX, USA, 9–11 February 2016. [Google Scholar]
- Safari, R.; Lewis, R.; Ma, X.; Mutlu, U.; Ghassemi, A. Infill-Well Fracturing Optimization in Tightly Spaced Horizontal Wells. SPE J. 2017, 22, 582–595. [Google Scholar] [CrossRef]
- Kumar, D.; Feizi Masouleh, S.; Ghassemi, A.; Riley, S.; Elliott, B. A 3D geomechanical analysis of horizontal refracturing and frac-hits. In Proceedings of the 52nd US Rock Mechanics/Geomechanics Symposium, Seattle, WA, USA, 17–20 June 2018. [Google Scholar]
- Kumar, D.; Ghassemi, A. 3D geo-mechanical analysis of refracturing of horizontal wells. In Proceedings of the SPE/AAPG/SEG Unconventional Resources Technology Conference, Austin, Texas, USA, 24–26 July 2017. [Google Scholar]
- Perkins, T.; Gonzalez, J. The Effect of Thermoelastic Stresses on Injection Well Fracturing. Soc. Pet. Eng. J. 1985, 25, 78–88. [Google Scholar] [CrossRef]
- Warpinski, N.R.; Branagan, P.T. Altered-Stress Fracturing. J. Pet. Technol. 1989, 41, 990–997. [Google Scholar] [CrossRef]
- Elbel, J.L.; Mack, M.G. Refracturing: Observations and Theories. In Proceedings of the SPE 25464, the Production Operations Symposium, Oklahoma City, OK, USA, 21–23 March 1993. [Google Scholar]
- Palmer, I.D. Induced stresses due to propped hydraulic fracture in coalbed methane wells. In Low Permeability Reservoirs Symposium. In In Proceedings of the Society of Petroleum Engineers, Denver, CO, USA, 26–28 April 1993. [Google Scholar]
- Wright, C.A.; Conant, R.A. Hydraulic fracture reorientation in primary and secondary recovery from low-permeability reservoirs. In Proceedings of the SPE Annual Technical Conference and Exhibition, Dallas, TX, USA, 22–25 October 1995. [Google Scholar]
- Berchenko, I.; Detournay, E. Deviation of Hydraulic Fractures through Poroelastic Stress Changes Induced by Fluid Injection and Pumping. Int. J. Rock Mech. Min. Sci. 1997, 34, 1009–1019. [Google Scholar] [CrossRef]
- Siebrits, E.; Elbel, J.L.; Detournay, E.; Detournay-Piette, C.; Christianson, M.; Robinson, B.M.; Diyashev, I.R. Parameters affecting azimuth and length of a secondary fracture during a fracture treatment. In Proceedings of the SPE 48928: SPE Annual Technical Conference and Exhibition, New Orleans, LA, USA, 27–30 September 1998. [Google Scholar]
- Zhang, Q. A Boundary Element Method for Thermo-Poroelasticity with Applications in Rock Mechanics. Master’s Thesis, University of North Dakota, Grand Forks, ND, USA, 2004. [Google Scholar]
- Ghassemi, A.; Zhang, Q. Poro-thermoelastic response of a stationary crack using the displacement discontinuity method. ASCE J. Eng. Mech. 2006, 132, 26–33. [Google Scholar] [CrossRef]
- Siebrits, E.; Elbel, J.L.; Hoover, R.S.; Diyashev, I.R.; Griffin, L.G.; Demetrius, S.L.; Wright, C.A.; Davidson, B.M.; Steinsberger, N.P.; Hill, D.G. Refracture reorientation enhances gas production in Barnett shale tight gas wells. In Proceedings of the SPE Annual Technical Conference and Exhibition, Society of Petroleum Engineers, Dallas, TX, USA, 1–4 October 2000. [Google Scholar]
- Rezaei, A.; Rafiee, M.; Bornia, G.; Soliman, M.; Morse, S. Protection Refrac: Analysis of pore pressure and stress change due to refracturing of Legacy Wells. In Proceedings of the SPE/AAPG/SEG Unconventional Resources Technology Conference, Austin, TX, USA, 24–26 July 2017. [Google Scholar]
- Rezaei, A.; Bornia, G.; Rafiee, M.; Soliman, M.; Morse, S. Analysis of refracturing in horizontal wells: Insights from the poroelastic displacement discontinuity method. Int. J. Numer. Anal. Methods Géoméch. 2018, 42, 1306–1327. [Google Scholar] [CrossRef]
- Chun, K.H. Thermo-Poroelastic Fracture Propagation Modeling with Displacement Discontinuity Boundary Element Method. Ph.D. Thesis, Texas A&M University, College Station, TX, USA, 2013. [Google Scholar]
- Roussel, N.P.; Florez, H.A.; Rodriguez, A.A. Hydraulic fracture propagation form infill horizontal wells. In Proceedings of the SPE 166503, SPE Annual Technical Conference and Exhibition, New Orleans, LA, USA, 30 September–2 October 2013. [Google Scholar]
- Safari, R.; Ghassemi, A. 3D thermo-poroelastic analysis of the fracture network deformation and induced micro-seismicity in enhanced geothermal systems. Geothermics 2015, 58, 1–14. [Google Scholar] [CrossRef]
- Sangnimnuan, A.; Li, J.; Wu, K.; Holditch, S.A. Impact of Parent Well Depletion on Stress Changes and Infill Well Completion in Multiple Layers in Permian Basin. In Proceedings of the Unconventional Resources Technology Conference (URTeC), Denver, CO, USA, 22–24 July 2019. [Google Scholar]
- Biot, M.A. Theory of Elasticity and Consolidation for a Porous Anisotropic Solid. J. Appl. Phys. 1955, 26, 182–185. [Google Scholar] [CrossRef]
- Geertsma, J. The Effect of Fluid Pressure Decline on Volumetric Changes of Porous Rocks. Trans. AIME 1957, 210, 331–340. [Google Scholar] [CrossRef]
- Cheng, A.H.D. Poroelasticity; Springer International Publishing: Cham, Switzerland, 2016; Volume 27. [Google Scholar]
- Rice, J.R.; Cleary, M.P. Some basic stress diffusion solutions for fluid saturated elastic media with compressible constituents. Rev. Geophys. Space Phys. 1976, 14, 227–241. [Google Scholar] [CrossRef]
- Detournay, E.; Cheng, A.H.-D. Poroelastic response of a borehole in a non-hydrostatic stress field. Int. J. Rock Mech. Min. Sci. Géoméch. Abstr. 1988, 25, 171–182. [Google Scholar] [CrossRef]
- Gidley, J.L.; Holditch, S.A.; Nierode, D.E.; Veatch, R.W.J. Recent advances in hydraulic fracturing. In Proceedings of the SPE Monograph Series. Society of Petroleum Engineers, Richardson, TX, USA, 6–8 March 1989. [Google Scholar]
- Witherspoon, P.A.; Wang, J.S.Y.; Iwai, K.; Gale, J.E. Validity of Cubic Law for fluid flow in a deformable rock fracture. Water Resour. Res. 1980, 16, 1016–1024. [Google Scholar] [CrossRef] [Green Version]
- Gale, J.F.; Elliott, S.J.; Laubach, S.E. Hydraulic fractures in core from stimulated reservoirs: Core fracture description of HFTS slant core. In Proceedings of the Unconventional Resources Technology Conference (URTEC), Midland Basin, TX, USA, 23–25 July 2018. [Google Scholar]
- Batchelor, G.K. An Introduction to Fluid Dynamics; Cambridge University Press: Cambridge, UK, 1967; ISBN 9780521663960. [Google Scholar]
- Yew, C.H. Mechanics of Hydraulic Fracturing; Gulf Publishing Company: Houston, TX, USA, 1997. [Google Scholar]
- Ghassemi, A.; Zhou, X. A three-dimensional thermo-poroelastic model for fracture response to injection/extraction in enhanced geothermal systems. Geothermics 2011, 40, 39–49. [Google Scholar] [CrossRef]
- Ghassemi, A.; Zhou, X.; Rawal, C. A three-dimensional poroelastic analysis of rock failure around a hydraulic fracture. J. Pet. Sci. Eng. 2013, 108, 118–127. [Google Scholar] [CrossRef]
- Garagash, D.I. Propagation of a plane-strain hydraulic fracture with a fluid lag: Early-time solution. Int. J. Solids Struct. 2006, 43, 5811–5835. [Google Scholar] [CrossRef] [Green Version]
- Zienkiewicz, O.C.; Taylor, R.L. Finite Element Method, 5th ed.; Butterworth-Heinemann: Amsterdam, The Netherlands, 2000. [Google Scholar]
- Smith, I.M.; Griffiths, D.V.; Margetts, L. Programming the Finite Element Method, 5th ed.; Wiley: Hoboken, NJ, USA, 2013. [Google Scholar]
- Curran, J.; Carvalho, J.L. A displacement discontinuity model for fluid-saturated porous media. In Proceedings of the 6th ISRM Congress, International Society for Rock Mechanics and Rock Engineering, Montreal, QC, Canada, 30 August–3 September 1987. [Google Scholar]
- Safari, R.; Ghassemi, A. Three-dimensional poroelastic modeling of injection induced permeability enhancement and microseismicity. Int. J. Rock Mech. Min. Sci. 2016, 84, 47–58. [Google Scholar] [CrossRef] [Green Version]
- Kumar, D.; Ghassemi, A. Three-dimensional poro-elastic modeling of multiple hydraulic fracture propagation from horizontal wells. Int. J. Rock Mech. Mining Sci. 2018, 105, 192–209. [Google Scholar] [CrossRef]
- Zhou, X.; Ghassemi, A. Three-dimensional poroelastic analysis of a pressurized natural fracture. Int. J. Rock Mech. Min. Sci. 2011, 48, 527–534. [Google Scholar] [CrossRef]
- Kumar, D.; Ghassemi, A. A three-dimensional analysis of simultaneous and sequential fracturing of horizontal wells. J. Pet. Sci. Eng. 2016, 146, 1006–1025. [Google Scholar] [CrossRef]
- Kamali, A.; Ghassemi, A. Analysis of natural fracture shear slip and propagation in response to injection. In Proceedings of the 41st Workshop on Geothermal Reservoir Engineering, Stanford University, Stanford, CA, USA, 22–24 February 2016. [Google Scholar]
- Kamali, A.; Ghassemi, A. Poroelastic Analysis of Natural Fracture Propagation and Coalescence. In Proceedings of the Geothermal Resource Council, Annual Meeting, Sacramento, CA, USA, 23–26 October 2016. [Google Scholar]
- Kumar, D.; Ghassemi, A. 3D simulation of multiple fracture propagation from horizontal wells. In Proceedings of the 49th US Rock Mechanics/Geomechanics Symposium, San Francisco, CA, USA, 28 June–1 July 2015. [Google Scholar]
- Crouch, S.L.; Starfield, A.M. Boundary Element Methods in Solid Mechanics; George Allen & Unwin: London, UK, 1983. [Google Scholar]
- Griffith, A.A., VI. The phenomena of rupture and flow in solids. Philos. Trans. R. Soc. Lond. 1921, 221, 163–198. [Google Scholar] [CrossRef] [Green Version]
- Irwin, G.R. Analysis of stresses and strains near the end of a crack. J. Appl. Mech. 1957, 24, 361–364. [Google Scholar]
- Schöllmann, M.; Richard, H.A.; Kullmer, G.; Fulland, M. A new criterion for the prediction of crack development in multi-axially loaded structures. Int. J. Fract. 2002, 117, 129–141. [Google Scholar] [CrossRef]
- Richard, H.; Fulland, M.; Sander, M. Theoretical crack path prediction. Fatigue Fract. Eng. Mater. Struct. 2005, 28, 3–12. [Google Scholar] [CrossRef]
- Gupta, P.; Duarte, C.A. Coupled hydromechanical-fracture simulations of nonplanar three-dimensional hydraulic fracture propagation. Int. J. Numer. Anal. Methods Géoméch. 2017, 42, 143–180. [Google Scholar] [CrossRef]
- Geuzaine, C.; Remacle, J.-F. Gmsh: A 3-D finite element mesh generator with built-in pre- and post-processing facilities. Int. J. Numer. Methods Eng. 2009, 79, 1309–1331. [Google Scholar] [CrossRef]
- Zhou, X.; Ghassemi, A. Three-dimensional poroelastic displacement discontinuity simulation of natural fractures. In Proceedings of the 43rd US Rock Mechanics Symposium and 4th U.S.-Canada Rock Mechanics Symposium, Asheville, NC, USA, 28 June–1 July 2009. [Google Scholar]
- Safari, R.; Ghassemi, A. 3D analysis of huff and puff and injection tests in geothermal reservoirs. In Proceedings of the 36th Workshop on Geothermal Reservoir Engineering, Stanford University, Stanford, CA, USA, 31 January–2 February 2011. [Google Scholar]
- Savitski, A.; Detournay, E. Propagation of a penny-shaped fluid-driven fracture in an impermeable rock: Asymptotic solutions. Int. J. Solids Struct. 2002, 39, 6311–6337. [Google Scholar] [CrossRef]
- Detournay, E. Propagation Regimes of Fluid-Driven Fractures in Impermeable Rocks. Int. J. Géoméch. 2004, 4, 35–45. [Google Scholar] [CrossRef]
- Sneddon, I.N. The distribution of stress in the neighborhood of a crack in an elastic solid. Proc. R. Soc. Lond. Ser. A 1946, 187, 29–260. [Google Scholar]
- Warpinski, N.; Wolhart, S.; Wright, C. Analysis and Prediction of Microseismicity Induced by Hydraulic Fracturing. SPE J. 2004, 9, 24–33. [Google Scholar] [CrossRef]
- Ge, J.; Ghassemi, A. Analytical modeling on 3D stress redistribution and fault reactivation during hydraulic fracturing stimulation. In Proceedings of the 48th US Rock Mechanics/Geomechanics Symposium, Minneapolis, MN, USA, 1–4 June 2014. [Google Scholar]
- Yue, K.; Olson, J.E.; Schultz, R.A. The effect of layered modulus on hydraulic-fracture modeling and fracture-height containment. SPE Drill. Completion 2019, 34, 356–371. [Google Scholar] [CrossRef]
- Zhou, X.; Ghassemi, A. Finite element analysis of coupled chemo-poro-thermo-mechanical effects around a wellbore in swelling shale. Int. J. Rock Mech. Min. Sci. 2009, 46, 769–778. [Google Scholar] [CrossRef]
- Wang, X.; Ghassemi, A. A 3D thermal-poroelastic model for geothermal reservoir stimulation. In Proceedings of the 37th Workshop on Geothermal Reservoir Engineering, Stanford, CA, USA, 30 January–1 February 2012. [Google Scholar]
- Cheng, Q.; Wang, X.; Ghassemi, A. Numerical simulation of reservoir stimulation with reference to the Newberry EGS. Geothermics 2019, 77, 327–343. [Google Scholar] [CrossRef]
- Mayerhofer, M.J.; Lolon, E.; Warpinski, N.R.; Cipolla, C.L.; Walser, D.W.; Rightmire, C.M. What Is Stimulated Reservoir Volume? SPE Prod. Oper. 2010, 25, 89–98. [Google Scholar] [CrossRef]
- Gray, K.E.; Podnos, E.; Becker, E. Finite-Element Studies of Near-Wellbore Region During Cementing Operations: Part I. SPE Drill. Complet. 2009, 24, 127–136. [Google Scholar] [CrossRef]
- Feng, Y.; Podnos, E.; Gray, K.E. Well integrity analysis: 3D numerical modeling of cement interface debonding. In Proceedings of the 50th US Rock Mechanics/Geomechanics Symposium, Houston, TX, USA, 26–29 June 2016. [Google Scholar]
- Rutqvist, J.; Rinaldi, A.P.; Cappa, F.; Moridis, G.J. Modeling of fault reactivation and induced seismicity during hydraulic fracturing of shale-gas reservoirs. J. Pet. Sci. Eng. 2013, 107, 31–44. [Google Scholar] [CrossRef]
- Lyons, W.G.; Plisga, B.S.; Lorenz, M. Standard Handbook of Petroleum Engineering and Natural Gas Engineering, 3rd ed.; Chapter 5-Reservoir Engineering; Gulf Professional Publishing: Houston, TX, USA, 2015. [Google Scholar]
- Sesetty, V.; Ghassemi, A. Numerical modeling of hydraulic fracture propagation from horizontal wells in anisotropic shale. In Proceedings of the 48th US Rock Mechanics/Geomechanics Symposium, Houston, TX, USA, 26–29 June 2016. [Google Scholar]


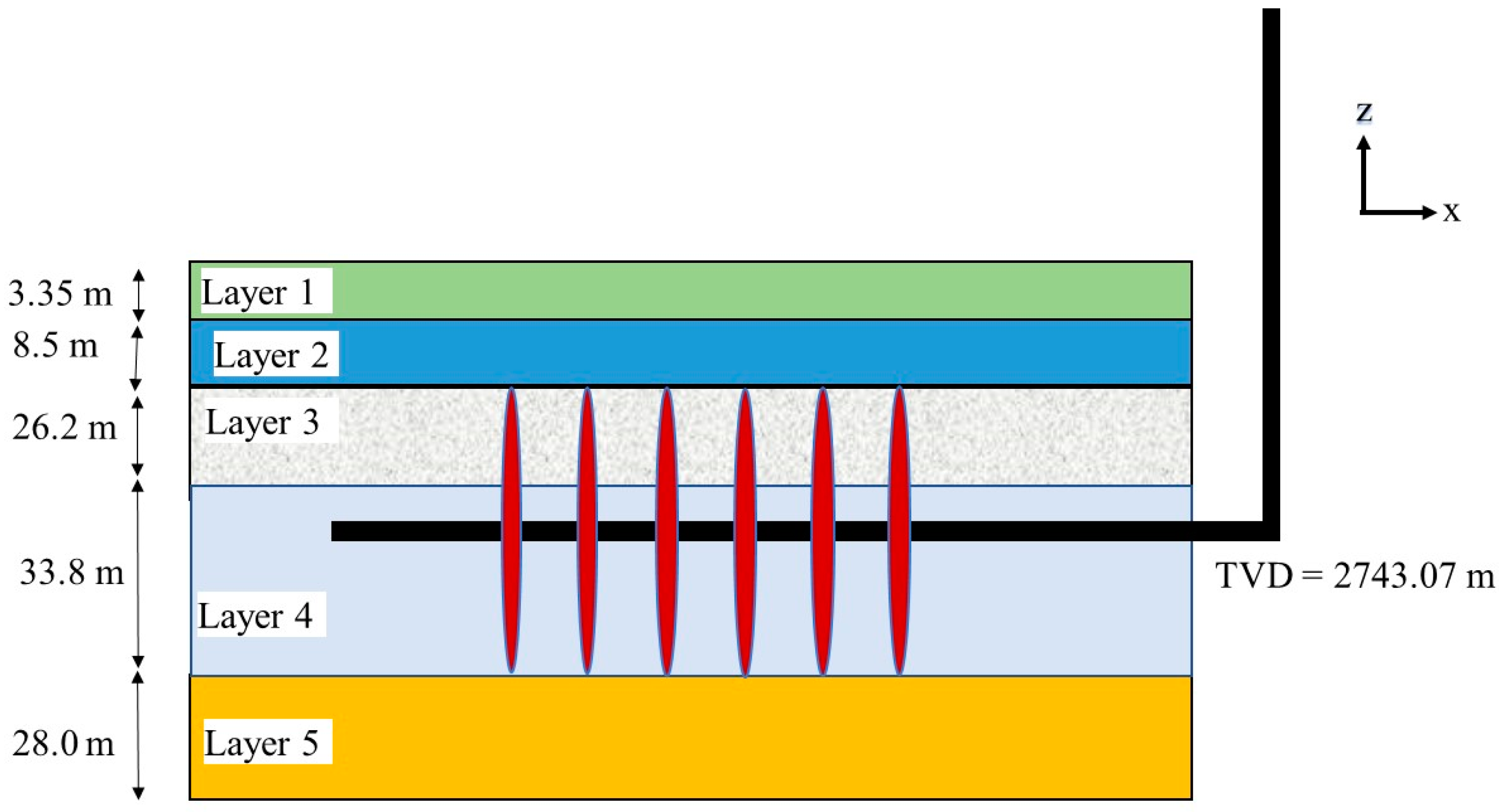


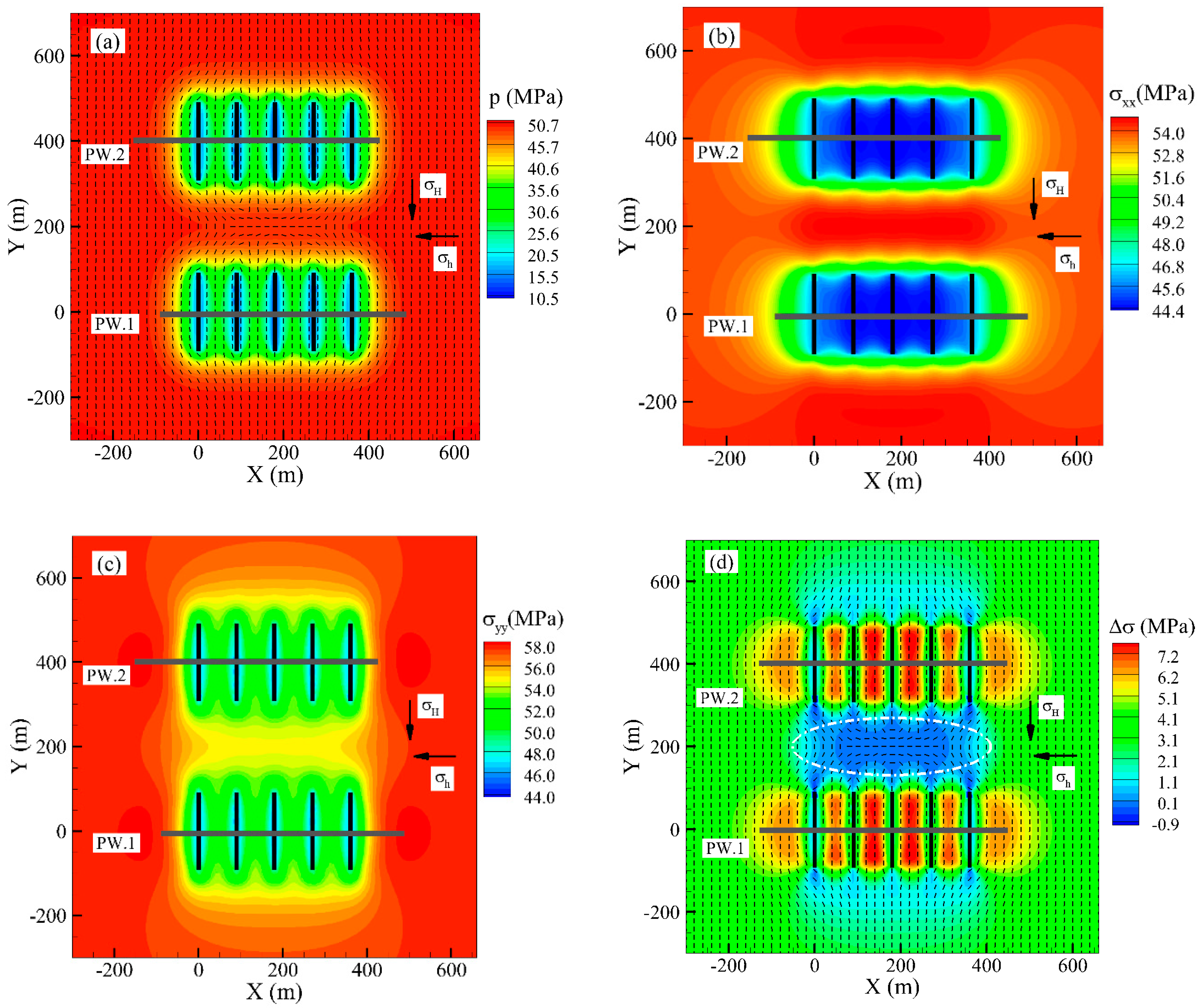
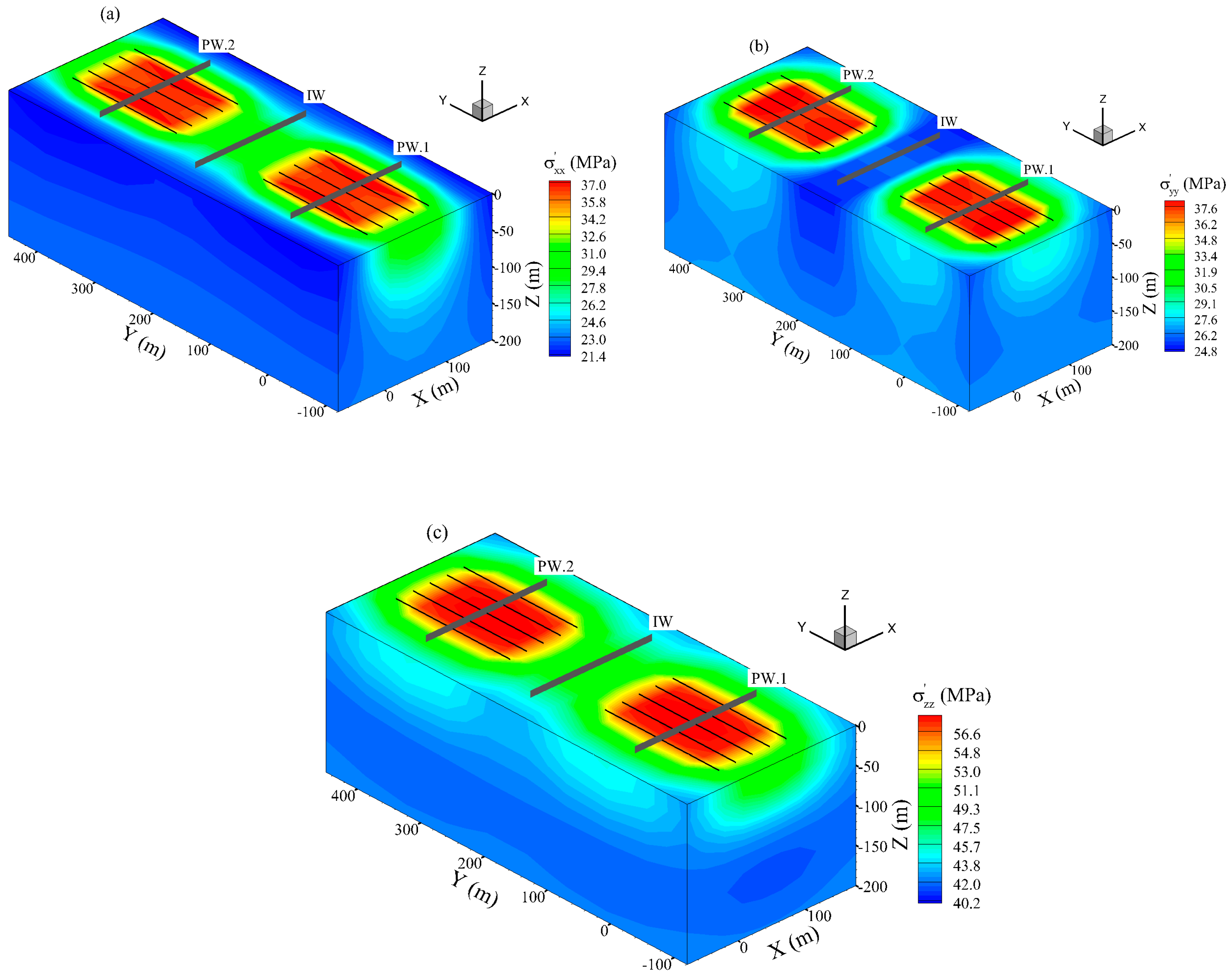


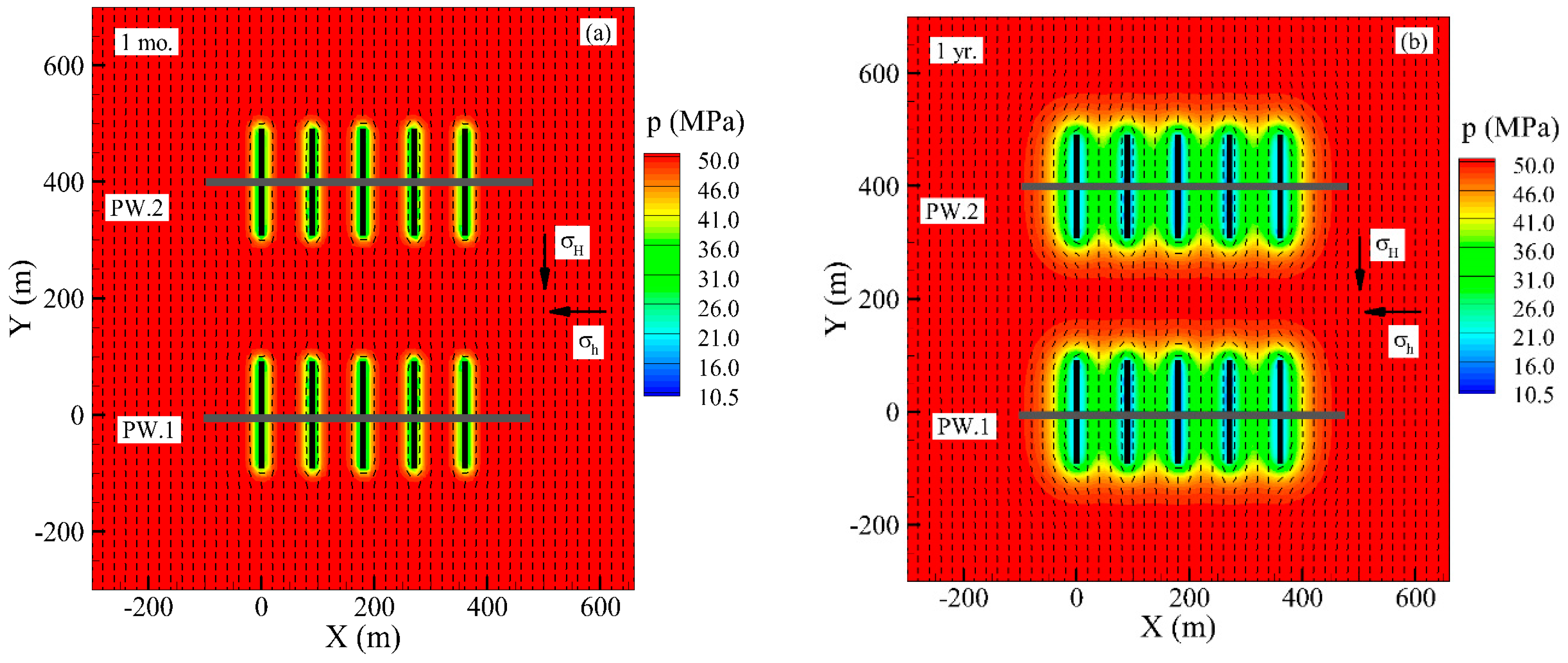
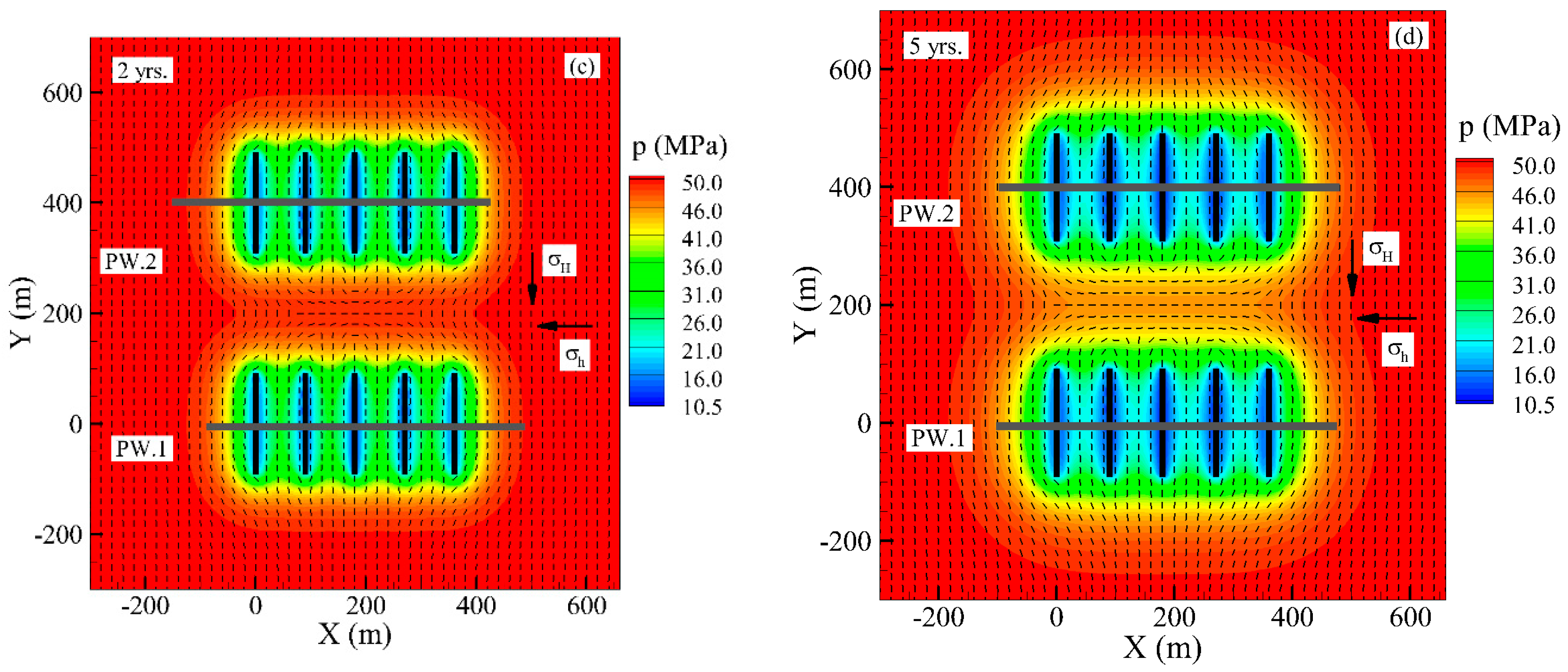



| Parameter | Layer-1 | Layer-2 | Layer-3 | Layer-4 | Layer-5 |
|---|---|---|---|---|---|
| Layer thickness, d (m) | 3.35 | 8.5 | 26.2 | 33.8 | 28.0 |
| True vertical depth, h (m) | 2696.22 | 2699.57 | 2708.07 | 2734.27 | 2773.07 |
| Young’s modulus, E (GPa) | 57.28 | 56.88 | 53.57 | 53.64 | 60.1 |
| Poisson’s ratio (ν) | 0.29 | 0.29 | 0.29 | 0.29 | 0.29 |
| Permeability, k (μd) | 0.07 | 1.84 | 3.04 | 2.96 | 1.84 |
| Porosity, ϕ (%) | 2.1 | 3.6 | 4.5 | 4.0 | 3.3 |
| Biot’s coefficient (α) | 0.65 | 0.65 | 0.65 | 0.65 | 0.65 |
| Parameter | Value |
|---|---|
| Fluid viscosity, μ (Pa.s) | 0.00025 |
| Fluid compressibility, (MPa−1) | 5.0 × 10−4 |
| Vertical stress () gradient (MPa/m) | 0.0266 |
| Max. horizontal stress ( ) gradient (MPa/m) | 0.021 |
| Min. horizontal stress ( gradient (MPa/m) | 0.0198 |
| Reservoir pore pressure (p) gradient (MPa/m) | 0.0184 |
| Fracture toughness, (MPa.m0.5) | 2.0 |
| Fracture normal stiffness, (GPa/m) | 300.0 |
| Fracture shear stiffness, (GPa/m) | 3000.0 |
Publisher′s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Masouleh, S.F.; Kumar, D.; Ghassemi, A. Three-Dimensional Geomechanical Modeling and Analysis of Refracturing and “Frac-Hits” in Unconventional Reservoirs. Energies 2020, 13, 5352. https://doi.org/10.3390/en13205352
Masouleh SF, Kumar D, Ghassemi A. Three-Dimensional Geomechanical Modeling and Analysis of Refracturing and “Frac-Hits” in Unconventional Reservoirs. Energies. 2020; 13(20):5352. https://doi.org/10.3390/en13205352
Chicago/Turabian StyleMasouleh, Shahla Feizi, Dharmendra Kumar, and Ahmad Ghassemi. 2020. "Three-Dimensional Geomechanical Modeling and Analysis of Refracturing and “Frac-Hits” in Unconventional Reservoirs" Energies 13, no. 20: 5352. https://doi.org/10.3390/en13205352
APA StyleMasouleh, S. F., Kumar, D., & Ghassemi, A. (2020). Three-Dimensional Geomechanical Modeling and Analysis of Refracturing and “Frac-Hits” in Unconventional Reservoirs. Energies, 13(20), 5352. https://doi.org/10.3390/en13205352





