Numerical Simulation and Experimental Investigation of Temperature and Residual Stress Distributions in a Circular Patch Welded Structure
Abstract
1. Introduction
2. Experimental Investigations
2.1. Model Geometry, Welding Conditions and Material Properties
2.2. Temperature Measurements
3. Numerical Model
3.1. Thermal Analysis
3.2. Mechanical Analysis
3.3. Finite Element Mesh
4. Results and Discussion
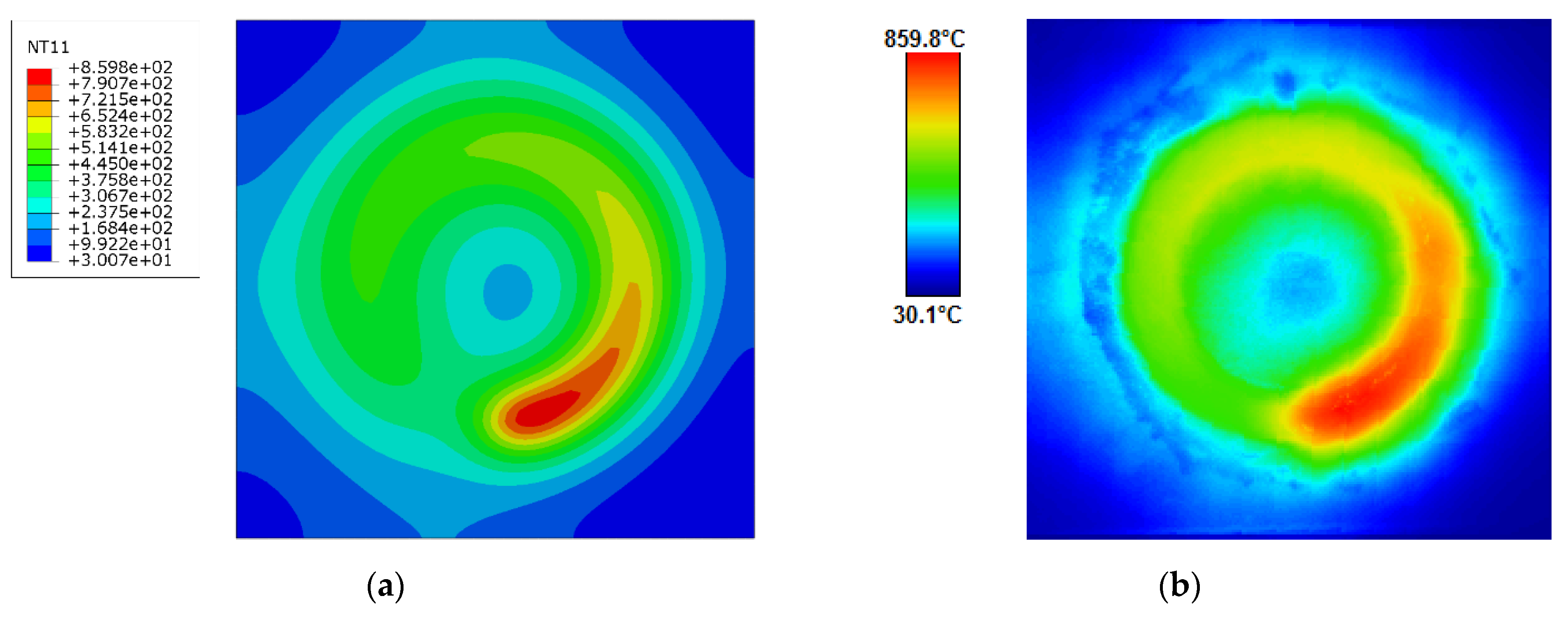
4.1. Temperature Field Distribution
4.2. Residual Stress Field Distribution
5. Conclusions
- Temperature histories recorded by thermocouples match well with the numerical calculated ones. The effect of simplified heat flux affects the temperature distribution in the weld bead and its close vicinity, while this effect on the temperature distribution away from the weld bead is significantly smaller.
- By measuring temperatures with the application of an IR camera on the upper surface of the model, a good correlation with the numerical simulation results was confirmed, whereas the largest deviations of the results were found in the weld bead and its vicinity.
- The numerical simulation residual stress results showed that the circumferential and radial residual stresses within the circular disk are primarily tensile.
- The circumferential residual stresses turn from tensile to compressive outside the disk. The radial residual stresses disappear towards the ends of the plate.
- The residual stress symmetries also show a steady state tendency following the path of the electrode, except in the welding start/end area.
Author Contributions
Funding
Conflicts of Interest
References
- Ryu, H.-S.; Kang, S.; Lee, K. Numerical Analysis and Experiments of Butt Welding Deformations for Panel Block Assembly. Appl. Sci. 2020, 10, 1669. [Google Scholar] [CrossRef]
- Lorza, R.L.; García, R.E.; Martinez, R.F.; Martinez Calvo, M.Á. Using Genetic Algorithms with Multi-Objective Optimization to Adjust Finite Element Models of Welded Joints. Metals 2018, 8, 230. [Google Scholar] [CrossRef]
- Perić, M.; Garašić, I.; Nižetić, S.; Dedić-Jandrek, H. Numerical Analysis of Longitudinal Residual Stresses and Deflections in a T-joint Welded Structure Using a Local Preheating Technique. Energies 2018, 11, 3487. [Google Scholar] [CrossRef]
- Chen, X.; Xu, H.; Xu, G.; Singh, A.R.; Jayalakshmi, S.; Konovalov, S.; Gupta, M. Stress and Temperature Distribution Simulation for Arc Weld-based Rapid Prototyping of Titanium Alloy Tc4. Mater. Express 2019, 9, 99–111. [Google Scholar] [CrossRef]
- Kik, T. Heat Source Models in Numerical Simulations of Laser Welding. Materials 2020, 13, 2653. [Google Scholar] [CrossRef] [PubMed]
- Gao, H.; Wu, S.; Wu, Q.; Li, B.; Gao, Z.; Zhang, Y.; Mo, S. Experimental and Simulation Investigation on Thermal-Vibratory Stress Relief Process for 7075 Aluminium Alloy. Mater. Des. 2020, 195, 108954. [Google Scholar] [CrossRef]
- Gao, H.; Zhang, Y.; Wu, Q.; Song, J.; Wen, K. Fatigue Life of 7075-t651 Aluminium Alloy Treated with Vibratory Stress Relief. Int. J. Fatigue 2018, 108, 62–67. [Google Scholar] [CrossRef]
- Chen, Y.; Sun, S.; Zhang, T.; Zhou, X.; Li, S. Effects of Post-Weld Heat Treatment on the Microstructure and Mechanical Properties of Laser-Welded NiTi/304SS Joint with Ni Filler. Mater. Sci. Eng. A 2020, 771, 138545. [Google Scholar] [CrossRef]
- Dong, P.; Song, S.; Zhang, J. Analysis of Residual Stress Relief Mechanisms in Post-Weld Heat Treatment. Int. J. Press. Vessel. Pip. 2014, 122, 6–14. [Google Scholar] [CrossRef]
- Karalis, D. Increasing the Efficiency of Computational Welding Mechanics by Combining Solid and Shell Elements. Mater. Today Commun. 2020, 22, 100836. [Google Scholar] [CrossRef]
- Lorza, R.L.; Bobadilla, M.C.; Calvo, M.; Ángeles, M.; Roldán, P.M.V. Residual Stresses with Time-Independent Cyclic Plasticity in Finite Element Analysis of Welded Joints. Metals 2017, 7, 136. [Google Scholar] [CrossRef]
- Seleš, K.; Perić, M.; Tonković, Z. Numerical Simulation of a Welding Process Using a Prescribed Temperature Approach. J. Constr. Steel Res. 2018, 145, 49–57. [Google Scholar] [CrossRef]
- Walker, T.; Bennett, C.J. An Automated Inverse Method to Calibrate Thermal Finite Element Models for Numerical Welding Applications. J. Manuf. Process. 2019, 47, 263–283. [Google Scholar] [CrossRef]
- Xiong, Y.; Li, C.; Chen, Z.; He, J.; Xin, H. The Evolution of Residual Stress in Rib-Diaphragm Joints of Orthotropic Steel Decks Subjected to Thermal Cutting and Welding. Materials 2020, 13, 3804. [Google Scholar] [CrossRef] [PubMed]
- Chandrasekaran, R.R.; Benoit, M.J.; Barrett, J.M.; Gerlich, A.P. Multi-Variable Statistical Models for Predicting Bead Geometry in Gas Metal Arc Welding. Int. J. Adv. Manuf. Technol. 2019, 105, 1573–1584. [Google Scholar] [CrossRef]
- Tian, L.; Luo, Y. The Effect of Process Parameters and Plate Thickness on in-Plane Inherent Deformations in T-Joint Fillet Weld. Eng. Comput. 2020. [Google Scholar] [CrossRef]
- Adamczuk, P.C.; Machado, I.G.; Mazzaferro, J.A.E. Methodology for Predicting the Angular Distortion in Multi-Pass Butt-Joint Welding. J. Mater. Process. Technol. 2017, 240, 305–313. [Google Scholar] [CrossRef]
- Costa, E.S.; Assunção, P.D.C.; Dos Santos, E.B.F.; Feio, L.G.; Bittencourt, M.S.Q.; Braga, E.M. Residual Stresses in Cold-Wire Gas Metal Arc Welding. Sci. Technol. Weld. Join. 2017, 22, 706–713. [Google Scholar] [CrossRef]
- Heinze, C.; Schwenk, C.; Rethmeier, M. Effect of Heat Source Configuration on the Result Quality of Numerical Calculation of Welding-Induced Distortion. Simul. Model. Pract. Theory 2012, 20, 112–123. [Google Scholar] [CrossRef]
- Kung, C.-L.; Hung, C.-K.; Hsu, C.-M.; Chen, C.-Y. Residual Stress and Deformation Analysis in Butt Welding on 6 mm SUS304 Steel with Jig Constraints Using Gas Metal Arc Welding. Appl. Sci. 2017, 7, 982. [Google Scholar] [CrossRef]
- Deng, D.; Zhou, Y.; Bi, T.; Liu, X. Experimental and Numerical Investigations of Welding Distortion Induced by CO2 Gas Arc Welding in Thin-Plate Bead-on Joints. Mater. Des. 2013, 52, 720–729. [Google Scholar] [CrossRef]
- Deng, D.; Liang, W.; Murakawa, H. Determination of Welding Deformation in Fillet-Welded Joint by Means of Numerical Simulation and Comparison with Experimental Measurements. J. Mater. Process. Technol. 2007, 183, 219–225. [Google Scholar] [CrossRef]
- Perić, M.; Tonković, Z.; Rodić, A.; Surjak, M.; Garašić, I.; Boras, I.; Švaić, S. Numerical Analysis and Experimental Investigation of Welding Residual Stresses and Distortions in a T-Joint Fillet Weld. Mater. Des. 2014, 53, 1052–1063. [Google Scholar] [CrossRef]
- Perić, M.; Tonković, Z.; Karsaj, I.; Stamenković, D. A Simplified Engineering Method for a T-Joint Welding Simulation. Therm. Sci. 2018, 22, 20. [Google Scholar] [CrossRef]
- Prajadhiana, K.P.; Manurung, Y.; Sulaiman, M.S.; Adenan, M.S. Investigation on Welded T-Joint Distortion Using Virtual Manufacturing Tools with Simplified Procedure. J. Korean Soc. Precis. Eng. 2020, 37, 91–97. [Google Scholar] [CrossRef]
- Deng, D.; Murakawa, H.; Liang, W. Prediction of Welding Distortion in a Curved Plate Structure by Means of Elastic Finite Element Method. J. Mater. Process. Technol. 2008, 203, 252–266. [Google Scholar] [CrossRef]
- Azad, N.; Iranmanesh, M.; Darvazi, A.R. A Study on the Effect of Welding Sequence on Welding Distortion in Ship Deck Structure. Ships Offshore Struct. 2019, 15, 355–367. [Google Scholar] [CrossRef]
- Zhang, Y.; Wang, Y. The Influence of Welding Mechanical Boundary Condition on the Residual Stress and Distortion of a Stiffened-Panel. Mar. Struct. 2019, 65, 259–270. [Google Scholar] [CrossRef]
- Podder, D.; Gupta, O.P.; Das, S.; Mandal, N.R. Experimental and Numerical Investigation of Effect of Welding Sequence on Distortion of Stiffened Panels. Weld. World 2019, 63, 1275–1289. [Google Scholar] [CrossRef]
- Perić, M.; Seleš, K.; Tonković, Z.; Lovrenić-Jugović, M. Numerical Simulation of Welding Distortions in Large Structures with a Simplified Engineering Approach. Open Phys. 2019, 17, 719–730. [Google Scholar] [CrossRef]
- Wu, C.; Lee, C.; Kim, J.-W. Numerical Simulation of Bending Deformation Induced by Multi-Seam Welding of a Steel-Pipe Structure. J. Mech. Sci. Technol. 2020, 34, 2121–2131. [Google Scholar] [CrossRef]
- Zhao, M.; Wei, F.; Huang, W.Q.; Lei, Y. Experimental and Numerical Investigation on Combined Girth Welding of API X80 Pipeline Steel. Sci. Technol. Weld. Join. 2015, 20, 622–630. [Google Scholar] [CrossRef]
- Lin, J.; Ma, N.; Lei, Y.; Murakawa, H. Measurement of Residual Stress in Arc Welded Lap Joints by Cosα X-Ray Diffraction Method. J. Mater. Process. Technol. 2017, 243, 387–394. [Google Scholar] [CrossRef]
- Baba, H.; Era, T.; Ueyama, T.; Tanaka, M. Single Pass Full Penetration Joining for Heavy Plate Steel Using High Current GMA Process. Weld. World 2017, 61, 963–969. [Google Scholar] [CrossRef]
- Perić, M.; Garašić, I.; Tonković, Z.; Vuherer, T.; Nižetić, S.; Dedić-Jandrek, H. Numerical Prediction and Experimental Validation of Temperature and Residual Stress Distributions in Buried-Arc Welded Thick Plates. Int. J. Energy Res. 2019, 43, 3590–3600. [Google Scholar] [CrossRef]
- Perić, M.; Nižetić, S.; Garašić, I.; Gubeljak, N.; Vuherer, T.; Tonković, Z. Numerical Calculation and Experimental Measurement of Temperatures and Welding Residual Stresses in a Thick-Walled T-Joint Structure. J. Therm. Anal. Calorim. 2020, 141, 313–322. [Google Scholar] [CrossRef]
- Charkhi, M.; Akbari, D. Experimental and Numerical Investigation of the Effects of the Pre-Heating in the Modification of Residual Stresses in the Repair Welding Process. Int. J. Press. Vessel. Pip. 2019, 171, 79–91. [Google Scholar] [CrossRef]
- Salerno, G.; Bennett, C.J.; Sun, W.; Becker, A.A. Residual Stress Analysis and Finite Element Modelling of Repair-Welded Titanium Sheets. Weld. World 2017, 61, 1211–1223. [Google Scholar] [CrossRef]
- Jiang, W.; Liu, Z.; Gong, J.; Tu, S. Numerical Simulation to Study the Effect of Repair Width on Residual Stresses of a Stainless Steel Clad Plate. Int. J. Press. Vessel. Pip. 2010, 87, 457–463. [Google Scholar] [CrossRef]
- Pilipenko, A. Computer Simulation of Residual Stress and Distortion of Thick Plates in Multi-Electrode Submerged Arc Welding: Their Mitigation Techniques. Ph.D. Thesis, Norwegian University of Science and Technology, Trondheim, Norway, 2001. [Google Scholar]
- Nasouri, R.; Nguyen, K.; Montoya, A.; Matamoros, A.; Bennett, C.; Li, J. Simulating the Hot Dip Galvanizing Process of High Mast Illumination Poles. Part I: Finite Element Model Development. J. Constr. Steel Res. 2019, 162, 105705. [Google Scholar] [CrossRef]
- Nguyen, K.; Nasouri, R.; Bennett, C.R.; Matamoros, A.; Li, J.; Montoya, A.H. Thermomechanical Modeling of Welding and Galvanizing a Steel Beam Connection Detail to Examine Susceptibility to Cracking. Mater. Perform. Charact. 2018, 7, 165–190. [Google Scholar] [CrossRef]
- Deng, D. FEM Prediction of Welding Residual Stress and Distortion in Carbon Steel Considering Phase Transformation Effects. Mater. Des. 2009, 30, 359–366. [Google Scholar] [CrossRef]
- Perić, M.; Tonković, Z.; Maksimović, K.S.; Stamenković, D. Numerical Analysis of Residual Stresses in a T-Joint Fillet Weld Using a Submodeling Technique. FME Trans. 2019, 47, 183–189. [Google Scholar] [CrossRef]
- Perić, M.; Tonković, Z.; Garašić, I.; Vuherer, T. An Engineering Approach for a T-Joint Fillet Welding Simulation Using Simplified Material Properties. Ocean Eng. 2016, 128, 13–21. [Google Scholar] [CrossRef]
- Deng, D.; Kiyoshima, S. FEM Prediction of Welding Residual Stresses in a SUS304 Girth-Welded Pipe with Emphasis on Stress Distribution Near Weld Start/End Location. Comput. Mater. Sci. 2010, 50, 612–621. [Google Scholar] [CrossRef]

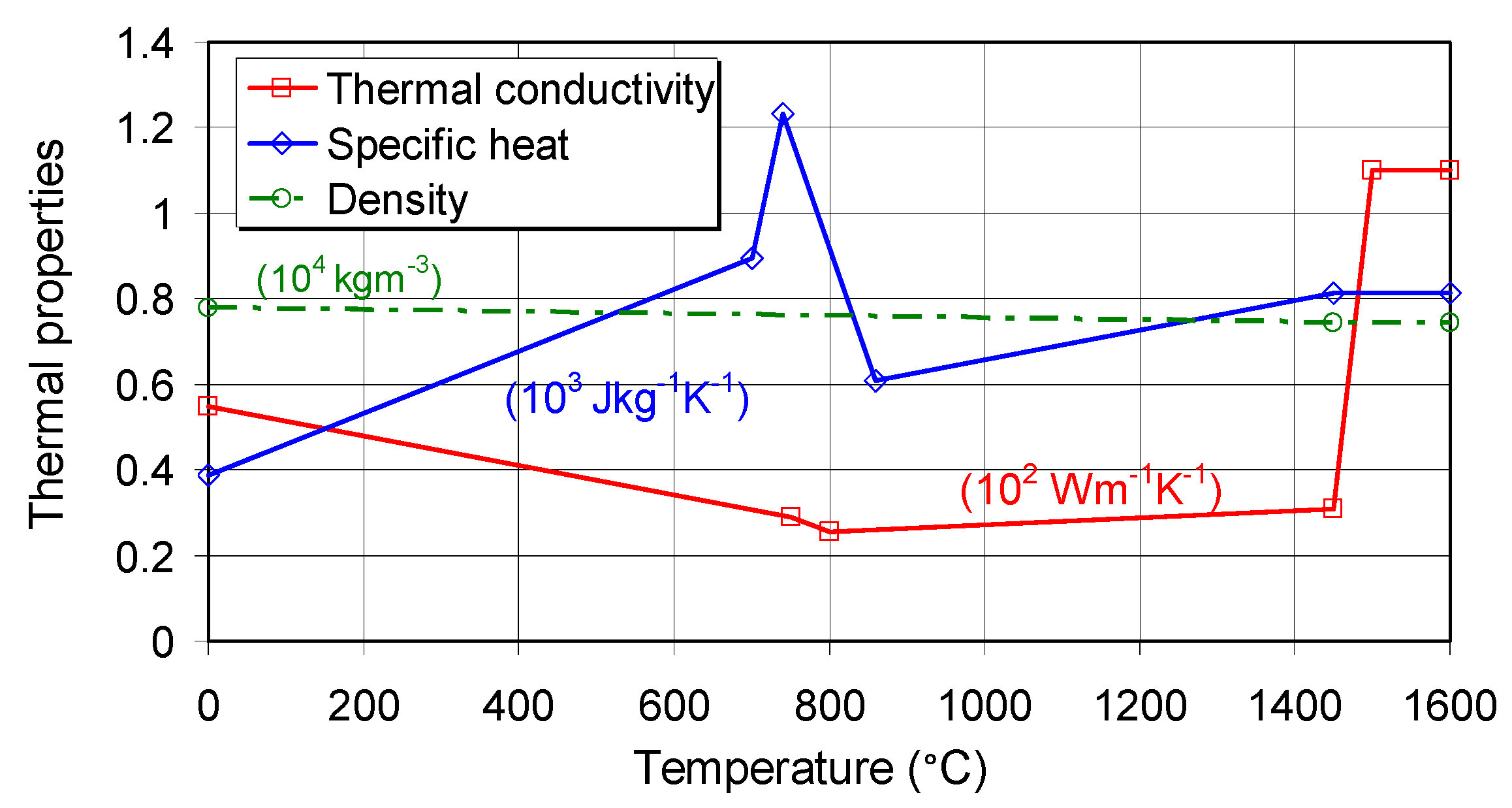





| C | Si | Mn | P | S | N | Cu | Cr | Ni | Mo | Al | V | Ti | Nb |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.17 | 0.24 | 1.25 | 0.016 | 0.006 | 0.008 | 0.23 | 0.06 | 0.1 | 0.11 | 0.0032 | 0.005 | 0.025 | 0.033 |
| Welding Current I | Welding Voltage U | Heat Input Efficiency η | Welding Speed | Wire Diameter | Wire Feed Speed | Shielding Gas Composition | Shielding Gas Flow |
|---|---|---|---|---|---|---|---|
| 540 A | 41 V | 0.85 | 304 mm/min | 1.6 mm | 10.4 m/min | 100% CO2 | 25 L/min |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Perić, M.; Nižetić, S.; Tonković, Z.; Garašić, I.; Horvat, I.; Boras, I. Numerical Simulation and Experimental Investigation of Temperature and Residual Stress Distributions in a Circular Patch Welded Structure. Energies 2020, 13, 5423. https://doi.org/10.3390/en13205423
Perić M, Nižetić S, Tonković Z, Garašić I, Horvat I, Boras I. Numerical Simulation and Experimental Investigation of Temperature and Residual Stress Distributions in a Circular Patch Welded Structure. Energies. 2020; 13(20):5423. https://doi.org/10.3390/en13205423
Chicago/Turabian StylePerić, Mato, Sandro Nižetić, Zdenko Tonković, Ivica Garašić, Ivan Horvat, and Ivanka Boras. 2020. "Numerical Simulation and Experimental Investigation of Temperature and Residual Stress Distributions in a Circular Patch Welded Structure" Energies 13, no. 20: 5423. https://doi.org/10.3390/en13205423
APA StylePerić, M., Nižetić, S., Tonković, Z., Garašić, I., Horvat, I., & Boras, I. (2020). Numerical Simulation and Experimental Investigation of Temperature and Residual Stress Distributions in a Circular Patch Welded Structure. Energies, 13(20), 5423. https://doi.org/10.3390/en13205423






