Performance Evaluation of a Novel Thermal Power Plant Process with Low-Temperature Selective Catalytic Reduction
Abstract
1. Introduction
2. Model Descriptions for the Process Analysis
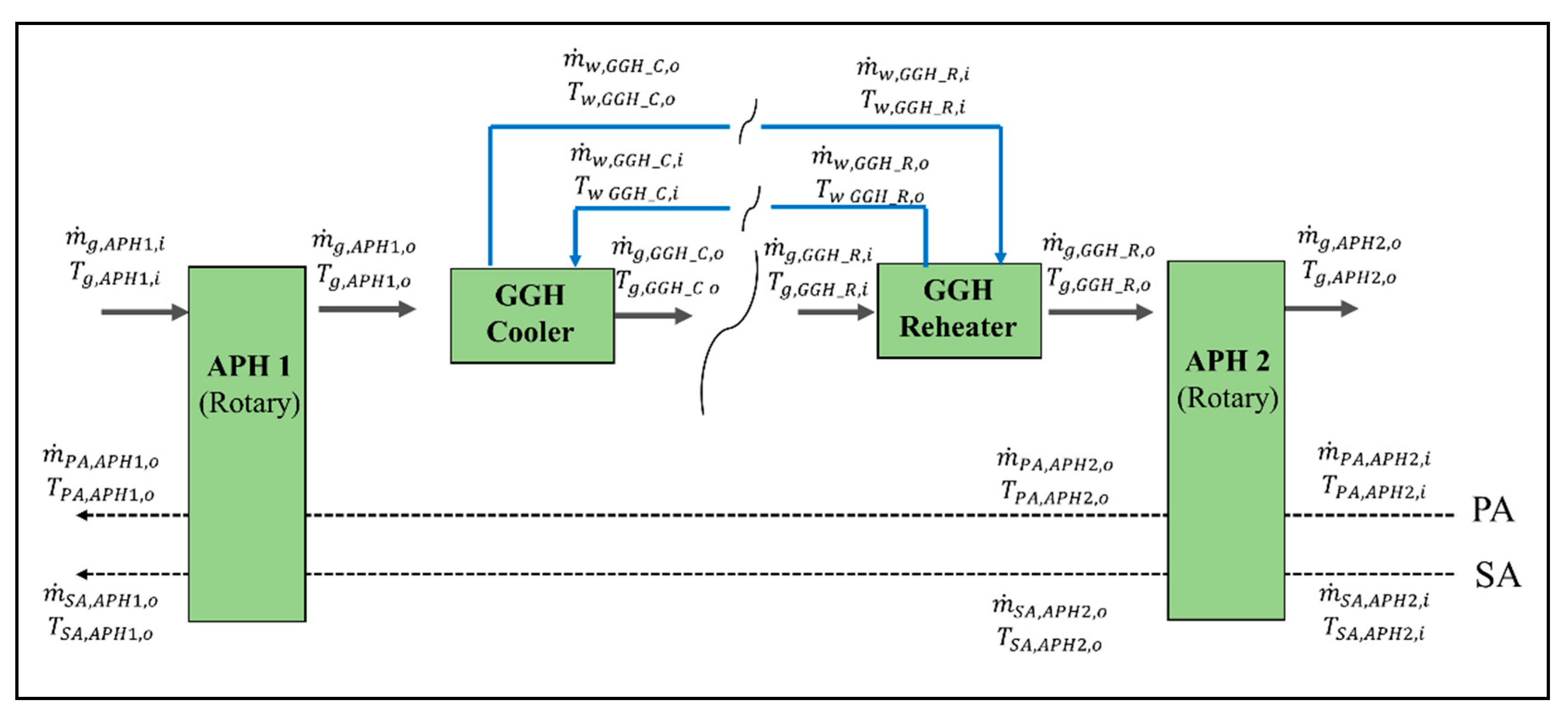
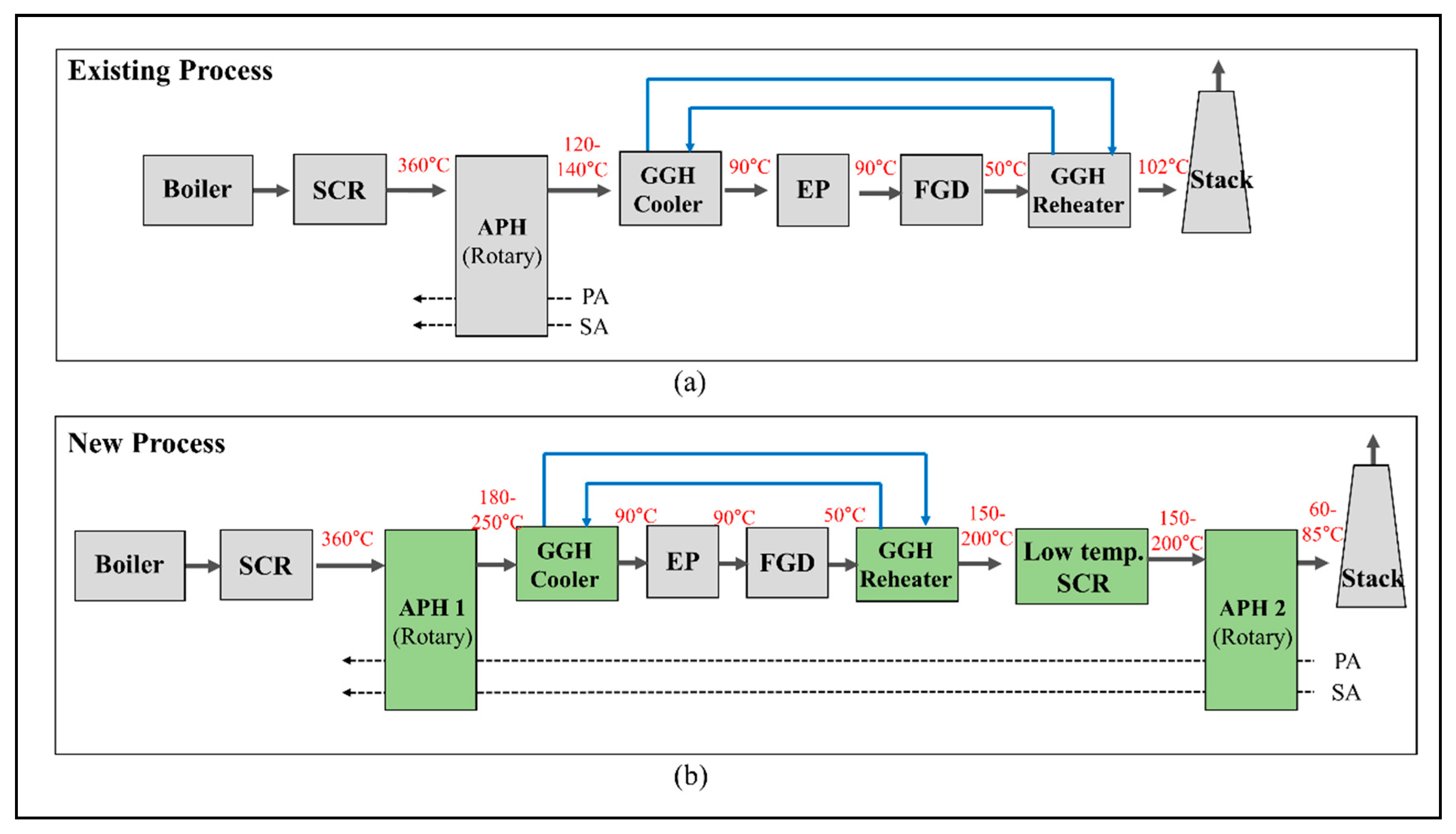
2.1. Target Power Plant
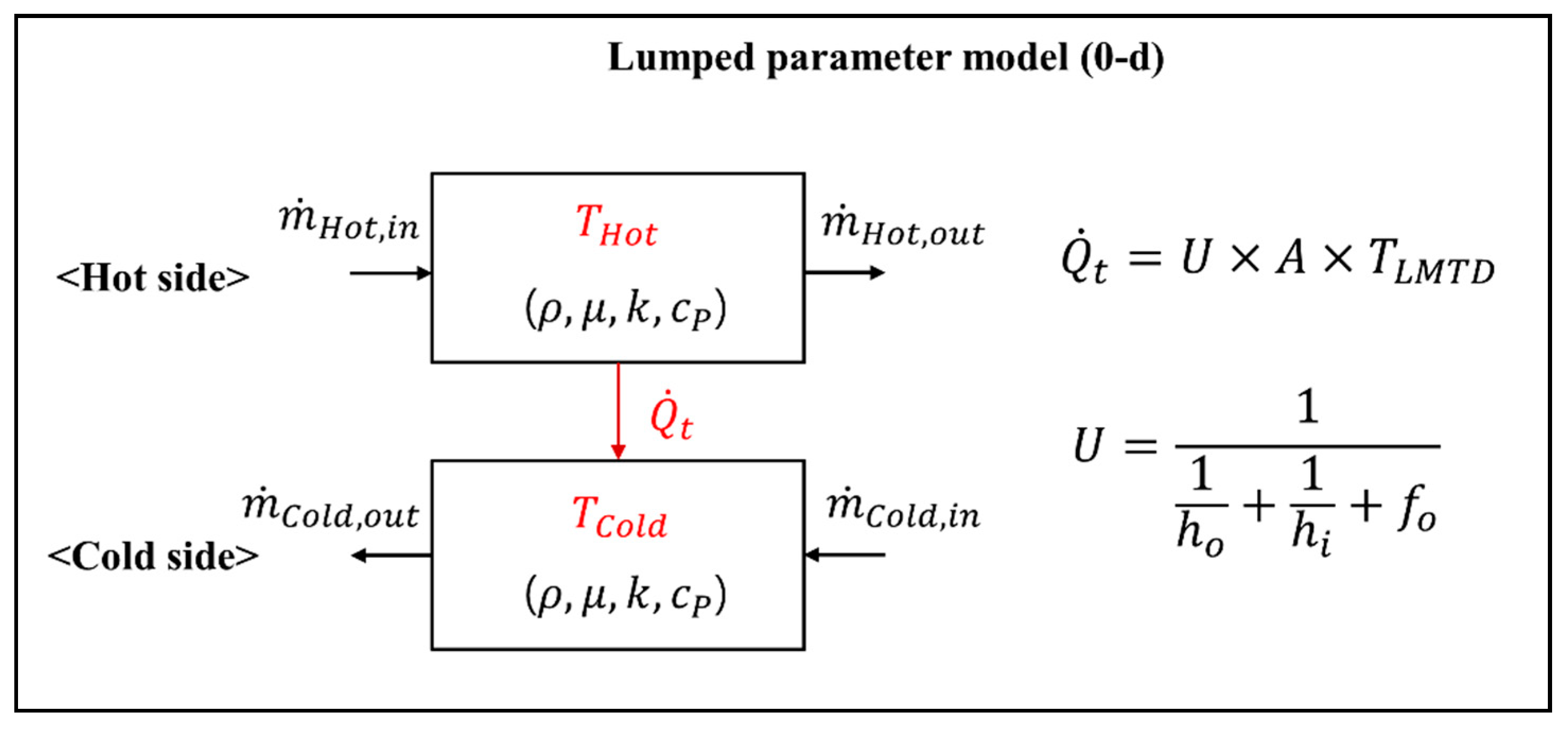
2.2. A Lumped Parameter Model for a Power Plant Heat Exchanger Block
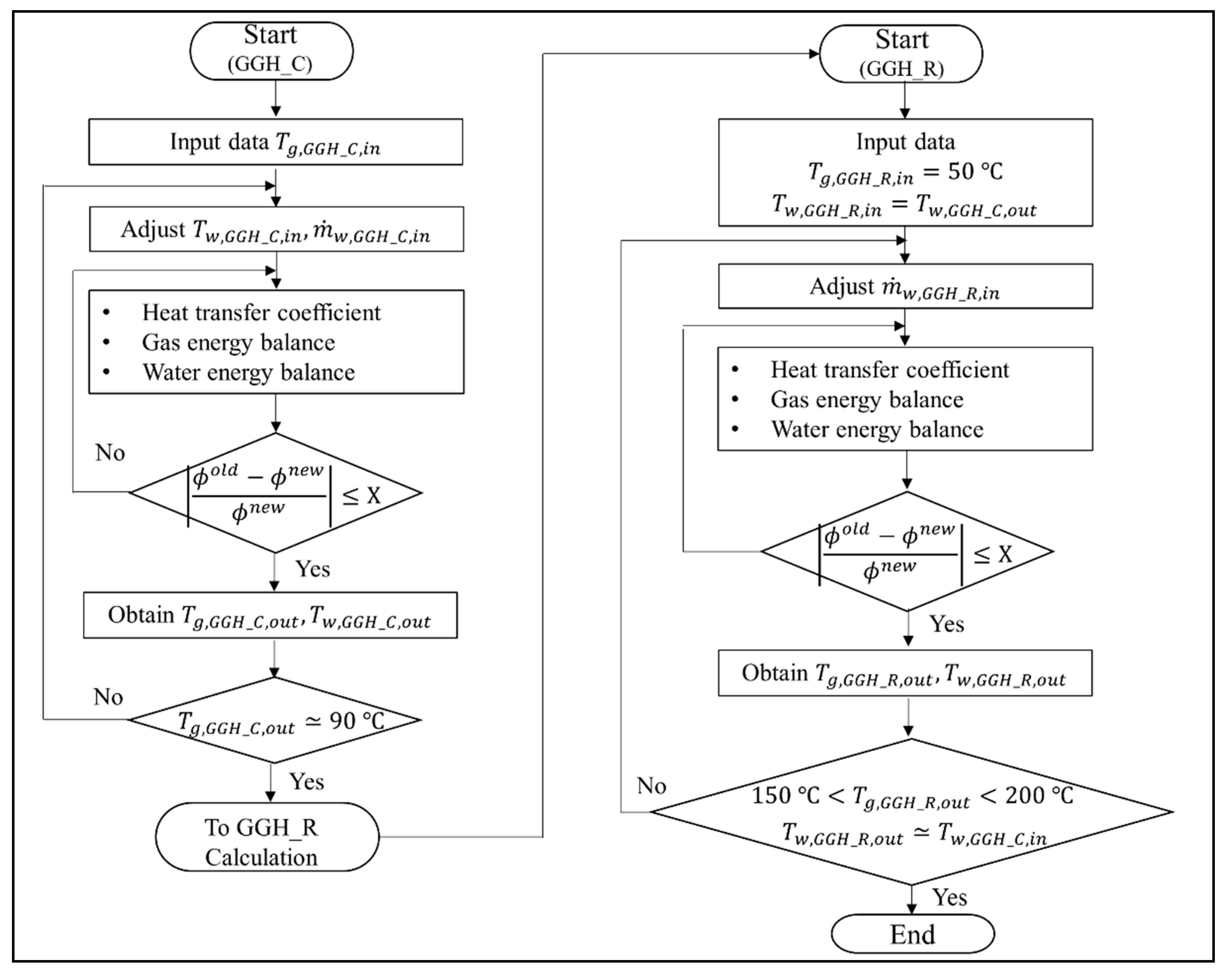
2.3. Modeling Methodology for the New Process
- (1)
- The energy balances for the APH 1, APH 2, GGH_C, and GGH_R were calculated in the post-gas flow system model. The exit temperature of the FGD has a constant value based on the design data of 50 °C for all load conditions.
- (2)
- The newly added APH 2 has the identical heat transfer area as the existing APH 1 and uses the suggested fitting equation for the HTC. The air leak rate from the design data was used for APH 1 and APH 2, and is listed in Table A2.
- (3)
- The exit temperature of the flue gas in the GGH_C should be near 90 °C, which is the optimum operating temperature in the FGD. The flue gas temperature at the exit of the GGH_R should range from 150 to 200 °C, which is the optimum operating temperature of the low-temperature SCR.
- (4)
- To minimize the use of the heat medium heater in the GGH, the operating conditions of the waterside of the GGH ensure the minimization of the temperature difference in the circulating water through the GGH_C and GGH_R. The water temperature of the GGH should be maintained below the saturation temperature at the working pressure to prevent steam generation.
- (5)
- The heat transfer area in the GGH for the new process was calculated using the required heat duty, TLMTD, and HTC values from the existing design data.
2.4. Process Analysis Model for the Existing and New Processes for the Target Power Plant
3. Process Simulation Results and Discussion
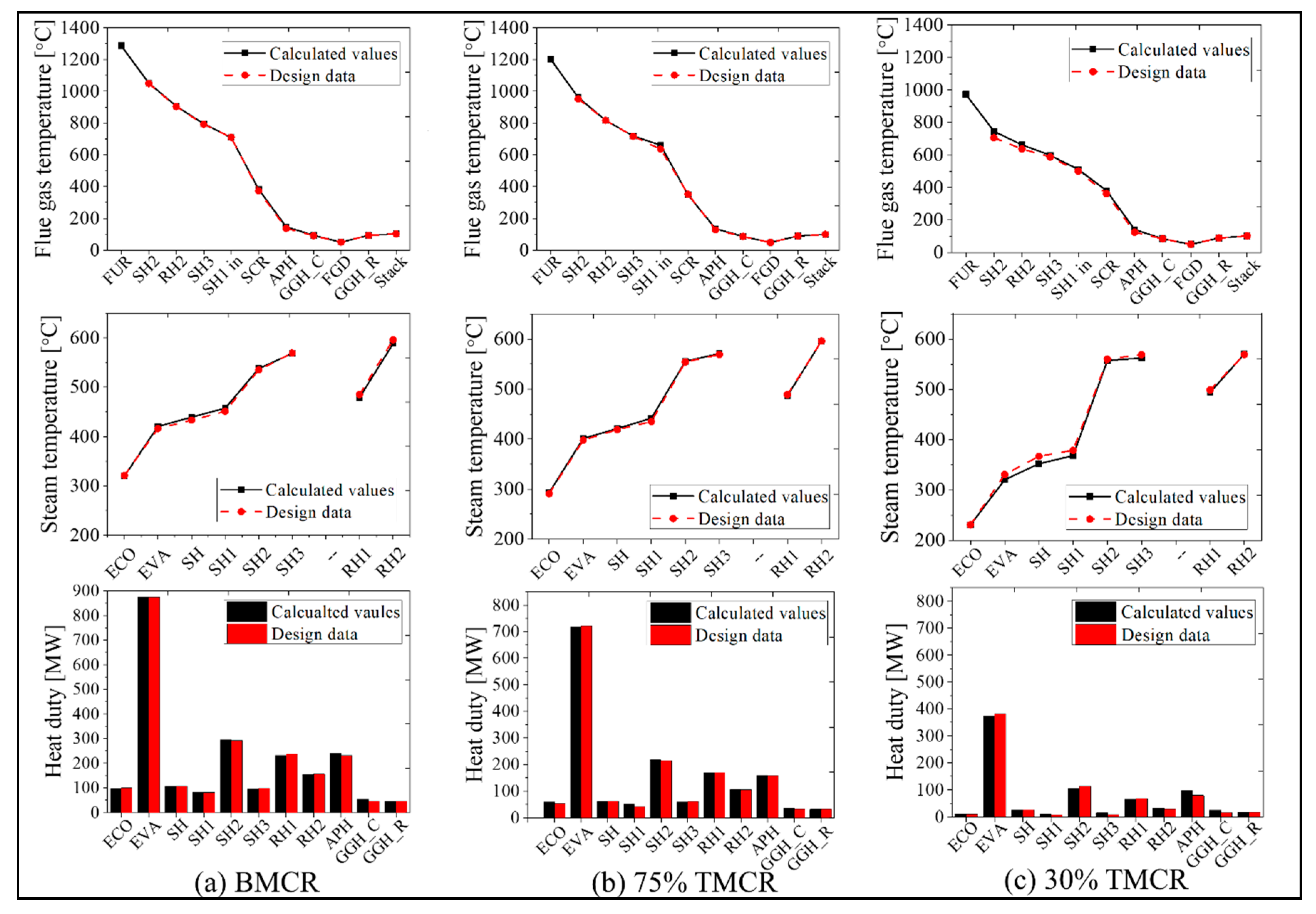
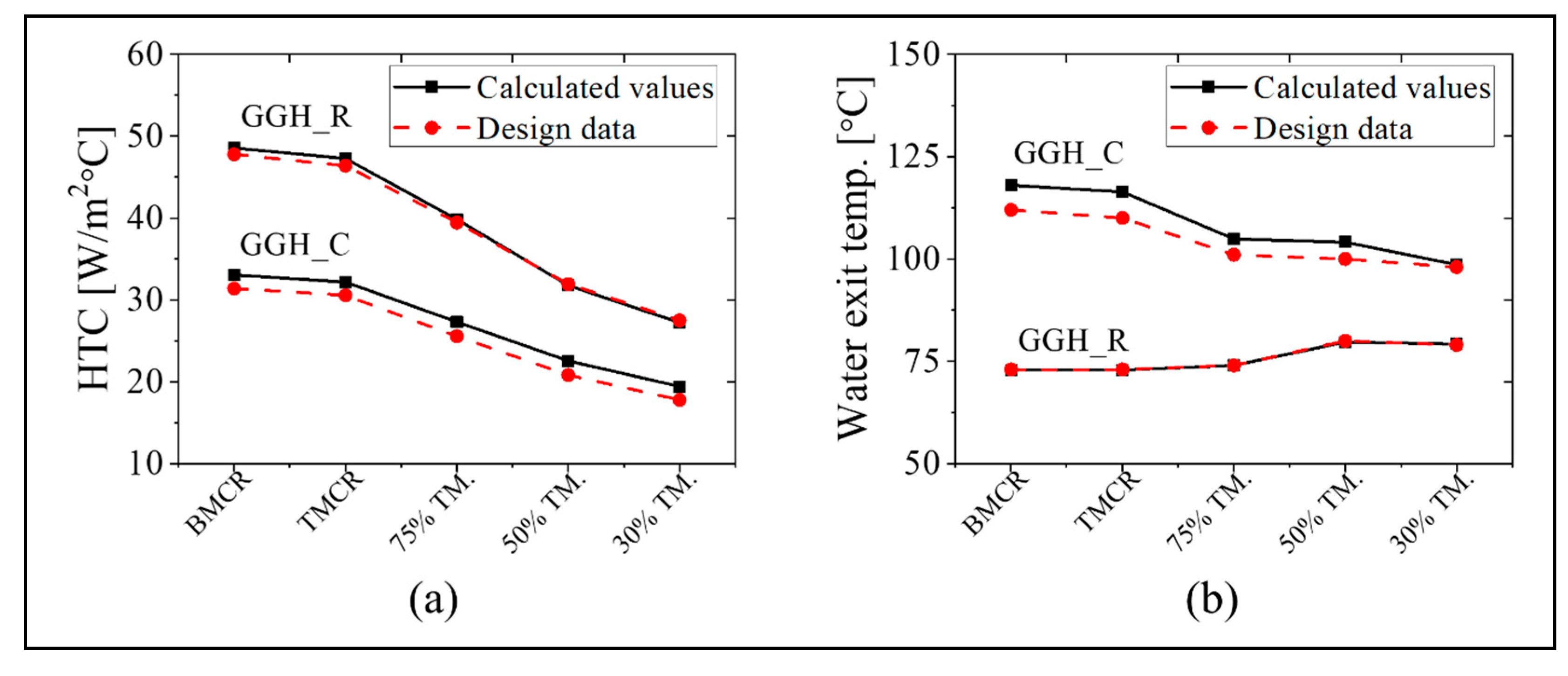
3.1. Validation of the Model for the Existing Process
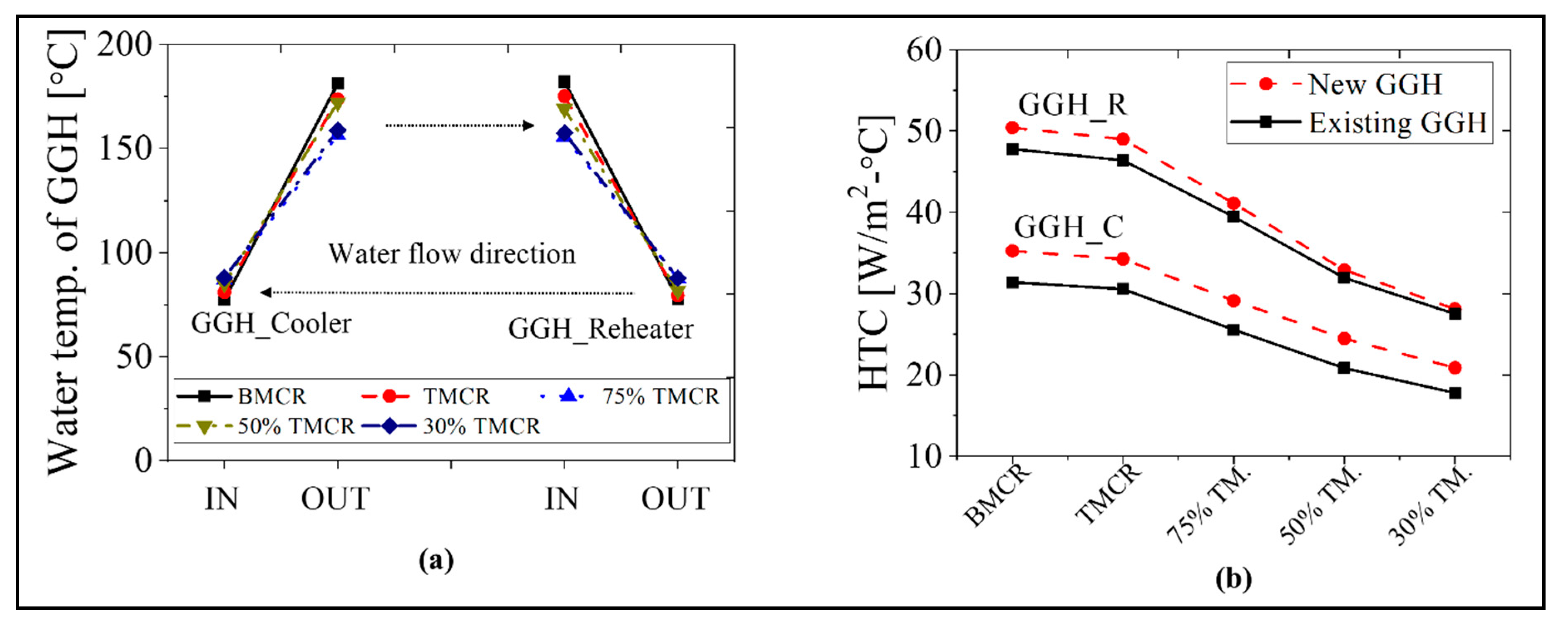
3.2. New Process Simulation Results
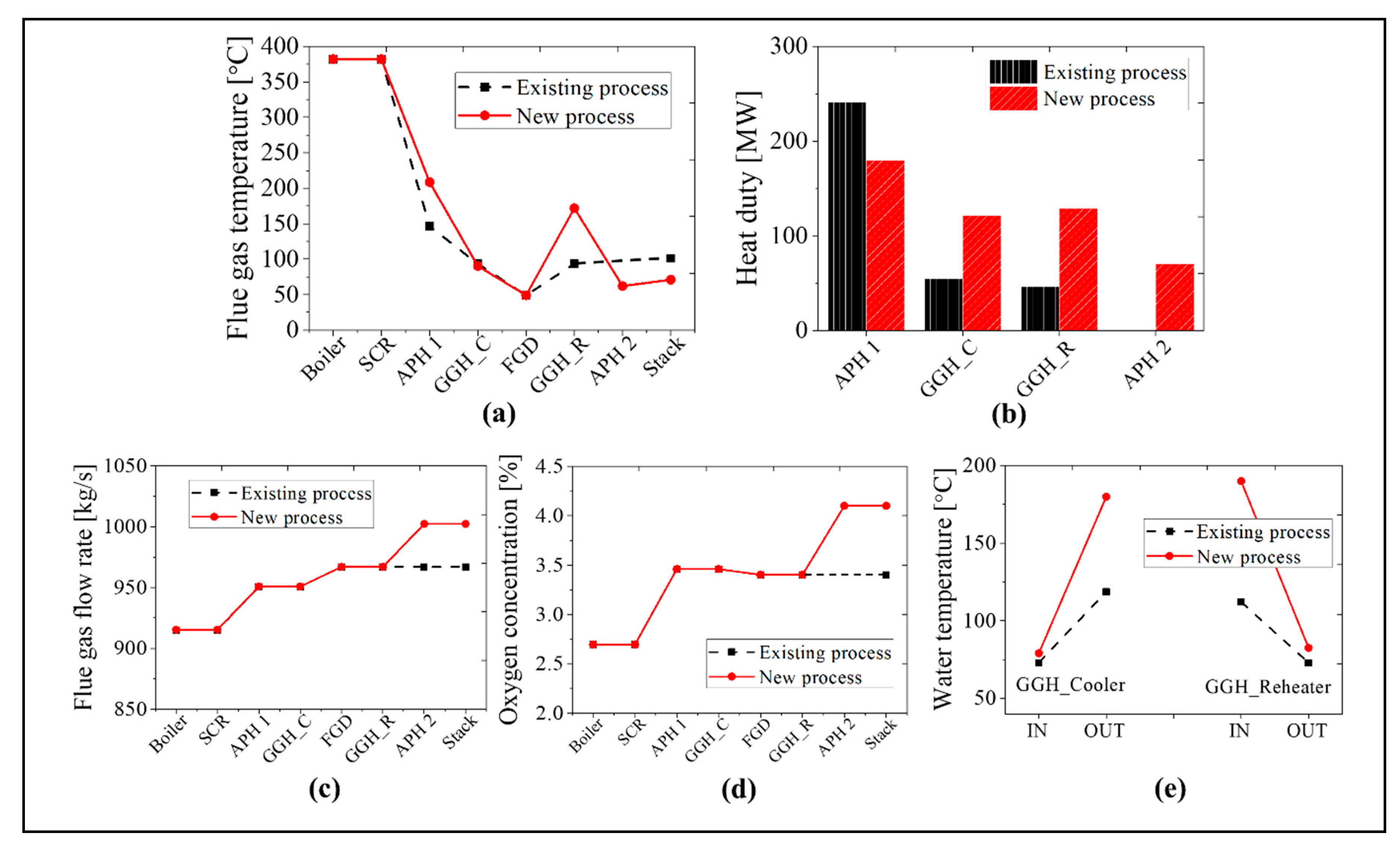
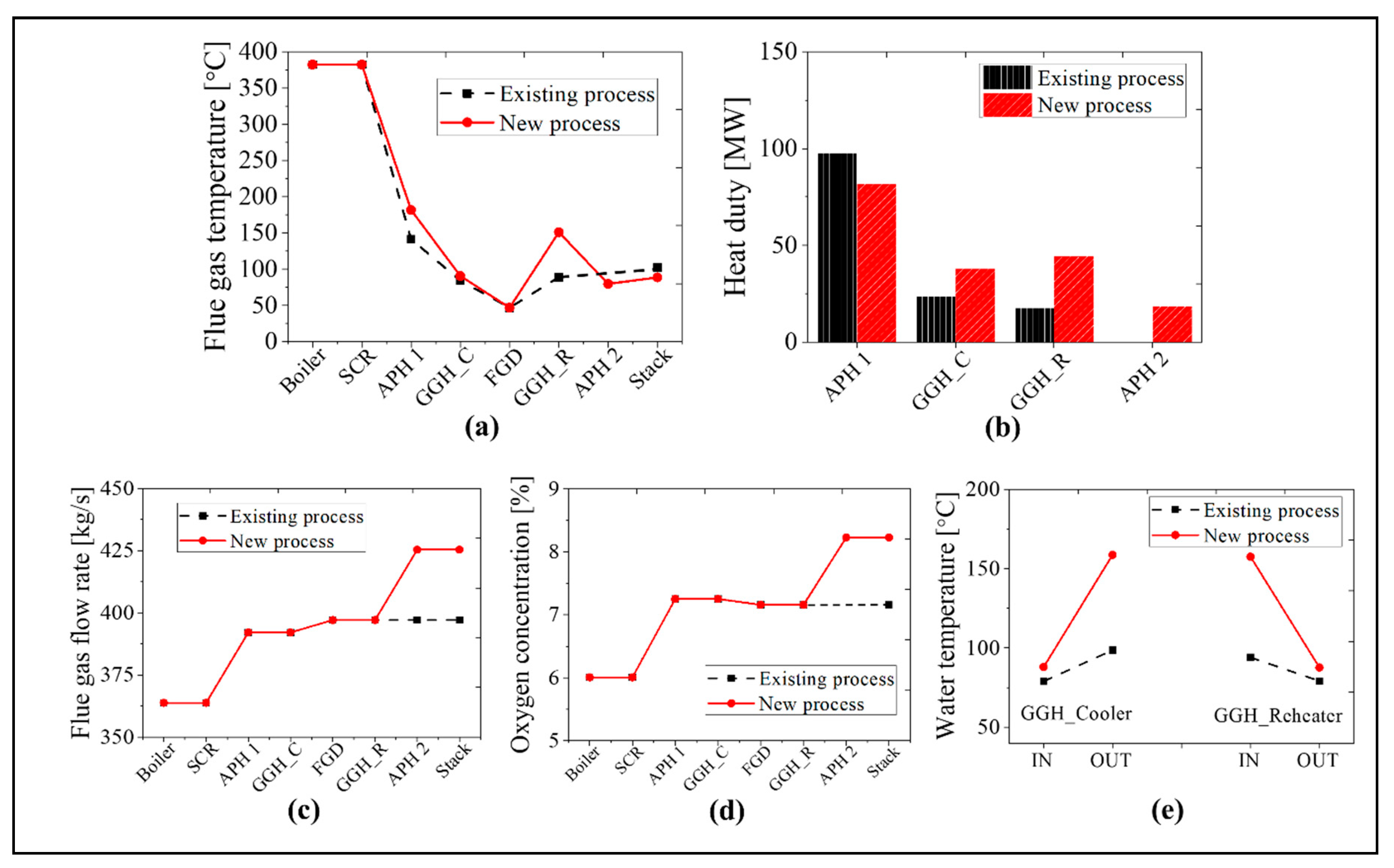
3.3. Comparison of the Existing and New Process
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Heat transfer surface area [m2] | |
| Specific heat [kJ/kg/°C] | |
| Pressure drop [MPa] | |
| Friction coefficient [-] | |
| Fouling factor [-] | |
| Gravity [m/s2] | |
| Enthalpy [kJ/kg] | |
| Outer heat transfer coefficient [kW/m2/°C] | |
| Inner heat transfer coefficient [kW/m2/°C] | |
| Thermal conductivity [kW/m-°C] | |
| Tube length [m] | |
| Mass flow rate [kg/s] | |
| Pressure [MPa] | |
| Heat transfer rate [kW] | |
| Temperature [°C] | |
| Overall heat transfer coefficient [kW/m2/°C] | |
| Velocity [m/s] | |
| Greek | |
| Viscosity [N∙s/m2] | |
| Density [kg/m3] | |
| Subscript | |
| g | Gas side |
| i | In |
| k | Gas species (CO2, N2, O2, H2O, and SO2) |
| o | Out |
| w | Water side |
| ws | Water-steam side |
| Abbreviation | |
| APH | Air preheater |
| BMCR | Boiler maximum continuous rating |
| BUF | Booster up fan |
| EAR | Excess air ratio |
| ECO | Economizer |
| EP | Electrostatic precipitator |
| FGD | Flue gas desulfurization |
| FUR | Furnace |
| FW | Feedwater |
| GGH_C | Gas–gas heater cooler |
| GGH_R | Gas–gas heater reheater |
| LMTD | Logarithmic mean temperature difference |
| HTC | Heat transfer coefficient |
| NR | Normal rating |
| PA | Primary air |
| RH | Reheater |
| SA | Secondary air |
| SCR | Selective catalyst reduction |
| SNCR | Selective non-catalyst reduction |
| SH | Superheater |
| TMCR | Turbine maximum continuous rating |
Appendix A. Calculation Process and Simulation Input Conditions

| Operating Conditions | BMCR | TMCR | 75% TMCR | 50% TMCR | 30% TMCR |
|---|---|---|---|---|---|
| Fuel [kg/s] | 97.93 | 93.87 | 71.53 | 49.80 | 32.54 |
| EAR | 1.15 | 1.15 | 1.15 | 1.15 | 1.4 |
| FW (Feed Water) mass flow [kg/s] | 710.5 | 674.2 | 488.8 | 320.2 | 201.5 |
| FW Temperature [] | 294 | 290 | 269 | 245 | 220 |
| FW Pressure [MPa] | 28.89 | 28.36 | 22.35 | 10.35 | 9.15 |
| RH mass flow [kg/s] | 604 | 574.6 | 428.2 | 287.7 | 184.4 |
| RH Temperature [] | 326 | 321 | 319 | 327 | 331 |
| RH Pressure [MPa] | 4.76 | 4.53 | 3.35 | 2.21 | 1.36 |
| Operating Conditions. | BMCR | TMCR | 75% TMCR | 50% TMCR | 30% TMCR | |
|---|---|---|---|---|---|---|
| Air leakage rate of APH [%] | 3.9 | 4.1 | 5.5 | 6.9 | 7.8 | |
| Air inlet Temperature [°C] | PA | 50 | 50 | 50 | 49 | 51 |
| SA | 45 | 44 | 43 | 51 | 43 | |
| Air flow rate [kg/s] | PA | 162 | 160 | 145 | 111 | 82 |
| SA | 645 | 614 | 461 | 313 | 263 | |
| Water inlet Temperature [°C] | GGH_C | 73 | 73 | 74 | 80 | 79 |
| GGH_R | 112 | 110 | 101 | 100 | 94 | |
| Water flow rate [kg/s] | GGH_C | 282 | 280 | 282 | 289 | 202 |
| GGH_R | 279 | 277 | 279 | 286 | 219 | |
Appendix B. Governing Equations for the New Process in the Post-Gas Treatment System
- APH 1 energy balance
- GGH_C energy balance
- GGH_R energy balance
- APH 2 energy balance
References
- Dolanc, G.; StrmcÏnik, S.; PetrovcÏic, J. NOX selective catalytic reduction control based on simple models. J. Process Control 2001, 11, 35–51. [Google Scholar] [CrossRef]
- Price, D.; Birnbaum, R.; Mccullouh, M.; Smith, R. Nitrogen Oxides: Impacts on Public Health and the Environment; EPA-452/R-97-002 (NTIS PB98-104631, August); Environmental Protection Agency: Washington, DC, USA, 1997. [Google Scholar]
- Boningari, T.; Smirniotis, P.G. Impact of nitrogen oxides on the environment and human health: Mn-based materials for the NOx abatement. Curr. Opin. Chem. Eng. 2016, 13, 133–141. [Google Scholar] [CrossRef]
- Xu, Y.; Zhang, Y.; Wang, J.; Yuan, J. Application of CFD in the optimal design of a SCR–DeNOx system for a 300 MW coal-fired power plant. Comput. Chem. Eng. 2013, 49, 50–60. [Google Scholar] [CrossRef]
- Lietti, L.; Nova, I.; Camurri, S.; Tronconi, E.; Forzatti, P. Dynamics of the SCR-DeNOx reaction by the transient-response method. AIChE J. 1997, 43, 2559–2570. [Google Scholar] [CrossRef]
- Sloss, L.L.; Hjalamasson, A.K.; Soud, H.N.; Campbell, L.M.; Stome, D.K.; Shareef, G.S.; Emnel, T.; Maibodi, M.; Livengood, C.D.; Markussen, J. Nitrogen Oxides Technology Fact Book; Noyes Data Corporation: Park Ridge, NJ, USA, 1992. [Google Scholar]
- Niu, Y.; Zhang, X.; Zhang, H.; Liang, Y.; Li, S.; Yao, Q.; Wang, D. Performance of Low-temperature SCR of NO with NH3 over MnOx/Ti-based catalysts. Can. J. Chem. Eng. 2019, 97, 1407–1417. [Google Scholar] [CrossRef]
- Park, T.; Jeong, S.; Hong, S.; Hong, S. Selective catalytic reduction of nitrogen oxides with NH3 over natural manganese ore at low temperature. Ind. Eng. Chem. Res. 2001, 40, 4491–4495. [Google Scholar] [CrossRef]
- IEA Clean Coal Centre. Emission Standards. Available online: https://www.iea-coal.org/library/emission-standards/ (accessed on 22 October 2020).
- Improving Emission Regulation for Coal-fired Power Plants in ASEAN, ERIA Research Project Report. Available online: https://www.eria.org/RPR_FY2016_02.pdf (accessed on 22 October 2020).
- Gao, F.; Tang, X.; Yi, H.; Zhao, S.; Li, C.; Li, J.; Shi, Y.; Meng, X. A review on selective catalytic reduction of NOx by NH3 over Mn–based catalysts at low temperatures: Catalysts, mechanisms, kinetics and DFT calculations. Catalysts 2017, 7, 199. [Google Scholar] [CrossRef]
- Wang, W.; Du, X.; Liu, S.; Yang, G.; Chen, Y.; Zhang, L.; Tu, X. Understanding the deposition and reaction mechanism of ammonium bisulfate on a vanadia SCR catalyst: A combined DFT and experimental study. Appl. Catal. B Environ. 2020, 260, 118168. [Google Scholar] [CrossRef]
- Thirupath, B.; Smirniotis, P.G. Nickel-doped Mn/TiO2 as an efficient catalyst for the low-temperature SCR of NO with NH3: Catalytic evaluation and characterizations. J. Catal. 2012, 288, 74–83. [Google Scholar] [CrossRef]
- Deng, S.; Meng, T.; Xu, B.; Gao, F.; Ding, Y.; Yu, L.; Fan, Y. Advanced MnOx/TiO2 catalyst with preferentially exposed anatase {001} facet for low-temperature SCR of NO. ACS Catal. 2016, 6, 5807–5815. [Google Scholar] [CrossRef]
- Kim, Y.J.; Kwon, H.J.; Nam I-SChoung, J.W.; Kil, J.K.; Kim, H.-J.; Cha, M.-S.; Yeo, G.K. High deNOx performance of Mn/TiO2 catalyst by NH3. Catal. Today 2010, 151, 244–250. [Google Scholar] [CrossRef]
- Gao, X.; Du, X.; Cui, L.; Fu, Y.; Luo, Z.; Cen, K. A Ce–Cu–Ti oxide catalyst for the selective catalytic reduction of NO with NH3. Catal. Commun. 2010, 12, 255–258. [Google Scholar] [CrossRef]
- Ali, S.; Chen, L.; Li, Z.; Zhang, T.; Li, R.; Bakhtiar, S.u.H.; Leng, X.; Yuan, F.; Niu, X.; Zhu, Y. Cux-Nb1.1-x (x = 0.45, 0.35, 0.25, 0.15) bimetal oxides catalysts for the low temperature selective catalytic reduction of NO with NH3. Appl. Catal. B Environ. 2018, 236, 25–35. [Google Scholar] [CrossRef]
- Yang, G.; Ran, J.; Du, X.; Wang, X.; Chen, Y.; Zhang, L. Different copper species as active sites for NH3-SCR reaction over Cu-SAPO-34 catalyst and reaction pathways: A periodic DFT study. Microporous Mesoporous Mater. 2018, 266, 223–231. [Google Scholar] [CrossRef]
- Jangjou, Y.; Wang, D.; Kumar, A.; Li, J.; Epling, W.S. SO2 poisoning of the NH3-SCR reaction over Cu-SAPO-34: Effect of ammonium sulfate versus other S-containing species. ACS Catal. 2016, 6, 6612–6622. [Google Scholar] [CrossRef]
- Wang, A.; Arora, P.; Bernin, D.; Kumar, A.; Kamasamudram, K.; Olsson, L. Investigation of the robust hydrothermal stability of Cu/LTA for NH3-SCR reaction. Appl. Catal. B Environ. 2019, 246, 242–253. [Google Scholar] [CrossRef]
- Kim, Y.; Lee, J.; Min, K.; Hong, S.; Nam, I.; Cho, B. Hydrothermal stability of Cu-SSZ13 for reducing NOx by NH3. J. Catal. 2014, 311, 447–457. [Google Scholar] [CrossRef]
- Ryu, T.; Kim, H.; Hong, S. Nature of active sites in Cu-LTA NH3-SCR catalysts: A comparative study with Cu-SSZ-13. Appl. Catal. B Environ. 2019, 245, 513–521. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, B.; Liu, B.; Sun, S. A review of Mn-containing oxide catalysts for low temperature selective catalytic reduction of NOx with NH3: Reaction mechanism and catalyst deactivation. RSC Adv. 2017, 7, 26226–26242. [Google Scholar] [CrossRef]
- Krishnan, A.; Boehman, A. Selective catalytic reduction of nitric oxide with ammonia at low temperatures. Appl. Catal. B 2017, 18, 189–198. [Google Scholar] [CrossRef]
- Damma, D.; Ettireddy, P.R.; Reddy, B.M.; Smirniotis, P.G. A review of low temperature NH3-SCR for Removal of NOx. Catalysts 2019, 9, 349. [Google Scholar] [CrossRef]
- Kim, T.; Choi, S.; Kim, J. Performance prediction of a large-scale circulating fluidized bed boiler by heat exchangers block simulation. Proc. Inst. Mech. Eng. Part A J. Power Energy 2015, 229, 298–308. [Google Scholar] [CrossRef]
- Pioro, I.L.; Duffey, R.B.; Dumouchel, T.J. Hydraulic resistance of fluids flowing in channels at supercritical pressures (survey). Nucl. Eng. Des. 2004, 231, 187–197. [Google Scholar] [CrossRef]
- Hottel, H.C. Radiation from Carbon Dioxide, Water Vapor and Soot; American Flame Committee: California, USA, 1985. [Google Scholar]
- Kakac, S. Boilers, Evaporators, and Condensers; Chap. 6; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 1991. [Google Scholar]
- Incropera, F.P.; Dewitt, D.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer; Wiley: New York, NY, USA, 2008. [Google Scholar]
- Sallevelt, J.L.H.P.; Withag, J.A.M.; Bramer, E.A.; Brilman, D.W.F.; Brem, G. One-dimensional model for heat transfer to a supercritical water flow in tube. J. Supercrit. Fluids 2012, 68, 1–12. [Google Scholar] [CrossRef]
- NIST. Reference Fluid Thermodynamic and Transport Properties Database (REFPROP): Version 10. Available online: https://www.nist.gov/srd/refprop (accessed on 22 October 2020).
- Kim, S.; Choi, S. Practical suggestion for calculating supercritical water-steam properties. Trans. Korean Soc. Mech. Eng. B 2016, 40, 809–814. (In Korean) [Google Scholar] [CrossRef]
- Wagner, W.; Kretzschmar, H.J. Release on the IAPWS (The International Association for the Properties of Water-Steam Properties). In Industrial Formulation 1997 for the Thermodynamic Properties of Water and Steam; IAPWS Meeting: Erlangen, Germany, 1997. [Google Scholar]






| Countries | Previous Standard | Reinforced Standard | |
|---|---|---|---|
| Limit Value | Remark | ||
| Korea [9] | 50 ppm (102.5 mg/m3) | 15 ppm (30.7 mg/m3) | After 2020 100 MWe |
| China [10] | 200 ppm (410 mg/m3) | 97.5 ppm (200 mg/m3) | After January 2012 |
| Japan [9] | - | 200 ppm (410 mg/m3) | 700,000 m3/h |
| India [9] | 146.3 ppm (300 mg/m3) | 48.7 ppm (100 mg/m3) | New plants installed from 1 January 2017 |
| EU [9] | 97.5 ppm (200 mg/m3) | 73.2 ppm (150 mg/m3) | >300 MWth After 1 November 2010 |
| U.S.A. [9] | 180 ng/J * (*gross output) | 88 ng/J * (*gross output) | After May 2011 |
| Main Operating Conditions (at BMCR load) | |
|---|---|
| Coal flow rate | 97.9 kg/s |
| Excess air ratio | 1.15 |
| Main steam conditions | 2664 t/h, 25.4 MPa, 569 °C |
| Reheat steam conditions | 2174 t/h, 4.5 MPa, 596 °C |
| Total boiler heat duty | 1944 MW |
| Boiler exit temperature (SCR inlet temperature) | 360 °C |
| FGD Inlet tem temperature/Outlet temperature | 90 °C/50 °C |
| Stack exit temperature | 102 °C |
| Boiler thermal efficiency | 89.3% |
| Parameters | BMCR | TMCR (Turbine Maximum Continuous Rating) | NR (Normal Rating) | 75% TMCR | 50% TMCR | 30% TMCR |
|---|---|---|---|---|---|---|
| Flue gas flow rate [kg/s] | 916 | 878 | 872 | 669 | 466 | 364 |
| HTC (W/m2/°C) | 13.6 | 13.1 | 13.2 | 10.4 | 6.8 | 5.9 |
| HTC and Properties | Mechanism | Reference | |
|---|---|---|---|
| ho (outer HTC) | Furnace | Radiation | [28] |
| Convective heat exchangers and GGH | Radiation | [29] | |
| Convection | [30] | ||
| Air preheater | Fitting equation based on the flue gas mass flow rate | - | |
| hi (inner HTC) | Subcritical | Dittus–Bolter equation | [30] |
| Supercritical | Sallevelt equation | [31] | |
| Properties | Air-flue gas side | Refprop code | [32] |
| Water-steam side | In-house code based on IAPWS-IF 97 | [33,34] | |
| GGH Cooler | BMCR | TMCR | 75% TMCR | 50% TMCR | 30% TMCR | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| Gas | Water | Gas | Water | Gas | Water | Gas | Water | Gas | Water | |
| Ti (°C) | 207.3 | 77.3 | 203.3 | 81 | 188 | 87 | 194.5 | 85 | 183.9 | 88 |
| To (°C) | 89.8 | 181.2 | 90.3 | 173.7 | 91.2 | 156.3 | 90.8 | 172.1 | 90.7 | 158.7 |
| (kg/s) | 950.8 | 272.1 | 913.5 | 282 | 705.8 | 250 | 497.7 | 150 | 392.1 | 130 |
| TLMTD (°C) | 18.47 | 17.53 | 13.61 | 12.29 | 10.07 | |||||
| U (W/m2/°C) | 35.25 | 34.25 | 29.12 | 24.472 | 20.87 | |||||
| (MW) | 120.7 | 111.46 | 73.59 | 55.65 | 39.1 | |||||
| GGH Reheater | BMCR | TMCR | 75% TMCR | 50% TMCR | 30% TMCR | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| Gas | Water | Gas | Water | Gas | Water | Gas | Water | Gas | Water | |
| Ti (°C) | 50 | 182.1 | 50 | 175.1 | 50 | 155.8 | 50 | 169 | 50 | 157.5 |
| To (°C) | 168 | 77.8 | 163.5 | 79.4 | 151.3 | 87.6 | 163.4 | 81.6 | 155.1 | 87.7 |
| (kg/s) | 967 | 280 | 928.5 | 282 | 715.4 | 275 | 504.3 | 170 | 397.1 | 155 |
| TLMTD (°C) | 21 | 20 | 16 | 16 | 14 | |||||
| U (W/m2/°C) | 50.42 | 49 | 41.09 | 32.91 | 28.1 | |||||
| (MW) | 124.79 | 115.12 | 79.69 | 63.29 | 46.03 | |||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, S.; Chae, T.; Lee, Y.; Yang, W.; Hong, S. Performance Evaluation of a Novel Thermal Power Plant Process with Low-Temperature Selective Catalytic Reduction. Energies 2020, 13, 5558. https://doi.org/10.3390/en13215558
Kim S, Chae T, Lee Y, Yang W, Hong S. Performance Evaluation of a Novel Thermal Power Plant Process with Low-Temperature Selective Catalytic Reduction. Energies. 2020; 13(21):5558. https://doi.org/10.3390/en13215558
Chicago/Turabian StyleKim, Seongil, Taeyoung Chae, Yongwoon Lee, Won Yang, and Sungho Hong. 2020. "Performance Evaluation of a Novel Thermal Power Plant Process with Low-Temperature Selective Catalytic Reduction" Energies 13, no. 21: 5558. https://doi.org/10.3390/en13215558
APA StyleKim, S., Chae, T., Lee, Y., Yang, W., & Hong, S. (2020). Performance Evaluation of a Novel Thermal Power Plant Process with Low-Temperature Selective Catalytic Reduction. Energies, 13(21), 5558. https://doi.org/10.3390/en13215558




