The Influence of the Shape of Granite on the Heat Storage Process in a Rock Bed
Abstract
:1. Introduction
2. Materials and Methods
2.1. Filling of the Storage Bed
2.2. Test Stand and Experiment Plan
2.3. Thermal Balance of the Storage Bed
- C = 0.5, k = 0 if < 10−3.
- C = 1.18, k = 1/8 if 10−3 < .
- C = 0.54, .
- C = 0.135, k = 1/3 if .
2.4. Calculation of the First-Law and Second-Law Efficiencies
3. Results
3.1. Experimental Research Results
3.2. Thermal Efficiency of the Charging Process
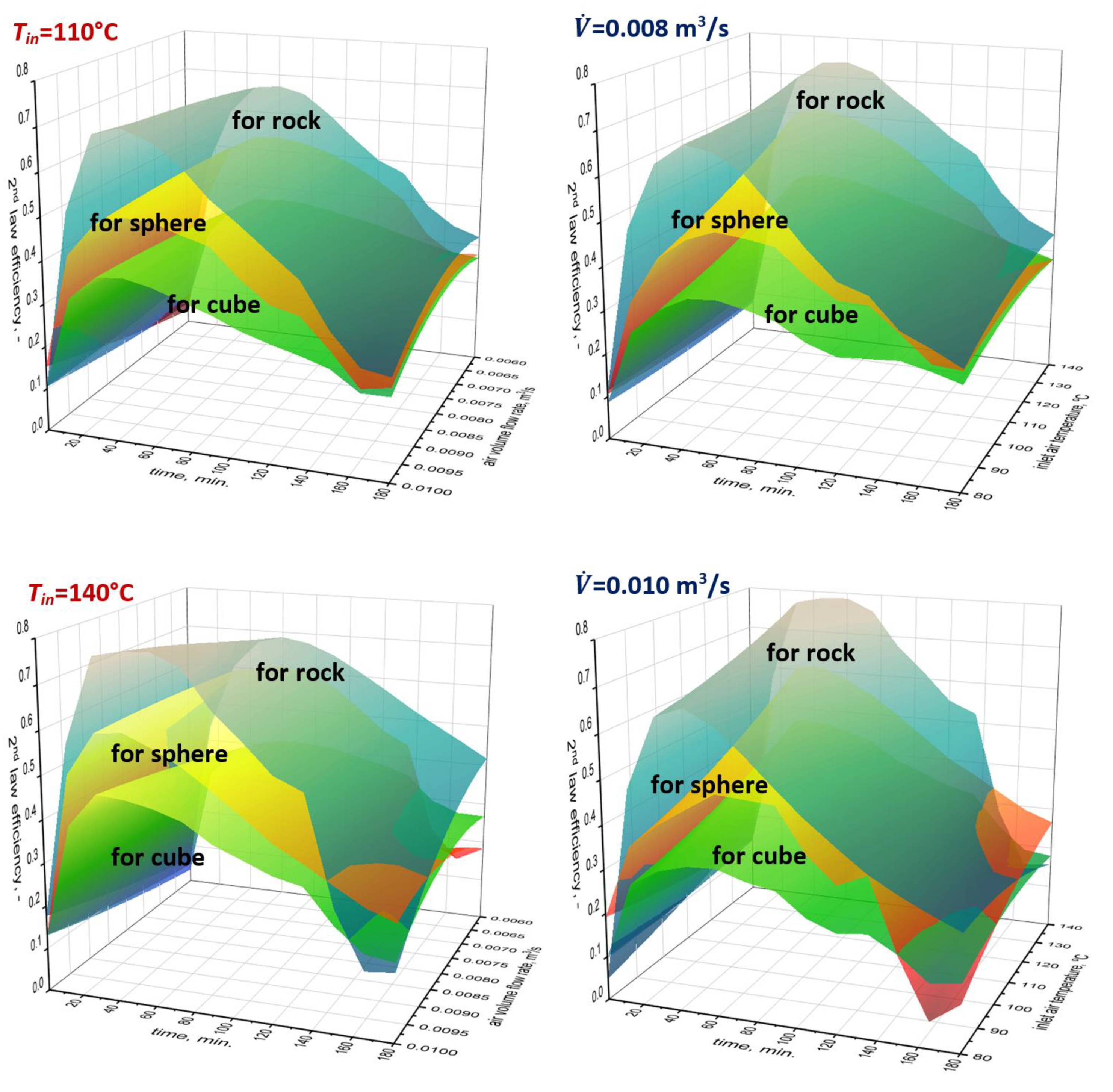
3.3. The Generation of Entropy—The Second-Law Efficiency
4. Discussion and Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| a | internal width and length of the bed, m |
| the area of the inner wall of the bed, m2 | |
| area of single element of granite in storage bed, m2 | |
| the area of the outer wall of the bed, m2 | |
| the average value of the partition’s surface area, m2 | |
| b | internal height of the bed, m |
| b0, b1, …, b10 | regression coefficients from Hartley plan of experiment |
| C | constant |
| characteristic dimension of the upper and lower inner surface of the bed, m | |
| specific heat of air at constant pressure, | |
| specific heat of the granite storage material, | |
| characteristic dimension of the upper and lower outer surface of the bed, m | |
| flow exergy, J | |
| Grashof number | |
| convection heat transfer coefficient, W/(m2·K) | |
| convective heat transfer coefficient on the inside of the bed, W/(m2·K) | |
| radiative heat transfer coefficient on the outer side of the bed, W/(m2·K) | |
| k | constant |
| mass air flow rate, | |
| mass of single element of granite in storage bed, | |
| n | amount of granite elements in storage bed |
| number of heat transfer units | |
| Nusselt number | |
| Prandtl number calculated for the average temperature of the working medium | |
| Prandtl number calculated for the mean wall temperature | |
| stream of accumulated heat, W | |
| heat flux supplied with hot air, W | |
| heat loss flux from the surface of the bed, W | |
| total heat loss flux from the bed surfaces, W | |
| heat transfer to the ambient, | |
| entropy generation rate, | |
| t | time, s |
| ambient temperature, | |
| average air temperature inside the bed, | |
| inlet air temperature, | |
| outlet air temperature, | |
| Tin | temperature of the inner wall surface of the bed, |
| granite temperature, | |
| temperature of the outer wall of the bed, | |
| volumetric air flow rate, | |
| x | characteristic dimension, m |
| heat exchanger parameter | |
| unknown parameter from Hartley plan of experiment, (variable) | |
| Greek symbols | |
| kinematic viscosity, m2/s | |
| β | thermal expansion coefficient, 1/K |
| first-law efficiency | |
| second-law efficiency | |
| dimensionless time | |
| density of the air, | |
| dimensionless temperature | |
| insulation thickness, m | |
| thermal conductivity of air, W/(m·K) | |
| thermal conductivity of the insulation, W/(m·K) | |
| the emissivity coefficient of the outer wall of the storage bed | |
| Ψ | the sphericity |
| time step, s | |
| Index | |
| bot | bottom surface of the bed |
| in | inside the bed |
| out | outside the bed |
| side | side surface of the bed |
| top | top surface of the bed |
References
- Gautam, A.; Saini, R.P. A review on technical, applications and economic aspect of packed bed solar thermal energy storage system. J. Energy Storage 2020, 27, 101046. [Google Scholar] [CrossRef]
- Singh, S. Thermohydraulic performance of double pass solar thermal collector with inline, staggered and hybrid fin configurations. J. Energy Storage 2020, 27, 101080. [Google Scholar] [CrossRef]
- Bellos, E.; Tzivanidis, C.; Daniil, I.; Antonopoulos, K.A. The impact of internal longitudinal fins in parabolic trough collectors operating with gases. Energy Convers. Manag. 2017, 135, 35–54. [Google Scholar] [CrossRef]
- Singh, H.; Saini, R.P.; Saini, J.S. A review on packed bed solar energy storage systems. Renew. Sustain. Energy Rev. 2010, 14, 1059–1069. [Google Scholar] [CrossRef]
- Rosen, M.A. The exergy of stratified thermal energy storages. Sol. Energy 2001, 71, 173–185. [Google Scholar] [CrossRef]
- Zanganeh, G.; Pedretti, A.; Haselbacher, A.; Steinfeld, A. Design of packed bed thermal energy storage systems for high-temperature industrial process heat. Appl. Energy 2015, 137, 812–822. [Google Scholar] [CrossRef]
- Allen, K.G.; von Backström, T.W.; Kröger, D.G. Packed bed pressure drop dependence on particle shape, size distribution, packing arrangement and roughness. Powder Technol. 2013, 246, 590–600. [Google Scholar] [CrossRef]
- Sarbu, I.; Sebarchievici, C. A comprehensive review of thermal energy storage. Sustainability 2018, 10, 191. [Google Scholar] [CrossRef] [Green Version]
- Yang, B.; Wang, Y.; Bai, F.; Wang, Z. Experimental and numerical investigation of a packed-bed thermal energy storage device. AIP Conf. Proc. 2017, 1850. [Google Scholar] [CrossRef] [Green Version]
- Yang, J.; Hu, Y.; Qian, P.; Guo, Z.; Wang, Q. Experimental Study of Forced Convective Heat Transfer in Packed Beds With Uniform and Non-Uniform Spheres. Heat Transf. Eng. 2020, 41, 351–360. [Google Scholar] [CrossRef]
- Yang, J.; Wang, Q.; Zeng, M.; Nakayama, A. Computational study of forced convective heat transfer in structured packed beds with spherical or ellipsoidal particles. Chem. Eng. Sci. 2010, 65, 726–738. [Google Scholar] [CrossRef]
- Agrawal, P.; Gautam, A.; Kunwar, A.; Kumar, M.; Chamoli, S. Performance assessment of heat transfer and friction characteristics of a packed bed heat storage system embedded with internal grooved cylinders. Sol. Energy 2018, 161, 148–158. [Google Scholar] [CrossRef]
- Singh, H.; Saini, R.P.; Saini, J.S. Performance of a packed bed solar energy storage system having large sized elements with low void fraction. Sol. Energy 2013, 87, 22–34. [Google Scholar] [CrossRef]
- Sagara, K.; Nakahara, N. Thermal performance and pressure drop of rock beds with large storage materials. Sol. Energy 1991, 47, 157–163. [Google Scholar] [CrossRef]
- Domanski, R.; Fellah, G. Exergy as a tool for designing and operating thermal storage units. J. Power Technol. 1995, 23–45. [Google Scholar]
- Bellecci, C.; Conti, M. Thermal energy storage in a porous medium: An entropy generation approach in a power production perspective. Nuovo Cim. C 1992, 15, 275–287. [Google Scholar] [CrossRef]
- Bejan, A. Entropy Generation Minimization: The Method of Thermodynamic Optimization of Finite-Size Systems and Finite-Time Processes (Mechanical and Aerospace Engineering Series); CRC Press: Boca Raton, FL, USA, 1996; ISBN 0-8493-9651-4. [Google Scholar]
- Osiecka, E. Materiały Budowlane. Kamień—Ceramika—Szkło; Oficyna Wydawnicza Politechniki Warszawskiej: Warsaw, Poland, 2010; ISBN 978-83-7207-865-0. (In Polish) [Google Scholar]
- Nemś, M.; Nemś, A.; Pacyga, P. A Granite Bed Storage for a Small Solar Dryer. Materials (Basel) 2018, 11, 1969. [Google Scholar] [CrossRef] [Green Version]
- Hartley, H.O. Smallest Composite Designs for Quadratic Response Surfaces. Biometrics 1959. [Google Scholar] [CrossRef]
- Singh, R.; Saini, R.P.; Saini, J.S. Nusselt number and friction factor correlations for packed bed solar energy storage system having large sized elements of different shapes. Sol. Energy 2006, 80, 760–771. [Google Scholar] [CrossRef]
- Kostowski, E. Zbiór Zadań z Przepływu Ciepła; Wydawnictwo Politechniki Śląskiej: Gliwice, Poland, 2006; ISBN 83-88000-18-7. (In Polish) [Google Scholar]
- Cengel, Y.A.; Boles, M.A. Thermodynamics: An Engineering Approach, 8th ed.; McGraw-Hill Education: New York, NY, USA, 2014; ISBN 9780073398174. [Google Scholar]
- Ranz, W.E.; Marshall, W.R. Evaporation from drops. Parts I & II. Chem. Eng. Progr. 1952. [Google Scholar] [CrossRef]
- Melissari, B.; Argyropoulos, S.A. Development of a heat transfer dimensionless correlation for spheres immersed in a wide range of Prandtl number fluids. Int. J. Heat Mass Transf. 2005, 48, 4333–4341. [Google Scholar] [CrossRef]
- Kunii, D.; Levenspiel, O. Fluidization Engineering, 2nd ed.; Elsevier: Cambridge, MA, USA, 1991; ISBN 0-409-90233-0. [Google Scholar]
- Wiśniewski, S.; Wiśniewski, T.S. Wymiana Ciepła; Wydawnictwo WNT: Warsaw, Poland, 2013; ISBN 9788379260478. (In Polish) [Google Scholar]
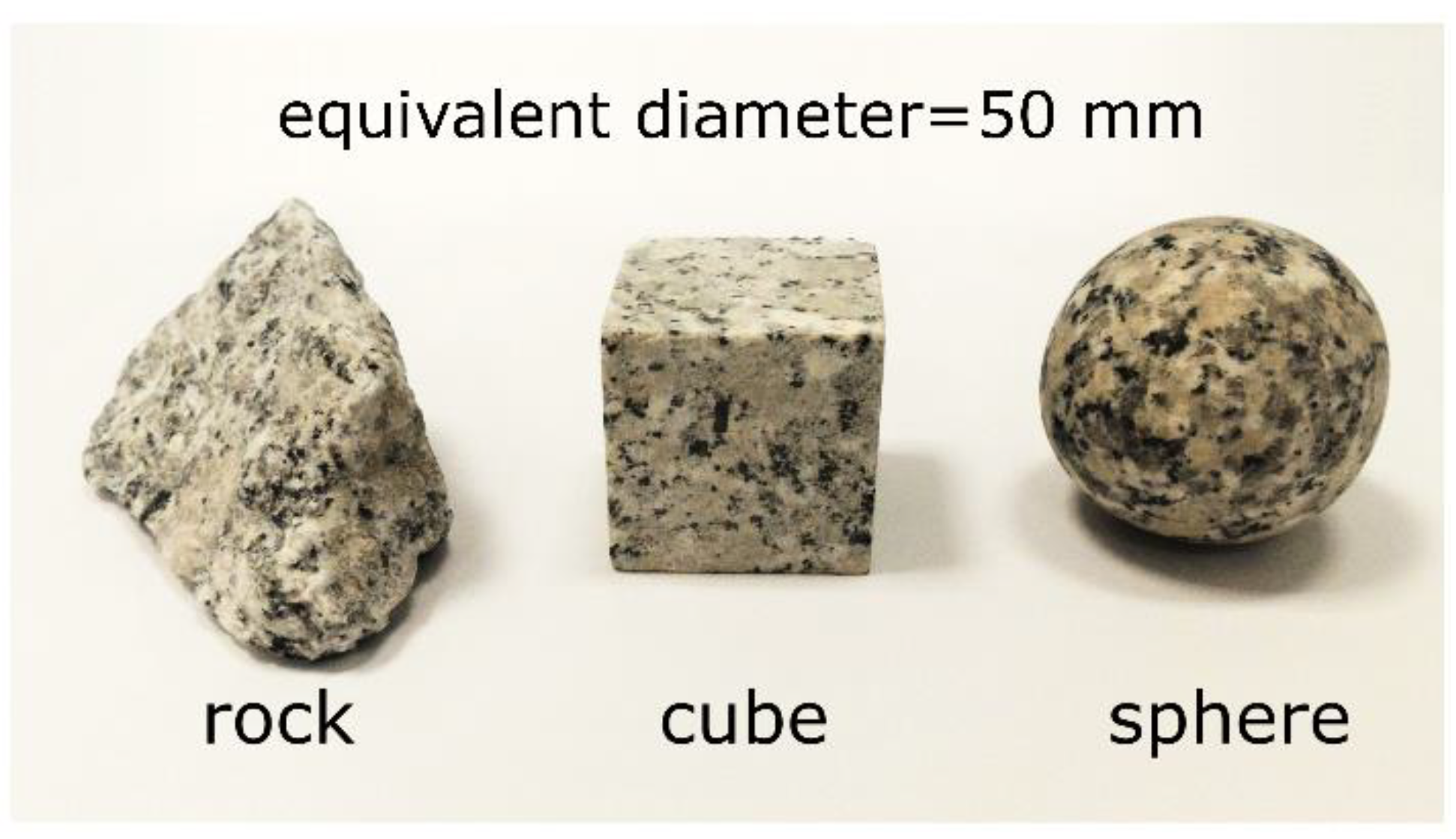
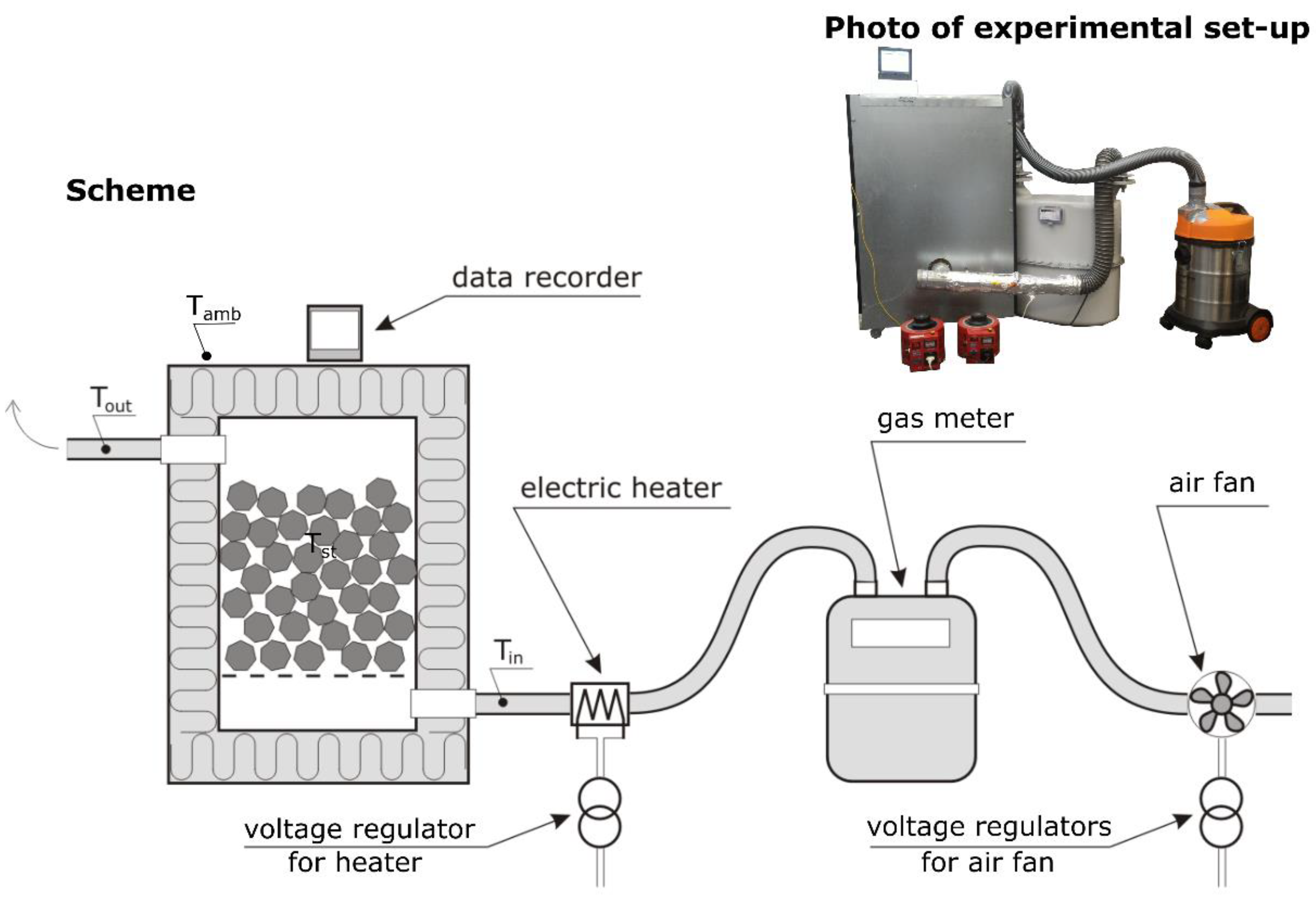

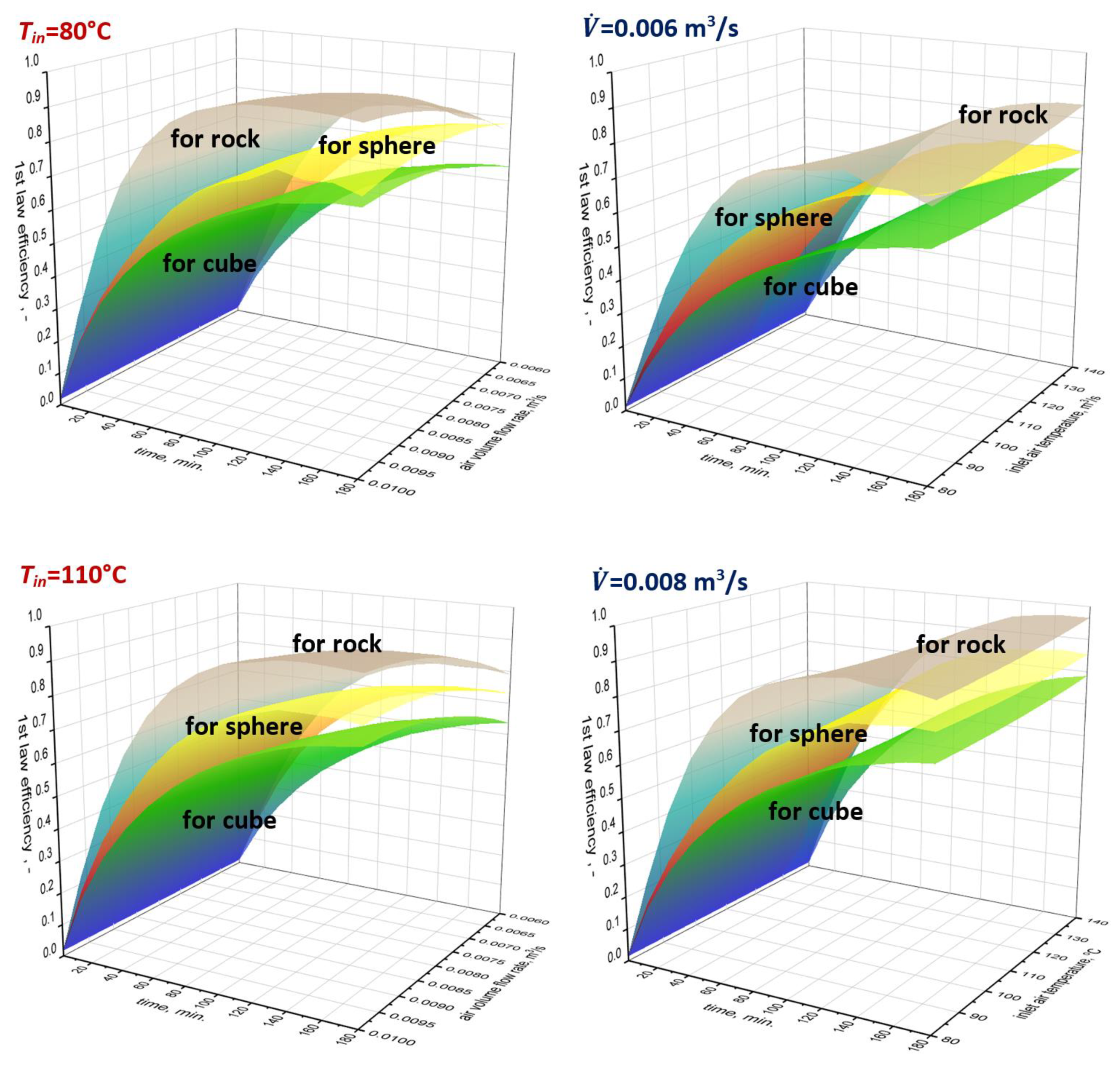
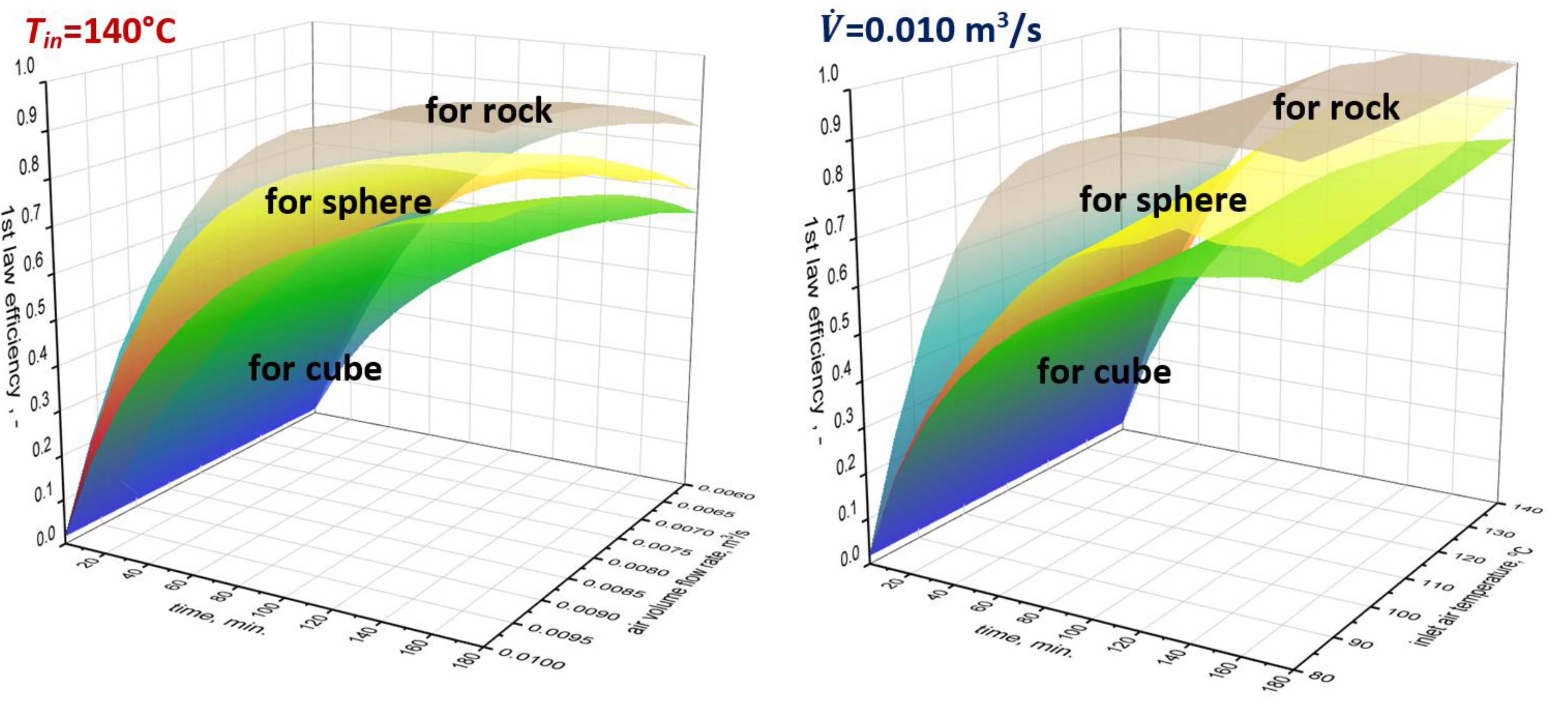

| Rock Bed | Internal Height | b | 0.5 m |
| Internal thickness | a | 0.3 m | |
| Internal length | a | 0.3 m | |
| Storage Material | Specific heat | 780 J/(kg·K) | |
| Mass | 0.174 kg | ||
| Number of elements | n | 230 | |
| Insulation | Top insulation thickness | 0.20 m | |
| Bottom insulation thickness | 0.15 m | ||
| Side insulation thickness | 0.15 m | ||
| Thermal conductivity | 0.039 W/(m·K) |
| Variable | −1 | 0 | +1 |
|---|---|---|---|
| Ψ, - | 0.6 | 0.8 | 1.0 |
| Tair_inlet, °C | 80 | 110 | 140 |
| , m3/s | 0.006 | 0.008 | 0.010 |
| No. | Ψ | Tair_in | |
|---|---|---|---|
| - | °C | m3/s | |
| 1 | 1.0 | 140 | 0.010 |
| 2 | 1.0 | 80 | 0.006 |
| 3 | 0.6 | 140 | 0.006 |
| 4 | 0.6 | 80 | 0.010 |
| 5 | 1.0 | 110 | 0.008 |
| 6 | 0.6 | 110 | 0.008 |
| 7 | 0.8 | 140 | 0.008 |
| 8 | 0.8 | 80 | 0.008 |
| 9 | 0.8 | 110 | 0.010 |
| 10 | 0.8 | 110 | 0.006 |
| 11 | 0.8 | 110 | 0.008 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nemś, M.; Nemś, A.; Gębarowska, K. The Influence of the Shape of Granite on the Heat Storage Process in a Rock Bed. Energies 2020, 13, 5662. https://doi.org/10.3390/en13215662
Nemś M, Nemś A, Gębarowska K. The Influence of the Shape of Granite on the Heat Storage Process in a Rock Bed. Energies. 2020; 13(21):5662. https://doi.org/10.3390/en13215662
Chicago/Turabian StyleNemś, Magdalena, Artur Nemś, and Kamila Gębarowska. 2020. "The Influence of the Shape of Granite on the Heat Storage Process in a Rock Bed" Energies 13, no. 21: 5662. https://doi.org/10.3390/en13215662
APA StyleNemś, M., Nemś, A., & Gębarowska, K. (2020). The Influence of the Shape of Granite on the Heat Storage Process in a Rock Bed. Energies, 13(21), 5662. https://doi.org/10.3390/en13215662







