Super-Twisting Algorithm Applied to Velocity Control of DC Motor without Mechanical Sensors Dependence
Abstract
:1. Introduction
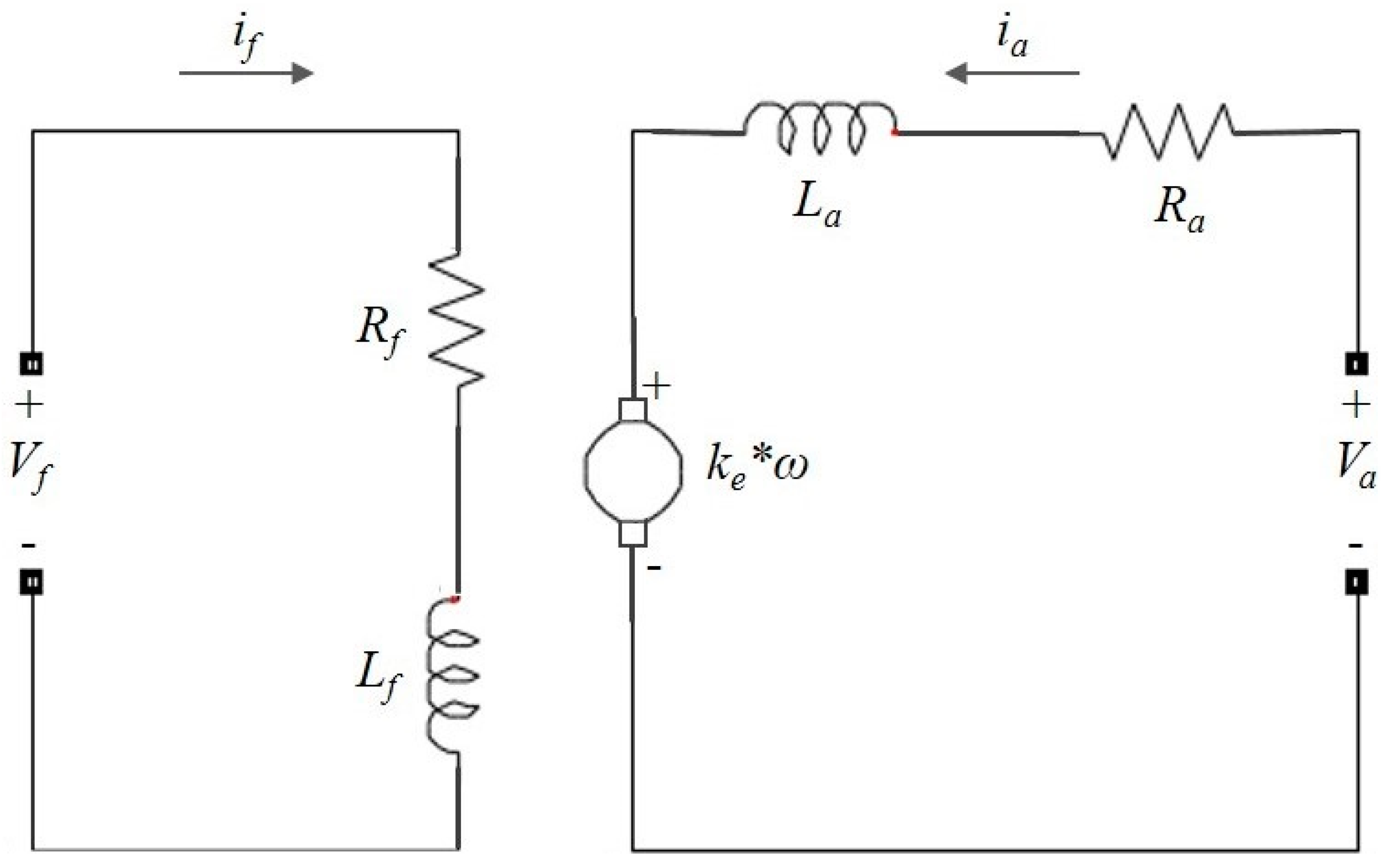
2. DC Motor Model
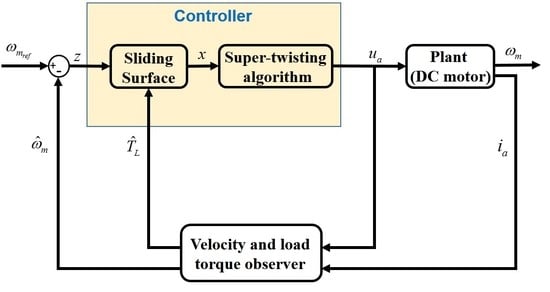
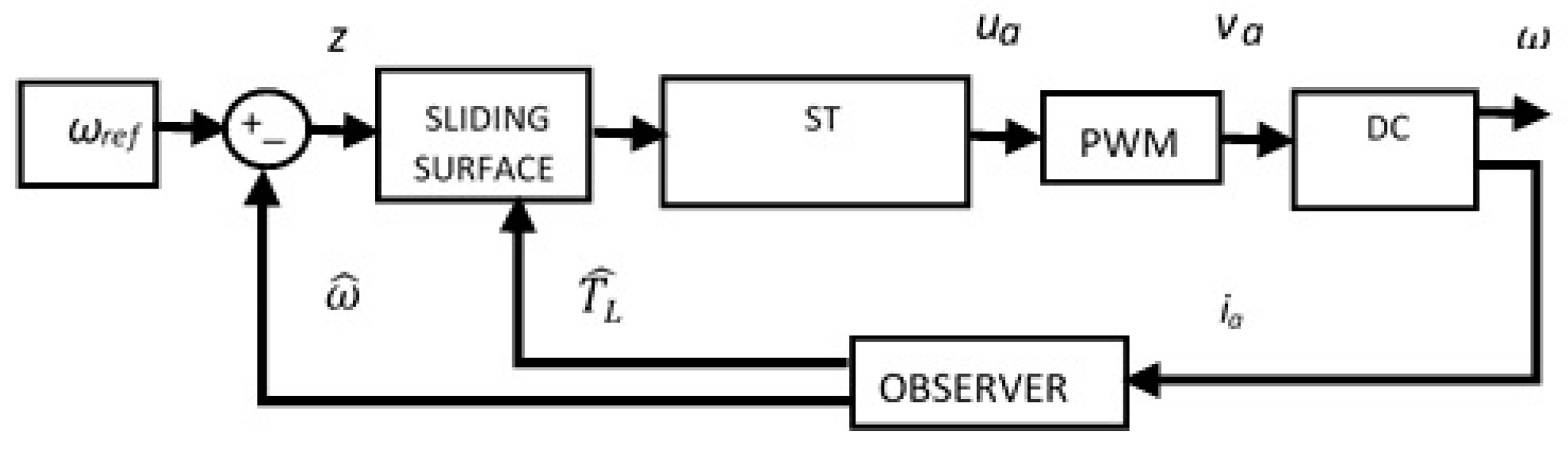
3. Controller Design
Super-Twisting Control Algorithm
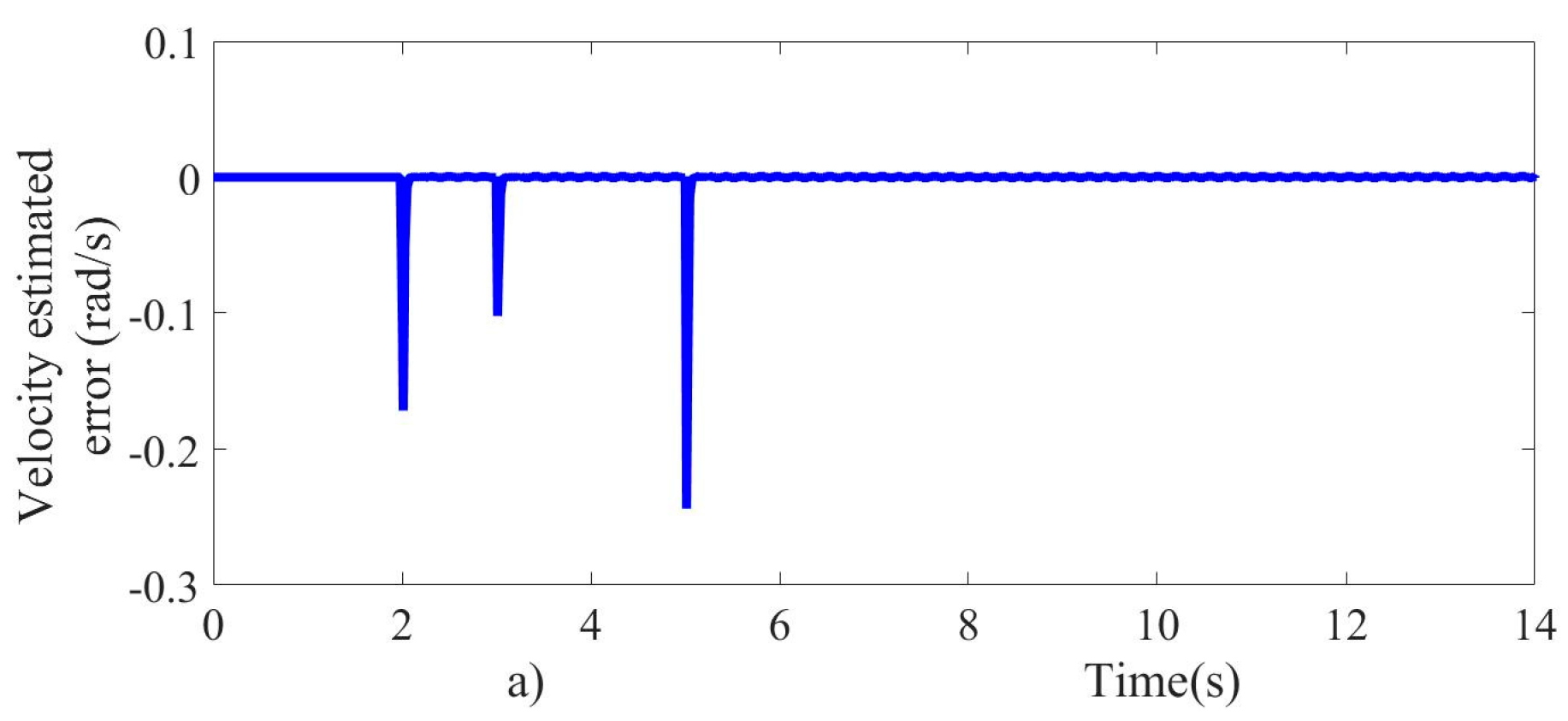
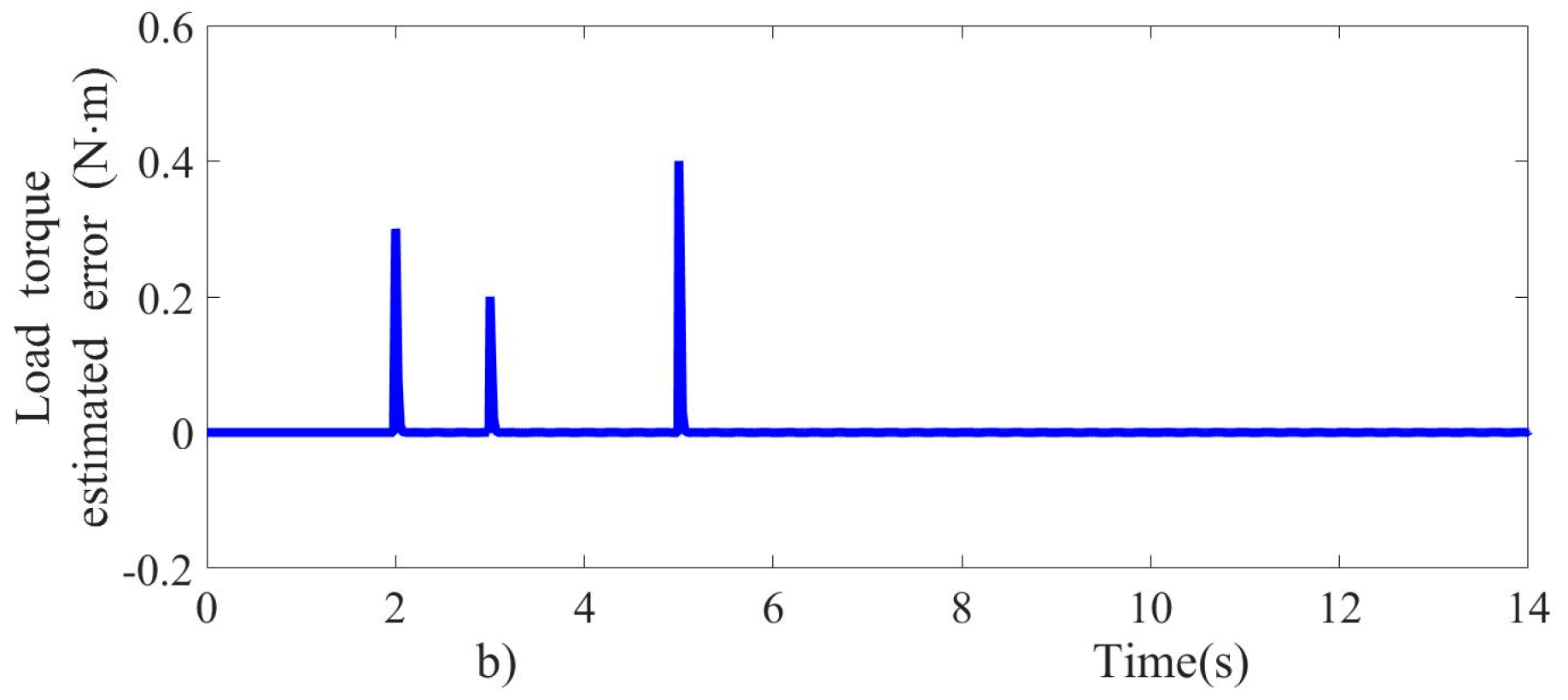
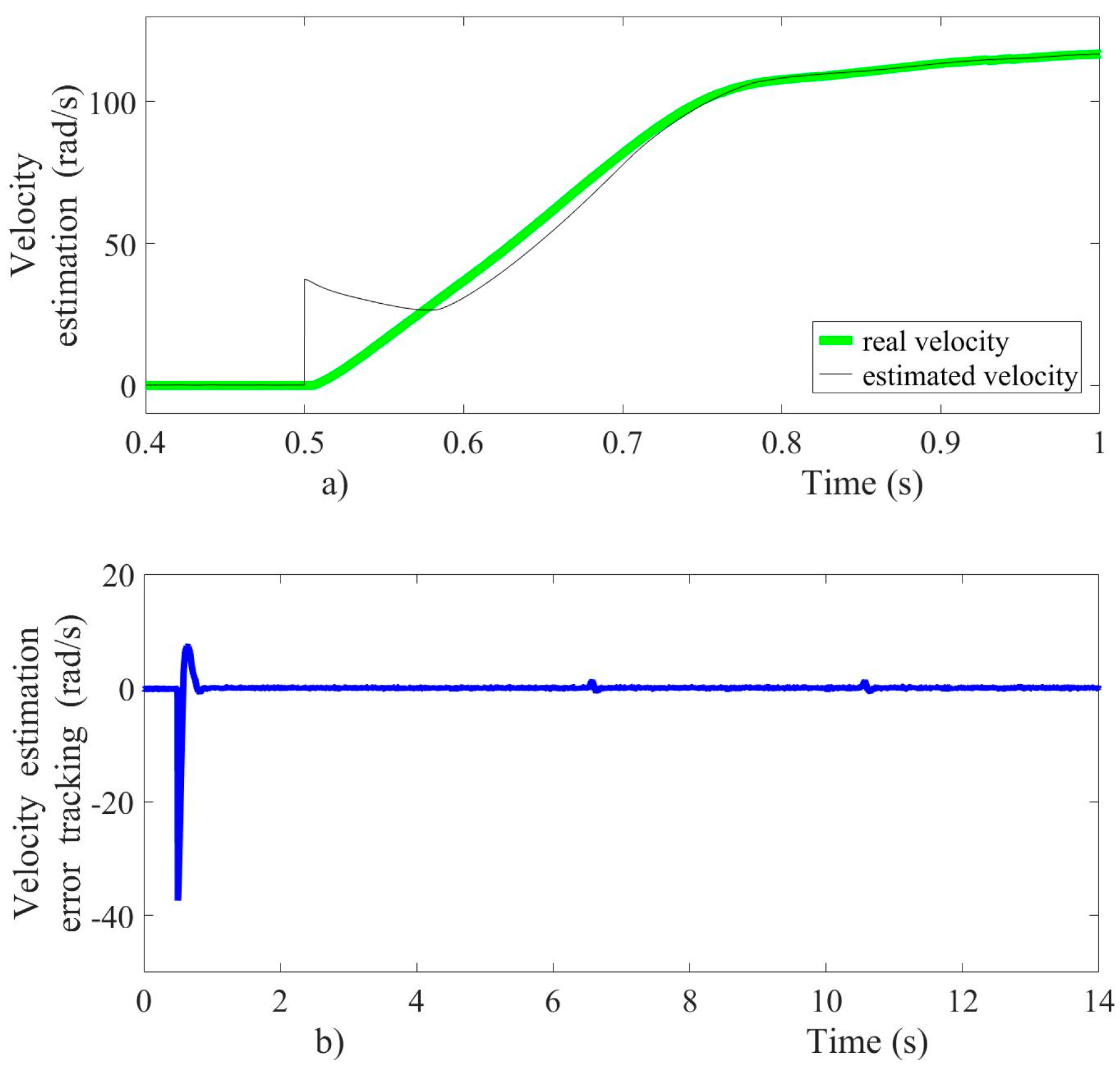
4. Velocity Observer
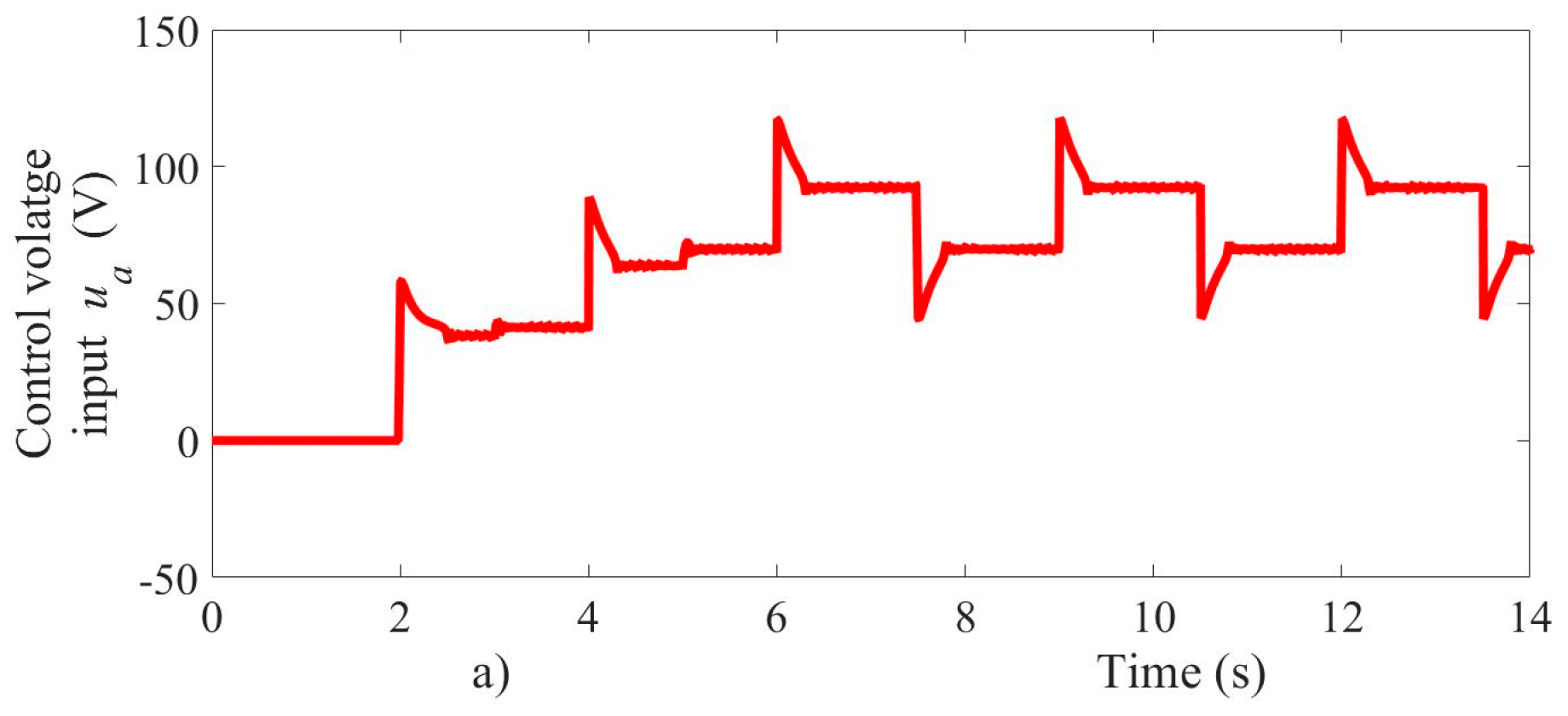
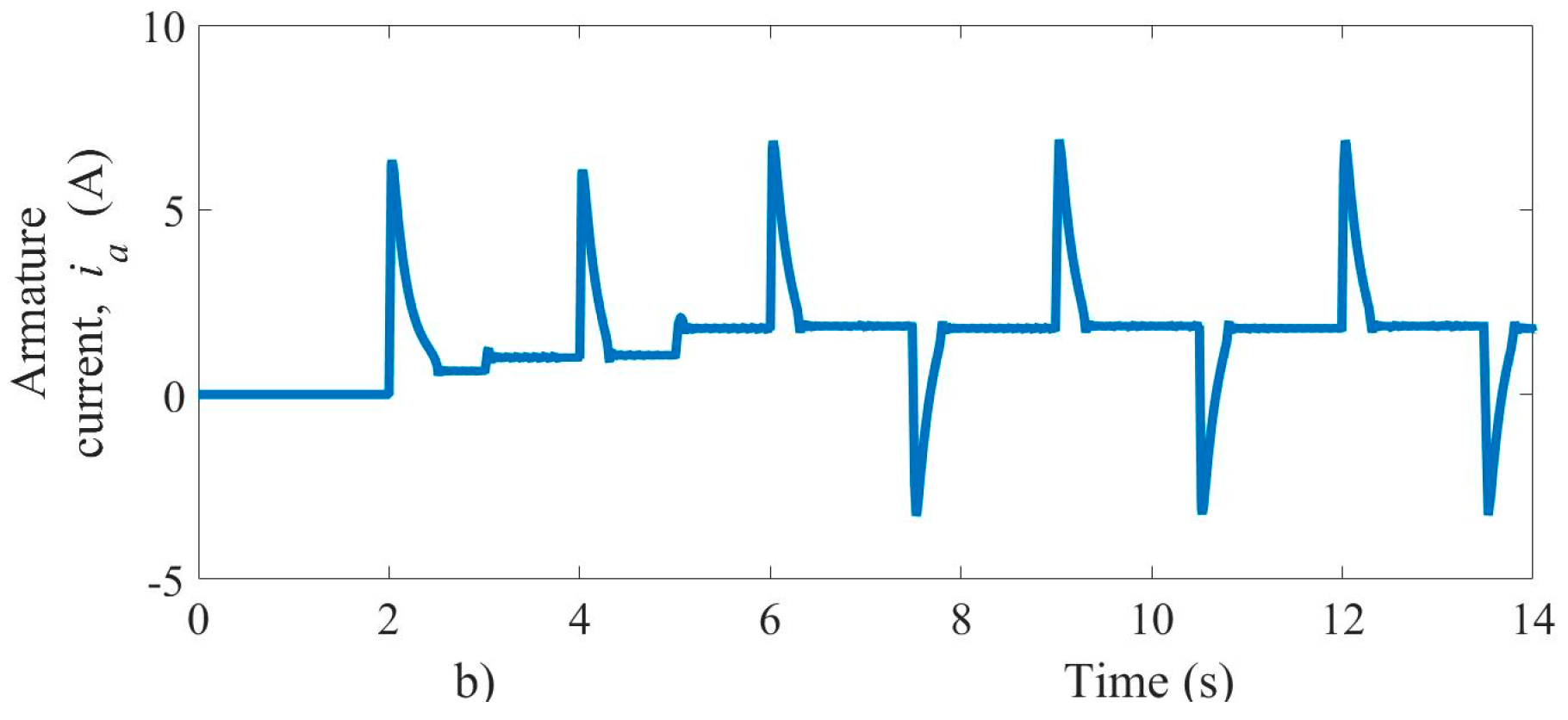
5. Control Scheme Validation
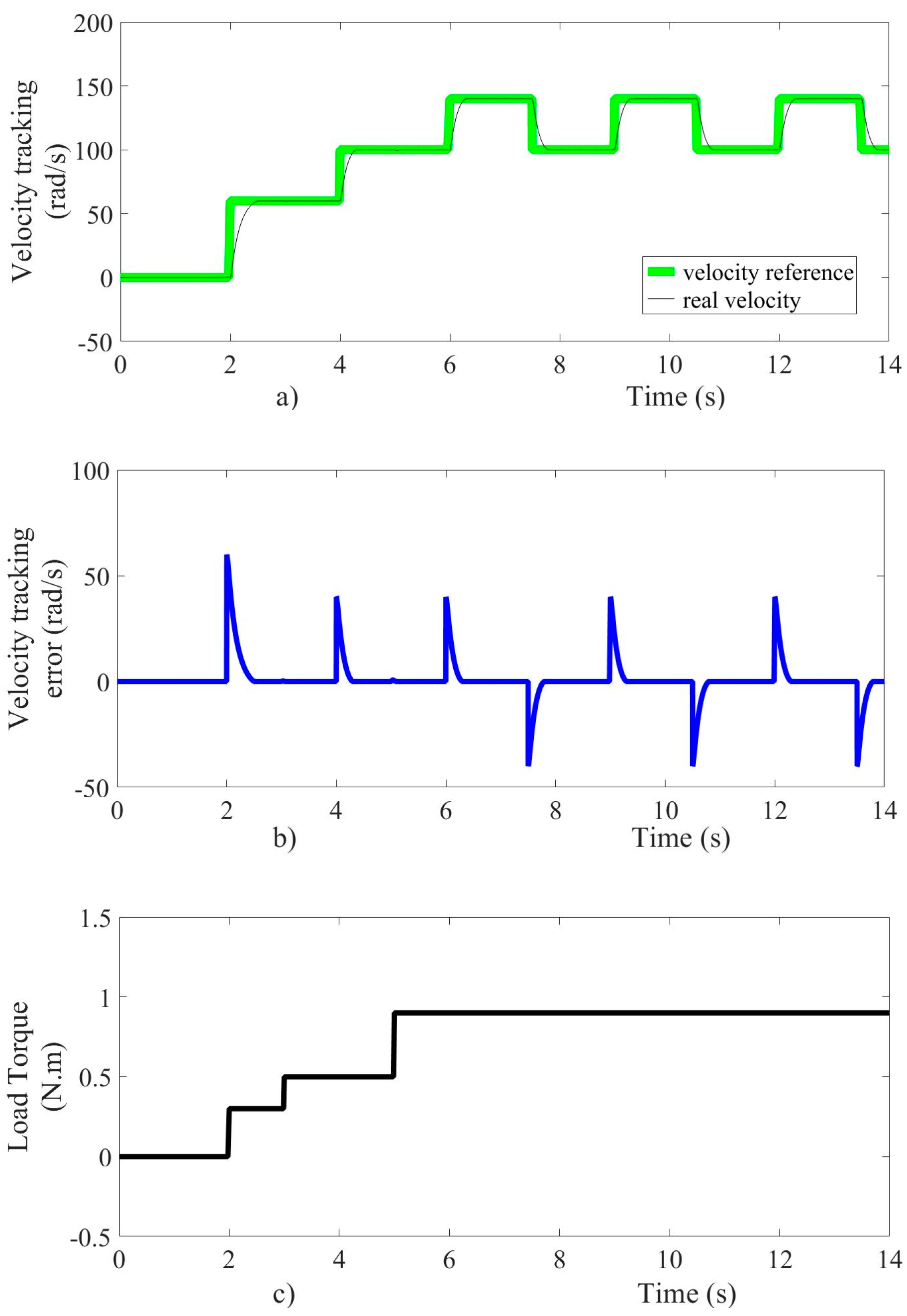
5.1. Simulation Results
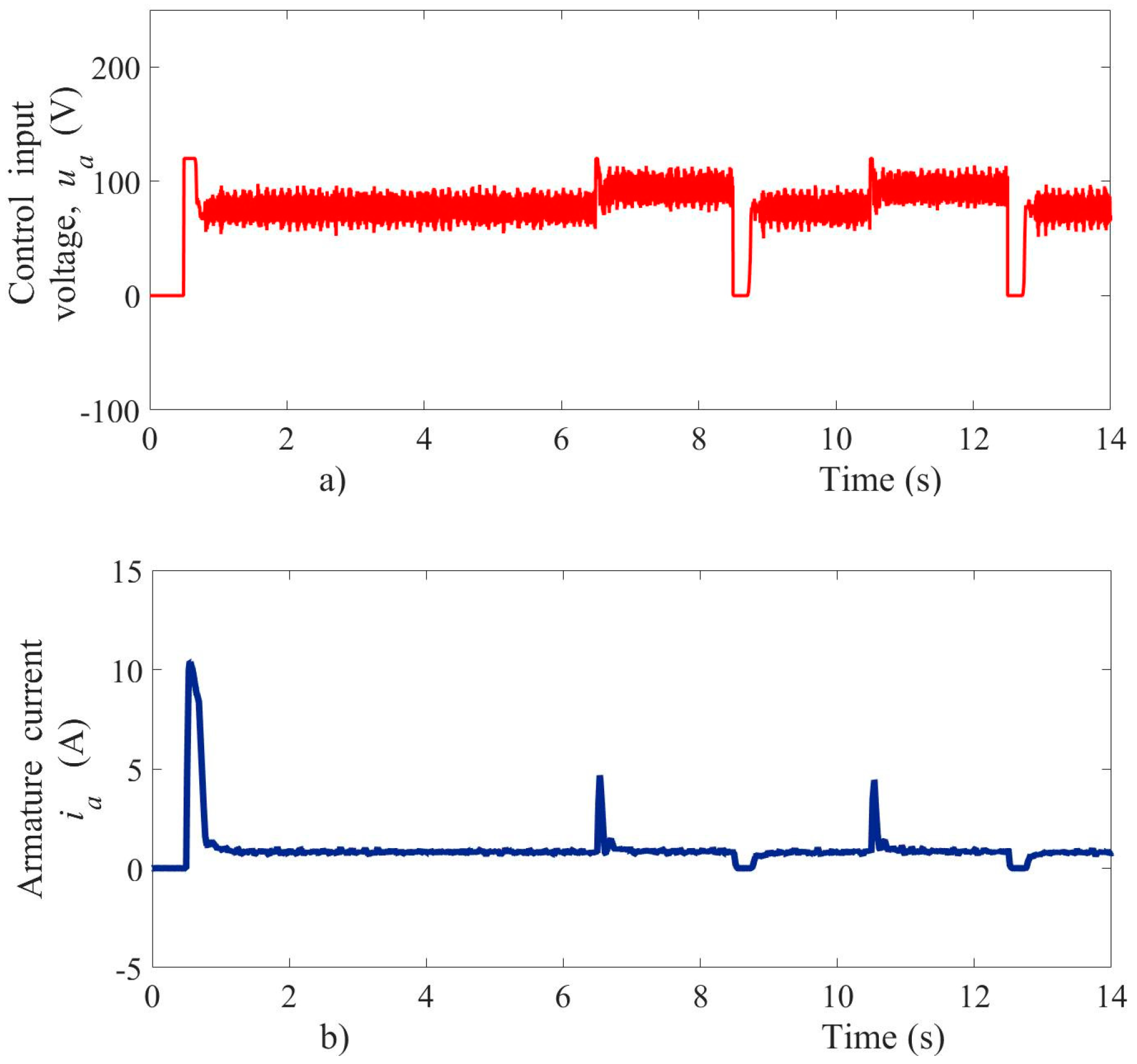
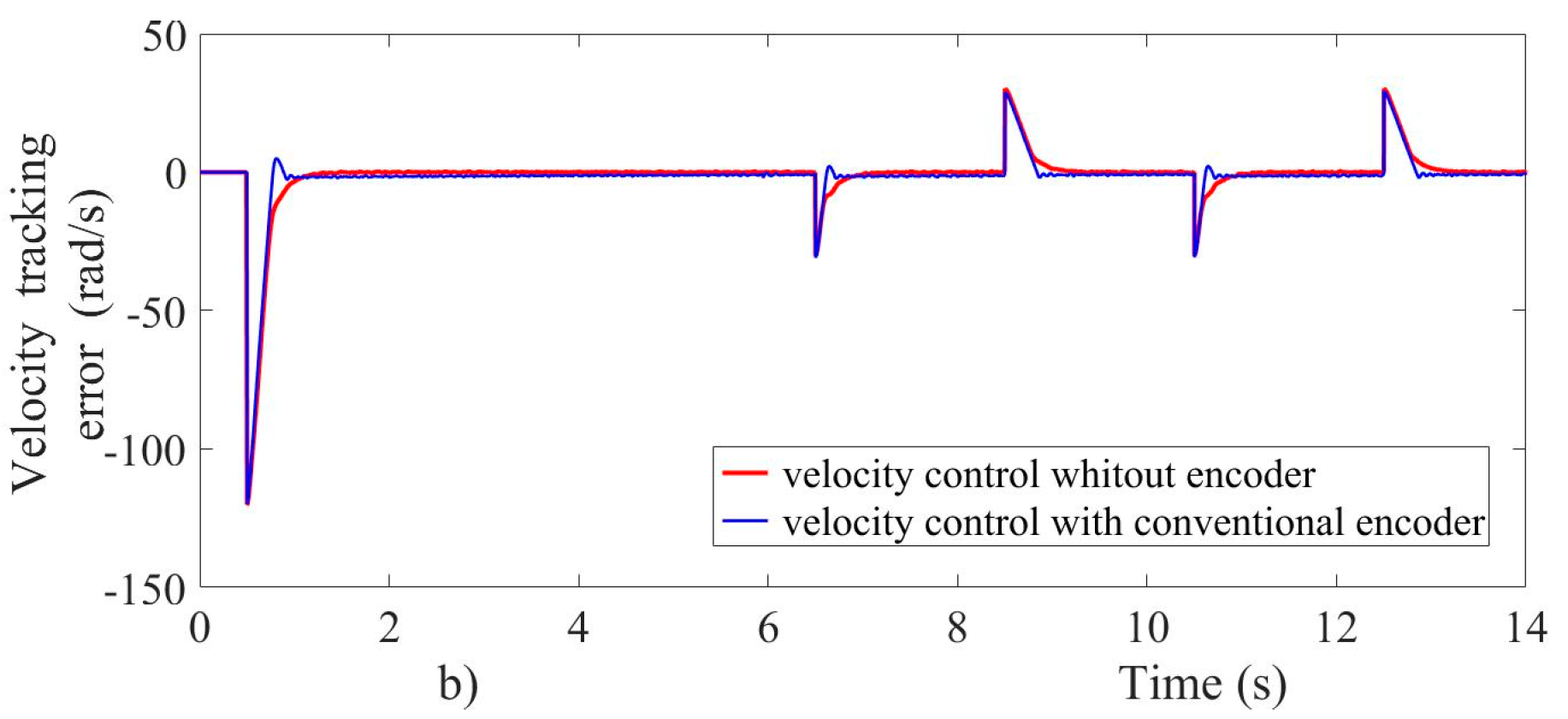
5.2. Experimental Results
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Hughes, A.; Drury, B. Electric Motors and Drives Fundamentals, Types and Applications, 5th ed.; Elsevier Ltd.: Amsterdam, The Netherlands, 2019. [Google Scholar] [CrossRef]
- Anatoli, S.; Naung, Y.; Lin, H.O.; Khaing, Z.M.; Zaw, K.Y. The comparative analysis of modelling of simscape physical plant system design and armature-controlled system design of DC motor. In Proceedings of the 2017 IEEE Conference of Russian Young Researchers in Electrical and Electronic Engineering (EIConRus), St. Petersburg, Russia, 1–3 February 2017; pp. 998–1002. [Google Scholar] [CrossRef]
- Ramírez, R.; Valenzuela, F.A.; Martínez, F.; Castañeda, C.E.; Morfin, O.A.; Olmos, J.A. Speed control of a DC motor based on armature current measurement. Ing. Investig. y Tecnol. 2018, 19. [Google Scholar] [CrossRef]
- Wei, Z.; Zhao, J.; Ji, D.; Tseng, K.J. A multi-timescale estimator for battery state of charge and capacity dual estimation based on an online identified model. Appl. Energy 2017, 204, 1264–1274. [Google Scholar] [CrossRef]
- Wei, Z.; Dong, G.; Zhang, X.; Pou, J.; Quan, Z.; He, H. Noise-immune model identification and state of charge estimation for lithium-ion battery using bilinear parameterization. IEEE Trans. Ind. Electron. 2020. [Google Scholar] [CrossRef]
- Utkin, V.; Guldner, J.; Shi, J. Sliding Mode Control in Electro-Mechanical Systems; CRC Press, Taylor & Francis Group: New York, NY, USA, 2009. [Google Scholar] [CrossRef]
- Tang, Y.; Zhang, Y.; Hasankhani, A.; Zwieten, J.V. Adaptive Super-Twisting Sliding Mode Control for Ocean Current Turbine-Driven Permanent Magnet Synchronous Generator. In Proceedings of the 2020 American Control Conference (ACC), Denver, CO, USA, 1–3 July 2020; pp. 211–217. [Google Scholar] [CrossRef]
- Ferrara, A.; Incremona, G.P.; Sangiovanni, B. Tracking control via switched Integral Sliding Mode with application to robot manipulators. Control Eng. Pract. 2019, 90, 257–266. [Google Scholar] [CrossRef] [Green Version]
- Yousri, M.S.; Derbeli, M.; Barambones, O.; Cheknane, A. Design and Implementation of High Order Sliding Mode Control for PEMFC Power System. Energies 2020, 13, 4317. [Google Scholar] [CrossRef]
- Ruderman, M.; Fridman, L.; Pasolli, P. Virtual sensing of load forces in hydraulic actuators using second- and higher-order sliding modes. Control Eng. Pract. 2019, 92, 104151. [Google Scholar] [CrossRef]
- Fridman, L.; Levant, A. Higher Order Sliding Modes. In Sliding Mode Control in Engineering; Barbot, J.P., Perruguetti, W., Eds.; Marcel Dekker: New York, NY, USA, 2002. [Google Scholar]
- Levant, A. Principles of 2-sliding mode design. Automatica 2007, 43, 576–586. [Google Scholar] [CrossRef] [Green Version]
- Bartolini, G.; Fridman, L.; Pisano, A.; Usai, E. Modern Sliding Mode Control Theory: New Perspectives and Applications; Springer Science: New York, NY, USA, 2008; Volume 375. [Google Scholar]
- Ahmed, Q.; Bhatti, A.I.; Iqbal, S.; Kazmi, I.H. 2-sliding mode based robust control for 2-DOF helicopter. In Proceedings of the 2010 11th International Workshop on Variable Structure Systems (VSS), Mexico City, Mexico, 26–28 June 2010; pp. 481–486. [Google Scholar] [CrossRef]
- Bartolini, G.; Pisano, A.; Punta, E.; Usai, E. A Survey of Applications of Second-order Sliding Mode Control to Mechanical Systems. Int. J. Control 2003, 76, 875–892. [Google Scholar] [CrossRef]
- Davila, J.; Fridman, L.; Levant, A. Second-order sliding-mode observer for mechanical systems. IEEE Trans. Autom. Control 2005, 50, 1785–1789. [Google Scholar] [CrossRef]
- Krause, P.C.; Wasynczuk, O.; Sudhoff, S.D. Analysis of Electric Machinery and Drive Systems; IEEE Press: Piscataway, NJ, USA, 2002. [Google Scholar]
- Isidori, A. Nonlinear Control Systems; Springer: New York, NY, USA, 1995. [Google Scholar]
- Li, Y.; Zhang, H.; Liang, X.; Huang, B. Event-Triggered-Based Distributed Cooperative Energy Management for Multienergy Systems. IEEE Trans. Ind. Inform. 2018, 15, 2008–2022. [Google Scholar] [CrossRef]
- Li, Y.; Gao, D.W.; Gao, W.; Zhang, H.; Zhou, J. Double-Mode Energy Management for Multi-Energy System via Distributed Dynamic Event-Triggered Newton-Raphson Algorithm. IEEE Trans. Smart Grid 2020, 11, 5339–5356. [Google Scholar] [CrossRef]
- Zhou, J.; Xu, Y.; Sun, H.; Wang, L.; Chow, M.Y. Distributed Event-Triggered H∞ Consensus Based Current Sharing Control of DC Microgrids Considering Uncertainties. IEEE Trans. Ind. Inform. 2020, 16, 7413–7425. [Google Scholar] [CrossRef]
- Yi, Z.; Xu, Y.; Gu, W.; Wu, W. A Multi-Time-Scale Economic Scheduling Strategy for Virtual Power Plant Based on Deferrable Loads Aggregation and Disaggregation. IEEE Trans. Sustain. Energy 2020, 11, 1332–1346. [Google Scholar] [CrossRef]
- Yi, Z.; Xu, Y.; Zhou, J.; Wu, W.; Sun, H. Bi-Level Programming for Optimal Operation of an Active Distribution Network With Multiple Virtual Power Plants. IEEE Trans. Sustain. Energy 2020, 11, 2855–2869. [Google Scholar] [CrossRef]
- Khalil, H.K. Nonlinear Systems, 3rd ed.; Pearson Education: New York, NY, USA, 2002. [Google Scholar]





| Parameter | Value |
|---|---|
| gain L1 | 174 |
| gain L2 | −14 |
| gain αo | 0.075 |
| gain λo | 0.75 |
| Parameter | Value |
|---|---|
| Power | 0.175 Kw |
| Armature rated DC voltage | 120 V |
| Field rated DC voltage | 120 V |
| Armature rated current | 2.8 A |
| Rated angular velocity | 1800 rpm |
| Armature resistance, Ra | 8.32 Ω |
| Armature inductance, La | 0.0813 H |
| Field resistance, Rf | 343 Ω |
| Mutual inductance, Laf | 1.57 H |
| Motor constant, km | 0.549 V/rad/s |
| Rotor inertial moment, Je | 0.0099 Kgm2 |
| Rotor friction coefficient, Bm | 0.00083 Nm/rad/s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Valenzuela, F.A.; Ramírez, R.; Martínez, F.; Morfín, O.A.; Castañeda, C.E. Super-Twisting Algorithm Applied to Velocity Control of DC Motor without Mechanical Sensors Dependence. Energies 2020, 13, 6041. https://doi.org/10.3390/en13226041
Valenzuela FA, Ramírez R, Martínez F, Morfín OA, Castañeda CE. Super-Twisting Algorithm Applied to Velocity Control of DC Motor without Mechanical Sensors Dependence. Energies. 2020; 13(22):6041. https://doi.org/10.3390/en13226041
Chicago/Turabian StyleValenzuela, Fredy A., Reymundo Ramírez, Fermín Martínez, Onofre A. Morfín, and Carlos E. Castañeda. 2020. "Super-Twisting Algorithm Applied to Velocity Control of DC Motor without Mechanical Sensors Dependence" Energies 13, no. 22: 6041. https://doi.org/10.3390/en13226041
APA StyleValenzuela, F. A., Ramírez, R., Martínez, F., Morfín, O. A., & Castañeda, C. E. (2020). Super-Twisting Algorithm Applied to Velocity Control of DC Motor without Mechanical Sensors Dependence. Energies, 13(22), 6041. https://doi.org/10.3390/en13226041