Generic Dynamical Model of PEM Electrolyser under Intermittent Sources
Abstract
:1. Introduction
2. BG Technique for Model Building
2.1. BG Elements and BG Variables
2.2. Different Levels of Modelling Abstraction
2.3. Modular Building (Capsules)
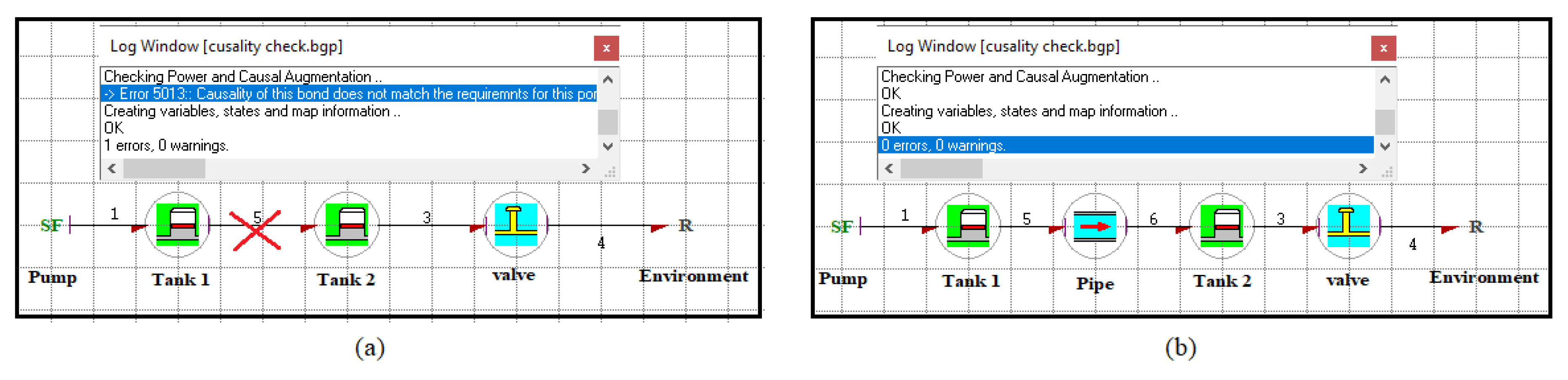
Grammar and Connectivity Rules
3. PEM Electrolyser Modelling
- The cells constituting the stack are identical in nature and connected in series. Thus, the stack with N cells can be modelled as an equivalent single cell that has the same dynamics of the stack.
- Uniform fluid flows and current distribution are considered between cells.
- Overpotential due to mass transport or diffusion is negligible with the assumption that PEM system usually operates at low current density.
- Electrolysis reaction kinetics is assumed firmly as a Faradic process and considers that there is no mass limitation problem in the system.
- Gases produced are assumed to have similar properties as that of an ideal gas and the partial pressures of these gases are governed by Dalton’s law.
- Temperature is homogenous throughout the stack.
- Cell is operated below the boiling temperature of the water.
- The system parameters are considered as lumped parameters. Pumps and fans are assumed as perfect mass flow sources.
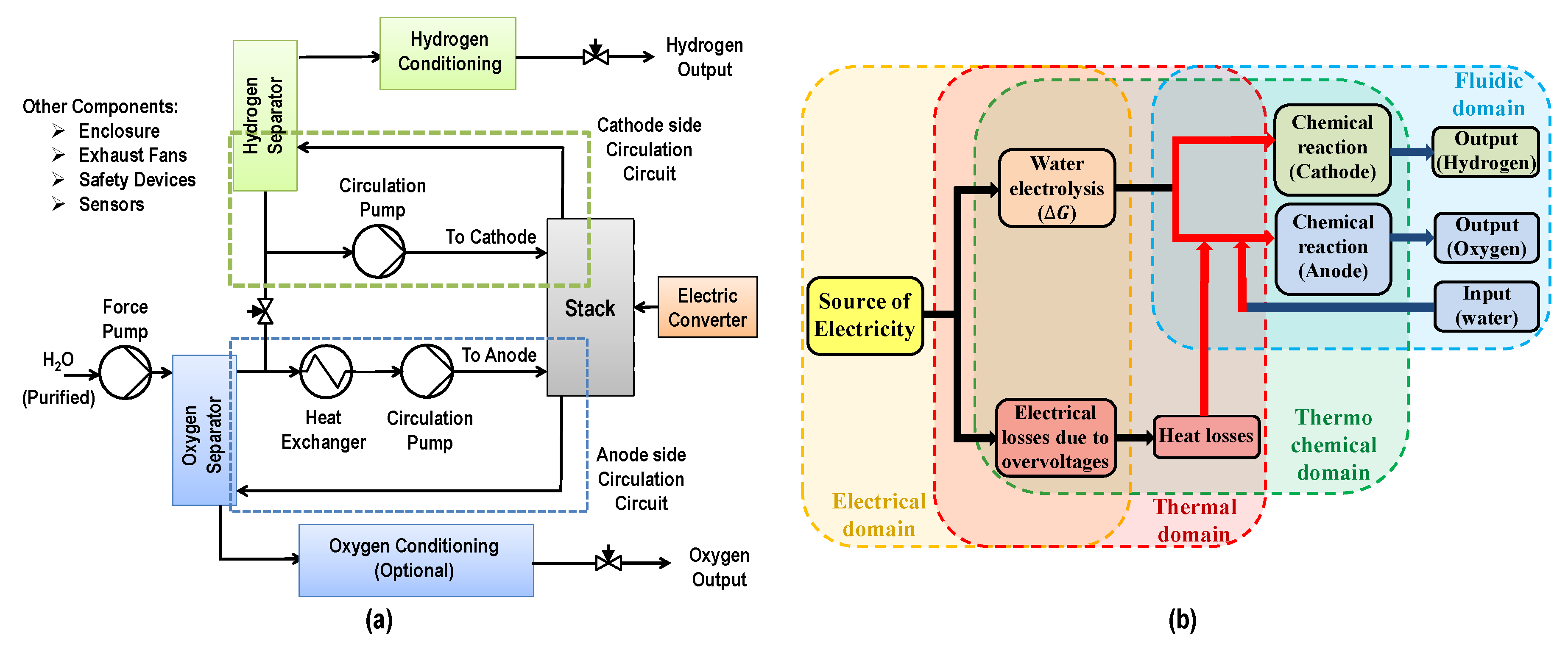
3.1. Technological Representation
3.2. Modular Representation
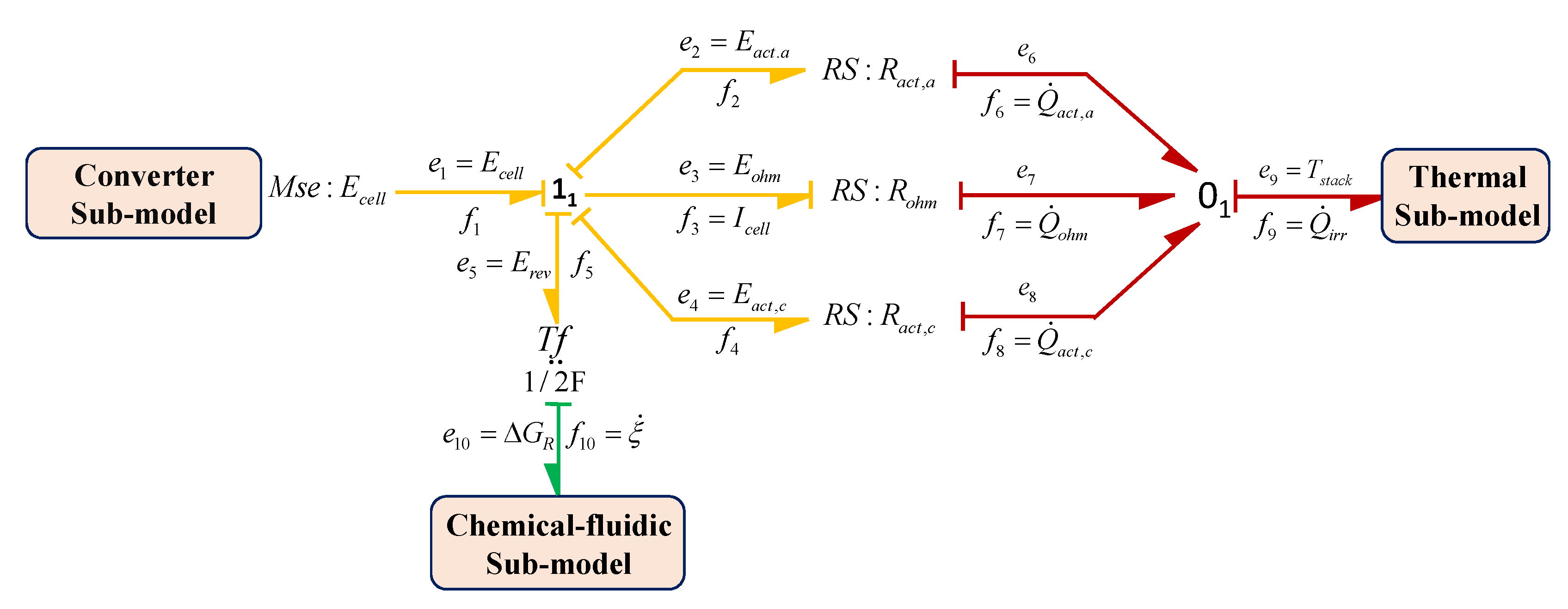
3.2.1. Stack Model
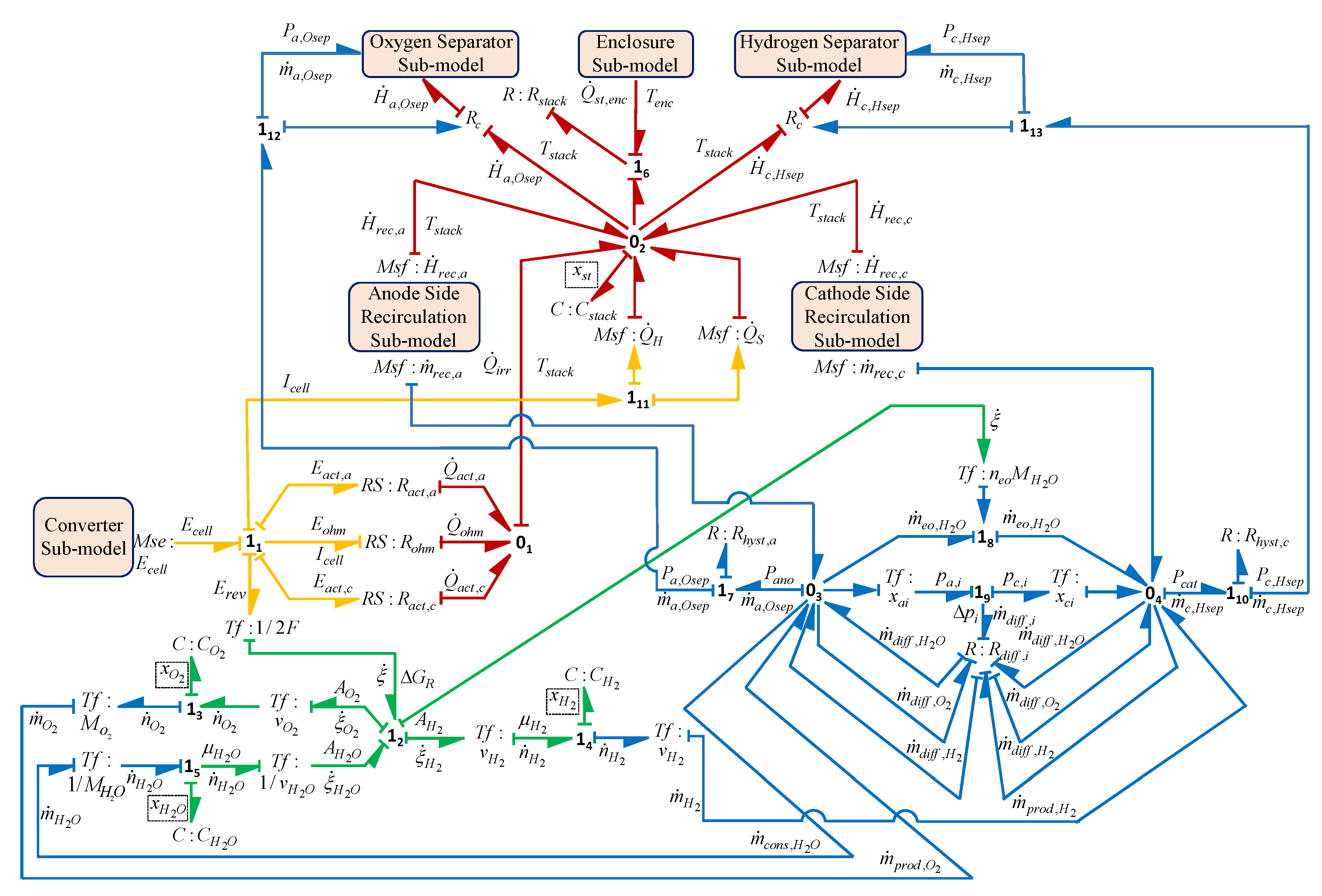
Electrochemical Sub-Model of the Stack
Chemical-Fluidic Sub-Model of the Stack
Thermal Sub-Model of the Stack
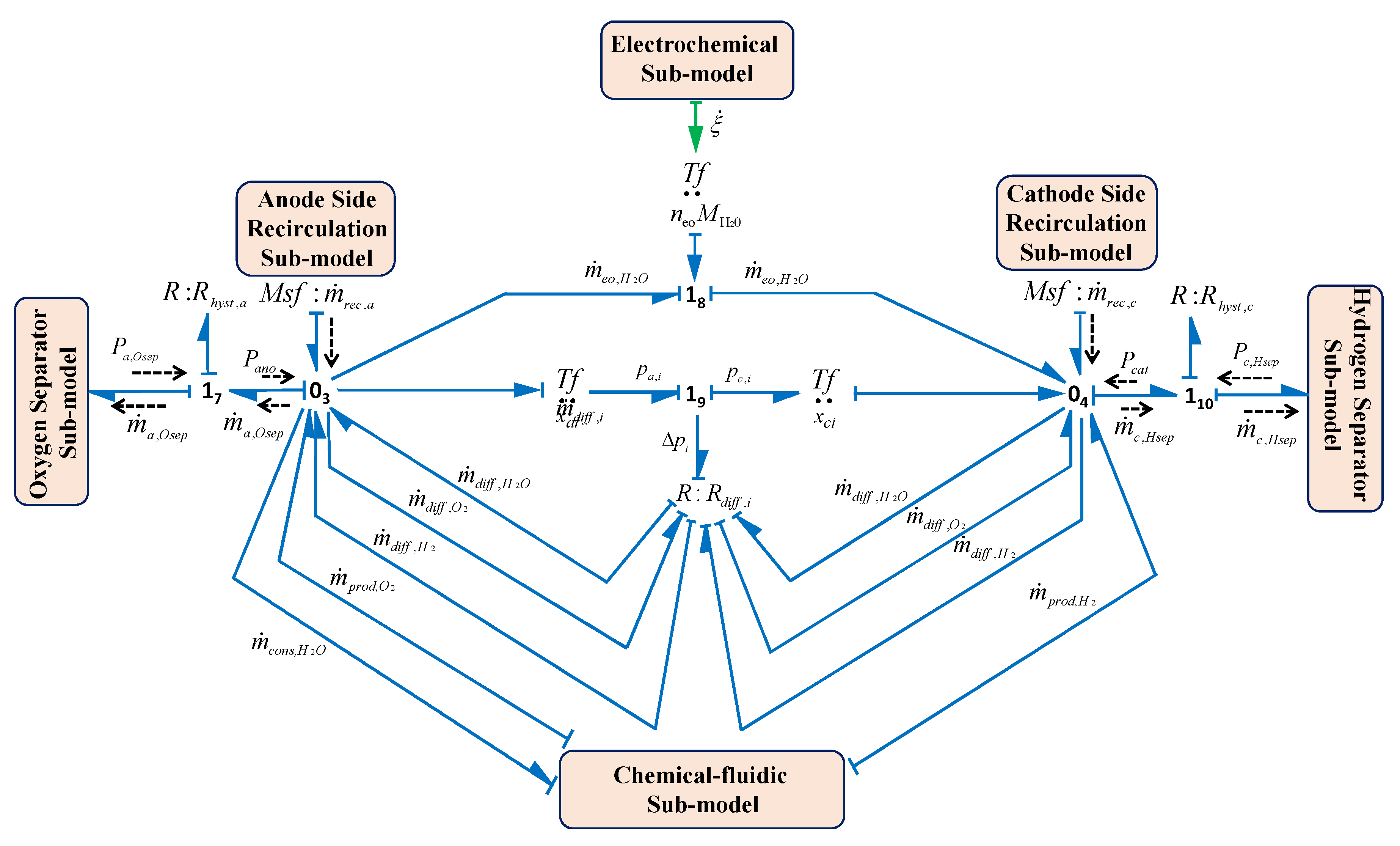
Fluidic and Mass Transfer Sub-Model
3.2.2. Converter Sub-Model
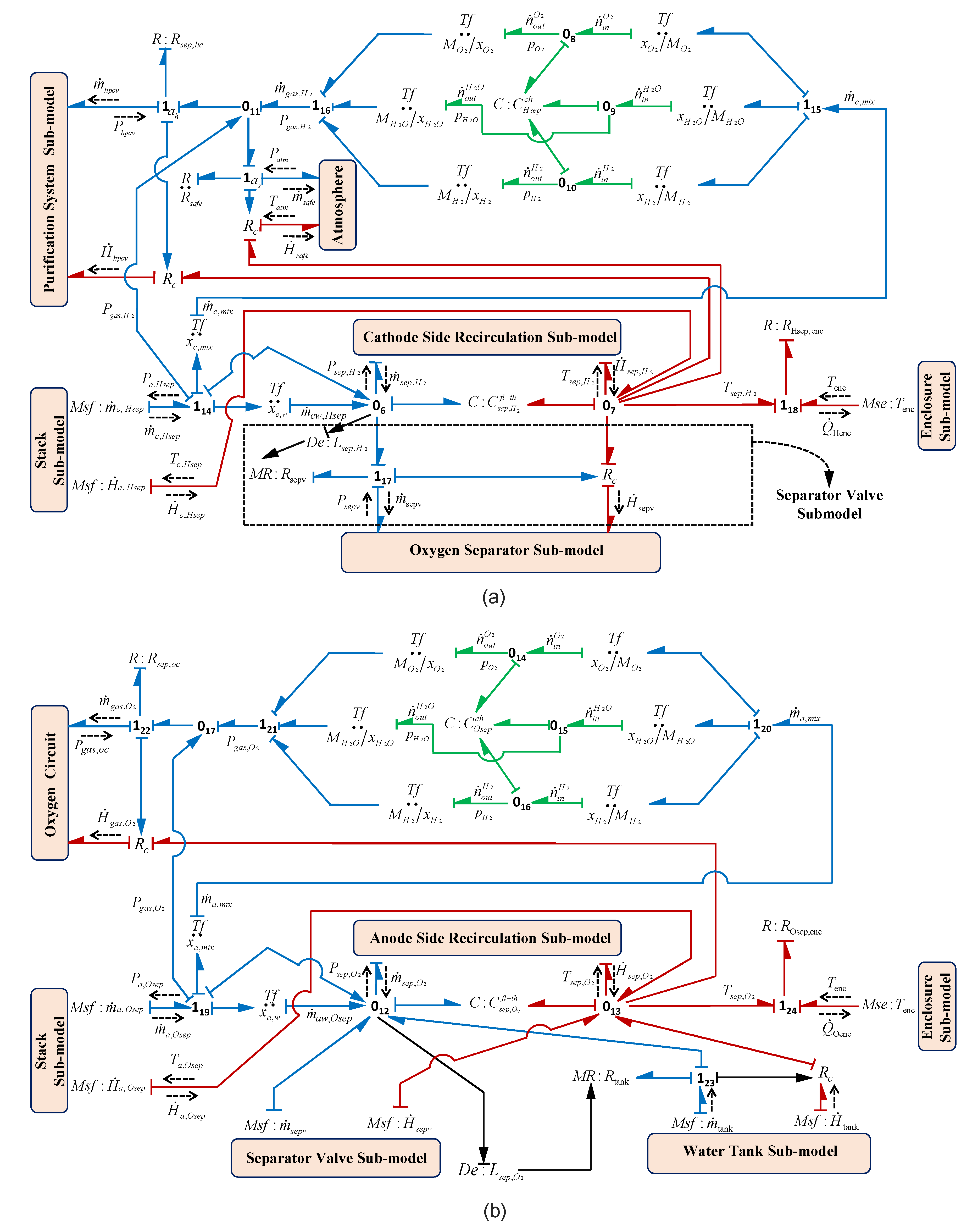
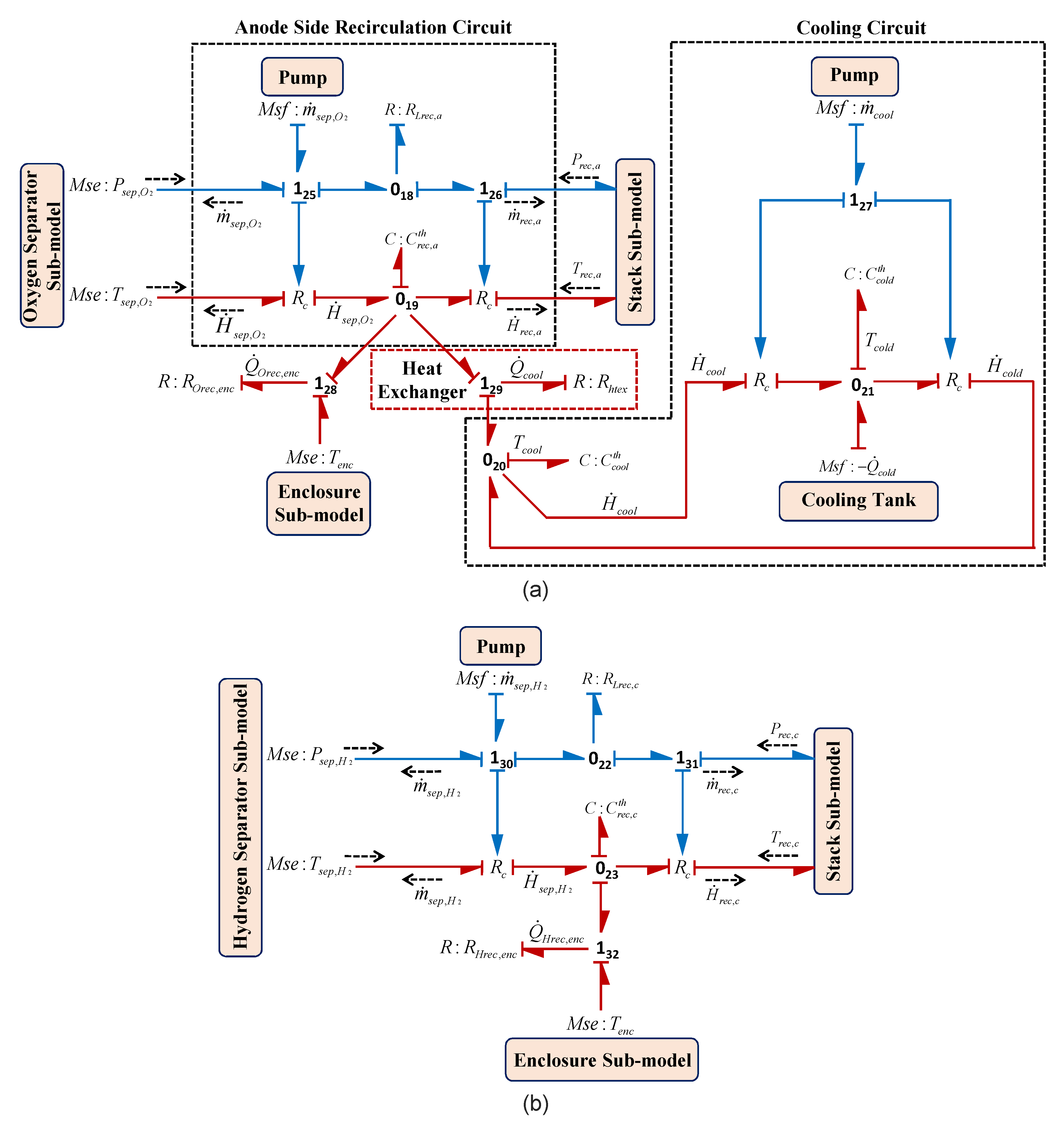
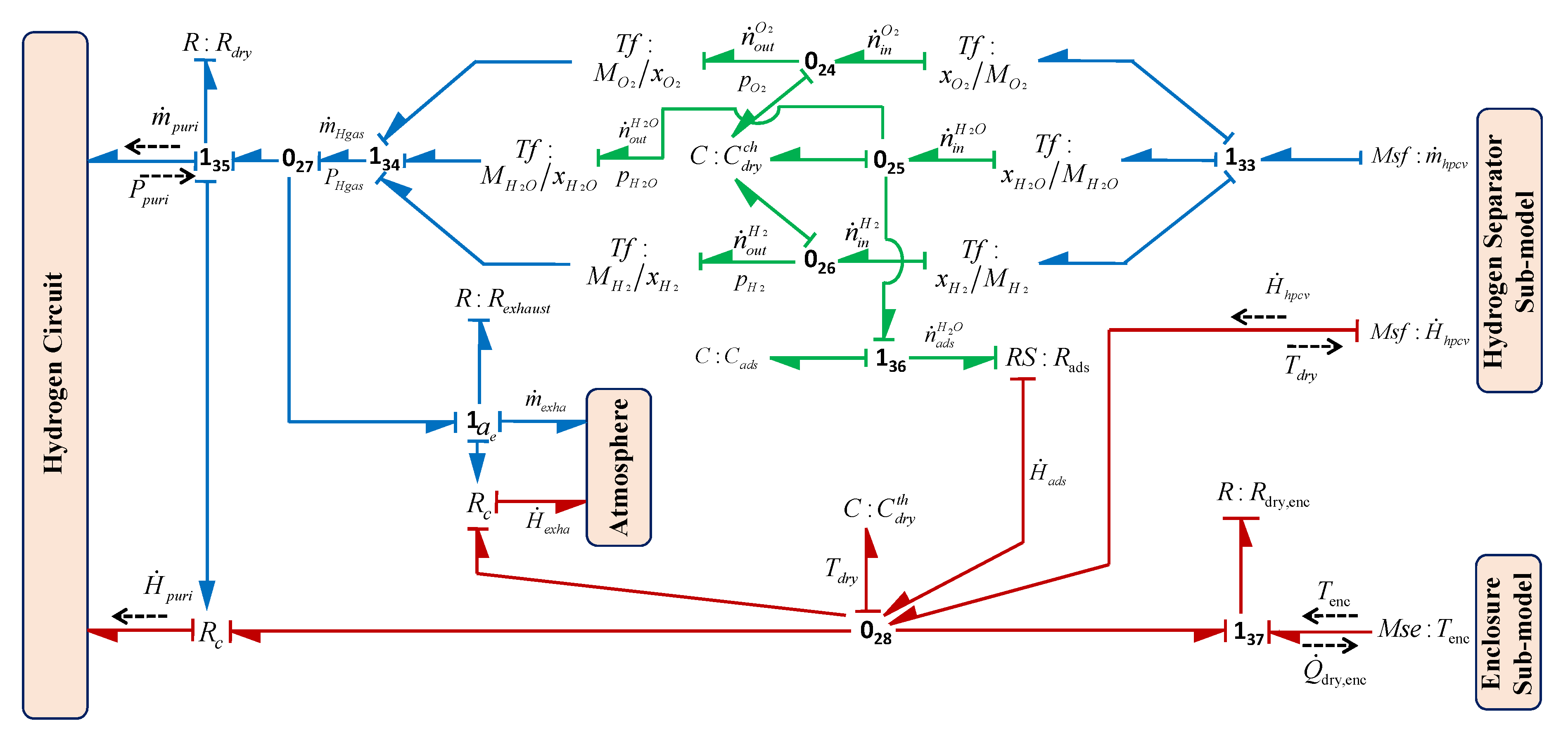
3.2.3. Separator Sub-Models
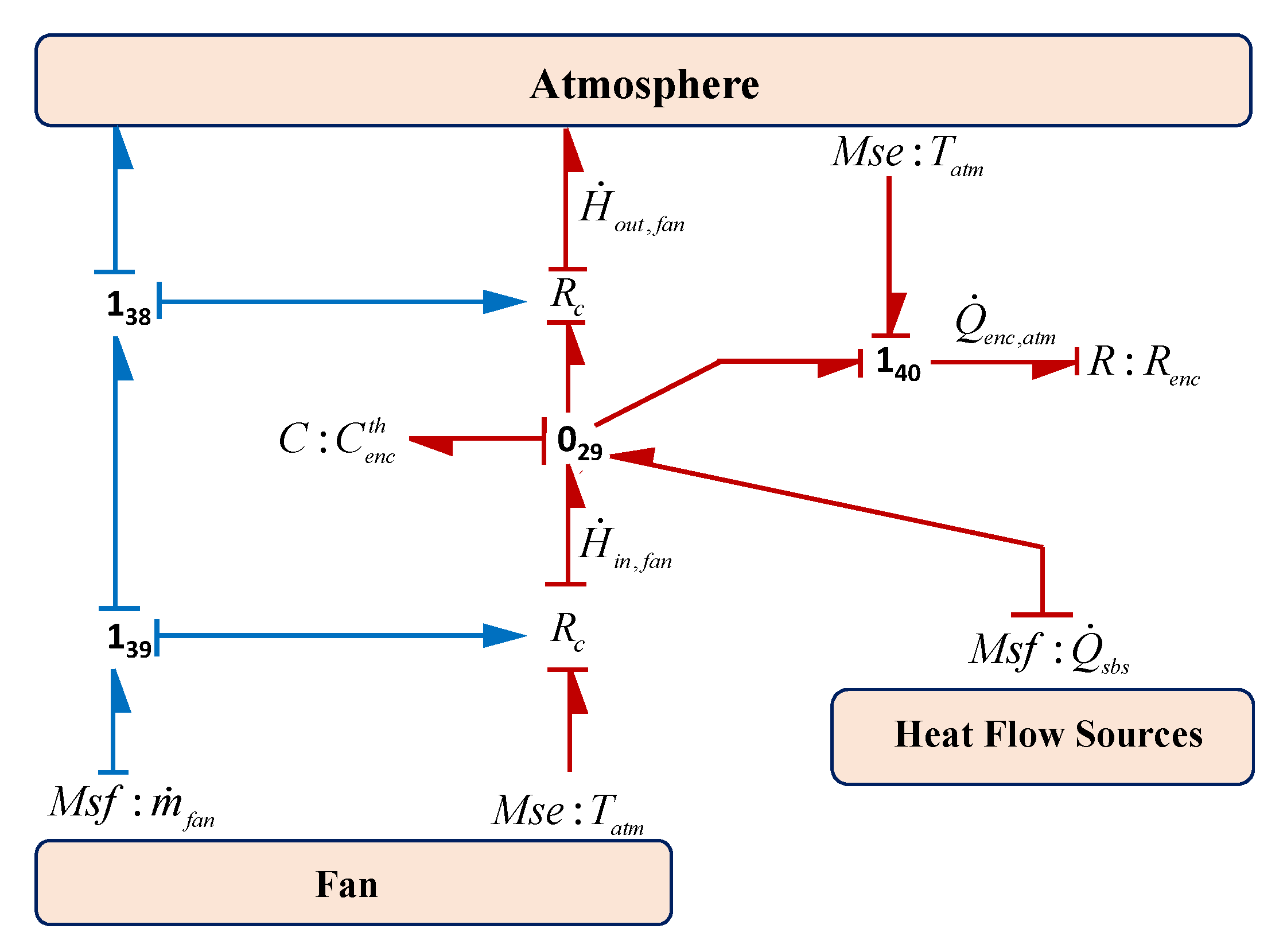
3.2.4. Cooling and Recirculation Circuits
3.2.5. Hydrogen Purification Subsystem
3.2.6. System Enclosure
3.3. Efficiency of the PEM Electrolysis System
3.3.1. Efficiency of Cell/Stack
3.3.2. Efficiency of System Including the Auxiliaries
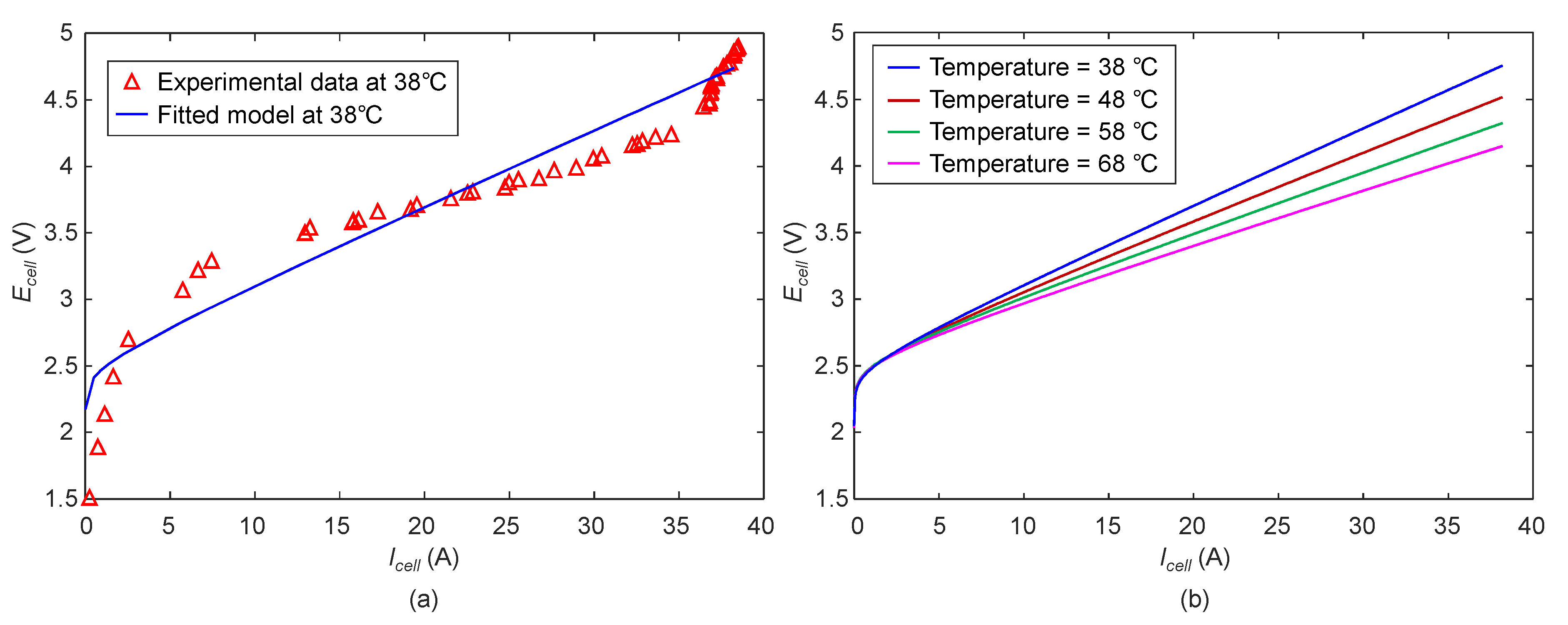
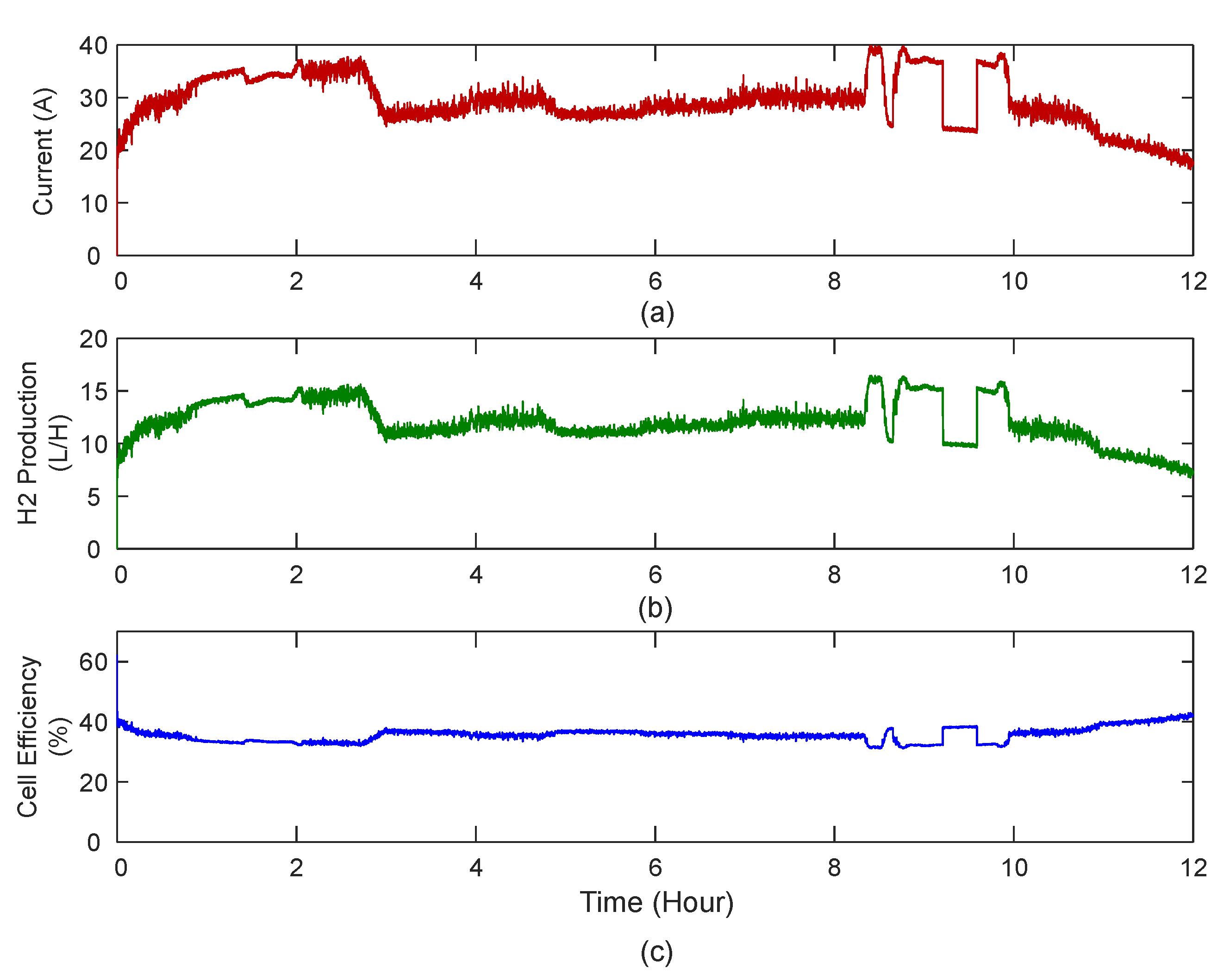
4. Experimental Validation and Results
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| AEM | Anion Exchange Membrane |
| BG | Bond Graph |
| DC | Direct Current |
| HHV | Higher Heating Value |
| HSV | Hydrogen Separator Vessel |
| LHV | Lower Heating Value |
| MEA | Membrane Electrode Assembly |
| NTU | Number of Transfer Unit |
| OSV | Oxygen Separator Vessel |
| PEM | Proton Exchange Membrane |
| RES | Renewable Energy Sources |
| Nomenclature | |
| Charge transfer or symmetry factor coefficients for electrode | |
| Transformer coefficient of the converter | |
| Gibb’s free energy of water dissociation reaction, J·mol | |
| Enthalpy Change of water dissociation reaction, J·mol | |
| Entropy change of water dissociation reaction, J·mol·K | |
| Rate of reaction flow, mol·s | |
| Enthalpy rate, J·s | |
| Mass flow rate of the species, kg·s | |
| Gas mass flow rate for species, kg·s | |
| Rate of heat flow, J·s | |
| Hydration of the membrane | |
| Coefficient of stoichiometry for species | |
| Water density, kg·m | |
| Conductivity of the membrane, S·m | |
| Efficiency | |
| Cross-sectional area of the membrane, m | |
| Cross-sectional area of the separator, m | |
| Chemical activity of water | |
| Matter storage capacity of the species (), kg·J | |
| Specific heat at constant pressure, J·kg·K | |
| Thermal capacitance of cooling tank, J·K | |
| Thermal capacitance of cooling circuit, J·K | |
| Dryer’s chemical capacitance of the purification unit for the species, mol·Pa | |
| Dryer’s thermal capacitance of the purification unit, J·K | |
| Thermal capacity of the enclosure, J·K | |
| Chemical capacitance of hydrogen separator for the species, mol·Pa | |
| Chemical capacitance of oxygen separator for the species, mol·Pa | |
| Thermal capacitance of recirculation circuit (anode/cathode side), J·K | |
| Field capacitance element representing fluidic capacitance and thermal capacitance of hydrogen separator | |
| Field capacitance element representing fluidic capacitance and thermal capacitance of oxygen separator | |
| Thermal capacitance of the stack, J·K | |
| Parameter for diffusion, m·s | |
| Ratio of length to the cross-sectional area of the membrane, m | |
| Activation overvoltages for electrode, V | |
| Cell voltage, V | |
| Ohmic overvoltage, V | |
| Reversible voltage, V | |
| Standard reversible cell voltage at STP, V | |
| g | Acceleration due to gravity, m·s |
| Henry’s Parameter, Pa·m·mol | |
| Cell current, A | |
| J | Current density, A·m |
| Standard current exchange density for electrode, A·m | |
| Length of the membrane, m | |
| Water level in Separators (HSV and OSV), m | |
| Water level of the separator, m | |
| Molar mass for species, kg mol | |
| Electro-osmosis coefficient | |
| P | Pressure, Pa |
| Partial pressure of species, Pa | |
| Coupling element for fluidic flow to thermal flow | |
| Non linear activation resistance for electrode | |
| Coupling resistance of adsorbed water molar flow and the enthalpy flow towards dryer | |
| Diffusion resistance of the species, Pa·s·kg | |
| Thermal resistance between purification unit and enclosure, K·s·J | |
| Internal pneumatic resistance of the dryer, Pa·s·kg | |
| Thermal resistance between the enclosure and the atmosphere, K·s·J | |
| Pneumatic resistance of the exhaust valve, Pa·s·kg | |
| Thermal resistance between hydrogen recirculation circuit and enclosure, K·s·J | |
| Thermal resistance between hydrogen separator and enclosure, K·s·J | |
| Thermal resistance of heat exchanger, K·s·J | |
| Internal fluidic resistance of the stack at th electrode side, Pa·s·kg | |
| Hydraulic resistance representing leakage in recirculation circuit (anode/cathode side), Pa·s·kg | |
| Total ohmic resistance of the cell, | |
| Thermal resistance between oxygen recirculation circuit and enclosure, K·s·J | |
| Thermal resistance between oxygen separator and enclosure, K·s·J | |
| Ohmic resistance of the cell except membrane, | |
| Pneumatic resistance between hydrogen separator and hydrogen circuit, Pa·s·kg | |
| Pneumatic resistance between oxygen separator and oxygen circuit, Pa·s·kg | |
| Hydraulic resistance of the separator valve, Pa·s·kg | |
| Thermal resistance of the stack, K·s·J | |
| Hydraulic resistance between tank and oxygen Separator, Pa·s·kg | |
| T | Temperature, K |
| Volume of the species in HSV, m | |
| Volume of the species in OSV, m | |
| Mass fraction of the species | |
| Chemical potential of the species, J·kg | |
| Chemical affinity of the species, J·mol | |
| Water adsorption capacity of the purification unit, mol·Pa | |
| F | Faraday’s constant, C·mol |
| R | Ideal gas constant, J·mol·K |
References
- Jain, I.P. Hydrogen the fuel for 21st century. Int. J. Hydrogen Energy 2009, 34, 7368–7378. [Google Scholar] [CrossRef]
- Olivier, P.; Bourasseau, C.; Ould-Bouamama, B. Low-temperature electrolysis system modelling: A review. Renew. Sustain. Energ. Rev. 2017, 78, 280–300. [Google Scholar] [CrossRef]
- David, M.; Ocampo-Martínez, C.; Sánchez-Peña, R. Advances in alkaline water electrolyzers: A review. J. Energy Storage 2019, 23, 392–403. [Google Scholar] [CrossRef] [Green Version]
- Vincent, I.; Bessarabov, D. Low cost hydrogen production by anion exchange membrane electrolysis: A review. Renew. Sustain. Energ. Rev. 2018, 81, 1690–1704. [Google Scholar] [CrossRef]
- Sood, S.; Ould-Bouamama, B.; Dieulot, J.Y.; Bressel, M.; Li, X.; Ullah, H.; Loh, A. Bond graph based multiphysic modelling of anion exchange membrane water electrolysis cell. In Proceedings of the 28th Mediterranean Conference on Control and Automation (MED), Saint-Raphaël, France, 15–18 September 2020; pp. 752–757. [Google Scholar] [CrossRef]
- Ipsakis, D.; Voutetakis, S.; Seferlis, P.; Stergiopoulos, F.; Elmasides, C. Power management strategies for a stand-alone power system using renewable energy sources and hydrogen storage. Int. J. Hydrogen Energy 2009, 34, 7081–7095. [Google Scholar] [CrossRef]
- Giddey, S.; Badwal, S.P.; Ju, H. Polymer electrolyte membrane technologies integrated with renewable energy for hydrogen production. In Current Trends and Future Developments on (Bio-) Membranes; Elsevier: Amsterdam, The Netherlands, 2019; pp. 235–259. [Google Scholar] [CrossRef]
- Carmo, M.; Fritz, D.L.; Mergel, J.; Stolten, D. A comprehensive review on PEM water electrolysis. Int. J. Hydrogen Energy 2013, 38, 4901–4934. [Google Scholar] [CrossRef]
- Wirkert, F.J.; Roth, J.; Jagalski, S.; Neuhaus, P.; Rost, U.; Brodmann, M. A modular design approach for PEM electrolyser systems with homogeneous operation conditions and highly efficient heat management. Int. J. Hydrogen Energy 2020, 45, 1226–1235. [Google Scholar] [CrossRef]
- Falcão, D.; Pinto, A.M.F.R. A review on PEM Electrolyzer Modelling: Guidelines for beginners. J. Clean Prod. 2020, 261, 121184:1–121184:10. [Google Scholar] [CrossRef]
- Saeed, W.; Warkozek, G. Modeling and analysis of renewable PEM fuel cell system. Energy Procedia 2015, 74, 87–101. [Google Scholar] [CrossRef] [Green Version]
- Xie, B.; Zhang, G.; Xuan, J.; Jiao, K. Three-dimensional multi-phase model of PEM fuel cell coupled with improved agglomerate sub-model of catalyst layer. Energy Convers. Manag. 2019, 199, 112051:1–112051:12. [Google Scholar] [CrossRef]
- Vuppala, R.K.; Chaedir, B.A.; Jiang, L.; Chen, L.; Aziz, M.; Sasmito, A.P. Optimization of Membrane Electrode Assembly of PEM Fuel Cell by Response Surface Method. Molecules 2019, 24, 3097. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vijay, P.; Samantaray, A.K.; Mukherjee, A. A bond graph model-based evaluation of a control scheme to improve the dynamic performance of a solid oxide fuel cell. Mechatronics 2009, 19, 489–502. [Google Scholar] [CrossRef]
- Amiri, A.; Vijay, P.; Tadé, M.O.; Ahmed, K.; Ingram, G.D.; Pareek, V.; Utikar, R. Solid oxide fuel cell reactor analysis and optimisation through a novel multi-scale modelling strategy. Comput. Chem. Eng. 2015, 78, 10–23. [Google Scholar] [CrossRef] [Green Version]
- Hernández-Gómez, Á.; Ramirez, V.; Guilbert, D. Investigation of PEM electrolyzer modeling: Electrical domain, efficiency, and specific energy consumption. Int. J. Hydrogen Energy 2020, 45, 14625–14639. [Google Scholar] [CrossRef]
- Ruuskanen, V.; Koponen, J.; Huoman, K.; Kosonen, A.; Niemelä, M.; Ahola, J. PEM water electrolyzer model for a power-hardware-in-loop simulator. Int. J. Hydrogen Energy 2017, 42, 10775–10784. [Google Scholar] [CrossRef]
- Liso, V.; Savoia, G.; Araya, S.S.; Cinti, G.; Kær, S.K. Modelling and experimental analysis of a polymer electrolyte membrane water electrolysis cell at different operating temperatures. Energies 2018, 11, 3273. [Google Scholar] [CrossRef] [Green Version]
- Fragiacomo, P.; Genovese, M. Modeling and energy demand analysis of a scalable green hydrogen production system. Int. J. Hydrogen Energy 2019, 44, 30237–30255. [Google Scholar] [CrossRef]
- Hernández-Gómez, Á.; Ramirez, V.; Guilbert, D.; Saldivar, B. Cell voltage static-dynamic modeling of a PEM electrolyzer based on adaptive parameters: Development and experimental validation. Renew. Energy 2021, 163, 1508–1522. [Google Scholar] [CrossRef]
- Aubras, F.; Rhandi, M.; Deseure, J.; Kadjo, A.J.J.; Bessafi, M.; Majasan, J.; Grondin-Perez, B.; Druart, F.; Chabriat, J.P. Dimensionless approach of a polymer electrolyte membrane water electrolysis: Advanced analytical modelling. J. Power Sources 2021, 481, 228858:1–228858:10. [Google Scholar] [CrossRef]
- Zhou, T.; Francois, B.; el hadi Lebbal, M.; Lecoeuche, S. Modeling and control design of hydrogen production process by using a causal ordering graph for wind energy conversion system. In Proceedings of the IEEE International Symposium on Industrial Electronics, Vigo, Spain, 4–7 June 2007; pp. 3192–3197. [Google Scholar] [CrossRef]
- Agbli, K.; Péra, M.; Hissel, D.; Rallières, O.; Turpin, C.; Doumbia, I. Multiphysics simulation of a PEM electrolyser: Energetic Macroscopic Representation approach. Int. J. Hydrogen Energy 2011, 36, 1382–1398. [Google Scholar] [CrossRef]
- Olivier, P.; Bourasseau, C.; Ould-Bouamama, B. Dynamic and multiphysic PEM electrolysis system modelling: A bond graph approach. Int. J. Hydrogen Energy 2017, 42, 14872–14904. [Google Scholar] [CrossRef]
- Samantaray, A.K.; Ould-Bouamama, B. Model-Based Process Supervision: A Bond Graph Approach; Springer: London, UK, 2008. [Google Scholar] [CrossRef]
- Merzouki, R.; Samantaray, A.K.; Pathak, P.M.; Ould-Bouamama, B. Intelligent Mechatronic Systems: Modeling, Control and Diagnosis; Springer: London, UK, 2012. [Google Scholar] [CrossRef]
- Ould-Bouamama, B.; El Harabi, R.; Abdelkrim, M.N.; Gayed, M.K.B. Bond graphs for the diagnosis of chemical processes. Comput. Chem. Eng. 2012, 36, 301–324. [Google Scholar] [CrossRef]
- Prakash, O.; Samantaray, A.K.; Bhattacharyya, R. Model-based diagnosis of multiple faults in hybrid dynamical systems with dynamically updated parameters. IEEE Trans. Syst. Man Cybern. Syst. 2017, 49, 1053–1072. [Google Scholar] [CrossRef]
- Prakash, O.; Samantaray, A.K.; Bhattacharyya, R. Model-based multi-component adaptive prognosis for hybrid dynamical systems. Control Eng. Pract. 2018, 72, 1–18. [Google Scholar] [CrossRef]
- Prakash, O.; Samantaray, A.K.; Bhattacharyya, R. Adaptive Prognosis of Hybrid Dynamical System for Dynamic Degradation Patterns. IEEE Trans. Ind. Electron. 2019, 67, 5717–5728. [Google Scholar] [CrossRef]
- Paynter, H.M. Analysis and Design of Engineering Systems; The M.I.T. Press: Cambridge, MA, USA, 1961. [Google Scholar]
- Thoma, J.; Ould-Bouamama, B. Modelling and Simulation in Thermal and Chemical Engineering: A Bond Graph Approach; Springer-Verlag: Berlin/Heidelberg, Germany, 2000. [Google Scholar] [CrossRef]
- Ould-Bouamana, B.; Cassar, J.P. Integrated bond graph modelling in process engineering linked with economic systems. In Proceedings of the 14th European Simulation Multiconference on Simulation and Modelling: Enablers for a Better Quality of Life, Ghent, Belgium, 23–26 May 2000; pp. 340–347. [Google Scholar]
- Ould-Bouamama, B.; Abdalah, I.; Gehin, A.L. Bond graphs as mechatronic approach for supervision design of multisource renewable energy system. In Proceedings of the 5th International Conference on Mechanics and Mechatronics Research (ICMMR), Tokyo, Japan, 19–21 July 2018; pp. 012033:1–012033:5. [Google Scholar] [CrossRef] [Green Version]
- Samantaray, A.K.; Mukherjee, A. Users manual of SYMBOLS Shakti; High-Tech Consultants, STEP, Indian Institute of Technology: Kharagpur, India, 2006. [Google Scholar]
- Kleijn, C.; Groothuis, M.A.; Differ, H.G. 20-Sim 4.3 Reference Manual; Controllab Products: Hengelosestraat, The Netherlands, 2013. [Google Scholar]
- Ould-Bouamama, B.; Samantaray, A.K.; Medjaher, K.; Staroswiecki, M.; Dauphin-Tanguy, G. Model builder using functional and bond graph tools for FDI design. Control Eng. Pract. 2005, 13, 875–891. [Google Scholar] [CrossRef]
- Ould-Bouamama, B.; Staroswiecki, M.; Samantaray, A.K. Software for supervision system design in process engineering industry. IFAC Proc. Vol. 2006, 39, 646–650. [Google Scholar] [CrossRef]
- Ould-Bouamama, B. Bond graph approach as analysis tool in thermofluid model library conception. J. Frankl. Inst. 2003, 340, 1–23. [Google Scholar] [CrossRef]
- Görgün, H. Dynamic modelling of a proton exchange membrane (PEM) electrolyzer. Int. J. Hydrogen Energy 2006, 31, 29–38. [Google Scholar] [CrossRef]
- Abdin, Z.; Webb, C.; Gray, E.M. Modelling and simulation of a proton exchange membrane (PEM) electrolyser cell. Int. J. Hydrogen Energy 2015, 40, 13243–13257. [Google Scholar] [CrossRef]
- Bessarabov, D.; Wang, H.; Li, H.; Zhao, N. PEM Electrolysis for Hydrogen Production: Principles and Applications, 1st ed.; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar] [CrossRef]
- Abdallah, I.; Gehin, A.L.; Ould-Bouamama, B. Event driven Hybrid Bond Graph for Hybrid Renewable Energy Systems part I: Modelling and operating mode management. Int. J. Hydrogen Energy 2018, 43, 22088–22107. [Google Scholar] [CrossRef]
- Marangio, F.; Santarelli, M.; Cali, M. Theoretical model and experimental analysis of a high pressure PEM water electrolyser for hydrogen production. Int. J. Hydrogen Energy 2009, 34, 1143–1158. [Google Scholar] [CrossRef]
- García-Valverde, R.; Espinosa, N.; Urbina, A. Simple PEM water electrolyser model and experimental validation. Int. J. Hydrogen Energy 2012, 37, 1927–1938. [Google Scholar] [CrossRef]
- Kim, H.; Park, M.; Lee, K.S. One-dimensional dynamic modeling of a high-pressure water electrolysis system for hydrogen production. Int. J. Hydrogen Energy 2013, 38, 2596–2609. [Google Scholar] [CrossRef]
- Nafchi, F.M.; Afshari, E.; Baniasadi, E.; Javani, N. A parametric study of polymer membrane electrolyser performance, energy and exergy analyses. Int. J. Hydrogen Energy 2019, 44, 18662–18670. [Google Scholar] [CrossRef]
- Awasthi, A.; Scott, K.; Basu, S. Dynamic modeling and simulation of a proton exchange membrane electrolyzer for hydrogen production. Int. J. Hydrogen Energy 2011, 36, 14779–14786. [Google Scholar] [CrossRef]
- Grigoriev, S.; Porembskiy, V.; Korobtsev, S.; Fateev, V.; Auprêtre, F.; Millet, P. High-pressure PEM water electrolysis and corresponding safety issues. Int. J. Hydrogen Energy 2011, 36, 2721–2728. [Google Scholar] [CrossRef]
- Chandesris, M.; Médeau, V.; Guillet, N.; Chelghoum, S.; Thoby, D.; Fouda-Onana, F. Membrane degradation in PEM water electrolyzer: Numerical modeling and experimental evidence of the influence of temperature and current density. Int. J. Hydrogen Energy 2015, 40, 1353–1366. [Google Scholar] [CrossRef]
- Cengel, Y.; Ghajar, A. Heat and Mass Transfer: Fundamentals and Applications, 5th ed.; McGraw Hill Education: New Delhi, India, 2017. [Google Scholar]
- Scheepers, F.; Stähler, M.; Stähler, A.; Rauls, E.; Müller, M.; Carmo, M.; Lehnert, W. Improving the efficiency of PEM electrolyzers through membrane-specific pressure optimization. Energies 2020, 13, 612. [Google Scholar] [CrossRef] [Green Version]
- LeRoy, R.L.; Bowen, C.T.; LeRoy, D.J. The thermodynamics of aqueous water electrolysis. J. Electrochem. Soc. 1980, 127, 1954–1962. [Google Scholar] [CrossRef]
- Bessarabov, D.; Millet, P. PEM Water Electrolysis; Academic Press: Cambridge, MA, USA, 2017; Volume 1. [Google Scholar] [CrossRef]
- Bessarabov, D.; Millet, P. PEM Water Electrolysis; Academic Press: Cambridge, MA, USA, 2018; Volume 2. [Google Scholar] [CrossRef]


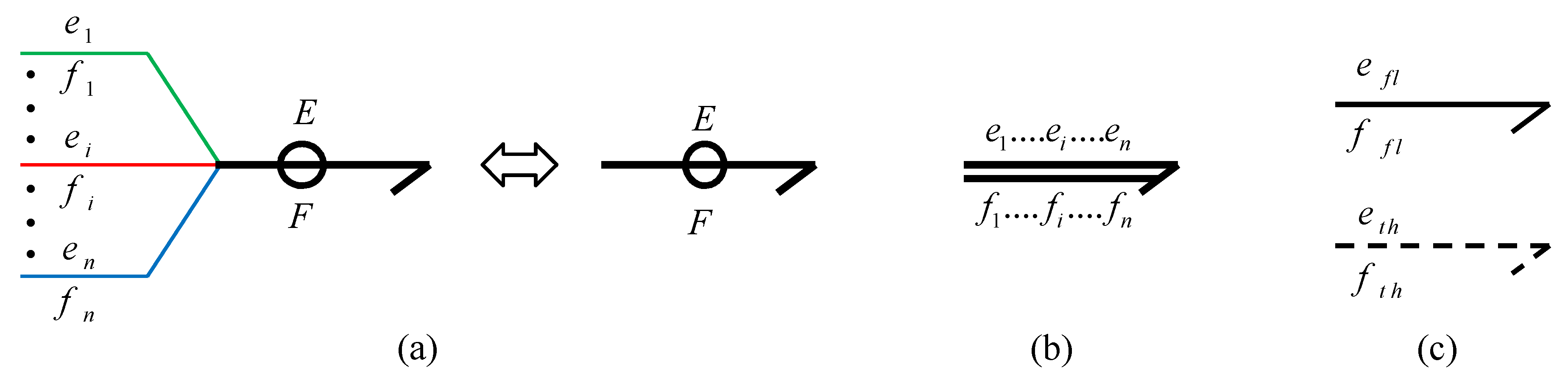










| Energy Domain | Flow (f) | Effort (e) |
|---|---|---|
| Electrical | Current intensity (A) | Voltage (V) |
| Fluidic | Volume flow rate (m·s) | Pressure (Pa) |
| Fluidic (Pseudo BG) | Mass flow (kg·s) | Pressure (Pa) |
| Thermal | Entropy flow (J·K·s) | Temperature (K) |
| Thermal (Pseudo BG) | Thermal flow (J·s) | Temperature (K) |
| Chemical (Transformation) | Molar flow (mol·s) | Chemical potential (J·mol) |
| Chemical (Kinetic) | Reaction flow rate (mol·s) | Chemical affinity (J·mol) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sood, S.; Prakash, O.; Boukerdja, M.; Dieulot, J.-Y.; Ould-Bouamama, B.; Bressel, M.; Gehin, A.-L. Generic Dynamical Model of PEM Electrolyser under Intermittent Sources. Energies 2020, 13, 6556. https://doi.org/10.3390/en13246556
Sood S, Prakash O, Boukerdja M, Dieulot J-Y, Ould-Bouamama B, Bressel M, Gehin A-L. Generic Dynamical Model of PEM Electrolyser under Intermittent Sources. Energies. 2020; 13(24):6556. https://doi.org/10.3390/en13246556
Chicago/Turabian StyleSood, Sumit, Om Prakash, Mahdi Boukerdja, Jean-Yves Dieulot, Belkacem Ould-Bouamama, Mathieu Bressel, and Anne-Lise Gehin. 2020. "Generic Dynamical Model of PEM Electrolyser under Intermittent Sources" Energies 13, no. 24: 6556. https://doi.org/10.3390/en13246556
APA StyleSood, S., Prakash, O., Boukerdja, M., Dieulot, J.-Y., Ould-Bouamama, B., Bressel, M., & Gehin, A.-L. (2020). Generic Dynamical Model of PEM Electrolyser under Intermittent Sources. Energies, 13(24), 6556. https://doi.org/10.3390/en13246556






