Relative Pressure Drop Model for Hydrate Formation and Transportability in Flowlines in High Water Cut Systems
Abstract
1. Introduction
2. Materials and Methods
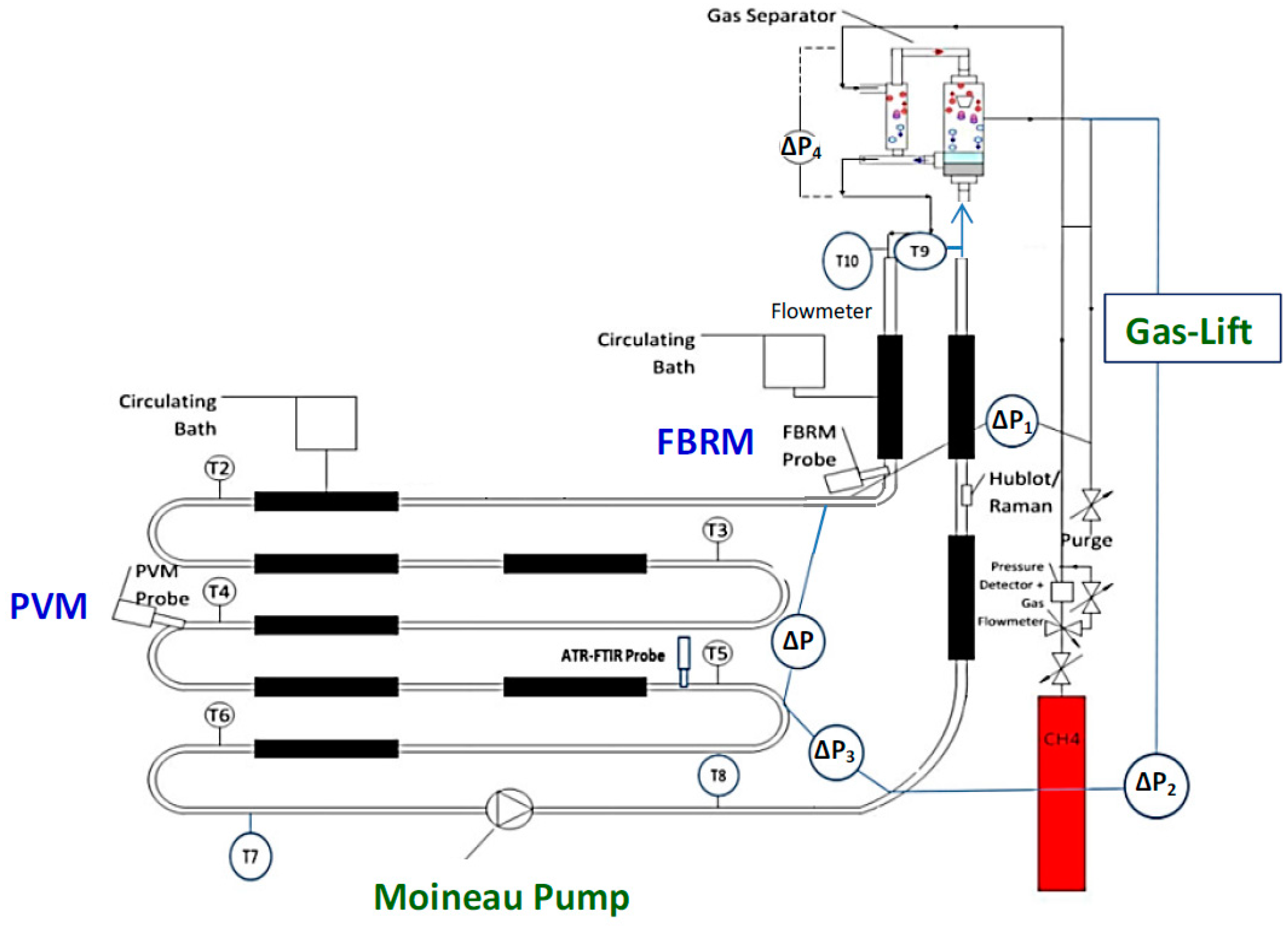
2.1. Apparatus
2.2. Materials
2.3. Experimental Procedure
2.4. Measurements and Calculations
3. Results and Discussion
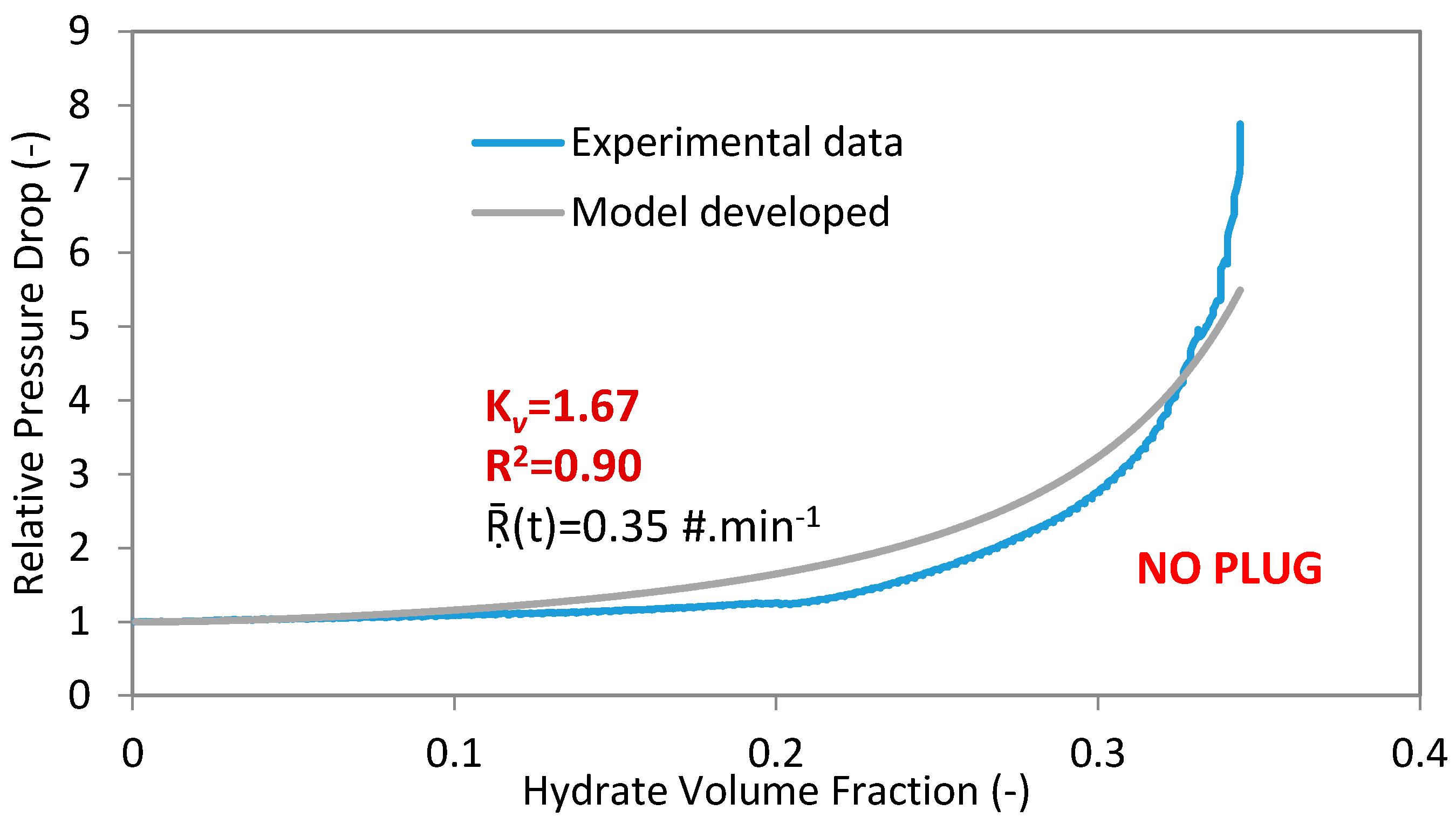
3.1. Relative Pressure Drop Models of Suspension
3.2. Relative Pressure Drop Model Developed
3.3. Homogeneous
3.4. Heterogeneous
- It is supposed that φmax is constant (0.74), regardless of flowrate, salt, and AA-LDHI presence. The fact is that φmax might be a function of shearing [21] depends on the presence of salt and/or AA-LDHI.
- In this study, it is supposed that Kv is constant. In fact, the Kv can change until hydrate particles reach a maximum packing volume fraction, φmax [21]. Kv might be dependent on crowding and shearing effects on the formation of agglomerates.
- In this model, the hydrate deposition phenomenon was not completely taken into account.
- The coefficient of multiple determinations (R2) is in the range of 0.76 to 0.98, and approaches 0.9 in the case hydrate agglomerates are homogeneously distributed in the flow (model results fit better with experimental data in case of homogeneous hydrate flow).
4. Conclusions
- At higher values of Kv, larger hydrate agglomerates were observed, with higher liquid quantities entrapped. The existence of these larger hydrate agglomerates caused a higher relative pressure drop and a more heterogeneous flow, with higher risk of hydrate plugging.
- At intermediary Kv, higher amounts of AA-LDHI led to a higher rate of crystallization (AA-LDHIs promoted hydrate formation) which promoted a heterogeneous hydrate flow. At low Kv, the use of AA-LDHI decreased the size of hydrate particles and agglomerates, which led to lower RPD (decrease in Kv), preventing plugging.
Author Contributions
Funding
Conflicts of Interest
Appendix A. Composition of Kerdane® Used
| Properties/Composition | Kerdane® |
|---|---|
| Chemical Composition | Hydrocarbons, C11–C14, n-alkanes, iso-alkanes, cyclic, <2% aromatics |
| Appearance | Clear light yellow |
| Boiling Point/Interval | 180–260 °C |
| Flash Point | >64 °C |
| Density | 790–825 kg·m−3 at 15 °C |
| Kinematic Viscosity | 2.1 mm2·s−1 at 25 °C |
References
- Sloan, E.; Koh, C.A. Clathrate Hydrates of Natural Gases, 3rd ed.; Taylor and Francis Group: Abingdon, UK, 2008. [Google Scholar]
- Wei, N.; Sun, W.; Meng, Y.; Zhao, J.; Kvamme, B.; Zhou, S.; Zhang, L.; Li, Q.; Zhang, Y.; Jiang, L.; et al. Hydrate Formation and Decomposition Regularities in Offshore Gas Reservoir Production Pipelines. Energies 2020, 13, 248. [Google Scholar] [CrossRef]
- Straume, E.O.; Kakitani, C.; Merino-Garcia, D.; Morales, R.E.M.; Sum, A.K. Experimental study of the formation and deposition of gas hydrates in non-emulsifying oil and condensate systems. Chem. Eng. Sci. 2016, 155, 111–126. [Google Scholar] [CrossRef]
- Dong, S.B.; Li, M.Z.; Firoozabadi, A. Effect of salt and water cuts on hydrate anti-agglomeration in a gas condensate system at high pressure. FUEL 2017, 210, 713–720. [Google Scholar] [CrossRef]
- Peng, B.Z.; Chen, J.; Sun, C.Y.; Dandekar, A.; Guo, S.H.; Liu, B.; Mu, L.; Yang, L.Y.; Li, W.Z.; Chen, G.J. Flow characteristics and morphology of hydrate slurry formed from (natural gas+diesel oil/condensate oil+water) system containing anti-agglomerant. Chem. Eng. Sci. 2012, 84, 333–344. [Google Scholar] [CrossRef]
- Camargo, R. Rheological Properties of Hydrate Suspensions in an Asphaltenic Crude Oil. In Proceedings of the 4th International Conference on Gas Hydrates, Yokohama Symposia, Yokohama, Japan, 19–23 May 2002; pp. 880–885. [Google Scholar]
- Colombel, E.; Gateau, P.; Barré, L.; Gruy, F.; Palermo, T. Discussion sur les mécanismes d’agglomération entre particules d’hydrate dans les émulsions eau dans huile. Oil Gas Sci. Technol. 2009, 64, 629–636. [Google Scholar] [CrossRef]
- Fidel-Dufour, A.; Gruy, F.; Herri, J.M. Rheology of methane hydrate slurries during their crystallization in a water in dodecane emulsion under flowing. Chem. Eng. Sci. 2006, 61, 505–515. [Google Scholar] [CrossRef]
- Palermo, T.; Arla, D.; Borregales, M.; Dalmazzone, C. Study of the agglomeration between hydrate particles in oil using differential scanning calorimetry (DSC). In Proceedings of the Fifth International Conference on Gas Hydrates, Trondheim, Norway, 12–16 June 2005. [Google Scholar]
- Leba, H.; Cameirao, A.; Herri, J.M.; Darbouret, M.; Peytavy, J.L.; Glénat, P. Chord length distributions measurements during crystallization and agglomeration of gas hydrate in a water-in-oil emulsion: Simulation and experimentation. Chem. Eng. Sci. 2010, 65, 1185–1200. [Google Scholar] [CrossRef]
- Pauchard, V.; Palermo, T.; Peytavy, J.L.; Mussumeci, A. Transformation of a Concentrated Oil/Water Emulsion into A Gel Due to Slight Hydrates Formation. In Proceedings of the 5th International Conference on Gas Hydrates, Trondheim, Norway, 12–16 June 2005. [Google Scholar]
- Austvik, T.; Li, X.; Gjertsen, L.H. Hydrate Plug Properties: Formation and Removal of Plugs. Ann. N. Y. Acad. Sci. 2000, 912, 294–303. [Google Scholar] [CrossRef]
- Turner, D.J.; Miller, K.T.; Dendy Sloan, E. Methane hydrate formation and an inward growing shell model in water-in-oil dispersions. Chem. Eng. Sci. 2009, 64, 3996–4004. [Google Scholar] [CrossRef]
- Glenat, P.; Pagezy, L.; Devoisselle, R.; Bourg, P.; Pere, M.; Melchuna, A. Rheological Study of Liquid Hydrates Slurries in Presence of Commercial Hydrates Dispersants (AA-LDHIs); ICGH: Denver, CO, USA, 2017. [Google Scholar]
- Melchuna, A.; Cameirão, A.; Herri, J.-M.; Glénat, P. Topological modeling of methane hydrate crystallization from low to high water cut emulsion systems. Fluid Phase Equilibria 2016, 413, 158–169. [Google Scholar] [CrossRef]
- Fidel-Dufour, A. Influence d’additifs anti-agglomérants sur l’agrégation et les propriétés de transport des hydrates de méthane dans des émulsions eau/dodécane. Ph.D. Thesis, Ecole des Mines de Saint-Etienne, Saint Etienne, France, 2004. [Google Scholar]
- Giavarini, C.; Hester, K. Gas Hydrates. Green Energy and Technology; Springer: London, UK, 2011. [Google Scholar]
- Midoux, N. Mécanique et Rhéologie des Fluides en Génie Chimique; Edition Lavoisier: Paris, France, 1993. [Google Scholar]
- Mills, P. Non-Newtonian behaviour of flocculated suspensions. J. Phys. Lett. 1985, 46, 301–309. [Google Scholar] [CrossRef]
- Moradpour, H.; Chapoy, A.; Tohidi, B. Bimodal model for predicting the emulsion-hydrate mixture viscosity in high water cut systems. Fuel 2011, 90, 3343–3351. [Google Scholar] [CrossRef]
- Quemada, D. Rheology of Concentrated Disperse Systems and Minimum Energy-Dissipation Principle 1. Viscosity-Concentration Relationship. Rheol. Acta 1977, 16, 82–94. [Google Scholar] [CrossRef]
- Krieger, I. Rheology of monodisperse latices. Adv. Colloid Interface Sci. 1972, 3, 111–136. [Google Scholar] [CrossRef]
- Hoekstra, L.L.; Vreeker, R.; Agterof, W.G.M. Aggregation of colloidal nickel hydroxycarbonate studied by light scattering. J. Colloid Interface Sci. 1992, 151, 17–25. [Google Scholar] [CrossRef]
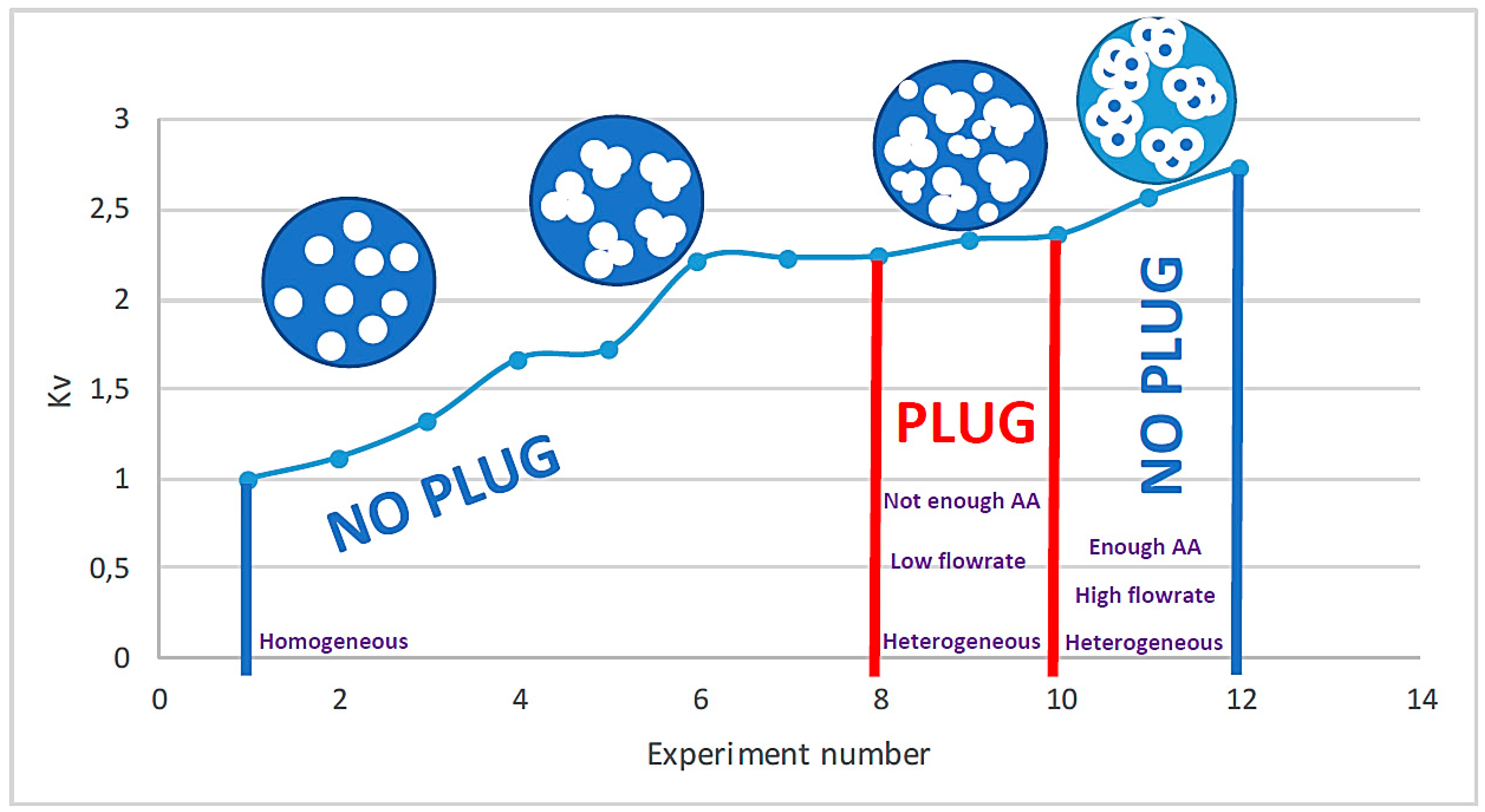
| Exp. | WC | Flowrate | Pressure | AA-LDHI | NaCl | Ṝ(t) | Structural Agglomerate Factor | Coefficient of Multiple Determination | PLUG | Hydrate Volume Fraction |
|---|---|---|---|---|---|---|---|---|---|---|
| No. | (%) | (L·h−1) | (Bar) | (wt.% of Water) | (g·L−1(H2O)) | (.min−1) | (Kv) | (R2) | - | (-) |
| 1 | 100 | 150 | 75 | 1 | 30 | 0.16 | 1 | 0.98 | NO | 0.51 |
| 2 | 80 | 150 | 75 | 1 | 30 | 0.1 | 1.12 | 0.76 | NO | 0.37 |
| 3 | 100 | 400 | 75 | 0.5 | 30 | 0.35 | 1.33 | 0.95 | NO | 0.44 |
| 4 | 100 | 400 | 75 | 1 | 30 | 0.35 | 1.67 | 0.9 | NO | 0.34 |
| 5 | 80 | 400 | 75 | 1 | 30 | 0.18 | 1.73 | 0.95 | NO | 0.38 |
| 6 | 80 | 400 | 75 | 1 | 0 | 0.72 | 2.22 | 0.83 | NO | 0.25 |
| 7 | 100 | 400 | 70 | 2 | 0 | 0.35 | 2.23 | 0.89 | NO | 0.27 |
| 8 | 100 | 150 | 75 | 1 | 0 | 0.47 | 2.24 | 0.9 | YES | 0.22 |
| 9 | 100 | 400 | 75 | 0 | 30 | 0.08 | 2.33 | 0.98 | YES | 0.23 |
| 10 | 100 | 400 | 75 | 0.5 | 0 | 0.43 | 2.36 | 0.92 | YES | 0.26 |
| 11 | 100 | 400 | 75 | 2 | 0 | 0.53 | 2.57 | 0.93 | NO | 0.23 |
| 12 | 100 | 400 | 75 | 1 | 0 | 0.81 | 2.74 | 0.78 | NO | 0.23 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pham, T.-K.; Cameirao, A.; Melchuna, A.; Herri, J.-M.; Glénat, P. Relative Pressure Drop Model for Hydrate Formation and Transportability in Flowlines in High Water Cut Systems. Energies 2020, 13, 686. https://doi.org/10.3390/en13030686
Pham T-K, Cameirao A, Melchuna A, Herri J-M, Glénat P. Relative Pressure Drop Model for Hydrate Formation and Transportability in Flowlines in High Water Cut Systems. Energies. 2020; 13(3):686. https://doi.org/10.3390/en13030686
Chicago/Turabian StylePham, Trung-Kien, Ana Cameirao, Aline Melchuna, Jean-Michel Herri, and Philippe Glénat. 2020. "Relative Pressure Drop Model for Hydrate Formation and Transportability in Flowlines in High Water Cut Systems" Energies 13, no. 3: 686. https://doi.org/10.3390/en13030686
APA StylePham, T.-K., Cameirao, A., Melchuna, A., Herri, J.-M., & Glénat, P. (2020). Relative Pressure Drop Model for Hydrate Formation and Transportability in Flowlines in High Water Cut Systems. Energies, 13(3), 686. https://doi.org/10.3390/en13030686




