CO₂ Convective Dissolution in Oil-Saturated Unconsolidated Porous Media at Reservoir Conditions

Abstract

During CO₂ storage, CO₂ plume mixes with the water and oil present at the reservoir, initiated by diffusion followed by a density gradient that leads to a convective flow. Studies are available where CO₂ convective mixing have been studied in water phase but limited in oil phase. This study was conducted to reach this gap, and experiments were conducted in a vertically packed 3-dimensional column with oil-saturated unconsolidated porous media at 100 bar and 50 °C (representative of reservoir pressure and temperature conditions). N-Decane and crude oil were used as oils, and glass beads as porous media. A bromothymol blue water solution-filled sapphire cell connected at the bottom of the column was used to monitor the CO₂ breakthrough. With the increase of the Rayleigh number, the CO₂ transport rate in n-decane was found to increase as a function of a second order polynomial. $Ra$ number vs. dimensionless time $\tau$ had a power relationship in the form of $Ra = c\times {\tau }^{-n}$. The overall pressure decay was faster in n-decane compared to crude oil for similar permeability (4 D), and the crude oil had a breakthrough time three times slower than in n-decane. The results were compared with similar experiments that have been carried out using water.

1. Introduction

CO₂ storage is a commonly considered topic when it comes to climate change mitigation. Injection of CO₂ to active and abandoned oil and gas fields is a well-discovered solution for a viable utilization of CO₂ due to its commercial benefits of enhancing the oil recovery (EOR) as well as achieving permanent CO₂ storage [1,2]. During CO₂ injection into existing oil fields for EOR, the added CO₂ will swell and reduce the viscosity and will lead to an increase of the oil recovery percentage [3,4]. EOR for CO₂ utilization can also reduce a significant cost of the whole CCS value chain [5,6,7].

When CO₂ is injected into the oil fields, a CO₂ plume will usually develop above the fluid phases inside the porous media due to the low density of CO₂ compared to the density of the reservoir fluids, as shown in Figure 1 [8]. Initially, this CO₂ plume mixes with the oil and water phases present in the reservoir mainly due to diffusion. The mixing process creates a density gradient (e.g., increase the density of oil). This phenomenon leads to a convective flow, which will accelerate the CO₂ mixing and mass transfer and will significantly enhance the underground CO₂ storage rate as well as the oil production [7,9,10,11].

It is essential to know the behavior of the CO₂ plume in the reservoir along with how CO₂ will dissolve convectively into the oil phase. This helps to understand how CO₂ will be transported during long-term storage after injection for storage and EOR. The convectively driven dissolution has been extensively studied for accelerated CO₂ dissolution in saline water for CO₂ storage in 2-dimensional (2-dim) Hele-Shaw experimental setups [12,13,14,15,16,17,18] and using 3-dimensional (3-dim) confined experimental setups [19,20,21,22,23,24]. An extensive review on available CO₂ convective mixing experiments in water is available in Amarasinghe et al. [12].

However, similar studies with the presence of oil (or residual oil) are still very limited. This represents a gap in defining and validating the adequate mathematical models and upscaling procedures for CO₂ storage and EOR, and the lack of input parameters for uncertainty estimation. In the literature, Amarasinghe et al. [25] and Khosrokhavar et al. [16] have conducted CO₂ convective dissolution visualization experiments into the oil phase inside a Hele-Shaw cell using the Schlieren visualization method. Farajzadeh et al. [26] performed a few PVT experiments using n-decane and n-hexadecane to investigate the CO₂ mass transfer at gaseous conditions. They concluded that CO₂ mass transfer increases in n-decane with the increase of pressure, while mass transfer in n-hexadecane is slower compared to n-decane. Zhao et al. [21] investigated and visualized CO₂ flooding in porous media (bead pack) inside a vertical high-pressure PVT cell. They monitored CO₂ front movement, piston-like miscible regions, CO₂ channeling, and fingering using MRI technology for both supercritical and gaseous miscible conditions in n-decane. Meanwhile, Seyyedsar and Sohrabi [27] visually investigated the formation of a new oil phase during immiscible CO₂ injection into heavy oil-saturated porous media under reservoir conditions. Wei et al. [28] carried out a visualization study on oil swelling due to CO₂ miscibility inside a high-pressure cylindrical cell under reservoir conditions. In terms of simulations, Gasda and Elenius [11], Gasda et al. [29], Both et al. [30], Ahmed et al. [31], and Rongy et al. [32] have conducted CO₂ gravity-driven convective mixing in oil. They showed the CO₂ interaction with single component oil types and phase behavior including gravity convection fingering.

Furthermore, it is important to know the CO₂ transport rate through an oil-saturated porous media to obtain a better understanding of real geological CO₂ storage. It will provide a better indication of the behavior of CO₂ plume and location of CO₂ front at a given time. The results also can be further used to develop and validate mathematical models in order to upscale towards the whole reservoir. In the literature, such an experimental study has not been found. The same authors conducted an experimental study to investigate CO₂ convective dissolution and breakthrough time in water-saturated unconsolidated porous media [24]. The objective of the presented work was to investigate CO₂ convective dissolution in oil-saturated unconsolidated porous media of different permeabilities at realistic reservoir conditions. In this study, we only focused on pressure and temperature with relation to reservoir conditions. In terms of other reservoir properties such as usage of actual reservoir rock and three-phase systems, we have not addressed them in this study. This study reduces the gap of experimental results of CO₂ convective mixing in oil, which will lead to a better understanding of the process in reservoirs.

2. Experimental Method

2.1. Materials

Hydrophilic glass beads of different diameters were used to prepare porous media of different permeabilities (0.5 D, 4 D, 16 D, and 76 D). The permeability of the bead packs was determined by the waterflooding of packed glass bead tubes. The particle size distribution of each glass bead type is shown in Figure 2. n-Decane and a North Sea crude oil (see

Table 1. Crude oil composition.

Component	mol %
C5	2
C6	3
C7	5
C8	5
C9	6
C10	4
C11	4
C12	4
C13	4
C14	3
C15	3
C15+	57
Total	100

for the composition) were used as oils. Bromothymol blue (BTB) pH indicator solution (0.004 wt % BTB with 0.01M NaOH prepared in deionized water) of pH around 8 was used as the water phase. The BTB solution changes color from blue to yellow when the pH changes due to CO₂ mixing.

2.2. Experimental Setup

A steel cell with an inner height of 27.5 cm and an inner diameter of 7.75 cm (approximately 1.3 L of volume) was used to carry out CO₂ mixing experiments in oil-saturated porous media. The steel cell was vertically placed and was connected to water and oil-filled sapphire cell at the bottom (see in Figure 3, Figure 4a, Figure 5 and Figure 6a). The end piece at the bottom of the steel cell had a single hole that connected the steel cell and the sapphire cell (see Figure 4b). A Spectrum Spectra Mesh woven filter (Supplier: Spectrum Laboratories, USA) was added at the bottom of the cell to prevent glass beads penetrating the sapphire cell (see Figure 3). The sapphire cell was connected to a back-pressure regulator set at 100 bar. A piston cell filled with CO₂ was used as the CO₂ source and another piston cell was filled with the type of oil that was being used. A Quizix pump (Supplier: Chandler Engineering, USA) was used to control injection and pressure monitoring. A simplified sketch of the whole experimental setup is given in Figure 5, including the main valves that are used in following text to describe the experimental procedure. All the experiments were carried out at 50 °C using the pressure decay method starting from 100 bar.

2.3. Experimental Procedure

1.

The steel column was wet packed with glass beads manually (filled the column with oil type first and dry glass beads into oil), with the specified size to a height of 18 cm, and filled the rest of the volume to the top with the oil type that was being used.

2.

After mounting all the devices, V1, V3, and V5 were opened and the oil was pumped through the packed column using the Quizix pump and pressurized to 100 bar until produced through the back-pressure regulator to make sure 100% oil saturation was obtained.

3.

The pump was stopped and waterside from the CO₂ piston cell (V2) was opened while V1, V3, and V5 were kept opened.

4.

Then, the system was heated to 50°. With the temperature increase, CO₂ inside the CO₂ piston cell expanded. Hence, water from the CO₂ piston cell was transferred to the oil piston cell where pressurized oil was transferred through the packed column via the back-pressure regulator. This way, it was made sure that packed column pressure and the CO₂ piston cell pressure stayed the same.

5.

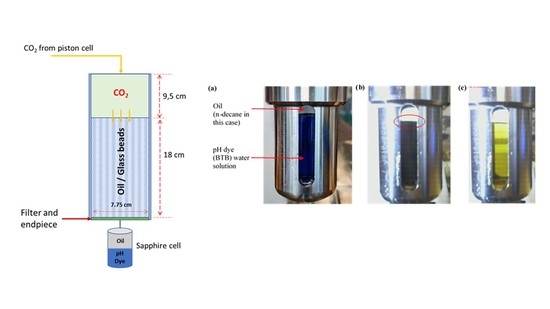
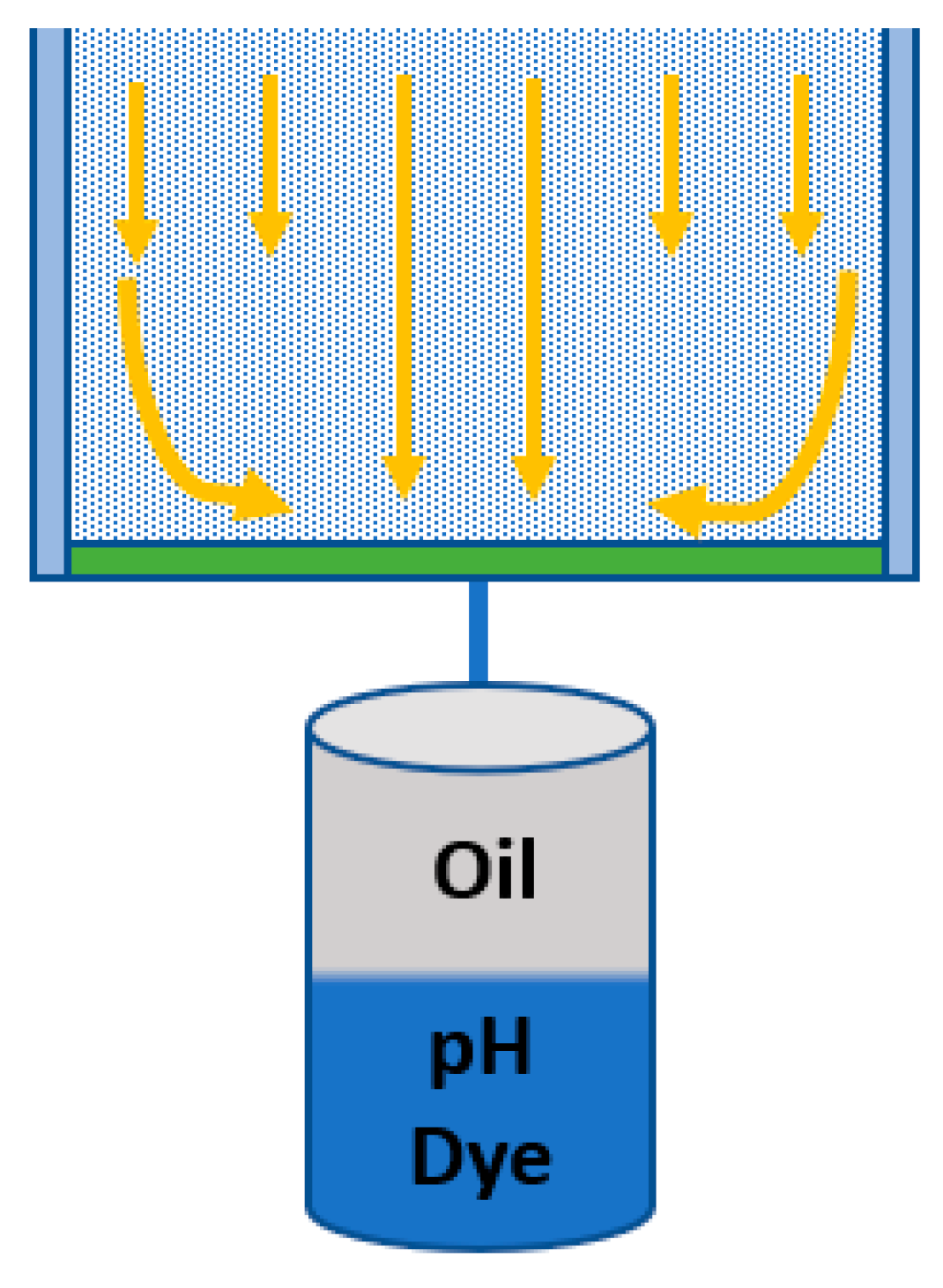
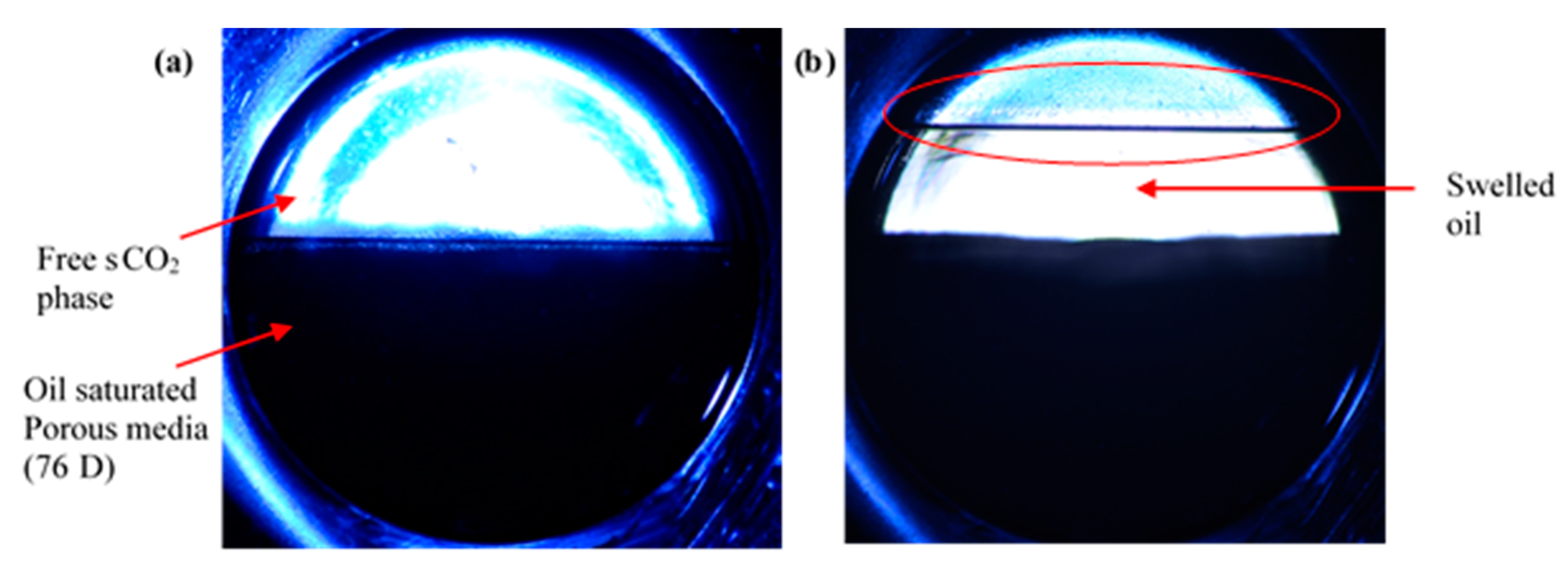
Afterward, V1 and V3 were closed. CO₂ piston cell was introduced to the packed column by opening V4. A specified amount of CO₂ (450 mL) was injected at a high rate (50 mL/min) and out through the back-pressure regulator (V5 was still opened) to create a 9.5 cm height of free phase of CO₂ on top of the oil-saturated porous media (as shown in Figure 3). With previous experience, it was calculated that a height of 9.5 cm was required to compensate for the oil swelling so that swelled oil due to CO₂ mixing was not transported into the CO₂ piston cell.

6.

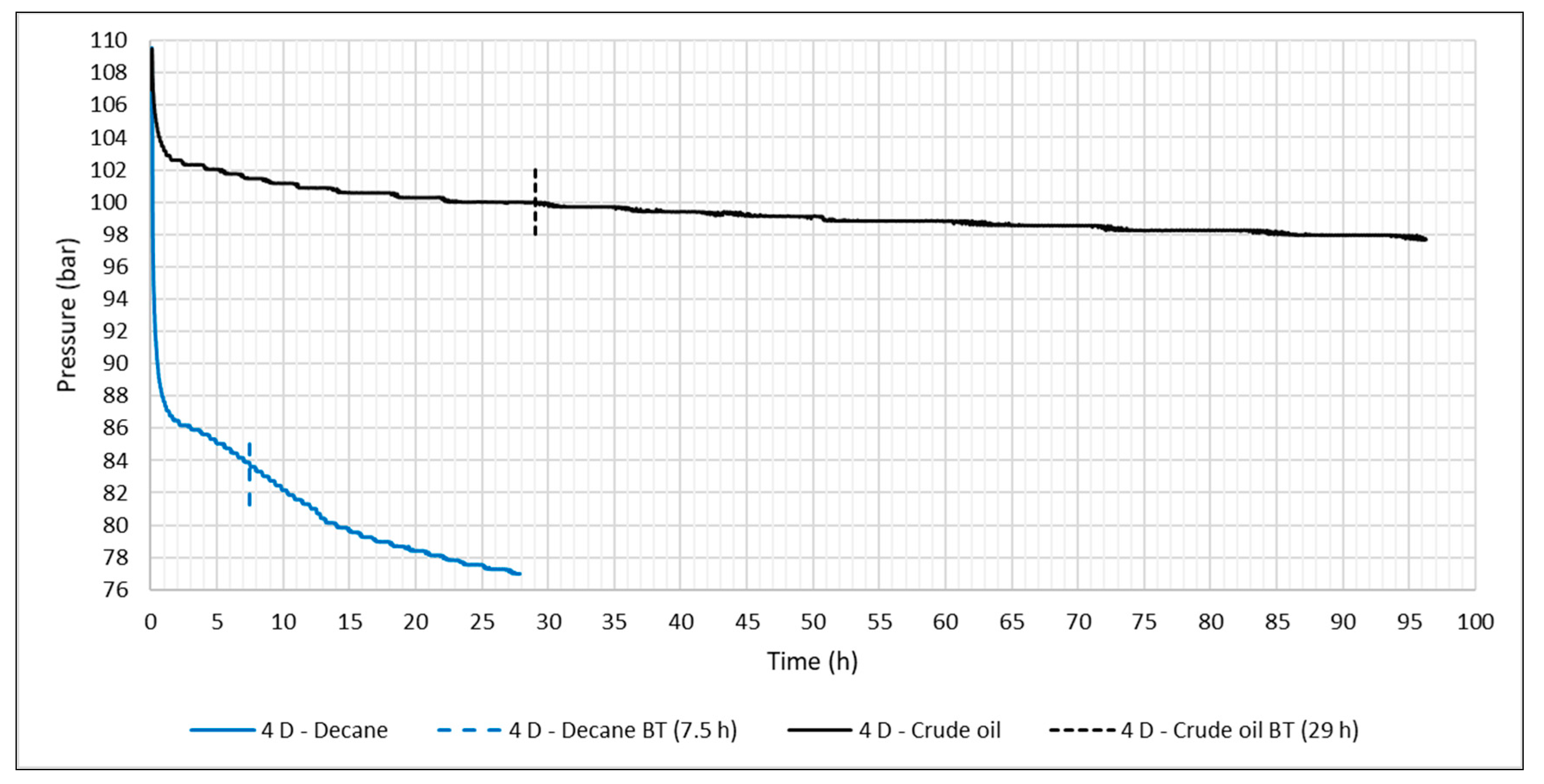
The connection between the packed column and back-pressure regulator (V5) was closed after the injection and the pump was stopped and pressure decay data were logged using the computer application.

7.

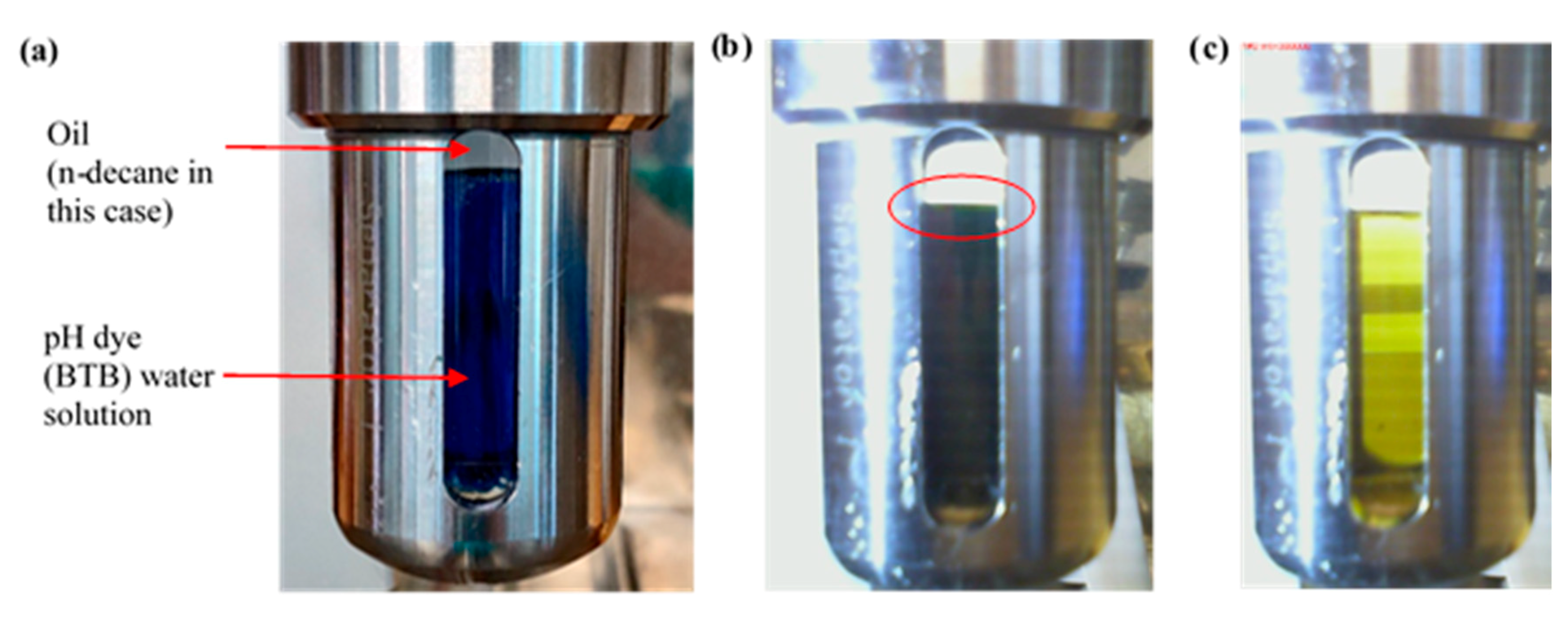
A small web camera with an interval timer shooting was used to monitor the breakthrough of CO₂ into the sapphire cell. CO₂ was transported through the oil-saturated porous media and a breakthrough was observed through the color change of water solution from blue to yellow (see Figure 6b).

2.4. Set of Experiments

The set of experiments carried out is shown in

Table 2. Set of experimental cases with CO₂/oil at 100 bar and 50 °C, and average breakthrough time and average CO₂ transport speed.

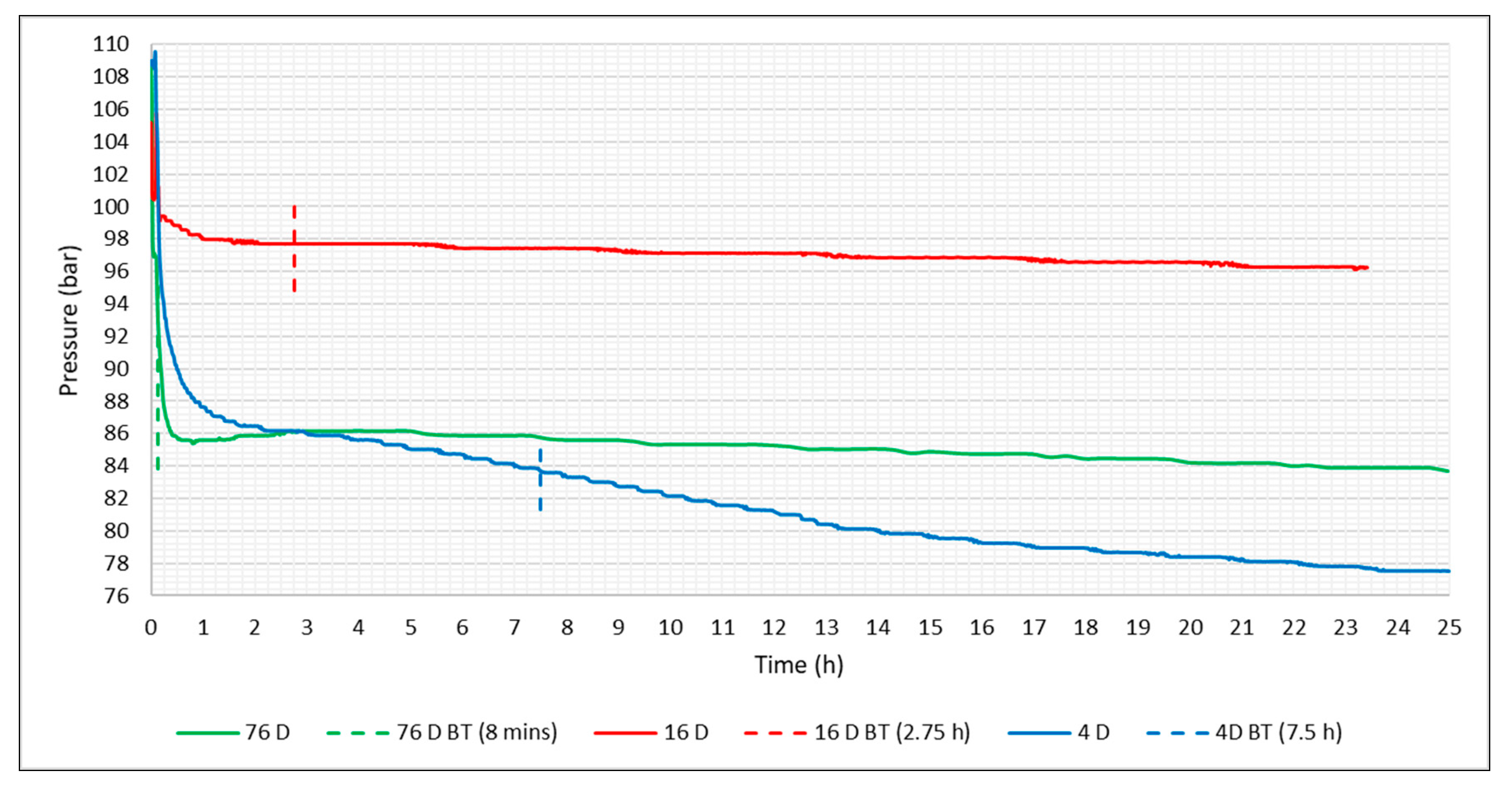
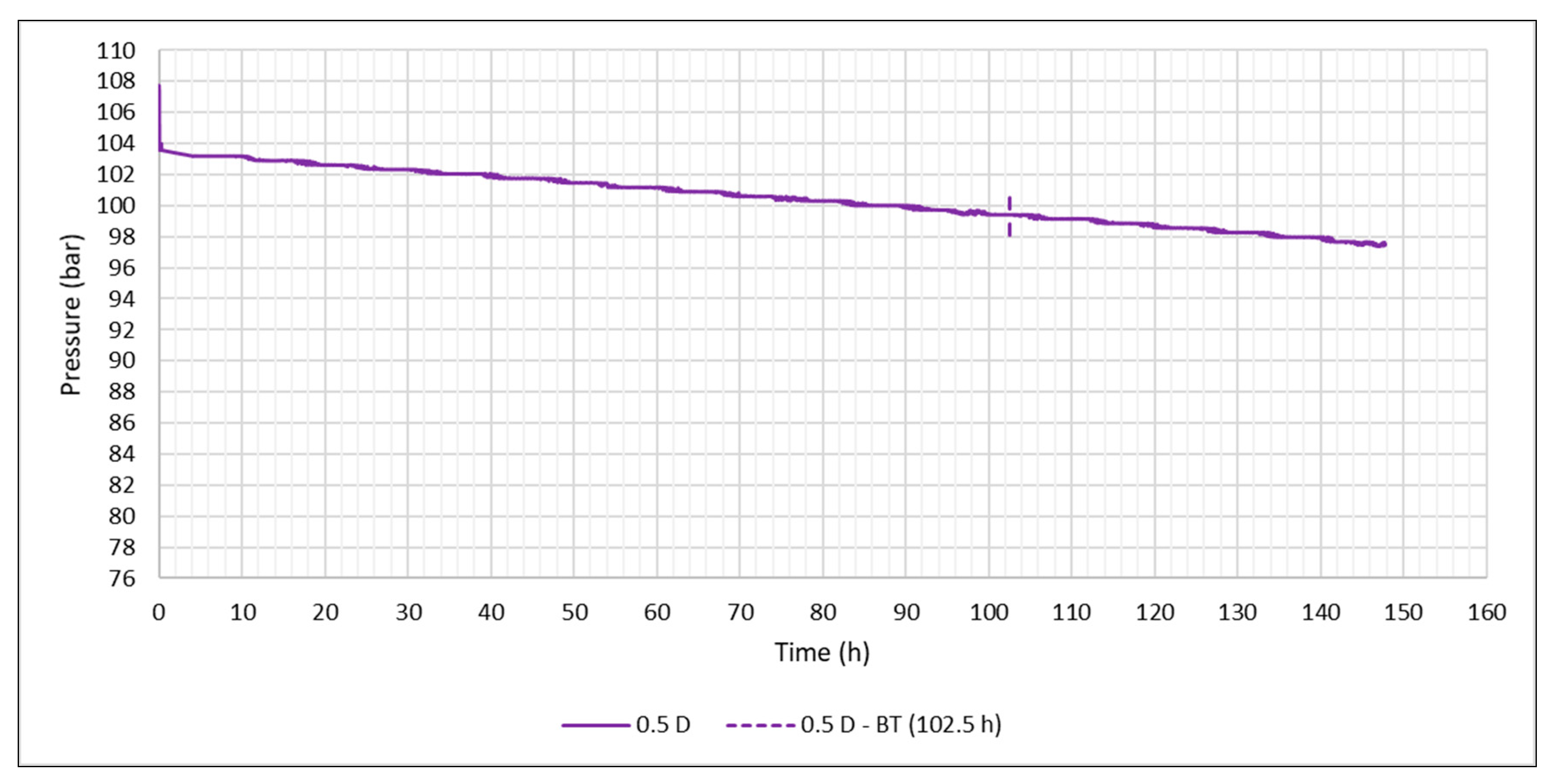
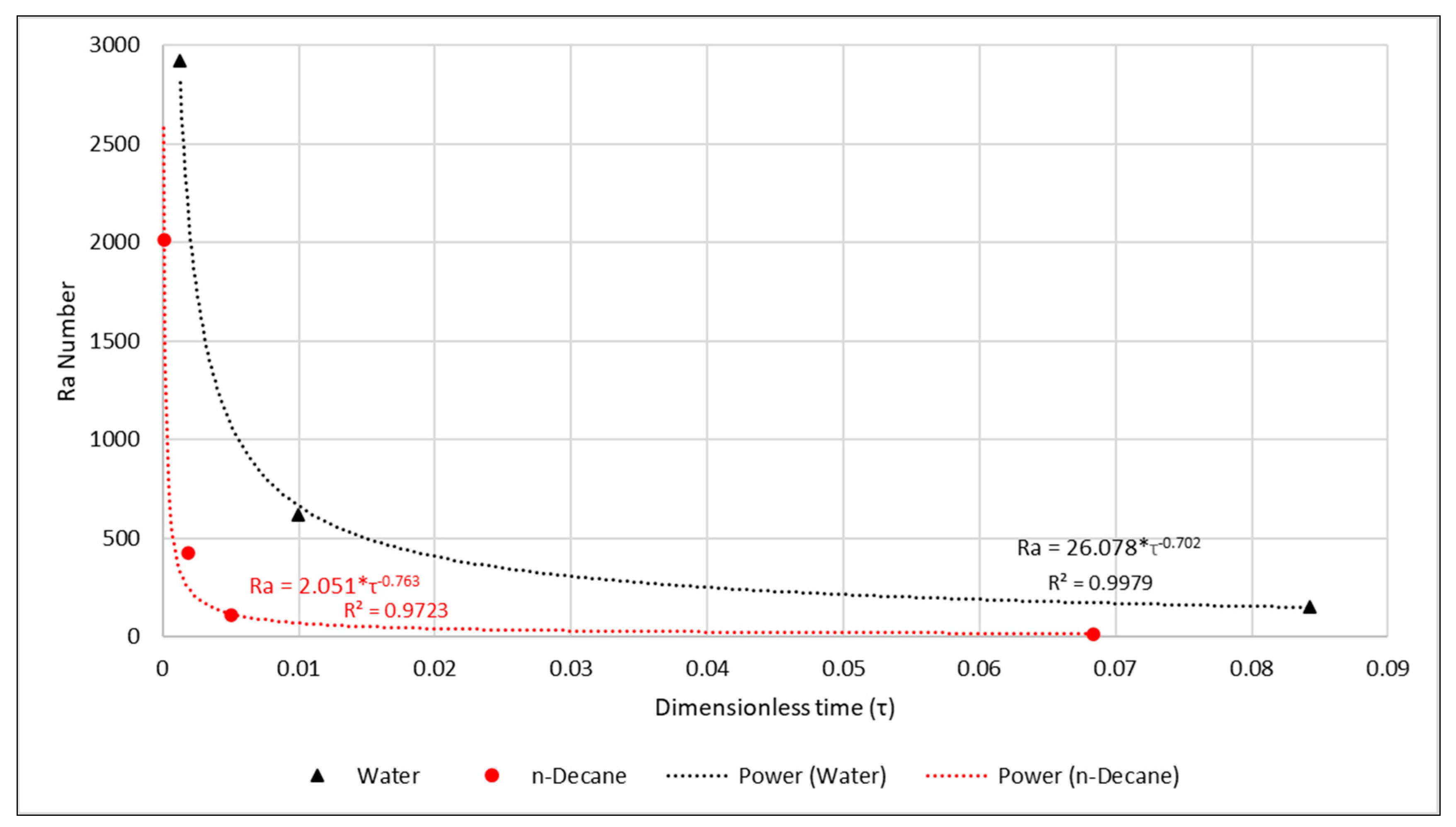
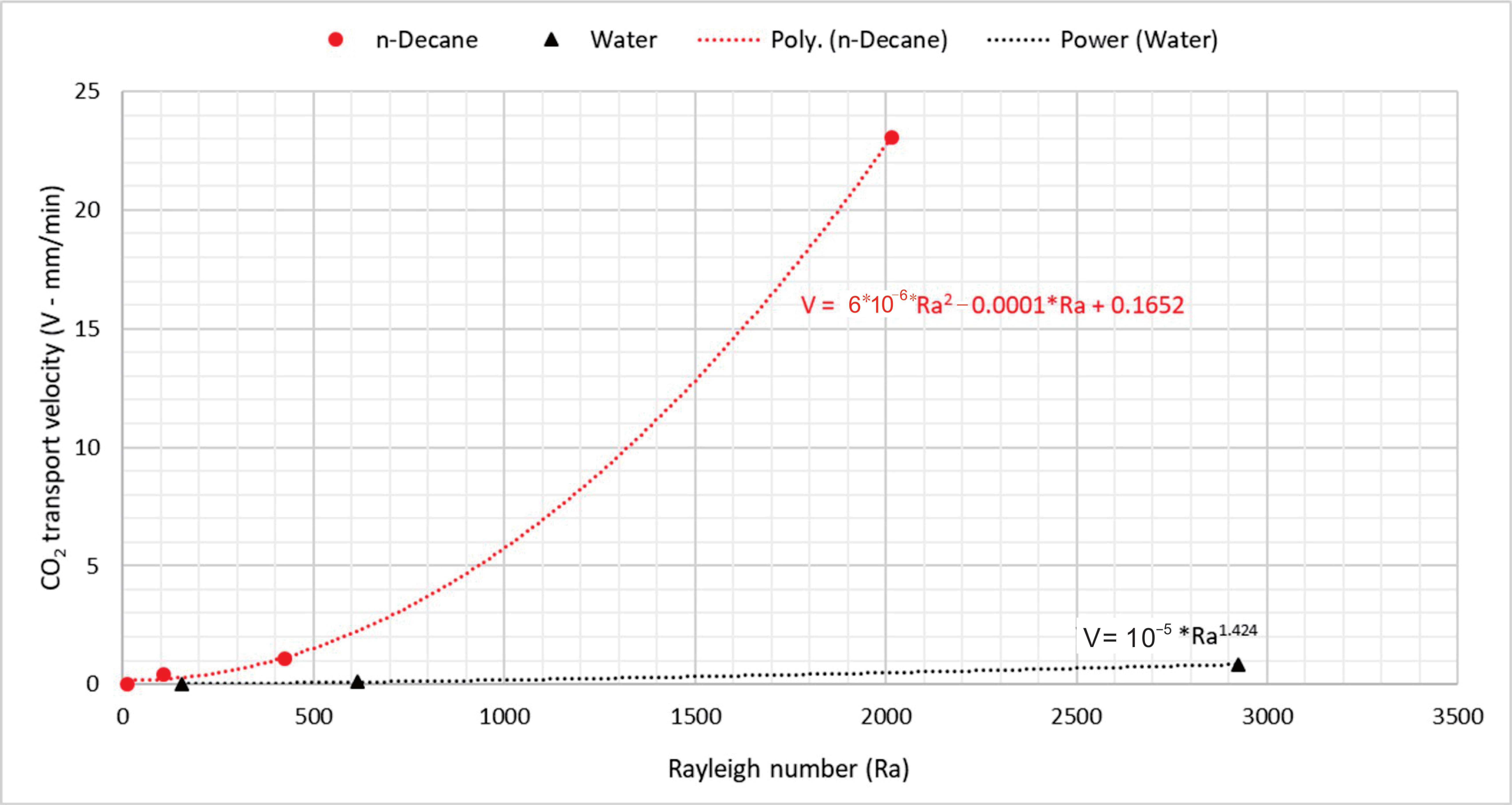
Test No.	Glass Beads (µm)	Oil Type	Estimated Permeability (D) *	Rayleigh Number (Ra)	Average Breakthrough Time	Average CO2 Transport Speed (V—mm/min)
1	400–600	n-decane	76	2015	8 min	23.08
2	100–200	n-decane	16	424	2.75 h	1.09
3 (2 repeats)	70–110	n-decane	4	106	7.5 h	0.11
4	0–50	n-decane	0.5	13	102.5 h	0.03
5	70–110	crude oil	4	not calculated	29 h	0.1

* Determined in waterflooding of packed tubes.

, together with the results of average breakthrough times and average CO₂ transport speed. Rayleigh number $\left(Ra\right)$ was calculated using the equation $Ra=\left(\mathrm{∆}\rho gkH\right)/\left(\mu D\Phi \right),$ where ∆$\rho$ is the density increase of oil due to CO₂ dissolution, $g$ is the acceleration of gravity, $k$ is the permeability of the porous media, $H$ is the height of porous media, $\mu$ is the dynamic viscosity of the oil, $D$ is the molecular diffusion coefficient of CO₂ in oil, and $\Phi$ is the porosity of porous media. The height of porous media was 18 cm, while the other parameter values used to calculate the $Ra$ number are given in

Table 3. Parameters for Ra number calculation.

Parameter	Value **		Units
${\rho }_{{CO}_2}$	384.67		kg/m3
$H$	0.18		m
$g$	9.81		m/s2
$\mathsf{\Phi }$	0.4		-
	n-Decane	Crude Oil
${\rho }_{oil}$	730	913	kg/m3
${\rho }_{\left(oil+{CO}_2\right)mix }$	755.2 [33]	N/A	kg/m3
$\mathrm{∆}\rho$	25.2	N/A	kg/m3
$D$	$6\times$10−9 [34]	N/A	m2/s
$\mu$	$6.9\times$10−4 [35]	0.045	kg/s·m

** Obtained at 100 bar/50 °C.

. $Ra$ number was calculated only for experiments with n-decane due to the unavailability of ${\rho }_{\left(oil+{CO}_2\right)mix }$ value and diffusion co-efficient of CO₂ in crude oil value for crude oil.

3. Results and Discussions

The pressure decay data and the breakthrough times for 76 D (test 1), 16 D (test 2), and 4 D (test 3) are presented in Figure 7. The pressure decay data and the breakthrough time for the 0.5 D (test 4) is presented in Figure 8. In Figure 9 shows pressure decay data and the breakthrough times comparison for the experiments with 4 D permeability with n-decane (test 3) and crude oil (test 5).

The CO₂ breakthrough time in n-decane-saturated 76 D porous media was very quick (8 min), which indicates that CO₂ was mixing with oil instantly [25]. The breakthrough time was found to increase with the decrease of permeability (see Table 2). Due to the high miscibility of CO₂ in oil, the pressure decreased rapidly at the beginning and was then gradually reduced. With the reduction of permeability, the initial pressure decay rate was reduced (see Figure 7). In 0.5 D porous media, a significant initial instant pressure decay was not observed. This was due to the low permeability, which led to a $Ra$ number ($Ra$ = 13) lower than the $R{a}_{critical}$ value of $4{\pi }^2$. The theory says that if the $Ra\le R{a}_{critical}$, then the flow is diffusion-dominant (i.e., natural convection is insignificant) [15,36].

For the crude oil, the initial pressure decay rate was slower than for n-decane in 4 D porous media. Moreover, the pressure decay rate was higher in n-decane compared to crude oil. The breakthrough time for n-decane-saturated porous media with 4 D permeability (7.5 h) was three times faster than for crude oil-saturated porous media with the same permeability (29 h). The overall pressure decay was also higher in n-decane than in crude oil, which indicated more CO₂ was mixed in n-decane compared to in crude oil (see Figure 9). Generally, CO₂ diffusion co-efficient in crude oil is lower than n-decane due to its presence of heavy carbon numbers (see Table 1) [34]. Hence, lower transport rate of CO₂ in crude oil can be expected in comparison to n-decane.

Due to the CO₂ mixing process inside the 3-dim porous media being random and fingering occurring randomly, the location of the CO₂ front fingers at the bottom varies [37]. Especially when CO₂ reached the bottom along the boundary, CO₂ had to be transported to the connection of sapphire cell along the bottom surface (see Figure 10). Since the sapphire cell was connected to the bottom end piece from its center (see Figure 4), different breakthrough times were reasonable.

After breakthrough of CO₂ produced a slight color change of the water solution in the sapphire cell, it took several minutes to change the color of the water solution completely from blue to yellow (see Figure 6c). This indicates that even after the breakthrough, CO₂ was still invading the sapphire cell and still CO₂ convection took place inside the porous media. From the pressure data (as in Figure 7, Figure 8 and Figure 9), the pressure was still decaying after the observation of the CO₂ breakthrough.

For scaling purposes of the 3-dim experiments, we have used dimensionless time ($\tau$), $\tau = \left(D/H^2\right)\times t_s$, where $t_s$ is considered as the breakthrough time. The relationship between τ and $Ra$ number was compared for n-decane (this study) and water [24] (see Figure 11). We found that the $Ra$ number vs. $\tau$ had a power relationship in the form of $Ra=c\times {\tau }^{-n}$, with constants $c=2.051$ and $n=0.763$ for n-decane and $c=26.078$ and $n=0.702$ for water. A similar power trend has been found by Faisal et al. [15] and Farajzadeh et al. [38] in their study of the water phase.

The CO₂ transport rate in oil was observed to increase with increasing permeability. Comparing the results with similar experiments carried out by Amarasinghe et al. [24] using water, we observed that the CO₂ transport rate was generally lower in water than in n-decane. Density increase in water and oil due to CO₂ mixing are 14.75 kg/m³ [39,40] and 25.2 kg/m³ [33], respectively. Hence, faster CO₂ mixing in n-decane compared to water can be justified. With increasing $Ra$ number, the increase of the CO₂ transport rate (V) increased as a function of power $\left(\mathrm{V} = 1\times {10}^{-5}\times R{a}^{1.424}\right)$ in water and as a function of a second order polynomial $\left(\mathrm{V} = 6\times {10}^{-6}\times R{a}^2-0.0001\times Ra+0.1652\right)$ for n-decane (see Figure 12 for the relationships between $Ra$ number and CO₂ transport rate in both water and n-decane).

In this kind of experiment, measurement/calculation of CO₂ mass transferred into the oil phase would be significant data. Due to the swelling of the oil phase, the CO₂–oil boundary inside the vertical column moves upwards, as observed by Amarasinghe et al. [25] in their 2-dim Hele-Shaw experiments (see Figure 13). There is a disturbance to the CO₂–oil interface during CO₂ injection to generate a free volume of CO₂ on top of the porous media. This may affect the breakthrough time. However, we neglected the effects for the observation of breakthrough time and the calculation of CO₂ transport speed through the porous media. In the 3-dim experiments, due to boundary effects, slight heterogeneities within the porous pack, contact area CO₂, and porous media, internal fingering merging does add substantial complication to the fingering phenomenon compared to 2-dim experiments [9,25,41].

The scaled experimental data forms a basis for the fine-tuning of the existing mathematical model and scaling-up [11,42]. As further work, we suggest carrying out more experiments in more different oil types (e.g., mixture of oil and different crude oil types with known compositions) using a wider range of permeabilities to gather more data.

4. Conclusions

We experimentally investigated CO₂ convective mixing inside an oil-saturated porous media at realistic reservoir pressure and temperature conditions (100 bar and 50 °C). CO₂ breakthrough time was quantitatively measured with porous media of different permeabilities. It was found that $Ra$ number vs. dimensionless time τ had a relationship in the form of $Ra =c\times {\tau }^{-n}$. In crude oil, the initial pressure decay rate was lower than for n-decane inside 4 D porous media. The overall pressure decay also was higher in n-decane than in crude oil for similar permeability (4 D), and crude oil had a breakthrough time that was three times slower than in n-decane. The results also were compared with similar experiments carried out by the same authors using water. It was shown that CO₂ transport rate was generally lower in water compared to n-decane due to the lower density increase of the fluid mixture. With the increase of $Ra$ number, the increase of the CO₂ transport rate increased as a form of power of $V = 1\times {10}^{-5}\times R{a}^{1.424}$ in water and as a function of a second order polynomial for n-decane. It was concluded that due to geometry, boundary effects, slight heterogeneities within the porous pack, the contact area between CO₂—porous media are responsible for the different results for 2-dim and 3-dim experiments. The scaled experimental data formed a basis for the validation of the existing mathematical model and scaling-up to further understanding of CO₂ geological storage processes and plume behavior.