Sliding Mode Control with Minimization of the Regulation Time in the Presence of Control Signal and Velocity Constraints
Abstract
:1. Introduction
2. Sliding Mode Controller Design
- When the magnitude of the control signal is constrained;
- When the magnitude of the system’s velocity is constrained;
- When the magnitude of the control signal and the absolute value of the system’s velocity are both constrained.
3. Optimization of the Controller Parameters
- The sliding line moves, then it stops and stays stationary until the control process finishes;
- The sliding line moves during the entire control process.
- In this case, we obtain:
- The left-hand side limit of in is equal to and the right-hand side limit is Hence:
- By equating one-sided limits at , we obtain:From the above equation, we obtain the formula describing the regulation time, which is our quality index:
3.1. Control Signal Constraint
- Using Equation (4), one can observe that function c is constant This means that its derivative with respect to time is always equal to Hence, we can rewrite formula (26) as follows:After calculations, we obtain the following inequality for :for which the magnitude of the input is constrained from above by the parameter However, the constraint depends on the parameter Therefore, in order to determine the admissible set of parameters and , we have to consider the situation when the state is on the moving sliding line.
- Again, using Equation (4), we obtain Calculating the derivative, we obtain In this case, inequality (26) can be rewritten asUsing Theorem 1 and the fact that the state converges to the demand state on the sliding line, one can deduce that the first state variable is monotonically increasing or monotonically decreasing, depending on the initial point. Hence:
3.2. System’s Velocity Constraint
- Convergence along the moving switching curve.In this case, our goal is to constrain the system’s velocity given by Equation (22). If the state converges to the desired point along the moving switching curve, then the admissible set will consist of only one constraint, as in this case . The first step will be deriving the moment in which the velocity reaches its extreme value. Now, the derivative of the first equation in (22) will be calculated and equated to zero, obtaining:Solving this equation for t and taking into account that it has to be positive, we obtain:Substituting (37) into the first equation in (22), one can obtain that the maximum absolute value of the system’s velocity is given byTherefore, in order to limit the magnitude of the system’s velocity by , inequality:has to be satisfied. Hence, the admissible set for the case, when the demand state is reached along the moving switching curve, is given as
- Convergence along the fixed switching curve.In this case, the representative point reaches the demand state at time Let us notice that taking into account the time interval and the shape of the switching curve, one can conclude that the maximum absolute value of the system’s velocity is reached at the time Therefore, we have yet to consider the case when In this scenario, the maximum absolute value of the system’s velocity will be reached at or given by (37). Now, let us consider two cases:
- In this case, the maximum of the absolute value of the system’s velocity is reached at Let us notice that first formulas in (20) and (22) are the same. Hence, following a similar procedure as in the first scenario, we obtain that the absolute value of the system’s velocity is attained at the moment:and similarly, as in the previous scenario, the admissible set is given by (40).
3.3. Control Signal and System’s Velocity Constraints
3.4. Settling Time Minimization
- When the magnitude of the control signal is constrained;
- When the magnitude of the system’s velocity is constrained;
- When the magnitude of the control signal and the absolute value of the system’s velocity are both constrained.
3.4.1. Settling Time Minimization with the Control Signal Constraint
- In this case, the regulation time is given by Equation (18). In order to determine its minimum value, we will consider as a function of and At first, let us calculate the partial derivative of with respect to :Taking into account the fact that and are positive, we conclude that the right hand side of (46) is always negative. Therefore, the regulation time is a decreasing function with respect to and its minimum value is obtained on the edge of the admissible set. Let us consider the maximum admissible value of which is equal to:In order to calculate the minimum value of the regulation time, we obtain the derivative of the function (48) with respect to as follows:Equating the above formula to zero, taking into account that and solving this equation, we obtain that the only stationary point is:We have to examine whether the above point belongs to the admissible set. Substituting value (47) into the inequality (28), we obtain:Hence, the value (50) does not belong to the admissible set. Therefore, the minimum value of the regulation time is obtained for:and is equal to:Now, let us consider the second edge of the admissible set, i.e., the line given asCalculating the derivative of the above function with respect to :we obtain that it is always negative; thus, is a decreasing function and its smallest value is obtained for the maximum admissible Therefore, again, the minimum of the regulation time is given as (53) and the optimal sliding line parameters are obtained for and as in Equations (52).
- In this case, the state reaches the desired point along the non-stationary switching curve and the regulation time is given by Equation (23). Calculating the derivative of this function with respect to , we obtain:This value is always negative. Hence, again the minimum regulation time will be obtained for the maximum admissible value of From inequality (34), we obtain that:Substituting the above value into Equation (23), we obtain the minimum regulation time:
3.4.2. Settling Time Minimization with the Velocity Constraint
3.4.3. Settling Time Minimization with Both Control Signal and Velocity Constraints
- The first step will be to determine the intersection point of lines describing the maximum admissible Equating the right-hand sides of inequality (28) and Equation (41), we find that these lines cross for:Using inequality (33), we can conclude that the above does not belong to the admissible set. Therefore, one of the functions in the admissible set is always greater than the other one. Let us observe that if increases, then the function:decays to zero faster than:Hence, in the admissible set, value (70) is always greater than value (71). Selecting the maximum admissible value of and then of , we conclude that the limitation of the system’s velocity will be fulfilled without any excess. In order to calculate the minimum value of the regulation time, we will analyze the edge of the admissible set described by the line:Substituting the above into Equation (18) and calculating its derivative with respect to , one obtains:Equating the right-hand side of formula (73) to 0, we obtain that the minimized regulation time is obtained for:Moreover, in order to constrain the control signal for the whole control process, we have to take into account that must satisfy condition (51). Let us observe that the right-hand side of the inequality (51) is smaller than the left-hand side in the maximum function of formula (74). Moreover, we have to consider the limitation given by inequality (28). Therefore, the minimum of the regulation time is obtained for:Let us derive the minimum value of the regulation time. If:then:however, if:then:or:We will substitute proper values of and into Equation (18):
- and In this case:
- and . Then:
- and In this case:
- .In this case, the minimum of the regulation time will again be obtained for the maximum value of parameter In order to constrain both the input and system’s velocity, both inequalities (34) and (40) must hold. We have to select the more restrictive constraint. Hence:Substituting the above value into Equation (23), we obtain the minimum value of the regulation time:
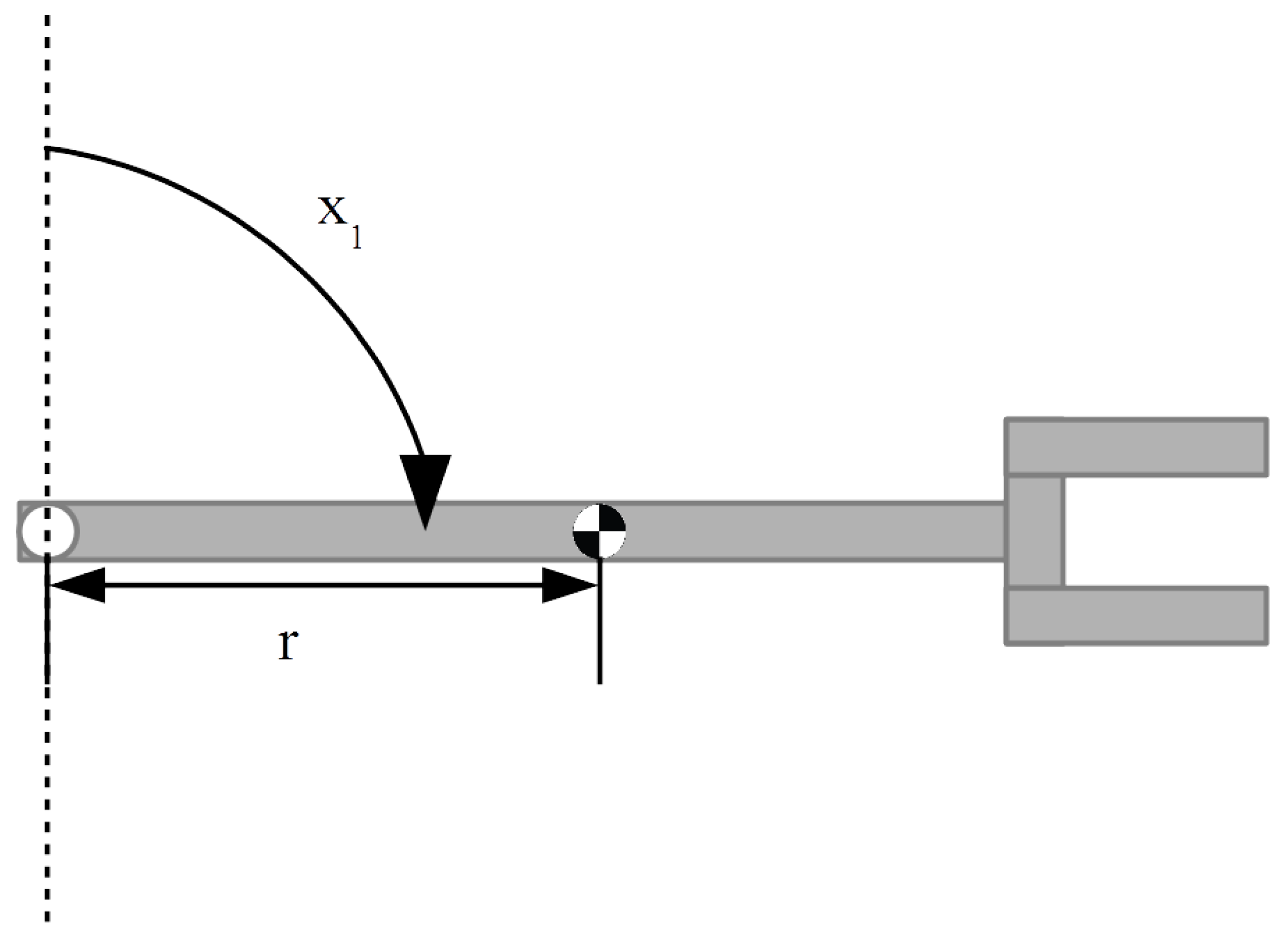
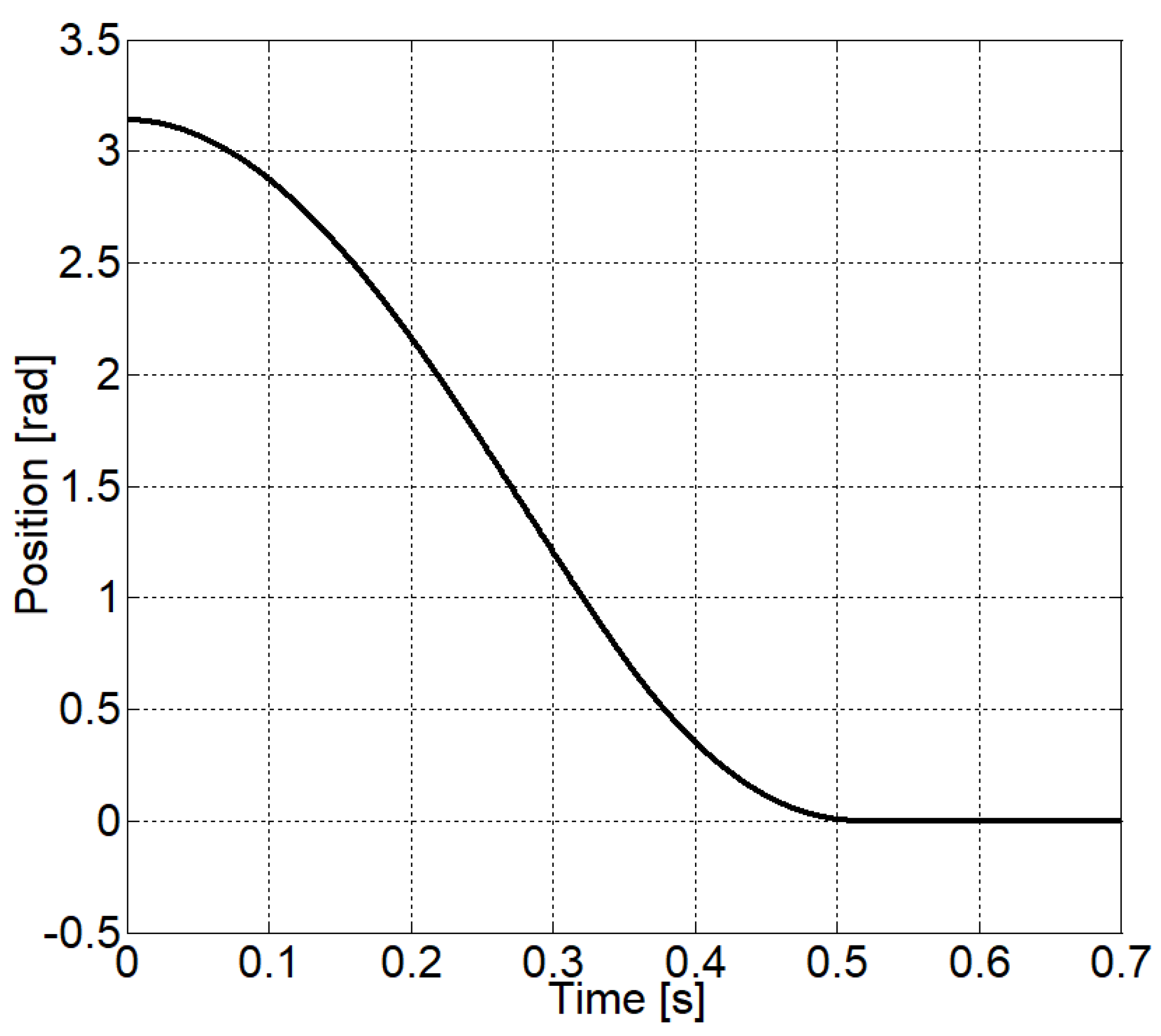
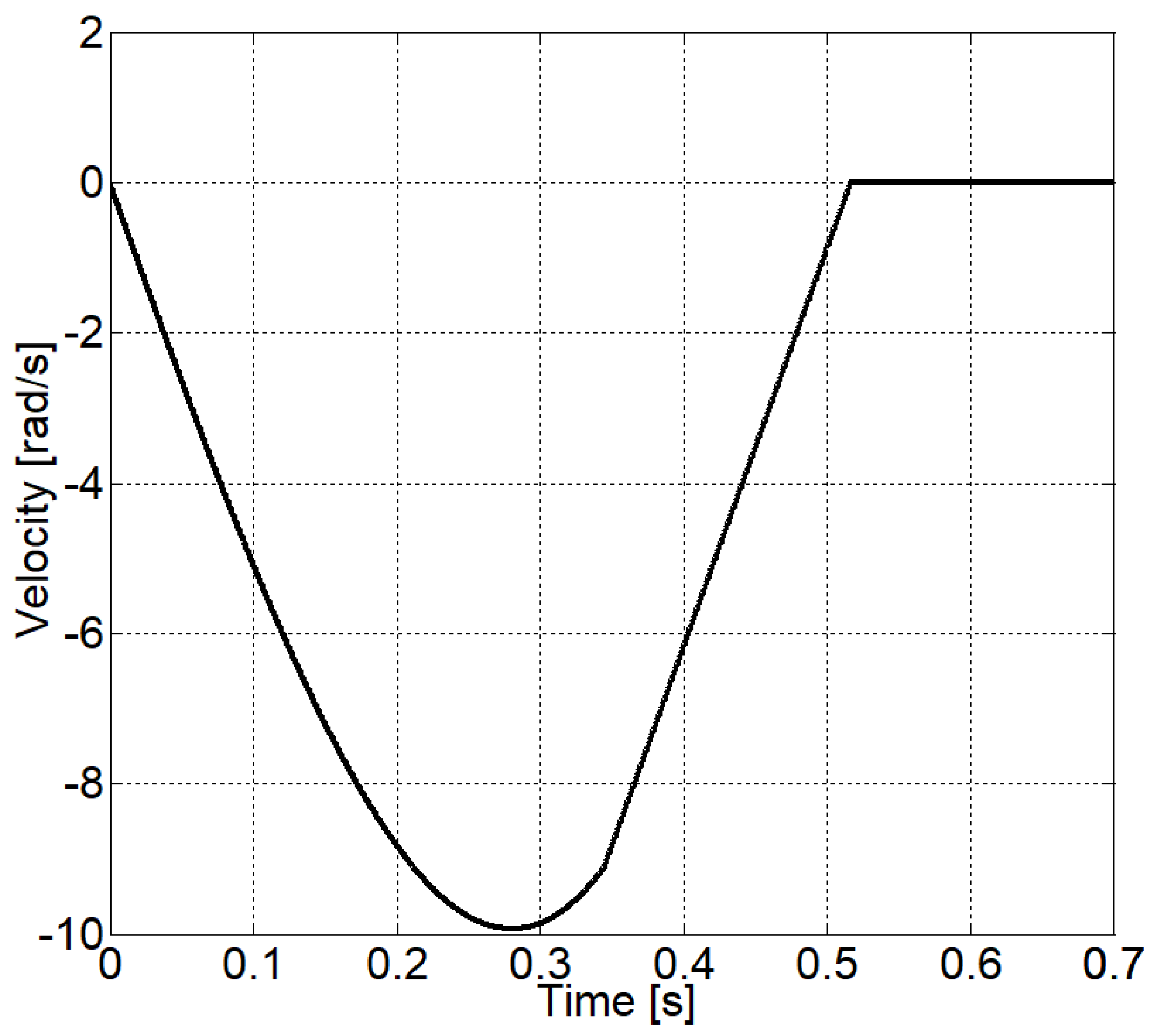
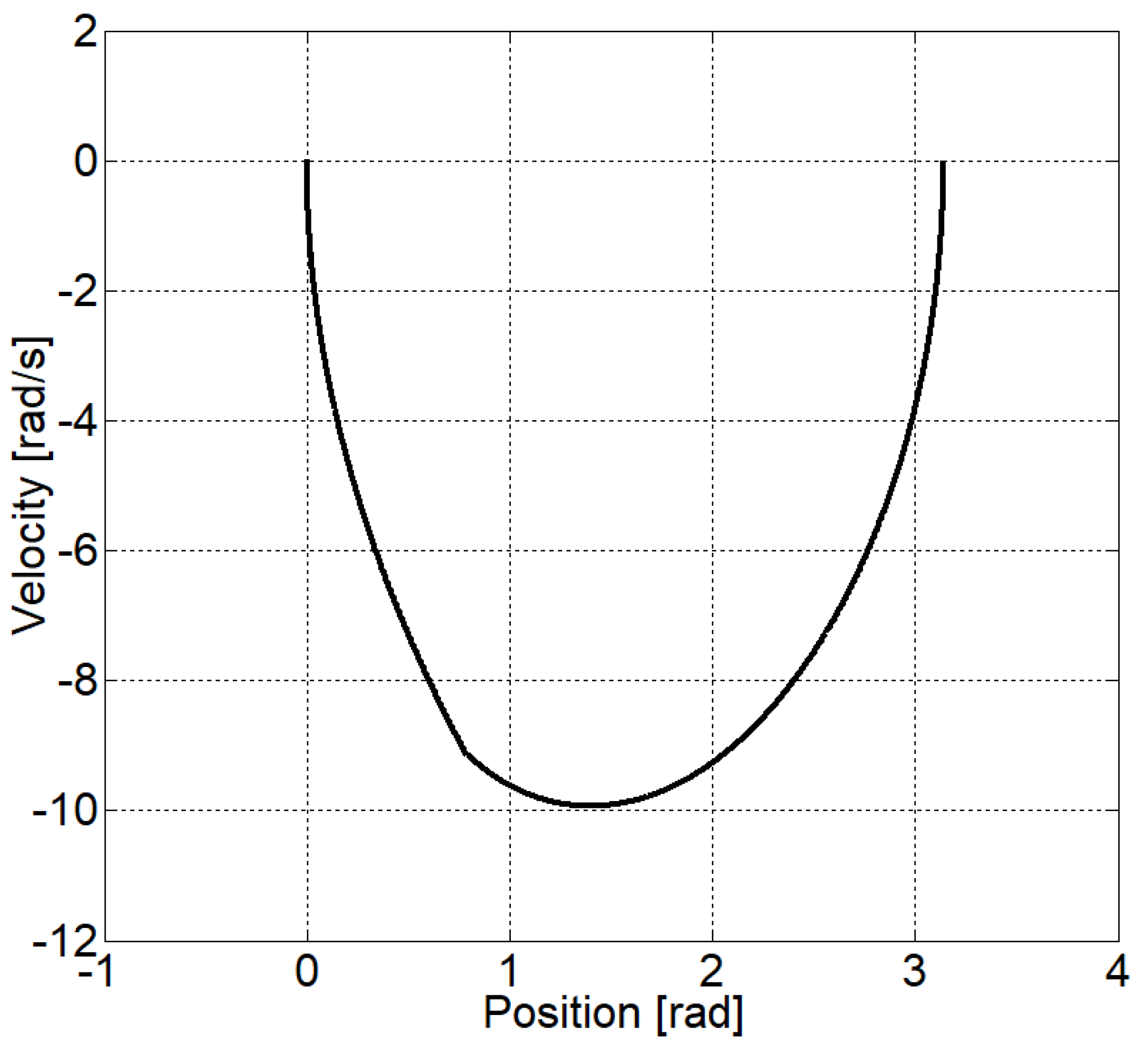
4. Simulation Example
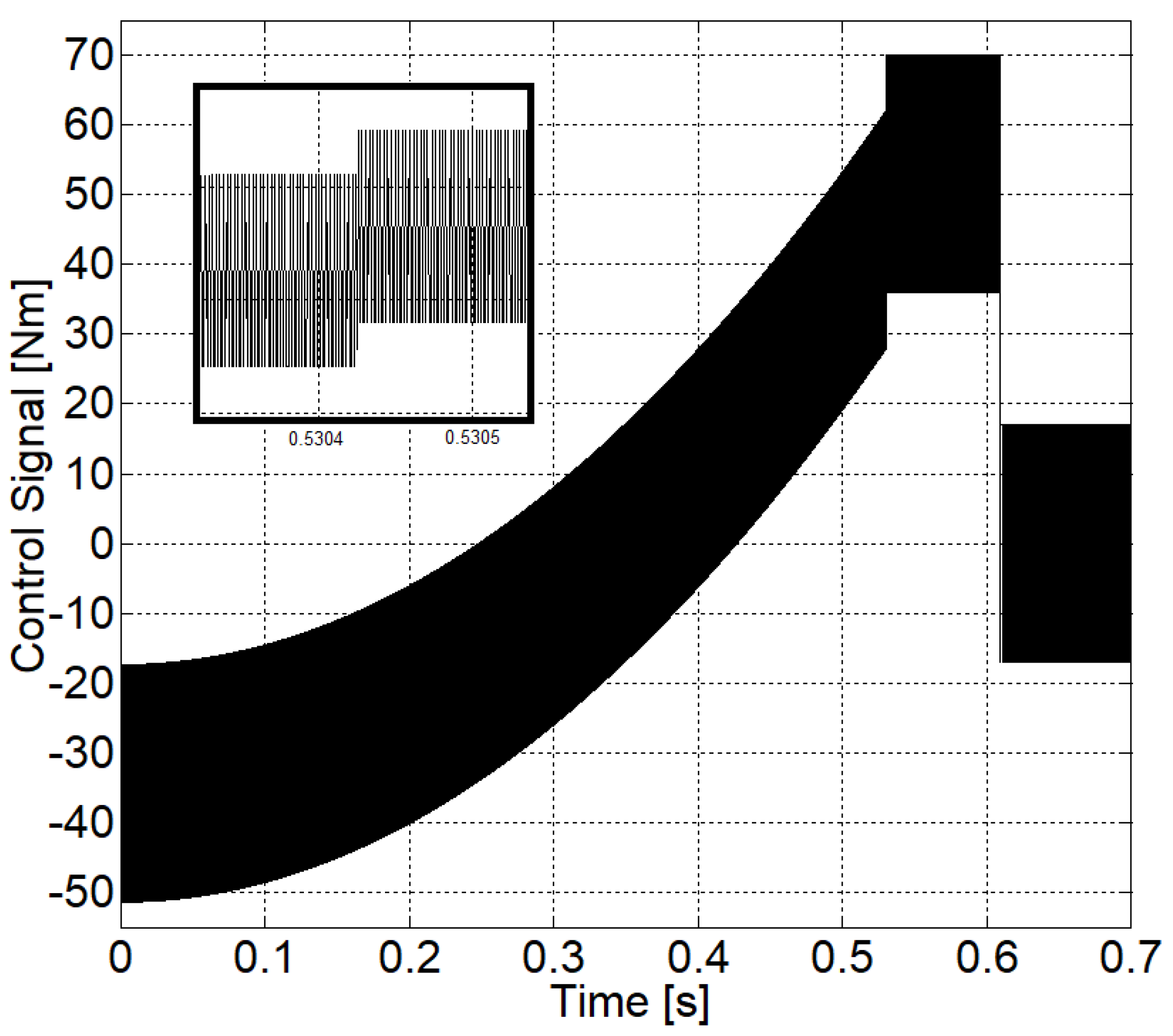
4.1. Control Signal Constraint
4.2. System’s Angular Velocity Constraint
4.3. Both Control Signal and System’s Angular Velocity Constraints
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Basin, M.; Fridman, L.; Shi, P. (Eds.) Optimal sliding mode algorithms for dynamic systems. J. Frankl. Inst. 2012, 349, 1317–1616. [Google Scholar] [CrossRef]
- Gao, W. (Ed.) Variable structure control. IEEE Trans. Ind. Electron. 1993, 40, 1–165. [Google Scholar]
- Kaynak, O.; Bartoszewicz, A.; Utkin, V.I. (Eds.) Sliding mode control in industrial applications. IEEE Trans. Ind. Electron. 2009, 56, 3271–3274. [Google Scholar]
- Misawa, A.; Utkin, V.I. (Eds.) Variable structure systems. Trans. Asme J. Dyn. Syst. Meas. Control. 2000, 122, 585–819. [Google Scholar]
- Utkin, V.I. (Ed.) Sliding mode control. Int. J. Control 1993, 57, 1003–1259. [Google Scholar]
- Shtessel, Y.; Spurgeon, S.; Fridman, L. Advances in sliding mode observation and estimation. Intern. J. Syst. Sci. 2007, 38, 845–942. [Google Scholar] [CrossRef]
- Utkin, V.I. Variable structure systems with sliding modes. IEEE Trans. Autom. Control 1977, 22, 212–222. [Google Scholar] [CrossRef]
- Furuta, K. Sliding mode control of a discrete system. Syst. Control Lett. 1990, 14, 145–152. [Google Scholar] [CrossRef]
- Milosavljević, Č. General conditions for the existence of a quasisliding mode on the switching hyperplane in discrete variable structure systems. Autom. Remote. Control 1985, 46, 307–314. [Google Scholar]
- Utkin, V.I.; Drakunow, S.V. On discrete-time sliding mode control. IFAC Conf. Nonlinear Control 1989, 484–489. [Google Scholar]
- Choi, S.B.; Park, D.W.; Jayasuriya, S. A time-varying sliding surface for fast and robust tracking control of second-order uncertain systems. Automatica 1994, 30, 899–904. [Google Scholar] [CrossRef]
- Yilmaz, C.; Hurmuzlu, Y. Eliminating the reaching phase from variable structure control. J. Dyn. Syst. Meas. Control. 2000, 122, 753–757. [Google Scholar] [CrossRef] [Green Version]
- Man, Z.; Yu, X.H. Terminal sliding mode control of MIMO linear systems. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 1997, 44, 1065–1070. [Google Scholar]
- Man, Z.; Paplinski, A.; Wu, H.R. A robust MIMO terminal sliding mode control scheme for rigid robotic manipulators. IEEE Trans. Autom. Control 1994, 39, 2464–2469. [Google Scholar]
- Tang, Y. Terminal sliding mode control for rigid robots. Automatica 1998, 34, 51–56. [Google Scholar] [CrossRef]
- Hosseinzadeh, M.; Cotorruelo, A.; Limon, D.; Garone, E. Constrained Control of Linear Systems Subject to Combinations of Intersections and Unions of Concave Constraints. IEEE Control Syst. Lett. 2019, 3, 571–576. [Google Scholar] [CrossRef]
- O’Rourke, I.; Kolmanowvsky, I.; Garone, E.; Girard, A. Explicit Reference Governor for Constrained Maneuver and Shape Control of a Seven-State Multibody Aircraft. In AIAA Scitech 2020 Forum; AIAA: Reston, VA, USA, 2020; p. 1830. [Google Scholar]
- Gao, P.; Zhang, G.; Lv, X. Model-Free Control Using Improved Smoothing Extended State Observer and Super-Twisting Nonlinear Sliding Mode Control for PMSM Drives. Energies 2021, 14, 922. [Google Scholar] [CrossRef]
- Milosavljević, Č.; Peruničić-Draženović, B.; Veselić, B.; Petronijević, M. High-performance discrete-time chattering-free sliding mode-based speed control of induction motor. Electr. Eng. 2017, 99, 583–593. [Google Scholar] [CrossRef]
- Kali, Y.; Saad, M.; Doval-Gandoy, J.; Rodas, J. Discrete terminal super-twisting current control of a six-phase induction motor. Energies 2021, 14, 1339. [Google Scholar] [CrossRef]
- Ali, N.; Liu, Z.; Armghan, H.; Ahmad, I.; Hou, Y. LCC-S-Based integral terminal sliding mode controller for a hybrid energy storage system using a wireless power system. Energies 2021, 14, 1693. [Google Scholar] [CrossRef]
- Mobayen, S.; Bayat, F.; Lai, C.-C.; Taheri, A.; Fekih, A. Adaptive global sliding mode controller design for perturbed DC-DC buck converters. Energies 2021, 14, 1249. [Google Scholar] [CrossRef]
- Oliveira, T.; Caseiro, L.; Mendes, A.; Cruz, S.; Perdigão, M. Model Predictive Control for Paralleled Uninterruptible Power Supplies with an Additional Inverter Leg for Load-Side Neutral Connection. Energies 2021, 14, 2270. [Google Scholar] [CrossRef]
- Vásquez, L.O.P.; Ramírez, V.M.; Langarica Córdova, D.; Redondo, J.L.; Álvarez, J.D.; Torres-Moreno, J.L. Optimal Management of a Microgrid with Radiation and Wind-Speed Forecasting: A Case Study Applied to a Bioclimatic Building. Energies 2021, 14, 2398. [Google Scholar] [CrossRef]

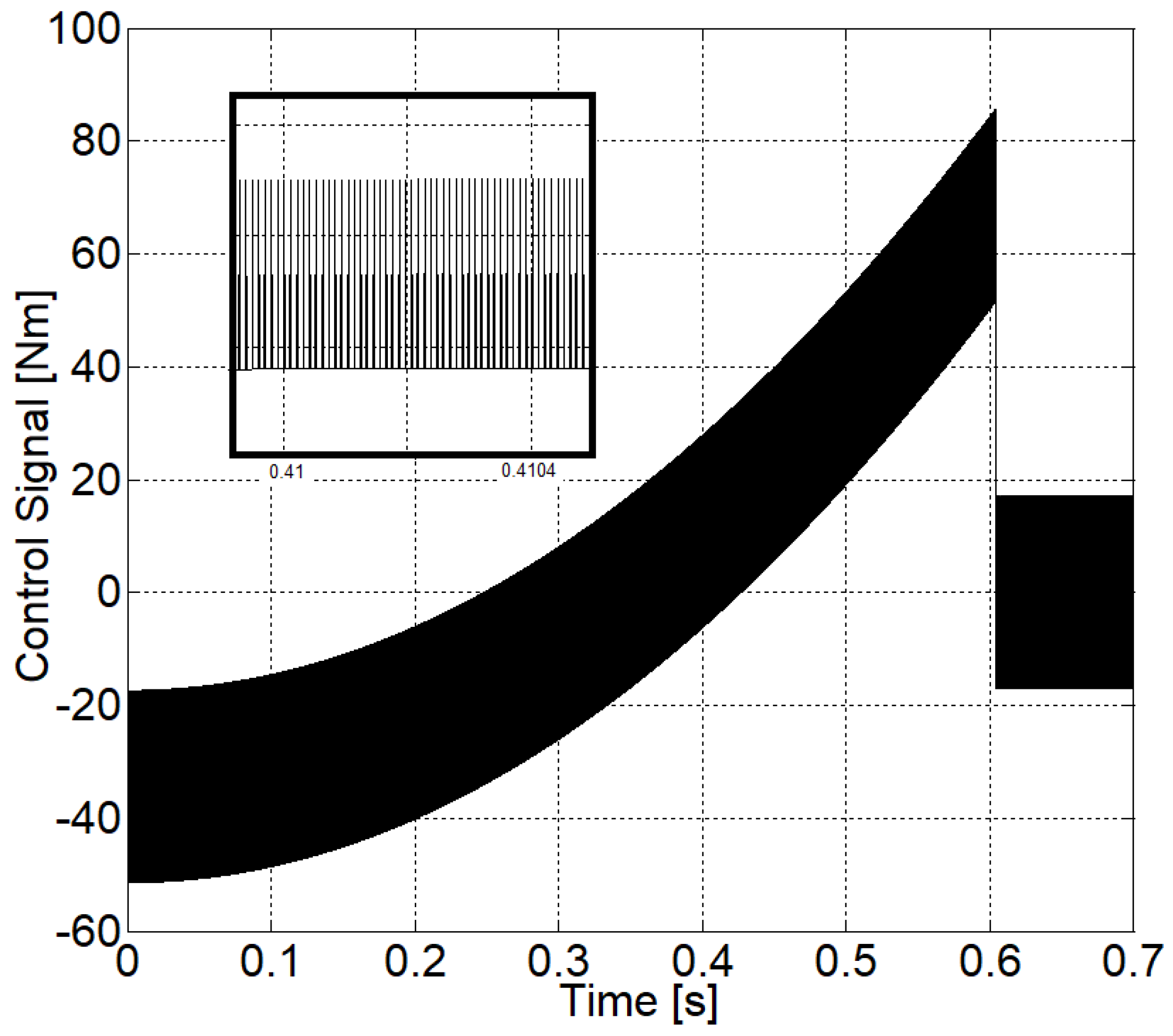
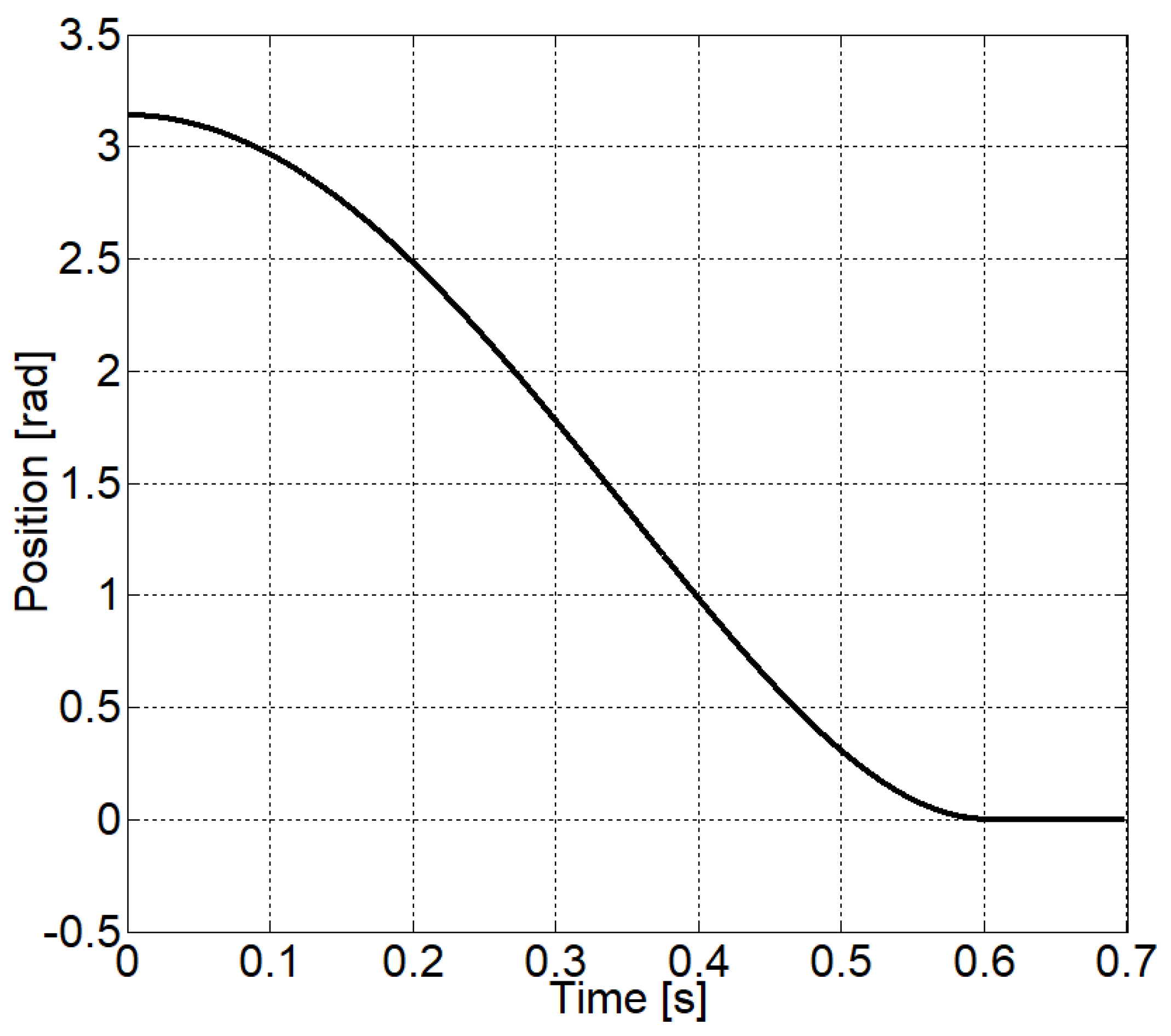
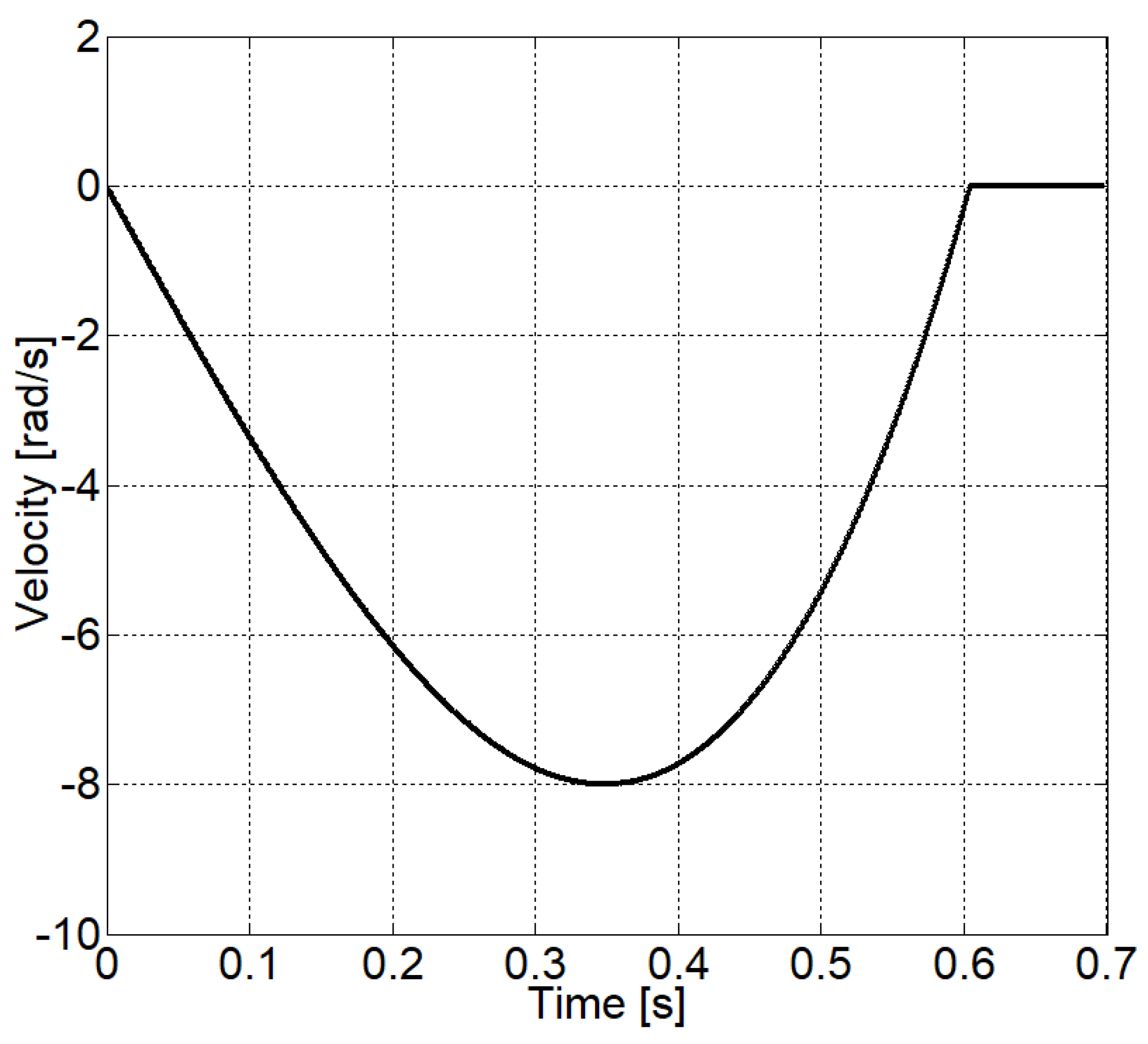
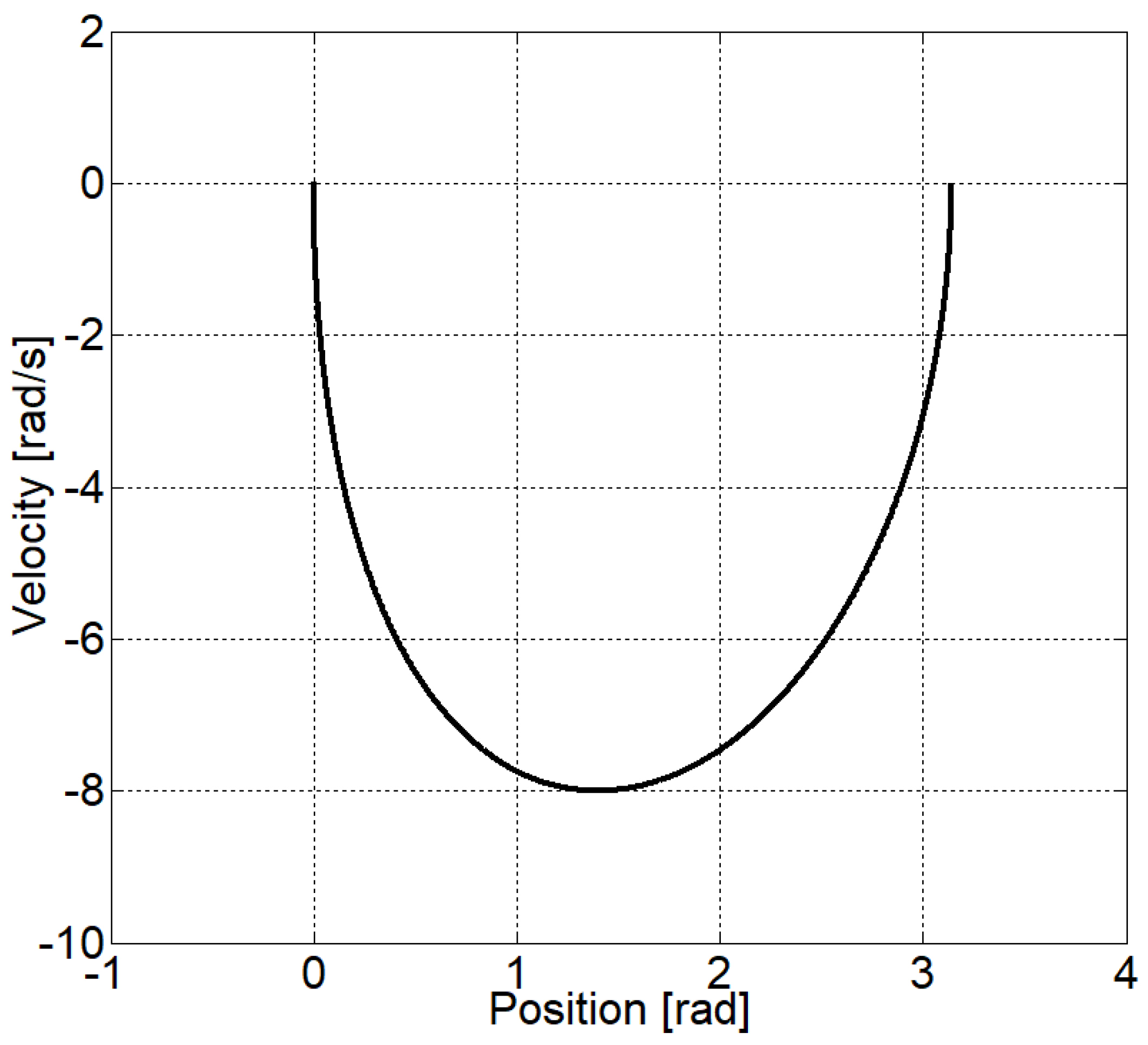



| IAE | ITAE | ||
|---|---|---|---|
| u limitation | 0.5163 | 0.8197 | 0.1260 |
| limitation | 0.6043 | 1.0120 | 0.1911 |
| both limitations | 0.6094 | 1.0121 | 0.1912 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pietrala, M.; Leśniewski, P.; Bartoszewicz, A. Sliding Mode Control with Minimization of the Regulation Time in the Presence of Control Signal and Velocity Constraints. Energies 2021, 14, 2887. https://doi.org/10.3390/en14102887
Pietrala M, Leśniewski P, Bartoszewicz A. Sliding Mode Control with Minimization of the Regulation Time in the Presence of Control Signal and Velocity Constraints. Energies. 2021; 14(10):2887. https://doi.org/10.3390/en14102887
Chicago/Turabian StylePietrala, Mateusz, Piotr Leśniewski, and Andrzej Bartoszewicz. 2021. "Sliding Mode Control with Minimization of the Regulation Time in the Presence of Control Signal and Velocity Constraints" Energies 14, no. 10: 2887. https://doi.org/10.3390/en14102887
APA StylePietrala, M., Leśniewski, P., & Bartoszewicz, A. (2021). Sliding Mode Control with Minimization of the Regulation Time in the Presence of Control Signal and Velocity Constraints. Energies, 14(10), 2887. https://doi.org/10.3390/en14102887







