Optimization of Air Cooling System Using Adjoint Solver Technique
Abstract
:1. Introduction
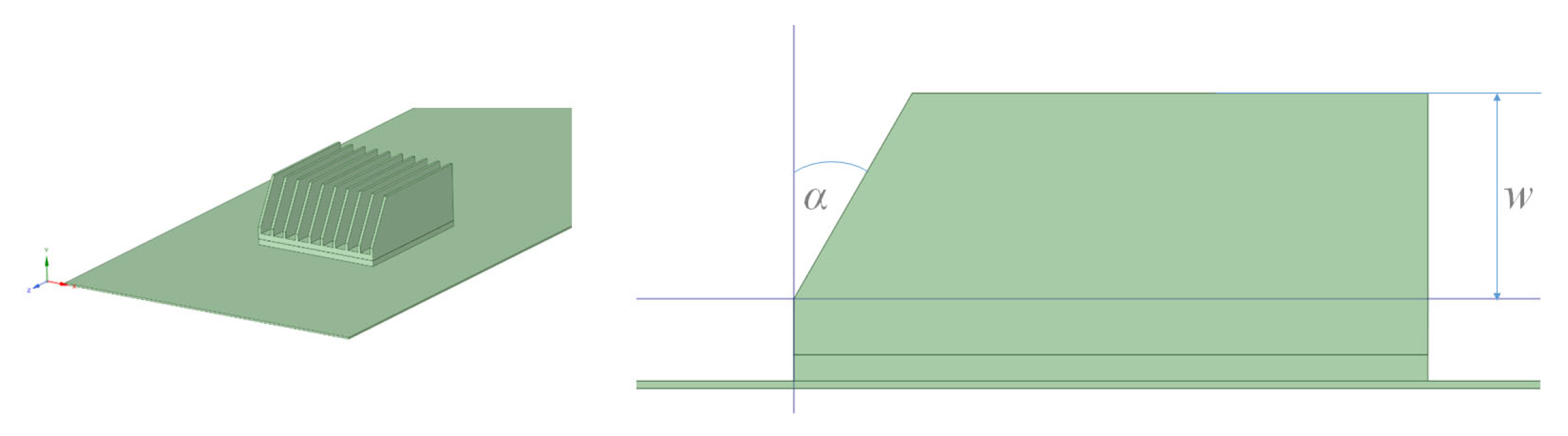
2. Research Object
3. Computational Model
3.1. Mathematical Model
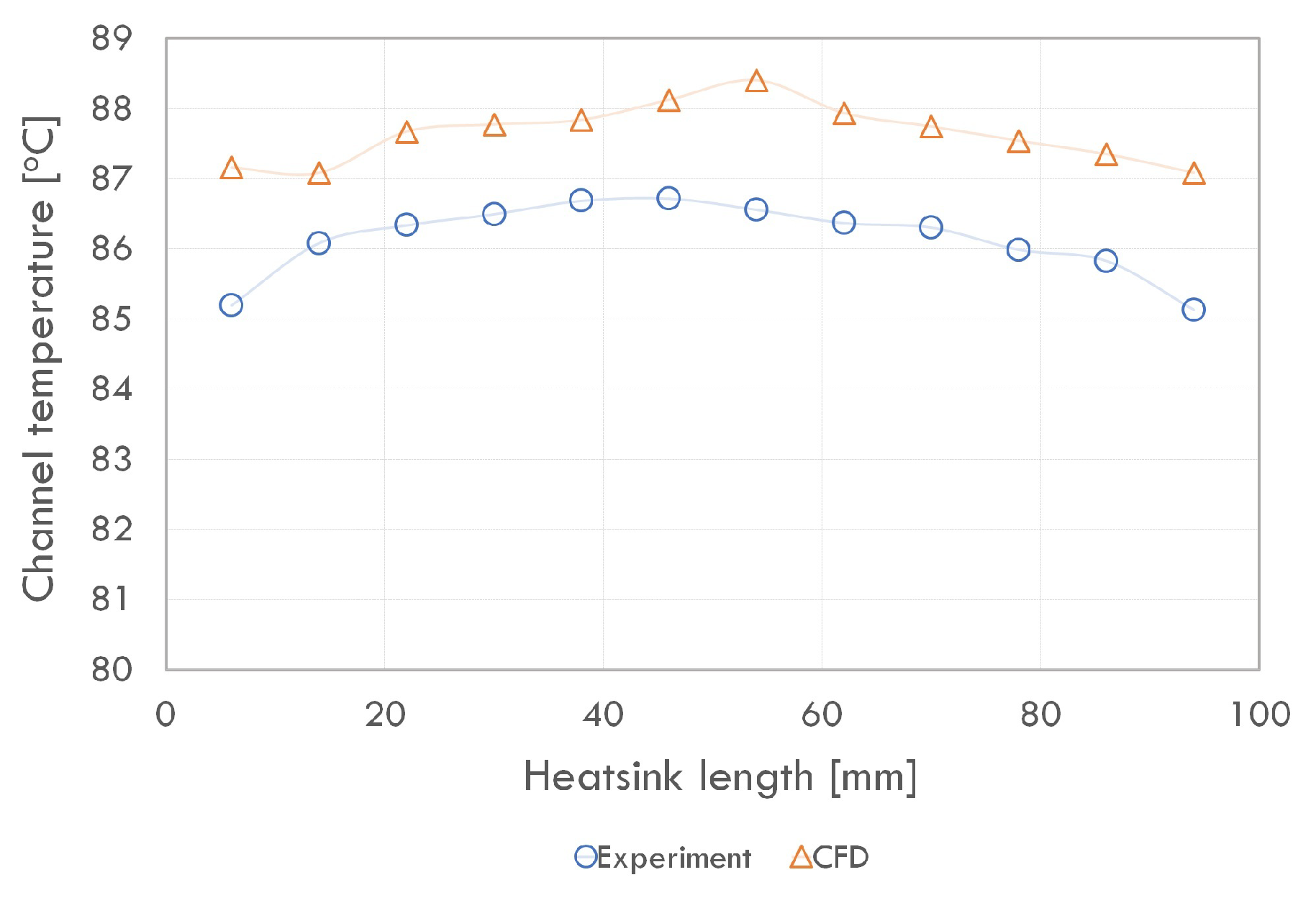
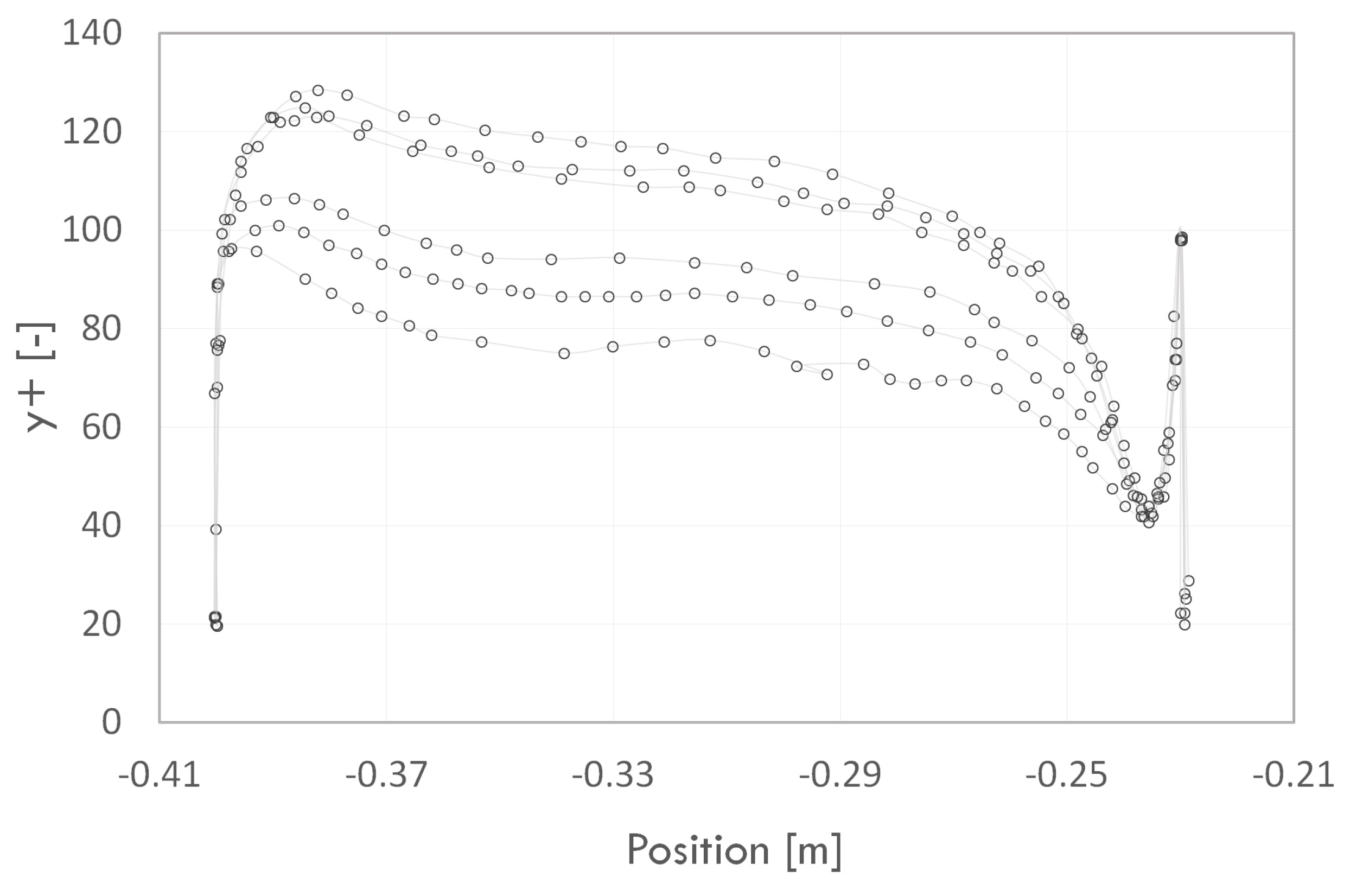
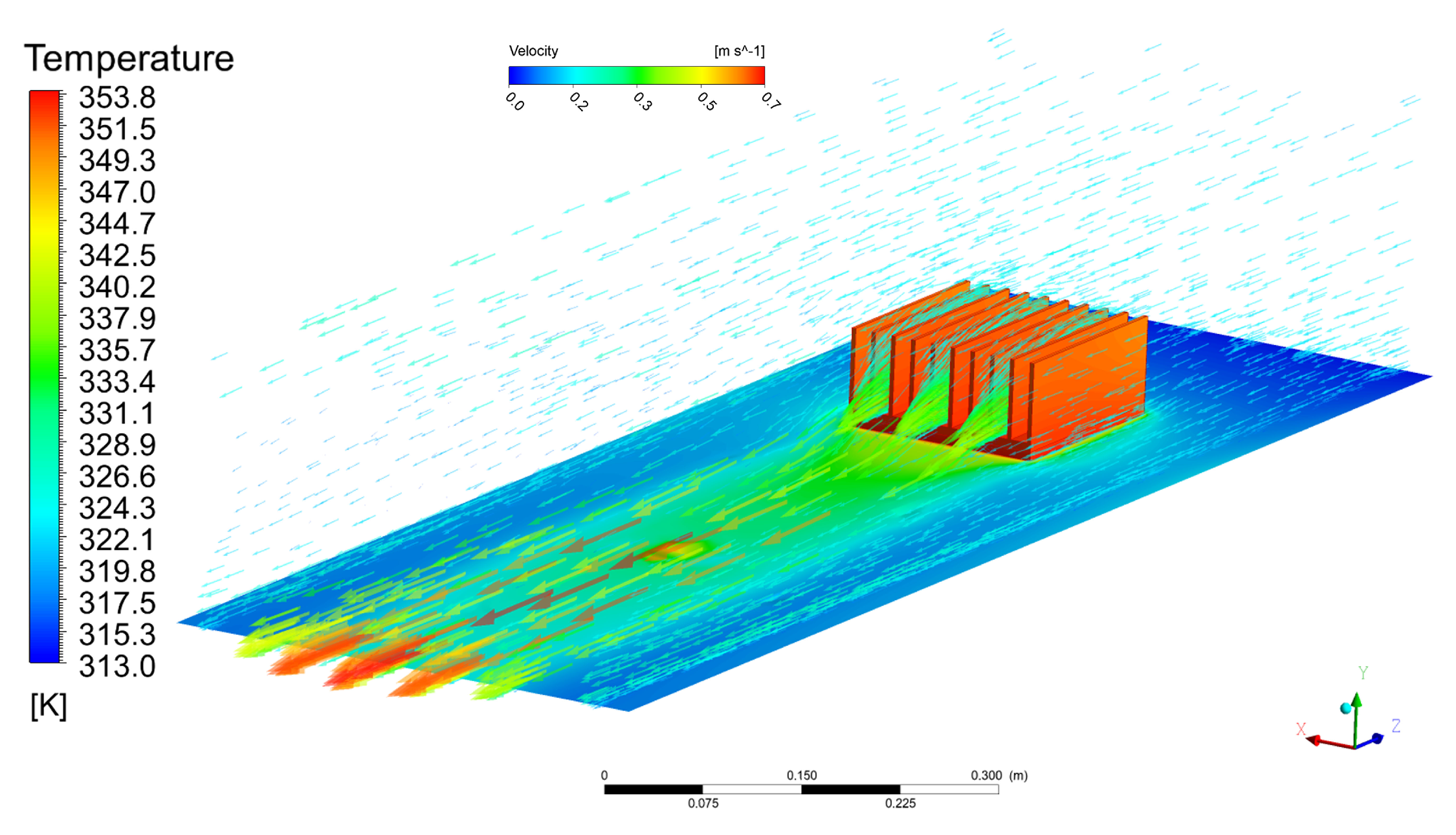
3.2. Validation of the Adopted Model
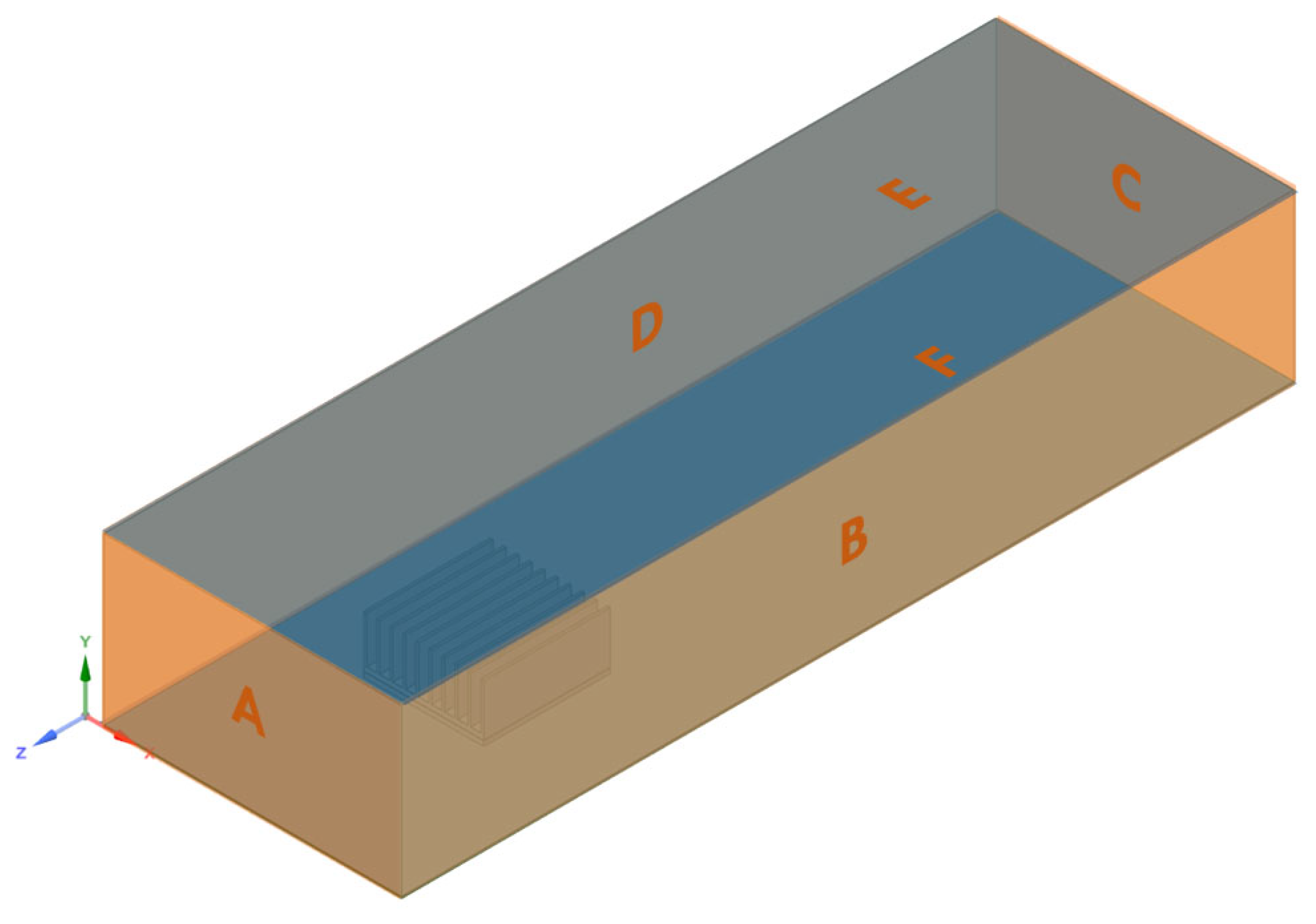
3.3. Computational Conditions
- a pressure inlet type boundary condition was assumed on surface A; relative pressure value 0 Pa, surface temperature 40 C, flow direction normal to the surface;
- a pressure outlet type boundary condition was assumed on surface C; relative pressure value 0 Pa, surface temperature 40 C, flow direction normal to the surface;
- a wall type boundary condition was assumed on surfaces B, D, E, F; stationary wall, no slip (v = 0);
- the emissivity value of the outer surface of the heat sink is equal to 0.9;
- the emissivity value of the outer surface of the heat source is equal to 0.3;
- the emissivity value of the upper base surface is equal to 0.9;
- the emissivity value of the surfaces B, D, E is equal to 0.9.
Material Properties and Solver Settings
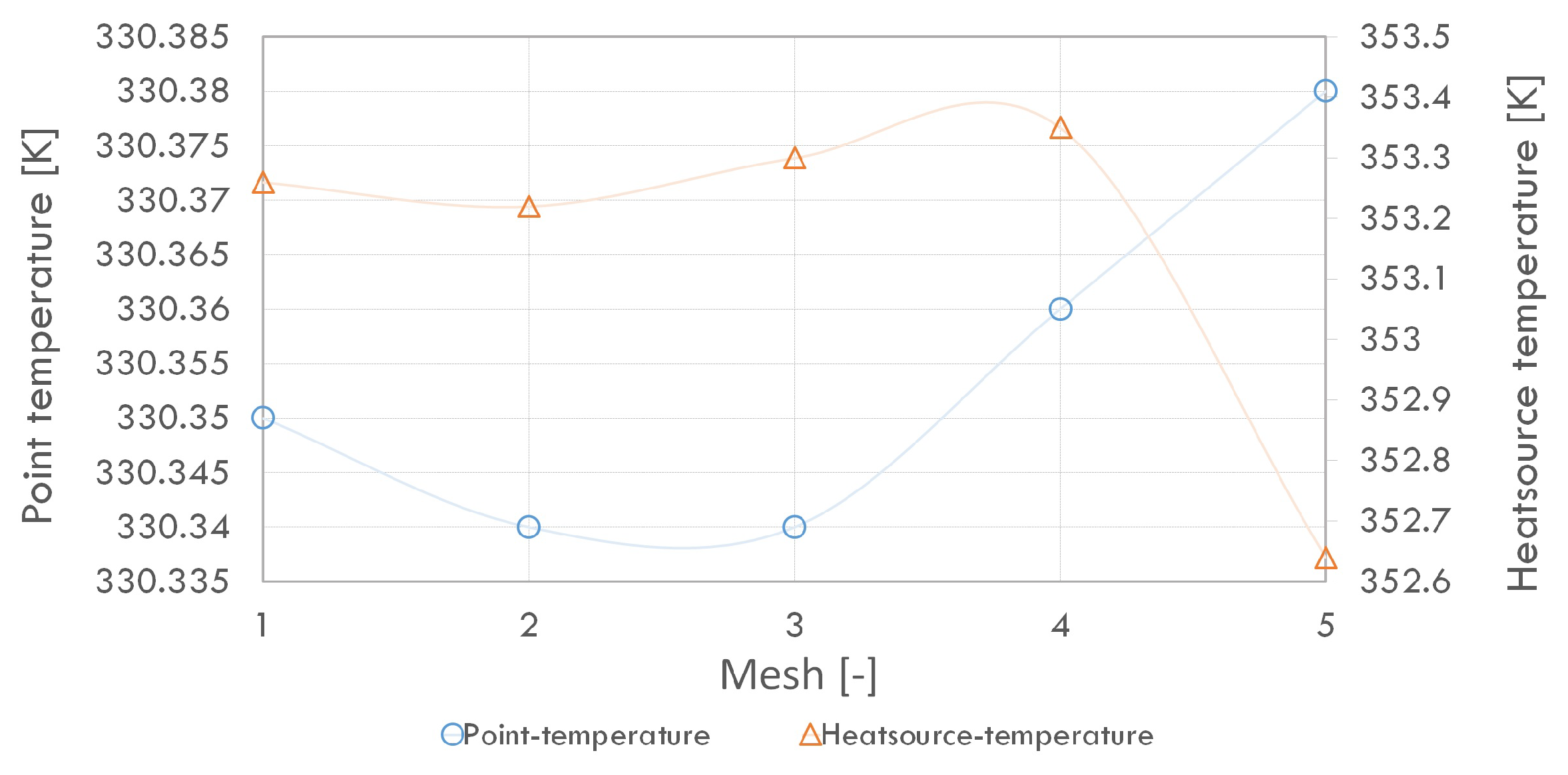
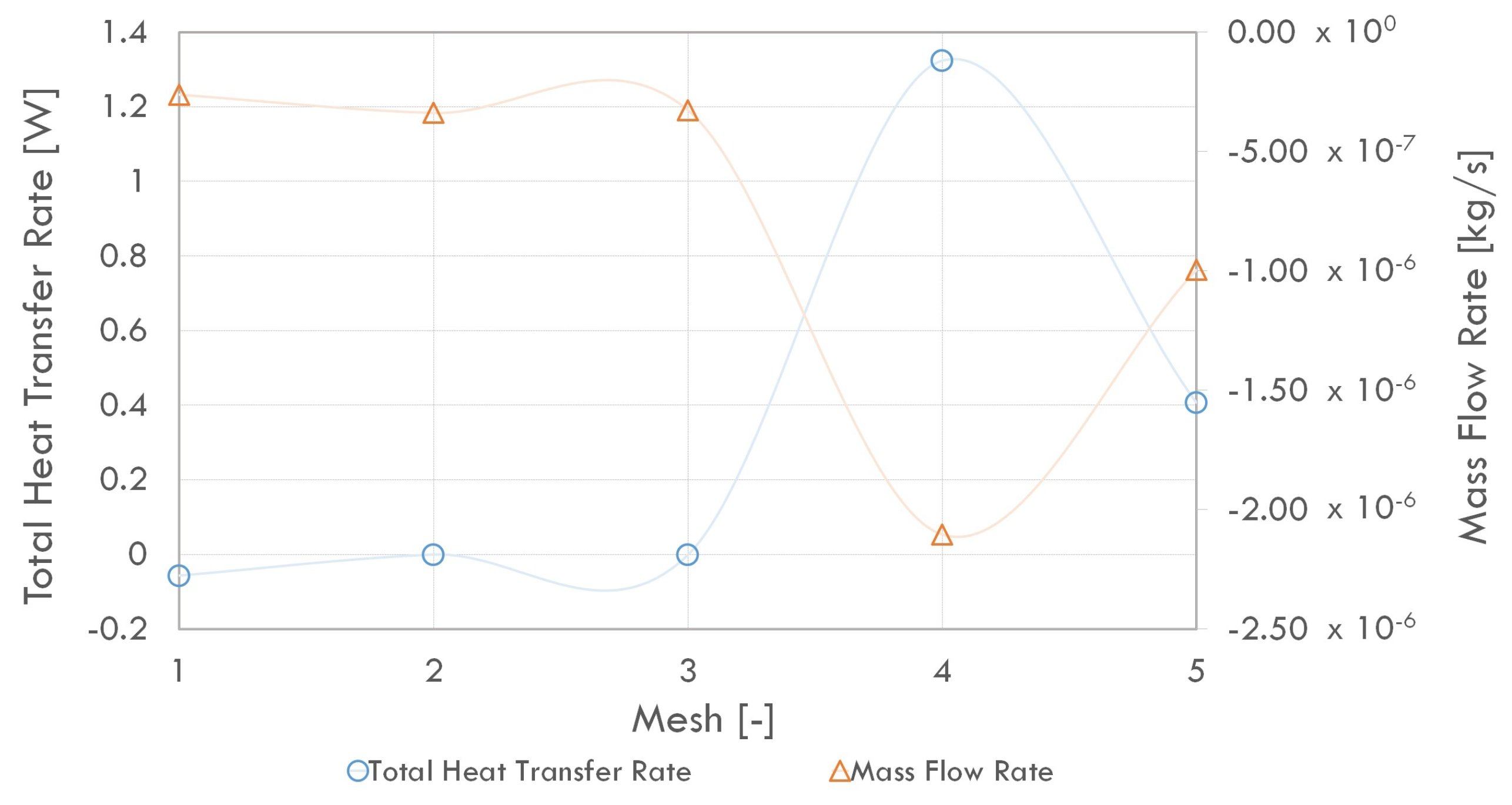
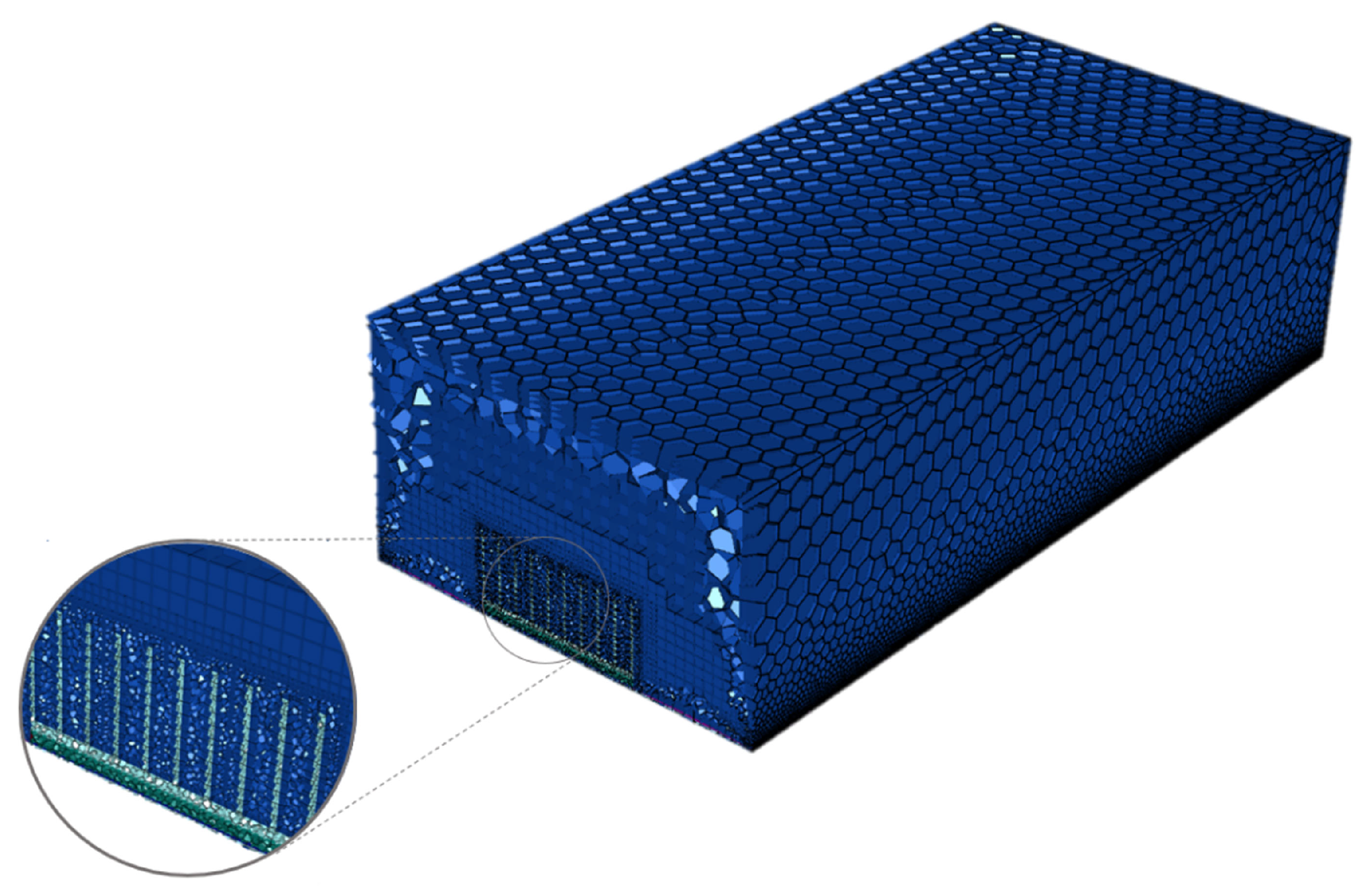
3.4. Adopted Numerical Grid
4. Results and Discussion of the Numerical Calculations
4.1. Effect of Radiation on the Solution
4.2. Effect of Fin Shape on the Maximum Temperature in the Cooling System
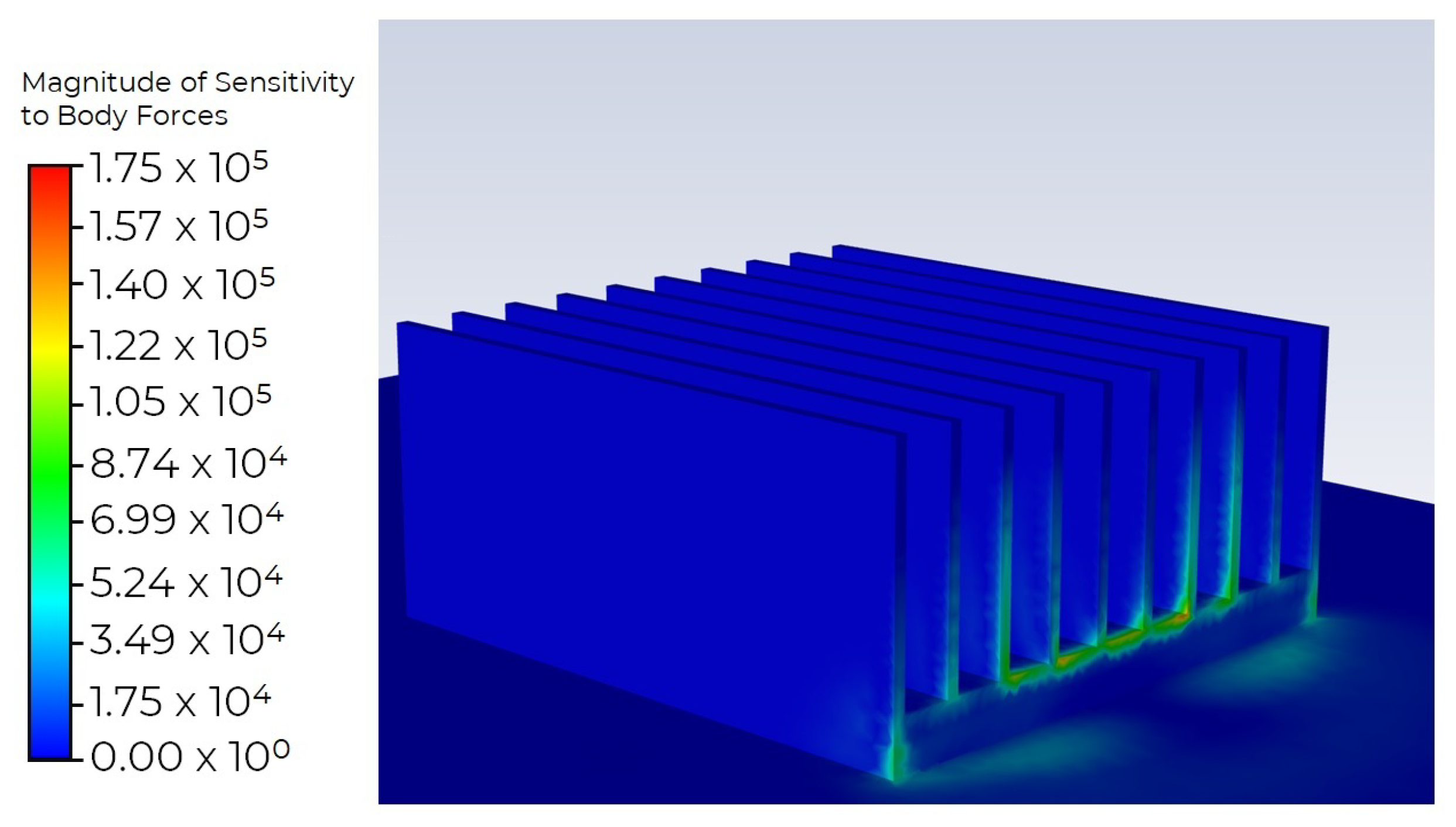
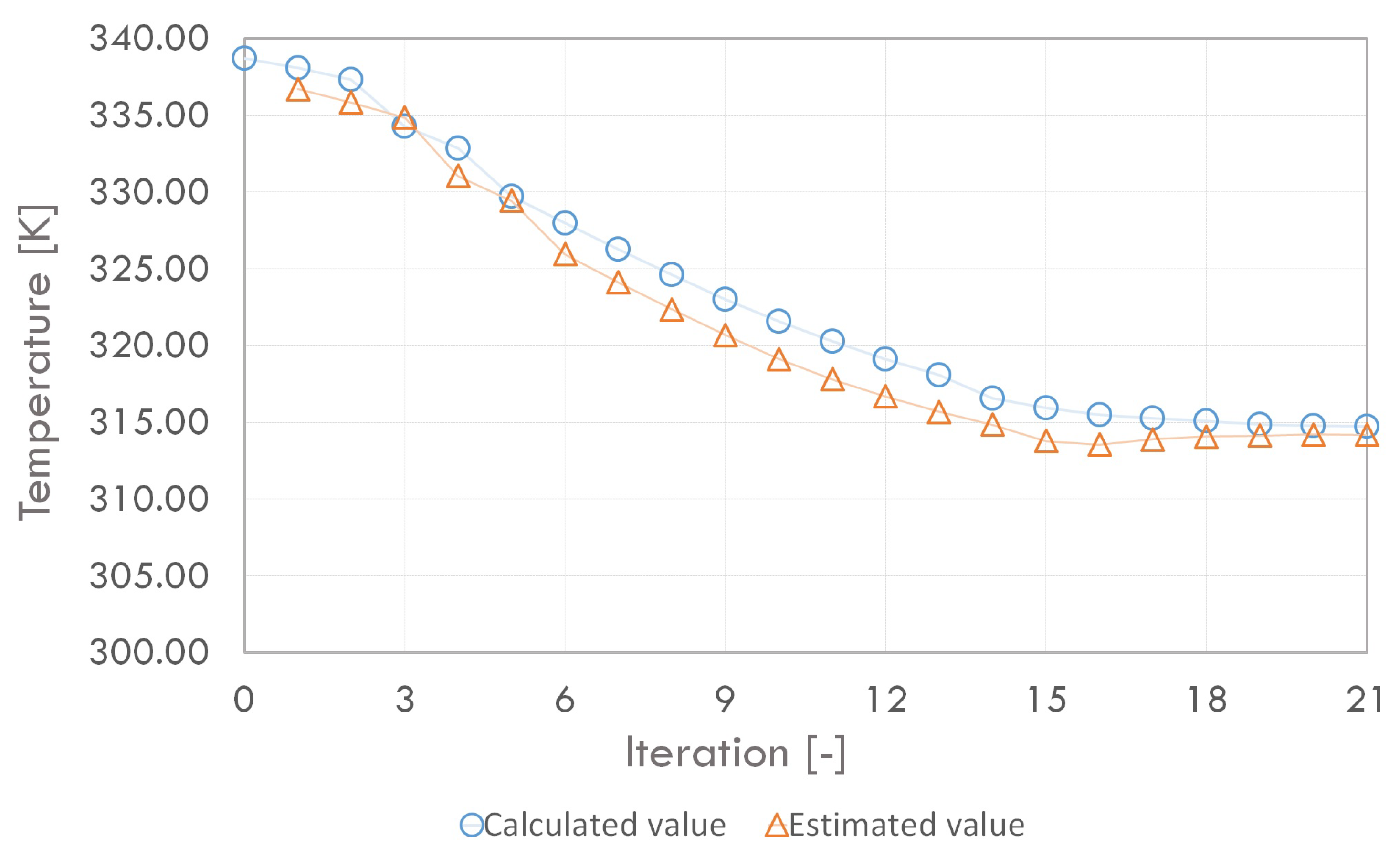
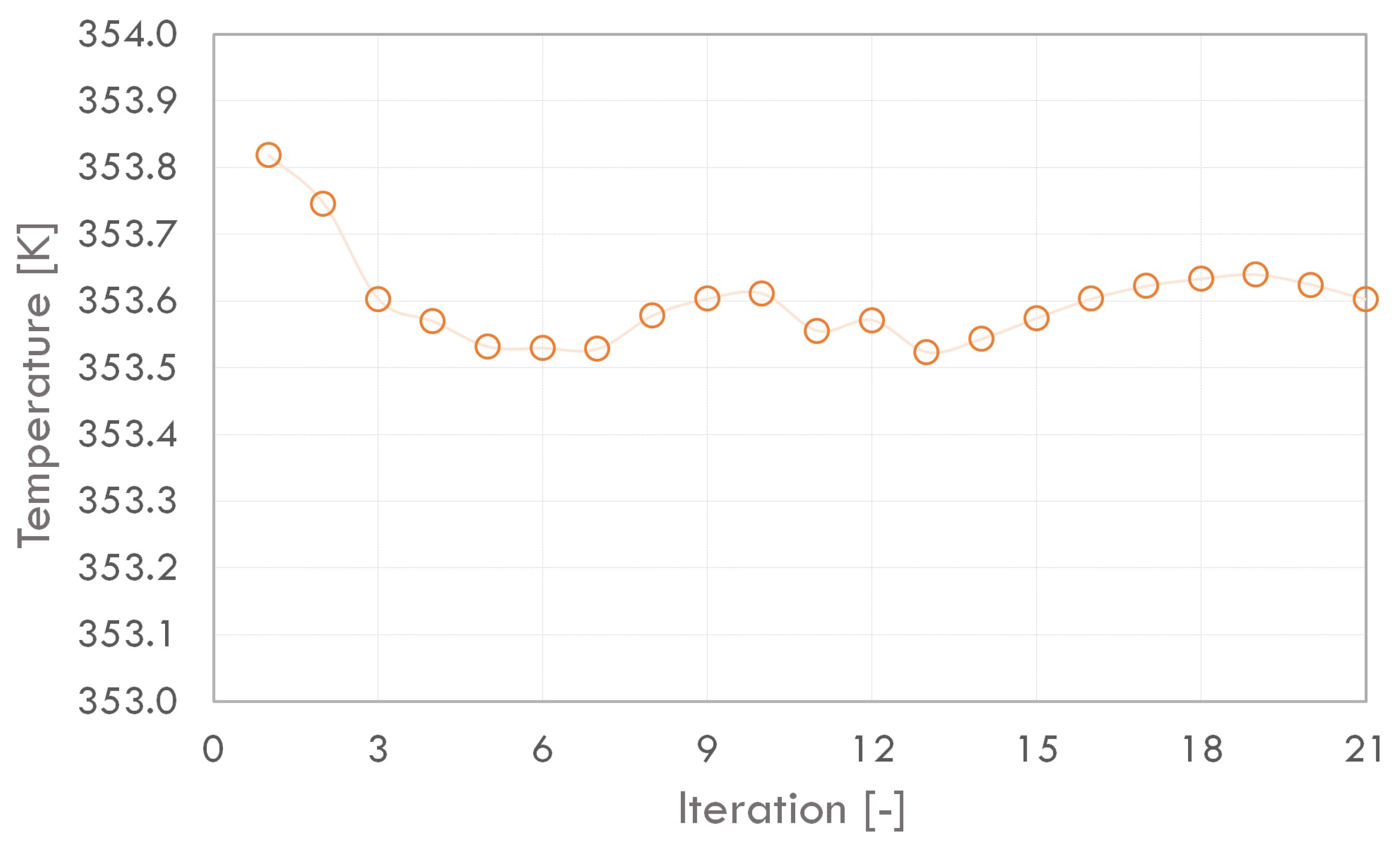
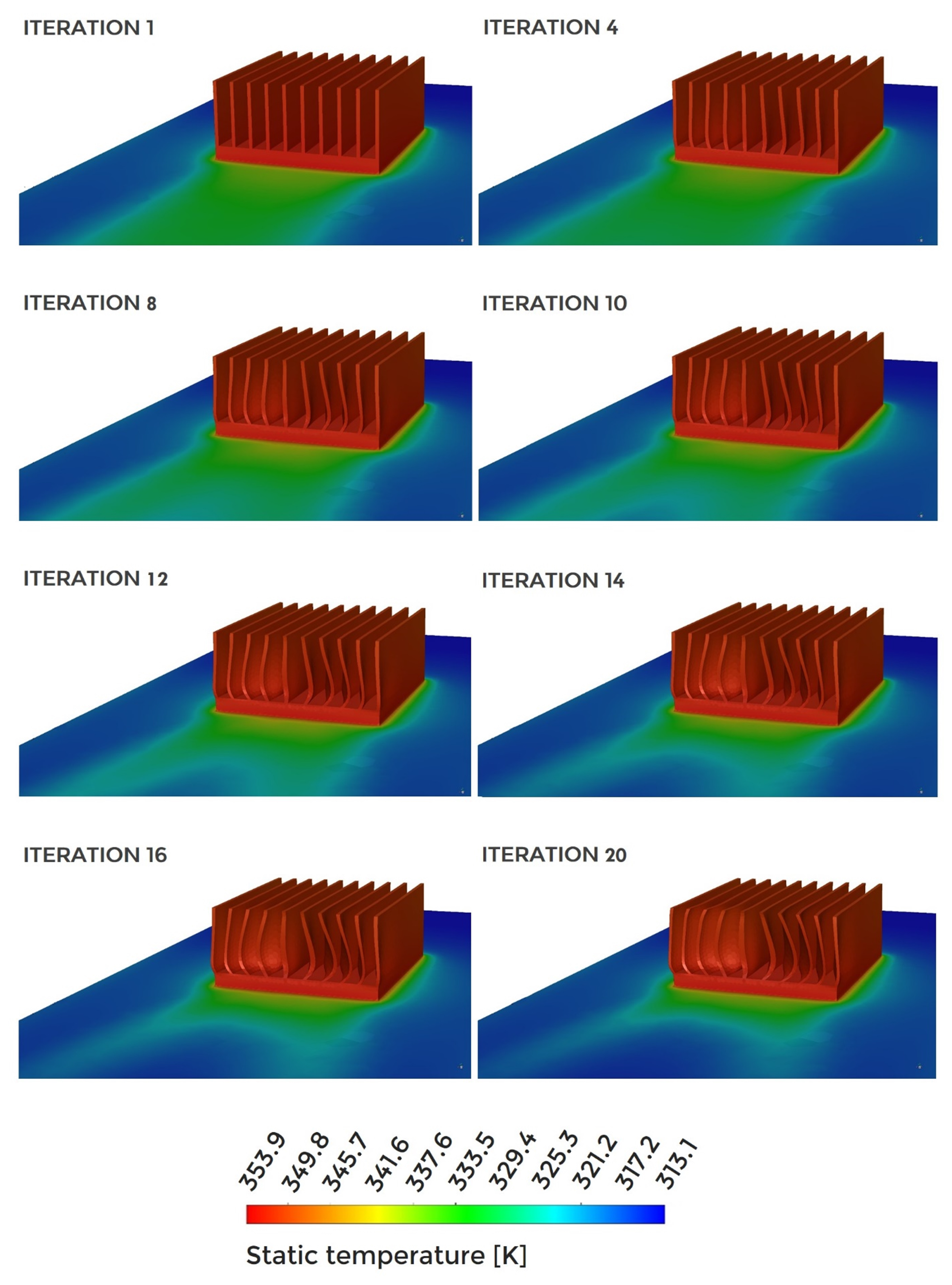
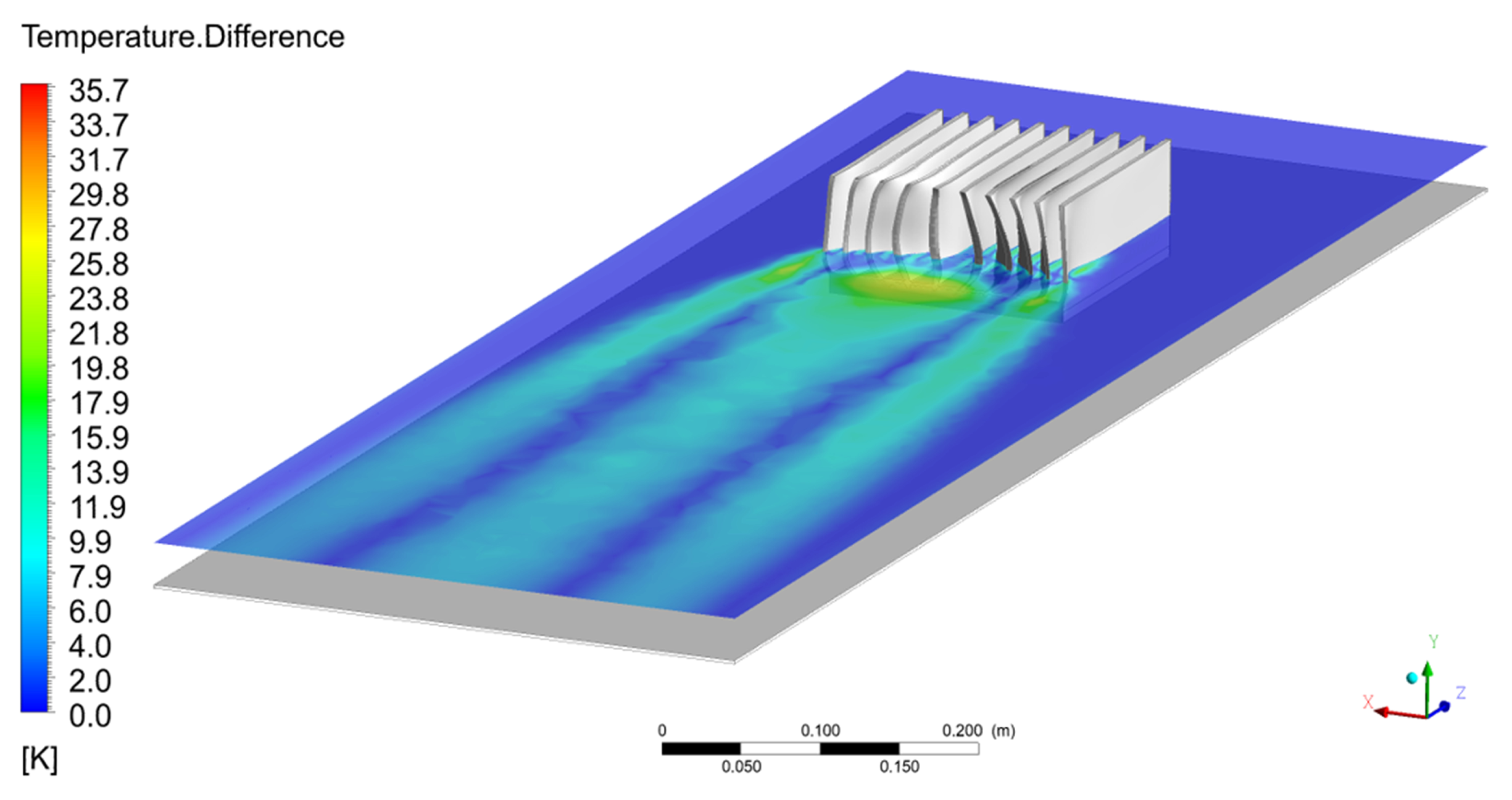
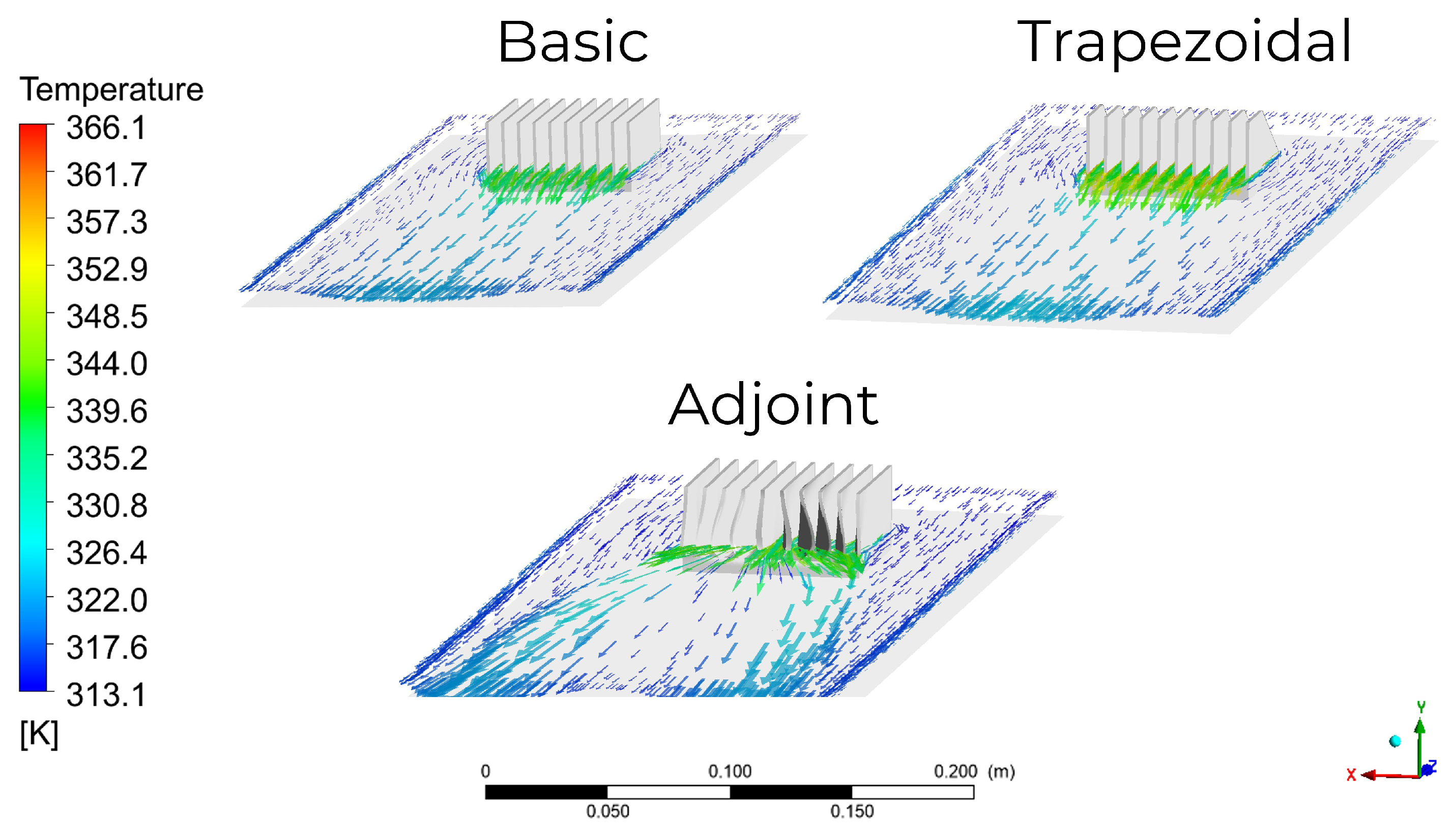
4.3. Use of Adjoint Solver Technique for Heat Sink Shape Optimization
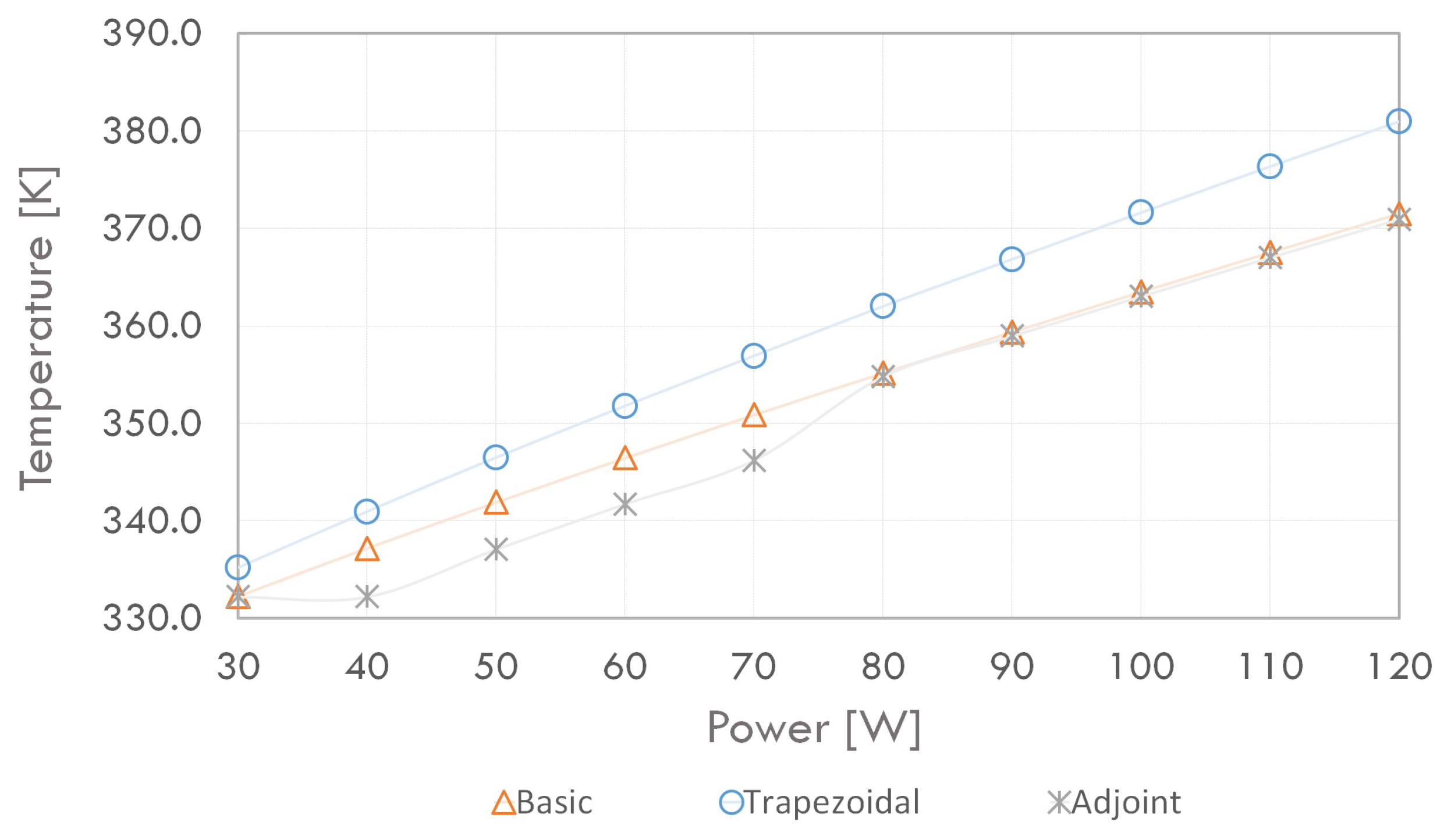
4.4. Effect of Power Dissipated by an Element on the Temperature in the Cooling System
5. Summary and Conclusions
- The optimisation performed using the Adjoint Solver technique allowed for a reduction of the assumed temperature by 25 K. This did lead to a decrease in the minimum value of the orthogonal grid quality. However, in this case, it is possible to re-generate the grid for the optimised geometric model.
- Neglecting the radiation phenomenon in the tested system led to overestimated heat sink temperatures (by nearly 14 K).
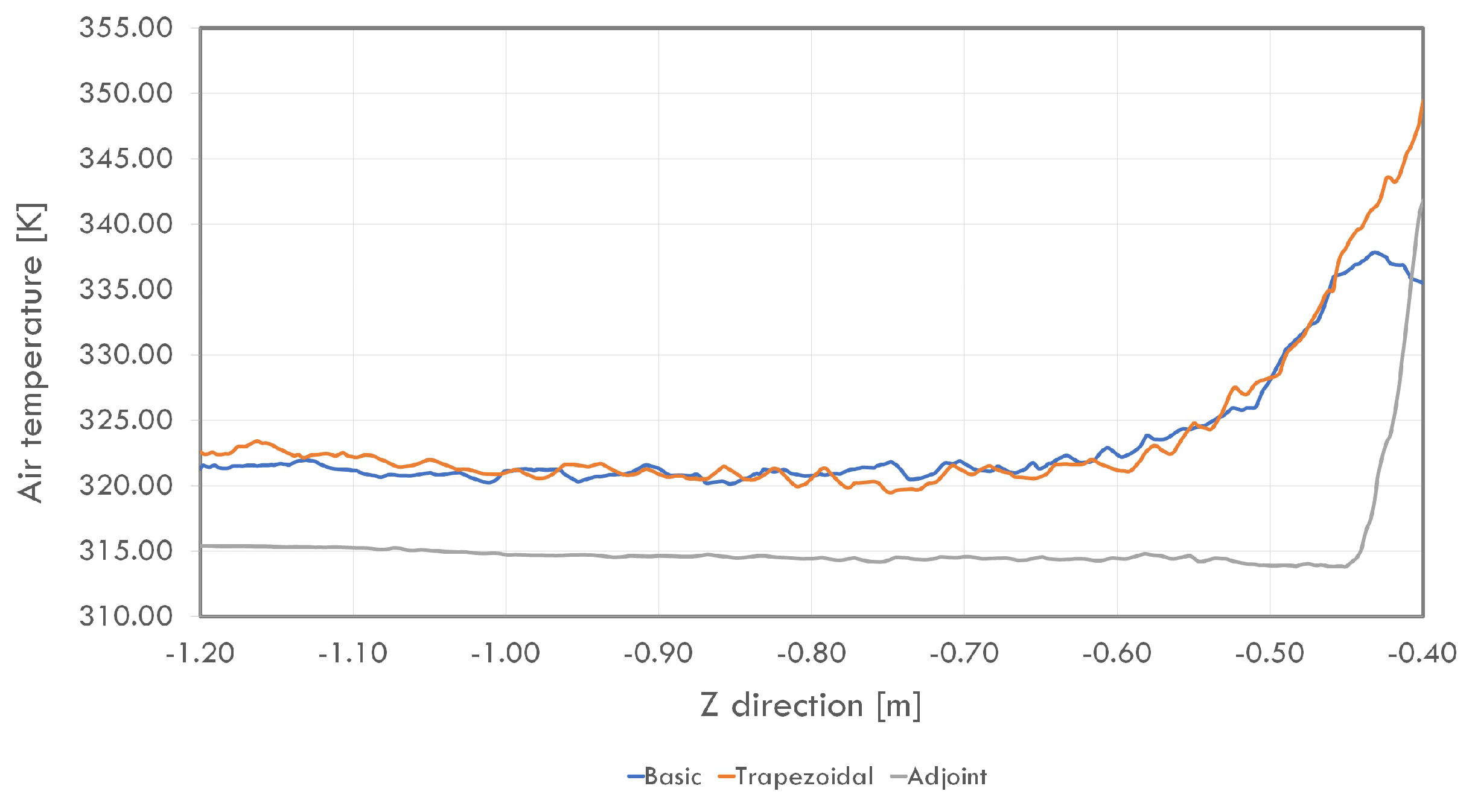
- Increasing the angle of the fin () and increasing the value of the parameter (w) allows a lower value of the air temperature behind the heat sink, which on the other hand, adversely affects the heating of the heat source component. The maximum values of the parameters and w cause a 1.5 K decrease in the temperature value behind the heat sink and a 6 K increase in the heat source temperature.
- The proposed optimal fin design ensures that the increase in heat source power does not significantly affect the temperature in the area behind the heat sink.
- The heat source temperature for optimal fin design and heat flux up to 80 W is lower than the basic design and trapezoidal fins.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| c | specific heat of fluid [J/(kg·K)], design parameters, |
| gravitational acceleration in a given direction [m/s], | |
| J | objective function, |
| k | turbulent kinetic energy [m/s], |
| L | Lagrangian function, |
| p | pressure [Pa], |
| q | heat generation [W/m], flow condition function, |
| R | residual variable, |
| T | temperature [K], |
| t | time [s], |
| t | pseudo time step [s], |
| ,, | Cartesian velocity coordinates [m/s], |
| w | fins height [mm], |
| x, y, z | Cartesian coordinates [m], |
| angle of inclination [], | |
| rate of dissipation of turbulent kinetic energy [m/s], | |
| fluid thermal conductivity [W/(m·K)], vector of adjoint variables, | |
| fluid dynamic viscosity [Pa·s], | |
| fluid density [kg/m] |
References
- Sui, Y.; Teo, C.J.; Lee, P.S.; Chew, Y.T.; Shu, C. Fluid flow and heat transfer in wavy microchannels. Int. J. Heat Mass Trans. 2010, 53, 2760–2772. [Google Scholar] [CrossRef]
- Sivasamy, A.; Selladurai, V.; Kanna, P.R. Mixed convection on jet impingement cooling of a constant heat flux horizontal porous layer. Int. J. Therm. Sci. 2010, 49, 1238–1246. [Google Scholar] [CrossRef]
- Starner, K.E.; McManus, H.N., Jr. An Experimental Investigation of Free-Convection Heat Transfer From Rectangular-Fin Arrays. J. Heat Transf. 1963, 85, 273–277. [Google Scholar] [CrossRef]
- Chaddock, J.B. Free convection heat transfer from vertical rectangular fin arrays. ASHRAE J. 1970, 12, 53–60. [Google Scholar]
- Leung, C.W.; Probert, S.D.; Shilston, M.J. Heat exchanger: Optimal separation for vertical rectangular fins protruding from a vertical rectangular base. Appl. Energy 1985, 19, 77–85. [Google Scholar] [CrossRef]
- Leung, C.W.; Probert, S.D.; Shilston, M.J. Heat exchanger design: Optimal uniform separation between rectangular fins protruding from a vertical rectangular base. Appl. Energy 1985, 19, 287–299. [Google Scholar] [CrossRef]
- Leung, C.W.; Probert, S.D.; Shilston, M.J. Heat transfer performances of vertical rectangular fins protruding from rectangular bases: Effect of fin length. Appl. Energy 1986, 22, 313–318. [Google Scholar] [CrossRef]
- Leung, C.W.; Probert, S.D. Heat-exchanger performance: Effect of orientation. Appl. Energy 1989, 33, 235–252. [Google Scholar] [CrossRef]
- Leung, C.W.; Probert, S.D. Thermal effectiveness of short-protrusion rectangular, heat-exchanger fins. Appl. Energy 1989, 34, 1–8. [Google Scholar] [CrossRef]
- de Lieto Vollaro, A.; Grignaffi, S.; Gugliermetti, F. Optimum design of vertical rectangular fin arrays. Int. J. Therm. Sci. 1999, 38, 525–529. [Google Scholar] [CrossRef]
- Baskaya, S.; Sivrioglu, M.; Ozek, M. Parametric study of natural convection heat transfer from horizontal rectangular fin arrays. Int. J. Therm. Sci. 2000, 39, 797–805. [Google Scholar] [CrossRef]
- Harahap, F.; Setio, D. Correlations for heat dissipation and natural convection heat-transfer from horizontally-based, vertically-finned arrays. Appl. Energy 2001, 69, 29–38. [Google Scholar] [CrossRef]
- Edwards, J.A.; Chaddock, J.B. An experimental investigation of the radiation and free convection heat transfer from a cylindrical disk extended surface. ASHRAE Trans. 1963, 69, 313–322. [Google Scholar]
- Azarkish, H.; Sarvari, S.M.H.; Behzadmehr, A. Optimum geometry design of a longitudinal fin with volumetric heat generation under the influences of natural convection and radiation. Energy Convers. Manag. 2010, 51, 1938–1946. [Google Scholar] [CrossRef]
- Rao, V.D.; Naidu, S.V.; Rao, B.G.; Sharma, K.V. Heat transfer from a horizontal fin array by natural convection and radiation—A conjugate analysis. Int. J. Heat Mass Transf. 2006, 49, 3379–3391. [Google Scholar] [CrossRef]
- Wu, Y.; Gao, N.; Niu, J.; Zang, J.; Caoa, Q. Numerical study on natural ventilation of the wind tower: Effects of combining with different window configurations in a low-rise house. Build. Environ. 2021, 188, 107450. [Google Scholar] [CrossRef]
- Soltani, M.; Dehghani-Sanij, A.; Sayadnia, A.; Kashkooli, F.M.; Gharali, K.; Mahbaz, S.; Dusseault, M.B. Investigation of Airflow Patterns in a New Design of Wind Tower with a Wetted Surface. Energies 2018, 11, 1100. [Google Scholar] [CrossRef] [Green Version]
- Mehryan, S.A.M.; Kashkooli, F.M.; Soltani, M. Comprehensive study of the impacts of surrounding structures on the aero-dynamic performance and flow characteristics of an outdoor unit of split-type air conditioner. Build. Simul. 2018, 11, 325–337. [Google Scholar] [CrossRef]
- Kashkooli, F.M.; Soltani, M.; Zargar, B.; Ijaz, M.K.; Taatizadeh, E.; Sattar, S.A. Analysis of an indoor air decontamination device inside an aerobiology chamber: A numerical-experimental study. Air Qual. Atmos. Health 2020, 13, 281–288. [Google Scholar] [CrossRef]
- Kashkooli, F.M.; Sefidgar, M.; Soltani, M.; Anbari, S.; Shahandashti, S.-A.; Zargar, B. Numerical Assessment of an Air Cleaner Device under Different Working Conditions in an Indoor Environment. Sustainability 2021, 13, 369. [Google Scholar] [CrossRef]
- Xie, Z.; Xiao, Y.; Jiang, C.; Ren, Z.; Li, X.; Yu, K. Numerical research on airflow-dust migration behavior and optimal forced air duct installation position in a subway tunnel during drilling operation. Powder Technol. 2021, 388, 176–191. [Google Scholar] [CrossRef]
- Tari, I.; Mehrtash, M. Natural convection heat transfer from inclined plate-fin heat sinks. Int. J. Heat Mass Transf. 2013, 56, 574–593. [Google Scholar] [CrossRef]
- Kim, T.H.; Kim, D.-K.; Do, K.-H. Correlation for the fin Nusselt number of natural convective heat sinks with vertically oriented plate-fins. Heat Mass Transf. 2013, 49, 413–425. [Google Scholar] [CrossRef]
- Kulkarni, V.M.; Dotihal, B. CFD and conjugate heat transfer analysis of heat sinks with different fin geometries subjected to forced convection used in electronics cooling. Int. J. Res. Eng. Technol. 2013, 4, 158–163. [Google Scholar]
- Maji, A.; Bhanja, D.; Patowari, P.K. Numerical investigation on heat transfer enhancement of heat sink using perforated pin fins with inline and staggered arrangement. Appl. Therm. Eng. 2017, 125, 596–616. [Google Scholar] [CrossRef]
- Shadlaghani, A.; Tavakoli, M.R.; Farzaneh, M.; Salimpour, M.R. Optimization of triangular fins with/without longitudinal perforate for thermal performance enhancement. J. Mech. Sci. Technol. 2016, 30, 1903–1910. [Google Scholar] [CrossRef]
- Al-Sallami, W.; Al-Damook, A.; Thompson, H.M. A numerical investigation of the thermal-hydraulic characteristics of perforated plate fin heat sinks. Int. J. Therm. Sci. 2017, 121, 266–277. [Google Scholar] [CrossRef] [Green Version]
- Saraiya, A.; Chandramohan, V.P.; Balasubramanian, K. Optimization of Horizontal Rectangular Fin Heat Sink: A CFD with Response Surface Analysis and Parametric Study. J. Inst. Eng. India 2020, 101, 149–158. [Google Scholar] [CrossRef]
- Patel, H.; Matawala, V.K. Performance Evaluation and parametric optimization of a Heat Sink for Cooling of Electronic Devices with Entropy Generation Minimization. Eur. J. Sustain. Dev. Res. 2019, 3, em0100. [Google Scholar] [CrossRef]
- Iradukunda, A.-C.; Vargas, A.; Huitink, D.; Lohan, D. Transient thermal performance using phase change material integrated topology optimized heat sinks. Appl. Therm. Eng. 2020, 179, 115723. [Google Scholar] [CrossRef]
- Feppon, F.; Allaire, G.; Dapogny, C.; Jolivet, P. Body-fitted topology optimization of 2D and 3D fluid-to-fluid heat exchangers. Comput. Methods Appl. Mech. Eng. 2021, 376, 113638. [Google Scholar] [CrossRef]
- Sun, S.; Liebersbach, P.; Qian, X. 3D topology optimization of heat sinks for liquid cooling. Appl. Therm. Eng. 2020, 178, 115540. [Google Scholar] [CrossRef]
- Nemati, H.; Moghimi, M.A.; Sapin, P.; Markides, C.N. Shape optimisation of air-cooled finned-tube heat exchangers. Int. J. Therm. Sci. 2020, 150, 106233. [Google Scholar] [CrossRef]
- Menrath, T.; Rosskopf, A.; Simon, F.B.; Groccia, M.; Schuster, S. Shape Optimization of a Pin Fin Heat Sink. In Proceedings of the 36th Semiconductor Thermal Measurement, Modeling & Management Symposium, San Jose, CA, USA, 16–20 March 2020. [Google Scholar]
- Ghosh, S.; Mondal, S.; Fernandez, E.; Kapat, J.S.; Roy, A. Parametric Shape Optimization of Pin-Fin Arrays Using a Surrogate Model-Based Bayesian Method. In Proceedings of the American Institute of Aeronautics and Astronautics AIAA Propulsion and Energy 2019 Forum, Indianapolis, IN, USA, 19–22 August 2019. [Google Scholar]
- Das, S.; Sutradhar, A. Multi-physics topology optimization of functionally graded controllable porous structures: Application to heat dissipating problems. Mater. Des. 2020, 193, 108775. [Google Scholar] [CrossRef]
- Lee, J.S.; Ha, M.Y.; Min, J.K. A finite-volume based topology optimization procedure for an aero-thermal system with a simplified sensitivity analysis method. Int. J. Heat Mass Transf. 2020, 163, 120524. [Google Scholar] [CrossRef]
- Høghøj, L.C.; Nørhave, D.R.; Alexandersen, J.; Sigmund, O.; Andreasen, C.S. Topology optimization of two fluid heat exchangers. Int. J. Heat Mass Transf. 2020, 163, 120543. [Google Scholar] [CrossRef]
- Lee, G.; Lee, I.; Kim, S.J. Topology optimization of a heat sink with an axially uniform cross-section cooled by forced convection. Int. J. Heat Mass Transf. 2021, 168, 120732. [Google Scholar] [CrossRef]
- Zeng, T.; Wang, H.; Yang, M.; Alexandersen, J. Topology optimization of heat sinks for instantaneous chip cooling using a transient pseudo-3D thermofluid model. Int. J. Heat Mass Transf. 2020, 154, 119681. [Google Scholar] [CrossRef] [Green Version]
- Lee, J.S.; Yoon, S.Y.; Kim, B.; Lee, H.; Ha, M.Y.; Min, J.K. A topology optimization based design of a liquid-cooled heat sink with cylindrical pin fins having varying pitch. Int. J. Heat Mass Transf. 2021, 172, 121172. [Google Scholar] [CrossRef]
- Dhar, P.L. Review of Fundamentals. In Thermal System Design and Simulation; Lisa Reading; Academic Press: Cambridge, MA, USA, 2017; p. 129. [Google Scholar]
- Cengel, Y. Natural Convection. In Heat and Mass Transfer: A Practical Approach, 3rd ed.; McGraw-Hill: New York, NY, USA, 2006; p. 467. [Google Scholar]
- Dixit, H.N.; Babu, V. Simulation of high Rayleigh number natural convection in a square cavity using the lattice Boltzmann method. Int. J. Heat Mass Transf. 2006, 49, 727–739. [Google Scholar] [CrossRef]
- Lampio, K. Optimization of Fin Arrays Cooled by Forced or Natural Convection. Ph.D. Thesis, Tampere University of Technology, Tampere, Finland, 2018. [Google Scholar]
- Meng, X.; Zhu, J.; Wei, X.; Yan, Y. Natural convection heat transfer of a straight-fin heat sink. Int. J. Heat Mass Transf. 2018, 123, 561–568. [Google Scholar] [CrossRef]
- ANSYS FLUENT 12.0 User’s Guide. Available online: https://www.afs.enea.it/project/neptunius/docs/fluent/html/th/node116.htm (accessed on 20 December 2020).
- Schramm, M.; Stoevesandt, B.; Peinke, J. Optimization of Airfoils Using the Adjoint Approach and the Influence of Adjoint Turbulent Viscosity. Computation 2018, 6, 5. [Google Scholar] [CrossRef] [Green Version]
- Wang, M.; Wang, Q.; Zaki, T.A. Discrete adjoint of fractional-step incompressible Navier-Stokes solver in curvilinear coordinates and application to data assimilation. J. Comput. Phys. 2019, 369, 427–450. [Google Scholar] [CrossRef]
- Lang, M. CFD-Method for 3D Aerodynamic Adjoint Simulations. Master’s Thesis, Linkoping University, Linkoping, Sweden, 2019. [Google Scholar]
- Roth, R.; Ulbrich, S. A Discrete Adjoint Approach for the Optimization of Unsteady Turbulent Flows. Flow Turbul. Combust. 2013, 90, 763–783. [Google Scholar] [CrossRef]
- Gkaragkounis, K.T. Conjugate Heat Transfer Shape Optimization Based On The Continuous Adjoint Method. In Proceedings of the International VII International Conference on Computational Methods for Coupled Problems in Science and Engineering (COUPLED 2017), Rhodes Island, Greece, 12–14 June 2017. [Google Scholar]
- Sen, A.; Towara, M.; Naumann, U. Sensitivity computation for ducted flows using adjoint of implicit pressure-velocity coupled solver based on Foam. In Proceedings of the Eurogen, Glasgow, UK, 14–16 September 2015. [Google Scholar]
- Tzanakis, A. Duct optimization using CFD software ‘ANSYS Fluent Adjoint Solver’. Master’s Thesis, Chalmers University of Technology, Goteborg, Sweden, 2014. [Google Scholar]
- Plutecki, Z.; Sattler, P.; Ryszczyk, K. Innowacyjny proces projektowania i optymalizacji produktów z wykorzystaniem solvera Adjoint. In Proceedings of the Innowacje w Zarządzaniu i inżYnierii Produkcji, Zakopane, Poland, 1–3 March 2015; Volume 18. [Google Scholar]
- Kalinowski, M.; Szczepanik, M. Aerodynamic Shape Optimization of Racing Car Front Wing; IOP Conference Series: Materials Science and Engineering; IOP Publishing Ltd.: Bristol, UK, 2021; Volume 1037. [Google Scholar]
- Day, H.; Ingham, D.; Ma, L.; Pourkashanian, M. Adjoint based optimisation for efficient VAWT blade aerodynamics using CFD. J. Wind Eng. Ind. Aerodyn. 2021, 208, 104431. [Google Scholar] [CrossRef]
- Towara, M.; Lotz, J.; Naumann, U. Discrete adjoint approaches for CHT applications in OpenFOAM. Comput. Methods Appl. Sci. 2021, 55, 163–178. [Google Scholar]
- Rana, R.A. Numerical and Experimental Study on Orientation Dependency of Free Convection Heat Sinks. Master’s Thesis, The University of British Columbia, Okangan, BC, Canada, 2015. [Google Scholar]





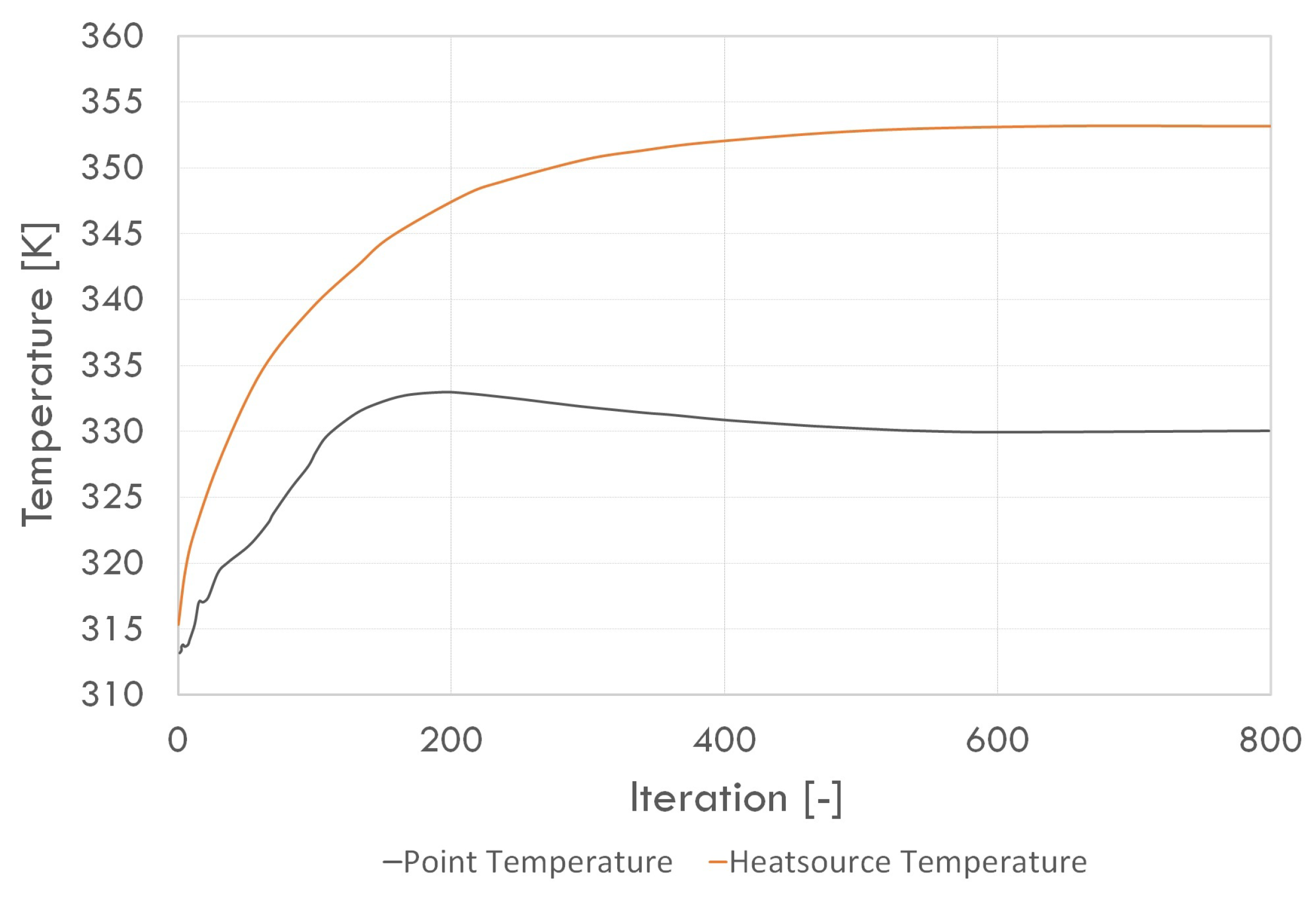

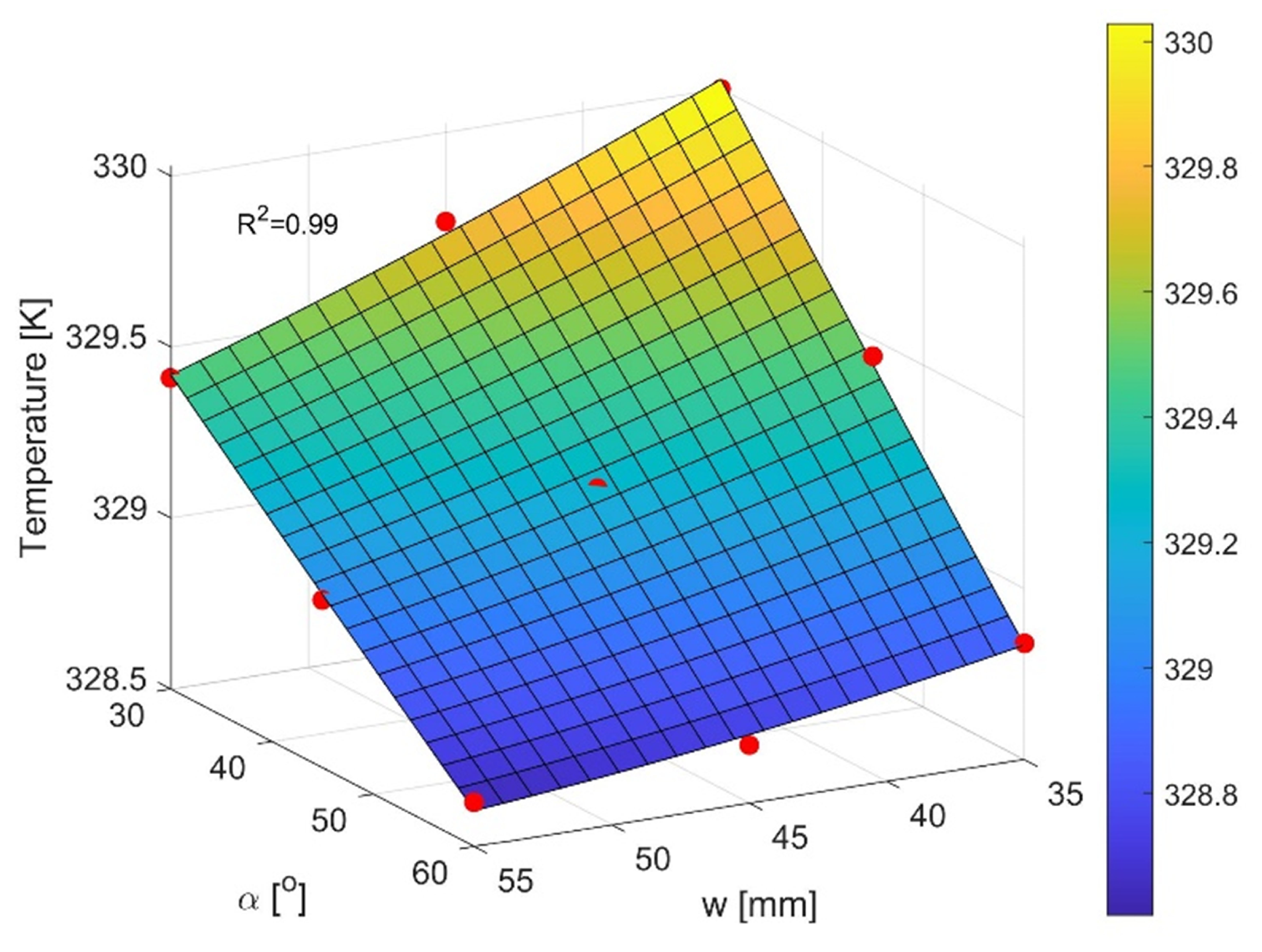
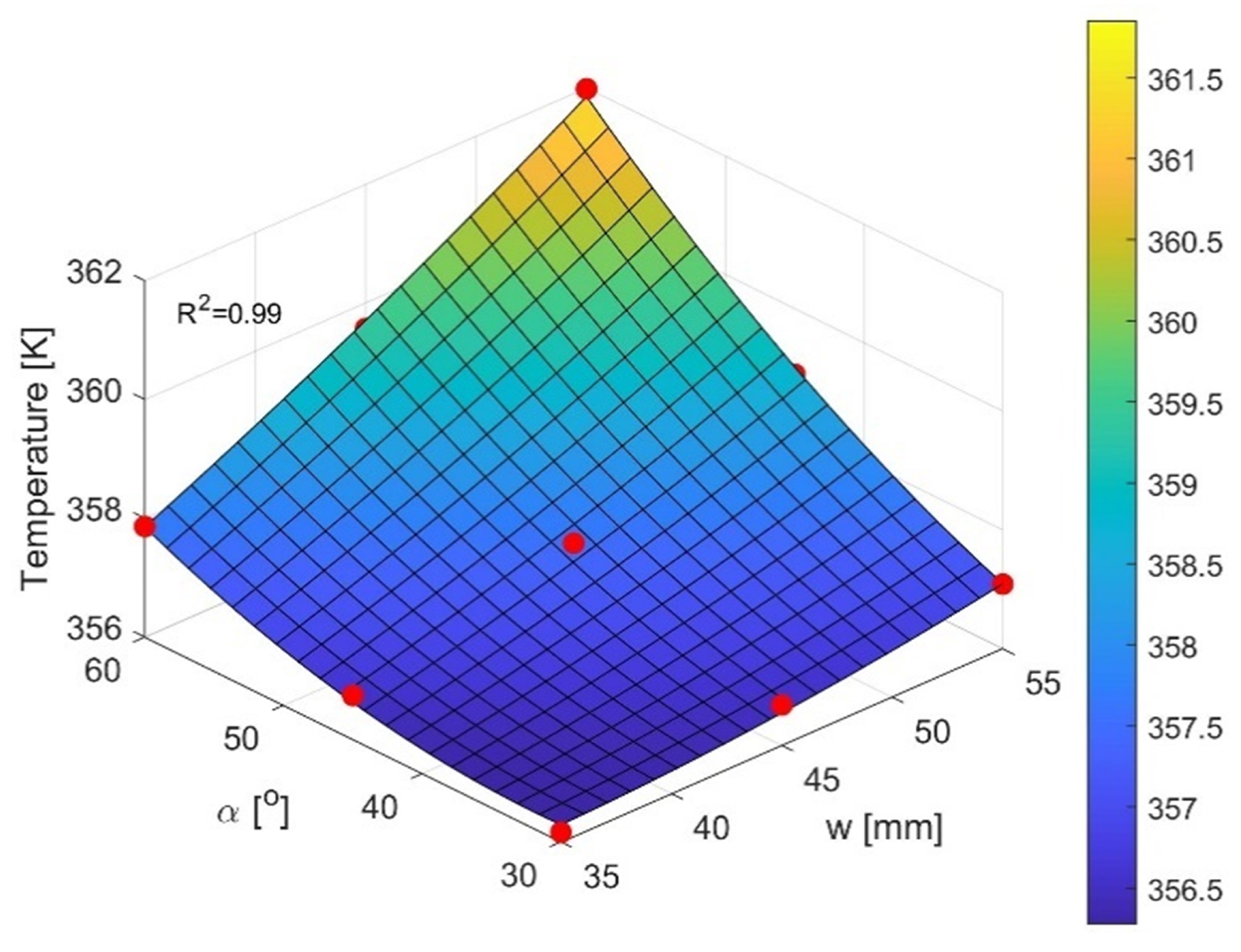

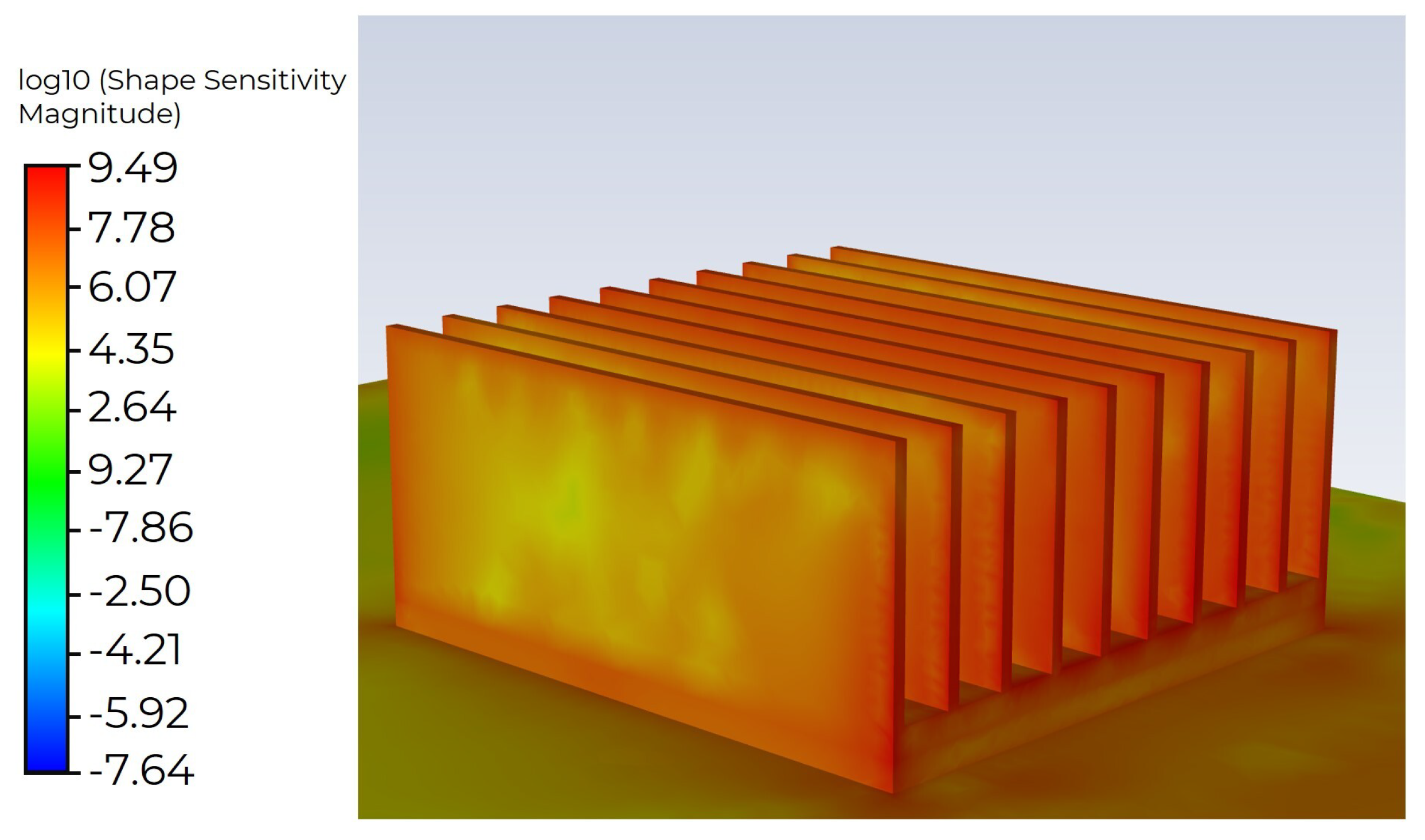


| No. | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
|---|---|---|---|---|---|---|---|---|---|
| w [mm] | 55 | 55 | 55 | 45 | 45 | 45 | 35 | 35 | 35 |
| [] | 30 | 45 | 60 | 30 | 45 | 60 | 30 | 45 | 60 |
| Part of Domain | Material | Density | Specific Heat | Thermal Conductivity |
|---|---|---|---|---|
| [kg/m] | c [J/(kg·K)] | [W/(m·K)] | ||
| solid_heat sink | copper | 8978 | 381 | 387.6 |
| solid_base | fr-4 | 381 | 1300 | 795 |
| solid_heatsource | component | 387.6 | 0.35 | 10 |
| No. | Local_1 | Minimum Size | Maximum Size | Number of Elements | min OQ |
|---|---|---|---|---|---|
| [mm] | [mm] | [mm] | [-] | [-] | |
| 1 | 3.0 | 2.50 | 25 | 486,382 | 0.1129 |
| 2 | 3.5 | 1.17 | 25 | 379,152 | 0.1017 |
| 3 | 4.5 | 2.50 | 25 | 342,902 | 0.1011 |
| 4 | 6.0 | 2.50 | 50 | 312,676 | 0.1499 |
| 5 | 7.0 | 2.50 | 60 | 286,189 | 0.1500 |
| Power [W] | Heat Generation [W/m] |
|---|---|
| 30 | 156,584 |
| 40 | 208,779 |
| 50 | 260,974 |
| 60 | 313,169 |
| 70 | 365,364 |
| 80 | 417,558 |
| 90 | 469,753 |
| 100 | 521,948 |
| 110 | 574,143 |
| 120 | 626,337 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Czerwiński, G.; Wołoszyn, J. Optimization of Air Cooling System Using Adjoint Solver Technique. Energies 2021, 14, 3753. https://doi.org/10.3390/en14133753
Czerwiński G, Wołoszyn J. Optimization of Air Cooling System Using Adjoint Solver Technique. Energies. 2021; 14(13):3753. https://doi.org/10.3390/en14133753
Chicago/Turabian StyleCzerwiński, Grzegorz, and Jerzy Wołoszyn. 2021. "Optimization of Air Cooling System Using Adjoint Solver Technique" Energies 14, no. 13: 3753. https://doi.org/10.3390/en14133753
APA StyleCzerwiński, G., & Wołoszyn, J. (2021). Optimization of Air Cooling System Using Adjoint Solver Technique. Energies, 14(13), 3753. https://doi.org/10.3390/en14133753






