Single Well Productivity Prediction Model for Fracture-Vuggy Reservoir Based on Selected Seismic Attributes
Abstract
:1. Introduction
2. Construction of Productivity Prediction Model for Fracture-Vuggy Reservoir
- (1)
- The reservoir is infinitely homogeneous and has isotropic permeability;
- (2)
- The well is produced at constant pressure;
- (3)
- The reservoir fluid is single phase;
- (4)
- The seepage law satisfies Darcy’s law and the compressibility coefficient is constant.
- ;
3. Productivity Prediction Method for a Single Well
4. Comparison with Other Methods
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Li, L. Study of Numerical Simulation in Carbonate Fracture-Vuggy Reservoir with Multi-Scale Features. Ph.D. Thesis, Southwest Petroleum University, Chengdu, China, 2013. [Google Scholar]
- Zhang, L.; Li, C.; Zhao, Y. Review on the Seepage Mechanisms of Oil and Gas Flow in Fractured Carbonate Reservoirs. Earth Sci. 2017, 42, 1273. [Google Scholar]
- Fan, J. Characteristics of carbonate reservoirs for oil and gas fields in the world and essential controlling factors for their formation. Earth Sci. Front. 2005, 12, 23–30. [Google Scholar]
- Zhang, K. The discovery of tahe oilfifld and its. Oil Gas Geol. 1999, 20, 120–124. [Google Scholar]
- Wang, J.P.; Shen, A.J.; Cai, X.Y.; Luo, Y.C.; Li, Y. A review of the ordovician carbonate reservoirs in the world. J. Stratigr. 2008, 32, 363–373. [Google Scholar]
- Wang, G. Geological model of carbonate reservoirs in Tahe oil field. Pet. Explor. Dev. 2002, 29, 109–111. [Google Scholar]
- Hou, J. Modelling of carbonate fracture-vuggy reservoir: A case study of Ordovician reservoir of 4th block in Tahe Oilfield. Earth Sci. Front. 2012, 19, 59–66. [Google Scholar]
- Zhang, H. Study on prediction of connected multiple fractured acid fracturing of horizontal well productivity. Pet. Geol. Recovery Effic. 2011, 18, 86–89. [Google Scholar]
- Zhao, Y. Flow rate and pressure variation modeling for production wells in fractured-vuggy carbonates reservoirs. Oil Gas Geol. 2010, 31, 54–56. [Google Scholar]
- Wang, Y. Discussion on deliverability evaluation method of fractured-vuggy carbonate reservoir. Fault Block Oil Gas Field 2011, 18, 637–640. [Google Scholar]
- Han, G.H.; Qi, L.X.; Li, Z.J. Prediction of the Ordovician fractured-vuggy carbonate reservoirs in Tahe oilfield. Oil Gas Geol. 2006, 27, 860–870. [Google Scholar]
- Wan, L. Study on Multiple Regression Analysis in Prediction of Tight Oil Reservoir and Its Application. Well Test. 2015, 24, 17–19. [Google Scholar]
- Yu, Y. Productivity analysis of single well in fractured-vuggy reservoir. Fault Block Oil Gas Field 2014, 21, 746–749. [Google Scholar]
- Warren, J.E.; Root, P.J. The Behavior of Naturally Fractured Reservoirs. Soc. Pet. Eng. J. 1963, 3, 245–255. [Google Scholar] [CrossRef] [Green Version]
- Gilman, J.R.; Kazemi, H. Improvements in Simulation of Naturally Fractured Reservoirs. Soc. Pet. Eng. J. 1982, 23, 4. [Google Scholar] [CrossRef]
- Kasap, E.; Lake, L.W. Calculating the Effective Permeability Tensor of a Gridblock. SPE Form. Eval. 1990, 5, 192–200. [Google Scholar] [CrossRef]
- Lee, S.H.; Durlofsky, L.J.; Lough, M.F.; Chen, W.H. Finite Difference Simulation of Geologically Complex Reservoirs with Tensor Permeabilities. SPE Res. Eval. Eng. 1998, 1, 567–574. [Google Scholar] [CrossRef]
- Abdassah, D.; Ershaghi, I. Triple-Porosity Systems for Representing Naturally Fractured Reservoirs. SPE Form. Eval. 1986, 1, 113–127. [Google Scholar] [CrossRef]
- Nelson, R.A. Geologic Analysis of Naturally Fractured Reservoirs. Geologic Analysis of Naturally Fractured Reservoirs; Elsevier: Amsterdam, The Netherlands, 1985; pp. xix–xx. [Google Scholar]
- Zhang, S. An Efficient Calculation of Equivalent Permeability of Fractured Porous Media Based on Discrete Fracture Model. Sci. Technol. Eng. 2014, 14, 36–40. [Google Scholar]
- Liu, J. The Equivalent Continuum Media Model of Fracture Sand Stone Reservoir. J. Chongqing Univ. 2000, 23, 158–161. [Google Scholar]
- Feng, J. A Theoretical Model of Steady-State Flow for Naturally Fractured Low-Permeability Reservoir. Xinjiang Pet. Geol. 2006, 27, 316–318. [Google Scholar]
- Junrui, C. Discussion on Seepage through Continuum and Seepage through Non-continuum. Hongshui River 2002, 21, 43–45. [Google Scholar]
- Hunt, A.G.; Sahimi, M. Flow, Transport, and Reaction in Porous Media: Percolation Scaling, Critical-Path Analysis, and Effective Medium Approximation. Rev. Geophys. 2017, 55, 993–1078. [Google Scholar] [CrossRef]
- Parteli, R.; Silva, L.J., Jr. Self-organized percolation in multi-layered structures. J. Stat. Mech. Theory Exp. 2010, 2010, P03026. [Google Scholar] [CrossRef] [Green Version]
- Guo, J. Geological Characteristics and Formation Mechanism of Karst Reservoir Seismic Reflection in Tazhong Area. J. Chongqing Univ. Sci. Technol. 2012, 2, 29–32. [Google Scholar]
- Liu, X. Seismic Reflection Characteristics of high Efficient Wells in Carbonate Reservoirs in Western Tazhong Area, Tarim Basin. Xinjiang Pet. Geol. 2011, 32, 97–100. [Google Scholar]
- Xu, H. Discuss on Relationship between “Beaded” Seismic Reflection and “Beaded” Model at Well Test Analysis. Well Test. 2015, 24, 6–9. [Google Scholar]
- Wu, Y.; Ge, J. The transient flow in naturally fractured reservoirs with three-porosity systems. Acta Mech. Sin. 1983, 14, 81–84. [Google Scholar]
- Lang, Y. Application of Neural-Network Prediction to Pre-fracturing Evaluation of Shaximiao Reservoir, Xinchang Area. Nat. Gas Technol. 2012, 6, 27–29. [Google Scholar]
- Cristianini, N.J.; Shawe-Taylor, J. An Introduction to Support Vector Machines and Other Kernel-Based Learning Methods: Preface; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
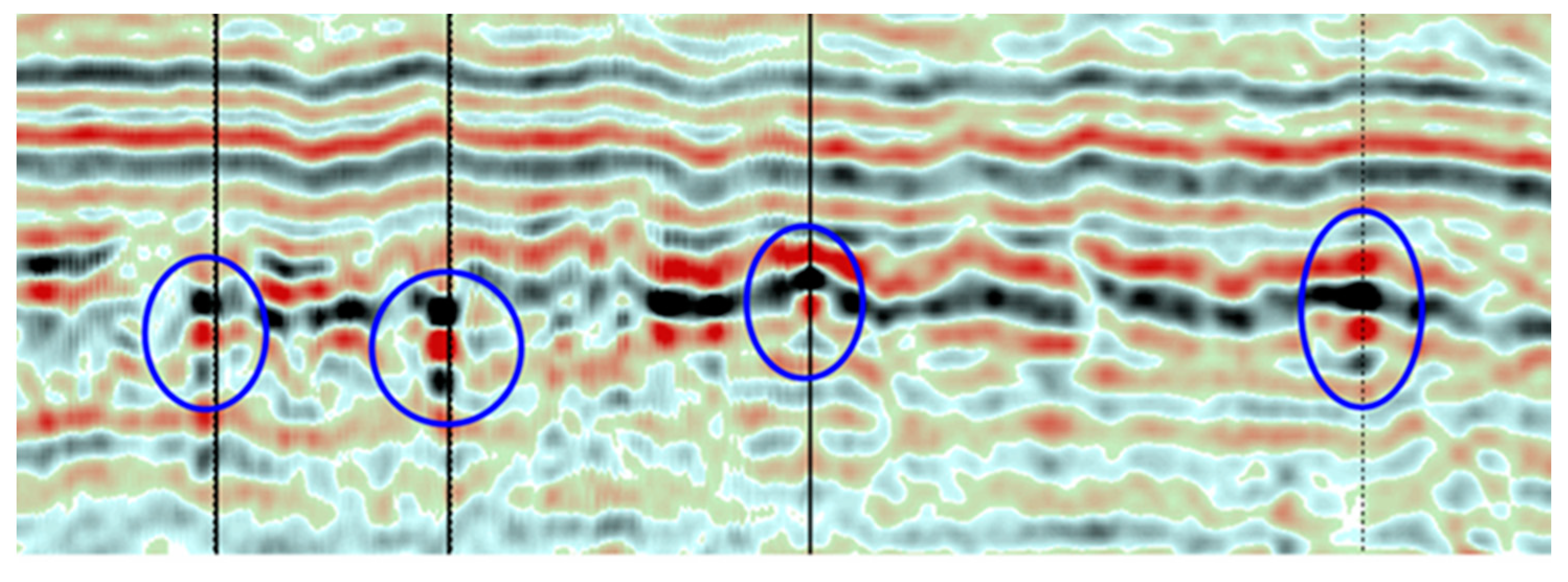

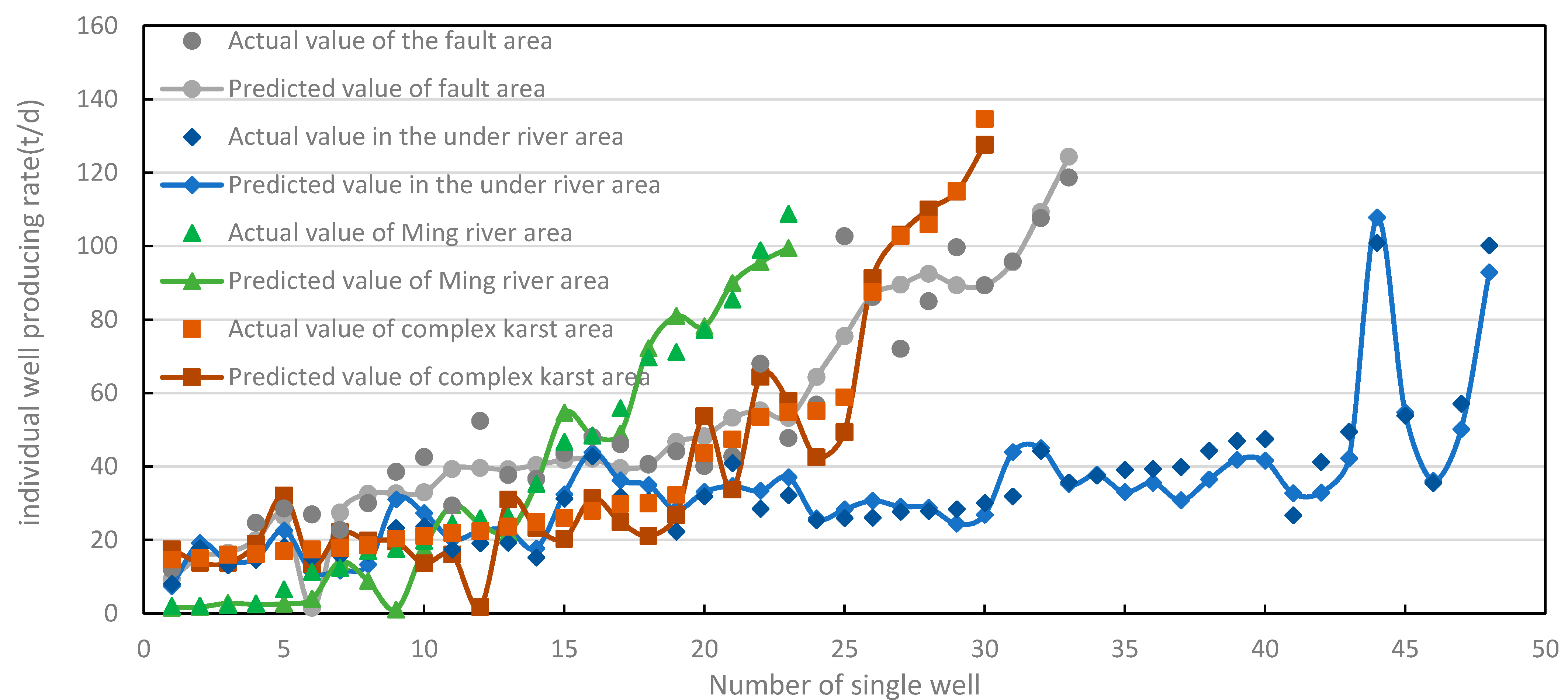
| Individual Well Producing Rate | Correlation Coefficient | X1 | X2 | X3 | X4 | X5 | X6 | X7 |
|---|---|---|---|---|---|---|---|---|
| fault area | Pearson | −0.401 | −0.095 | −0.064 | 0.718 | 0.692 | 0.294 | −0.222 |
| Spearman | −0.473 | −0.132 | 0.037 | 0.676 | 0.475 | 0.434 | −0.278 | |
| under river area | Pearson | −0.11 | 0.116 | −0.247 | 0.875 | 0.853 | 0.815 | 0.012 |
| Spearman | −0.113 | 0.139 | −0.167 | 0.725 | 0.743 | 0.745 | 0.001 | |
| Ming river area | Pearson | −0.11 | 0.116 | −0.247 | 0.875 | 0.853 | 0.815 | 0.012 |
| Spearman | −0.113 | 0.139 | −0.167 | 0.725 | 0.743 | 0.745 | 0.001 | |
| complex karst area | Pearson | −0.38 | 0.805 | 0.75 | 0.829 | 0.527 | −0.354 | 0.000 |
| Spearman | −0.411 | 0.791 | 0.612 | 0.737 | 0.237 | −0.335 | 0.000 |
| fault area | X1 | distance | Ming river area | X1 | distance |
| X2 | RMS | X2 | RMS | ||
| X3 | frequency attenuation coefficient | X3 | Frequency attenuation coefficient | ||
| X4 | beaded area | X4 | beaded area | ||
| X5 | rate of amplitude change | X5 | rate of amplitude change | ||
| X6 | sweet spot minimum | X6 | sweet spot minimum | ||
| X7 | sweet spot maximum | X7 | sweet spot maximum | ||
| under river area | X1 | distance | complex karst area | X1 | RMS |
| X2 | RMS | X2 | frequency attenuation coefficient | ||
| X3 | frequency attenuation coefficient | X3 | beaded area | ||
| X4 | beaded area | X4 | rate of amplitude change | ||
| X5 | rate of amplitude change | X5 | sweet spot minimum | ||
| X6 | sweet spot minimum | X6 | sweet spot maximum | ||
| X7 | sweet spot maximum |
| Area | Parameter | Estimated Value | Parameter | Estimated Value |
|---|---|---|---|---|
| fault area | a1 | −2.50015 | c1 | 1.090775 |
| a2 | −13.2065 | c2 | −1.92896 | |
| a3 | 20.33426 | c3 | −85.7551 | |
| b1 | 0.047811 | d1 | −0.00572 | |
| b2 | 0.060151 | d2 | −0.00717 | |
| b3 | 0.008436 | d3 | −0.00086 | |
| under river area | a1 | −2.50015 | c1 | 1.0907751 |
| a2 | −13.2065 | c2 | −1.928956 | |
| a3 | 20.33426 | c3 | −85.75511 | |
| b1 | 0.047811 | d1 | −0.005718 | |
| b2 | 0.060151 | d2 | −0.007175 | |
| b3 | 0.008436 | d3 | −0.000859 | |
| Ming river area | a1 | 3.29385 | c1 | 59.76628 |
| a2 | −0.05509 | c2 | 3.13136 | |
| a3 | −3.80572 | c3 | 5.747533 | |
| b1 | 3.732016 | d1 | −0.75274 | |
| b2 | 1.359635 | d2 | −0.19553 | |
| b3 | −2.70759 | d3 | 0.55742 | |
| complex karst area | a1 | 3.29385 | c1 | 59.76628 |
| a2 | −0.05509 | c2 | 3.13136 | |
| a3 | −3.80572 | c3 | 5.747533 | |
| b1 | 3.732016 | d1 | −0.75274 | |
| b2 | 1.359635 | d2 | −0.19553 | |
| b3 | −2.70759 | d3 | 0.55742 |
| Oil Region | Precision | Oil Region | Precision | ||
|---|---|---|---|---|---|
| fault area | multiple linear regression | 78.05% | under river area | multiple linear regression | 69.17% |
| BP neural network | 62% | BP neural network | 70.95% | ||
| support vector machine | 72.25% | support vector machine | 82.46% | ||
| multivariate nonlinear regression | 85% | multivariate nonlinear regression | 87% | ||
| Ming river area | multiple linear regression | 75.57% | complex karst area | multiple linear regression | 73.78% |
| BP neural network | 75.57% | BP neural network | 71.08% | ||
| support vector machine | 72% | support vector machine | 77.50% | ||
| multivariate nonlinear regression | 80% | multivariate nonlinear regression | 80% | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.; Wang, S.; Yu, C.; Liu, H. Single Well Productivity Prediction Model for Fracture-Vuggy Reservoir Based on Selected Seismic Attributes. Energies 2021, 14, 4134. https://doi.org/10.3390/en14144134
Wang S, Wang S, Yu C, Liu H. Single Well Productivity Prediction Model for Fracture-Vuggy Reservoir Based on Selected Seismic Attributes. Energies. 2021; 14(14):4134. https://doi.org/10.3390/en14144134
Chicago/Turabian StyleWang, Shuozhen, Shuoliang Wang, Chunlei Yu, and Haifeng Liu. 2021. "Single Well Productivity Prediction Model for Fracture-Vuggy Reservoir Based on Selected Seismic Attributes" Energies 14, no. 14: 4134. https://doi.org/10.3390/en14144134
APA StyleWang, S., Wang, S., Yu, C., & Liu, H. (2021). Single Well Productivity Prediction Model for Fracture-Vuggy Reservoir Based on Selected Seismic Attributes. Energies, 14(14), 4134. https://doi.org/10.3390/en14144134





