Torque Analysis for Rotational Devices with Nonmagnetic Rotor Driven by Magnetic Fluid Filled in Air Gap
Abstract
:1. Introduction
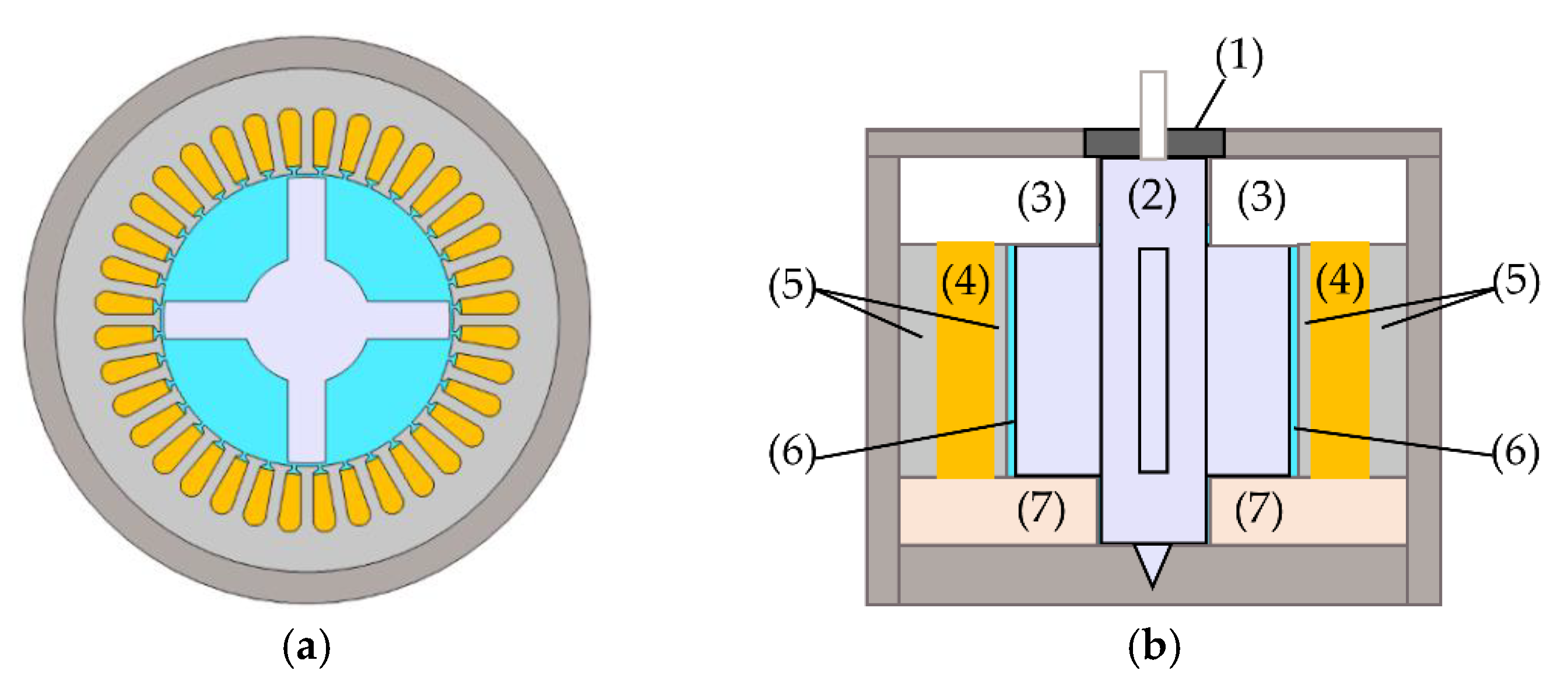
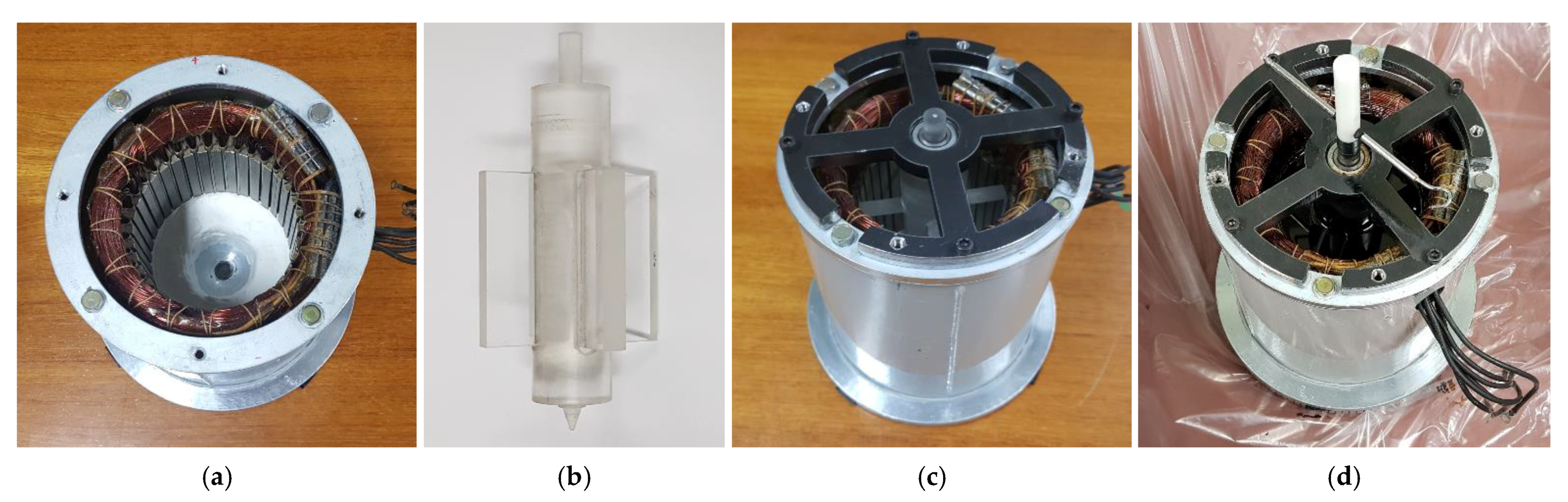
2. Structure of the Experimental Motor for Analysis
3. Controversies of Magnetic Force Density Formula in Magnetic Fluid
- where is the density.
- is the fluid velocity vector.
- is the pressure of fluid.
- is the magnetic force density vector.
- is the first coefficient of viscosity.
- is the local acceleration vector due to gravity.
- where is the magnetic induction field vector.
- is the magnetic field intensity vector.
- is the free current density vector.
- is the permeability of vacuum.
- is the magnetization vector.
4. Virtual Work Principle for Torque Analysis
- where, when the rotor angle is ,
- is the rotor torque by pressure of the magnetic fluid at .
- the subscript means to fix the free current density on all spaces.
- is the total magnetic co-energy:

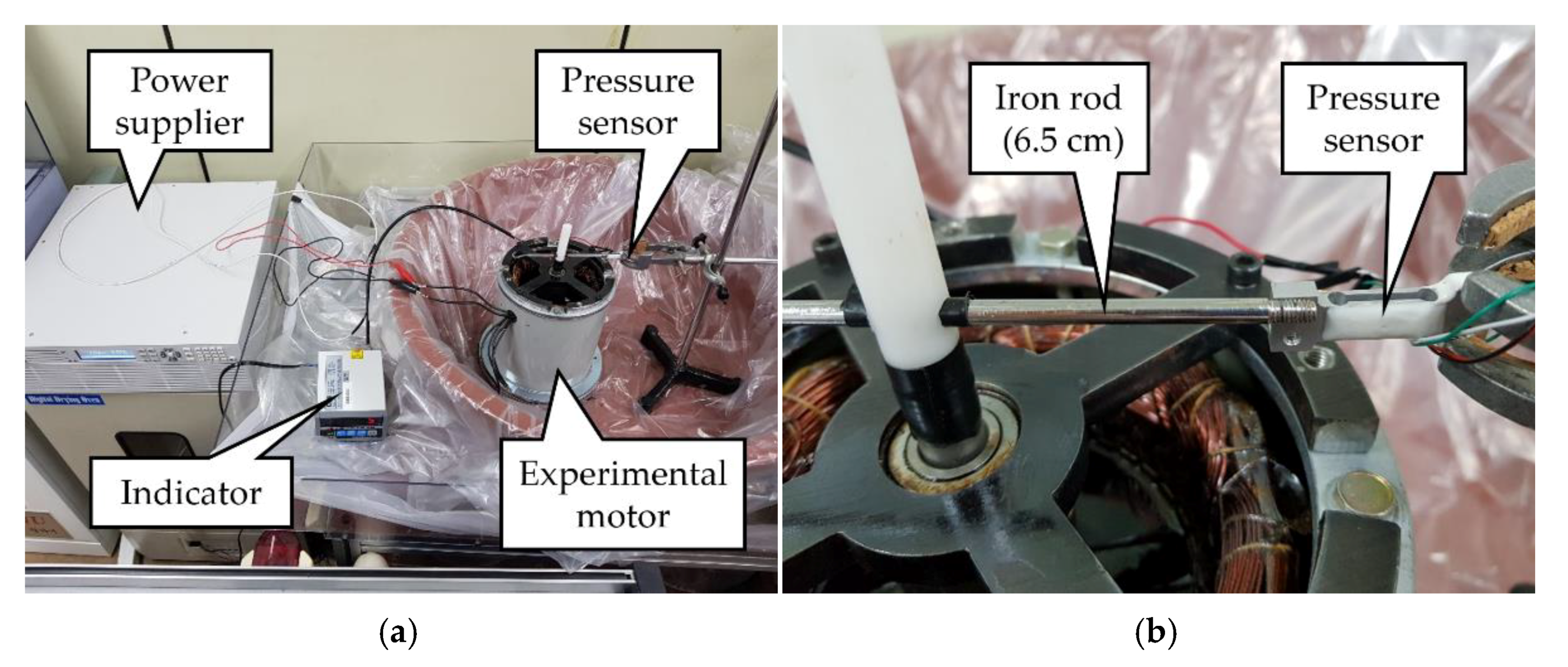
5. Numerical Analysis and Experiment
6. Discussion
6.1. The Significance of Results
6.2. Additional Derivations for Stator and Magnetic Fluid
- where, when the rotor angle is ,
- is the total rotor torque.
- is the rotor torque by its magnetic force.
- is the rotor torque by pressure of the magnetic fluid.
- where, when the rotor angle is ,
- is the total stator torque.
- is the stator torque by its magnetic force.
- is the stator torque by pressure of the magnetic fluid.
- can also be obtained by the virtual work principle, which applies the virtual rotation to the stator; however, if the coordinate system is fixed to the stator, it turns out that the virtual rotation of the stator is the same operation that differs only toward the virtual rotation of the rotor. So,
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| magnetic induction field vector (T) | |
| magnetic force density vector (N/m3) | |
| local acceleration vector due to gravity (m/s2) | |
| magnetic field intensity vector (A/m) | |
| free current density vector (A/m2) | |
| magnetization vector (T) | |
| pressure (N/m2) | |
| fluid velocity vector (m/s) | |
| all spaces (m3) | |
| space occupied by (m3) | |
| total magnetic co-energy (J) | |
| total gravitational energy (J) | |
| Greek letters | |
| first coefficient of viscosity ((N/m2)∙s) | |
| rotor angle (rad) | |
| permeability of vacuum (N/A2) | |
| density (Kg/m3) | |
| magnetic fluid torque by its magnetic force (N∙m) | |
| total rotor torque (N∙m) | |
| rotor torque by its magnetic force (N∙m) | |
| rotor torque by pressure of the magnetic fluid (N∙m) | |
| total stator torque (N∙m) | |
| stator torque by its magnetic force (N∙m) | |
| stator torque by pressure of the magnetic fluid (N∙m) | |
| Subscript | |
| fix free current density in all spaces |
Appendix A
| Quantity | Value | Quantity | Value |
|---|---|---|---|
| Phase number | 3 | Stack length | 81 mm |
| Pole number | 4 | Stator outer diameter | 160 mm |
| Slot number | 36 | Stator inner diameter | 93 mm |
| Area per slot | 108 mm2 | Rotor outer diameter | 91 mm |
| Turn number | 48 | Air gap length | 1 mm |
| Parallel number | No | Rotor axis diameter | 40 mm |
| Phase resistive | 3.3 Ω | Rotor wing width | 12 mm |
| Quantity | Value | Quantity | Value |
|---|---|---|---|
| Magnetic fluid initial susceptibility 1 | 2.2 | Core initial Susceptibility 2 | 322 |
| Magnetic fluid saturation magnetization 1 | 0.04 T | Core saturation Magnetization 2 | 1.7 T |
Appendix B

- where at the position vector of surface ,
- is the magnetic flux density on the side of material 1.
- is the magnetic flux density on the side of material 2.
- is the magnetic field intensity on the side of material 1.
- is the magnetic field intensity on the side of material 2.
- is the normal vector.
- is the tangent vector.
References
- Rosensweig, R.E. Ferrohydrodynamics; Dover Publications, Inc.: Mineola, NY, USA, 1997. [Google Scholar]
- Raj, K.; Moskowitz, B.; Casciari, R. Advances in ferrofluid technology. J. Magn. Magn. Mater. 1995, 149, 174–180. [Google Scholar] [CrossRef]
- Scherer, C.; Neto, A.M.F. Ferrofluids: Properties and applications. Braz. J. Phys. 2005, 35, 718–727. [Google Scholar] [CrossRef]
- Torres-Diaz, I.; Rinaldi, C. Recent progress in ferrofluids research: Novel applications of magnetically controllable and tunable fluids. Soft Matter 2014, 10, 8584–8602. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Sun, L.; Yu, Y.; Zhao, Y. Flexible Ferrofluids: Design and Applications. Adv. Mater. 2019, 31, e1903497. [Google Scholar] [CrossRef] [PubMed]
- Sohail, A.; Fatima, M.; Ellahi, R.; Akram, K.B. A videographic assessment of ferrofluid during magnetic drug targeting: An application of artificial intelligence in nanomedicine. J. Mol. Liq. 2019, 285, 47–57. [Google Scholar] [CrossRef]
- Mody, V.V.; Cox, A.G.; Shah, S.; Singh, A.; Bevins, W.; Parihar, H. Magnetic nanoparticle drug delivery systems for targeting tumor. Appl. Nanosci. 2013, 4, 385–392. [Google Scholar] [CrossRef] [Green Version]
- Hatch, A.; Kamholz, A.; Holman, G.; Yager, P.; Bohringer, K. A ferrofluidic magnetic micropump. J. Microelectromech. Syst. 2001, 10, 215–221. [Google Scholar] [CrossRef] [Green Version]
- Chitnis, G.; Ziaie, B. A ferrofluid-based wireless pressure sensor. J. Micromech. Microeng. 2013, 23. [Google Scholar] [CrossRef]
- Kurtoğlu, E.; Bilgin, A.; Şeşen, M.; Mısırlıoğlu, B.; Yıldız, M.; Acar, H.F.Y.; Koşar, A. Ferrofluids actuation with varying magnetic fluids for micropumping applications. Microfluid. Nanofluid. 2012, 13, 683–694. [Google Scholar] [CrossRef]
- Engelmann, S.; Nethe, A.; Scholz, T.h.; Stahlmann, H.-D. Concept of a new type of electric machines using ferrofluids. J. Magn. Magn. Mater. 2005, 293, 685–689. [Google Scholar] [CrossRef]
- Zeng, G.; Xiang-Yu, Y.; Yin, H.; Pei, Y.; Zhao, S.; Cao, J.; Qiu, L. Asynchronous Machine with Ferrofluid in Gap: Modeling, Simulation, and Analysis. IEEE Trans. Magn. 2019, 56, 1–4. [Google Scholar] [CrossRef]
- Engelmann, S.; Nethe, A.; Scholz, T.; Stahlmann, H.-D. Experiments with a ferrofluid-supported linear electric motor. Appl. Organomet. Chem. 2004, 18, 529–531. [Google Scholar] [CrossRef]
- Yang, I.-J.; Song, S.-W.; Kim, D.-H.; Kim, K.-S.; Kim, W.-H. Improvement in Torque Density by Ferrofluid Injection into Magnet Tolerance of Interior Permanent Magnet Synchronous Motor. Energies 2021, 14, 1736. [Google Scholar] [CrossRef]
- Livens, G.H. The Theory of Electricity; Cambridge University Press: Cambridge, UK, 1918; pp. 212–220. [Google Scholar]
- Stratton, J.A. Electromagnetic Theory; McGraw-Hill: New York, NY, USA, 2015. [Google Scholar] [CrossRef]
- Chu, L.; Haus, H.; Penfield, P. The force density in polarizable and magnetizable fluids. Proc. IEEE 1966, 54, 920–935. [Google Scholar] [CrossRef] [Green Version]
- Landau, L.D.; Pitaevskii, L.P.; Lifshitz, E.M. Electrodynamics of Continuous Media, 2nd ed.; Butterworth-Heinemann: Oxford, UK, 1984; p. 127. [Google Scholar]
- Mansuripur, M. Electromagnetic stress tensor in ponderable media. Opt. Express 2008, 16, 5193–5198. [Google Scholar] [CrossRef] [Green Version]
- Shevchenko, A.; Hoenders, B.J. Microscopic derivation of electromagnetic force density in magnetic dielectric media. New J. Phys. 2010, 12. [Google Scholar] [CrossRef]
- Penfield, P.; Haus, H.A. Electrodynamics of Moving Media; The MIT Press: Cambridge, MA, USA, 1968; pp. 75–77. [Google Scholar] [CrossRef] [Green Version]
- Bobbio, S. Electrodynamics of Materials; Academic Press: San Diego, CA, USA, 2000. [Google Scholar]
- Odenbach, S.; Liu, M. Invalidation of the Kelvin Force in Ferrofluids. Phys. Rev. Lett. 2001, 86, 328–331. [Google Scholar] [CrossRef] [Green Version]
- Rinaldi, C.; Brenner, H. Body versus surface forces in continuum mechanics: Is the Maxwell stress tensor a physically objective Cauchy stress? Phys. Rev. E 2003, 65, 036615. [Google Scholar] [CrossRef]
- Bethune-Waddell, M.; Chau, K.J. Simulations of radiation pressure experiments narrow down the energy and momentum of light in matter. Rep. Prog. Phys. 2015, 78, 122401. [Google Scholar] [CrossRef]
- Mansuripur, M. Force, torque, linear momentum, and angular momentum in classical electrodynamics. Appl. Phys. A 2017, 123, 653. [Google Scholar] [CrossRef]
- Reich, F.A.; Rickert, W.; Müller, W.H. An investigation into electromagnetic force models: Differences in global and local effects demonstrated by selected problems. Contin. Mech. Thermodyn. 2017, 30, 233–266. [Google Scholar] [CrossRef]
- Panofsky, W.K.H.; Phillips, M.; Morse, P.M. Classical Electricity and Magnetism; Dover Publications, Inc.: Mineola, NY, USA, 1956; pp. 22–24. [Google Scholar] [CrossRef] [Green Version]
- Reichet, K.; Freundl, H.; Vogt, W. The calculation of forces and torques within numerical magnetic field calculation methods. Proc. Compumag. 1976, 76, 64–74. [Google Scholar]
- Bobbio, S.; Girdinio, P.; Molfino, P.; Delfino, F. Equivalent sources methods for the numerical evaluation of magnetic force with extension to nonlinear materials. IEEE Trans. Magn. 2000, 36, 663–666. [Google Scholar] [CrossRef]
- Kabashima, T.; Kawahara, A.; Goto, T. Force calculation using magnetizing currents. IEEE Trans. Magn. 1988, 24, 451–454. [Google Scholar] [CrossRef]
- Coulomb, J.C.; Meunier, G.; Sabonnadiere, J.C. An original stationary method using local jacobian derivative for direct finite element computation of electromechanical force, torque and siftness. J. Magn. Magn. Mater. 1982, 26, 337–339. [Google Scholar] [CrossRef]
- Reddy, J.N. Energy Principles and Variational Methods in Applied Mechanics, 3rd ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2017; p. 131. [Google Scholar]
- Jiles, D. Introduction to Magnetism and Magnetic Materials, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2015; pp. 99–102. [Google Scholar]
- Choi, H.-S.; Park, I.-H.; Lee, S.-H. Electromagnetic body force calculation based on virtual air gap. J. Appl. Phys. 2006, 99, 08H903. [Google Scholar] [CrossRef]
- Choi, H.S.; Kim, Y.S.; Lee, J.H.; Park, I.H. An Observation of Body Force Distributions in Electric Machines. IEEE Trans. Magn. 2007, 43, 1861–1864. [Google Scholar] [CrossRef]
- Choi, H.S.; Park, I.H.; Lee, S.H. Concept of virtual air gap and its applications for force calculation. IEEE Trans. Magn. 2006, 42, 663–666. [Google Scholar] [CrossRef]
- Choi, H.S.; Park, I.H.; Lee, S.H. Force Calculation of Magnetized Bodies in Contact Using Kelvin’s Formula and Virtual Air-Gap. IEEE Trans. Appl. Supercond. 2006, 16, 1832–1835. [Google Scholar] [CrossRef]
- Choi, H.S.; Lee, S.H.; Kim, Y.S.; Kim, K.T.; Park, I.H. Implementation of Virtual Work Principle in Virtual Air Gap. IEEE Trans. Magn. 2008, 44, 1286–1289. [Google Scholar] [CrossRef]
- Park, B.S.; Choi, H.S.; Park, J.O.; Wang, J.H.; Park, I.H. Equality of the Kelvin and Korteweg–Helmholtz Force Densities Inside Dielectric Materials. IEEE Trans. Magn. 2020, 56, 4. [Google Scholar] [CrossRef]
- Kemp, B.A. Resolution of the Abraham-Minkowski debate: Implications for the electromagnetic wave theory of light in matter. J. Appl. Phys. 2011, 109, 111101. [Google Scholar] [CrossRef]
- Frias, W.; Smolyakov, A.I. Electromagnetic forces and internal stresses in dielectric media. Phys. Rev. E 2012, 85, 046606. [Google Scholar] [CrossRef] [PubMed]
- Mahdy, M.R.C.; Gao, D.; Ding, W.; Mehmood, M.Q.; Nieto-Vesperinas, M.; Qui, C.-W. A unified theory correcting Einstein-Laub electrodynamics solves dilemmas in the photon momenta and electromagnetic stress tensors. arXiv 2015, arXiv:1509.06971. [Google Scholar]
- Kemp, B.A.; Sheppard, C.J. Electromagnetic and material contributions to stress, energy, and momentum in metamaterials. Adv. Electromagn. 2017, 6, 11–19. [Google Scholar] [CrossRef] [Green Version]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, G.-H.; Choi, H.-S. Torque Analysis for Rotational Devices with Nonmagnetic Rotor Driven by Magnetic Fluid Filled in Air Gap. Energies 2021, 14, 4669. https://doi.org/10.3390/en14154669
Kim G-H, Choi H-S. Torque Analysis for Rotational Devices with Nonmagnetic Rotor Driven by Magnetic Fluid Filled in Air Gap. Energies. 2021; 14(15):4669. https://doi.org/10.3390/en14154669
Chicago/Turabian StyleKim, Gui-Hwan, and Hong-Soon Choi. 2021. "Torque Analysis for Rotational Devices with Nonmagnetic Rotor Driven by Magnetic Fluid Filled in Air Gap" Energies 14, no. 15: 4669. https://doi.org/10.3390/en14154669
APA StyleKim, G.-H., & Choi, H.-S. (2021). Torque Analysis for Rotational Devices with Nonmagnetic Rotor Driven by Magnetic Fluid Filled in Air Gap. Energies, 14(15), 4669. https://doi.org/10.3390/en14154669






