Experimental Investigation on Flow Boiling Heat Transfer and Pressure Drop of a Low-GWP Refrigerant R1234ze(E) in a Horizontal Minichannel
Abstract
:1. Introduction
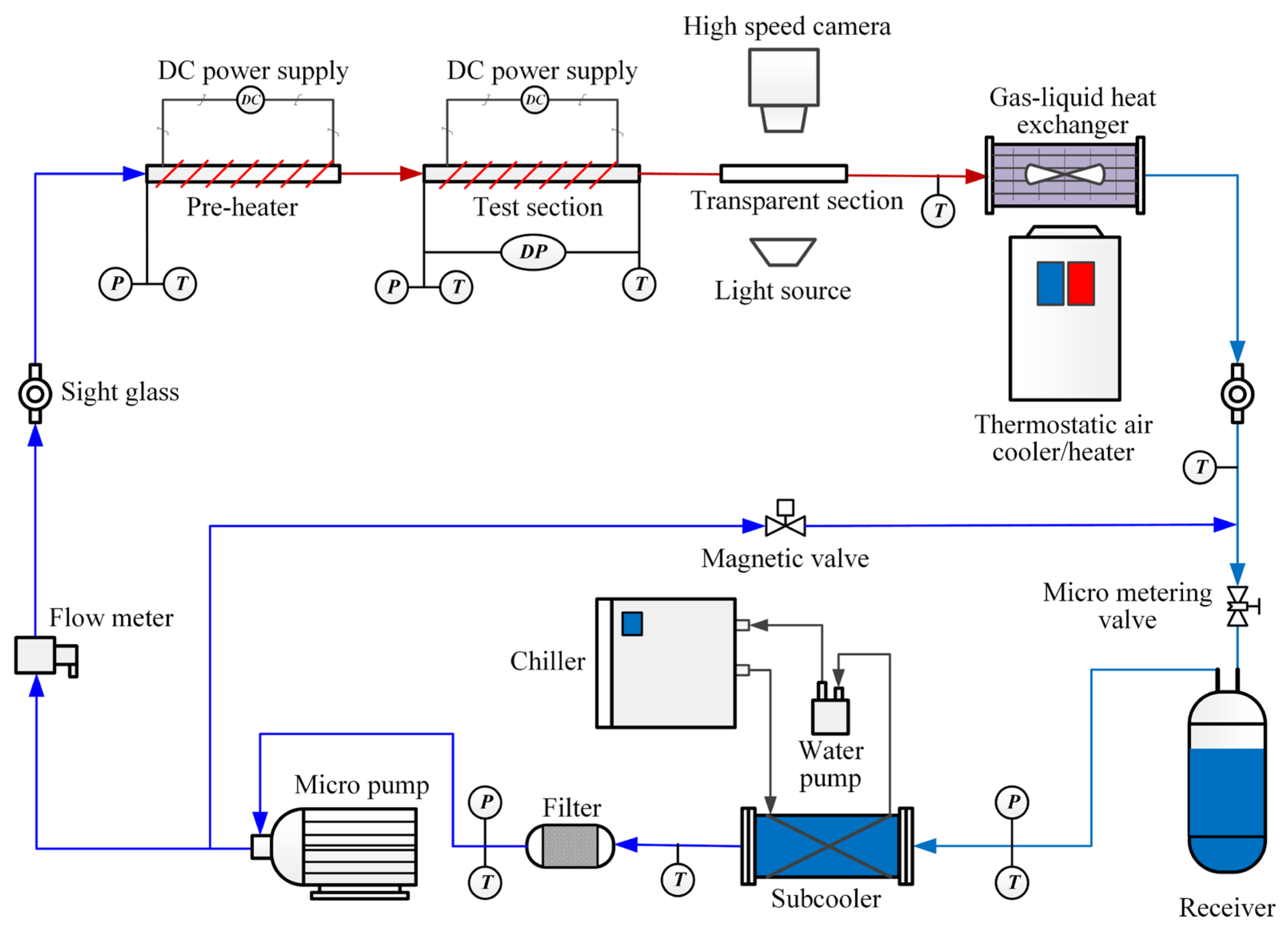
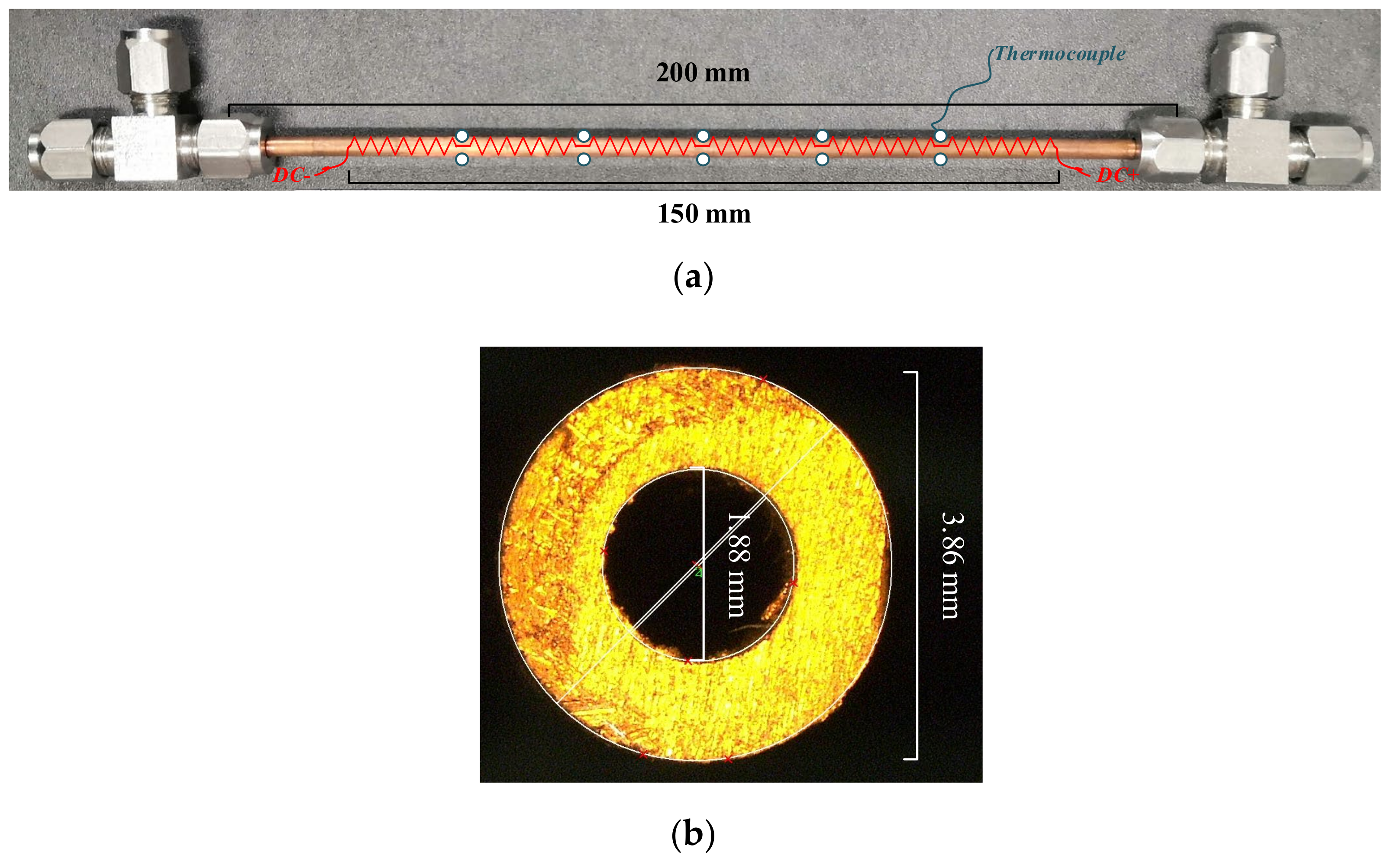
2. Experimental Apparatus
3. Data Reduction and Uncertainty Analysis
4. Experimental Results and Discussion
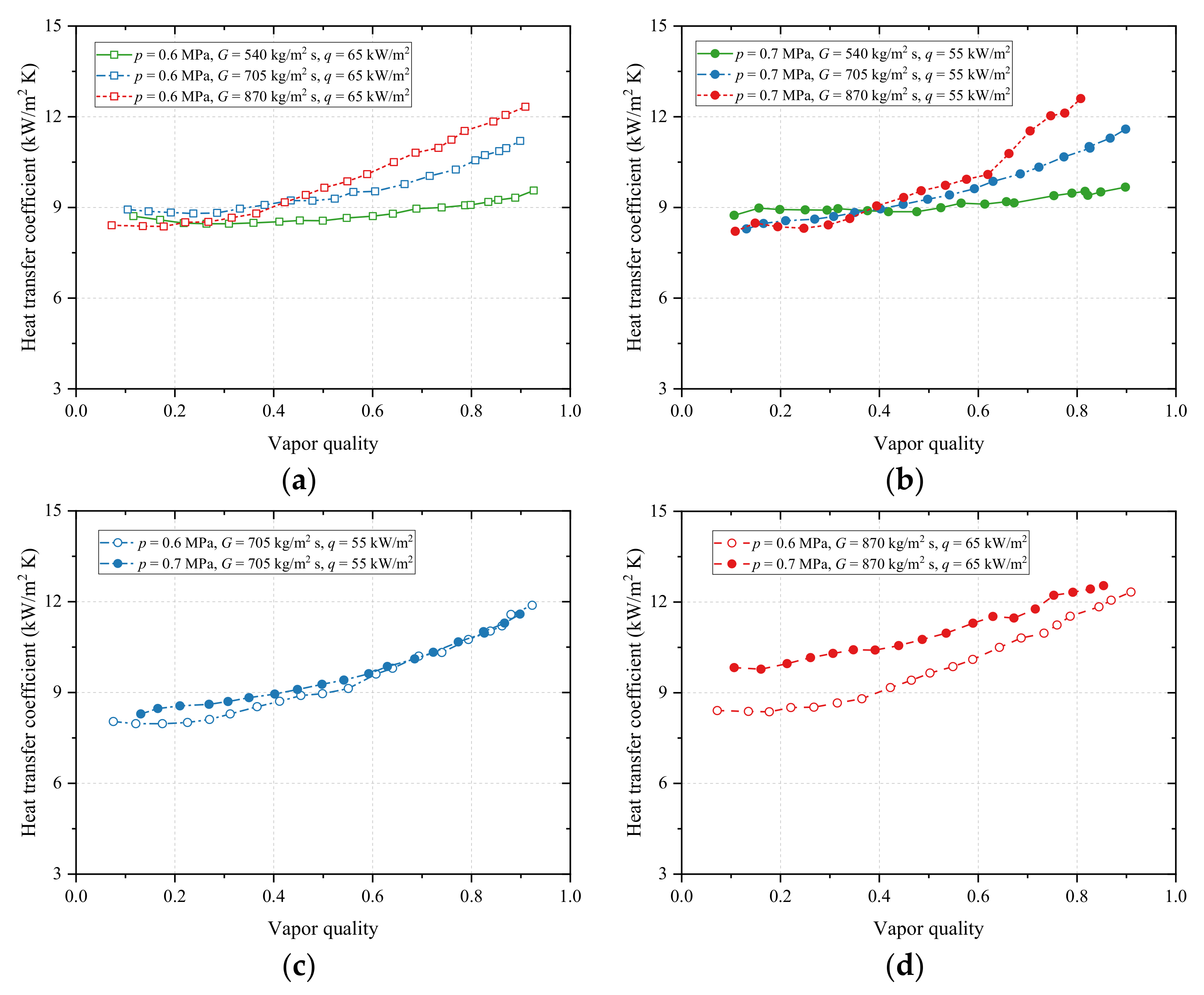
4.1. Flow Boiling HTC of R1234ze(E)
4.2. Comparison with HTC Correlations
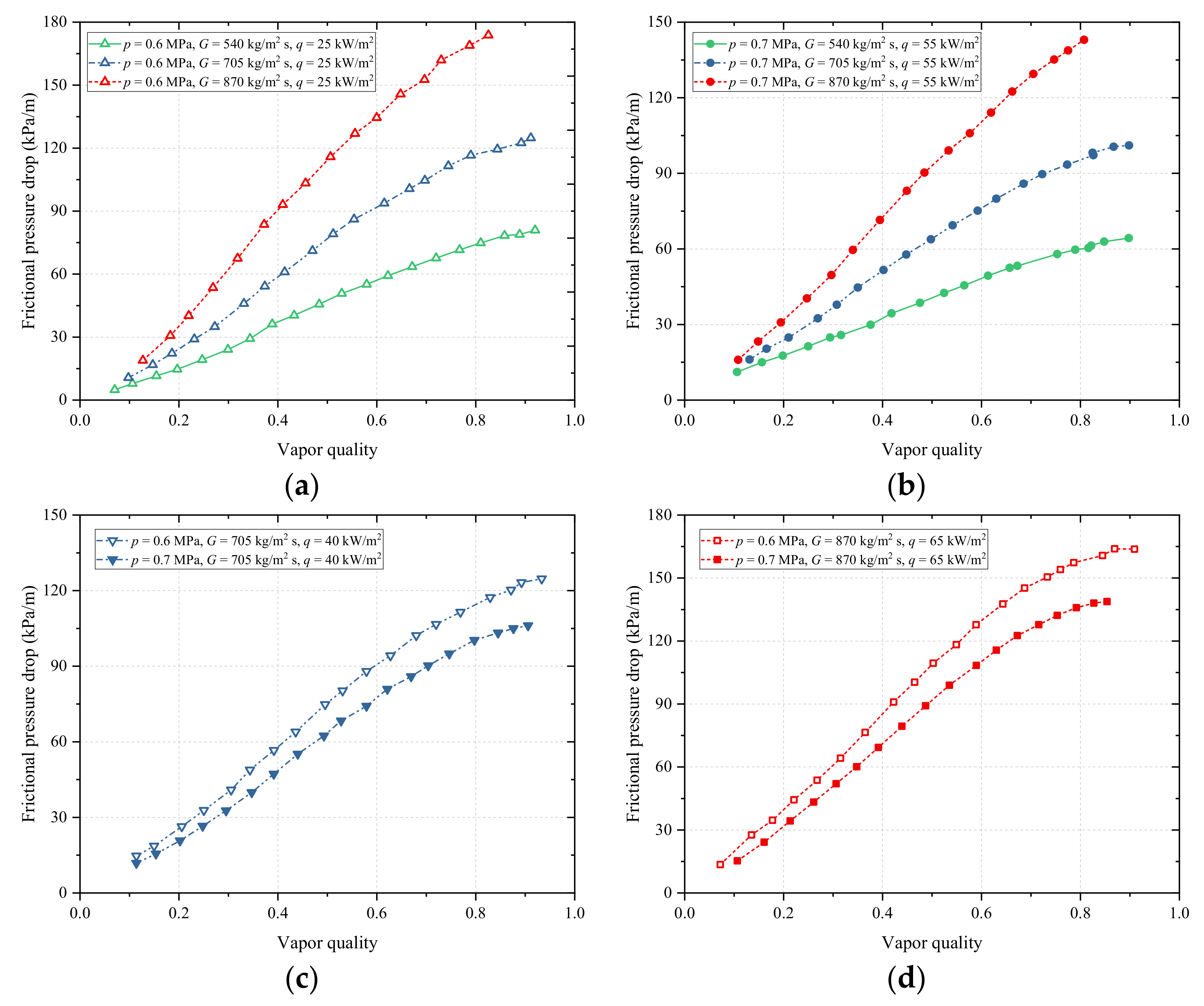
4.3. Flow Boiling FPD of R1234ze(E)
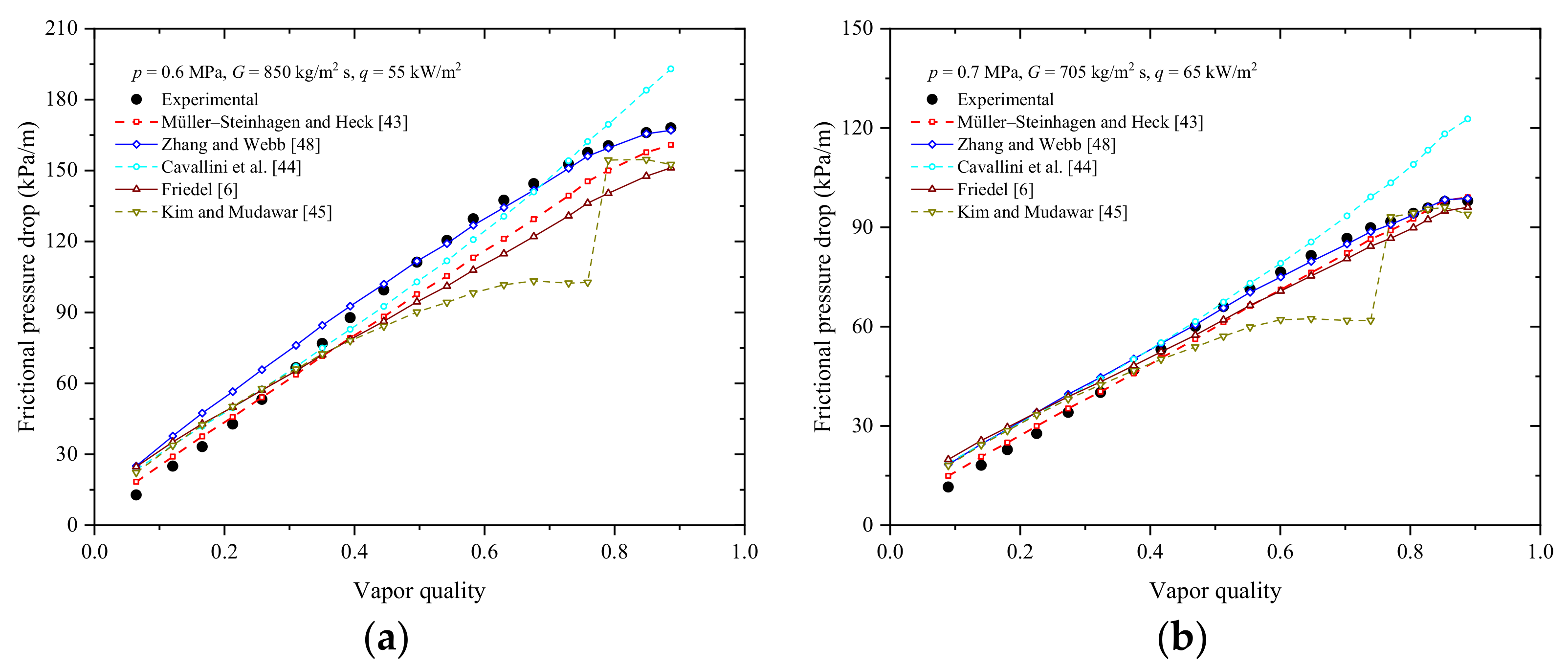
4.4. Comparison with FPD Correlations
5. Conclusions
- (1)
- The R1234ze(E) flow boiling HTC and FPD inside a 1.88 mm horizontal circular minichannel was investigated experimentally. The saturation pressure, mass flux, and heat flux fell in the scopes of 0.6–0.7 MPa, 540–870 kg/m2 s, and 25–65 kW/m2, respectively.
- (2)
- The influences of various factors on flow boiling HTC and FPD are analyzed thoroughly. The nucleate boiling and convective boiling dominate the lower and higher vapor quality regions with a threshold vapor quality nearby 0.4. HTC mainly rises with heat flux for nucleate boiling, but increases with mass flux and vapor quality for convective boiling. HTC rises with saturation pressure, especially at lower vapor quality. Larger FPD is found for larger mass flux and vapor quality, but smaller saturation pressure. FPD is not sensitive to heat flux in most conditions.
- (3)
- Twelve existing correlations of two-phase HTC and ten ones of two-phase FPD are compared with the current experimental data, respectively. The correlations of Saitoh et al. [15] and Müller-Steinhagen and Heck [43] yield the best predictions and tracking performances on the flow boiling HTC and FPD with MADs of 5.4% and 10.9%, respectively. The reasonable prediction results indicate that the previous correlations suggested for R134a are mostly effective for R1234ze(E), as the thermophysical properties of R1234ze (E) are similar to those of R134a and their flow boiling characteristics are close.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| A | Sectional area (m2) |
| Ac | Cross sectional aera of fin (m) |
| Cc | Contraction coefficient (–) |
| Di | Internal diameter (m) |
| Dh | Hydraulic diameter (m) |
| Do | Outer diameter (m) |
| Fr | Froude number |
| G | Mass flux (kg/m2 s) |
| g | Gravitational acceleration (m/s2) |
| h | Enthalpy (J/kg) |
| H | Fin length (m) |
| H′ | Modified fin length (m) |
| hlv | Latent heat of vaporization (J/kg) |
| L | Test section length (m) |
| Lheat | Heating length (m) |
| L′heat | Modified heating length (m) |
| m | Fin parameter (–) |
| n | Data point number |
| p | Pressure (Pa) |
| P | Cross-sectional perimeter of fin (m) |
| pr | Reduced pressure (–) |
| q | Heat flux (W/m2) |
| Qdis | Heat dissipation (W) |
| Qeff | Effective heating power (W) |
| r | Radius (m) |
| Re | Reynolds number |
| t | Temperature (°C) |
| tw | Inner wall temperature (°C) |
| tw,o | Outer wall temperature (°C) |
| V | Volume flow rate (m3/s) |
| x | Vapor quality |
| Δp | Pressure drop (Pa) |
| Greek Symbols | |
| α | Heat transfer coefficient (W/m2 K) |
| δ | Fin thickness (m) |
| ε | Void fraction (–) |
| ηf | Fin efficiency (–) |
| ρ | Density (kg/m3) |
| λ | Thermal conductivity (W/m K) |
| σc | Contraction area ratio (–) |
| σe | Expansion area ratio (–) |
| Subscripts | |
| exp | Experimental |
| in | Inlet |
| ins | Insulating layer |
| l | Liquid |
| out | Outlet |
| pre | Pre-heater |
| pred | Predicted |
| sat | Saturation |
| t | Total |
| test | Test section |
| v | Vapor |
References
- Diani, A.; Mancin, S.; Rossetto, L. R1234ze(E) flow boiling inside a 3.4 mm ID microfin tube. Int. J. Refrig. 2014, 47, 105–119. [Google Scholar] [CrossRef]
- Longo, G.A.; Mancin, S.; Righetti, G.; Zilio, C. R1234yf and R1234ze(E) as environmentally friendly replacements of R134a: Assessing flow boiling on an experimental basis. Int. J. Refrig. 2019, 108, 336–346. [Google Scholar] [CrossRef]
- Longo, G.A.; Mancin, S.; Righetti, G.; Zilio, C. Saturated flow boiling of HFC134a and its low GWP substitute HFO1234ze(E) inside a 4 mm horizontal smooth tube. Int. J. Refrig. 2016, 64, 32–39. [Google Scholar] [CrossRef]
- Diani, A.; Mancin, S.; Cavallini, A.; Rossetto, L. Experimental investigation of R1234ze(E) flow boiling inside a 2.4 mm ID horizontal microfin tube. Int. J. Refrig. 2016, 69, 272–284. [Google Scholar] [CrossRef]
- Kim, S.-M.; Mudawar, I. Review of databases and predictive methods for heat transfer in condensing and boiling mini/micro-channel flows. Int. J. Heat Mass Transf. 2014, 77, 627–652. [Google Scholar] [CrossRef]
- Friedel, L. Improved friction pressure drop correlations for horizontal and vertical two phase pipe flow. In Proceedings of the European Two-Phase Flow Group Meeting, Ispra, Italy, 5–8 June 1979; pp. 485–491. [Google Scholar]
- Bortolin, S.; Bortolato, M.; Azzolin, M.; Del Col, D. Comparative experimental procedures for measuring the local heat transfer coefficient during flow boiling in a microchannel. Exp. Therm. Fluid Sci. 2018, 90, 231–245. [Google Scholar] [CrossRef]
- Sun, L.; Mishima, K. An evaluation of prediction methods for saturated flow boiling heat transfer in mini-channels. Int. J. Heat Mass Transf. 2009, 52, 5323–5329. [Google Scholar] [CrossRef]
- Bertsch, S.S.; Groll, E.A.; Garimella, S.V. A composite heat transfer correlation for saturated flow boiling in small channels. Int. J. Heat Mass Transf. 2009, 52, 2110–2118. [Google Scholar] [CrossRef] [Green Version]
- Sempértegui-Tapia, D.F.; Ribatski, G. Flow boiling heat transfer of R134a and low GWP refrigerants in a horizontal micro-scale channel. Int. J. Heat Mass Transf. 2017, 108, 2417–2432. [Google Scholar] [CrossRef]
- Kanizawa, F.T.; Tibiriçá, C.B.; Ribatski, G. Heat transfer during convective boiling inside microchannels. Int. J. Heat Mass Transf. 2016, 93, 566–583. [Google Scholar] [CrossRef]
- Kim, S.M.; Mudawar, I. Universal approach to predicting saturated flow boiling heat transfer in mini/micro-channels—Part II. Two-phase heat transfer coefficient. Int. J. Heat Mass Transf. 2013, 64, 1239–1256. [Google Scholar] [CrossRef]
- Jige, D.; Kikuchi, S.; Eda, H.; Inoue, N. Flow boiling in horizontal multiport tube: Development of new heat transfer model for rectangular minichannels. Int. J. Heat Mass Transf. 2019, 144, 118668. [Google Scholar] [CrossRef]
- Gungor, K.E.; Winterton, R.H.S. A general correlation for flow boiling in tubes and annuli. Int. J. Heat Mass Transf. 1986, 29, 351–358. [Google Scholar] [CrossRef]
- Saitoh, S.; Daiguji, H.; Hihara, E. Correlation for boiling heat transfer of R-134a in horizontal tubes including effect of tube diameter. Int. J. Heat Mass Transf. 2007, 50, 5215–5225. [Google Scholar] [CrossRef]
- Kim, S.-M.; Mudawar, I. Universal approach to predicting saturated flow boiling heat transfer in mini/micro-channels—Part I. Dryout incipience quality. Int. J. Heat Mass Transf. 2013, 64, 1226–1238. [Google Scholar] [CrossRef]
- Vakili-Farahani, F.; Agostini, B.; Thome, J.R. Experimental study on flow boiling heat transfer of multiport tubes with R245fa and R1234ze(E). Int. J. Refrig. 2013, 36, 335–352. [Google Scholar] [CrossRef]
- Thome, J.R.; Dupont, V.; Jacobi, A.M. Heat transfer model for evaporation in microchannels. Part I: Presentation of the model. Int. J. Heat Mass Transf. 2004, 47, 3375–3385. [Google Scholar] [CrossRef]
- Li, H.; Hrnjak, P. Heat transfer coefficient, pressure drop, and flow patterns of R1234ze(E) evaporating in microchannel tube. Int. J. Heat Mass Transf. 2019, 138, 1368–1386. [Google Scholar] [CrossRef]
- Mancin, S.; Diani, A.; Doretti, L.; Rossetto, L. R134a and R1234ze(E) liquid and flow boiling heat transfer in a high porosity copper foam. Int. J. Heat Mass Transf. 2014, 74, 77–87. [Google Scholar] [CrossRef]
- Diani, A.; Mancin, S.; Doretti, L.; Rossetto, L. Low-GWP refrigerants flow boiling heat transfer in a 5 PPI copper foam. Int. J. Multiph. Flow 2015, 76, 111–121. [Google Scholar] [CrossRef]
- Xu, Y.; Fang, X. Correlations of void fraction for two-phase refrigerant flow in pipes. Appl. Therm. Eng. 2014, 64, 242–251. [Google Scholar] [CrossRef]
- Collier, J.G.; Thome, J.R. Convective Boiling and Condensation; Oxford University Press: New York, NY, USA, 1994. [Google Scholar]
- Fu, B.-R.; Tsou, M.-S.; Pan, C. Flow-pattern-based correlations for pressure drop during flow boiling of ethanol-water mixtures in a microchannel. Int. J. Heat Mass Transf. 2013, 61, 332–339. [Google Scholar] [CrossRef]
- Kim, S.-M.; Mudawar, I. Consolidated method to predicting pressure drop and heat transfer coefficient for both subcooled and saturated flow boiling in micro-channel heat sinks. Int. J. Heat Mass Transf. 2012, 55, 3720–3731. [Google Scholar] [CrossRef]
- Schmidt, J.; Friedel, L. Two-phase pressure drop across sudden contractions in duct areas. Int. J. Multiph. Flow 1997, 23, 283–299. [Google Scholar] [CrossRef]
- Abdelall, F.F.; Hahn, G.; Ghiaasiaan, S.M.; Abdel-Khalik, S.I.; Jeter, S.S.; Yoda, M.; Sadowski, D.L. Pressure drop caused by abrupt flow area changes in small channels. Exp. Therm. Fluid Sci. 2005, 29, 425–434. [Google Scholar] [CrossRef]
- Kawahara, A.; Chung, P.M.Y.; Kawaji, M. Investigation of two-phase flow pattern, void fraction and pressure drop in a microchannel. Int. J. Multiph. Flow 2002, 28, 1411–1435. [Google Scholar] [CrossRef]
- Kline, S.J.; McClintock, F.A. Describing Uncertainties in Single-Sample Experiments. Mech. Eng. 1953, 75, 3–8. [Google Scholar]
- Belyaev, A.V.; Varava, A.N.; Dedov, A.V.; Komov, A.T. An experimental study of flow boiling in minichannels at high reduced pressure. Int. J. Heat Mass Transf. 2017, 110, 360–373. [Google Scholar] [CrossRef]
- Xu, Y.; Fang, X.; Li, G.; Li, D.; Yuan, Y. An experimental study of flow boiling heat transfer of R134a and evaluation of existing correlations. Int. J. Heat Mass Transf. 2016, 92, 1143–1157. [Google Scholar] [CrossRef]
- Fang, X. A new correlation of flow boiling heat transfer coefficients based on R134a data. Int. J. Heat Mass Transf. 2013, 66, 279–283. [Google Scholar] [CrossRef]
- Wattelet, J.P.; Chato, J.C.; Souza, A.L.; Christoffersen, B.R. Evaporative characteristics of R-12, R-134a, and a mixture at low mass fluxes. ASHRAE Trans. 1994, 100, 603–615. [Google Scholar]
- Gungor, K.E.; Winterton, R.H.S. Simplified general correlation for saturated flow boiling and comparisons of correlations with data. Chem. Eng. Res. Des. 1987, 65, 148–156. [Google Scholar]
- Shah, M.M. Chart correlation for saturated boiling heat transfer: Equations and further study. ASHRAE Trans. 1982, 88, 185–196. [Google Scholar]
- Liu, Z.; Winterton, R.H.S. A general correlation for saturated and subcooled flow boiling in tubes and annuli, based on a nucleate pool boiling equation. Int. J. Heat Mass Transf. 1991, 34, 2759–2766. [Google Scholar] [CrossRef]
- Jung, D.S.; McLinden, M.; Radermacher, R.; Didion, D. A study of flow boiling heat transfer with refrigerant mixtures. Int. J. Heat Mass Transf. 1989, 32, 1751–1764. [Google Scholar] [CrossRef]
- Li, W.; Wu, Z. A general criterion for evaporative heat transfer in micro/mini-channels. Int. J. Heat Mass Transf. 2010, 53, 1967–1976. [Google Scholar] [CrossRef]
- Tran, T.N.; Wambsganss, M.W.; France, D.M. Small circular- and rectangular-channel boiling with two refrigerants. Int. J. Multiph. Flow 1996, 22, 485–498. [Google Scholar] [CrossRef]
- Cooper, M.G. Heat Flow Rates in Saturated Nucleate Pool Boiling—A Wide-Ranging Examination Using Reduced Properties. Adv. Heat Transf. 1984, 16, 157–239. [Google Scholar]
- Xu, Y.; Fang, X.; Li, D.; Li, G.; Yuan, Y.; Xu, A. An experimental study of flow boiling frictional pressure drop of R134a and evaluation of existing correlations. Int. J. Heat Mass Transf. 2016, 98, 150–163. [Google Scholar] [CrossRef]
- Xu, Y.; Fang, X. A new correlation of two-phase frictional pressure drop for evaporating flow in pipes. Int. J. Refrig. 2012, 35, 2039–2050. [Google Scholar] [CrossRef]
- Müller-Steinhagen, H.; Heck, K. A simple friction pressure drop correlation for two-phase flow in pipes. Chem. Eng. Process. 1986, 20, 297–308. [Google Scholar] [CrossRef]
- Cavallini, A.; Censi, G.; Del Col, D.; Doretti, L.; Longo, G.A.; Rossetto, L. Condensation of halogenated refrigerants inside smooth tubes. HVAC R Res. 2002, 8, 429–451. [Google Scholar] [CrossRef]
- Kim, S.M.; Mudawar, I. Universal approach to predicting two-phase frictional pressure drop for adiabatic and condensing mini/micro-channel flows. Int. J. Heat Mass Transf. 2012, 55, 3246–3261. [Google Scholar] [CrossRef]
- Cicchitti, A.; Lombardi, C.; Silvestri, M.; Soldaini, G.; Zavattarelli, R. Two-phase cooling experiments-pressure drop, heat transfer, and burnout measurements. Energ. Nucl. 1960, 7, 407–425. [Google Scholar]
- Grönnerud, R. Investigation of liquid hold-up, flow-resistance and heat transfer in circulation type evaporators, part IV: Two-phase flow resistance in boiling refrigerants. Bull. I’Inst. Du Froid 1979, 16, 1972–1981. [Google Scholar]
- Zhang, M.; Webb, R.L. Correlation of two-phase friction for refrigerants in small-diameter tubes. Exp. Therm. Fluid Sci. 2001, 25, 131–139. [Google Scholar] [CrossRef]
- Shannak, B.A. Frictional pressure drop of gas liquid two-phase flow in pipes. Nucl. Eng. Des. 2008, 238, 3277–3284. [Google Scholar] [CrossRef]
- Li, W.; Wu, Z. A general correlation for adiabatic two-phase pressure drop in micro/mini-channels. Int. J. Heat Mass Transf. 2010, 53, 2732–2739. [Google Scholar] [CrossRef]







| Properties | p = 0.6 MPa | p = 0.7 MPa |
|---|---|---|
| Saturation temperature (°C) | 31.3 | 36.7 |
| Liquid density (kg/m3) | 1142.2 | 1123.3 |
| Vapor density (kg/m3) | 31.673 | 37.027 |
| Liquid viscosity (Pa s) | 1.8519 × 10−4 | 1.7367 × 10−4 |
| Vapor viscosity (Pa s) | 1.2516 × 10−5 | 1.2770 × 10−5 |
| Liquid conductivity (W/m K) | 0.0721 | 0.0703 |
| Vapor conductivity (W/m K) | 0.0141 | 0.0146 |
| Surface tension (N/m) | 8.0483 × 10−3 | 7.3648 × 10−3 |
| Latent enthalpy (kJ/kg) | 162.05 | 157.61 |
| Parameter | Uncertainty |
|---|---|
| Diameter | ±1 μm |
| Length | ±1 mm |
| Temperature | ±0.1 °C |
| Flow rate | ±0.5% |
| Relative pressure | ±0.3%FS (0–1.6 MPa) |
| Differential pressure | ±0.075%FS (0–100 kPa) |
| Heat flux | ±1.44% |
| Vapor quality | ±1.66% |
| HTC | ±4.01% |
| Correlation | MAD | MRD | PPN10 | PPN20 |
|---|---|---|---|---|
| Saitoh et al. [15] | 5.4 | −0.7 | 85.0 | 99.2 |
| Tran et al. [39] | 8.6 | −4.0 | 66.4 | 88.9 |
| Wattelet et al. [33] | 12.7 | −6.9 | 47.8 | 79.1 |
| Fang [32] | 13.3 | −10.1 | 49.0 | 74.3 |
| Shah [35] | 13.6 | −1.2 | 36.8 | 76.3 |
| Gungor and Winterton [34] | 17.6 | 8.5 | 29.6 | 60.5 |
| Cooper [40] | 18.1 | −18.0 | 19.0 | 64.4 |
| Li and Wu [38] | 18.1 | −15.1 | 35.6 | 59.3 |
| Sun and Mishima [8] | 18.3 | 17.0 | 24.1 | 60.1 |
| Jung et al. [37] | 18.7 | 18.3 | 23.7 | 61.7 |
| Liu and Winterton [36] | 21.4 | −21.4 | 17.0 | 47.0 |
| Bertsch et al. [9] | 27.1 | −27.0 | 9.1 | 48.6 |
| Correlation | MAD | MRD | PPN10 | PPN20 |
|---|---|---|---|---|
| Müller–Steinhagen and Heck [43] | 10.9 | −0.3 | 64.4 | 90.7 |
| Zhang and Webb [48] | 15.7 | 12.9 | 67.4 | 75.2 |
| Cavallini et al. [44] | 17.7 | 16.3 | 46.3 | 72.2 |
| Friedel [6] | 17.7 | 5.6 | 43.4 | 80.0 |
| Kim and Mudawar [45] | 19.7 | −0.7 | 33.9 | 62.5 |
| Cicchitti et al. [46] | 21.5 | –12.8 | 18.7 | 42.5 |
| Xu and Fang [42] | 32.3 | 32.3 | 8.4 | 49.3 |
| Shannak [49] | 32.9 | −25.4 | 11.4 | 21.1 |
| Li and Wu [50] | 33.1 | −32.2 | 4.8 | 11.2 |
| Grönnerud [47] | 54.5 | 54.2 | 3.2 | 8.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, Y.; Yan, Z.; Li, L. Experimental Investigation on Flow Boiling Heat Transfer and Pressure Drop of a Low-GWP Refrigerant R1234ze(E) in a Horizontal Minichannel. Energies 2021, 14, 5972. https://doi.org/10.3390/en14185972
Xu Y, Yan Z, Li L. Experimental Investigation on Flow Boiling Heat Transfer and Pressure Drop of a Low-GWP Refrigerant R1234ze(E) in a Horizontal Minichannel. Energies. 2021; 14(18):5972. https://doi.org/10.3390/en14185972
Chicago/Turabian StyleXu, Yu, Zihao Yan, and Ling Li. 2021. "Experimental Investigation on Flow Boiling Heat Transfer and Pressure Drop of a Low-GWP Refrigerant R1234ze(E) in a Horizontal Minichannel" Energies 14, no. 18: 5972. https://doi.org/10.3390/en14185972
APA StyleXu, Y., Yan, Z., & Li, L. (2021). Experimental Investigation on Flow Boiling Heat Transfer and Pressure Drop of a Low-GWP Refrigerant R1234ze(E) in a Horizontal Minichannel. Energies, 14(18), 5972. https://doi.org/10.3390/en14185972







