Flow and Heat Transfer Characteristics of a Swirling Impinging Jet Issuing from a Threaded Nozzle of 45 Degrees
Abstract
:- (a)
- The 45° threaded nozzle is found to increase radial cooling effectiveness and uniformity by the superposition of the vertical jet and swirl.
- (b)
- The 45° threaded nozzle is studied and compared with the CIJ by both numerical and experimental methods.
- (c)
- The heat transfer correlation of the swirling impinging jet is built and compared with the CIJ.
- (d)
- The pressure loss coefficient of SIJ 45° and the CIJ are studied.
1. Introduction
2. Experimental System and Data Reduction
2.1. Experimental Facility
2.2. Data Reduction
3. Numerical Method
3.1. Model Setup
3.2. Verification of the Numerical Method
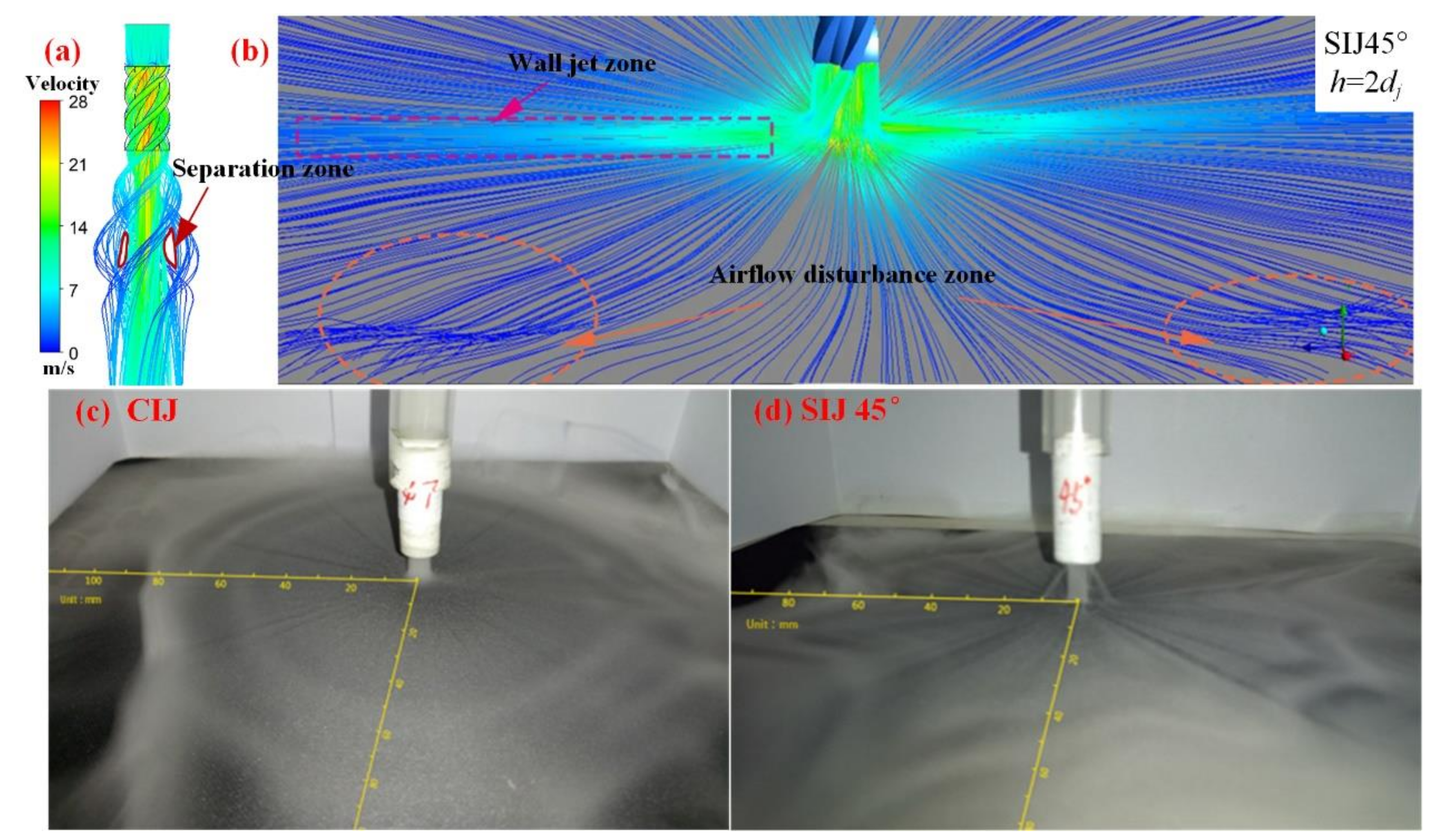
4. Flow Field Characteristic Analysis
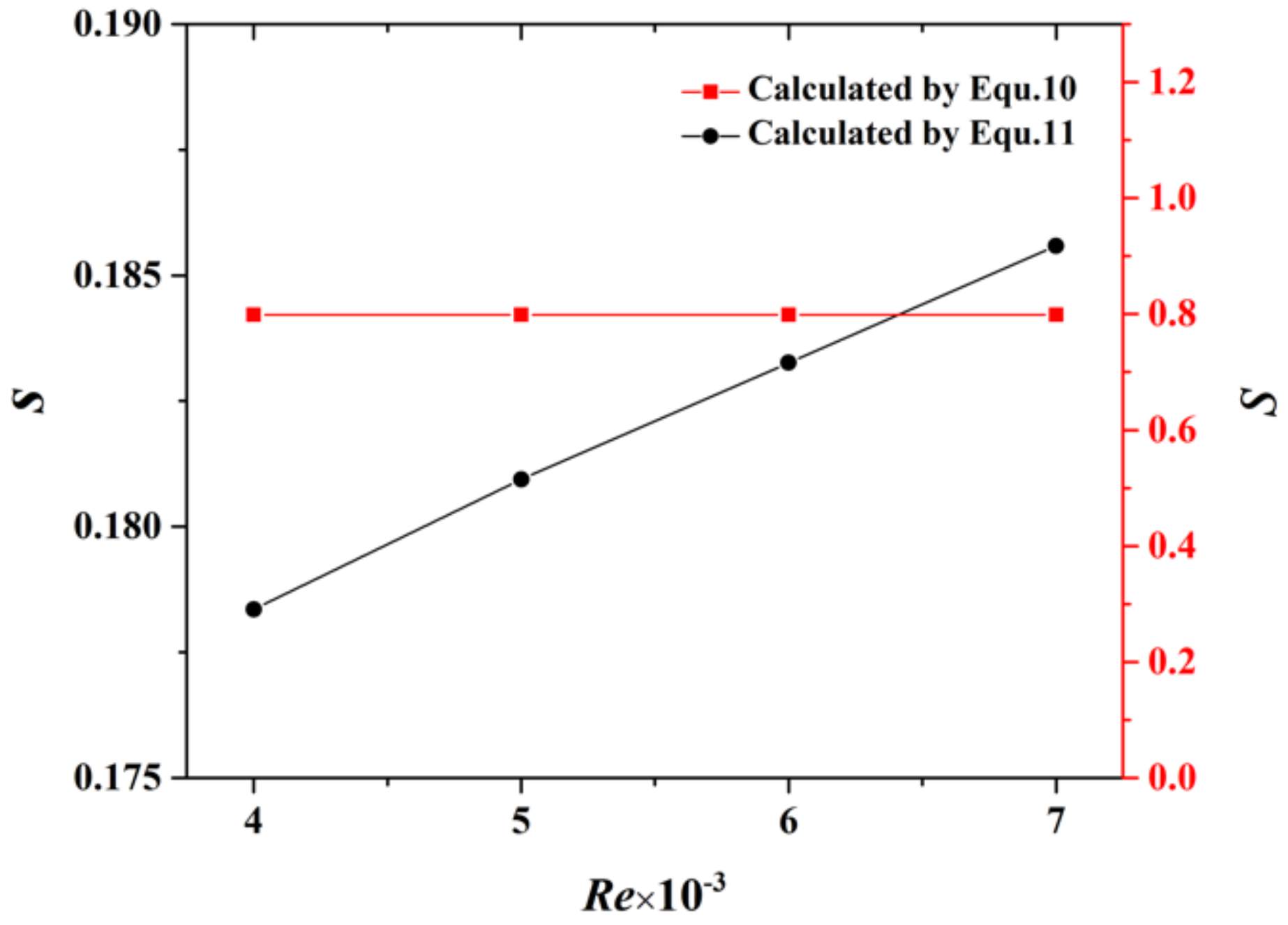
4.1. Analysis of the Swirl Number
4.2. Analysis of Velocity Distribution of Different Sections
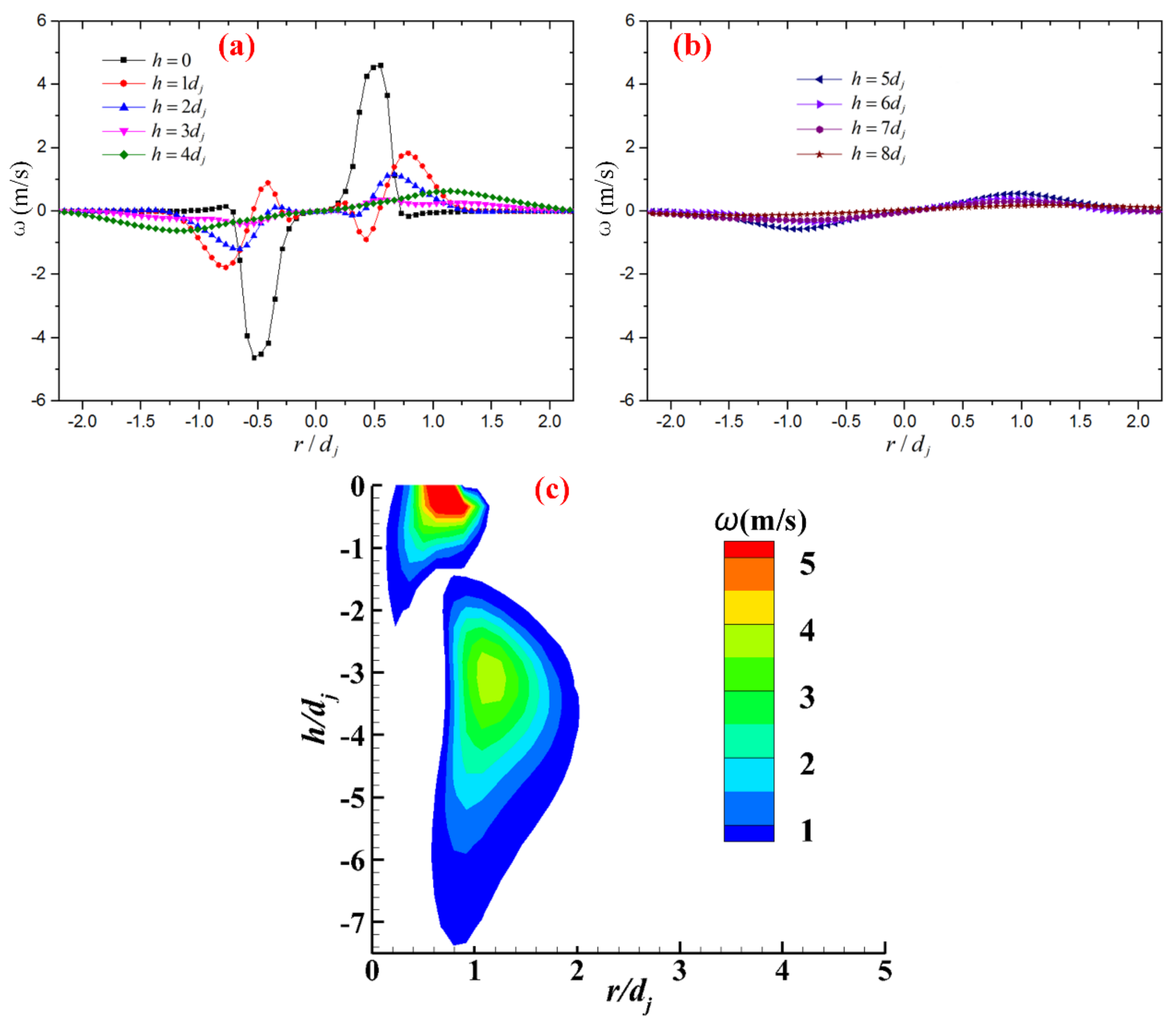
4.3. Analysis of the Attenuation of Tangential Velocity
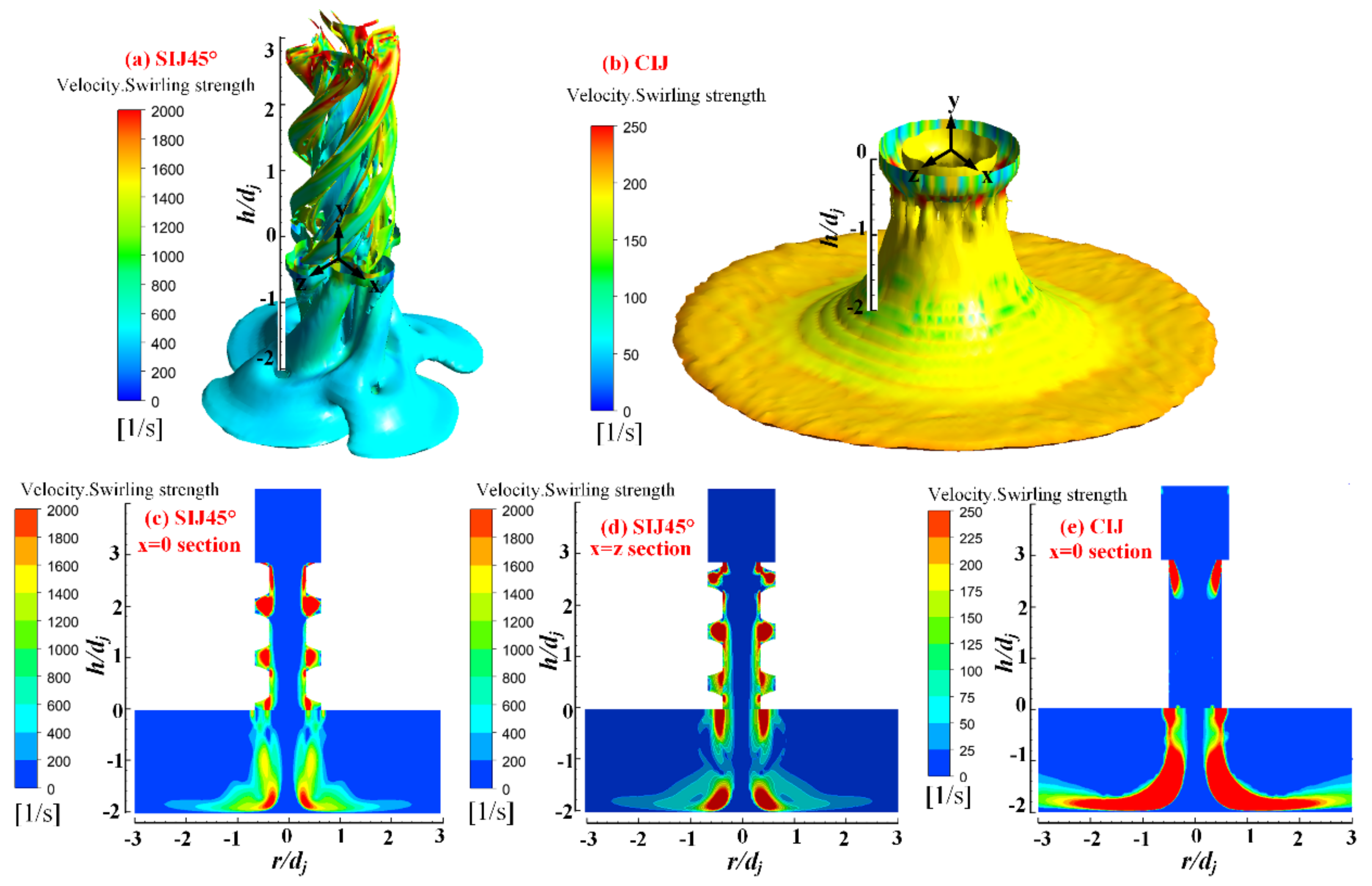
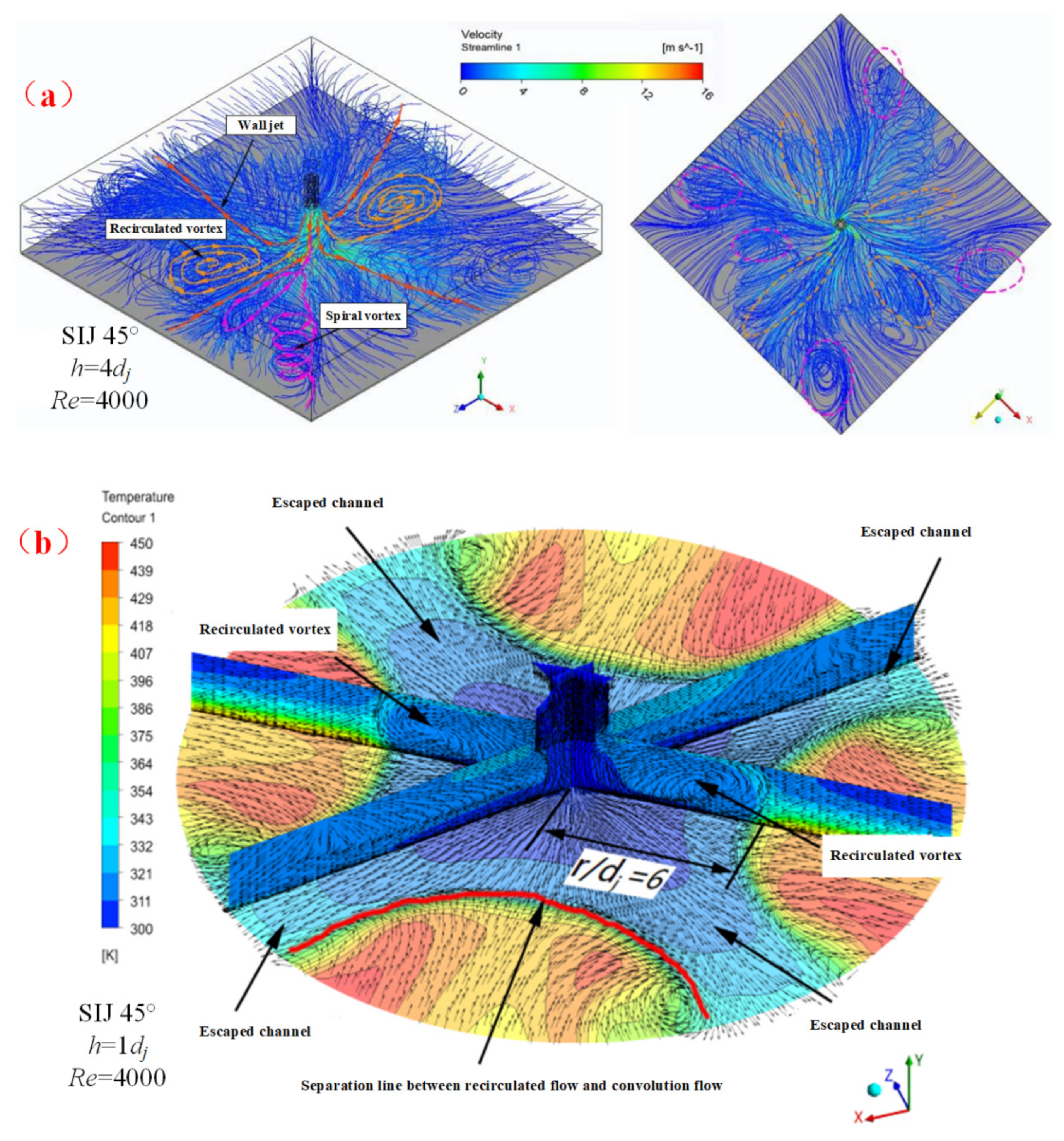
4.4. Analysis of the Vortex
4.5. Analysis of the Flow Field Structure
4.6. Flow Model for Swirling Impinging Jets
5. Heat Transfer Characteristics of the Jets from the Threaded Nozzles
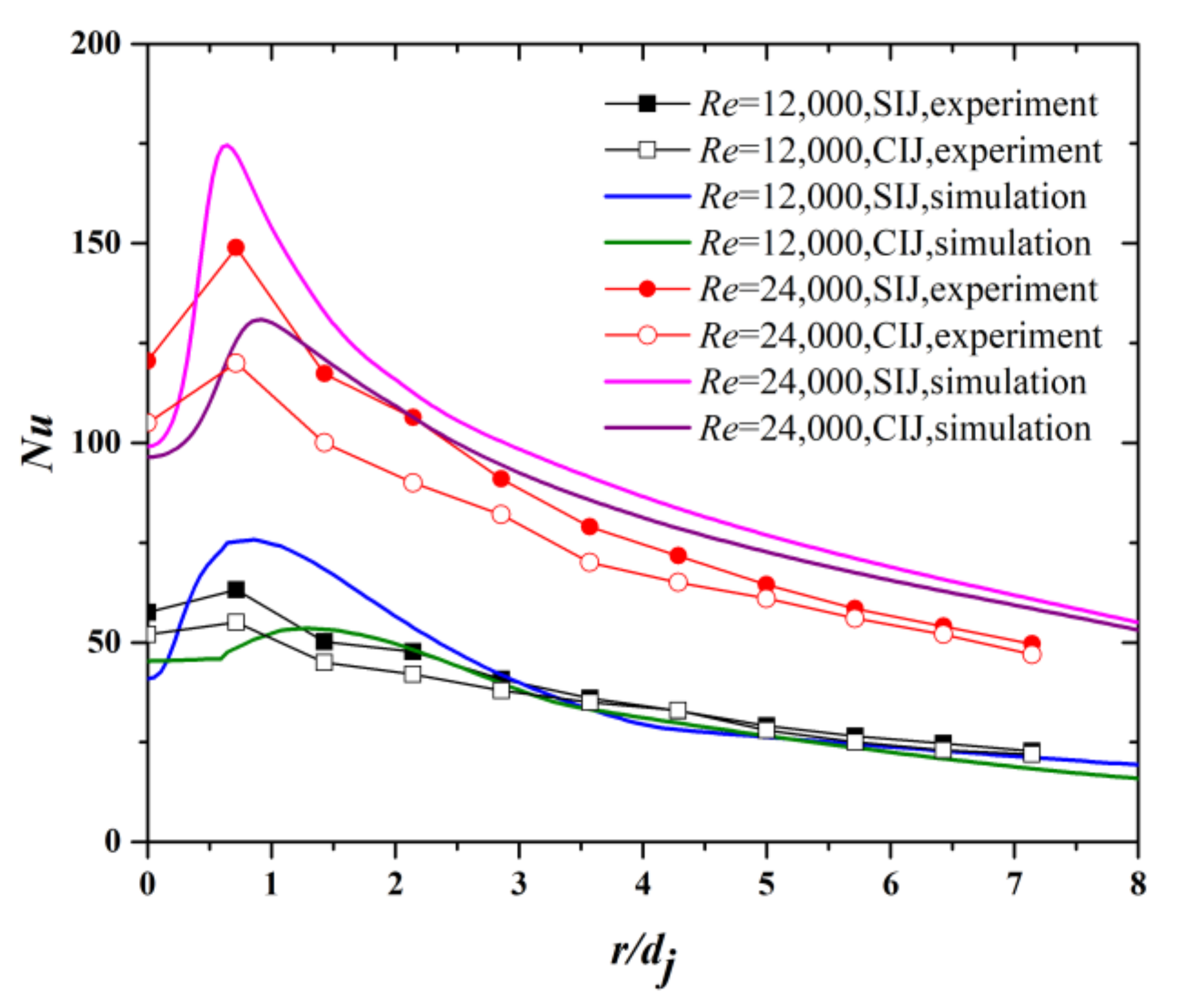
5.1. Comparison of Heat Transfer and Flow Characteristics of SIJ 45° and CIJ
5.2. Effect of Re on Heat Transfer Characteristics
5.3. Effect of Jet Spacing on Heat Transfer Characteristics
5.4. Comparison of Pressure Loss Coefficient
5.5. Globally Averaged
6. Conclusions
- SIJ 45° generates swirling flow and a central vertical jet. Due to the tangential velocity, flow diffusivity is enhanced compared with that of the CIJ, which takes away more heat.
- The airflow ejected from SIJ 45° rotates and diffuses around and forms four strong vortices with a symmetrical distribution, which explains why the tangential velocity appears with multiple peaks of positive and negative alternations.
- The flow field ejected from SIJ 45° can be considered a complex flow field, in which the vertical impingement flow and the swirling flow interact with each other.
- As the increases, the Nu for SIJ gradually decreases, and the maximum Nu transfers to the peripheral area, which enhances the heat transfer uniformity.
- An empirical correlation of is built within the range of 1 ≤ h/dj ≤ 8 for SIJ , which agrees well with the experimental results.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| CIJ | Conventional impinging jet |
| dj | Equivalent diameter (mm) |
| G | Ratio of the maximum rotational velocity and axial velocity |
| Axial flux of linear momentum | |
| h | Jet spacing (distance from nozzle to impingement surface) (mm) |
| K | Pressure loss coefficient |
| Nu | Nusselt number |
| R2 | Fitting accuracy |
| r | Radial distance from the stagnation point (mm) |
| SIJ45° | Swirling jet with a swirling angle of 45° |
| Average test temperature of the target (K) | |
| Ωij | Antisymmetric part of the velocity gradient tensor (s-1) |
| Maximum tangential velocity (m/s) | |
| θ | Swirl angle (°) |
| Mean coolant velocity (m/s) | |
| Aerodynamic viscosity (Pa s) | |
| Inverse effective Prandtl numbers for | |
| d | Minimum inner diameter of the threaded nozzle (mm) |
| D | Maximum inner diameter of the threaded nozzle (mm) |
| Turbulence kinetic energy due to the mean velocity gradients | |
| axial flux of swirl momentum | |
| Heat transfer coefficient (W/m2 K) | |
| k | Turbulent kinetic energy |
| R | Nozzle radius (mm) |
| Re | Reynolds number |
| S | Swirl number |
| Inlet temperature of the jet (K) | |
| Average velocity (m/s) | |
| Ψij | Positive symmetric part of the velocity gradient tensor. |
| Maximum axial velocity (m/s) | |
| λ | Thermal conductivity of air (W/m K) |
| Coolant density (kg/m3) | |
| Inverse effective Prandtl numbers for | |
| Effective viscosity coefficient. |
References
- Alenezi, A.; Almutairi, A.; Alhajeri, H.; Addali, A.; Gamil, A. Flow Structure and Heat Transfer of Jet Impingement on a Rib-Roughened Flat Plate. Energies 2018, 11, 1550. [Google Scholar] [CrossRef] [Green Version]
- Kareem, Z.S.; Balla, H.H.; AbdulWahid, A.F. Heat transfer enhancement in single circular impingement jet by CuO-water nanofluid. Case Stud. Therm. Eng. 2019, 15, 100508. [Google Scholar] [CrossRef]
- Kura, T.; Wajs, J.; Fornalik-Wajs, E.; Kenjeres, S.; Gurgul, S. Thermal and Hydrodynamic Phenomena in the Stagnation Zone—Impact of the Inlet Turbulence Characteristics on the Numerical Analyses. Energies 2020, 14, 105. [Google Scholar] [CrossRef]
- Martínez-Filgueira, P.; Zulueta, E.; Sánchez-Chica, A.; Fernández-Gámiz, U.; Soriano, J. Multi-Objective Particle Swarm Based Optimization of an Air Jet Impingement System. Energies 2019, 12, 1627. [Google Scholar] [CrossRef] [Green Version]
- Yang, Y.; Liu, H.; Mao, W.; Song, Z.; Wang, H. Study on the Impact Pressure of Swirling-Round Supercritical CO2 Jet Flow and Its Influencing Factors. Energies 2020, 14, 106. [Google Scholar] [CrossRef]
- Mohamed Illyas, S.; Ramesh Bapu, B.R.; Venkata Subba Rao, V. Experimental Analysis of Heat Transfer and Multi Objective Optimization of Swirling Jet Impingement on a Flat Surface. J. Appl. Fluid Mech. 2019, 12, 803–817. [Google Scholar] [CrossRef]
- Singh, P.; Chander, S. Study of flow field and heat transfer characteristics for an interacting pair of counter-rotating dual-swirling impinging flames. Int. J. Therm. Sci. 2019, 144, 191–211. [Google Scholar] [CrossRef]
- Fénot, M.; Dorignac, E.; Lalizel, G. Heat transfer and flow structure of a multichannel impinging jet. Int. J. Therm. Sci. 2015, 90, 323–338. [Google Scholar] [CrossRef]
- Ahmed, Z.U.; Al-Abdeli, Y.M.; Guzzomi, F.G. Flow field and thermal behaviour in swirling and non-swirling turbulent impinging jets. Int. J. Therm. Sci. 2017, 114, 241–256. [Google Scholar] [CrossRef]
- Ahmed, Z.U.; Al-Abdeli, Y.M.; Matthews, M.T. The effect of inflow conditions on the development of non-swirling versus swirling impinging turbulent jets. Comput. Fluids 2015, 118, 255–273. [Google Scholar] [CrossRef]
- Wu, F.; Li, L.; Wang, J.; Fan, X.; Du, C. Numerical investigations on flow and heat transfer of swirl and impingement composite cooling structures of turbine blade leading edge. Int. J. Heat Mass Transf. 2019, 144, 118625. [Google Scholar] [CrossRef]
- Debnath, S.; Khan, M.H.U.; Ahmed, Z.U. Turbulent swirling impinging jet arrays: A numerical study on fluid flow and heat transfer. Therm. Sci. Eng. Prog. 2020, 19, 100580. [Google Scholar] [CrossRef]
- Chouaieb, S.; Kriaa, W.; Mhiri, H.; Bournot, P. Swirl generator effect on a confined coaxial jet characteristics. Int. J. Hydrogen Energy 2017, 42, 29014–29025. [Google Scholar] [CrossRef]
- Liu, L.; Zhang, J.; Liu, S.; Wang, K.; Gu, H. Decay law and swirl length of swirling gas-liquid flow in a vertical pipe. Int. J. Multiph. Flow 2021, 137, 103570. [Google Scholar] [CrossRef]
- Yan, J.; Gui, N.; Xie, G.; Gao, J. Direct Numerical Simulation and Visualization of Biswirling Jets. Adv. Mech. Eng. 2015, 6, 193731. [Google Scholar] [CrossRef]
- Wannassi, M.; Monnoyer, F. Fluid flow and convective heat transfer of combined swirling and straight impinging jet arrays. Appl. Therm. Eng. 2015, 78, 62–73. [Google Scholar] [CrossRef]
- Huang, L.; El-Genk, M.S. Heat transfer and flow visualization experiments of swirling, multi-channel, and conventional impinging jets.pdf. Int. J. Heat Mass Transf. 1998, 41, 583–600. [Google Scholar] [CrossRef]
- Markal, B. The effect of Total flowrate on the cooling performance of swirling coaxial impinging jets. Heat Mass Transf. 2019, 55, 3275–3288. [Google Scholar] [CrossRef]
- Hindasageri, V.; Vedula, R.P.; Prabhu, S.V. Heat transfer distribution of swirling flame jet impinging on a flat plate using twisted tapes. Int. J. Heat Mass Transf. 2015, 91, 1128–1139. [Google Scholar] [CrossRef]
- Duangthongsuk, W.; Wongwises, S. An experimental investigation of the heat transfer and pressure drop characteristics of a circular tube fitted with rotating turbine-type swirl generators. Exp. Therm. Fluid Sci. 2013, 45, 8–15. [Google Scholar] [CrossRef]
- Kumar, S.S.; Hindasageri, V.; Prabhu, S.V. Local heat transfer distribution on a flat plate impinged by a swirling jet generated by a twisted tape. Int. J. Therm. Sci. 2017, 111, 351–368. [Google Scholar] [CrossRef]
- Wongcharee, K.; Chuwattanakul, V.; Eiamsaard, S. Influence of CuO/water nanofluid concentration and swirling flow on jet impingement cooling. Int. Commun. Heat Mass Transf. 2017, 88, 277–283. [Google Scholar] [CrossRef]
- Wongcharee, K.; Chuwattanakul, V.; Eiamsaard, S. Heat transfer of swirling impinging jets with TiO2-water nanofluids. Chem. Eng. Process. Process. Intensif. 2017, 114, 16–23. [Google Scholar] [CrossRef]
- Chang, S.W.; Shen, H.-D. Heat transfer characteristics of swirling impinging jet-arrays issued from nozzle plates with and without webbed grooves. Int. J. Therm. Sci. 2020, 148, 106155. [Google Scholar] [CrossRef]
- Ortega-Casanova, J. CFD and correlations of the heat transfer from a wall at constant temperature to an impinging swirling jet. Int. J. Heat Mass Transf. 2012, 55, 5836–5845. [Google Scholar] [CrossRef]
- Ortega-Casanova, J.; Granados-Ortiz, F.J. Numerical simulation of the heat transfer from a heated plate with surface variations to an impinging jet. Int. J. Heat Mass Transf. 2014, 76, 128–143. [Google Scholar] [CrossRef]
- Amini, Y.; Mokhtari, M.; Haghshenasfard, M.; Barzegar Gerdroodbary, M. Heat transfer of swirling impinging jets ejected from Nozzles with twisted tapes utilizing CFD technique. Case Stud. Therm. Eng. 2015, 6, 104–115. [Google Scholar] [CrossRef] [Green Version]
- Chattopadhyay, H. Numerical investigations of heat transfer from impinging annular jet. Int. J. Heat Mass Transf. 2004, 47, 3197–3201. [Google Scholar] [CrossRef]
- Salman, S.D.; Kadhum, A.A.H.; Takriff, M.S.; Mohamad, A.B. Experimental and Numerical Investigations of Heat Transfer Characteristics for Impinging Swirl Flow. Adv. Mech. Eng. 2015, 6, 631081. [Google Scholar] [CrossRef]
- Hayat, M.Z.; Nandan, G.; Tiwari, A.K.; Sharma, S.K.; Shrivastava, R.; Singh, A.K. Numerical study on heat transfer enhancement using twisted tape with trapezoidal ribs in an internal flow. Mater. Today Proc. 2020, 46, 5412–5419. [Google Scholar] [CrossRef]
- Nozaki, A.; Igarashi, Y.; Hishida, K. Heat transfer mechanism of a swirling impinging jet in a stagnation region. Heat Transf. Asian Res. 2003, 32, 663–673. [Google Scholar] [CrossRef]
- Xu, L.; Lan, J.; Ma, Y.; Gao, J.; Li, Y. Numerical study on heat transfer by swirling impinging jets issuing from a screw-thread nozzle. Int. J. Heat Mass Transf. 2017, 115, 232–237. [Google Scholar] [CrossRef]
- Xu, L.; Xiong, Y.; Xi, L.; Gao, J.; Li, Y.; Zhao, Z. Numerical Simulation of Swirling Impinging Jet Issuing from a Threaded Hole under Inclined Condition. Entropy 2019, 22, 15. [Google Scholar] [CrossRef] [Green Version]
- Xu, L.; Yang, T.; Sun, Y.; Xi, L.; Gao, J.; Li, Y.; Li, J. Flow and heat transfer characteristics of a swirling impinging jet issuing from a threaded nozzle. Case Stud. Therm. Eng. 2021, 25, 100970. [Google Scholar] [CrossRef]
- Orzag, S.A.; Yakhot, V. Renormalization-Group Analysis of Turbulence. Phys. Rev. Lett. 1986, 57, 1722–1724. [Google Scholar]
- Drake, P.F.; Hubard, E.H. Effect of air swirl on the completeness of combustion. J. Inst. Fuel 1963, 36, 389. [Google Scholar]
- Bakirci, K.; Bilen, K. Visualization of heat transfer for impinging swirl flow. Exp. Therm. Fluid Sci. 2007, 32, 182–191. [Google Scholar] [CrossRef]
- Yang, H.Q.; Kim, T.; Lu, T.J.; Ichimiya, K. Flow structure, wall pressure and heat transfer characteristics of impinging annular jet with/without steady swirling. Int. J. Heat Mass Transf. 2010, 53, 4092–4100. [Google Scholar] [CrossRef]
- Gupta, A.K. Swirl Flows; Tunbridge Wells Kent England Abacus Press: Kent, UK, 1984. [Google Scholar]
- Forthmann, E. Uber turbulence strahlausbreitung. Diss. Gott. 1934, 319, 36–38. [Google Scholar]
- Kolář, V. Vortex identification: New requirements and limitations. Int. J. Heat Fluid Flow 2007, 28, 638–652. [Google Scholar] [CrossRef]
- Jeong, J.; Hussain, F. On the identification of a vortex. J. Fluid Mech. 1995, 285, 69–94. [Google Scholar] [CrossRef]
- Xu, L.; Ren, D.; Ma, Y.; Lan, J.; Gao, J. Pressure loss and heat transfer characteristics experiment of swirling impinging jet with different shape nozzles. J. Aerosp. Power 2018, 33, 2678–2686. [Google Scholar]
- Goldstein, R.J.; Seol, W.S. Heat transfer to a row of impinging circular air jets including the effect of entrainment. Int. J. Heat Mass Transf. 1991, 34, 2133–2147. [Google Scholar] [CrossRef]
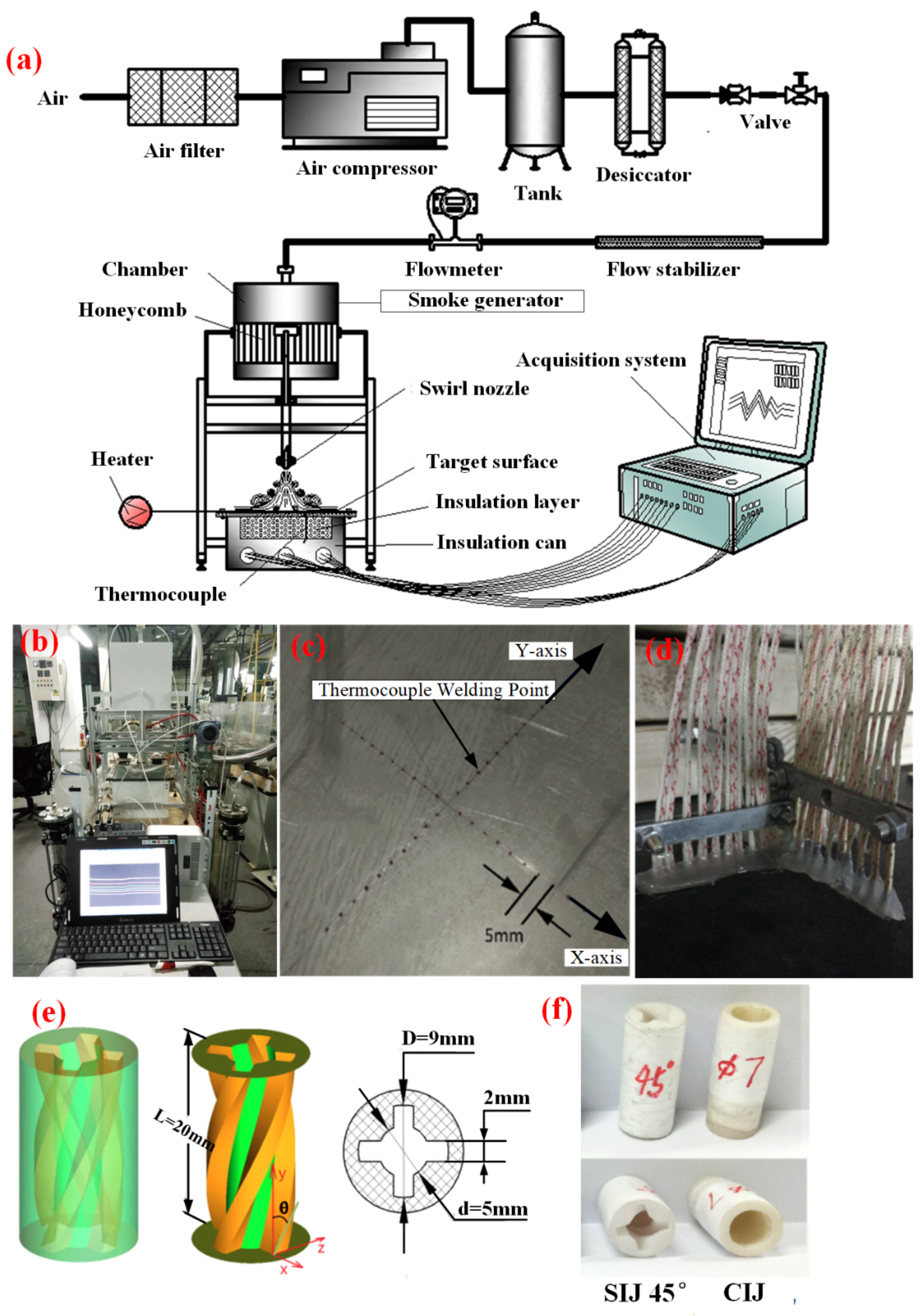












| Measurement Parameters | Symbols | Measuring Instruments | Uncertainty |
|---|---|---|---|
| Temperature | T | E-Type thermocouple | 1% |
| flow rate | Q | Flowmeter | 2.5% |
| Pressure | P | differential pressure gauge | 0.05% |
| Geometric parameters | L | Digital caliper | 0.01 mm |
| Voltage | U | Voltmeter | 0.2% |
| Current | I | Ammeter | 0.2% |
| Condition | Swirl Angle (°) | Chamber Pressure (Pa) | Flow Rate | Jet Spacing | Heat Flux | |||
|---|---|---|---|---|---|---|---|---|
| Case 1 | 45 | 296.27 | 310.10 | 298.45 | 166 | 1.83 | 3 | 2625 |
| Case 2 | 45 | 294.71 | 301.22 | 296.12 | 590 | 2.65 | 3 | 2746 |
| Case 3 | 7 (CIJ) | 297.55 | 306.23 | 295.42 | 108 | 1.52 | 4 | 1035 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, L.; Yang, T.; Sun, Y.; Xi, L.; Gao, J.; Li, Y. Flow and Heat Transfer Characteristics of a Swirling Impinging Jet Issuing from a Threaded Nozzle of 45 Degrees. Energies 2021, 14, 8412. https://doi.org/10.3390/en14248412
Xu L, Yang T, Sun Y, Xi L, Gao J, Li Y. Flow and Heat Transfer Characteristics of a Swirling Impinging Jet Issuing from a Threaded Nozzle of 45 Degrees. Energies. 2021; 14(24):8412. https://doi.org/10.3390/en14248412
Chicago/Turabian StyleXu, Liang, Tao Yang, Yanhua Sun, Lei Xi, Jianmin Gao, and Yunlong Li. 2021. "Flow and Heat Transfer Characteristics of a Swirling Impinging Jet Issuing from a Threaded Nozzle of 45 Degrees" Energies 14, no. 24: 8412. https://doi.org/10.3390/en14248412
APA StyleXu, L., Yang, T., Sun, Y., Xi, L., Gao, J., & Li, Y. (2021). Flow and Heat Transfer Characteristics of a Swirling Impinging Jet Issuing from a Threaded Nozzle of 45 Degrees. Energies, 14(24), 8412. https://doi.org/10.3390/en14248412








