Free Convection Heat Transfer from Horizontal Cylinders
Abstract
1. Introduction
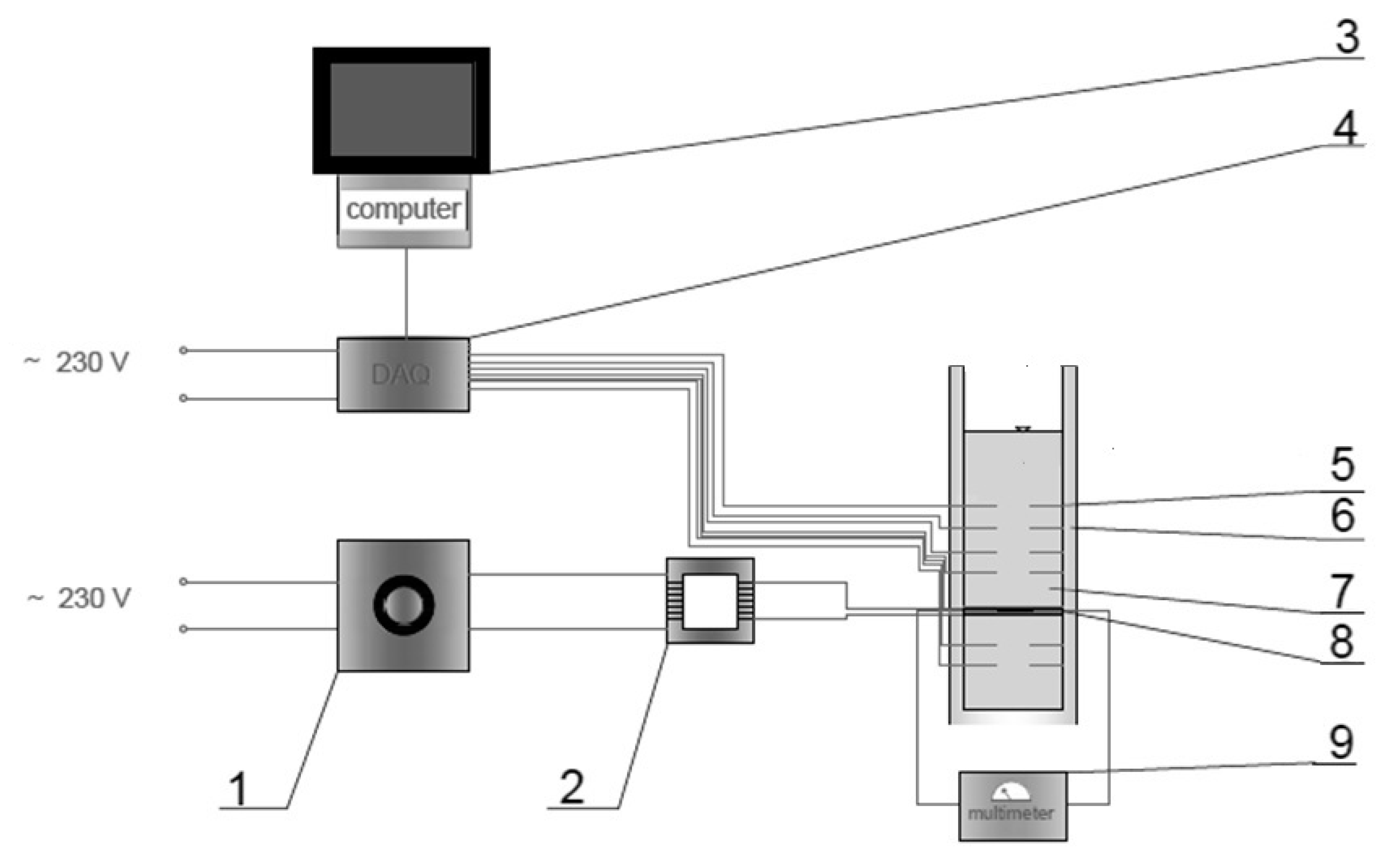
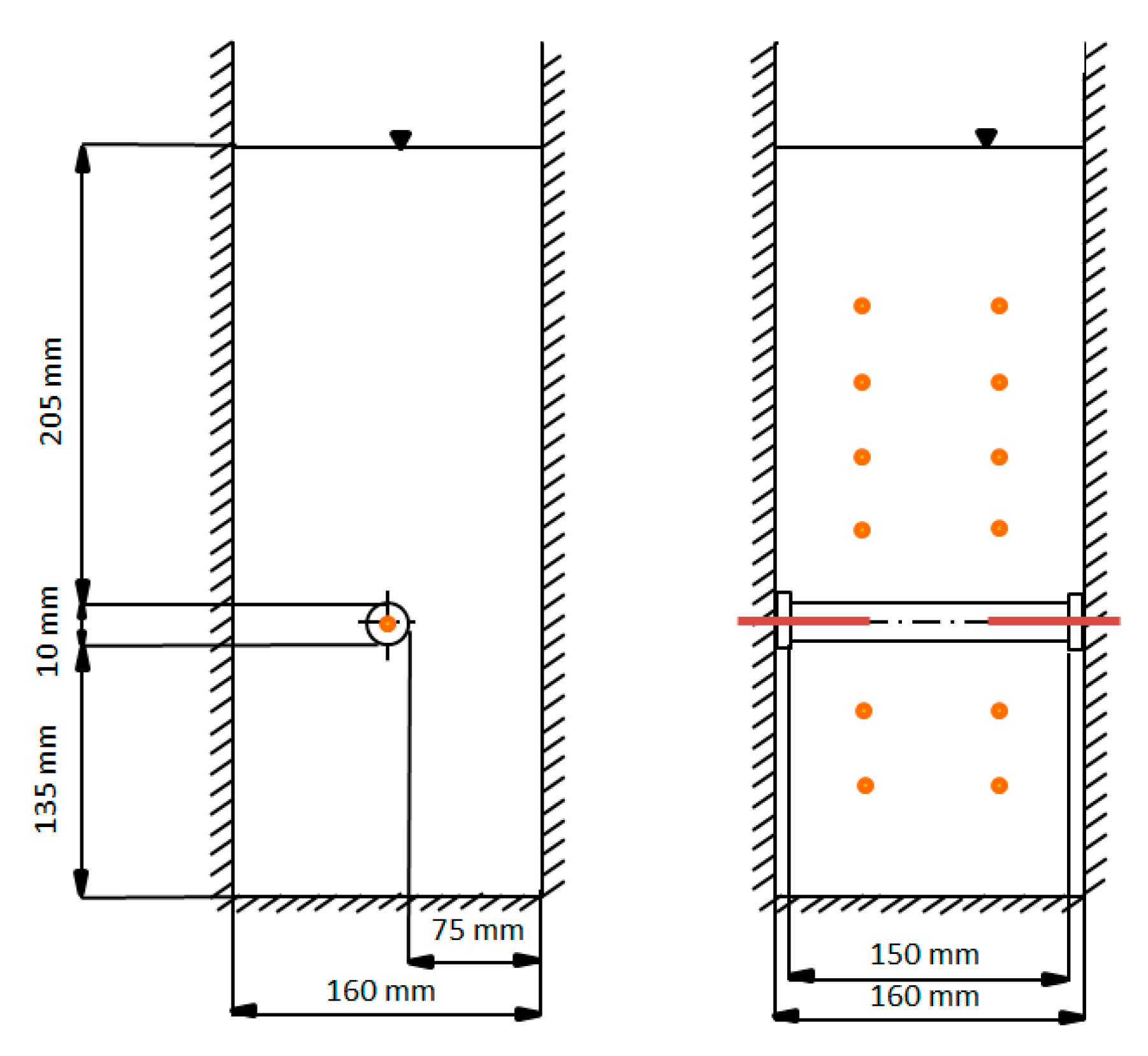
2. Experimental Setup
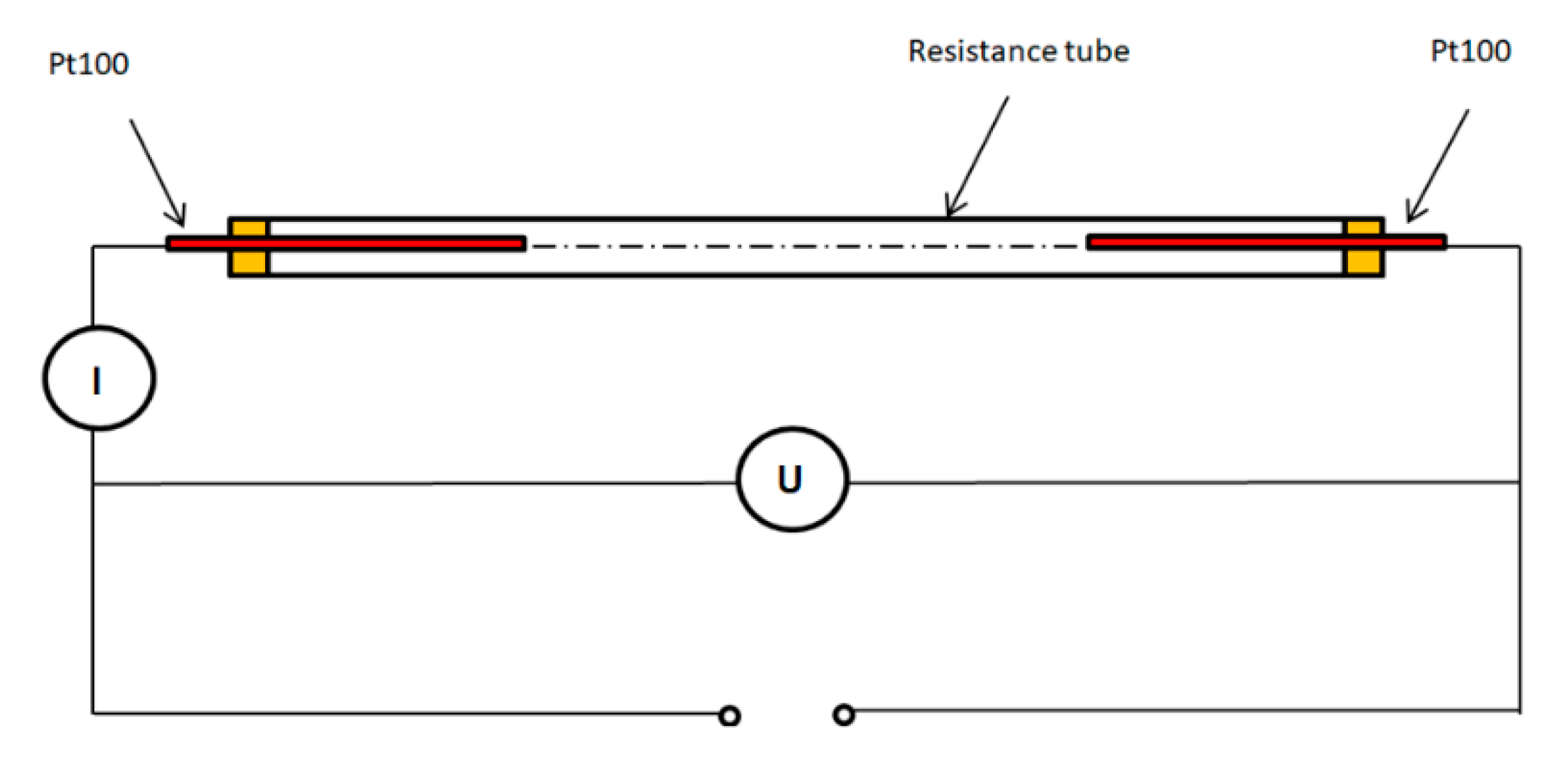
2.1. Experimental Apparatus
2.2. Experimental Procedure
2.3. Data Reduction and Measurement Error
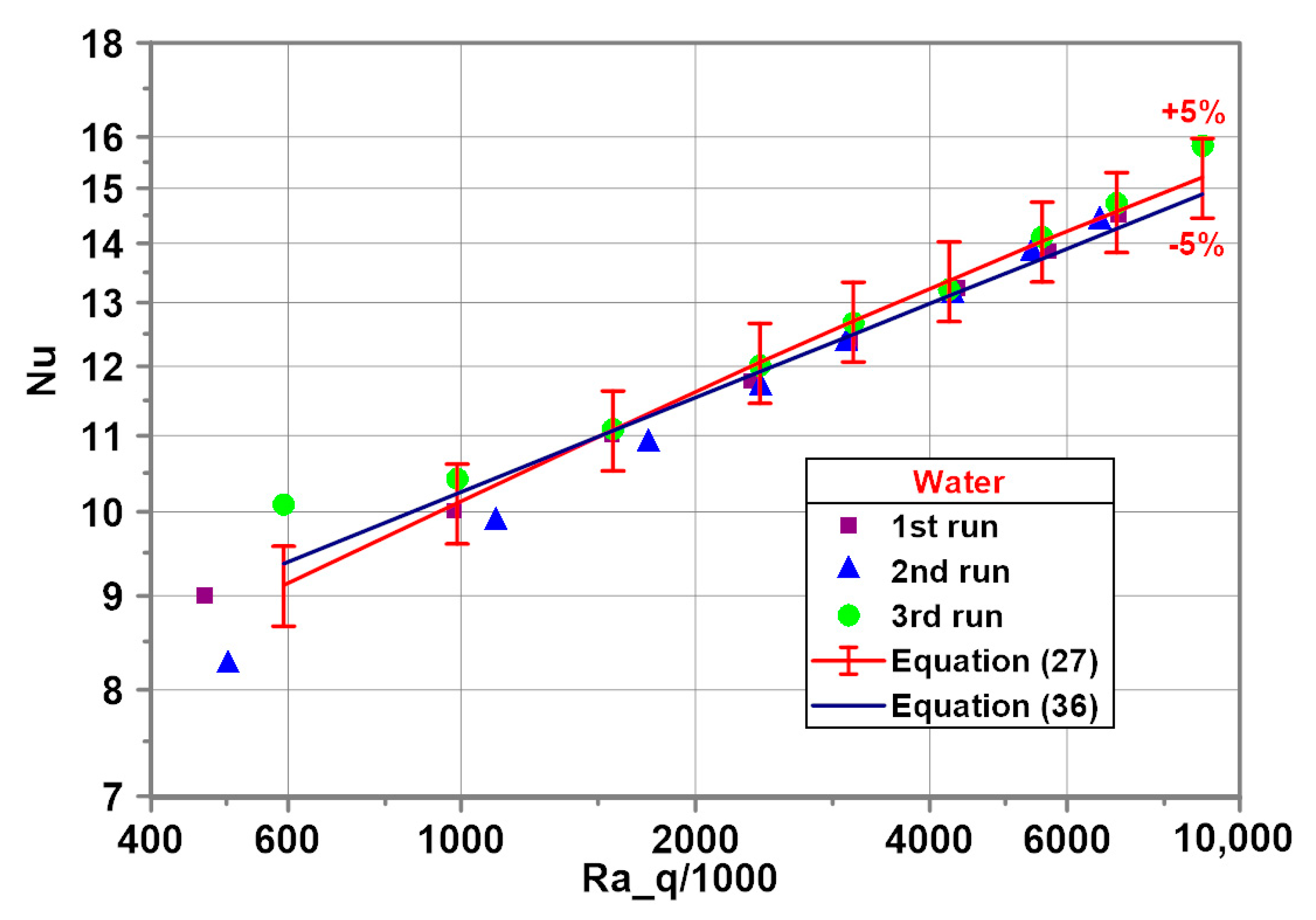
3. Results
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| a | Thermal diffusivity | (m2/s) |
| b = πD/2 | Boundary layer length | (m) |
| cp | Specific heat | (J/(kg K)) |
| D | Diameter of heated cylinder | (m) |
| G | Gebhart number | (-) |
| h | Average heat transfer coefficient | (W/(m2 K)) |
| H | Liquid depth between bottom wall and free liquid surface | (m) |
| HB | Distance between periphery of cylinder and bottom wall | (m) |
| HT | Submersion depth | (m) |
| I | Current | (A) |
| k | Thermal conductivity | (W/(m K)) |
| L | Effective length of cylinder | (m) |
| Lchar | Characteristic length | (m) |
| Nusselt number | (-) | |
| P | Electrical power | (W) |
| Prandtl number | (-) | |
| q | Heat flux | (W/m2) |
| Rayleigh number related to temperature difference | (-) | |
| Rayleigh number based on b as characteristic length | (-) | |
| Rayleigh number related to heat flux | (-) | |
| SW | Distance between periphery of cylinder and side wall | (m) |
| t | Temperature | (°C) |
| T | Temperature | (K) |
| ΔT | Temperature difference | (K) |
| U | Voltage | (V) |
| W | Width | (m) |
| Thermal expansion coefficient | (1/K) | |
| Dynamic viscosity | (Pa s) | |
| Kinematic viscosity | (m2/s) | |
| Density | (kg/m3) | |
| Subscripts | ||
| cyl | Cylinder | |
| el | Electrical | |
| f | Liquid | |
| fm | Film temperature | |
| in | Inside | |
| o | Outside | |
| uni | Uniform | |
| v | Volume | |
| w | Wall | |
| q | Based on heat flux | |
| ∞ | Infinity | |
References
- Boetcher, S.K.S. Natural Convection from Circular Cylinders; Springer Briefs in Thermal Engineering and Applied Science; Springer: Cham, Switzerland, 2014. [Google Scholar] [CrossRef]
- Morgan, V.T. The Overall Convective Heat Transfer from Smooth Circular Cylinders. Adv. Heat Transf. 1975, 11, 199–263. [Google Scholar]
- Churchill, S.; Usagi, R. A general expression for the correlation of rates of transfer and other phenomena. AICHE J. 1972, 18, 1121–1128. [Google Scholar] [CrossRef]
- Churchill, S.; Chu, H. Correlating equations for laminar and turbulent free convection from a horizontal cylinder. Int. J. Heat Mass Transf. 1975, 18, 1049–1053. [Google Scholar] [CrossRef]
- Morgan, V.T. Heat transfer by Natural Convection from a Horizontal Isothermal Circular Cylinder in Air. Heat Transf. Eng. 1997, 18, 25–33. [Google Scholar] [CrossRef]
- Kuehn, T.H.; Goldstein, R.J. Correlating equations for natural convection heat transfer between horizontal circular cylinders. Int. J. Heat Mass Transf. 1976, 19, 1127–1134. [Google Scholar] [CrossRef]
- Fand, R.M.; Morris, E.W.; Lum, M. Natural convection heat transfer from horizontal cylinders to air, water and silicone oils for Rayleigh numbers between 3 × 102 to 2 × 107. Int. J. Heat Mass Transf. 1977, 20, 1173–1184. [Google Scholar] [CrossRef]
- Kuehn, T.H.; Goldstein, R.J. Numerical solution to the Navier-Stokes equations for laminar natural convection about a horizontal isothermal circular cylinder. Int. J. Heat Mass Transf. 1980, 23, 971–979. [Google Scholar] [CrossRef]
- Farouk, B.; Güçeri, S.I. Natural convection from a horizontal cylinder—laminar regime. Trans. ASME J. Heat Transf. 1981, 103, 522–527. [Google Scholar] [CrossRef]
- Fand, R.M.; Brucker, J. A correlation for heat transfer by natural convection from horizontal cylinders that accounts for viscous dissipation. Int. J. Heat Mass Transfer 1983, 26, 709–726. [Google Scholar] [CrossRef]
- Sparrow, E.M.; Pfeil, D.R. Enhancement of natural convection heat transfer from a horizontal cylinder due to vertical shrouding surfaces. Trans. ASME J. Heat Transf. 1984, 106, 124–130. [Google Scholar] [CrossRef]
- Sparrow, E.M.; Ansari, M.A. All-modes heat transfer from a horizontal cylinder situated adjacent to adiabatic enclosing walls. Int. J. Heat Mass Transf. 1984, 22, 1855–1864. [Google Scholar] [CrossRef]
- Warrington, R.O.; Powe, R.E. The transfer of heat by natural convection between bodies and their enclosures. Int. J. Heat Mass Transf. 1985, 28, 319–330. [Google Scholar] [CrossRef]
- Karim, F.; Farouk, B.; Namer, I. Natural Convection Heat Transfer from a Horizontal Cylinder between Vertical Confining Adiabatic Walls. Trans. ASME J. Heat Transf. 1986, 108, 291–298. [Google Scholar] [CrossRef]
- Warrington, P.; Smith, S.; Powe, R.; Mussulman, R. Boundary effects on natural convection heat transfer for cylinders and cubes. Int. J. Heat Mass Transf. 1988, 31, 1322–1325. [Google Scholar] [CrossRef]
- Ghaddar, N.K. Natural Convection heat transfer between a uniformly heated cylindrical element and its rectangular enclosure. Int. J. Heat Mass Transf. 1992, 35, 2327–2334. [Google Scholar] [CrossRef]
- Saitoh, T.; Sajiki, T.; Maruhara, K. Bench mark solutions to natural convection heat transfer problem around a horizontal circular cylinder. Int. J. Heat Mass Transf. 1993, 36, 1251–1259. [Google Scholar] [CrossRef]
- Moukalled, F.; Acharya, S. Natural convection in the annulus between concentric horizontal circular and square cylinders. J. Thermophys. Heat Transf. 1996, 10, 524–531. [Google Scholar] [CrossRef]
- Kitamura, K.; Kami-iwa, F.; Misumi, T. Heat transfer and fluid flow of natural convection around large horizontal cylinders. Int. J. Heat Mass Transf. 1999, 42, 4093–4106. [Google Scholar] [CrossRef]
- Cesini, G.; Paroncini, M.; Cortella, G.; Manzan, M. Natural convection from a horizontal cylinder in a rectangular cavity. Int. J. Heat Mass Transf. 1999, 42, 1801–1811. [Google Scholar] [CrossRef]
- Sadeghipour, M.S.; Razi, Y.P. Natural convection from a confined horizontal cylinder: The optimum distance between the confining walls. Int. J. Heat Mass Transf. 2001, 44, 367–374. [Google Scholar] [CrossRef]
- Atmane, M.A.; Chan, V.S.S.; Murray, D.B. Natural convection around a horizontal heated cylinder: The effects of vertical confinement. Int. J. Heat Mass Transf. 2003, 46, 3661–3672. [Google Scholar] [CrossRef]
- Ashjaee, M.; Eshtiaghi, A.H.; Yaghoubi, M.; Yousefi, T. Experimental investigation on free convection from a horizontal cylinder beneath an adiabatic ceiling. Exp. Therm. Fluid Sci. 2007, 32, 614–623. [Google Scholar] [CrossRef]
- Atayılmaz, Ş.Ö.; Teke, İ. Experimental and numerical study of the natural convection from a heated horizontal cylinder. Int. Commun. Heat Mass Transf. 2009, 36, 731–738. [Google Scholar] [CrossRef]
- Angeli, D.; Levoni, P.; Barozzi, G.S. Numerical predictions for stable buoyant regimes within a square cavity containing a heated horizontal cylinder. Int. J. Heat Mass Transf. 2008, 51, 553–565. [Google Scholar] [CrossRef]
- Kim, B.S.; Lee, D.S.; Ha, M.Y.; Yoon, H.S. A numerical study of natural convection in a square enclosure with a circular cylinder at different vertical locations. Int. J. Heat Mass Transf. 2008, 51, 1888–1906. [Google Scholar] [CrossRef]
- Yoon, H.S.; Ha, M.Y.; Kim, B.S.; Yu, D.H. Effect of the position of a circular cylinder in a square enclosure on natural convection at Rayleigh number of 107. Phys. Fluids 2009, 21, 047101. [Google Scholar] [CrossRef]
- Angeli, D.; Pagano, A.; Corticelli, M.A.; Fichera, A.; Barozzi, G.S. Bifurcations of natural convection flows from an enclosed cylindrical heat source. Front. Heat Mass Transf. 2011, 2, 023003. [Google Scholar] [CrossRef]
- Ashjaee, M.; Yazdani, S.; Bigham, S.; Yousefi, T. Experimental and Numerical Investigation on Free Convection from a Horizontal Cylinder Located Above an Adiabatic Surface. Heat Transf. Eng. 2012, 33, 213–224. [Google Scholar] [CrossRef]
- Kang, D.H.; Ha, M.Y.; Yoon, H.S.; Choi, C.H. Bifurcation to unsteady natural convection in square enclosure with a circular cylinder at Rayleigh number of 107. Int. J. Heat Mass Transf. 2013, 64, 926–944. [Google Scholar] [CrossRef]
- Sebastian, G.; Shine, S.R. Natural convection from horizontal heated cylinder with and without horizontal confinement. Int. J. Heat Mass Transf. 2015, 82, 325–334. [Google Scholar] [CrossRef]
- Abou-Ziyan, H.; Kalender, A.; Shedid, M.; Abdel-Hameed, H. Experimental investigation of free convection from short horizontal cylinder to Newtonian and power-law liquids of large Prandtl numbers. Exp. Therm. Fluid Sci. 2017, 86, 102–116. [Google Scholar] [CrossRef]
- Rath, S.; Dash, M.K.; Dash, S.K. Natural convection from horizontal cylinders of different shapes in a rectangular enclosure. In Proceedings of the 24th National and 2nd International ISHMT-ASTFE Heat and Mass Transfer Conference (IHMTC-2017), Hyderabad, India, 27–30 December 2017. [Google Scholar]
- Lin, K.C.; Bhosale, Y.; Huang, C.-Y.Z. 3D-CFD investigation into free convection flow above a heated horizontal cylinder: Comparisons with experimental data. Appl. Therm. Eng. 2017, 120, 277–288. [Google Scholar] [CrossRef]
- Ma, H.; He, L.; Rane, S. Heat Transfer-Fluid Flow Interaction in Natural Convection around Heated Cylinder and Its Thermal Chimney Effect. In Proceedings of the International Conference Innovative Applied Energy (IAPE’19), Oxford, UK, 14–15 March 2019. [Google Scholar]
- Kuehner, J.P.; Pflug, J.R.; Tessier, F.A., Jr.; Hamed, A.M.; Marin, F.J.M. Velocity measurements in the free convection flow above a heated horizontal cylinder. Int. J. Heat Mass Transf. 2012, 55, 4711–4723. [Google Scholar] [CrossRef]
- Fiscaletti, D.; Angeli, D.; Tarozzi, L.; Barozzi, G.S. Buoyancy-induced transitional flows around an enclosed horizontal cylinder: An experiment. Int. J. Heat Mass Transf. 2013, 58, 619–631. [Google Scholar] [CrossRef]
- Grafsrønningen, S.; Jensen, A. Natural convection heat transfer from two horizontal cylinders at high Rayleigh numbers. Int. J. Heat Mass Transf. 2012, 55, 5552–5564. [Google Scholar] [CrossRef]
- American Society of Heating, Refrigerating and Air-Conditioning Engineers. ASHRAE Handbook—Fundamentals; ASHRAE: Atlanta, GA, USA, 2005. [Google Scholar]
- Cieśliński, J.T.; Pudlik, W. Laminar free-convection from spherical segments. Int. J. Heat Fluid Flow 1988, 9, 405–409. [Google Scholar] [CrossRef]
- Lewandowski, W.M.; Bieszk, H.; Cieśliński, J.T. Free convection from horizontal screened plates. Wärme-und Stoffübertragung 1992, 27, 481–488. [Google Scholar] [CrossRef]
- Cieśliński, J.T.; Krygier, K. Free convection of water-Al2O3 nanofluid from horizontal porous coated tube. Key Eng. Mater. 2014, 597, 15–20. [Google Scholar] [CrossRef]
- Lienhard, J.H. On the commonality of equations for natural convection from immersed bodies. Int. J. Heat Mass Transf. 1973, 16, 2121–2123. [Google Scholar] [CrossRef]

| Authors [Reference] | Equation | Nu-Ra Relationship | Ra and Pr Range; tch and Lch | Surface Condition |
|---|---|---|---|---|
| Churchill and Chu [4] | Equation (1a) Equation (1b) | tuni and quni | ||
| any | ||||
| tch = tfm | ||||
| Lch = D | ||||
| Morgan [2] | Equation (2) | B = 1.02 and m = 0.148, 10−2 < Ra ≤ 102 B = 0.85 and m = 0.188, 102 < Ra ≤ 104 B = 0.48 and m = 0.25, 104 < Ra ≤ 107 B = 0.125 and m = 0.333, 107 < Ra ≤ 1012 | tuni | |
| Pr—not stated | ||||
| tch = tfm | ||||
| Lchar = D | ||||
| Kuehn and Goldstein [6] | Equation (3) | tuni | ||
| any Pr | ||||
| tch—not stated | ||||
| Lch = D | ||||
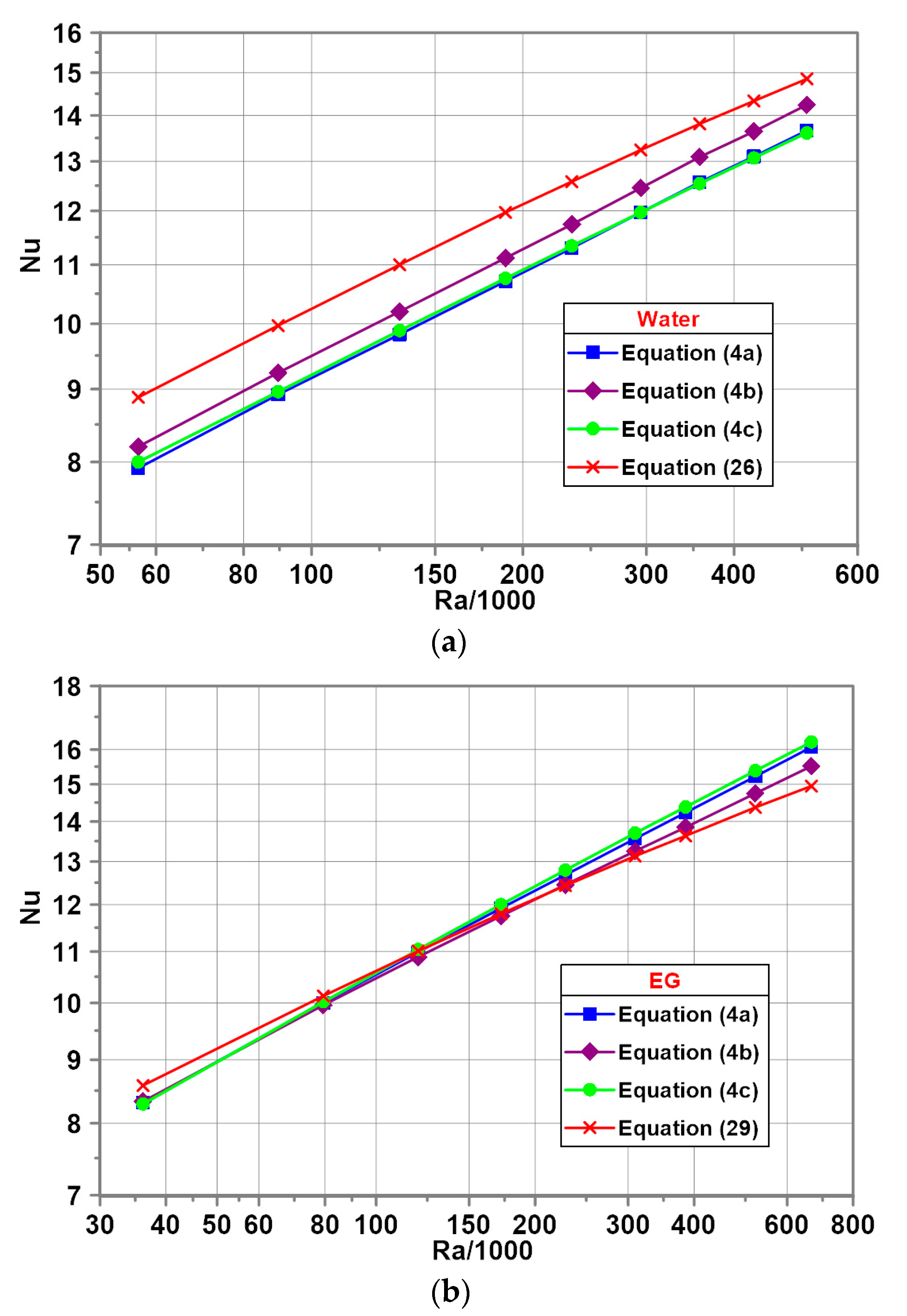
| Equation (4a) | tuni | |||
| 0.7 3090 | ||||
| tch = t∞ and tch = tw | ||||
| Lch = D | ||||
| Fand, Morris, Lum [7] | Equation (4b) | tchar = t∞ + 0.32(tw − t∞) | ||
| Equation (4c) | tuni | |||
| Fand and Brucker [10] | Equation (5) | |||
| 0.7 | ||||
| Lch = D | ||||
| Warrington and Powe [13] | Equation (6a) | , Ri—hypothetical radius of an inner sphere of a volume equal to the cylindrical body Ro—outer body hypothetical radius equal to the radius of a sphere having a volume equal to the volume of the outer body | tuni | |
| 0.7 | ||||
| Equation (6b) | ||||
| Lch = G | ||||
| Karim et al. [14] | Equation (7) | tuni | ||
| Pr = 0.7 | ||||
| Lch = D | ||||
| Ghaddar [16] | Equation (8a) | —see Equation (6a) | quni | |
| Pr = 0.7 | ||||
| Lch=G | ||||
| Equation (8b) | ||||
| Pr = 0.7 | ||||
| Lch = b | ||||
| Kitamura et al. [19] | Equation (9) | quni | ||
| Pr = 6 | ||||
| Lch = D | ||||
| Sadeghipour and Razi [21] | Equation (10) | and | tuni | |
| Pr = 0.7 | ||||
| Lch = D | ||||
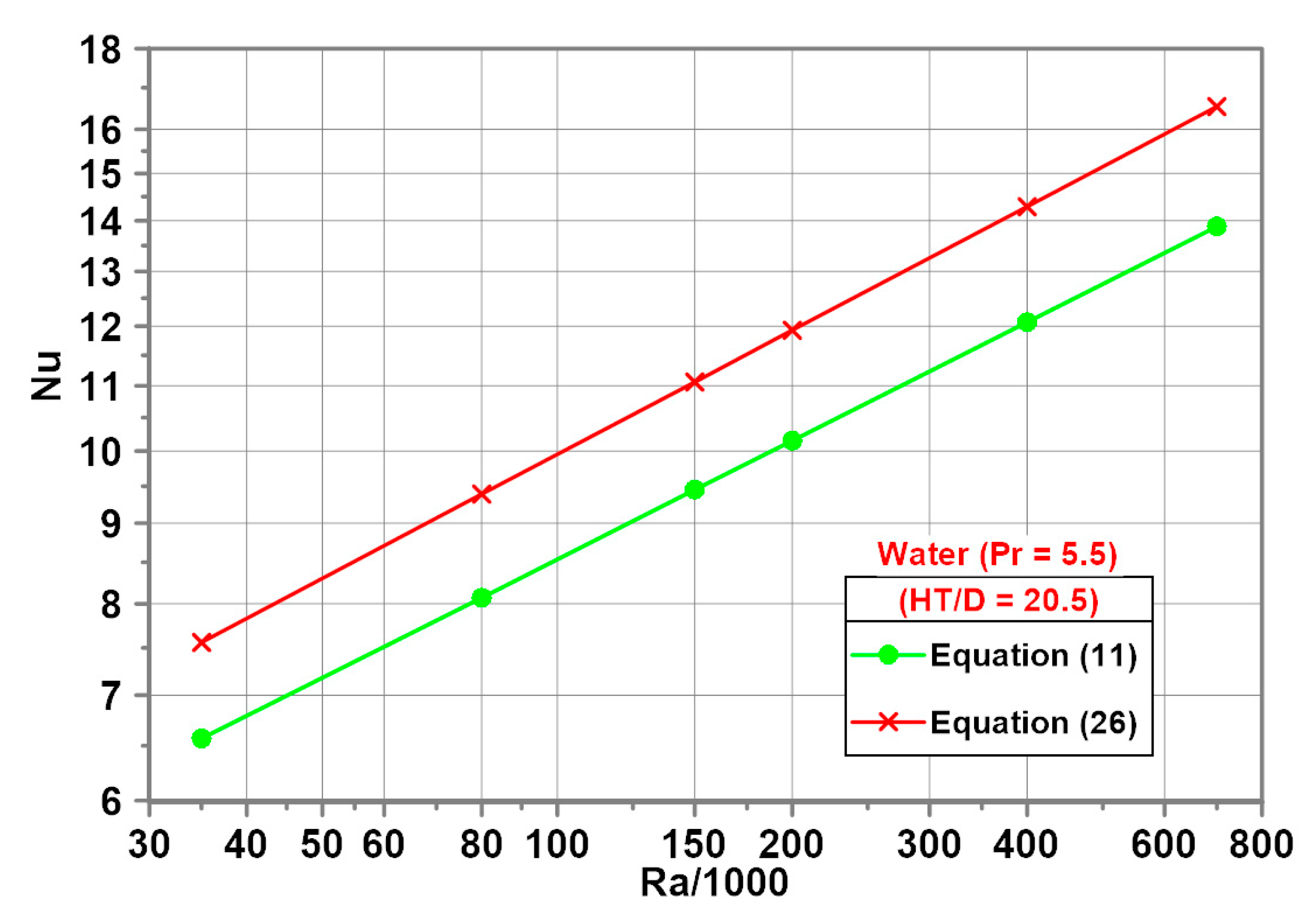
| Ashjaee et al. [23] | Equation (11) | tuni | ||
| Pr = 0.7 | ||||
| Lch = D | ||||
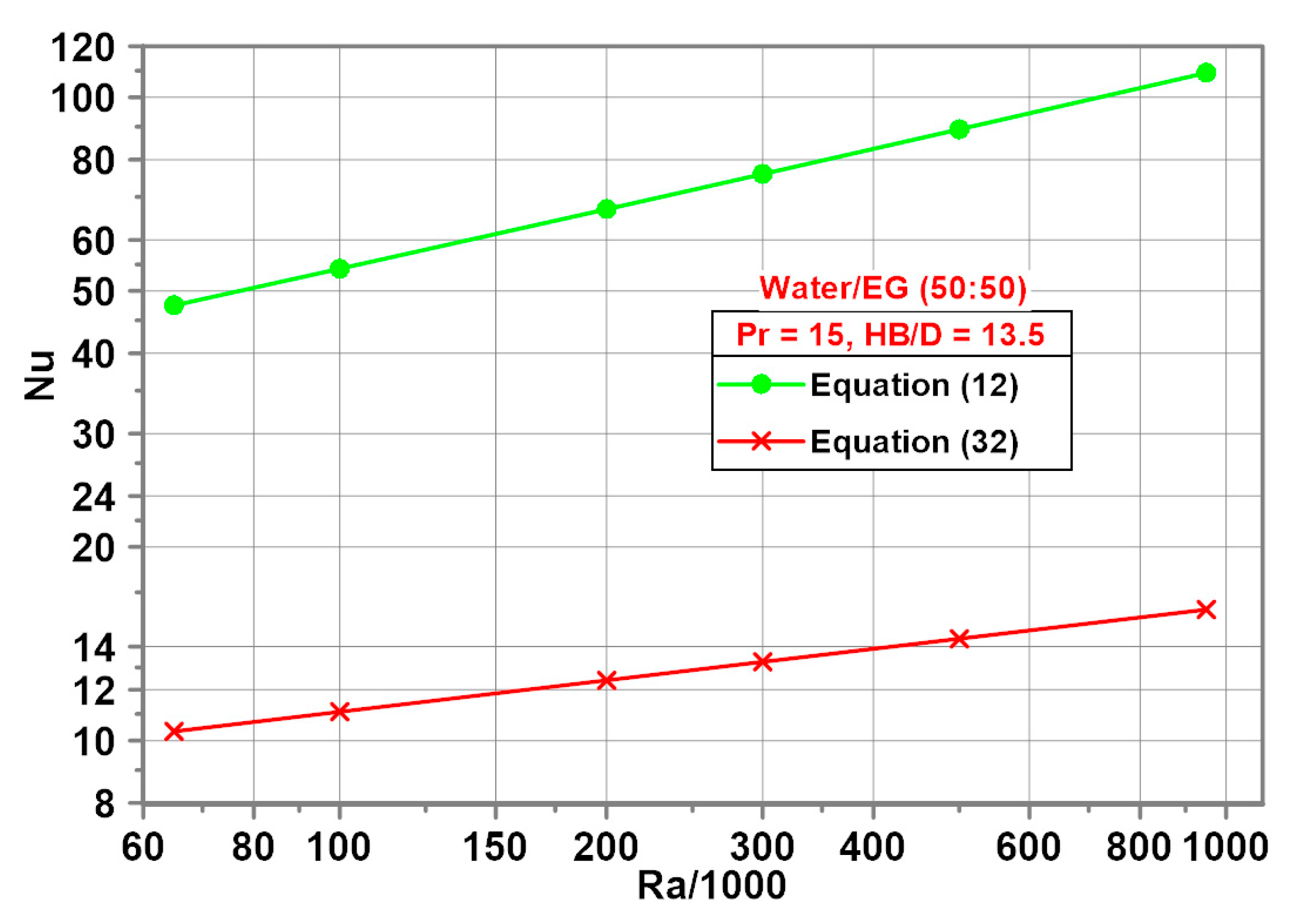
| Ashjaee et al. [29] | Equation (12) | tuni | ||
| Pr = 0.7 | ||||
| Lch = D | ||||
| Abou-Ziyan et al. [32] | Equation (13) | γ—heat generation parameter , | quni | |
| tchar = t∞ + 0.32(tw − t∞) | ||||
| Lchar = D |
| Liquid | Present Correlation | Equation Number |
|---|---|---|
| Water | Equation (14) | |
| EG | Equation (15) | |
| Water/EG (50:50) | Equation (16) |
| Liquid | Correlation | Equation Number |
|---|---|---|
| Water | Equation (17) | |
| EG | Equation (18) | |
| Water/EG (50:50) | Equation (19) |
| Liquid | ρ [kg/m3] | cp [J/(kgK)] | β [1/K] |
|---|---|---|---|
| Water | |||
| EG | |||
| Mixture W/EG (50/50) by vol. |
| Liquid | Equation Number | Relationship | Ra Range | Pr Range | Data within 5% Equation |
|---|---|---|---|---|---|
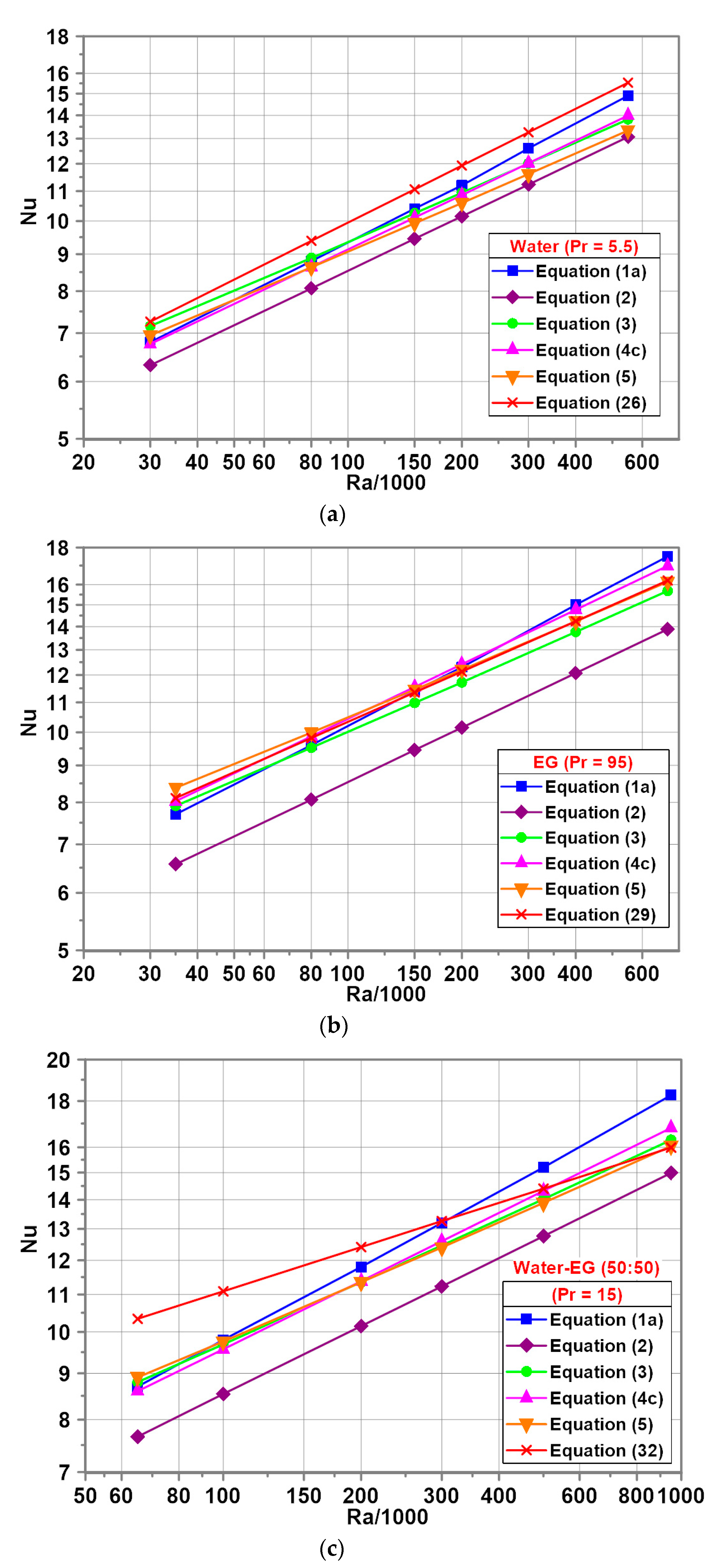
| Water | Equation (26) | 5.2 × 104 < Ra < 5.1 × 105 | 4.5 < Pr < 7 | 88% | |
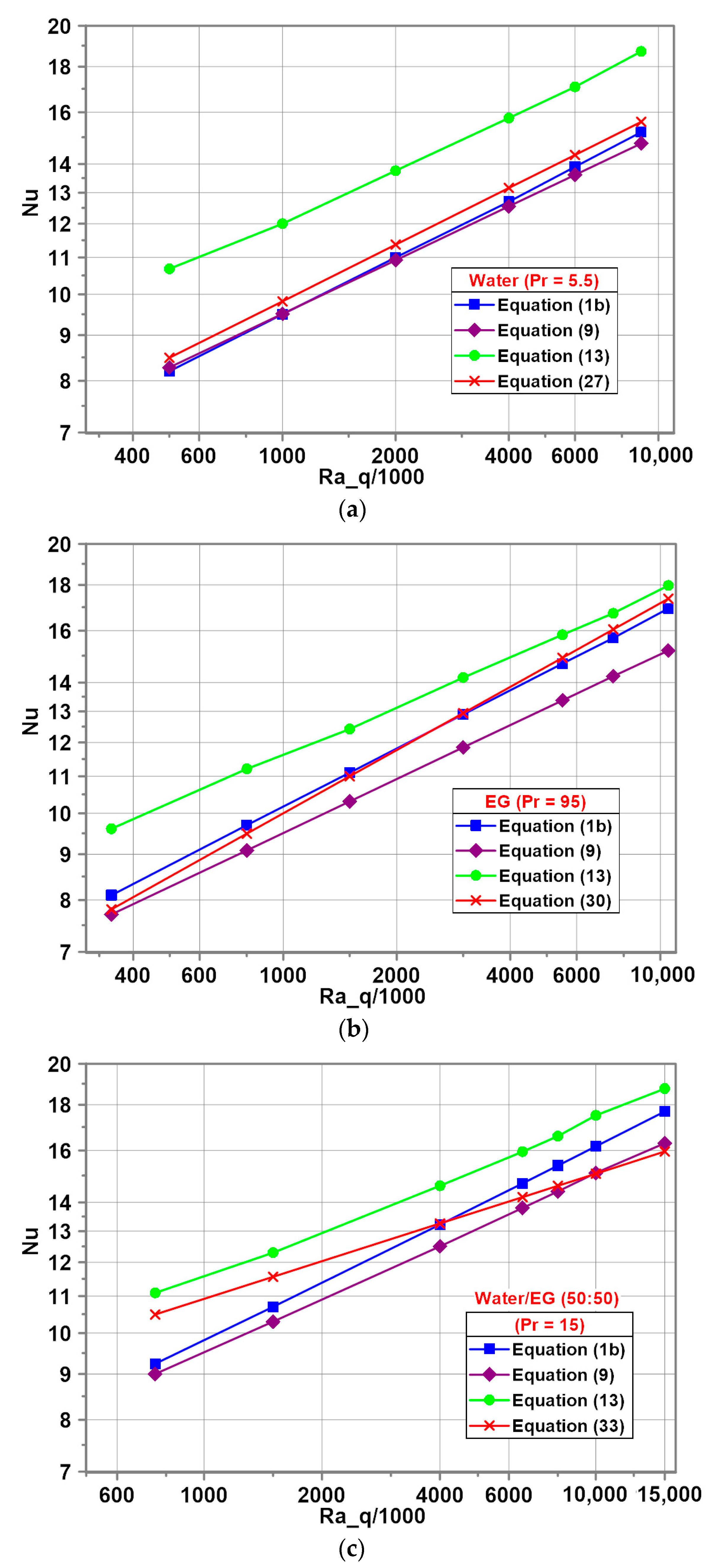
| Equation (27) | 4.7 × 105 < Raq < 9 × 106 | 92% | |||
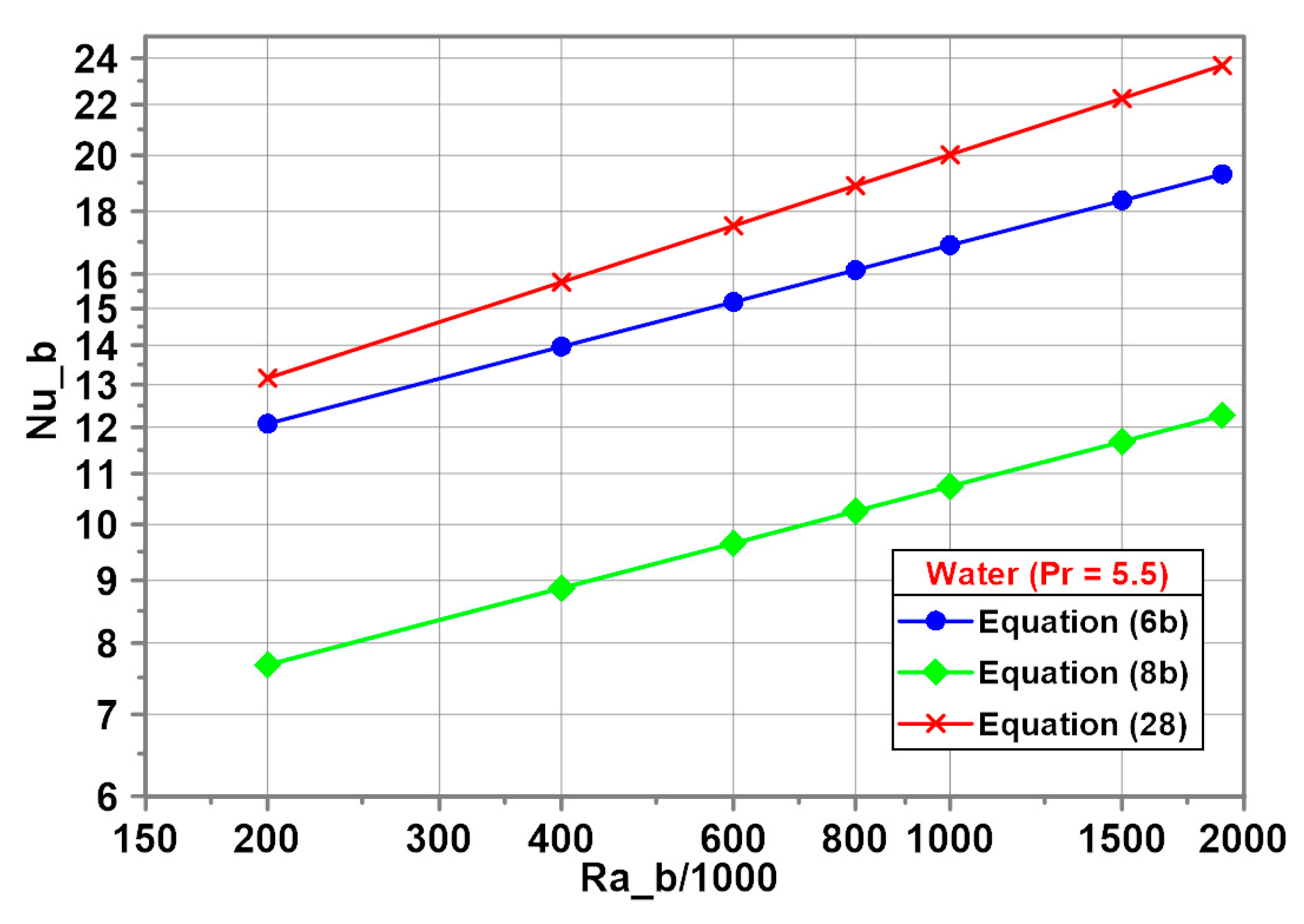
| Equation (28) | 2 × 105 < Rab < 2 × 106 | 88% | |||
| EG | Equation (29) | 3.6 × 104 < Ra< 6.7 × 105 | 45 < Pr < 160 | 64% | |
| Equation (30) | 3.6 × 105 < Raq < 10.3 × 106 | 72% | |||
| Equation (31) | 1.4 × 105 < Rab < 2.6 × 106 | 64% | |||
| W/EG (50:50) | Equation (32) | 6.8 × 104 < Ra < 9.2 × 105 | 10 < Pr < 25 | 100% | |
| Equation (33) | 7.3 × 105 < Raq < 14.8 × 106 | 100% | |||
| Equation (34) | 2.7 × 105 < Rab < 3.6 × 106 | 100% | |||
| All | Equation (35) | 3.6 × 104 < Ra < 9.2 × 105 | 4.5 < Pr < 160 | 72% | |
| Equation (36) | 3.6 × 105 < Raq < 14.8 × 106 | 76% | |||
| Equation (37) | 1.4 × 105 < Rab < 3.6 × 106 | 72% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cieśliński, J.T.; Smolen, S.; Sawicka, D. Free Convection Heat Transfer from Horizontal Cylinders. Energies 2021, 14, 559. https://doi.org/10.3390/en14030559
Cieśliński JT, Smolen S, Sawicka D. Free Convection Heat Transfer from Horizontal Cylinders. Energies. 2021; 14(3):559. https://doi.org/10.3390/en14030559
Chicago/Turabian StyleCieśliński, Janusz T., Slawomir Smolen, and Dorota Sawicka. 2021. "Free Convection Heat Transfer from Horizontal Cylinders" Energies 14, no. 3: 559. https://doi.org/10.3390/en14030559
APA StyleCieśliński, J. T., Smolen, S., & Sawicka, D. (2021). Free Convection Heat Transfer from Horizontal Cylinders. Energies, 14(3), 559. https://doi.org/10.3390/en14030559





