Analysis and Experimental Verification of a Variable Speed Turbo Air Centrifugal Compressor System for Energy Saving
Abstract
:1. Introduction
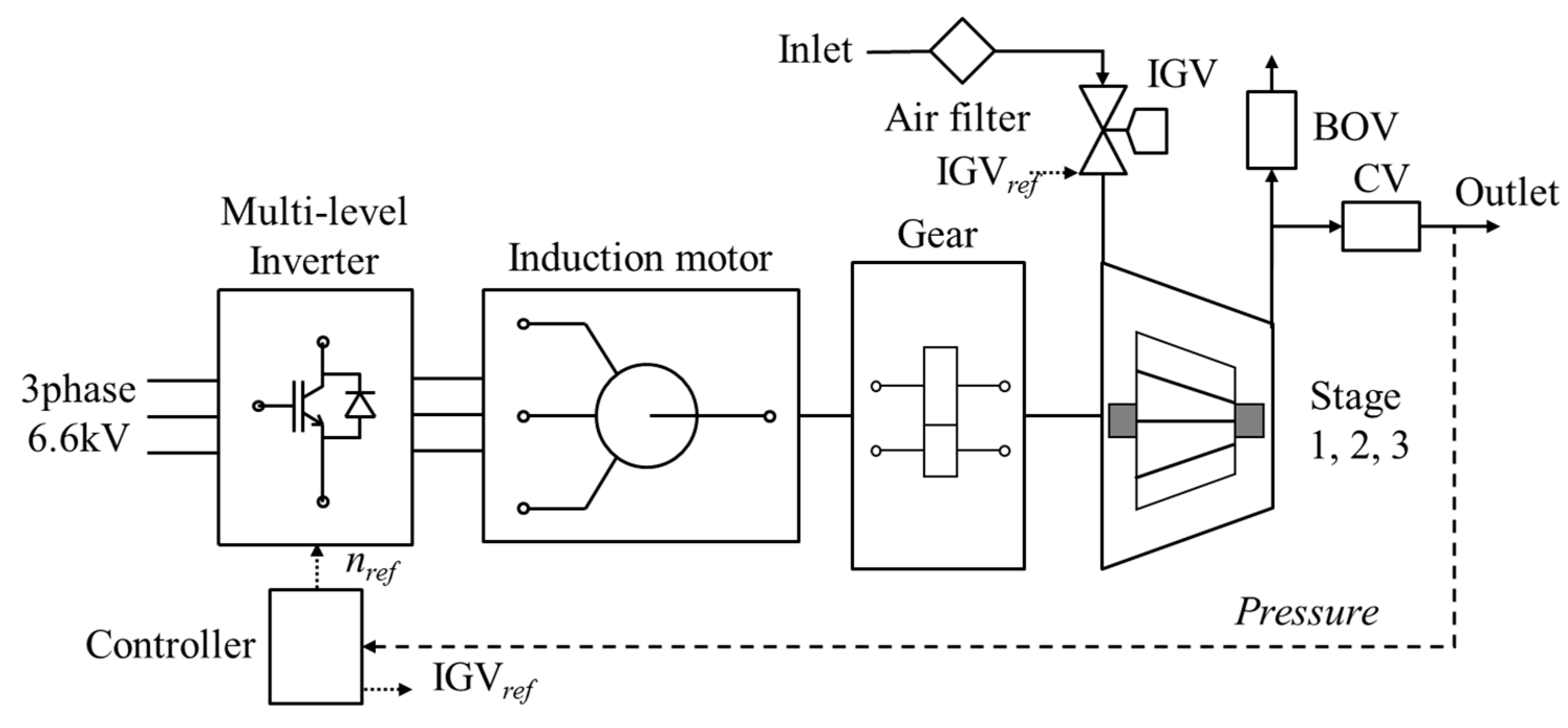
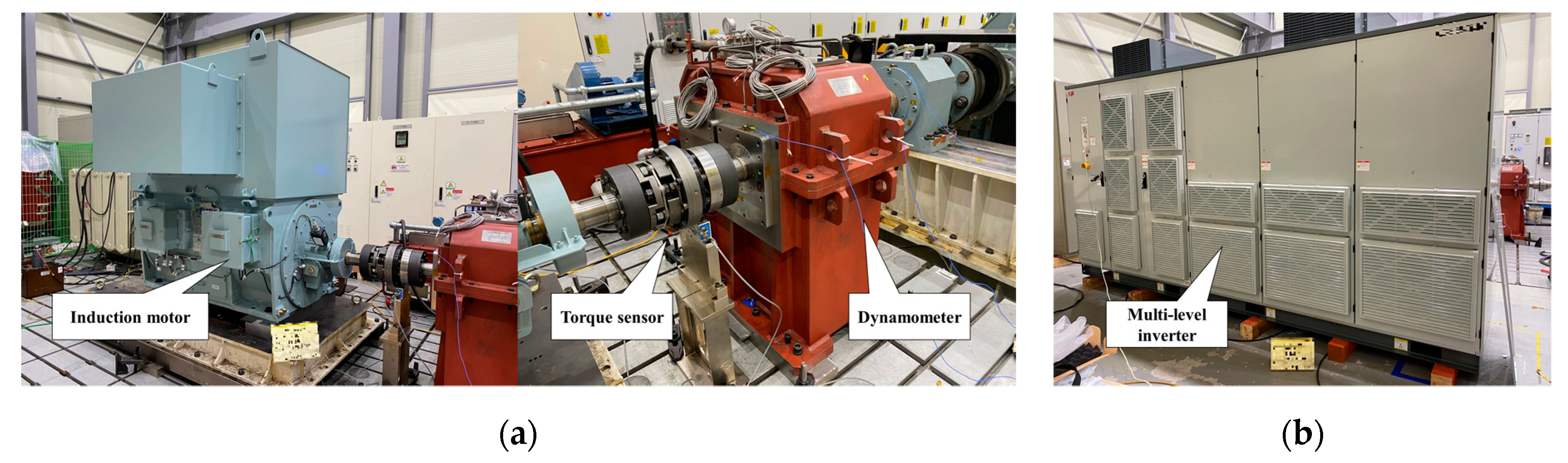
2. Configuration of TACCS
3. Performance Analysis of EMDS and TACCS
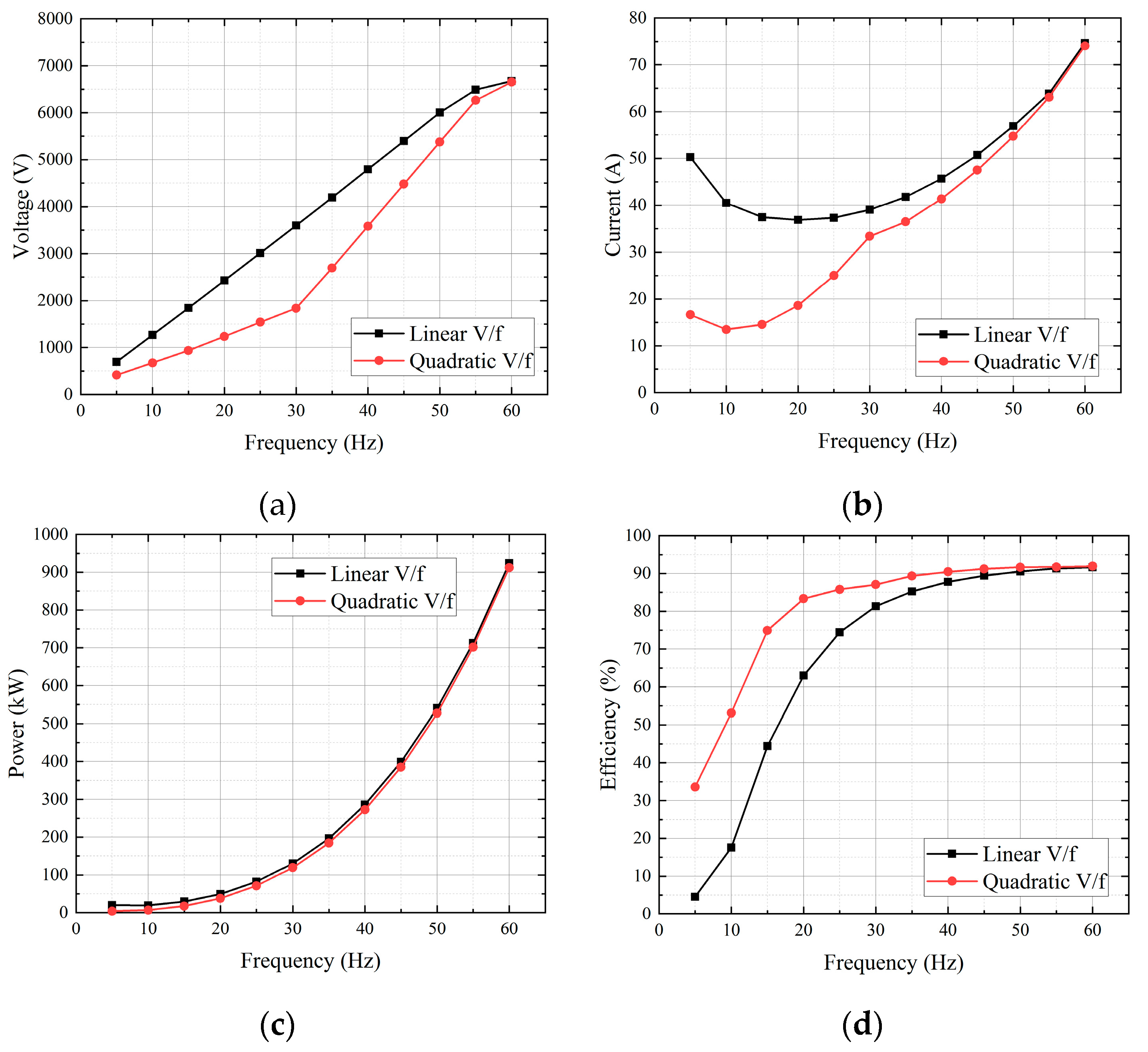
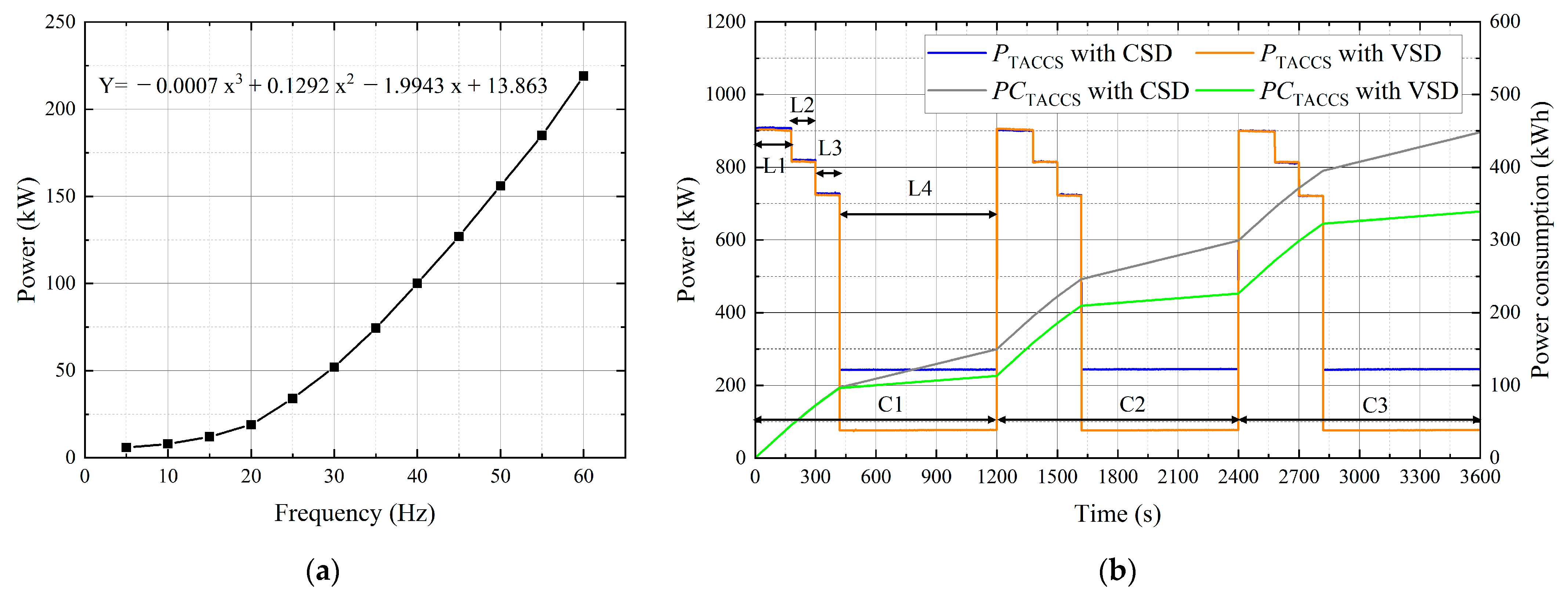
3.1. Performance Analysis of EMDS
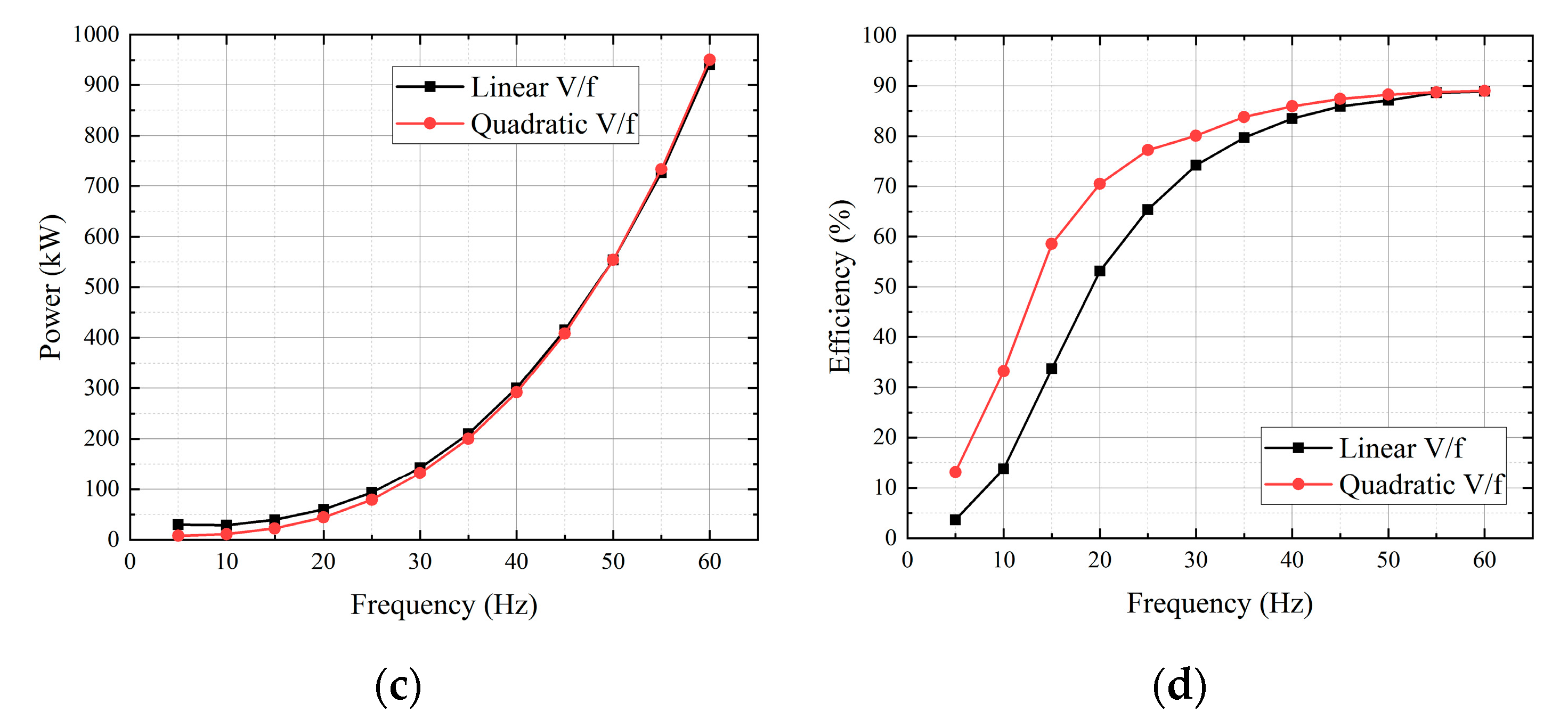
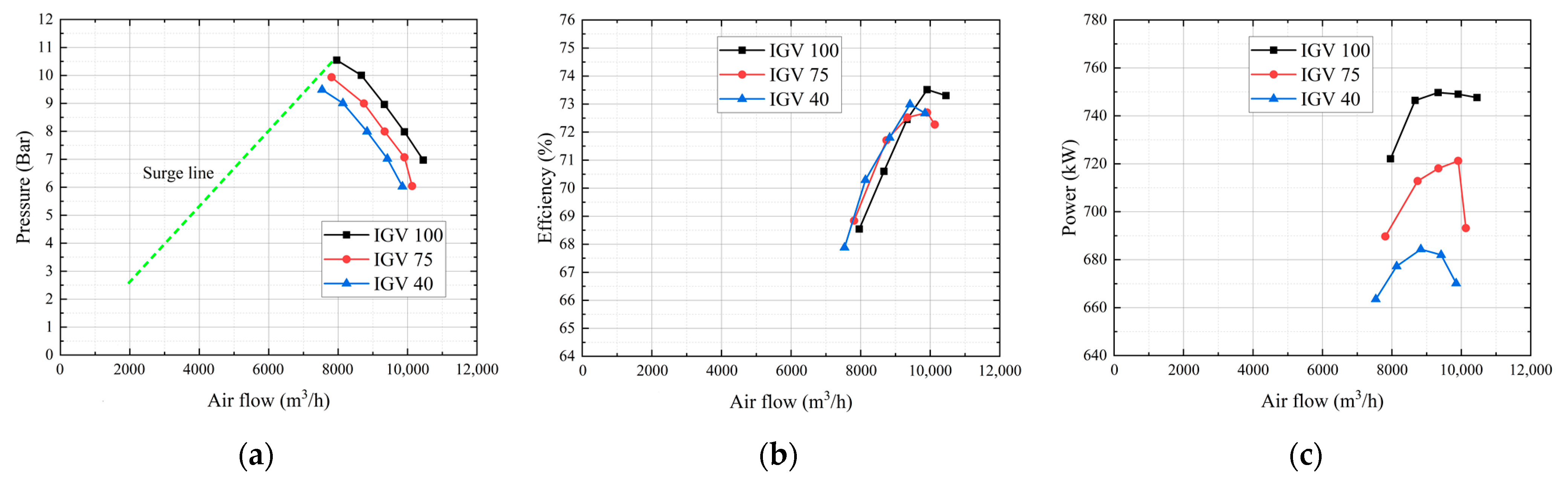
3.2. Perfomance Analysis of TACCS
4. Economic Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Li, H.; Zhang, X.; Xu, F. Experimental Investigation on Centrifugal Compressor Blade Crack Classification Using the Squared Envelope Spectrum. Sensors 2013, 13, 12548–12563. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Baek, G.Y.; Seo, D.H.; In, B.S. Gas compressor introduction and development trend. KSFM J. Fluid Mach. 2009, 12, 76–80. [Google Scholar]
- Gwon, U. Industrial compressor technology status and development. J. KSME 2012, 52, 45–48. [Google Scholar]
- Cha, B.J. Compressor research trend. KSFM J. Fluid Mach. 2007, 10, 85–90. [Google Scholar] [CrossRef]
- Robison, D.H.; Beaty, P.J. Compressor types, classifications, and applications. In Proceedings of the 21st Turbomachinery Symposium, Texas A&M University, Turbomachinery Laboratories, Dallas, TX, USA, 28 September–1 October 1992. [Google Scholar]
- Jiang, W.; Khan, J.; Dougal, R.A. Dynamic centrifugal compressor model for system simulation. J. Power Sources 2006, 158, 1333–1343. [Google Scholar] [CrossRef]
- Zhao, D.; Blunier, B.; Dou, M.; Miraoui, A. Control of an ultra high speed centrifugal compressor for the air management of fuel cell systems. In Proceedings of the 2012 IEEE Industry Applications Society Annual Meeting, Las Vegas, NV, USA, 7–11 October 2012; pp. 1–8. [Google Scholar]
- Xu, C.; Muller, M. Development and Design of a Centrifugal Compressor Volute. Int. J. Rotating Mach. 2005, 2005, 190–196. [Google Scholar] [CrossRef] [Green Version]
- Munari, E.; D’Elia, G.; Morini, M.; Mucchi, E.; Pinelli, M.; Spina, P.R. Experimental Investigation of Vibrational and Acoustic Phenomena for Detecting the Stall and Surge of a Multistage Compressor. J. Eng. Gas Turbines Power 2018, 140, 092605. [Google Scholar] [CrossRef]
- Casey, M.; Robinson, C. A Method to Estimate the Performance Map of a Centrifugal Compressor Stage. J. Turbomach. 2012, 135, 021034. [Google Scholar] [CrossRef]
- Mojaddam, M.; Pullen, K.R. Optimization of a Centrifugal Compressor Using the Design of Experiment Technique. Appl. Sci. 2019, 9, 291. [Google Scholar] [CrossRef] [Green Version]
- Kim, S.-A.; Hong, K.-P. Development of Average Model to Analysis Dynamic Characteristics of Variable Speed Driven Turbo Air Compressor System with Multi Level Inverter. Trans. Korean Inst. Electr. Eng. 2020, 69, 1200–1207. [Google Scholar] [CrossRef]
- Ristanovic, D.; Taher, M.; Getschmann, T.; Bhatia, N. Large Synchronous Motors as Drivers for Centrifugal Compressors in LNG Liquefaction Plants. IEEE Trans. Ind. Appl. 2020, 56, 6083–6093. [Google Scholar] [CrossRef]
- Mohseni, A.; Goldhahn, E.; Braembussche, R.A.V.D.; Seume, J.R. Novel IGV Designs for Centrifugal Compressors and Their Interaction with the Impeller. J. Turbomach. 2011, 134, 021006. [Google Scholar] [CrossRef]
- Bentaleb, T.; Cacitti, A.; De Franciscis, S.; Garulli, A. Multivariable control for regulating high pressure centrifugal compressor with variable speed and IGV. In Proceedings of the 2014 IEEE Conference on Control Applications (CCA), Juan Les Antibes, France, 8–10 October 2014; pp. 486–491. [Google Scholar]
- Lim, J.; Yoon, M.S.; Al-Qahtani, T.; Nam, Y. Feasibility Study on Variable-Speed Air Conditioner under Hot Climate based on Real-Scale Experiment and Energy Simulation. Energies 2019, 12, 1489. [Google Scholar] [CrossRef] [Green Version]
- Chen, I.Y.; Chen, Y.M.; Chang, Y.J.; Wei, C.S.; Wang, C.C. A comparative study between a constant-speed air-conditioner and a variable-speed air-conditioner. ASHRAE Trans. 2009, 115, 326–332. [Google Scholar]
- Al-Tamimi, O.; Kassas, M. Experimental investigation of energy consumption of VFD and ON/OFF A/C systems in residential area in Saudi Arabia. In Proceedings of the 2018 IEEE International Conference on Industrial Technology (ICIT), Lyon, France, 20–22 February 2018; pp. 401–406. [Google Scholar]
- Yussif, N.; Sabry, O.H.; Abdel-Khalik, A.S.; Ahmed, S.; Mohamed, A.M. Enhanced Quadratic V/f-Based Induction Motor Control of Solar Water Pumping System. Energies 2020, 14, 104. [Google Scholar] [CrossRef]






| Item | Value | Item | Value | ||
|---|---|---|---|---|---|
| Turbo air compressor | Number of stages | 3 | Motor | Motor type | Induction |
| Rated air flow rate | 10,000 m3/h | Rated voltage | 6.6 kV | ||
| Shaft power | 848.9 kW | No. of poles | 2 | ||
| Rated pressure | 8 bar | Rated power | 1050 kW | ||
| Shaft speed | 3600 rpm | Inverter | Input voltage | 6.6 KV | |
| Type | Centrifugal | Topology | 11 level | ||
| Gear ratios | 1:8.8:8.8:10.6 | Switching frequency | 10 kHz | ||
| Control method | V/f | ||||
| Section | Power Consumption (kWh) of the CSD | Power Consumption (kWh) of the VSD | ||||
|---|---|---|---|---|---|---|
| Cycle 1 | Cycle 2 | Cycle 3 | Cycle 1 | Cycle 2 | Cycle 3 | |
| 1 | 45.42 | 45.07 | 44.95 | 45.22 | 44.99 | 44.95 |
| 2 | 27.33 | 27.17 | 27.08 | 27.18 | 27.15 | 27.14 |
| 3 | 24.25 | 24.13 | 24.05 | 24.13 | 24.08 | 24.06 |
| 4 | 52.74 | 52.95 | 52.90 | 16.72 | 16.71 | 16.70 |
| Subtotal | 149.74 | 149.33 | 149.01 | 113.15 | 113.17 | 112.85 |
| Total | 448.08 | 339.17 | ||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, S.-A.; Hong, K.-P. Analysis and Experimental Verification of a Variable Speed Turbo Air Centrifugal Compressor System for Energy Saving. Energies 2021, 14, 1208. https://doi.org/10.3390/en14041208
Kim S-A, Hong K-P. Analysis and Experimental Verification of a Variable Speed Turbo Air Centrifugal Compressor System for Energy Saving. Energies. 2021; 14(4):1208. https://doi.org/10.3390/en14041208
Chicago/Turabian StyleKim, Sung-An, and Kyung-Pyo Hong. 2021. "Analysis and Experimental Verification of a Variable Speed Turbo Air Centrifugal Compressor System for Energy Saving" Energies 14, no. 4: 1208. https://doi.org/10.3390/en14041208
APA StyleKim, S.-A., & Hong, K.-P. (2021). Analysis and Experimental Verification of a Variable Speed Turbo Air Centrifugal Compressor System for Energy Saving. Energies, 14(4), 1208. https://doi.org/10.3390/en14041208







