Rain Erosion Load and Its Effect on Leading-Edge Lifetime and Potential of Erosion-Safe Mode at Wind Turbines in the North Sea and Baltic Sea
Abstract
1. Introduction
2. Data, Methodology, and Analysis
2.1. Data Selection
2.2. Data Quality Control and Precipitation Data Filtering
2.3. Turbine Aerodynamic Model
2.4. Rain Environmental Load
2.5. Modeling the Erosion Rate and Optimize the Profit Using Erosion-Safe Mode Operation
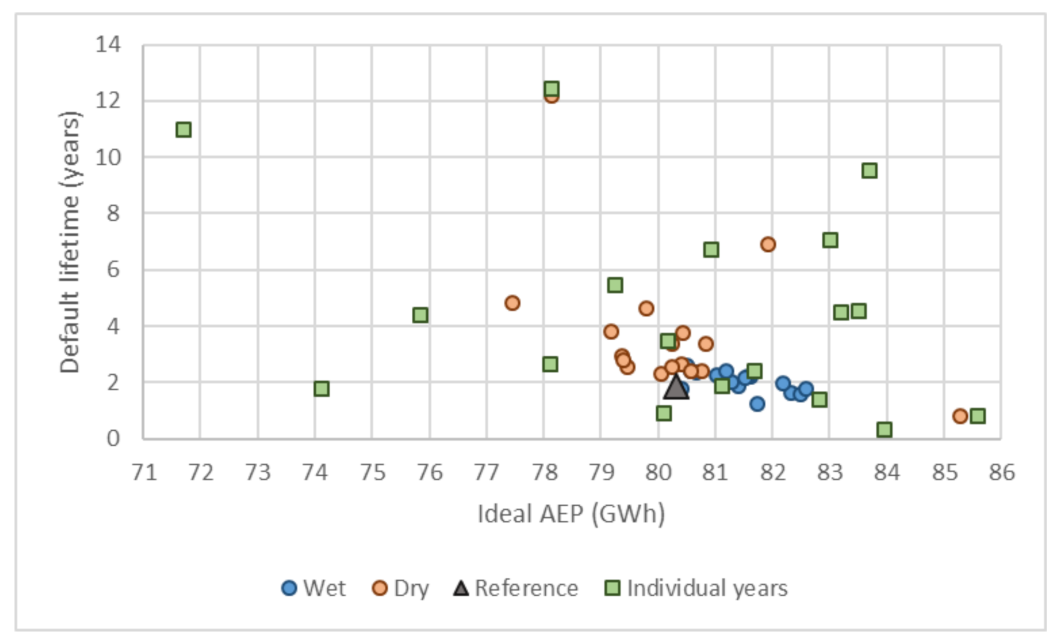
- The first case is the assumption of ideal blades. The ideal blades do not suffer from LEE and have a clean mechanical power curve throughout the lifetime. The time to repair is infinite. The AEP in percentage is 100%. This represents perfect coatings. AEP in GWh varies between stations due to variations in the wind resource but is completely unaffected by rain conditions.
- The second case is default operation, including a damage model. LEE is initiated during time, causing rougher blades. The roughening of the blades affects the aerodynamic performance. The loss in production due to rougher blades is modeled as a gradual progression using the eroded power curve. When the damage is at a certain level, determined by the damage model, the repair is necessary. The time until this happens is the average repair time (or blade lifetime). We call this default lifetime. After repair, the clean power curve is used again, followed by another cycle of erosion, etc. This represents the current practice in the wind industry. AEP and repair interval are functions of the wind speeds and the rain rate.
- The third case is erosion-safe mode (ESM) operation, including a damage model. As for the second case, the assumptions of LEE gradually growing during time and a change from a clean to an eroded power curve are the same. What is new is that the turbine is operated with lower revolutions per minute (RPM) during specific rain rates and wind speeds defined in the damage model as causing fast erosion. The reduction in RPM results in lower tip speed, hence in less LEE. This delay in the damage process ensures the time before the repair is longer. Furthermore, the loss in AEP due to severely eroded blades in operation using the eroded power curve is postponed. Potentially repair can be avoided with sufficient RPM reduction, but the balance is to optimize for profit for the turbine owner. This is to balance costs for repair and downtime versus earnings on AEP. AEP and repair interval are functions of the wind speeds and the rain rate as for the default case but with the difference that active choices are made to limit the impact of rain to the blades. Note the optimization is done for profit.
2.6. Sensitivity to Length of Time-Series
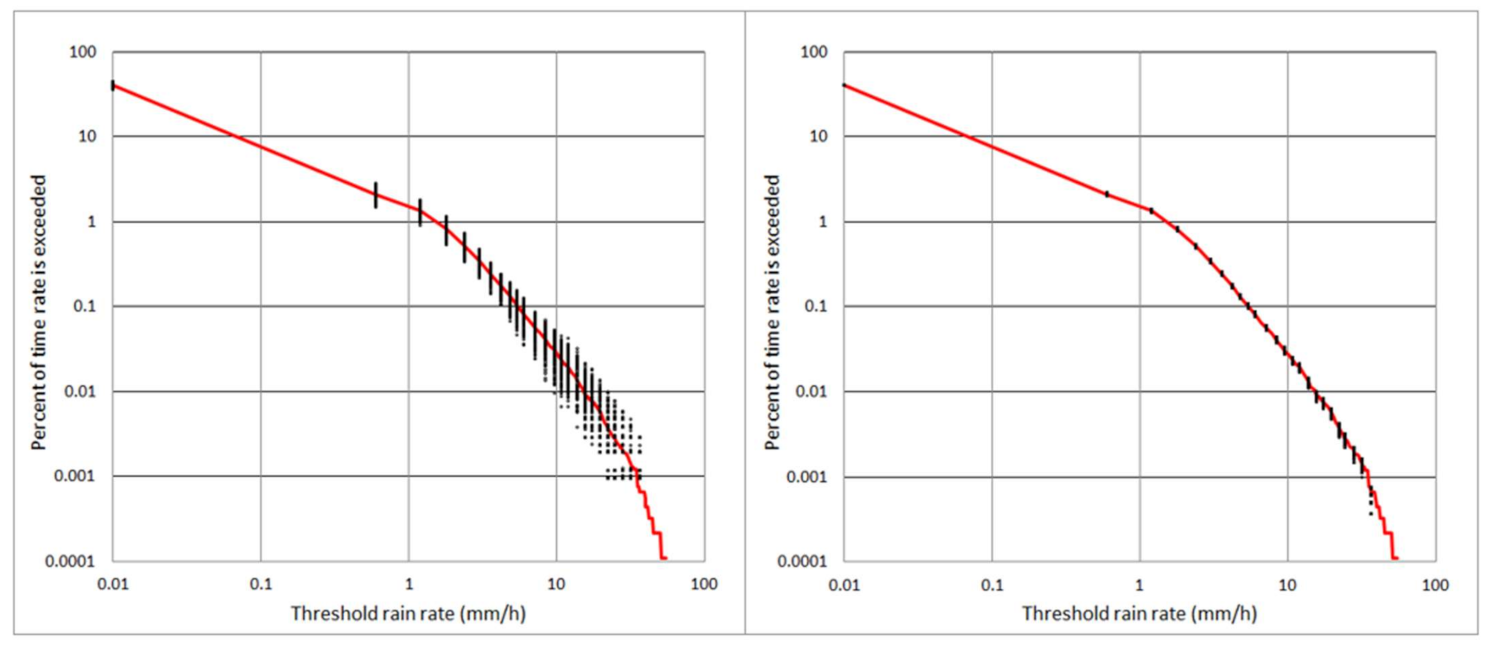
- Variation in percentage of time exceedance of rain rates for 2 sample sizes (2 years and 16 years), including all data.
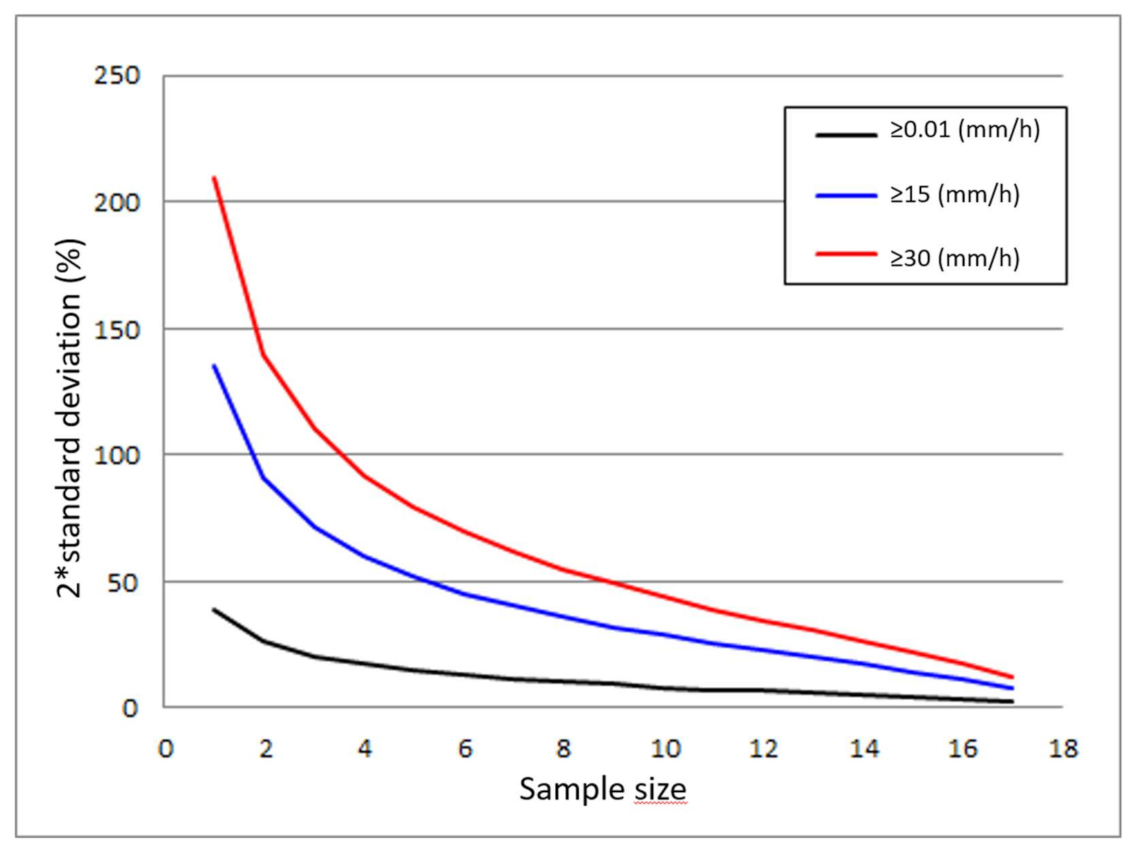
- Variation in +2 standard deviations (wet conditions) for 3 rain rates (≥0.01 mm h−1, ≥15 mm h−1, and ≥30 mm h−1) for 17 sample sizes including all data.
- Variation in lifetime in default operation for ±2 standard deviations (wet and dry conditions) for 17 sample sizes including representative selected data.
- Variation in lifetime based on 18 individual years from 2002 to 2019.
- The Reference is the entire 18 years’ time series.
2.7. Sensitivity to Correction of Rain Rate during High Wind Speed
3. Results
3.1. Results on Site-Specific Variability in Lifetime and Profit from ESM Operation
3.2. Results on Sensitivity to Length of Time Series for Predicted Lifetime
3.3. Results on Wind Speed Correction of Rain Rate Observations and Predicted Lifetime
4. Discussion
5. Conclusions
- Time series of more than 10 years duration appear to give robust results on blade lifetime, while shorter time series are likely to over-predict blade lifetime.
- Correction for wind speed on rain amount for specific rain gauge types is relevant as the uncorrected (under-catch time series) will over-predict blade lifetime.
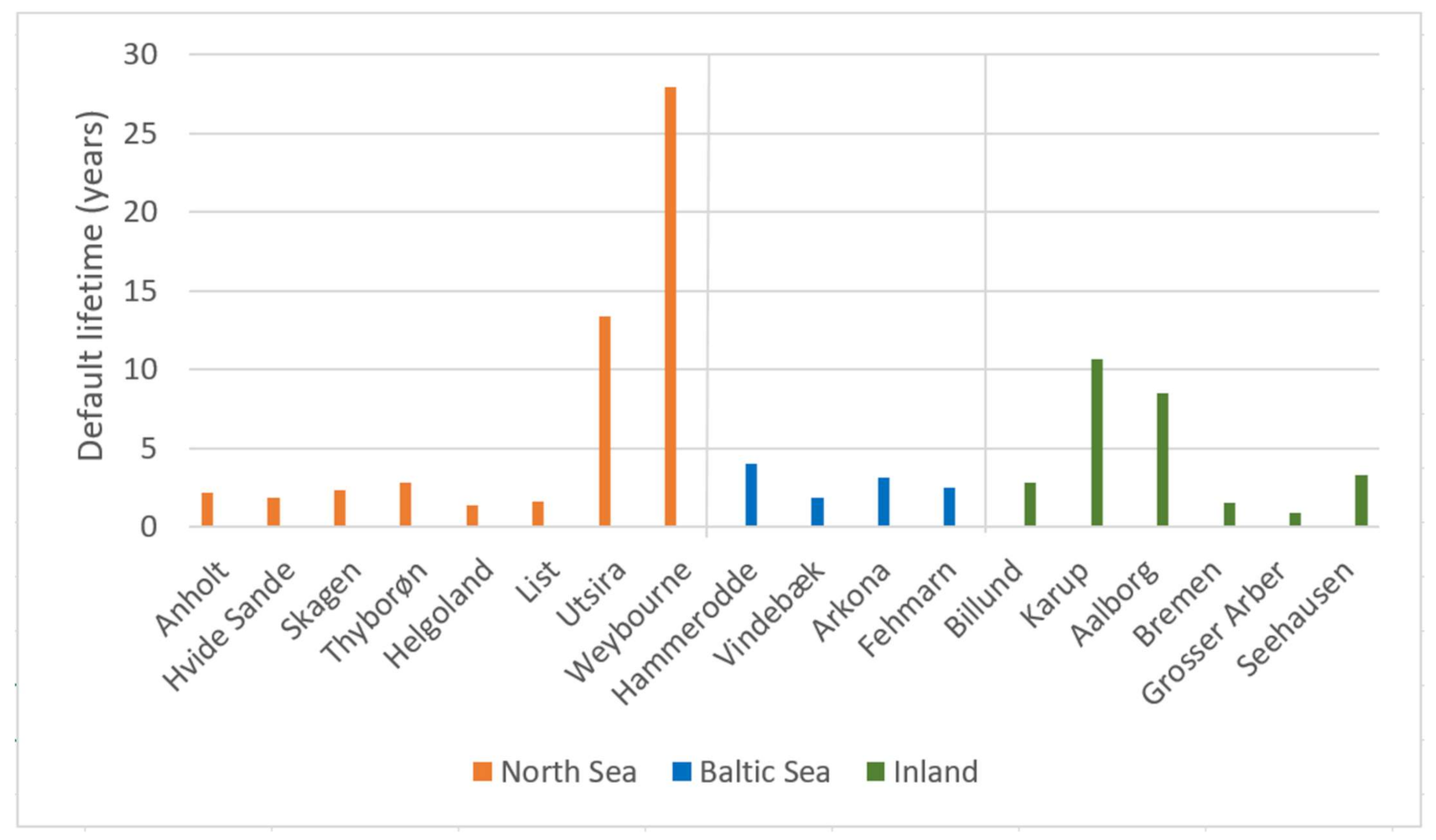
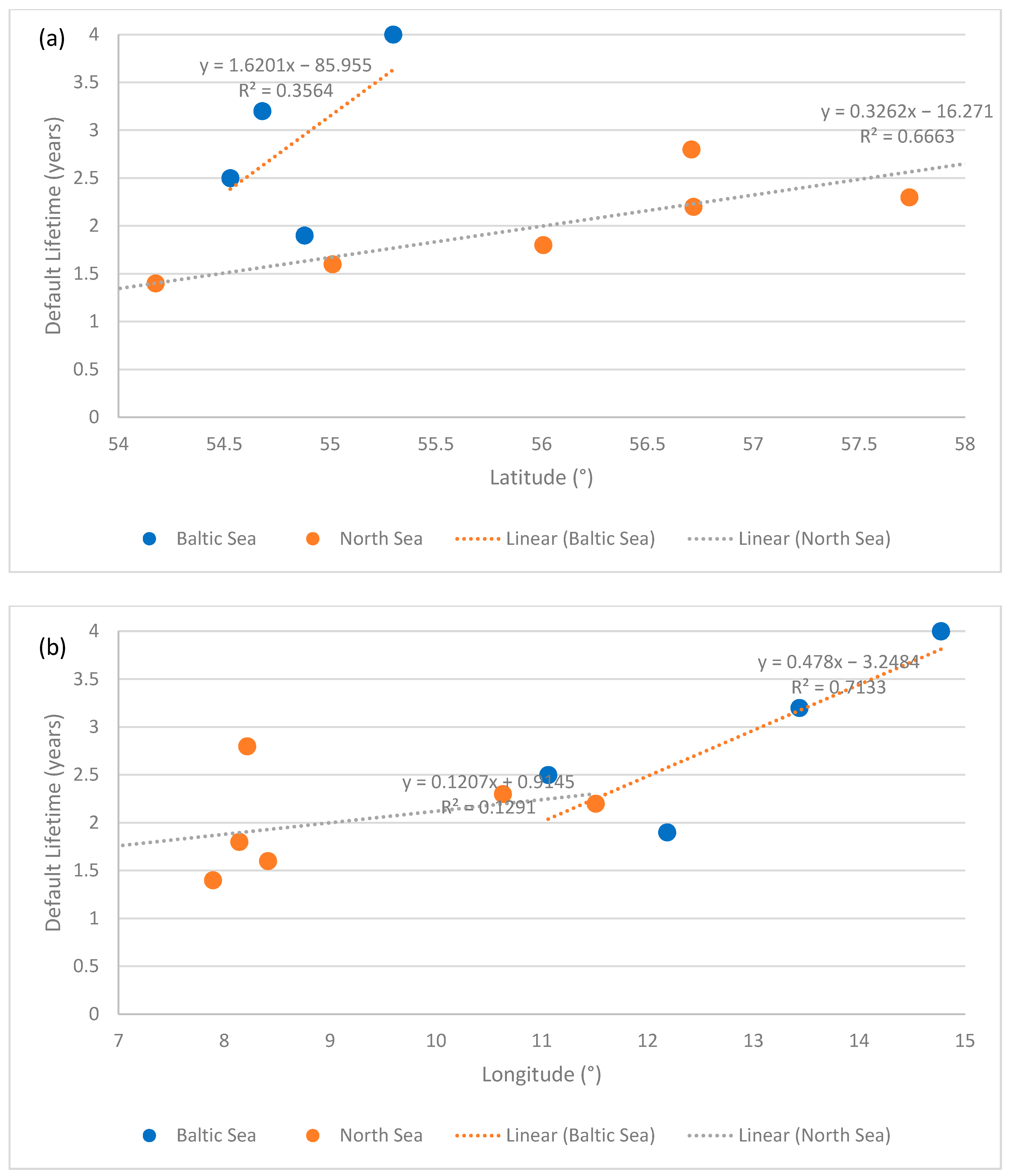
- The lifetime increases from the South to the North along the German and Danish North Sea coastline: Helgoland (1.4 years), List (1.6 years), Hvide Sande (1.8 years), and Thyborøn (2.8 years). The time series are from 18 to 23 years long.
- The lifetime increases from the West to the East in the Baltic Sea: Fehmarn (2.5 years) and Vindebæk (1.9 years) in the western part while Arkona (3.2 years) and Hammerodde (4.0 years) are located further East. The time series are from 13 to 28 years long.
- Weybourne’s lifetime (27.9 years) calculated from a time series of 2.6 years duration may be over-predicted. Weybourne is located in a region with low annual rainfall, thus a longer lifetime than in the eastern part of the North Sea appears likely.
- Utsira’s lifetime (13.3 years) calculated from a time series of 3.3 years may also be over-predicted. In contrast to Weybourne, Utsira is located in a region with very high annual rainfall. Thus further analysis is needed to conclude on the lifetime, if lifetime is truly much longer than in the Danish North Sea.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Precipitation Type | Symbol | Rimco, Pluvio2 | Geonor, Shielded |
|---|---|---|---|
| Snow | β0 | 0.04587 | −0.12159 |
| β1 | 0.23677 | 0.18546 | |
| β2 | 0.017979 | 0.006918 | |
| β3 | −0.015407 | −0.005254 | |
| Rain | γ0 | 0.007697 | 0.007697 |
| γ1 | 0.034331 | 0.034331 | |
| γ2 | −0.00101 | −0.00101 | |
| γ3 | −0.012177 | −0.012177 | |
| c | 0.0 | −0.05 |
References
- Slot, H.M.; Gelinck, E.R.M.; Rentrop, C.; van der Heide, E. Leading edge erosion of coated wind turbine blades: Review of coating life models. Renew. Energy 2015, 80, 837–848. [Google Scholar] [CrossRef]
- Eisenberg, D.; Laustsen, S.; Stege, J. Wind turbine blade coating leading edge rain erosion model: Development and validation. Wind Energy 2018, 21, 1–10. [Google Scholar] [CrossRef]
- Punge, H.J.; Kunz, M. Hail observations and hailstorm characteristics in Europe: A review. Atmos. Res. 2016, 176–177, 159–184. [Google Scholar] [CrossRef]
- Keegan, M.H.; Nash, D.; Stack, M. On erosion issues associated with the leading edge of wind turbine blades. J. Phys. D Appl. Phys. 2013, 46, 383001. [Google Scholar] [CrossRef]
- Letson, F.; Barthelmie, R.J.; Pryor, S.C. Radar-derived precipitation climatology for wind turbine blade leading edge erosion. Wind Energy Sci. 2020, 5, 331–347. [Google Scholar] [CrossRef]
- Macdonald, H.; Infield, D.; Nash, D.H.; Stack, M.M. Mapping hail meteorological observations for prediction of erosion in wind turbines. Wind Energy 2016, 19, 777–784. [Google Scholar] [CrossRef]
- Papi, F.; Balduzzi, F.; Ferrara, G.; Bianchini, A. Uncertainty quantification on the effects of rain-induced erosion on annual energy production and performance of a Multi-MW wind turbine. Renew. Energy 2021, 165. [Google Scholar] [CrossRef]
- Prieto, R.; Karlsson, T. A model to estimate the effect of variables causing erosion in wind turbine blades. Wind Energy 2021, 22, 1–14. [Google Scholar] [CrossRef]
- Bartolomé, L.; Teuwen, J. Methodology for the energetic characterisation of rain erosion on wind turbine blades using meteorological data: A case study for The Netherlands. Wind Energy 2021, 1–13. [Google Scholar] [CrossRef]
- Dashtkar, A.; Hadavinia, H.; Sahinkaya, M.N.; Williams, N.A.; Vahid, S.; Ismail, F.; Turner, M.A.; Vahid, S.; Ismail, F.; Turner, M. Rain erosion-resistant coatings for wind turbine blades: A review. Polym. Polym. Compos. 2019, 27, 443–475. [Google Scholar]
- Herring, R.; Dyer, K.; Martin, F.; Ward, C. The increasing importance of leading edge erosion and a review of existing protection solutions. Renew. Sustain. Energy Rev. 2019, 115, 109382. [Google Scholar] [CrossRef]
- Mishnaevsky, L., Jr. Repair of wind turbine blades: Review of methods and related computational mechanics problems. Renew. Energy 2019, 140, 828–839. [Google Scholar] [CrossRef]
- IEA International Energy Agency Offshore. Wind Outlook 2019, World Energy Outlook Special Report, Technology report—November. Available online: https://www.iea.org/reports/offshore-wind-outlook-2019 (accessed on 31 March 2021).
- Wind Europe. Our Energy, Our Future, How Offshore Wind Will Help Europe Go Carbon-Neutral. 2019. Available online: https://windeurope.org/about-wind/reports/our-energy-our-future/ (accessed on 31 March 2021).
- Bech, J.I.; Hasager, C.B.; Bak, C. Extending the life of wind turbine blade leading edges by reducing the tip speed during extreme precipitation events. Wind Energ. Sci. 2018, 3, 729–748. [Google Scholar] [CrossRef]
- Hasager, C.B.; Vejen, F.; Bech, J.I.; Skrzypiński, W.R.; Tilg, A.-M.; Nielsen, M. Assessment of the rain and wind climate with focus on wind turbine blade leading edge erosion rate and expected lifetime in Danish Seas. Renew. Energy 2020, 149, 91–102. [Google Scholar] [CrossRef]
- Skrzypiński, W.R.; Bech, J.I.; Hasager, C.B.; Tilg, A.-M.; Bak, C. Optimization of the erosion-safe operation of the IEA Wind 15 MW Reference Wind Turbine. J. Phys: Conf. Ser. 2020, 1618, 052034. [Google Scholar] [CrossRef]
- Bak, C.; Forsting, A.M.; Sørensen, N.N. The influence of leading edge roughness, rotor control and wind climate on the loss in energy production. J. Phys. Conf. Ser. 2020, 1618. [Google Scholar] [CrossRef]
- Gaertner, E.; Rinker, J.; Sethuraman, L.; Zahle, F.; Anderson, B.; Barter, G.; Abbas, N.; Meng, F.; Bortolotti, P.; Skrzypiński, W.R.; et al. Definition of the IEA 15-Megawatt Offshore Reference Wind Turbine (NREL/TP-5000-75698); National Renewable Energy Laboratory: Golden, CO, USA. Available online: https://www.nrel.gov/docs/fy20osti/75698.pdf (accessed on 31 March 2021).
- Nešpor, V.; Sevruk, B. Estimation of Wind-Induced Error of Rainfall Gauge Measurement Using a Numerical Simulation. J. Atmos. Ocean. Technol. 1999, 16, 450–464. [Google Scholar] [CrossRef]
- Natural Environment Research Council; Met Office; Pickering, B.S.; Neely, R., III; Harrison, D. The Disdrometer Verification Network (DiVeN) Particle Diameter and Fall Velocity Measurements from a Network of Thies Laser Precipitation Monitors Around the UK (2017–2019); Centre for Environmental Data Analysis: Chilton, UK, 2019. [Google Scholar] [CrossRef]
- Quante, M.; Colijn, F.; Bakker, J.P.; Härdtle, W.; Heinrich, H.; Lefebvre, C.; Nöhren, I.; Olesen, J.E.; Pohlmann, T.; Sterr, H.; et al. Introduction to the Assessment—Characteristics of the Region. In North Sea Region Climate Change Assessment, Regional Climate Studies; Quante, M., Colijn, F., Eds.; Springer: Cham, Switzerland, 2016. [Google Scholar] [CrossRef]
- Tilg, A.-M.; Skrzypiński, W.R.; Hasager, C.B. Effect of drop-size parametrization and rain amount on blade-lifetime calculations considering leadindg-edge erosion. Wind Energy 2021. in review. [Google Scholar]
- Bak, C.; Skrzypiński, W.; Fischer, A.; Gaunaa, M.; Brønnum, N.F.; Kruse, E.K. Wind tunnel tests of an airfoil with 18% relative thickness equipped with vortex generators. J. Phys. Conf. Ser. 2018, 1037. [Google Scholar] [CrossRef]
- Best, A.C. The size of distribution of raindrops. Quart. J. R. Met. Soc. 1950, 76, 16. [Google Scholar] [CrossRef]
- Brualdi, R.A. Introductory Combinatorics, 5th ed.; Pearson Prentice Hall: Upper Saddle River, NJ, USA, 2010; ISBN 978-0-13-602040-0. [Google Scholar]
- Sevruk, B.; Hamon, W.R. International Comparison of National Precipitation Gauges with a Reference Pit Gauge; World Meteorological Organization: Geneva, Switzerland, 1984. [Google Scholar]
- Goodison, B.E.; Louie, P.Y.T.; Yang, D. WMO Solid Precipitation Measurement Intercomparison: Final Report. Instruments and Observing Methods, Report No. 67 (WMO/TD No. 872); World Meteorological Organization: Geneva, Switzerland, 1998. [Google Scholar]
- Allerup, P.; Madsen, H. Accuracy of point precipitation measurements. Nord. Hydrol. 1980, 11, 57–70. [Google Scholar] [CrossRef]
- Allerup, P.; Madsen, H.; Vejen, F. A Comprehensive Model for Correcting Point Precipitation. Nord. Hydrol. 1997, 28, 1–20. [Google Scholar] [CrossRef]
- Sevruk, B.; Klemm, S. Types of standard precipitation gauges. In Proceedings of the WMO, IAHS, ETH International Workshop on Precipitation Measurement, St. Moritz, Switzerland, 3–7 December 1989; pp. 227–232. [Google Scholar]
- Allerup, P.; Dahlström, B.; Elomaa, E.; Jónsson, T.; Madsen, H.; Perälä, J.; Rissanen, P.; Vedin, H.; Vejen, F. Manual for Operational Correction of Nordic Precipitation Data (Nordic Working Group on Precipitation, Report Nr. 24/96), 1st ed.; Førland, E.J., Ed.; DNMI: Copenhagen, Denmark, 1996; pp. 1–66. [Google Scholar]
- Rasmussen, R.; Baker, B.; Kochendorfer, J.; Meyers, T.; Landolt, S.; Fischer, A.P.; Black, J.; Thériault, J.M.; Kucera, P.; Gochis, D.; et al. How well are we measuring snow? The NOAA/FAA/NCAR Winter Precipitation Test Bed. BAMS 2012, 811–829. [Google Scholar] [CrossRef]
- Niemczynowicz, J. Dynamic calibration of tipping-bucket rain gauges. Nord. Hydrol. 1986, 17, 203–214. [Google Scholar] [CrossRef]
- Vejen, F.; Vilic, K.; Jensen, H.; Kern-Hansen, C. Korrigeret Nedbør 1989–2010, 2011–2012 & 2013—Konsulentopgave Udført for Dce—Nationalt Center for Miljø Og Energi, Aarhus Universitet, DMI Technical Report, Tr14-13; Danish Meteorological Institute: Copenhagen, Denmark, 2014; pp. 1–228. [Google Scholar]
- Sevruk, B. Wind speed estimation at precipitation gauge orifice level. In Instruments and Observing Methods, Report No. 33, Proceedings of the WMO Technical Conference on Instruments and Methods of Observation (TECO-1988), Leipzig, Germany, 16–20 May 1988; World Meteorological Organization (WMO): Geneva, Switzerland, 1988; p. 4. [Google Scholar]
- World Meteorological Organization. WMO Guide to Meteorological Instruments and Methods of Observation, WMO-No. 8, 7th ed.; Secretariat of the World Meteorological Organization: Geneva, Switzerland, 2008. [Google Scholar]
- Frich, P.; Rosenørn, S.; Madsen, H.; Jensen, J.J. Observed Precipitation in Denmark, 1961–90; Technical Report 97-8; Danish Meteorological Institute, Ministry of Transport: Copenhagen, Denmark, 1997; Available online: https://www.dmi.dk/fileadmin/user_upload/Rapporter/TR/1997/tr97-8.pdf (accessed on 31 March 2021).
- Tilg, A.-M.; Hagen, M.; Vejen, F.; Hasager, C.B. Variation of leading-edge-erosion relevant precipitation parameters with location and weather type. Meteorol. Z. 2021, accepted. [Google Scholar]
- Tait, A.B.; Barrett, E.C.; Beaumont, M.J.; Brown, P.A.; Taberner, M.J.; Todd, M.C. Interpretation of an atlas of passive microwave-derived rainfall over the eastern North Atlantic Ocean and North Sea. Int. J. Climatol. 1999, 19, 231–252. [Google Scholar] [CrossRef]
- Panagos, P.; Ballabio, C.; Borrelli, P.; Meusburger, K.; Klik, A.; Rousseva, S.; Tadic, M.P.; Michaelides, S.; Hrabalikova, M.; Olsen, P.; et al. Rainfall erosivity in Europe. Sci. Total Environ. 2015, 511, 801–814. [Google Scholar] [CrossRef]
- World Meteorological Organization. WMO Field Intercomparison of Rainfall Intensity Gauges. In Instruments and Observing Methods, Report No. 99; WMO/TD-No. 1504; World Meteorological Organization: Geneva, Switzerland, 2009. [Google Scholar]
- Sareen, A.; Sapre, C.A.; Selig, M.S. Effects of leading edge erosion on wind turbine blade performance: Effects of leading edge erosion. Wind Energy 2014, 17, 1531–1542. [Google Scholar] [CrossRef]
- Han, W.; Kim, J.; Kim, B. Effects of contamination and erosion at the leading edge of blade tip airfoils on the annual energy production of wind turbines. Renew. Energy 2018, 115. [Google Scholar] [CrossRef]
- Law, H.; Koutsos, V. Leading edge erosion of wind turbines: Effect of solid airborne particles and rain on operational wind farms. Wind Energy 2020, 23, 10–1955. [Google Scholar] [CrossRef]
- Punge, H.; Bedka, K.; Kunz, M.; Werner, A. A new physically based stochastic event catalog for hail in Europe. Nat. Hazards 2014, 1625–1645. [Google Scholar] [CrossRef]
- Tilg, A.-M.; Hasager, C.B.; Kirtzel, H.-J.; Hummelshøj, P. Brief communication: Nowcasting of precipitation for leading-edge-erosion-safe mode. Wind Energ. Sci. 2020, 5, 977–981. [Google Scholar] [CrossRef]
- Mishnaevsky, L., Jr.; Hasager, C.B.; Bak, C.; Tilg, A.-M.; Bech, J.I.; Rad, S.D.; Fæster, S. Leading edge erosion of wind turbine blades: Understanding, prevention and protection. Renew. Energy 2021, 169, 953–969. [Google Scholar] [CrossRef]
- Letson, F.; Shepherd, T.; Barthelmie, R.; Pryor, S.C. WRF Modeling of Deep Convection and Hail for Wind Power Applications. J. Appl. Meteorol. Climatol. 2020, 59, 1717–1733. [Google Scholar] [CrossRef]
- Rios Gaona, M.F.; Overeem, A.; Leijnse, H.; Uijlenhoet, R. First-Year Evaluation of GPM Rainfall over the Netherlands: IMERG Day 1 Final Run (V03D). J. Hydrometeorol. 2016, 7, 2799–2814. [Google Scholar] [CrossRef]

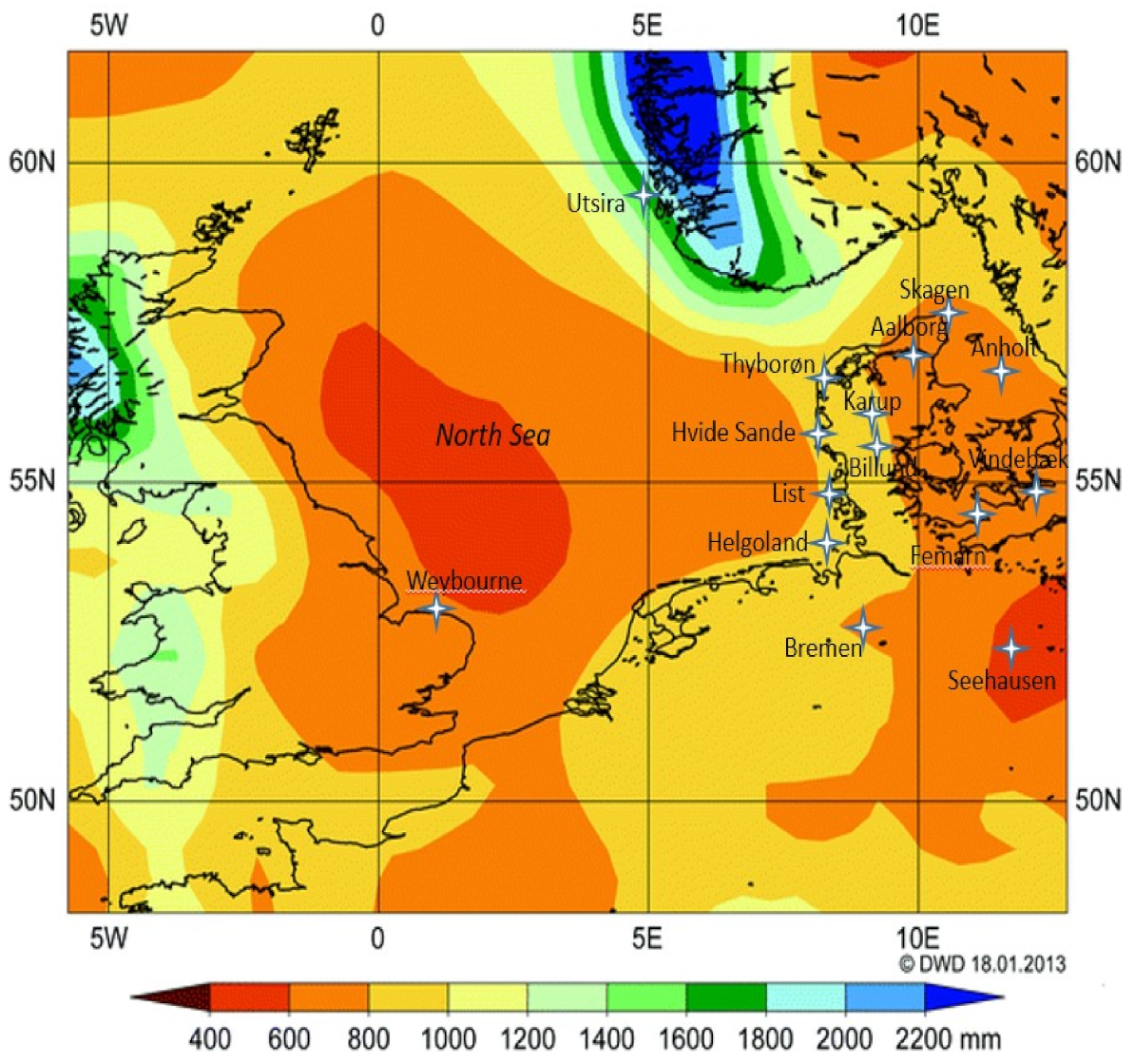
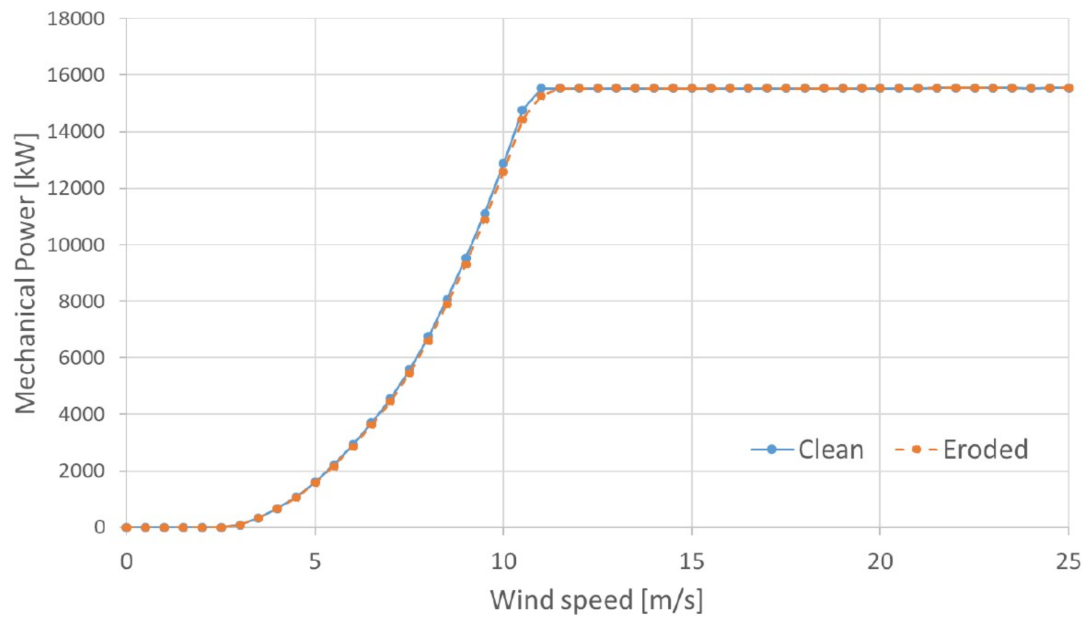
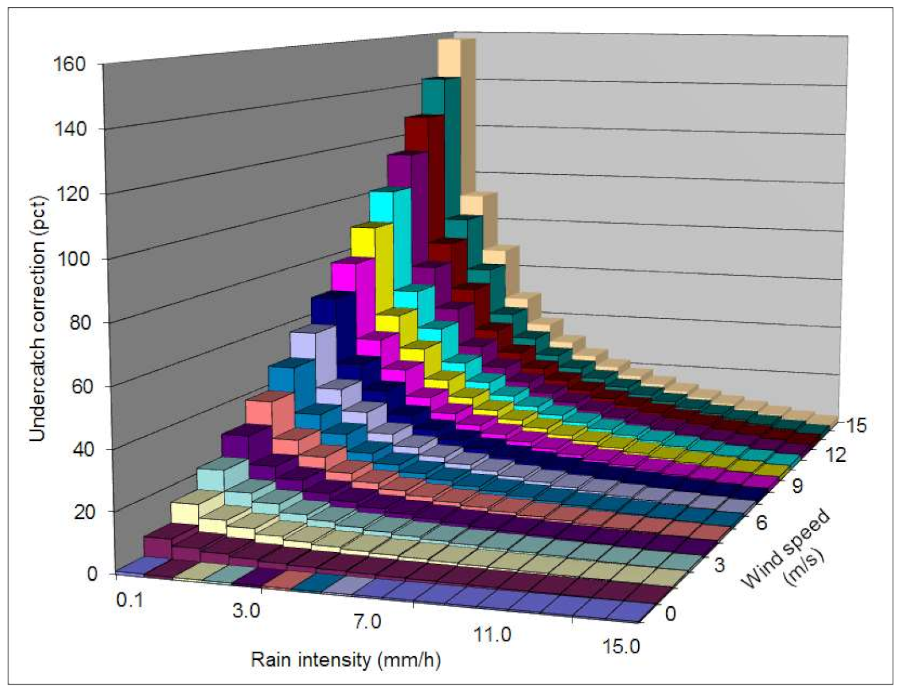
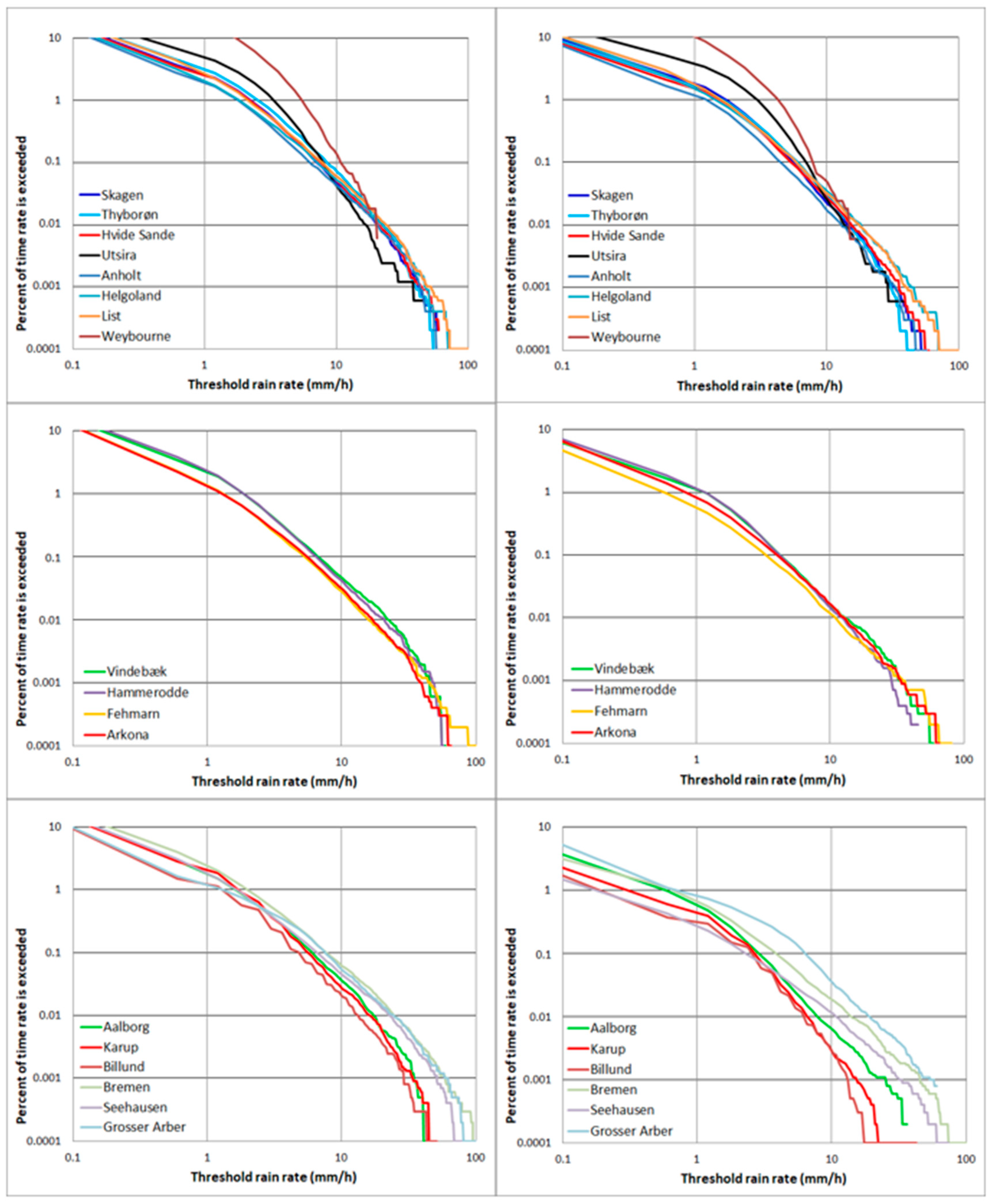

| Location | Country | Data Provider | WMO Station | Rain Sensor Type | Dates (Start, End) | Number of Years |
|---|---|---|---|---|---|---|
| Aalborg | DK | DMI | 603000 | Theiss, Pluvio | 28/02/2003, 31/12/2019 | 16.9 |
| Anholt | DK | DMI | 607900 | Geonor | 01/01/2002, 31/12/2019 | 17.9 |
| Arkona | DE | DWD | 1009100 | NG 200, Pluvio, rain[e]H3 | 01/11/1991, 31/12/2019 | 28.2 |
| Billund | DK | DMI | 610400 | Rimco, Pluvio | 22/08/2003, 31/12/2019 | 16.4 |
| Bremen | DE | DWD | 1022400 | Tipping bucket, Pluvio | 29/02/1991, 31/12/2019 | 28.2 |
| Fehmarn | DE | DWD | 1005500 | Tipping bucket, Pluvio, rain[e]H3 | 01/06/1996, 31/12/2019 | 23.6 |
| Grosser Arber | DE | DWD | 1079100 | Pluvio, rain[e]H3 | 26/02/1998, 31/12/2019 | 21.9 |
| Hammerodde | DK | DMI | 619300 | Geonor, Pluvio | 30/08/2001, 31/12/2019 | 18.3 |
| Helgoland | DE | DWD | 1001500 | Tipping bucket, Pluvio | 20/12/1996, 31/12/2019 | 23.0 |
| Hvide Sande | DK | DMI | 605800 | Geonor | 01/01/2002, 31/12/2019 | 18.0 |
| Karup | DK | DMI | 606000 | Rimco, Pluvio | 13/02/2003, 31/12/2019 | 16.9 |
| List | DE | DWD | 1002000 | Tipping bucket, Pluvio, rain[e]H3 | 02/12/1995, 31/12/2019 | 24.1 |
| Seehausen | DE | DWD | 1026100 | NG 200, Pluvio, rain[e]H3 | 01/11/1991, 31/12/2019 | 28.2 |
| Skagen | DK | DMI | 604100 | Geonor | 01/01/2002, 31/12/2019 | 18.0 |
| Thyborøn | DK | DMI | 605200 | Geonor, Pluvio | 01/01/2002, 31/12/2019 | 18.0 |
| Utsira | NO | NMI | 140300 | Geonor | 15/13/1916, 28/06/2019 | 3.3 |
| Vindebæk | DK | DMI | 614700 | Geonor | 29/05/2006, 31/12/2019 | 13.6 |
| Weybourne | UK | CEDA | - | Thies LPM | 22/02/1917, 30/09/2019 | 2.6 |
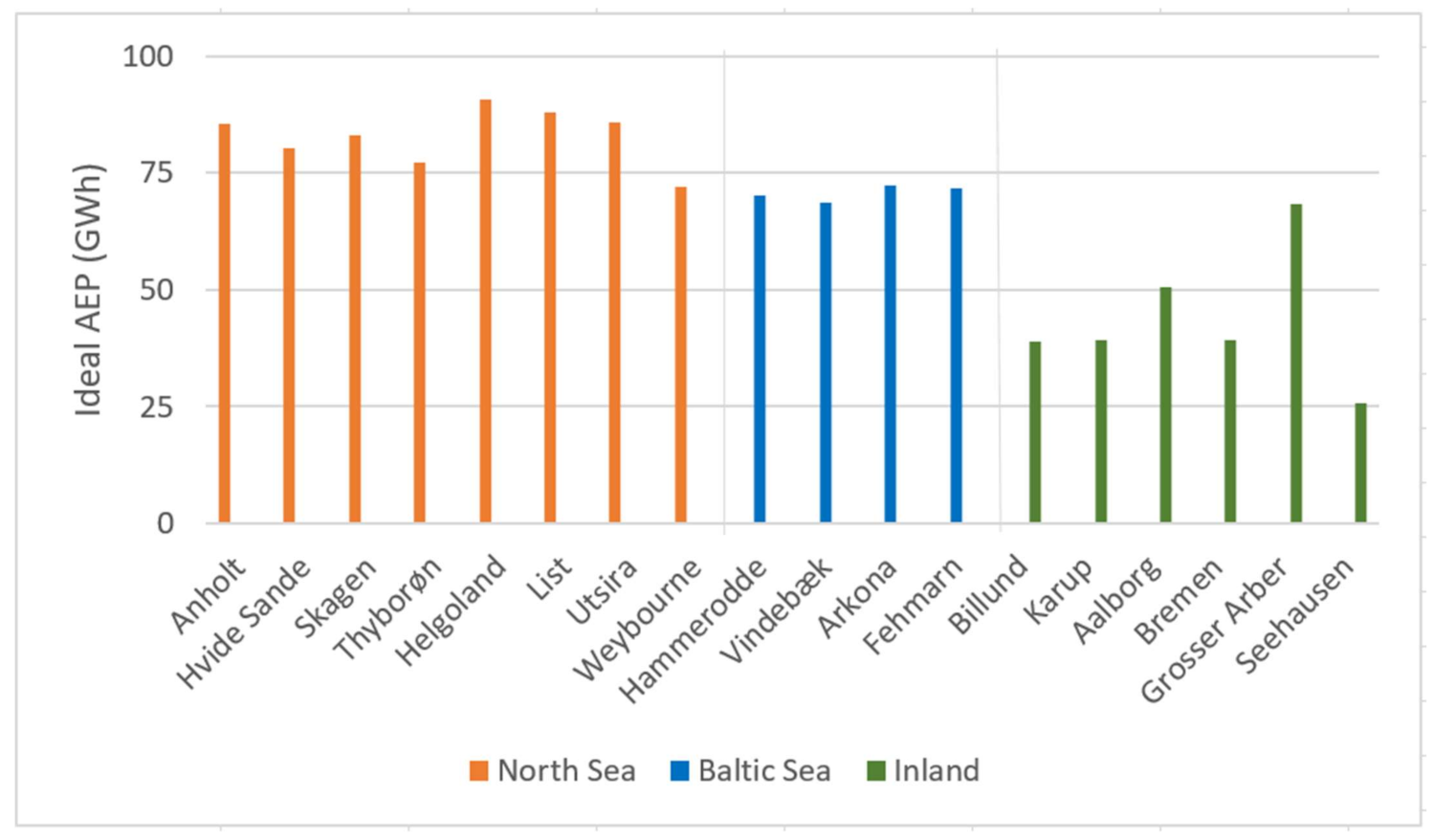
| Area | Station | Lifetime | AEP Ideal (GWh) | AEP Loss (%) | ||
|---|---|---|---|---|---|---|
| Ideal (years) | Default (years) | ESM (years) | ||||
| North Sea | Anholt | ∞ | 2.2 | 13.1 | 85.4 | 0.35 |
| Helgoland | ∞ | 1.4 | 9.7 | 90.7 | 0.26 | |
| Hvide Sande | ∞ | 1.8 | 11.8 | 80.3 | 0.46 | |
| List | ∞ | 1.6 | 10.2 | 87.9 | 0.36 | |
| Skagen | ∞ | 2.3 | 13.0 | 83.1 | 0.43 | |
| Thyborøn | ∞ | 2.8 | 13.6 | 77.2 | 0.53 | |
| Utsira | ∞ | 13.3 | 62.5 | 85.9 | 0.04 | |
| Weybourne | ∞ | 27.9 | 45.1 | 72.0 | 0.07 | |
| Average North Sea | ∞ | 6.7 | 22.4 | 82.8 | 0.31 | |
| Average North Sea DE and DK only | ∞ | 2.0 | 11.9 | 84.1 | 0.40 | |
| Baltic Sea | Arkona | ∞ | 3.2 | 18.3 | 72.4 | 0.51 |
| Fehmarn | ∞ | 2.5 | 12.5 | 71.6 | 0.58 | |
| Hammerodde | ∞ | 4.0 | 20.8 | 70.2 | 0.32 | |
| Vindebæk | ∞ | 1.9 | 23.8 | 68.5 | 0.61 | |
| Average Baltic Sea | ∞ | 2.9 | 18.9 | 70.7 | 0.50 | |
| Inland Stations | Billund | ∞ | 2.8 | 14.8 | 38.9 | 0.52 |
| Bremen | ∞ | 1.5 | 9.0 | 39.2 | 0.74 | |
| Grosser Arber | ∞ | 0.9 | 5.3 | 68.4 | 0.42 | |
| Karup | ∞ | 10.7 | 25.4 | 39.4 | 0.66 | |
| Seehausen | ∞ | 3.3 | 14.5 | 25.8 | 0.73 | |
| Aalborg | ∞ | 8.5 | 33.3 | 50.7 | 0.75 | |
| Average Inland stations | ∞ | 4.6 | 17.1 | 43.7 | 0.63 | |
| All | Average | ∞ | 5.16 | 19.82 | 67.1 | 0.46 |
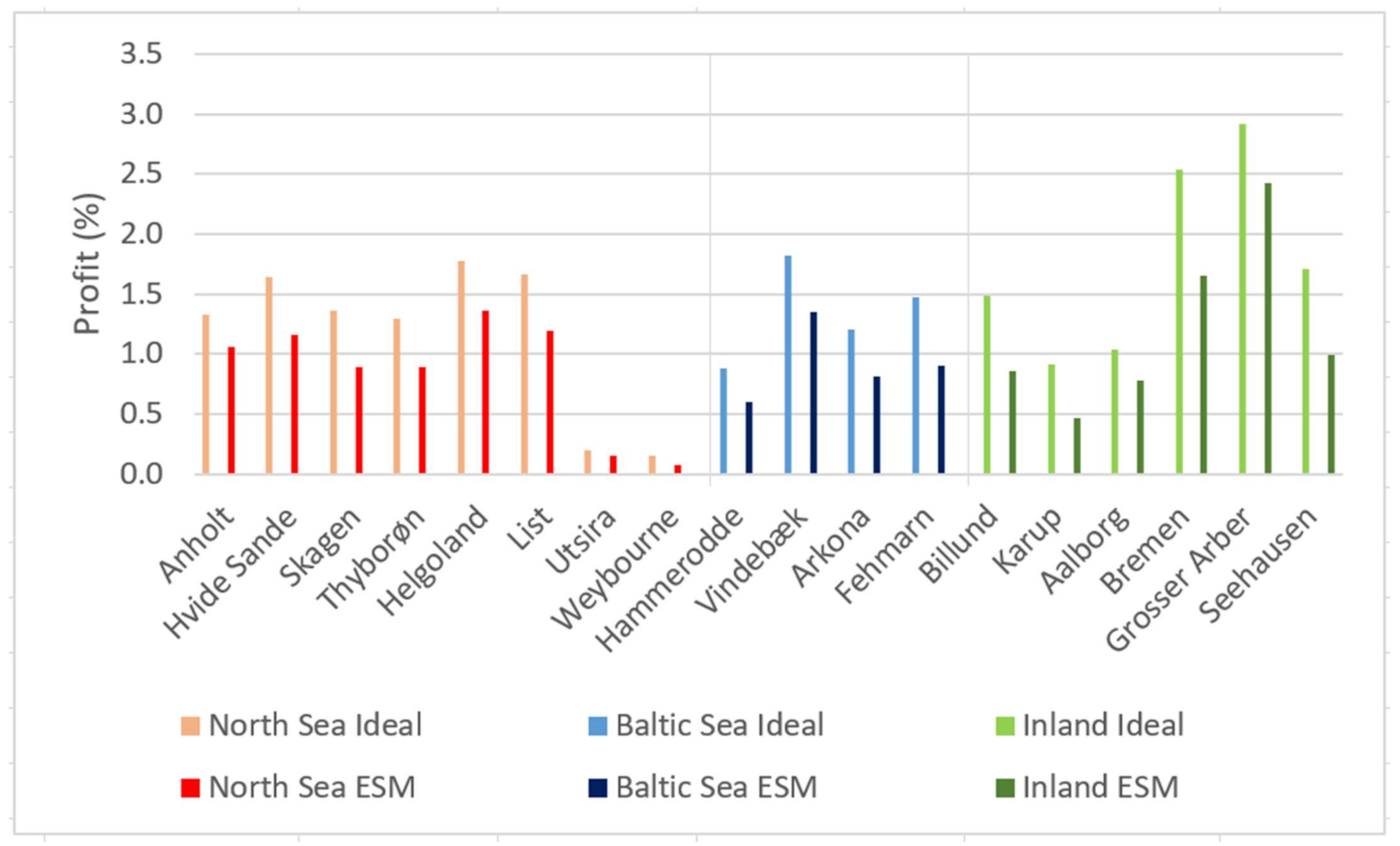
| Area | Station | Ideal vs. Default Profit (%) | Profit ESM vs. Default (%) | Profit Site-Specific Optimized ESM vs. Default (%) |
|---|---|---|---|---|
| North Sea | Anholt | 1.33 | 0.87 | 1.06 |
| Helgoland | 1.78 | 1.24 | 1.36 | |
| Hvide Sande | 1.64 | 0.88 | 1.16 | |
| List | 1.66 | 1.04 | 1.20 | |
| Skagen | 1.36 | 0.76 | 0.89 | |
| Thyborøn | 1.30 | 0.61 | 0.89 | |
| Utsira | 0.19 | 0.14 | 0.16 | |
| Weybourne | 0.15 | 0.06 | 0.08 | |
| Average North Sea | 1.18 | 0.70 | 0.85 | |
| Average North Sea DE and DK only | 1.51 | 0.90 | 1.09 | |
| Baltic Sea | Arkona | 1.21 | 0.57 | 0.82 |
| Fehmarn | 1.47 | 0.63 | 0.90 | |
| Hammerodde | 0.88 | 0.37 | 0.60 | |
| Vindebæk | 1.83 | 1.31 | 1.35 | |
| Average Baltic Sea | 1.35 | 0.72 | 0.92 | |
| Inland stations | Billund | 1.48 | 0.51 | 0.86 |
| Bremen | 2.54 | 1.43 | 1.66 | |
| Grosser Arber | 2.92 | 2.06 | 2.43 | |
| Karup | 0.91 | 0.17 | 0.46 | |
| Seehausen | 1.72 | 0.66 | 0.99 | |
| Aalborg | 1.04 | 0.58 | 0.79 | |
| Average Inland stations | 1.77 | 0.90 | 1.20 | |
| All | Average | 1.41 | 0.77 | 0.98 |
| Jan. | Feb. | Mar. | Apr. | May | Jun. | Jul. | Aug. | Sep. | Oct. | Nov. | Dec. | Year | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Corrected (mm) | Geonor | 39.3 | 26.7 | 25.3 | 22.9 | 27.7 | 46.0 | 49.5 | 77.1 | 72.0 | 68.5 | 61.3 | 54.4 | 570.6 |
| Pluvio2 | 41.3 | 28.0 | 26.6 | 24.0 | 28.9 | 47.8 | 51.3 | 79.7 | 74.9 | 71.5 | 64.1 | 57.1 | 595.1 | |
| Rimco | 41.9 | 28.5 | 27.2 | 25.0 | 30.2 | 49.3 | 53.0 | 81.6 | 76.4 | 72.7 | 65.1 | 57.8 | 608.6 | |
| Under-Catch (%) | Geonor | 8.0 | 7.7 | 6.3 | 4.8 | 3.9 | 3.3 | 2.2 | 1.8 | 3.0 | 4.3 | 4.9 | 6.3 | 4.3 |
| Pluvio2 | 13.5 | 13.0 | 11.5 | 9.6 | 8.6 | 7.4 | 6.1 | 5.4 | 7.1 | 8.9 | 9.8 | 11.4 | 8.8 | |
| Rimco | 15.2 | 15.3 | 14.2 | 14.0 | 13.5 | 10.7 | 9.5 | 7.8 | 9.3 | 10.8 | 11.4 | 12.9 | 11.2 | |
| Average (m –s) Wind Speed | 9.8 | 9.3 | 8.3 | 7.6 | 7.3 | 7.6 | 6.7 | 6.9 | 8.1 | 8.3 | 8.2 | 9.0 | 8.2 | |
| Average (mm h−1) Rain Rate | 1.37 | 1.30 | 1.33 | 1.49 | 1.63 | 2.30 | 2.49 | 3.04 | 2.59 | 2.00 | 1.70 | 1.50 | 1.91 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hasager, C.B.; Vejen, F.; Skrzypiński, W.R.; Tilg, A.-M. Rain Erosion Load and Its Effect on Leading-Edge Lifetime and Potential of Erosion-Safe Mode at Wind Turbines in the North Sea and Baltic Sea. Energies 2021, 14, 1959. https://doi.org/10.3390/en14071959
Hasager CB, Vejen F, Skrzypiński WR, Tilg A-M. Rain Erosion Load and Its Effect on Leading-Edge Lifetime and Potential of Erosion-Safe Mode at Wind Turbines in the North Sea and Baltic Sea. Energies. 2021; 14(7):1959. https://doi.org/10.3390/en14071959
Chicago/Turabian StyleHasager, Charlotte Bay, Flemming Vejen, Witold Robert Skrzypiński, and Anna-Maria Tilg. 2021. "Rain Erosion Load and Its Effect on Leading-Edge Lifetime and Potential of Erosion-Safe Mode at Wind Turbines in the North Sea and Baltic Sea" Energies 14, no. 7: 1959. https://doi.org/10.3390/en14071959
APA StyleHasager, C. B., Vejen, F., Skrzypiński, W. R., & Tilg, A.-M. (2021). Rain Erosion Load and Its Effect on Leading-Edge Lifetime and Potential of Erosion-Safe Mode at Wind Turbines in the North Sea and Baltic Sea. Energies, 14(7), 1959. https://doi.org/10.3390/en14071959







