System Parameter Based Performance Optimization of Solar PV Systems with Perturbation Based MPPT Algorithms
Abstract
:1. Introduction
- To study the effect of input capacitance on the system time constant and determine system time constant using a simple step test (Step test 01).
- To study the digital filter’s impact on the system time constant as seen by the controller (Step test 02).
- To address the choice of the sampling frequency of PV voltage and current based on the estimated system time constant for optimal energy extraction using perturbation techniques.
2. Solar PV Fed Boost Converter
2.1. Input Capacitance and Its Effect on the System Time Constant
2.2. Digital Filter Design and Its Effect on System Time Constant
2.3. Effect of Sampling time on System Performance
2.4. Mathematical Model
3. Proposed Tests and Guidelines for System Parameter Based Performance Optimization
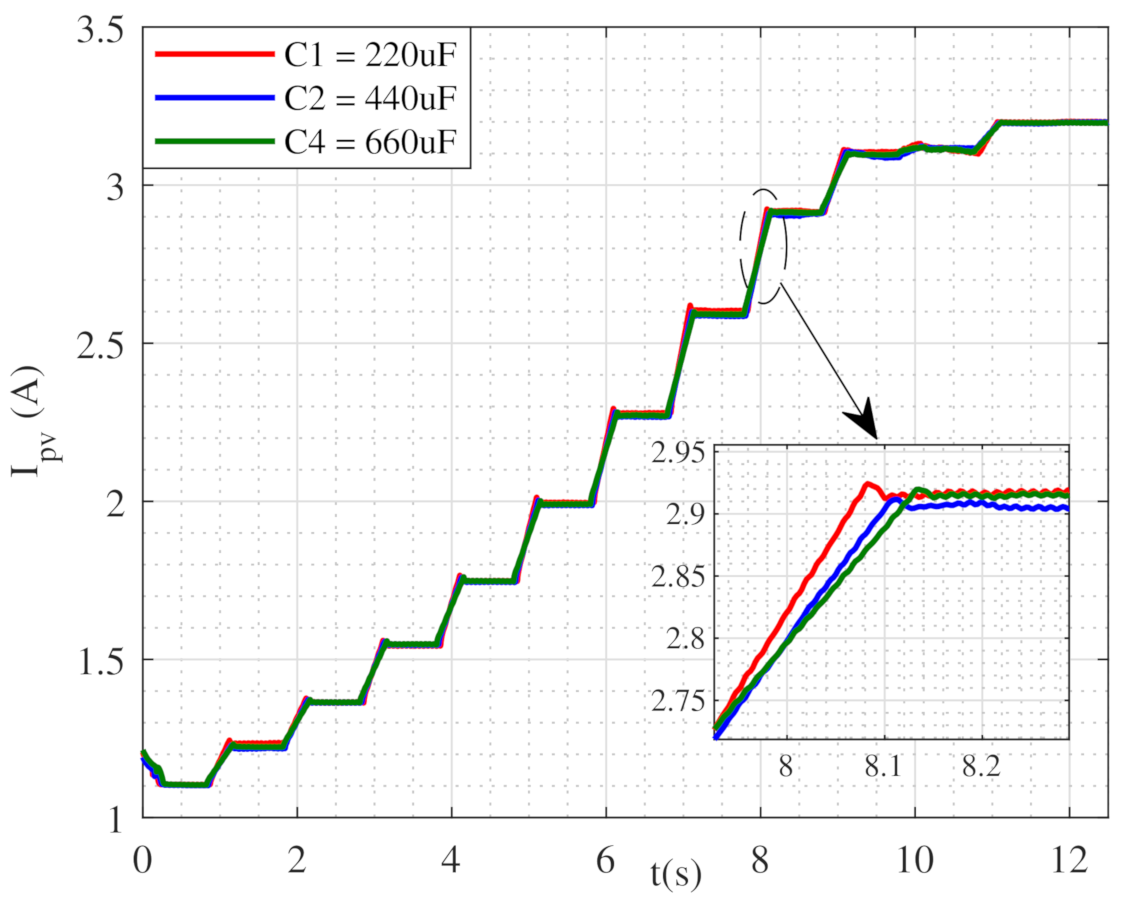
3.1. Step Test 01—Determination of Optimum Value of Input Capacitance
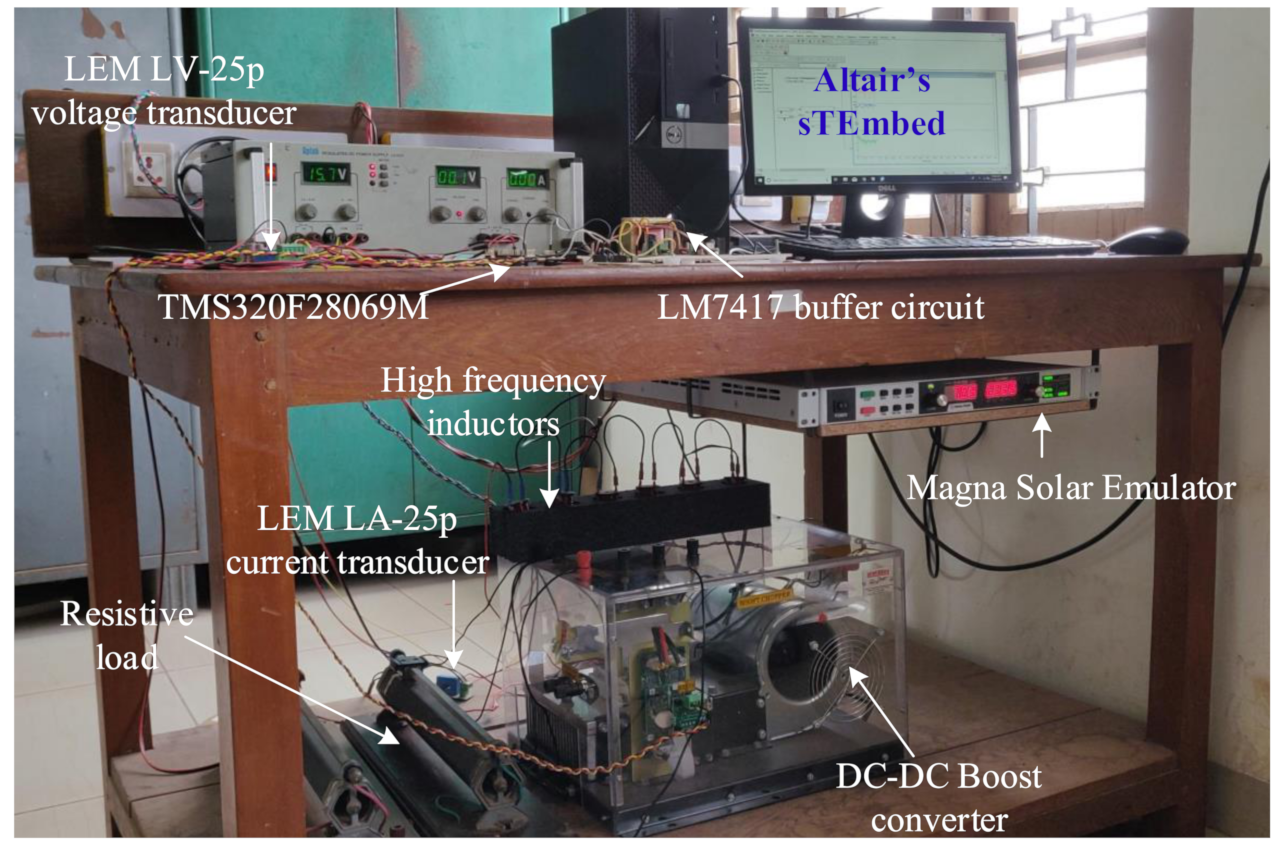
- Configure an experimental setup consisting of Solar PV array, and the power converter under test (setup is shown in Figure 1 for this study)
- Choose appropriate value of and vary the duty ratio from minimum to maximum value and capture the responses of . (In this study, is chosen and D is varied from 0.1 to 0.7 in steps of 0.05)
- Repeat step 2 for different values of , to see the effect of input capacitance on . (In this study, responses are investigated for , 440 ).
- The value of depends on the switching frequency of the power converter, ripple in PV voltage and PV current and is calculated using (11).
- The value of is directly proportional to the settling time of responses of PV voltage and current.
- The of the system is 0.2 s for .
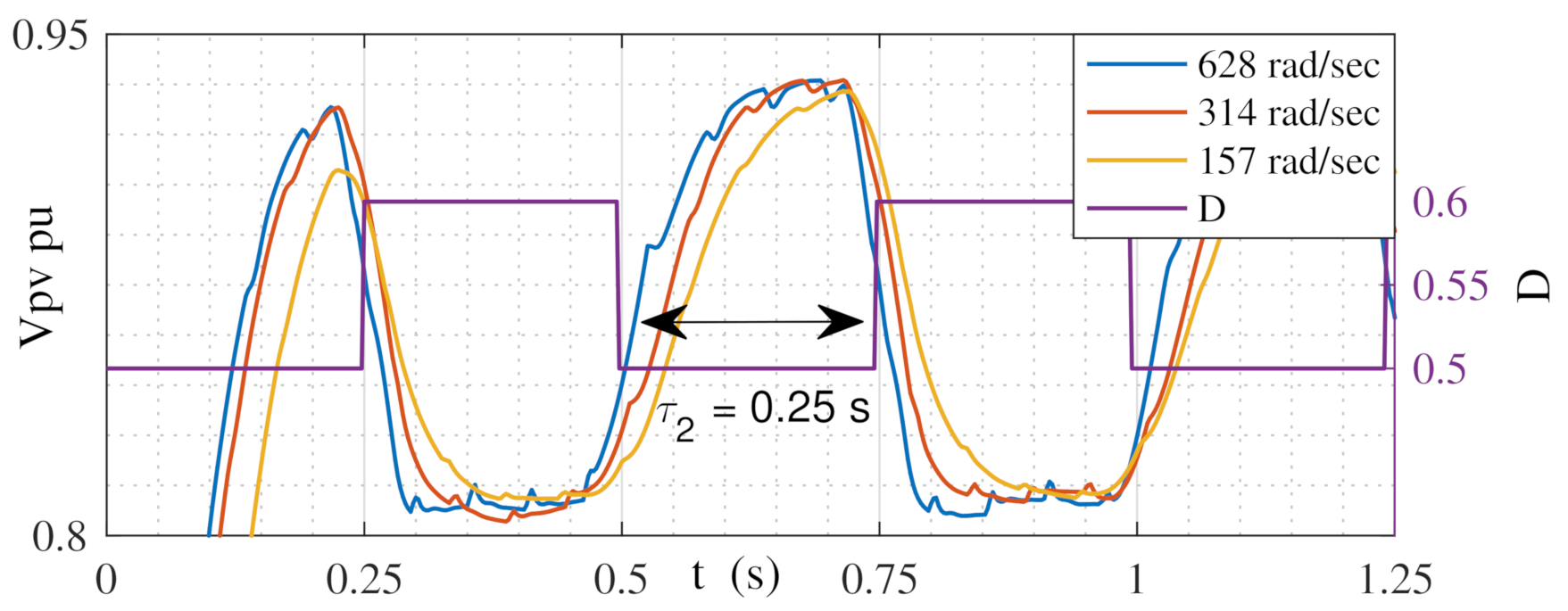
3.2. Step Test 02—Determination of Optimum Value of Digital Filter Cut-Off Frequency
- Configure experiment set-up with chosen value of in step test-01. ( )
- Configure digital filter to eliminate/reduce the noise due to ADC. (First order filter with cut-off frequency of 157 rad/s, 314 rad/sec and 628 rad/s are used in this study, first cut-off frequency (100 Hz = 628 rad/s) is empirically chosen as 0.01 times switching frequency (10 kHz))
- Introduce a step change in D (from 0.5 to 0.6) of S and record the response of digital filter output () for different cut-off frequencies.(During experimentation, the response of was observed to be slightly slower compared to , hence was chosen as criteria over )
3.3. Proposed Guidelines for the Choice of Sampling Time
- For a given switching frequency , obtain at value of using Equation (11) ().
- Conduct step test for system under test using control variable (D, in this case) and determine the of the system under test ( s).
- Determine of the experimental setup as seen from the controller, for chosen cut-off frequency of low pass digital filter, as discussed in Section 2.2. ( s).
- Select sample time for perturbation based algorithm () more than (), () approximately.
- (a)
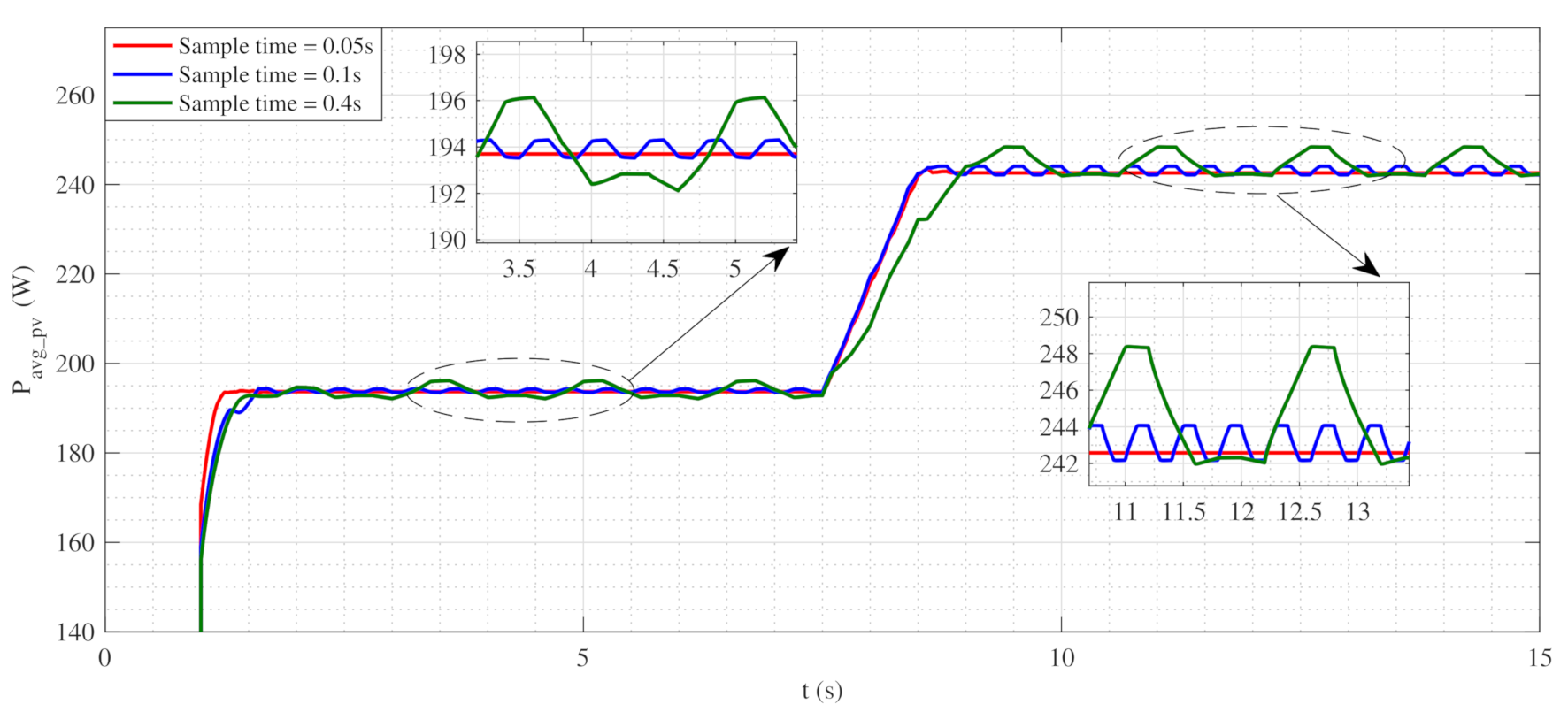
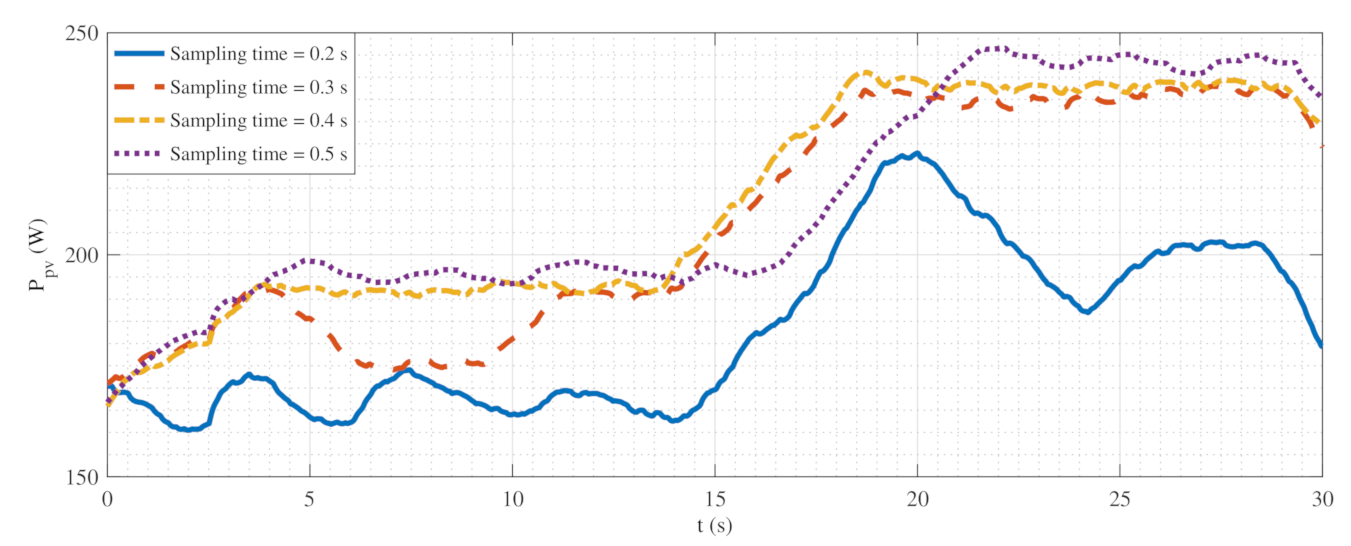
- Experiments are conducted for four different (0.5 s, 0.4 s, 0.3 s, 0.2 s)
- (b)
- Behaviour of and are presented for () and ().
- (c)
- Average PV power extraction is discussed for all four sampling times.
- (d)
- Scaling factor of 1.5 in is chosen empirically.
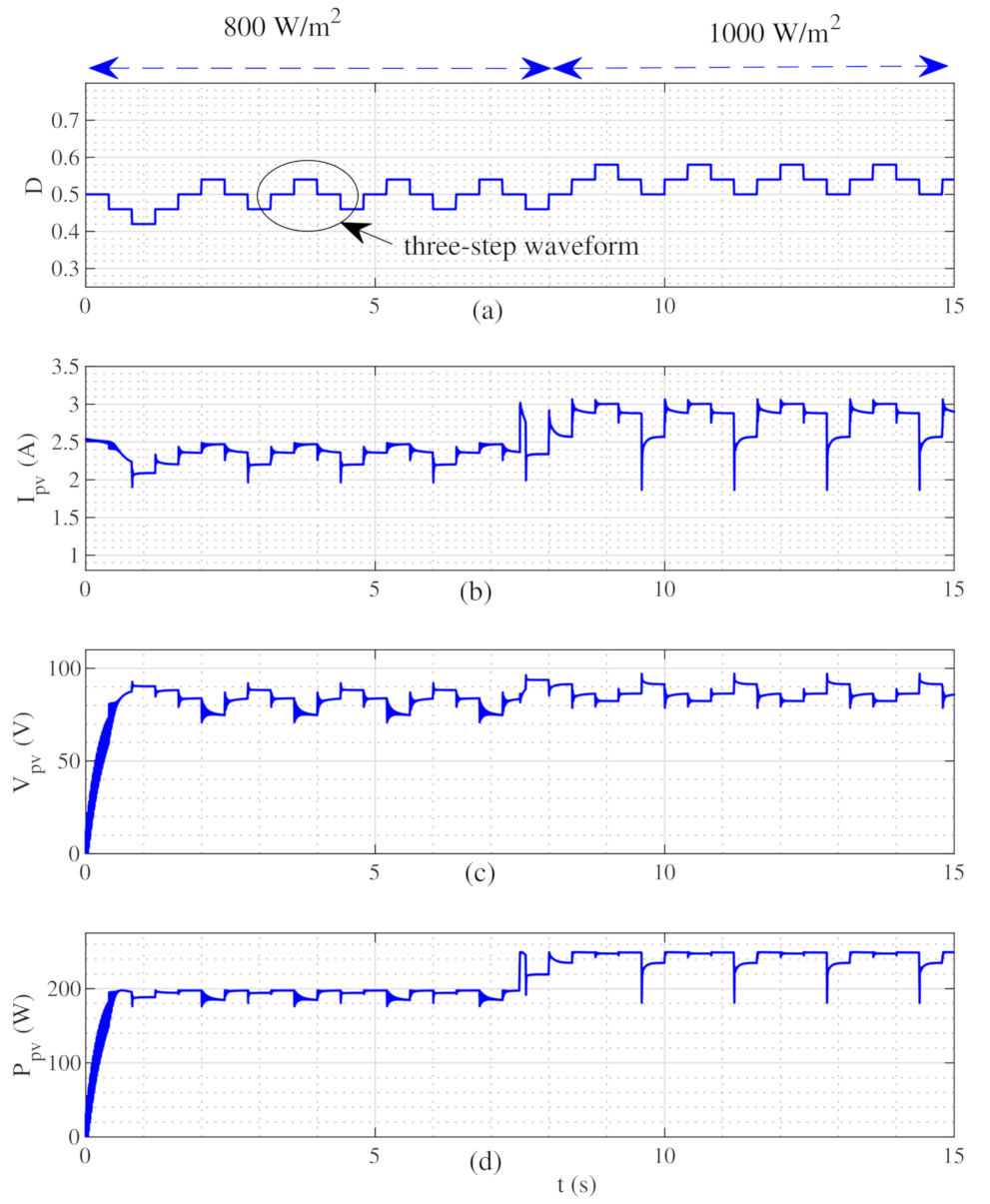
- Energize the system under test with suitable perturbation based algorithm and chosen sample time (), and observe the behavior of control variable (D) and system variables () to exhibit sustained three-step-waveform around their respective steady-state values.
- The sample time can further be optimized based on the required tracking speed and the energy extraction.
- Check the behaviour of control variable and the system variables for step-change in irradiances to ensure three-step waveform or quasi three-step waveform.
4. Results and Discussions
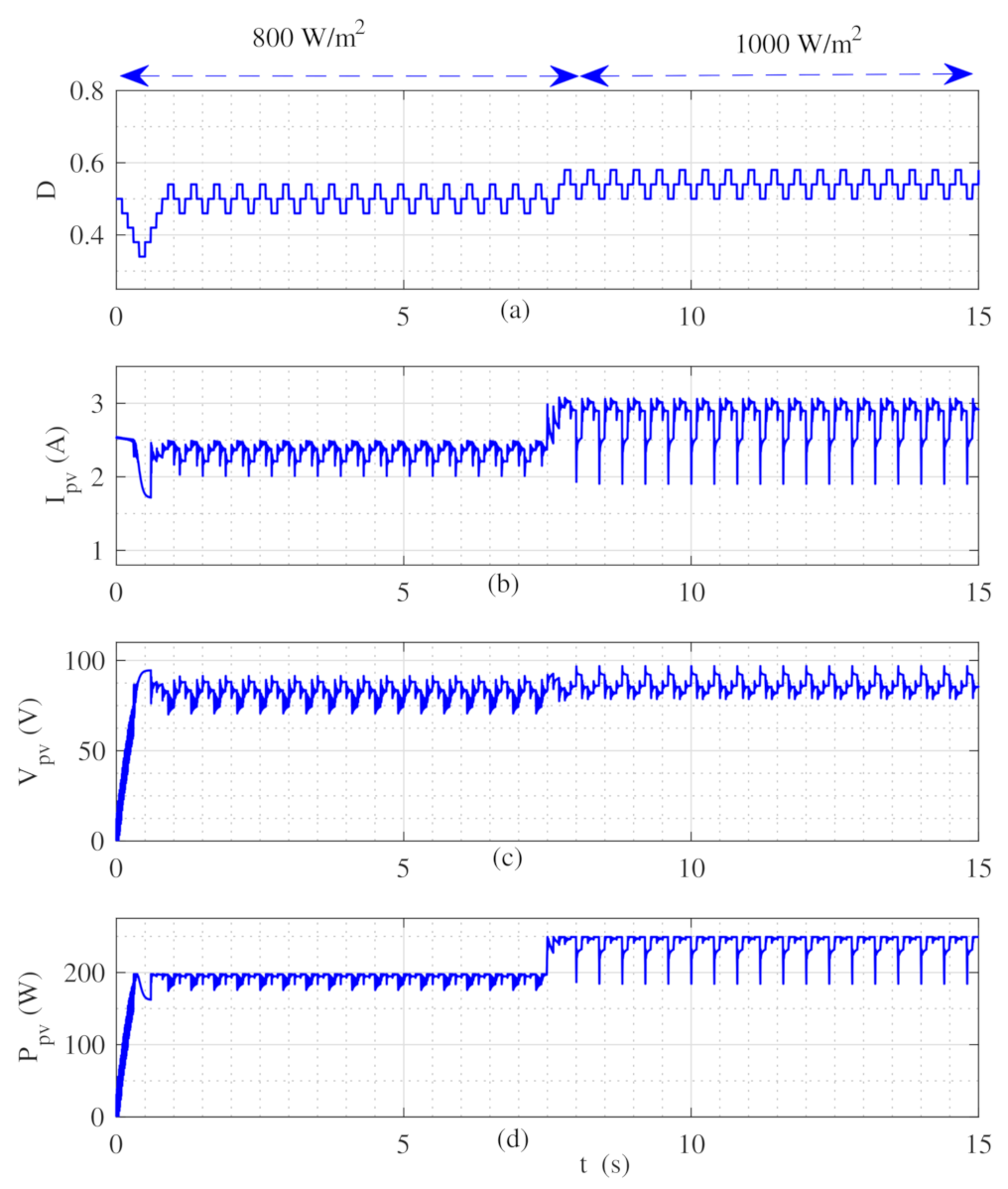
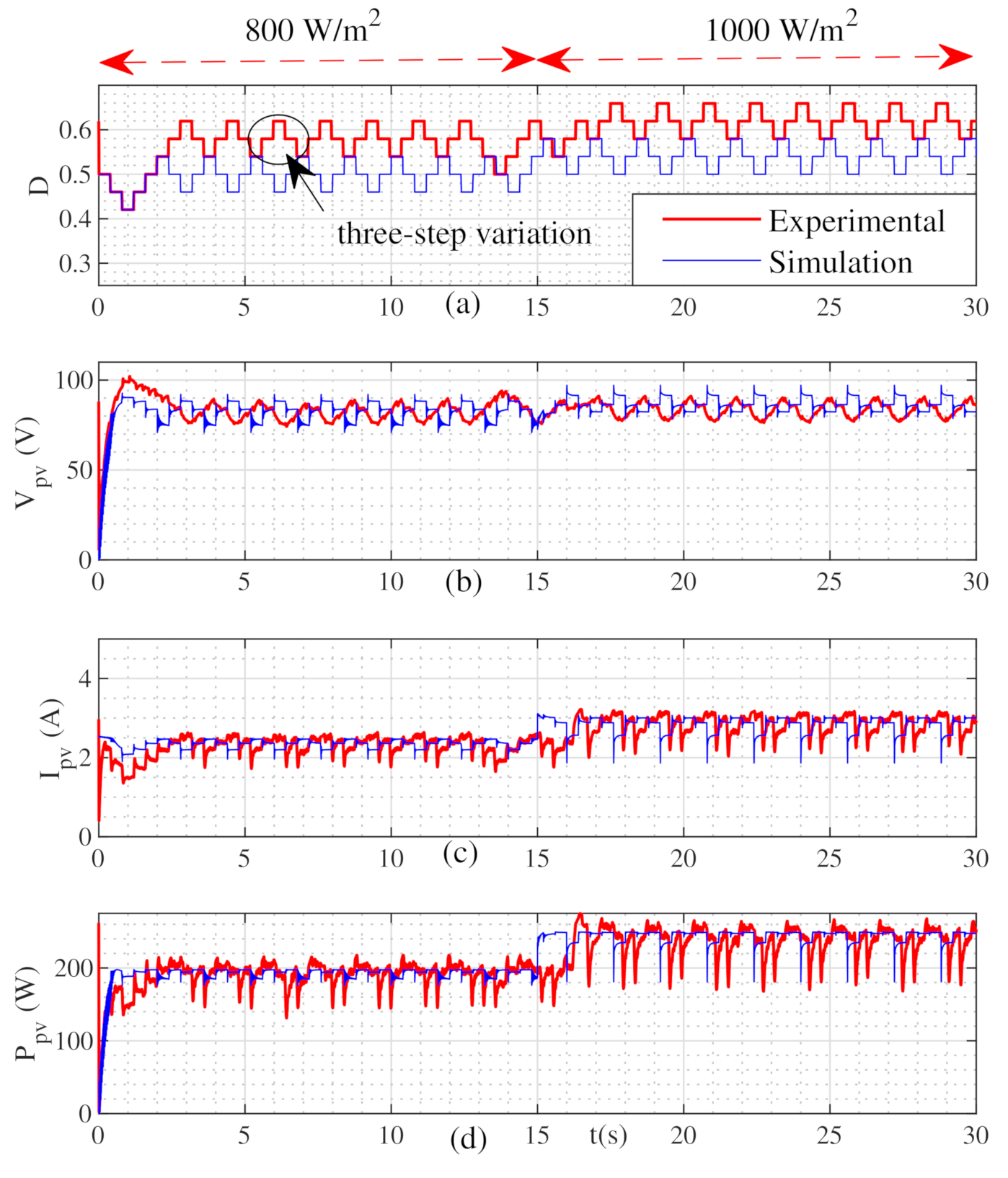
4.1. Simulation Results
4.2. Experimental Results
4.3. Discussions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Elavarasan, R.M. Comprehensive Review on India’s Growth in Renewable Energy Technologies in Comparison With Other Prominent Renewable Energy Based Countries. J. Sol. Energy Eng. 2020, 142. [Google Scholar] [CrossRef]
- Ayop, R.; Tan, C.W. Design of boost converter based on maximum power point resistance for photovoltaic applications. Sol. Energy 2018, 160, 322–335. [Google Scholar] [CrossRef]
- Yahyaoui, I. Advances in Renewable Energies and Power Technologies: Volume 1: Solar and Wind Energies; Elsevier: Amsterdam, The Netherlands, 2018. [Google Scholar]
- Ahmad, R.; Murtaza, A.F.; Sher, H.A. Power tracking techniques for efficient operation of photovoltaic array in solar applications—A review. Renew. Sustain. Energy Rev. 2019, 101, 82–102. [Google Scholar] [CrossRef]
- Motahhir, S.; El Hammoumi, A.; El Ghzizal, A. The most used MPPT algorithms: Review and the suitable low-cost embedded board for each algorithm. J. Clean. Prod. 2020, 246, 118983. [Google Scholar] [CrossRef]
- Ahmed, M.; Abdelrahem, M.; Harbi, I.; Kennel, R. An Adaptive Model-Based MPPT Technique with Drift-Avoidance for Grid-Connected PV Systems. Energies 2020, 13, 6656. [Google Scholar] [CrossRef]
- Ahmed, J.; Salam, Z. An enhanced adaptive P&O MPPT for fast and efficient tracking under varying environmental conditions. IEEE Trans. Sustain. Energy 2018, 9, 1487–1496. [Google Scholar]
- Jana, S.; Kumar, N.; Mishra, R.; Sen, D.; Saha, T.K. Development and implementation of modified MPPT algorithm for boost converter-based PV system under input and load deviation. Int. Trans. Electr. Energy Syst. 2020, 30, e12190. [Google Scholar] [CrossRef]
- Yang, Y.; Wen, H. Adaptive perturb and observe maximum power point tracking with current predictive and decoupled power control for grid-connected photovoltaic inverters. J. Mod. Power Syst. Clean Energy 2019, 7, 422–432. [Google Scholar] [CrossRef] [Green Version]
- Li, X.; Wen, H.; Hu, Y.; Du, Y.; Yang, Y. A comparative study on photovoltaic MPPT algorithms under EN50530 dynamic test procedure. IEEE Trans. Power Electron. 2020, 36, 4153–4168. [Google Scholar] [CrossRef]
- Xu, L.; Cheng, R.; Yang, J. A Modified INC Method for PV String Under Uniform Irradiance and Partially Shaded Conditions. IEEE Access 2020, 8, 131340–131351. [Google Scholar] [CrossRef]
- Femia, N.; Petrone, G.; Spagnuolo, G.; Vitelli, M. Optimization of perturb and observe maximum power point tracking method. IEEE Trans. Power Electron. 2005, 20, 963–973. [Google Scholar] [CrossRef]
- Femia, N.; Petrone, G.; Spagnuolo, G.; Vitelli, M. A technique for improving P&O MPPT performances of double-stage grid-connected photovoltaic systems. IEEE Trans. Ind. Electron. 2009, 56, 4473–4482. [Google Scholar]
- Elgendy, M.A.; Zahawi, B.; Atkinson, D.J. Assessment of perturb and observe MPPT algorithm implementation techniques for PV pumping applications. IEEE Trans. Sustain. Energy 2011, 3, 21–33. [Google Scholar] [CrossRef]
- Elgendy, M.A.; Zahawi, B.; Atkinson, D.J. Assessment of the incremental conductance maximum power point tracking algorithm. IEEE Trans. Sustain. Energy 2012, 4, 108–117. [Google Scholar] [CrossRef]
- Kivimäki, J.; Kolesnik, S.; Sitbon, M.; Suntio, T.; Kuperman, A. Design guidelines for multiloop perturbative maximum power point tracking algorithms. IEEE Trans. Power Electron. 2017, 33, 1284–1293. [Google Scholar] [CrossRef]
- Suntio, T.; Kuperman, A. Maximum Perturbation Step Size in MPP-Tracking Control for Ensuring Predicted PV Power Settling Behavior. Energies 2019, 12, 3984. [Google Scholar] [CrossRef] [Green Version]
- Kivimäki, J.; Kolesnik, S.; Sitbon, M.; Suntio, T.; Kuperman, A. Revisited perturbation frequency design guideline for direct fixed-step maximum power point tracking algorithms. IEEE Trans. Ind. Electron. 2017, 64, 4601–4609. [Google Scholar] [CrossRef]
- Dadkhah, J.; Niroomand, M. Real-Time MPPT Optimization of PV Systems by Means of DCD-RLS Based Identification. IEEE Trans. Sustain. Energy 2018, 10, 2114–2122. [Google Scholar] [CrossRef]
- Tang, L.; Xu, W.; Mu, C. Analysis for step-size optimisation on MPPT algorithm for photovoltaic systems. IET Power Electron. 2017, 10, 1647–1654. [Google Scholar] [CrossRef]
- Yan, K.; Du, Y.; Ren, Z. MPPT perturbation optimization of photovoltaic power systems based on solar irradiance data classification. IEEE Trans. Sustain. Energy 2018, 10, 514–521. [Google Scholar] [CrossRef]
- Bhattacharyya, S.; Patnam, D.S.K.; Samanta, S.; Mishra, S. Steady Output and Fast Tracking MPPT (SOFT MPPT) for P&O and InC Algorithms. IEEE Trans. Sustain. Energy 2020, 12, 293–302. [Google Scholar]
- Al-Atrash, H.; Batarseh, I.; Rustom, K. Effect of measurement noise and bias on hill-climbing MPPT algorithms. IEEE Trans. Aerosp. Electron. Syst. 2010, 46, 745–760. [Google Scholar] [CrossRef]
- Villalva, M.G.; Gazoli, J.R.; Ruppert Filho, E. Comprehensive approach to modeling and simulation of photovoltaic arrays. IEEE Trans. Power Electron. 2009, 24, 1198–1208. [Google Scholar] [CrossRef]
- Fannakh, M.; Elhafyani, M.L.; Zouggar, S. Hardware implementation of the fuzzy logic MPPT in an Arduino card using a Simulink support package for PV application. IET Renew. Power Gener. 2018, 13, 510–518. [Google Scholar] [CrossRef]
- Angadi, S.; Yaragatti, U.R.; Suresh, Y.; Raju, A. Implementation of Perturbation-Based MPPT Technique Using Model-Based Design. In Electronic Systems and Intelligent Computing; Springer: Berlin, Germany, 2020; pp. 155–166. [Google Scholar]
- Erickson, R.W.; Maksimovic, D. Fundamentals of Power Electronics; Springer Science & Business Media: New York, NY, USA, 2007. [Google Scholar]
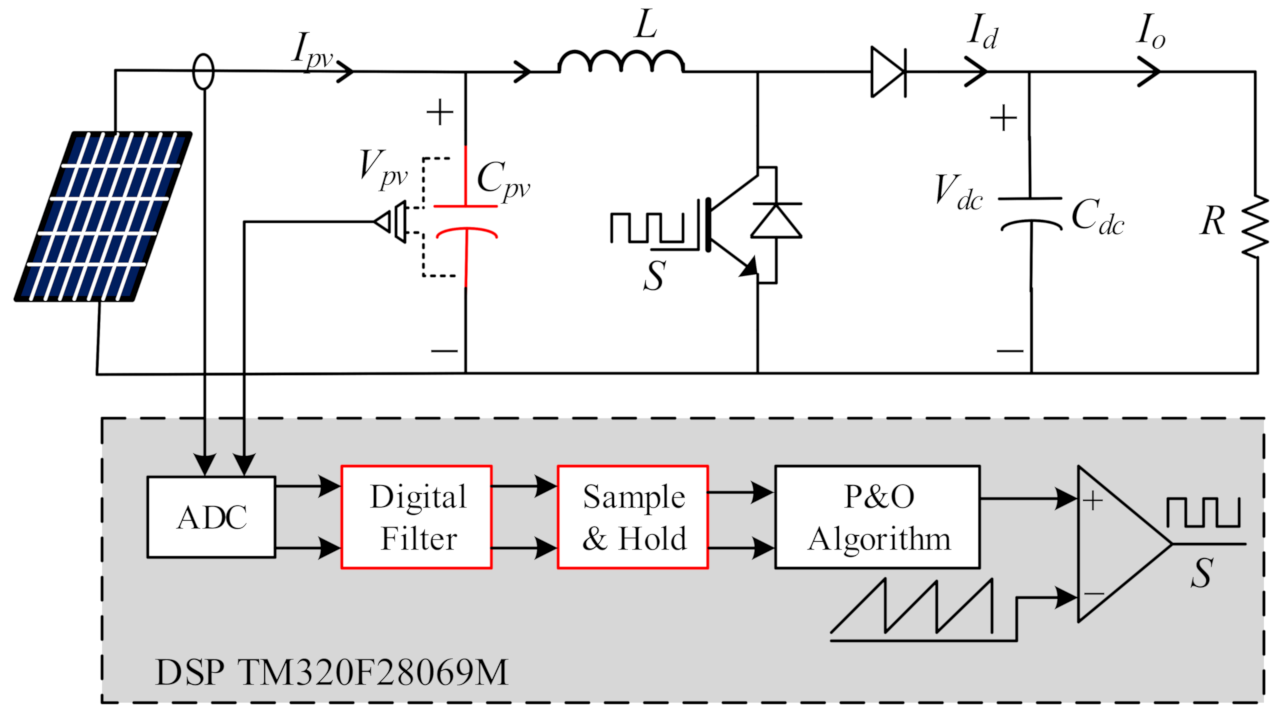





| System Parameter | Value (Unit) |
|---|---|
| Open circuit Voltage, | 21.47 V |
| Short circuit current, | 3.11 A |
| Voltage at Maximum Power, | 17.21 V |
| Current at Maximum Power, | 2.91 A |
| Module maximum power, | 50 W |
| Temperature coefficient of Current , | 0.0154 C/V) |
| Temperature coefficient of Voltage, | −0.2775 C/mA) |
| PV array power (5 modules in series) | 250 W |
| G (W/) | (V) | (A) | () | (W) | |
|---|---|---|---|---|---|
| 800 | 86.14 | 2.331 | 36.95 | 200 | 0.45 |
| 1000 | 85.63 | 2.91 | 29.42 | 250 | 0.5 |
| Component | Type/Value |
|---|---|
| IGBT Module | SKM 200 GB12E4 (Semikron, Nuremberg, Germany) |
| IGBT drivers | SKYPER 32 Pro (Semikron, Nuremberg, Germany) |
| Input capacitor | 220 |
| DC link Capacitor | 2350 |
| Load resistor | 120 |
| Switching Frequency | 10 kHz |
| Inductor | 3.5 mH |
| Current Sensor | LA 25-P |
| Voltage sensor | LV 25-P |
| 250 W Solar PV Array | Magna SL 300-5 Emulator |
| Controller | TMS320F28069M |
| Parameters | G (W/m) | (V) | (A) | () | (W) | |
|---|---|---|---|---|---|---|
| Theoretical results | 800 1000 | 86.14 85.63 | 2.331 2.91 | 36.95 29.42 | 200 250 | 0.45 0.5 |
| Simulation results | 800 1000 | 82.7 87.5 | 2.35 2.81 | 35.19 31.13 | 194 245.875 | 0.5 0.54 |
| Experimental results | 800 1000 | 82.15 83.46 | 2.27 2.843 | 35.24 30.12 | 186.48 237.27 | 0.58 0.62 |
| Parameters\Ref | [12,13] | [14,15] | [16,18] | [19] | Proposed |
|---|---|---|---|---|---|
| System time constant | SSM | SSM | SSM | DCD-RLS | Step test -01 |
| Digital Filter delay | Neglected | 100 Hz (0.01 s) | Neglected | Neglected | Step-test - 02 |
| Sampling time | 1.1* | Trial and error | 1.5 | ||
| Comprehensiveness | No | No | No | No | Yes |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Angadi, S.; Yaragatti, U.R.; Suresh, Y.; Raju, A.B. System Parameter Based Performance Optimization of Solar PV Systems with Perturbation Based MPPT Algorithms. Energies 2021, 14, 2007. https://doi.org/10.3390/en14072007
Angadi S, Yaragatti UR, Suresh Y, Raju AB. System Parameter Based Performance Optimization of Solar PV Systems with Perturbation Based MPPT Algorithms. Energies. 2021; 14(7):2007. https://doi.org/10.3390/en14072007
Chicago/Turabian StyleAngadi, Sachin, Udaykumar R. Yaragatti, Yellasiri Suresh, and A. B. Raju. 2021. "System Parameter Based Performance Optimization of Solar PV Systems with Perturbation Based MPPT Algorithms" Energies 14, no. 7: 2007. https://doi.org/10.3390/en14072007
APA StyleAngadi, S., Yaragatti, U. R., Suresh, Y., & Raju, A. B. (2021). System Parameter Based Performance Optimization of Solar PV Systems with Perturbation Based MPPT Algorithms. Energies, 14(7), 2007. https://doi.org/10.3390/en14072007






