Laboratory Study of the Influence of Fluid Rheology on the Characteristics of Created Hydraulic Fracture
Abstract
:1. Introduction
2. Materials and Methods
2.1. Material Description
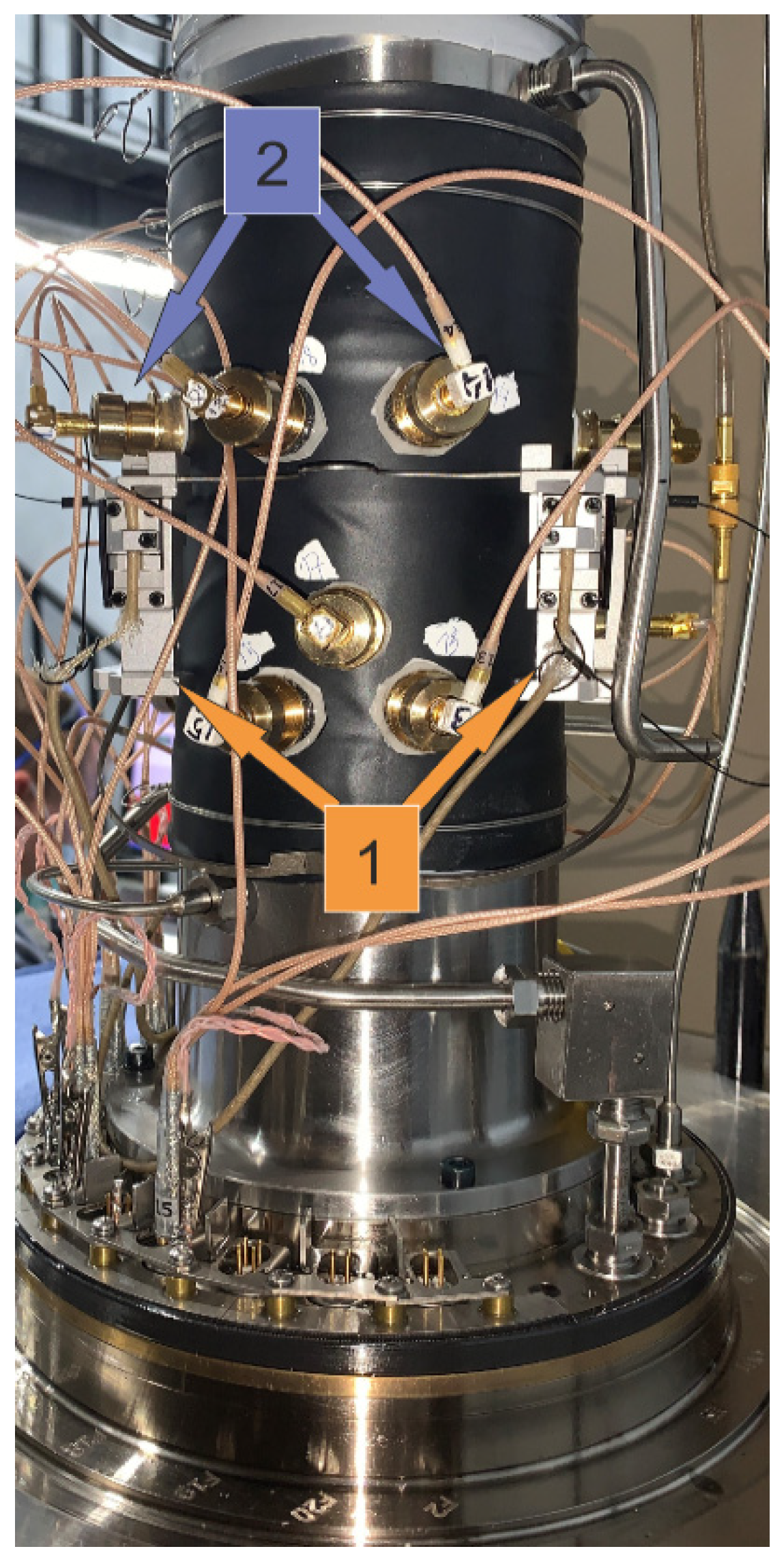
2.2. Experimental Setup
2.3. Fracturing Fluids
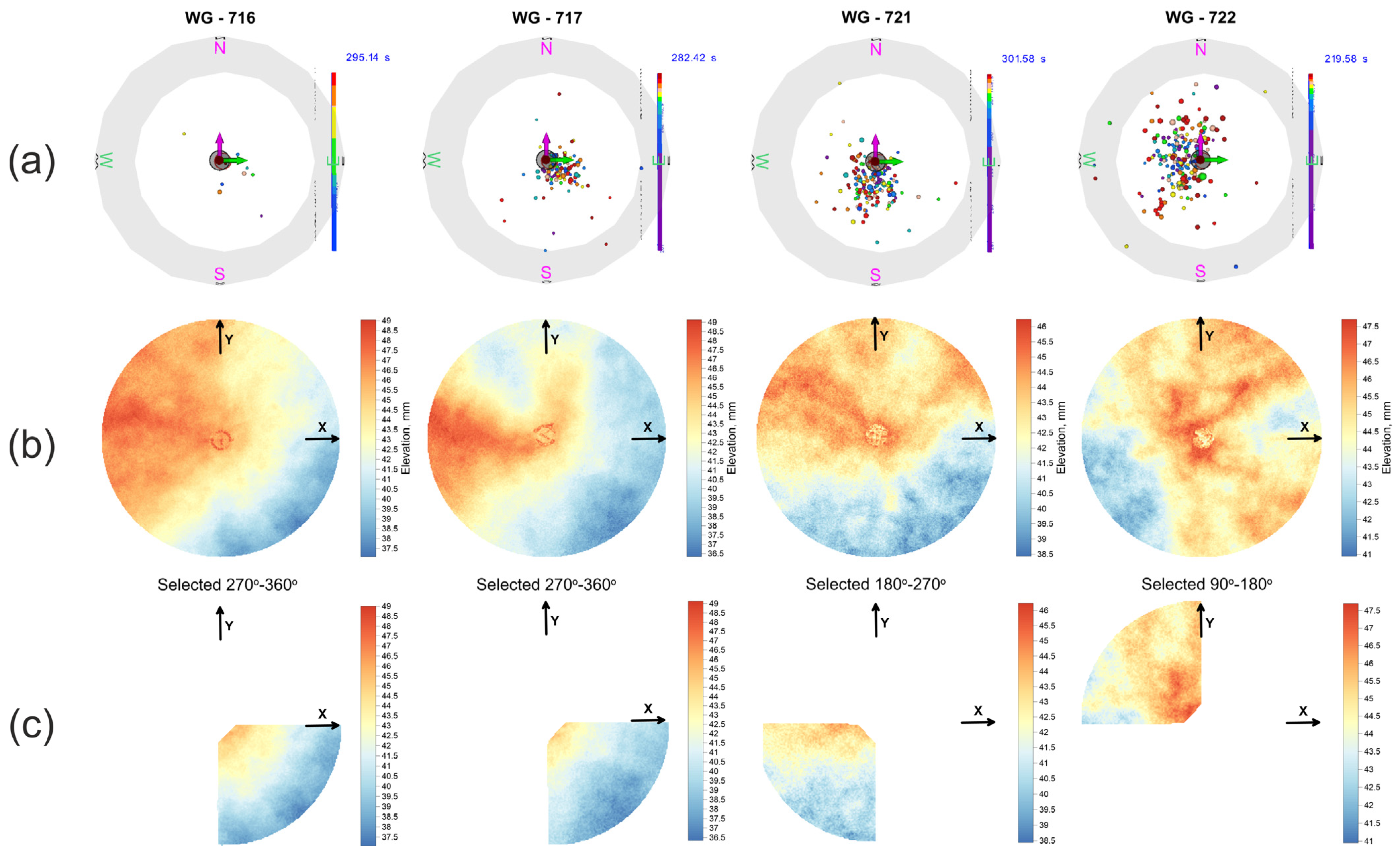
3. Results
3.1. Injection of the Non-Polymer Composition Based on Ampholyte Surfactant (NEFTENOL-VES)
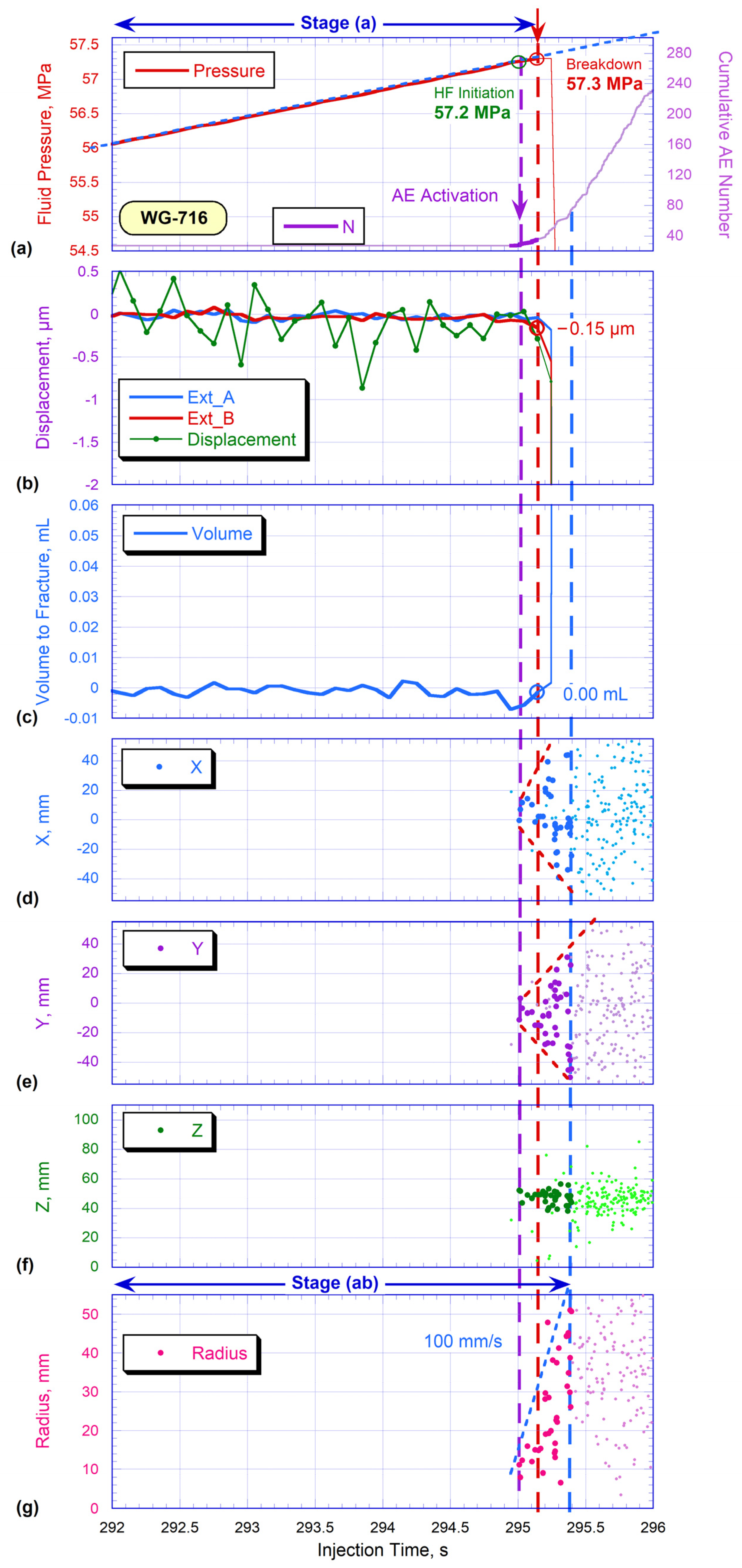
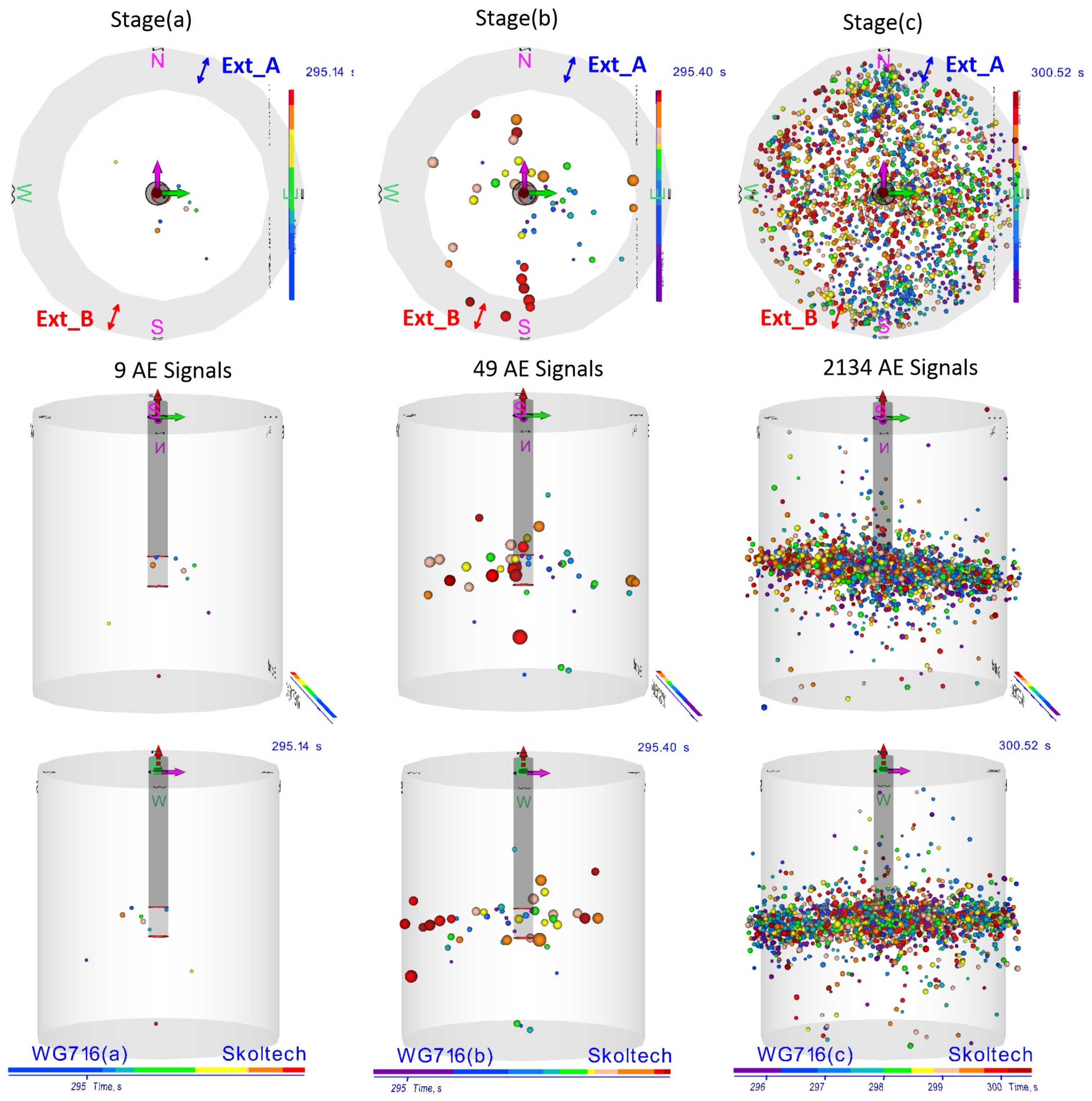
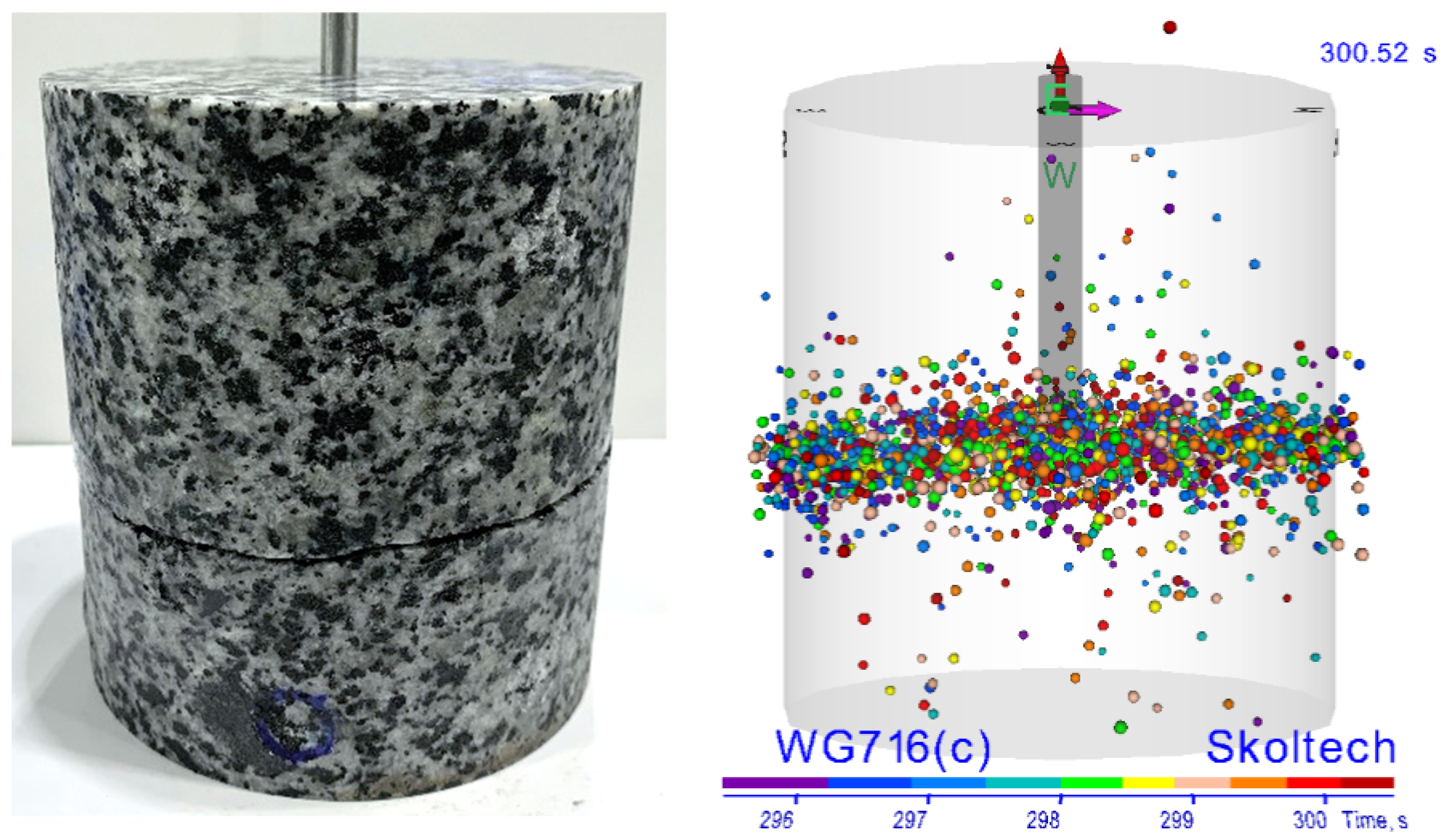
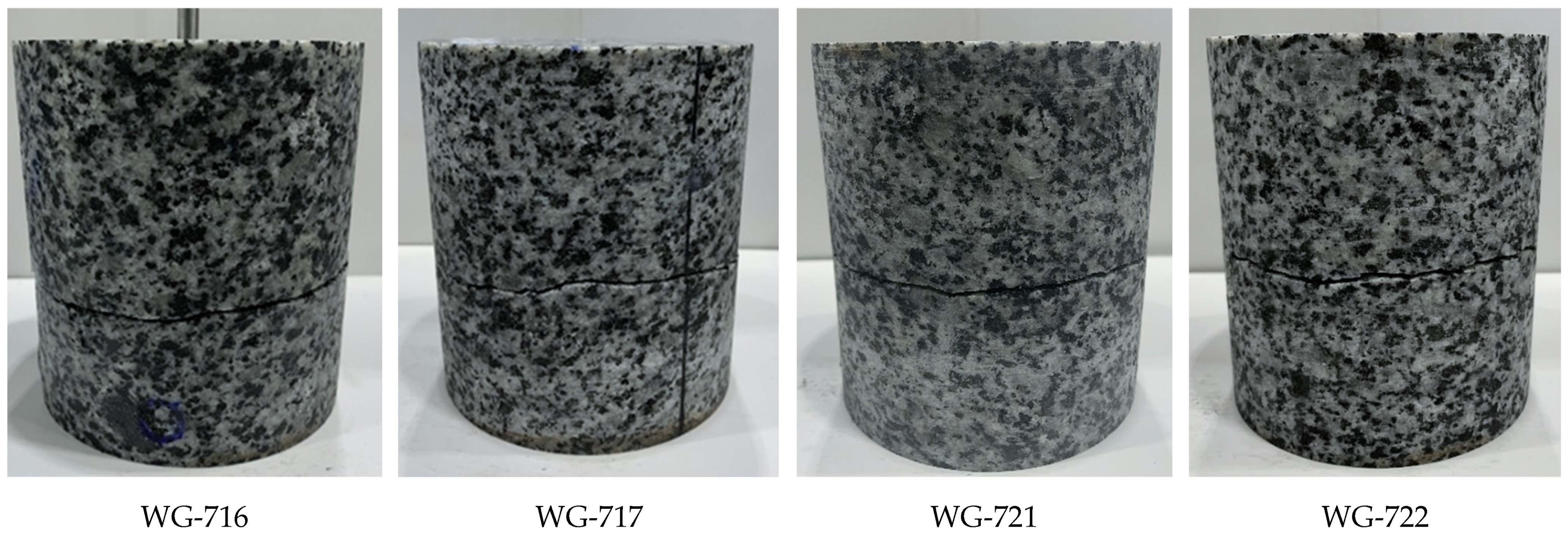
- Sample WG-716
3.2. Injection of the Water-Based Synthetic Polymer Solution (PolyGel)
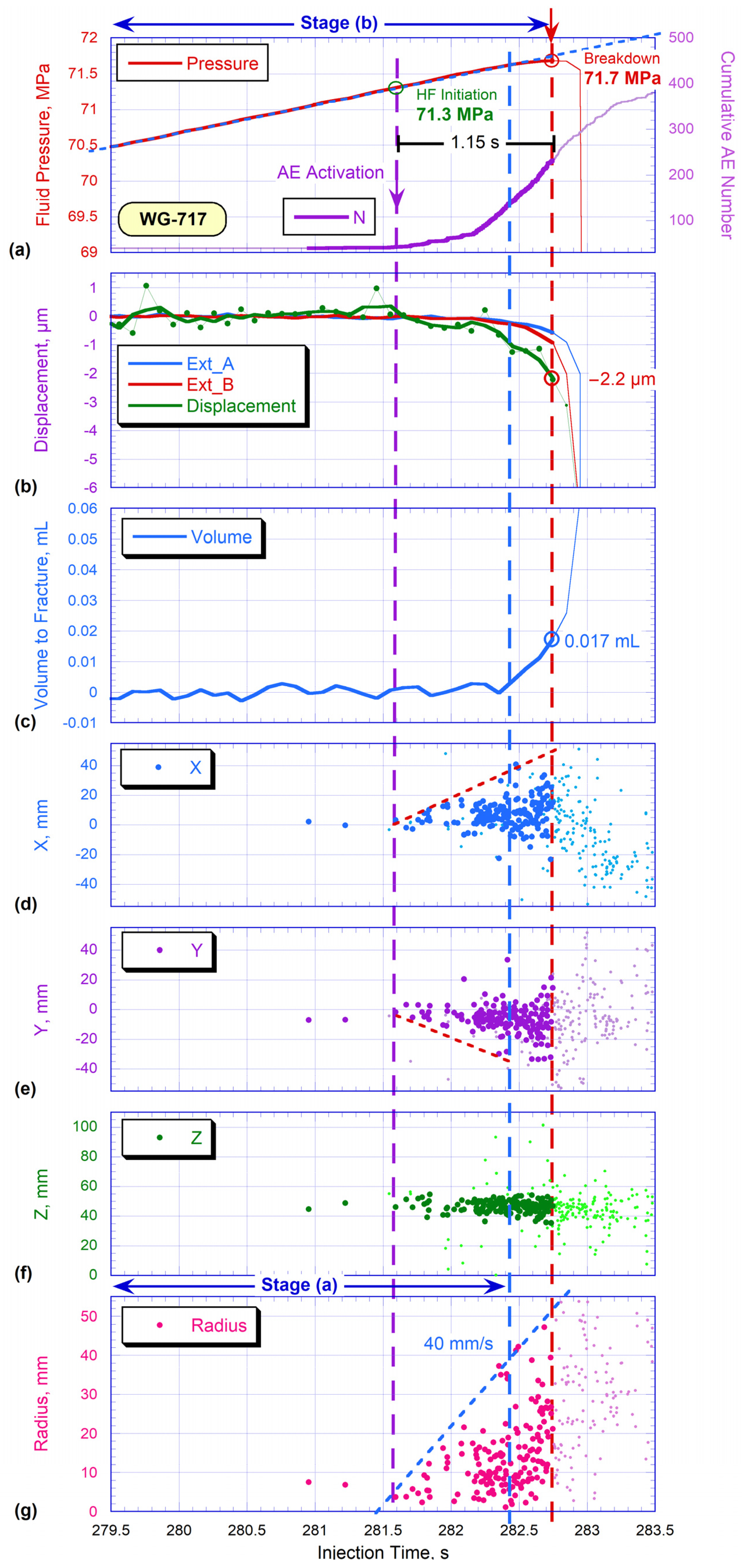
- Sample WG-717
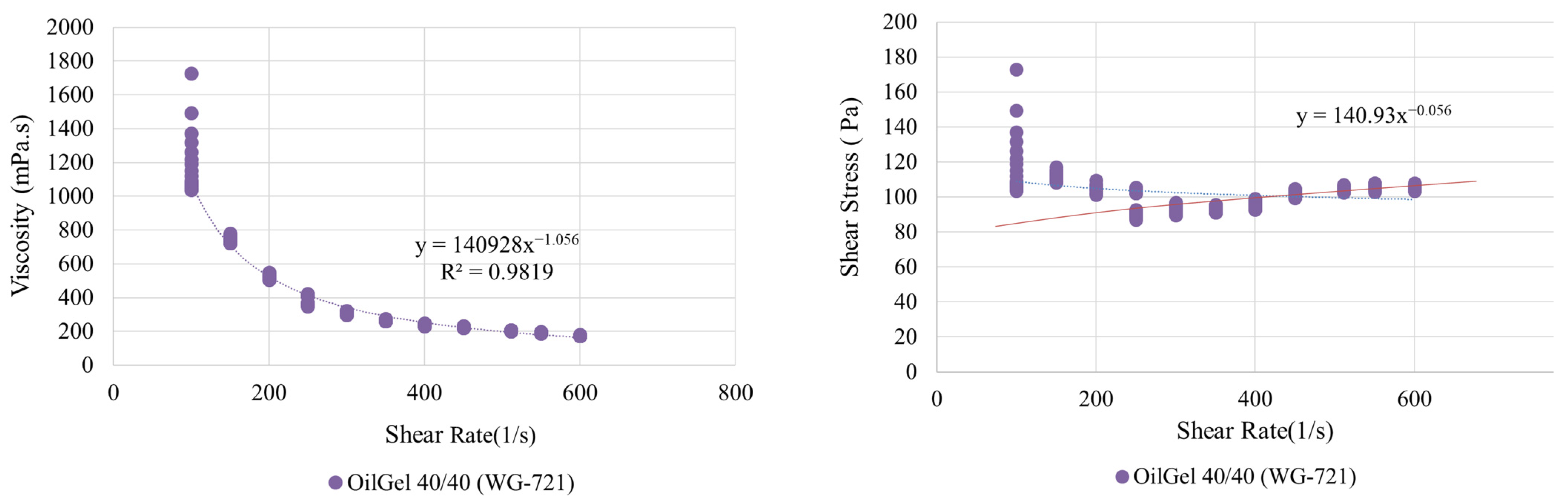
3.3. Injection of Diesel Fuel-Based Fracturing Fluid with Gelling Complex Grade “N” (OilGel 40/40; OilGel 7/7)
- Sample WG-721
- Sample WG-722
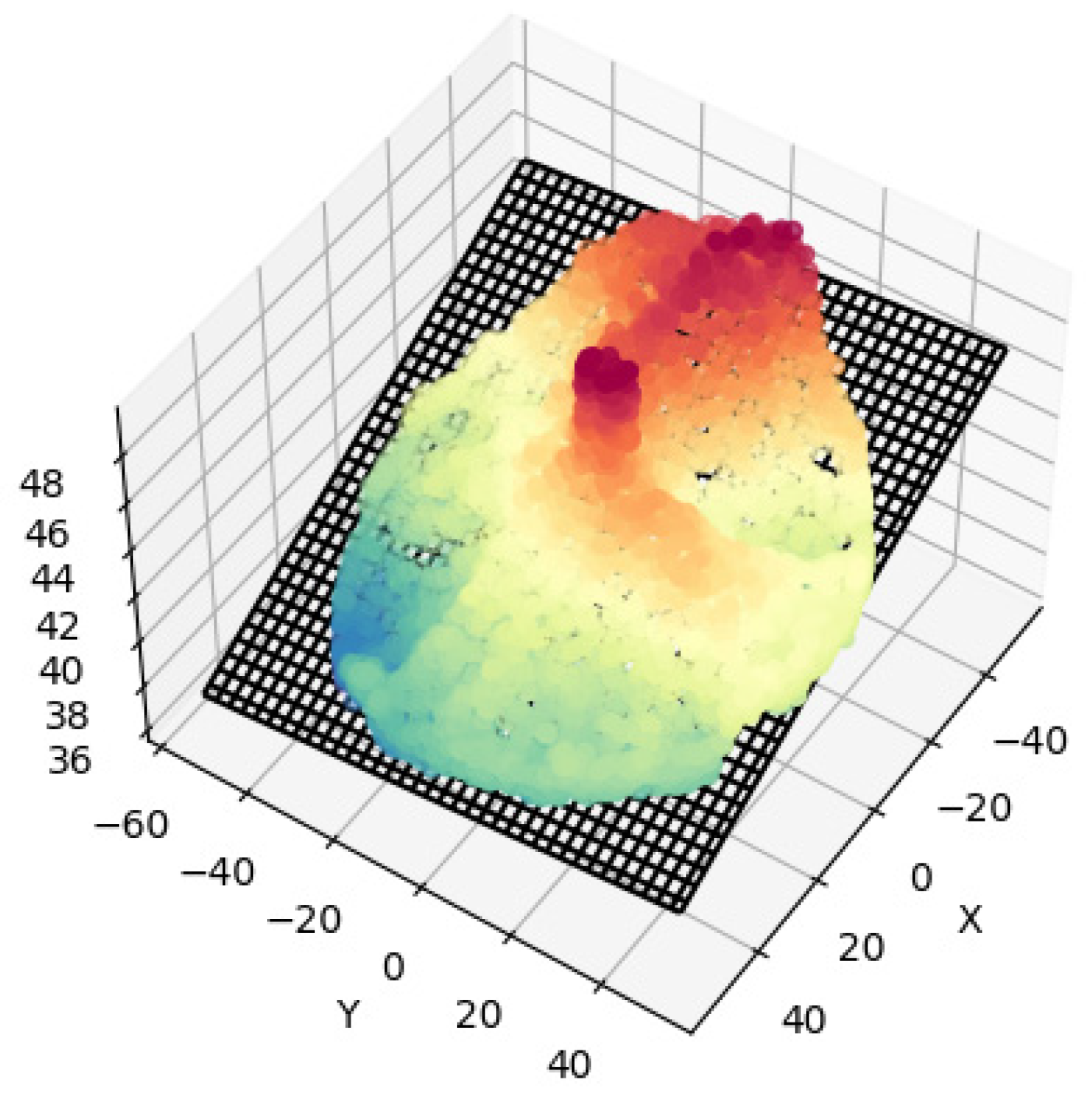
4. Discussion
5. Conclusions
- (1)
- For our experiments, we used two water-based systems (non-polymer composition (VES), PAA (PolyGel)) and two non-aqueous (OilGel 7/7, OilGel 40/40) fluids for the HF initiation. In contrast to the traditionally used cross-linked liquids based on guar solution, they have a structural difference. It is generally accepted (in Russian service companies) that the fracturing fluid must have a viscosity of at least 350 mPa·s at 100 s−1 for proper proppant retention and transport. The described fluids have a branched spatial structure, which allows to hold and transport the proppant despite their low viscosity (100–200 mPa·s at 100 s−1). Moreover, hydraulic fracturing induced by injection of these systems had not been previously tested in laboratory conditions. The fluids were provided by the department of the international scientific center “Rational development of liquid hydrocarbon planet’s reserves” at the National University of Oil and Gas “Gubkin University”;
- (2)
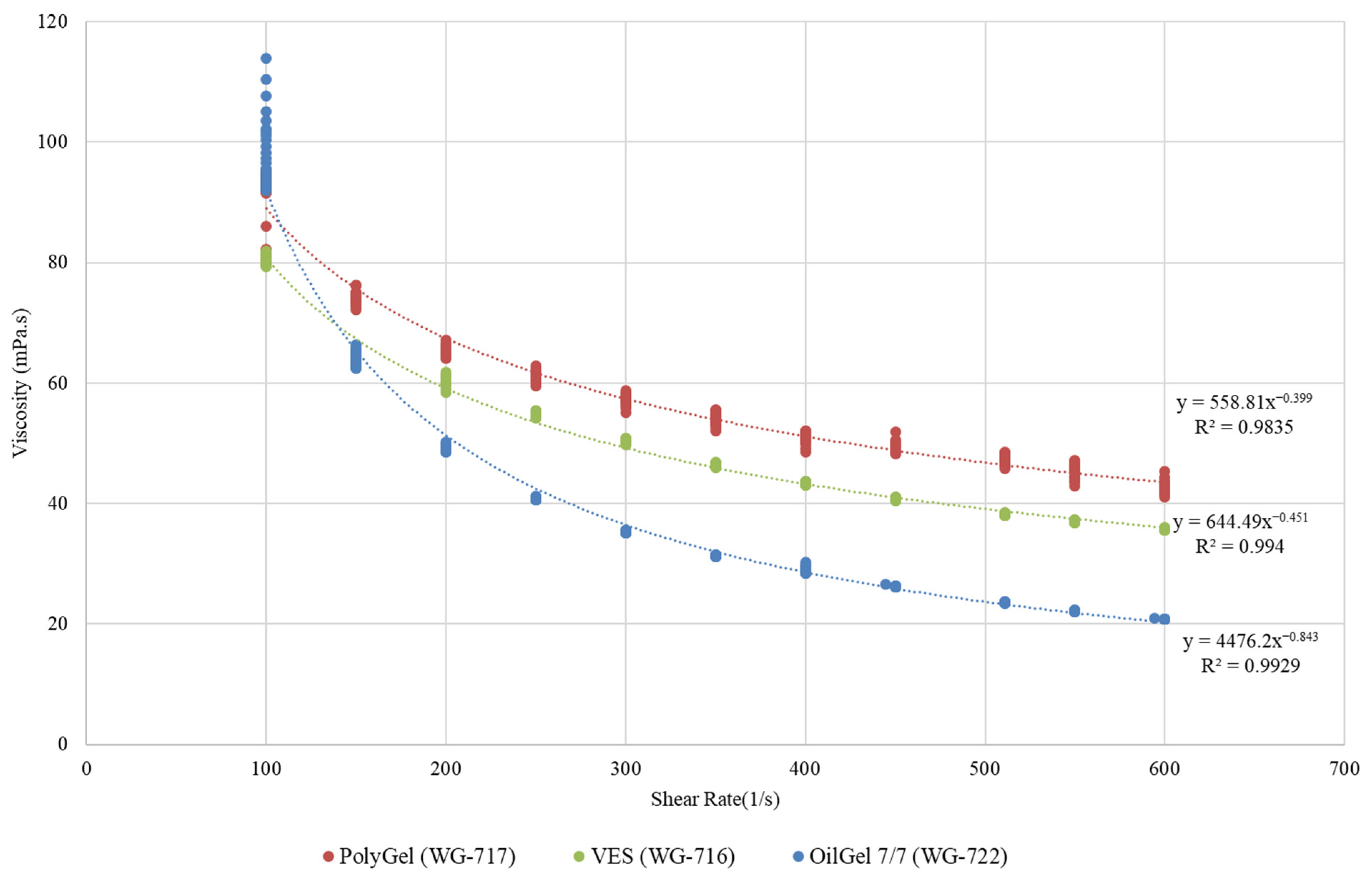
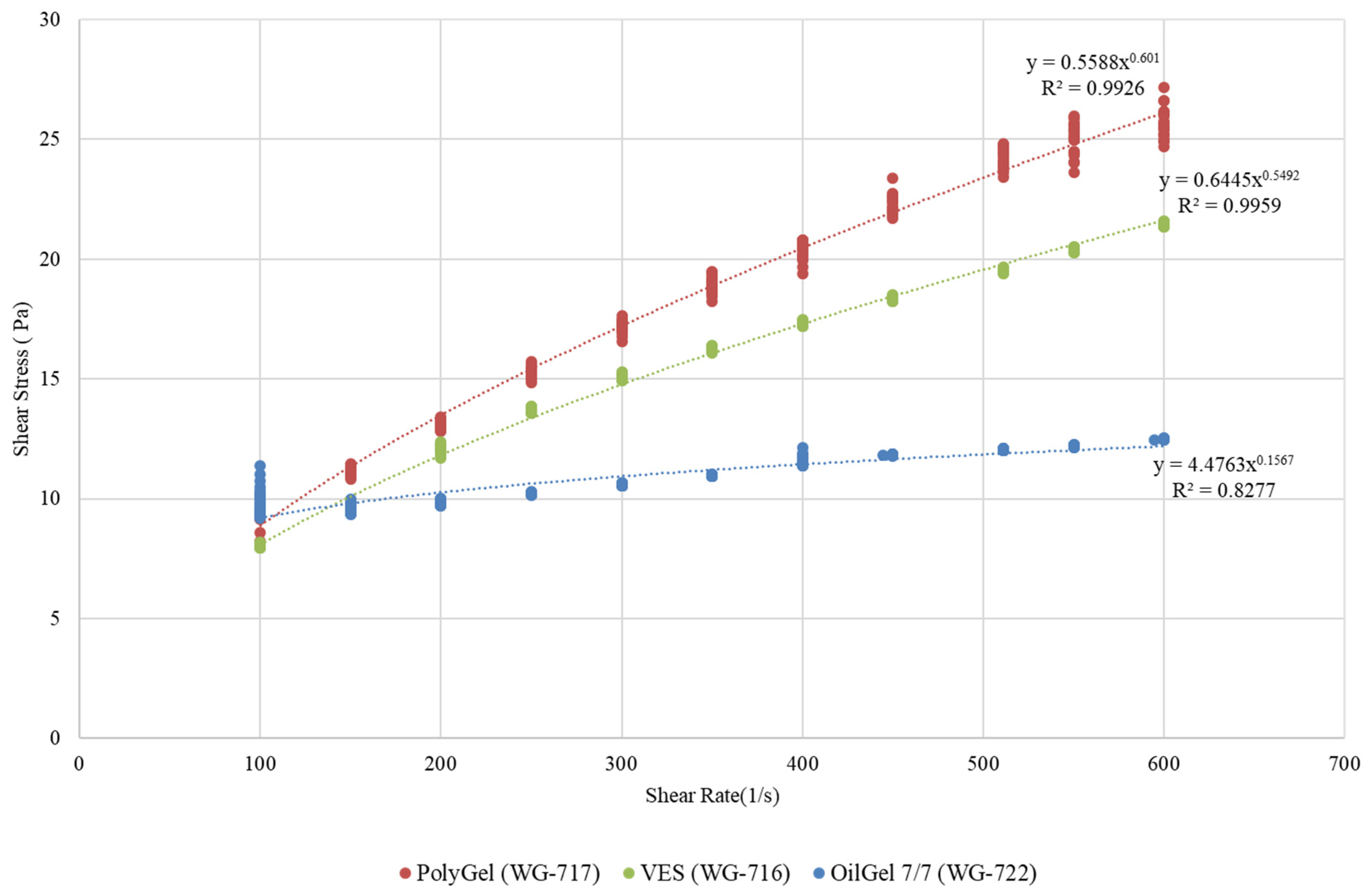
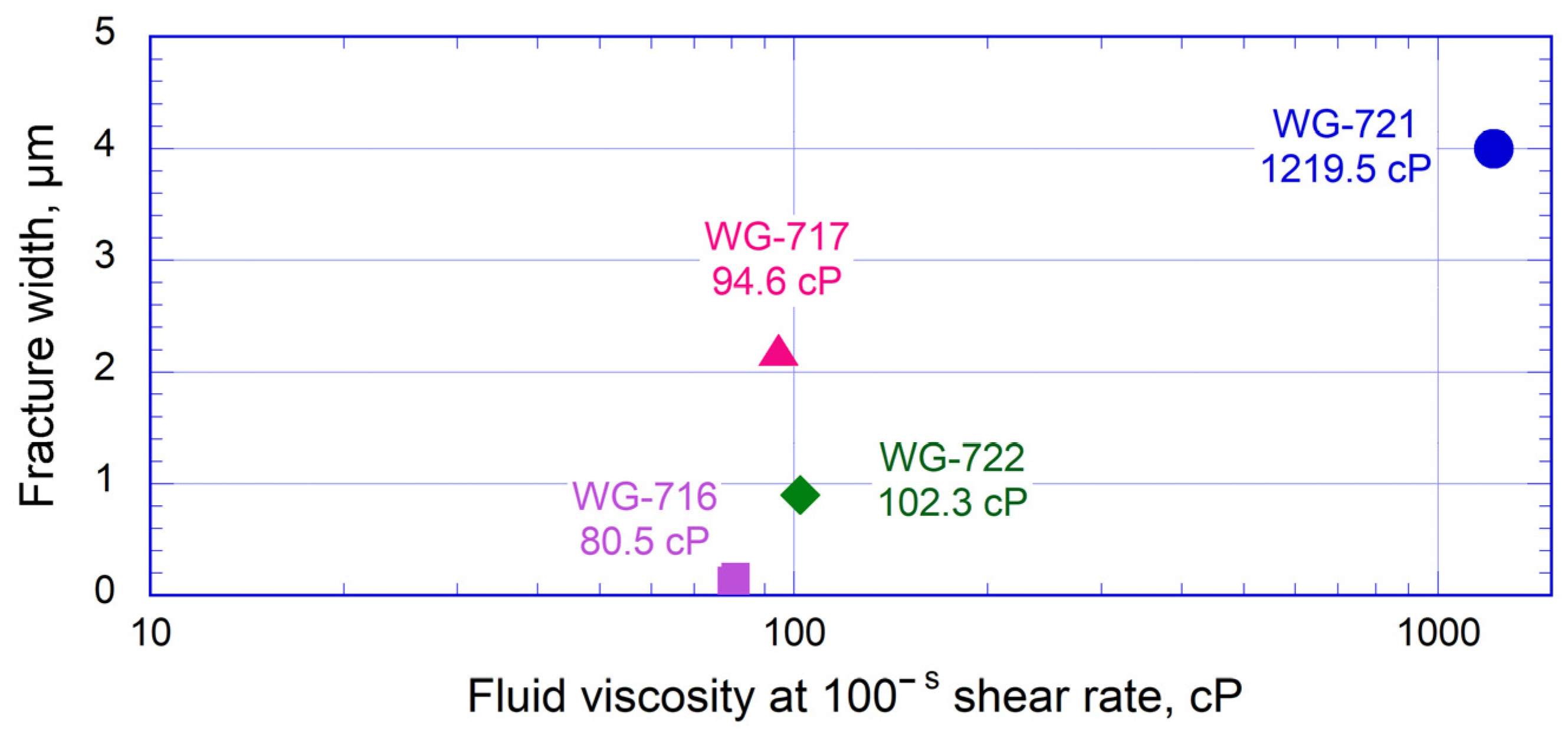
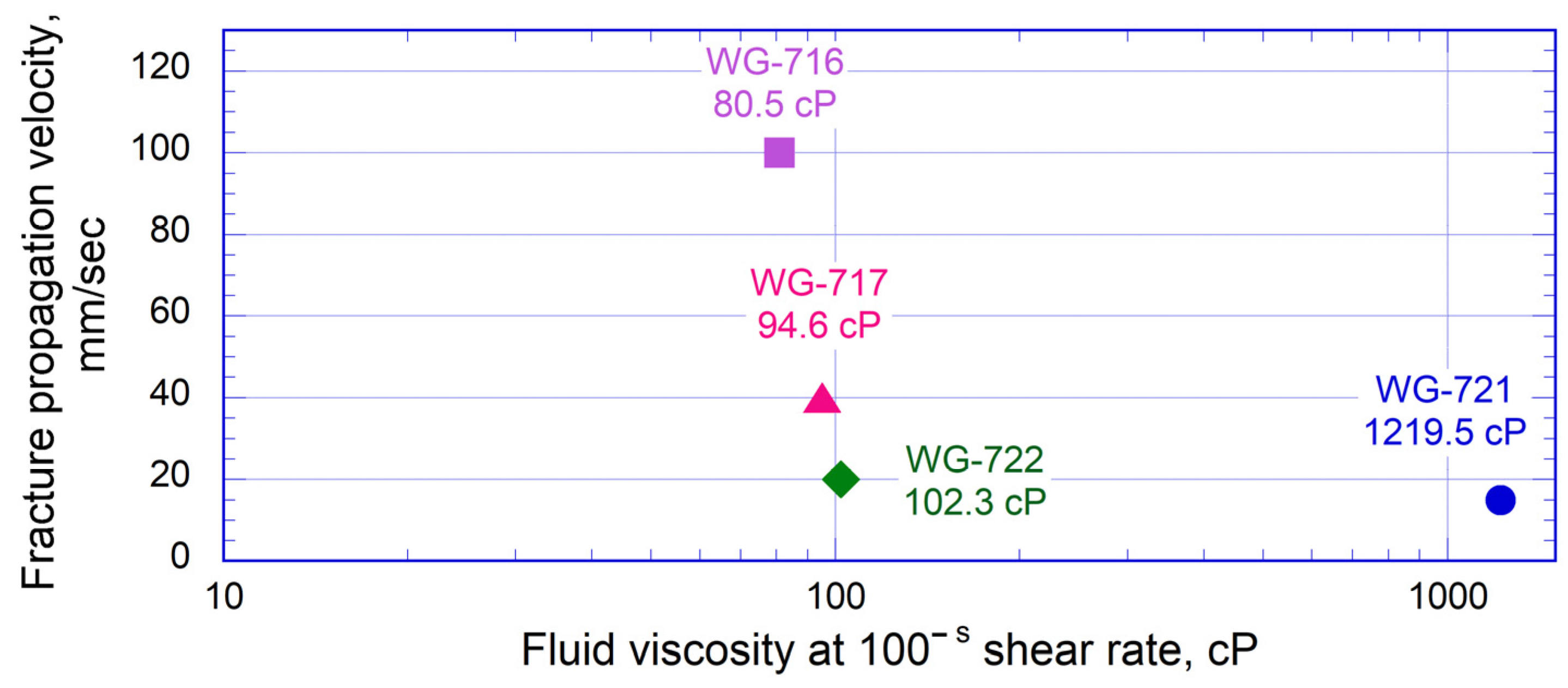
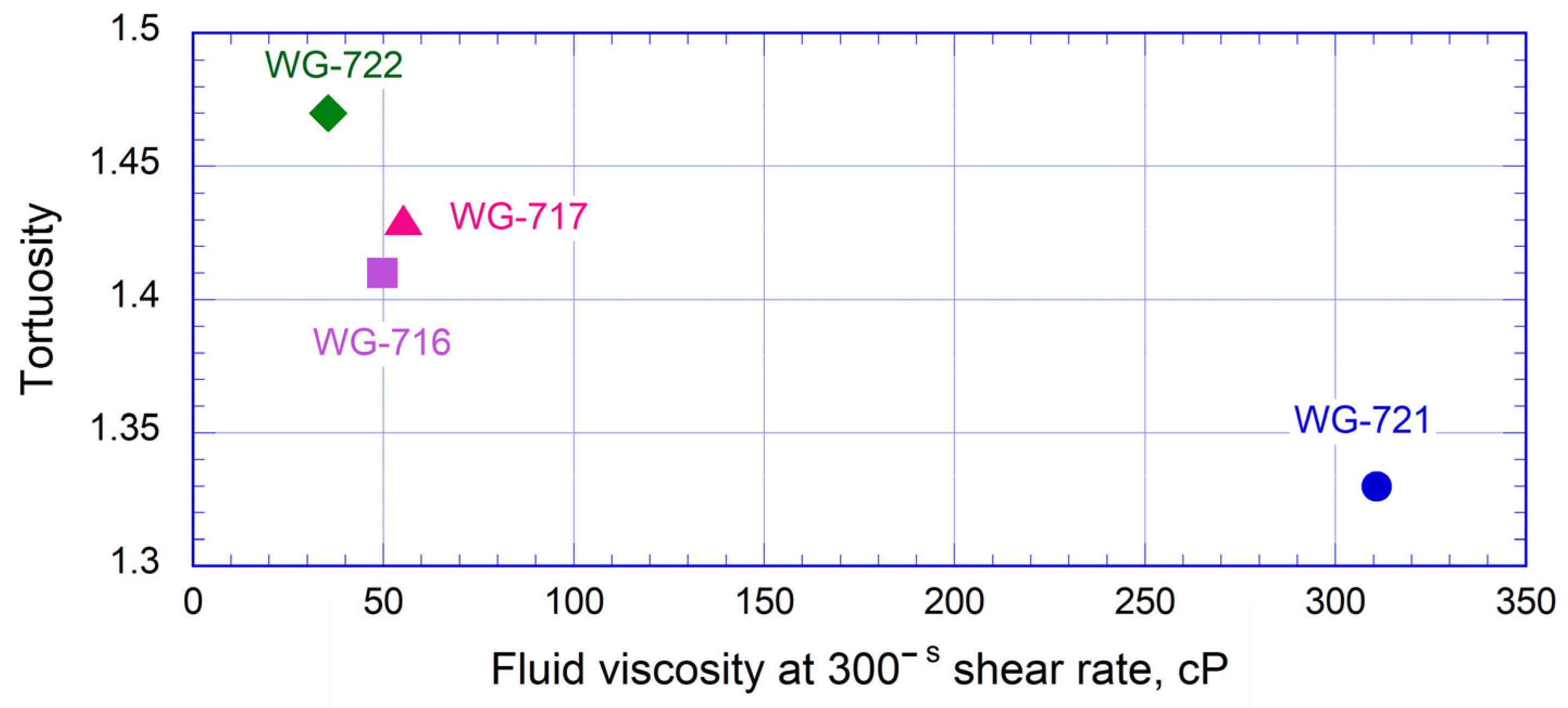
- We measured hydraulic fracture opening width and the volume of fluid injected into the fracture at the moment of wellbore pressure breakdown. We have found a good correlation between these parameters and viscosity values. Initially, the results of two tests with water-based fluids were compared. The behavior of the fracture created by injection of the non-polymer composition (VES) demonstrated quick propagation, minor opening, insignificant volume of fluid filling the fracture, and low breakdown pressure. This behavior of hydraulic fracture can characterize the injection of low-viscosity fluids. In case of PolyGel fluid injection, the hydraulic fracture propagated more slowly, the extensometers registered a bigger opening width, and hydraulic fracture occurred at the highest maximum pressure among the tests.
- (3)
- It has also been revealed that the topography of the created fracture surface is related to the viscosity of the fracturing fluid too. We have observed that the fracture induced by the most viscous fluid had the least tortuous surface. Based on the data of our study, the tortuosity decreased with the increase in fracturing fluid viscosity, which is in good agreement with [46,47];
- (4)
- The observed relationships should be taken into account during modeling of the hydraulic fracture propagation. This may lead to the creation of more realistic models, and as a result, to an increase in the efficiency of the field hydraulic fracturing.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lin, C.; Mao, J.; He, J.; Li, X.; Zhao, J. Propagation characteristics and aperture evolution of hydraulic fractures in heterogeneous granite cores. Arab. J. Geosci. 2019, 12, 684. [Google Scholar] [CrossRef]
- Suppachoknirun, T.; Tutuncu, A.N. Hydraulic Fracturing and Production Optimization in Eagle Ford Shale Using Coupled Geomechanics and Fluid Flow Model. Rock Mech. Rock Eng. 2017, 50, 3361–3378. [Google Scholar] [CrossRef]
- Mojid, M.R.; Negash, B.M.; Abdulelah, H.; Jufar, S.R.; Adewumi, B.K. A state–of–art review on waterless gas shale fracturing technologies. J. Pet. Sci. Eng. 2021, 196, 108048. [Google Scholar] [CrossRef]
- Turuntaev, S.B.; Zenchenko, E.V.; Zenchenko, P.E.; Trimonova, M.A. Hydraulic Crack Growth Dynamics from Ultrasound Transmission Monitoring in Laboratory Experiments. Izv. Phys. Solid Earth 2021, 57, 671–685. [Google Scholar] [CrossRef]
- Bukharov, D.F.; Alekseev, Y.V.; Prodan, A.S.; Nenko, A.V. Potential and Possible Technological Solutions for Field Development of Unconventional Reservoirs: Bazhenov Formation. In Proceedings of the SPE Russian Petroleum Technology Conference, Virtual, 26 October 2020. [Google Scholar]
- Barati, R.; Liang, J.-T. A review of fracturing fluid systems used for hydraulic fracturing of oil and gas wells. J. Appl. Polym. Sci. 2014, 131, 40735. [Google Scholar] [CrossRef]
- Silin, M.A.; Magadova, L.; Malkin, D.; Krisanova, P.K.; Krashevnikova, V. Development of a viscoelastic composition based on surfactants for hydraulic fracturing. Proc. Gubkin Russ. State Univ. Oil Gas 2020, 1, 142–154. [Google Scholar] [CrossRef]
- Magadova, L.A.; Silin, M.A.; Gluschenko, V.N. Technological Aspects and Materials for Hydraulic Fracturing; National University of Oil and Gas «Gubkin University»: Moscow, Russia, 2012; ISBN 9785919610755. [Google Scholar]
- Zhang, R.; Zheng, X.; Li, S.; Jin, K.; Zheng, H.; Wang, L. Environmentally friendly hydraulic fracturing and water-free fracturing technologies. Int. J. Oil, Gas Coal Technol. 2018, 17, 375–390. [Google Scholar] [CrossRef]
- Alharith, A.; Batarseh, S.; San Roman Alerigi, D.; Asiri, W. Overview of Recent Waterless Stimulation Technologies. In Proceedings of the Abu Dhabi International Petroleum Exhibition & Conference, Abu Dhabi, United Arab Emirates, 9 November 2020. [Google Scholar]
- Duan, Y.; Xu, Y.; Weng, D.; Lu, Y.; Qiu, X.; Wang, L. Research on Waterless Fracturing Technology in Tight Gas Formation in China. In Proceedings of the International Field Exploration and Development Conference 2019, SSGG; Lin, J., Ed.; Springer: Singapore, 2020; pp. 265–275. [Google Scholar]
- Yang, Y.; Liu, S.; Chang, X. Fracture stiffness evaluation with waterless cryogenic treatment and its implication in fluid flowability of treated coals. Int. J. Rock Mech. Min. Sci. 2021, 142, 104727. [Google Scholar] [CrossRef]
- Zhang, Z.; Mao, J.; Yang, X.; Zhao, J.; Smith, G.S. Advances in waterless fracturing technologies for unconventional reservoirs. Energy Sources, Part A Recover. Util. Environ. Eff. 2019, 41, 237–251. [Google Scholar] [CrossRef]
- Fu, C.; Liu, N. Journal of Natural Gas Science and Engineering Waterless fluids in hydraulic fracturing—A review. J. Nat. Gas Sci. Eng. 2019, 67, 214–224. [Google Scholar] [CrossRef]
- Almubarak, M.; Almubarak, T.; Ng, J.H.; Hernandez, J.; Nasr-El-Din, H. Recent Advances in Waterless Fracturing Fluids: A Review. In Proceedings of the Abu Dhabi International Petroleum Exhibition & Conference, Abu Dhabi, United Arab Emirates, 9 November 2020. [Google Scholar]
- Gaurina-Međimurec, N.; Brkić, V.; Topolovec, M.; Mijić, P. Fracturing Fluids and Their Application in the Republic of Croatia. Appl. Sci. 2021, 11, 2807. [Google Scholar] [CrossRef]
- Deb, P.; Salimzadeh, S.; Vogler, D.; Düber, S.; Clauser, C.; Settgast, R.R. Verification of Coupled Hydraulic Fracturing Simulators Using Laboratory-Scale Experiments. Rock Mech. Rock Eng. 2021, 54, 2881–2902. [Google Scholar] [CrossRef]
- Cha, M.; Yin, X.; Kneafsey, T.; Johanson, B.; Alqahtani, N.; Miskimins, J.; Patterson, T.; Wu, Y.S. Cryogenic fracturing for reservoir stimulation–Laboratory studies. J. Pet. Sci. Eng. 2014, 124, 436–450. [Google Scholar] [CrossRef] [Green Version]
- Yang, R.; Hong, C.; Huang, Z.; Wen, H.; Li, X.; Huang, P.; Liu, W.; Chen, J. Liquid Nitrogen Fracturing in Boreholes under True Triaxial Stresses: Laboratory Investigation on Fractures Initiation and Morphology. SPE J. 2021, 26, 135–154. [Google Scholar] [CrossRef]
- Tao, J.; Meng, S.; Jin, X.; Xu, J.; Yang, Q.; Wang, X.; Liu, H.; Peng, B. Stimulation and Sequestration Mechanism of CO2 Waterless Fracturing for Continental Tight Oil Reservoirs. ACS Omega 2021, 6, 20758–20767. [Google Scholar] [CrossRef]
- Wang, L.; Yao, B.; Cha, M.; Alqahtani, N.B.; Patterson, T.W.; Kneafsey, T.J.; Miskimins, J.L.; Yin, X.; Wu, Y.-S. Waterless fracturing technologies for unconventional reservoirs-opportunities for liquid nitrogen. J. Nat. Gas Sci. Eng. 2016, 35, 160–174. [Google Scholar] [CrossRef] [Green Version]
- Kalam, S.; Afagwu, C.; Al Jaberi, J.; Siddig, O.M.; Tariq, Z.; Mahmoud, M.; Abdulraheem, A. A review on non-aqueous fracturing techniques in unconventional reservoirs. J. Nat. Gas Sci. Eng. 2021, 95, 104223. [Google Scholar] [CrossRef]
- Yang, J.; Lian, H.; Li, L. Fracturing in coals with different fluids: An experimental comparison between water, liquid CO2, and supercritical CO2. Sci. Rep. 2020, 10, 18681. [Google Scholar] [CrossRef]
- Abbas, M.; Manouchehr, H.; Dane, K. An experimental investigation into surface roughness of a hydraulic fracture. J. Aust. Pet. Prod. Explor. Assoc. 2018, 58, 728–732. [Google Scholar]
- Bobrova, M.; Stanchits, S.; Shevtsova, A.; Filev, E.; Stukachev, V.; Shayahmetov, T. Laboratory Investigation of Hydraulic Fracture Behavior of Unconventional Reservoir Rocks. Geosciences 2021, 11, 292. [Google Scholar] [CrossRef]
- Daeffler, C.; Perroni, D.; Makarychev-Mikhailov, S.; Mirakyan, A. Internal Viscoelastic Surfactant Breakers from In-Situ Oligomerization. In Proceedings of the SPE International Conference on Oilfield Chemistry, Galveston, TX, USA, 8 April 2019. [Google Scholar]
- Durairaj, R. Rheology-New Concepts, Applications and Methods; InTech: London, UK, 2013; ISBN 978-953-51-0953-2. [Google Scholar]
- Al-Muntasheri, G.A. A Critical Review of Hydraulic Fracturing Fluids over the Last Decade. In Proceedings of the SPE Western North American and Rocky Mountain Joint Meeting, Denver, CO, USA, 17 April 2014. [Google Scholar]
- Feng, Y.; Zhaolong, G.; Jinlong, Z.; Zhiyu, T. Viscoelastic surfactant fracturing fluid for underground hydraulic fracturing in soft coal seams. J. Pet. Sci. Eng. 2018, 169, 646–653. [Google Scholar] [CrossRef]
- Lu, Y.; Yang, F.; Ge, Z.; Wang, Q.; Wang, S. Influence of viscoelastic surfactant fracturing fluid on permeability of coal seams. Fuel 2017, 194, 1–6. [Google Scholar] [CrossRef]
- Chieng, Z.H.; Mohyaldinn, M.E.; Hassan, A.M.; Bruining, H. Experimental Investigation and Performance Evaluation of Modified Viscoelastic Surfactant (VES) as a New Thickening Fracturing Fluid. Polymers 2020, 12, 1470. [Google Scholar] [CrossRef]
- Yang, C.; Hu, Z.; Song, Z.; Bai, J.; Zhang, Y.; Luo, J.Q.; Du, Y.; Jiang, Q. Self-assembly properties of ultra-long-chain gemini surfactant with high performance in a fracturing fluid application. J. Appl. Polym. Sci. 2017, 134, 44602. [Google Scholar] [CrossRef]
- Yang, X.; Mao, J.; Zhang, Z.; Zhang, H.; Yang, B.; Zhao, J. Rheology of Quaternary Ammonium Gemini Surfactant Solutions: Effects of Surfactant Concentration and Counterions. J. Surfactants Deterg. 2018, 21, 467–474. [Google Scholar] [CrossRef]
- Yang, Z.; Xu, Y.; Wang, X.; Duan, Y.; Che, M.; Lu, Y. Study and Application of Novel Cellulose Fracturing Fluid in Ordos Basin. In IOP Conference Series: Earth and Environmental Science; IOP Publishing: Bristol, UK, 2018; Volume 170, p. 22145. [Google Scholar]
- Li, J.; Tellakula, R.; Rosencrance, S. Cross-Linked Acrylamide Polymer or Copolymer Gel and Breaker Compositions and Methods of Use. International Patent WO2015103203A1, 9 July 2015. [Google Scholar]
- Li, Y.; Wang, S.; Guo, J.; Gou, X.; Jiang, Z.; Pan, B. Reduced adsorption of polyacrylamide-based fracturing fluid on shale rock using urea. Energy Sci. Eng. 2018, 6, 749–759. [Google Scholar] [CrossRef]
- Horton, R.L.; Land, S. Oil-Based Hydraulic Fracturing Fluids and Breakers and Methods of Preparation and Use. U.S. Patent 2010/0252259 A1, 7 October 2010. [Google Scholar]
- Goodfellow, S.D.; Nasseri, M.H.B.; Maxwell, S.C.; Young, R.P. Hydraulic fracture energy budget: Insights from the laboratory. Geophys. Res. Lett. 2015, 42, 3179–3187. [Google Scholar] [CrossRef]
- Hu, L.; Ghassemi, A.; Riley, S.; Kahn, D. Lab-Scale Experiments Investigating the Possibility of Bedding Plane Slip Events During Microseismic Monitoring of Hydraulic Fracturing. In Proceedings of the 53rd US Rock Mechanics/Geomechanics Symposium, New York, NY, USA, 23 June 2019; p. ARMA-2019-1870. [Google Scholar]
- Stanchits, S.; Surdi, A.; Edelman, E.; Suarez-Rivera, R. Acoustic Emission And Ultrasonic Transmission Monitoring of Hydraulic Fracture Propagation In Heterogeneous Rock Samples. In Proceedings of the 46th U.S. Rock Mechanics/Geomechanics Symposium, Chicago, IL, USA, 24 June 2012; p. ARMA-2012-527. [Google Scholar]
- Rivas, E.; Gracie, R. A monolithic coupled hydraulic fracture model with proppant transport. Comput. Methods Appl. Mech. Eng. 2020, 372, 113361. [Google Scholar] [CrossRef]
- Stanchits, S.; Burghardt, J.; Surdi, A. Hydraulic Fracturing of Heterogeneous Rock Monitored by Acoustic Emission. Rock Mech. Rock Eng. 2015, 48, 2513–2527. [Google Scholar] [CrossRef]
- Yin, Q.; Liu, R.; Jing, H.; Su, H.; Yu, L.; He, L. Experimental Study of Nonlinear Flow Behaviors through Fractured Rock Samples after High-Temperature Exposure. Rock Mech. Rock Eng. 2019, 52, 2963–2983. [Google Scholar] [CrossRef]
- Abe, S.; Deckert, H. Roughness of fracture surfaces in numerical models and laboratory experiments. Solid Earth 2021, 12, 2407–2424. [Google Scholar] [CrossRef]
- Cheng, C.; Milsch, H. Hydromechanical Investigations on the Self-propping Potential of Fractures in Tight Sandstones. Rock Mech. Rock Eng. 2021, 54, 5407–5432. [Google Scholar] [CrossRef]
- Chen, Y.; Nagaya, Y.; Ishida, T. Observations of Fractures Induced by Hydraulic Fracturing in Anisotropic Granite. Rock Mech. Rock Eng. 2015, 48, 1455–1461. [Google Scholar] [CrossRef]
- Jia, Y.; Lu, Z.; Liu, H.; Wang, J.; Cheng, Y.; Zhang, X. Fracture Propagation and Morphology Due to Non-Aqueous Fracturing: Competing Roles between Fluid Characteristics and In Situ Stress State. Minerals 2020, 10, 428. [Google Scholar] [CrossRef]









| Average Porosity, % | Average Effective N2Permeability, nD | Young’s Modulus E, GPa | Poisson’s Ratio | Tensile Strength, MPa | Comprehensive Strength, MPa | Cohesion, MPa | Friction Angle, Grad° |
|---|---|---|---|---|---|---|---|
| 1.22 | 656.4 | 68.79 | 0.22 | 7.2 | 364.20 | 28 | 44.2 |
| Sample Id | Diameter, mm | Length, mm | Weight, g | Density, g/cm3 |
|---|---|---|---|---|
| WG-716 | 99.31 | 108.24 | 2288.44 | 2.73 |
| WG-717 | 99.26 | 108.88 | 2299.70 | 2.73 |
| WG-721 | 99.43 | 109.10 | 2304.35 | 2.72 |
| WG-722 | 99.61 | 110.84 | 2355.62 | 2.73 |
| PAA Suspension (Gelling Agent PolyGel), L/m3 | Ampholytic Surfactant (NEFTENOL-VES), L/m3 | Structuring Reagent (R s−1), L/m3 | Clay Stabilizer, L/m3 | Demulsifier, L/m3 | Destructor, kg/m3 | Sample Id | |
|---|---|---|---|---|---|---|---|
| Liquid based on synthetic polymer | 8.00 | - | - | 2.00 | 2.00 | 0.50 | WG-717 |
| Non-polymer composition (VES) | - | 70.00 | 18.00 | - | - | - | WG-716 |
| Himeko N Gelling Agent, L/m3 | Himeko N Activator, L/m3 | Destructor, kg/m3 | Sample Id | |
|---|---|---|---|---|
| Low viscous OilGel 7/7 | 7.00 | 7.00 | - | WG-722 |
| High viscous OilGel 40/40 | 40.00 | 40.00 | - | WG-721 |
| Sample Id | WG-716 | WG-717 | WG-721 | WG-722 |
|---|---|---|---|---|
| Type of fluid | Non-Newtonian | |||
| Base of agent/fluid | Water-based | Oil-based | ||
| Fracturing fluid | Non-polymer composition (VES) | PolyGel | OilGel 40/40 | OilGel 7/7 |
| Injection rate, mL/min | 5 | |||
| Sigma 1, MPa | 37 | 22 | ||
| Sigma 3, MPa | 23 | 8 | ||
| Fluid viscosity (100 s−1), cP | 81 | 95 | 1220 | 102 |
| Breakdown pressure, MPa | 57.30 | 71.70 | 39.90 | 39.23 |
| Fracture aperture, μm | 0.15 | 2.2 | 4 | 0.9 |
| Fluid volume into the fracture, ml | ~0 | 0.017 | 0.026 | 0.01 |
| HF propagation velocity, mm/s | 100 | 40 | 15 | 20 |
| Time of the fluid filled the fracture before breakdown, s | - | - | 1 | 0.6 |
| Time interval from the AE initiation till the breakdown, s | 0.15 | 1.15 | 3.50 | 1.50 |
| Parameter | WG-722 | WG-716 | WG-717 | WG-721 | |
|---|---|---|---|---|---|
| mm | 2.29 | 1.94 | 2.21 | 1.92 | |
| mm | 0.62 | 0.55 | 0.61 | 0.65 | |
| mm | 0.76 | 0.67 | 0.75 | 0.74 | |
| Tortuosity | - | 1.47 | 1.41 | 1.43 | 1.33 |
| Fluid viscosity (300 s−1) | cP | 35.58 | 49.75 | 55.19 | 310.81 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shevtsova, A.; Stanchits, S.; Bobrova, M.; Filev, E.; Borodin, S.; Stukachev, V.; Magadova, L. Laboratory Study of the Influence of Fluid Rheology on the Characteristics of Created Hydraulic Fracture. Energies 2022, 15, 3858. https://doi.org/10.3390/en15113858
Shevtsova A, Stanchits S, Bobrova M, Filev E, Borodin S, Stukachev V, Magadova L. Laboratory Study of the Influence of Fluid Rheology on the Characteristics of Created Hydraulic Fracture. Energies. 2022; 15(11):3858. https://doi.org/10.3390/en15113858
Chicago/Turabian StyleShevtsova, Anna, Sergey Stanchits, Maria Bobrova, Egor Filev, Sergey Borodin, Vladimir Stukachev, and Lyubov Magadova. 2022. "Laboratory Study of the Influence of Fluid Rheology on the Characteristics of Created Hydraulic Fracture" Energies 15, no. 11: 3858. https://doi.org/10.3390/en15113858
APA StyleShevtsova, A., Stanchits, S., Bobrova, M., Filev, E., Borodin, S., Stukachev, V., & Magadova, L. (2022). Laboratory Study of the Influence of Fluid Rheology on the Characteristics of Created Hydraulic Fracture. Energies, 15(11), 3858. https://doi.org/10.3390/en15113858







