1. Introduction and Background
To achieve greenhouse gas emission targets that many countries have set in the last years [
1], a significant increase in the penetration of Renewable Energy Sources (RES) in the power grid is required. However, the growth in non-programmable resources such as solar photovoltaics and wind [
2,
3] hinders the reliability and stability of the electric grid [
4] because (i) their discontinuous and uncertain generation causes unforeseeable oscillations in the net load and (ii) their modularity favors the diffusion of distributed generators, connected to the distribution grid and close to the demand. Thus, energy flows become unpredictable both in quantity and direction.
To allow the deployment of RES to grow without affecting the grid reliability, it is necessary to rethink the design, operation, and planning of the power system, both on a technical and on an economic level. On the technical side, it is necessary to increase the system flexibility with fast-ramping dispatchable plants, energy storage systems, demand-side management, and interconnections to the adjacent power grids for trading [
5,
6,
7,
8]. On the economic side, the regulatory framework must be adjusted to allow new services and business models. New rules for the electricity market have been set in the European Union with the Clean Energy for All European package [
9], which aims at helping the energy markets to better manage the energy flows across Europe, to empower the consumers, and to include more renewables. With these rules, the prosumers (both producers and consumers) can vary their consumption and production based on price changes, thus participating in the energy market and contributing to grid efficiency and integration of RES.
At the same time, in this scenario characterized by stringent greenhouse gas reduction targets, the development of a hydrogen economy has gained renewed interest. Indeed, the key role that hydrogen can play in fostering the clean energy transition has been recognized at an international level by the Hydrogen Council, gathering a number of industrial entities [
10], as well as by the International Energy Agency [
11]. On the one hand, the deployment of hydrogen technologies has the potential to increase the penetration of renewables in the power system, allowing demand–supply balancing and avoiding renewable energy curtailment. On the other hand, hydrogen technologies have sector coupling capability, and clean hydrogen can be used to decarbonize either the industrial sector, the transport sector, or the heating and cooling sector. For instance, hydrogen is used in refinery and for the production of clean fuels (e.g., ammonia and methanol).
At the European level, the European Green Deal [
12] includes the European hydrogen strategy, aiming at making clean hydrogen a viable solution to decarbonize the different energy sectors over time across Europe, enabling the European Union to play a global pioneering role in this field. The priority is the development of renewable hydrogen, although in the short-term, other forms of low-carbon hydrogen are necessary to support the creation of a profitable market. Another important aspect is that the targets set by the European hydrogen strategy (e.g., 24% of hydrogen in its energy mix by 2050) also require the import of renewable electricity or of green hydrogen from overseas. Thus, the European hydrogen strategy promotes the deployment of renewable energy sources in developing countries, and especially in the countries of the African Union, bridging the energy transition between advanced and developing countries [
13].
In this framework, this work identifies MW-scale Fuel Cell (FC) power plants based on low temperature Proton Exchange Membrane (PEM) cells as a source of flexibility for the future high-RES penetration electric grid, providing grid ancillary services thanks to their fast ramp rate and the load-following capability. The potential of this technology is being investigated within the EU H2020 project GRASSHOPPER, whose main features are described in
Section 2. This work focuses on the development of a dynamic numerical model of a flexible PEM FC power plant, based on the layout of the 100-kW pilot unit built within the GRASSHOPPER project. The purpose is to develop an instrument to simulate the system operation, aimed at maximizing the system efficiency and flexibility while limiting the degradation rate.
The topic of dynamic modeling of fuel cell systems has been already addressed in the literature, where the case of stationary fuel cell power plants has been discussed, mostly focusing on the case of high temperature fuel cells, in particular solid oxide fuel cells for stand-alone and hybrid configurations, including combined heat and power applications [
14,
15,
16]. Their off-design and transient behavior is investigated by the point of view of their safe and reliable operation as well as performance improvement, degradation minimization, and control improvement [
17,
18,
19,
20]. On the other hand, PEM-based systems have been mostly addressed for mobile applications [
21,
22,
23,
24], and in few cases for stationary use [
25].
This work instead focuses on low temperature systems for stationary use, with the particular target of discussing fast-ramping applications for grid support. A detailed description of the model is presented in
Section 3, while in
Section 4, the model is tested against experimental data and subsequently applied to simulate plant load-following operation and cold start up.
2. GRASSHOPPER Project
The demonstration of the feasibility of stationary large-scale FC power plants, using PEM cells, has been accomplished in many projects. For example, ref. [
26] describes a 70-kW
el PEM FC power plant that uses by-product hydrogen from the electrolysis of brine. The pilot plant performance is analyzed over 30,000 h of operation, showing cell irreversible and reversible decay. Ref. [
27] shows that the operation of a 50-kW
el PEM FC plant over 4400 h, with relatively low-quality hydrogen from a sodium chlorate production process with standard industry purification, does not lead to extensive cell degradation. The feasibility of MW-scale PEM FC power plant has been demonstrated in [
28,
29], which tested a 2-MW
e plant with by-product hydrogen from a chlor-alkali process. The flexibility of PEM FC stacks is also well demonstrated since PEM FCs are used in the automotive sector. However, the flexibility of stationary FC power plant has never been tested.
The GRASSHOPPER project studies how to use FC power plants, adopting low temperature PEM cells to provide balancing services to the power grid. The aim is to demonstrate MW-scale plant capability to operate dynamically, to realize the next-generation cost-effective FC power plant for stationary application and grid stabilization. The target CAPEX for a production of 25 MWel per year is below 1500 €/kWel, reached through joint design of MEA (membrane electrode assembly), stack, and system.
The project has designed a 100 kWel pilot unit, whose construction was finalized in September 2021. The plant successfully completed factory acceptance tests and is to be demonstrated in the field in Delfzij (NL), with 8 months of continuous operation.
Experience from the 100-kWel pilot plant is the basis for the design of a MW-scale unit. To support the scale-up, important outcomes are also derived from numerical simulations. The work presented in this paper falls within this scope.
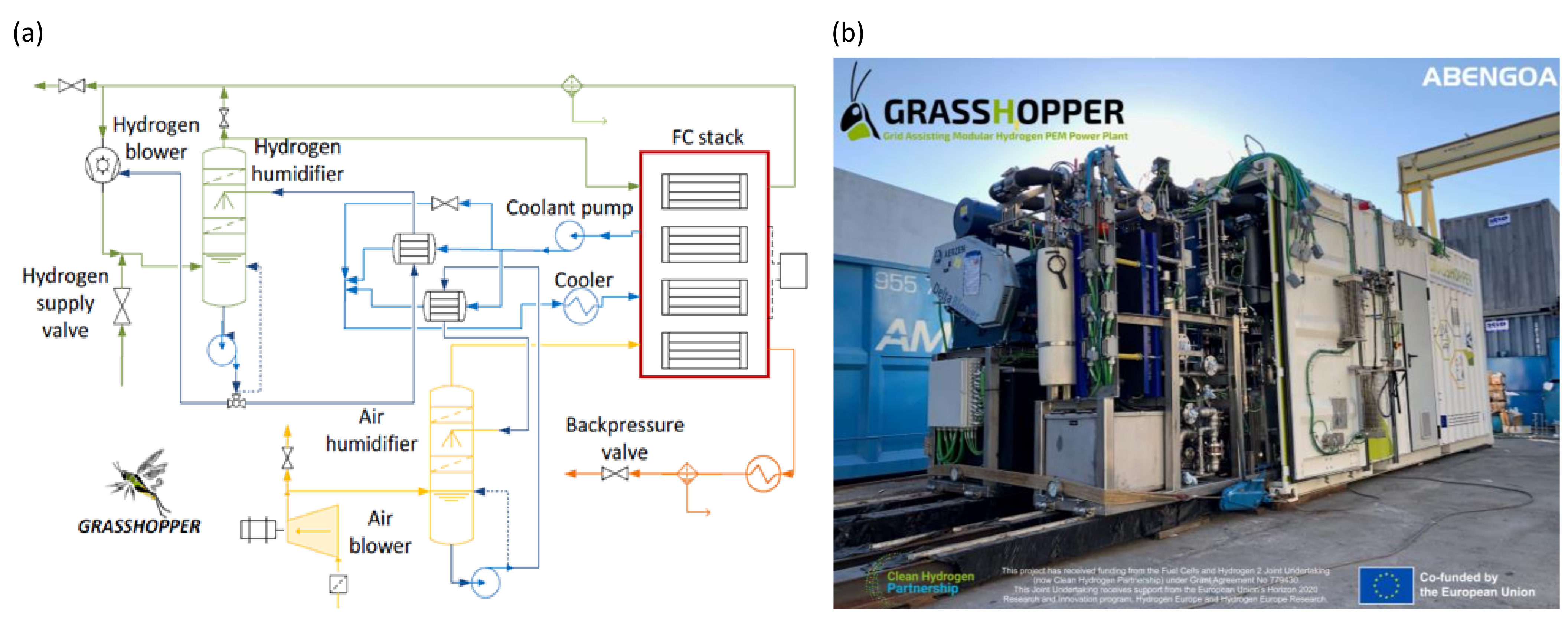
100 kW Pilot Plant Layout
The layout of the GRASSHOPPER 100 kW
el plant is shown in
Figure 1a, while
Figure 1b shows the real plant configuration.
Filtered air and pure hydrogen are humidified and supplied to the stack. The operating temperature of the stack is maintained at the desired value by the coolant, flowing in a dedicated loop. Heat exchangers allow the recovery of the stack dissipated thermal power for air and hydrogen humidification. The excess heat is dissipated in a dedicated cooler. The cathode backpressure is controlled with a valve, while the regulation of the rotational speed of the air blower and a purge valve allow the control of the ‘air stoichiometry’ (i.e., the ratio of air flowrate with respect to the stoichiometric flow). Fresh hydrogen is injected into the system through a controlled valve (hydrogen it is available above 4 bar). A liquid ring compressor recirculates the excess hydrogen and the ‘hydrogen stoichiometry’ (i.e., the ratio of hydrogen flowrate with respect to the stoichiometric flowrate) is controlled with a stack bypass.
The system has been designed to work flexibly between 20% (200 mA/cm2) and 100% (1000 mA/cm2) of the nominal current density value. Its performance is optimized and stack degradation is limited thanks to a precise control system.
3. Methods for Dynamic Modeling
This work implements, with the software Simulink, a dynamic model of a PEM FC plant, referring to the Grasshopper 100 kWel plant configuration. Models that solve energy and mass balances during operation with a variable load are built for each plant component, as described in the following paragraphs, reporting also the assumptions for model scale-up. The models of the components are combined to build the model of the entire system. To allow control of the system operation, PI-type and on-off controllers are then implemented.
3.1. Thermodynamic Properties
The model is based on the hypothesis of ideal gas, ideal gas mixture, and ideal liquid. For water and coolant, enthalpy is computed, assuming constant specific heat capacity (Equation (1)), equal to 4.186 kJ/kg/K for water and to 3.52 kJ/kg/K for the water–glycol mixture.
For the gas, enthalpy is computed with NASA polynomial equations [
30] (Equation (2)), where
–
are numerical coefficients available on NASA thermodynamic libraries for 1130 solid, liquid, and gaseous pure chemical species.
When a gaseous mixture of different species is present (e.g., moist air/hydrogen), its enthalpy is computed from the enthalpy (
) and the molar fraction (
) of each species in the mixture, assuming the ideal gas mixture behavior (Equation (3)). For liquid water, the density is assumed to be constant and enthalpy variation with pressure is taken into account.
Water in the liquid phase and in the vapor phase is treaded as two different species. When the partial pressure of the water in the mixture is below its saturation pressure, the water is in the vapor phase. Otherwise, it is partially in the condensate liquid phase. The maximum molar fraction of water in the vapor phase coincides with the water molar fraction at saturation. Saturation pressure of water is computed as a function of the temperature, according to a polynomial equation (Equations (4) and (5)) derived in [
31] and valid for temperature values ranging from water triple point (
= 273.16 K) to the critical point (
647.096 K,
= 220.64 bar).
3.2. PEM FC Stack
A single cell is modeled with a lumped-volume approach, considering performance data acquired from detailed cell simulations, thus allowing to simulate large scale effects. Given the stack modularity, the FC stack model is then built, assuming that many identical cells are electrically connected in series.
The model is divided into three subsystems: electrochemical model, fluid dynamic model, and thermodynamic model.
Regarding the electrochemical model, FC voltage and gross electrical power are calculated with semi-empirical current-voltage curves, combining cell theoretical polarization curve equations and experimental datasets, as detailed in [
32]. The resulting formulation is shown in Equation (6), where the first term represents an apparent open circuit voltage, the second term the ohmic losses, and the third and the last terms the activation and the concentration overvoltage, respectively. The equation takes into account voltage dependence on backpressure, air stoichiometry, and air relative humidity. It catches the real cells performance with a good approximation, mainly for the range of current density where the cell will generally operate (relative errors <1% for relevant currents values: 200–1000 mA/cm
2). A detailed description of the terms appearing in Equation (6) is presented in [
32], together with the coefficients calibration procedure.
In order to simulate cold start-up, semi-empirical corrections have been considered to model the effects of the cell temperature on ohmic losses and activation losses. Temperature effects on the concentration losses are not modeled since they impact on the cell performance only with high current density, outside the range of operability of the cells. The ohmic resistance
varies exponentially with the temperature [
33], according to Equation (7), while the activation losses vary because of the exponential variation with the temperature of the exchange current density (
) [
33], according to Equation (8).
The values of the coefficients
and
are determined by comparing the regressed polarization curves with an experimental dataset obtained from a similar FC stack, characterized by temperatures ranging between 15 °C and 62 °C, constant stoichiometry, relative humidity, and backpressure. The coefficients are chosen such that the gradient with the temperature for the ohmic resistance and the open circuit overvoltage are equal to the ones measured in the reference database. The value of temperature adopted in the equations is the average temperature of the coolant flowing over the stack, assumed as representative of the stack temperature. The reference temperature is equal to 65 °C. Polarization curves obtained with this model for different values of temperature and air ratio to stoichiometry are shown in
Figure 2, where backpressure is 1.35 bar and the average air relative humidity is 100% (i.e.,
/
= 1). The numerical values of the regressed coefficients are summarized in
Table 1.
The electrochemical model computes the stack voltage and the gross DC electric power respectively with Equations (9) and (10), where
is the number of cells composing the stack and
is the active area of each cell.
In regard to the cell dynamic behavior, the charge and discharge of the cell double layer is the main phenomenon affecting the cell voltage when the load is varied [
34,
35,
36,
37]. It can be modeled though a capacitance, whose value varies between 0.01 and 0.05 F/cm
2 according to literature [
35]. Since these phenomena are much faster than other dynamic effects, such as the ones associated with the thermal inertia, the double layer capacitance is not included in this model.
Regarding the fluid dynamic model, it solves mass conservation equations over the FC stack for each chemical species (H
2, O
2, N
2, and H
2O) flowing through the anodic and the cathodic channels, to determine flowrate and composition of the anodic and cathodic streams leaving the stacks. Hydrogen and oxygen consumptions and water production are computed as a function of the stack current density, knowing the reaction in the stacks, according to Equations (11)–(13).
Additionally, according to experimental evidence, the model considers a net transport of H2O from cathode to anode equal to 0.1 mol/h, a net diffusion of H2 from anode to cathode corresponding to an internal current density equal to 0.002 A/cm2, a net transport of N2 form cathode to anode such that the mass fraction of N2 in the outlet anodic stream is 10%, and a null net transport of O2 from cathode to anode or vice versa.
The accumulation of gasses in the cell channels is not included in the model because the channel volume is negligible with respect to the other component volumes.
Pressure drops in the channels are linearly dependent on the volumetric flowrate of coolant and of reactants at the stack inlet, according to prior experimental stack tests and justified by the low velocities in the flow field, which leads to a laminar regime.
Regarding the thermodynamic model, it solves energy balances on the stack (Equation (14)) to determine the average temperature of the cell and the temperature of the flows leaving the FC stack. Temperature dynamic (Equation (15)) is taken into account through the heat capacity of the stack, which is lumped in the Bipolar Plates (BP). The gross DC electric power (
) is an output of the electrochemical subsystem. Heat transfer from the reactants to the bipolar plants (
) and to the coolant fluid (
) is computed, assuming constant heat transfer coefficients. No heat losses to the environment are assumed.
The system can be scaled up by increasing the number of cells in each stack or by installing more stacks of the same size. In the first case, more cells are connected in series, resulting in higher voltage and requiring and increase in the flowrate of hydrogen and air supplied to each stack. The resulting wider voltage range is not compatible with commercially available Power Control Systems. In the second case, which is applied in this work, a greater number of stacks is electrically connected in parallel, requiring more converters or a converter able to work with a higher current, although each stack remains identical in geometry and operation.
3.3. Air Blower
The air blower model follows a lumped-parameter approach. It includes dynamic effects related to the mechanical inertia and thermal inertia, while it neglects mass accumulation.
The unit stationary performances are based on the machine performance maps, available on the commercial datasheet and implemented through polynomial functions. The maps give the volumetric flowrate of air processed by the machine, its temperature gain at thermal equilibrium, and the blower mechanical power, as a function of air inlet temperature and pressure (ambient conditions), air outlet pressure (determined by the pressure drops in the downstream components), and the blower rotational speed. Isentropic efficiency and mechanical efficiency are computed.
The blower rotational speed (ω
c [rad/s]) is computed according to Equation (16), representing a balance between the torque generated by the electric motor (
, defined in Equation (17)) and the torque required by the blower (
, defined in Equation (18)), where
is compressor-motor block inertia [
38],
is the electrical power supplied to the motor (external input),
is the motor electrical efficiency, and
is the blower mechanical power.
The blower thermal inertia was initially not included in the model, but experimental data showed the importance of the temperature dynamics: the temperature gain of the air flowing through the blower matches the performance maps value in stationary conditions, but a smaller gain is shown during warm-up.
In general, the thermal power generated in the compression phase (
is in part responsible for the air temperature increase (
and in part transferred to the machine (
). This second part is in turn partially absorbed by the machine steel mass (
), and partially dissipated towards the environment (
) (Equation (19)).
At thermal equilibrium (
, the air temperature gain is known from the performance maps. This allows to compute the heat absorbed by the air (Equation (20)), the heat dissipated (Equation (21)), and therefore the generated heat. According to the lumped volume approach, the steel temperature is homogeneous and equal, at thermal equilibrium, to the average air temperature between inlet and outlet (Equation (22)).
During thermal transients, the generated heat (
is the same computed for steady state, and Equations (23)–(26) allow computing the air temperature at blower outlet (
).
The values of the heat transfer coefficients ( and the blower heat capacity (), and the mechanical inertia ( have been calibrated on experimental data.
The model scale-up procedure assumes:
Flowrate (
, isentropic efficiency (), and mechanical efficiency () curves derived from the regressed performance curves, assuming different sizes but the same efficiency profile for the blowers.
Blower heat capacity proportional to the blower mass (representing the case assembly and excluding the motor, whose mass is expected to increase less than linearly).
The blower surface in contact with the compressed air (named ‘Internal area’) and the blower surface in contact with the environment (named ‘external area’) are functions of the machine diameter. Indeed, by approximating the rotary lobe compressors as two identical cylinders (the lobes, with diameter DL and height L) contained in a larger cylinder (the case, with diameter DC and height L), and assuming that the diameter of the single lobe is equal to half the diameter of the case (DL = DC/2), the relations in Equations (27) and (28) are obtained. Additionally, neglecting the thickness of the case, the volume of the machine can be expressed as the sum of the volume of the lobes and the void volume, which is occupied by air (Equation (29)). Assuming that the void volume is proportional to the nominal volumetric air flowrate and that the ratio between the cylinders diameters remains the same for blowers of different sizes, the ratio between the diameters of the two machines of size A and B is expressed as a function of the ratio between the nominal volumetric air flowrates by Equation (30).
3.4. Humidifier
The humidifier is composed by two sections, the packed-bed column and the water tank. These two sections are modeled separately with a lumped-volume approach.
Regarding the packed-bed column, the gas (hydrogen or air) enters at the bottom and flows upwards, while the water is sprayed at the top and flows downwards. Thus, the humidity of the gas increases while flowing thorough the column because of water evaporation. The column model computes flowrate, composition, pressure, and temperature of the outlet flows by knowing the same properties at inlet. It is assumed, according to industrial experience, that the humidifier effectiveness is 100%: the outlet gas is fully saturated and in thermal equilibrium with the water spray. Additionally, a demister at the top of the column removes the water drops that may be present in the outlet gas. Temperature dynamic in the column is negligible with respect to the water tank and is therefore neglected by the model. For the air humidifier, although the void fraction of the column is the 90% of its total volume, the column model neglects air build-up (the volume of all the air supply line components is lumped in the air manifold). Conversely, for the hydrogen humidifier, the column model includes hydrogen build-up. Pressure drops of the gasses flowing through the humidifier are then taken as constant and set equal to the nominal values reported on the datasheet.
The water tank collects the water that is sprayed in the column. The water tank model includes water build-up and temperature dynamic (the thermal inertia is associated to the heat capacity of the stored water). Perfect mixing in the water tank is assumed. The inputs to the model are the flowrate of the outlet water stream, set by the recirculation pump, and the flowrate and temperature of the water coming from the packed bed column. In addition, a purge or a refill of water can be introduced to regulate the water level in the tank. With the given assumptions, the model computes the amount of water that is accumulated in the tank, its temperature and the temperature of the water that is pumped out.
The model of the humidifier is scaled up, assuming that the size of the water tank is proportional to the system nominal power. The pressure drops in the humidifier column are assumed constant.
3.5. Air Supply Manifold
The air supply manifold model allows to simulate the build-up of the air in the supply line. Indeed, the volume of all components in the supply line is lumped in the manifold, which is located between the air blower and the air humidifier.
The manifold model assumes uniform temperature in the manifold itself (equal to the temperature of the inlet air) and ideal gas behavior. It computes the time variation of the pressure in the air supply line as in Equation (31). The air blower sets the flowrate at manifold inlet (
), while the pressure drops in the components downstream set the air flowrate at the manifold outlet (
).
To scale up the model, the manifold volume is varied proportionally to the void volume in the air humidifier column, which most contributes to the volume of the air supply line.
3.6. Backpressure Valve
The backpressure valve is modeled as an orifice, through which a one-dimensional isentropic flow of a compressible fluid flows [
39,
40]. The orifice model reported for subcritical flow is adopted, since the ratio between valve inlet pressure (max 1.6 bar) and outlet pressure (ambient pressure) is always above the critical pressure ratio. According to Equation (32), the flowrate of air through the valve is computed knowing the upstream pressure and temperature, the pressure ratio across the valve, and the valve effective area (
.
To scale-up the model, the valve effective area is varied proportionally to the nominal air flowrate.
3.7. Liquid Ring Compressor
The liquid ring compressor is modeled through a stationary model because its rotational speed can vary only in a narrow range, making dynamic effects negligible. Two sequential steps are considered: hydrogen compression and hydrogen-water mixing.
In the first step, the hydrogen stream coming from the FC anode and from the hydrogen humidifier (if hydrogen recirculation over the humidifier is required to guarantee the minimum hydrogen flow to the liquid ring compressor) is compressed. Knowing flowrate, composition, temperature, and pressure of the inlet moist hydrogen, as well as the outlet pressure that the stream has to reach (determined by the hydrogen humidifier located downstream), the model computes the temperature of the hydrogen flow after compression and the electrical power required for hydrogen compression, assuming constant isentropic efficiency () and mechanical efficiency (. The water contained in the inlet hydrogen streams is assumed to be in vapor phase.
In the second step, the compressed hydrogen flow is mixed with the water stream from the hydrogen compressor tank. The mixing process is modeled as an adiabatic process. The flowrate of the water is assumed to be constant since the pump is not controlled and works with a constant rotational speed. The inlet water stream is assumed to be in the liquid phase.
The liquid ring compressor model is scaled up, assuming constant isentropic and mechanical efficiencies and that the water flowrate required by the compressor is proportional to the nominal hydrogen flowrate.
3.8. Heat Exchanger
The heat exchangers are plate-type counter-current heat exchangers, working with liquid on both sides. Given the modular structure of the plates and assuming that the fluids equally distribute among the channels, the model considers a number of identical sub-units. Each of them includes a plate and half of the adjacent hot and cold channels. In each sub-unit, control volumes are then identified through a discretization along the channel direction (1D-model). The model solves mass and energy balances for each control volume, to compute the heat transferred, the plates temperature, and the outlet streams temperature. The model assumes that, in the control volume, the temperature of each plate is uniform, and it considers constant values for the heat transfer coefficients and the heat capacities. The temperature dynamic is associated to the heat capacity of the heat exchanger materials. Conduction along the direction of the flows, thermal resistance of the plate, heat losses toward the environment, and mass accumulation in the channels are neglected. Pressure drops are modeled, assuming the pressure will vary linearly with the volumetric flowrates.
The model is scaled up by increasing the number of plates, so that the total area of heat transfer varies proportionally to the system nominal power. The heat transfer coefficients and the pressure drops are assumed unchanged. The model is representative of a counter-current plate-type heat exchanger or of a more compact plate-and-shell heat exchanger.
3.9. Pumps
A stationary model of the pump is realized, since they are expected to work at constant rotational speed (such as in the air and hydrogen humidifier water circuit) or the dynamic effects are assumed negligible (as for the coolant circuit pump, whose rotational speed is varied to control the coolant temperature gain over the stack). The model computes the pump electrical consumption and the outlet flow pressure and temperature, assuming constant mechanical and isentropic efficiency and knowing flowrate, temperature, and pressure of the inlet flow, as well as the pressure gain that the pump has to provide.
When the model is scaled up, mechanical and isentropic efficiencies are assumed unchanged.
3.10. Pipelines
Pipelines are used to connect the plant components and are responsible of transport delays and pressure drops. These phenomena are included in the pipeline model. The transport delay depends on pipe diameter and length and on the volumetric flowrate, as in Equation (33). Pressure drops depend on the volumetric flowrate, as in Equation (34), where λ is assumed to be constant. The fluid is assumed to be incompressible (as already mentioned, hydrogen build-up is lumped in the hydrogen humidifier volume and air build-up in the air supply manifold volume).
The pipeline model scale-up is based on the assumption that bigger components need to be more distant from each other, therefore the length of the pipes connecting the components increases with the plant nominal power. Additionally, the scale-up assumes that the nominal pressure drops are the same for systems of different sizes. Thus, being the pressure drops calculated with the assumption of turbulent flowrate (Equation (34)), the relationship between the pipes diameters for the two systems of size A and B is given by Equation (35).
3.11. Power Conversion System (PCS)
The PCS includes the components required to connect the plant to the electric grid, namely a DC/DC converter and an inverter. The PCS is simulated through a constant conversion efficiency, which is set equal to 80%, as experimentally measured on the 100 kWel unit at low current density operation. The choice of these value is conservative since higher values are expected at higher currents, but an experimental performance curve is not available. Additionally, the DC/DC converter is not required for the MW size plant, where a higher stack voltage is expected.
3.12. Control Method
The control strategy is implemented in the model with PID controllers, when a precise control of the variable is required, and with on-off controllers, when it is sufficient to guarantee that the variable remains within a given range of values.
A PID controller is generally characterized by three different control actions: a proportional action, an integral action, a derivative action. In this system, the derivative part in not implemented and a PI configuration is used, characterized by the proportional gain
and the integral gain
(Equation (36)).
Each controller takes into account the physical characteristic of the system components, allowing the manipulated variable to vary only within a given range of values. To avoid wind-up, clamping of the integral is adopted: the integral in each PI controller is switched off ( = 0) when the manipulated variable reaches its minimum or maximum limit, allowing a faster response when the error changes its sign.
Table 2 details the controller implemented in the model.
3.13. System Performance Evaluation
The performances of the fuel cell system are evaluated through the stack gross power (defined by Equation (10)), the system net power (Equation (37)), the stack gross efficiency (Equation (38)), and the system net efficiency (Equation (39)). Stack gross efficiency and net efficiency are referred to the Low Heating Value (LHV) of the consumed hydrogen.
5. Conclusions
Future evolution of the electric grid in a low-CO2 emission and high renewable-based scenario will increasingly require the availability of clean and dispatchable energy resources. In this framework, hydrogen-based fuel cell systems could provide a modular, reliable, and zero-emission solution to the necessity of distributed grid support, providing grid ancillary services thanks to their fast ramp rate and the load-following capability. This work contributes to the analysis of the dynamic behavior of fuel cell power plants based on low temperature Proton Exchange Membrane cells, focusing on the development of a dynamic numerical model of a flexible PEM FC power plant based on the layout of the 100-kW pilot unit built within the EU Horizon 2020 GRASSHOPPER project. The purpose is to develop an instrument to simulate the system operation, aiming at maximizing the system efficiency and flexibility while limiting the degradation rate.
The dynamic model includes FC stack, all main plant components, and controllers. The comparison of simulation results with experimental data from a 100 kWel plant has shown that the model reproduces, with good accuracy, both the components stationary behavior at different load (e.g., stationary values of pressure, temperature, power consumptions, etc.) and the system dynamics (e.g., thermal transients, effects related to delays in mass transfer, etc.)
The validated model has been applied to assess the flexible performance of a 1-MWel unit, providing a suggestion to improve the plant scale-up.
Load-following operation simulations showed that the system is able to follow a load setpoint. When the current setpoint is changed stepwise, a precise backpressure control and a good temperature control are possible. Conversely, deviations of the air and fuel utilization factors from their setpoints affect air and fuel average relative humidity over the stack and suggest the adoption of more advanced controllers (e.g., smith predictors). Both stack gross efficiency and system net efficiency increase while the load decreases. The power conversion system, having an 80% efficiency, results in the main component limiting the system net efficiency, and an improvement of the plant net efficiency up to 10 percentage points is possible by acting on the PCS efficiency only. Indeed, a PCS efficiency higher than 80% is expected for the MW-scale system where, thanks to the higher number of cells and the consequently higher stack voltage, the DC/DC converter is not needed and only the inverter (with efficiency higher than 90%) can be installed.
Simulations of cold start-up show that, starting from 20 °C and limiting the current density at low temperature, the plant takes 2 h 38 min to reach the nominal point. The warm-up time decreases substantially if the stack is operated at maximum current (34 min), thanks to the higher thermal losses of the stack. However, operating at high current and low temperature may reduce the lifetime of the cells. Other solutions to reduce the warm-up time, without affecting the stack, consider a reduction of the water inventory. Halving the water content in the humidifier slightly reduces the warm-up time (−9%), while using pipes three times shorter to connect the plant components appears as the best solution (−70%). The design of a compact system should therefore be a priority in the perspective of building a flexible unit, able to rapidly start-up.
These suggestions have been taken into account in the design of the flexible 1-MW FC power plant, which is the target of the Grasshopper project. Thus, the commercial FC system design will combine the technical improvements deriving from the simulation results with improvements deriving from an economic analysis.