Optimised Adjoint Sensitivity Analysis Using Adjoint Guided Mesh Adaptivity Applied to Neutron Detector Response Calculations
Abstract
:1. Introduction
2. Optimised Adjoint Sensitivity Formulation for Neutron Transport Problems
2.1. Summary of First-Order Adjoint Sensitivity Analysis
2.2. Adjoint Error Metrics for Adaptive Mesh Refinement
2.3. Adaptive Mesh Refinement
3. Numerical Example—Maynard Problem
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Cacuci, D.G. Sensitivity and Uncertainty Analysis, Volume I: Theory; Chapman and Hall/CRC: London, UK, 2003. [Google Scholar]
- Cacuci, D.G.; Fang, R.; Favorite, J.A. Comprehensive Second-Order Adjoint Sensitivity Analysis Methodology (2nd-ASAM) Applied to a Subcritical Experimental Reactor Physics Benchmark. VI: Overall Impact of 1st- and 2nd-Order Sensitivities on Response Uncertainties. Energies 2020, 13, 1674. [Google Scholar] [CrossRef] [Green Version]
- Favorite, J.A.; Bledsoe, K.C. Eigenvalue sensitivity to system dimensions. Ann. Nucl. Energy 2010, 37, 522–528. [Google Scholar] [CrossRef]
- Cacuci, D.G. A Paradigm-Shifting Methodology for Sensitivity Analysis of Critical Multiplying Nuclear Systems. Nucl. Sci. Eng. 2017, 185, 361–383. [Google Scholar] [CrossRef]
- Evans, R.T.; Cacuci, D.G. A Parallel Krylov-Based Adjoint Sensitivity Analysis Procedure. Nucl. Sci. Eng. 2012, 172, 216–222. [Google Scholar] [CrossRef]
- Ionescu-Bujor, M.; Cacuci, D.G. Adjoint Sensitivity Analysis of the RELAP5/MOD3.2 Two-Fluid Thermal-Hydraulic Code System—II: Applications. Nucl. Sci. Eng. 2000, 136, 85–121. [Google Scholar] [CrossRef]
- Perkó, V.; Lathouwers, D.; Kloosterman, J.L.; Hagen, T.V. Adjoint-Based Sensitivity Analysis of Coupled Criticality Problems. Nucl. Sci. Eng. 2012, 173, 118–138. [Google Scholar]
- Cacuci, D.G. Application of the Second-Order Comprehensive Adjoint Sensitivity Analysis Methodology to Compute First- and Second-Order Sensitivities of Flux Functionals in a Multiplying System with Source. Nucl. Sci. Eng. 2019, 193, 555–600. [Google Scholar] [CrossRef] [Green Version]
- Cacuci, D.G.; Fang, R.; Favorite, J.A. Comprehensive Second-Order Adjoint Sensitivity Analysis Methodology (2nd-ASAM) Applied to a Subcritical Experimental Reactor Physics Benchmark: I. Effects of Imprecisely Known Microscopic Total and Capture Cross Section. Energies 2019, 12, 4219. [Google Scholar] [CrossRef] [Green Version]
- Cacuci, D.G. Towards Overcoming the Curse of Dimensionality: The Third-Order Adjoint Method for Sensitivity Analysis of Response-Coupled Linear Forward/Adjoint Systems, with Applications to Uncertainty Quantification and Predictive Modeling. Energies 2019, 12, 4216. [Google Scholar] [CrossRef] [Green Version]
- Aussourd, C. A Multidimensional AMR SN Scheme. Nucl. Sci. Eng. 2003, 143, 281–290. [Google Scholar]
- Ragusa, J.C.; Wang, Y. A two-mesh adaptive mesh refinement technique for SN neutral-particle transport using a higher-order DGFEM. Nucl. Sci. Eng. 2010, 233, 3178–3188. [Google Scholar]
- Baker, R.S. A Block Adaptive Mesh Refinement Algorithm for the Neutral Particle Transport Equation. Nucl. Sci. Eng. 2002, 141, 1–12. [Google Scholar] [CrossRef]
- Lathouwers, D. Spatially adaptive eigenvalue estimation for the SN equations on unstructured triangular meshes. Ann. Nucl. Energy 2011, 38, 1867–1876. [Google Scholar] [CrossRef]
- Goffin, M.A.; Baker, C.M.J.; Buchan, A.G.; Pain, C.C.; Eaton, M.D.; Smith, P.N. Minimising the error in eigenvalue calculations involving the Boltzmann transport equation using goal-based adaptivity on unstructured meshes. J. Comput. Phys. 2013, 242, 726–752. [Google Scholar] [CrossRef] [Green Version]
- Buchan, A.G.; Pain, C.C.; Eaton, M.D.; Smedley-Stevenson, R.P.; Goddard, A.J.H. Self Adaptive Spherical Wavelets for Angular Discretisation of the Boltzmann Transport Equation. Nucl. Sci. Eng. 2006, 158, 244–263. [Google Scholar] [CrossRef]
- Dargaville, S.; Goffin, M.A.; Buchan, A.G.; Pain, C.C.; Smedley-Stevenson, R.P.; Smith, P.N.; Gorman, G. Goal-based angular adaptivity for Boltzmann transport in the presence of ray-effects. J. Comput. Phys. 2020, 421, 109759. [Google Scholar] [CrossRef]
- Park, H.; Oliveira, C. Adaptive angular resolution for the finite element-spherical harmonics method. Trans. Am. Nucl. Soc. 2005, 93, 517–519. [Google Scholar]
- Dargaville, S.; Buchan, A.G.; Smedley-Stevenson, R.P.; Smith, P.N.; Pain, C.C. Angular adaptivity with spherical harmonics for Boltzmann transport. J. Comput. Phys. 2019, 397, 108846. [Google Scholar] [CrossRef] [Green Version]
- Sadeghi, K.H.; Abbasi, M.; Zolfaghari, A. Adaptive refinement for PN neutron transport equation based on conjoint variational formulation and discontinuous finite element method. Prog. Nucl. Energy 2018, 104, 16–31. [Google Scholar] [CrossRef]
- Hughes, A.C.; Buchan, A.G. A discontinuous and adaptive reduced order model for the angular discretization of the Boltzmann transport equation. Int. J. Numer. Methods Eng. 2020, 121, 5647–5666. [Google Scholar] [CrossRef]
- Kópházi, J.; Lathouwers, D. A space—Angle DGFEM approach for the Boltzmann radiation transport equation with local angular refinement. J. Comput. Phys. 2015, 297, 637–668. [Google Scholar] [CrossRef]
- Dargaville, S.; Goffin, M.A.; Buchan, A.G.; Pain, C.C.; Smedley-Stevenson, R.P.; Smith, P.N.; Gorman, G. Solving the Boltzmann transport equations with multigrid and adaptive space/angle discretisations. Ann. Nucl. Energy 2015, 86, 99–107. [Google Scholar] [CrossRef]
- Park, H.; de Oliveira, C.R.E. Coupled Space-Angle Adaptivity for Radiation Transport Calculations. Nucl. Sci. Eng. 2009, 161, 216–234. [Google Scholar] [CrossRef]
- Baker, C.M.J.; Buchan, A.G.; Pain, C.C.; Tollit, B.S.; Goffin, M.A.; Merton, S.R.; Warner, P. Goal based mesh adaptivity for fixed source radiation transport calculations. Ann. Nucl. Energy 2013, 55, 169–183. [Google Scholar] [CrossRef]
- Pain, C.C.; Umpleby, A.P.; de Oliveira, C.R.E.; Goddard, A.J.H. Tetrahedral mesh optimisation and adaptivity for steady-state and transient finite element calculations. Comput. Methods Appl. Mech. Eng. 2001, 190, 3771–3796. [Google Scholar] [CrossRef]
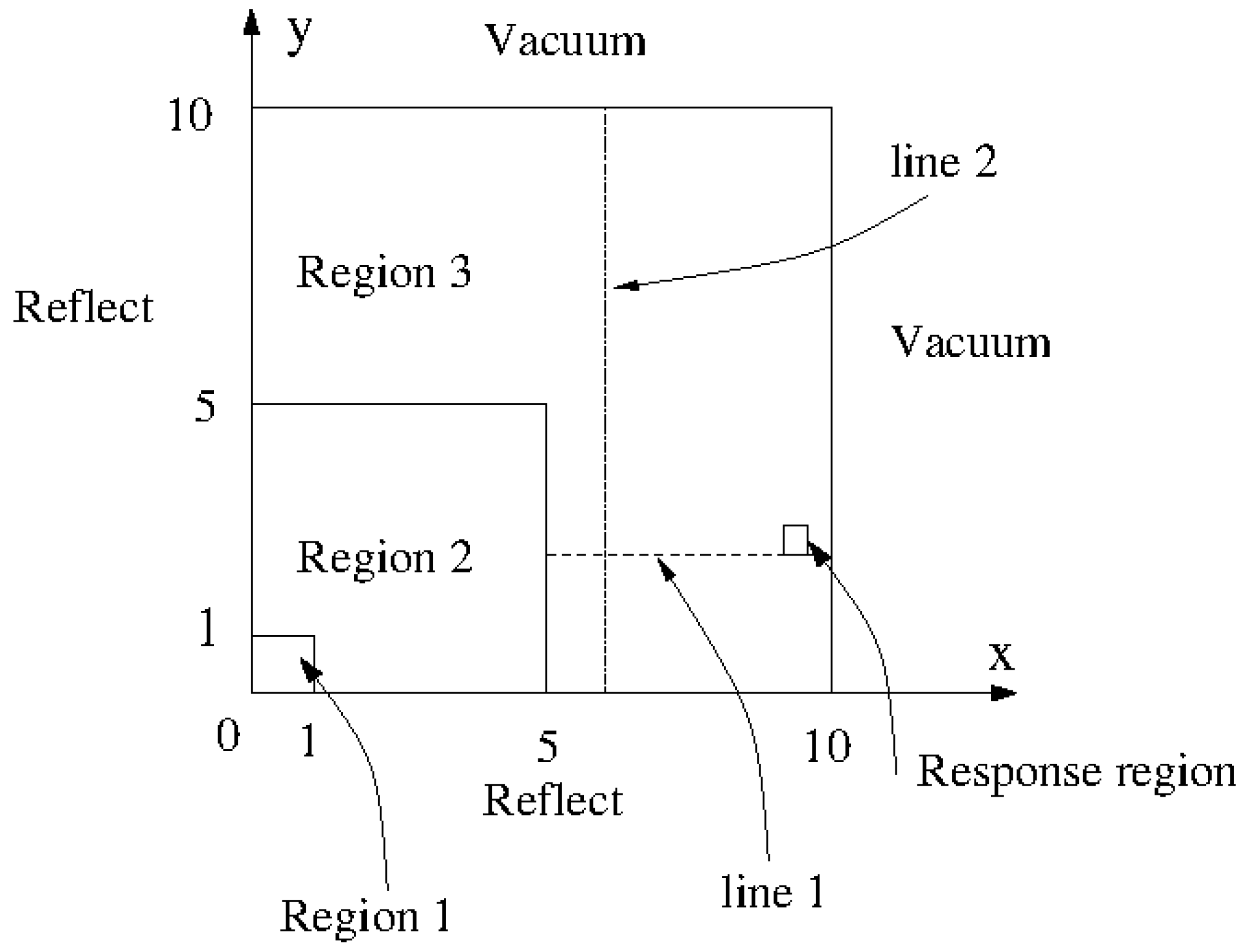


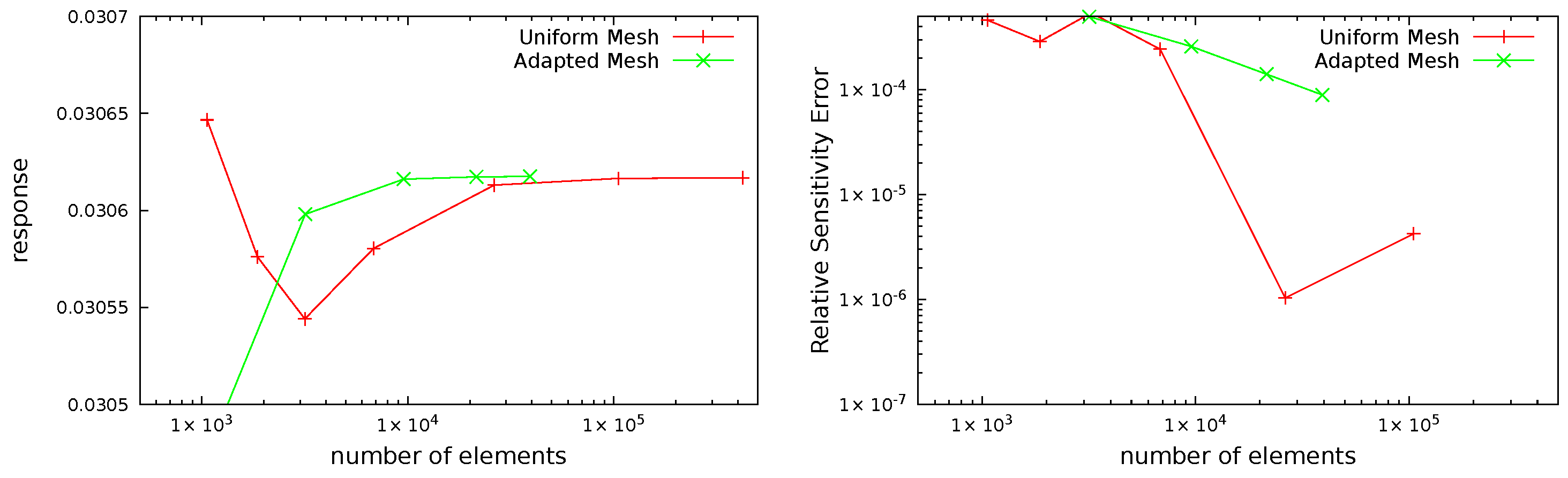


| Region | S | ||
|---|---|---|---|
| 1 | 0.2 | 0.19 | 1.0 |
| 2 | 0.0 | 0.0 | 0.0 |
| 3 | 0.2 | 0.19 | 0.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Buchan, A.G.; Cacuci, D.G.; Dargaville, S.; Pain, C.C. Optimised Adjoint Sensitivity Analysis Using Adjoint Guided Mesh Adaptivity Applied to Neutron Detector Response Calculations. Energies 2022, 15, 5102. https://doi.org/10.3390/en15145102
Buchan AG, Cacuci DG, Dargaville S, Pain CC. Optimised Adjoint Sensitivity Analysis Using Adjoint Guided Mesh Adaptivity Applied to Neutron Detector Response Calculations. Energies. 2022; 15(14):5102. https://doi.org/10.3390/en15145102
Chicago/Turabian StyleBuchan, Andrew G., Dan G. Cacuci, Steven Dargaville, and Christopher C. Pain. 2022. "Optimised Adjoint Sensitivity Analysis Using Adjoint Guided Mesh Adaptivity Applied to Neutron Detector Response Calculations" Energies 15, no. 14: 5102. https://doi.org/10.3390/en15145102
APA StyleBuchan, A. G., Cacuci, D. G., Dargaville, S., & Pain, C. C. (2022). Optimised Adjoint Sensitivity Analysis Using Adjoint Guided Mesh Adaptivity Applied to Neutron Detector Response Calculations. Energies, 15(14), 5102. https://doi.org/10.3390/en15145102









