Diagnosis and Location of Power Cable Faults Based on Characteristic Frequencies of Impedance Spectroscopy
Abstract
:1. Introduction
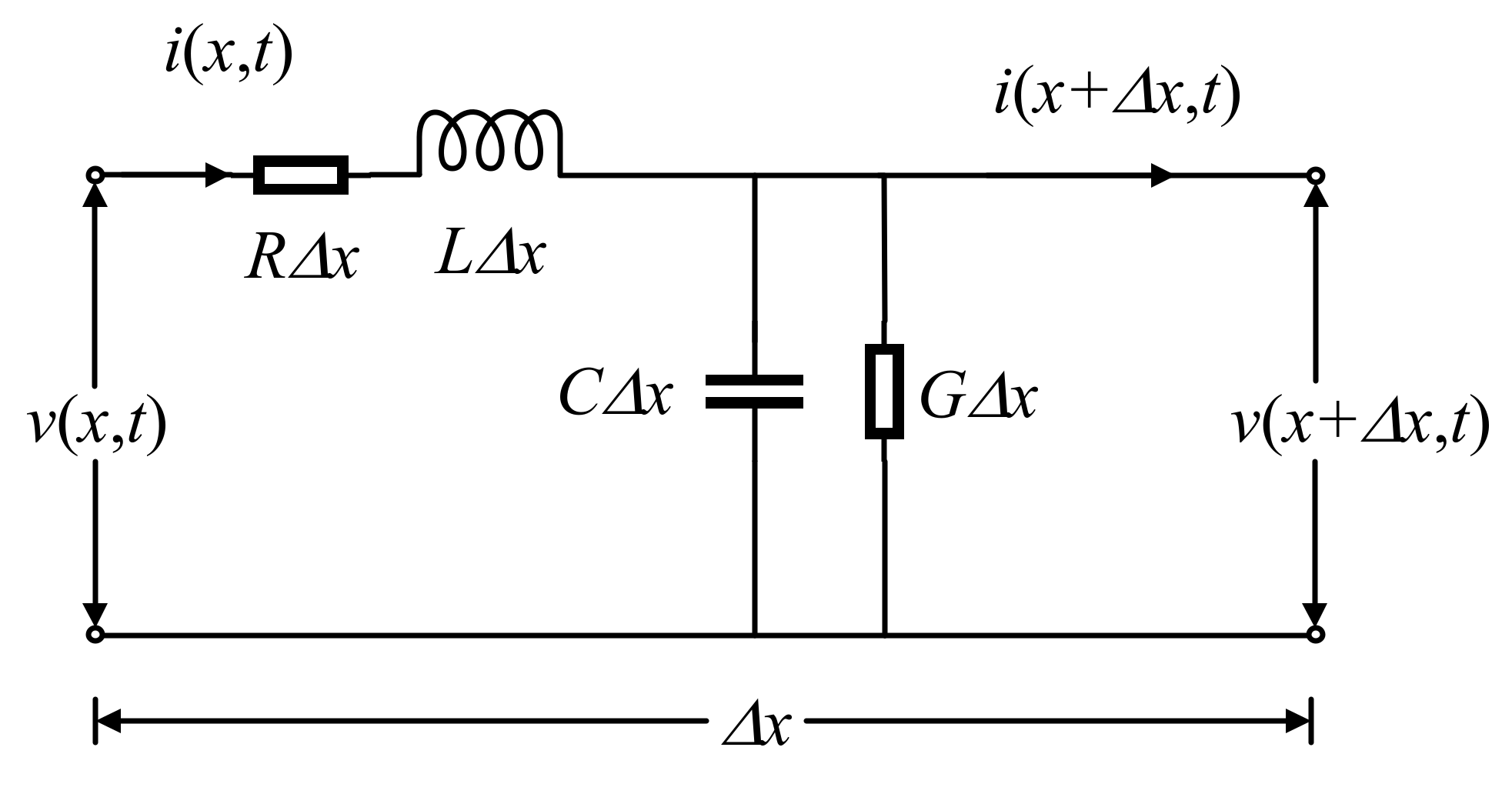
2. The Impedance Spectroscopy of Power Cables
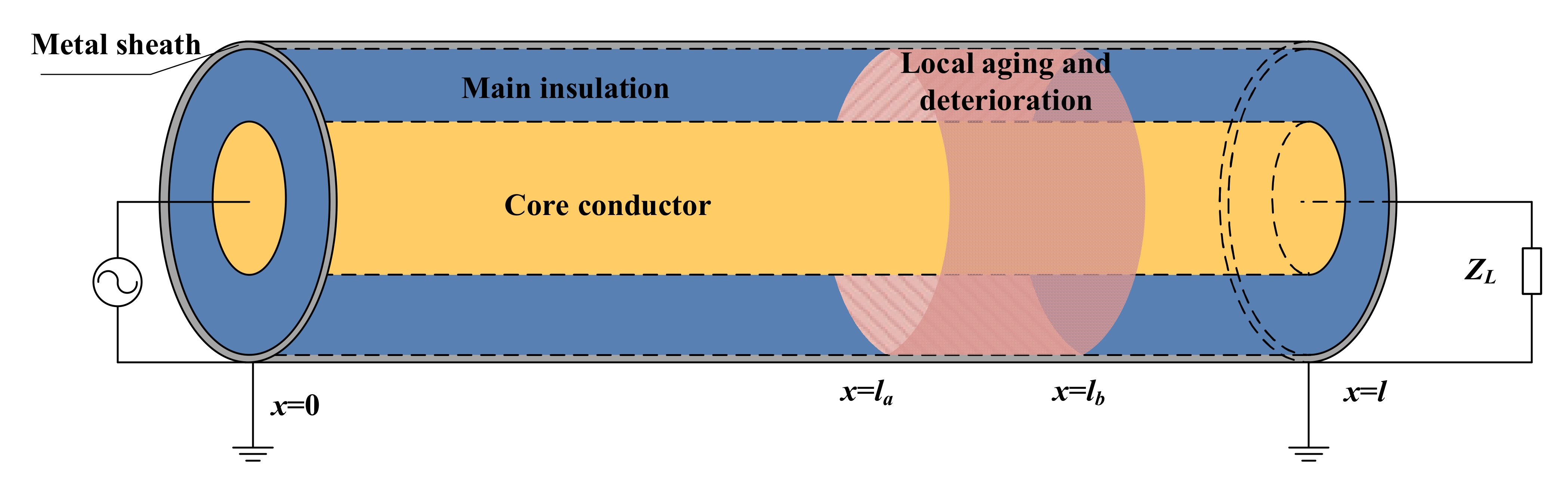
2.1. Typical Structure of Power Cables
2.2. Theoretical Basis
2.3. The Impedance Spectroscopy
2.4. Determination of Local Degradation
3. Simulations and Results
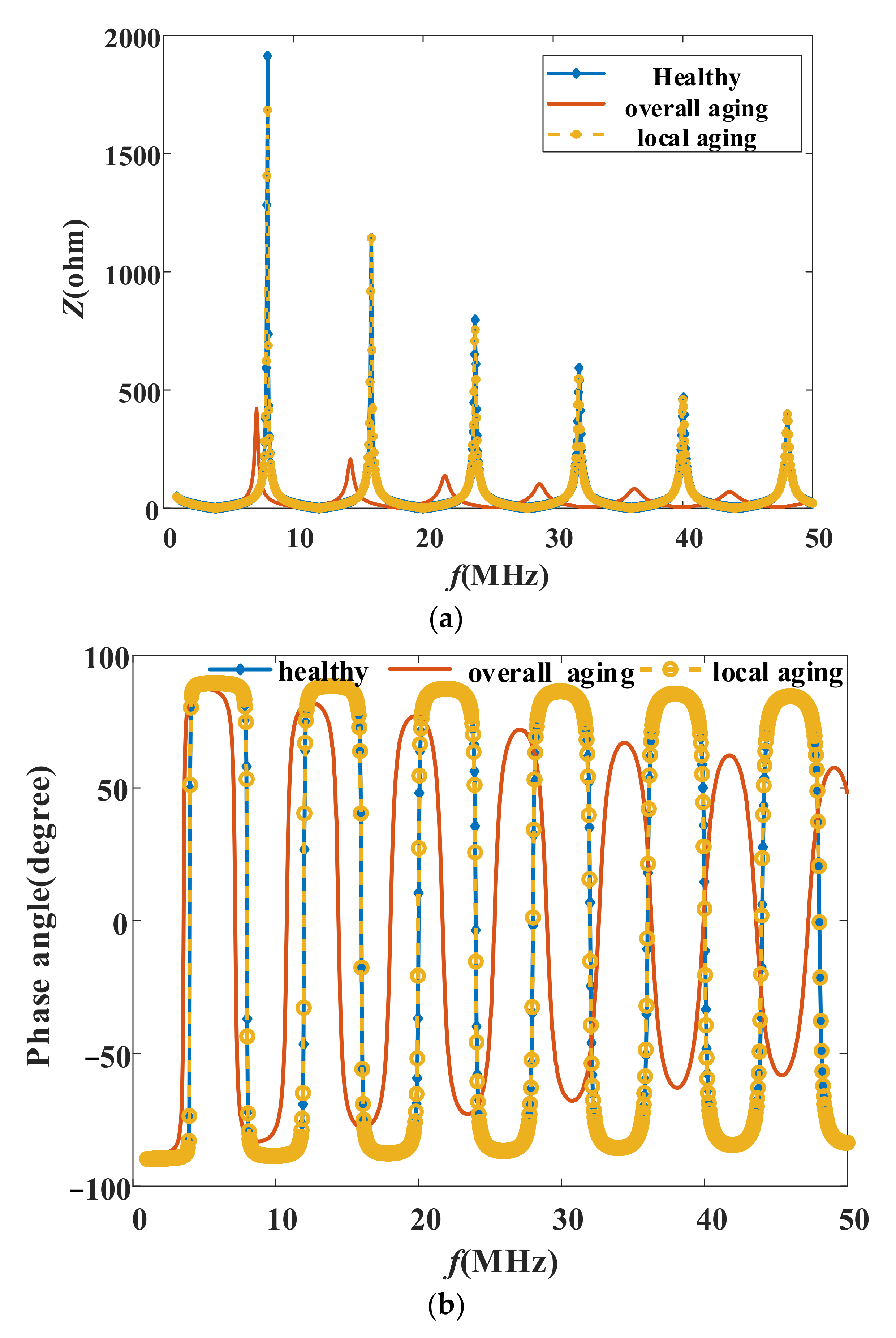
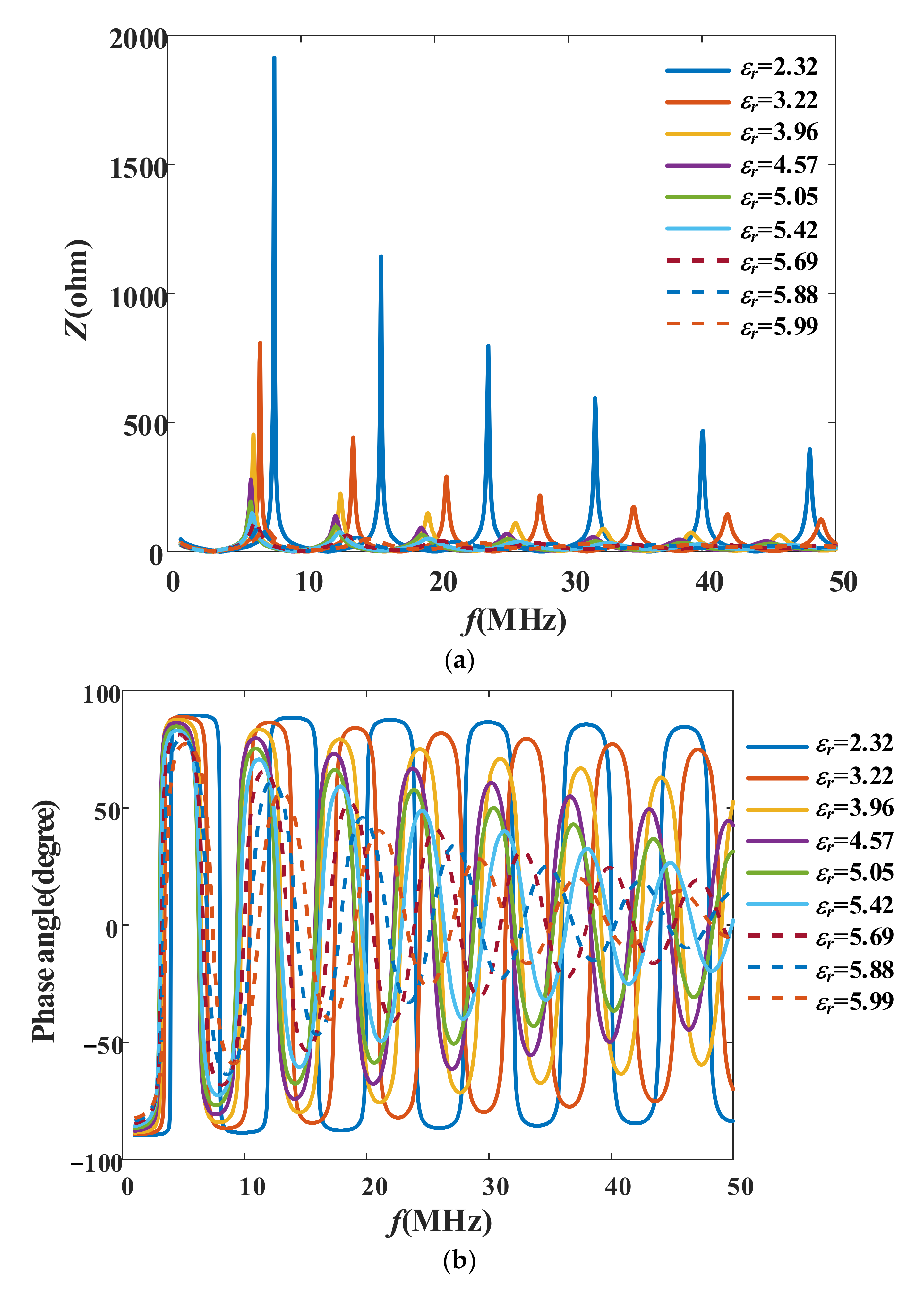
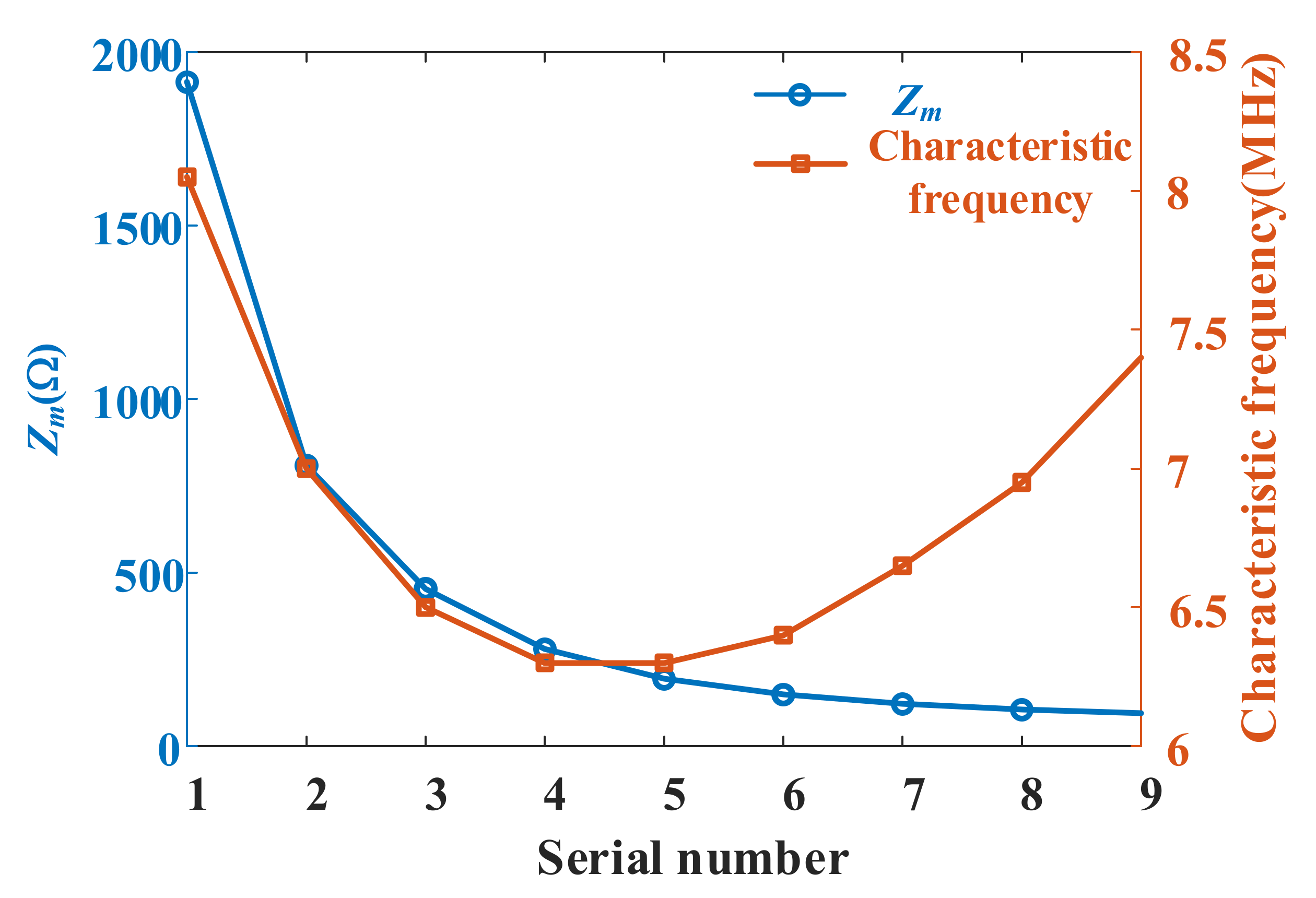
3.1. The Impedance Spectroscopy for Healthy and Aged Cables
3.2. The Frequency Range
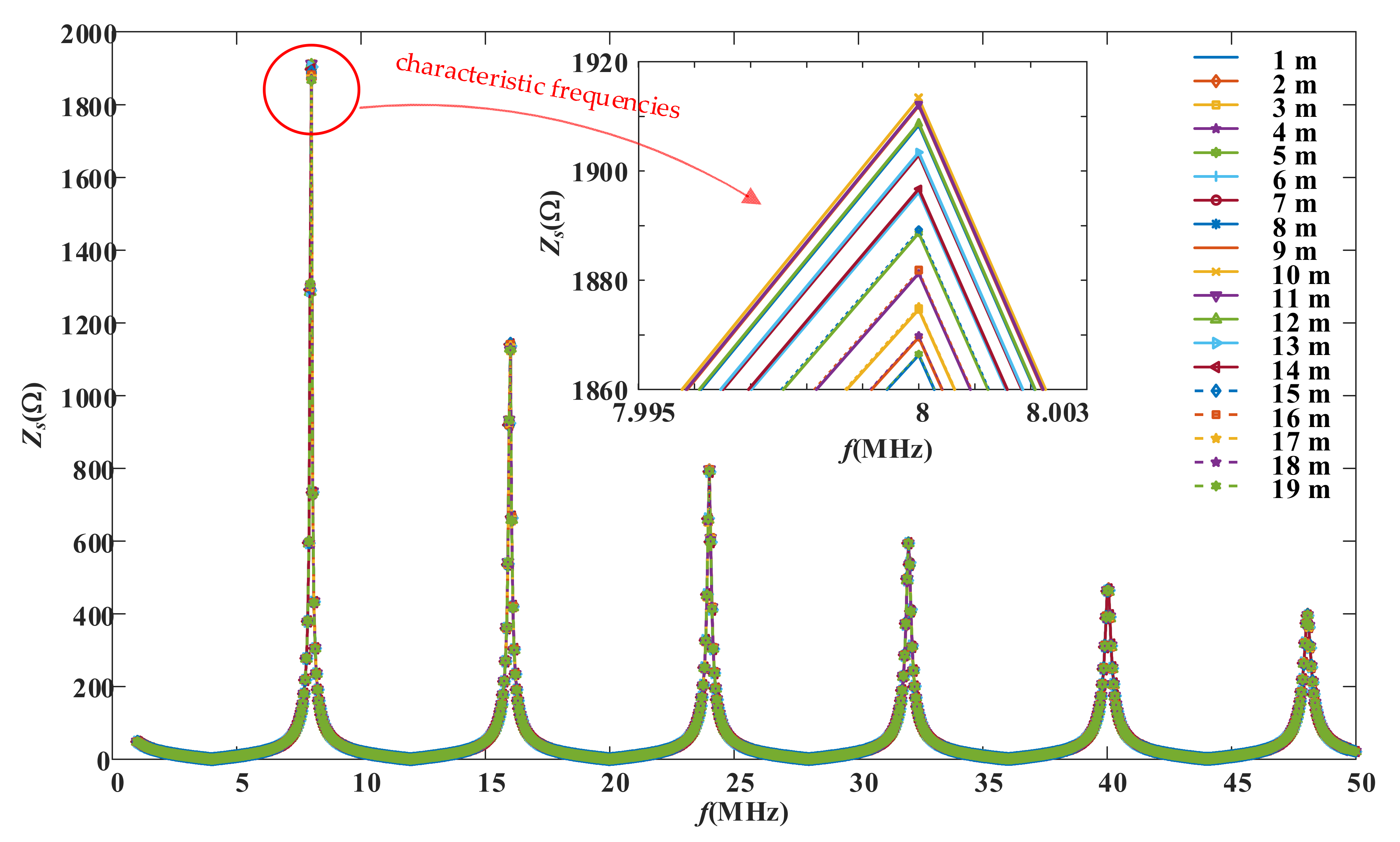
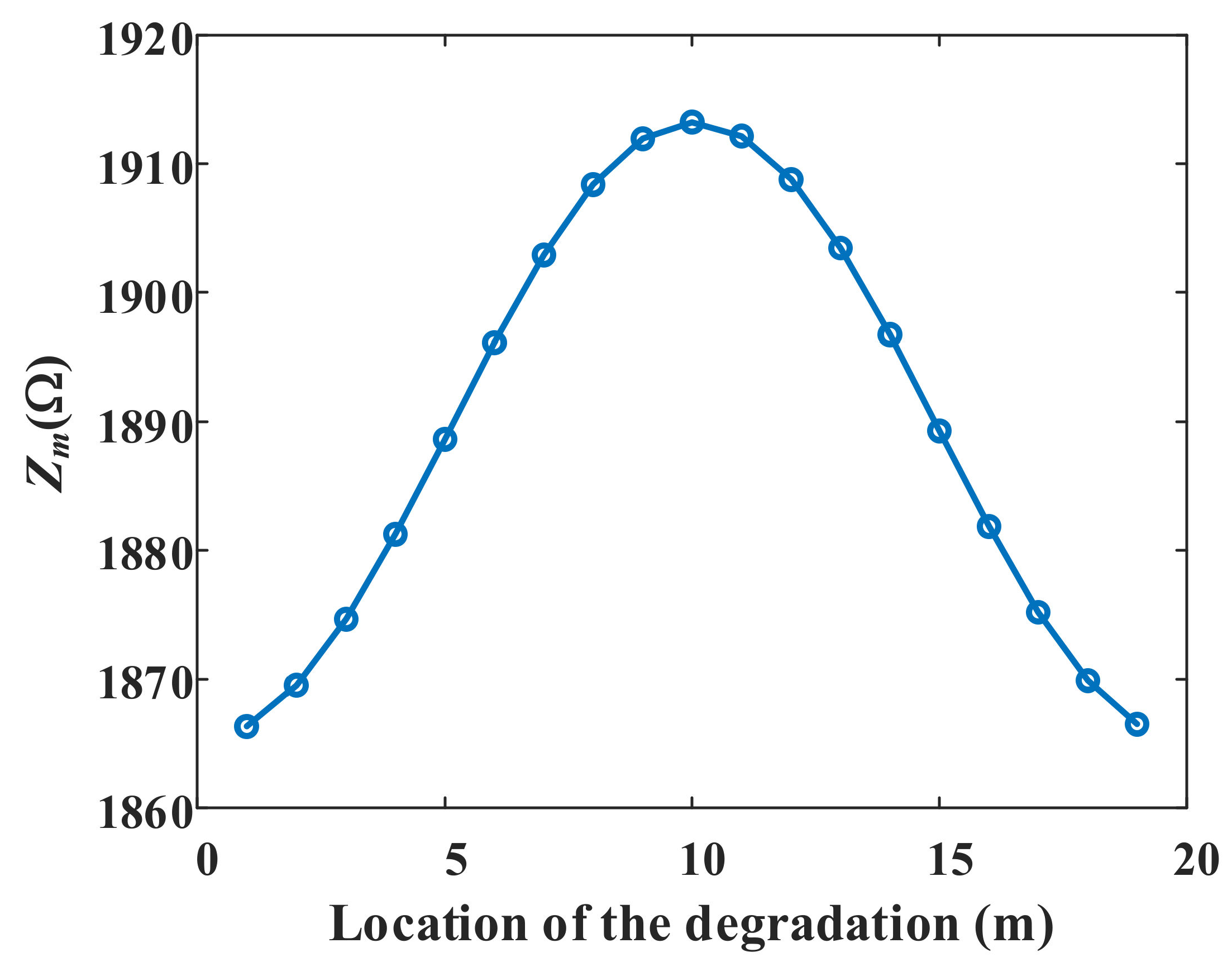
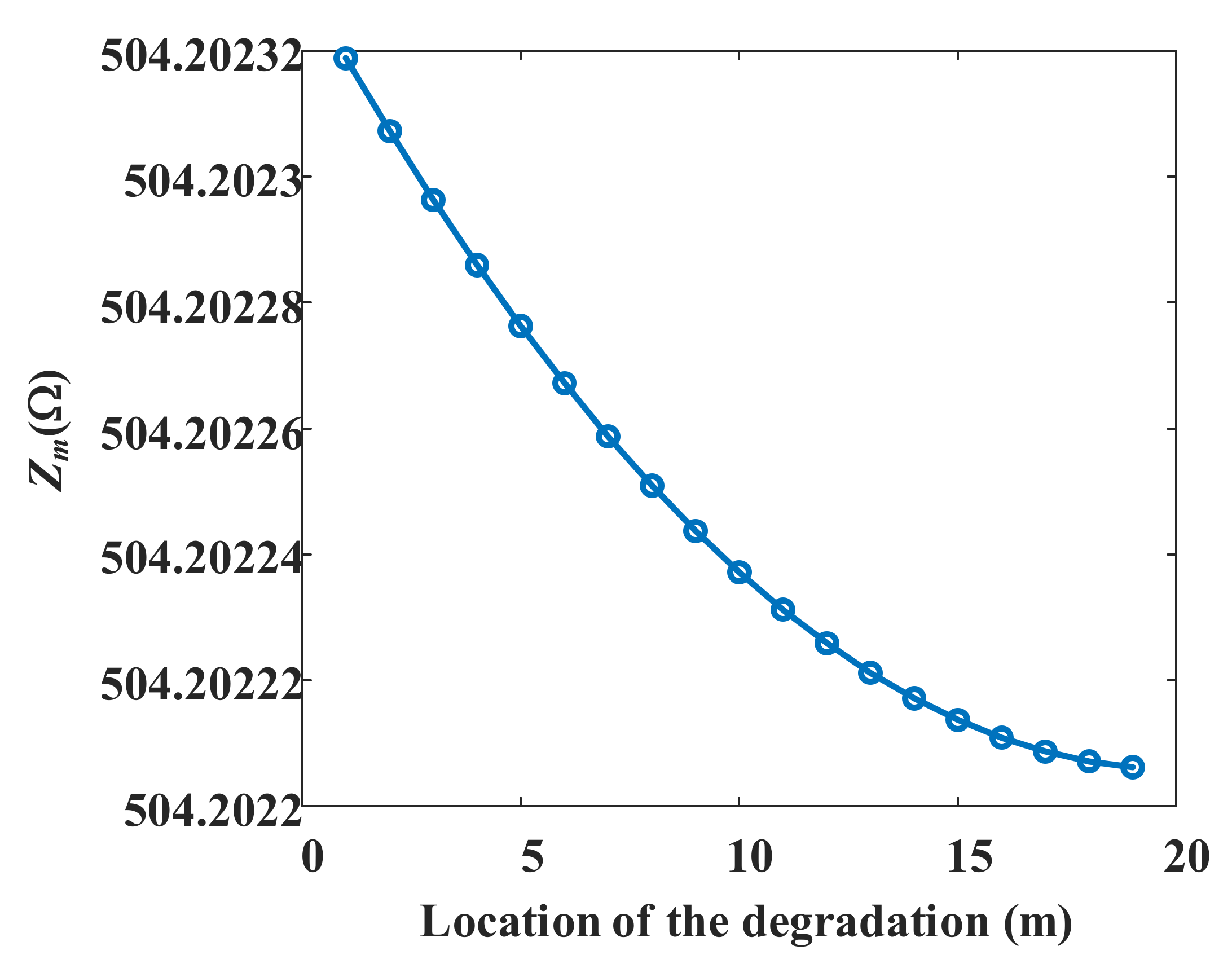
3.3. The Location for Local Degradation
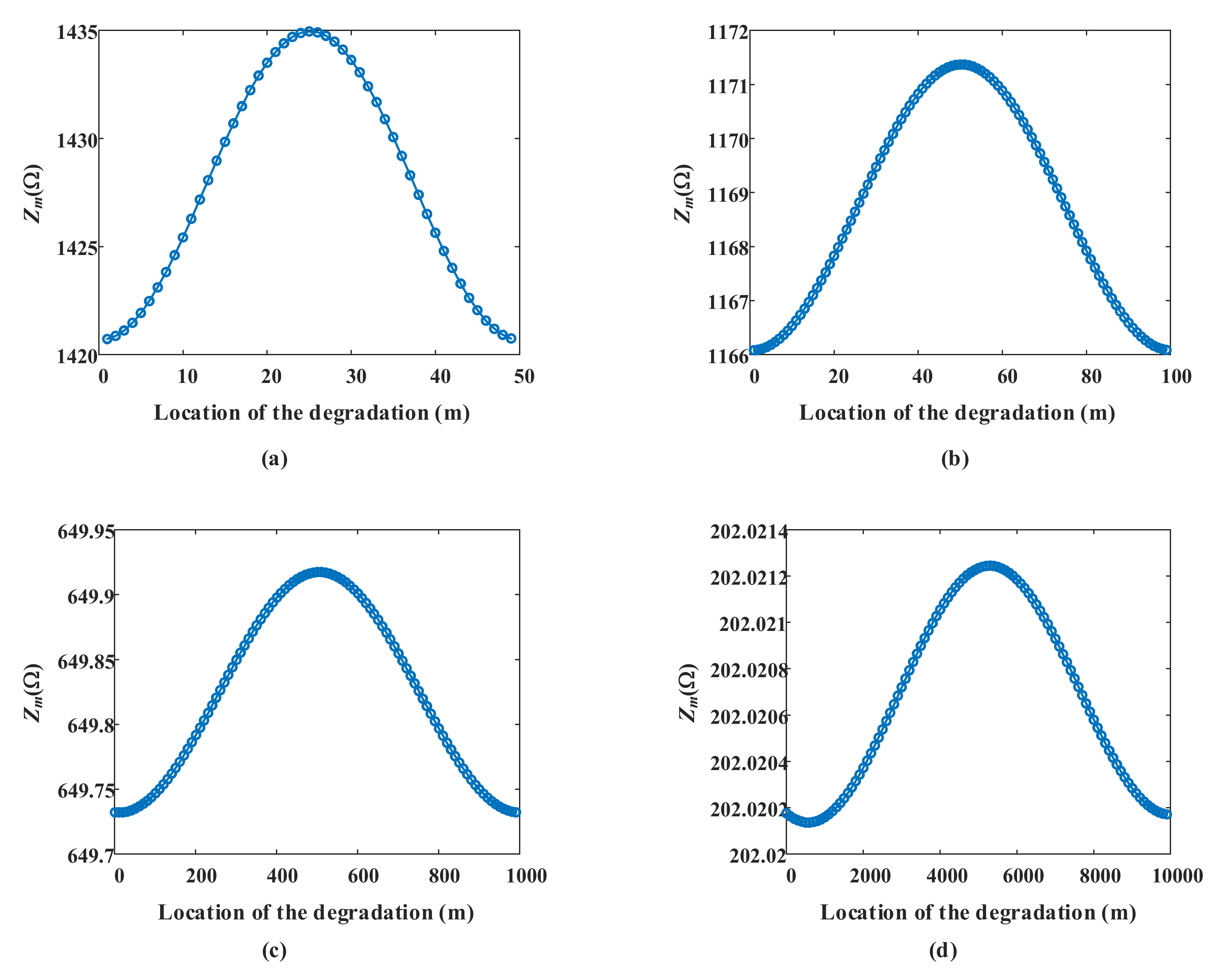
3.3.1. Fault Location for Different Cable Lengths
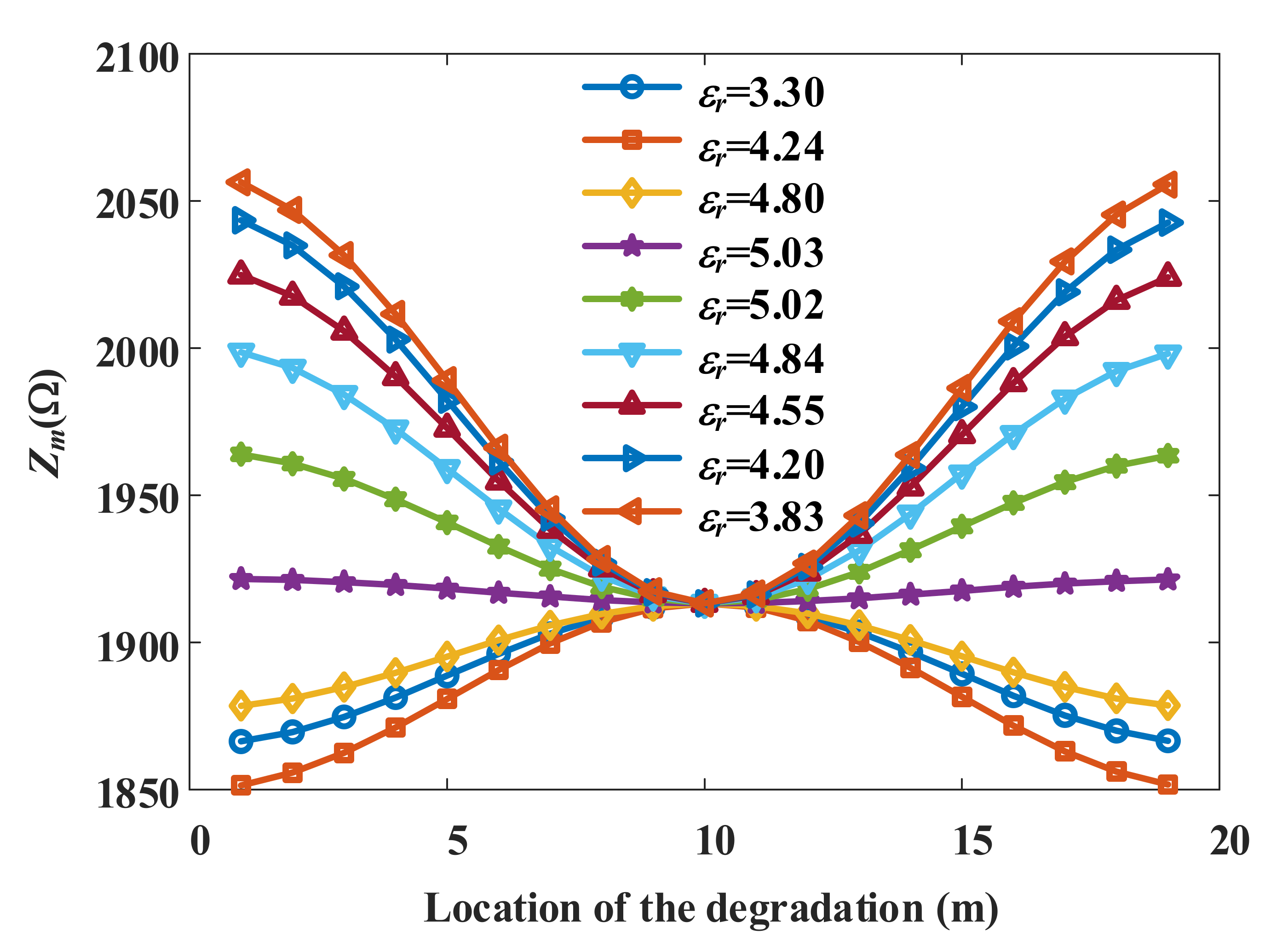
3.3.2. The Location for Different Degrees of the Degradation
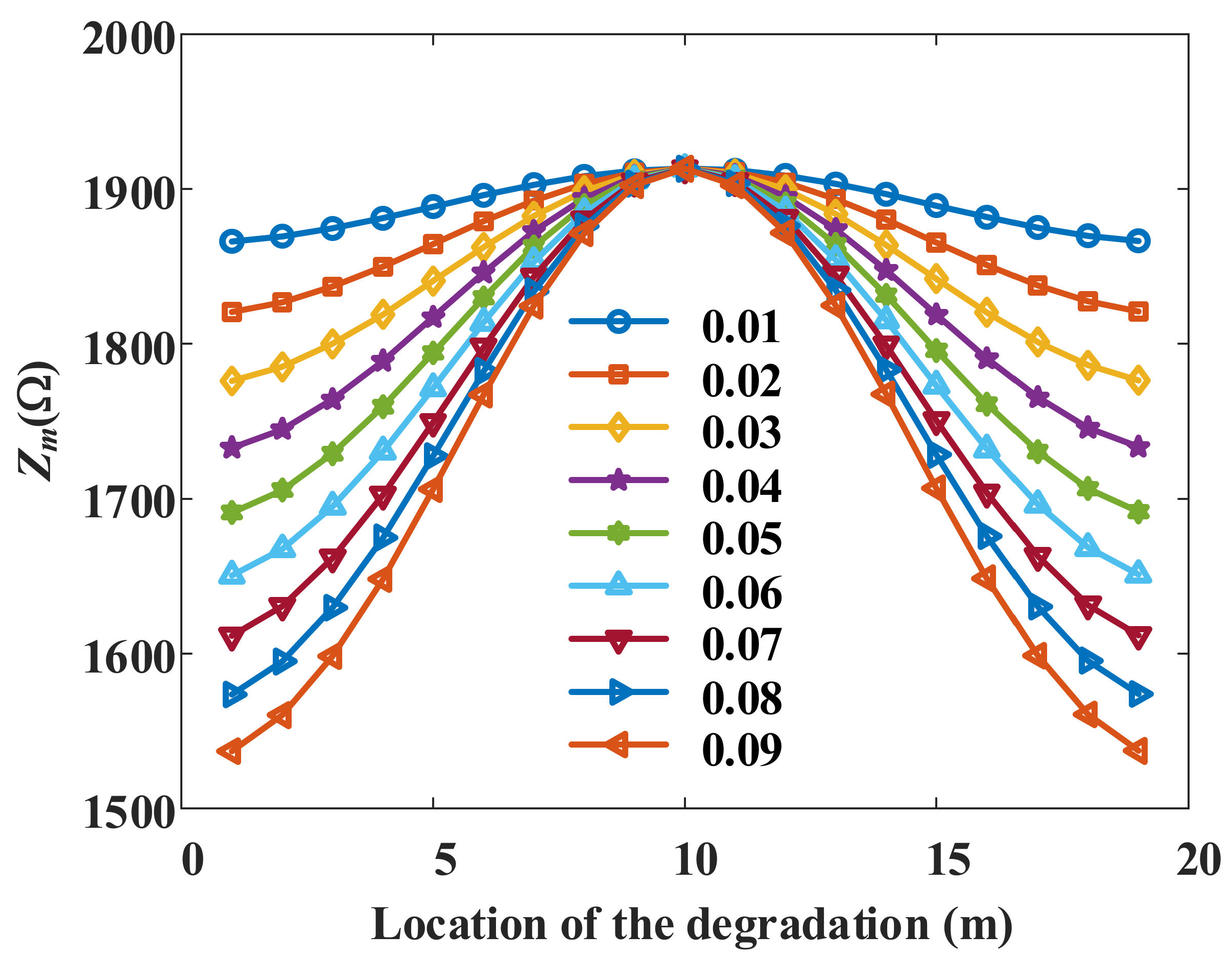
3.3.3. The Location for Different Local Degradation Sizes
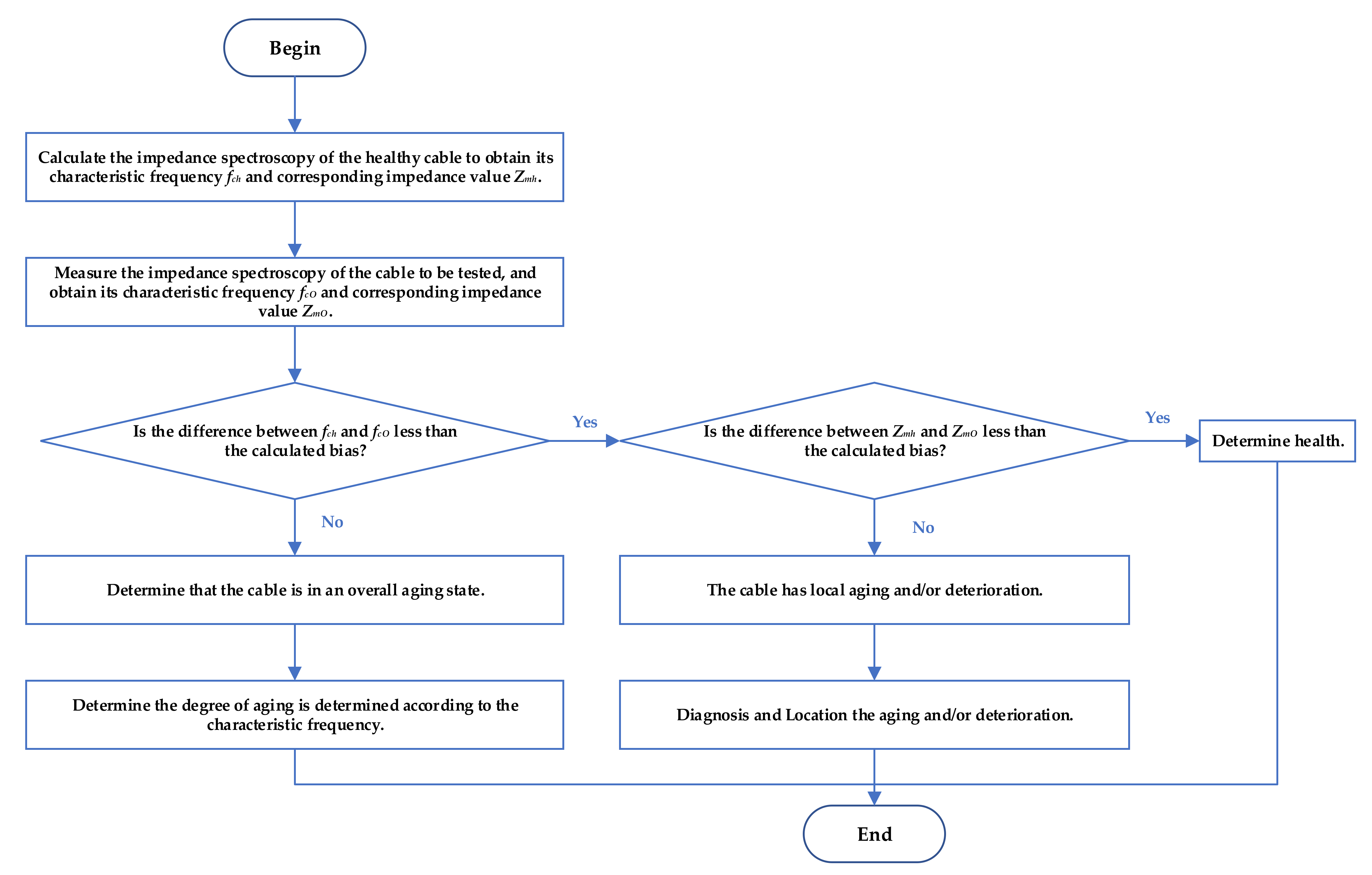
4. The Criterion for the Diagnosis and Location
5. Discussion
5.1. The Frequency Range
5.2. The Advantages of the Proposed Method
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhou, C.; Yi, H.; Dong, X. Review of recent research towards power cable life cycle management. High Volt. 2017, 2, 179–187. [Google Scholar] [CrossRef]
- Fabiani, D.; Mazzanti, G.; Suraci, S.V.; Diban, B. Innovative Development and Application of a Stress-Strength Model for Reliability Estimation of Aged LV Cables for Nuclear Power Plants. IEEE Trans. Dielectr. Electr. Insul. 2021, 28, 2083–2090. [Google Scholar] [CrossRef]
- Montanari, G.C.; Hebner, R.; Morshuis, P.; Seri, P. An approach to insulation condition monitoring and life assessment in emerging electrical environments. IEEE Trans. Power Deliv. 2019, 34, 1357–1364. [Google Scholar] [CrossRef]
- Hedir, A.; Moudoud, M.; Lamrous, O.; Rondot, S.; Jbara, O.; Dony, P. Ultraviolet radiation aging impact on physicochemical properties of crosslinked polyethylene cable insulation. J. Appl. Polym. Sci. 2020, 137, 48575. [Google Scholar] [CrossRef]
- Fabiani, D.; Suraci, S.V. Broadband Dielectric Spectroscopy: A Viable Technique for Aging Assessment of Low-Voltage Cable Insulation Used in Nuclear Power Plants. Polymers 2021, 13, 494. [Google Scholar] [CrossRef]
- Jahromi, A.N.; Pattabi, P.; Densley, J.; Lamarre, L. Medium voltage XLPE cable condition assessment using frequency domain spectroscopy. IEEE Electr. Insul. Mag. 2020, 36, 9–18. [Google Scholar] [CrossRef]
- Alghamdi, A.S.; Desuqi, R.K. A study of expected lifetime of XLPE insulation cables working at elevated temperatures by applying accelerated thermal ageing. Heliyon 2020, 6, e03120. [Google Scholar] [CrossRef] [Green Version]
- Montanari, G.C.; Seri, P.; Bononi, S.F.; Albertini, M. Partial discharge behavior and accelerated aging upon repetitive DC cable energization and voltage supply polarity inversion. IEEE Trans. Power Del. 2021, 36, 578–586. [Google Scholar] [CrossRef]
- Mor, A.R.; Munoz, F.A.; Wu, J.; Heredia, L.C.C. Automatic partial discharge recognition using the cross wavelet transform in high voltage cable joint measuring systems using two opposite polarity sensors. Int. J. Electr. Power Energy Syst. 2020, 117, 105695. [Google Scholar]
- Algwari, Q.T.; Saleh, D.N. Numerical Modeling of Partial Discharge in a Void Cavity Within High-Voltage Cable Insulation. IEEE Trans. Plasma Sci. 2021, 49, 1536–1542. [Google Scholar] [CrossRef]
- Dong, X.; Yang, Y.; Zhou, C.; Hepburn, D.M. Online Monitoring and Diagnosis of HV Cable Faults by Sheath System Currents. IEEE Trans. Power Del. 2017, 32, 2281–2290. [Google Scholar] [CrossRef]
- Yang, Y.; Hepburn, D.M.; Zhou, C.; Zhou, W.; Bao, Y. On-line monitoring of relative dielectric losses in cross-bonded cables using sheath currents. IEEE Trans. Dielectr. Electr. Insul. 2017, 24, 2677–2685. [Google Scholar] [CrossRef] [Green Version]
- Shi, Q.; Troeltzsch, U.; Kanoun, O. Detection and localization of cable faults by time and frequency domain measurements. In Proceedings of the 7th International Multi-Conference on Systems, Signals and Devices, Amman, Jordan, 27–30 June 2010; pp. 1–6. [Google Scholar]
- Shi, Q.; Kanoun, O. A new method of locating the single wire fault. In Proceedings of the 12th International Conference on Signal Processing (ICSP), HangZhou, China, 26–30 October 2014; pp. 2262–2267. [Google Scholar]
- Fantoni, P.F.; Toman, G.J. Cable Aging Assessment and Condition Monitoring Using Line Resonance Analysis (LIRA); American Nuclear Society—ANS: La Grange Park, IL, USA, 2006; Volume 12, pp. 177–186. [Google Scholar]
- Maier, T.; Lick, D.; Leibfried, T. Frequency-Domain Cable Model with a Real Representation of Joints and Smooth Failure Changes and a Diagnostic Investigation with Line Resonance Analysis. In Proceedings of the 2018 Condition Monitoring and Diagnosis (CMD), Perth, Australia, 23–26 September 2018; pp. 1–6. [Google Scholar]
- Ohki, Y.; Hirai, N. Effects of the structure and insulation material of a cable on the ability of a location method by FDR. IEEE Trans. Dielectr. Electr. Insul. 2016, 23, 77–84. [Google Scholar] [CrossRef]
- Hirai, N.; Ohki, Y. Highly sensitive detection of distorted points in a cable by frequency domain reflectometry. In Proceedings of the International Symposium on Electrical Insulating Materials, Niigata City, Japan, 1–5 June 2014; pp. 144–147. [Google Scholar]
- Yamada, T.; Hirai, N.; Ohki, Y. Improvement in sensitivity of broadband impedance spectroscopy for locating degradation in cable insulation by ascending the measurement frequency. In Proceedings of the International Conference on Condition Monitoring and Diagnosis, Bali, Indonesia, 23–27 September 2012; pp. 677–680. [Google Scholar]
- Zhou, Z.; Zhang, D.; He, J.; Li, M. Local degradation diagnosis for cable insulation based on broadband impedance spectroscopy. IEEE Trans. Dielectr. Electr. Insul. 2015, 22, 2097–2107. [Google Scholar] [CrossRef]
- Mo, S.; Zhang, D.; Li, Z.; Wan, Z. The Possibility of Fault Location in Cross-Bonded Cables by Broadband Impedance Spectroscopy. IEEE Trans. Dielectr. Electr. Insul. 2021, 28, 1416–1423. [Google Scholar] [CrossRef]
- Pan, W.; Li, X.; Zhu, Z.; Zhao, K.; Xie, C.; Su, Q. Detection Sensitivity of Input Impedance to Local Defects in Long Cables. IEEE Access 2020, 99, 55702–55710. [Google Scholar] [CrossRef]
- Rogovin, D.; Lofaro, R. Evaluation of the Broadband Impedance Spectroscopy Prognostic/Diagnostic Technique for Electric Cables Used in Nuclear Power Plants. U.S Nuclear Regulatory Commission. Available online: https://www.nrc.gov/reading-rm/doc-collections/nuregs/contract/cr6904/cr6904.pdf (accessed on 18 July 2022).
- Wedepohl, L.M.; Wilcox, D.J. Transient analysis of underground power-transmission systems. System-model and wave-propagation characteristics. Proc. Inst. Electr. Eng. 1973, 120, 253–260. [Google Scholar] [CrossRef]
- Teng, C.; Zhou, Y.; Zhang, L.; Zhang, Y.; Huang, X.; Chen, J. Improved electrical resistivity-temperature characteristics of insulating epoxy composites filled with polydopamine-coated ceramic particles with positive temperature coefficient. Compos. Sci. Technol. 2022, 221, 109365. [Google Scholar] [CrossRef]
- Said, A.; Ghunem, R.A. A Techno-Economic Framework for Replacing Aged XLPE Cables in the Distribution Network. IEEE Trans. Power Del. 2020, 35, 2387–2393. [Google Scholar]
- Arikan, O.; Uydur, C.C.; Kumru, C.F. Prediction of dielectric parameters of an aged mv cable: A comparison of curve fitting, decision tree and artificial neural network methods. Electr. Power Syst. Res. 2022, 208, 107892. [Google Scholar] [CrossRef]
- Paramane, A.; Chen, X.; Dai, C.; Guan, H.; Yu, L.; Tanaka, Y. Electrical insulation performance of cross-linked polyethylene/MgO nanocomposite material for ±320 kV high-voltage direct-current cables. Polymer Compos. 2020, 41, 1936–1949. [Google Scholar] [CrossRef]
- IEC 60287-1-1:2006+A1:2014; Electric Cables—Calculation of the Current Rating—Part1-1: Current Rating Equations (100% Load factor) and Calculation of Losses—General. International Electrotechnical Commission: Geneva, Switzerland, 2014.

| The Structural Parameters | Value (mm) | The Material Parameters | Value (Ω·m) |
|---|---|---|---|
| r1 | 4 | ρc | 1.68 × 10−8 |
| r2 | 8.9 | ρs | 1.68 × 10−8 |
| r3 | 9.5 |
| Serial Number | ε Parameters | Relative Permittivity | Tan δ |
|---|---|---|---|
| 1 | A = 4.26, B = 0.22, p = 0.05 | 3.30 | 0.02 |
| 2 | A = 6.39, B = 0.33, p = 0.075 | 4.24 | 0.04 |
| 3 | A = 8.52, B = 0.44, p = 0.1 | 4.80 | 0.07 |
| 4 | A = 10.65, B = 0.55, p = 0.125 | 5.03 | 0.10 |
| 5 | A = 12.78, B = 0.66, p = 0.15 | 5.02 | 0.14 |
| 6 | A = 14.91, B = 0.77, p = 0.175 | 4.84 | 0.19 |
| 7 | A = 17.04, B = 0.88, p = 0.2 | 4.55 | 0.24 |
| 8 | A = 19.17, B = 0.99, p = 0.225 | 4.20 | 0.28 |
| 9 | A = 21.3, B = 1.10, p = 0.25 | 3.83 | 0.34 |
| Serial Number | ε Parameters | Relative Permittivity | Tan δ |
|---|---|---|---|
| 1 | A = 2.68, B = 0.14, p = 0.02 | 2.32 | 0.0043 |
| 2 | A = 4.02, B = 0.21, p = 0.03 | 3.22 | 0.0094 |
| 3 | A = 5.36, B = 0.28, p = 0.04 | 3.96 | 0.0164 |
| 4 | A = 6.70, B = 0.35, p = 0.05 | 4.57 | 0.0250 |
| 5 | A = 8.04, B = 0.42, p = 0.06 | 5.05 | 0.0351 |
| 6 | A = 9.38, B = 0.49, p = 0.07 | 5.42 | 0.0465 |
| 7 | A = 10.72, B = 0.56, p = 0.08 | 5.69 | 0.0591 |
| 8 | A = 12.06, B = 0.63, p = 0.09 | 5.88 | 0.0728 |
| 9 | A = 13.40, B = 0.70, p = 0.10 | 5.99 | 0.0873 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, G.; Chen, J.; Li, H.; Hu, L.; Zhou, W.; Zhou, C.; Li, M. Diagnosis and Location of Power Cable Faults Based on Characteristic Frequencies of Impedance Spectroscopy. Energies 2022, 15, 5617. https://doi.org/10.3390/en15155617
Li G, Chen J, Li H, Hu L, Zhou W, Zhou C, Li M. Diagnosis and Location of Power Cable Faults Based on Characteristic Frequencies of Impedance Spectroscopy. Energies. 2022; 15(15):5617. https://doi.org/10.3390/en15155617
Chicago/Turabian StyleLi, Gen, Jie Chen, Hongze Li, Libin Hu, Wenjun Zhou, Chengke Zhou, and Mingzhen Li. 2022. "Diagnosis and Location of Power Cable Faults Based on Characteristic Frequencies of Impedance Spectroscopy" Energies 15, no. 15: 5617. https://doi.org/10.3390/en15155617
APA StyleLi, G., Chen, J., Li, H., Hu, L., Zhou, W., Zhou, C., & Li, M. (2022). Diagnosis and Location of Power Cable Faults Based on Characteristic Frequencies of Impedance Spectroscopy. Energies, 15(15), 5617. https://doi.org/10.3390/en15155617





