Experimental Study on Static Pressure Sedimentation for a Thick-Walled Bucket Foundation in Sand
Abstract
:1. Introduction
2. Model Tests
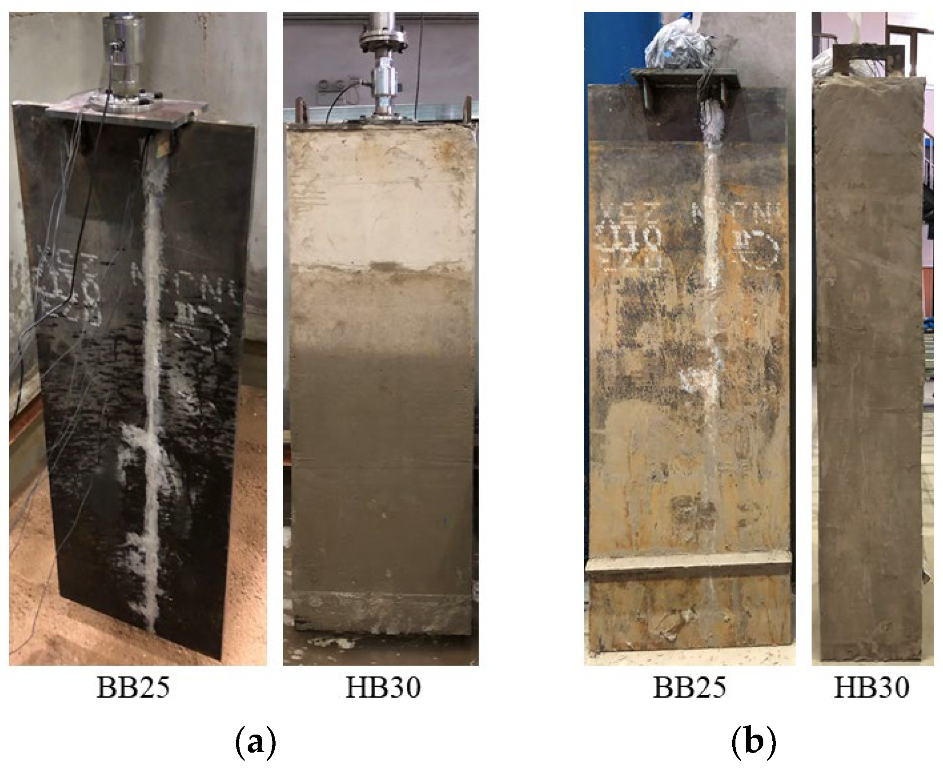
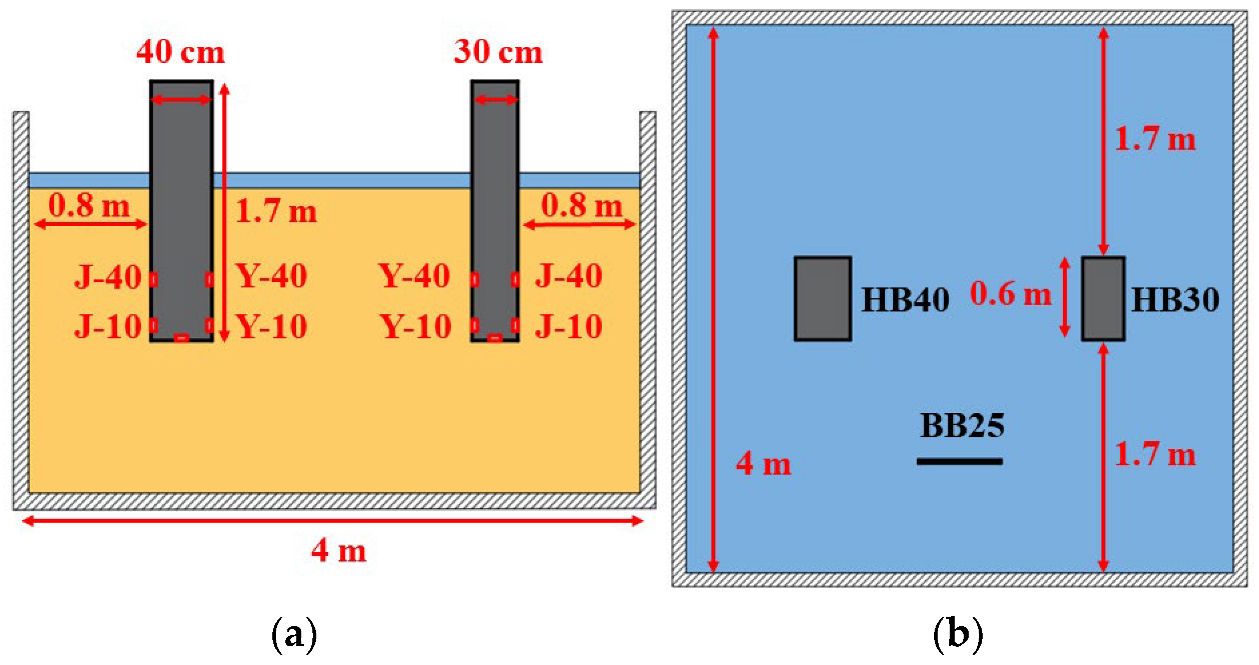
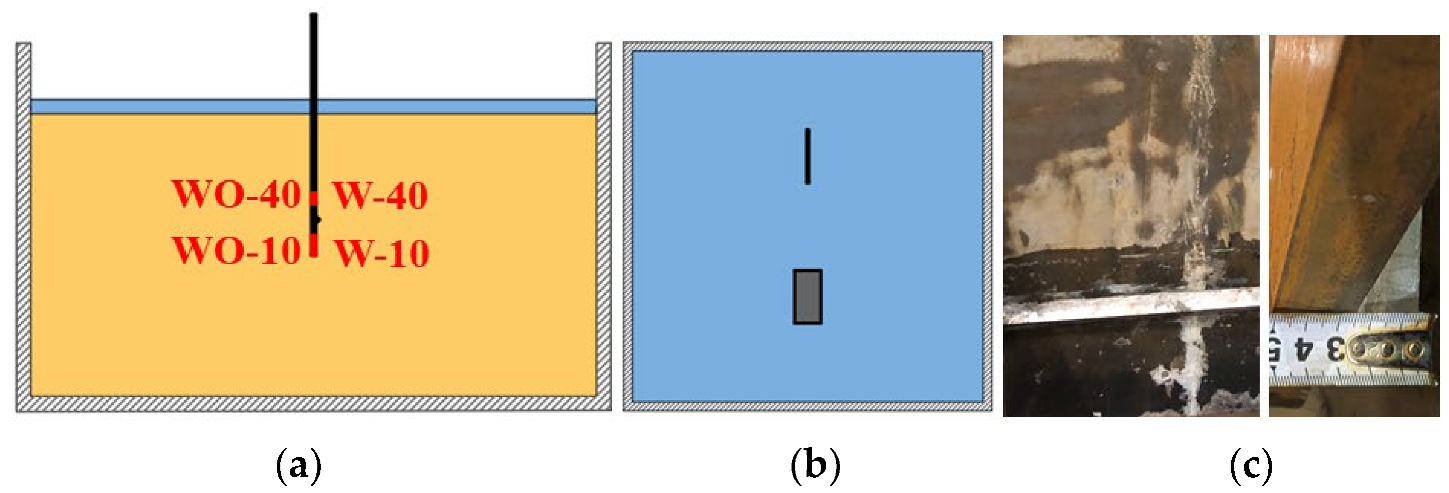
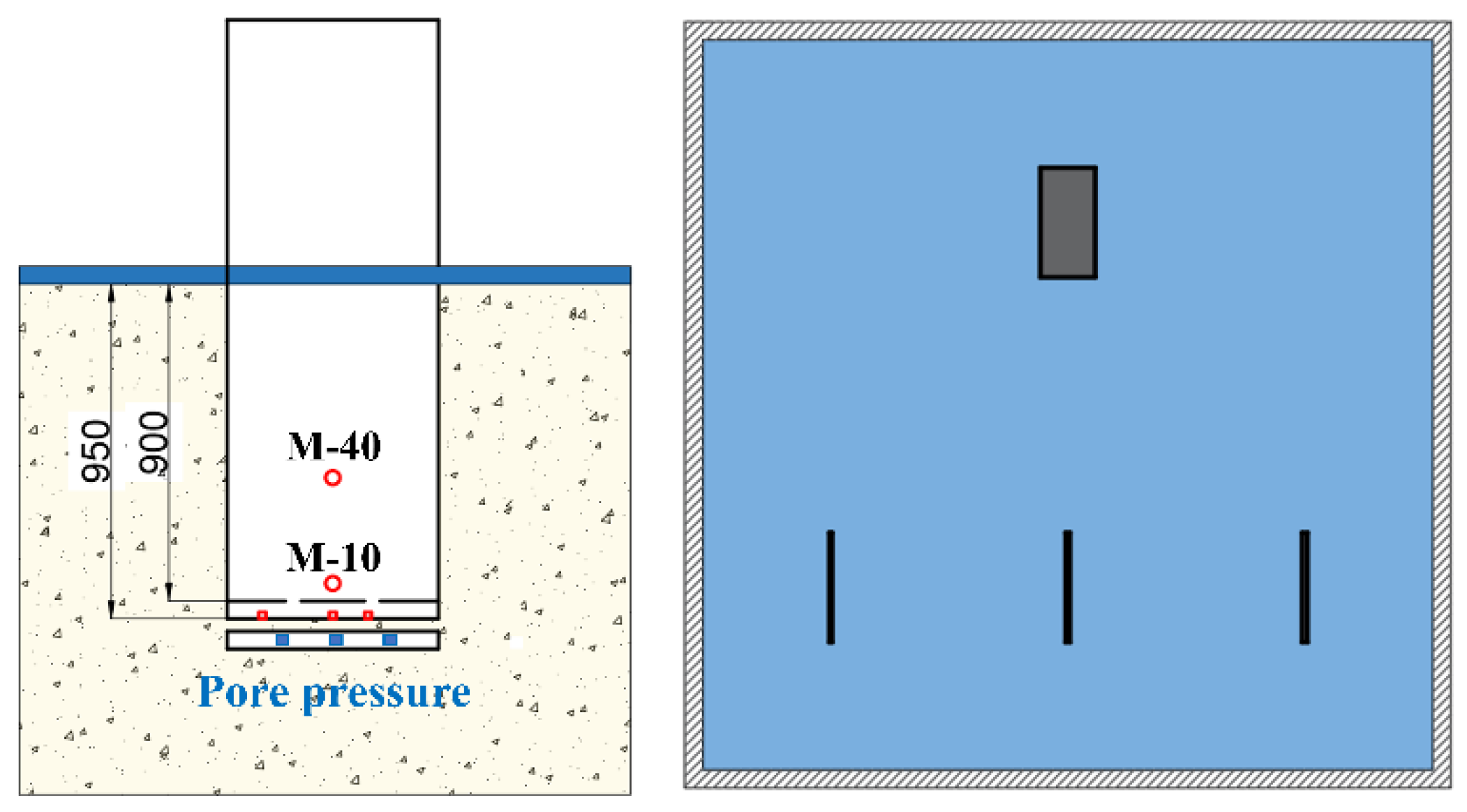
2.1. Model Design and Sensor-Acquisition System
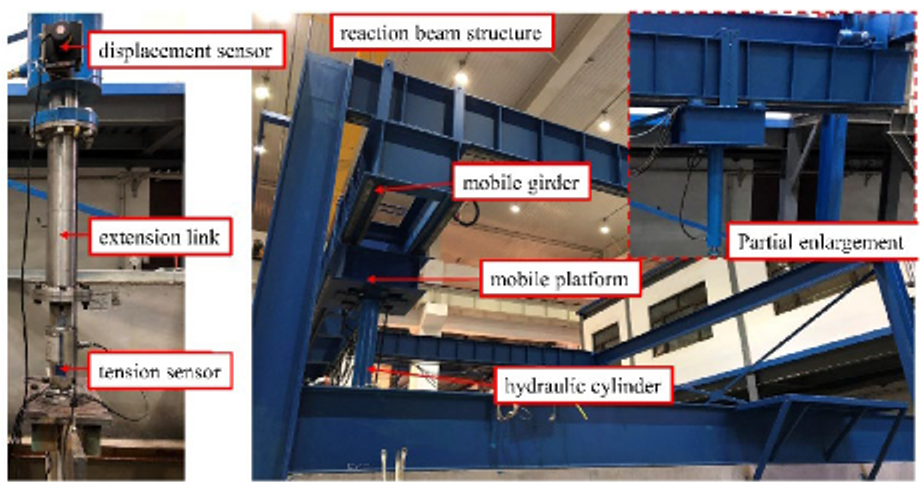
2.2. Equipment and Method
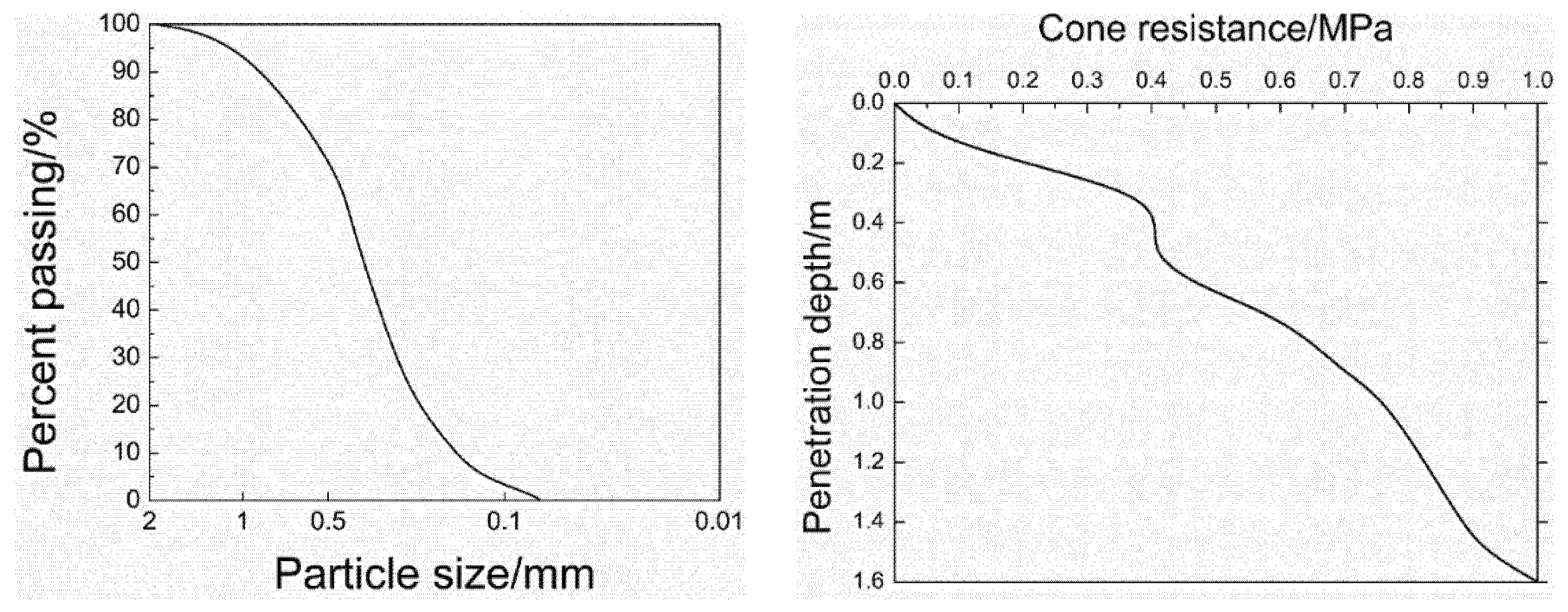
2.3. Sand
3. Test Results
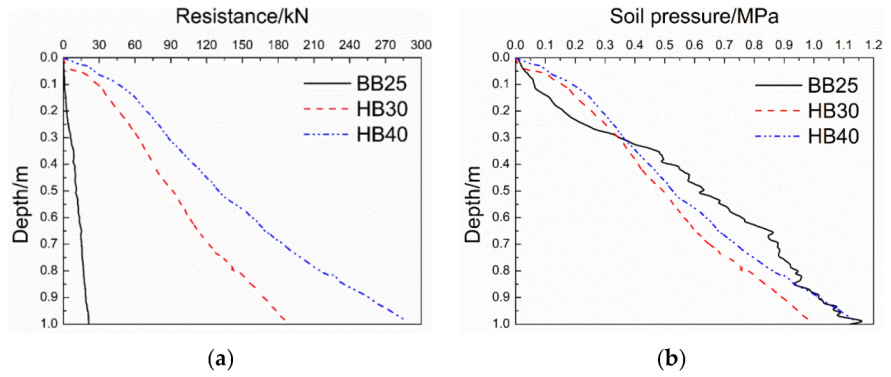
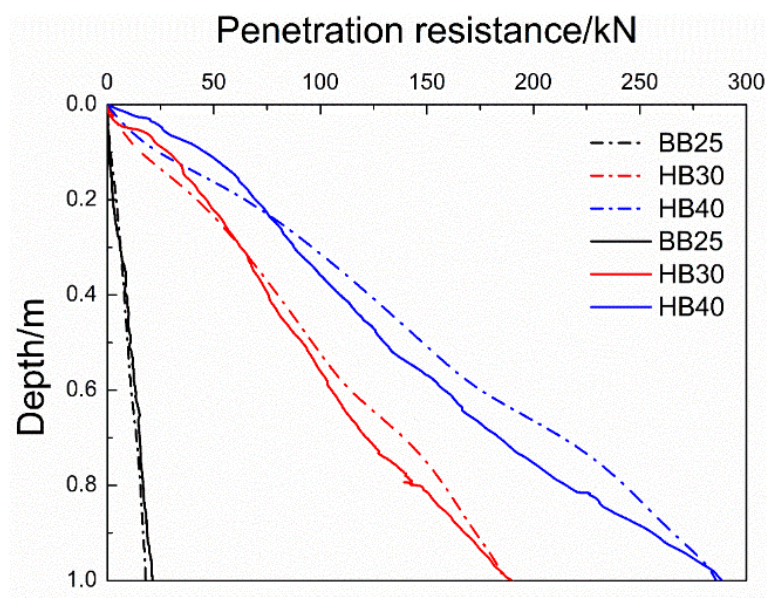
3.1. Sinking Resistance of the Thin-Walled and Thick-Walled Models
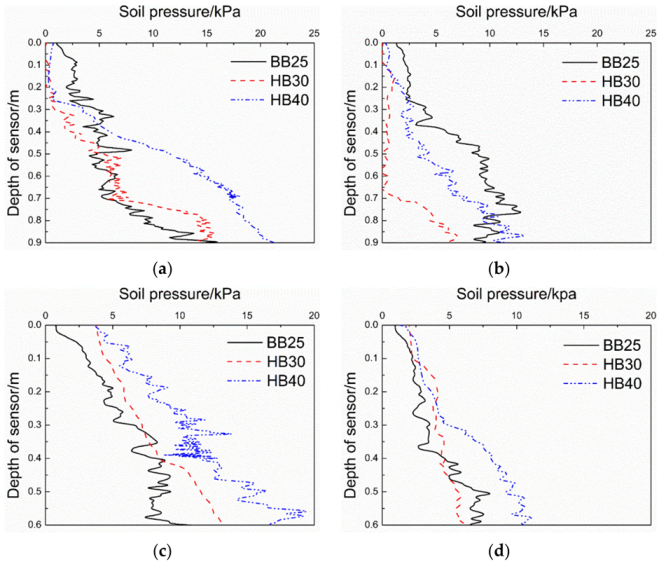
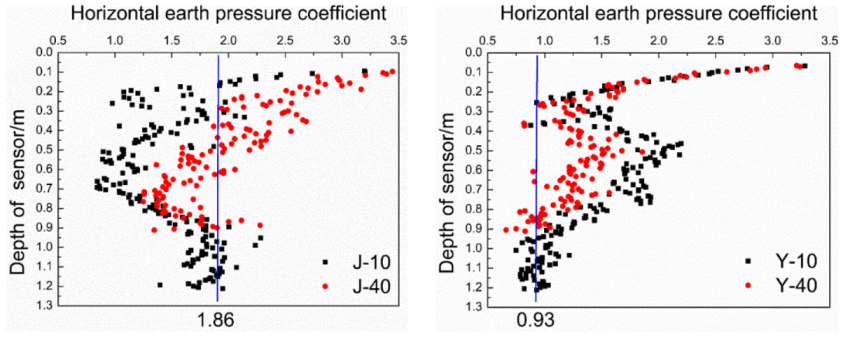
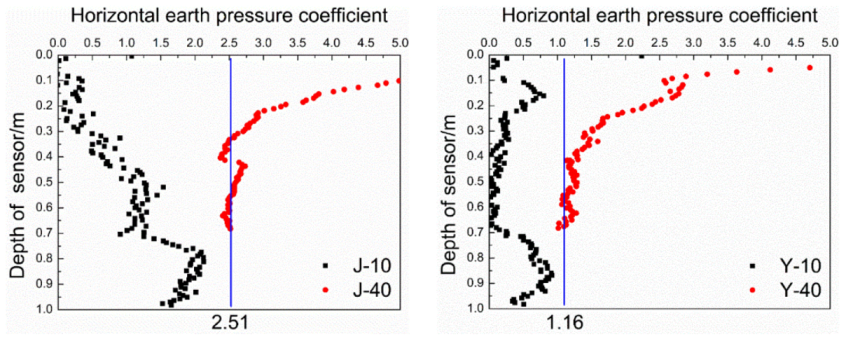
3.2. Horizontal-Soil-Pressure Coefficient
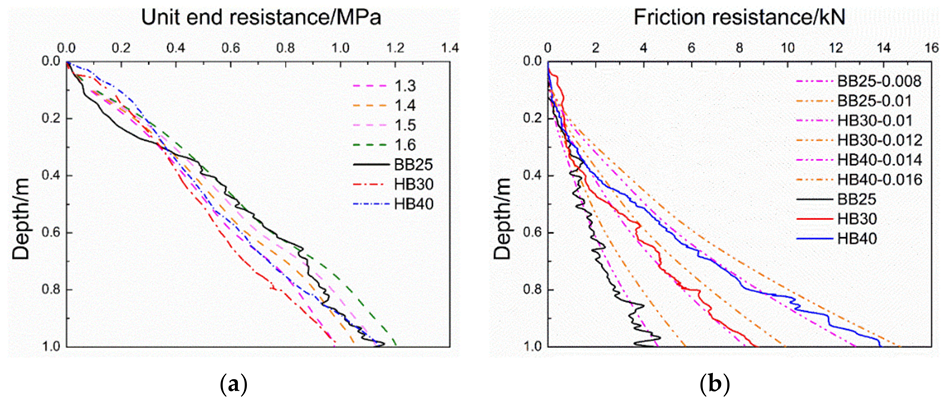
3.3. CPT Coefficient Fitting
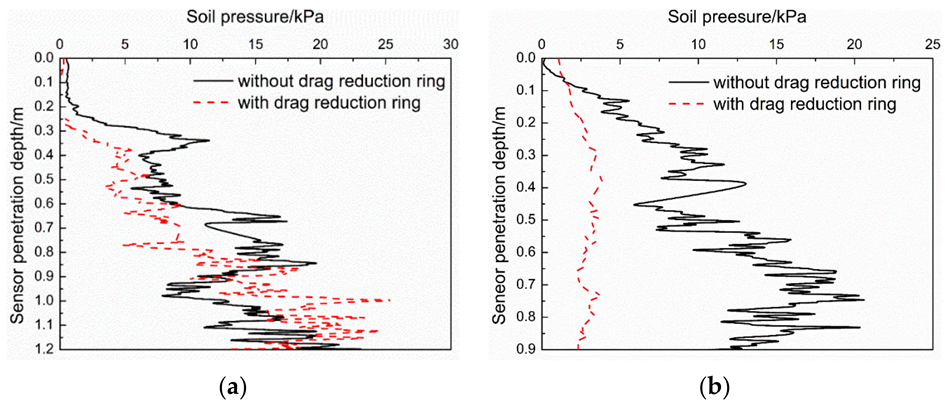
4. Drag Reduction Strategy
4.1. Anti-Drag Ring
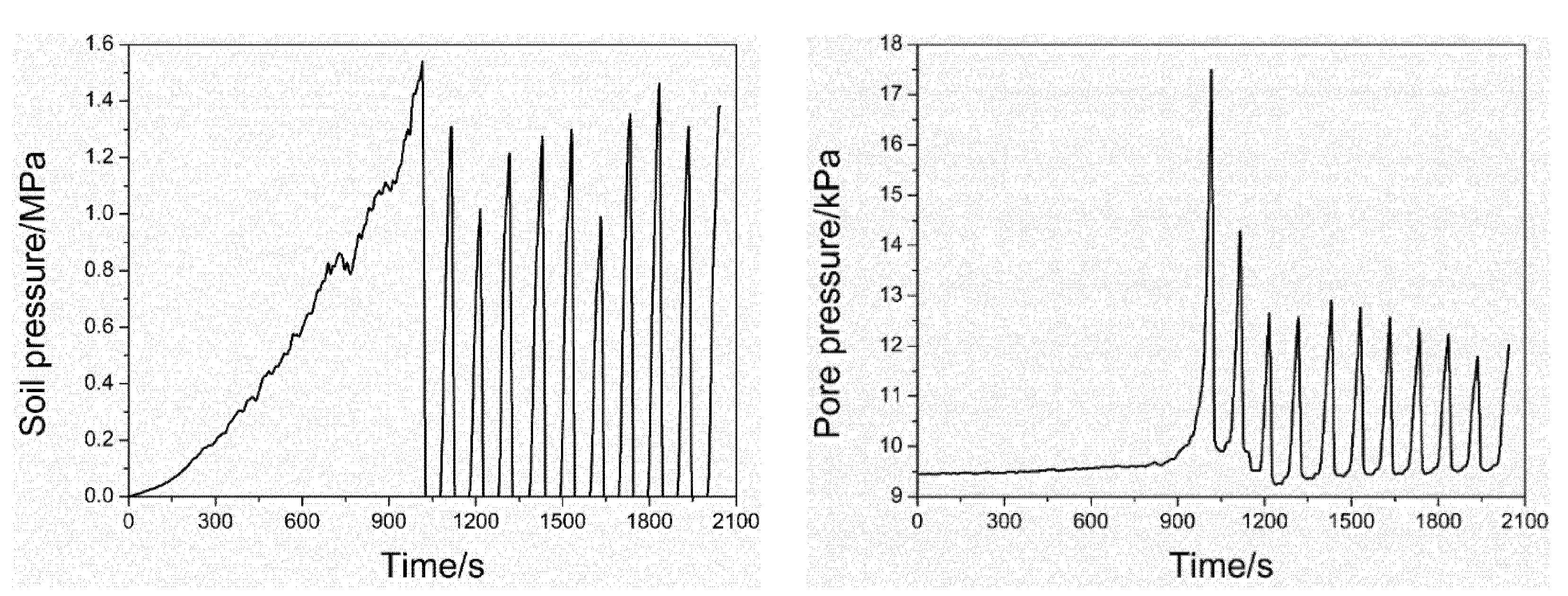
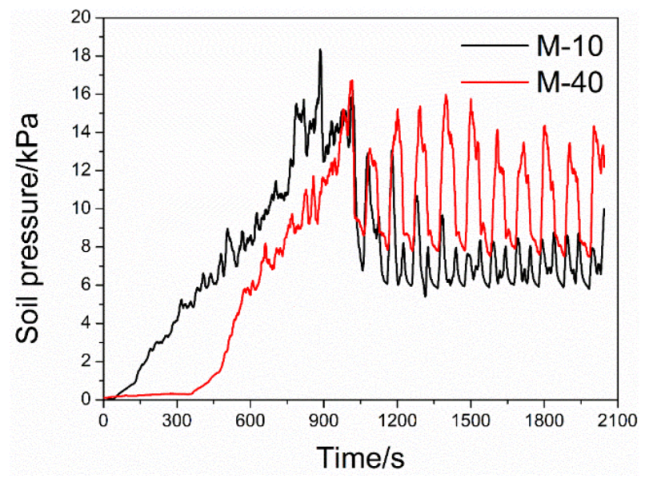
4.2. Pressure-Pull-Out Loading Method
5. Conclusions
- (1)
- The resistance characteristics of the thin-walled model and two thick-walled models were compared through an experiment. The end pressure of the thick-walled models was different from that of the thin-walled model under the condition of the experimental depth, but there were no clear multiple increases. The horizontal-soil-pressure coefficient of the near-wall side was larger than that of the far-wall side for models because of the compaction effect. The horizontal-soil-pressure coefficients at the near-wall and far-wall sides were 1.86 and 0.93 for BB25, 2.51 and 1.16 for HB30, and 3.54 and 2.14 for HB40, respectively.
- (2)
- The suggested values of the end and skin-friction coefficients of the models under the test conditions were presented. The end coefficient of the thin-walled model was 1.5, and the skin-friction coefficient was 0.008. The end and skin-friction coefficients of the HB30 model were 1.4 and 0.012, respectively. The end and skin-friction coefficients of the HB40 model were 1.5 and 0.016, respectively.
- (3)
- The anti-drag ring can reduce the soil pressure at its upper side, and the drag-reduction effect was evident. However, the setting of the anti-drag ring will increase the end resistance, so attention should be paid to the practical engineering application.
- (4)
- The pressure-pull-out loading method can significantly reduce the end resistance of the thin-walled model, and the resistance will not continue to decrease with the increase in cycle times. For the thick-walled model, this strategy has no drag-reduction effect, and the resistance will grow with the increase in cycle times. The pressure-pull-out loading method is suitable for the thin-walled foundation, but it is not recommended for thick-walled foundations.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zheng, H.; Du, W.; Li, Y. Current situation of the offshore wind power development all over the world. Hydropower New Energy 2018, 6, 75–77. [Google Scholar]
- Lian, J.; Jiang, Q.; Dong, X.; Zhao, Y. Dynamic impedance of the wide-shallow bucket foundation for offshore wind turbine using coupled finite–infinite element method. Energies 2019, 12, 4370. [Google Scholar] [CrossRef] [Green Version]
- Wu, X.; Hu, Y.; Li, Y. Foundations of offshore wind turbines: A review. Renew. Sustain. Energy Rev. 2019, 104, 379–393. [Google Scholar] [CrossRef] [Green Version]
- Tabassum, A.; Premalatha, M.; Abbasi, T.; Abbasi, S.A. Wind energy: Increasing deployment, rising environmental concerns. Renew. Sustain. Energy Rev. 2014, 31, 270–288. [Google Scholar] [CrossRef]
- Oh, K.; Nam, W.; Ryu, M. A review of foundations of offshore wind energy convertors: Current status and future perspectives. Renew. Sustain. Energy Rev. 2018, 88, 16–36. [Google Scholar] [CrossRef]
- Liu, X. Study on Design and Security of Large-Diameter and Wide-Shallow Bucket Foundation for Offshore Wind Power. Ph.D. Thesis, Tianjin University, Tianjin, China, 2010. [Google Scholar]
- Jijian, L.; Hongyan, D.; Puyang, Z. Design of large-scale prestressing bucket foundation for offshore wind turbines. Trans. Tianjin Univ. 2010, 18, 79–84. [Google Scholar]
- Jia, N.; Zhang, P.; Liu, Y. Bearing capacity of composite bucket foundations for offshore wind turbines in silty sand. Ocean Eng. 2018, 151, 1–11. [Google Scholar] [CrossRef]
- Lian, J.; Sun, L.; Zhang, J. Bearing capacity and technical advantages of composite bucket foundation of offshore wind turbines. Trans. Tianjin Univ. 2011, 17, 132–137. [Google Scholar] [CrossRef]
- Liu, R.; Chen, G.; Lian, J. Vertical bearing behaviour of the composite bucket shallow foundation of offshore wind turbines. J. Renew. Sustain. Energy. 2015, 7, 013123. [Google Scholar] [CrossRef]
- Zhang, P.; Ding, H.; Le, C. Towing characteristics of large-scale composite bucket foundation for offshore wind turbines. J. Southeast Univ. Engl. Ed. 2013, 29, 300–304. [Google Scholar]
- Lian, J.; Chen, F.; Yang, X. Suction installation and leveling of composite bucket foundation for offshore wind turbines. J. Tianjin Univ. 2014, 47, 987–993. [Google Scholar]
- Lian, J.; Chen, F.; Wang, H. Laboratory tests on soil-skirt interaction and penetration resistance of suction caissons during installation in sand. Ocean Eng. 2014, 84, 1–13. [Google Scholar] [CrossRef]
- Tran, M.N.; Randolph, M.F. Variation of suction pressure during caisson installation in sand. Géotechnique 2008, 58, 1–11. [Google Scholar] [CrossRef]
- Tran, M.N.; Randolph, M.F.; David, W.A. Study of Seepage Flow and Sand Plug Loosening in Installation of Suction Caissons in Sand. In Proceedings of the 15th International Offshore and Polar Engineering Conference, Seoul, Korea, 19–24 June 2005; pp. 516–521. [Google Scholar]
- Tran, M.N. Installation of Suction Caisson in Dense Sand and the Influence of Silt and Cemented Layers. Ph.D. Thesis, Department of Civil Engineering, University of Sydney, Sydney, Australia, 2005. [Google Scholar]
- Zhu, B.; Kong, D.; Tong, J. Model tests on penetration and pullout of suction caissons in silt. Chin. J. Geotech. Eng. 2011, 33, 1045–1053. [Google Scholar]
- Houlsby, G.T.; Byrne, B.W. Design procedures for installation of suction caissons in clay and other materials. Geotech. Eng. 2005, 158, 75–82. [Google Scholar] [CrossRef]
- Cao, J.; Phillips, R.; Popescu, R. Penetration Resistance of Suction Caissons in Clay. In Proceedings of the 12th International Offshore and Polar Engineering Conference, Kitakyushu, Japan, 26−31 May 2002; pp. 800–806. [Google Scholar]
- Lian, J.; Ma, Y.; Wang, H. Experimental study on static pressure sedimentation for bucket foundation in silty clay. Rock Soil Mech. 2017, 38, 1856–1862. [Google Scholar]
- Liu, R.; Qi, Y.; Lian, J. Experimental study on static pressure penetration of bucket foundation in cohesionless soil. Shuili Xuebao. 2016, 47, 1473–1483. [Google Scholar]
- Ma, W.; Liu, R.; Lian, J. The research on penetration resistance of bucket foundation in silt. Rock Soil Mech. 2018, 40, 1307–1312. [Google Scholar]
- Chen, F.; Lian, J.; Ma, Y. Model tests on bucket-soil interaction during installation of bucket foundation in silt sand. Chin. J. Geotech. Eng. 2015, 37, 683–691. [Google Scholar]
- Andersen, K.H.; Jostad, H.P. Foundation design of skirted foundations and anchors in clay. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 3–6 May 1999. [Google Scholar]
- Doherty, P.; Gavin, K. Shaft capacity of open-ended piles in clay. J. Geotech. Geoenviron. Eng. 2011, 137, 1090–1102. [Google Scholar] [CrossRef] [Green Version]
- American Petroleum Institute. Recommended Practice for Geotechnical and Foundation Design Considerations, 1st ed.; American Petroleum Institute: Washington, DC, USA, 2011. [Google Scholar]
- DET Norske Veritas. Foundations; Det Norske Veritas: Høvik, Norway, 1992. [Google Scholar]
- Wei, W. Research on the Influence of Wall Deformation on the Penetration Resistance in Sand of Bucket Foundation. Master’s Thesis, Tianjin University, Tianjin, China, 2017. [Google Scholar]
- Andersen, K.H.; Jostad, H.P.; Dyvik, R. Penetration resistance of offshore skirted foundations and anchors in dense sand. J. Geotech. Geoenviron. Eng. 2008, 134, 106–116. [Google Scholar] [CrossRef]
- Wang, X.F.; Zeng, X.W.; Li, J.L. Vertical performance of suction bucket foundation for offshore wind turbines in sand. Ocean Eng. 2019, 180, 40–48. [Google Scholar] [CrossRef] [Green Version]
- Chen, F. Research on the Soil-Skirt Interaction of Suction Buckets for Wind Turbines During Installation in Sand. Ph.D. Thesis, Tianjin University, Tianjin, China, 2014. [Google Scholar] [CrossRef]





| Prototype Diameter (Height)/m | Prototype Skirt Thickness/m | Scale | Model Diameter (Height)/cm | Model Skirt Thickness/cm |
|---|---|---|---|---|
| 30–10 | 0.025 | 1:30 | 100–33.3 | 0.083 |
| 30–10 | 0.3 | 1:30 | 100–33.3 | 1 |
| 30–10 | 0.025 | 1:20 | 150–50 | 0.125 |
| 30–10 | 0.3 | 1:20 | 150–50 | 1.5 |
| Particles (mm) | 2–1 | 1–0.5 | 0.5–0.25 | 0.25–0.075 | <0.075 |
|---|---|---|---|---|---|
| Content (%) | 3.1 | 20 | 53.3 | 22.4 | 1.2 |
| Cumulative content (%) | 100 | 96.9 | 76.9 | 23.6 | 1.2 |
| Saturated Density (g/cm3) | Specific Gravity | Relative Density | Void Ratio | Compression Modulus (MPa) | Cohesion (kPa) | Internal Friction Angle |
|---|---|---|---|---|---|---|
| 1.89 | 2.69 | 0.63 | 0.665 | 20.06 | 0.3 | 31.9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, H.; Zheng, H.; Lian, J. Experimental Study on Static Pressure Sedimentation for a Thick-Walled Bucket Foundation in Sand. Energies 2022, 15, 5786. https://doi.org/10.3390/en15165786
Zhao H, Zheng H, Lian J. Experimental Study on Static Pressure Sedimentation for a Thick-Walled Bucket Foundation in Sand. Energies. 2022; 15(16):5786. https://doi.org/10.3390/en15165786
Chicago/Turabian StyleZhao, Hao, Hongjie Zheng, and Jijian Lian. 2022. "Experimental Study on Static Pressure Sedimentation for a Thick-Walled Bucket Foundation in Sand" Energies 15, no. 16: 5786. https://doi.org/10.3390/en15165786
APA StyleZhao, H., Zheng, H., & Lian, J. (2022). Experimental Study on Static Pressure Sedimentation for a Thick-Walled Bucket Foundation in Sand. Energies, 15(16), 5786. https://doi.org/10.3390/en15165786






