Performance Analysis and Optimization of a Cooling System for Hybrid Solar Panels Based on Climatic Conditions of Islamabad, Pakistan
Abstract
:1. Introduction
2. Problem Description and Methodology
3. Mathematical Modeling
3.1. Analytical Model of a PV Panel
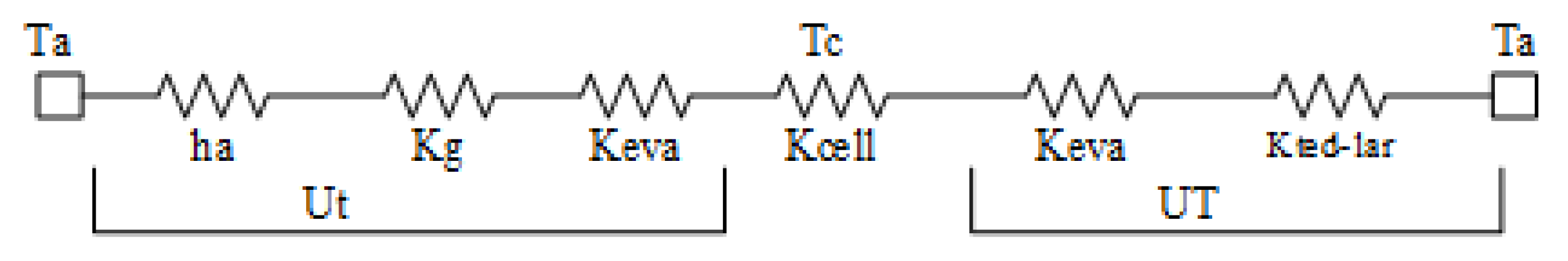
3.1.1. Temperature Modeling
3.1.2. Irradiance Modeling
3.2. Design of Absorber: Hybrid PV Panel
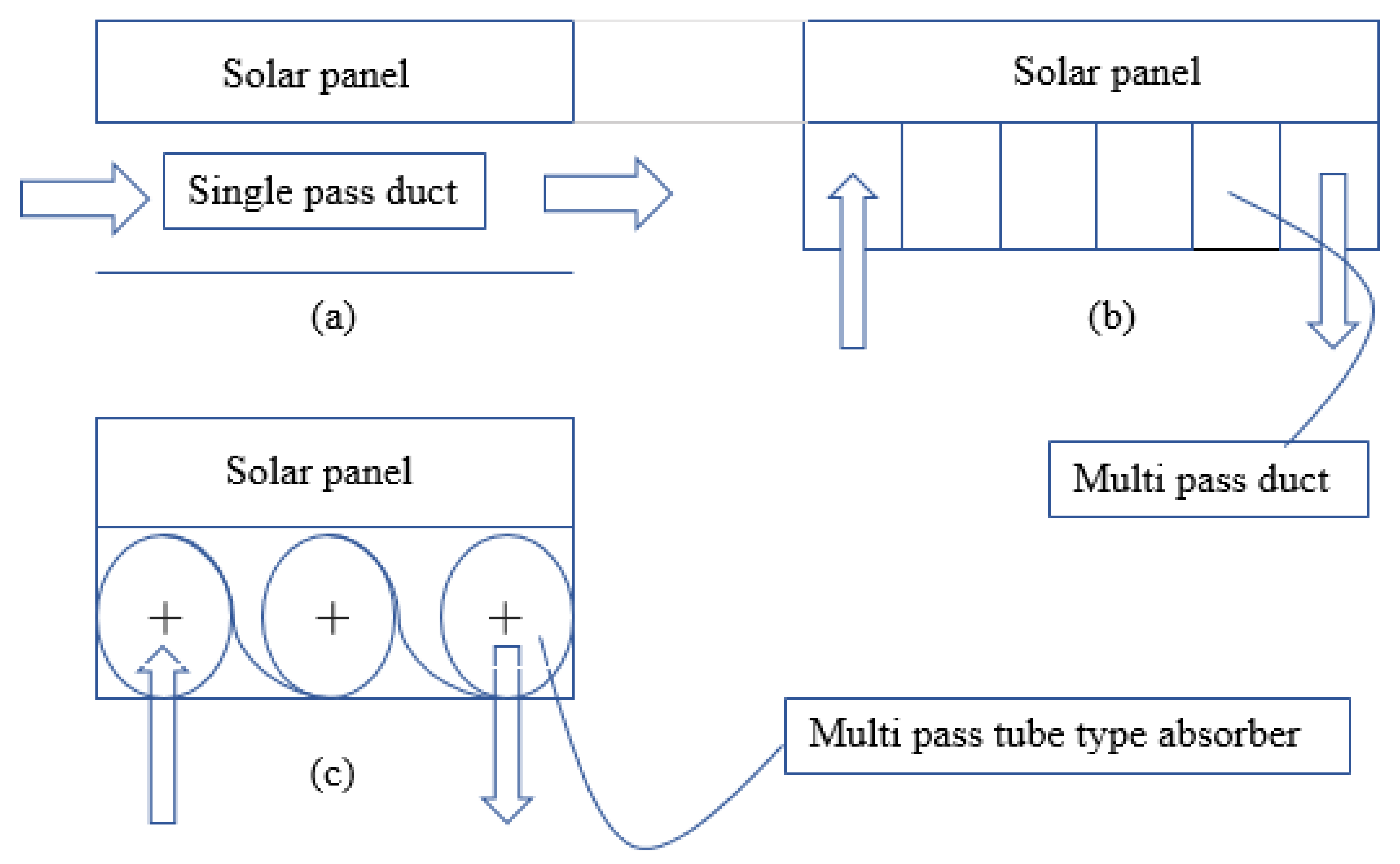
3.2.1. Single-Pass Duct
3.2.2. Multi-Pass Duct
3.2.3. Tube-Type Absorber
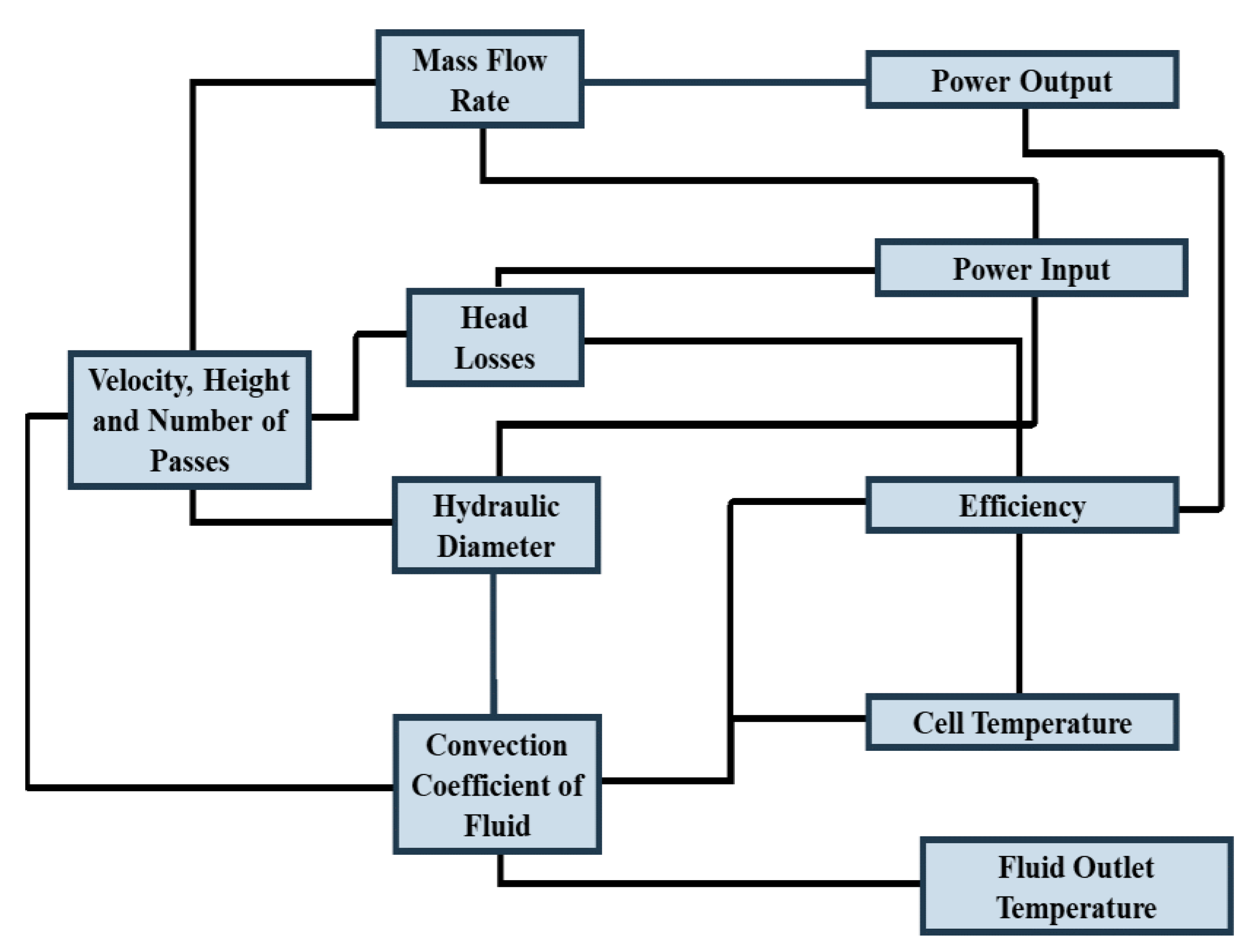
4. Optimization
- (i)
- Expressions for Duct-Type Absorber
- (ii)
- Expressions for Multi-Pass Duct
- (iii)
- Expressions for Tube-Type Absorber
4.1. Genetic Algorithm
4.2. Comparative Analysis for Different Types of Absorbers
4.3. Energy Produced by Hybrid Multi-Pass PV and Uncooled PV Module
5. Validation of Analytical Results through Virtual Experiments
5.1. Simulation Analysis of the PV Module without Cooling
5.2. Simulation Analysis of Hybrid PV Panel with a Multi-Pass Duct
6. Conclusions
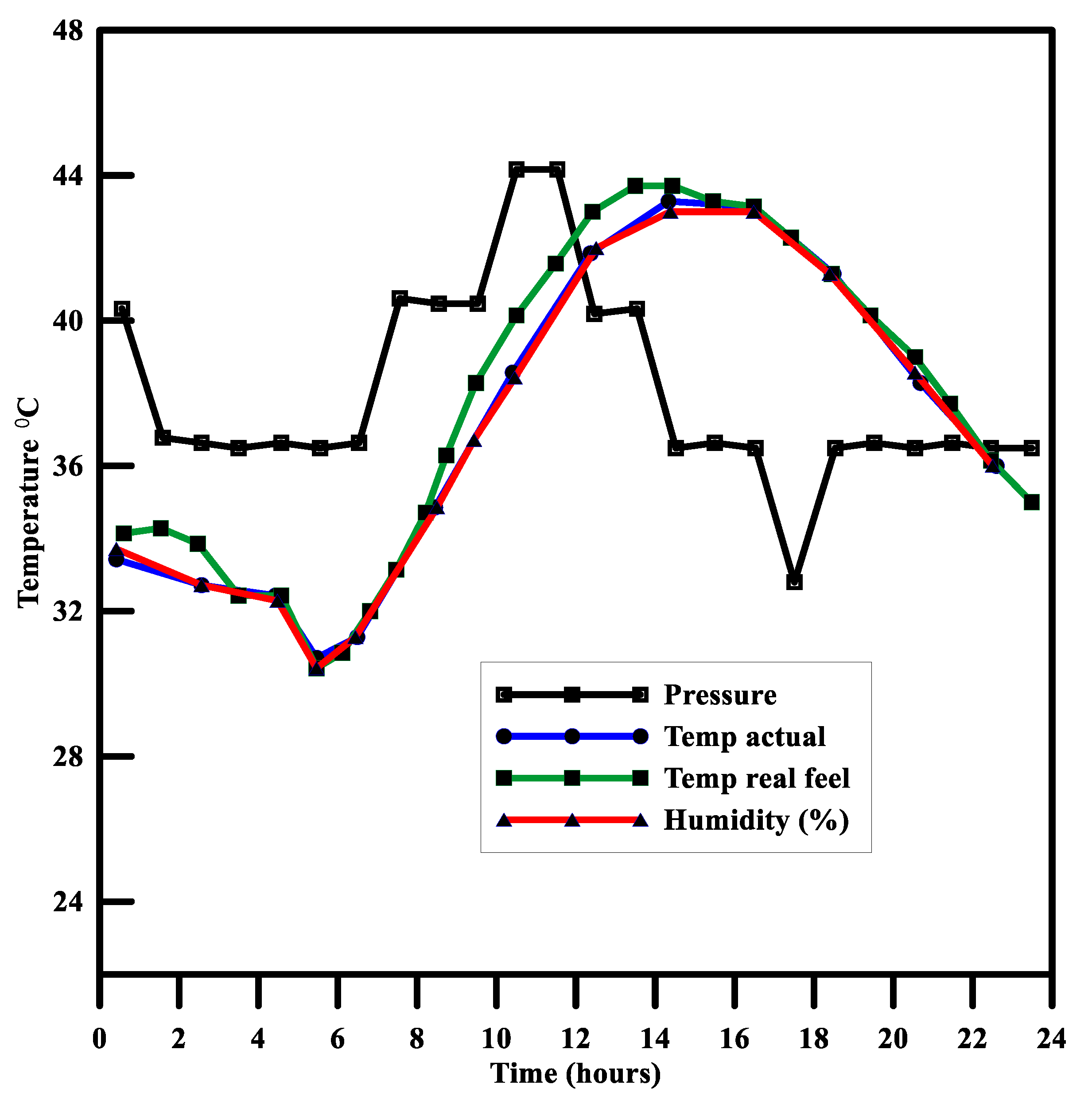
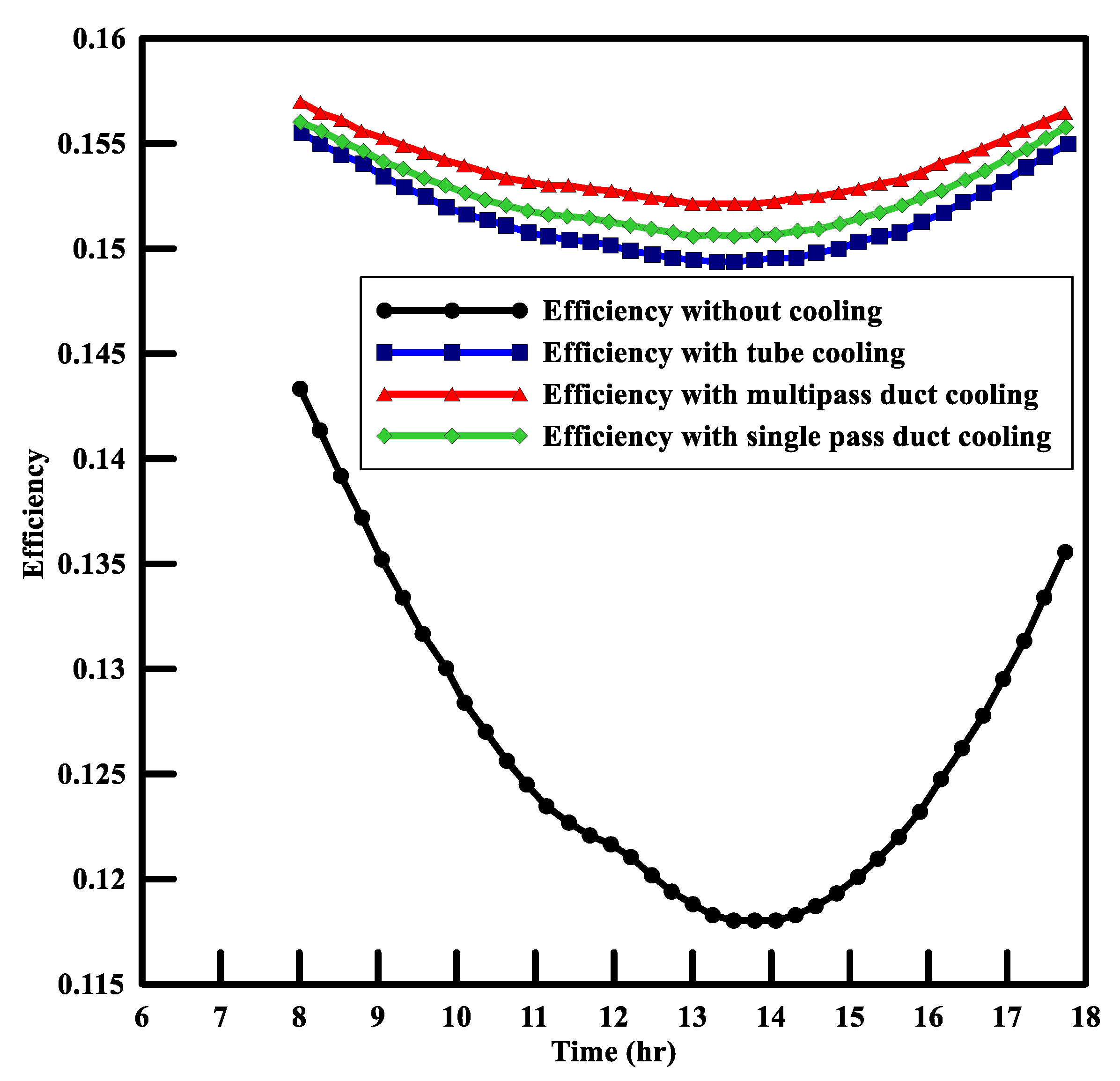
- PV and PV/T modules were analytically modeled using irradiance and ambient air conditions of Islamabad in June. For climate modeling, Erbs, Liu, and Jordan’s model and the sin linking day’s model were used. The optimum angle for solar panel tilt is 23°. Boundary conditions obtained by these models were incorporated in the analytical model, which was then linked with the optimization algorithm. According to analytical calculations, the hybrid system can produce about 0.15 kWh more energy than the ordinary system in June. Results clarify that cooling enhances the net output of the panel by reducing the effect of efficiency loss due to temperature.
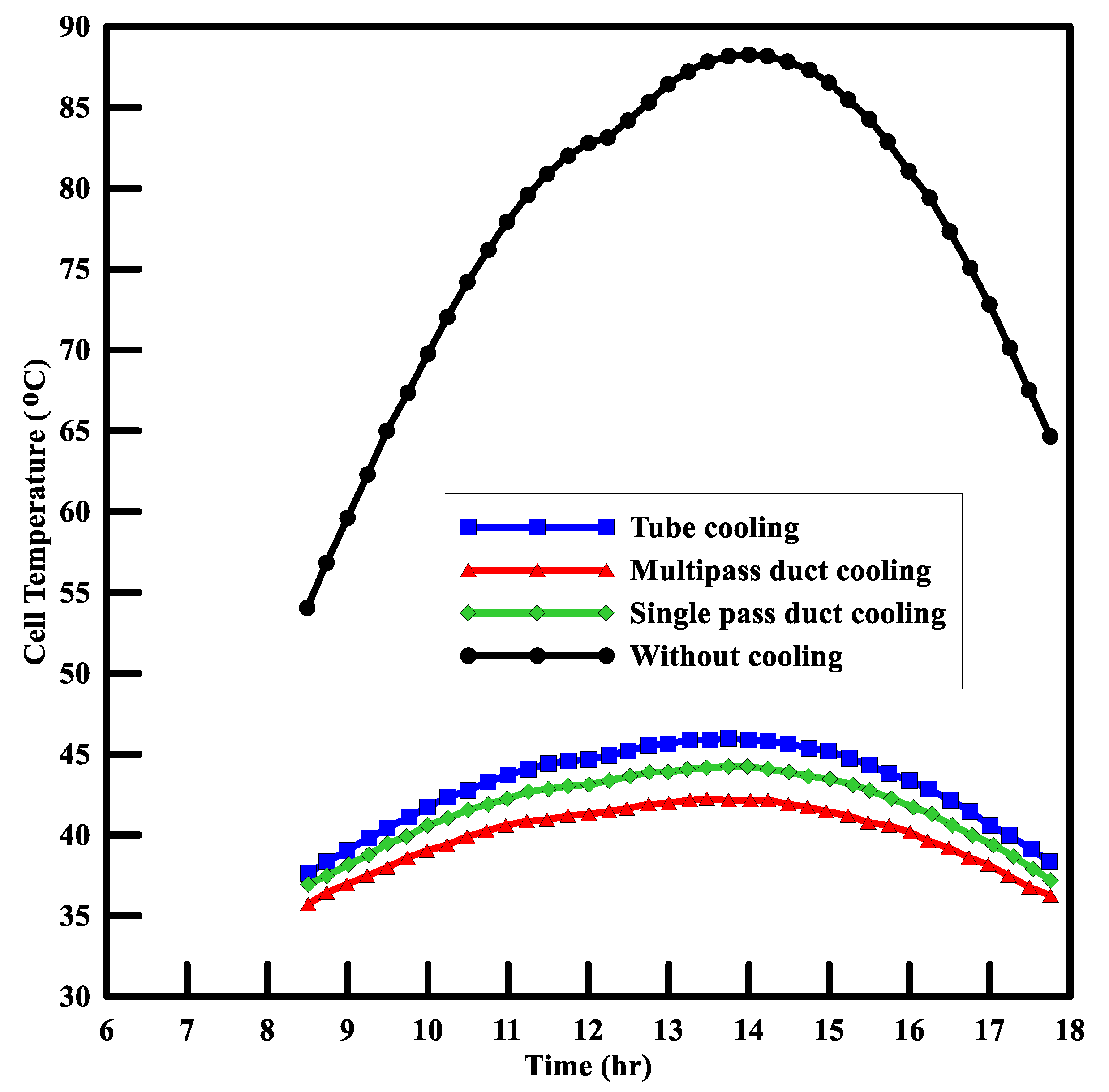
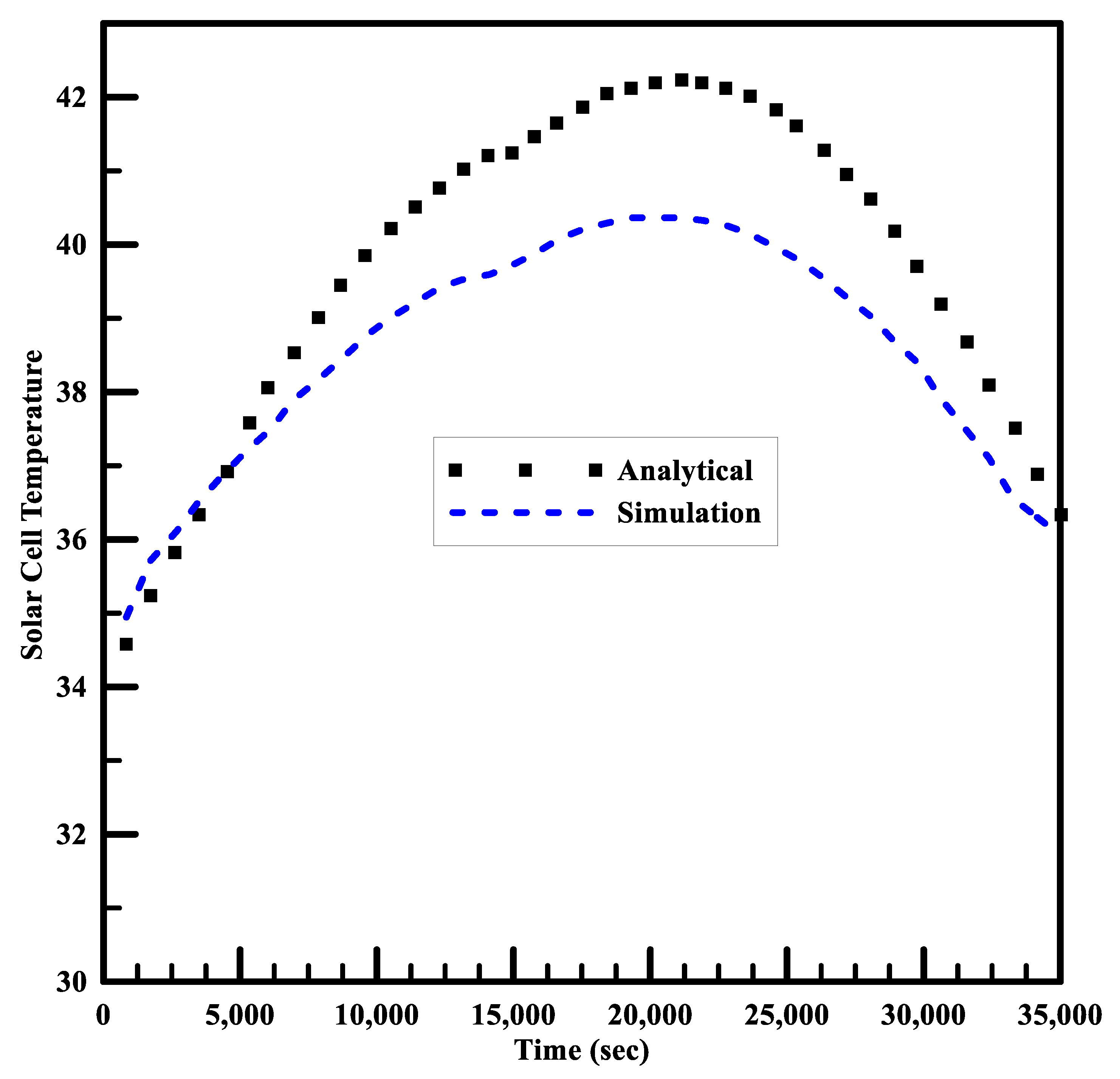
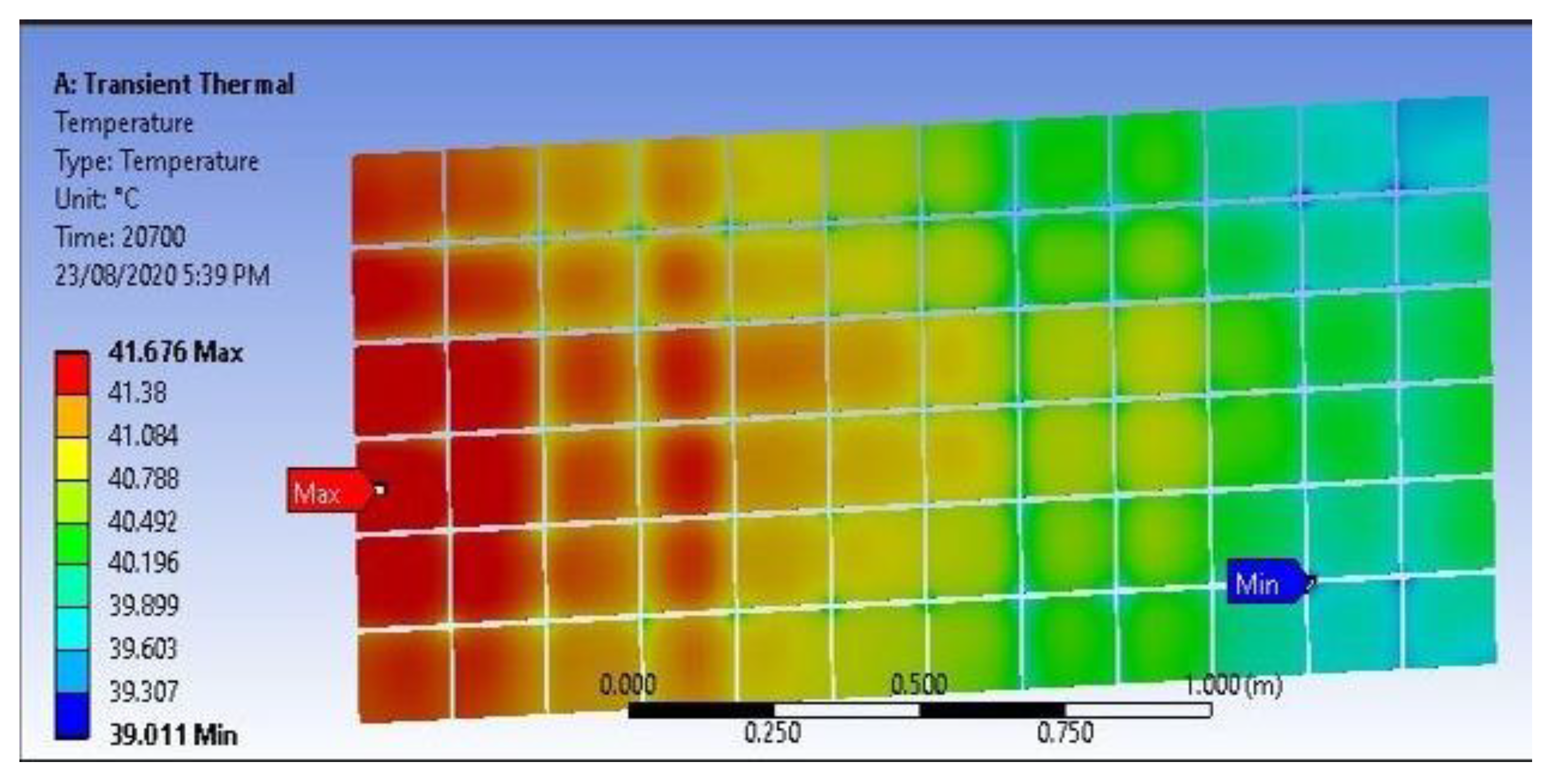
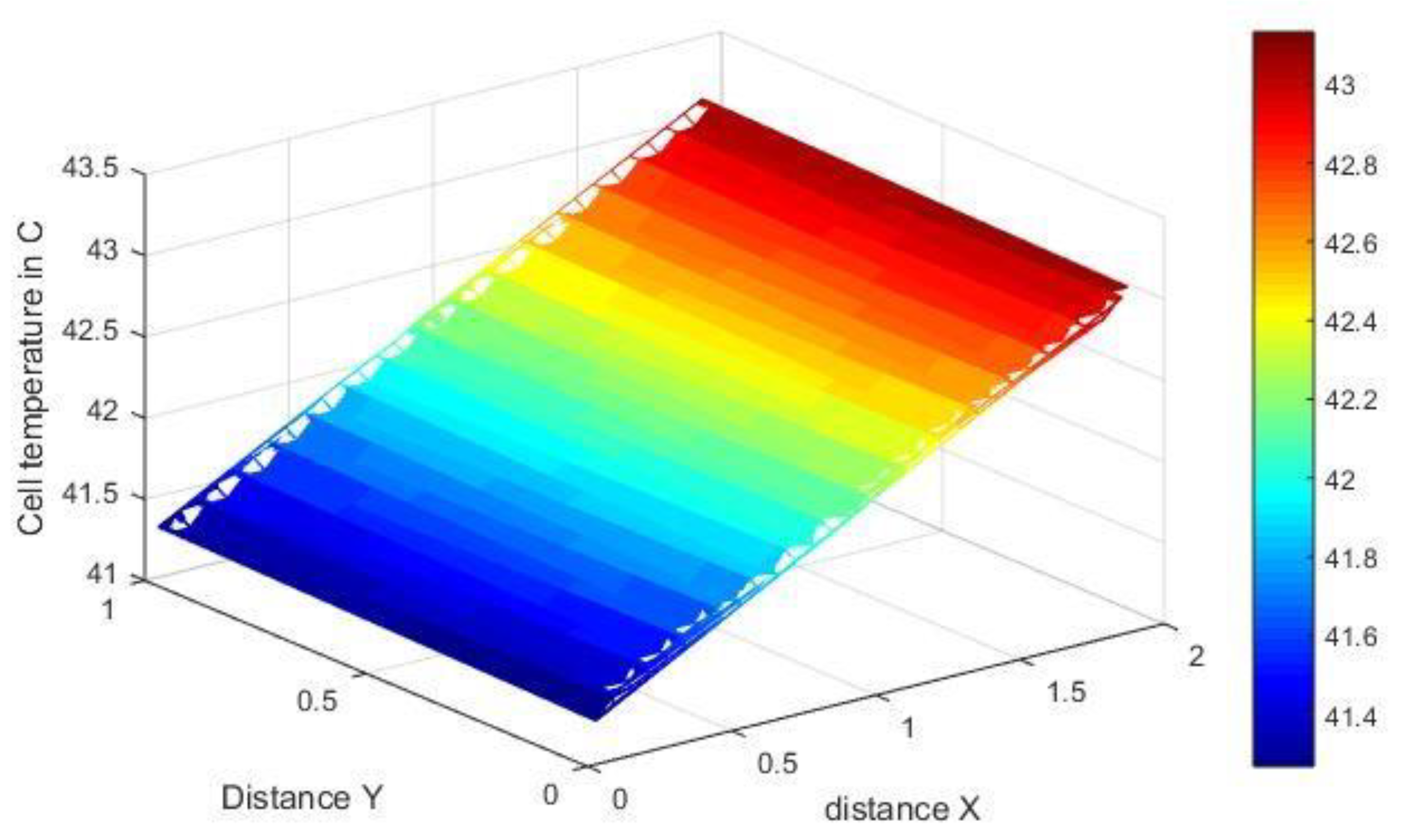
- The analytical results were validated using fluent and thermal modules in ANSYS. The fluid flow regime is considered in the transition domain close to turbulent flow. Good agreement between analytical and numerical results for maximum cell temperature of PV panel was observed. The efficiency of the solar panel was directly dependent on the temperature of the cell; thus, the numerical results verified the efficiency of temperature behavior for a multi-pass hybrid solar panel.
- A comparison of PV and hybrid PV panels was carried out considering the power required by a commercial pump that provides the same head and flow rate of the fluid. In a multi-pass absorber, the inlet width was also varied as the number of passes was changed. Due to these facts, the multi-pass absorber can have relatively higher velocities of fluid at lower head losses. The cell temperature is also reduced as higher velocities increase the heat transfer coefficient.
- Absorbers of different types were modeled and compared. The hybrid solar panel with a multi-pass duct system was compared with a PV system without cooling. In March, the output of both the systems is almost equal, but the difference in output increases as ambient temperature increases. The trends and temperature behavior of the PV module and designed Hybrid PV module were verified by ANSYS simulations. However, the optimized result was achieved for the multi-pass duct with 31 passes that delivers a maximum power output of 186.713 W at a mass flow rate of 0.14 kg/s. The maximum cell temperature achieved for this configuration was 37.810 °C at a velocity of 0.092 m/s.
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| I | incident radiation |
| transmissivity of the glass cover | |
| efficiency | |
| αc | absorptivity of solar panel |
| β | packing factor |
| αt | absorptivity of tedlar |
| STC | standard test Conditions |
| PV | photovoltaic |
| Ta | ambient temperature |
| Tc | cell temperature |
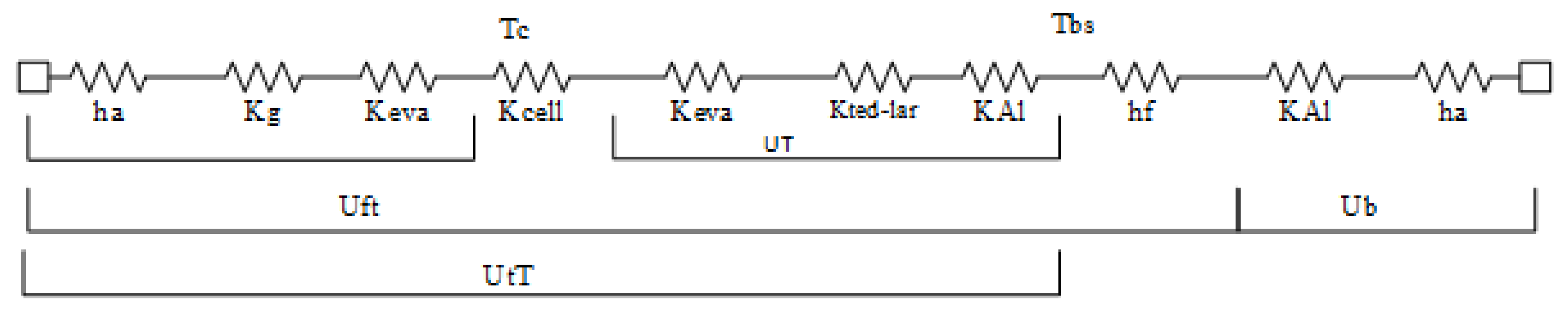
| Ut | Overall heat transfer coefficient from PV to ambient from the top of the panel through glass |
| UT | overall heat transfer coefficient from PV to ambient from the bottom of panel trough tedlar |
| Io | hourly extraterrestrial radiation |
| Γ | day angle |
| ω | hour angle |
| ST | local solar time |
| LT | local standard time |
| Ls | standard meridian |
| LL | longitude of the location |
| Mt | clearness index |
| β | tilt angle |
| Iββ | total Irradiance on the tilted surface |
| Tbs | temperature back surface |
| b | breath |
| dx | element length |
| hf | heat transfer coefficient of fluid |
| Tf-avg | average temperature of fluid |
| Cp | specific heat |
| Ub | an overall heat transfer coefficient from bottom to ambient |
| hp1 | penalty factor |
| hp2 | penalty factor |
| UTt | Overall Heat transfer Coefficient from Tedlar to Ambient from the top of the panel across glass |
| Dh | hydraulic Diameter |
| k | conduction coefficient |
| Ub | overall heat transfer coefficient to ambient from the back side of absorber |
| UL | an overall heat transfer coefficient from solar cell to ambient through top and back surface of insulation |
| L | length |
| m | mass |
| Tf-out | fluid outlet temperature |
| Tf-in | fluid inlet temperature |
| P | pressure |
| Utf | overall heat transfer coefficient from fluid to ambient from the top of the panel through glass |
| Subscripts | |
| A | Ambient Air |
| f | Fluid |
| t | Tedlar |
| g | Glass |
| bs | Back surface |
| c | Solar Cell |
References
- Awda, L.; Khalaf, Y.; Salih, S. Analysis of temperature effect on a crystalline silicon photovoltaic module performance. Int. J. Eng. 2016, 29, 722–727. [Google Scholar]
- Kandeal, A.; Thakur, A.K.; Elkadeem, M.; Elmorshedy, M.F.; Ullah, Z.; Sathyamurthy, R.; Sharshir, S.W. Photovoltaics performance improvement using different cooling methodologies: A state-of-art review. J. Clean. Prod. 2020, 273, 122772. [Google Scholar] [CrossRef]
- Osma-Pinto, G.; Ordóñez-Plata, G. Dynamic thermal modelling for the prediction of the operating temperature of a PV panel with an integrated cooling system. Renew. Energy 2020, 152, 1041–1054. [Google Scholar] [CrossRef]
- Idoko, L.; Anaya-Lara, O.; McDonald, A. Enhancing PV modules efficiency and power output using multi-concept cooling technique. Energy Rep. 2018, 4, 357–369. [Google Scholar] [CrossRef]
- Prudhvi, P.; Sai, P.C. Efficiency improvement of solar PV panels using active cooling. In Proceedings of the 2012 11th International Conference on Environment and Electrical Engineering, Venice, Italy, 18–25 May 2012; pp. 1093–1097. [Google Scholar]
- Hasan, M.A.; Sumathy, K. Photovoltaic thermal module concepts and their performance analysis: A review. Renew. Sustain. Energy Rev. 2010, 14, 1845–1859. [Google Scholar] [CrossRef]
- Sargunanathan, S.; Elango, A.; Mohideen, S.T. Performance enhancement of solar photovoltaic cells using effective cooling methods: A review. Renew. Sustain. Energy Rev. 2016, 64, 382–393. [Google Scholar] [CrossRef]
- Al-Waeli, A.H.; Sopian, K.; Kazem, H.A.; Chaichan, M.T. Photovoltaic/Thermal (PV/T) systems: Status and future prospects. Renew. Sustain. Energy Rev. 2017, 77, 109–130. [Google Scholar] [CrossRef]
- García, M.A.; Balenzategui, J. Estimation of photovoltaic module yearly temperature and performance based on nominal operation cell temperature calculations. Renew. Energy 2004, 29, 1997–2010. [Google Scholar] [CrossRef]
- Kurnik, J.; Jankovec, M.; Brecl, K.; Topic, M. Outdoor testing of PV module temperature and performance under different mounting and operational conditions. Sol. Energy Mater. Sol. Cells 2011, 95, 373–376. [Google Scholar] [CrossRef]
- Cuce, E.; Cuce, P.M.; Karakas, I.H.; Bali, T. An accurate model for photovoltaic (PV) modules to determine electrical characteristics and thermodynamic performance parameters. Energy Convers. Manag. 2017, 146, 205–216. [Google Scholar] [CrossRef]
- Bayrak, F.; Oztop, H.F.; Selimefendigil, F. Experimental study for the application of different cooling techniques in photovoltaic (PV) panels. Energy Convers. Manag. 2020, 212, 112789. [Google Scholar] [CrossRef]
- Shahsavar, A.; Ameri, M.; Gholampour, M. Energy and exergy analysis of a photovoltaic-thermal collector with natural air flow. J. Sol. Energy Eng. 2012, 134, 011014. [Google Scholar] [CrossRef]
- Dwivedi, P.; Sudhakar, K.; Soni, A.; Solomin, E.; Kirpichnikova, I. Advanced cooling techniques of PV modules: A state of art. Case Stud. Therm. Eng. 2020, 21, 100674. [Google Scholar] [CrossRef]
- Tonui, J.; Tripanagnostopoulos, Y. Improved PV/T solar collectors with heat extraction by forced or natural air circulation. Renew. Energy 2007, 32, 623–637. [Google Scholar] [CrossRef]
- Dubey, S.; Solanki, S.; Tiwari, A. Energy and exergy analysis of PV/T air collectors connected in series. Energy Build. 2009, 41, 863–870. [Google Scholar] [CrossRef]
- Joshi, A.; Tiwari, A.; Tiwari, G.; Dincer, I.; Reddy, B. Performance evaluation of a hybrid photovoltaic thermal (PV/T)(glass-to-glass) system. Int. J. Therm. Sci. 2009, 48, 154–164. [Google Scholar] [CrossRef]
- Othman, M.Y.; Yatim, B.; Sopian, K.; Bakar, M.N.A. Performance studies on a finned double-pass photovoltaic-thermal (PV/T) solar collector. Desalination 2007, 209, 43–49. [Google Scholar] [CrossRef]
- Sarhaddi, F.; Farahat, S.; Ajam, H.; Behzadmehr, A.; Adeli, M.M. An improved thermal and electrical model for a solar photovoltaic thermal (PV/T) air collector. Appl. Energy 2010, 87, 2328–2339. [Google Scholar] [CrossRef]
- Saygin, H.; Nowzari, R.; Mirzaei, N.; Aldabbagh, L. Performance evaluation of a modified PV/T solar collector: A case study in design and analysis of experiment. Sol. Energy 2017, 141, 210–221. [Google Scholar] [CrossRef]
- Mojumder, J.C.; Chong, W.T.; Ong, H.C.; Leong, K. An experimental investigation on performance analysis of air type photovoltaic thermal collector system integrated with cooling fins design. Energy Build. 2016, 130, 272–285. [Google Scholar] [CrossRef]
- Benato, A.; Stoppato, A.; De Vanna, F.; Schiro, F. Spraying Cooling System for PV Modules: Experimental Measurements for Temperature Trends Assessment and System Design Feasibility. Designs 2021, 5, 25. [Google Scholar] [CrossRef]
- Sharma, R.; Gupta, A.; Nandan, G.; Dwivedi, G.; Kumar, S. Life span and overall performance enhancement of solar photovoltaic cell using water as coolant: A recent review. Mater. Today Proc. 2018, 5, 18202–18210. [Google Scholar] [CrossRef]
- Silva, V.; Martinez, J.; Heideier, R.; Bernal, J.; Gimenes, A.; Udaeta, M.; Saidel, M. A Long-Term Analysis of the Architecture and Operation of Water Film Cooling System for Commercial PV Modules. Energies 2021, 14, 1515. [Google Scholar] [CrossRef]
- Bahaidarah, H.; Subhan, A.; Gandhidasan, P.; Rehman, S. Performance evaluation of a PV (photovoltaic) module by back surface water cooling for hot climatic conditions. Energy 2013, 59, 445–453. [Google Scholar] [CrossRef]
- Mah, C.-Y.; Lim, B.-H.; Wong, C.-W.; Tan, M.-H.; Chong, K.-K.; Lai, A.-C. Investigating the performance improvement of a photovoltaic system in a tropical climate using water cooling method. Energy Procedia 2019, 159, 78–83. [Google Scholar] [CrossRef]
- Nižetić, S.; Čoko, D.; Yadav, A.; Grubišić-Čabo, F. Water spray cooling technique applied on a photovoltaic panel: The performance response. Energy Convers. Manag. 2016, 108, 287–296. [Google Scholar]
- Tripanagnostopoulos, Y.; Nousia, T.; Souliotis, M.; Yianoulis, P. Hybrid photovoltaic/thermal solar systems. Sol. Energy 2002, 72, 217–234. [Google Scholar] [CrossRef]
- Fudholi, A.; Sopian, K.; Yazdi, M.H.; Ruslan, M.H.; Ibrahim, A.; Kazem, H.A. Performance analysis of photovoltaic thermal (PVT) water collectors. Energy Convers. Manag. 2014, 78, 641–651. [Google Scholar] [CrossRef]
- Yazdanifard, F.; Ebrahimnia-Bajestan, E.; Ameri, M. Investigating the performance of a water-based photovoltaic/thermal (PV/T) collector in laminar and turbulent flow regime. Renew. Energy 2016, 99, 295–306. [Google Scholar] [CrossRef]
- Al-Amri, F.; Maatallah, T.S.; Al-Amri, O.F.; Ali, S.; Ali, S.; Ateeq, I.S.; Zachariah, R.; Kayed, T.S. Innovative technique for achieving uniform temperatures across solar panels using heat pipes and liquid immersion cooling in the harsh climate in the Kingdom of Saudi Arabia. Alex. Eng. J. 2022, 61, 1413–1424. [Google Scholar] [CrossRef]
- Han, X.; Guo, Y.; Wang, Q.; Phelan, P. Optical characterization and durability of immersion cooling liquids for high concentration III-V photovoltaic systems. Sol. Energy Mater. Sol. Cells 2018, 174, 124–131. [Google Scholar] [CrossRef]
- Mehrotra, S.; Rawat, P.; Debbarma, M.; Sudhakar, K. Performance of a solar panel with water immersion cooling technique. Int. J. Sci. Environ. Technol. 2014, 3, 1161–1172. [Google Scholar]
- Abdolzadeh, M.; Ameri, M. Improving the effectiveness of a photovoltaic water pumping system by spraying water over the front of photovoltaic cells. Renew. Energy 2009, 34, 91–96. [Google Scholar] [CrossRef]
- Tabaei, H.; Ameri, M. Improving the effectiveness of a photovoltaic water pumping system by using booster reflector and cooling array surface by a film of water. Iran. J. Sci. Technol. Trans. Mech. Eng. 2015, 39, 51. [Google Scholar]
- Odeh, S.; Behnia, M. Improving photovoltaic module efficiency using water cooling. Heat Transf. Eng. 2009, 30, 499–505. [Google Scholar] [CrossRef]
- Figaj, R.; Żołądek, M. Operation and Performance Assessment of a Hybrid Solar Heating and Cooling System for Different Configurations and Climatic Conditions. Energies 2021, 14, 1142. [Google Scholar] [CrossRef]
- Figaj, R.; Żołądek, M. Experimental and numerical analysis of hybrid solar heating and cooling system for a residential user. Renew. Energy 2021, 172, 955–967. [Google Scholar] [CrossRef]
- Gado, M.G.; Megahed, T.F.; Ookawara, S.; Nada, S.; El-Sharkawy, I.I. Performance and economic analysis of solar-powered adsorption-based hybrid cooling systems. Energy Convers. Manag. 2021, 238, 114134. [Google Scholar] [CrossRef]
- Ziapour, B.M.; Palideh, V.; Mohammadnia, A. Study of an improved integrated collector-storage solar water heater combined with the photovoltaic cells. Energy Convers. Manag. 2014, 86, 587–594. [Google Scholar] [CrossRef]
- Tiwari, A.; Sodha, M.; Chandra, A.; Joshi, J. Performance evaluation of photovoltaic thermal solar air collector for composite climate of India. Sol. Energy Mater. Sol. Cells 2006, 90, 175–189. [Google Scholar] [CrossRef]
- Chow, D.; Levermore, G.J. New algorithm for generating hourly temperature values using daily maximum, minimum and average values from climate models. Build. Serv. Eng. Res. Technol. 2007, 28, 237–248. [Google Scholar] [CrossRef]
- Kalogirou, S.A. Environmental characteristics. In Solar Energy Engineering; Elsevier: Amsterdam, The Netherlands, 2009; pp. 49–120. [Google Scholar]
- Mousavi Maleki, S.A.; Hizam, H.; Gomes, C. Estimation of hourly, daily and monthly global solar radiation on inclined surfaces: Models re-visited. Energies 2017, 10, 134. [Google Scholar] [CrossRef]
- Erbs, D.; Klein, S.; Duffie, J. Estimation of the diffuse radiation fraction for hourly, daily and monthly-average global radiation. Sol. Energy 1982, 28, 293–302. [Google Scholar] [CrossRef]
- Liu, B.; Jordan, R. Daily insolation on surfaces tilted towards equator. ASHRAE J. 1961, 10, 526–541. [Google Scholar]
- Rao, S.S. Engineering Optimization: Theory and Practice; John Wiley & Sons: Hoboken, NJ, USA, 2019. [Google Scholar]



| Length (m) | Width (m) | Efficiency at STC | Max Power (W) | Temp Coefficient of Pmax |
|---|---|---|---|---|
| 1.956 | 0.992 | 16.5 | 320 | 0.45%/°C |
| Variable/Parameter | Value |
|---|---|
| Fill Factor of PV module | 0.83 |
| Coefficient of Convection of Air (W/m2K) | 6.5 |
| Thickness of Glass (m) | 0.0032 |
| Thickness of Tedlar (m) | 0.0005 |
| Thickness of EVA (m) | 0.0005 |
| Conductivity of Tedlar (W/(mK)) | 0.033 |
| Conductivity of EVA (W/(mK)) | 0.23 |
| Conductivity of Glass (W/(mK)) | 1 |
| Conductivity of Solar Cell (W/(mK)) | 148 |
| Thickness of Cell (m) | 0.00035 |
| Duct and tube material | Aluminium |
| Aluminium Thickness for Duct Walls (m) | 0.003 |
| Aluminium Conductivity (W/(mK)) | 205 |
| Tube Dimensions | 15 × 25 (sq.mm) |
| Absorptivity of Ted-lar | 0.50 |
| Absorptivity of Solar Cell | 0.90 |
| Transmissibility of Glass | 0.95 |
| Diameter of External Circuit pipe (mm) | 0.0142 |
| Fluid Inlet Temperature (°C) | 0.8 times the maximum Ambient temperature of the Month |
| Tube Type | Passes | Velocity (m/s) | Tc Max (°C) | Mass Flow Rate (kg/s) | Power Input (W) | Max Power Output (W) |
| ✓7.00 | 0.28 | 41.94 | 0.11 | 5.18 | 178.97 | |
| 8.00 | 0.26 | 41.64 | 0.10 | 5.48 | 178.94 | |
| 12.00 | 0.18 | 41.75 | 0.07 | 5.50 | 178.82 | |
| 13.00 | 0.16 | 41.91 | 0.06 | 5.40 | 178.78 | |
| Single-pass duct | Height(m) | Velocity (m/s) | Tc Max (°C) | Mass Flow Rate (kg/s) | Power Input (W) | Max Power Output (W) |
| ✓0.012 | 0.009 | 39.985 | 0.114 | 0.878 | 185.027 | |
| 0.012 | 0.006 | 40.699 | 0.069 | 0.499 | 184.767 | |
| 0.016 | 0.007 | 40.835 | 0.110 | 0.843 | 184.301 | |
| 0.018 | 0.006 | 41.380 | 0.103 | 0.776 | 183.879 |
| Multi-Pass Duct | Height (m) | Velocity (m/s) | Passes | Tc Max (°C) | Mass Flow Rate (kg/s) | Power Input (W) | Max Power Output (W) |
| 0.016 | 0.100 | 32.000 | 38.169 | 0.095 | 0.723 | 186.810 | |
| 0.029 | 0.107 | 38.000 | 37.638 | 0.149 | 1.241 | 186.768 | |
| ✓0.025 | 0.092 | 31.000 | 37.810 | 0.140 | 1.141 | 186.713 | |
| 0.024 | 0.064 | 29.000 | 38.534 | 0.098 | 0.739 | 186.468 |
| Month | Monthly Average Temperate (Hi/Low) (°C) | Cooling Water inlet Temperature (°C) | Power Input Commercially Available Pump (W) | Net Energy Produced KWh | |
|---|---|---|---|---|---|
| PV System without Cooling | PV System with Multi-Pass Duct Cooling | ||||
| March | 24/10 | 20 | 10 | 0.99 | 1.01 |
| April | 30/15 | 25 | 10 | 1.148 | 1.23 |
| May | 35/19 | 28 | 10 | 1.227 | 1.35 |
| June | 38/24 | 30 | 10 | 1.2306 | 1.38 |
| July | 34/24 | 28 | 10 | 1.234 | 1.369 |
| August | 33.4/23.5 | 28 | 10 | 1.193 | 1.30 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sattar, M.; Rehman, A.; Ahmad, N.; Mohammad, A.; Al Ahmadi, A.A.; Ullah, N. Performance Analysis and Optimization of a Cooling System for Hybrid Solar Panels Based on Climatic Conditions of Islamabad, Pakistan. Energies 2022, 15, 6278. https://doi.org/10.3390/en15176278
Sattar M, Rehman A, Ahmad N, Mohammad A, Al Ahmadi AA, Ullah N. Performance Analysis and Optimization of a Cooling System for Hybrid Solar Panels Based on Climatic Conditions of Islamabad, Pakistan. Energies. 2022; 15(17):6278. https://doi.org/10.3390/en15176278
Chicago/Turabian StyleSattar, Mariyam, Abdul Rehman, Naseem Ahmad, AlSharef Mohammad, Ahmad Aziz Al Ahmadi, and Nasim Ullah. 2022. "Performance Analysis and Optimization of a Cooling System for Hybrid Solar Panels Based on Climatic Conditions of Islamabad, Pakistan" Energies 15, no. 17: 6278. https://doi.org/10.3390/en15176278
APA StyleSattar, M., Rehman, A., Ahmad, N., Mohammad, A., Al Ahmadi, A. A., & Ullah, N. (2022). Performance Analysis and Optimization of a Cooling System for Hybrid Solar Panels Based on Climatic Conditions of Islamabad, Pakistan. Energies, 15(17), 6278. https://doi.org/10.3390/en15176278





