Simulation Analysis of Ejector Optimization for High Mass Entrainment under the Influence of Multiple Structural Parameters
Abstract
:1. Introduction
2. Numerical Model
3. Simulation Results and Analysis
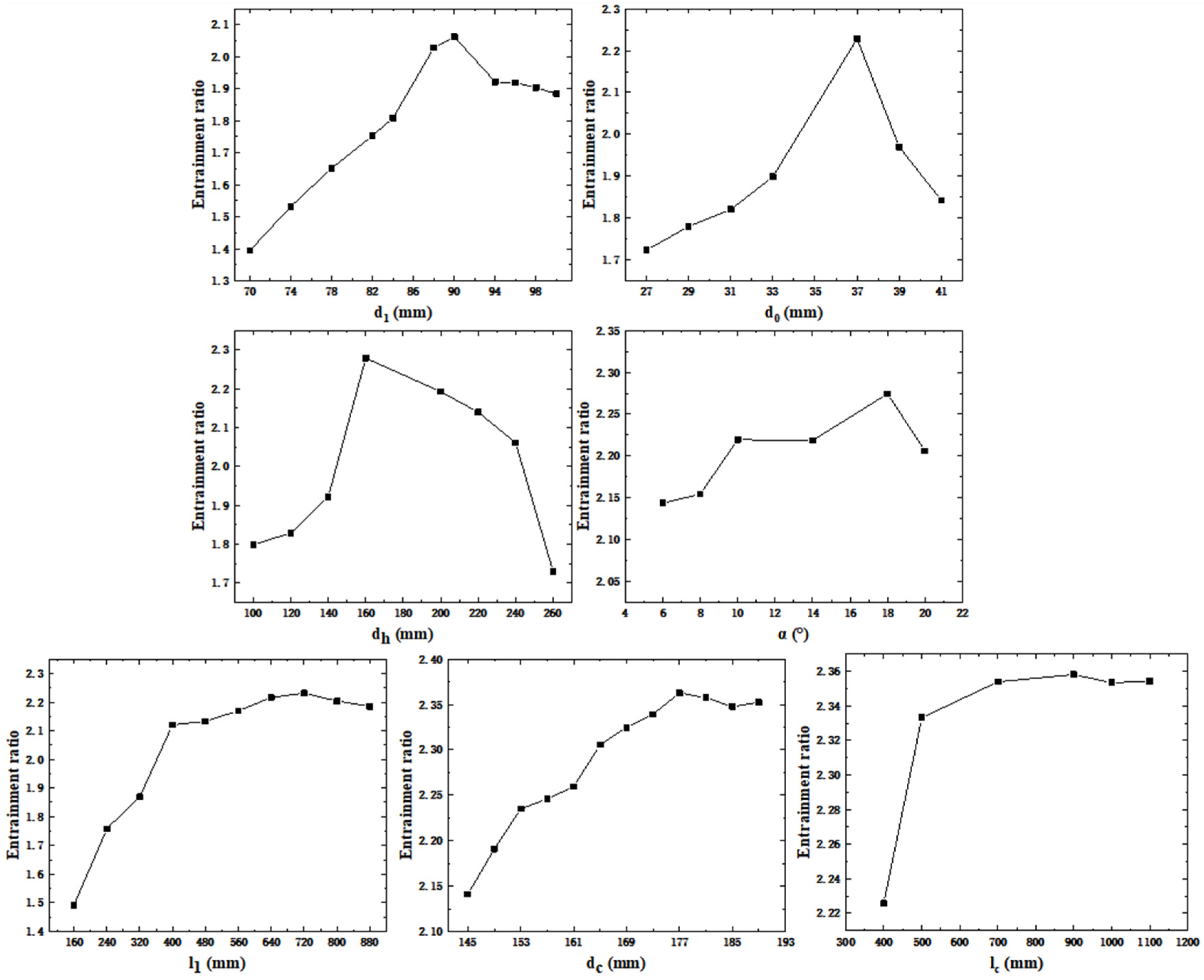
3.1. Single Factor Optimization of Ejector Structure
3.2. Response Surface Method Optimization of Ejector Structure
3.2.1. Response Surface Method
3.2.2. Selection of Optimization Parameters and Determination of Optimal Value Range
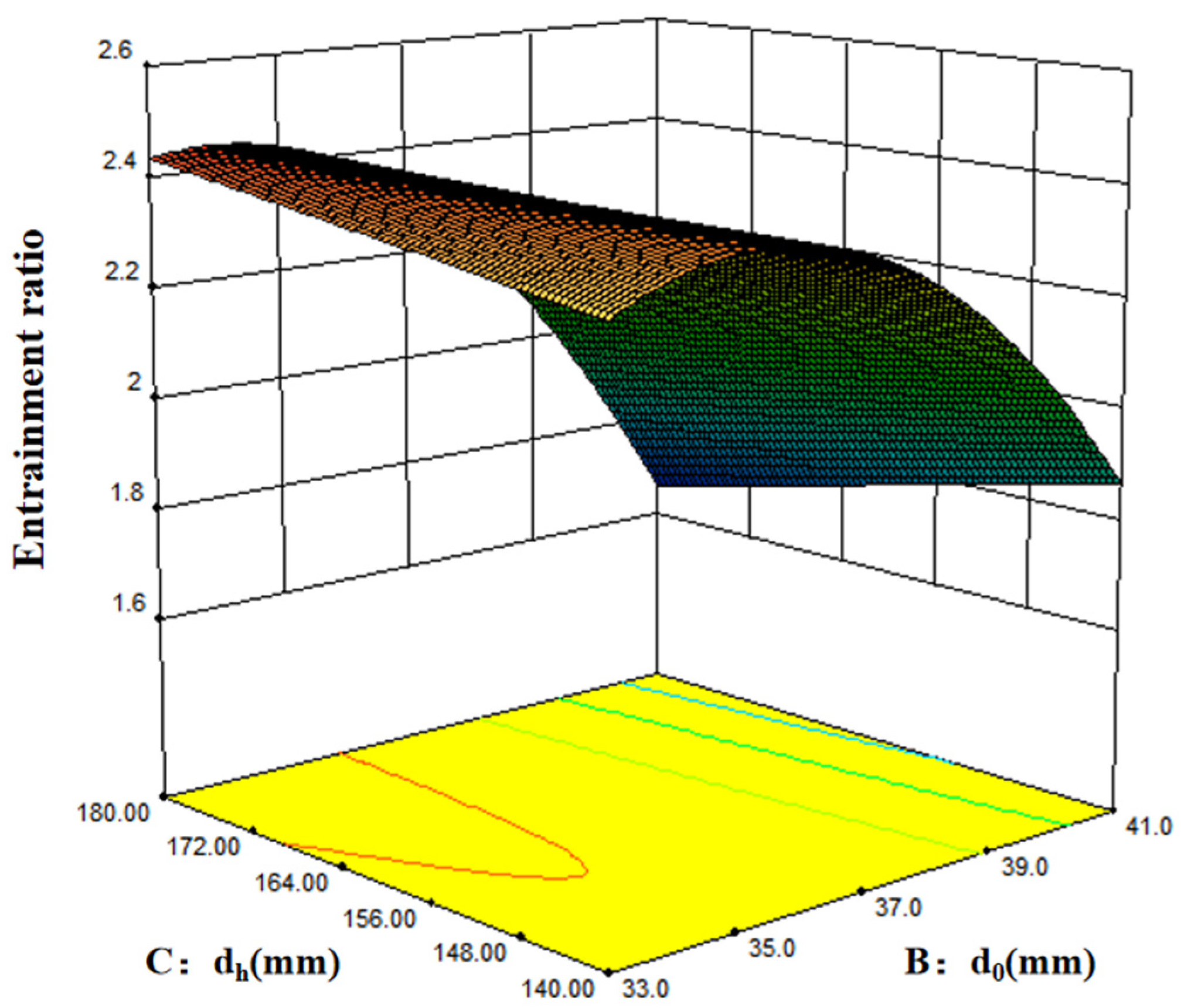
3.2.3. Response Surface Method Test Results and Analysis
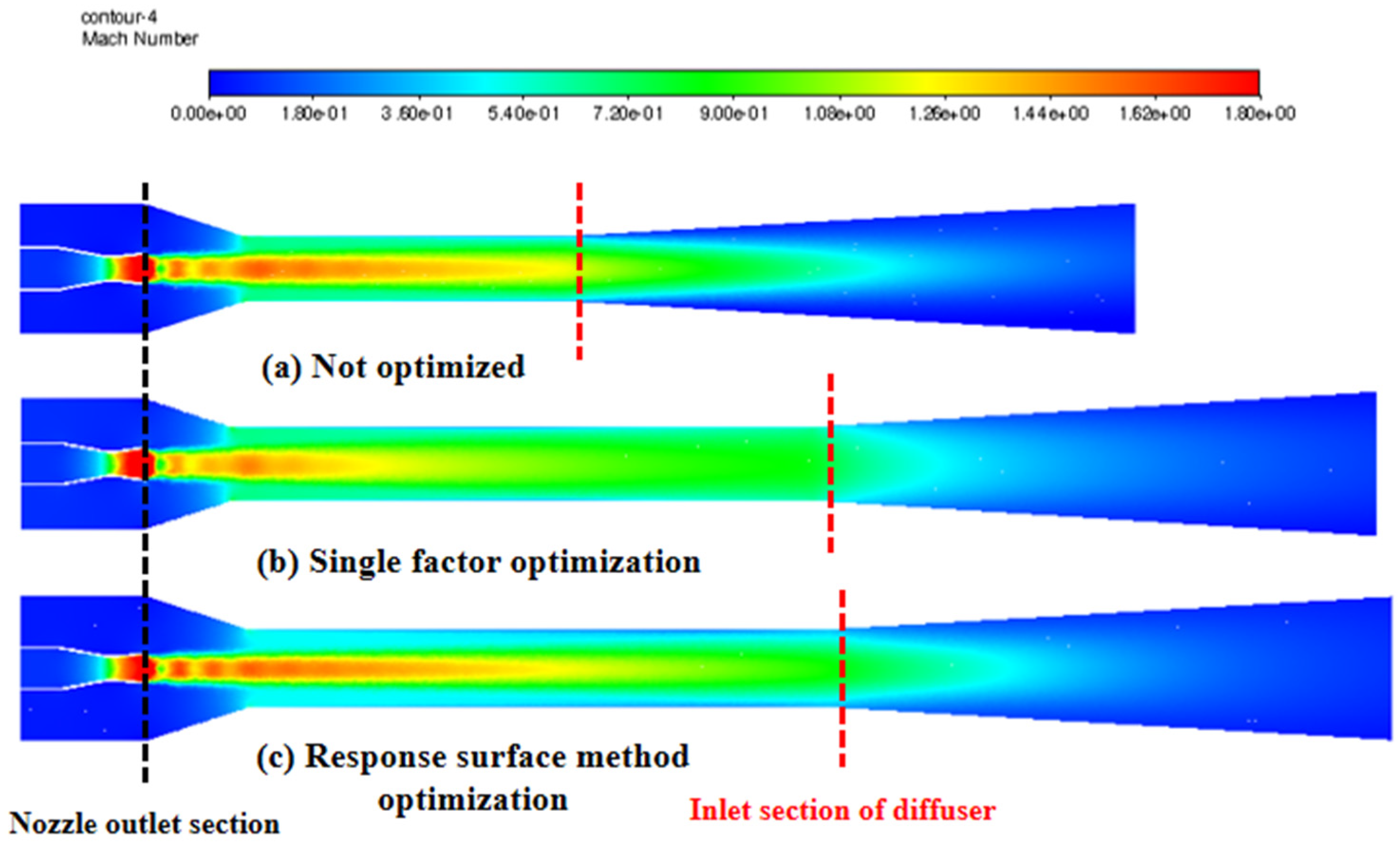
3.2.4. Flow Field Analysis of Different Optimization Methods
4. Conclusions
- (1)
- The entrainment ratio of the ejector optimized by the response surface method is 35.4% higher than that of the initial structure designed by the one-dimensional model and 5.1% higher than that of the ejector optimized by the single-factor optimization method. The ejector optimized by the response surface method has better performance.
- (2)
- The error between the predicted entrainment ratio using the one-dimensional model and the simulated entrainment ratio is 11.4%. Compared with the simulated entrainment ratio, the error of the predicted entrainment ratio using the second-order regression equation is 0.95%. The prediction equation of entrainment rate obtained by the response surface method is more reliable.
- (3)
- The structural dimensions of the ejector will affect each other. The change of structural parameters will cause variation in the flow field, and the variation of the flow field will affect the entrainment ratio of the ejector. The response surface method can obtain the optimal structure size through the combined effect of multiple structural parameters on the entrainment ratio.
Author Contributions
Funding
Conflicts of Interest
References
- Dong, J.; Hu, Q.; Yu, M.; Han, Z.; Cui, W.; Liang, D.; Ma, H.; Pan, X. Numerical investigation on the influence of mixing chamber length on steam ejector performance. Appl. Therm. Eng. 2020, 174, 115204. [Google Scholar] [CrossRef]
- Chen, W.; Chong, D.; Yan, J.; Liu, J. The numerical analysis of the effect of geometrical factors on natural gas ejector performance. Appl. Therm. Eng. 2013, 59, 21–29. [Google Scholar] [CrossRef]
- Chen, W.; Chen, H.; Shi, C.; Wang, Y.; Chong, D.; Yan, J. Experimental investigation of influences of primary nozzle exit position on ejector performance. J. Univ. Chin. Acad. Sci. 2016, 33, 253–257. [Google Scholar]
- Bai, T.; Xie, H.; Liu, S.; Yan, G.; Yu, J. Experimental investigation on the influence of ejector geometry on the pull-down performance of an ejector-enhanced auto-cascade low-temperature freezerÉtude expérimentale de l’influence de la géométrie de l’éjecteur sur la performance de mise en régime d’un congélateur basse température en auto-cascade avec éjecteur amélioré. Int. J. Refrig. 2021, 131, 41–50. [Google Scholar]
- Bauzvand, A.; Tavousi, E.; Noghrehabadi, A.; Behbahani-Nejad, M. Study of a novel inlet geometry for ejectors. Int. J. Refrig. 2022, 139, 113–127. [Google Scholar] [CrossRef]
- Yang, Y.; Karvounis, N.; Walther, J.H.; Ding, H.; Wen, C. Effect of area ratio of the primary nozzle on steam ejector performance considering nonequilibrium condensations. Energy 2021, 237, 121483. [Google Scholar] [CrossRef]
- Sun, W.; Ma, X.; Zhang, Y.; Jia, L.; Xue, H. Performance analysis and optimization of a steam ejector through streamlining of the primary nozzle. Case Stud. Therm. Eng. 2021, 27, 101356. [Google Scholar] [CrossRef]
- Fu, W.; Liu, Z.; Li, Y.; Wu, H.; Tang, Y. Numerical study for the influences of primary steam nozzle distance and mixing chamber throat diameter on steam ejector performance. Int. J. Therm. Sci. 2018, 132, 509–516. [Google Scholar] [CrossRef]
- Xue, K.; Li, K.; Chen, W.; Chong, D.; Yan, J. Numerical investigation on the performance of different primary nozzle structures in the supersonic ejector. Energy Procedia 2017, 105, 4997–5004. [Google Scholar] [CrossRef]
- Tu, J.V. Advantages and disadvantages of using artificial neural networks versus logistic regression for predicting medical outcomes. J. Clin. Epidemiol. 1996, 49, 1225–1231. [Google Scholar] [CrossRef]
- Falcone, R.; Ciaramella, A.; Carrabs, F.; Strisciuglio, N.; Martinelli, E. Artificial neural network for technical feasibility prediction of seismic retrofitting in existing RC structures. Structures 2022, 41, 1220–1234. [Google Scholar] [CrossRef]
- Zhou, S.; Qiu, J.; Zhang, C.; Guo, Y.; Pan, Q.; Zhou, Q.; Shuai, Y. Fast design and optimization method for an ultra-wideband perfect absorber based on artificial neural network acceleration. Int. J. Therm. Sci. 2022, 179, 107680. [Google Scholar] [CrossRef]
- Jeon, Y.; Lee, D.; Cho, H. Optimization of motive nozzle position in a modified two-phase ejector expansion household refrigeration cycle using an artificial neural network. Energy Rep. 2022, 8, 1114–1123. [Google Scholar] [CrossRef]
- Wu, Y.; Zhao, H.; Zhang, C.; Wang, L.; Han, J. Optimization analysis of structure parameters of steam ejector based on CFD and orthogonal test. Energy 2018, 151, 79–93. [Google Scholar] [CrossRef]
- Tong, X.; Wang, Y.; Gao, R.; Wang, W. Optimization of extraction conditions of total alkaloids from Lasiosphaera fenzlii by a combination of orthogonal design with response surface method. Pharm. Biotechnol. 2013, 20, 245–249. [Google Scholar]
- Pan, Y.; Yu, L.; Zhang, Z.; Han, L. Research on lightweight design of mobile car machine based on response surface method. Comput. Simul. 2020, 37, 91–95. [Google Scholar]
- Fu, H.; Wu, R. Optimization of thrust characteristics of tubular permanent magnet linear motor based on response surface method. Small Spec. Electr. Mach. 2022, 50, 31–35. [Google Scholar]
- Ouyang, S.; Xiong, Z.; Zhao, J.; Li, Z. Separator performance modeling and analysis using artificial neural network and response surface method. Ann. Nucl. Energy 2022, 174, 109139. [Google Scholar] [CrossRef]
- Tayyeban, E.; Deymi-Dashtebayaz, M.; Dadpour, D. Multi objective optimization of MSF and MSF-TVC desalination systems with using the surplus low-pressure steam (an energy, exergy and economic analysis). Comput. Chem. Eng. 2022, 160, 107708. [Google Scholar] [CrossRef]
- Deymi-Dashtebayaz, M.; Tayyeban, E. Multi objective optimization of using the surplus low pressure steam from natural gas refinery in the thermal desalination process. J. Clean. Prod. 2019, 238, 117945. [Google Scholar] [CrossRef]
- Huang, B.J.; Chang, J.M.; Wang, C.P.; Petrenko, V.A. A 1-D analysis of ejector performance. Int. J. Refrig. 1999, 22, 354–364. [Google Scholar] [CrossRef]
- Sriveerakul, T.; Aphornratana, S.; Chunnanond, K. Performance prediction of steam ejector using computational fluid dynamics: Part 1. Validation of the CFD results. Int. J. Therm. Sci. 2006, 46, 812–822. [Google Scholar] [CrossRef]
- Hou, Y.; Chen, F.; Zhang, S.; Chen, W.; Zheng, J.; Chong, D.; Yan, J. Numerical simulation study on the influence of primary nozzle deviation on the steam ejector performance. Int. J. Therm. Sci. 2022, 179, 107633. [Google Scholar] [CrossRef]



| Structure Name | Value |
|---|---|
| Nozzle inlet diameter (di) | 48 mm |
| Nozzle throat diameter (dt) | 25.96 mm |
| Nozzle throat length (lt) | 5 mm |
| Nozzle outlet diameter (d0) | 35 mm |
| Mixing chamber inlet diameter (dh) | 160 mm |
| Inclination angle of contraction section of mixing chamber (α) | 18° |
| Throat diameter of mixing chamber (d1) | 80.5 mm |
| Throat length of mixing chamber (l1) | 400 mm |
| Diffuser outlet diameter (dc) | 161 mm |
| Diffuser length (lc) | 700 mm |
| Number of Grids | Pressure (Pa) | Absolute Value of Relative Error (%) | Velocity (m/s) | Absolute Value of Relative Error (%) |
|---|---|---|---|---|
| 361,449 | 590,477 | - | 918.053 | - |
| 514,450 | 601,920 | 1.93 | 914.074 | 0.43 |
| 820,227 | 591,419 | 1.74 | 917.09 | 0.33 |
| 1,545,968 | 586,748 | 0.79 | 918.982 | 0.21 |
| 2,270,250 | 595,874 | 1.56 | 915.052 | 0.43 |
| 3,768,720 | 597,252 | 0.23 | 914.45 | 0.07 |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| di | 48 mm | dc | 177 mm |
| dt | 25.96 mm | lt | 5 mm |
| d0 | 37 mm | α | 18° |
| dh | 160 mm | l1 | 720 mm |
| d1 | 90 mm | lc | 700 mm |
| Parameter | Maximum Interval [a, b] | Maximum Entrainment Ratio Change Rate | |
|---|---|---|---|
| a | b | ||
| d1 | 84 m | 96 mm | 10.7% |
| d0 | 33 mm | 41 mm | 13.8% |
| dh | 140 mm | 180 mm | 15.7% |
| α | 14° | 18° | 3.0% |
| l1 | 640 mm | 800 mm | 1.2% |
| dc | 173 mm | 181 mm | 1.0% |
| lc | 800 mm | 1000 mm | 0.2% |
| Level | Factor | ||
|---|---|---|---|
| A-d1 | B-d0 | C-dh | |
| −1 | 84 mm | 33 mm | 140 mm |
| 0 | 90 mm | 37 mm | 160 mm |
| 1 | 96 mm | 41 mm | 180 mm |
| Serial Number | Factor | Response (Entrainment Ratio) | ||
|---|---|---|---|---|
| A-d1 | B-d0 | C-dh | ||
| 1 | 0 | 0 | 0 | 2.2673 |
| 2 | 1 | 0 | 0 | 2.3451 |
| 3 | 0 | 0 | 0 | 2.2676 |
| 4 | 0 | 0 | 0 | 2.2832 |
| 5 | −1 | 0 | 0 | 2.1107 |
| 6 | 1 | −1 | 1 | 2.4804 |
| 7 | 0 | 0 | 0 | 2.2853 |
| 8 | −1 | 1 | 1 | 1.6401 |
| 9 | 1 | 1 | 1 | 1.6233 |
| 10 | 0 | 0 | −1 | 2.3189 |
| 11 | 0 | 0 | 1 | 2.2829 |
| 12 | 0 | 0 | 0 | 2.2135 |
| 13 | 0 | 1 | 0 | 1.7905 |
| 14 | 1 | −1 | −1 | 2.3531 |
| 15 | 0 | −1 | 0 | 2.2702 |
| 16 | −1 | 1 | −1 | 1.8657 |
| 17 | 1 | 1 | −1 | 1.8172 |
| 18 | −1 | −1 | 1 | 2.0909 |
| 19 | 0 | 0 | 0 | 2.3085 |
| 20 | −1 | −1 | −1 | 1.9723 |
| Source | Sum of Squares | df | Mean Square | F Valve | p Valve | Significance |
|---|---|---|---|---|---|---|
| Model | 1.22 | 7 | 0.17 | 257.88 | <0.0001 | ** |
| A-d1 | 0.088 | 1 | 0.088 | 130.56 | <0.0001 | ** |
| B-d0 | 0.59 | 1 | 0.59 | 873.70 | <0.0001 | ** |
| dh | 4.393 × 10−3 | 1 | 4.393 × 10−3 | 6.50 | 0.0255 | * |
| AB | 0.087 | 1 | 0.087 | 129.13 | <0.0001 | ** |
| BC | 0.055 | 1 | 0.055 | 81.88 | <0.0001 | ** |
| A2 | 8.076 × 10−3 | 1 | 8.076 × 10−3 | 11.95 | 0.0047 | ** |
| B2 | 0.20 | 1 | 0.20 | 290.69 | <0.0001 | ** |
| Residual | 8.111 × 10−3 | 12 | 6.759 × 10−4 | |||
| Lack of Fit | 3.020 × 10−3 | 7 | 4.314 × 10−4 | 0.42 | 0.8532 | |
| Pure Error | 5.091 × 10−3 | 5 | 1.018 × 10−3 | |||
| Cor Total | 1.23 | 19 |
| Type | Throat Diameter of Mixing Chamber (mm) | Nozzle Outlet Diameter (mm) | Mixing Chamber Inlet Diameter (mm) | Entrainment Ratio Predicted by One-Dimensional Model [19] | Entrainment Ratio Predicted by the Second-Order Regression Equation | Simulated Entrainment Ratio |
|---|---|---|---|---|---|---|
| Not optimized | 80.5 | 29 | 160 | 2.036 | - | 1.827 |
| Single factor optimization | 90 | 37 | 160 | 2.297 | - | 2.354 |
| Response surface method optimization | 96 | 33.58 | 180 | 2.626 | 2.497 | 2.473 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, J.; Hou, Y.; Tian, Z.; Jiang, H.; Chen, W. Simulation Analysis of Ejector Optimization for High Mass Entrainment under the Influence of Multiple Structural Parameters. Energies 2022, 15, 7058. https://doi.org/10.3390/en15197058
Zheng J, Hou Y, Tian Z, Jiang H, Chen W. Simulation Analysis of Ejector Optimization for High Mass Entrainment under the Influence of Multiple Structural Parameters. Energies. 2022; 15(19):7058. https://doi.org/10.3390/en15197058
Chicago/Turabian StyleZheng, Jiantao, Yuyan Hou, Zhongwei Tian, Hongkui Jiang, and Weixiong Chen. 2022. "Simulation Analysis of Ejector Optimization for High Mass Entrainment under the Influence of Multiple Structural Parameters" Energies 15, no. 19: 7058. https://doi.org/10.3390/en15197058
APA StyleZheng, J., Hou, Y., Tian, Z., Jiang, H., & Chen, W. (2022). Simulation Analysis of Ejector Optimization for High Mass Entrainment under the Influence of Multiple Structural Parameters. Energies, 15(19), 7058. https://doi.org/10.3390/en15197058







