Fluidic Thrust, Propulsion, Vector Control of Supersonic Jets by Flow Entrainment and the Coanda Effect
Abstract
:1. Introduction
2. Experimental Apparatus and Procedure
2.1. Schematics of the Fluidic Coanda Nozzle
2.2. Experimental Procedure
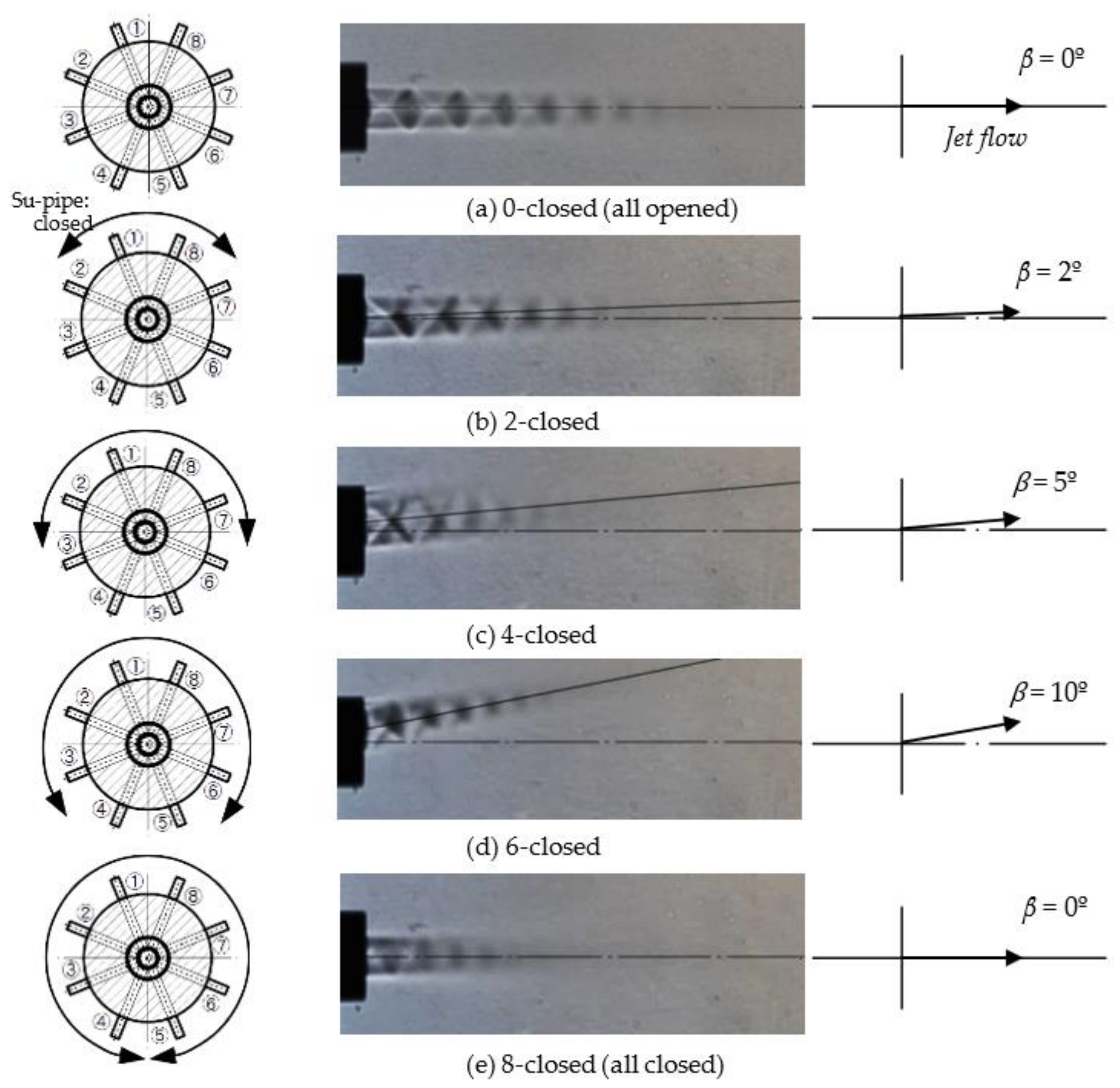
3. Propulsion Vectoring Method
4. Results and Discussion
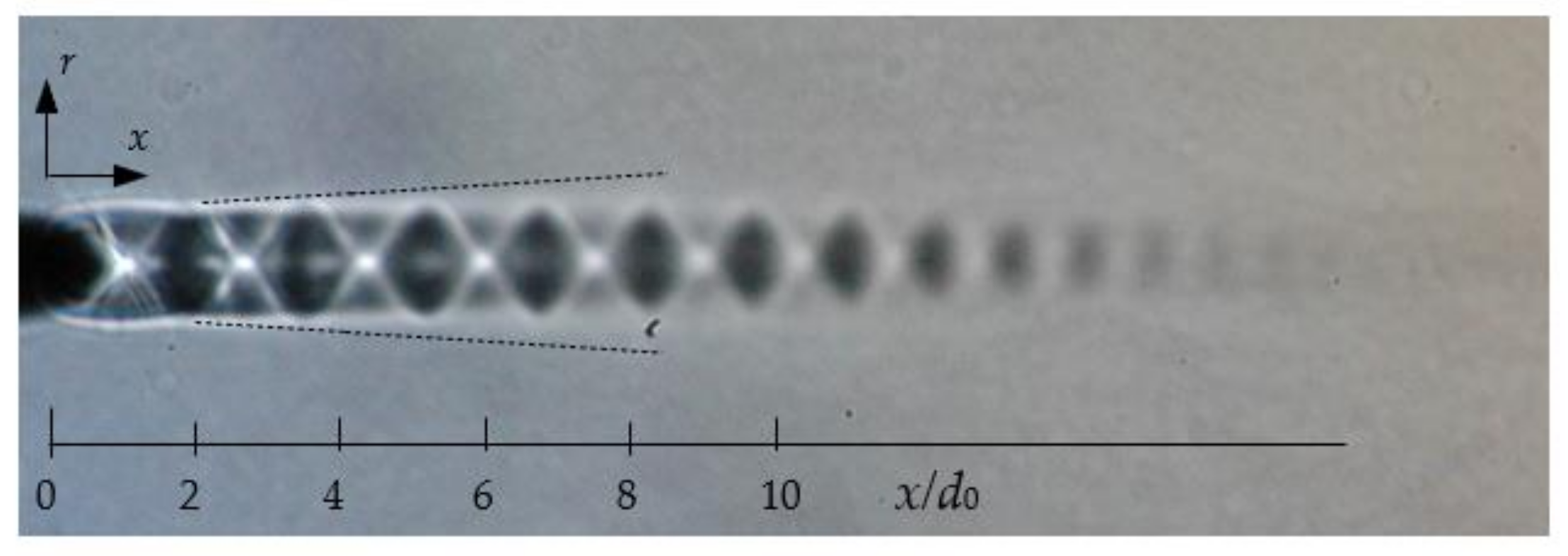
4.1. Jet Flow from the Pi-Nozzle
4.1.1. Visualized Flow Pattern
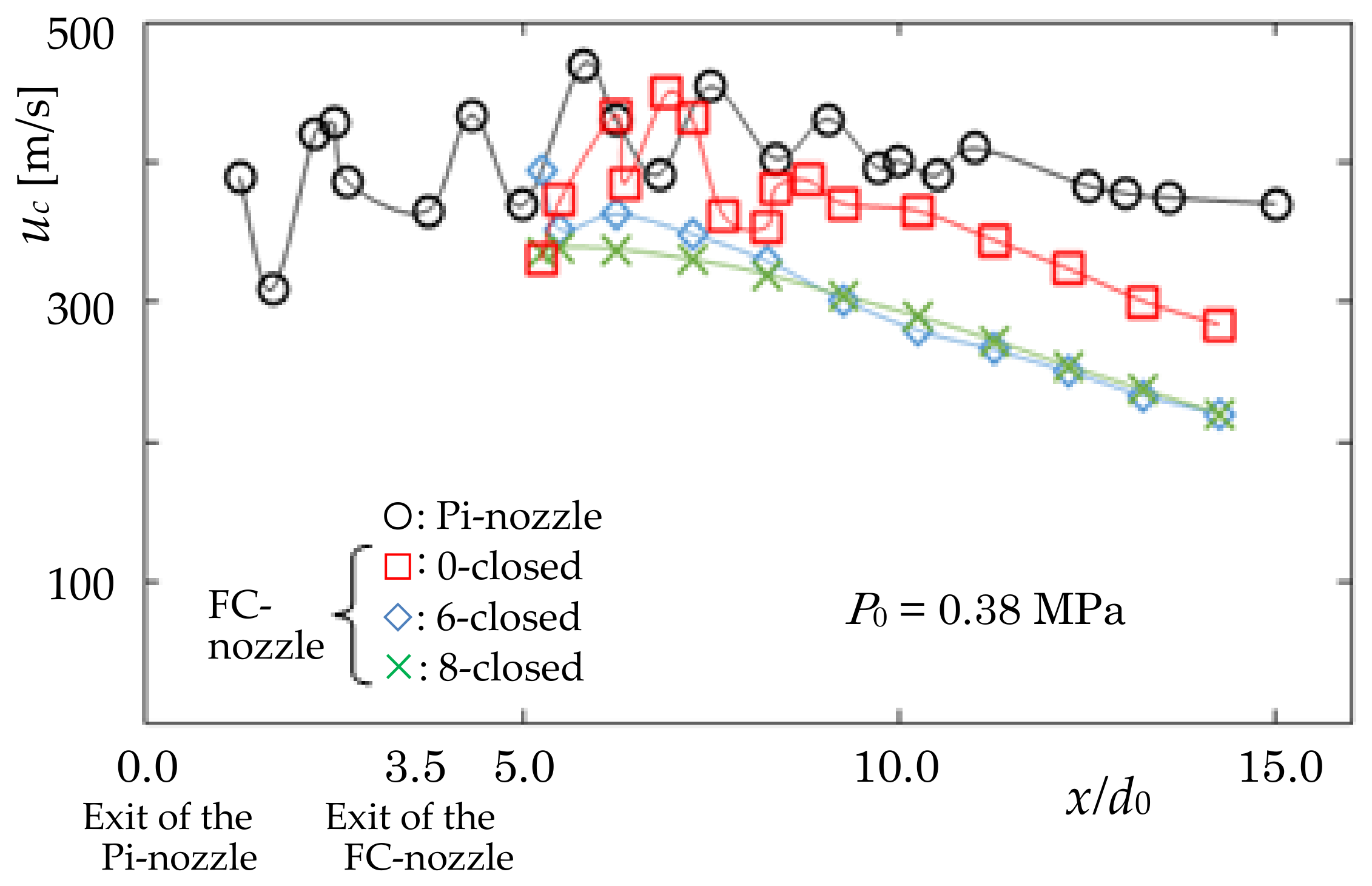
4.1.2. Jet Centerline Velocity Distribution
4.2. Jet Flow from the FC-Nozzle
4.2.1. Visualized Flow Pattern
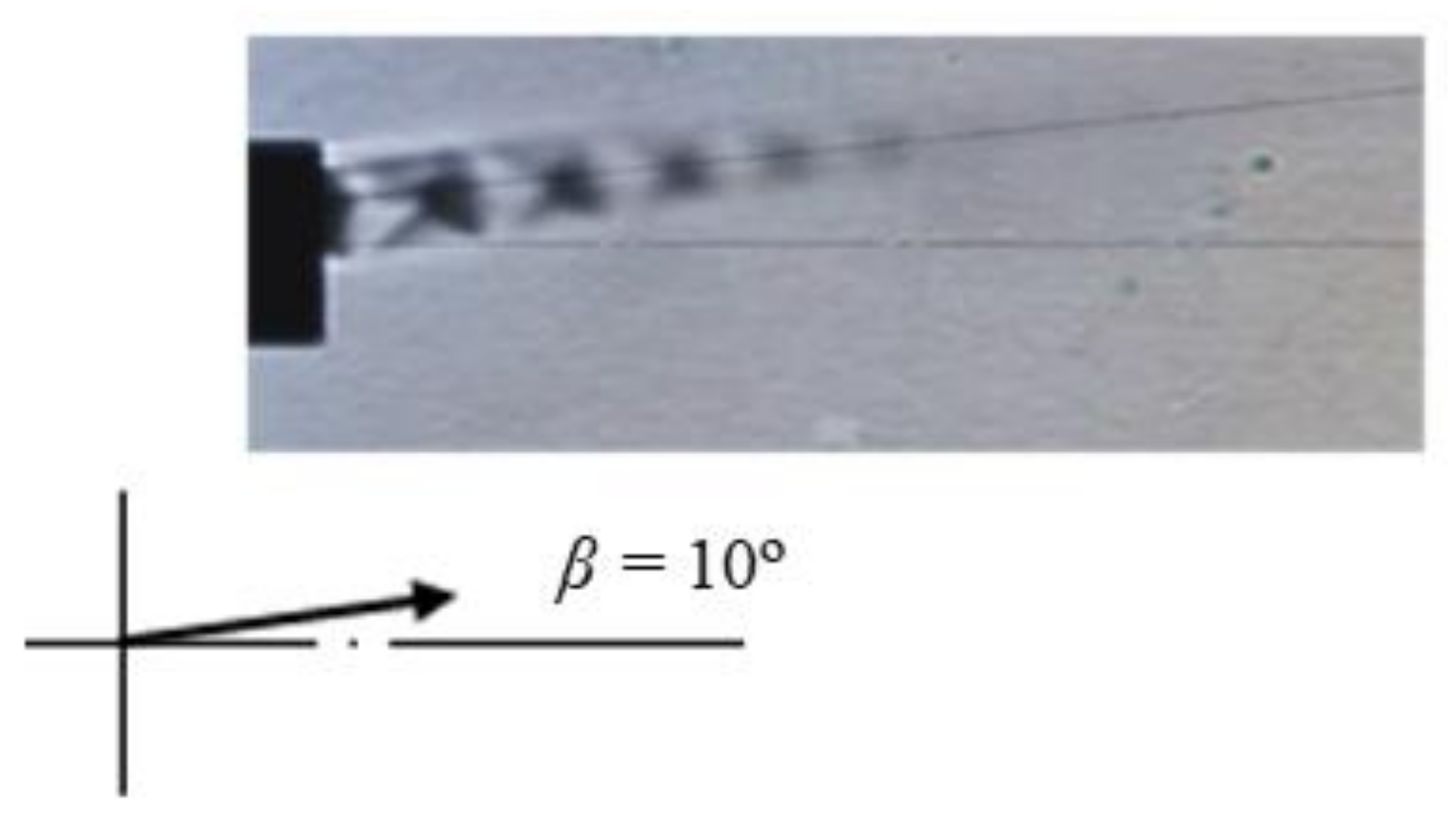
4.2.2. Deflection Angle, β
4.2.3. Practical Operation
4.3. Velocity Distribution
4.3.1. Jet Centerline Velocity Distribution
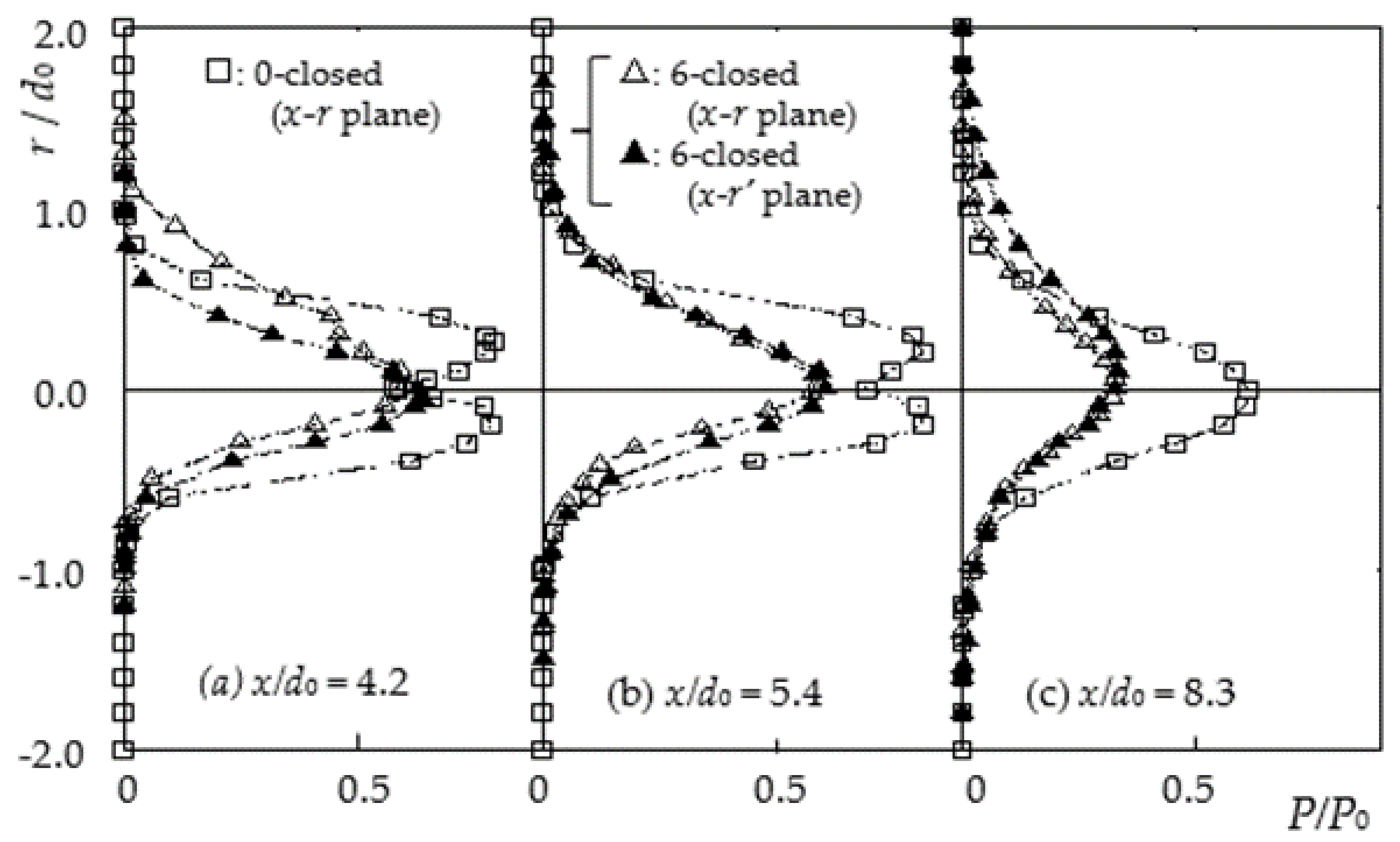
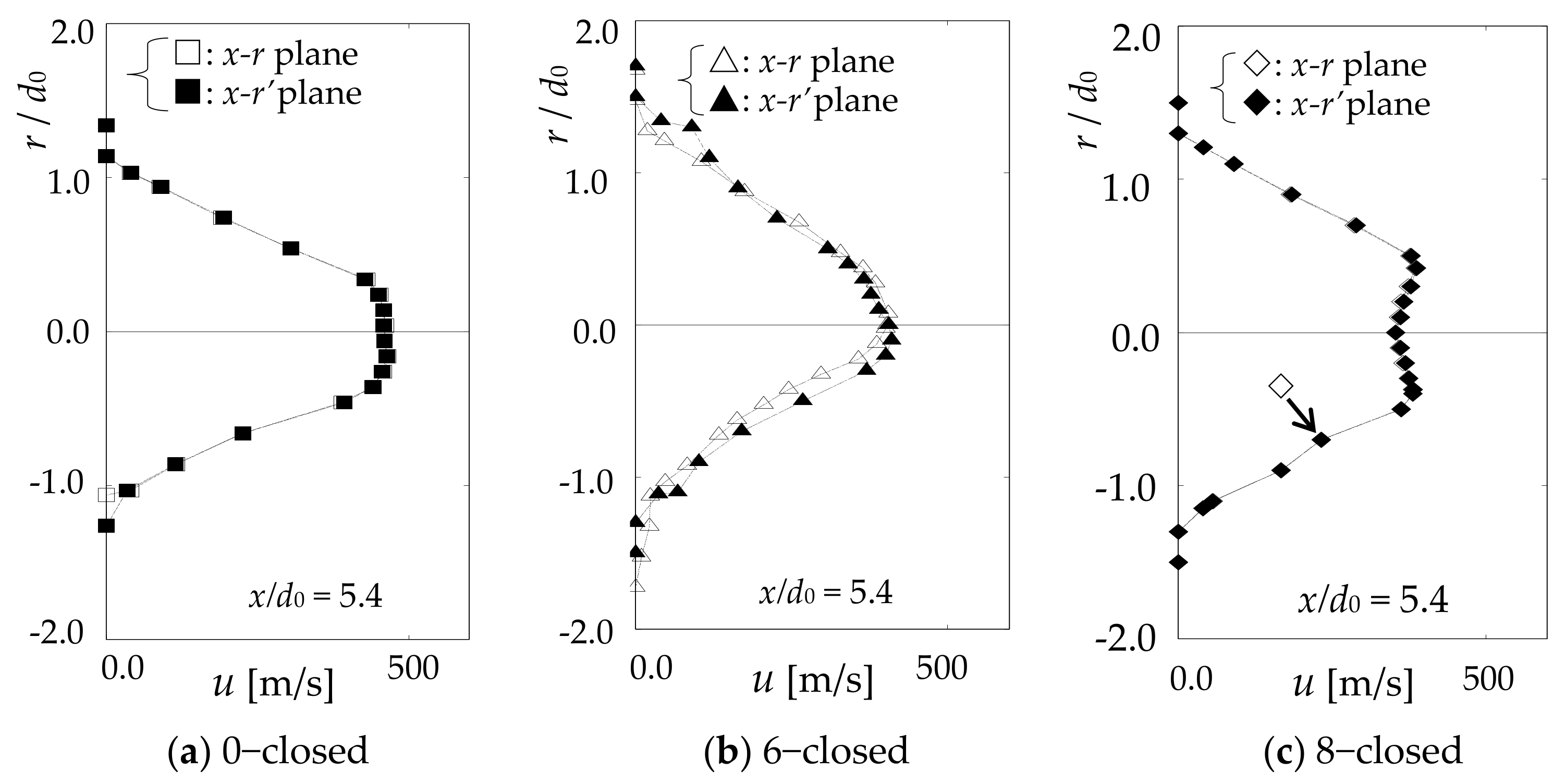
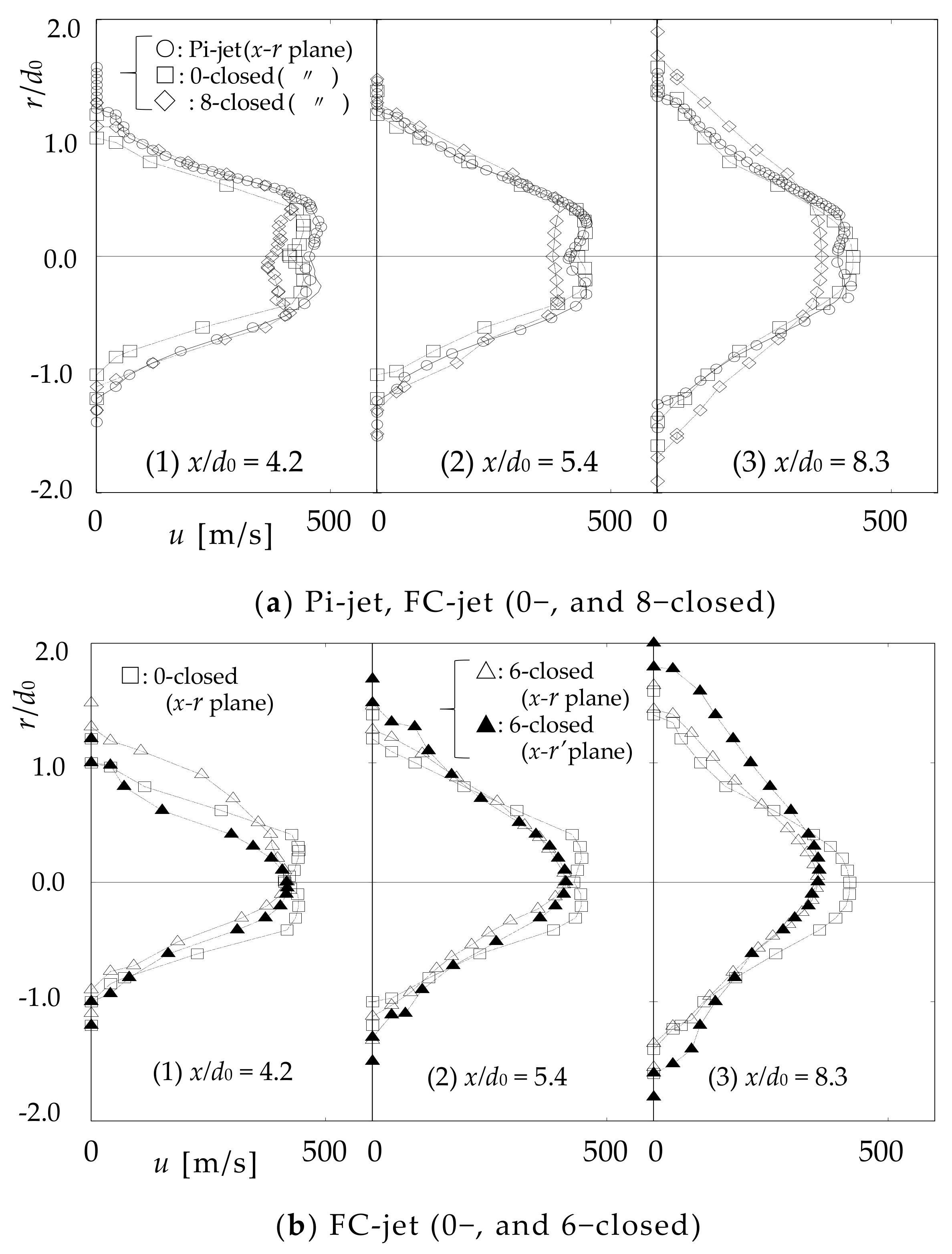
4.3.2. Cross-Sectional Pressure and Velocity Distributions
- 1.
- Total Pressure Distribution
- 2.
- Static Pressure Distribution
- 3.
- Sectional Velocity Distribution
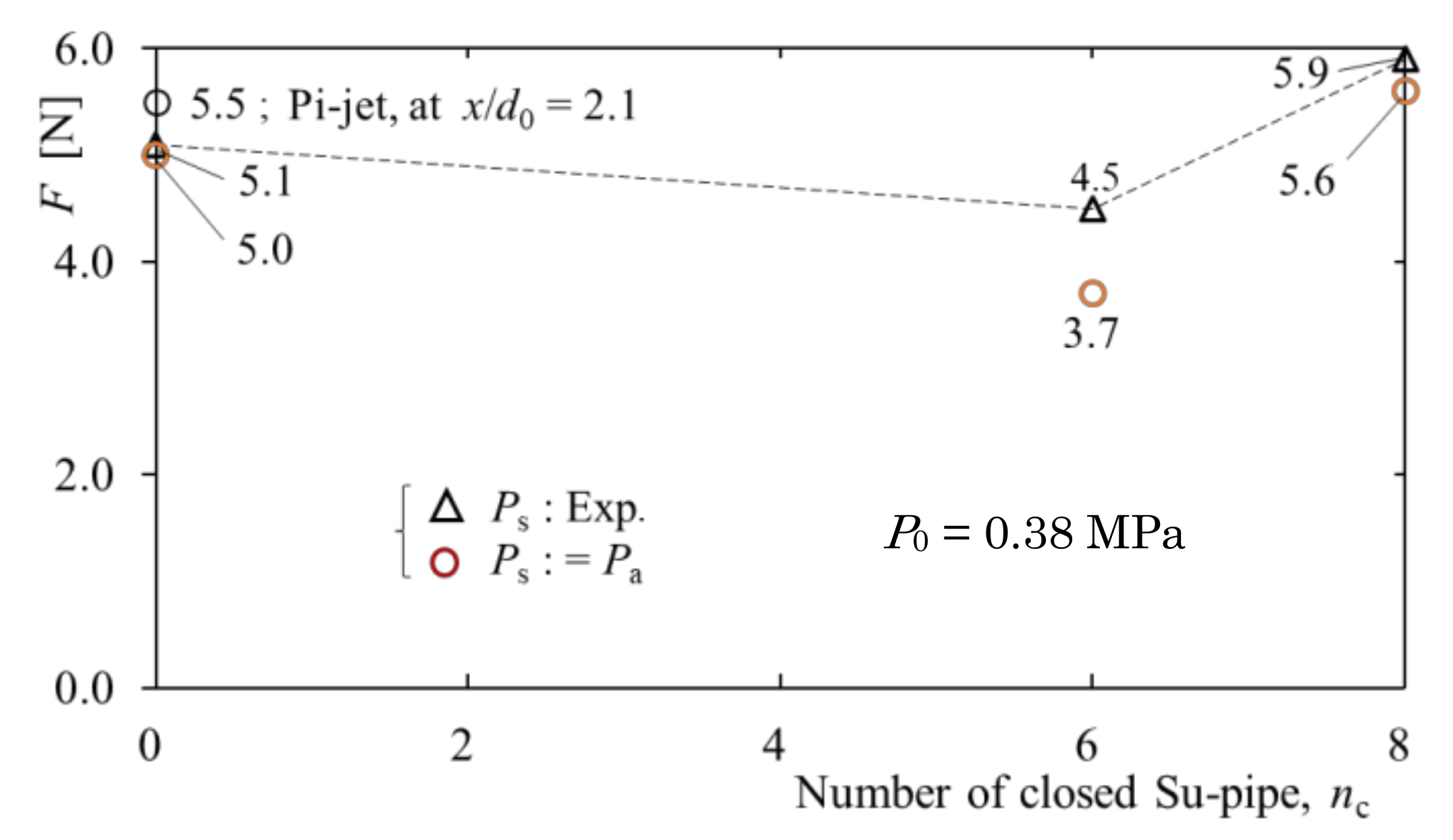
4.3.3. Thrust, Approximate Estimation
4.3.4. Thrust Vectoring by Sub Jets
- 1.
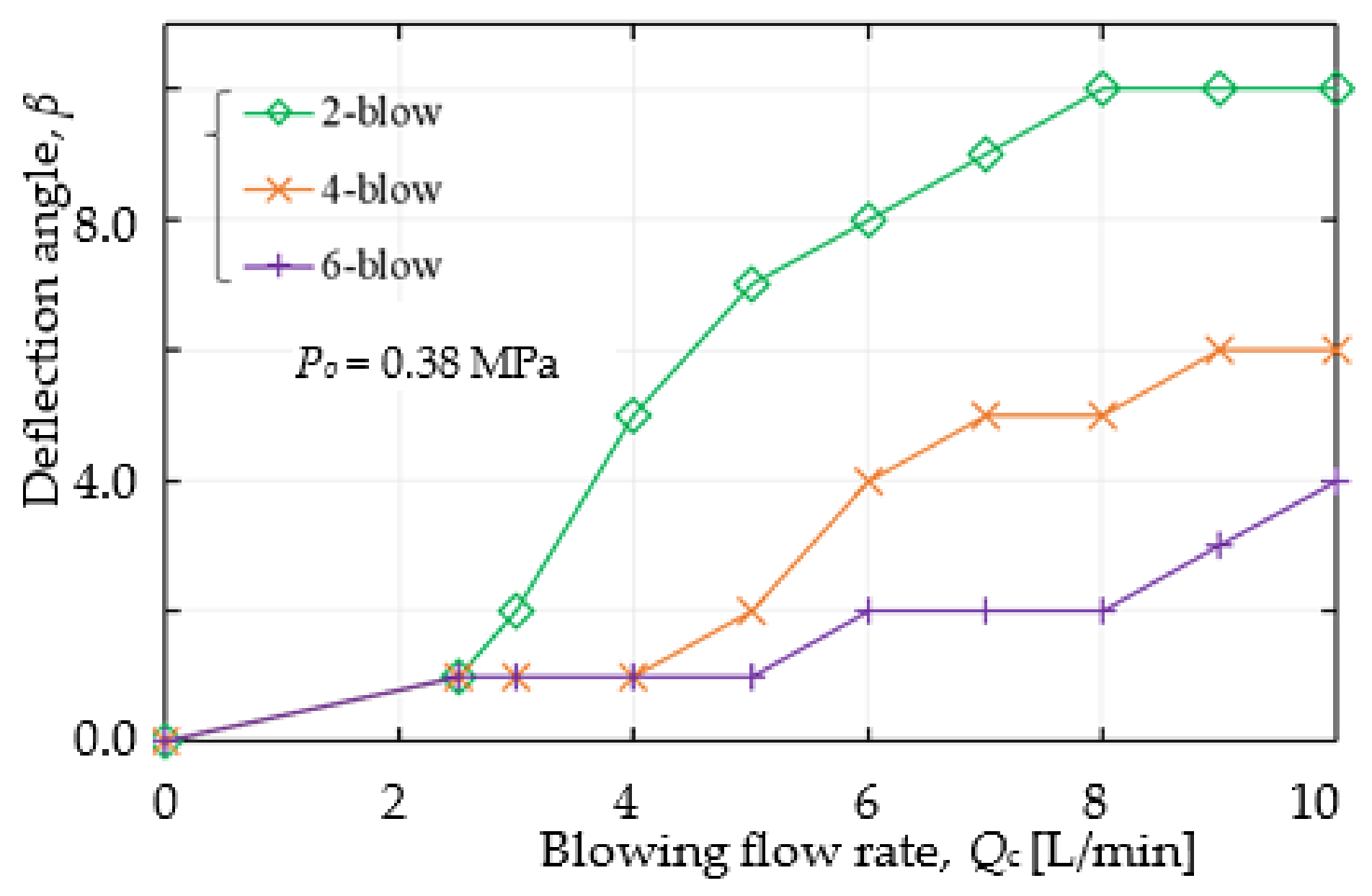
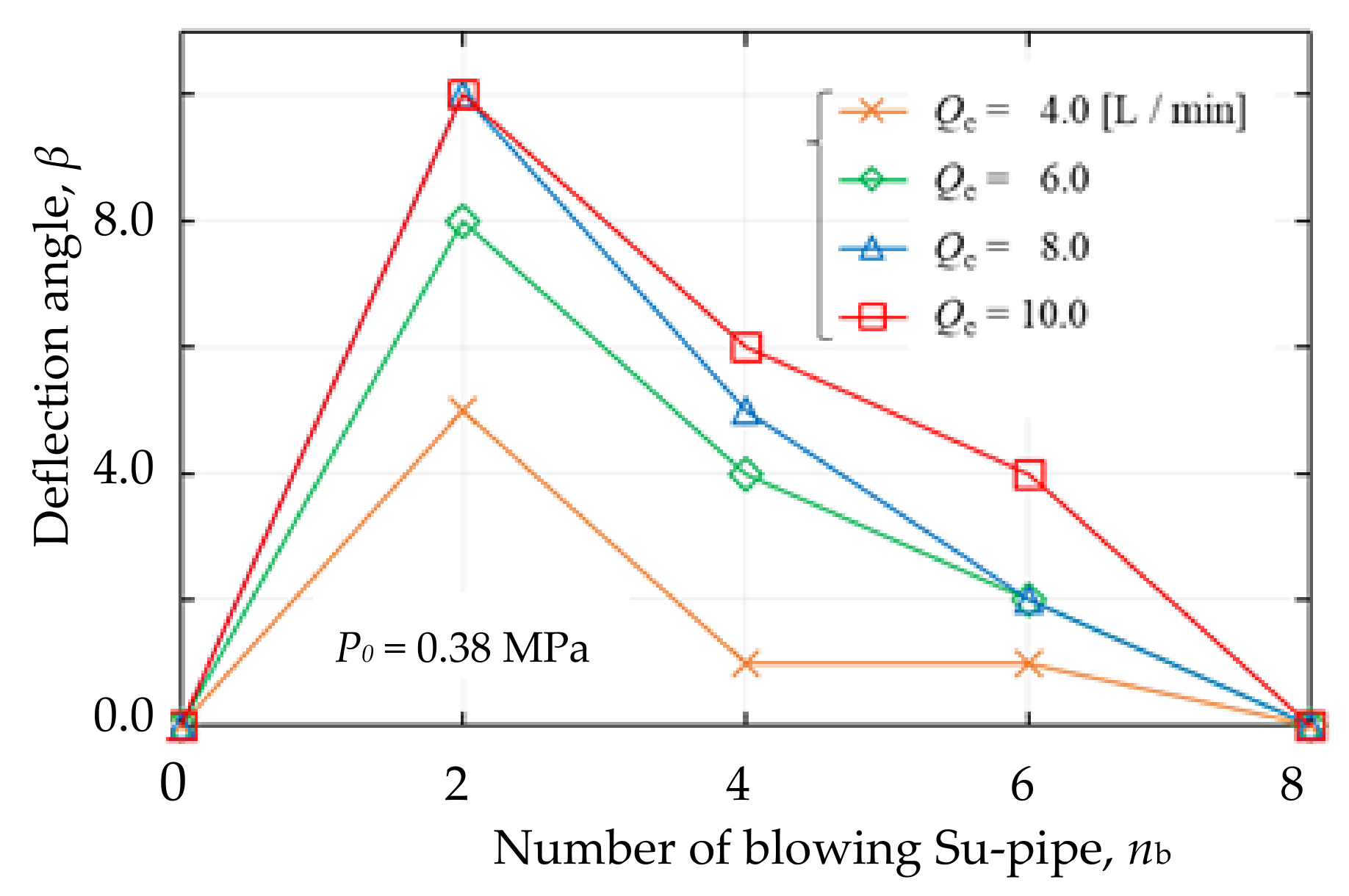
- Effect of the Blowing Flow Rate Qc on the Deflection Angle β Figure 12 shows the relationship between β and Qc when air is blown from Su-pipes 2, 4, and 6. β increases as Qc increases, and β also increases as the number of blowing nozzles, nb, decreases, because by increasing nb, the blowing flow rate per Su-pipe decreases. The deflection angle has a maximum value of β = 10° at nb = 2 and Qc = 8 [L/min].
- 2.
- Effect of the Number of Blowing Nozzles, nb, on the Deflection Angle β
5. Conclusions
- (1)
- By changing the number of the Su-pipes and the locations at which the Su-pipes close, the deflection angle and circumferential position of the jet can be controlled. That is, vector control of the thrust of the supersonic jet can be performed with this new and simple method and apparatus.
- (2)
- This new fluidic thrust vector control method for supersonic jets is extremely simple compared to other conventional methods that require control with additional devices, such as secondary jets.
- (3)
- In practical applications, it is conceivable that a computer-controlled solenoid valve could be used to change the number of Su-pipes and the locations at which they close.
- (4)
- When there is no fluid to be entrained by the jet, that is, when the surroundings are in a vacuum state, the same type of control can be gained by blowing a small amountof fluid from the Su-pipe.
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Ae | exit area of the Pi-nozzle |
| dic | diameter of the Su-pipe |
| dis | inlet diameter of the Co-nozzle |
| d0 | diameter of the Pi-nozzle |
| F | thrust |
| Lc | length of the Co-nozzle |
| n | number of Su-pipes |
| nb | number of blowing Su-pipes |
| nc | number of closing Su-pipes |
| Pa | ambient pressure (atmospheric pressure) |
| Pe | exit pressure of the Pi-nozzle |
| Ps | static pressure |
| Pt | total pressure |
| P0 | supply pressure |
| Qc | total blowing flow rate |
| r, r’ | perpendicular directions of the x-axis, respectively (Figure 1) |
| s | distance in the x-direction from the exit of the FC-nozzle |
| u | velocity in the x direction |
| uc | jet centerline velocity |
| x | direction of jet flow (Figure 1) |
| α | expansion angle of Co-nozzle |
| β | jet deflection angle |
| ρ | density of fluid (air) |
| Abbreviations | |
| Co-nozzle | Coanda nozzle |
| FC-nozzle | fluidic Coanda nozzle |
| Pi-nozzle | pipe nozzle |
| SSU-jet | supersonic under-expanded jet flow |
| Su-pipe | suction pipe |
References
- Donaldson, S.D.; Snedeker, R.S. Abst: Under-Expanded Jet. 1971. Available online: http://www.kbwiki.ercoftac.org/w/index.pkp/Abstr:Under-expanded_jet (accessed on 1 March 2019).
- Kojima, T.; Matsuoka, Y. Study of an under-expanded sonic jet (1st Report, On the mechanism of pseudo-shock waves and pressure and velocity in jets). Trans. Jpn. Soc. Mech. Eng. JSME 1987, 259, 190–191. (In Japanese) [Google Scholar]
- Nourl, J.M.; Whitelaw, J.H. Flow characteristics of an under-expanded jet and its application to the study of droplet breakup. Exp. Fluids 1996, 21, 243–247. [Google Scholar] [CrossRef]
- Zapryagaez, V.I.; Kiselev, N.P.; Pivovarov, A.A. Gas dynamic structure of an axisymmetric supersonic under-expanded jet. Fluid Dyn. 2015, 50, 95–107. [Google Scholar]
- Shakouchi, T.; Tanoue, T.; Tsujimoto, K.; Ando, T. Flow characteristics of sub- and supersonic jets issued from orifice and notched orifice nozzles, and application to ejector. J. Fluid Sci. Technol. JSME 2019, 14, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Shakouchi, T. Passive flow control of jets. J. Mech. Eng. Rev. JSME 2020, 17, 1–18. [Google Scholar] [CrossRef] [Green Version]
- Shadow, K.C.; Gutmark, E.; Wilson, W.J. Compressible spreading rates of supersonic coaxial jets. Exp. Fluids 1990, 10, 161–167. [Google Scholar] [CrossRef]
- Gutmark, E.J.; Callender, B.W.; Martens, S. Aeroacoustics of Turbulent Jets: Flow Structure, Noise Sources, and Control. JSME Int. J. Ser. Fluid Therm. Eng. 2006, 49, 1078–1085. [Google Scholar] [CrossRef]
- Deere, K.A. Summary of fluidic thrust vectoring research conducted at NASA Langley Research Center. In Proceedings of the 21st AIAA Applied Aerodynamics Conference, Orlando, FL, USA, 1 January 2003; AIAA-2003-3800. pp. 1–18. [Google Scholar]
- Pascoa, L.; Dumas, A.; Trancossi, M.; Stewart, P.; Vucinic, D. A review of thrust vectoring in support of V/STOL non-moving mechanical propulsion system. Cent. Eur. J. Phys. 2013, 3, 374–388. [Google Scholar] [CrossRef] [Green Version]
- Heo, J.Y.; Too, K.H.; Lee, Y.; Sung, H.G.; Cho, S.H.; Jeon, Y.J. Fluidic thrust vector control of supersonic jet using co-flow injection. In Proceedings of the 45th AIAA/ASME/SAE/ASEE Joint Propulsion Conference Exhibit, Denver, CO, USA, 2–5 August 2009; AIAA 2009-517. pp. 1–2. [Google Scholar]
- Dumitrache, A.; Frunzulica, F.; Preotu, O.; Ionescu, T. Thrust and jet directional control using the Coanda effect. IN-CAS Bull. 2018, 10, 199–205. [Google Scholar] [CrossRef]
- Wu, K.; Kim, H.D.; Jin, Y. Fluidic thrust vector control based on counter-flow concept. Proc. Inst. Mech. Eng. Part G J. Aerosp. Eng. 2018, 233, 1412–1422. [Google Scholar] [CrossRef]
- Trancossi, M.; Dumas, A. Coanda Synthetic Jet Deflection Apparatus and Control; No. 2011-01-2590; SAE International: Warrendale, PA, USA, 2011. [Google Scholar] [CrossRef] [Green Version]
- Liu, J.F.; Luo, Z.B.; Deng, X.; Zhao, Z.J.; Li, S.Q.; Liu, Q.; Zhu, Y.X. Dual Synthetic jets actuator and its applications—Part II: Novel fluidic thrust-vectoring method based on dual synthetic jets actuator. Actuators 2022, 11, 209. [Google Scholar] [CrossRef]
- Resta, E.; Malsilio, R.; Ferlauto, M. Thrust vectoring of a fixed axisymmetric supersonic nozzle using the shock-vector control method. Fluids 2021, 6, 441. [Google Scholar] [CrossRef]
- Miller, R.; Yagle, P.J.; Hamstra, J.W. Fluidic throat skewing for thrust vectoring in fixed-geometry nozzles. In Proceedings of the 37th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 11–14 January 1999; p. AIAA-99-0365. [Google Scholar] [CrossRef]
- Allen, D.; Smith, B.L. Axisymmetric Coanda-assisted vectoring. Exp. Fluids 2009, 46, 55–64. [Google Scholar] [CrossRef] [Green Version]
- Pourhashem, H.; Kumar, S.; Kalkhoran, I.M. Flow field characteristics of a supersonic jet influenced by downstream micro jet fluidic injection. Aerosp. Sci. Technol. AESCTE 2019, 93, 1–15. [Google Scholar] [CrossRef] [Green Version]
- Yan, D.; Wei, Z.; Xie, K.; Wang, N. Simulation of thrust control by fluidic injection and pintle in a solid rocket motor. Aerosp. Sci. Technol. AESCTE 2020, 99, 1–10. [Google Scholar] [CrossRef]
- Trancossi, M. An overview of Scientific and Technical Literature on Coanda Effect Applied to Nozzles. SAE Tech. Pap. 2011, 2011, 2591. [Google Scholar] [CrossRef]
- Trancossi, M.; Dumas, A. ACHEON: Aerial Coanda High Efficiency Orienting-Jet Nozzle; No. 2011-01-2737; SAE International: Warrendale, PA, USA, 2011. [Google Scholar] [CrossRef]
- Trancossi, M.; Madonia, M.; Dumas, A.; Angeli, D.; Bingham, C.; Sumanta Das, S.; Grimaccia, F.; Marques, J.P.; Porreca, E.; Smith, T.; et al. A new aircraft architecture based on the ACHEON Coanda nozzle: Flight model and energy evaluation. Eur. Transp. Res. Rev. 2016, 8, 1–21. [Google Scholar] [CrossRef] [Green Version]
- Trancossi, M.; Stewart, J.; Maharshi, S.; Angeli, D. Mathematical model of a constructal Coanda effect nozzle. J. Appl. Fluid Mech. 2016, 9, 2813–2822. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shakouchi, T.; Fukushima, S. Fluidic Thrust, Propulsion, Vector Control of Supersonic Jets by Flow Entrainment and the Coanda Effect. Energies 2022, 15, 8513. https://doi.org/10.3390/en15228513
Shakouchi T, Fukushima S. Fluidic Thrust, Propulsion, Vector Control of Supersonic Jets by Flow Entrainment and the Coanda Effect. Energies. 2022; 15(22):8513. https://doi.org/10.3390/en15228513
Chicago/Turabian StyleShakouchi, Toshihiko, and Shunsuke Fukushima. 2022. "Fluidic Thrust, Propulsion, Vector Control of Supersonic Jets by Flow Entrainment and the Coanda Effect" Energies 15, no. 22: 8513. https://doi.org/10.3390/en15228513
APA StyleShakouchi, T., & Fukushima, S. (2022). Fluidic Thrust, Propulsion, Vector Control of Supersonic Jets by Flow Entrainment and the Coanda Effect. Energies, 15(22), 8513. https://doi.org/10.3390/en15228513






