Research and Application of Surface Throttling Technology for Ultra-High-Pressure Sour Natural Gas Wells in Northwestern Sichuan Basin
Abstract
:1. Introduction
2. Prediction of Formation Conditions of Ultra-High-Pressure Sour Gas Hydrate
2.1. vdW-P Hydrate Thermodynamic Model
2.2. Description of the Polar System Based on CPA EoS
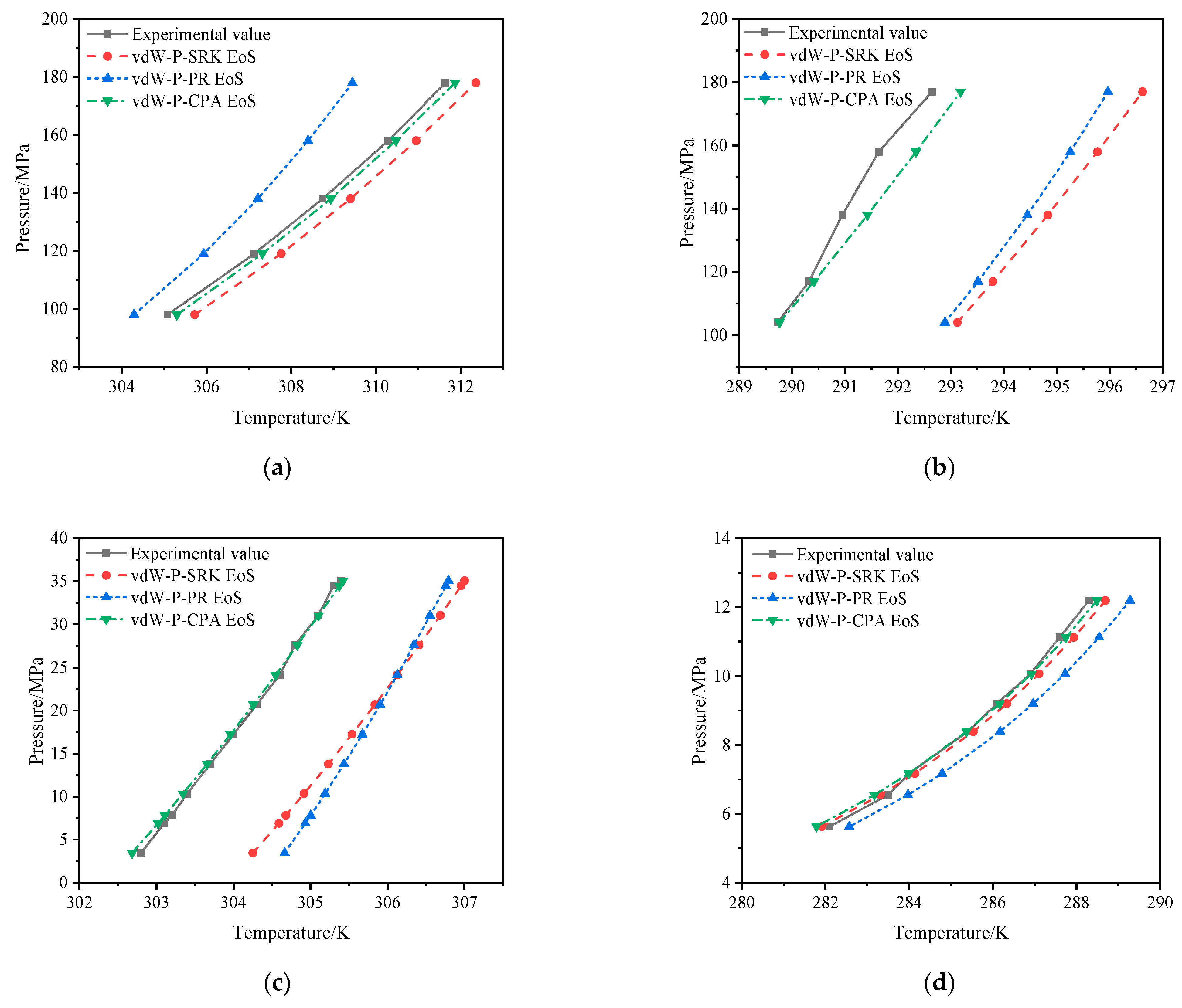
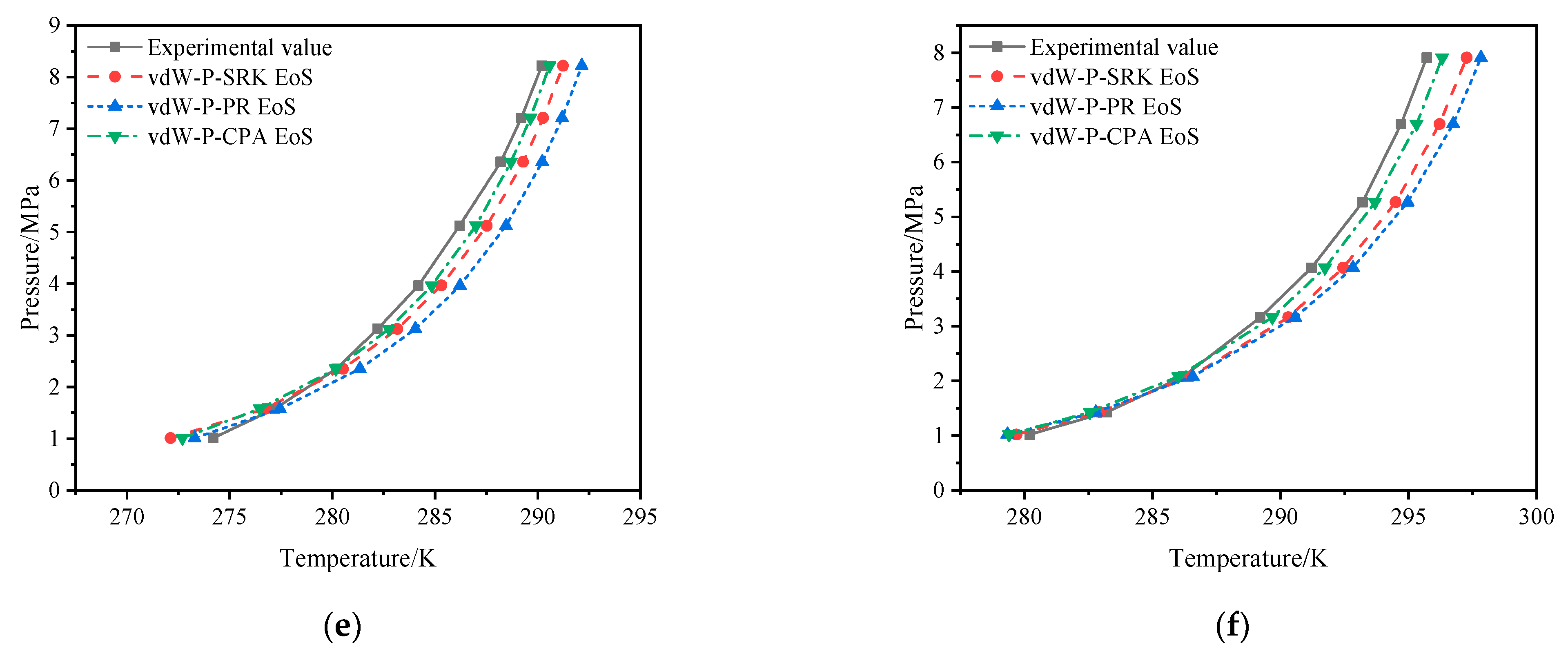
2.3. Verification of Hydrate Formation Condition Prediction Model
3. Calculation Method of Throttling Temperature and Throttling Pressure Difference
3.1. Throttling Temperature Prediction Model
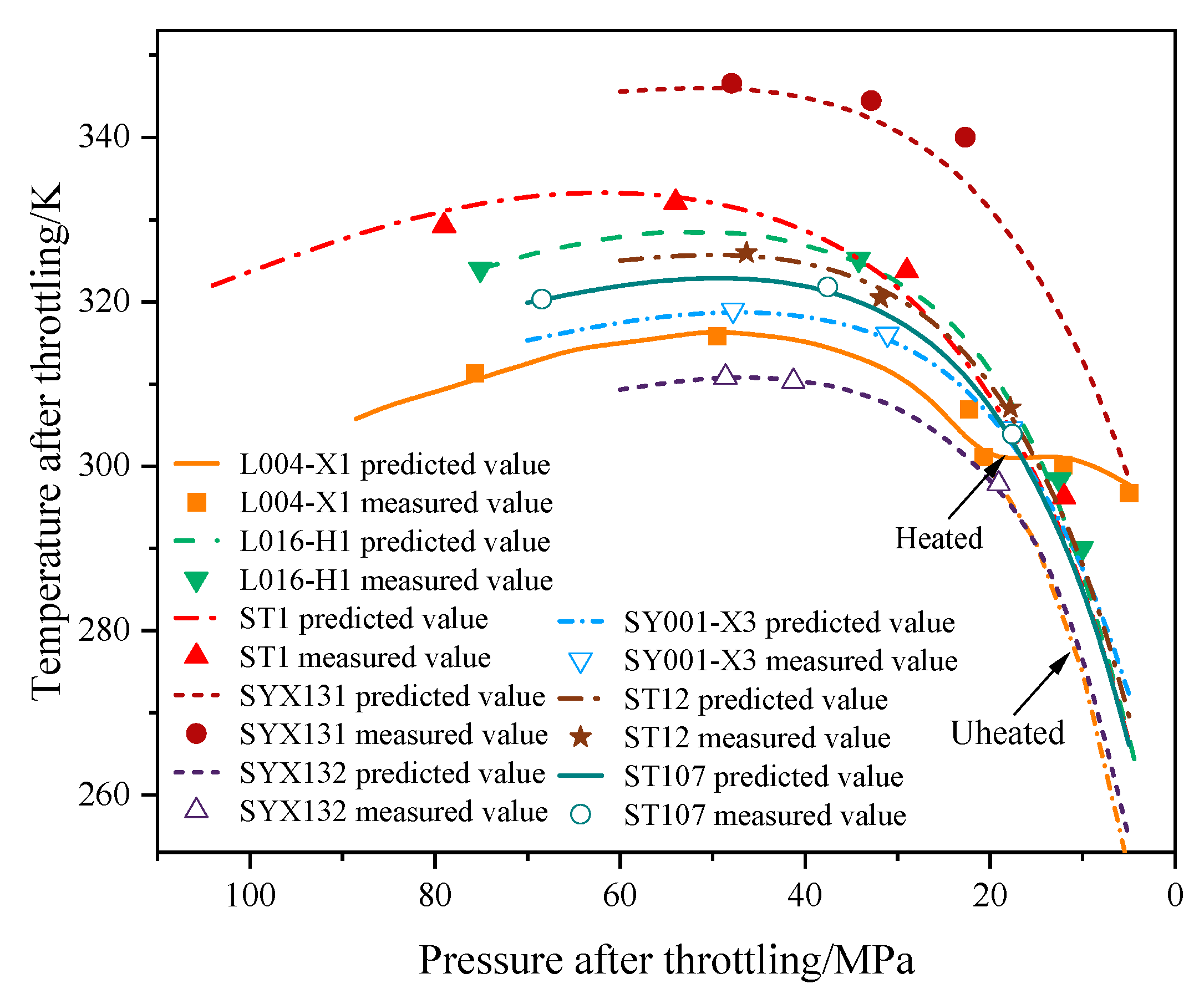
3.2. Model Verification and Throttling Temperature Variation Analysis
3.3. Calculation Method of Throttling Pressure Difference
4. Distribution of Throttling Pressure Difference in Ultra-High-Pressure Sour Gas Wells
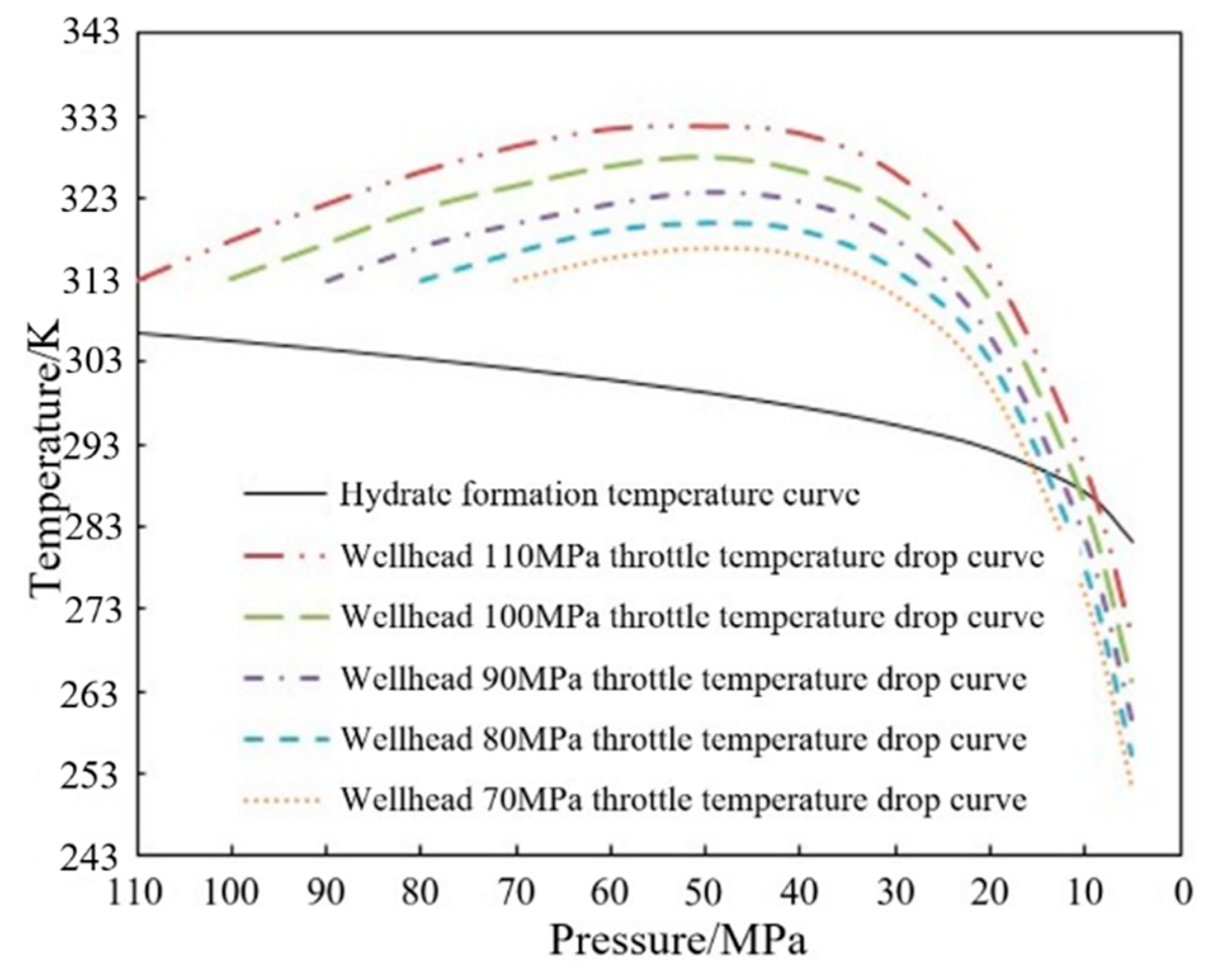
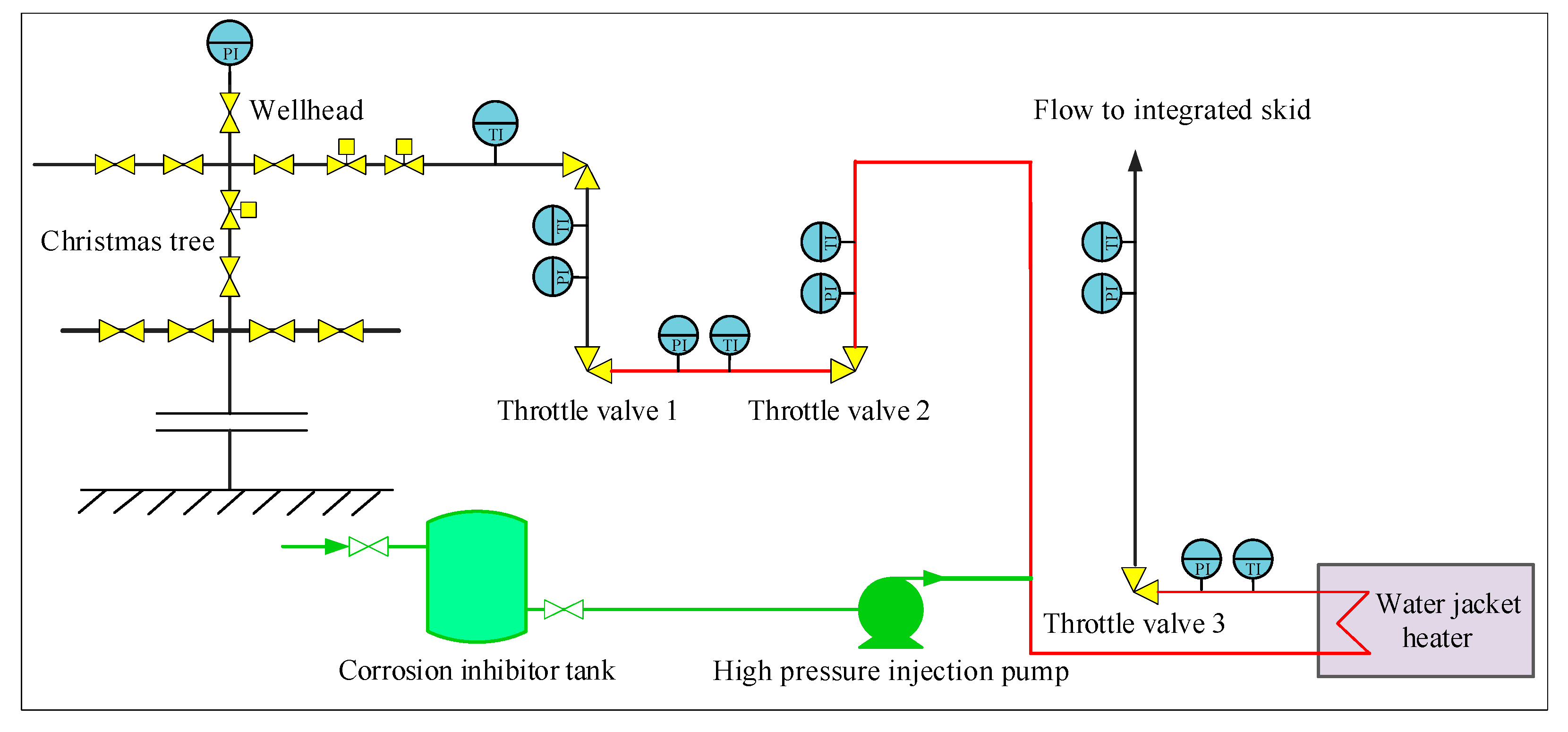
4.1. Traditional Throttling Technology for Ultra-High-Pressure Gas Wells in Northwestern Sichuan
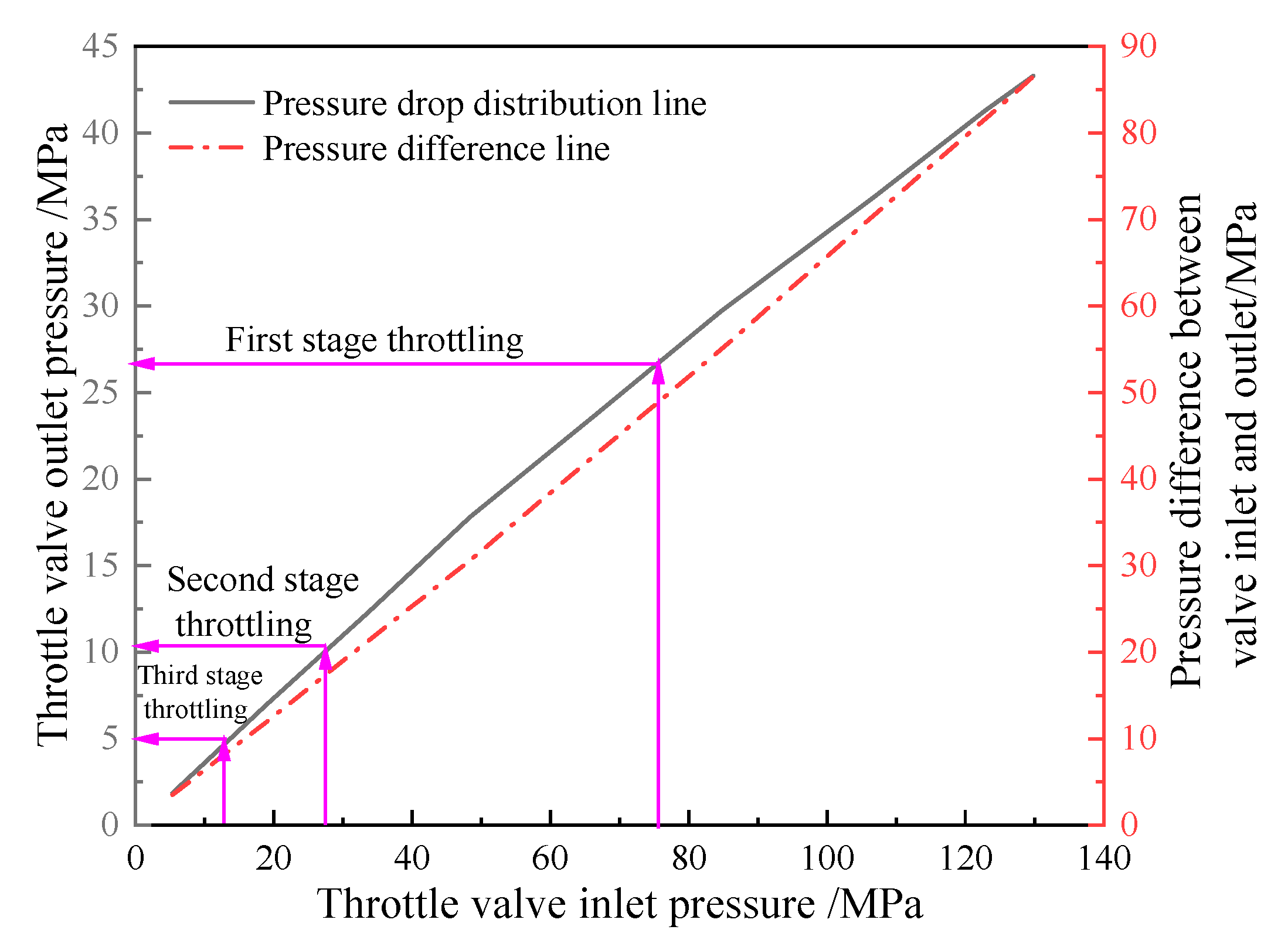
4.2. Optimization of Throttling Technology for Ultra-High-Pressure Sour Gas Wells
5. Conclusions and Recommendations
- (1)
- This work presents an accurate method for predicting hydrate formation conditions of ultra-high-pressure sour gas. The prediction model is constructed based on the vdW-P model and CPA EoS and considers the influence of the intermolecular association between acid gases (H2S, CO2) and H2O. In a wide range of pressures (1.02–178 MPa), the average absolute deviation of the predicted hydrate formation temperature is less than 1 K, and its calculation accuracy is superior to those of the vdW-P model and PR/SRK EoSs;
- (2)
- Based on the Lee–Kesler EoS and the isenthalpic throttling principle, the prediction model for throttling temperature of ultra-high-pressure sour natural gas is proposed. This paper puts forward a method of process simplification that involves making full use of the advantages of temperature rise after throttling in the high-pressure and ultra-high-pressure throttling sections, thus reasonably distributing the throttling pressure difference to simplify the surface throttling series and equipment;
- (3)
- Based on the field test and application of gas wells L004-X1 and L016-H1, the feasibility of selecting a three-stage throttling process for high-pressure and ultra-high-pressure sour natural gas wells was confirmed. The innovatively adopted ultra-high-pressure throttling technology based on the fixed throttle valve with a large pressure difference has fewer throttling stages and requires less equipment compared with the traditional five-stage throttling process;
- (4)
- The exploration and practice of large pressure difference throttling technology provide a solution to the surface hydrate plugging problem in the process of trial production and the development of ultra-high-pressure sour gas. However, the influence of sour media on throttling valve erosion and the reliability of fixed throttling valve application, under conditions of a large pressure difference and high flow rate, need to be further studied. The proposed method can also be further extended to similar industrial processes, such as the injection–production process for depleted gas fields with H2 storage and CO2 sequestration, for hydrate prevention and throttling process optimization.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yang, Y.; Chen, C.; Wen, L.; Chen, X.; Liang, H.; Liu, R.; Guan, X.; Luo, B.; Xie, C. Characteristics of buried structures in northern Longmenshan mountains and its significance to oil and gas exploration in the Sichuan Basin. Nat. Gas Ind. B 2019, 6, 175–182. [Google Scholar] [CrossRef]
- Zhang, B.; Xie, J.; Yin, H.; Hu, X.; Wang, Y.; Yang, X.; Pei, S. Characteristics and exploration direction of the Middle Permian carbonate reservoirs in the Longmenshan mountain areas, western Sichuan Basin. Nat. Gas Ind. 2018, 38, 33–42. [Google Scholar]
- Liu, Q.; Tang, Y.; Luo, Z.; Tian, Z.; Xiao, Z.; Zhou, G. The surface gathering and transportation technology suitable for ultrahigh pressure sulfur gas wells in the northwestern Sichuan Basin. Nat. Gas Ind. 2017, 37, 101–107. [Google Scholar]
- Jia, W.; Yang, F.; Wu, X.; Li, C.; Wang, Y. Predictions on temperatures of high-pressure gas/water/MEG mixtures flowing through wellhead chokes. J. Nat. Gas Sci. Eng. 2020, 74, 103108. [Google Scholar] [CrossRef]
- Vasheghani Farahani, M.; Jahanpeyma, Y.; Taghikhani, V. Dynamic Modeling and Numerical Simulation of Gas Lift Performance in Deviated Oil Well Using the Two-Fluid Model. In Proceedings of the 80th EAGE Conference and Exhibition 2018, Copenhagen, Denmark, 11–14 June 2018; Volume 2018, pp. 1–5. [Google Scholar]
- Zeng, T.; Li, C.; Jia, W. Surface Throttling Process of Abnormally High Pressure Jiulongshan Gas Field. Oil-Gas Field Surf. Eng. 2016, 35, 59–61. [Google Scholar]
- Platteeuw, J.C.; van der Waals, J.H. Thermodynamic properties of gas hydrates. Mol. Phys. 1958, 1, 91–96. [Google Scholar] [CrossRef]
- Ng, H.J.; Robinson, D.B. The measurement and prediction of hydrate formation in liquid hydrocarbon-water systems. Ind. Eng. Chem. Fundam. 1976, 15, 293–298. [Google Scholar] [CrossRef]
- Valiollahi, S.; Kavianpour, B.; Raeissi, S.; Moshfeghian, M. A new Peng-Robinson modification to enhance dew point estimations of natural gases. J. Nat. Gas Sci. Eng. 2016, 34, 1137–1147. [Google Scholar] [CrossRef]
- Epelle, E.I.; Bennett, J.; Abbas, H.; Schmidt, K.A.G.; Vesovic, V. Correlation of binary interaction coefficients for hydrate inhibition using the Soave-Redlich-Kwong Equation of State and the Huron-Vidal mixing rule. J. Nat. Gas Sci. Eng. 2020, 77, 103259. [Google Scholar] [CrossRef]
- Jia, W.L.; Yang, F.; Li, C.J.; Huang, T.; Song, S.S. A unified thermodynamic framework to compute the hydrate formation conditions of acidic gas/water/alcohol/electrolyte mixtures up to 186.2 MPa. Energy 2021, 230, 120735. [Google Scholar] [CrossRef]
- Kontogeorgis, G.M.; Folas, G.K. Thermodynamic Models for Industrial Applications; John Wiley & Sons: New York, NY, USA, 2009. [Google Scholar]
- Hassanpouryouzband, A.; Farahani, M.V.; Yang, J.; Tohidi, B.; Chuvilin, E.; Istomin, V.; Bukhanov, B. Solubility of Flue Gas or Carbon Dioxide-Nitrogen Gas Mixtures in Water and Aqueous Solutions of Salts: Experimental Measurement and Thermodynamic Modeling. Ind. Eng. Chem. Res. 2019, 58, 3377–3394. [Google Scholar] [CrossRef]
- Lee, B.I.; Kesler, M.G. A generalized thermodynamic correlation based on three-parameter corresponding states. AIChE J. 1975, 21, 510–527. [Google Scholar] [CrossRef]
- Li, C.; Jia, W.; Wu, X. Temperature Prediction for High Pressure High Temperature Condensate Gas Flow Through Chokes. Energies 2012, 5, 670–682. [Google Scholar] [CrossRef]
- Leung, J.C. A generalized correlation for one-component homogeneous equilibrium flashing choked flow. AIChE J. 1986, 32, 1743–1746. [Google Scholar] [CrossRef]
- Henry, R.E.; Fauske, H.K. The Two-Phase Critical Flow of One-Component Mixtures in Nozzles, Orifices, and Short Tubes. J. Heat Transf. 1971, 93, 179–187. [Google Scholar] [CrossRef]
- Schmidt, J.; Claramunt, S. Sizing of rupture disks for two-phase gas/liquid flow according to HNE-CSE-model. J. Loss Prev. Process Ind. 2016, 41, 419–432. [Google Scholar] [CrossRef]
- Avlonitis, D. The determination of kihara potential parameters from gas hydrate data. Chem. Eng. Sci. 1994, 49, 1161–1173. [Google Scholar] [CrossRef]
- Sloan, E.D., Jr.; Koh, C.A.; Koh, C.A. Clathrate Hydrates of Natural Gases; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Voutsas, E.C.; Kontogeorgis, G.M.; Yakoumis, I.V.; Tassios, D.P. Correlation of liquid-liquid equilibria for alcoholhydrocarbon mixtures using the CPA equation of state. Fluid Phase Equilibria 1997, 132, 61–75. [Google Scholar] [CrossRef]
- Tsivintzelis, I.; Kontogeorgis, G.M.; Michelsen, M.L.; Stenby, E.H. Modeling phase equilibria for acid gas mixtures using the CPA equation of state. Part II: Binary mixtures with CO2. Fluid Phase Equilibria 2011, 306, 38–56. [Google Scholar] [CrossRef]
- Tsivintzelis, I.; Kontogeorgis, G.M.; Michelsen, M.L.; Stenby, E.H. Modeling phase equilibria for acid gas mixtures using the CPA equation of state. I. Mixtures with H2S. AIChE J. 2010, 56, 2965–2982. [Google Scholar] [CrossRef]
- Nakano, S.; Moritoki, M.; Ohgaki, K. High-Pressure Phase Equilibrium and Raman Microprobe Spectroscopic Studies on the Methane Hydrate System. J. Chem. Eng. Data 1999, 44, 254–257. [Google Scholar] [CrossRef]
- Nakano, S.; Moritoki, M.; Ohgaki, K. High-Pressure Phase Equilibrium and Raman Microprobe Spectroscopic Studies on the CO2 Hydrate System. J. Chem. Eng. Data 1998, 43, 807–810. [Google Scholar] [CrossRef]
- Selleck, F.T.; Carmichael, L.T.; Sage, B.H. Phase behavior in the hydrogen sulfide-water system. Ind. Eng. Chem. 1952, 44, 2219–2226. [Google Scholar] [CrossRef]
- Aghajanloo, M.; Taheri, Z.; Behbahani, T.J.; Mohammadi, A.H.; Ehsani, M.R.; Heydarian, H. Experimental determination and thermodynamic modeling of clathrate hydrate stability conditions in methane + hydrogen sulfide + water system. J. Nat. Gas Sci. Eng. 2020, 83, 103549. [Google Scholar] [CrossRef]
- Huang, Q.; Sun, C.; Chen, G.; Yang, L. Hydrate formation conditions of (CH4+CO2+H2S) ternary sour natural gases. CIESC J. 2005, 48, 1159–1163. [Google Scholar]
- Teng, L.; Zhang, D.; Li, Y.; Wang, W.; Wang, L.; Hu, Q.; Ye, X.; Bian, J.; Teng, W. Multiphase mixture model to predict temperature drop in highly choked conditions in CO2 enhanced oil recovery. Appl. Therm. Eng. 2016, 108, 670–679. [Google Scholar] [CrossRef] [Green Version]
- Jia, W.L. Basic Theory and Simulation for High Temperature and High Pressure Condensate Gas Choke Process. Master’s Thesis, Southwest Petroleum University, Chengdu, China, 2011. [Google Scholar]
- API. American Petroleum Institute Data Book, 7th ed.; EPCON International: Houston, TX, USA, 2005. [Google Scholar]
- Hassanpouryouzband, A.; Joonaki, E.; Edlmann, K.; Haszeldine, R.S. Offshore Geological Storage of Hydrogen: Is This Our Best Option to Achieve Net-Zero? ACS Energy Lett. 2021, 6, 2181–2186. [Google Scholar] [CrossRef]

| Gas Components | H2O | CH4 | H2S | CO2 |
|---|---|---|---|---|
| H2O | 0.0000 | −0.0398 | 0.0816 | −0.0559 |
| CH4 | −0.0398 | 0.0000 | 0.0888 | 0.0956 |
| H2S | 0.0816 | 0.0888 | 0.0000 | 0.1150 |
| CO2 | −0.0559 | 0.0956 | 0.1150 | 0.0000 |
| Molecular A | Molecular B | Association Scheme | βAB | εAB (Pa∙m3mol−1) | Reference |
|---|---|---|---|---|---|
| H2O | H2O | 4C | 0.0692 | 16.6550 | [12] |
| H2S | H2O | 3B | 0.2329 | 3.7810 | [23] |
| CO2 | H2O | Solvation | 0.1836 | 8.3275 | [21] |
| Component | Mole Fraction (mol%) | |||||
|---|---|---|---|---|---|---|
| NG1 | NG2 | NG3 | NG4 | NG5 | NG6 | |
| CH4 | 100.00 | 0.00 | 0.00 | 99.50 | 87.65 | 77.71 |
| CO2 | 0.00 | 100.00 | 0.00 | 0.00 | 7.40 | 7.31 |
| H2S | 0.00 | 0.00 | 100.00 | 0.50 | 4.95 | 14.98 |
| NG1 [24] | NG2 [25] | NG3 [26] | NG4 [27] | NG5 [28] | NG6 [29] | |
|---|---|---|---|---|---|---|
| Temperature (K) | 305.08~311.64 | 289.73~292.64 | 302.80~305.40 | 282.10~288.30 | 282.20~297.20 | 276.20~291.20 |
| Pressure (MPa) | 98.00–178.00 | 104.00~177.00 | 3.45~35.07 | 5.63~12.19 | 0.95~8.68 | 1.11~8.02 |
| Experimental System | Number of Data Points | Pressure Range (MPa) | AAD (K) | ||
|---|---|---|---|---|---|
| vdW-P -PR EoS | vdW-P -SRK EoS | vdW-P -CPA EoS | |||
| NG1 | 5 | 98.00–178.00 | 1.52 | 0.66 | 0.20 |
| NG2 | 5 | 104.00~177.00 | 3.36 | 3.77 | 0.37 |
| NG3 | 12 | 3.45~35.07 | 1.64 | 1.54 | 0.05 |
| NG4 | 8 | 5.63~12.19 | 0.77 | 0.23 | 0.14 |
| NG5 | 9 | 1.04~8.22 | 1.59 | 1.05 | 0.74 |
| NG6 | 8 | 1.02~7.91 | 1.32 | 0.97 | 0.54 |
| Well Number | Inlet of the Throttle Valve | Outlet of the Throttle Valve | Deviation (K) | |||
|---|---|---|---|---|---|---|
| Measured Pressure (MPa) | Measured Temperature (K) | Measured Pressure (MPa) | Measured Temperature (K) | Predicted Temperature (K) | ||
| L004-X1 | 88.50 | 305.60 | 75.70 | 311.20 | 310.46 | −0.74 |
| 75.70 | 311.20 | 49.50 | 315.70 | 316.42 | 0.72 | |
| 49.50 | 315.70 | 22.30 | 306.80 | 303.35 | −3.45 | |
| 22.30 | 306.80 | 20.70 | 301.00 | 301.21 | 0.21 | |
| 21.00 | 320.80.2 | 12.10 | 300.02 | 302.05 | 2.03 | |
| 12.00 | 320.40 2 | 5.00 | 296.61 | 297.60 | 0.99 | |
| L016-H1 | 75.10 | 323.81 | 34.20 | 325.00 | 325.17 | 0.17 |
| 34.20 | 325.00 | 12.50 | 298.20 | 296.06 | −2.14 | |
| 12.50 | 298.20 | 10.21 | 289.59 | 291.25 | 1.66 | |
| 10.21 | 289.59 | 10.07 | 289.84 | 289.29 | −0.55 | |
| ST1 | 104.00 | 321.84 | 79.00 | 329.12 | 332.24 | 3.15 |
| 79.00 | 329.12 | 54.00 | 331.94 | 334.47 | 2.80 | |
| 54.00 | 331.94 | 29.00 | 323.58 | 325.43 | 1.85 | |
| 29.00 | 323.58 | 12.00 | 296.10 | 294.96 | −1.14 | |
| SYX131 | 47.94 | 346.42 | 32.82 | 344.31 | 343.13 | −1.18 |
| 32.82 | 344.31 | 22.67 | 339.86 | 337.24 | −2.62 | |
| SY132 | 48.57 | 310.67 | 41.25 | 310.15 | 310.38 | 0.23 |
| 41.25 | 310.15 | 19.05 | 297.71 | 296.62 | −1.09 | |
| SY001-X3 | 47.78 | 318.84 | 31.07 | 315.92 | 316.03 | 0.11 |
| 31.07 | 315.92 | 17.69 | 304.42 | 304.67 | 0.25 | |
| ST12 | 46.31 | 325.80 | 31.83 | 320.34 | 322.21 | 1.87 |
| 31.83 | 320.34 | 17.80 | 306.95 | 306.63 | −0.32 | |
| ST107 | 68.44 | 320.16 | 37.54 | 321.66 | 321.65 | −0.01 |
| 37.54 | 321.66 | 17.59 | 303.72 | 305.42 | 1.70 | |
| Average absolute deviation | 1.29 | |||||
| Well Number | L004-X1 | L016-H1 | ||||
|---|---|---|---|---|---|---|
| Location | Pressure (MPa) | Temperature (K) | Hydrate Formation Temperature (K) | Pressure (MPa) | Temperature (K) | Hydrate Formation Temperature (K) |
| Wellhead | 75.70 | 310.3 | 303.0 | 77.49 | 324.6 | 302.7 |
| Outlet of throttle valve 1 | 49.50 | 312.8 | 299.4 | 34.40 | 319.0 | 295.7 |
| Outlet of throttle valve 2 | 22.30 | 298.8 | 293.4 | 12.50 | 297.0 | 287.8 |
| Outlet of throttle valve 3 | 20.70 | 296.5 | 292.9 | 10.20 | 289.2 | 286.0 |
| Outlet of throttle valve 4 | 12.10 | 300.0 | 288.9 | 10.10 | 289.5 | 286.0 |
| Outlet of throttle valve 5 (unheated) | 5.00 | 273.3 | 281.4 | 4.70 | 267.5 | 278.9 |
| Well Number | L004-X1 | L016-H1 | ||||
|---|---|---|---|---|---|---|
| Location | Pressure (MPa) | Temperature (K) | Hydrate Formation Temperature (K) | Pressure (MPa) | Temperature (K) | Hydrate Formation Temperature (K) |
| Wellhead | 75.7 | 310.3 | 303.0 | 77.5 | 324.6 | 302.7 |
| Outlet of throttle valve 1 | 26.5 | 308.1 | 294.6 | 28.1 | 322.5 | 294.1 |
| Outlet of throttle valve 2 | 10.5 | 276.3 | 287.8 | 10.5 | 291.4 | 286.3 |
| Outlet of throttle valve 3 1 | 5.0 | 251.2 | 281.4 | 5.0 | 269.2 | 279.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luo, Z.; Liu, Q.; Yang, F.; Li, Z.; Wang, H.; Wang, B.; Peng, Z.; Jia, W. Research and Application of Surface Throttling Technology for Ultra-High-Pressure Sour Natural Gas Wells in Northwestern Sichuan Basin. Energies 2022, 15, 8641. https://doi.org/10.3390/en15228641
Luo Z, Liu Q, Yang F, Li Z, Wang H, Wang B, Peng Z, Jia W. Research and Application of Surface Throttling Technology for Ultra-High-Pressure Sour Natural Gas Wells in Northwestern Sichuan Basin. Energies. 2022; 15(22):8641. https://doi.org/10.3390/en15228641
Chicago/Turabian StyleLuo, Zhaoqian, Qilin Liu, Fan Yang, Ziyuan Li, Huanhuan Wang, Bo Wang, Zhouyu Peng, and Wenlong Jia. 2022. "Research and Application of Surface Throttling Technology for Ultra-High-Pressure Sour Natural Gas Wells in Northwestern Sichuan Basin" Energies 15, no. 22: 8641. https://doi.org/10.3390/en15228641
APA StyleLuo, Z., Liu, Q., Yang, F., Li, Z., Wang, H., Wang, B., Peng, Z., & Jia, W. (2022). Research and Application of Surface Throttling Technology for Ultra-High-Pressure Sour Natural Gas Wells in Northwestern Sichuan Basin. Energies, 15(22), 8641. https://doi.org/10.3390/en15228641






