Analysis and Optimization of a Novel Flux Reversal Machine with Auxiliary Teeth
Abstract
1. Introduction
2. Topology and Operation Principle
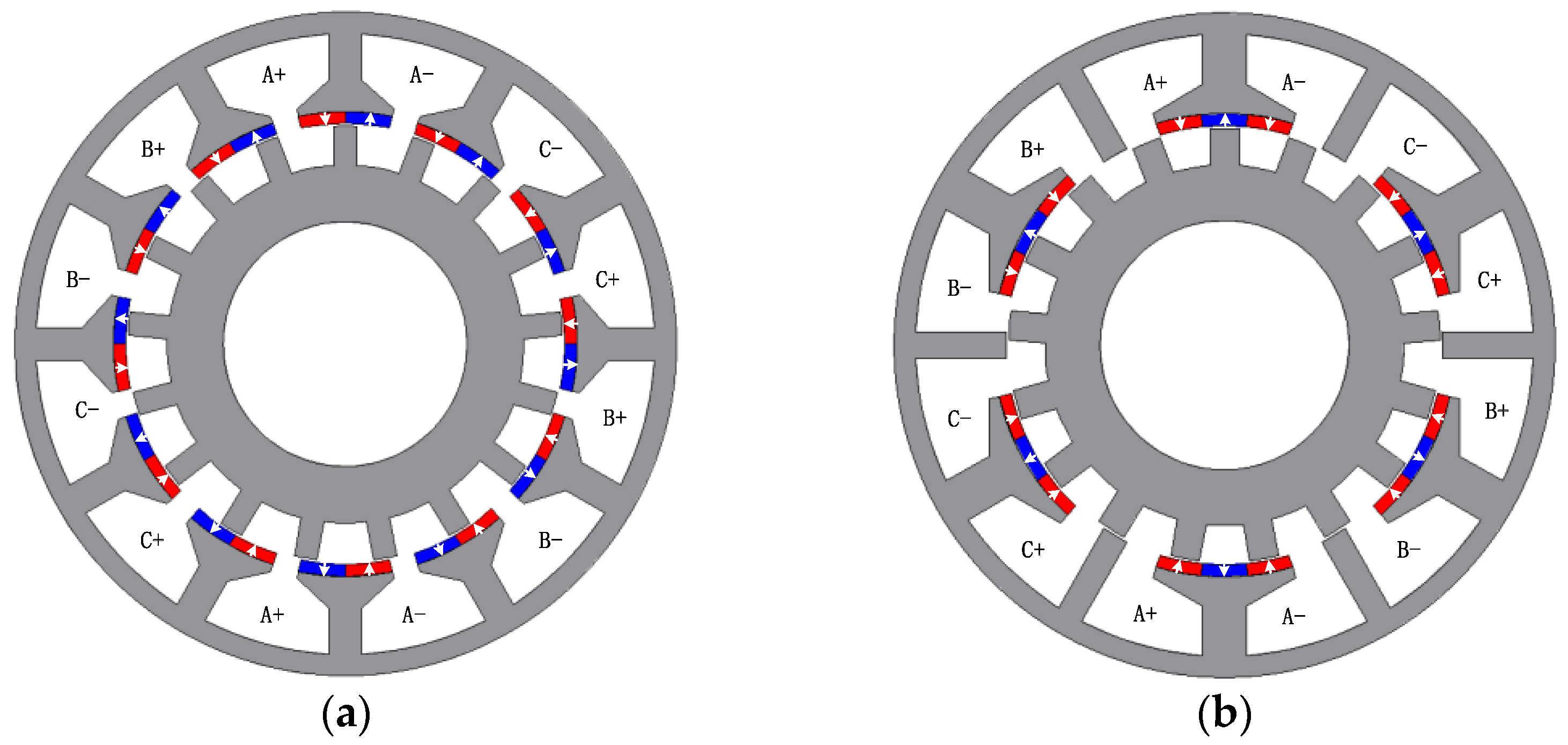
2.1. Topology
2.2. Operation Principle
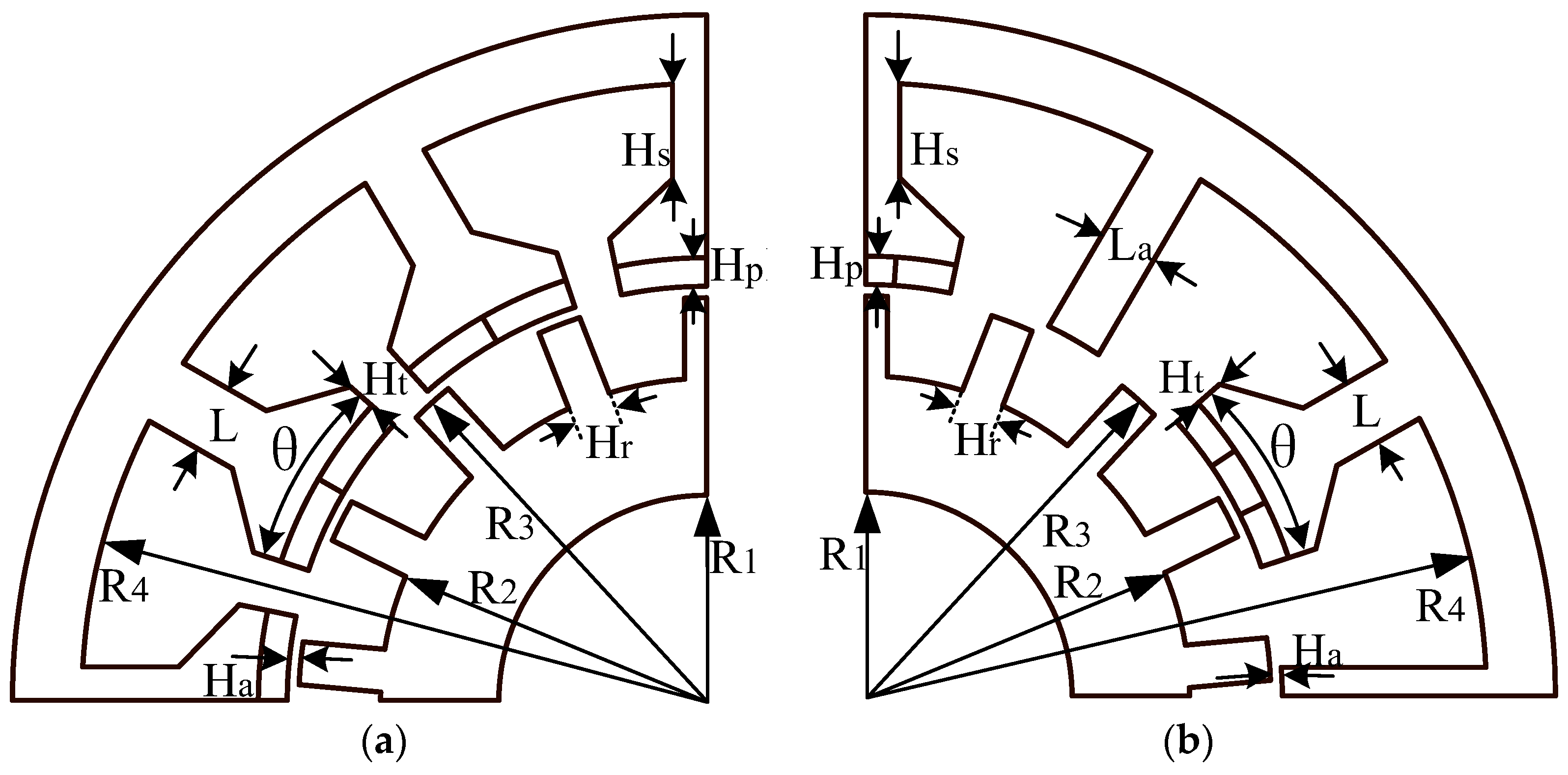
3. Parametric Optimization
4. Comparison of Electromagnetic Performance
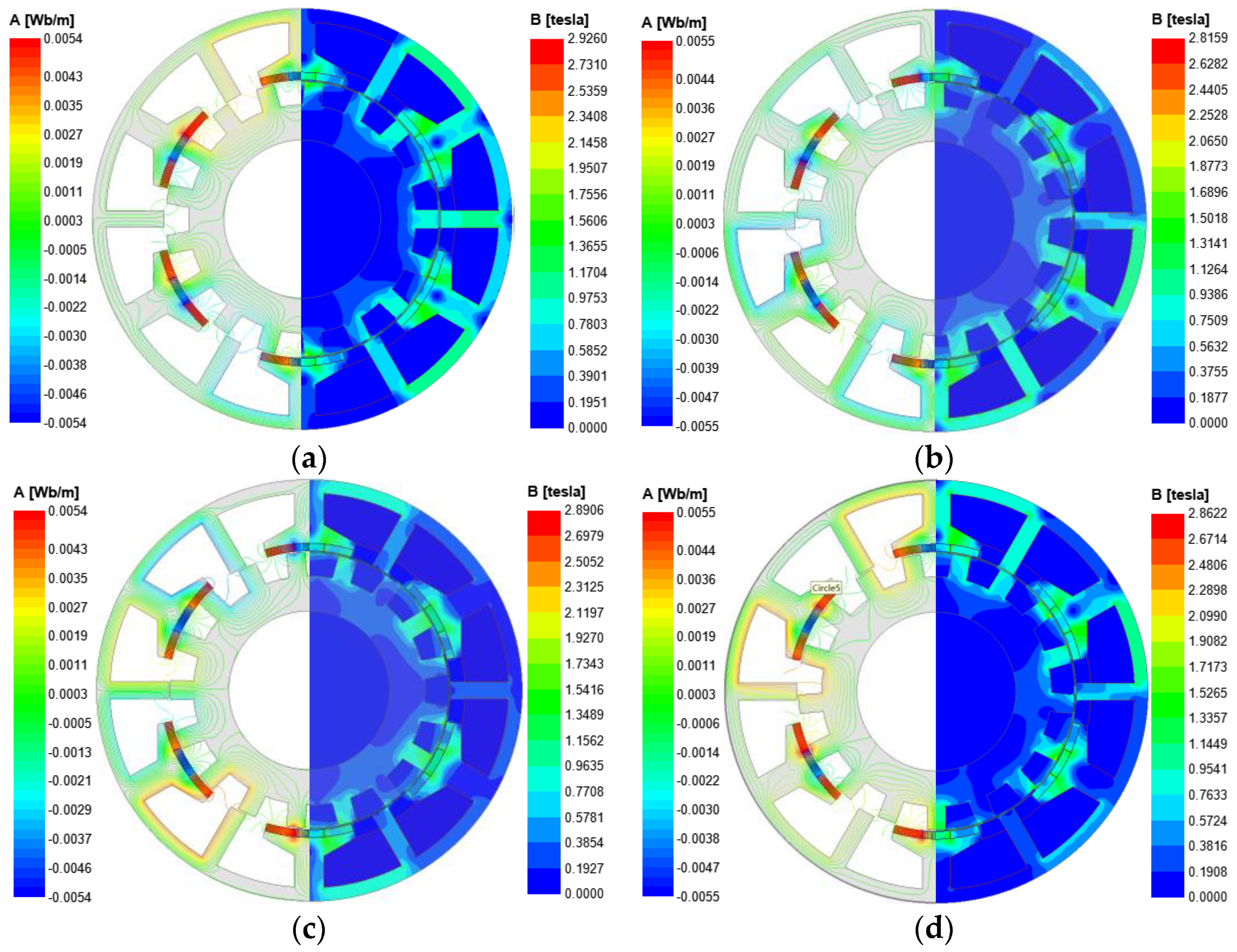
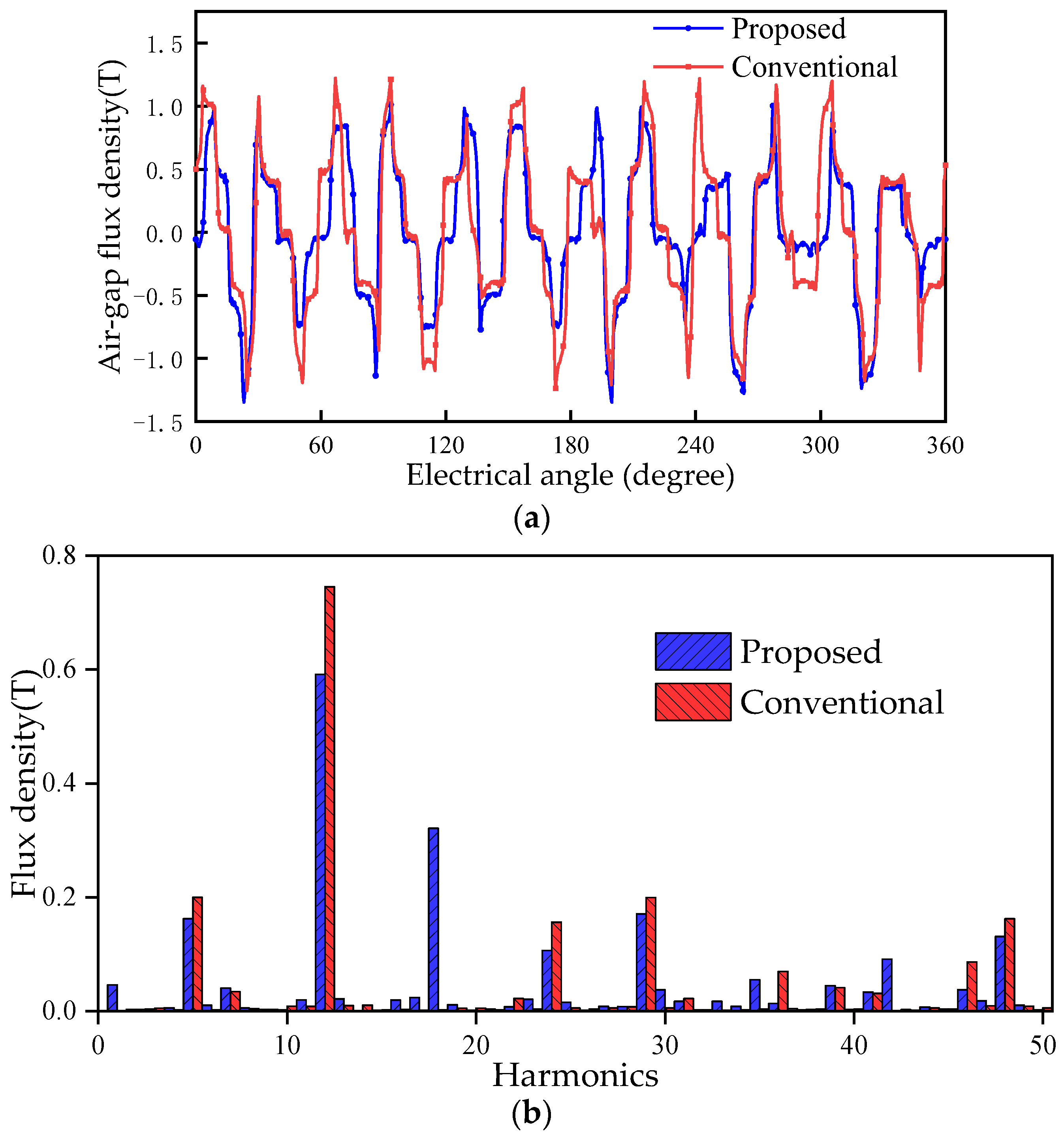
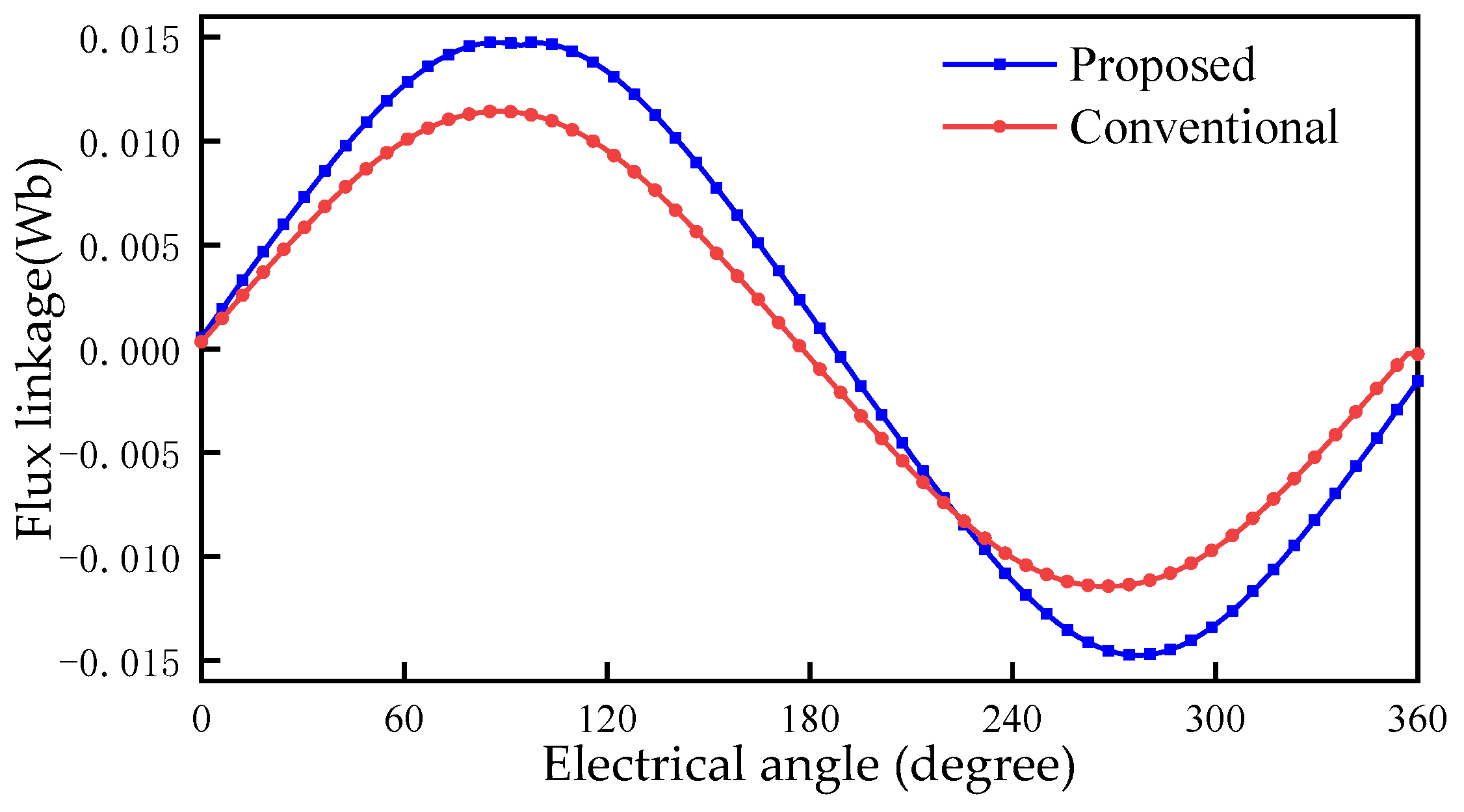
4.1. Flux Density
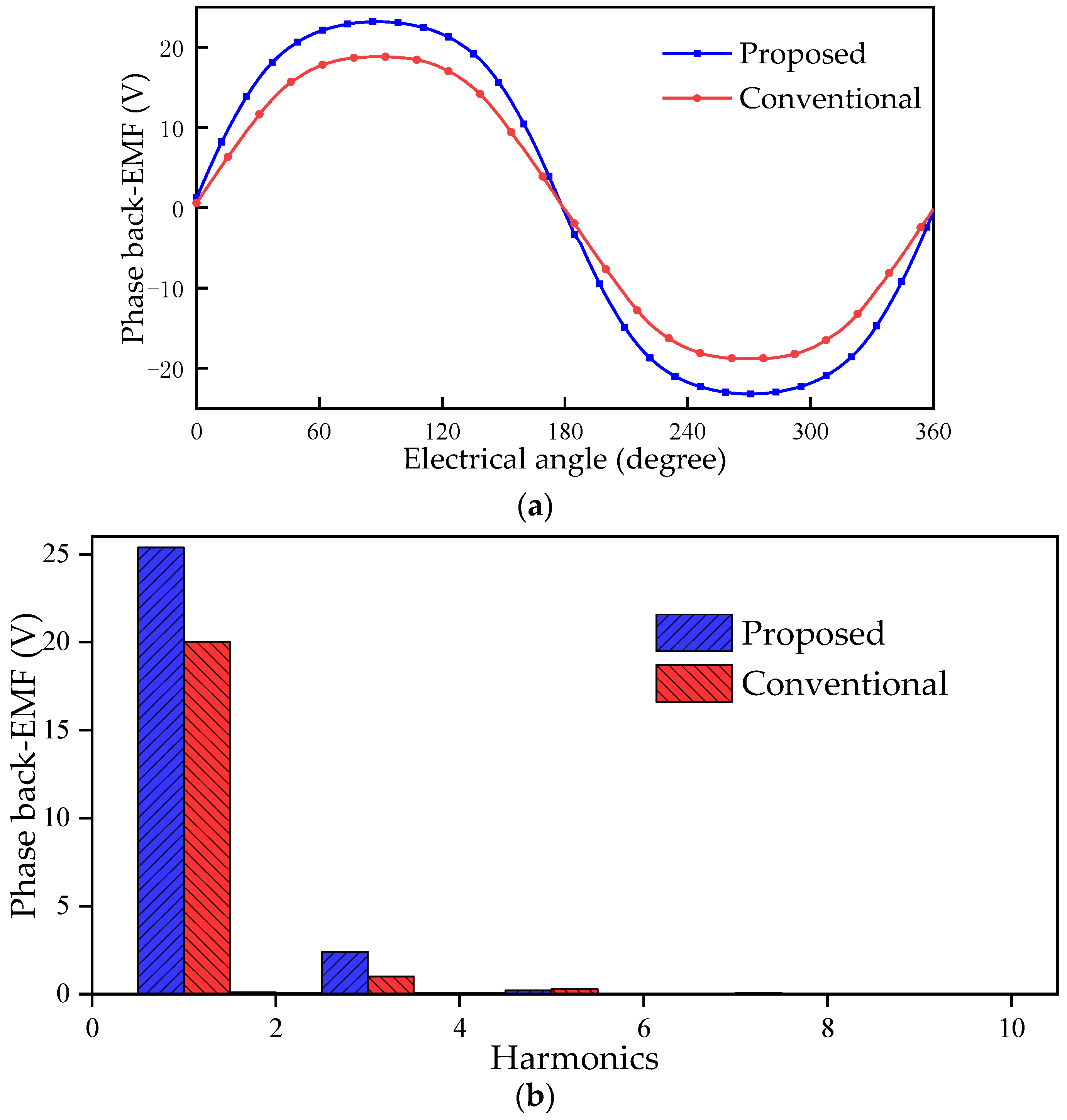
4.2. Back EMF
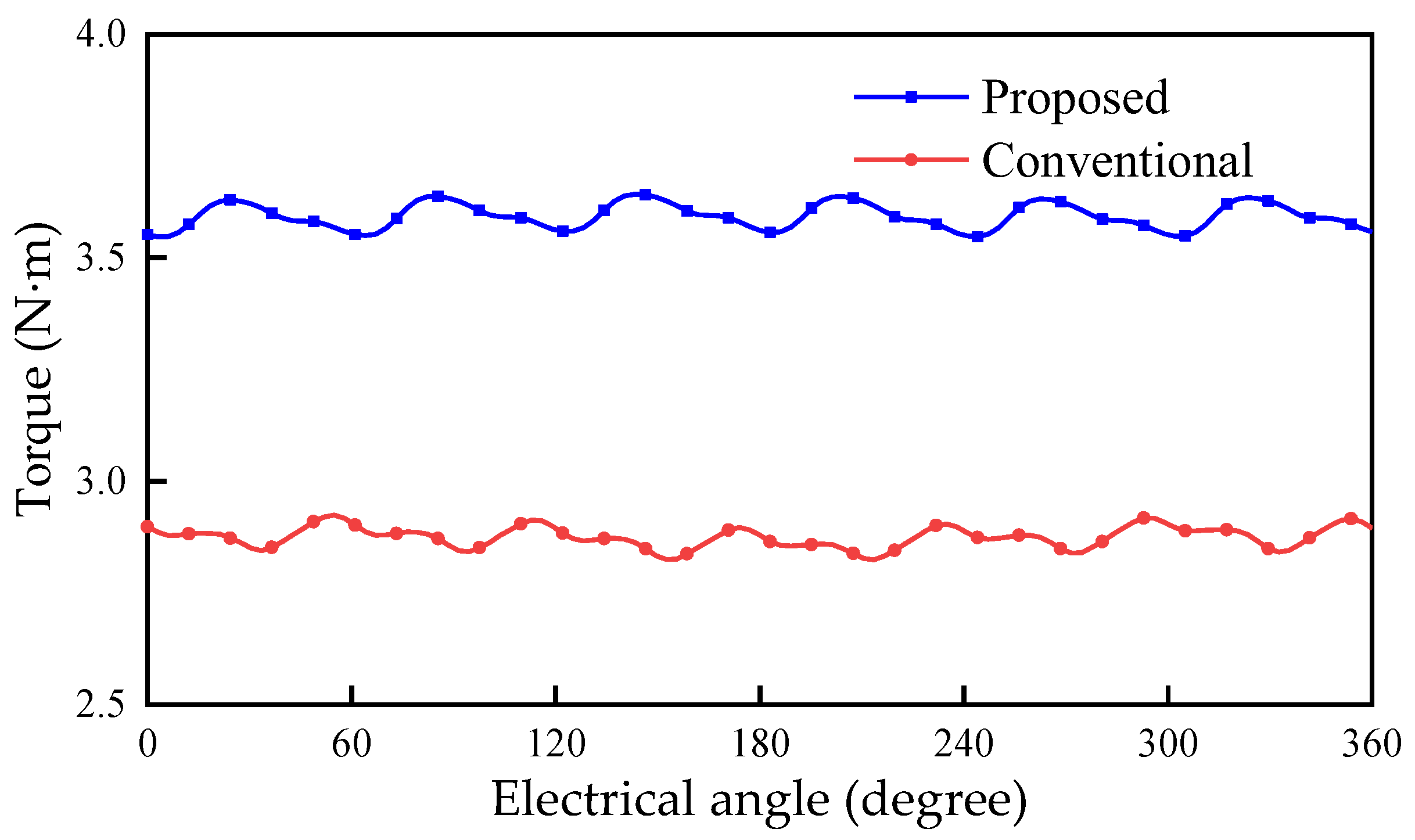
4.3. Torque
4.4. Overload Capability
4.5. Power Factor
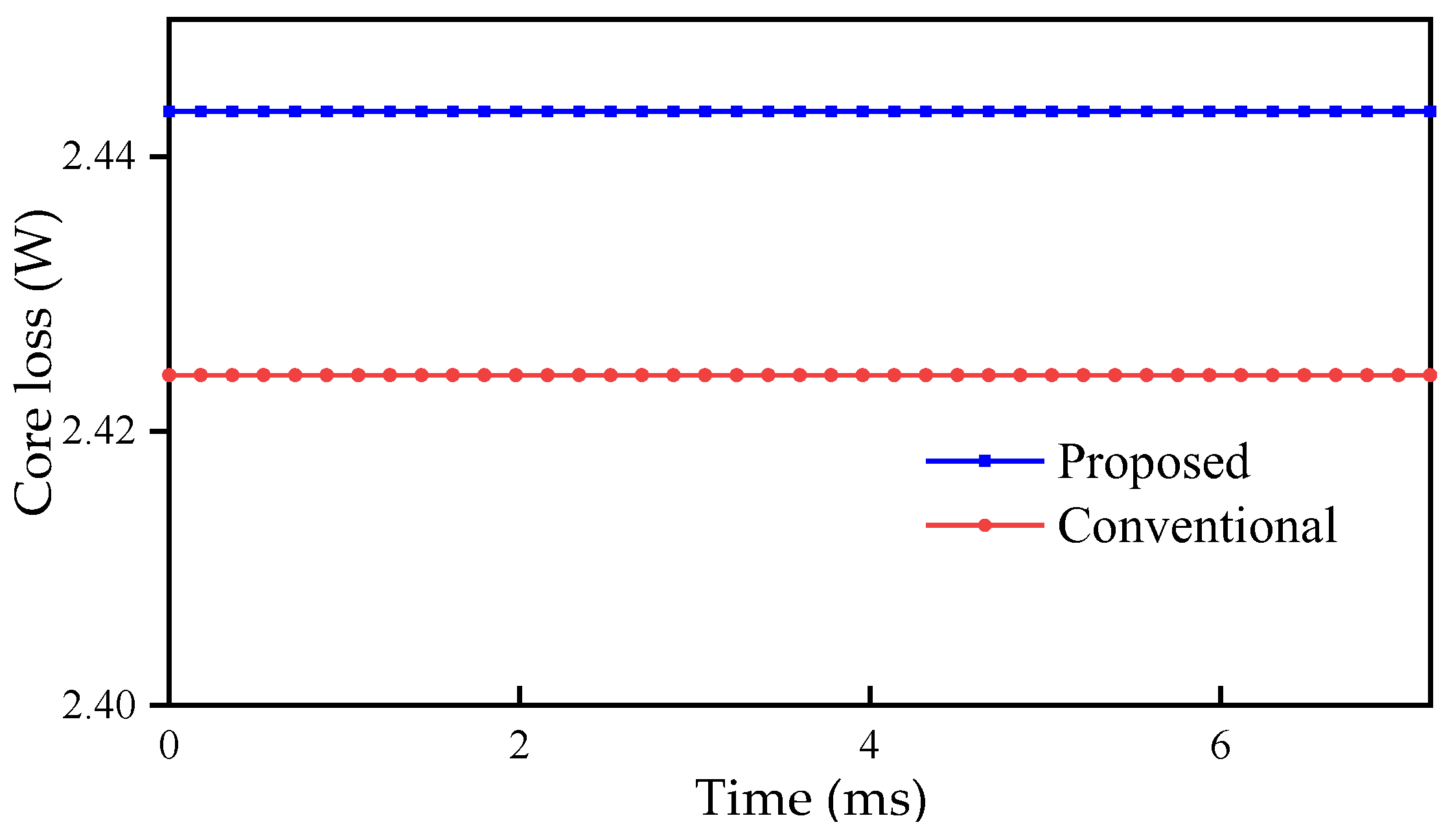
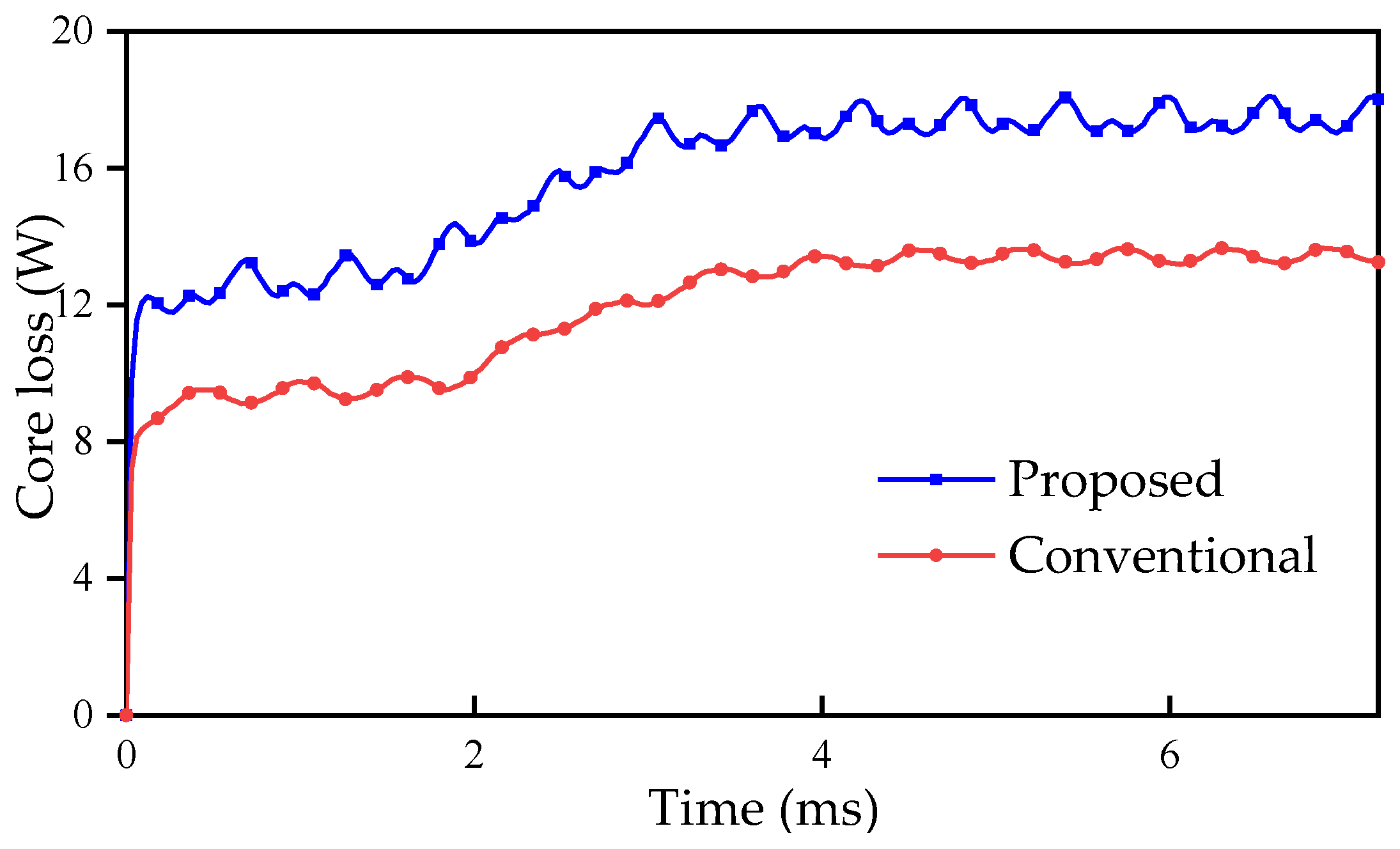
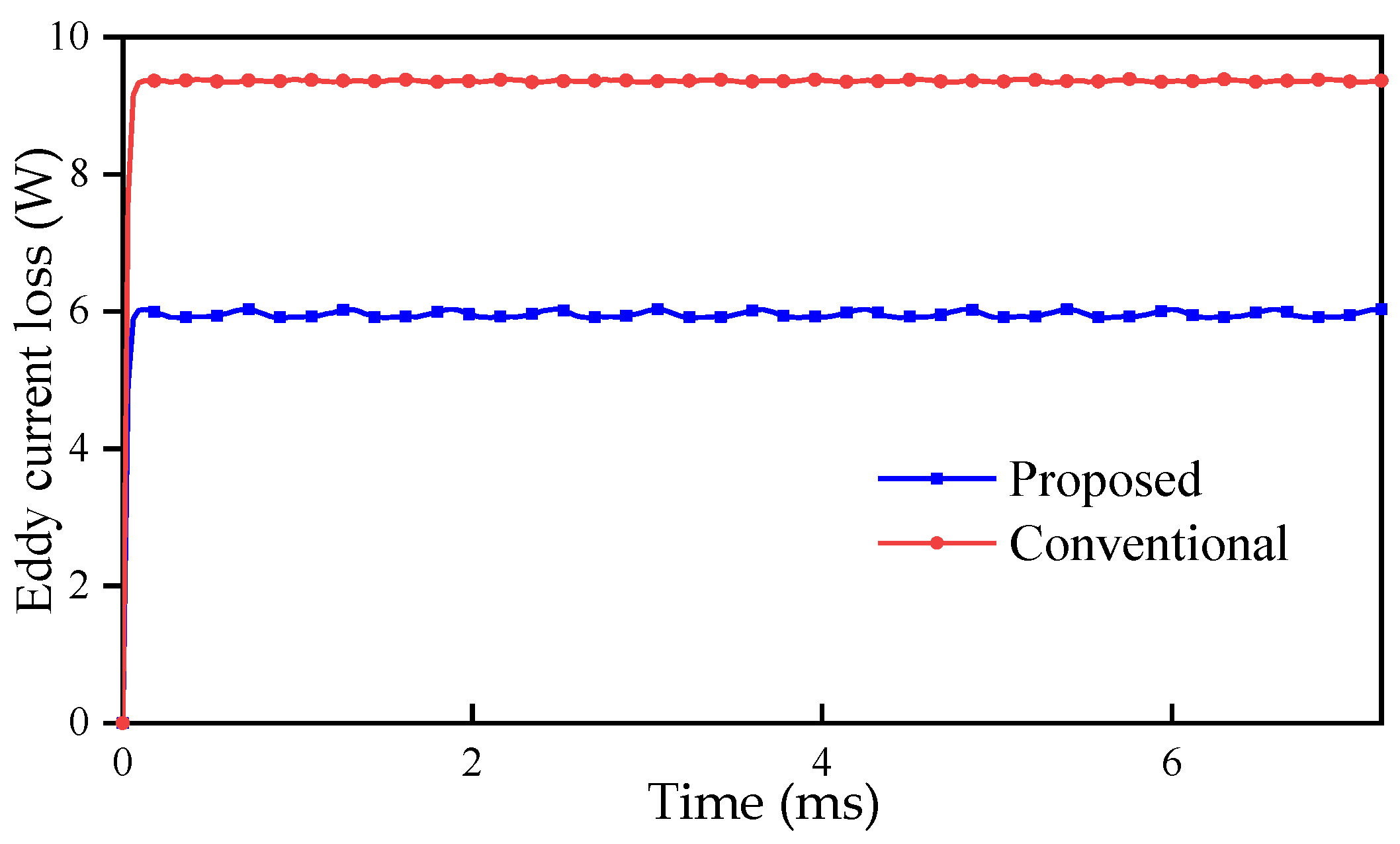
4.6. Loss and Efficiency
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Jing, L.; Pan, Y.; Wang, T.; Qu, R.; Cheng, P. Transient Analysis and Verification of a Magnetic Gear Integrated Permanent Magnet Brushless Machine with Halbach Arrays. IEEE J. Emerg. Sel. Top. Power Electr. 2022, 10, 1881–1890. [Google Scholar] [CrossRef]
- Manne, B.; Kiran Kumar, M.; Akuru, U.B. Design and Performance Assessment of a Small-Scale Ferrite-PM Flux Reversal Wind Generator. Energies 2020, 13, 5565. [Google Scholar] [CrossRef]
- Gao, Y.; Qu, R.; Li, D.; Li, J. Torque Performance Analysis of Three-Phase Flux Reversal Machines. IEEE Trans. Ind. Appl. 2017, 53, 2110–2119. [Google Scholar] [CrossRef]
- Dmitrievskii, V.; Prakht, V.; Kazakbaev, V.; Sarapulov, S. Optimal Design of a High-Speed Single-Phase Flux Reversal Motor for Vacuum Cleaners. Energies 2018, 11, 3334. [Google Scholar] [CrossRef]
- Gao, Y.; Li, D.; Qu, R.; Li, J. Design Procedure of Flux Reversal Permanent Magnet Machines. IEEE Trans. Ind. Appl. 2017, 53, 4232–4241. [Google Scholar] [CrossRef]
- Zhu, X.; Hua, W.; Wang, W.; Huang, W. Analysis of Back-EMF in Flux-Reversal Permanent Magnet Machines by Air Gap Field Modulation Theory. IEEE Trans. Ind. Electron. 2019, 66, 3344–3355. [Google Scholar] [CrossRef]
- Hua, W.; Zhu, X.; Wu, Z. Influence of Coil Pitch and Stator-Slot/Rotor-Pole Combination on Back EMF Harmonics in Flux-Reversal Permanent Magnet Machines. IEEE Trans. Energy Convers. 2018, 33, 1330–1341. [Google Scholar] [CrossRef]
- Li, H.; Zhu, Z.Q.; Hua, H. Comparative Analysis of Flux Reversal Permanent Magnet Machines with Toroidal and Concentrated Windings. IEEE Trans. Ind. Electron. 2020, 67, 5278–5290. [Google Scholar] [CrossRef]
- Yang, H.; Lin, H.; Zhu, Z.Q.; Lyu, S.; Liu, Y. Design and Analysis of Novel Asymmetric-Stator-Pole Flux Reversal PM Machine. IEEE Trans. Ind. Electron. 2020, 67, 101–114. [Google Scholar] [CrossRef]
- Liu, W.; Yang, H.; Lin, H.; Qin, L. Hybrid Analytical Modeling of Air-Gap Magnetic Field in Asymmetric-Stator-Pole Flux Reversal Permanent Magnet Machine Considering Slotting Effect. IEEE Trans. Ind. Electron. 2022, 69, 1739–1749. [Google Scholar] [CrossRef]
- Wu, L.; Zheng, Y.; Fang, Y.; Huang, X. Novel Fault-Tolerant Doubly Fed Flux Reversal Machine with Armature Windings Wound on Both Stator and Rotor Teeth. IEEE Trans. Ind. Electron. 2021, 68, 4780–4789. [Google Scholar] [CrossRef]
- Zheng, Y.; Wu, L.; Li, H.; Wen, H.; Qiu, L. Harmonic Analysis of Airgap Magnetic Fields in Doubly-Fed Flux Reversal Permanent Magnet Machines. IEEE Access 2020, 8, 134856–134867. [Google Scholar] [CrossRef]
- Zheng, Y.; Wu, L.; Fang, Y.; Li, T.; Zheng, W. Comparative Analysis of Doubly Fed Flux-Reversal Permanent Magnet Machines with Different PM Arrangements and Consequent-Pole Topologies. IEEE Trans. Magn. 2021, 57, 1–6. [Google Scholar] [CrossRef]
- Li, D.; Gao, Y.; Qu, R.; Li, J.; Huo, Y.; Ding, H. Design and Analysis of a Flux Reversal Machine with Evenly Distributed Permanent Magnets. IEEE Trans. Ind. Appl. 2018, 54, 172–183. [Google Scholar] [CrossRef]
- Li, H.-Y.; Zhu, Z.Q. Optimal Number of Magnet Pieces of Flux Reversal Permanent Magnet Machines. IEEE Trans. Energy Convers. 2019, 34, 889–898. [Google Scholar] [CrossRef]
- Li, H.; Zhu, Z.Q. Influence of Adjacent Teeth Magnet Polarities on the Performance of Flux Reversal Permanent Magnet Machine. IEEE Trans. Ind. Appl. 2019, 55, 354–365. [Google Scholar] [CrossRef]
- Xie, K.; Li, D.; Qu, R.; Yu, Z.; Gao, Y.; Pan, Y. Analysis of a Flux Reversal Machine with Quasi-Halbach Magnets in Stator Slot Opening. IEEE Trans. Ind. Appl. 2019, 55, 1250–1260. [Google Scholar] [CrossRef]
- Zheng, Y.; Wu, L.; Zhu, J.; Fang, Y.; Qiu, L. Analysis of Dual-Armature Flux Reversal Permanent Magnet Machines with Halbach Array Magnets. IEEE Trans. Energy Convers. 2021, 36, 3044–3052. [Google Scholar] [CrossRef]
- Qu, H.; Zhu, Z.Q. Analysis of Spoke Array Permanent Magnet Flux Reversal Machines. IEEE Trans. Energy Convers. 2020, 35, 1688–1696. [Google Scholar] [CrossRef]
- Qu, H.; Zhu, Z.Q.; Shuang, B. Influences of PM Number and Shape of Spoke Array PM Flux Reversal Machines. IEEE Trans. Energy Convers. 2021, 36, 1131–1142. [Google Scholar] [CrossRef]
- Wei, F.; Zhu, Z.Q.; Qu, H.; Yan, L.; Qi, J. New Dual-PM Spoke-Type Flux-Reversal Machines for Direct-Drive Applications. IEEE Trans. Ind. Appl. 2022, 58, 6190–6202. [Google Scholar] [CrossRef]
- Cai, S.; Chen, H.; Yuan, X.; Wang, Y.-C.; Shen, J.-X.; Lee, C.H.T. Analysis of Synergistic Stator Permanent Magnet Machine with the Synergies of Flux-Switching and Flux-Reversal Effects. IEEE Trans. Ind. Electron. 2022, 69, 12237–12248. [Google Scholar] [CrossRef]
- Gao, Y.; Qu, R.; Li, D.; Li, J.; Zhou, G. Consequent-Pole Flux-Reversal Permanent-Magnet Machine for Electric Vehicle Propulsion. IEEE Trans. Appl. Supercond. 2016, 26, 1–5. [Google Scholar] [CrossRef]
- Li, H.Y.; Zhu, Z.Q. Analysis of Flux-Reversal Permanent-Magnet Machines with Different Consequent-Pole PM Topologies. IEEE Trans. Magn. 2018, 54, 8105305. [Google Scholar] [CrossRef]
- Qu, H.; Zhu, Z.Q.; Li, H. Analysis of Novel Consequent Pole Flux Reversal Permanent Magnet Machines. IEEE Trans. Ind. Appl. 2021, 57, 382–396. [Google Scholar] [CrossRef]
- Yang, K.; Zhao, F.; Wang, Y. Analysis of Double-Layer Permanent-Magnet Flux Reversal Machines with Different Permanent Magnet Arrangements in Stator. IEEE Trans. Magn. 2021, 57, 1–5. [Google Scholar] [CrossRef]
- Yang, K.; Zhao, F.; Wang, Y.; Bao, Z. Consequent-Pole Flux Reversal Permanent Magnet Machine with Halbach Array Magnets in Rotor Slot. IEEE Trans. Magn. 2021, 57, 1–5. [Google Scholar] [CrossRef]
- Yang, H.; Zhu, Z.Q.; Lin, H.; Li, H.; Lyu, S. Analysis of Consequent-Pole Flux Reversal Permanent Magnet Machine with Biased Flux Modulation Theory. IEEE Trans. Ind. Electron. 2020, 67, 2107–2121. [Google Scholar] [CrossRef]
- Hua, H.; Zhu, Z.Q. Investigation on Symmetrical Characteristics of Consequent-Pole Flux Reversal Permanent Magnet Machines with Concentrated Windings. IEEE Trans. Energy Convers. 2022, 37, 1815–1824. [Google Scholar] [CrossRef]
- Jing, L.; Tang, W.; Wang, T.; Ben, T.; Qu, R. Performance Analysis of Magnetically Geared Permanent Magnet Brushless Motor for Hybrid Electric Vehicles. IEEE Trans. Transp. Electr. 2022, 8, 2874–2883. [Google Scholar] [CrossRef]




| Parameters | Value |
|---|---|
| Axial length | 60 mm |
| Outer radius of machine | 60 mm |
| Supplied current | 7 A |
| Voltage | 36 V |
| Speed | 1000 rpm |
| PMs material | NdFeB |
| Steel material | DW315-50 |
| Conventional FRM | Proposed FRM | ||||||
|---|---|---|---|---|---|---|---|
| Parameters | Unit | Initial Value | Range | Final Value | Initial Value | Range | Final Value |
| Inner radius of rotor yoke R1 | mm | 22 | 20–25 | 22.02 | 22 | 20–25 | 22.57 |
| Outer radius of rotor yoke R2 | mm | 32 | 30–35 | 32.32 | 32 | 30–35 | 32.58 |
| Outer radius of rotor teeth R3 | mm | 40 | 37–42 | 39.39 | 40 | 37–42 | 39.16 |
| Width of rotor teeth Hr | mm | 4.5 | 3–6 | 4.2 | 4.5 | 3–6 | 5.29 |
| Air gap length Ha | mm | 0.5 | 0.4–0.6 | 0.47 | 0.5 | 0.4–0.6 | 0.51 |
| Thickness of PM Hp | mm | 2.5 | 2–3 | 2.06 | 2.5 | 2–3 | 2.21 |
| The radian of stator teeth θ | deg. | 23 | 20–25 | 23.42 | 35 | 30–40 | 34.39 |
| Thickness of stator yoke Ht | mm | 1.5 | 1–3 | 1.34 | 1.5 | 1–3 | 1.98 |
| Length of stator teeth Hs | mm | 8.5 | 7–10 | 8.2 | 8.5 | 7–10 | 8.98 |
| Width of stator teeth L | mm | 5.5 | 4–7 | 5.86 | 8 | 7–9 | 8.04 |
| Width of auxiliary teeth La | mm | 5 | 3–7 | 4.9 | |||
| Inner radius of stator yoke R4 | mm | 56 | 55–57 | 56.08 | 56 | 55–57 | 56.17 |
| Unit | Conventional | Proposed | |
|---|---|---|---|
| Phase flux linkage | Wb | 0.0114 | 0.0148 |
| Synchronous inductance | mH | 1.0064 | 1.3256 |
| Phase winding voltage drop | V | 0.1143 | 0.1152 |
| Power factor | 0.7507 | 0.7439 |
| Copper Loss | Core Loss | Eddy Current Loss | Additional Losses | Total Loss | Output Power | Efficiency | |
|---|---|---|---|---|---|---|---|
| Conventional | 2.424 W | 13.665 W | 9.389 W | 6.01 W | 31.5 W | 300.5 W | 90.51% |
| Proposed | 2.443 W | 18.105 W | 6.035 W | 7.52 W | 34.1 W | 376 W | 91.68% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jing, L.; Yang, K.; Gao, Y.; Kui, Z.; Min, Z. Analysis and Optimization of a Novel Flux Reversal Machine with Auxiliary Teeth. Energies 2022, 15, 8906. https://doi.org/10.3390/en15238906
Jing L, Yang K, Gao Y, Kui Z, Min Z. Analysis and Optimization of a Novel Flux Reversal Machine with Auxiliary Teeth. Energies. 2022; 15(23):8906. https://doi.org/10.3390/en15238906
Chicago/Turabian StyleJing, Libing, Kun Yang, Yuting Gao, Zhangtao Kui, and Zeyu Min. 2022. "Analysis and Optimization of a Novel Flux Reversal Machine with Auxiliary Teeth" Energies 15, no. 23: 8906. https://doi.org/10.3390/en15238906
APA StyleJing, L., Yang, K., Gao, Y., Kui, Z., & Min, Z. (2022). Analysis and Optimization of a Novel Flux Reversal Machine with Auxiliary Teeth. Energies, 15(23), 8906. https://doi.org/10.3390/en15238906







