Effect of Stagger Angle of Rotor Channels on the Wave Rotor
Abstract
:1. Introduction
2. Methodology
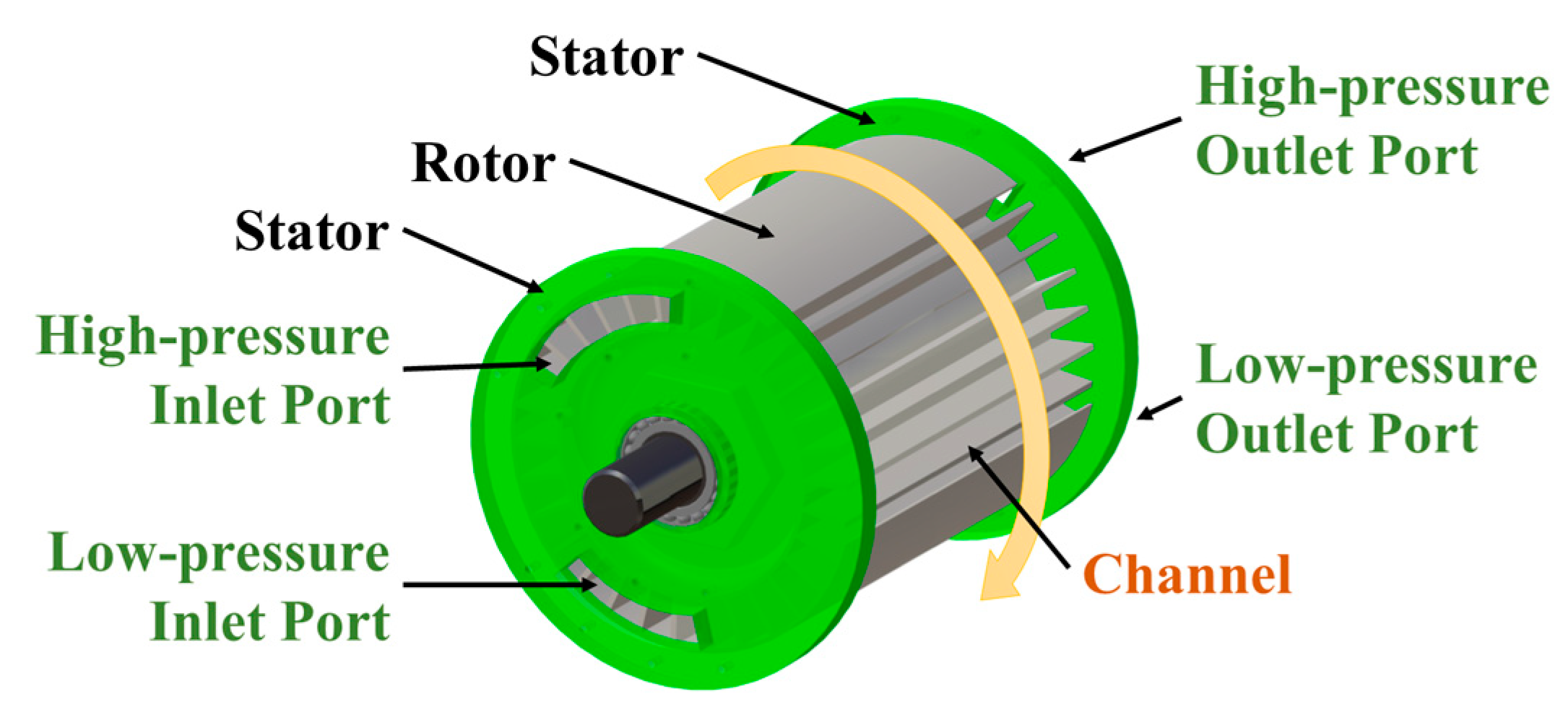
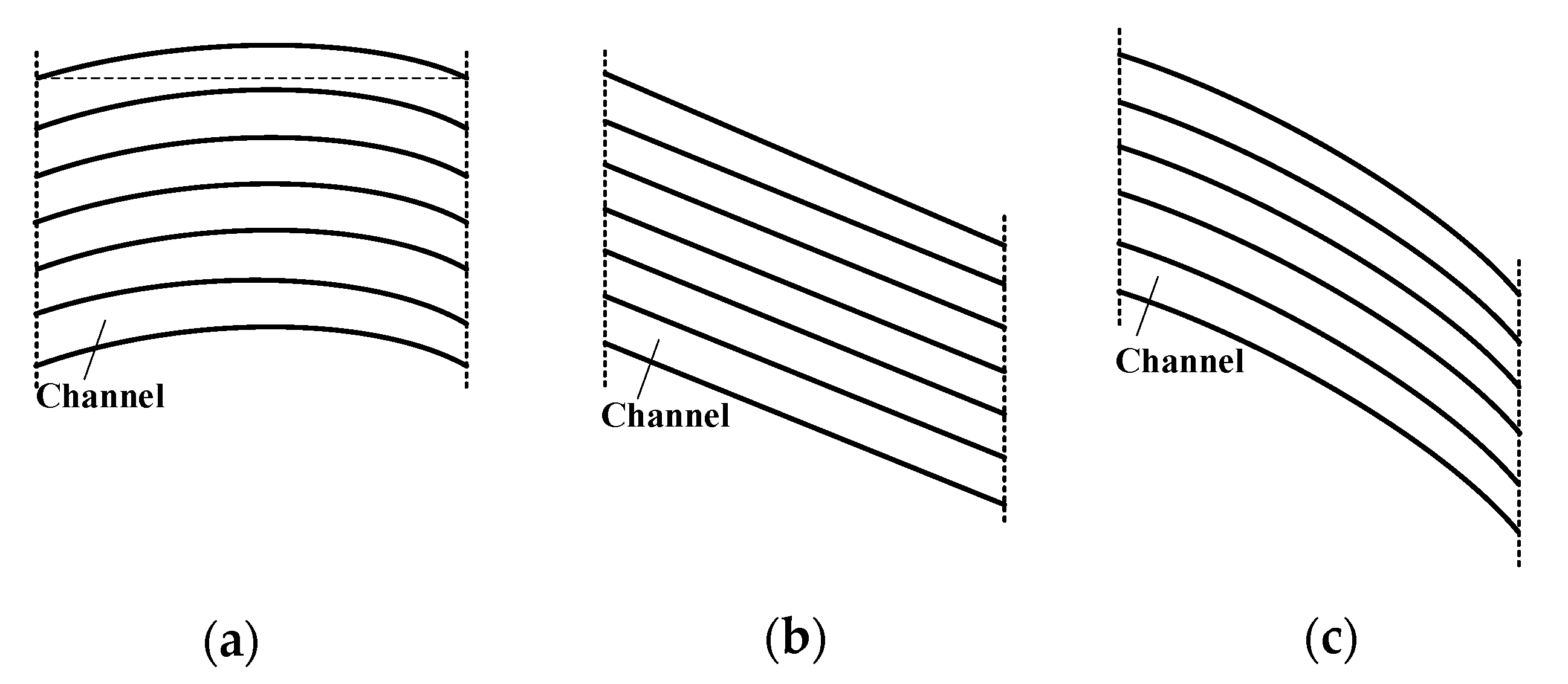
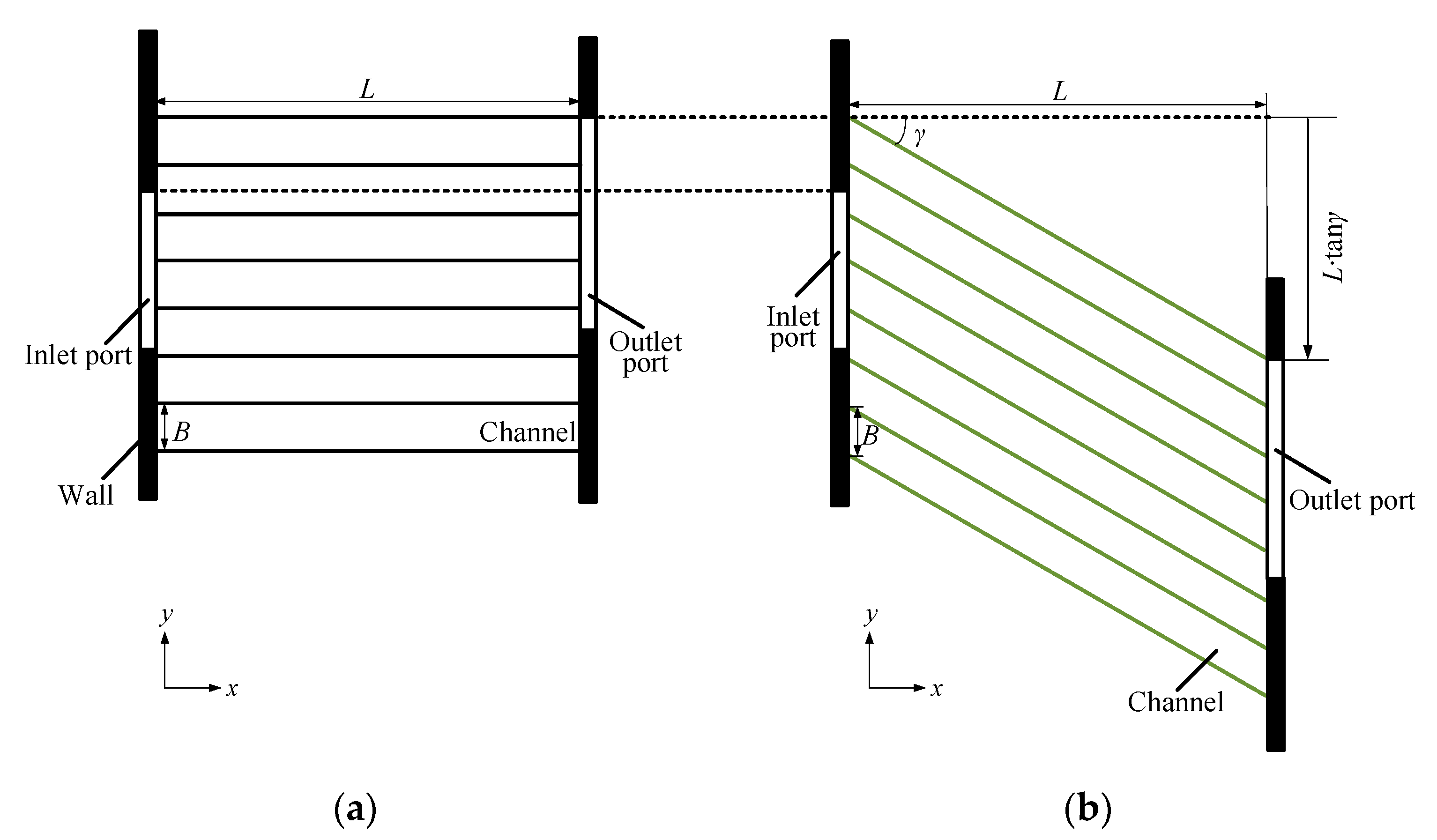
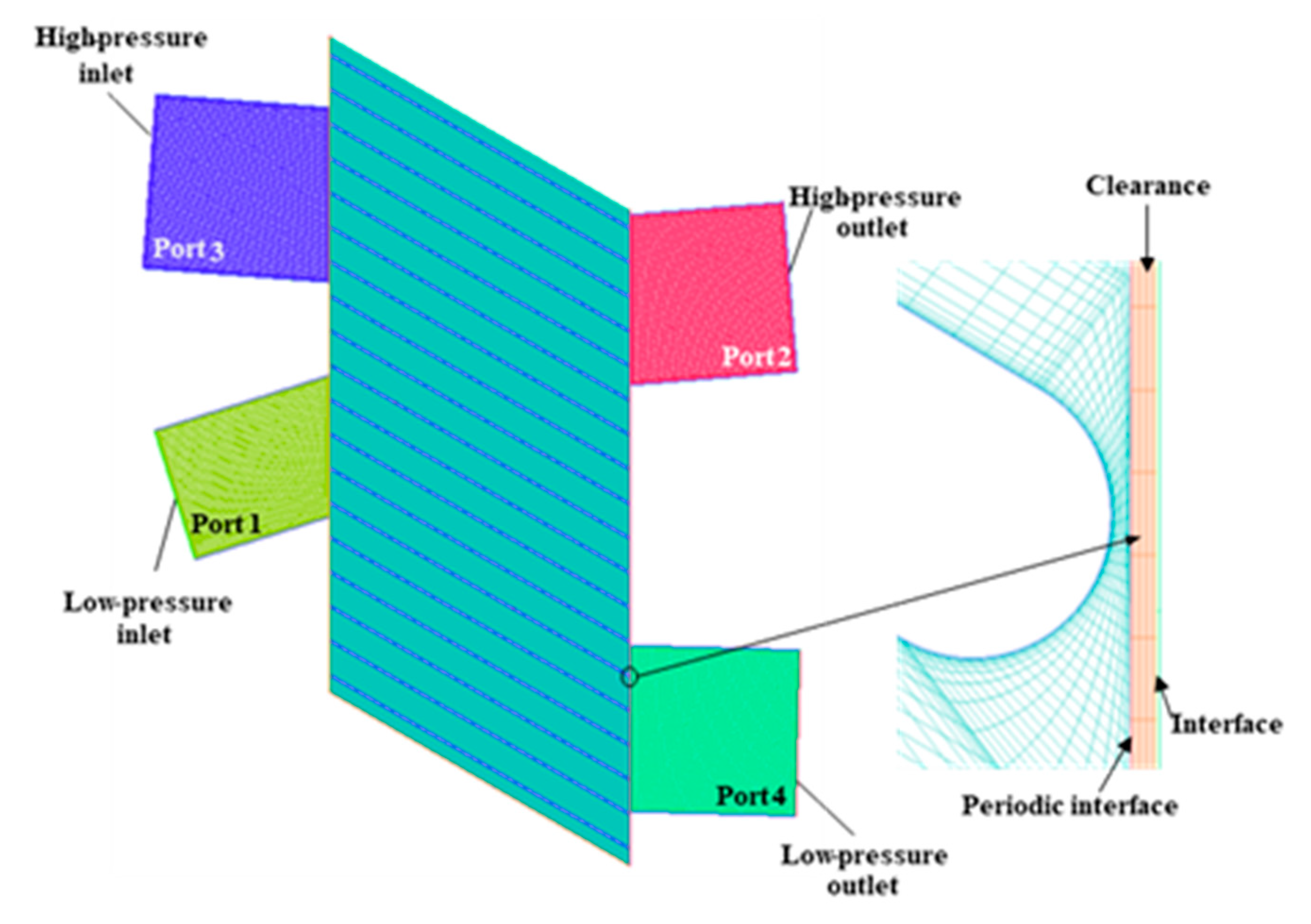
2.1. Geometric Modification
2.2. Numerical Model
2.2.1. Numerical Method
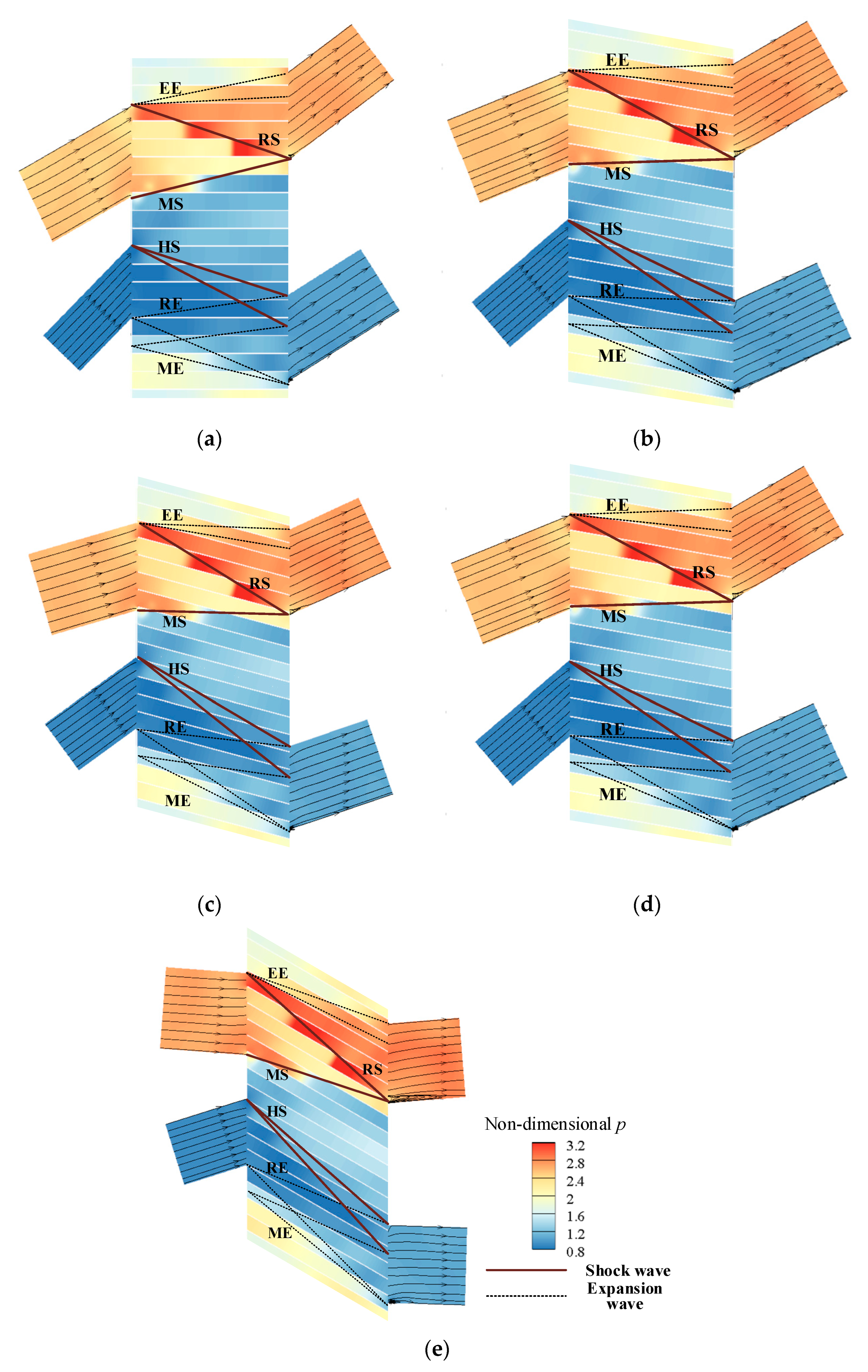
2.2.2. Model Validation
3. Results Analysis
3.1. The Effects of Stagger Angle on Pressure Ratios
3.2. The Effects of Stagger Angle on the Direction of Flow at Each Port
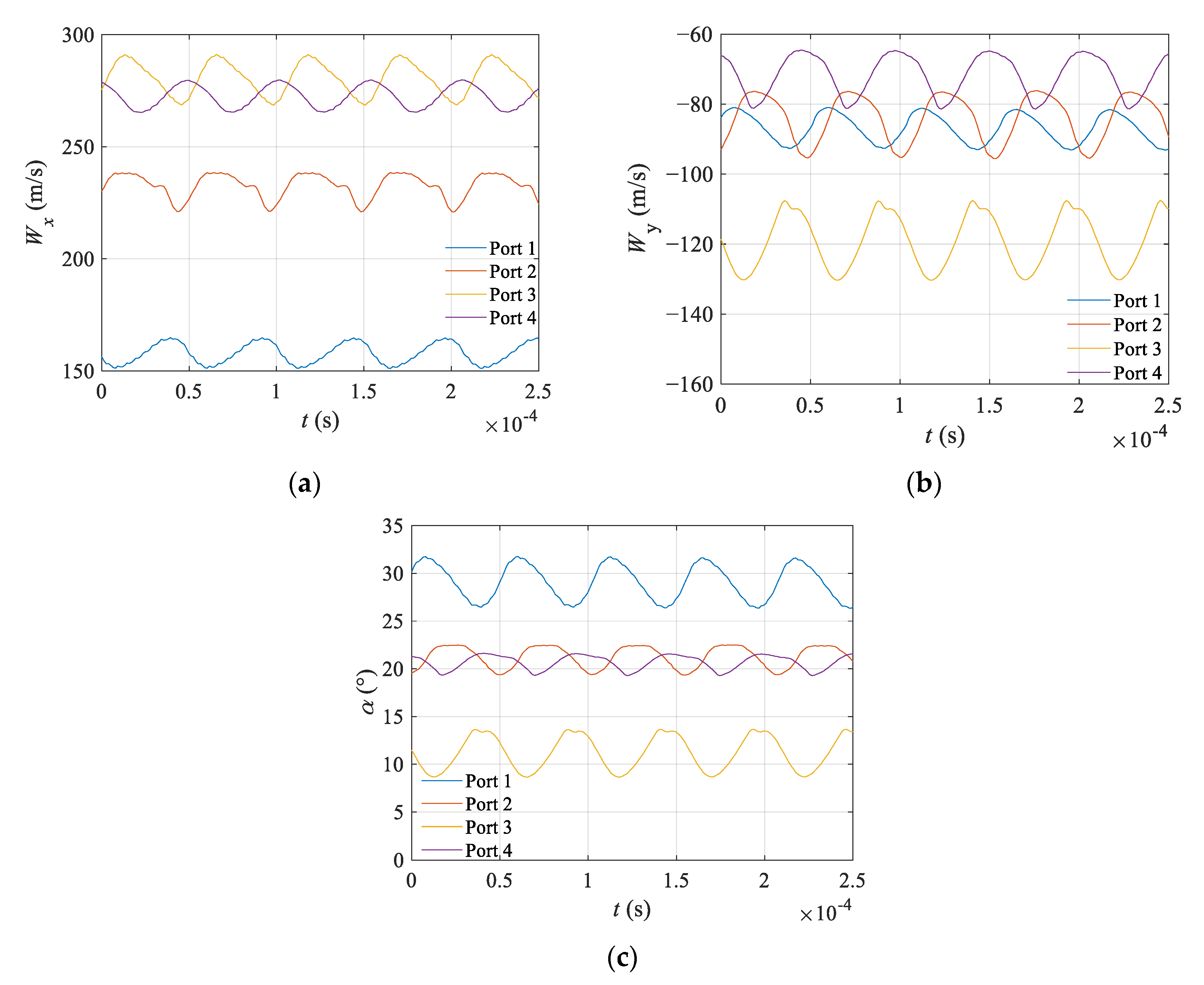
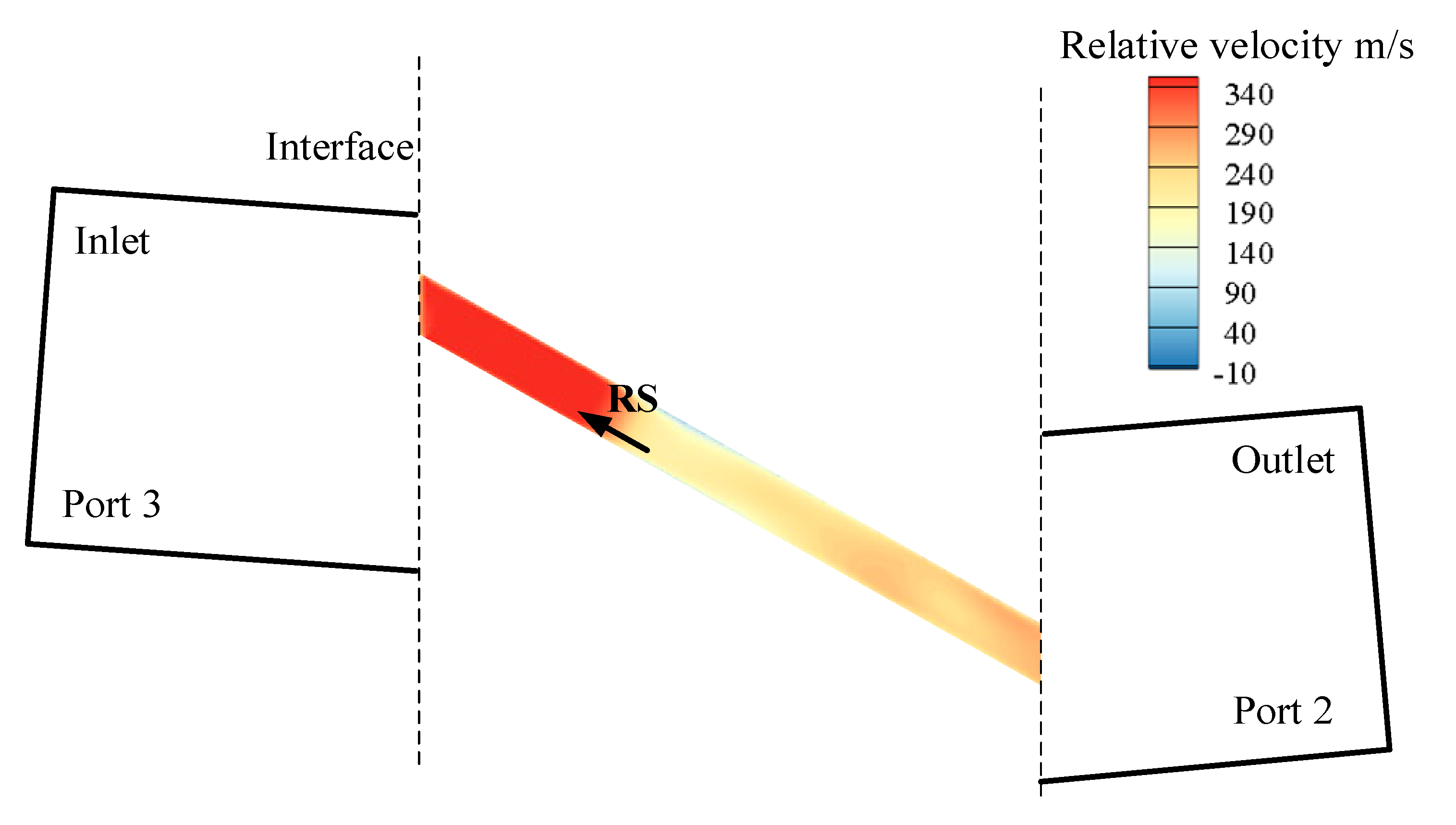
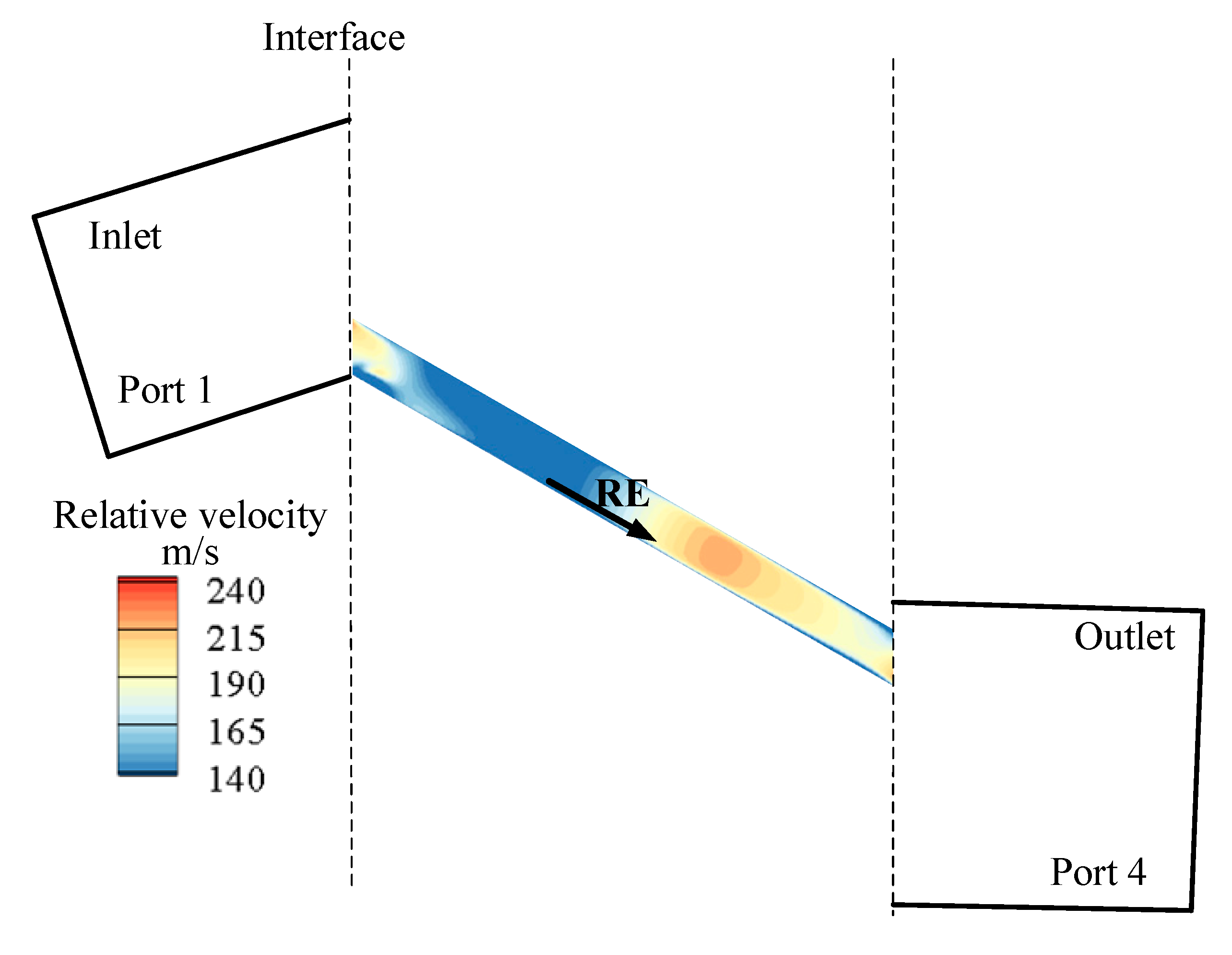
3.2.1. Unsteadiness of the Velocity
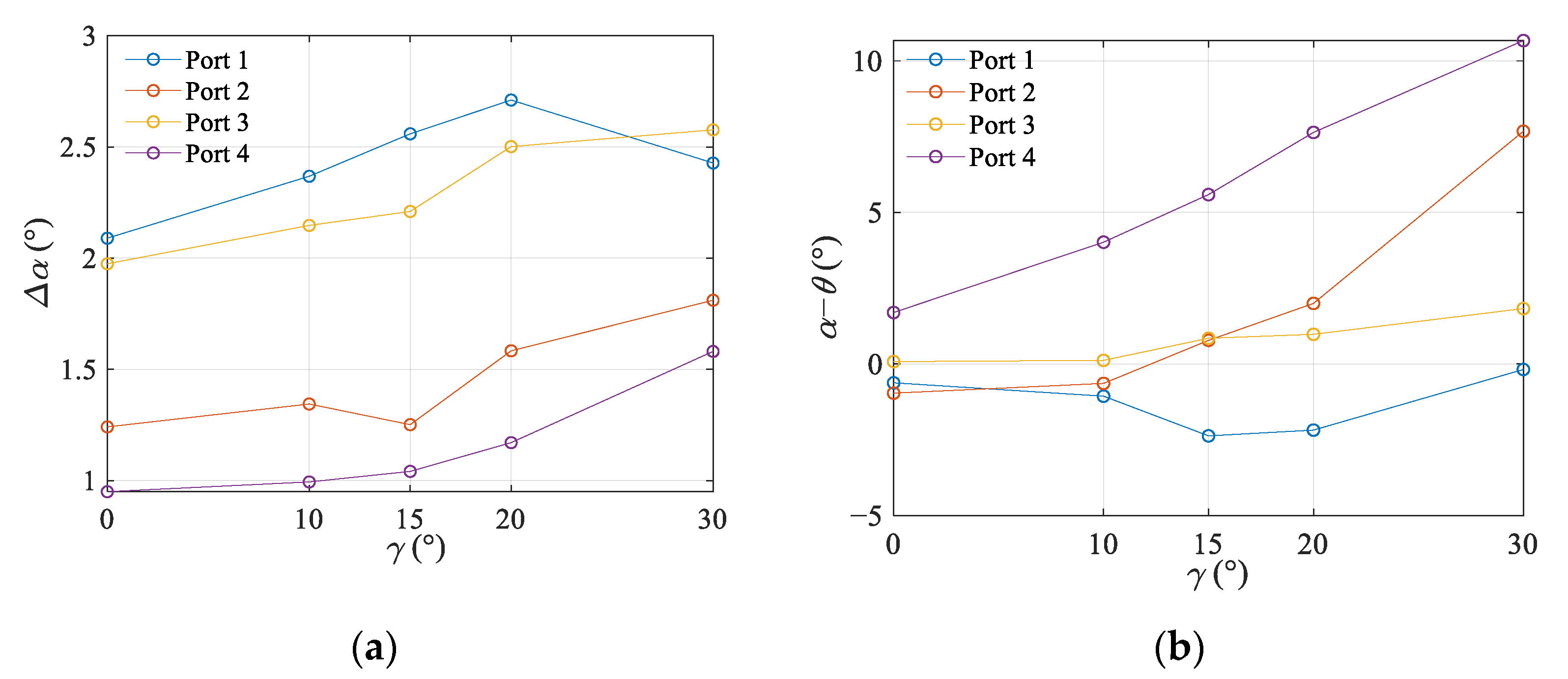
3.2.2. The Effect of Stagger Angle on Absolute Velocity Angle at Each Port
3.3. The Effect of Stagger Angle on Shaft Power
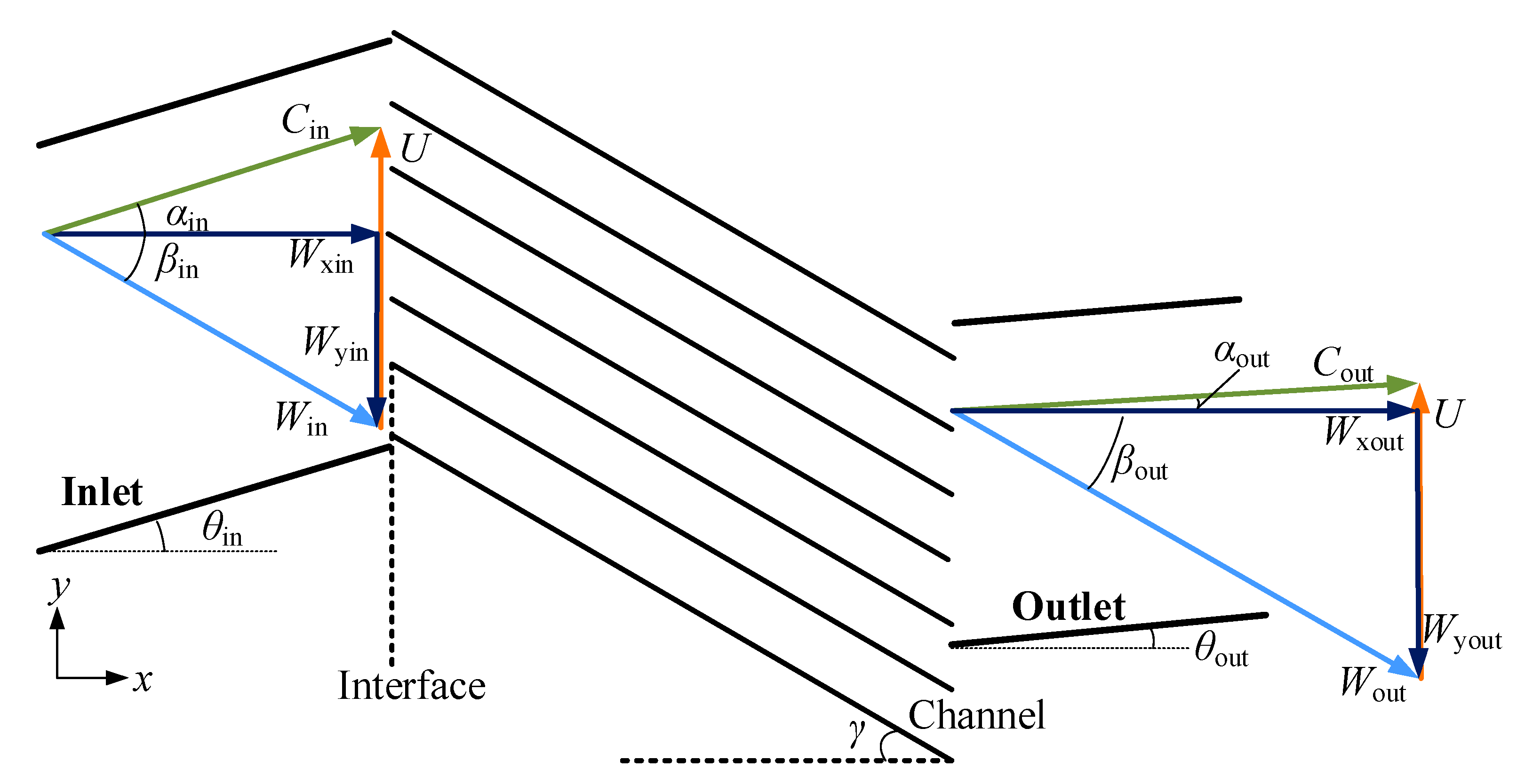
3.3.1. Mechanism of Velocity Triangles with Unsteady Pressure Waves
- (1)
- Velocity triangles of the high-pressure inlet and outlet ports
- (2)
- Velocity triangles of the low-pressure inlet and outlet ports
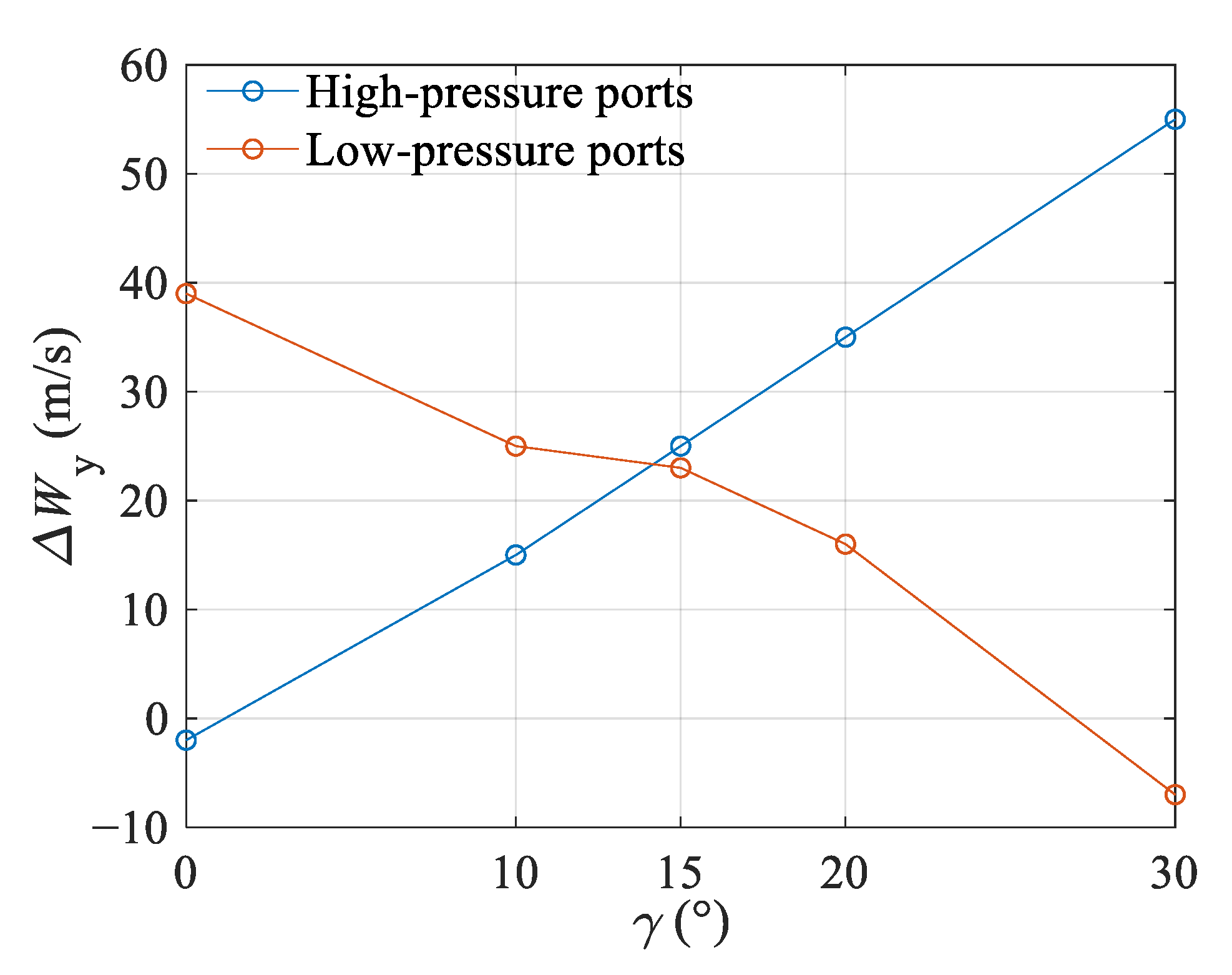
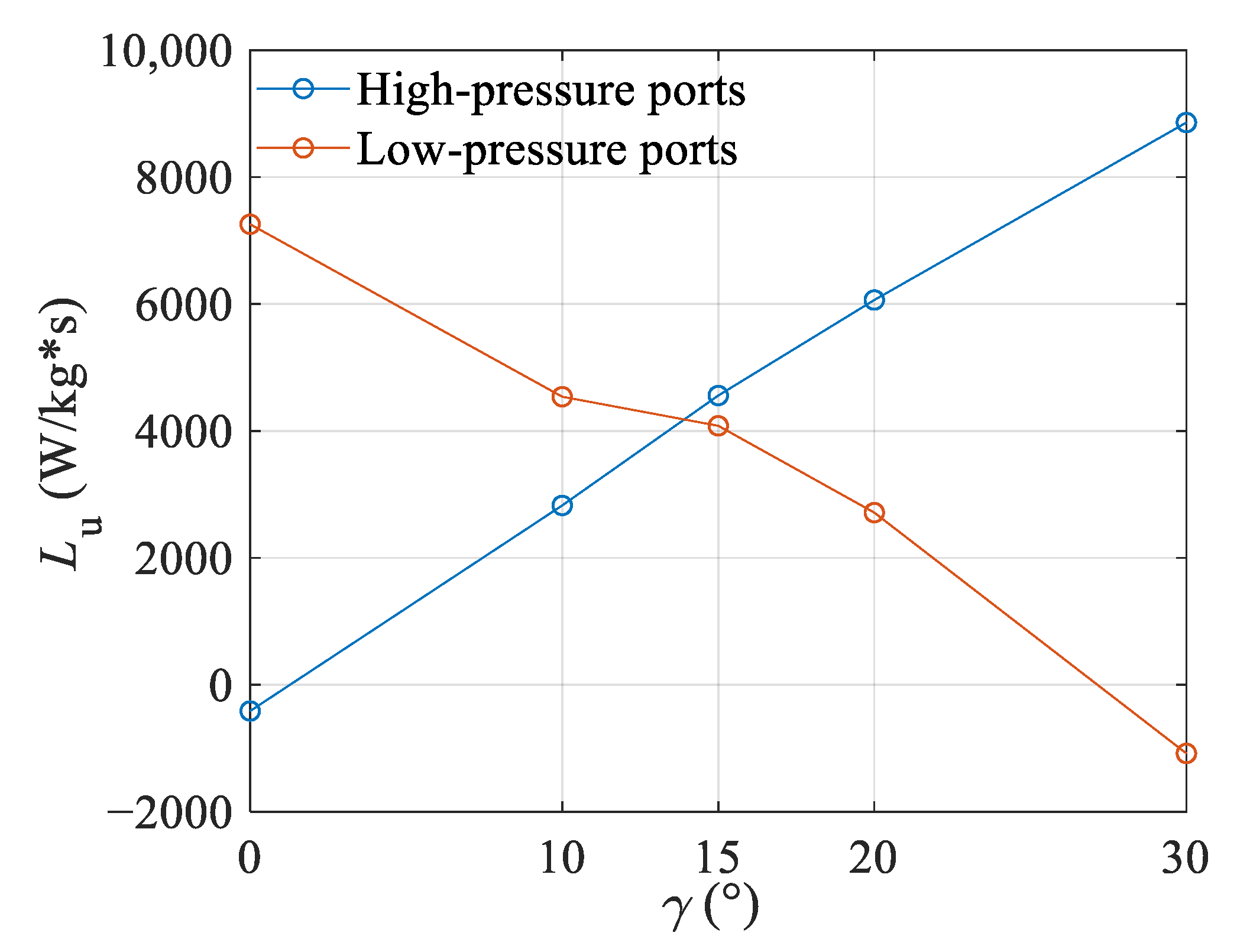
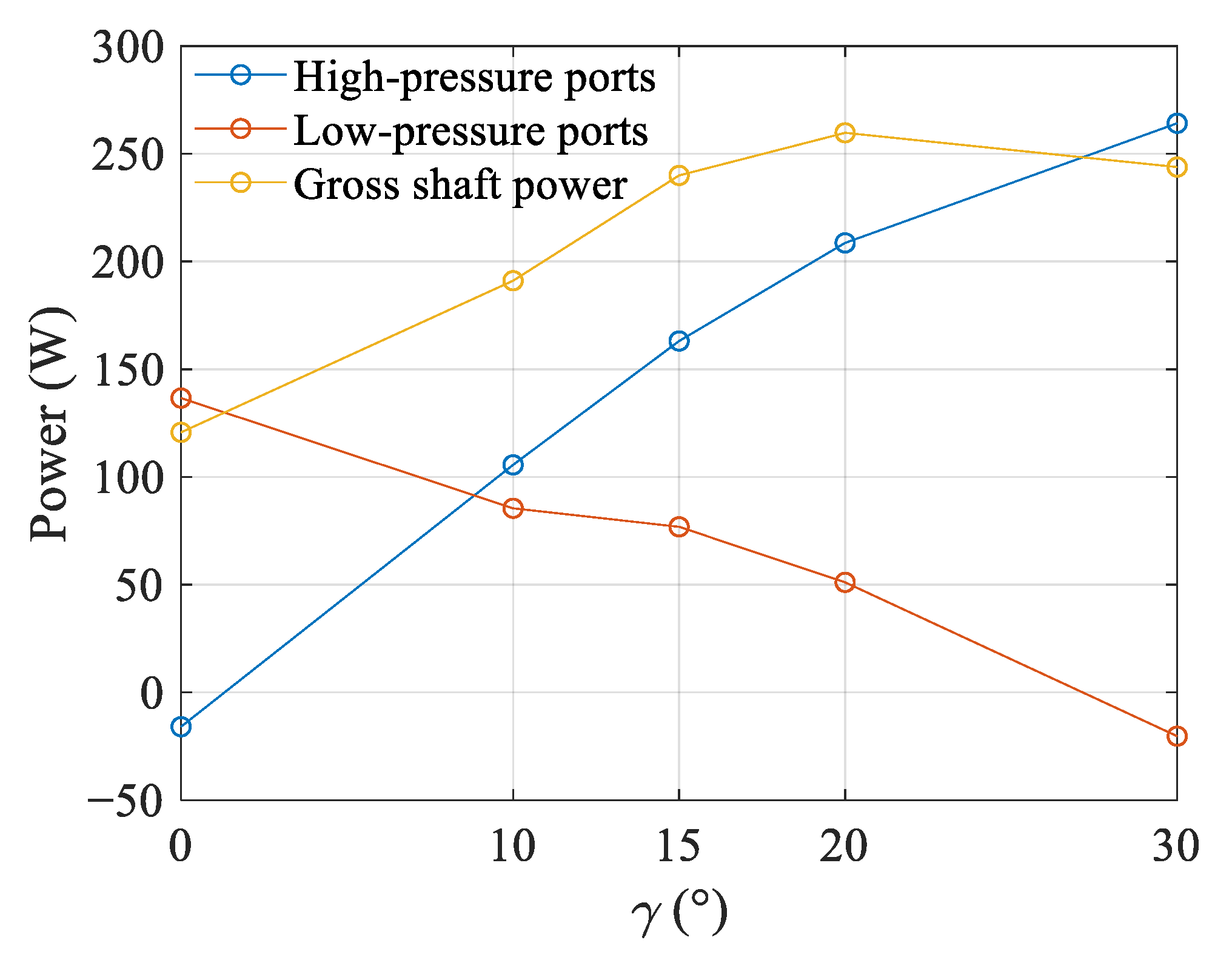
3.3.2. Effect of Stagger Angle on Shaft Power
- (1)
- Rim work estimation based on velocity triangles
- (2)
- Shaft power estimation
4. Conclusions
- (1)
- Geometric modifications on outlet port coordinates, rim velocity, and port inclination angles were made for different stagger angles based on the velocity triangle model. With such modifications, the unsteady pressure wave system in the wave rotor was kept similar to the baseline case (γ = 0°), and the inlet and outlet flow direction fits the inclination of ports with acceptable deviation. It verified such geometric modifications.
- (2)
- As the stagger angle increased, the pressure ratios of compression and expansion of the wave rotor was basically kept constant for different stagger angles. It means that the wave rotor would keep stable functions of compression and expansion in the optimization on the stagger angle.
- (3)
- As the stagger angle increased, the inlet and outlet flow of the wave rotor turned towards the axial direction as the adjacent compressor, combustor, or turbine would require. It was beneficial to compact and light-weight integration of the wave rotor to a gas turbine.
- (4)
- As the stagger angle increased, the rotor consumed relatively more shaft power in this work. It was a compromise of staggered channels of wave rotors, but the shaft power amount was low and acceptable at any stagger angle.
- (5)
- A recommendation of stagger angle optimization is the stagger angle of 30°. It produces nearly axial inlet and outlet flows with an acceptable compromise of shaft power consumption. A geometry of cambered and staggered channels would compensate for the shaft power consumption in future work.
- (6)
- A critical mechanism in this work was the rim work mechanism of straight and staggered channels of the wave rotor. The unsteady pressure wave between an inlet port and the opposite outlet port induced variation in flow velocity. A staggered channel makes the tangential component of the velocity variation produce some rim work. Such a mechanism was like the typical mechanism of rim work in a supersonic compressor stage.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature and Abbreviations
| Nomenclature | Greek Letters | ||
| C | absolute velocity (unit: m/s) | α | absolute velocity angle (unit: degree) |
| CFD | Computational fluid dynamics | β | relative velocity angle (unit: degree) |
| Lu | Rim work (unit: W/kg·s) | γ | stagger angle (unit: degree) |
| m | mass flow rate (unit: kg/s) | θ | inclination angel (unit: degree) |
| p | pressure (unit: Pa) | Δ | variation |
| P | shaft power (unit: W) | τ | channel passing period |
| t | time (unit: s) | Π | compression pressure ratio |
| U | implicated velocity (unit: m/s) | ε | expansion pressure ratio |
| W | relative velocity (unit: m/s) | ||
| x | axial coordinate (unit: m) | ||
| y | tangential coordinate (unit: m) | ||
| Subscripts | |||
| 1 | Port 1 | ||
| 2 | Port 2 | ||
| 3 | Port 3 | ||
| 4 | Port 4 | ||
| in | inlet | ||
| out | outlet | ||
| H | High-pressure ports | ||
| L | Low-pressure ports | ||
References
- Akbari, P.; Nalim, R.; Mueller, N. A Review of Wave Rotor Technology and Its Applications. J. Eng. Gas Turbines Power 2006, 128, 717–735. [Google Scholar] [CrossRef] [Green Version]
- Pring, P.; Onder, C.H.; Guzzella, L. EGR Control of Pressure-wave Supercharged IC Engines. Control Eng. Pract. 2007, 15, 1520–1532. [Google Scholar] [CrossRef]
- Kharazi, A.A.; Akbari, P.; Mueller, N. Preliminary Study of a Novel R718 Compression Refrigeration Cycle Using a Three-port Condensing Wave Rotor. J. Eng. Gas Turbines Power 2005, 127, 539–544. [Google Scholar] [CrossRef]
- Hu, D.; Yu, Y.; Liu, P. Enhancement of Refrigeration Performance by Energy Transfer of Shock Wave. Appl. Therm. Eng. 2018, 130, 309–318. [Google Scholar] [CrossRef]
- Akbari, P.; Nalim, R.; Mueller, N. Performance Enhancement of Microturbine Engines Topped with Wave Rotors. J. Eng. Gas Turbines Power 2006, 128, 190–202. [Google Scholar] [CrossRef] [Green Version]
- Berchtold, M.; Mayer, A. Supercharging with Comprex: Application and Experience. In Proceedings of the ONR/NAVAIR Wave Rotor Research and Technology Workshop, Naval Postgraduate School, Monterey, CA, USA, 1 January–31 March 1985. [Google Scholar]
- Zsiga, N.; Skopil, M.A.; Wang, M.; Klein, D.; Soltic, P. Comparison of Turbocharging and Pressure Wave Supercharging of a Natural Gas Engine for Light Commercial Trucks and Vans. Energies 2021, 14, 5306. [Google Scholar] [CrossRef]
- Pearson, R.D. A Gas Wave-Turbine Engine Which Developed 35 HP and Performed Over a 6: 1 Speed Range. In Proceedings of the ONR/NAVAIR Wave Rotor Research and Technology Workshop, Naval Postgraduate School, Monterey, CA, USA, 1 January–31 March 1985; pp. 125–170. [Google Scholar]
- Mathur, A. Design and Experimental Verification of Wave Rotor Cycles. In Proceedings of the ONR/NAVAIR Wave Rotor Research and Technology Workshop, Naval Postgraduate School, Monterey, CA, USA, 1 January–31 March 1985; pp. 215–228. [Google Scholar]
- Jagannath, R.R.; Bane, S.P.; Razi Nalim, M. Numerical Modeling of a Wave Turbine and Estimation of Shaft Work. J. Fluid Eng. 2018, 140, 101106. [Google Scholar] [CrossRef] [Green Version]
- Tüchler, S.; Copeland, C.D. Experimental Results from the Bath μ-wave Rotor Turbine Performance Tests. Energ. Convers. Manage. 2018, 189, 33–48. [Google Scholar] [CrossRef]
- Tüchler, S.; Copeland, C.D. Validation of a Numerical Quasi-One-Dimensional Model for Wave Rotor Turbines with Curved Channels. J. Eng. Gas Turbines Power 2020, 142, 021017-1–021017-15. [Google Scholar] [CrossRef]
- Tüchler, S.; Copeland, C.D. Experimental and Numerical Assessment of an Optimised, Non-axial Wave Rotor Turbine. Appl. Energy 2020, 268, 115013. [Google Scholar] [CrossRef]
- Tüchler, S.; Copeland, C.D. Numerical Optimisation of a Micro-wave Rotor Turbine Using a Quasi-two-dimensional CFD Model and a Hybrid Algorithm. Shock Waves 2021, 31, 271–300. [Google Scholar] [CrossRef]
- Liu, P.; Feng, M.; Liu, X.; Wang, H.; Hu, D. Performance Analysis of Wave Rotor Based on Response Surface Optimization Method. J. Energy Resour. Technol. 2022, 144, 061302. [Google Scholar] [CrossRef]
- Akbari, P.; Tait, C.J.; Brady, G.M.; Polanka, M.D.; Sell, B. Enhancement of the Radial Wave Engine. In Proceedings of the AIAA Propulsion and Energy 2019 Forum, Indianapolis, IN, USA, 19–22 August 2019. [Google Scholar] [CrossRef]
- Eidelman, S. Gradual Opening of Skewed Passages in Wave Rotors. J. Propul. Power 1986, 2, 379–381. [Google Scholar] [CrossRef]
- Welch, G.; Paxson, D. Wave turbine analysis tool development. In Proceedings of the 34th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Cleveland, OH, USA, 12–15 July 1998. [Google Scholar] [CrossRef]
- Chan, S.; Liu, H. Mass-based Design and Optimization of Wave Rotors for Gas Turbine Engine Enhancement. Shock Waves 2017, 27, 313–324. [Google Scholar] [CrossRef]
- Chan, S.; Liu, H.; Xing, F.; Song, H. Wave Rotor Design Method with Three Steps Including Experimental Validation. J. Eng. Gas Turbines Power 2018, 140, 111201–111213. [Google Scholar] [CrossRef]
- Hu, D.; Li, Y.; Liu, P.; Yu, Y.; Liu, F. Numerical Study on the Effect of Nozzle Incident Angle on the Overall Performance of Gas Wave Refrigerator. Int. J. Refrig. 2022, 138, 61–70. [Google Scholar] [CrossRef]
- Chan, S.; Liu, H.; Song, H.; Li, F.; Jiang, C.; Gao, Z. Investigation of Pressure Wave Behaviors in the Rotational Speed Effects on a Pressure-exchange Wave Rotor. Chin. J. Aeronaut. 2022, 35, 247–259. [Google Scholar] [CrossRef]



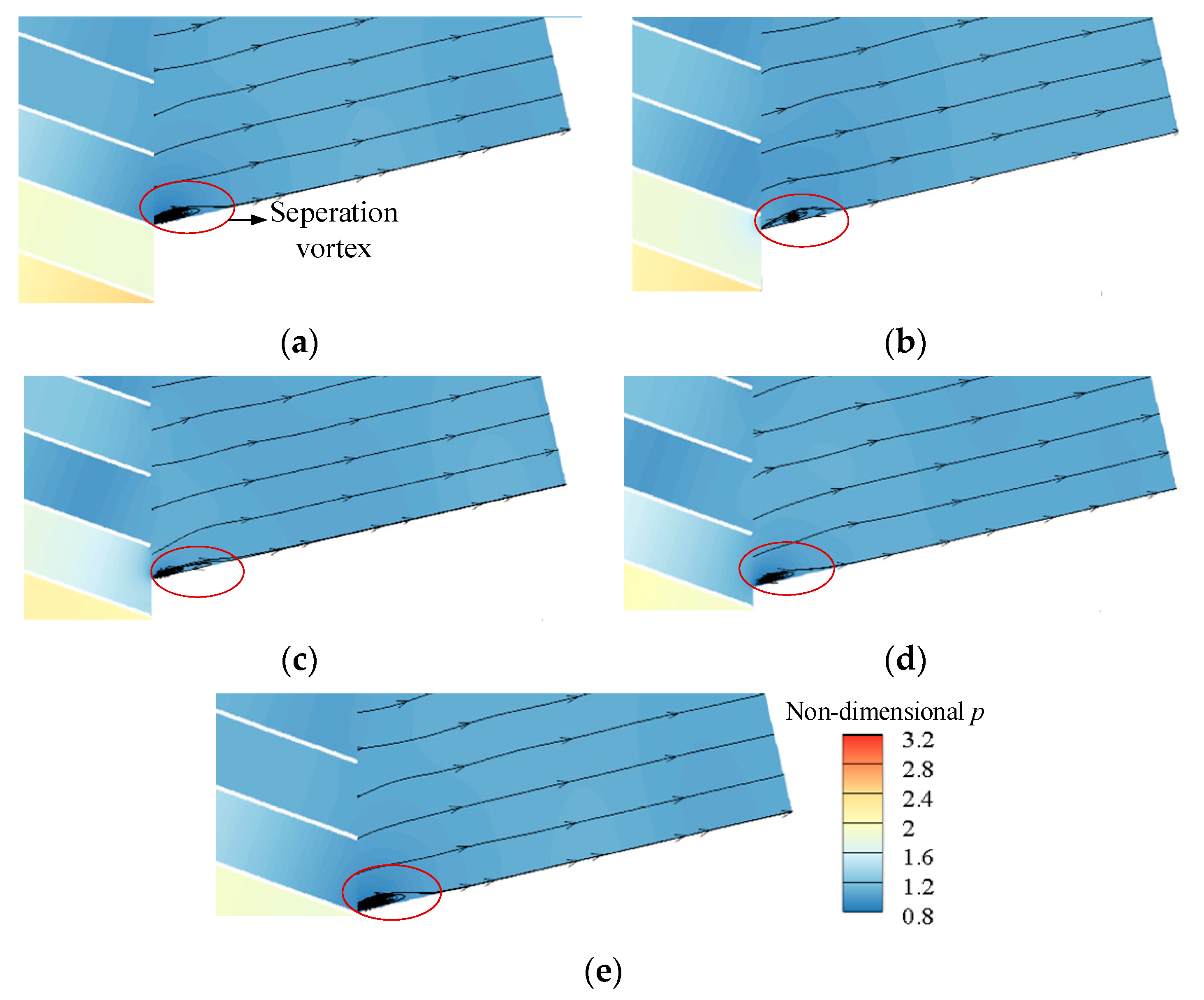



| γ = 0° | γ = 10° | γ = 15° | γ = 20° | γ = 30° | |
|---|---|---|---|---|---|
| Δy (m) | 0 | 0.014 | 0.021 | 0.029 | 0.046 |
| U (m/s) | 185.8 | 183.0 | 179.5 | 174.6 | 169.9 |
| θ1 | 45.9 | 40.3 | 36.1 | 31.2 | 17.6 |
| θ2 | 37.2 | 30.0 | 24.9 | 19.3 | 4.6 |
| θ3 | 30.1 | 22.0 | 16.3 | 10.2 | −4.3 |
| θ4 | 32.4 | 24.2 | 19.0 | 13.1 | −1.6 |
| Rotor | Port | Clearance | Total Number | |
|---|---|---|---|---|
| Grid resolution | 731,000 | 22,000 | 24,600 | 777,640 |
| γ = 0° | γ = 10° | γ = 15° | γ = 20° | γ = 30° | |
|---|---|---|---|---|---|
| Π | 3.018 | 3.023 | 3.017 | 3.015 | 3.017 |
| ε | 1.192 | 1.191 | 1.192 | 1.190 | 1.191 |
| γ = 0° | γ = 10° | γ = 15° | γ = 20° | γ = 30° | |
|---|---|---|---|---|---|
| Wx1 | 155.9 | 156.8 | 159.7 | 158.0 | 158.3 |
| Wx2 | 243.8 | 243.0 | 239.4 | 232.4 | 203.5 |
| Wx3 | 310.5 | 302.1 | 292.0 | 280.4 | 250.0 |
| Wx4 | 290.3 | 282.1 | 282.0 | 272.7 | 268.0 |
| Wy1 | −28.2 | −53.8 | −72.9 | −87.0 | −111.4 |
| Wy2 | −7.4 | −45.0 | −64.1 | −84.2 | −116.7 |
| Wy3 | −5.2 | −60.4 | −89.5 | −119.0 | −171.8 |
| Wy4 | 10.9 | −29.0 | −50.2 | −71.4 | −118.1 |
| α1 | 45.3 | 39.5 | 33.7 | 29.0 | 17.4 |
| α2 | 36.2 | 29.6 | 25.7 | 21.3 | 12.3 |
| α3 | 30.2 | 22.1 | 17.1 | 11.2 | −2.5 |
| α4 | 34.1 | 28.6 | 24.6 | 20.7 | 9.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chan, S.; Chen, Y.; Xing, F.; Liu, H. Effect of Stagger Angle of Rotor Channels on the Wave Rotor. Energies 2022, 15, 9455. https://doi.org/10.3390/en15249455
Chan S, Chen Y, Xing F, Liu H. Effect of Stagger Angle of Rotor Channels on the Wave Rotor. Energies. 2022; 15(24):9455. https://doi.org/10.3390/en15249455
Chicago/Turabian StyleChan, Shining, Yeyu Chen, Fei Xing, and Huoxing Liu. 2022. "Effect of Stagger Angle of Rotor Channels on the Wave Rotor" Energies 15, no. 24: 9455. https://doi.org/10.3390/en15249455
APA StyleChan, S., Chen, Y., Xing, F., & Liu, H. (2022). Effect of Stagger Angle of Rotor Channels on the Wave Rotor. Energies, 15(24), 9455. https://doi.org/10.3390/en15249455







