Study on the Viscosity Optimization of Polymer Solutions in a Heavy Oil Reservoir Based on Process Simulation
Abstract
:1. Introduction
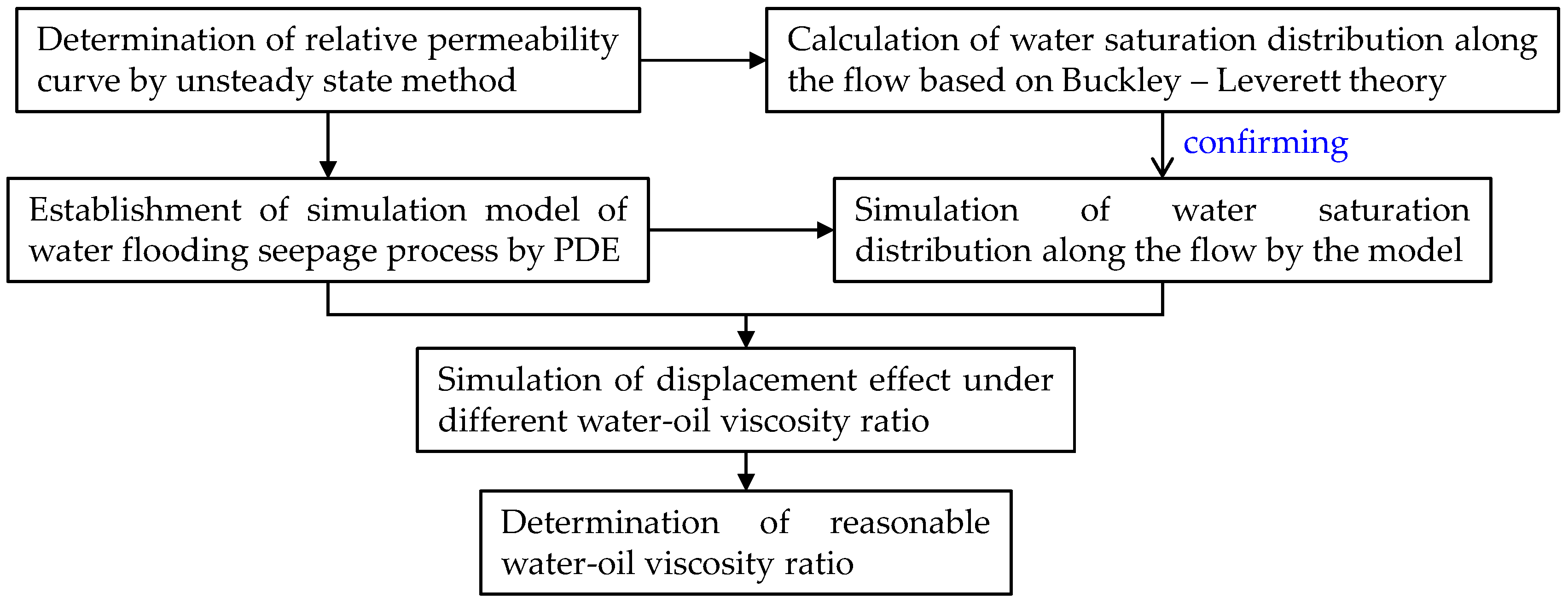
2. Experiments and Methodology
2.1. Measurement of Relative Permeability Curve
2.1.1. Materials
Experimental Core
Brine
Oil
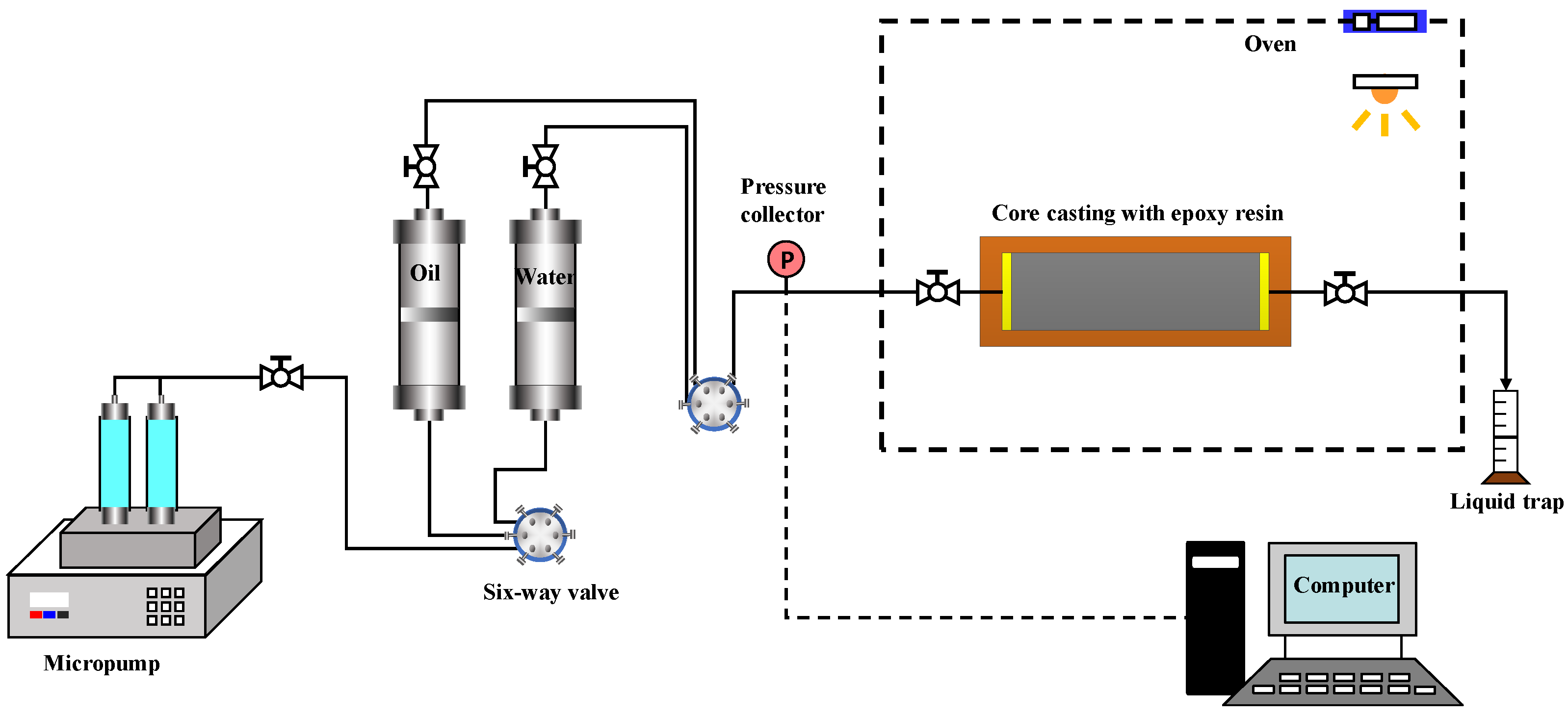
2.1.2. Experimental Procedures
- (1)
- Drain the saturated core with oil: the gradient flow rate method was used to flood the core with oil, the flow rates were 0.1 mL/min, 0.2 mL/min, 0.5 mL/min and 1.0 mL/min, successively. At each flow rate, the core was saturated with oil until the pressure was stable and no water was produced, then the water and oil production and displacement pressure data were collected [23].
- (2)
- Water flooding: Water displacement was carried out at a flow rate of 1.0 mL/min until the pressure was stable and no oil was produced. Water and oil production and displacement pressure data were collected. The experimental flowchart is presented in Figure 2.
- (3)
- Data processing: The JBN method was used to process the displacement data, then the relative permeability curve was standardized according to the Corey model.
2.2. Numerical Simulation
2.2.1. Model Building
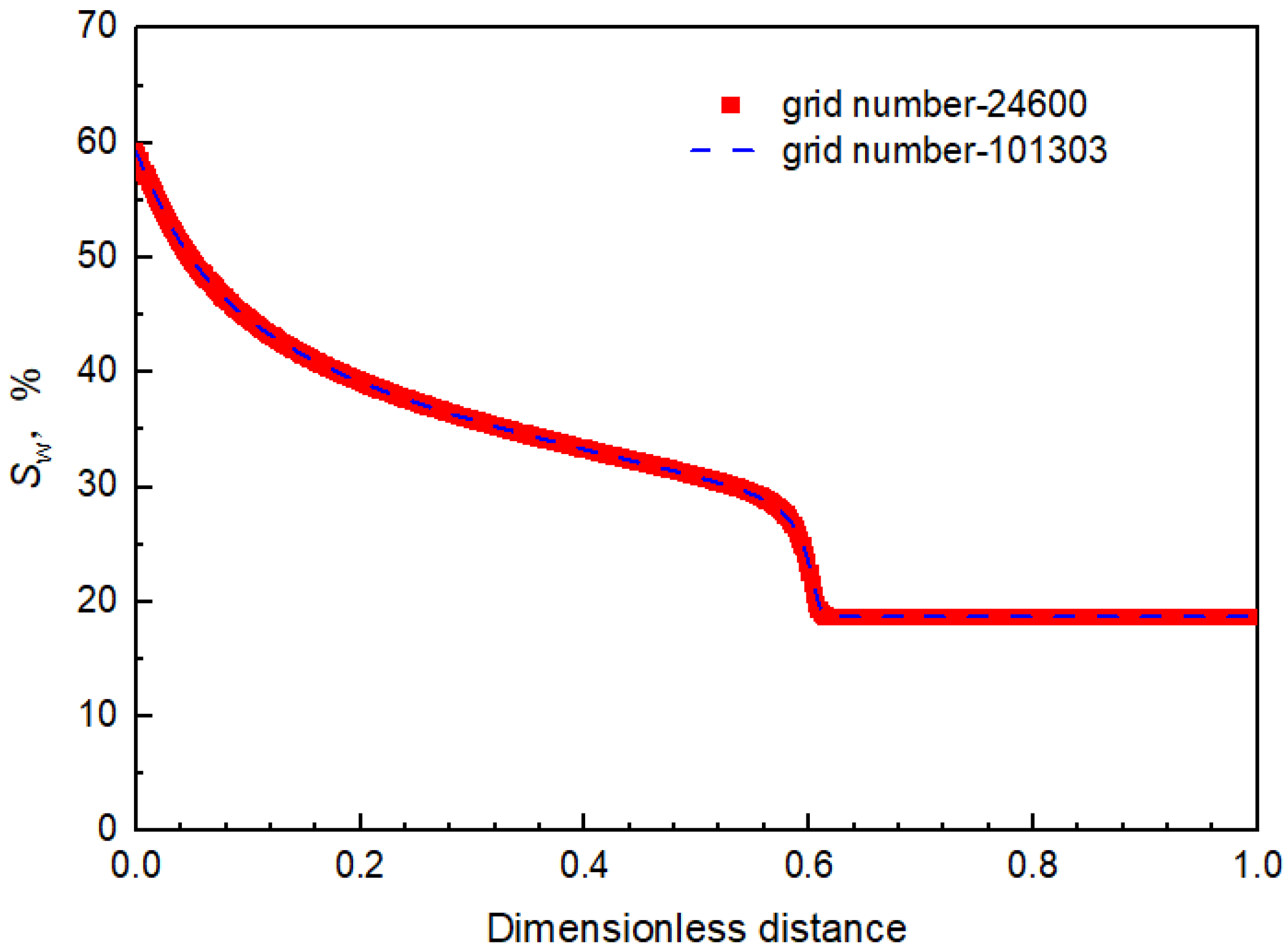
2.2.2. Model Validation
2.2.3. Simulation of the Effect of Viscosity on Displacement Process and Effect
3. Results and Discussions
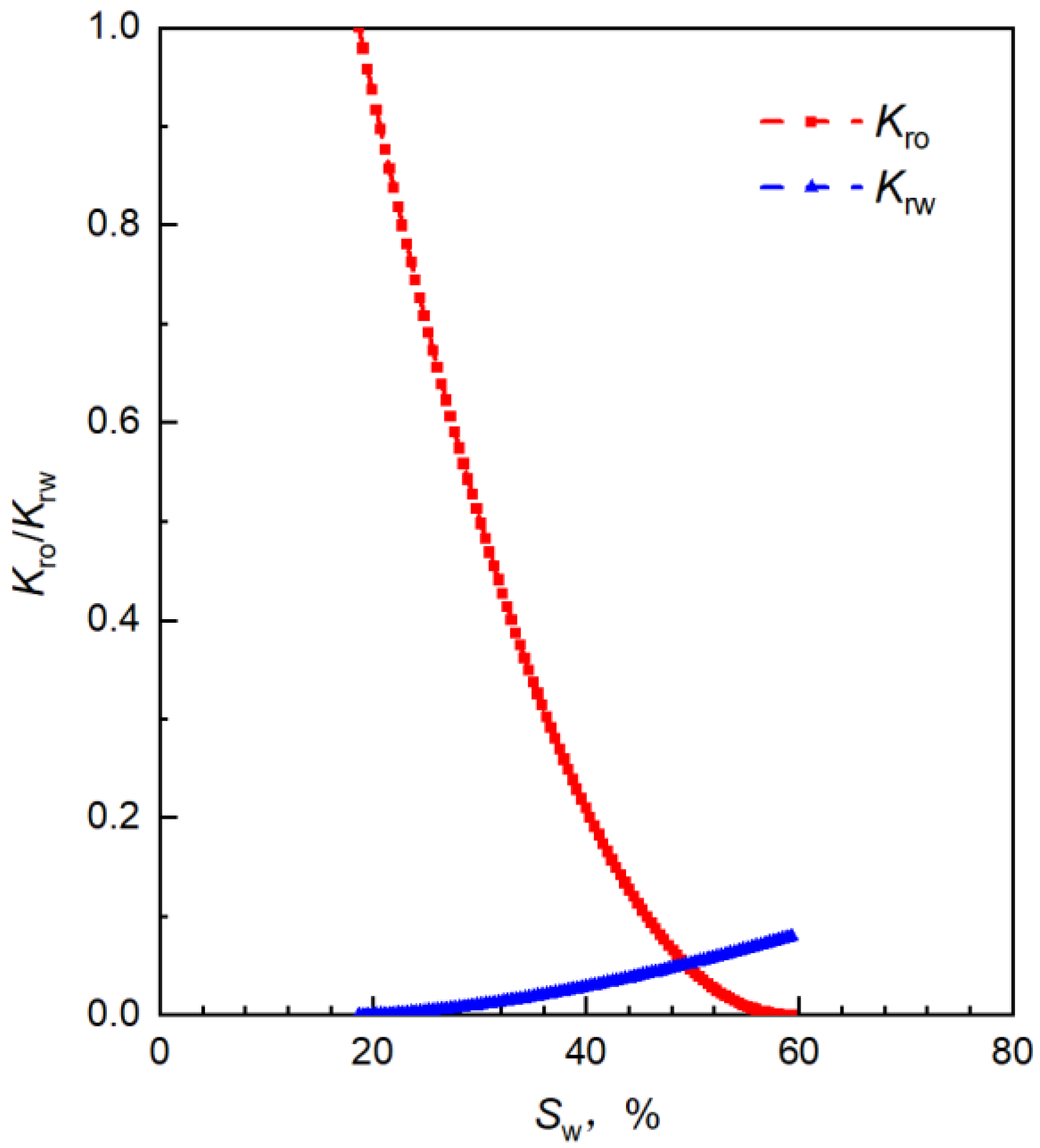
3.1. Oil-Water Relative Permeability Curve
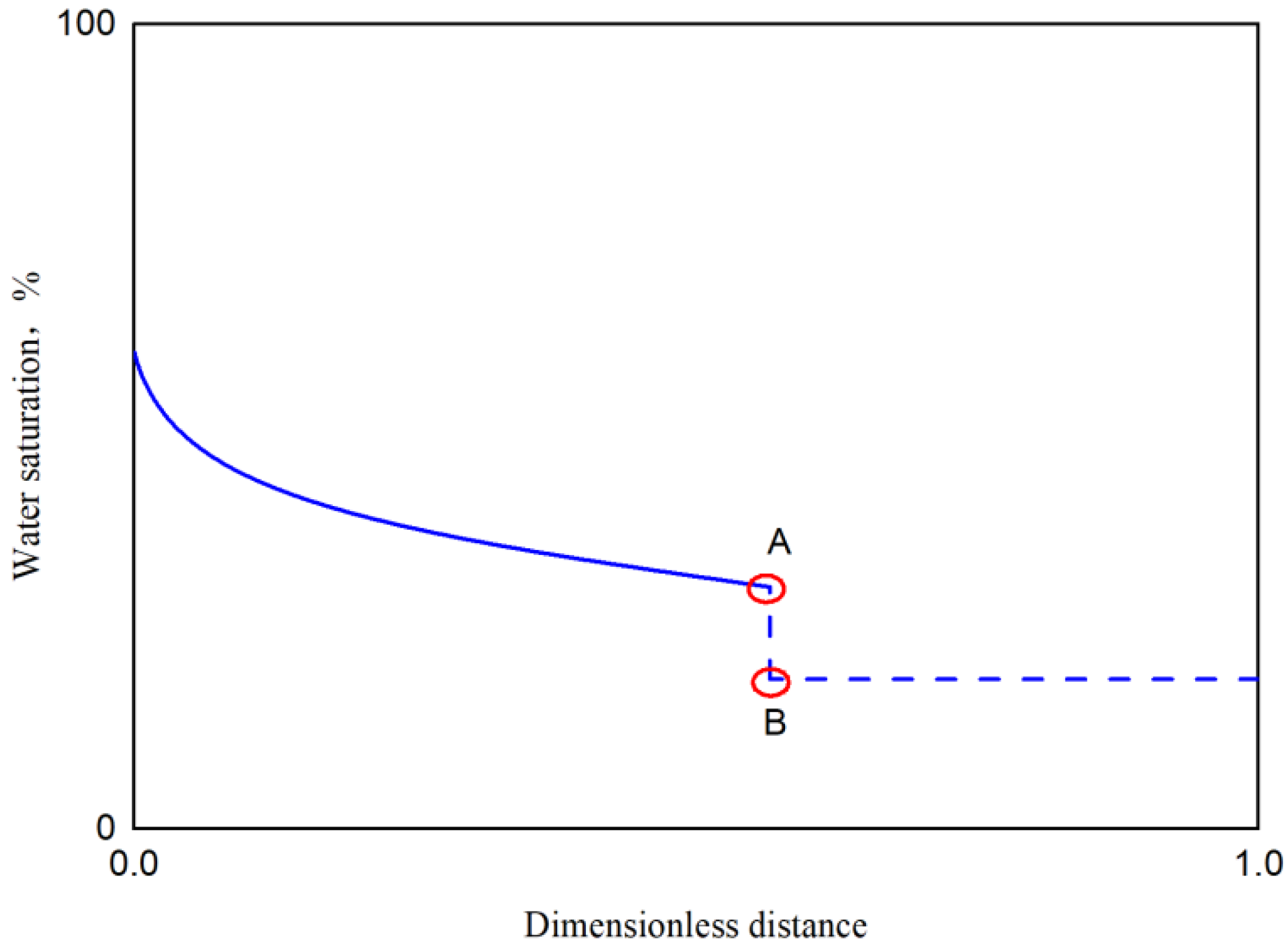
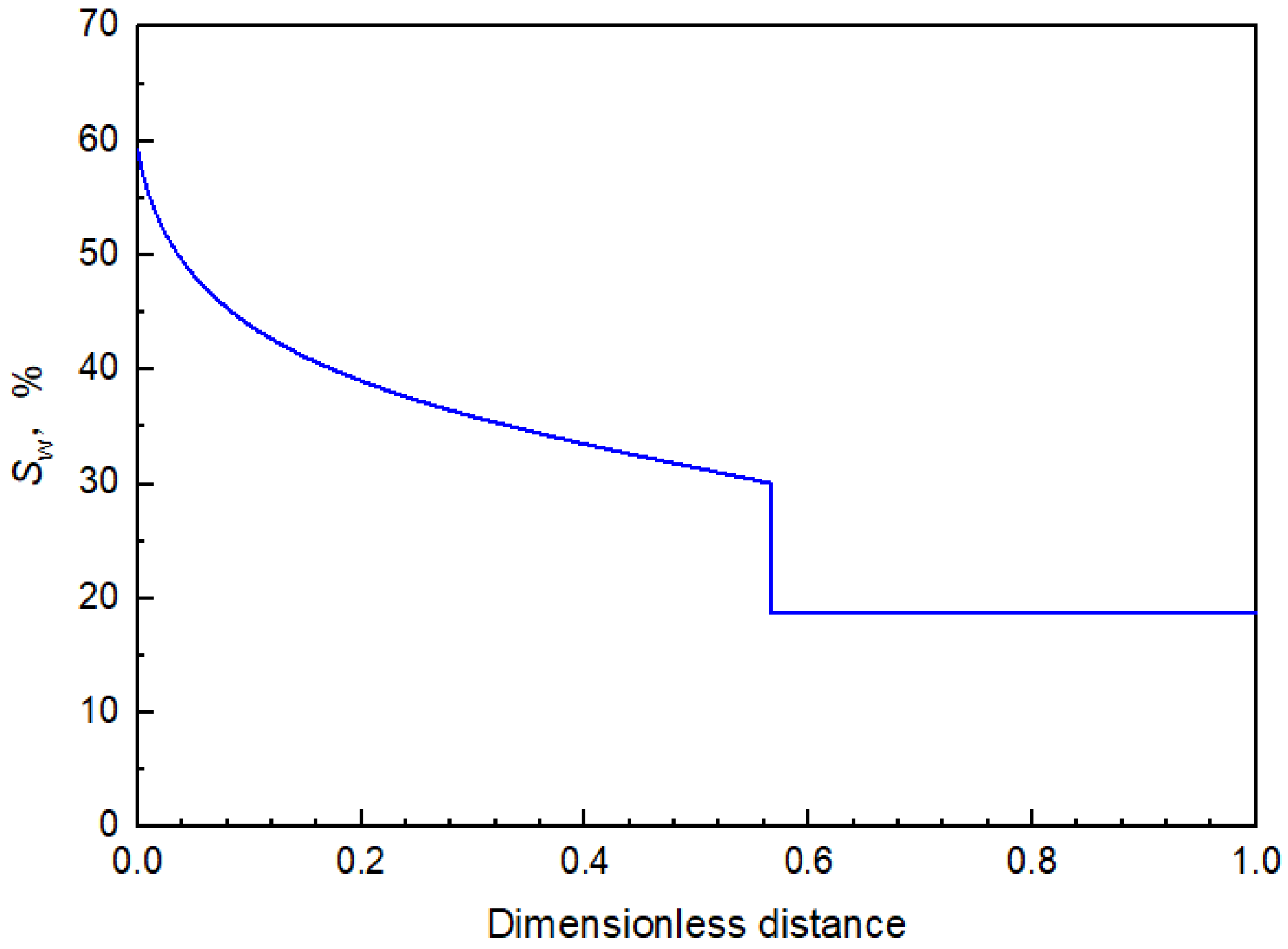
3.2. Model Validation
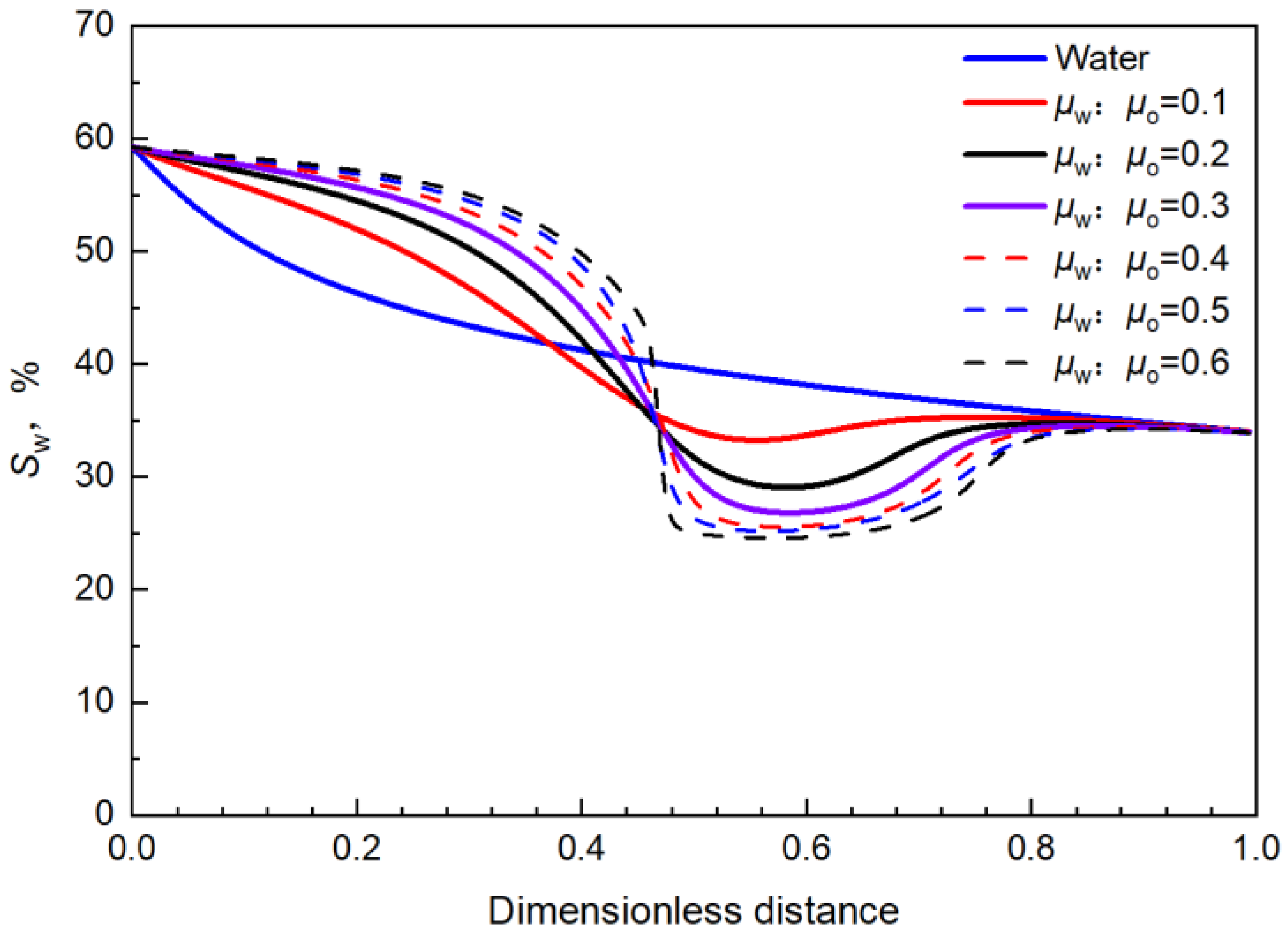
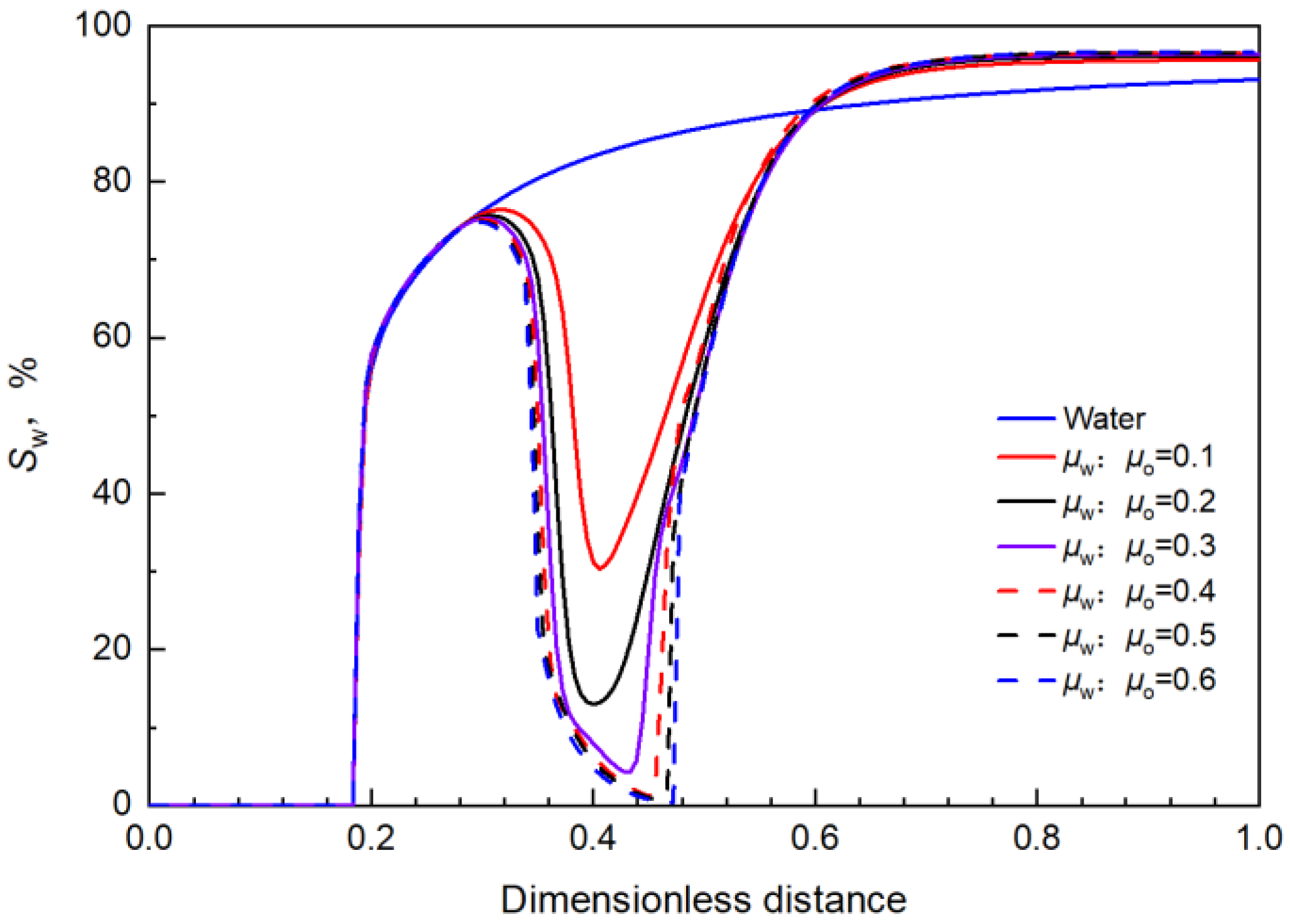
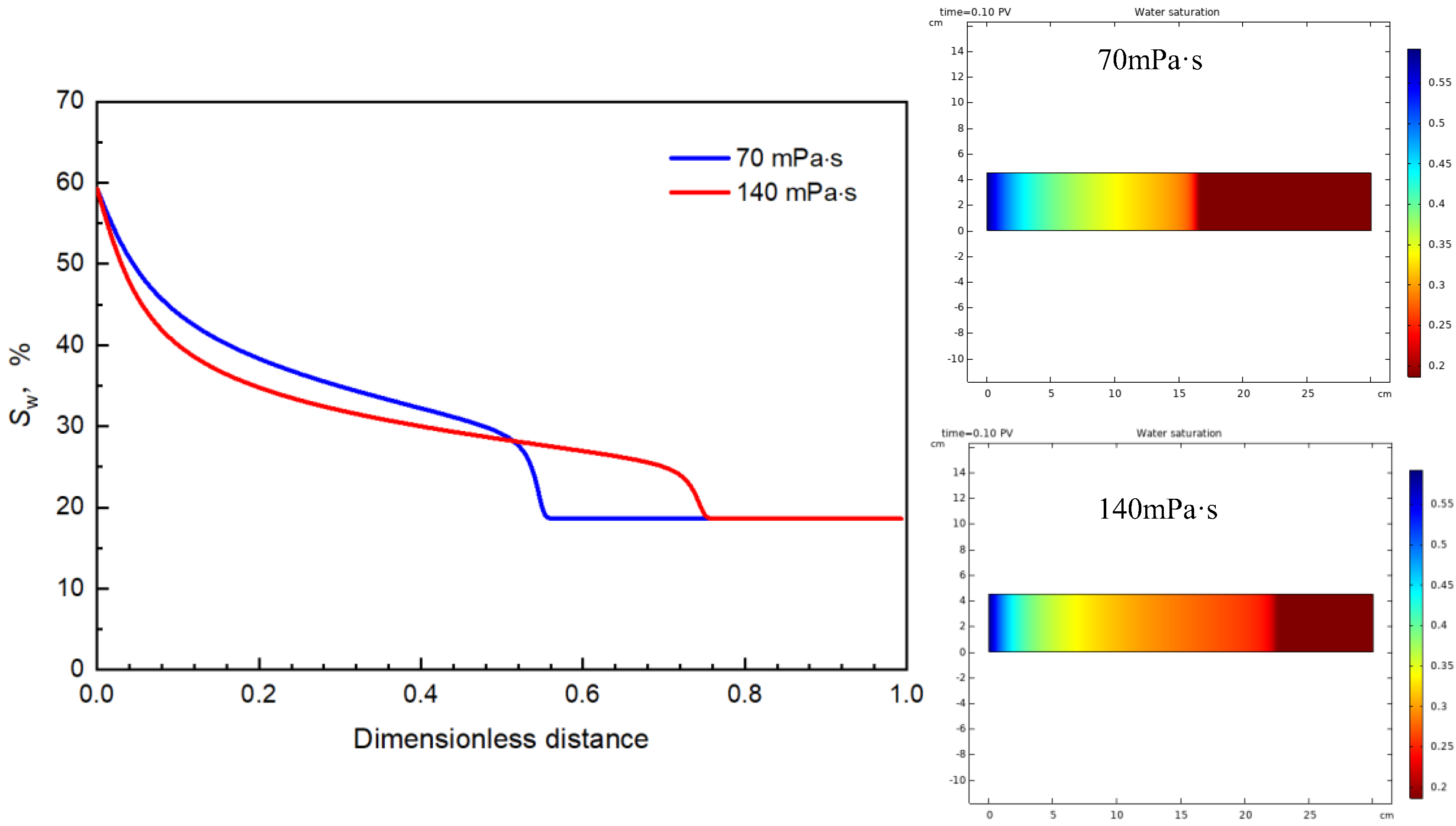
3.3. Study on the Influence of Different Viscosity Conditions on the Seepage Process
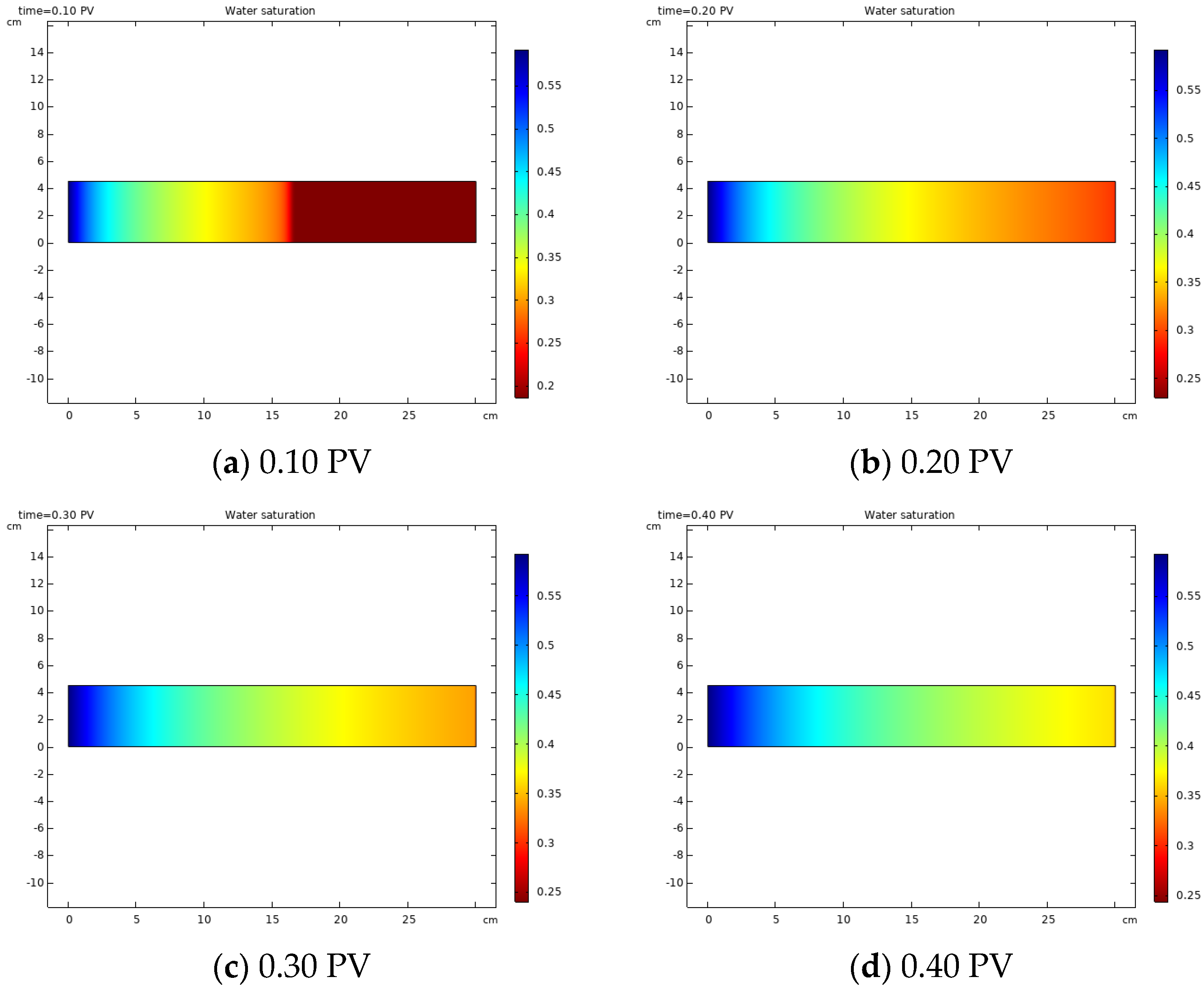
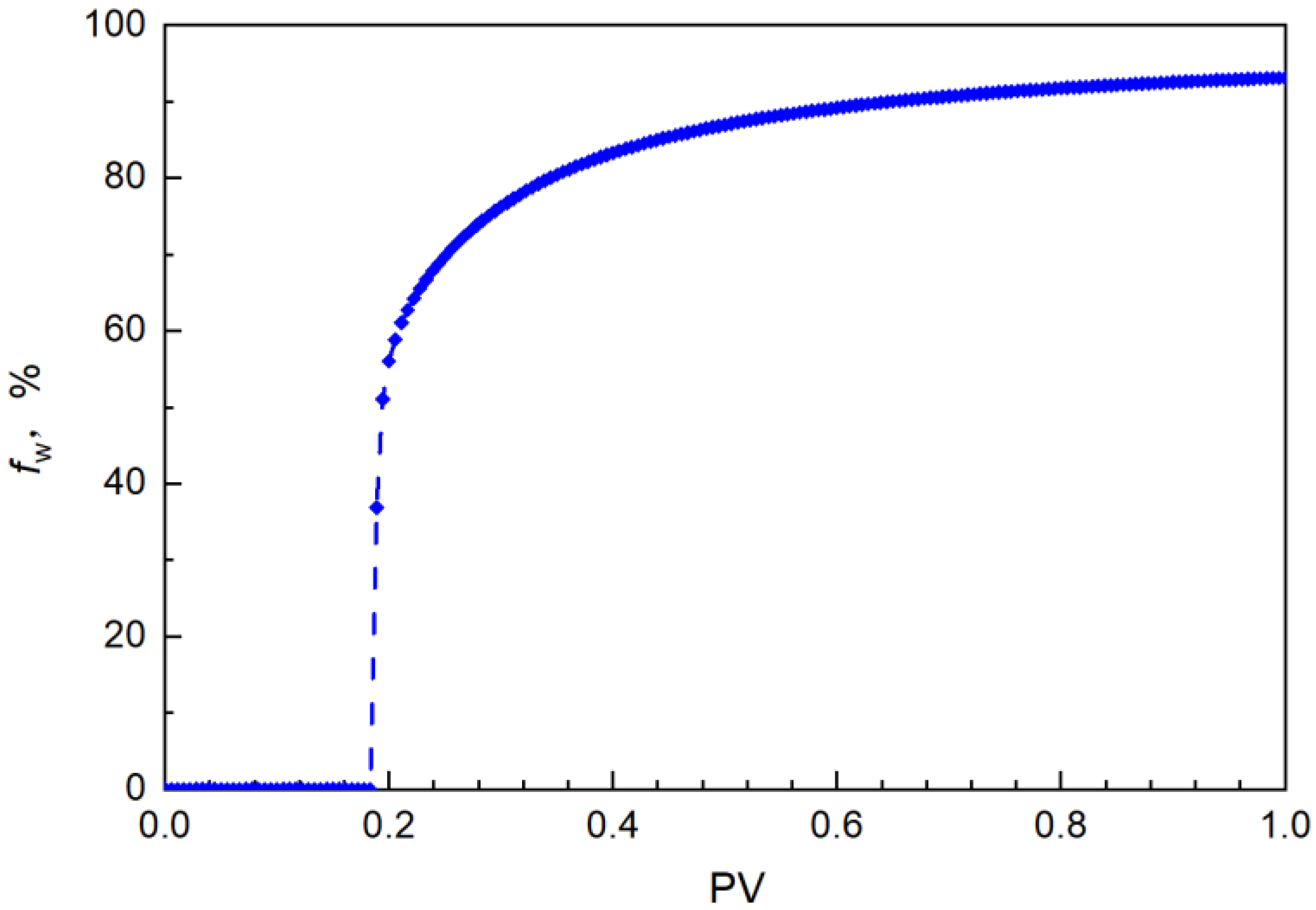
3.3.1. Simulation of the Water Flooding Process
3.3.2. Simulation the Process of Polymer Flooding after Water Flooding
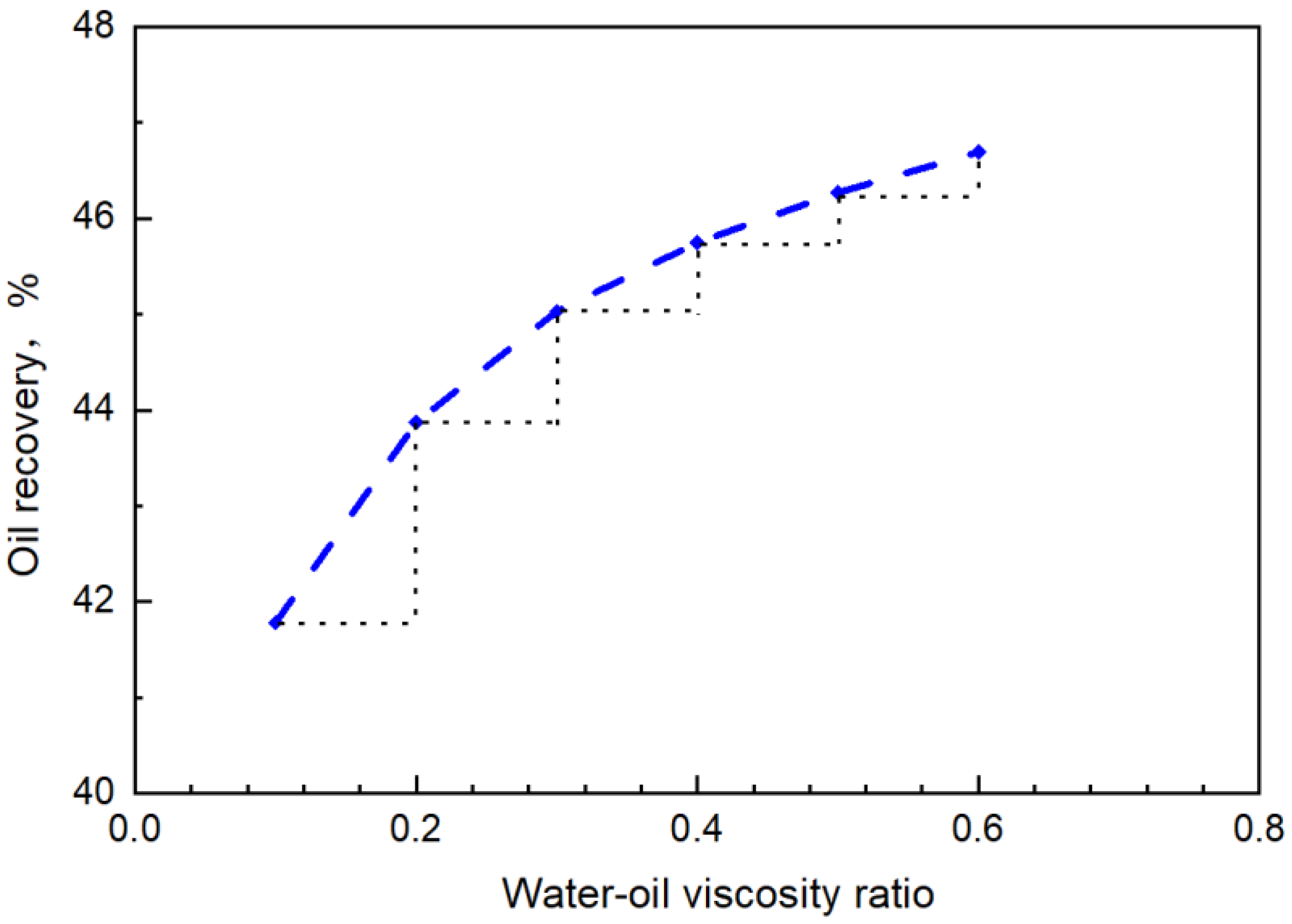
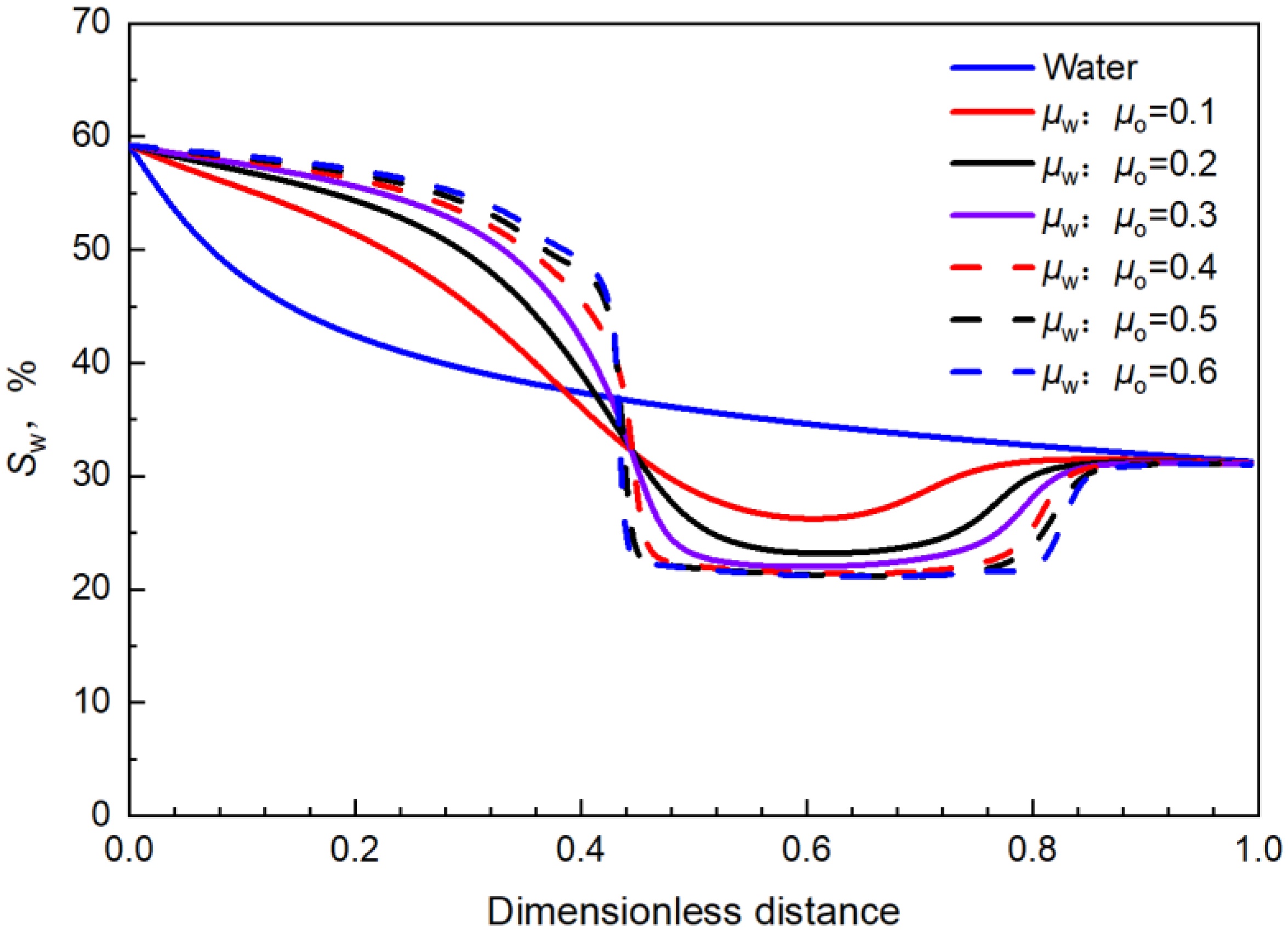
3.3.3. Comparison of Oil Displacement Effect under Different Water-Oil Viscosity Ratio Conditions
3.3.4. Effect of Increasing Oil Viscosity on the Displacement
4. Summary and Conclusions
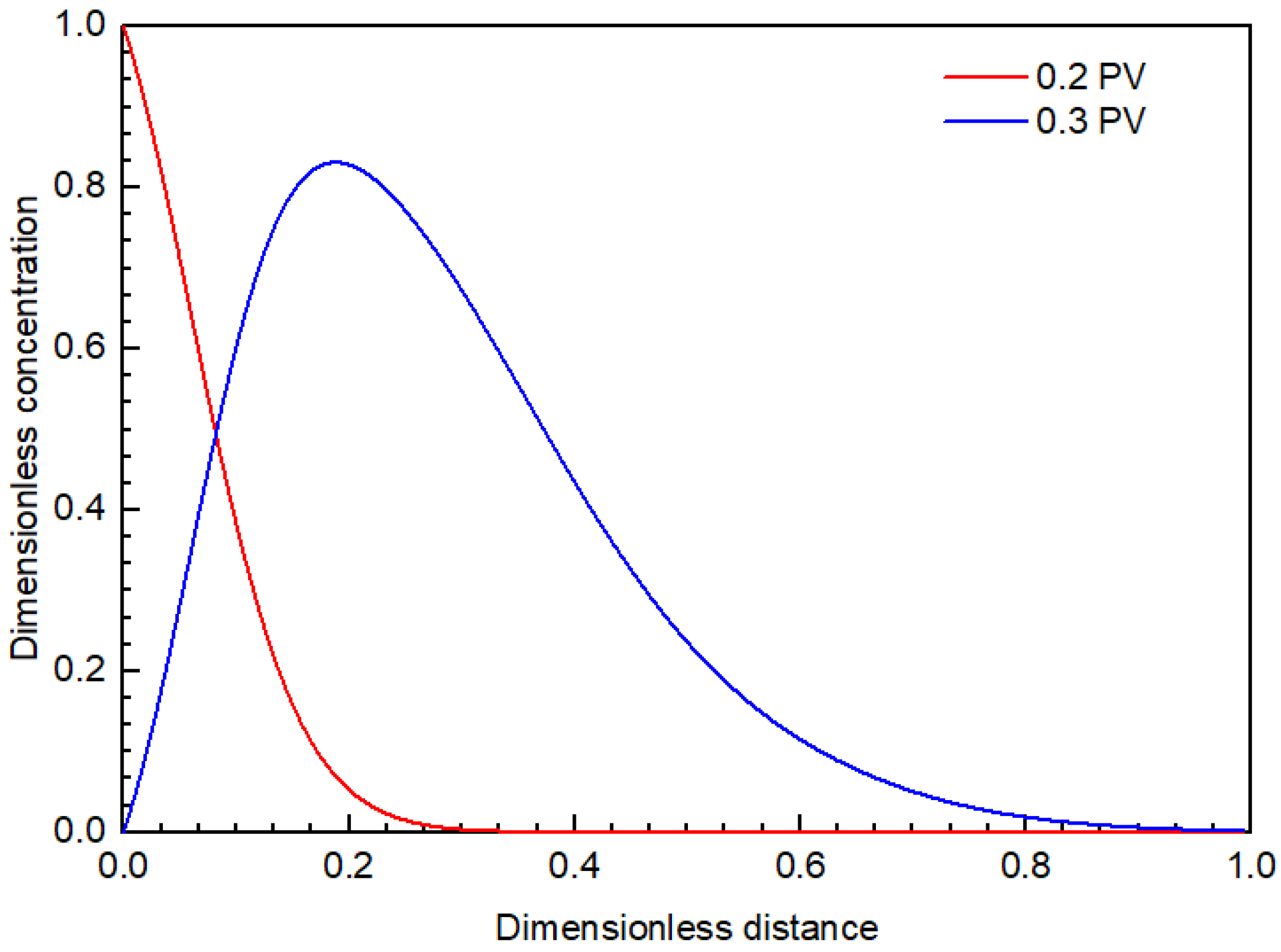
- The mobility control of polymer solution promotes oil phase accumulation, resulting in the decrease in water saturation along the flow, when the polymer solution viscosity increases, the water saturation decreasing region and the decreasing degree also increase.
- The viscosity of the polymer solution has an optimal value. When it reaches this value, the water cut will be reduced to the limit value under the action of the polymer solution. When the viscosity is further increased, the injection pressure is further increased, but the displacement effect will not change greatly.
- When the water-oil viscosity ratio increased from 0.1 to 0.6, oil viscosity of 70 mPa·s and 140 mPa·s have similar polymer solution displacement laws, and the optimal water-oil viscosity ratio under the two oil viscosity conditions is 0.4.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Shah, A.; Fishwick, R.; Wood, J.; Leeke, G.; Rigby, S.; Greaves, M. A review of novel techniques for heavy oil and bitumen extraction and upgrading. Energy Environ. Sci. 2010, 3, 700–714. [Google Scholar] [CrossRef]
- Mai, A.; Bryan, J.; Goodarzi, N.; Kantzas, A. Insights into Non-Thermal Recovery of Heavy Oil. J. Can. Pet. Technol. 2009, 48, 27–35. [Google Scholar] [CrossRef]
- Chen, Z.; Zhao, X.; Wang, Z.; Fu, M. A comparative study of inorganic alkaline/polymer flooding and organic alkaline/polymer flooding for enhanced heavy oil recovery. Colloids Surf. A Physicochem. Eng. Asp. 2015, 469, 150–157. [Google Scholar] [CrossRef]
- Khalilinezhad, S.S.; Hashemi, A.; Mobaraki, S.; Zakavi, M.; Jarrahian, K. Experimental Analysis and Numerical Modeling of Polymer Flooding in Heavy Oil Recovery Enhancement: A Pore-Level Investigation. Arab. J. Sci. Eng. 2019, 44, 10447–10465. [Google Scholar] [CrossRef]
- Mohammadi, S.; Masihi, M.; Ghazanfari, M. Characterizing the Role of Shale Geometry and Connate Water Saturation on Performance of Polymer Flooding in Heavy Oil Reservoirs: Experimental Observations and Numerical Simulations. Transp. Porous Media 2012, 91, 973–998. [Google Scholar] [CrossRef]
- Lie, K.A.; Nilsen, H.M.; Rasmussen, A.F.; Raynaud, X. Fast simulation of polymer injection in heavy-oil reservoirs on the basis of topological sorting and sequential splitting. SPE Journal 2014, 19, 991–1004. [Google Scholar] [CrossRef]
- Xin, X.; Yu, G.; Chen, Z.; Wu, K.; Dong, X.; Zhu, Z. Effect of Non-Newtonian Flow on Polymer Flooding in Heavy Oil Reservoirs. Polymers 2018, 10, 1225. [Google Scholar] [CrossRef] [Green Version]
- Shi, L.-T.; Zhu, S.; Zhang, J.; Wang, S.-X.; Xue, X.-S.; Zhou, W.; Ye, Z.-B. Research into polymer injection timing for Bohai heavy oil reservoirs. Pet. Sci. 2015, 12, 129–134. [Google Scholar] [CrossRef] [Green Version]
- Li, Z.; Hou, J.; Liu, W.; Liu, Y. Multi-molecular mixed polymer flooding for heavy oil recovery. J. Dispers. Sci. Technol. 2022, 43, 1700–1708. [Google Scholar] [CrossRef]
- Pei, H.; Zhang, G.; Ge, J.; Zhang, L.; Wang, H. Effect of polymer on the interaction of alkali with heavy oil and its use in improving oil recovery. Colloids Surf. A: Physicochem. Eng. Asp. 2014, 446, 57–64. [Google Scholar] [CrossRef]
- Wassmuth, F.; Arnold, W.; Green, K.; Cameron, N. Polymer Flood Application to Improve Heavy Oil Recovery at East Bodo. J. Can. Pet. Technol. 2009, 48, 55–61. [Google Scholar] [CrossRef] [Green Version]
- Asghari, K.; Nakutnyy, P. Experimental Results of Polymer Flooding of Heavy Oil Reservoirs. In Proceedings of the Canadian International Petroleum Conference, Calgary, Alberta, 17–19 June 2008. [Google Scholar] [CrossRef]
- Guo, H. How to Select Polymer Molecular Weight and Concentration to Avoid Blocking in Polymer Flooding? In Proceedings of the SPE Symposium: Production Enhancement and Cost Optimisation, Kuala Lumpur, Malaysia, 7–8 November 2017. [Google Scholar] [CrossRef]
- Delamaide, E.; Zaitoun, A.; Renard, G.; Tabary, R. Pelican Lake Field: First Successful Application of Polymer Flooding in a Heavy-Oil Reservoir. SPE Reserv. Eval. Eng. 2014, 17, 340–354. [Google Scholar] [CrossRef]
- Demin, W.; Gang, W.; Huifen, X. Large Scale High Viscous-Elastic Fluid Flooding in the Field Achieves High Recoveries. In Proceedings of the SPE Enhanced Oil Recovery Conference, Kuala Lumpur, Malaysia, 19–20 July 2011. [Google Scholar] [CrossRef]
- Seright, R.S. How Much Polymer Should Be Injected During a Polymer Flood? Review of Previous and Current Practices. SPE J. 2016, 22, 1–18. [Google Scholar] [CrossRef]
- Seright, R.S.; Wang, D.; Lerner, N.; Nguyen, A.; Sabid, J.; Tochor, R. Can 25-cp Polymer Solution Efficiently Displace 1600-cp Oil During Polymer Flooding? SPE J. 2018, 23, 2260–2278. [Google Scholar] [CrossRef]
- Levitt, D.; Jouenne, S.; Bondino, I.; Santanach-Carreras, E.; Bourrel, M. Polymer Flooding of Heavy Oil Under Adverse Mobility Conditions. In Proceedings of the SPE Enhanced Oil Recovery Conference, Kuala Lumpur, Malaysia, 2–4 July 2013. [Google Scholar] [CrossRef]
- Wang, J.; Dong, M. Optimum effective viscosity of polymer solution for improving heavy oil recovery. J. Pet. Sci. Eng. 2009, 67, 155–158. [Google Scholar] [CrossRef]
- Wang, J.; Dong, M. A Laboratory Study of Polymer Flooding for Improving Heavy Oil Recovery. In Proceedings of the Canadian International Petroleum Conference, Calgary, Alberta, 12–14 June 2007. [Google Scholar] [CrossRef]
- Guo, Z.; Dong, M.; Chen, Z.; Yao, J. A fast and effective method to evaluate the polymer flooding potential for heavy oil reservoirs in Western Canada. J. Pet. Sci. Eng. 2013, 112, 335–340. [Google Scholar] [CrossRef]
- Guo, Z.; Dong, M.; Chen, Z.; Yao, J. Dominant Scaling Groups of Polymer Flooding for Enhanced Heavy Oil Recovery. Ind. Eng. Chem. Res. 2013, 52, 911–921. [Google Scholar] [CrossRef]
- Olayiwola, S.O.; Dejam, M. Comprehensive experimental study on the effect of silica nanoparticles on the oil recovery during alternating injection with low salinity water and surfactant into carbonate reservoirs. J. Mol. Liq. 2021, 325, 115178. [Google Scholar] [CrossRef]
- Zhu, S.; Shi, L.; Wang, X.; Liu, C.; Xue, X.; Ye, Z. Investigation into mobility control mechanisms by polymer flooding in offshore high-permeable heavy oil reservoir. Energy Sources Part A Recover. Util. Environ. Eff. 2020, 1–14. [Google Scholar] [CrossRef]
- Manichand, R.N.; Mogollon, J.L.; Bergwijn, S.S.; Graanoogst, F.; Ramdajal, R. Preliminary Assessment of Tambaredjo Heavy Oilfield Polymer Flooding Pilot Test. In Proceedings of the SPE Latin American and Caribbean Petroleum Engineering Conference, Lima, Peru, 1–3 December 2010. [Google Scholar] [CrossRef]
- Gao, C. Scientific research and field applications of polymer flooding in heavy oil recovery. J. Pet. Explor. Prod. Technol. 2011, 1, 65–70. [Google Scholar] [CrossRef] [Green Version]
- Ameli, F.; Moghbeli, M.R.; Alashkar, A. On the effect of salinity and nano-particles on polymer flooding in a heterogeneous porous media: Experimental and modeling approaches. J. Pet. Sci. Eng. 2019, 174, 1152–1168. [Google Scholar] [CrossRef]
- Amiri, H.A.; Hamouda, A. Pore-scale modeling of non-isothermal two phase flow in 2D porous media: Influences of viscosity, capillarity, wettability and heterogeneity. Int. J. Multiph. Flow 2014, 61, 14–27. [Google Scholar] [CrossRef]
- Afzali, S.; Zendehboudi, S.; Mohammadzadeh, O.; Rezaei, N. Hybrid mathematical modelling of three-phase flow in porous media: Application to water-alternating-gas injection. J. Nat. Gas Sci. Eng. 2021, 94, 103966. [Google Scholar] [CrossRef]
- Honarpour, M.; Mahmood, S. Relative-Permeability Measurements: An Overview. J. Pet. Technol. 1988, 40, 963–966. [Google Scholar] [CrossRef]
- Johnson, E.; Bossler, D.; Bossler, V.N. Calculation of Relative Permeability from Displacement Experiments. Trans. AIME 1959, 216, 370–372. [Google Scholar] [CrossRef]
- Huang, Y.; Liu, F.; Yang, Z. Mass transfer of complex chemical fluids in porous media. Chin. J. Theor. Appl. Mech. 2002, 34, 256–260. (In Chinese) [Google Scholar]








| Ion Types | Na+, K+ | Ca2+ | Mg2+ | CO32− | HCO3− | SO42− | Cl− | TDS |
|---|---|---|---|---|---|---|---|---|
| Concentration (mg/L) | 3091.96 | 276.16 | 158.68 | 14.21 | 311.48 | 85.29 | 5436.34 | 9374.12 |
| Swi | Sor | Kro (Swi) | Krw (Sor) | no | nw |
|---|---|---|---|---|---|
| 0.19 | 0.41 | 1.00 | 0.08 | 2.10 | 1.60 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dou, X.; Wang, A.; Wang, S.; Shao, D.; Xing, G.; Qian, K. Study on the Viscosity Optimization of Polymer Solutions in a Heavy Oil Reservoir Based on Process Simulation. Energies 2022, 15, 9473. https://doi.org/10.3390/en15249473
Dou X, Wang A, Wang S, Shao D, Xing G, Qian K. Study on the Viscosity Optimization of Polymer Solutions in a Heavy Oil Reservoir Based on Process Simulation. Energies. 2022; 15(24):9473. https://doi.org/10.3390/en15249473
Chicago/Turabian StyleDou, Xiangji, An Wang, Shikai Wang, Dongdong Shao, Guoqiang Xing, and Kun Qian. 2022. "Study on the Viscosity Optimization of Polymer Solutions in a Heavy Oil Reservoir Based on Process Simulation" Energies 15, no. 24: 9473. https://doi.org/10.3390/en15249473
APA StyleDou, X., Wang, A., Wang, S., Shao, D., Xing, G., & Qian, K. (2022). Study on the Viscosity Optimization of Polymer Solutions in a Heavy Oil Reservoir Based on Process Simulation. Energies, 15(24), 9473. https://doi.org/10.3390/en15249473







