Levelized Cost of Electricity Generation by Small Hydropower Projects under Clean Development Mechanism in India
Abstract
:1. Introduction
1.1. Small Hydropower and Clean Development Mechanism Technology
1.2. CDM Potential of SHP
2. Methodology
2.1. Cost Correlations of SHP
2.2. LCOE Calculations
3. Results and Discussion
- (i)
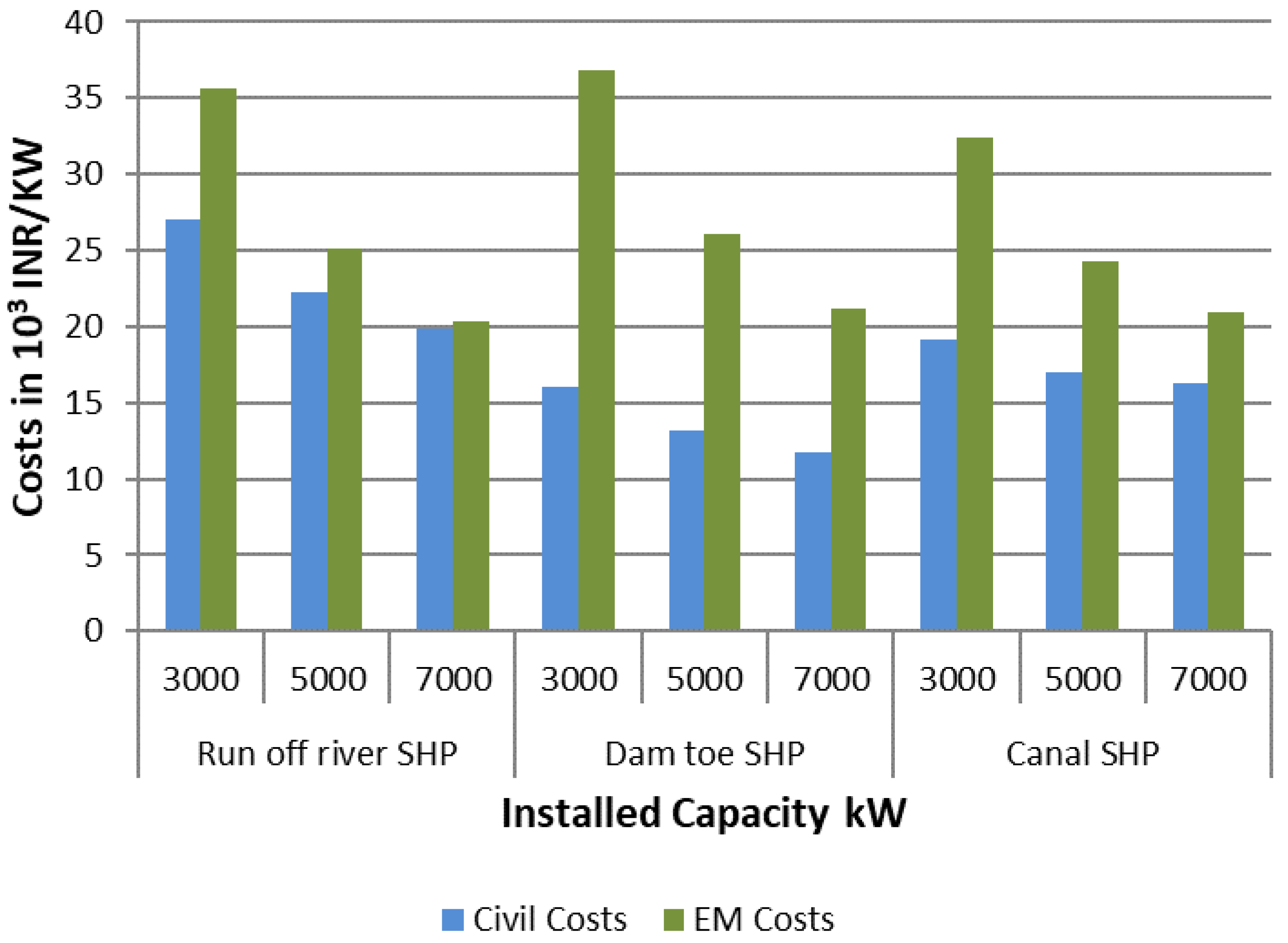
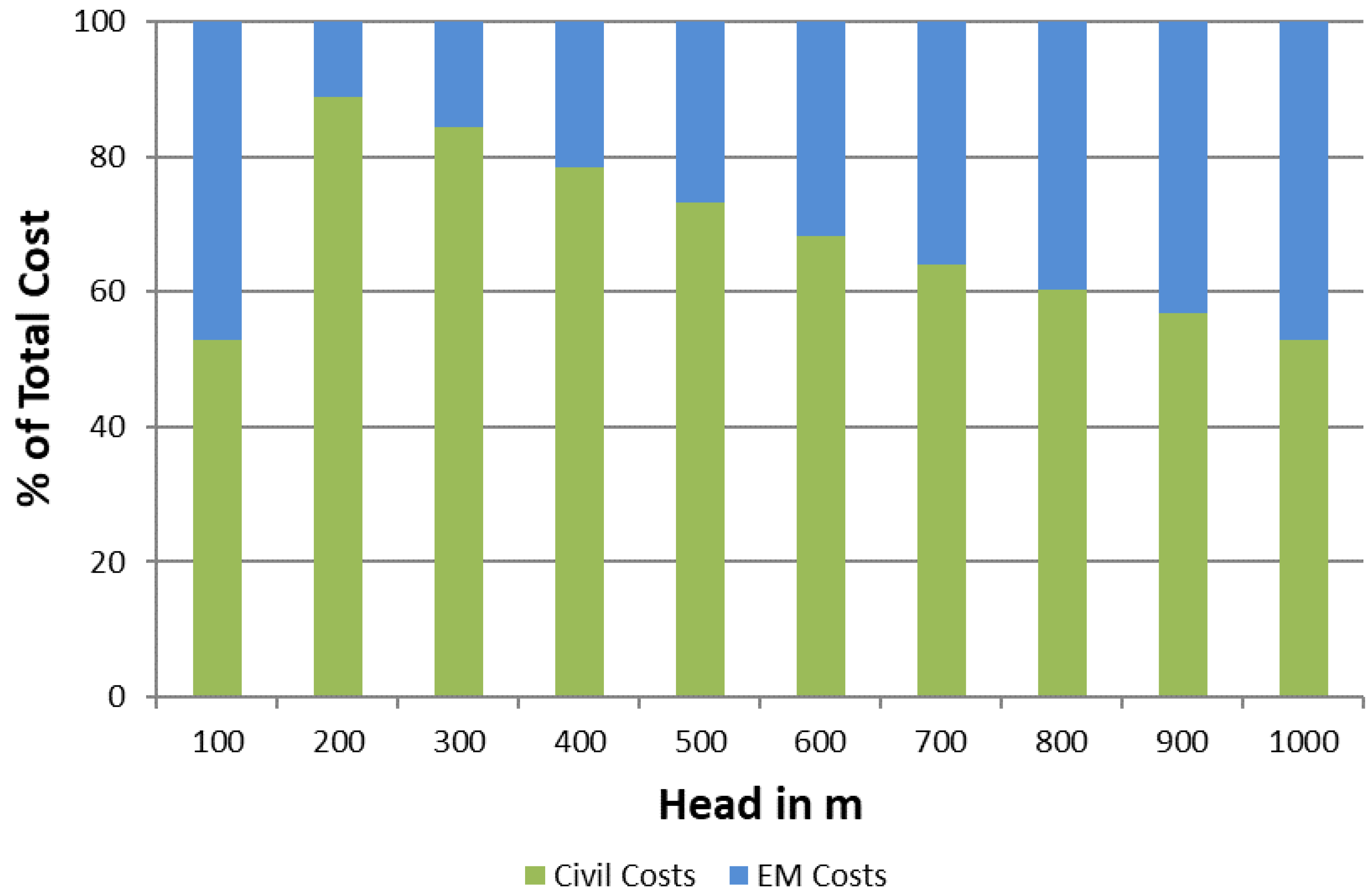
- In all the three cases of low head SHP via dam toe, run-off-the-river, and canal-based schemes, the costs of electro-mechanical work were more than civil works, as illustrated in Figure 2. This is because, in low head SHP schemes, the machine sizes are relatively larger, the size of powerhouse building and other civil works components are directly affected by the type and size of machines and, hence, electro-mechanical equipment has a bigger cost contribution than civil works. However, for high head SHP plants, civil works costs are more than electro-mechanical components cost works as illustrated in Figure 3. This is because the capital investment of medium and high head SHP schemes are governed by the civil works cost as these schemes are site specific.
- (ii)
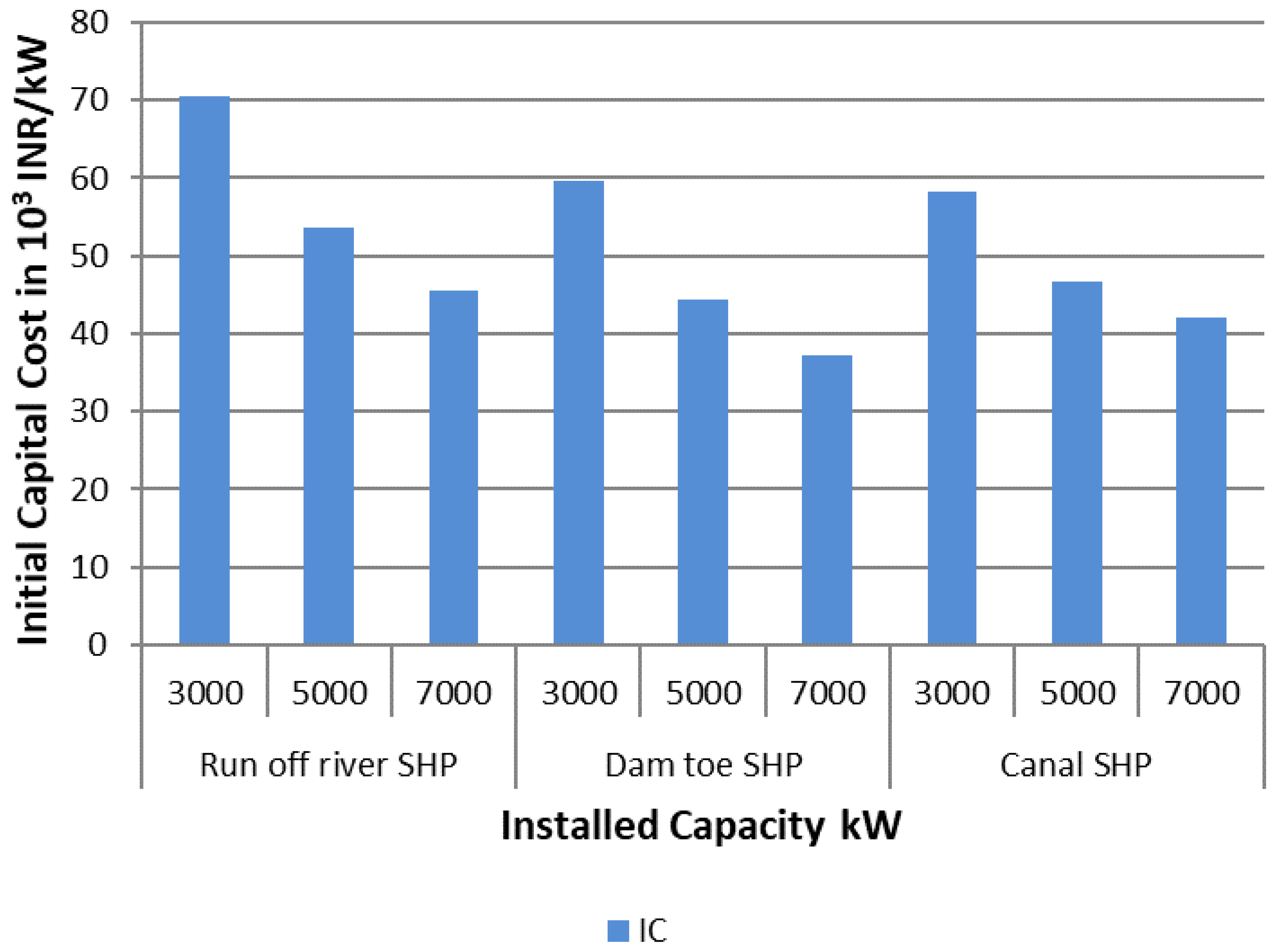
- IC, which is a combination of cost of civil works, electro-mechanical components and administrative, charges is more for SHP with low head and capacity. This decreases with an increase in head or capacity of the plant as illustrated in Figure 4 and Figure 5 for low and high head SHP plants, respectively.
- (iii)
- From Figure 4, it can be observed that for the same head and capacity, IC of run-off-river is highest followed by dam-toe, further followed by canal-based plants. An exception can be found in canal-based SHP plants where the cost is more as compared to dam-toe plants. This is due to the impractical techno-economic feasibility of having a canal-based SHP plant with high heads.
- (iv)
- LCC has been evaluated taking into account the present values of various cost components. Such as IC, OMC, RC and CER. The LCC of SHP plants for all cases has been calculated and is 85% or approximately seven times higher than the corresponding IC.
- (v)
- Compared to the benchmarked cost of SHP in India, i.e., INR 100 Million per MW, the analyzed costs are very close to benchmarked cost with a minor deviation of +0.42 to −0.16 for all the low head SHP plants under consideration.
- (vi)
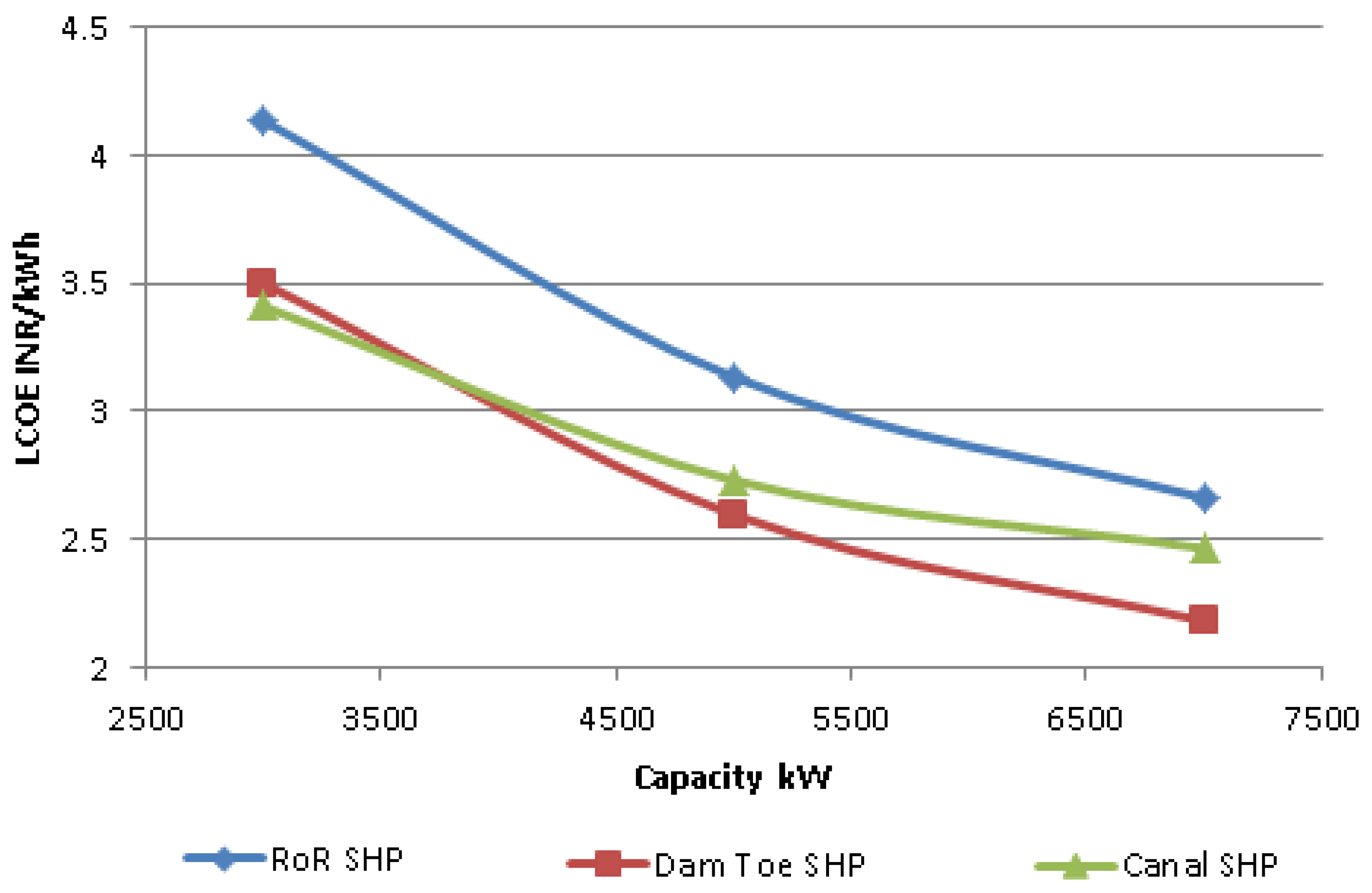
- Compared to the average benchmarked cost for sale of electricity generated from SHP in India, i.e., INR 5 per kW, the obtained LCOE with and without CER cost considerations is less, indicating scope for reasonable profits and sustainable operation SHP over its lifetime. In the case of low head SHP schemes, the LCOE reduces significantly with increased capacity as illustrated in Figure 6. A deviation in two combinations (2000 kW, 200 m and 300 m) for high head SHP projects can be observed, illustrated in Figure 7, which are accounted to the impractical techno-economic feasibility of constructing the plant with a combination of penstock material, i.e., HDPE (200 m and 300 m) and turgo impulse for 200 m and Pelton for 300 m, respectively.
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| AEP | Annual Energy Production | IC | Initial Capital |
| c | Cost of issuing 1 CER | INR | Indian Rupee |
| CAUX | Cost of auxiliaries | LCC | Life Cycle Cost |
| CCIV | Cost of civil works | LCOE | Levelized Cycle Cost of Electricity |
| CDC | Cost of desiltying chamber | NPV | Net Present Value |
| CDM | Clean Development Mechanism | OMC | Operation and Maintenance Cost |
| CDW | Cost of diversion weir | P | Plant Capacity |
| CDW&I | Cost of diversion weir and intake | PLF | Plant Load Factor |
| CEF | Carbon Emission Factor | Q | Discharge |
| CEM | Cost of electromechanical equipment | T | Project operating life |
| CER | Certified Emission Reductions | TVO | Total Value |
| CF&S | Cost of forebay and spillway | UNFCCC | United Nations Framework Convention on Climate Change |
| CGE | Cost of generator exciter system | X | Returns from electricity sale |
| CI | Cost of intake | Y | Returns from CER sale |
| CPC | Cost of power channel | η | Efficiency |
| CPHB | Cost of power house building | ρ | Water density |
| CPST | Cost of penstock | Electricity cost | |
| CS | Cost of spillway | CER cost | |
| CSHP | Cost of SHP plant | Weighted average price of electricity | |
| CT&SY | Cost of transformer and switchyard | Weighted average price of CER sale | |
| CTG | Cost of turbine governor system | XO | Electricity volume |
| CTR | Cost of tailrace | YO | CER volume |
| g | Acceleration due to gravity | Input Price Vector | |
| GCE | Gross Annual CO2 Emissions | State variables set | |
| GHG | Greenhouse Gases | τ | CER issuance period |
| H | Head |
References
- Zhao, Z.Y.; Li, Z.W.; Xia, B. The impact of the CDM (clean development mechanism) on the cost price of wind power electricity: A China study. Energy 2014, 69, 179–185. [Google Scholar] [CrossRef] [Green Version]
- Spalding-Fecher, R.; Joyce, B.; Winkler, H. Climate change and hydropower in the Southern African Power Pool and Zambezi River Basin: System-wide impacts and policy implications. Energy Policy 2017, 103, 84–97. [Google Scholar] [CrossRef]
- USEIA. Levelized Costs of New Generation Resources in the Annual Energy Outlook 2021; U.S. Energy Information Administration: Washington, DC, USA, 2021. Available online: https://www.eia.gov/outlooks/aeo/electricity_generation.php (accessed on 1 December 2021).
- Branker, K.; Pathak, M.J.M.; Pearce, J.M. A review of solar photovoltaic levelized cost of electricity. Renew. Sustain. Energy Rev. 2011, 15, 4470–4482. [Google Scholar] [CrossRef] [Green Version]
- Sims, R.E.H.; Rogner, H.H.; Gregory, K. Carbon emission and mitigation cost comparisons between fossil fuel, nuclear and renewable energy resources for electricity generation. Energy Policy 2003, 31, 1315–1326. [Google Scholar] [CrossRef]
- Larsson, S.; Fantazzini, D.; Davidsson, S.; Kullander, S.; Höök, M. Reviewing electricity production cost assessments. Renew. Sustain. Energy Rev. 2014, 30, 170–183. [Google Scholar] [CrossRef] [Green Version]
- Edomah, N.; Foulds, C.; Jones, A. Influences on energy supply infrastructure: A comparison of different theoretical perspectives. Renew. Sustain. Energy Rev. 2017, 79, 765–778. [Google Scholar] [CrossRef] [Green Version]
- Bhattacharyya, S.C. Economic Analysis of Energy Investments. In Energy Economics; Springer: London, UK, 2011; pp. 163–189. [Google Scholar] [CrossRef]
- Kishore, T.S.; Patro, E.R.; Harish, V.S.K.V.; Haghighi, A.T. A Comprehensive Study on the Recent Progress and Trends in Development of Small Hydropower Projects. Energies 2021, 14, 2882. [Google Scholar] [CrossRef]
- Cavazzini, G.; Santolin, A.; Pavesi, G.; Ardizzon, G. Accurate estimation model for small and micro hydropower plants costs in hybrid energy systems modelling. Energy 2016, 103, 746–757. [Google Scholar] [CrossRef]
- Carapellucci, R.; Giordano, L.; Pierguidi, F. Techno-economic evaluation of small-hydro power plants: Modelling and characterisation of the Abruzzo region in Italy. Renew. Energy 2015, 75, 395–406. [Google Scholar] [CrossRef]
- Zema, D.A.; Nicotra, A.; Tamburino, V.; Zimbone, S.M. A simple method to evaluate the technical and economic feasibility of micro hydro power plants in existing irrigation systems. Renew. Energy 2016, 85, 498–506. [Google Scholar] [CrossRef]
- Raimondi, A.; Bettoni, F.; Bianchi, A.; Becciu, G. Economic Sustainability of Small-Scale Hydroelectric Plants on a National Scale—The Italian Case Study. Water 2021, 13, 1170. [Google Scholar] [CrossRef]
- Balkhair, K.S.; Rahman, K.U. Sustainable and economical small-scale and low-head hydropower generation: A promising alternative potential solution for energy generation at local and regional scale. Appl. Energy 2017, 188, 378–391. [Google Scholar] [CrossRef]
- Filho, G.L.T.; dos Santos, I.F.S.; Barros, R.M. Cost estimate of small hydroelectric power plants based on the aspect factor. Renew. Sustain. Energy Rev. 2017, 77, 229–238. [Google Scholar] [CrossRef]
- Rahi, O.P.; Kumar, A. Economic analysis for refurbishment and uprating of hydro power plants. Renew. Energy 2016, 86, 1197–1204. [Google Scholar] [CrossRef]
- Quaranta, E.; Aggidis, G.; Boes, R.M.; Comoglio, C.; De Michele, C.; Patro, E.R.; Georgievskaia, E.; Harby, A.; Kougias, I.; Muntean, S.; et al. Assessing the energy potential of modernizing the European hydropower fleet. Energy Convers. Manag. 2021, 246, 114655. [Google Scholar] [CrossRef]
- Patro, E.R.; Voltz, T.J.; Kumar, A.; Grischek, T. Micro-hydropower in drinking water gravity pipelines: A case study in Uttarakhand, India. ISH J. Hydraul. Eng. 2018, 26, 332–342. [Google Scholar] [CrossRef]
- Mishra, S.; Singal, S.K.; Khatod, D.K. A review on electromechanical equipment applicable to small hydropower plants. Int. J. Energy Res. 2012, 36, 553–571. [Google Scholar] [CrossRef]
- Rahman, S.M.; Larson, D.F.; Dinar, A. Costs of greenhouse gas emissions abatement under the clean development mechanism. Clim. Chang. Econ. 2015, 6, 1550005. [Google Scholar] [CrossRef]
- GIZ. Carbon Market Roadmap for India Looking Back on CDM and Looking Ahead; German Development Cooperation: New Delhi, India, 2020; Available online: https://www.giz.de/en/worldwide/368.html (accessed on 1 December 2021).
- CEA. CDM—CO2 Baseline Database; Central Electricity Authority: New Delhi, India, 2019; Available online: https://cea.nic.in/cdm-co2-baseline-database/?lang=en (accessed on 1 December 2021).
- International Energy Agency India. 2020: Energy Policy Review; IEA: France, Paris, 2020; Available online: https://www.iea.org/events/india-energy-policy-review-2020 (accessed on 1 December 2021).
- Liu, D.; Liu, H.; Wang, X.; Kremere, E. World Small Hydropower Development Report 2019; United Nations Industrial Development Organization: Vienna, Austria, 2019; Available online: https://www.unido.org/our-focus-safeguarding-environment-clean-energy-access-productive-use-renewable-energy-focus-areas-small-hydro-power/world-small-hydropower-development-report (accessed on 1 December 2021).
- Rahman, S.M.; Spalding-Fecher, R.; Haites, E.; Kirkman, G.A. The levelized costs of electricity generation by the CDM power projects. Energy 2018, 148, 235–246. [Google Scholar] [CrossRef]
- Singal, S.K.; Saini, R.P.; Raghuvanshi, C.S. Analysis for cost estimation of low head run-of-river small hydropower schemes. Energy Sustain. Dev. 2010, 14, 117–126. [Google Scholar] [CrossRef]
- Singal, S.K.; Saini, R.P. Cost analysis of low-head dam-toe small hydropower plants based on number of generating units. Energy Sustain. Dev. 2008, 12, 55–60. [Google Scholar] [CrossRef]
- Singal, S.K.; Saini, R.P. Analytical approach for development of correlations for cost of canal-based SHP schemes. Renew. Energy 2008, 33, 2549–2558. [Google Scholar] [CrossRef]
- Mishra, S.; Singal, S.K.; Khatod, D.K. Cost Optimization of High Head Run of River Small Hydropower Projects. In Application of Geographical Information Systems and Soft Computation Techniques in Water and Water Based Renewable Energy Problems; Springer: Singapore, 2018; pp. 141–166. [Google Scholar] [CrossRef]
- Singh, V.K.; Singal, S.K. Operation of hydro power plants-a review. Renew. Sustain. Energy Rev. 2017, 69, 610–619. [Google Scholar] [CrossRef]
- Department of Hydro and Renewable Energy. Standards/Manuals/Guidelines for Small Hydro Development; Roorkee: Uttarakhand, India, 2014; Available online: https://www.iitr.ac.in/departments/HRE/pages/Publications+Standard_and_Guidelines.html (accessed on 1 December 2021).
- Lal, S.R.S.; Herbert, G.M.J.; Arjunan, P.; Suryan, A. Advancements in renewable energy transition in India: A review. In Energy Sources, Part A: Recovery, Utilization, and Environmental Effects; Taylor & Francis: Abingdon, UK, 2022; pp. 1–31. [Google Scholar] [CrossRef]
- Kumar, K.; Saini, R.P. A review on operation and maintenance of hydropower plants. Sustain. Energy Technol. Assess. 2022, 49, 101704. [Google Scholar] [CrossRef]
- Fonseca, M.; Bezerra, U.H.; Leite, J.C.; Rodríguez, J.L.M. Maintenance Tools applied to Electric Generators to Improve Energy Efficiency and Power Quality of Thermoelectric Power Plants. Energies 2017, 10, 1091. [Google Scholar] [CrossRef] [Green Version]
- Vera-García, F.; Rubio, J.A.P.; Grau, J.H.; Hernández, D.A. Improvements of a Failure Database for Marine Diesel Engines Using the RCM and Simulations. Energies 2019, 13, 104. [Google Scholar] [CrossRef] [Green Version]



| SHP Type → Components ↓ | Run of River SHP | Dam Toe SHP | Canal-Based SHP |
|---|---|---|---|
| Civil Works Components | Powerhouse building, diversion weir, power channel, desilting chamber, intake channel, forebay, penstock, spillway, tail race | Power house building, intake, penstock, tail race | Power house building, spillway, diversion weir |
| Electro-mechanical Components | Turbine with governing system; switch gear, generator with excitation system, control and protection equipment; mechanical and electrical auxiliaries; switchyard and main transformer equipment. | ||
| S.No. | States/UTs | Small Hydro Power | Installed (MW) | To Be Exploited (MW) | CEF | AEP (TWh) | Estimated CDM Potential (Million CER/Year) | Estimated Revenue (Million INR) |
|---|---|---|---|---|---|---|---|---|
| 1 | Andhra Pradesh | 409.32 | 162.11 | 247.21 | 0.86 | 1.30 | 1.12 | 81.57 |
| 2 | Arunachal Pradesh | 2064.92 | 131.11 | 1933.81 | 0.42 | 10.16 | 4.27 | 311.63 |
| 3 | Assam | 201.99 | 34.11 | 167.88 | 0.42 | 0.88 | 0.37 | 27.05 |
| 4 | Bihar | 526.98 | 70.70 | 456.28 | 1.05 | 2.40 | 2.52 | 183.82 |
| 5 | Chhattisgarh | 1098.20 | 76.00 | 1022.20 | 0.81 | 5.37 | 4.35 | 317.69 |
| 6 | Goa | 4.70 | 0.05 | 4.65 | 0.85 | 0.02 | 0.02 | 1.52 |
| 7 | Gujarat | 201.97 | 68.95 | 133.02 | 0.81 | 0.70 | 0.57 | 41.34 |
| 8 | Haryana | 107.40 | 73.50 | 33.90 | 0.80 | 0.18 | 0.14 | 10.41 |
| 9 | Himachal Pradesh | 3460.34 | 911.51 | 2548.83 | 0.80 | 13.40 | 10.72 | 782.36 |
| 10 | Jammu and Kashmir | 1707.45 | 180.48 | 1526.97 | 0.80 | 8.03 | 6.42 | 468.70 |
| 11 | Jharkhand | 227.96 | 4.05 | 223.91 | 1.05 | 1.18 | 1.24 | 90.21 |
| 12 | Karnataka | 3726.49 | 1280.73 | 2445.76 | 0.86 | 12.85 | 11.06 | 807.03 |
| 13 | Kerala | 647.15 | 222.02 | 425.13 | 0.86 | 2.23 | 1.92 | 140.28 |
| 14 | Madhya Pradesh | 820.44 | 95.91 | 724.53 | 0.81 | 3.81 | 3.08 | 225.17 |
| 15 | Maharashtra | 786.46 | 379.58 | 406.88 | 0.81 | 2.14 | 1.73 | 126.45 |
| 16 | Manipur | 99.95 | 5.45 | 94.50 | 0.42 | 0.50 | 0.21 | 15.23 |
| 17 | Meghalaya | 230.05 | 32.53 | 197.52 | 0.42 | 1.04 | 0.44 | 31.83 |
| 18 | Mizoram | 168.90 | 36.47 | 132.43 | 0.42 | 0.70 | 0.29 | 21.34 |
| 19 | Nagaland | 182.18 | 30.67 | 151.51 | 0.42 | 0.80 | 0.33 | 24.42 |
| 20 | Odisha | 286.22 | 64.63 | 221.59 | 1.05 | 1.16 | 1.22 | 89.27 |
| 21 | Punjab | 578.28 | 173.55 | 404.73 | 0.80 | 2.13 | 1.70 | 124.23 |
| 22 | Rajasthan | 51.67 | 23.85 | 27.82 | 0.80 | 0.15 | 0.12 | 8.54 |
| 23 | Sikkim | 266.64 | 52.11 | 214.53 | 0.42 | 1.13 | 0.47 | 34.57 |
| 24 | Tamil Nadu | 604.46 | 123.05 | 481.41 | 0.86 | 2.53 | 2.18 | 158.85 |
| 25 | Telangana | 102.25 | 90.87 | 11.38 | 0.86 | 0.06 | 0.05 | 3.76 |
| 26 | Tripura | 46.86 | 16.01 | 30.85 | 0.42 | 0.16 | 0.07 | 4.97 |
| 27 | Uttar Pradesh | 460.75 | 25.10 | 435.65 | 0.80 | 2.29 | 1.83 | 133.72 |
| 28 | Uttarakhand | 1664.31 | 214.32 | 1449.99 | 0.8. | 7.62 | 0.00 | 0.00 |
| 29 | West Bengal | 392.06 | 98.50 | 293.56 | 1.05 | 1.54 | 1.62 | 118.27 |
| 30 | Andaman and Nicobar | 7.27 | 5.25 | 2.02 | 0.85 | 0.01 | 0.01 | 0.66 |
| Total | 21,133.62 | 4683.17 | 16,450.45 | 21.60 | 86.46 | 60.07 | 4384.90 | |
| Components → SHP Type ↓ | Civil Works Components | Electromechanical Components |
|---|---|---|
| Run of River SHP | CPHB = 92,615 P−0.2351 H−0.0585 CDW&I = 12,415 P−0.2368 H−0.0597 CPC = 85,383 P−0.3811 H−0.0307 CDC = 20,700 P−0.2385 H−0.0611 CF&S = 25,402 P−0.2356 H−0.0589 CP = 7875 P−0.3806 H0.3804 CTR = 28,164 P−0.376 H−0.624 | CTG = 63,346 P−0.1913 H−0.2171 CGE = 78,661 P−0.1855 H−0.2083 CAUX = 40,860 P−0.1892 H−0.2118 CT&SY = 18,739 P−0.1803 H−0.2075 |
| Dam Toe SHP | CI = 17,940 P−0.2366 H−0.0596 CP = 7875 P−0.3806 H0.3804 CPHB = 85,717 P−0.2355 H−0.0588 CTR = 28,164 P−0.376 H−0.624 | CTG = 66,282 P−0.1866 H−0.2094 CGE = 79,927 P−0.1854 H−0.2097 CAUX = 39,372 P−0.1865 H−0.2107 CT&SY = 18,739 P−0.1803 H−0.2075 |
| Canal-based SHP | CPHB = 105,555 P−0.238 H−0.0602 CS = 36,778 P−0.2306 H−0.0644 CDW = 9909 P−0.2295 H−0.0623 | CTG = 63,346 P−0.1913 H−0.2171 CGE = 78,661 P−0.1855 H−0.2083 CAUX = 40,860 P−0.1892 H−0.2118 CT&SY = 18,739 P−0.1803 H−0.2075 |
| Real Discount Rate | 8% |
| Plant life | 50 years |
| Annual O&M cost | 1.5% |
| Turbine generator rehabilitation time | 25 years |
| Generator exciter rehabilitation time | 25 years |
| Auxiliary equipment rehabilitation time | 10 years |
| Transformer rehabilitation time | 30 years |
| Depreciation tax shield factor | 0.35 |
| Turbine efficiency | 85% |
| Generator efficiency | 90% |
| Annual plant load factor | 36% |
| Average CER cost | 2$ |
| 1 USD | 75 INR |
| The benchmarked sale price of electricity | 5 INR/kWh |
| Plant | Capacity (kW) | Head (m) | CCIV | CEM | CSHP | IC | OMC | RC | CER | LCC | LCOE | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Without CER | With CER | Without CER | With CER | ||||||||||
| Run-off-River SHP | 3000 | 3 | 26,891 | 35,599 | 70,613 | 494,293 | 706 | 4265 | 9228 | 499,264 | 490,036 | 4.14 | 4.06 |
| 5000 | 10 | 22,288 | 25,074 | 53,519 | 374,631 | 535 | 3003 | 9228 | 378,170 | 368,942 | 3.14 | 3.06 | |
| 7000 | 20 | 19,911 | 20,330 | 45,472 | 318,306 | 455 | 2435 | 9228 | 321,195 | 311,968 | 2.66 | 2.59 | |
| Dam Toe SHP | 3000 | 3 | 15,989 | 36,812 | 59,665 | 417,656 | 597 | 4263 | 9228 | 422,516 | 413,288 | 3.50 | 3.43 |
| 5000 | 10 | 13,169 | 26,017 | 44,280 | 309,960 | 443 | 3011 | 9228 | 313,414 | 304,186 | 2.60 | 2.52 | |
| 7000 | 20 | 11,784 | 21,137 | 37,201 | 260,405 | 372 | 2446 | 9228 | 263,223 | 253,995 | 2.18 | 2.11 | |
| Canal-Based SHP | 3000 | 3 | 19,131 | 32,348 | 58,171 | 407,199 | 582 | 3777 | 9228 | 411,558 | 402,330 | 3.41 | 3.34 |
| 5000 | 10 | 17,021 | 24,231 | 46,615 | 326,305 | 466 | 2829 | 9228 | 329,600 | 320,372 | 2.73 | 2.66 | |
| 7000 | 20 | 16,312 | 20,926 | 42,079 | 294,553 | 421 | 2443 | 9228 | 297,417 | 288,189 | 2.47 | 2.39 | |
| Capacity (kW) | Head (m) | CCIV | CEM | CSHP | IC | OMC | RC | CER | LCC | LCOE | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Without CER | With CER | Without CER | With CER | |||||||||
| 2000 | 100 | 38,485 | 34,234 | 82,172 | 575,207 | 822 | 3115 | 10,253 | 579,144 | 568,891 | 4.8 | 4.72 |
| 2000 | 200 | 233,449 | 28,984 | 296,549 | 2,075,845 | 2965 | 2638 | 10,253 | 2,081,448 | 2,071,195 | 17.26 | 17.17 |
| 2000 | 300 | 119,545 | 22,205 | 160,178 | 1,121,243 | 1602 | 2021 | 10,253 | 1,124,865 | 1,114,612 | 9.33 | 9.24 |
| 2000 | 400 | 76,310 | 20,917 | 109,867 | 769,067 | 1099 | 1903 | 10,253 | 772,069 | 761,816 | 6.4 | 6.32 |
| 2000 | 500 | 54,774 | 20,107 | 84,616 | 592,313 | 846 | 1830 | 10,253 | 594,989 | 584,736 | 4.93 | 4.85 |
| 2000 | 600 | 42,283 | 19,576 | 69,902 | 489,311 | 699 | 1781 | 10,253 | 491,792 | 481,538 | 4.08 | 3.99 |
| 2000 | 700 | 34,291 | 19,225 | 60,474 | 423,319 | 605 | 1749 | 10,253 | 425,673 | 415,420 | 3.53 | 3.44 |
| 2000 | 800 | 28,816 | 19,001 | 54,033 | 378,234 | 540 | 1729 | 10,253 | 380,503 | 370,250 | 3.15 | 3.07 |
| 2000 | 900 | 24,868 | 18,866 | 49,419 | 345,936 | 494 | 1717 | 10,253 | 348,147 | 337,894 | 2.89 | 2.80 |
| 2000 | 1000 | 21,186 | 18,797 | 45,181 | 316,268 | 452 | 1711 | 10,253 | 318,430 | 308,177 | 2.64 | 2.55 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Patro, E.R.; Kishore, T.S.; Haghighi, A.T. Levelized Cost of Electricity Generation by Small Hydropower Projects under Clean Development Mechanism in India. Energies 2022, 15, 1473. https://doi.org/10.3390/en15041473
Patro ER, Kishore TS, Haghighi AT. Levelized Cost of Electricity Generation by Small Hydropower Projects under Clean Development Mechanism in India. Energies. 2022; 15(4):1473. https://doi.org/10.3390/en15041473
Chicago/Turabian StylePatro, Epari Ritesh, Teegala Srinivasa Kishore, and Ali Torabi Haghighi. 2022. "Levelized Cost of Electricity Generation by Small Hydropower Projects under Clean Development Mechanism in India" Energies 15, no. 4: 1473. https://doi.org/10.3390/en15041473
APA StylePatro, E. R., Kishore, T. S., & Haghighi, A. T. (2022). Levelized Cost of Electricity Generation by Small Hydropower Projects under Clean Development Mechanism in India. Energies, 15(4), 1473. https://doi.org/10.3390/en15041473








