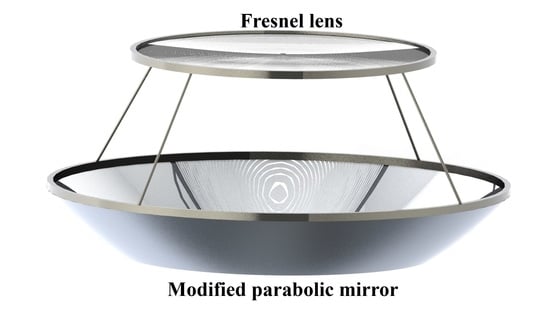
Highly Efficient Solar Laser Pumping Using a Solar Concentrator Combining a Fresnel Lens and Modified Parabolic Mirror
Abstract
:1. Introduction
2. Method
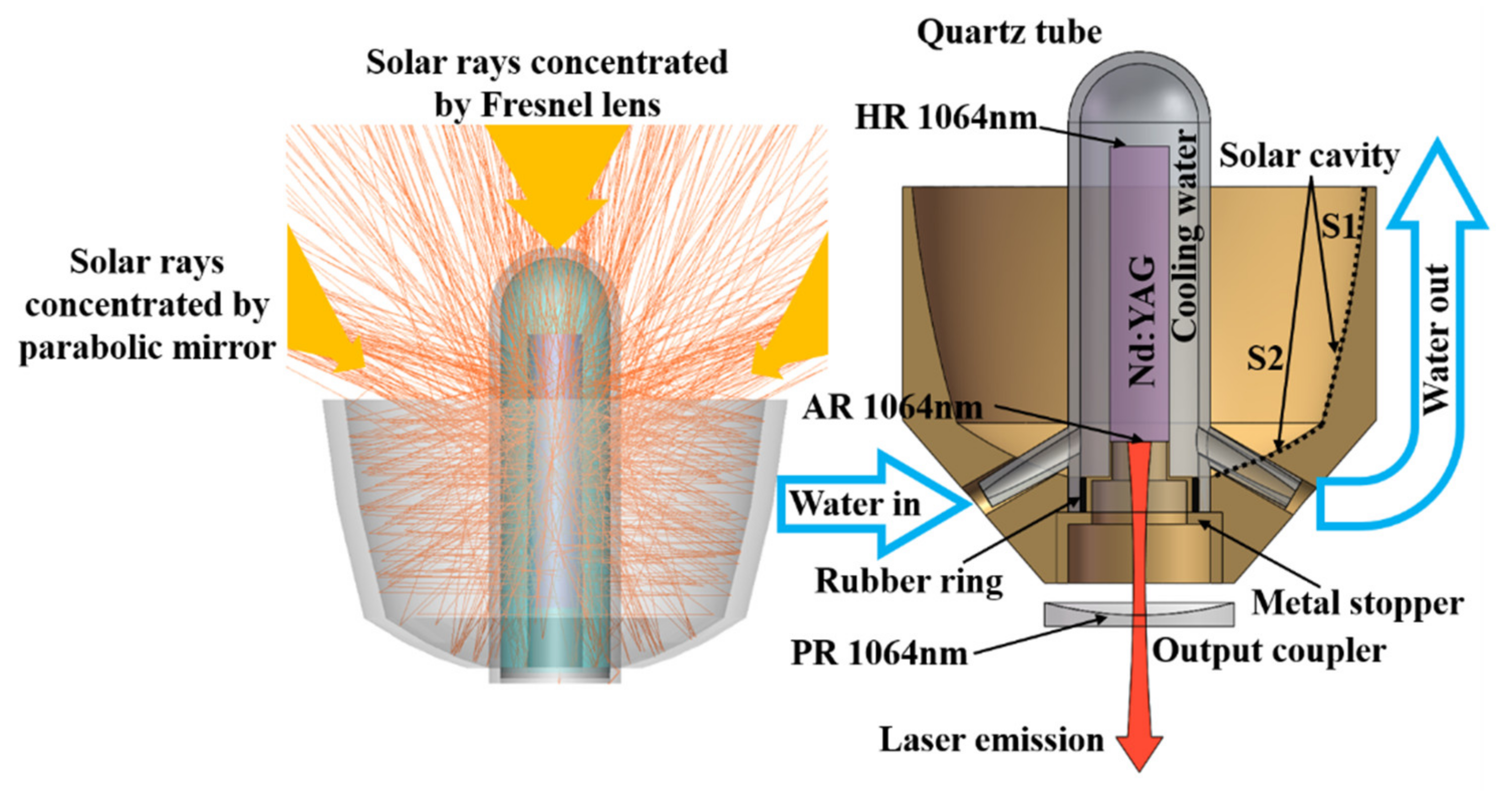
2.1. Solar Energy Collection and Concentrator System
2.2. Solar Laser Head
2.3. Optimization of the Optical Design Parameters Using TracePro
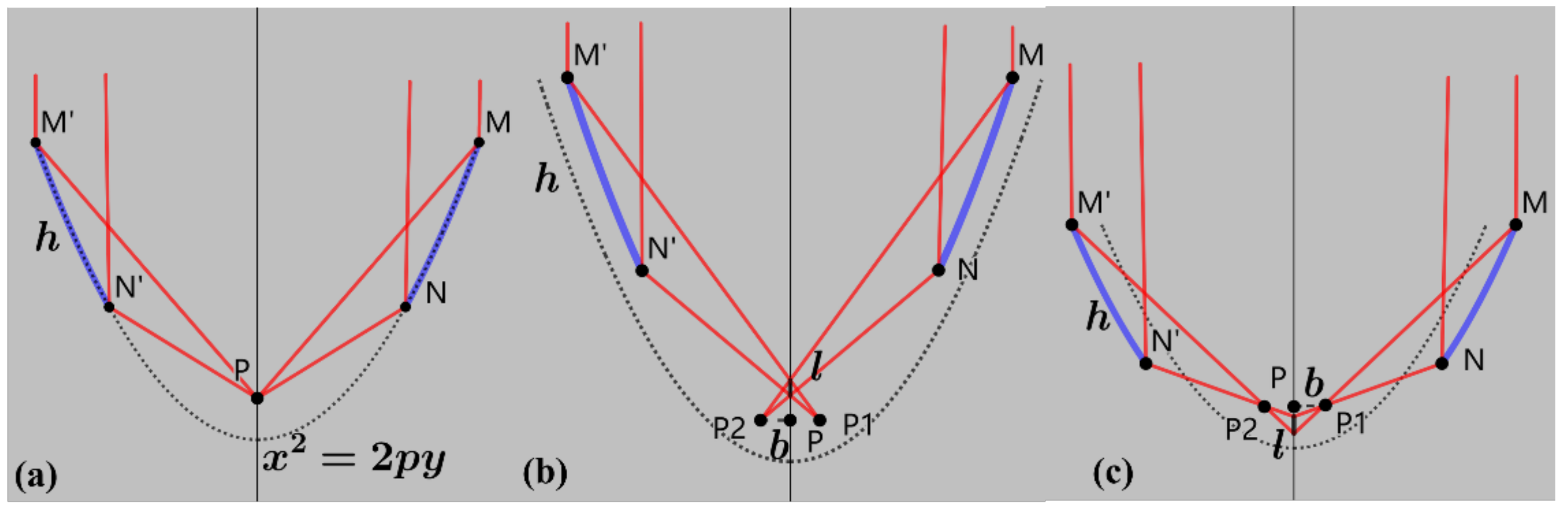
2.3.1. Step 1: Optimization of the Modified Parabolic Mirror
2.3.2. Step 2: Optimization of Parabolic Surface S1 in the Pump Cavity
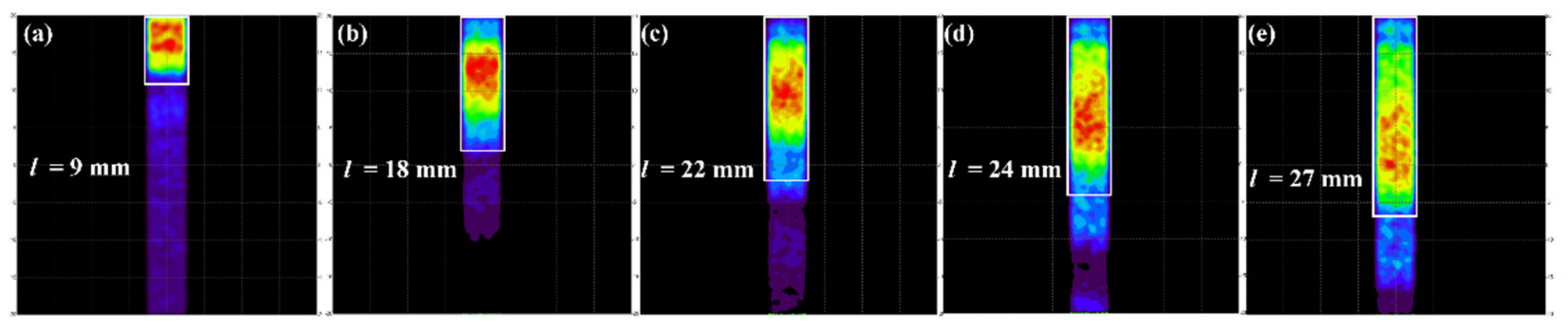
2.3.3. Step 3: Optimization of the Laser Rod Length
2.3.4. Step 4: Optimization of Parabolic Surface S2 in the Pump Cavity
3. Result
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yabe, T.; Uchida, S.; Ikuta, K.; Yoshida, K.; Baasandash, C.; Mohamed, M.S.; Sakurai, Y.; Ogata, Y.; Tuji, M.; Mori, Y.; et al. Demonstrated fossil-fuel-free energy cycle using magnesium and laser. Appl. Phys. Lett. 2006, 89, 261107. [Google Scholar] [CrossRef]
- Ohkubo, T.; Yabe, T.; Yoshida, K.; Uchida, S.; Funatsu, T.; Bagheri, B.; Oishi, T.; Daito, K.; Ishioka, M.; Nakayama, Y.; et al. Solar-pumped 80 W laser irradiated by a Fresnel lens. Opt. Lett. 2009, 34, 175–177. [Google Scholar] [CrossRef]
- Yabe, T.; Bagheri, B.; Ohkubo, T.; Uchida, S.; Yoshida, K.; Funatsu, T.; Oishi, T.; Daito, K.; Ishioka, M.; Yasunaga, N.; et al. 100 W-class solar pumped laser for sustainable magnesium-hydrogen energy cycle. J. Appl. Phys. 2008, 104, 083104. [Google Scholar] [CrossRef]
- Mori, M.; Kagawa, H.; Saito, Y. Summary of studies on space solar power systems of Japan Aerospace Exploration Agency (JAXA). Acta Astronaut. 2006, 59, 132–138. [Google Scholar] [CrossRef]
- Guan, Z.; Zhao, C.M.; Yang, S.H.; Wang, Y.; Ke, J.Y.; Zhang, H.Y. Demonstration of a free-space optical communication system using a solar-pumped laser as signal transmitter. Laser Phys. Lett. 2017, 14, 055804. [Google Scholar] [CrossRef]
- Takeda, Y.; Iizuka, H.; Mizuno, S.; Hasegawa, K.; Ichikawa, T.; Ito, H.; Kajino, T.; Ichiki, A.; Motohiro, T. Silicon photovoltaic cells coupled with solar-pumped fiber lasers emitting at 1064 nm. J. Appl. Phys. 2014, 116, 014501. [Google Scholar] [CrossRef]
- Young, C.G. A Sun-Pumped cw One-Watt Laser. Appl. Opt. 1966, 5, 993–997. [Google Scholar] [CrossRef]
- Arashi, H.; Oka, Y.; Sasahara, N.; Kaimai, A.; Ishigame, M. A Solar-Pumped cw 18 W Nd:YAG Laser. Jpn. J. Appl. Phys. 1984, 23, 1051–1053. [Google Scholar] [CrossRef]
- Weksler, M.; Shwartz, J. Solar-pumped solid-state lasers. IEEE J. Quantum Electron. 1988, 24, 1222–1228. [Google Scholar] [CrossRef]
- Benmair, R.M.J.; Kagan, J.; Kalisky, Y.; Noter, Y.; Oron, M.; Shimony, Y.; Yogev, A. Solar-pumped Er, Tm, Ho:YAG laser. Opt. Lett. 1990, 15, 36–38. [Google Scholar] [CrossRef]
- Lando, M.; Kagan, J.; Linyekin, B.; Dobrusin, V. A solar-pumped Nd:YAG laser in the high collection efficiency regime. Opt. Commun. 2003, 222, 371–381. [Google Scholar] [CrossRef]
- Yabe, T.; Ohkubo, T.; Uchida, S.; Yoshida, M.; Nakatsuka, M.; Funatsu, T.; Mabuti, A.; Oyama, A.; Nakagawa, K.; Oishi, T.; et al. High-efficiency and economical solar-energy-pumped laser with Fresnel lens and chromium codoped laser medium. Appl. Phys. Lett. 2007, 90, 261120. [Google Scholar] [CrossRef]
- Liang, D.; Almeida, J. Highly efficient solar-pumped Nd:YAG laser. Opt. Express 2011, 19, 26399–26405. [Google Scholar] [CrossRef] [PubMed]
- Dinh, T.H.; Ohkubo, T.; Yabe, T.; Kuboyama, H. 120 watt continuous wave solar-pumped laser with a liquid light-guide lens and an Nd:YAG rod. Opt. Lett. 2012, 37, 2670–2672. [Google Scholar] [CrossRef] [PubMed]
- Guan, Z.; Zhao, C.; Li, J.; He, D.; Zhang, H. 32.1 W/m2 continuous wave solar-pumped laser with a bonding Nd:YAG/YAG rod and a Fresnel lens. Opt. Laser Technol. 2018, 107, 158–161. [Google Scholar] [CrossRef]
- Liang, D.; Vistas, C.R.; Tibúrcio, B.D.; Almeida, J. Solar-pumped Cr:Nd:YAG ceramic laser with 6.7% slope efficiency. Sol. Energy Mater. Sol. Cells 2018, 185, 75–79. [Google Scholar] [CrossRef]
- Vistas, C.R.; Liang, D.; Almeida, J.; Tibúrcio, B.D.; Garcia, D.; Catela, M.; Costa, H.; Guillot, E. Ce:Nd:YAG side-pumped solar laser. J. Photonics Energy 2021, 11, 018001. [Google Scholar] [CrossRef]
- Tibúrcio, B.; Liang, D.; Almeida, J.; Garcia, D.; Vistas, C.R.; Morais, P. Highly efficient side-pumped solar laser with enhanced tracking-error compensation capacity. Opt. Commun. 2020, 460, 125156. [Google Scholar] [CrossRef]
- Liang, D.; Almeida, J.; Vistas, C.R.; Guillot, E. Solar-pumped TEM00 mode Nd:YAG laser by a heliostat—Parabolic mirror system. Sol. Energy Mater. Sol. Cells 2015, 134, 305–308. [Google Scholar] [CrossRef]
- Mehellou, S.; Liang, D.; Almeida, J.; Bouadjemine, R.; Vistas, C.R.; Guillot, E.; Rehouma, F. Stable solar-pumped TEM00-mode 1064 nm laser emission by a monolithic fused silica twisted light guide. Sol. Energy 2017, 155, 1059–1071. [Google Scholar] [CrossRef]
- Xiong, S.; He, Y.; Liu, X. Parabolic ring array concentrator for solar-pumped laser. J. Appl. Opt. 2014, 35, 531–536. [Google Scholar]
- Matos, R.; Liang, D.; Almeida, J.; Tibúrcio, B.D.; Vistas, C.R. High-efficiency solar laser pumping by a modified ring-array concentrator. Opt. Commun. 2018, 420, 6–13. [Google Scholar] [CrossRef]
- Boutaka, R.; Liang, D.; Bouadjemine, R.; Traiche, M.; Kellou, A. A Compact Solar Laser Side-Pumping Scheme Using Four Off-Axis Parabolic Mirrors. J. Russ. Laser Res. 2021, 42, 453–461. [Google Scholar] [CrossRef]
- Catela, M.; Liang, D.; Vistas, C.R.; Garcia, D.; Tibúrcio, B.D.; Costa, H.; Almeida, J. Doughnut-Shaped and Top Hat Solar Laser Beams Numerical Analysis. Energies 2021, 14, 7102. [Google Scholar] [CrossRef]
- Tibúrcio, B.D.; Liang, D.; Almeida, J.; Matos, R.; Vistas, C.R. Improving solar-pumped laser efficiency by a ring-array concentrator. J. Photonics Energy 2018, 8, 018002. [Google Scholar] [CrossRef]
- Koechner, W. Solid-State Laser Engineering, 6th ed.; Springer: New York, NY, USA, 2006. [Google Scholar]
- ASTM G173-03(2012). Standard Tables for Reference Solar Spectral Irradiances: Direct Normal and Hemispherical on 37° Tilted Surface; ASTM: West Conshohocken, PA, USA, 2012. [Google Scholar]
- Advanced Software for Laser Design (ASLD). Multiphysics Laser Simulation Software. Available online: http://www.asldweb.com/index.html (accessed on 4 February 2022).
- Zhao, B.; Zhao, C.; He, J.; Yang, S. The Study of Active Medium for Solar-Pumped Solid-State Lasers. Acta Opt. Sin. 2007, 27, 1797–1801. [Google Scholar]







| System No. | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| Focal length p/2 (mm) | 200 | 200 | 200 | 200 | 200 |
| Deviation distance b (mm) | 0 | 20 | 30 | 40 | 50 |
| Distance above the laser head (mm) | 109.5 | 89 | 78 | 68 | 58 |
| Absorbed solar power (W) | 177.2 | 157.1 | 157.2 | 157.2 | 157.2 |
| System No. | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| Distance behind the laser head (mm) | 4.5 | 5 | 6 | 7 | 10 |
| Diameter of input window (mm) | 21.8 | 35.5 | 39.4 | 51.2 | 56.6 |
| Focal length of the S1 parabola m/2 (mm) | 11 | 38 | 50 | 66 | 79 |
| Depth (mm) | 7 | 15 | 18 | 26 | 30 |
| Absorbed solar power (W) | 241.2 | 229.8 | 226.0 | 222.2 | 219.7 |
| System No. | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| Laser rod length (mm) | 9 | 18 | 22 | 24 | 27 |
| Absorbed solar power (W) | 187.2 | 202.5 | 202.5 | 200.9 | 199.0 |
| Reduction in resonator losses (%) | 77.5 | 55 | 45 | 40 | 32.5 |
| Reduction in absorbed solar power (%) | 22.4 | 11.9 | 10.4 | 9.6 | 9.4 |
| System No. | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| Focal length of S2 parabola n/2 (mm) | 6 | 16 | 22 | 32 | 42 |
| Absorbed solar power (W) | 210.6 | 228.7 | 232.3 | 230.7 | 233.3 |
| System No. | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| Output mirror reflectivity | 0.95 | 0.94 | 0.93 | 0.93 | 0.93 |
| Laser output power (W) | 70.2 | 73.5 | 74.6 | 68.4 | 71.5 |
| Laser collection efficiency (W/m2) | 39.8 | 41.6 | 42.2 | 38.7 | 40.5 |
| Mx2 | 51.8 | 35.7 | 34.0 | 37.1 | 36.3 |
| My2 | 53.3 | 36.9 | 35.1 | 39.2 | 37.1 |
| Conversion efficiency (%) | 4.06 | 4.25 | 4.31 | 3.95 | 4.13 |
| Parameters | Ref. [22] | Ref. [25] | Present Work | Fold Change with Respect to | |
|---|---|---|---|---|---|
| Ref. [22] | Ref. [25] | ||||
| Collection area (m2) | 1.76 | 1.71 | 1.76 | - | - |
| Pumping configuration | end-side-pumping | side-pumping | end-side-pumping | - | - |
| Laser output (W) | 67.8 | 49.89 | 74.6 | - | - |
| Collection efficiency (W/m2) | 38.4 | 29.18 | 42.2 | 1.1 | 1.45 |
| Conversion efficiency (%) | 4.0 | 3.07 | 4.31 | 1.08 | 1.40 |
| Average M2 factor | 55.0 | 33.0 | 34.6 | - | - |
| Figure of merit (W) | 0.024 | 0.046 | 0.063 | 2.625 | 1.370 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cai, Z.; Zhao, C.; Zhao, Z.; Yao, X.; Zhang, H.; Zhang, Z. Highly Efficient Solar Laser Pumping Using a Solar Concentrator Combining a Fresnel Lens and Modified Parabolic Mirror. Energies 2022, 15, 1792. https://doi.org/10.3390/en15051792
Cai Z, Zhao C, Zhao Z, Yao X, Zhang H, Zhang Z. Highly Efficient Solar Laser Pumping Using a Solar Concentrator Combining a Fresnel Lens and Modified Parabolic Mirror. Energies. 2022; 15(5):1792. https://doi.org/10.3390/en15051792
Chicago/Turabian StyleCai, Zitao, Changming Zhao, Ziyin Zhao, Xingyu Yao, Haiyang Zhang, and Zilong Zhang. 2022. "Highly Efficient Solar Laser Pumping Using a Solar Concentrator Combining a Fresnel Lens and Modified Parabolic Mirror" Energies 15, no. 5: 1792. https://doi.org/10.3390/en15051792
APA StyleCai, Z., Zhao, C., Zhao, Z., Yao, X., Zhang, H., & Zhang, Z. (2022). Highly Efficient Solar Laser Pumping Using a Solar Concentrator Combining a Fresnel Lens and Modified Parabolic Mirror. Energies, 15(5), 1792. https://doi.org/10.3390/en15051792