Along-Wind Aerodynamic Damping of Wind Turbine Towers: Determination by Wind Tunnel Tests and Impact on Tower Lifetime

Abstract

As wind turbines become larger and their towers more slender, aeroelastic effects play a bigger role in the wind turbine’s dynamic behavior. This study focuses on the along-wind aerodynamic damping of wind turbine towers, which has been determined by wind tunnel experiments using the forced oscillation method according to Steckley’s approach. Reynolds number scale effects have been considered through surface roughness modifications using sand paper and a dimple pattern, which have been described in detail. The wind tunnel measurements are performed in sub-critical, critical and trans-critical flow regimes, as well as in low- and high-turbulence conditions, which allows for an accurate description of the required relative roughness and Reynolds numbers for achieving trans-critical conditions. The resulting along-wind aerodynamic damping values according to Steckley’s and Holmes’ approaches are compared, and an analytical relation between them is established. Both approaches are then used in aeroelastic multi-body-simulations of an onshore 6 MW reference wind turbine and their impact on the wind turbine lifetime is evaluated through fatigue proofs at the tower base section. Holmes’ approach seems more appropriate for the application in aeroelastic multi-body simulations. A lifetime extension for the wind turbine tower of approximately 0.4% is achieved for the reference wind turbine tower, which roughly corresponds to 1 to 2 months for 20 years of operation. An analytical expression is given for the estimation of the tower’s aerodynamic damping in parked and operating conditions.

1. Introduction

Wind energy is still one of the main contributors to the global transition to renewable energies. With a wind energy installation of approximately 93 GW in 2020, wind energy represents around 6% of the global power mix [1]. To achieve the objective of remaining below a 2 °C global temperature increase, the percentage of wind energy should be increased to over 30% by 2050 [1]. This requires an increase in the annually wind energy installation and ideally cheaper and more efficient wind turbines. This has been classically achieved through higher wind turbines with larger rotors.

In the last few years, the number of studies about the aerodynamic damping of wind turbines has increased. These studies mainly focus on the aerodynamic damping of the wind turbine rotor oscillating in wind direction due to the first bending mode shape of the wind turbine tower, which can reach damping rations between 5% and 15% depending on rotor dimension and other parameters [2,3,4,5]. Several studies have presented techniques to identify the aerodynamic damping from monitoring data of full-scale turbines [6,7,8,9], and other studies present semi-analytical expressions after the consideration of certain assumptions [3,10,11,12]. While the aerodynamic damping of the rotor is already considered in state-of-the art aeroelastic software for wind turbines [3,4,11], the mentioned studies aim at its determination from real wind turbines or to its characterization and identification of its most relevant parameters.

Less attention has been paid to the aerodynamic damping of the support structure, classically a tube steel tower with a circular cross-section. The main reason for this is the relatively small value of the tower’s aerodynamic damping in comparison to the rotor’s. For instance, measurements by Devriendt [13] on a parked offshore Vestas Wind turbine V90 with 3 MW rated power showed a total damping ratio in the fore-aft and side-to-side directions of around 1.9% and 2.5%, respectively, the side-to-side damping being higher due to the parked blades having a pitch angle close to 90${}^{\circ }$. Koukoura [14] determined a mean standstill damping ratio of 1.93% for an offshore Siemens 3.6 MW wind turbine, and Damgaard [15] found a cross-wind total damping ratio of approximately 2.4%. Rezaei [16] mentions good agreement in the literature for parked conditions, the fore-aft and side-to-side aerodynamic damping being 1% and 1.5% of critical, respectively. In the BBTI-Project [17], the along-wind aerodynamic damping of towers was determined through wind tunnel experiments without consideration of Reynolds number effects, and its impact in the wind turbine dynamics was studied by Werkmeister [18] through Multi-Body Simulations, obtaining around 10% lower damage-equivalent base moments in the simulations where the along-wind aerodynamic damping was modeled. A summary of other study cases can be found in [19].

Larger turbine dimensions and soft-soft designs may increase the relevance of the tower aerodynamic damping in the system overall dynamics. As shown by Rezaei [16], a damping ratio increase of around 0.5% can already lead to a turbine lifetime extension of around 2.5 years. In this context, the consideration of the tower’s aerodynamic damping may play a more significant role than it has in the past.

This paper aims at determining the along-wind aerodynamic damping of wind turbine towers through wind tunnel measurements under the consideration of Reynolds number effects and quantifying its impact on the wind turbine’s lifetime. To ensure the necessary level of aerodynamic scaling quality, a thorough study of flow similarity for circular cross-sections has been carried out. In particular, methods to reduce the Reynolds number effects on the wind tunnel are studied in Section 2, which leads to the choice of models and surface roughness for the aerodynamic damping measurements. The presented relation between critical Reynolds number $R{e}_{crit}$ and different surface roughness conditions provides guidance for future experimental investigations, as well. In Section 3, the used aerodynamic damping approaches are presented, as well as the results of the wind tunnel measurements. Conceptually, the theoretical approaches from Steckley [20] and Holmes [21] are considered in this study. Both differ with respect to their assumption of generalizing the acting aerodynamic damping forces but have not been compared in previous research. The presented comparison with each other and with experimental data enables a more realistic wind turbine modeling.

Finally, in Section 4, the obtained results are applied in aeroelastic multi-body simulations of a 6 MW reference wind turbine to study their impact on the wind turbine’s lifetime.

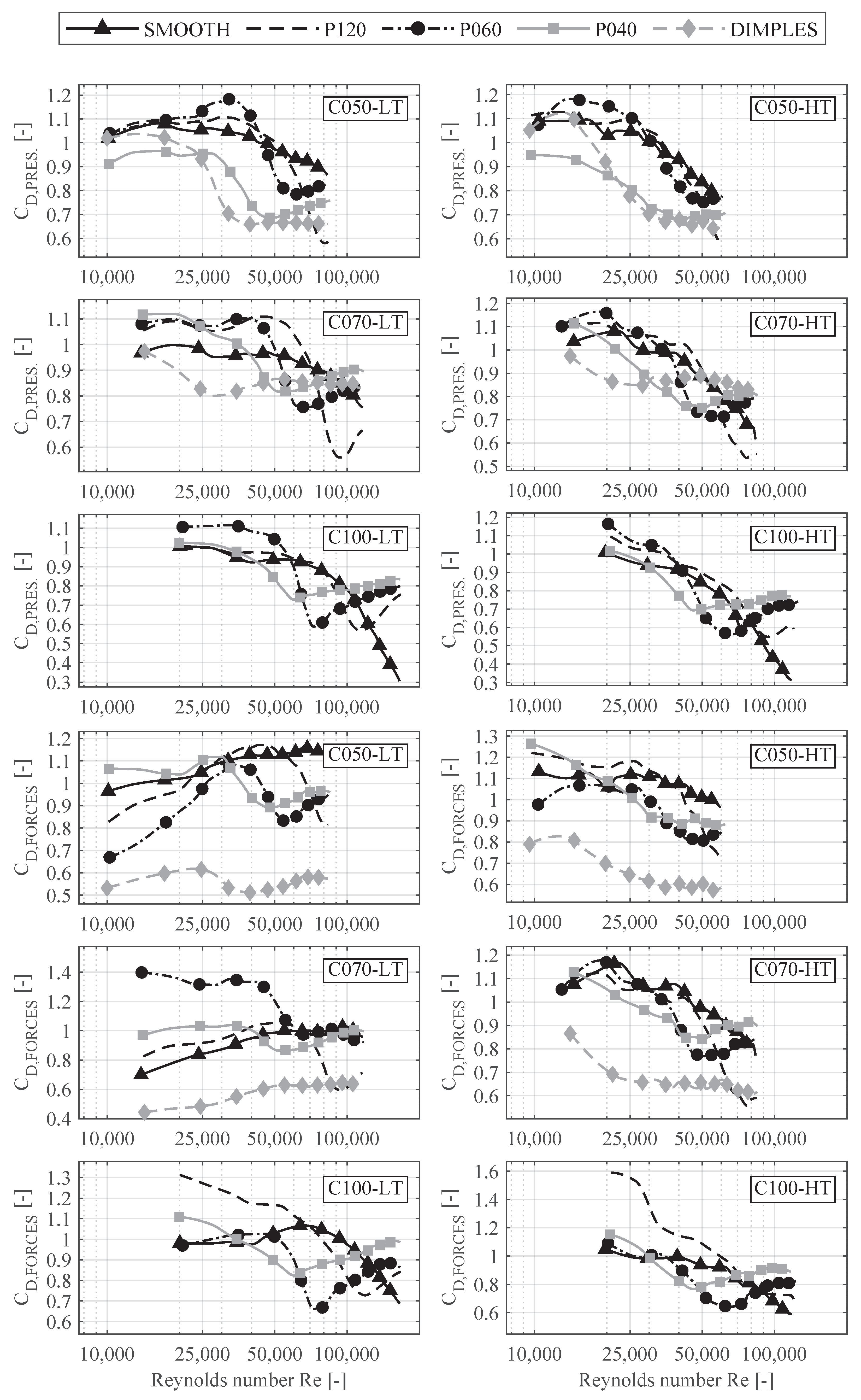
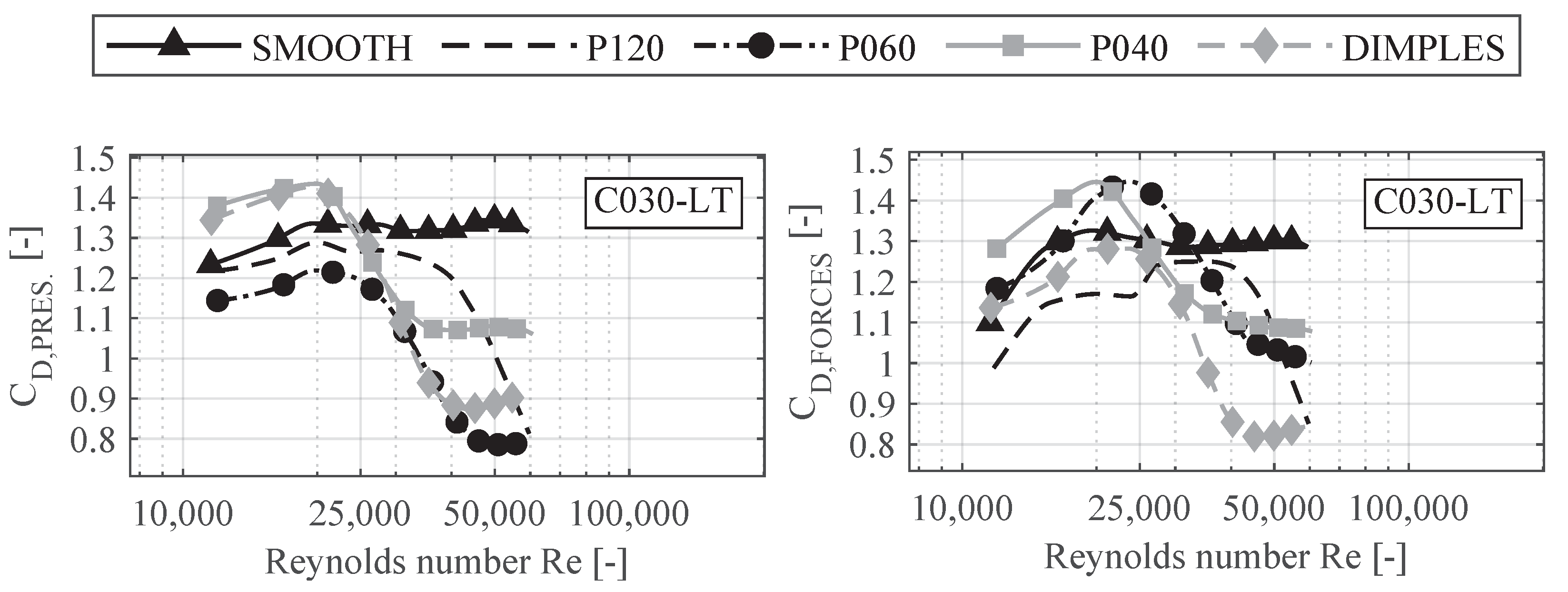
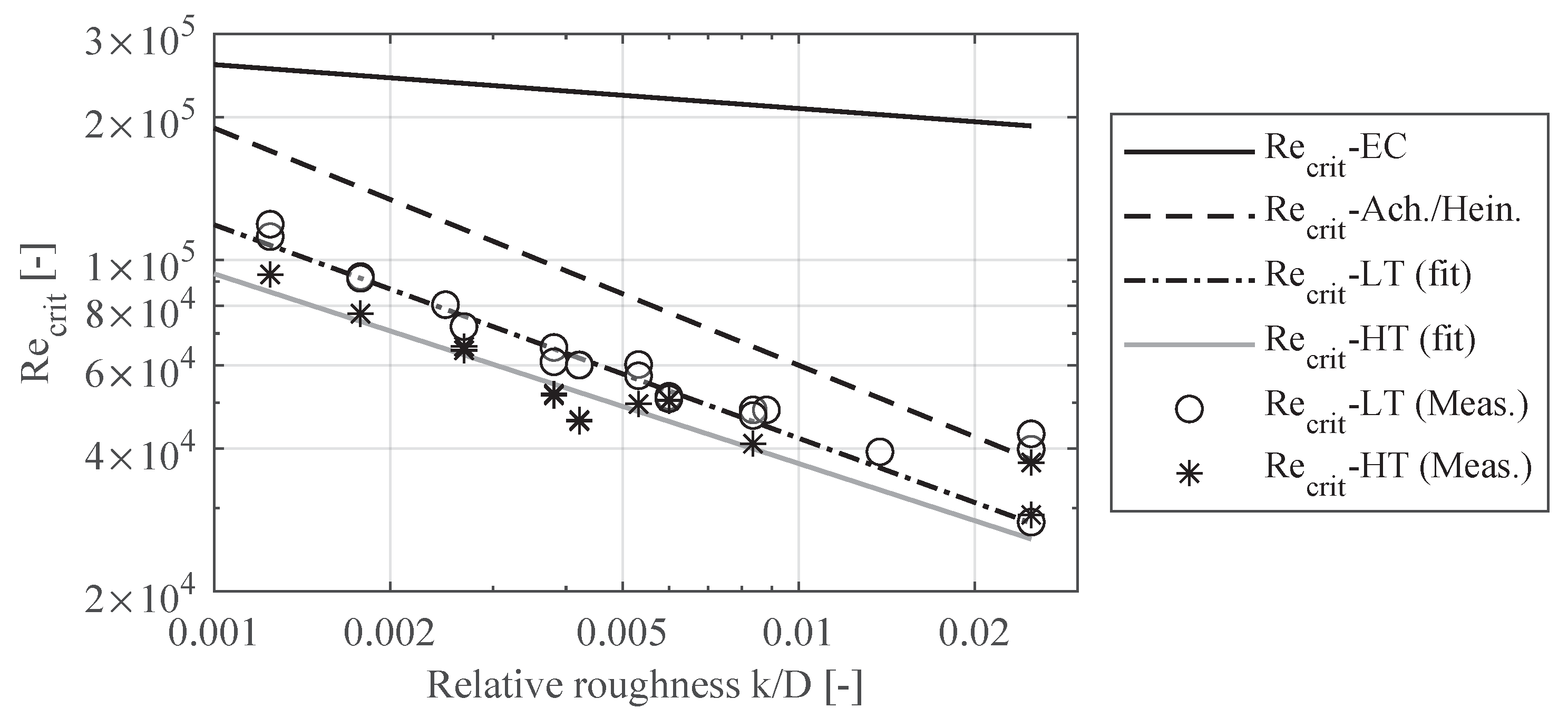
2. Aerodynamic Drag of Wind Turbine Tower Models in Wind Tunnel Experiments: Effects of Surface Roughness and Turbulence Modification

When performing small-scale wind tunnel experiments, similarity rules must be considered in order to faithfully represent the studied aerodynamic phenomena. In the case of wind turbine towers with circular cross-sections, the flow characteristics are highly dependent on the Reynolds number [22,23], which depends on the fluid viscosity $\nu$, the undisturbed flow speed v and a characteristic cross-section length D:

$$Re=\frac{vD}{\nu }$$

(1)

Wind tunnel models are typically hundreds of times smaller than their full-scale counterpart, which makes compensating the diameter reduction with a wind speed increase impracticable. As a result, the Reynolds number in wind tunnel tests does not match that in the full-scale prototype. However, the resulting scale-effects can be reduced to some extent by an increase in the model’s surface roughness [24,25,26,27,28,29] or through the modification of the experiment flow, either increasing flow turbulence [30] or using pressurized wind tunnels [31]. Many of these studies are summarized elsewhere [32,33,34,35,36].

In this study, both surface roughness and flow modification were used to mitigate scale-effects. To this end, cylinder models with circular cross-sections were placed in the wind tunnel horizontally with end plates to reduce tip effects. The diameters of the cylinders were between 30 mm and 100 mm and the lengths around 750 mm. The surface roughness was achieved through sandpapers P040, P060 and P120 (according to FEPA P notation) and a milled dimpled pattern.

The dimpled pattern is roughly based on the patterns shown by Bearman [26] and Hojo [28] and is described in detail for its potential use in Reynolds scale effects reduction in wind tunnel measurements. The dimpled pattern was defined through milling dimple spheres of diameter $D_{DIMPLE}$ equal to one-eighth of the model diameter, a depth of 0.025 times the corresponding model diameter, which results in a dimple width $D_{CUT}$ equal to $10\%$ of the model diameter. The deepest points of the dimples were separated from each other by a distance $A_{DIMPLE}$ equal to the dimple sphere diameter $D_{DIMLPE}$. The dimple pattern geometry is presented schematically in Figure 1.

The wind tunnel measurements were performed at wind speeds up to 25 to 30 m/s under low-turbulence (turbulence intensity $I_u=4\%$, noted as LT) and turbulent (turbulence intensity $I_u=11.5\%$, noted as HT) flow conditions. Support forces were measured at both model extremes, and 12 pressure taps were located chord-wise at the center of the model to measure the pressure distribution at the cross-section (for more details see [37]). Because the experiments occur under laboratory conditions, the main uncertainty source is attributed to the precision of the sensors, which is documented in the manufacturer data sheets. Applying these to the average measurement values, precision values of around ±2.5% for the measured wind speed (5.1% for pressure values) and ±4% for the measured forces and ±2.5% for the pressure sensors are estimated, which results in maximum errors of around ±9.5% (multiplication of wind speed error squared and force error) in force measurements and ±7.5% in pressure measurements (multiplication of wind speed error squared and pressure error). The measurements had a duration of 30 s each, which ensured a convergence of the average value within 0.1% of the end value. A schematic representation of the measurements is shown in Figure 2, while the measured models are summarized in

Table 1. Surface roughness of used cylinders, relative roughness $\kappa =\frac{k}{D}\left[{10}^{-3}\right]$ of models and performed measurements.

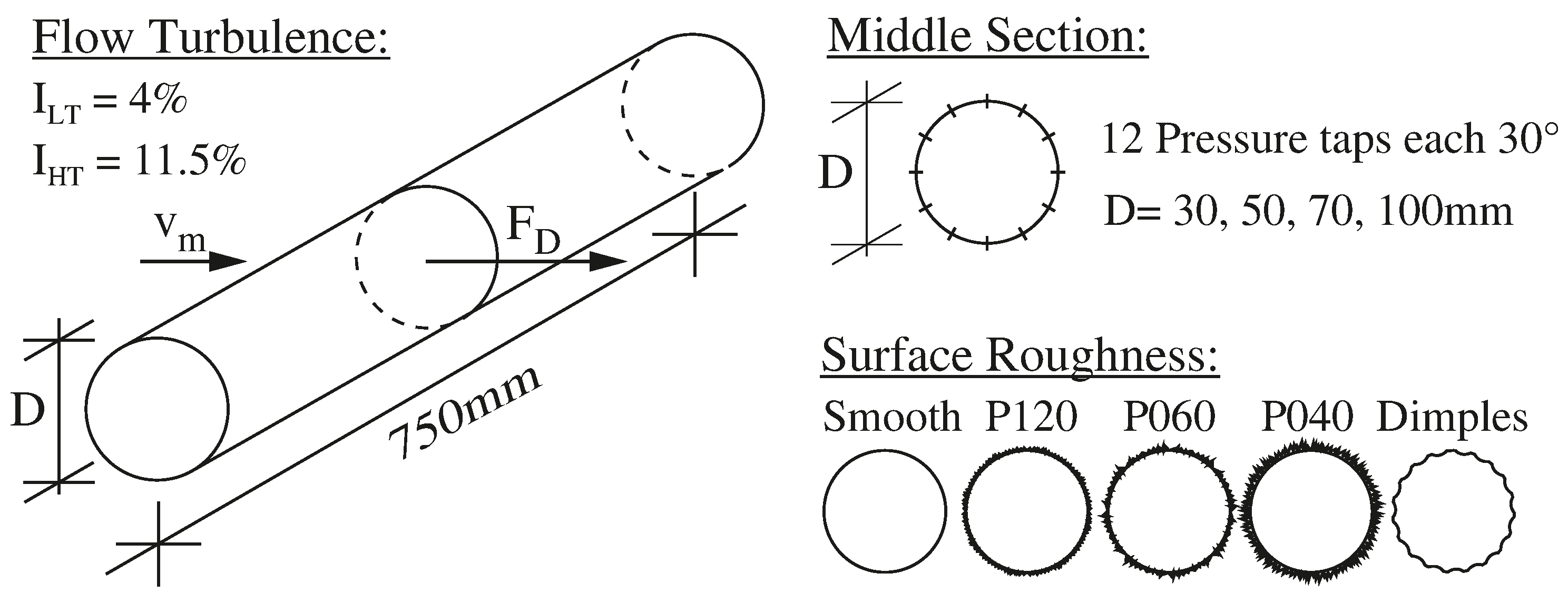
Roughness	Smooth		P040		P060		P120		Dimple
Equiv.k [$\mathsf{\mu }$m]	2		425		269		100		0.025D
D [mm]	LT	HT	LT	HT	LT	HT	LT	HT	LT	HT
30	0.07	-	14.17	-	8.97	-	3.33	-	25.00	-
50	0.04	0.04	8.50	8.50	5.38	5.38	2.00	2.00	25.00	25.00
70	0.03	0.03	6.07	6.07	3.84	3.84	1.43	1.43	25.00	25.00
100	0.02	0.02	4.25	4.25	2.69	2.69	1.00	1.00	-	-

.

For the determination of the drag coefficient, the following equation was used:

$$C_D=\frac{F_D}{0.5{\rho }_a{v}_m^{2}DL}$$

(2)

where $F_D$ is the average aerodynamic force projected in wind direction, ${\rho }_a$ is the air density, $v_m$ is the average wind speed, D is the model diameter and L is the model length, which in all cases was 750 mm. Because the relative surface $\kappa$ is not considered explicitly in the drag coefficient equation, each drag coefficient curve must be given with its correspondent equivalent roughness k or relative roughness $\kappa$. The measurement results vary depending on flow turbulence and measuring method, i.e., depending on whether the drag coefficients were determined through cross-section pressures or support reaction forces. In general, the results agree with some trends observed in the literature [25,36]. Consequently, larger surface roughness results in an increase in the drag coefficient in all flow regimes and in a reduction in the critical Reynolds number $R{e}_{crit}$, which is described as the Reynolds number corresponding to the minimum drag coefficient. The expected effect of turbulence varies in the literature. In this study, the effect of higher flow turbulence can be summarized through an anticipation of the critical transcritical flow regime but no alteration of the drag coefficient magnitude. The obtained drag coefficient curves are shown in Figure 3 and Figure 4.

The value of $R{e}_{crit}$ is specially important to determine the possibility of performing wind tunnel tests in the trans-critical flow regime, which is the flow regime found in full-scale wind turbine towers. To this end, the critical Reynolds number $R{e}_{crit}$ in all measurements was related to the relative roughness $\kappa =\frac{k}{D}$ for both analysis methods (cross-section pressures and model support reactions). The results were averaged due to their similarity and are thus expressed only as a function of flow turbulence. The curves for $R{e}_{crit}$ as a function of the relative roughness $\kappa$ for low- and high-turbulence conditions and the values from which they are derived are compared to equivalent curves given in Figure 7.28 of the Eurocode 1 [38] and by Achenbach and Heinecke [25]. Both Eurocode and Achenbach assume laminar flow conditions. Achenbach uses a turbulence intensity of $I_u=0.45\%$. All curves are shown in Figure 5.

The lines in Figure 5 can be expressed using an equation of the form given in ([25,36]) as follows:

$$R{e}_{crit}=\frac{\beta }{x^{\alpha }}$$

(3)

The coefficients of the curves determined in this study and their counterparts in the literature are given in

Table 2. Coefficients for determining $R{e}_{crit}$ according to Equation (3).

Author or Standard	Exponent $\mathit{\alpha }$	Coefficient $\mathit{\beta }$
Eurocode 1, Figure 7.28 [38]	0.10	13,600
Achenbach and Heinecke ($I_u=0.45\%$) [25]	0.50	6000
Own Measurements (LT, $I_u=4.0\%$)	0.45	5300
Own Measurements (HT, $I_u=11.5\%$)	0.40	5900

.

The measured results agree with the literature. While the Eurocode shows very conservative values (a large $R{e}_{crit}$ implies a larger wind speed range with higher drag coefficients), Achenbach [25] obtained larger $R{e}_{crit}$ values, probably due to the lower turbulence intensity in his experiments. As a result, Figure 5 quantifies how surface roughness and turbulence intensity reduce $R{e}_{crit}$, reducing the necessary wind speeds to achieve trans-critical flow regimes.

3. Aerodynamic Damping of Wind Turbine Towers with Circular Sections

The determination of the aerodynamic damping of wind turbine towers through wind tunnel tests is based on the works of Steckley, Tschanz and Davenport [20,39,40]. The High-Frequency Force Balance (HFFB) approach [40] is extended to include force oscillation measurements [20] and thus to determine the aerodynamic damping of slender tall structures (towers, chimneys, buildings) oscillating in the first natural bending mode. The HFFB approach consists of the use highly stiff and light models with the same shape as the studied prototype structure to define its aerodynamic admittance through a high-sensitivity balance sensor at the base of the model. In the case of structures with a first modal form similar to a linear equation of the form $\Phi \left(z\right)=z/H$, with H being the total structure height, the modal force spectrum equals the wind force spectrum weighted with the structure height, i.e., the moment measured at the model base corresponds to the modal wind force spectrum. The structural dynamics are added analytically after the measurements using random vibration theory [20]. A more detailed explanation and a benchmark case can be found in the literature [39,40,41]. Because the HFFB method cannot reproduce motion-induced oscillations, the approach was further developed by Steckley [20] to include forced oscillation measurements, which can be used to determine aeroelastic phenomena. In this case, the model is forced to pivot around an axis at the model’s base, simulating the first, approximately linear, modal shape of the structure. According to Steckley’s approach, the linearized wind base moment $M_b\left(t\right)$ is composed by a random moment component due to turbulence and unsteady wake effects with dimensionless instantaneous coefficient $C_r\left(t\right)$, and a motion-induced moment component. The wind base moment on a structure with circular cross-section [37] can then be expressed as follows:

$$M_b\left(t\right)=qD{H}^2{C}_r\left(t\right)-\frac{1}{6}{\omega }^2{\rho }_a{H}^2\pi D^2\left(\alpha y+\frac{\beta }{\omega }\dot{y}\right)$$

(4)

where q is the dynamic pressure, D is the structure’s diameter or width, H the structure’s height, $\omega$ is the angular frequency of the first modal form, ${\rho }_a$ is the air density, $\alpha$ and $\beta$ are the aerodynamic stiffness and damping coefficients, respectively, and y and $\dot{y}$ are the structure’s top oscillation amplitude and velocity, respectively. In wind tunnel measurements, the model is forced to oscillate in a sinusoidal manner with constant oscillation amplitude $\widehat{y}$. As a result, Equation (4) becomes:

$$M_b\left(t\right)=qD{H}^2{C}_r\left(t\right)-\frac{1}{6}{\omega }^2{\rho }_a{H}^2\pi D^2\widehat{y}\left[\alpha cos\left(\omega t\right)-\beta sin\left(\omega t\right)\right]$$

(5)

Equation (5) can be used in forced oscillation tests to find the aerodynamic damping and aerodynamic stiffness. First, measurements without wind are performed to determine the mechanical damping and inertial forces of the model and test bench. Afterwards, the measurements are repeated with wind acting on the model. The aerodynamic damping is obtained by subtracting the the measurements with wind to those without wind. The measured inertial and damping aerodynamic forces are identified using the cross power spectral density between measured accelerations and base moments. The measured aerodynamic damping and stiffness base moments are then normalized according to the measurement conditions, and the factors $\beta$ and $\alpha$ are determined. A more detailed explanation of this analysis is found elsewhere [20,42,43]. The application of Steckley’s approach is performed under the assumption that blade passing and tower tip effects due to the turbine components do not affect the general action of the aerodynamic damping.

A more general approach to along-wind aerodynamic damping of structures is given by Holmes [21]. Holmes exposes a derivation for drag forces acting on a tower, which results in the following aerodynamic damping force coefficient per unit height:

$$c\left(z\right)={\rho }_{a}D\left(z\right)v\left(z\right)C_d\left(z\right)$$

(6)

where $v\left(z\right)$ is the height-dependent wind profile, and $C_d\left(z\right)$ is the section-dependent aerodynamic drag coefficient. Analog to Steckley’s approach, it is assumed that the first modal form $\varphi \left(z\right)$ dominates the oscillation behavior, and the generalized aerodynamic damping coefficient for the first mode is calculated, yielding:

$$C_{AD}={\rho }_a{\int }_0^{H}D\left(z\right)v\left(z\right)C_d\left(z\right)\varphi \left(z\right)dz$$

(7)

Holmes’ approach expresses the aerodynamic damping as a function of the section’s aerodynamic drag coefficient. As a result, the expected aerodynamic damping forces can be calculated using the drag coefficients determined in Section 2. This is performed by applying Equation (7) to the particular conditions given in the wind tunnel. In this context, a constant diameter D, a constant drag coefficient $C_d$ and a uniform wind speed along the model height are assumed. Due to the pivoting oscillations, the first modal form can be expressed as $\varphi \left(z\right)=z/H$. This yields the following expression:

$$C_{AD}=\frac{{\rho }_a}{3}Dv{C}_{d}H$$

(8)

expressing the wind speed as a function of the reduced velocity $u_{red}=v/\left(f_{n}D\right)$ (with $f_n$ the first natural frequency). Replacing the excitation frequency for the angular frequency, it yields:

$$C_{AD}=\frac{{\rho }_a}{6\pi }{D}^2\omega H{C}_d{u}_{red}.$$

(9)

Equation (9) allows a comparison with Equation (4) of Steckley’s approach and will be used later to compare the measured aerodynamic damping with their expected values based on their drag coefficients.

3.1. Aerodynamic Damping Measurements Using the Forced Oscillations Method

Using the results of Section 2, 3 model diameters (50 mm, 70 mm and 100 mm) with 3 different surface roughnesses (Smooth surface, Sandpaper P040 and dimpled pattern) are chosen. The models were placed vertically on a six-axis force balance, and an end plate was located approximately one millimeter above the upper model extreme to minimize tip-effects. Additionally, the models were closed at their upper end. Figure 6 summarizes the main experiment parameters.

The cylinders’ drag coefficients were measured again using this experimental setup to study the correlation between aerodynamic damping and drag coefficient values and to check the previously measured drag coefficients. The drag coefficient curves are shown in Figure 7. The $R{e}_{crit}$ values agree with the curves shown in Figure 5, and the drag coefficients agree in general with previous values and values given in literature and standards for the corresponding surface roughness (for example, see [38]). Only the values for the dimpled cylinders are considerably larger than those given in Figure 3. This may be due to the finishing of the dimples in the aluminum cylinders being too rough and sharp, while the previously used PMA cylinders had a smoother finish. This again illustrates the sensibility of circular cross-sections against surface roughness modifications.

In the aerodynamic damping measurements, the sweep method as described by Steckley [20] has been used, i.e., the wind tunnel wind speed is fixed and the forced oscillation frequencies is varied (i.e. “sweeping” over a range of frequencies). This allows for performing all measurements with a constant Reynolds number and therefore constant flow regime. Using the previously defined expressions in Table 2 for the critical Reynolds number $R{e}_{crit}$, the parameters for the aerodynamic damping measurements are determined as shown in

Table 3. Models and conditions for the wind tunnel measurements of the aerodynamic damping.

Model Name	D[mm]	Roughn.	$\mathit{\kappa }\left[{10}^{-3}\right]$	${\mathit{v}}_{\mathit{m}}$ [m/s]	$\mathbf{Re}$	${\mathit{C}}_{\mathit{D}}$	Regime
CMA-050-SMO	50	Smooth	0.04	8.02	2.67$·{10}^4$	1.05	SC
CMA-050-P040	50	P040	8.50	7.81	2.60$·{10}^4$	1.02	SC
CMA-050-P040	50	P040	8.50	12.90	4.30$·{10}^4$	0.80	CC
CMA-050-DIMP	50	Dimple	25.00	7.90	2.63$·{10}^4$	0.70	CC
CMA-050-DIMP	50	Dimple	25.00	12.96	4.32$·{10}^4$	0.60	TC
CMA-070-SMO	70	Smooth	0.03	7.90	3.69$·{10}^4$	0.94	SC
CMA-070-SMO	70	Smooth	0.03	12.92	6.03$·{10}^4$	0.96	SC
CMA-070-P040	70	P040	6.07	7.96	3.72$·{10}^4$	1.00	CC
CMA-070-P040	70	P040	6.07	12.96	6.05$·{10}^4$	0.85	TC
CMA-070-DIMP	70	Dimple	25.00	7.95	3.71$·{10}^4$	0.70	TC
CMA-070-DIMP	70	Dimple	25.00	13.06	6.09$·{10}^4$	0.70	TC
CMA-100-SMO	100	Smooth	0.02	9.87	6.58$·{10}^4$	1.00	SC
CMA-100-P040	100	P040	4.25	8.88	5.92$·{10}^4$	0.80	CC
CMA-100-P040	100	P040	4.25	13.98	9.32$·{10}^4$	0.85	TC
CMA-100-DIMP	100	Dimple	25.00	8.89	5.92$·{10}^4$	-	TC
CMA-100-DIMP	100	Dimple	25.00	13.85	9.23$·{10}^4$	-	TC

. The models were measured in sub-critical (SC), critical (CC) and trans-critical (TC) flow conditions using oscillation frequencies between 4 and 11 Hz, yielding reduced speeds in the range of $u_{red}=10-45$ approximately. As performed previously, the uncertainty of the measurements is estimated through the sensor precision. Assuming precisions around ±2.5% for the measured wind speed, ±5.0% for the measured moments and ±1.0% for the accelerometer results in a maximum error of around ±9.0% for the aerodynamic damping determination (multiplication of all errors). Because the evaluation of the results is based on the correlation between two sinusoidal signals, a measurement duration of 90 s was chosen to ensure a minimum of 450 up to 900 oscillations per measurement. This proved to yield a convergence of the results within 5% of the end result.

3.2. Results: Aerodynamic Damping of Wind Turbine Towers

The determined aerodynamic damping coefficients $\beta$ as defined in Equation (4) are shown in Figure 8. The results show some dispersion, but a clear tendency is recognizable: the aerodynamic damping has a damping effect on the tower oscillations, and grows with increasing reduced speed.

The results shown in Figure 8 can be classified depending on their flow regimes in the SC, TC and CC regimes. A linear regression is used to fit lines to each case, which are forced to contain the origin of coordinates. The aerodynamic damping coefficient $\beta$ can then be expressed as a function of the reduced speed using Equation (10):

$$\beta =m_{\beta }·u_{red}$$

(10)

where $m_{\beta }$ is the fitted line slope. The measurements grouped in flow regimes and their fitted lines are shown in Figure 9.

The expression of the fitted lines for the aerodynamic damping and their goodness of fit $R^2$ are given in

Table 4. Equation parameters for the aerodynamic damping of wind turbine towers.

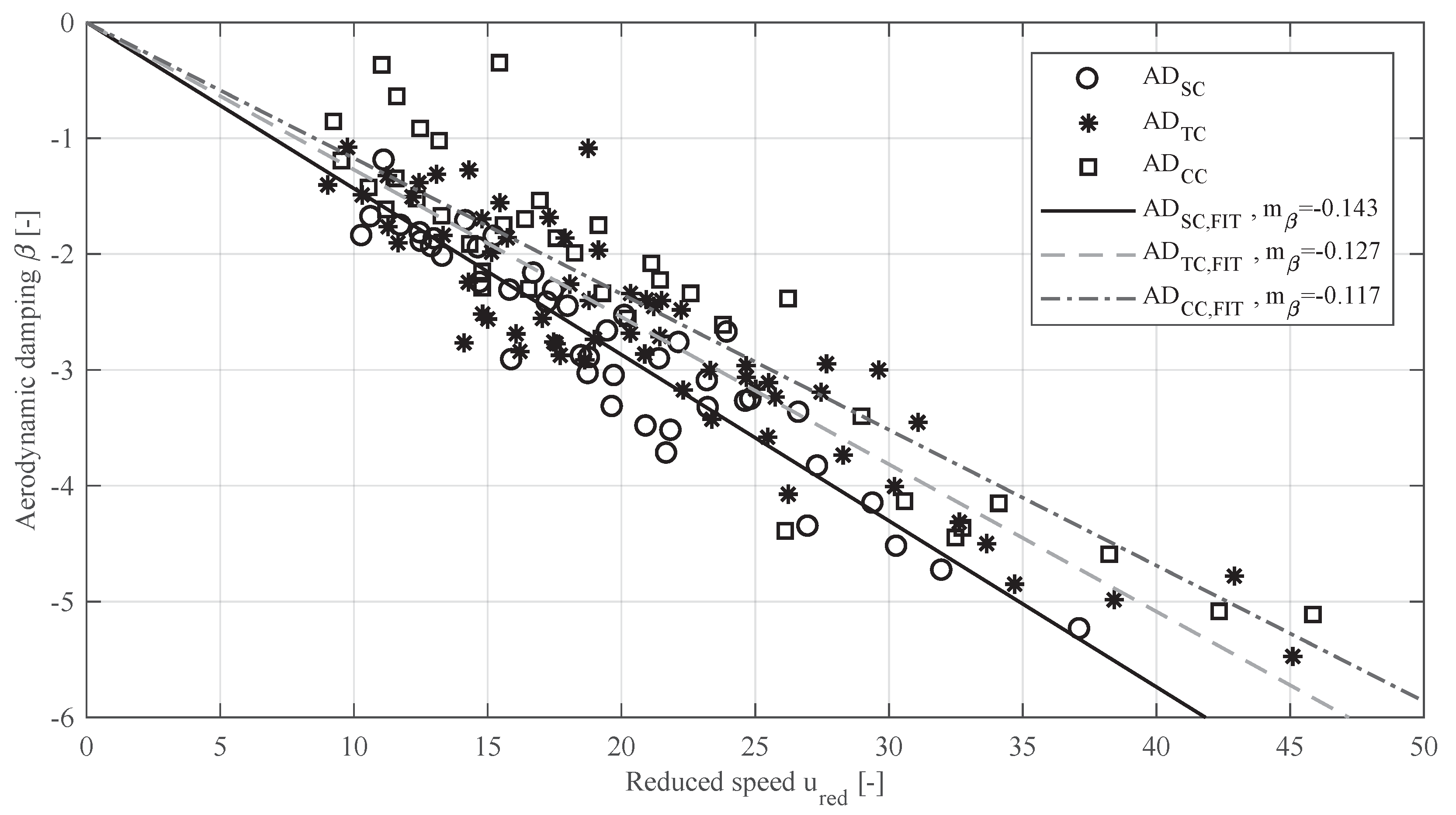
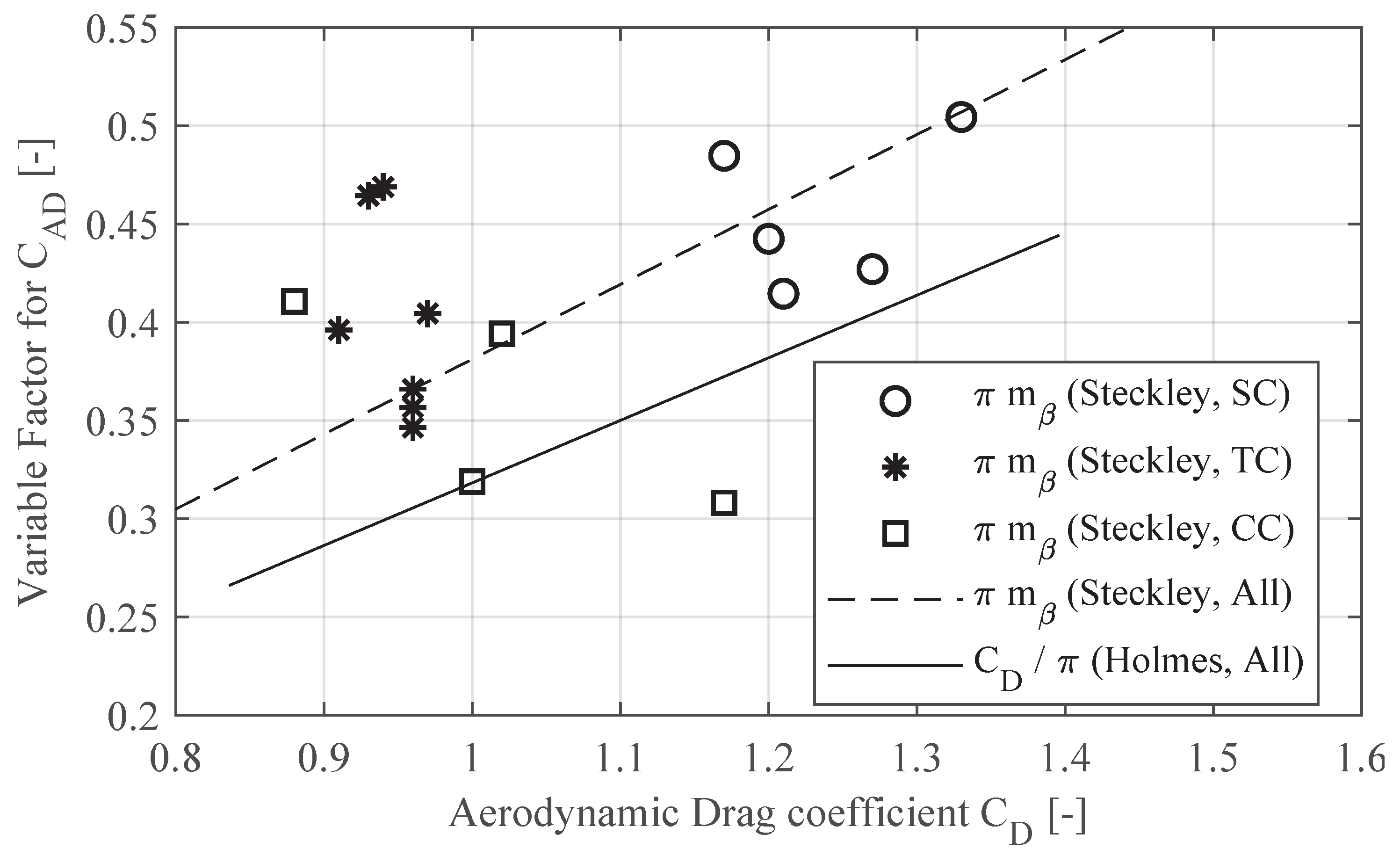
Flow Regime	Line Slope ${\mathit{m}}_{\mathit{\beta }}$	${\mathit{R}}^2$	Mean ${\mathit{C}}_{\mathit{D}}$	$\frac{{\mathit{C}}_{\mathit{D}}}{{\mathit{m}}_{\mathit{\beta }}}$
Sub-Critical	−0.143	0.89	1.24	−8.64
Trans-Critical	−0.127	0.81	0.95	−7.46
Critical	−0.117	0.86	1.02	−8.70
All regimes	−0.129	0.81	1.06	−8.17

. An additional fit was performed considering all measured points, which is also included in the table under the name of “All regimes”. The results are compared to the average drag values of each regime through the ratio $\frac{C_D}{m_{\beta }}$, which is also shown in Table 4.

The aerodynamic damping results are made dimensionless by using the normalization factor ${\lambda }_{\beta }$, which is given in Equation (11), and which is also used to upscale the aerodynamic damping to full size, as shown in

Table 5. Relevant parameters for the aerodynamic damping calculation of towers of RWTs.

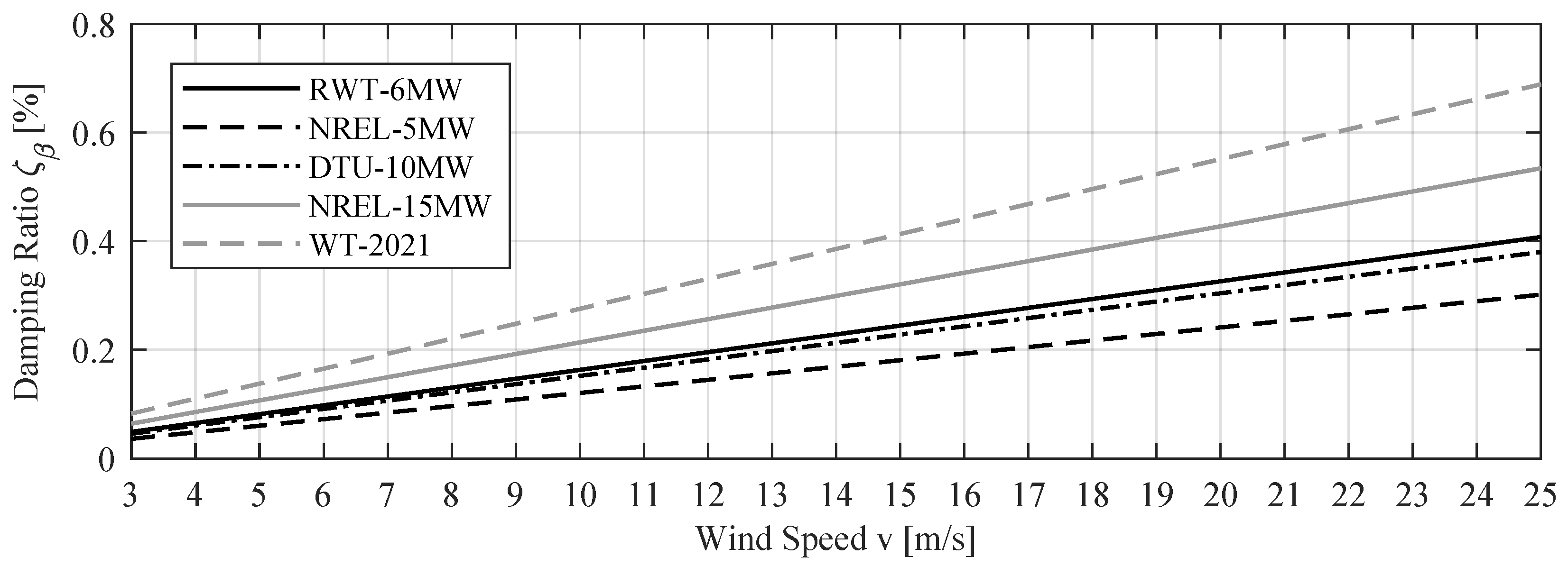
Parameter	RWT	NREL-5 MW	DTU-10 MW	NREL-15 MW
D [m]	5.63	4.2	5.95	6.78
H [m]	112	87.6	115.6	145
$f_E$ [Hz]	0.28	0.32	0.25	0.17
$M_{Modal}$ [kg]	562,405	388,195	736,967	1,102,116
${\lambda }_{\beta }$ [kg/s]	4006	1993	4123	4567
$c_{crit}$ [kg/s]	1,978,869	1,561,024.72	2,315,250.11	2,354,431.68

:

$${\lambda }_{\beta }=\frac{1}{6}{\rho }_a\pi D^2{H}^2{\left(2\pi f_E\right)}^2\widehat{Y}$$

(11)

where $f_E$ is the excitation frequency in the wind tunnel measurements or the first natural frequency of the full-scale structure, and $\widehat{Y}$ is the oscillation amplitude.

The relation between the drag and the aerodynamic damping coefficients $\frac{C_D}{m_{\beta }}$ is studied using Holmes’ approach. To this end, the aerodynamic term in Expression (4) is considered, and $\beta$ is replaced using Equation (10). Doing so, the generalized aerodynamic damping as defined by Steckley takes the following form:

$$C_{AD}=\frac{\rho }{6}{D}^2\omega H\pi m_{\beta }{u}_{red}$$

(12)

As Equations (9) and (12) are supposed to describe the same phenomenon, both equations can be equated to find the relation between $m_{\beta }$ and $C_D$. The result is a factor ${\pi }^2$, as shown in Equation (13):

$$\frac{C_D}{\pi }=\pi m_{\beta }$$

(13)

As seen in Table 4, the calculated ratio from the experiments $\frac{C_D}{m_{\beta }}$ is smaller than ${\pi }^2$, by approximately 15%. This indicates that the measured aerodynamic damping coefficients are higher than expected. The differing coefficients between Equations (9) and (12) are considered, i.e., $C_D/\pi$ in Holmes’ approach and $\pi m_{\beta }$ in Steckley’s approach, both as measured in the wind tunnel. For the aerodynamic damping measurements, a slope coefficient $m_{\beta }$ is determined for each measurement and expressed according to its previously measured $C_D$. In addition, the $m_{\beta }$ coefficients are grouped according to their flow regime. Figure 10 shows the differences between both approaches, which results in aerodynamic damping values approximately 15% higher in the wind tunnel measurements according to Steckley’s approach, which can be due to fundamental differences in the approaches, or to the wind tunnel measurement set-up (end-plate, tip effects, etc.).

4. Application of Results

In this section, the aerodynamic damping according to the approaches of Steckley and Holmes was applied in wind turbine load simulations. To study their impact on wind turbine dynamics, multi-body aeroelastic simulations of a reference wind turbine in operation were performed with and without the application of the aerodynamic damping, and fatigue proofs of the turbine bottom section were performed.

4.1. Description of Reference Wind Turbine and Its Aerodynamic Damping Forces

The reference wind turbine (RWT) used in the simulations is based on the 5 MW land-based reference wind turbine of the National Renewable Energy Laboratory (NREL) [44]. The rotor geometry remains unchanged, and the tower is modified to have a height of 112 m and a tapered diameter reaching from 5.5 m at the top to 6.5 m at the bottom, as well as a tower wall thickness linearly varying between 15 mm at the top and 60 mm at the bottom. The controller used by the NREL is replaced by a controller developed at the CWD of RWTH Aachen University using Simulink.

In accordance with the approach of Steckley, the aerodynamic damping forces can be applied as a oscillation-dependent force acting at the top of the tower. The aerodynamic damping force acts against the tower top oscillation velocity, and is therefore modeled as a linear damper acting at the tip of the tower. Following the notation in Equation (4), the aerodynamic damping force can be applied in SimPack at the tower top as follows:

$$F_{\beta }=-\left(\frac{{\pi }^2}{3}{\rho }_{a}H{D}^2{f}_E\beta \right)\dot{x}=-\left({\lambda }_{\beta }\beta \right)\dot{x}=-c_{\beta }\dot{x}$$

(14)

where $\dot{x}$ is the tower top oscillation speed. The damping coefficient $\beta$ is calculated using Formula (10) for the RWT. For comparability with Holmes’ approach, Equation (13) was used to determine $m_{\beta }$, and the drag coefficient $C_D$ was calculated using Eurocode 1 [38]. For the calculation of the reduced velocity, the wind speed was queried at the tower top, the used tower diameter was determined by weighting the tower diameters with the square of the first modal form, which corresponds roughly to the average diameter of the upper third of the tower. Table 5 summarizes some of the main properties of the studied RWT and other relevant RWTs regarding the aerodynamic damping calculation, while Table 5 summarizes the equivalent damping parameters for the RWT in the operating wind speeds. In Table 5, the resulting damping ratio ${\zeta }_{\beta }$ and logarithmic damping decrement ${\delta }_{\beta }$ are also given.

On the other hand, Holmes’ approach allows its application at each tower segment [21]. The aerodynamic damping acting in each segment is then given by:

$$dFx\left(z\right)=\frac{1}{2}{\rho }_{a}D\left(z\right)C_D\left(z\right)(\dot{x}{(z,t)}^2-2v(z,t)\dot{x}(z,t))$$

(15)

where all used parameters are queried at the studied segment. To calculate the instantaneous wind speed at the studied segment $v(z,t)$, the wind speed at the tower top was transferred to the corresponding height assuming the same exponential wind profile that is used in Turbsim to generate the acting wind fields. The drag coefficient $C_D\left(z\right)$ was calculated using Eurocode 1 [38]. For the Reynolds number calculation, the relative wind speed $v(z,t)-\dot{x}(z,t)$ was used. The minimum instantaneous drag coefficient was limited to $C_D(z,t)=0.4$.

4.2. Description of the Simulations

The RWT is modeled and simulated using Simpack, a multi-body simulation (MBS) software by Dassault Systèmes commonly used in the wind energy sector. The controller of the wind turbine is modeled using SimuLink. The wind turbine model consists of a tower, drive train and blades. The tower is modeled as a Timoshenko beam and split up into 11 elements. The drive train contains the main shaft, the simplified gearbox and the generator. The blades are linear flexible bodies imported from a finite element model using modal reduction.

For the generation of the wind turbine loads, the design load case 1.2 as defined in IEC 61400-1 [45] is considered. The objective is to reproduce realistic wind turbine in operating conditions in the simulations to study the impact of the aerodynamic damping on the tower in such conditions. To this end, turbulent wind fields are generated using NREL’s Turbsim and are integrated into the model using NREL’s AeroDyn V13 force element. For the simulation of the wind fields, the class “A” according to IEC 61400-1 [45] is chosen for the turbulence characteristic. The hub height is 115m, and the other necessary parameters are chosen according to recommendations of the IEC 61400-1 [45].

The simulations are then performed 6 times for every odd wind speed from 3 to 25 m/s and for the rated wind speed, with and without simulating the aerodynamic damping, as expressed in Equations (14) and (15), which results in a total of 216 simulations. The random seed of each generated wind field is changed for every individual simulation to improve independence from the wind conditions. To enable better comparability, the simulations with aerodynamic damping use the same wind fields as their counterparts without aerodynamic damping (i.e., only 72 independent wind fields are generated).

4.3. Results and Discussion

To study the effect of considering the aerodynamic damping of the tower, a fatigue analysis is performed at the bottom of the tower. The fatigue calculations are performed following indications of the Eurocode 3 [46]. First, the simulation time series of the internal forces are used to determine the time series of the stresses at the tower bottom. The stress cycles’ amplitudes and occurrences are identified in MATLAB using rainflow routines. Using the Wöhler curves given in EC3, the damage of each cycle is calculated and then added to the total damage using the Palmgren–Miner rule.

In the first step, the damage of each wind speed is calculated for the all the simulations with and without aerodynamic damping. The tower section zone with higher damage is studied, i.e., the upwind part of the section. The results are normalized with the total damage caused by all simulations without aerodynamic damping. This way, the effect of the aerodynamic damping in each wind speed can be easily compared. In this case, no probability distribution for the wind speeds is yet used. The normalized damage is shown in Figure 11.

As expected, the effect of the aerodynamic damping is more pronounced for higher wind speeds. The total normalized damage in the simulations without aerodynamic damping is 1.43% higher than in the simulations with aerodynamic damping according to Steckley and 0.89% higher than in the simulations with aerodynamic damping according to Holmes. The performed fatigue proofs showed that with Holmes’ approach, a lifetime extension of around 0.40% can be achieved, while Steckley’s approach allows a lifetime extension of around 0.7%. Steckley’s approach assumes that the tower oscillation occurs predominantly according to its first modal form, and the approach is applied globally through a unique aerodynamic force at the tower top. A global application of Holmes approach is also possible (see generalized aerodynamic damping and its assumption in Section 3 and Equations (8) and (9)), but the possibility to apply it segmentwise along the tower makes it more accurate in this case.

In the results of Werkmeister [18], the tower’s aerodynamic damping has a larger impact on the tower fatigue. This is due to the wind turbine used in the BBTI Project [17] being heavier and the tower more flexible than the ones in this study.

Finally, the current study has been performed on a 6MW onshore turbine, but it can be repeated for other common reference wind turbines, see relevant parameters in Table 5. The objective of these reference wind turbines is, however, to push the current design boundaries to the limit and therefore have a lot of optimization potential, which is usually developed afterwards by wind turbine OEMs. As a result, RWTs have generally larger tower diameters and component masses than market turbines with similar power capacity. To exemplify the magnitude of the tower aerodynamic damping in the context of a state-of-the-art wind turbine, a theoretical but plausible 5 MW onshore wind turbine “WT-2021” is considered based on the data available online and in the literature. This turbine is assumed to have a modally averaged diameter of 3.5 m, a hub height of 115 m, a first natural frequency of 0.17 Hz and a modal mass of 350 tonnes. If the procedure shown in

Table 6. Aerodynamic damping of the RWT Tower.

${\mathit{v}}_{\mathbf{wind}}$	${\mathit{u}}_{\mathbf{red}}$	$\mathit{\beta }[-]$	${\mathit{c}}_{\mathit{\beta }}$ [kg/s]	${\mathit{\zeta }}_{\mathit{\beta }}[-]$	${\mathit{\delta }}_{\mathit{\beta }}[-]$
3	1.90	−0.24	968	0.05%	0.003
5	3.17	−0.40	1614	0.08%	0.005
7	4.44	−0.56	2259	0.11%	0.007
9	5.71	−0.73	2905	0.15%	0.009
11	6.98	−0.89	3550	0.18%	0.011
13	8.25	−1.05	4196	0.21%	0.013
15	9.52	−1.21	4841	0.24%	0.015
17	10.78	−1.37	5486	0.28%	0.017
19	12.05	−1.53	6132	0.31%	0.019
21	13.32	−1.69	6777	0.34%	0.022
23	14.59	−1.85	7423	0.38%	0.024
25	15.86	−2.01	8068	0.41%	0.026

is repeated for this turbine and for all RWTs, the critical damping curves given in Figure 12 are obtained.

Figure 12 shows that, in the case of the RWTs in the literature, for each increase of 2.5 MW, the tower’s aerodynamic damping ratio is increased roughly by 0.1%. The main parameters determining the damping ratio can be found by introducing Equations (8) or (14) in the damping ratio equation, which yields the following expressions:

$${\zeta }_{\beta }=\frac{{\rho }_a}{12}\frac{HD}{M_M{f}_E}\frac{C_D}{\pi }{v}_{wind}=\frac{{\rho }_a}{12}\frac{HD}{M_M{f}_E}\pi m_{\beta }{v}_{wind}$$

(16)

where $M_M$ is the generalized modal mass. The damping ratio is then directly proportional to the wind turbine hub height and the averaged diameter and inversely proportional to its generalized mass and its first natural frequency (a similar behavior is expected for the second natural frequency). Although this expression is somewhat complex, because all these parameters are implicitly dependent on each other, it allows for an overview of which role the aerodynamic damping of the tower may play in the future. In onshore turbines with tubular steel towers, the diameter is usually constrained to 4.3–4.5 m, which consequently limits the realizable mass-to-hub height combinations, while the tendency towards soft-soft designs causes lower natural tower frequencies. In general, the tendency towards higher towers with lower natural frequencies and relatively similar turbine masses hints towards an increasing relevance of the tower’s aerodynamic damping. This can be seen in Figure 12, where the theoretical wind turbine WT-2021 shows a much higher aerodynamic damping ratio than all other RWTs, mostly due to its comparatively reduced head mass. It shall also be more relevant in offshore wind turbines, where larger oscillations imply larger oscillation speeds (for example, in the case of floating wind turbines), and the typically higher capacity factors (which range between 41% [47] and over 50% [48]) increase the economic repercussion of any lifetime extension.

5. Conclusions

The aerodynamic drag coefficients of cylinders with circular cross-sections and different surface roughnesses have been determined in wind tunnel tests with low ($I_u$ = 4%) and high ($I_u$ = 11.5%) turbulence flows. The localization of the transition to trans-critical flow conditions ($R{e}_{crit}$) has been documented in Figure 5 and Table 2 for guidance in experimental studies where the Reynolds number effects play an important role. The reproducibility of the surface roughness and specially the consideration of high-turbulence conditions is still relatively scarce in the literature.

The aerodynamic damping of wind turbine towers has been determined through wind tunnel experiments using Steckley’s approach and the forced oscillation method. The measurements have been performed in sub-critical, critical and trans-critical regimes, and the results were compared to a general approach by Holmes [21]. Both approaches were applied in aeroelastic multi-body simulations to study the impact of the tower’s aerodynamic damping on the tower fatigue of a 6MW reference wind turbine, obtaining a tower lifetime extension of approximately 0.4%. It can be concluded that while Steckley’s approach is useful for structures which oscillate primarily in their first modal form, its application as a generalized aerodynamic damping force is not accurate for wind turbine towers, where the second modal form and the loading on the rotor have a relevant contribution on the tower dynamic behavior.

The aerodynamic damping due to the tower may play a more important role for future turbines, as has been shown in Section 4.3. The results of this study support a better understanding of its nature and magnitude. In particular, Equation (16) enables a quick estimation of the tower aerodynamic damping and of its contribution to the total damping of wind turbines in operation and parked conditions, which can help in the interpretation of on-site measured responses such as the previously cited [13,14,15,16]. In addition, Equation (16) also enhances fatigue analysis in frequency domain ([49,50]). Finally, the application or modeling of the tower aerodynamic damping in aeroelastic software codes is possible in the design phase, which considers the resultant higher damping already in the design of all turbine components.

Future studies should consider the effect of the passing blade on the tower wind pressure distribution, so that its effect on the aerodynamic load and damping on the tower can also be considered. The presented findings of realistic modeling for wind tunnel investigations can contribute to future research for other dynamic effects such as vortex-induced vibrations.