Vertical Dynamic Impedance of a Viscoelastic Pile in Arbitrarily Layered Soil Based on the Fictitious Soil Pile Model
Abstract
:1. Introduction
2. Mathematical Model Construction
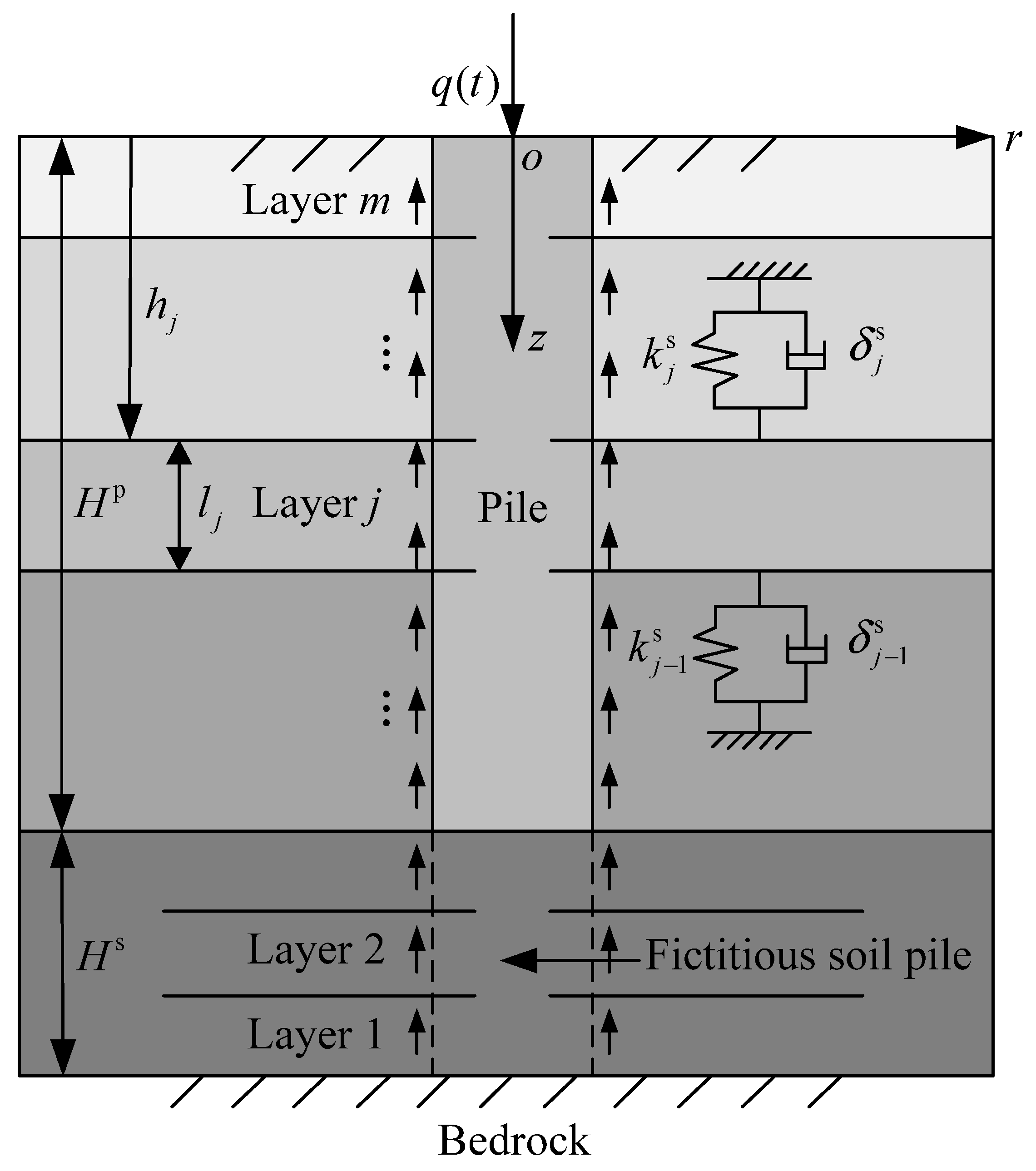
2.1. Geometry of Pile–Soil System
2.2. Dynamic Equilibrium Equations of the Pile–Soil System
2.3. Boundary Conditions (BCs) and Initial Conditions (ICs)
3. Solutions of Pile Surrounding Soil
4. Solutions of Pile and Fictitious Soil Pile
4.1. Solutions for the First FSP Segment
4.2. Solutions for the Viscoelastic Pile in Arbitrarily Layered Soil
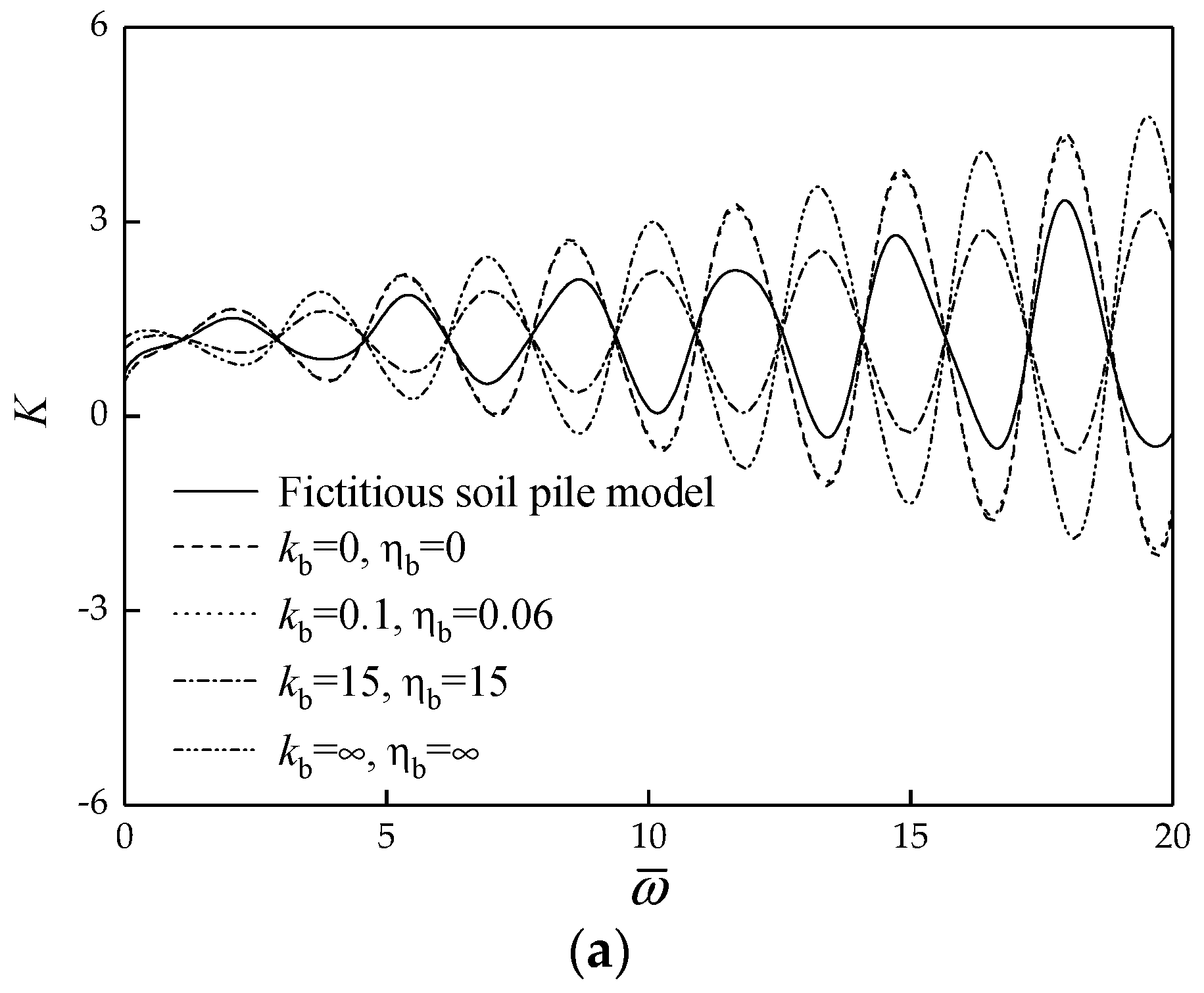
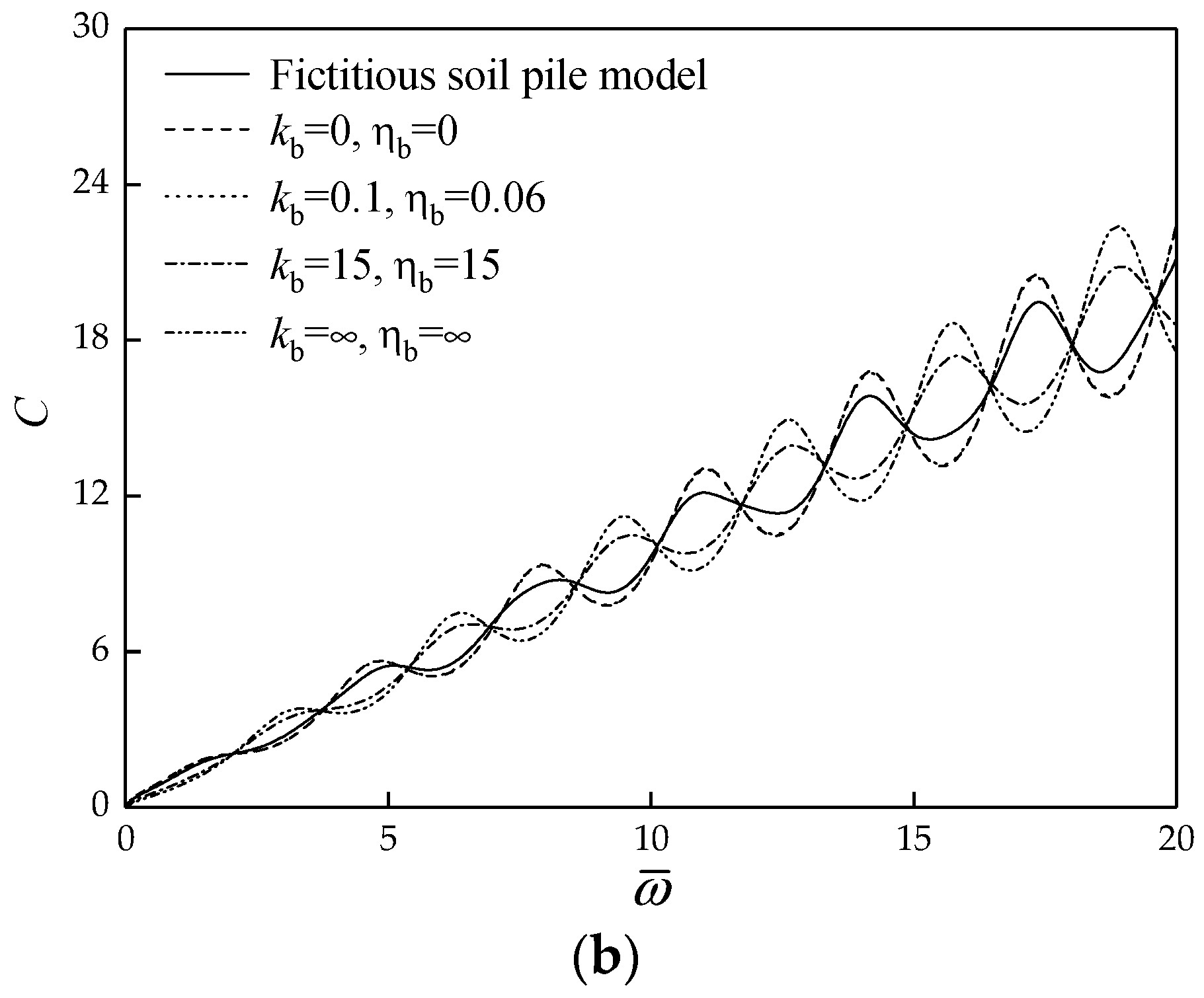
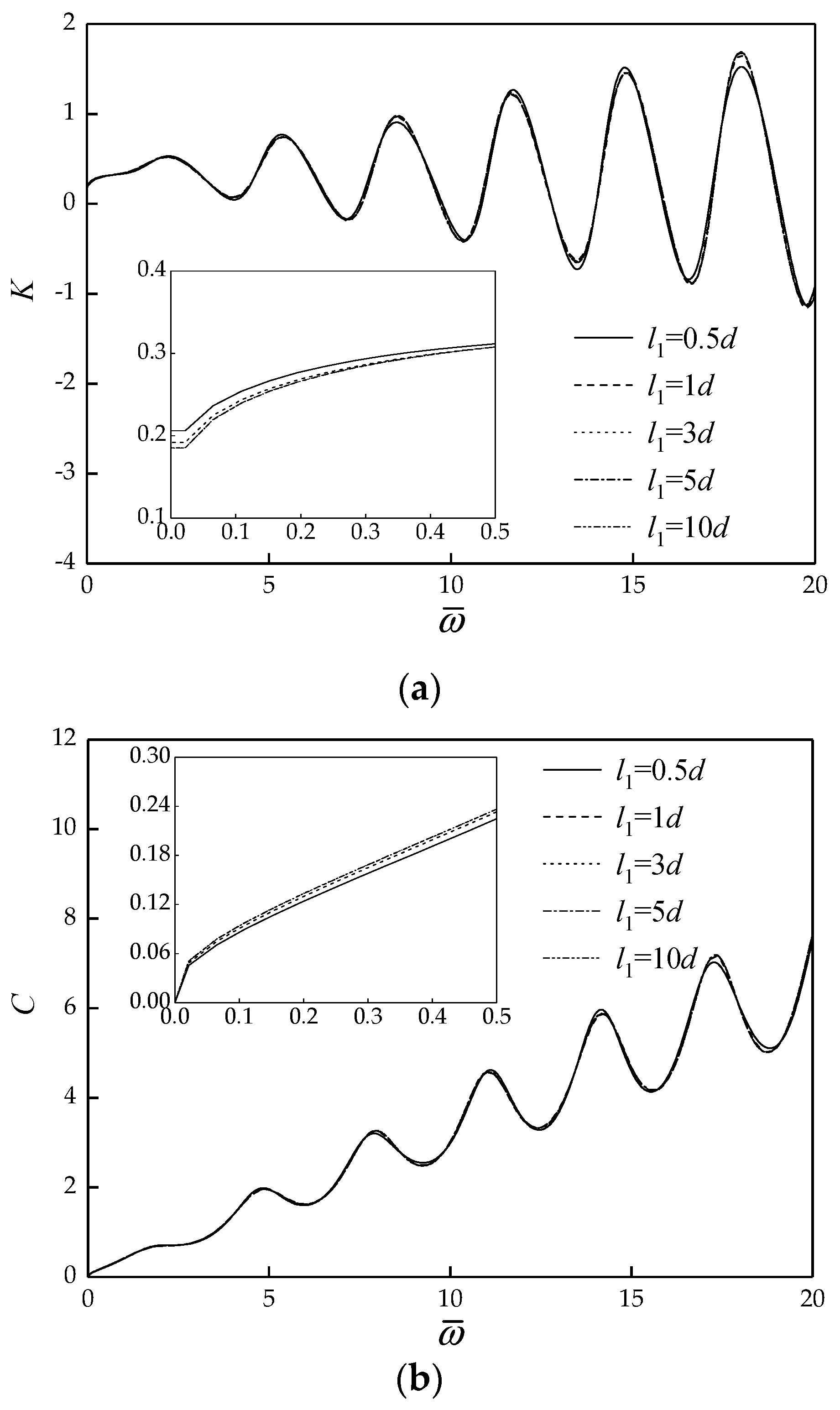
5. Rationality Analysis of the Present Solutions
6. Parametric Study
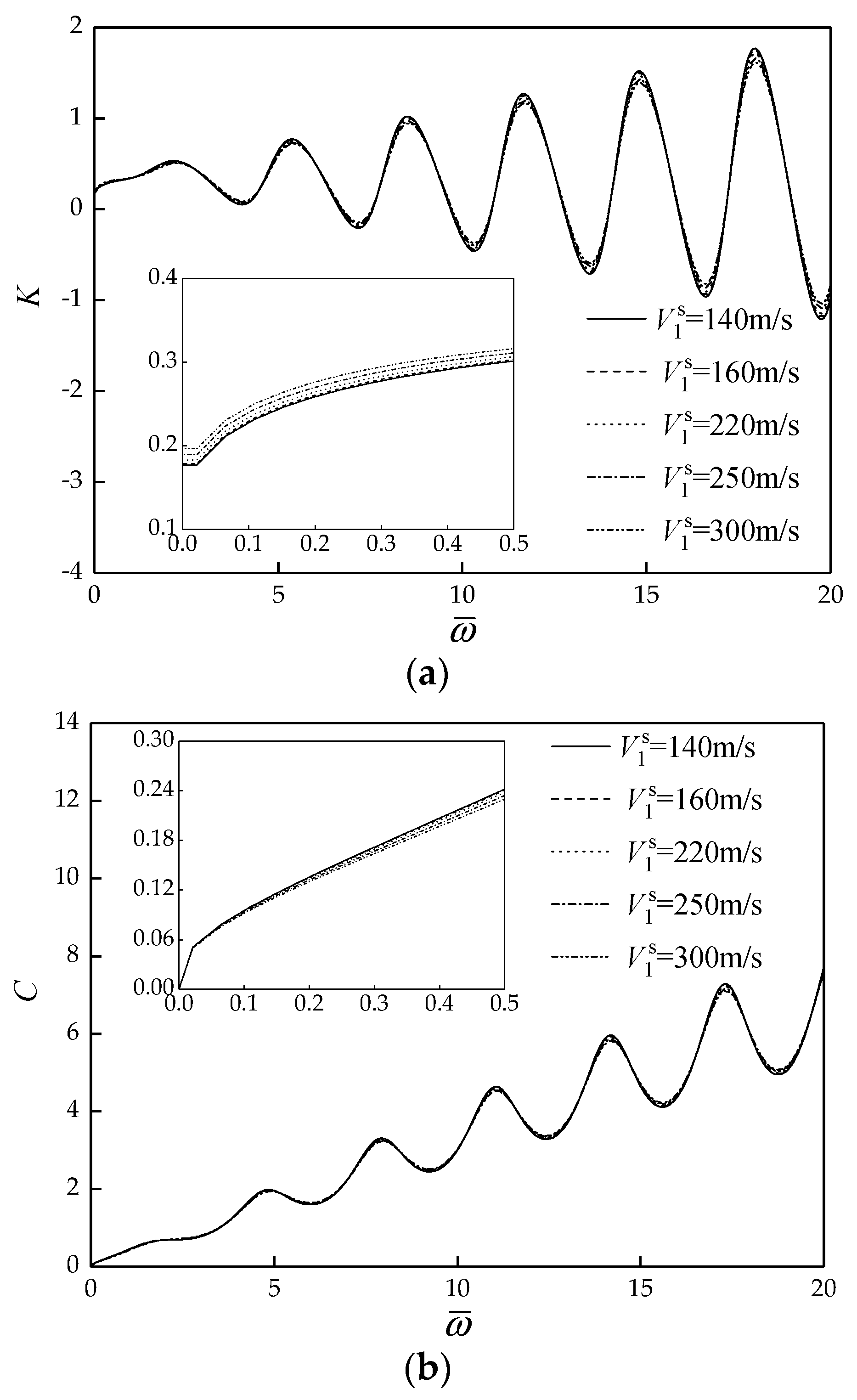
6.1. Effect of Single Homogeneous PES Layer on the Vertical Dynamic Impedance of Pile
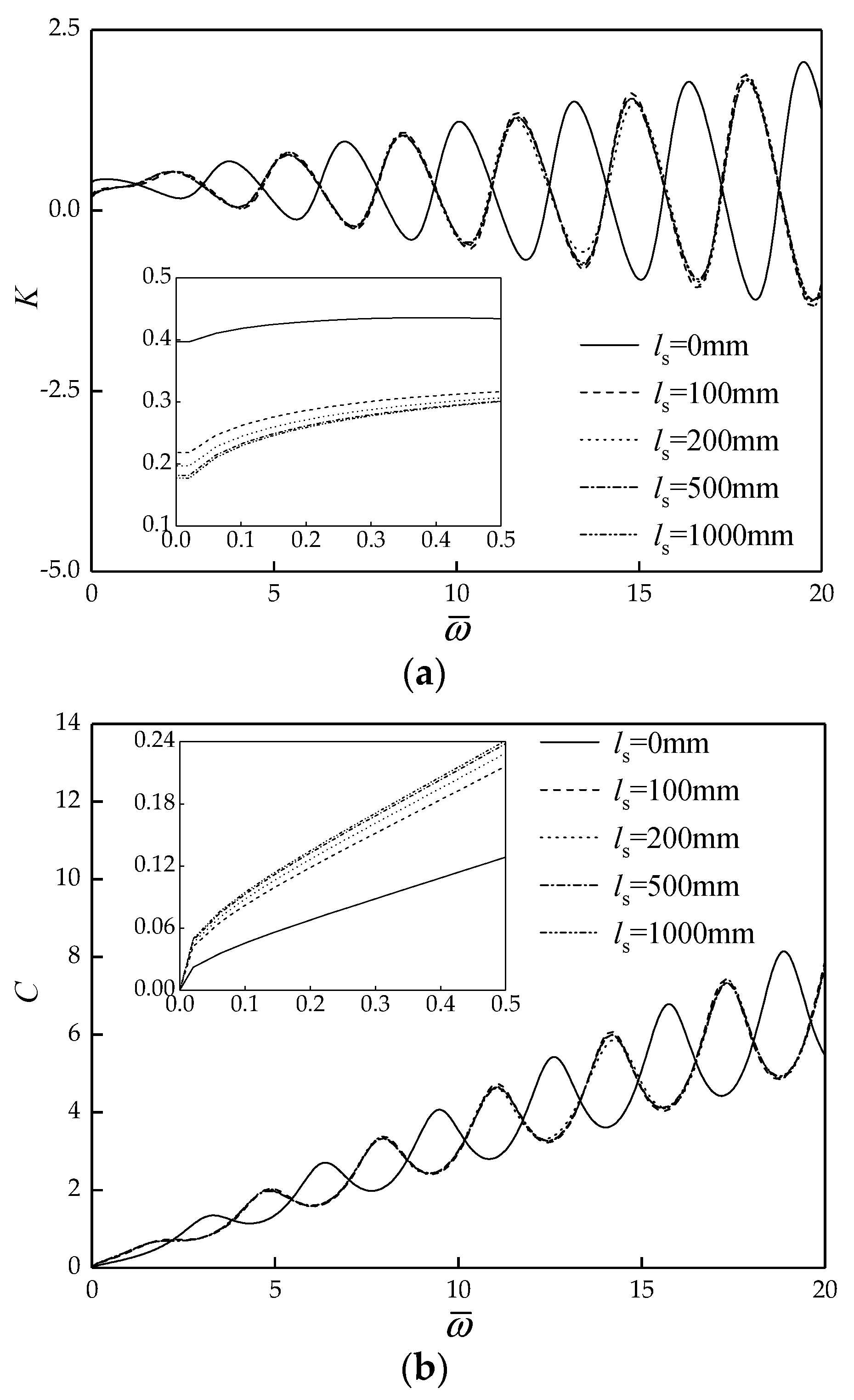
6.2. Effect of Two PES Layers on the Vertical Dynamic Impedance of Pile
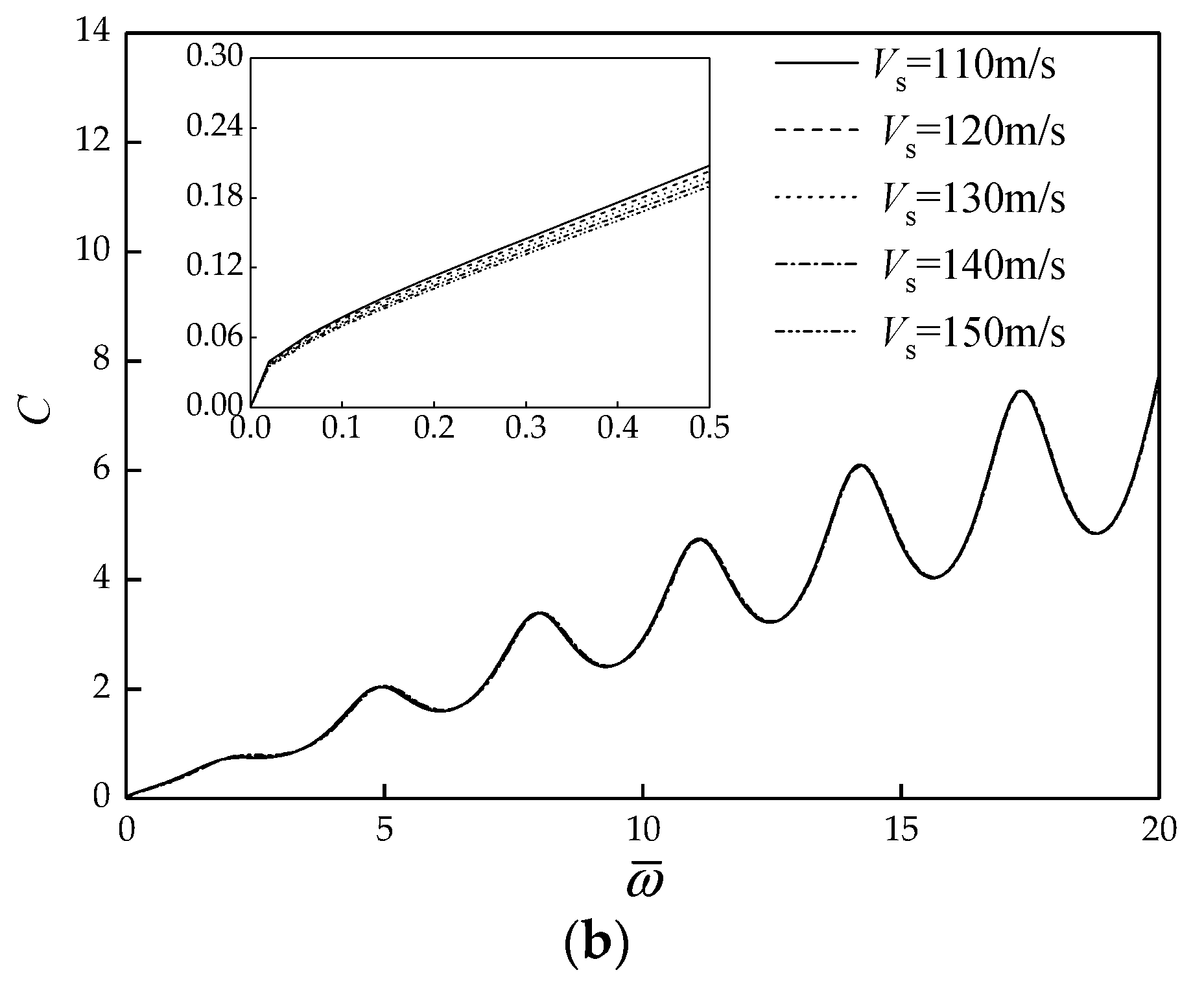
6.3. Effect of Sediment on the Vertical Dynamic Impedance of Rock-Socketed Pile
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, H.; Liang, F.Y.; Zheng, H.B. Dynamic impedance of monopiles for offshore wind turbines considering scour-hole dimensions. Appl. Ocean Res. 2021, 107, 102493. [Google Scholar] [CrossRef]
- Wu, J.T.; El Naggar, M.H.; Zhao, S.; Wen, M.J.; Wang, K.H. Beam-unequal length piles-soil coupled vibrating system considering pile-soil-pile interaction. J. Bridge Eng. 2021, 26, 04021086. [Google Scholar] [CrossRef]
- Li, L.C.; Zheng, M.Y.; Liu, X.; Wu, W.B.; Liu, H.; El Naggar, M.H.; Jiang, G.S. Numerical analysis of the cyclic loading behavior of monopile and hybrid pile foundation. Comput. Geotech. 2022, 144, 104635. [Google Scholar] [CrossRef]
- Shao, K.; Su, Q.; Liu, K.W.; Shao, G.X.; Zhong, Z.B.; Li, Z.Q.; Chen, C. A new modified approach to evaluating the installation power of large-diameter helical piles in sand validated by centrifuge and field data. Appl. Ocean Res. 2021, 114, 102756. [Google Scholar] [CrossRef]
- Novak, M. Vertical vibration of floating pile. J. Eng. Mech. Div. ASCE 1977, 103, 153–168. [Google Scholar] [CrossRef]
- Nogami, T.; Konagai, K. Time domain axial response of dynamically loaded single piles. J. Eng. Mech. Div. ASCE 1986, 112, 1241–1252. [Google Scholar] [CrossRef]
- Liao, S.T.; Roesset, J.M. Dynamic response of intact piles to impulse loads. Int. J. Numer. Anal. Met. 1997, 21, 255–275. [Google Scholar] [CrossRef]
- Michaelides, O.; Gazetas, G.; Bouckovalas, G.; Chrysikou, E. Approximate non-linear dynamic axial response of piles. Geotechnique 1998, 48, 33–53. [Google Scholar] [CrossRef] [Green Version]
- Militano, G.; Rajapakse, R.K.N.D. Dynamic response of a pile in a multi-layered soil to transient torsional and axial loading. Geotechnique 1999, 49, 91–109. [Google Scholar] [CrossRef]
- Wang, K.H.; Wu, W.B.; Zhang, Z.Q.; Leo, C.J. Vertical dynamic response of an inhomogeneous viscoelastic pile. Comput. Geotech. 2010, 37, 536–544. [Google Scholar] [CrossRef]
- Wu, W.B.; El Naggar, M.H.; Abdlrahem, M.; Mei, G.X.; Wang, K.H. New interaction model for vertical dynamic response of pipe piles considering soil plug effect. Can. Geotech. J. 2017, 54, 987–1001. [Google Scholar] [CrossRef]
- Chen, L.B.; Yang, X.Y.; Wu, W.B.; El Naggar, M.H.; Wang, K.H.; Chen, J.Y. Numerical analysis of the deformation performance of monopile under wave and current load. Energies 2020, 13, 6431. [Google Scholar] [CrossRef]
- Cui, C.Y.; Liang, Z.M.; Xu, C.S.; Xin, Y.; Wang, B.L.; Meng, K. New Analytical solution to predict the vertical impedance of a large-diameter pipe pile in soil considering wave propagation in visco-elastic continuum. J. Earthq. Tsunami 2021, 2140002. [Google Scholar] [CrossRef]
- Novak, M. Dynamic stiffness and damping of piles. Can. Geotech. J. 1974, 11, 574–598. [Google Scholar] [CrossRef]
- Mamoon, S.M.; Banerjee, P.K. Time-domain analysis of dynamically loaded single piles. J. Eng. Mech. Div. ASCE 1992, 118, 140–160. [Google Scholar] [CrossRef]
- El Naggar, M.H.; Novak, M. Nonlinear lateral interaction in pile dynamics. Soil Dyn. Earthq. Eng. 1995, 14, 141–157. [Google Scholar] [CrossRef]
- Wu, W.B.; Jiang, G.S.; Huang, S.G.; Mei, G.X.; Leo, C.J. A new analytical model to study the influence of weld on the vertical dynamic response of prestressed pipe pile. Int. J. Numer. Anal. Met. 2017, 41, 1247–1266. [Google Scholar] [CrossRef]
- Liu, H.; Wu, W.B.; Jiang, G.S.; El Naggar, M.H.; Mei, G.X.; Liang, R.Z. Benefits from using two receivers for the interpretation of low-strain integrity tests on pipe piles. Can. Geotech. J. 2019, 56, 1433–1447. [Google Scholar] [CrossRef]
- Liu, H.; Wu, W.B.; Yang, X.Y.; Jiang, G.S.; El Naggar, M.H.; Mei, G.S.; Liang, R.Z. Detection sensitivity analysis of pipe pile defects during low-strain integrity testing. Ocean Eng. 2019, 194, 106627. [Google Scholar] [CrossRef]
- Wu, W.B.; Liu, H.; Yang, X.Y.; Jiang, G.S.; El Naggar, M.H.; Mei, G.S.; Liang, R.Z. New method to calculate apparent phase velocity of open-ended pipe pile. Can. Geotech. J. 2020, 57, 127–138. [Google Scholar] [CrossRef]
- Zhang, Y.P.; Yang, X.Y.; Wu, W.B.; El Naggar, M.H.; Jiang, G.S.; Liang, R.Z. Torsional complex impedance of pipe pile considering pile installation and soil plug effect. Soil Dyn. Earthq. Eng. 2020, 131, 106010. [Google Scholar] [CrossRef]
- Guan, W.J.; Wu, W.B.; Jiang, G.S.; Leo, C.J.; Deng, G.D. Torsional dynamic response of tapered pile considering compaction effect and stress diffusion effect. J. Cent. South Univ. 2020, 27, 3839–3851. [Google Scholar] [CrossRef]
- Nogami, T.; Novak, M. Soil-pile interaction in vertical vibration. Earthq. Eng. Struct. D 1976, 4, 277–293. [Google Scholar] [CrossRef]
- Rajapakse, R.K.N.D.; Chen, Y.; Senjuntichai, T. Electroelastic field of a piezoelectric annular finite cylinder. Int. J. Solids Struct. 2005, 42, 3487–3508. [Google Scholar] [CrossRef]
- Wang, K.H.; Zhang, Z.Q.; Leo, C.J.; Xie, K.H. Dynamic torsional response of an end bearing pile in saturated poroelastic medium. Comput. Geotech. 2008, 35, 450–458. [Google Scholar] [CrossRef]
- Wu, W.B.; Wang, K.H.; Zhang, Z.Q.; Leo, C.J. Soil-pile interaction in the pile vertical vibration considering true three-dimensional wave effect of soil. Int. J. Numer. Anal. Met. 2013, 37, 2860–2876. [Google Scholar] [CrossRef]
- Luan, L.B.; Ding, X.M.; Zheng, C.J.; Kouretzis, G.; Wu, Q. Dynamic response of pile groups subjected to horizontal loads. Can. Geotech. J. 2020, 57, 469–481. [Google Scholar] [CrossRef]
- Qu, L.M.; Yang, C.W.; Ding, X.M.; Kouroussis, G.; Zheng, C.J. A continuum-based model on axial pile-head dynamic impedance in inhomogeneous soil. Acta Geotech. 2021, 16, 3339–3353. [Google Scholar] [CrossRef]
- Cui, C.Y.; Meng, K.; Xu, C.S.; Liang, Z.M.; Li, H.J.; Pei, H.F. Analytical solution for longitudinal vibration of a floating pile in saturated porous media based on a fictitious saturated soil pile model. Comput. Geotech. 2021, 131, 103942. [Google Scholar] [CrossRef]
- Zhang, Y.P.; Liu, H.; Wu, W.B.; Wang, L.X.; Jiang, G.S. A 3D analytical model for distributed low strain test and parallel seismic test of pipe piles. Ocean Eng. 2021, 225, 108828. [Google Scholar] [CrossRef]
- Luan, L.B.; Gao, L.; Kouretzis, G.; Ding, X.M.; Qin, H.Y.; Zheng, C.J. Response of pile groups in layered soil to dynamic lateral loads. Comput. Geotech. 2022, 142, 104564. [Google Scholar] [CrossRef]
- Zheng, C.J.; Cai, Y.J.; Kouretzis, G.; Luan, L.B. Horizontal vibration of rigid strip footings on poroelastic half-space. J. Sound Vib. 2022, 522, 116731. [Google Scholar] [CrossRef]
- Zhang, Y.P.; Jiang, G.S.; Wu, W.B.; El Naggar, M.H.; Liu, H.; Wen, M.J.; Wang, K.H. Analytical solution for distributed torsional low strain integrity test for pipe pile. Int. J. Numer. Anal. Met. 2022, 46, 47–67. [Google Scholar] [CrossRef]
- Zhao, M.; Huang, Y.M.; Wang, P.G.; Cao, Y.H.; Du, X.L. An analytical solution for the dynamic response of an end-bearing pile subjected to vertical P-waves considering water-pile-soil interactions. Soil Dyn. Earthq. Eng. 2022, 153, 107126. [Google Scholar] [CrossRef]
- Wu, W.B.; Yang, Z.J.; Liu, X.; Zhang, Y.P.; Liu, H.; El Naggar, M.H.; Xu, M.J.; Mei, G.X. Horizontal dynamic response of pile in unsaturated soil considering its construction disturbance effect. Ocean Eng. 2022, 245, 110483. [Google Scholar] [CrossRef]
- Zheng, C.J.; Gan, S.S.; Luan, L.B.; Ding, X.M. Vertical dynamic response of a pile embedded in a poroelastic soil layer overlying rigid base. Acta Geotech. 2021, 16, 977–983. [Google Scholar] [CrossRef]
- Wu, J.T.; El Naggar, M.H.; Ge, J.; Wang, K.H.; Zhao, S. Multipoint traveling wave decomposition method and its application in extended pile shaft integrity test. J. Geotech. Geoenviron. 2021, 147, 04021128. [Google Scholar] [CrossRef]
- Yang, D.Y.; Wang, K.H.; Zhang, Z.Q.; Leo, C.J. Longitudinal dynamic response of pile in a radially heterogeneous soil layer. Int. J. Numer. Anal. Met. 2008, 33, 1039–1054. [Google Scholar] [CrossRef]
- Zhang, Y.P.; Liu, H.; Wu, W.B.; Wang, S.; Wu, T.; Wen, M.J.; Jiang, G.S.; Mei, G.X. Interaction model for torsional dynamic response of thin-wall pipe piles embedded in both vertically and radially inhomogeneous soil. Int. J. Geomech. 2021, 21, 04021185. [Google Scholar] [CrossRef]
- Wu, W.B.; Wang, K.H.; Ma, S.J.; Leo, C.J. Longitudinal dynamic response of pile in layered soil based on virtual soil pile model. J. Cent. South Univ. 2012, 19, 1999–2007. [Google Scholar] [CrossRef]
- Wu, W.B.; Jiang, G.S.; Huang, S.G.; Leo, C.J. Vertical dynamic response of pile embedded in layered transversely isotropic soil. Math. Probl. Eng. 2014, 12, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Wu, W.B.; Liu, H.; El Naggar, M.H.; Mei, G.X.; Jiang, G.S. Torsional dynamic response of a pile embedded in layered soil based on the fictitious soil pile model. Comput. Geotech. 2016, 80, 190–198. [Google Scholar] [CrossRef]
- Zhang, Y.P.; Wu, W.B.; Jiang, G.S.; Wen, M.J.; Wang, K.H.; El Naggar, M.H.; Ni, P.P.; Mei, G.X. A new approach for estimating the vertical elastic settlement of a single pile based on the fictitious soil pile model. Comput. Geotech. 2021, 134, 104100. [Google Scholar] [CrossRef]
- Zhang, Y.P.; Wu, W.B.; Zhang, H.K.; El Naggar, M.H.; Wang, K.H.; Jiang, G.S.; Mei, G.X. A novel soil-pile interaction model for vertical pile settlement prediction. Appl. Math. Model 2021, 99, 478–496. [Google Scholar] [CrossRef]
- Wang, L.X.; Wu, W.B.; Zhang, Y.P.; Li, L.C.; Liu, H.; El Naggar, M.H. Nonlinear analysis of single pile settlement based on stress bubble fictitious soil pile model. Int. J. Numer. Anal. Met. 2022. [Google Scholar] [CrossRef]
- Lysmer, J.; Richart, F.E. Dynamic response of footing to vertical load. J. Soil Mech. Found. Div. ASCE 1966, 2, 65–91. [Google Scholar] [CrossRef]
- Novak, M.; Beredugo, Y.O. Vertical vibration of embedded footings. J. Soil Mech. Found. Div. ASCE 1972, 98, 1291–1310. [Google Scholar] [CrossRef]
- Meyerholf, G.G. Bearing capacity and settlement of pile foundations. J. Geotech. Eng. Div. ASCE 1976, 102, 195–228. [Google Scholar]
- Liang, R.Y.; Husein, A.I. Simplified dynamic method for pile-driving control. J. Geotech. Geoenviron. 1993, 119, 694–713. [Google Scholar] [CrossRef]
- JGJ 94-2008; Technical Code for Building Pile Foundations. China Construction Industry Press: Beijing, China, 2008.




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, X.; Wang, L.; Wu, W.; Liu, H.; Jiang, G.; Wang, K.; Mei, G. Vertical Dynamic Impedance of a Viscoelastic Pile in Arbitrarily Layered Soil Based on the Fictitious Soil Pile Model. Energies 2022, 15, 2087. https://doi.org/10.3390/en15062087
Yang X, Wang L, Wu W, Liu H, Jiang G, Wang K, Mei G. Vertical Dynamic Impedance of a Viscoelastic Pile in Arbitrarily Layered Soil Based on the Fictitious Soil Pile Model. Energies. 2022; 15(6):2087. https://doi.org/10.3390/en15062087
Chicago/Turabian StyleYang, Xiaoyan, Lixing Wang, Wenbing Wu, Hao Liu, Guosheng Jiang, Kuihua Wang, and Guoxiong Mei. 2022. "Vertical Dynamic Impedance of a Viscoelastic Pile in Arbitrarily Layered Soil Based on the Fictitious Soil Pile Model" Energies 15, no. 6: 2087. https://doi.org/10.3390/en15062087
APA StyleYang, X., Wang, L., Wu, W., Liu, H., Jiang, G., Wang, K., & Mei, G. (2022). Vertical Dynamic Impedance of a Viscoelastic Pile in Arbitrarily Layered Soil Based on the Fictitious Soil Pile Model. Energies, 15(6), 2087. https://doi.org/10.3390/en15062087






