Polar Voltage Space Vectors of the Six-Phase Two-Level VSI
Abstract
1. Introduction
2. State and Space Vectors of a Six-Phase Two-Level Voltage Source Inverter
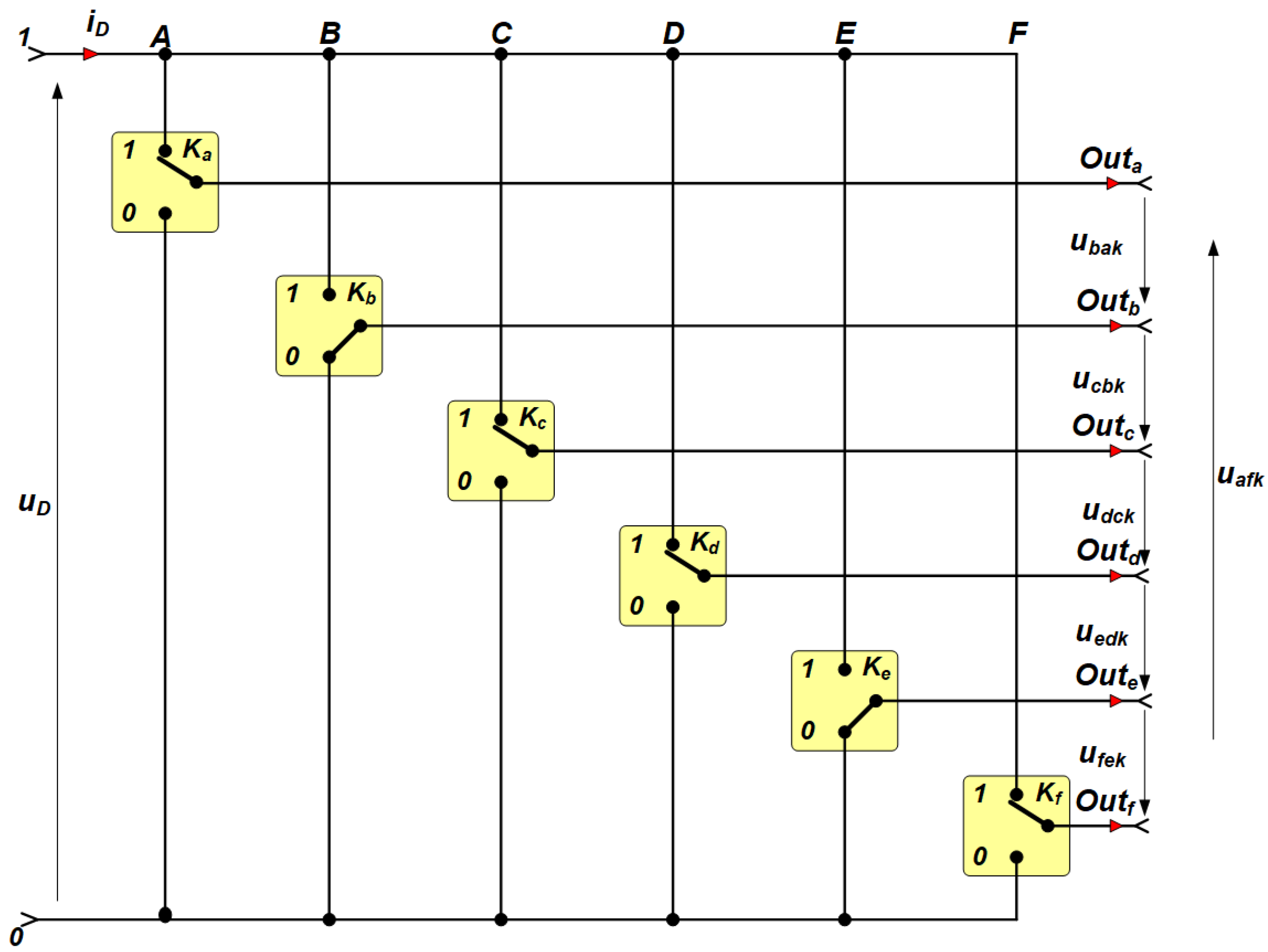
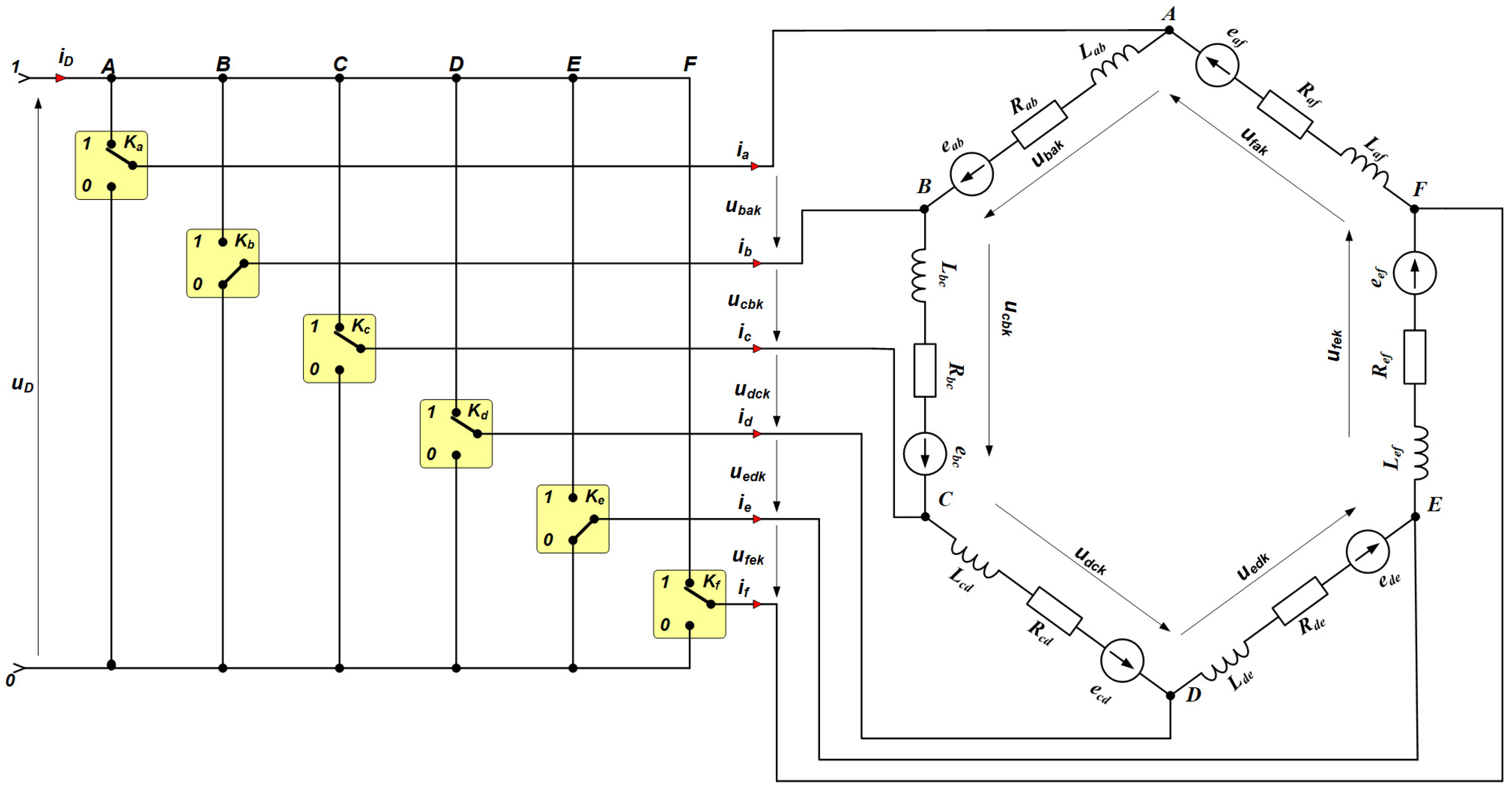
2.1. Model of Six-Phase Two-Level Inverter
2.2. State Vectors of the Six-Phase Two-Level Inverter
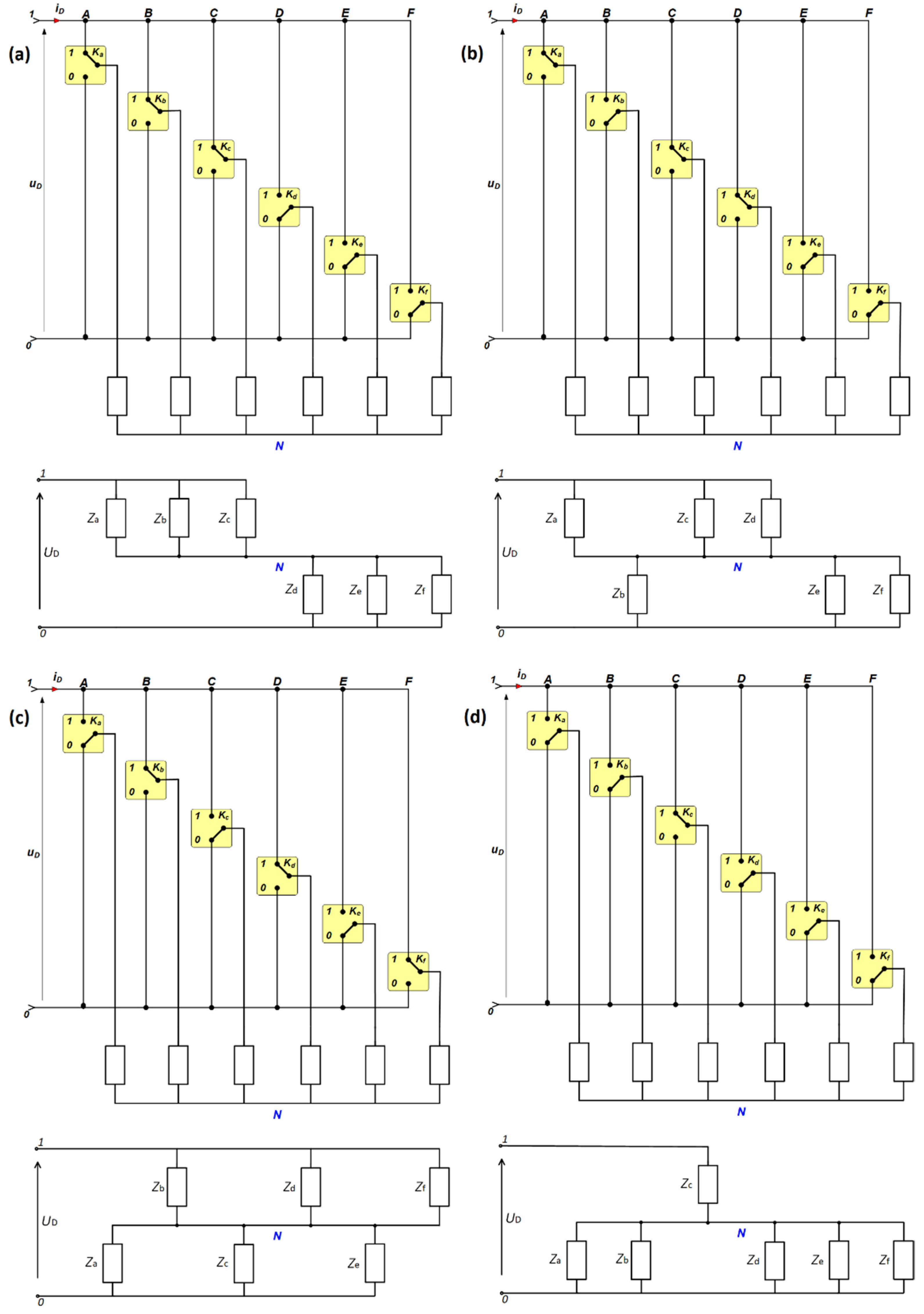
2.3. Star Connected Load of the Six-Phase Two-Level Inverter
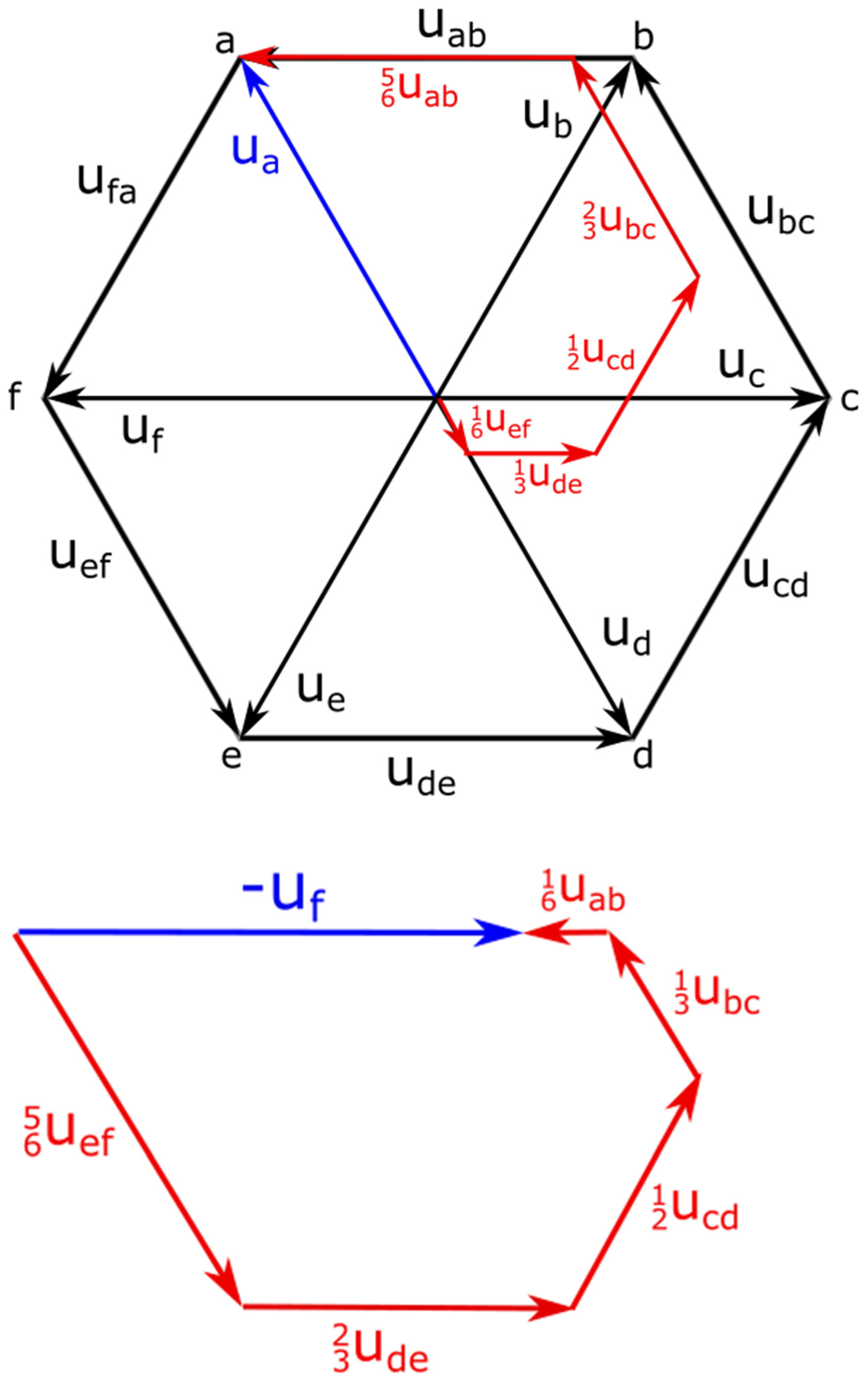
2.4. Polar Voltage Space Vectors of the Six-Phase Two-Level Voltage Source Inverter
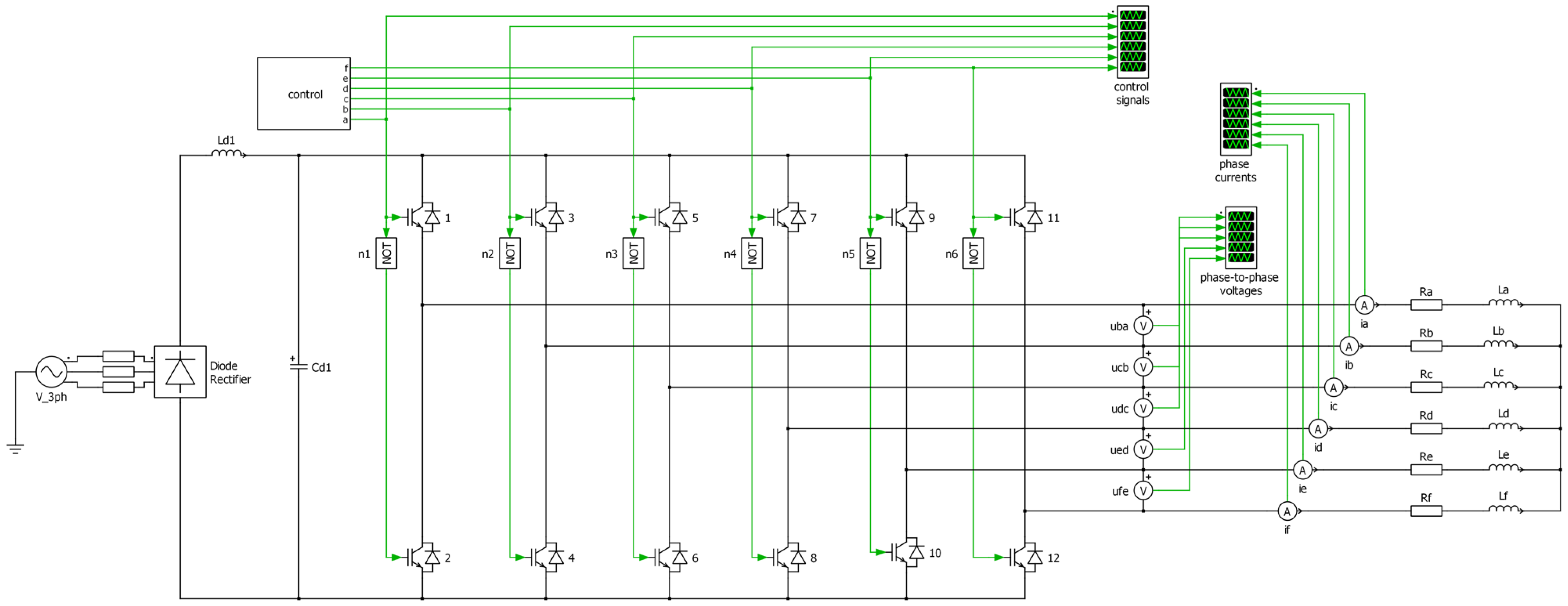
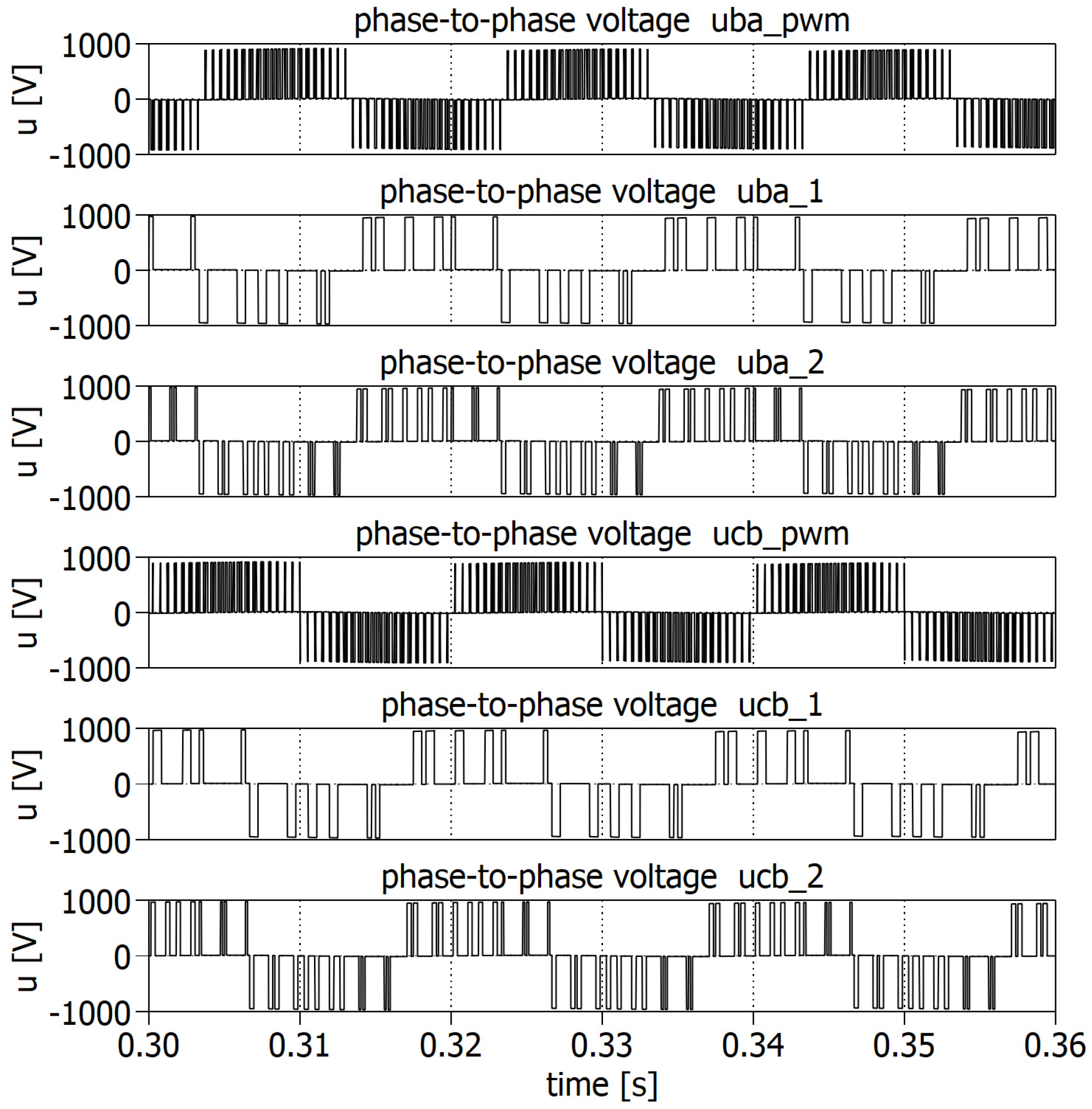
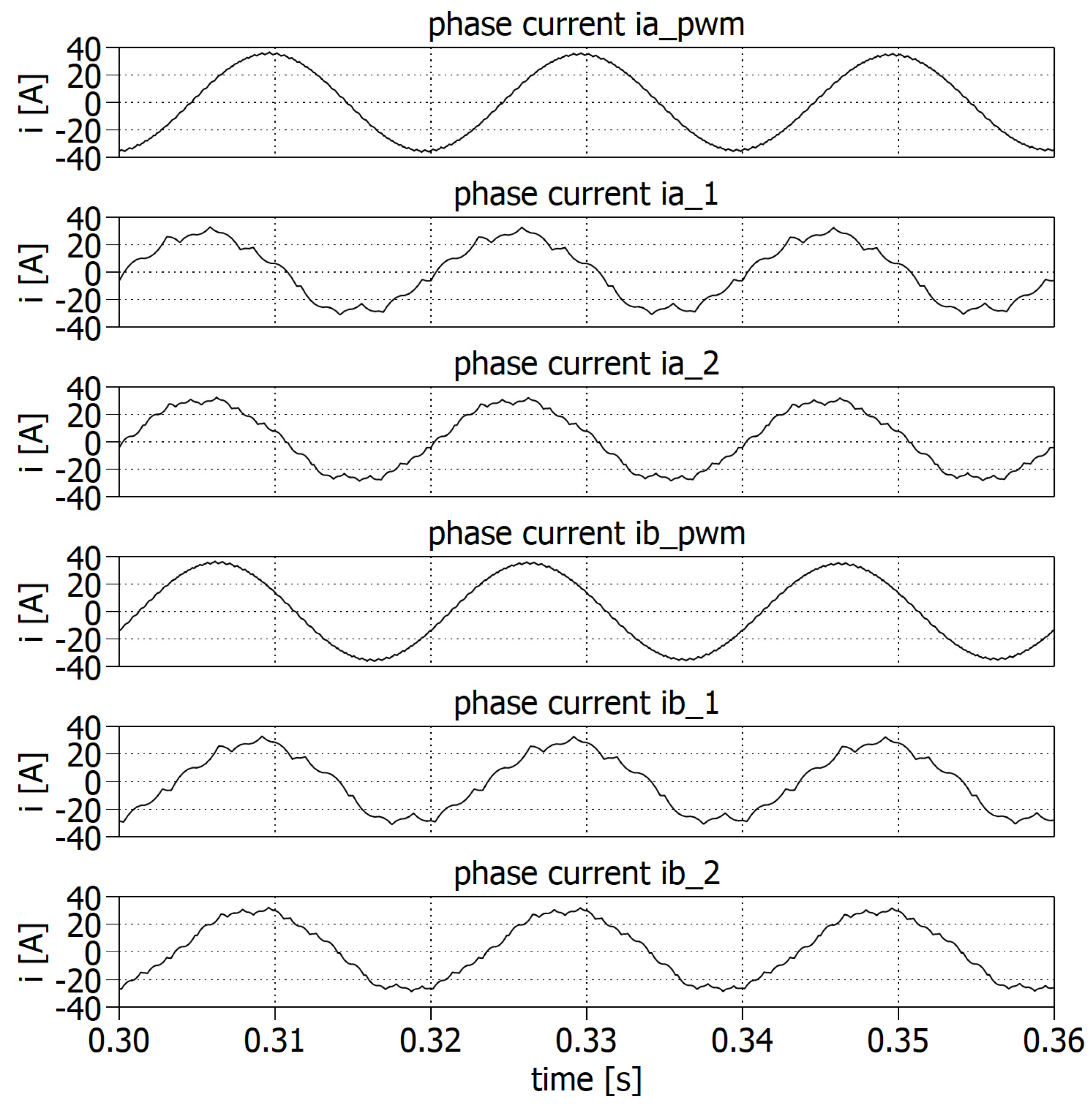
3. Simulation Experiment
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Listwan, J.; Pieńkowski, K. Field-Oriented Control of Five-Phase Induction Motor with Open-End Stator Winding. Arch. Electr. Eng. 2016, 65, 395–410. [Google Scholar]
- Levi, E.; Bojoi, R.; Profumo, F.; Toliyat, H.A.; Williamson, S. Multi-phase induction motor drives—A technology status review. IET Electr. Power Applications 2007, 1, 489–516. [Google Scholar] [CrossRef]
- Levi, E. Multi-phase electric machines for variable-speed applications. IEEE Transactions Ind. Electron. 2008, 55, 1893–1909. [Google Scholar] [CrossRef]
- Chavan, G.; Sridhar, S. Speed Control of Dual Induction Motor Using Five Leg Inverter. E3S Web Conf. 2020, 184, 01065. [Google Scholar] [CrossRef]
- Okayasu, M.; Sakai, K. Novel integrated motor design that supports phase and pole changes using multi-phase or single phase inverters. In Proceedings of the 2016 18th European Conference on Power Electronics and Applications (EPE’16 ECCE Europe), Karlsruhe, Germany, 5–9 September 2016; p. 135. [Google Scholar]
- Vasile, I.; Tudor, E.; Popescu, M.; Dumitru, C.; Popovici, L.; Sburlan, I.C. Electric Drives with Multi-phase Motors as a Better Solution for Traction Systems. In Proceedings of the 2019 11th International Symposium on Advanced Topics in Electrical Engineering (ATEE), Bucharest, Romania, 28–30 March 2019. [Google Scholar]
- Jakubiec, B. Multi-phase Permanent Magnet Synchronous Motor Drive for Electric Vehicle. Przegląd Elektrotechniczny 2015, 91, 125–128. [Google Scholar]
- Meinguet, F.; Nguyen, N.-K.; Sandulescu, P.; Kestelyn, X.; Semail, E. Fault-Tolerant Operation of an Open-End Winding Five-Phase PMSM Drive with Inverter. In Proceedings of the IECON 39th Annual Conference of the IEEE Industrial Electronics Society, Singapore, 18–21 October 2013. [Google Scholar]
- Omata, R.; Oka, K.; Furuya, A.; Matsumoto, S.; Nozawa, Y.; Matsuse, K. An Improved Performance of Five-Leg Inverter in Two Induction Motor Drives. In Proceedings of the 2006 CES/IEEE 5th International Power Electronics and Motion Control Conference, Shanghai, China, 14–16 August 2006. [Google Scholar]
- Ruba, M.; Surdu, F.; Szabo, L. Study of a Nine-Phase Fault Tolerant Permanent Magnet Starter-Alternator. J. Comput. Sci. Control Syst. 2011, 4, 149–154. [Google Scholar]
- Farsadi, M.; Kalashani, M.B. A novel hybrid multi-phase multilevel inverter topology. In Proceedings of the 10th International Conference on Electrical and Electronics Engineering (ELECO), Bursa, Turkey, 30 November–2 December 2017; pp. 305–308. [Google Scholar]
- Muqorobin, A.; Dahono, P.A.; Purwadi, A. Optimum phase number for multi-phase PWM inverters. In Proceedings of the 2017 4th International Conference on Electrical Engineering, Computer Science and Informatics (EECSI), Yogyakarta, Indonesia, 19–21 September 2017; pp. 1–6. [Google Scholar]
- Vitale, G.P.; Pipitone, E. A Six Legs Buck-boost Interleaved Converter for KERS Application. In Proceedings of the The International Conference ICREPQ’20, Granada, Spain, 8–10 April 2020. [Google Scholar]
- Furkan, A.; Yakup, T.; Enes, U.; Bulent, V.; Ismail, A. A bidirectional non-isolated multi-input DC–DC converter for hybrid energy storage systems in electric vehicles. IEEE Trans. Vehic. Technol. 2015, 65, 7944–7955. [Google Scholar]
- Padmanaban, S.; Hontz, M.R.; Khanna, R.; Wheeler, P.W.; Blaabjerg, F.; Ojo, J.O. Isolated/non-isolated quad-inverter configuration for multilevel symmetrical/asymmetrical dual six-phase star-winding converter. In Proceedings of the 2016 IEEE 25th International Symposium on Industrial Electronics (ISIE), Santa Clara, CA, USA, 8–10 June 2016; pp. 498–503. [Google Scholar]
- Moinoddin, S.; Iqbal, A.; Abu-Rub, H.; Khan, M.R.; Ahmed, S.M. Three-Phase to Seven-Phase Power Converting Transformer. IEEE Trans. Energy Convers. 2012, 27, 3. [Google Scholar]
- Abdel-Rahim, O.; Funato, H.; Abu-Rub, H.; Ellabban, O. Multi-phase Wind Energy Generation with Direct Matrix Converter. In Proceedings of the 2014 IEEE International Conference on Industrial Technology (ICIT), Busan, Korea, 26 February–1 March 2014. [Google Scholar]
- Koptjaew, E.; Popkov, E. AC-multi-phase Adjustable Electric Drive with Two-channel Conversion. In Proceedings of the International Conference on Industrial Engineering Applications and Manufacturing, ICIEAM, Pilsen, Czech Republic, 23–26 July 2019. [Google Scholar]
- Gao, Z.; Lu, Q. A hybrid cascaded multilevel converter based on three-level cells for battery energy management applied in electric vehicles. IEEE Trans. Power Electron. 2018, 34, 7326–7349. [Google Scholar] [CrossRef]
- Malinowski, M.; Gopakumar, K.; Rodriguez, J.; Perez, M.A. A Survey on Cascaded Multilevel Inverters. IEEE Trans. Ind. Electron. 2010, 57, 2197–2206. [Google Scholar] [CrossRef]
- Sleszynski, W.; Cichowski, A.; Mysiak, P. Suppression of supply current harmonics of 18-pulse diode rectifier by series active power filter with lc coupling. Energies 2020, 13, 6060. [Google Scholar] [CrossRef]
- Hui, P.; Wang, J.; Shen, W.; Shi, D.; Huang, Y. Controllable regenerative braking process for hybrid battery–ultracapacitor electric drive systems. IET Power Electron. 2018, 11, 2507–2514. [Google Scholar]
- Łebkowski, A.; Koznowski, W. Analysis of the use of electric and hybrid drives on swath ships. Energies 2020, 13, 6486. [Google Scholar] [CrossRef]
- Rybczak, M.; Rak, A. Prototyping and simulation environment of ship motion control system. TransNav 2020, 14, 367–374. [Google Scholar]
- Łebkowski, A. Analysis of the Use of Electric Drive Systems for Crew Transfer Vessels Servicing Offshore Wind Farms. Energies 2020, 13, 1466. [Google Scholar] [CrossRef]
- Nguyen, N.; Oruganti, S.K.; Na, K.; Bien, F. An adaptive backward control battery equalization system for serially connected lithium-ion battery packs. IEEE Trans. Veh. Technol. 2014, 63, 3651–3660. [Google Scholar] [CrossRef]
- Listwan, J. Application of Super-Twisting Sliding Mode Controllers in Direct Field-Oriented Control System of Six-Phase Induction Motor: Experimental Studies. Power Electron. Drives 2018, 3, 23–34. [Google Scholar] [CrossRef][Green Version]
- Liu, Z.; Zheng, Z.; Sudhoff, S.D.; Gu, C.; Li, Y. Reduction of common-mode voltage in multi-phase two-level inverters using SPWM with phase shifted carriers. IEEE Trans. Power Electron. 2016, 31, 6631–6645. [Google Scholar] [CrossRef]
- Nie, Z.; Preindl, M.; Schofield, N. SVM strategies for multi-phase voltage source inverters. In Proceedings of the 8th IET International Conference on Power Electronics, Machines and Drives (PEMD 2016), Glasgow, UK, 19–21 April 2016; pp. 1–6. [Google Scholar]
- Ippisch, M.; Gerling, D. Analytic Calculation of Space Vector Modulation Harmonic Content for Multi-phase Inverters with Single Frequency Output. In Proceedings of the IECON 2019—45th Annual Conference of the IEEE Industrial Electronics Society, Lisbon, Portugal, 14–17 October 2019; pp. 6187–6193. [Google Scholar]
- Karugaba, S.; Ojo, O. A carrier-based PWM modulation technique for balanced and unbalanced reference voltages in multi-phase voltage-source inverters. IEEE Trans. Ind. Appl. 2012, 48, 2102–2109. [Google Scholar] [CrossRef]
- Wang, Y.; Li, Y. Generalized Theory of Phase-Shifted Carrier PWM for Cascaded H-Bridge Converters and Modular Multilevel Converters. IEEE J. Emerg. Sel. Top. Power Electron. 2016, 4, 589–605. [Google Scholar] [CrossRef]
- Vafakhah, B.; Salmon, J.; Knight, A.M. A New Space-Vector PWM with Optimal Switching Selection for Multilevel Coupled Inductor Inverters. IEEE Trans. Ind. Electron. 2010, 57, 2354–2364. [Google Scholar] [CrossRef]
- Dujic, D.; Jones, M.; Levi, E. Generalized space vector PWM for sinusoidal output voltage generation with multi-phase voltage source inverters. Int. J. Ind. Electron. And Drives 2009, 1, 1–13. [Google Scholar] [CrossRef]
- Grandi, G.; Serra, G.; Tani, A. Space Vector Modulation of a Six Phase VSI based on three-phase decomposition. In Proceedings of the 2008 International Symposium on Power Electronics, Electrical Drives, Automation and Motion, Sorrento, Italy, 11–13 June 2008; pp. 674–679. [Google Scholar]
- Iwaszkiewicz, J.; Muc, A. State and Space Vectors of the 5-Phase 2-Level VSI. Energies 2020, 13, 4385. [Google Scholar] [CrossRef]
- Iwaszkiewicz, J.; Wolski, L. State and Space Vectors—Complementary Description of the 2-level VSI. In Proceedings of the International Conference on Renewable Energies and Power Quality (ICREPQ’14), Cordoba, Spain, 8–10 April 2014. [Google Scholar]


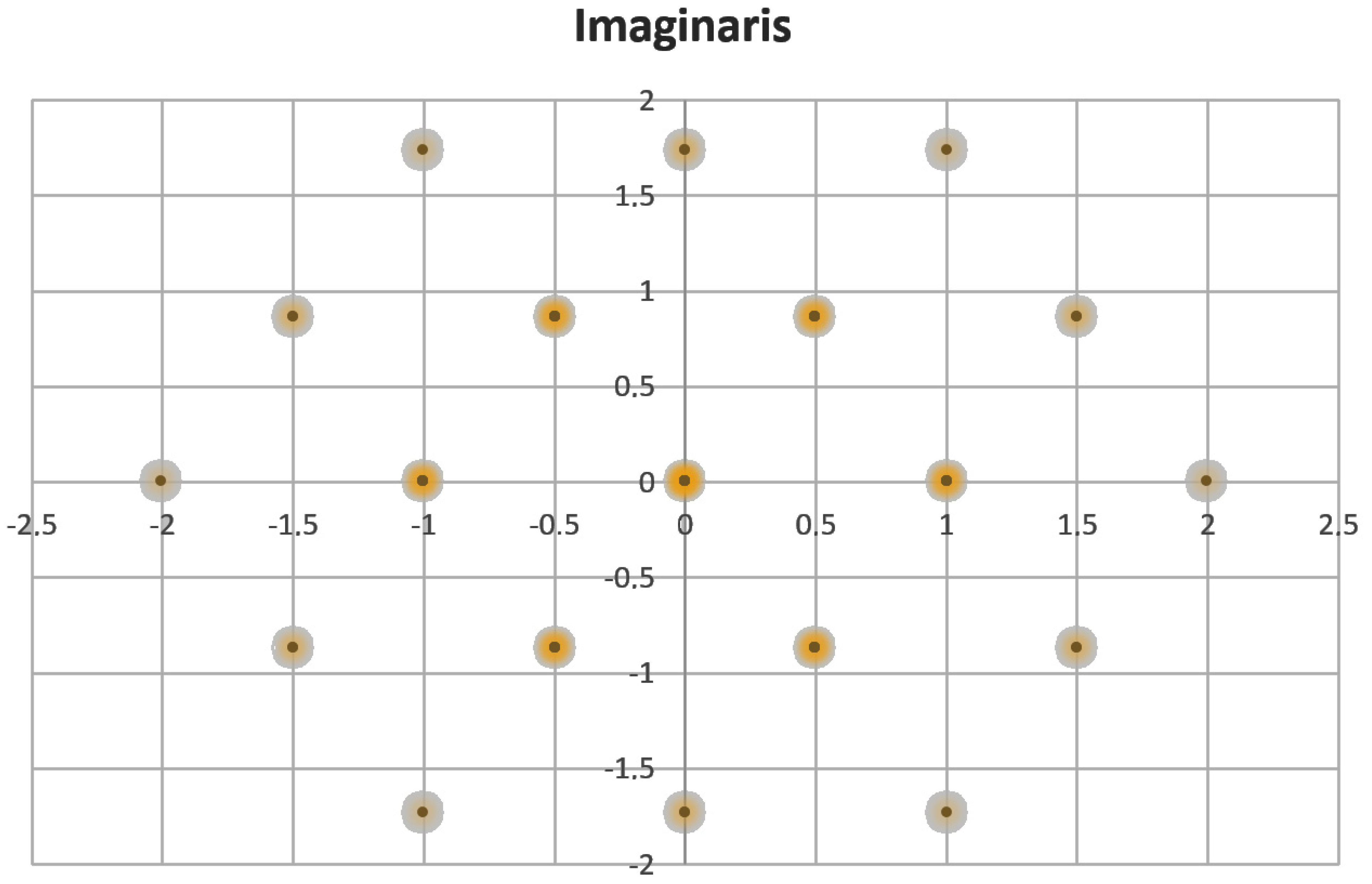
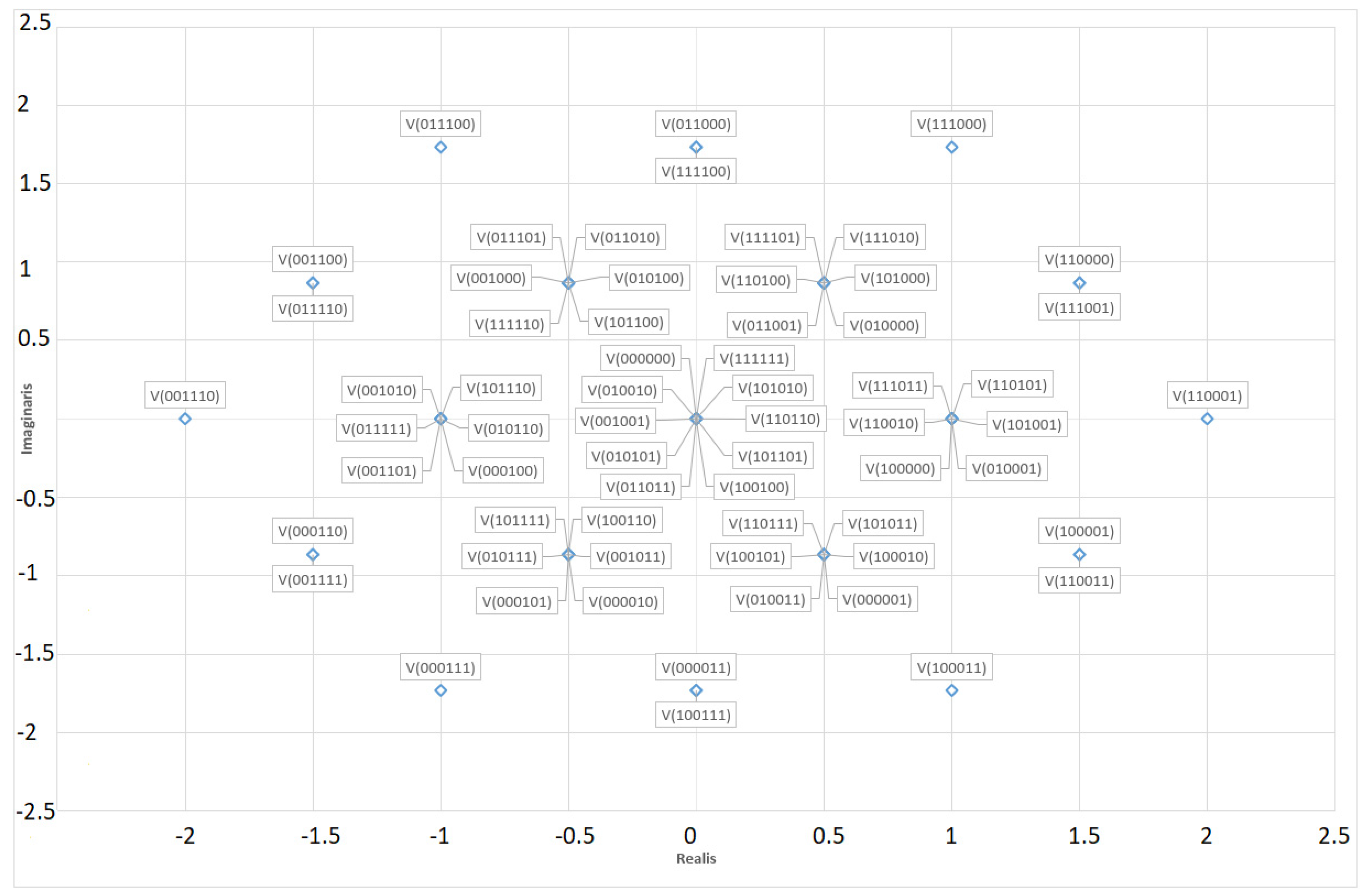
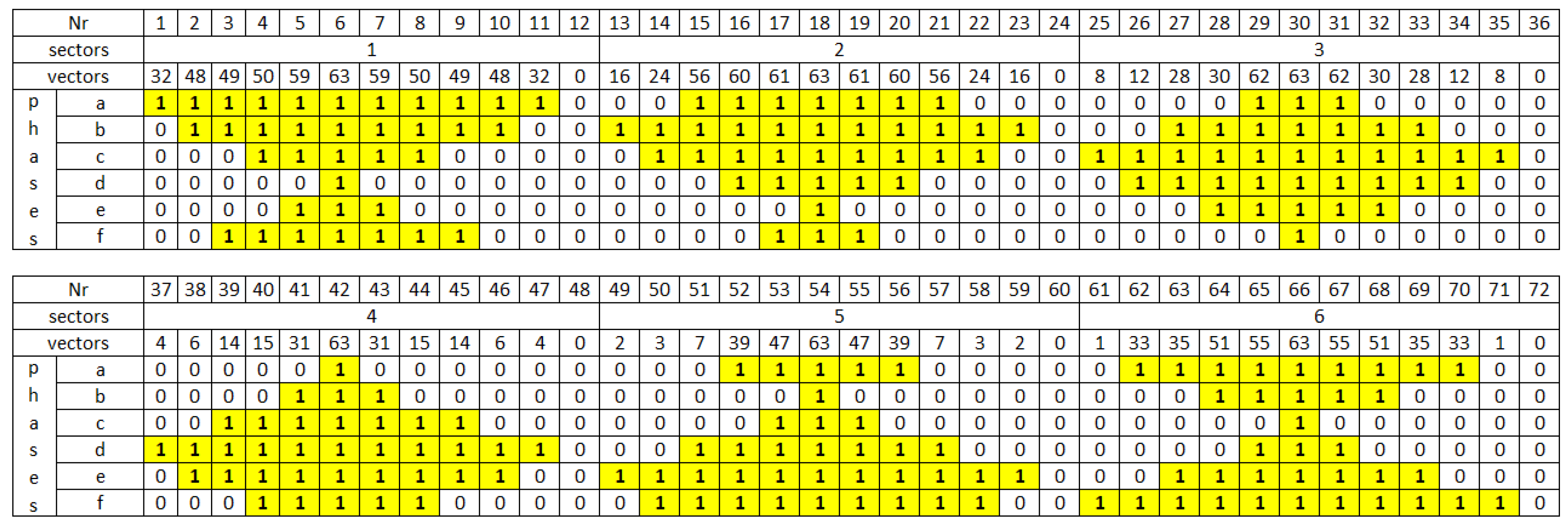
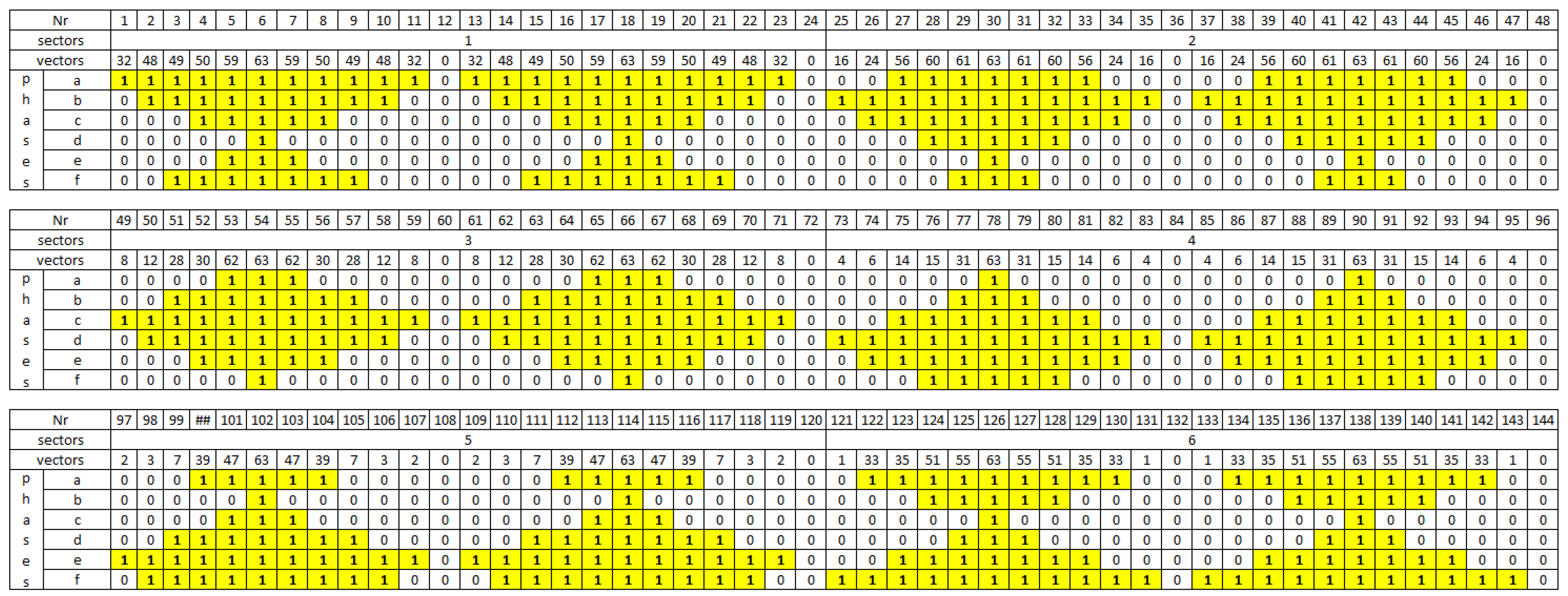

| Vk | a | b | c | d | e | f | Mk | φk | Vk | a | b | c | d | e | f | Mk | φk |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| V0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | * | V32 | 1 | 0 | 0 | 0 | 0 | 0 | 1 | 0 |
| V1 | 0 | 0 | 0 | 0 | 0 | 1 | 1 | 300 | V33 | 1 | 0 | 0 | 0 | 0 | 1 | 330 | |
| V2 | 0 | 0 | 0 | 0 | 1 | 0 | 1 | 240 | V34 | 1 | 0 | 0 | 0 | 1 | 0 | 1 | 300 |
| V3 | 0 | 0 | 0 | 0 | 1 | 1 | 270 | V35 | 1 | 0 | 0 | 0 | 1 | 1 | 2 | 300 | |
| V4 | 0 | 0 | 0 | 1 | 0 | 0 | 1 | 180 | V36 | 1 | 0 | 0 | 1 | 0 | 0 | 0 | * |
| V5 | 0 | 0 | 0 | 1 | 0 | 1 | 1 | 240 | V37 | 1 | 0 | 0 | 1 | 0 | 1 | 1 | 300 |
| V6 | 0 | 0 | 0 | 1 | 1 | 0 | 210 | V38 | 1 | 0 | 0 | 1 | 1 | 0 | 1 | 240 | |
| V7 | 0 | 0 | 0 | 1 | 1 | 1 | 2 | 240 | V39 | 1 | 0 | 0 | 1 | 1 | 1 | 270 | |
| V8 | 0 | 0 | 1 | 0 | 0 | 0 | 1 | 120 | V40 | 1 | 0 | 1 | 0 | 0 | 0 | 1 | 60 |
| V9 | 0 | 0 | 1 | 0 | 0 | 1 | 0 | * | V41 | 1 | 0 | 1 | 0 | 0 | 1 | 1 | 0 |
| V10 | 0 | 0 | 1 | 0 | 1 | 0 | 1 | 180 | V42 | 1 | 0 | 1 | 0 | 1 | 0 | 0 | * |
| V11 | 0 | 0 | 1 | 0 | 1 | 1 | 1 | 240 | V43 | 1 | 0 | 1 | 0 | 1 | 1 | 1 | 300 |
| V12 | 0 | 0 | 1 | 1 | 0 | 0 | 150 | V44 | 1 | 0 | 1 | 1 | 0 | 0 | 1 | 120 | |
| V13 | 0 | 0 | 1 | 1 | 0 | 1 | 1 | 180 | V45 | 1 | 0 | 1 | 1 | 0 | 1 | 0 | * |
| V14 | 0 | 0 | 1 | 1 | 1 | 0 | 2 | 180 | V46 | 1 | 0 | 1 | 1 | 1 | 0 | 1 | 180 |
| V15 | 0 | 0 | 1 | 1 | 1 | 1 | 210 | V47 | 1 | 0 | 1 | 1 | 1 | 1 | 1 | 240 | |
| V16 | 0 | 1 | 0 | 0 | 0 | 0 | 1 | 60 | V48 | 1 | 1 | 0 | 0 | 0 | 0 | 30 | |
| V17 | 0 | 1 | 0 | 0 | 0 | 1 | 1 | 0 | V49 | 1 | 1 | 0 | 0 | 0 | 1 | 2 | 0 |
| V18 | 0 | 1 | 0 | 0 | 1 | 0 | 0 | * | V50 | 1 | 1 | 0 | 0 | 1 | 0 | 1 | 0 |
| V19 | 0 | 1 | 0 | 0 | 1 | 1 | 1 | 300 | V51 | 1 | 1 | 0 | 0 | 1 | 1 | 330 | |
| V20 | 0 | 1 | 0 | 1 | 0 | 0 | 1 | 120 | V52 | 1 | 1 | 0 | 1 | 0 | 0 | 1 | 60 |
| V21 | 0 | 1 | 0 | 1 | 0 | 1 | 0 | * | V53 | 1 | 1 | 0 | 1 | 0 | 1 | 1 | 0 |
| V22 | 0 | 1 | 0 | 1 | 1 | 0 | 1 | 180 | V54 | 1 | 1 | 0 | 1 | 1 | 0 | 0 | * |
| V23 | 0 | 1 | 0 | 1 | 1 | 1 | 1 | 240 | V55 | 1 | 1 | 0 | 1 | 1 | 1 | 1 | 300 |
| V24 | 0 | 1 | 1 | 0 | 0 | 0 | 90 | V56 | 1 | 1 | 1 | 0 | 0 | 0 | 2 | 60 | |
| V25 | 0 | 1 | 1 | 0 | 0 | 1 | 1 | 60 | V57 | 1 | 1 | 1 | 0 | 0 | 1 | 30 | |
| V26 | 0 | 1 | 1 | 0 | 1 | 0 | 1 | 120 | V58 | 1 | 1 | 1 | 0 | 1 | 0 | 1 | 60 |
| V27 | 0 | 1 | 1 | 0 | 1 | 1 | 0 | * | V59 | 1 | 1 | 1 | 0 | 1 | 1 | 1 | 0 |
| V28 | 0 | 1 | 1 | 1 | 0 | 0 | 2 | 120 | V60 | 1 | 1 | 1 | 1 | 0 | 0 | 90 | |
| V29 | 0 | 1 | 1 | 1 | 0 | 1 | 1 | 120 | V61 | 1 | 1 | 1 | 1 | 0 | 1 | 1 | 60 |
| V30 | 0 | 1 | 1 | 1 | 1 | 0 | 150 | V62 | 1 | 1 | 1 | 1 | 1 | 0 | 1 | 120 | |
| V31 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | 180 | V63 | 1 | 1 | 1 | 1 | 1 | 1 | 0 | * |
| Vk | a | b | c | d | e | f |
|---|---|---|---|---|---|---|
| V21 | 0 | 1 | 0 | 1 | 0 | 1 |
| V42 | 1 | 0 | 1 | 0 | 1 | 0 |
| Three-Phase AC Drive | Three-Phase AC Drive | ||||||
|---|---|---|---|---|---|---|---|
| Vk | a | b | c | d | e | f | φk |
| V36 | 1 | 0 | 0 | 1 | 0 | 0 | 0 |
| V54 | 1 | 1 | 0 | 1 | 1 | 0 | 60° |
| V18 | 0 | 1 | 0 | 0 | 1 | 0 | 120° |
| V27 | 0 | 1 | 1 | 0 | 1 | 1 | 180° |
| V9 | 0 | 0 | 1 | 0 | 0 | 1 | 240° |
| V45 | 1 | 0 | 1 | 1 | 0 | 1 | 300° |
| Load | R = 2 Ω, L = 40 mH | |||||
|---|---|---|---|---|---|---|
| Control Strategy: | F | 40 | 45 | 50 | 55 | 60 |
| PWM | RMS | 23.9 | 24.3 | 24.0 | 23.5 | 22.8 |
| THDI | 1.4 | 1.3 | 1.3 | 1.4 | 1.5 | |
| 1 | RMS | 23.8 | 23.0 | 21.9 | 20.9 | 19.8 |
| THDI | 9.6 | 9.7 | 9.6 | 9.6 | 9.5 | |
| 2 | RMS | 24.1 | 23.2 | 22.2 | 21.0 | 19.9 |
| THDI | 5.5 | 5.5 | 5.4 | 5.4 | 5.4 | |
| Load | R = 2 Ω, L = 40 mH | |||||
|---|---|---|---|---|---|---|
| Control Strategy: | F | 40 | 45 | 50 | 55 | 60 |
| PWM | RMS | 27.7 | 26.7 | 25.5 | 24.3 | 23.1 |
| THDI | 2.1 | 2.3 | 2.6 | 2.8 | 3.1 | |
| 1 | RMS | 28.8 | 26.7 | 23.3 | 21.4 | 20.7 |
| THDI | 11.5 | 11.5 | 11.6 | 11.7 | 11.8 | |
| 2 | RMS | 29.2 | 26.6 | 24.3 | 23.1 | 21.9 |
| THDI | 7.4 | 7.4 | 7.5 | 7.5 | 7.4 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Iwaszkiewicz, J.; Muc, A.; Bielecka, A. Polar Voltage Space Vectors of the Six-Phase Two-Level VSI. Energies 2022, 15, 2763. https://doi.org/10.3390/en15082763
Iwaszkiewicz J, Muc A, Bielecka A. Polar Voltage Space Vectors of the Six-Phase Two-Level VSI. Energies. 2022; 15(8):2763. https://doi.org/10.3390/en15082763
Chicago/Turabian StyleIwaszkiewicz, Jan, Adam Muc, and Agata Bielecka. 2022. "Polar Voltage Space Vectors of the Six-Phase Two-Level VSI" Energies 15, no. 8: 2763. https://doi.org/10.3390/en15082763
APA StyleIwaszkiewicz, J., Muc, A., & Bielecka, A. (2022). Polar Voltage Space Vectors of the Six-Phase Two-Level VSI. Energies, 15(8), 2763. https://doi.org/10.3390/en15082763






