Fast Terminal Synergetic Control of PMVG-Based Wind Energy Conversion System for Enhancing the Power Extraction Efficiency
Abstract
:1. Introduction
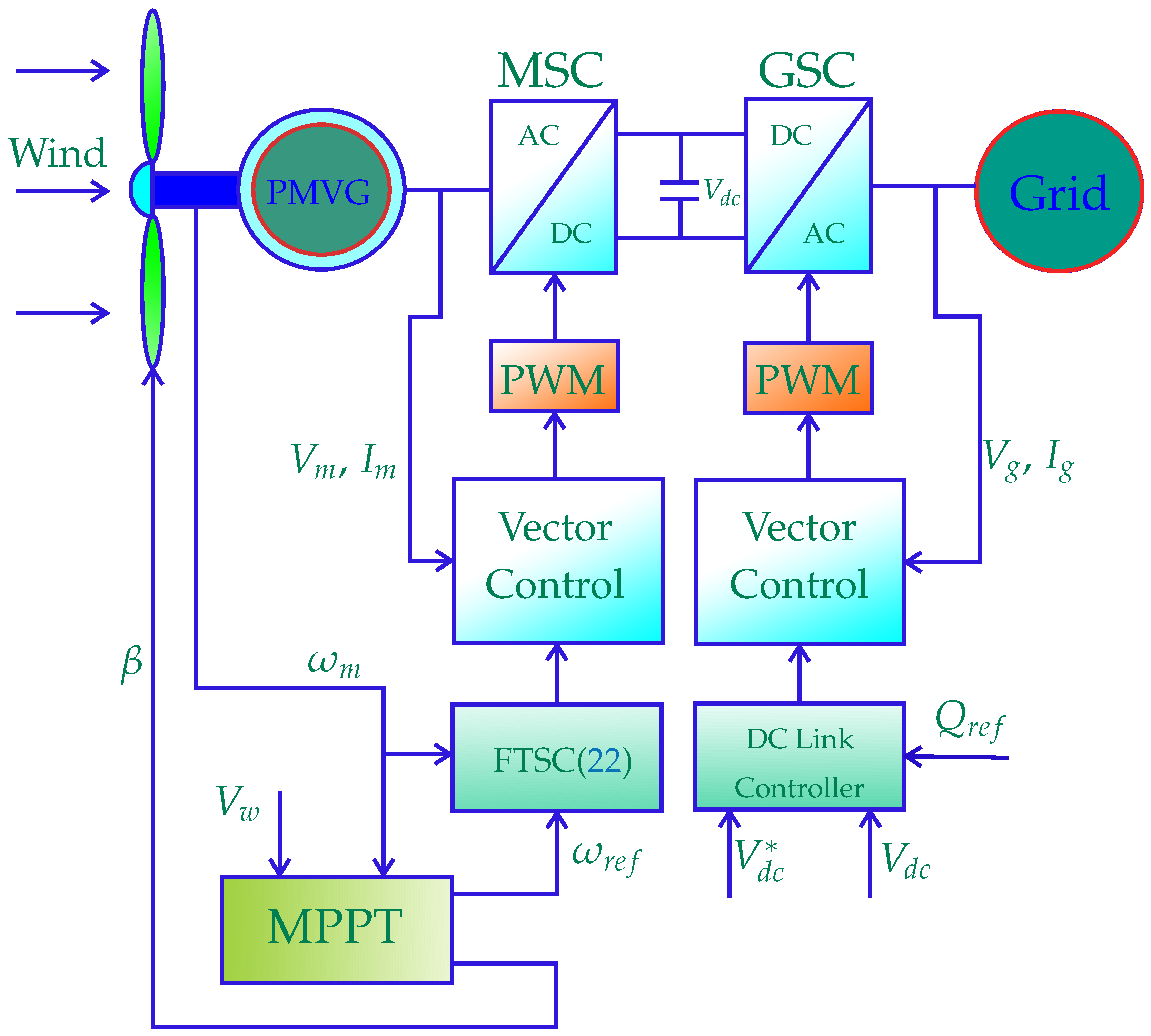
- The FSTC is presented with an integral macro variable to track the operating speed of PMVG to the optimum reference speed given by the TSR-based MPE scheme in MSC.
- The closed-loop FTSC with a macro variable and novel reaching law is presented to enhance the convergence rate of the error signal in finite time. Then, a zero chattering torque reference is generated to operate the system below and above rated wind conditions.
- The proposed method is validated by implementing it in the speed control loop of a 5 kW PMVG-based WECS in a simulation and experimental setup for two different wind speed conditions.
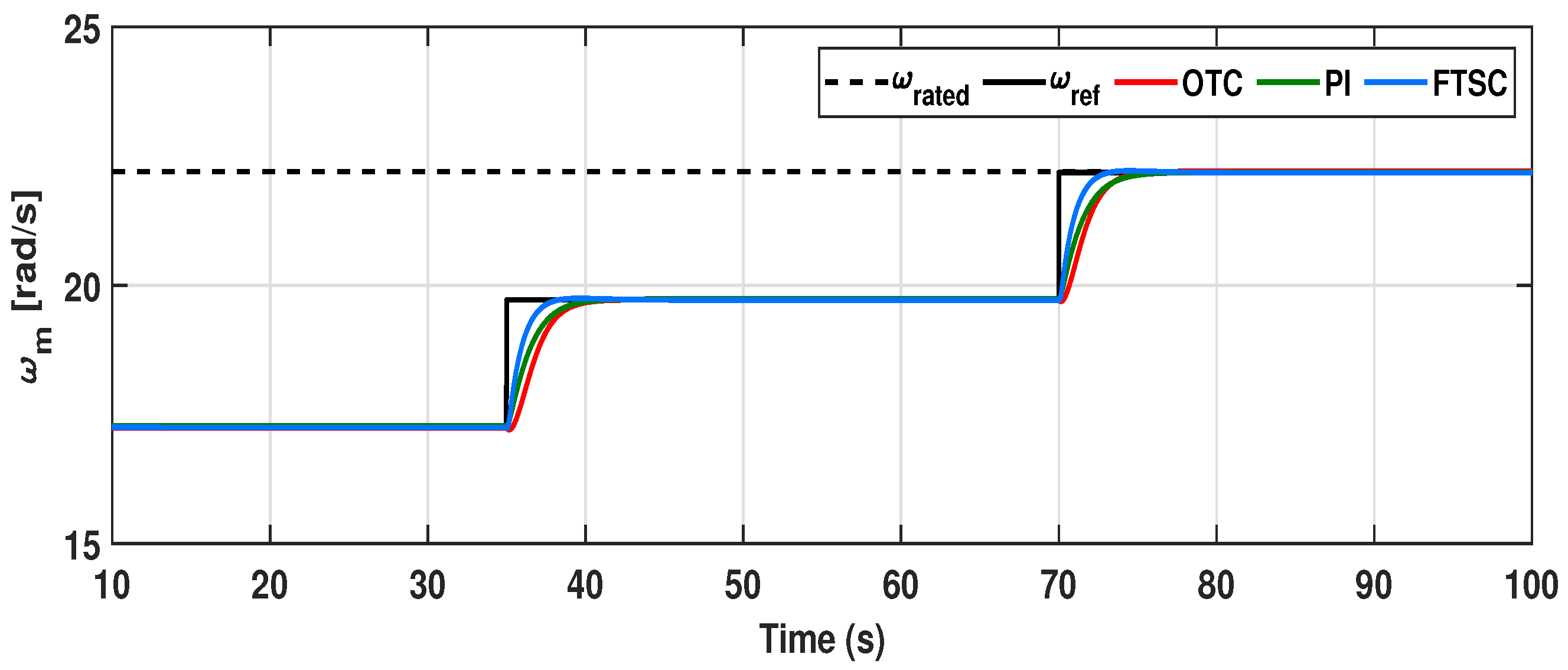
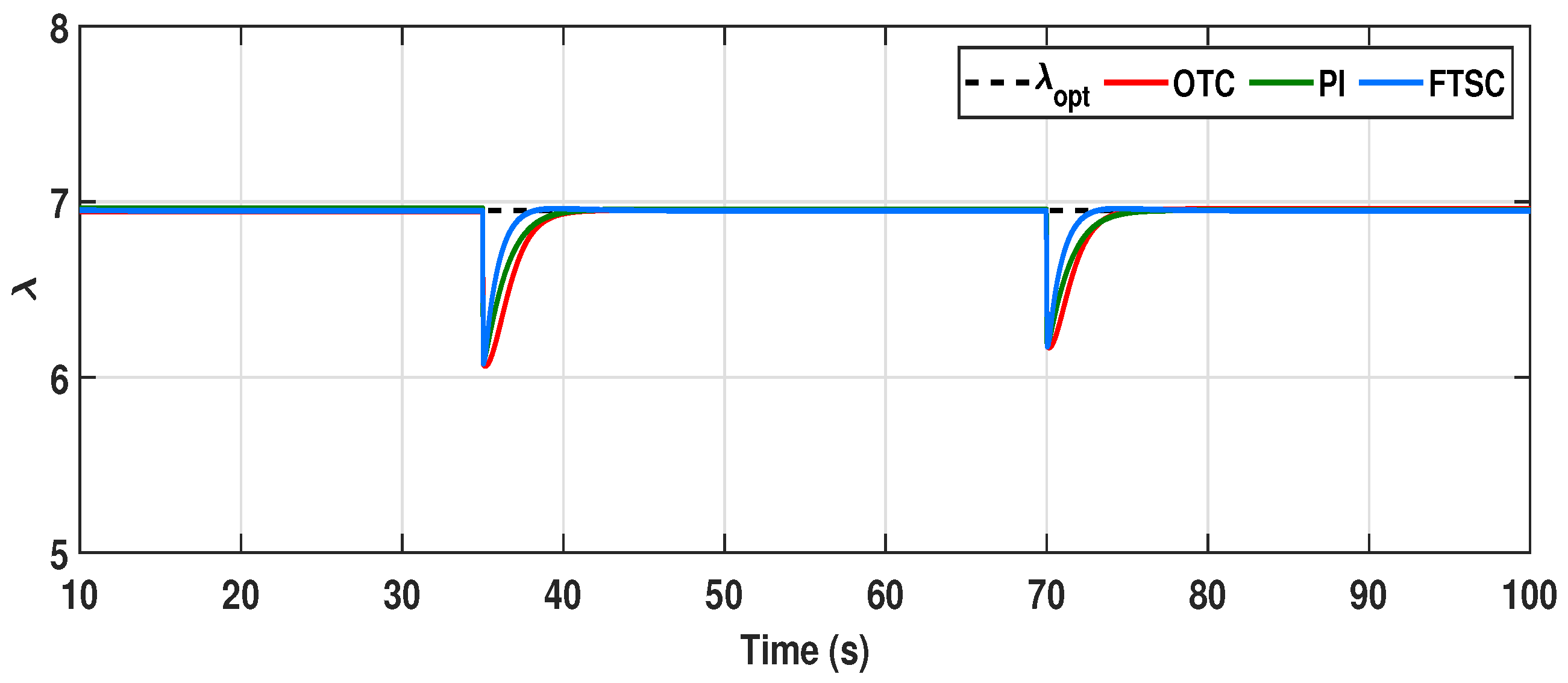
- Finally, the performance evaluation of the proposed FTSC is compared with conventional OTC and PI controllers [24] by analyzing the power extraction efficiencies of the PMVG-based WECS.
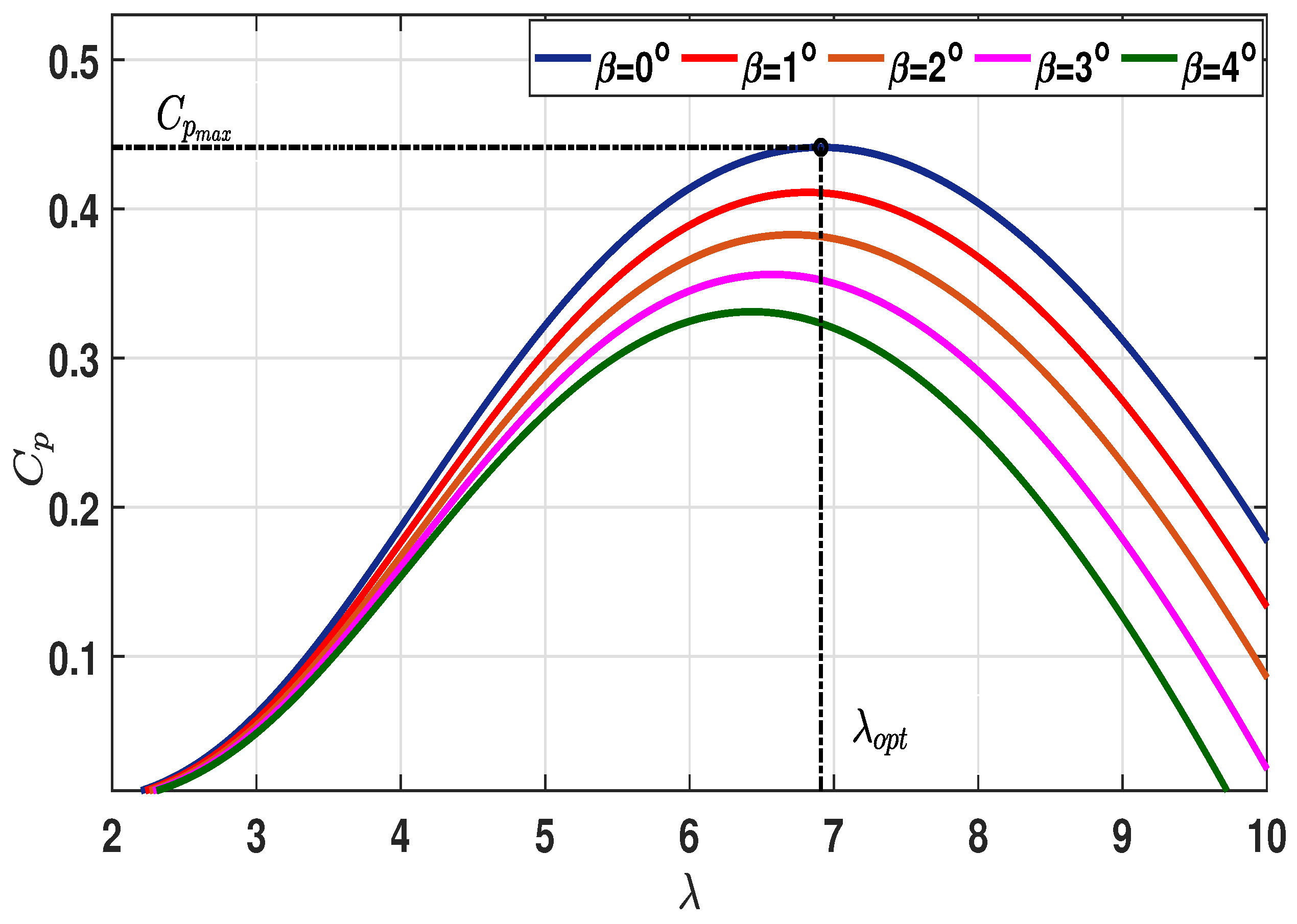
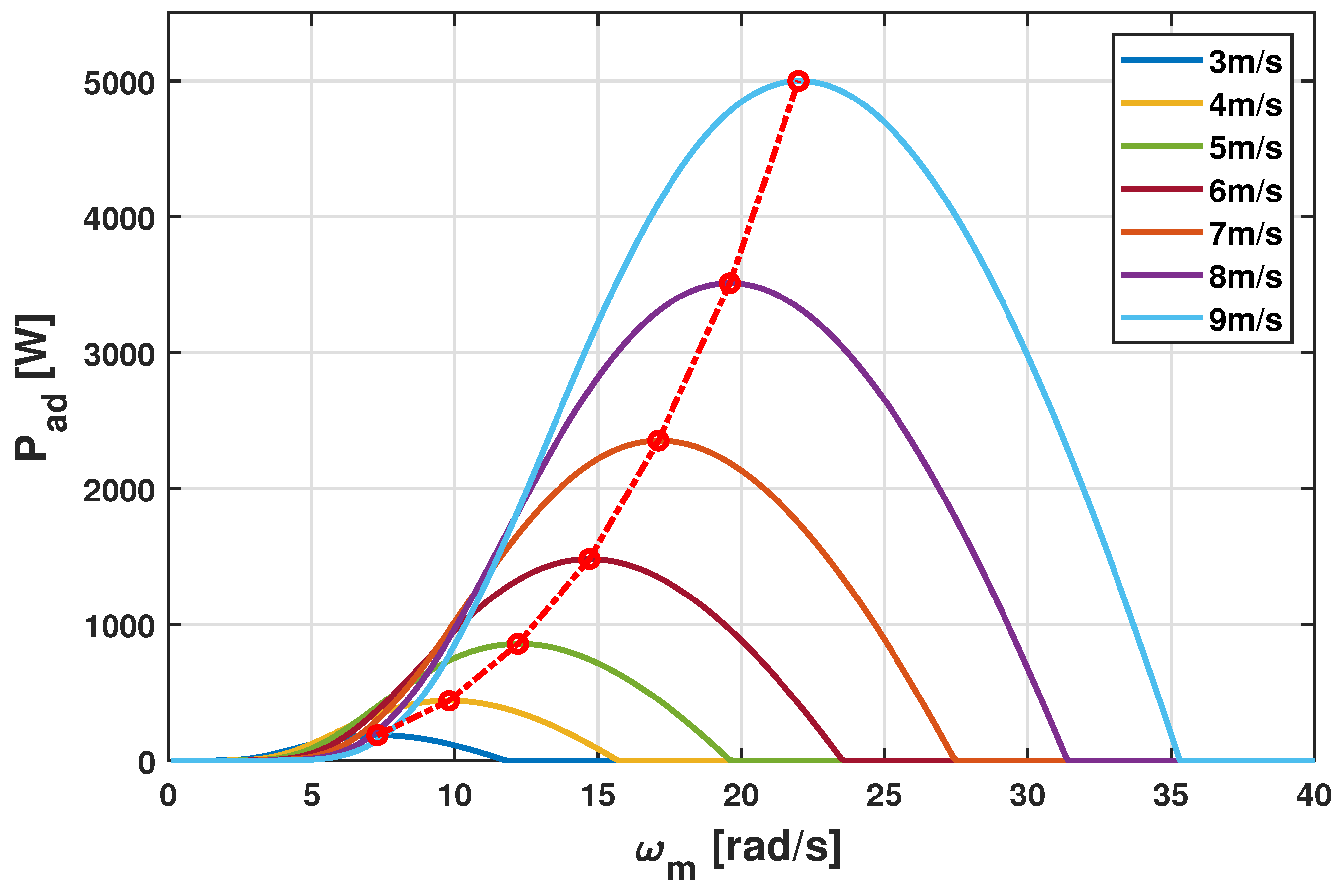
2. Wind Turbine Modeling
Dynamical Modeling of PMVG
3. Maximum Power Extraction Control Techniques for VS WECS
3.1. Conventional Optimum Torque Control Scheme
3.2. Synergetic Control
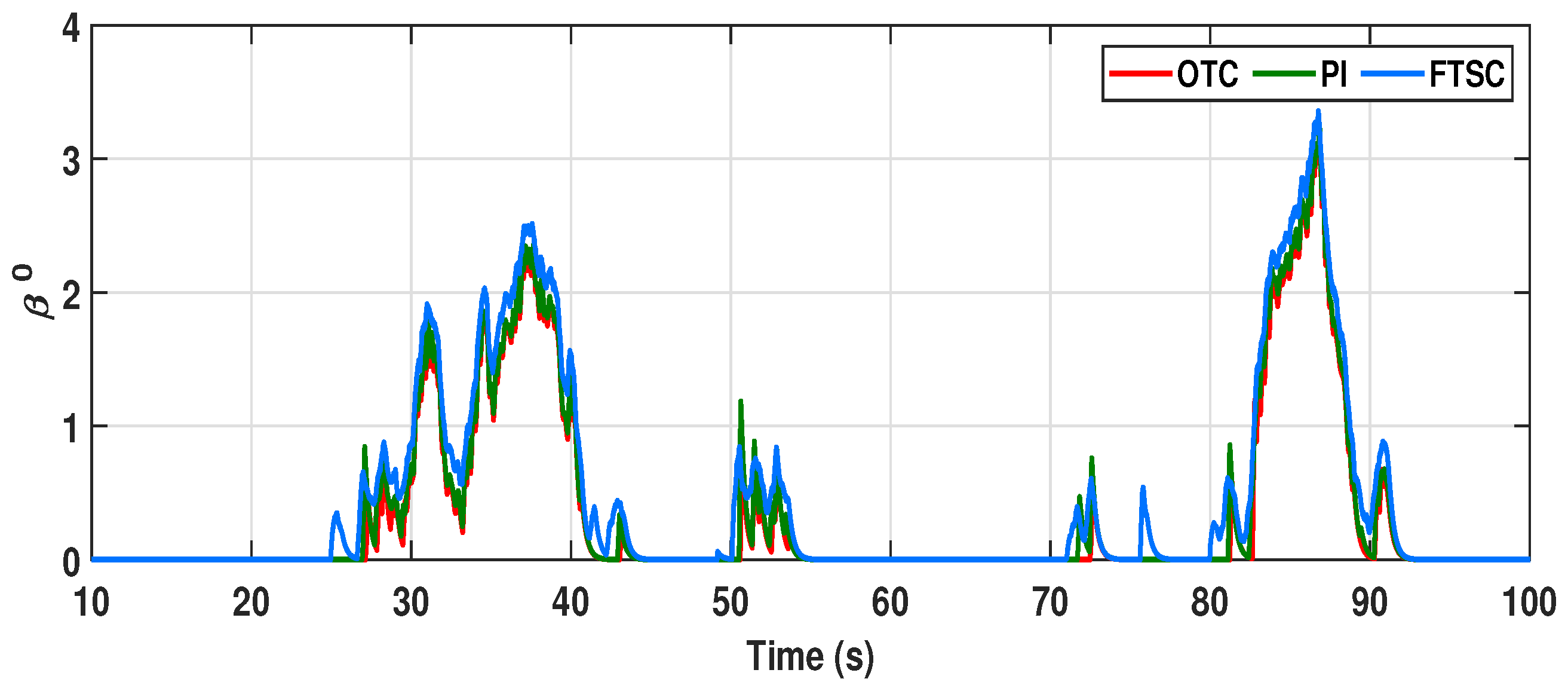
3.3. Fast Terminal Synergetic Control
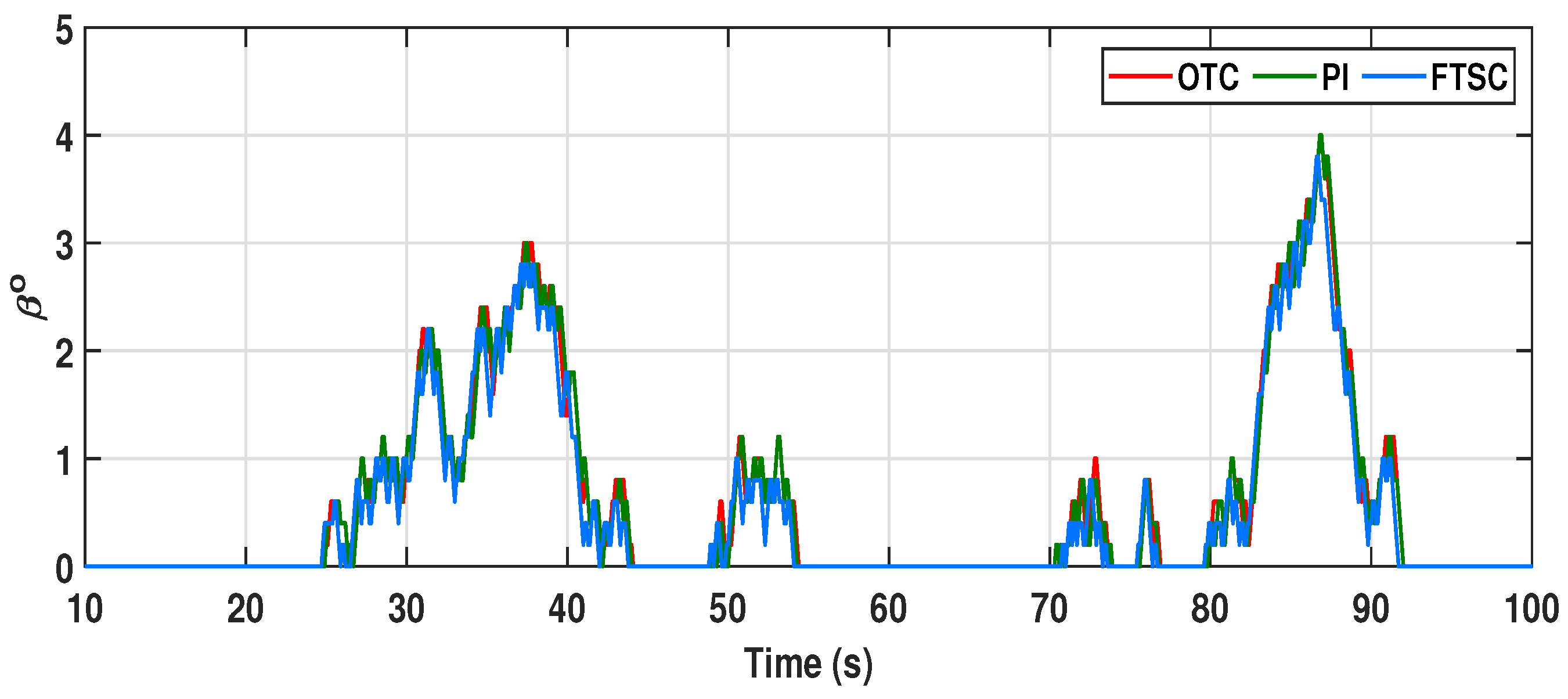
4. Performance Evaluation by Simulation and Experimentation Results
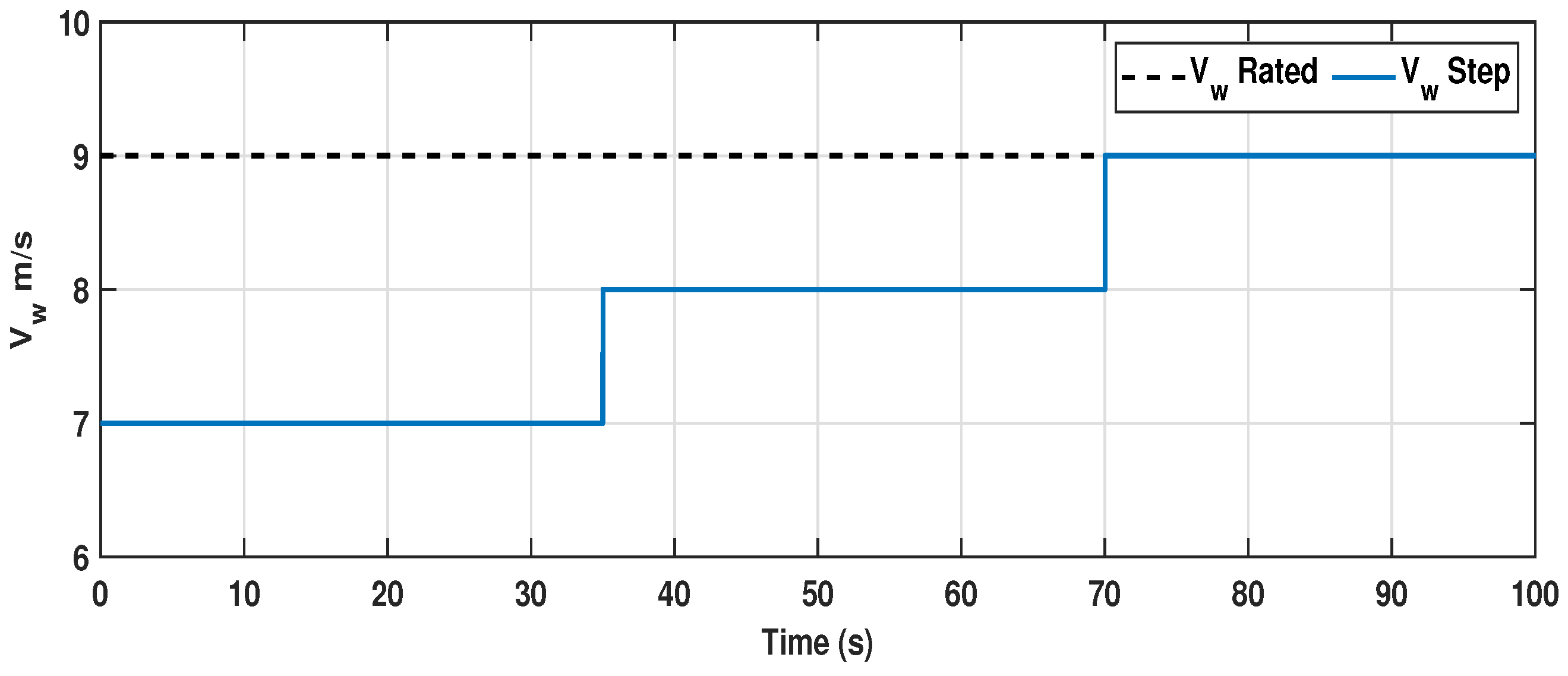
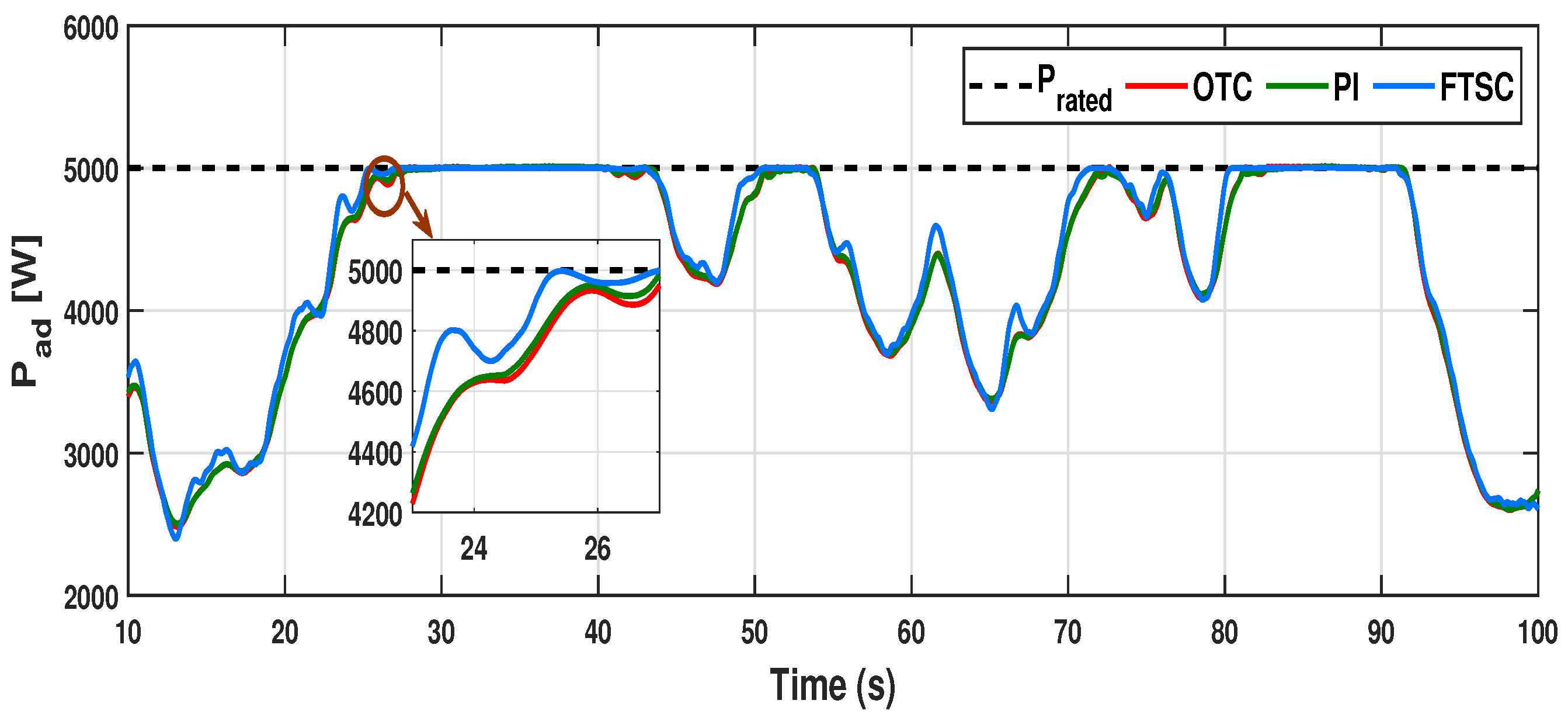
4.1. Simulation Results for Step Change in Wind Speed
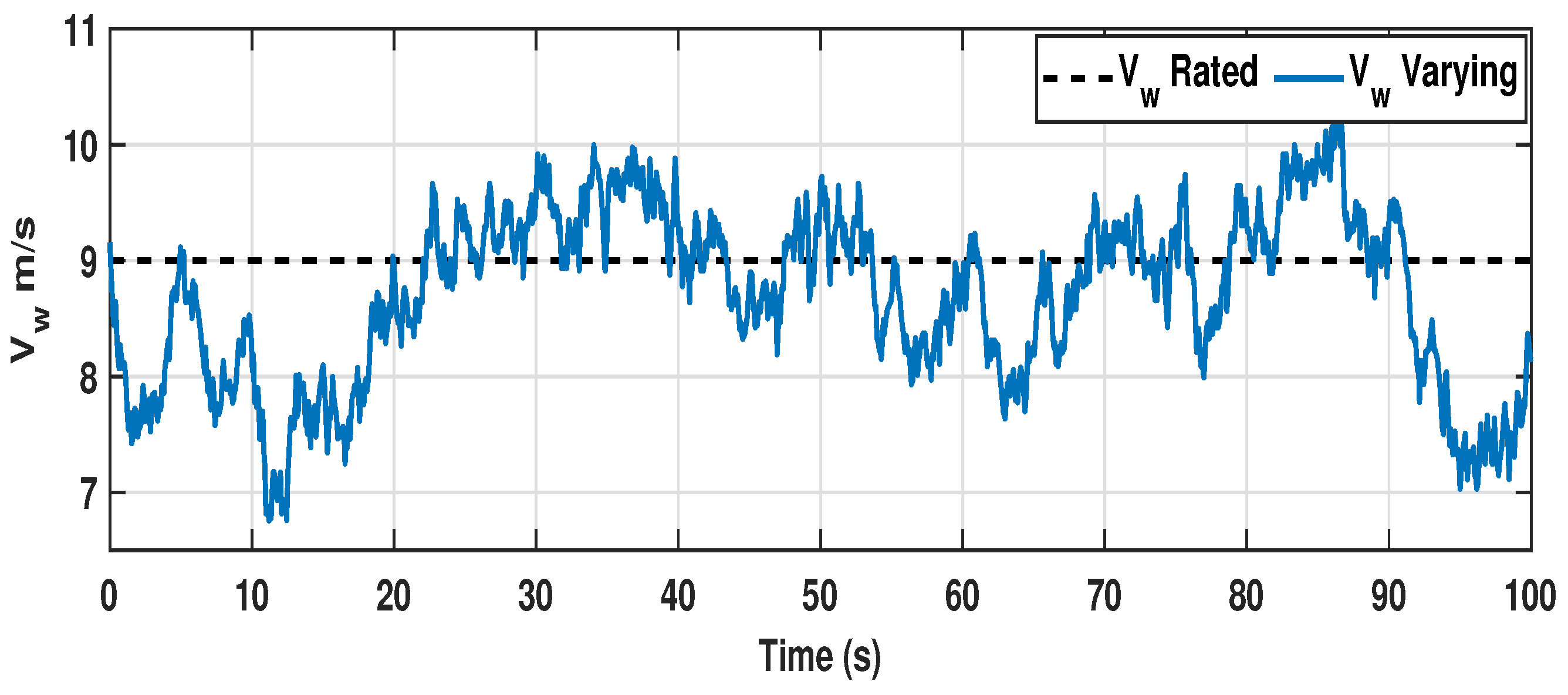
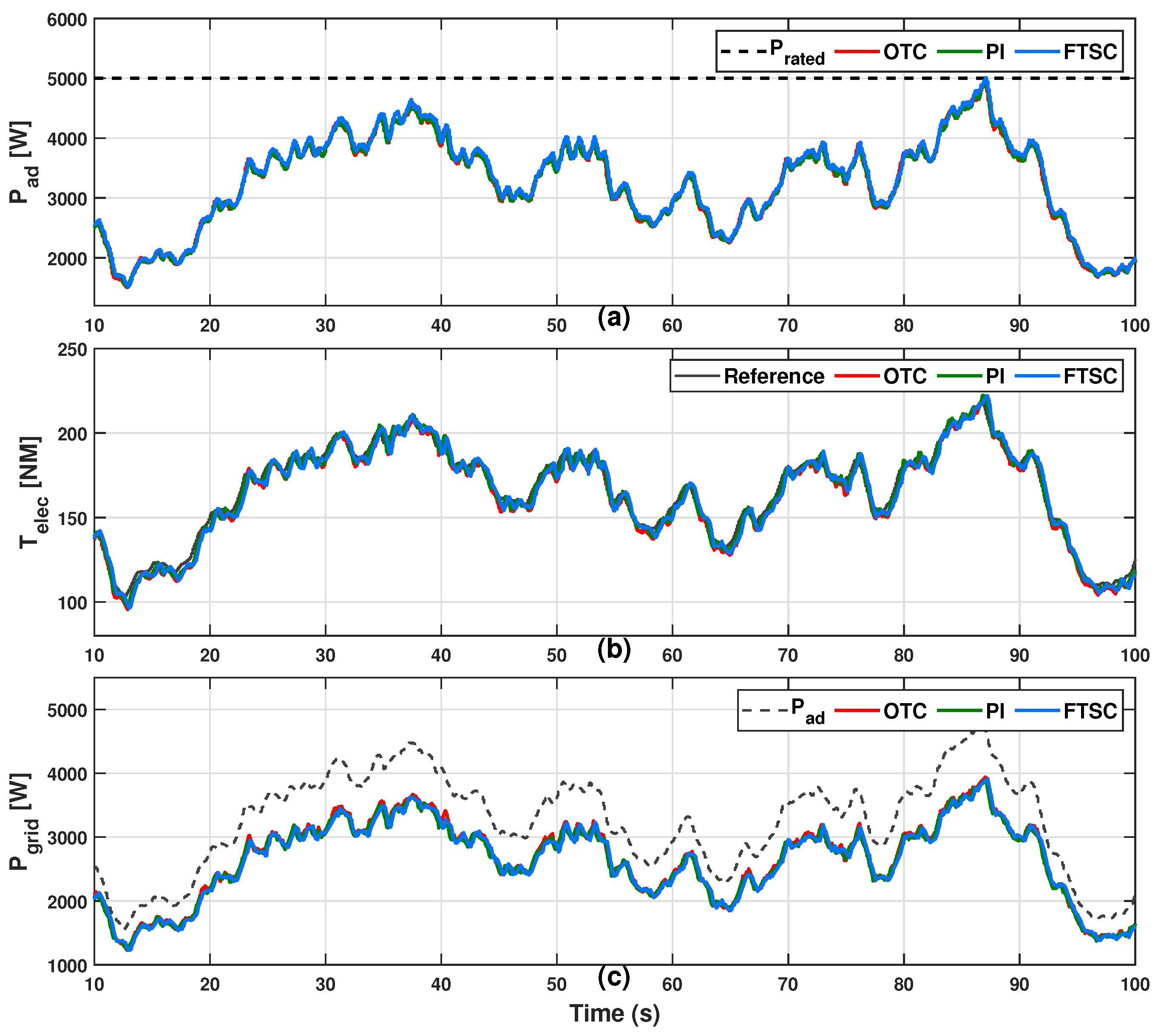
4.2. Simulation Results for Random Change in Wind Speed (Case-I)
4.3. Simulation Results for Random Change in Wind Speed (Case-II)
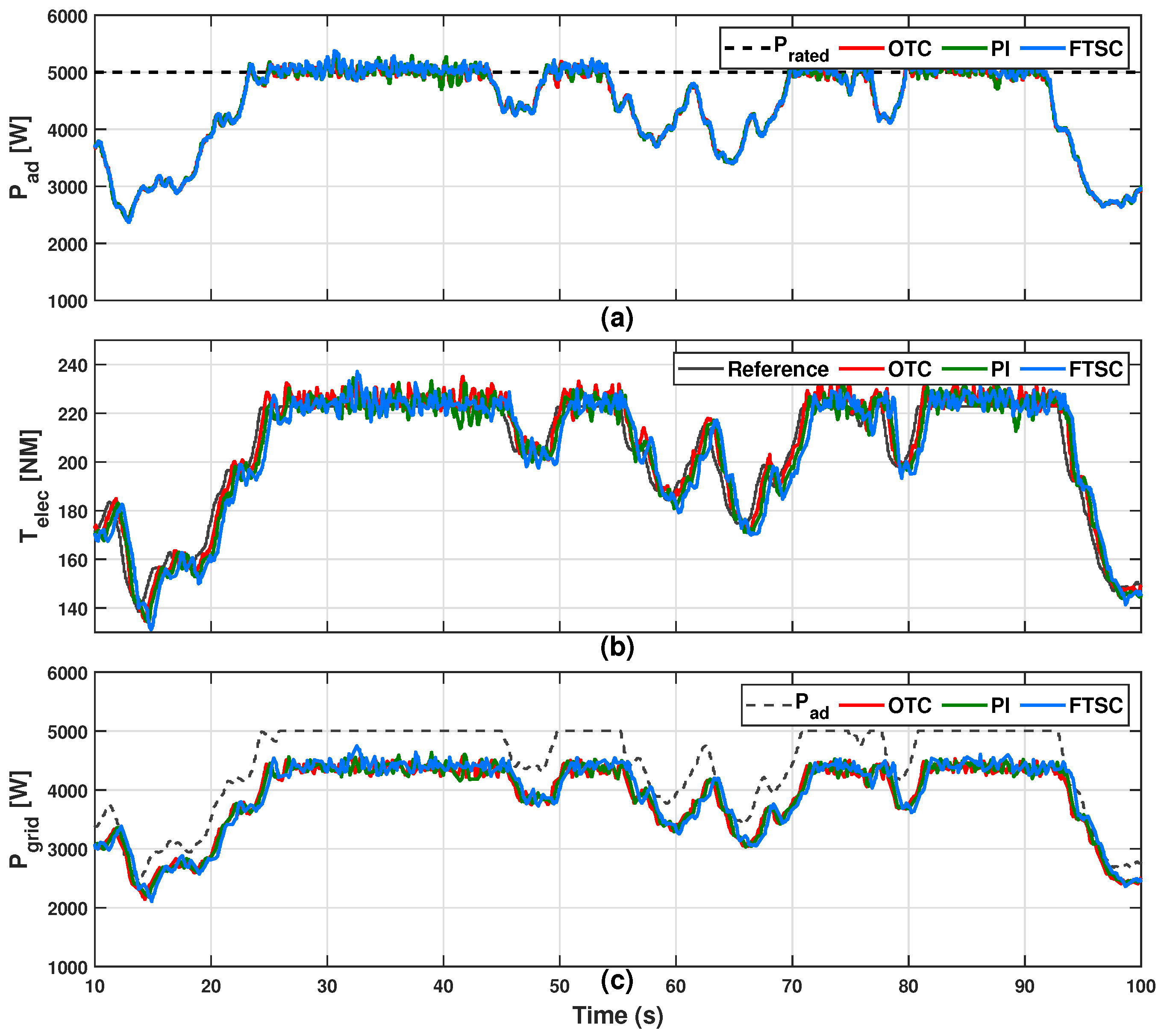
4.4. Experimentation Results for Random Change in Wind Speed (Case-I)
| 10 | 0.4 | mH | wb | 415 F |
| R | B | ||||
|---|---|---|---|---|---|
| 5 kW | 1.225 kg/m | m | 7 | N.m.s |
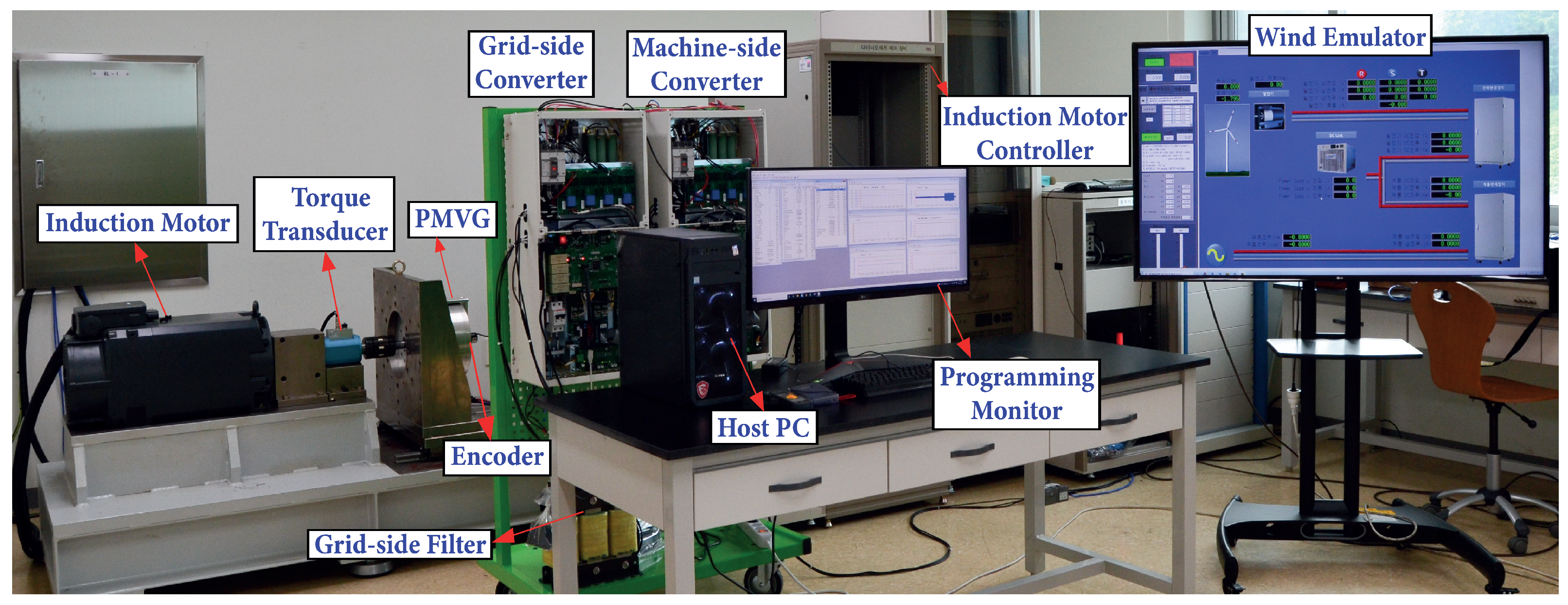
4.5. Experimentation Results for Random Change in Wind Speed (Case-II)
5. Quantitative Analysis for Power Extraction Efficiency
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| FTSC | Fast terminal synergetic control |
| PMVG | Permanent magnet vernier generator |
| WECS | Wind energy conversion system |
| VS | Variable speed |
| WT | Wind turbine |
| MPPT | Maximum power point tracking |
| SCIG | Squirrel cage induction generator |
| DFIG | Doubly fed induction generator |
| DD | Direct drive |
| PM | Permanent magnet |
| PMSG | Permanent magnet synchronous generator |
| MPE | Maximum power extraction |
| OTC | Optimum torque control |
| TSR | Tip speed ratio |
| FOPI | Fractional-order PI |
References
- Masters, G.M. Renewable and Efficient Electric Power Systems; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Elavarasan, R.M.; Shafiullah, G.; Padmanaban, S.; Kumar, N.M.; Annam, A.; Vetrichelvan, A.M.; Mihet-Popa, L.; Holm-Nielsen, J.B. A comprehensive review on renewable energy development, challenges, and policies of leading Indian states with an international perspective. IEEE Access 2020, 8, 74432–74457. [Google Scholar] [CrossRef]
- Newell, R.; Raimi, D.; Villanueva, S.; Prest, B. Global energy outlook 2021: Pathways from Paris. In Resources for the Future Report; Global Energy Outlook: Paris, France, 2021; pp. 11–21. [Google Scholar]
- Council, G.W.E. GWEC|Global Wind Report 2021; Global Wind Energy Council: Brussels, Belgium, 2021. [Google Scholar]
- Nelson, V. Wind Energy: Renewable Energy and the Environment; CRC Press: New York, NY, USA, 2009. [Google Scholar]
- Qais, M.H.; Hasanien, H.M.; Alghuwainem, S. A grey wolf optimizer for optimum parameters of multiple PI controllers of a grid-connected PMSG driven by variable speed wind turbine. IEEE Access 2018, 6, 44120–44128. [Google Scholar] [CrossRef]
- Wu, B.; Lang, Y.; Zargari, N.; Kouro, S. Power Conversion and Control of Wind Energy Systems; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Li, X.; Peng, T.; Dan, H.; Zhang, G.; Tang, W.; Jin, W.; Wheeler, P.; Rivera, M. A Modulated Model Predictive Control Scheme for the Brushless Doubly Fed Induction Machine. IEEE J. Emerg. Sel. Top. Power Electron. 2018, 6, 1681–1691. [Google Scholar] [CrossRef]
- Yaramasu, V.; Wu, B.; Sen, P.C.; Kouro, S.; Narimani, M. High-power wind energy conversion systems: State-of-the-art and emerging technologies. Proc. IEEE 2015, 103, 740–788. [Google Scholar] [CrossRef]
- Polinder, H.; Van der Pijl, F.F.; De Vilder, G.J.; Tavner, P.J. Comparison of direct-drive and geared generator concepts for wind turbines. IEEE Trans. Energy Convers. 2006, 21, 725–733. [Google Scholar] [CrossRef] [Green Version]
- Hansen, A.D.; Michalke, G. Multi-pole permanent magnet synchronous generator wind turbines’ grid support capability in uninterrupted operation during grid faults. IET Renew. Power Gener. 2009, 3, 333–348. [Google Scholar] [CrossRef]
- Yaramasu, V.; Wu, B. Model Predictive Control of Wind Energy Conversion Systems; John Wiley & Sons: Hoboken, NJ, USA, 2016. [Google Scholar]
- Kim, B. Design method of a direct-drive permanent magnet vernier generator for a wind turbine system. IEEE Trans. Ind. Appl. 2019, 55, 4665–4675. [Google Scholar] [CrossRef]
- Ghods, M.; Nasiri-Gheidari, Z.; Tootoonchian, F.; Oraee, H. Design improvement of a small, outer Rotor, permanent magnet vernier generator for supplying traffic enforcement camera. IEEE Trans. Energy Convers. 2018, 33, 1213–1221. [Google Scholar] [CrossRef]
- Padinharu, D.K.K.; Li, G.J.; Zhu, Z.Q.; Clark, R.; Thomas, A.S.; Azar, Z. System-level investigation of multi-MW direct-drive wind power PM vernier generators. IEEE Access 2020, 8, 191433–191446. [Google Scholar] [CrossRef]
- Padinharu, D.K.K.; Li, G.J.; Zhu, Z.Q.; Clark, R.; Thomas, A.; Azar, Z.; Duke, A. Permanent magnet vernier machines for direct-drive offshore wind power: Benefits and Challenges. IEEE Access 2022, 10, 20652–20668. [Google Scholar] [CrossRef]
- Basak, R.; Bhuvaneswari, G.; Pillai, R.R. Low-voltage ride-through of a synchronous generator-based variable speed grid-interfaced wind energy conversion system. IEEE Trans. Ind. Appl. 2019, 56, 752–762. [Google Scholar] [CrossRef]
- Gul, W.; Gao, Q.; Lenwari, W. Optimal design of a 5-MW double-stator single-rotor PMSG for offshore direct drive wind turbines. IEEE Trans. Ind. Appl. 2019, 56, 216–225. [Google Scholar] [CrossRef]
- Apata, O.; Oyedokun, D. An overview of control techniques for wind turbine systems. Sci. Afr. 2020, 10, e00566. [Google Scholar] [CrossRef]
- Hua, A.C.C.; Cheng, B.C.H. Design and implementation of power converters for wind energy conversion system. In Proceedings of the 2010 International Power Electronics Conference-ECCE ASIA, Sapporo, Japan, 21–24 June 2010; pp. 323–328. [Google Scholar]
- Carrillo, C.; Montaño, A.O.; Cidrás, J.; Díaz-Dorado, E. Review of power curve modelling for wind turbines. Renew. Sustain. Energy Rev. 2013, 21, 572–581. [Google Scholar] [CrossRef]
- Abdullah, M.; Yatim, A.; Tan, C. An online optimum-relation-based maximum power point tracking algorithm for wind energy conversion system. In Proceedings of the 2014 Australasian Universities Power Engineering Conference (AUPEC), Perth, WA, Australia, 28 September–1 October 2014; pp. 1–6. [Google Scholar]
- Hohm, D.; Ropp, M.E. Comparative study of maximum power point tracking algorithms. Prog. Photovolt. Res. Appl. 2003, 11, 47–62. [Google Scholar] [CrossRef]
- Fathabadi, H. Novel maximum electrical and mechanical power tracking controllers for wind energy conversion systems. IEEE J. Emerg. Sel. Top. Power Electron. 2017, 5, 1739–1745. [Google Scholar] [CrossRef]
- Nasiri, M.; Milimonfared, J.; Fathi, S. Modeling, analysis and comparison of TSR and OTC methods for MPPT and power smoothing in permanent magnet synchronous generator-based wind turbines. Energy Convers. Manag. 2014, 86, 892–900. [Google Scholar] [CrossRef]
- Abdullah, M.A.; Yatim, A.; Tan, C.W.; Saidur, R. A review of maximum power point tracking algorithms for wind energy systems. Renew. Sustain. Energy Rev. 2012, 16, 3220–3227. [Google Scholar] [CrossRef]
- Thongam, J.S.; Ouhrouche, M. MPPT control methods in wind energy conversion systems. Fundam. Adv. Top. Wind Power 2011, 15, 339–360. [Google Scholar]
- Yokoyama, H.; Tatsuta, F.; Nishikata, S. Tip speed ratio control of wind turbine generating system connected in series. In Proceedings of the 2011 International Conference on Electrical Machines and Systems, Beijing, China, 20–23 August 2011; pp. 1–4. [Google Scholar]
- Tonsing, B.; Vadhera, S.; Gupta, A.R. Comparative Analysis of Maximum Power Point Tracking Algorithms of Wind Energy Systems. In Proceedings of the 2019 International Conference on Computing, Power and Communication Technologies (GUCON), Greater Noida, India, 27–28 September 2019; pp. 337–342. [Google Scholar]
- Nadour, M.; Essadki, A.; Nasser, T. Comparative analysis between PI & backstepping control strategies of DFIG driven by wind turbine. Int. J. Renew. Energy Res. 2017, 7, 1307–1316. [Google Scholar]
- Azar, A.T.; Serrano, F.E.; Flores, M.A.; Kamal, N.A.; Ruiz, F.; Ibraheem, I.K.; Humaidi, A.J.; Fekik, A.; Alain, K.S.T.; Romanic, K.; et al. Fractional-order controller design and implementation for maximum power point tracking in photovoltaic panels. In Renewable Energy Systems; Azar, A.T., Kamal, N.A., Eds.; Advances in Nonlinear Dynamics and Chaos (ANDC); Academic Press: Cambridge, MA, USA, 2021; pp. 255–277. [Google Scholar] [CrossRef]
- Korayem, M.H.; Khademi, A.; Nekoo, S.R. A comparative study on SMC, OSMC and SDRE for robot control. In Proceedings of the 2014 Second RSI/ISM International Conference on Robotics and Mechatronics (ICRoM), Tehran, Iran, 15–17 October 2014; pp. 13–18. [Google Scholar] [CrossRef]
- Zerroug, N.; Harmas, M.N.; Benaggoune, S.; Bouchama, Z.; Zehar, K. DSP-based implementation of fast terminal synergetic control for a DC–DC Buck converter. J. Frankl. Inst. 2018, 355, 2329–2343. [Google Scholar] [CrossRef]
- Elnady, A.; Noureldin, A.; Adam, A.A. Integral terminal synergetic-based direct power control for distributed generation systems. IEEE Trans. Smart Grid 2022, 13, 1287–1297. [Google Scholar] [CrossRef]
- Soliman, M.A.; Hasanien, H.M.; Azazi, H.Z.; El-Kholy, E.E.; Mahmoud, S.A. An adaptive fuzzy logic control strategy for performance enhancement of a grid-connected PMSG-based wind turbine. IEEE Trans. Ind. Inform. 2019, 15, 3163–3173. [Google Scholar] [CrossRef]
- Periyanayagam, A.R.; Joo, Y.H. Integral sliding mode control for increasing maximum power extraction efficiency of variable-speed wind energy system. Int. J. Electr. Power Energy Syst. 2022, 139, 107958. [Google Scholar] [CrossRef]
- Kim, K.H.; Van, T.L.; Lee, D.C.; Song, S.H.; Kim, E.H. Maximum output power tracking control in variable-speed wind turbine systems considering rotor inertial power. IEEE Trans. Ind. Electron. 2012, 60, 3207–3217. [Google Scholar] [CrossRef]
- Kumar, D.; Chatterjee, K. A review of conventional and advanced MPPT algorithms for wind energy systems. Renew. Sustain. Energy Rev. 2016, 55, 957–970. [Google Scholar] [CrossRef]
- Jiang, Z.; Dougal, R. Synergetic control of power converters for pulse current charging of advanced batteries from a fuel cell power source. IEEE Trans. Power Electron. 2004, 19, 1140–1150. [Google Scholar] [CrossRef] [Green Version]
- Chen, Z.; Yuan, X.; Wu, X.; Yuan, Y.; Lei, X. Global fast terminal sliding mode controller for hydraulic turbine regulating system with actuator dead zone. J. Frankl. Inst. 2019, 356, 8366–8387. [Google Scholar] [CrossRef]
- Tang, X.; Yin, M.; Shen, C.; Xu, Y.; Dong, Z.Y.; Zou, Y. Active power control of wind turbine generators via coordinated rotor speed and pitch angle regulation. IEEE Trans. Sustain. Energy 2018, 10, 822–832. [Google Scholar] [CrossRef]
- Alzayed, M.; Chaoui, H.; Farajpour, Y. Maximum power tracking for a wind energy conversion system using cascade-forward neural networks. IEEE Trans. Sustain. Energy 2021, 12, 2367–2377. [Google Scholar] [CrossRef]
- Joo, Y.H.; Antonysamy, R.; Ramasamy, T.; Lee, S.R. Stable maximum power extraction and DC link voltage regulation for PMVG-based WECS. IEEE Trans. Ind. Electron. 2022. [Google Scholar] [CrossRef]


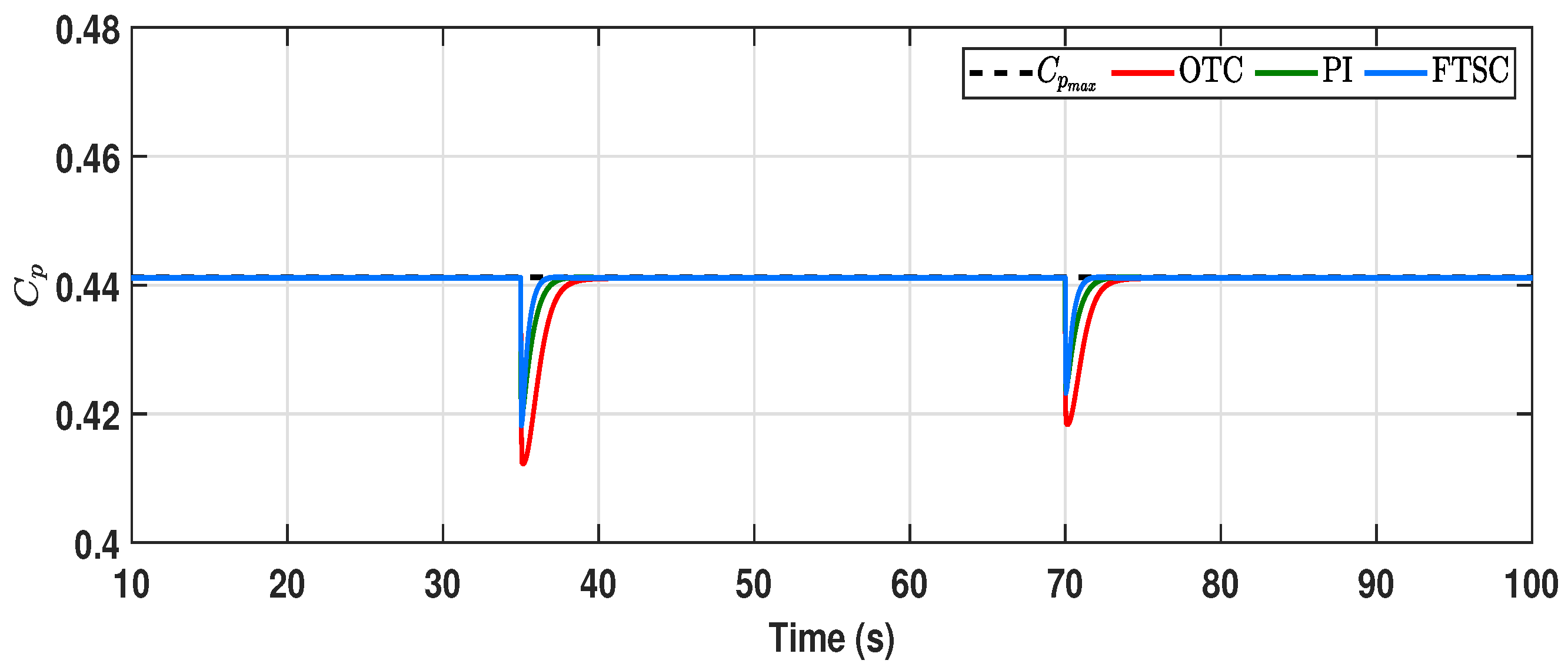
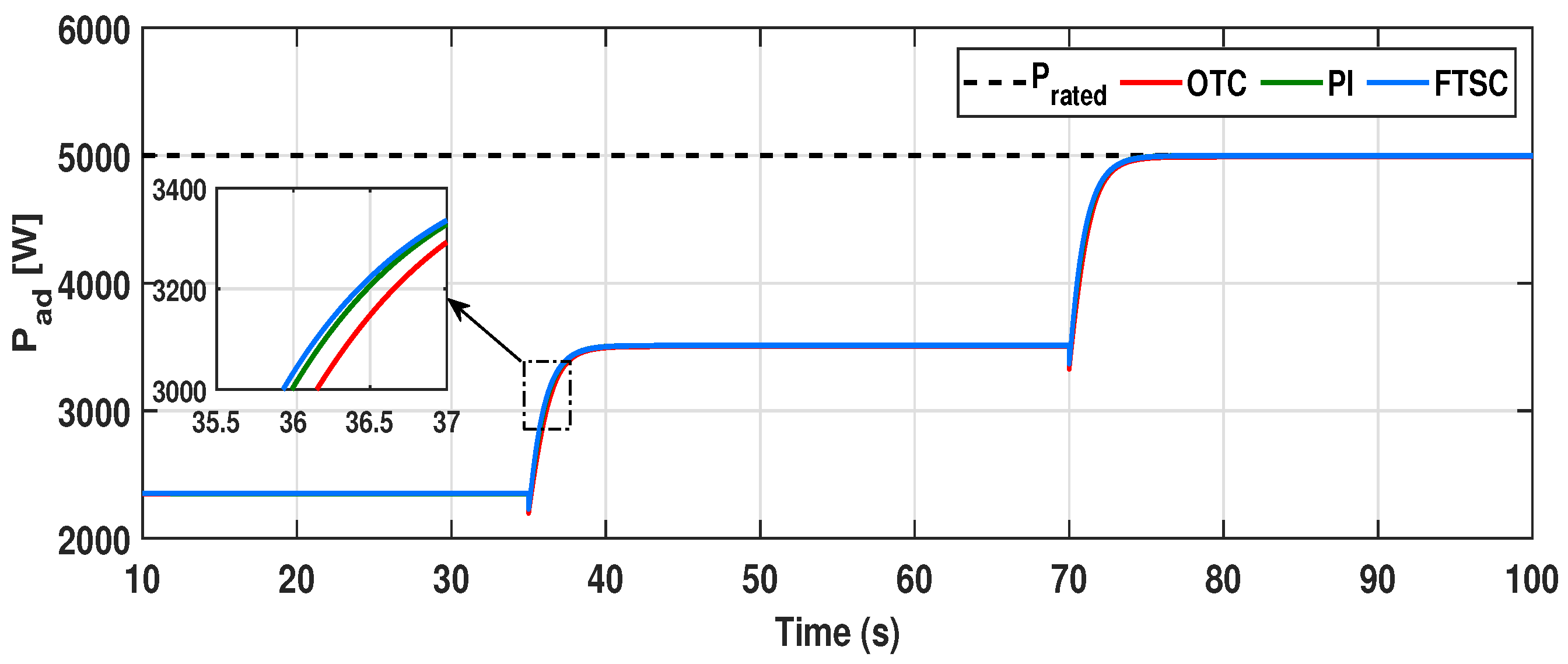
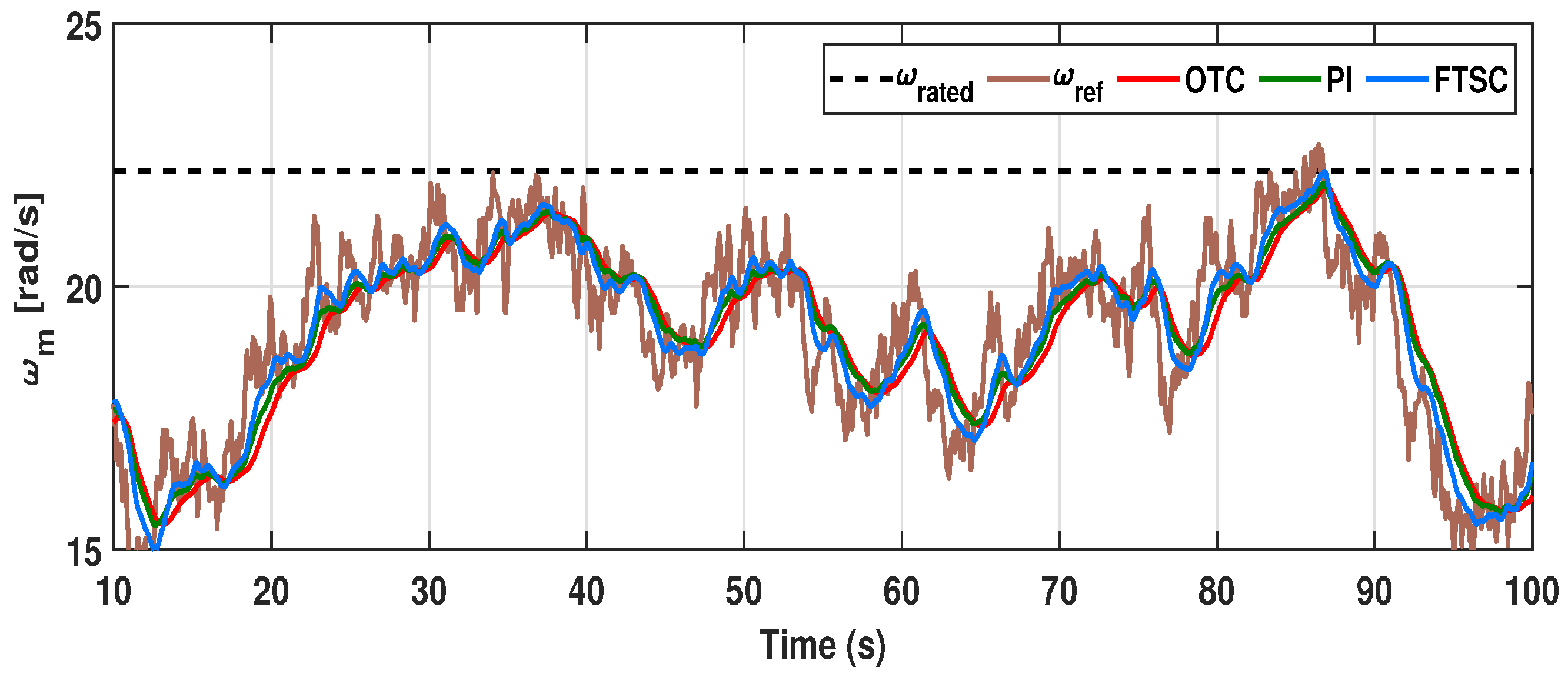
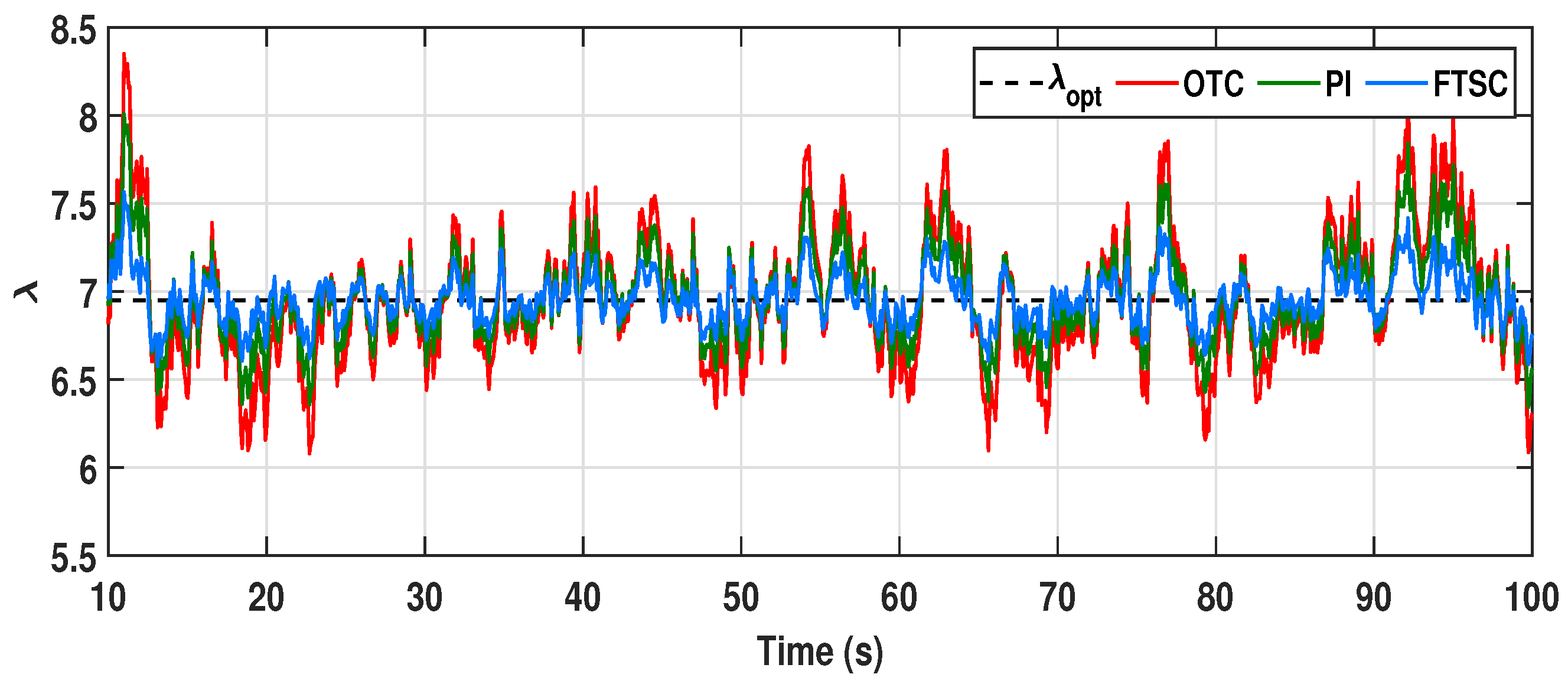
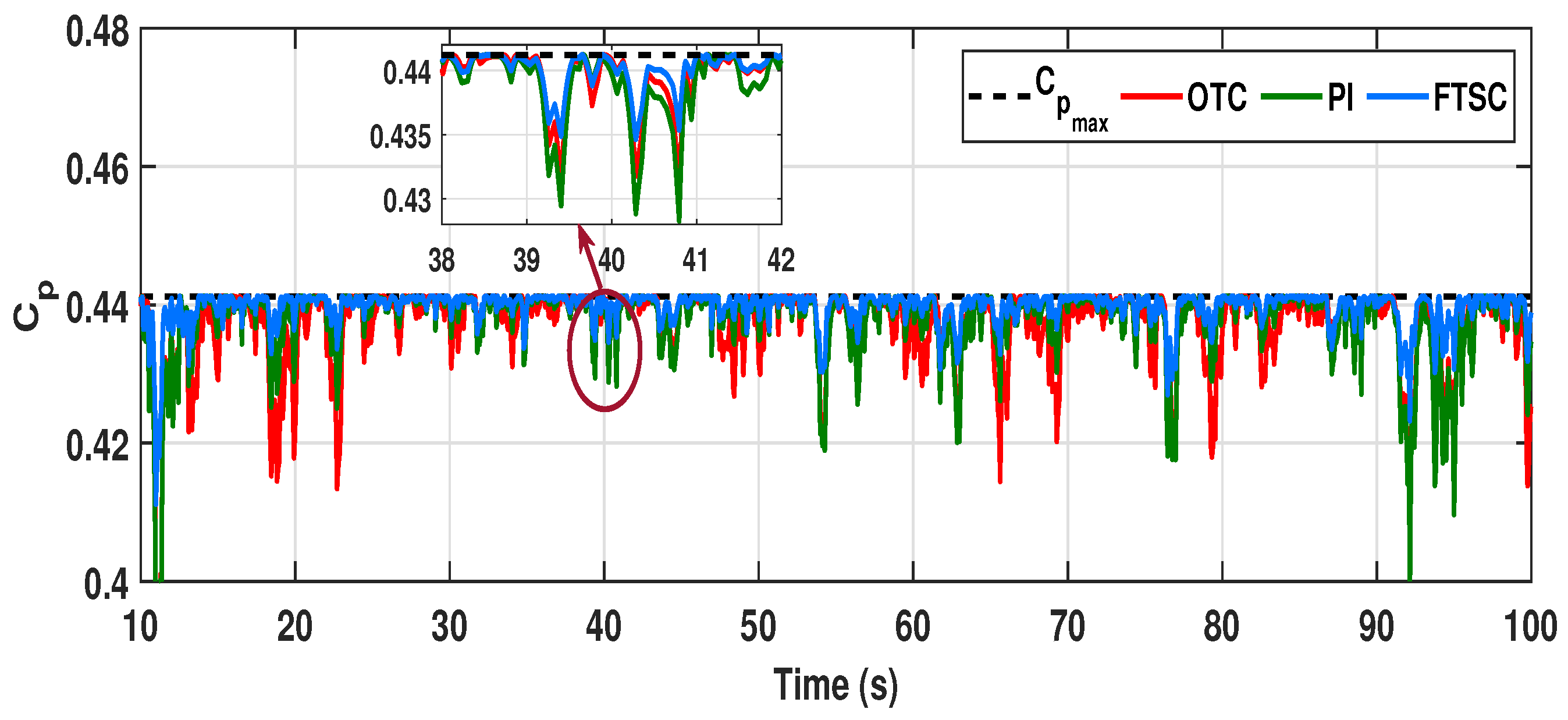
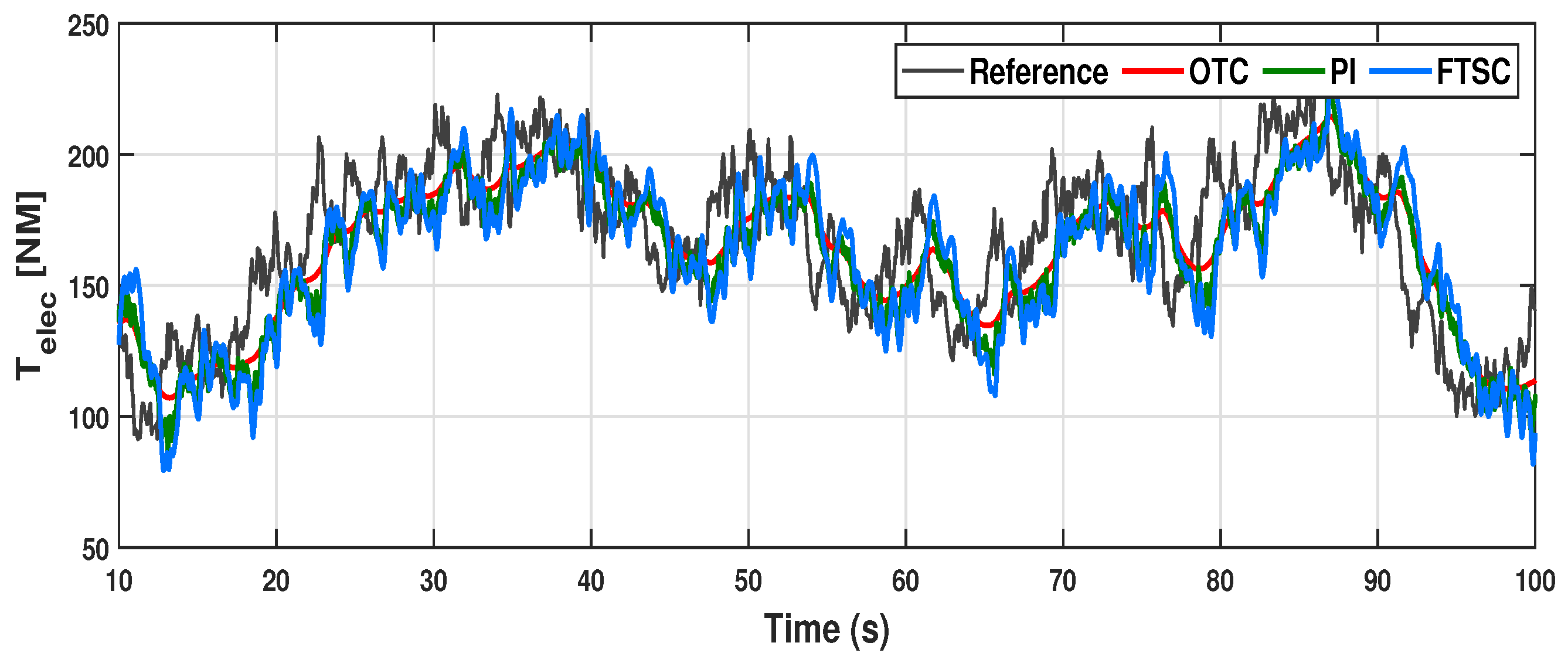
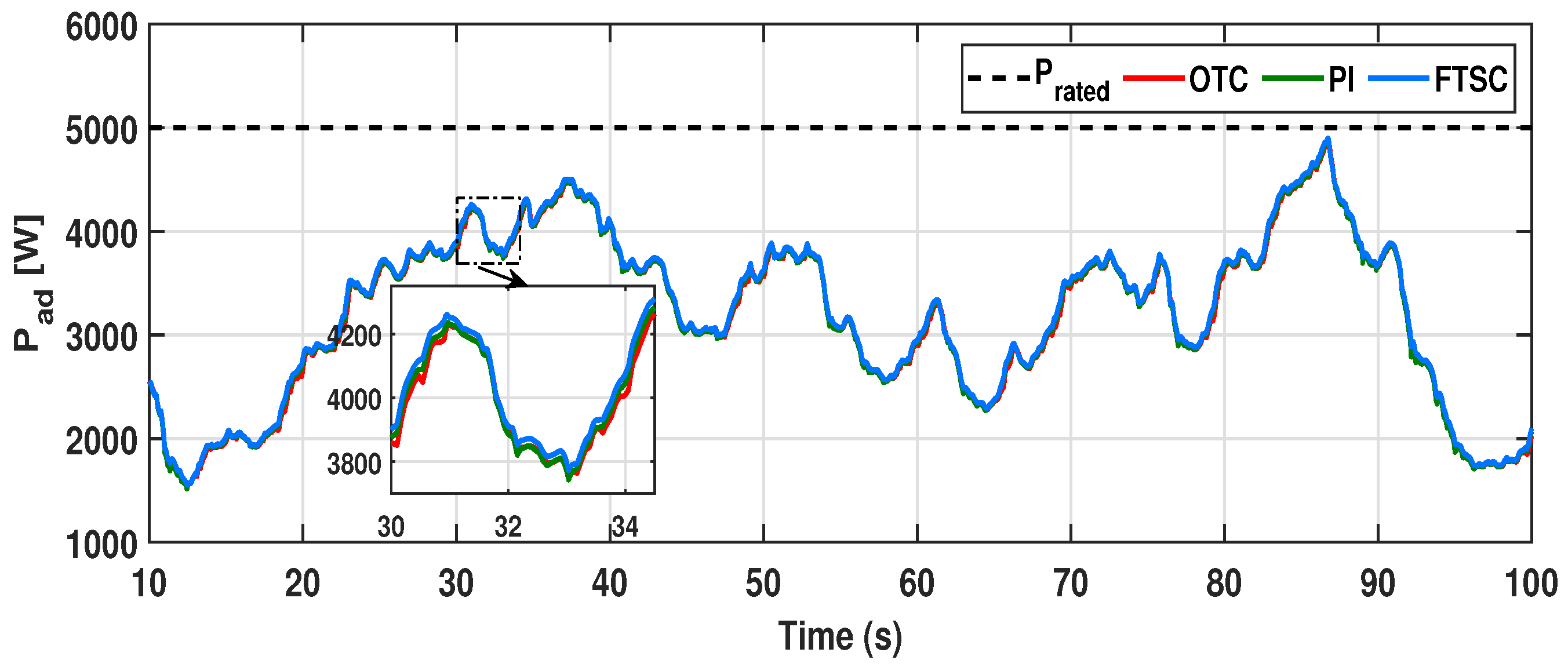

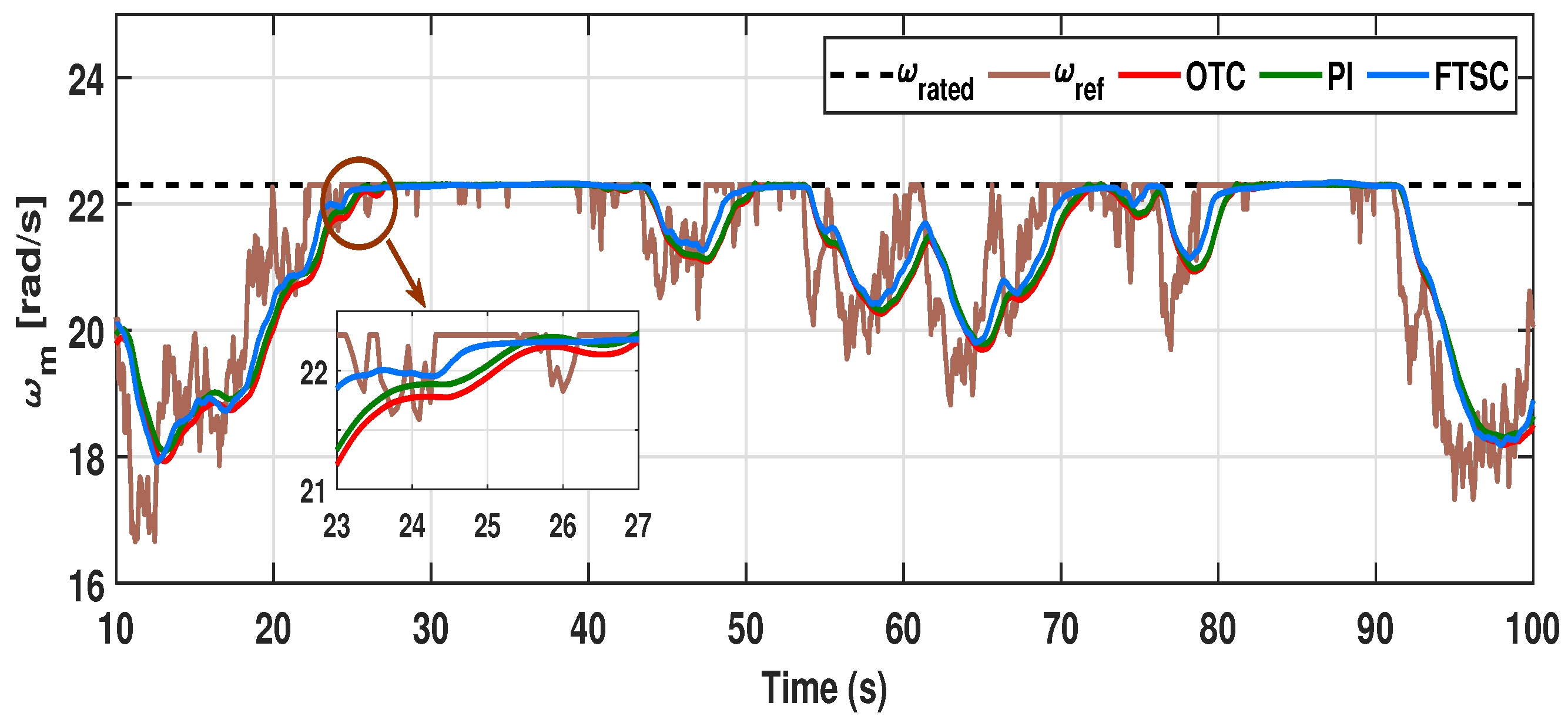


| Wind Profile | Efficiency | OTC | PI | FTSC |
|---|---|---|---|---|
| 8 m/s | % | % | % | |
| 8 m/s | % | % | % | |
| 9 m/s | % | % | % | |
| 9 m/s | % | % | % |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mayilsamy, G.; Natesan, B.; Joo, Y.H.; Lee, S.R. Fast Terminal Synergetic Control of PMVG-Based Wind Energy Conversion System for Enhancing the Power Extraction Efficiency. Energies 2022, 15, 2774. https://doi.org/10.3390/en15082774
Mayilsamy G, Natesan B, Joo YH, Lee SR. Fast Terminal Synergetic Control of PMVG-Based Wind Energy Conversion System for Enhancing the Power Extraction Efficiency. Energies. 2022; 15(8):2774. https://doi.org/10.3390/en15082774
Chicago/Turabian StyleMayilsamy, Ganesh, Balasubramani Natesan, Young Hoon Joo, and Seong Ryong Lee. 2022. "Fast Terminal Synergetic Control of PMVG-Based Wind Energy Conversion System for Enhancing the Power Extraction Efficiency" Energies 15, no. 8: 2774. https://doi.org/10.3390/en15082774
APA StyleMayilsamy, G., Natesan, B., Joo, Y. H., & Lee, S. R. (2022). Fast Terminal Synergetic Control of PMVG-Based Wind Energy Conversion System for Enhancing the Power Extraction Efficiency. Energies, 15(8), 2774. https://doi.org/10.3390/en15082774








