An Accurate Switching Transient Analytical Model for GaN HEMT under the Influence of Nonlinear Parameters
Abstract
:1. Introduction
- (1)
- An accurate model for nonlinear junction capacitances of GaN HEMT is given and an extraction method for nonlinear transconductance is proposed. The proposed approximation method for capacitances and transconductance have better performance than conventional approaches.
- (2)
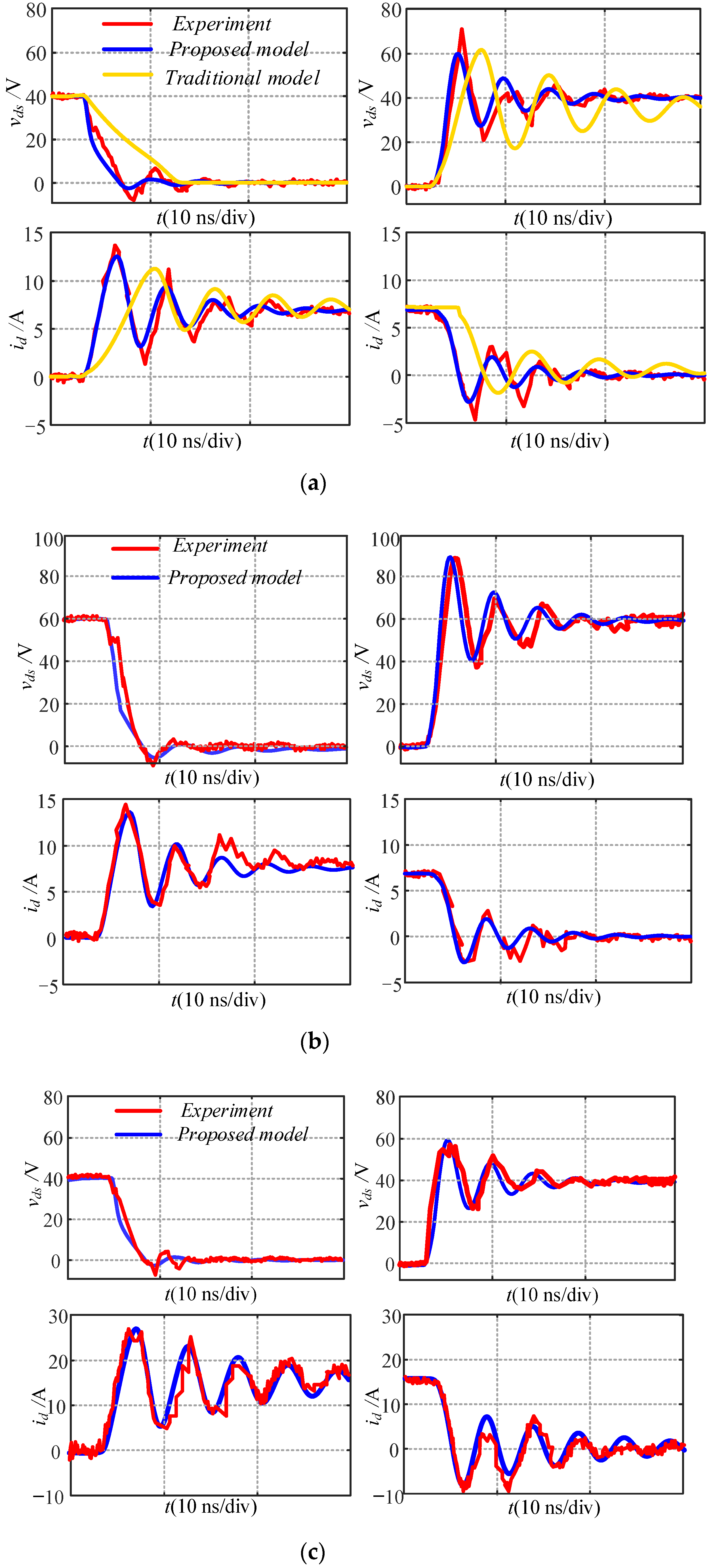
- An accurately switching transient analytical model for GaN HEMT considering the influence of nonlinear parameters is proposed in this paper. The analytical derivation of turn-ON process and turn-OFF process is illustrated in detail, and the equivalent circuits are derived for each switching transition. The accuracy of the proposed model is verified by DPT circuit. Experiment results verify that the proposed model is more precise compared with the traditional model. The experimental results show that the maximum relative error of the switching loss can be kept within 10% by the proposed model.
- (3)
- The effects of gate resistance, gate source capacitance, gate drain capacitance, drain source capacitance, and parasitic inductances on switching characteristic and switching losses are systematically investigated based on the proposed model.
2. Novel Analytical Model of GaN HEMT Device
3. Transient Characteristic Analysis of Turn-On Process Based on the Proposed Model
3.1. Stage I (t0–t1): Turn-On Delay Period
3.2. Stage II (t1–t2): Current Rise Period
3.3. Stage III (t2–t3): Voltage Decline Period
3.4. Stage IV (t3–t4): Ringing Period
4. Transient Characteristic Analysis of Turn-Off Process Based on the Proposed Model
4.1. Stage I (t5–t6): Turn-Off Delay Period
4.2. Stage II (t6–t7): Voltage Rise Period
4.3. Stage III (t7–t8): Current Decline Period
4.4. Stage IV (t8–t9): Ringing Period
5. Experimental Verifications
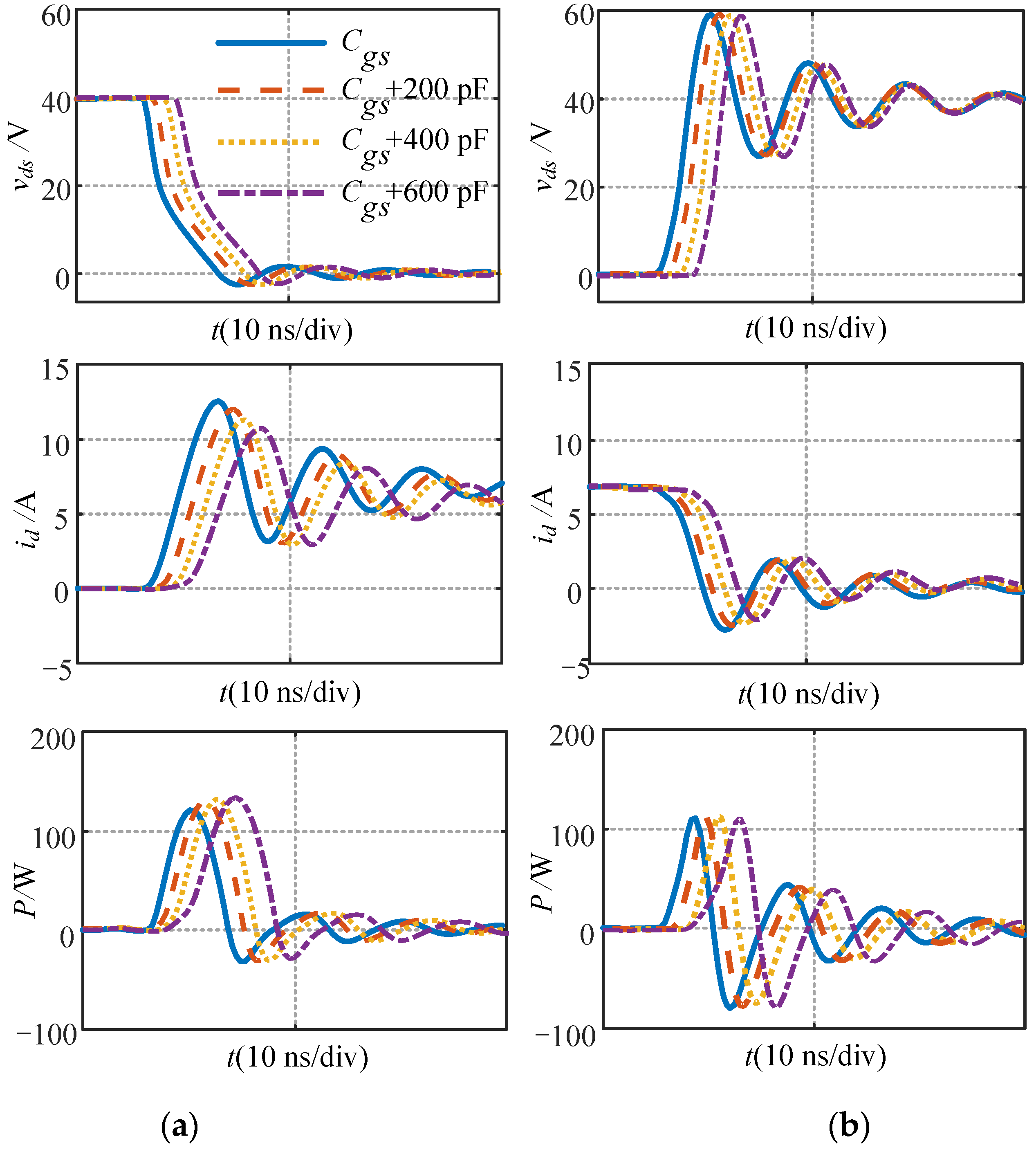
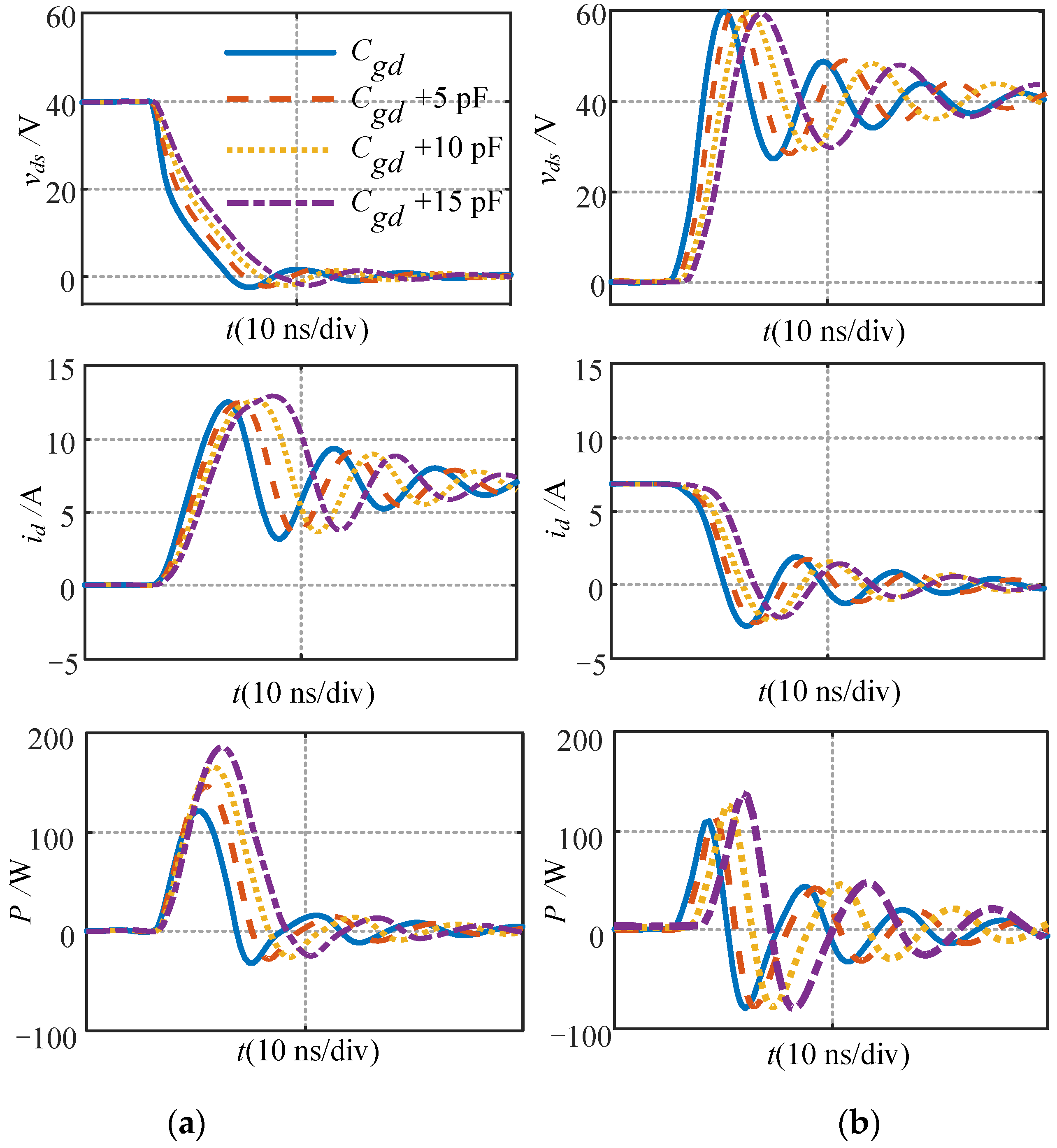
6. Effect of Parameter Variation on the Analytical Model
7. Conclusions
- (1)
- Switching speed. The switching process of the GaN HENT are essentially the charging process of its nonlinear junction capacitances. With the increases of Cgs, Cgd, Cds, Lloop and Rg, the switching speed is slowed down. Since the channel current is gate-source voltage dependent in the saturation region, it is out of the question that Cgs can affect the current slew rate. As for the voltage slew rate, it is clarified that the charging of Cgd leads to the change in the drain source voltage. The loop parasitic inductances Lloop and gate resistance Rg decrease the change rate of id and vds with the increase of Lloop and Rg.
- (2)
- Current and voltage overshoot. The current and voltage overshoot in switching transient can be suppressed by increasing Rg. The current overshoot can be suppressed by increasing Cgs. The current and voltage overshoot can be worsened by increasing Lloop. The current overshoot can be worsened by increasing Cds. Cgd cannot affect the current and voltage overshoot.
- (3)
- Switching loss. Both Cgs, Cgd, Cds, Lloop and Rg increases switching loss by slowing down the switching speed and prolonging the switching process.
- (4)
- Design guidelines. It can be concluded that parameter changes of the GaN HEMT have a profound influence on the switching performance; hence, it calls for special attention to the selection of components and circuit design. The voltage change rate is most sensitive to Cgd, Lloop and Rg; for high-speed high-voltage applications, these values should not be large. The current change rate is most sensitive to Cgs, Cds, Lloop, and Rg. Therefore, the PCB traces should be carefully routed to reduce the stray inductance in the circuit. The other parasitic elements should be minimized to achieve fast switching and low loss.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Afonso, J.L.; Tanta, M.; Pinto, J.G.O.; Monteiro, L.F.C.; Machado, L.; Sousa, T.J.C.; Monteiro, V. A Review on Power Electronics Technologies for Power Quality Improvement. Energies 2021, 14, 8585. [Google Scholar] [CrossRef]
- Maddi, H.L.R.; Yu, S.; Zhu, S.; Liu, T.; Shi, L.; Kang, M.; Xing, D.; Nayak, S.; White, M.H.; Agarwal, A.K. The Road to a Robust and Affordable SiC Power MOSFET Technology. Energies 2021, 14, 8283. [Google Scholar] [CrossRef]
- Ramachandran, R.; Nymand, M. Experimental demonstration of a 98.8% efficient isolated DC–DC GaN converter. IEEE Trans. Ind. Electron. 2017, 64, 9104–9113. [Google Scholar] [CrossRef]
- Ren, Y.C.; Xu, M.; Zhou, J.H.; Lee, F.C. Analytical loss model of power MOSFET. IEEE Trans. Power Electron. 2006, 21, 310–319. [Google Scholar]
- Xiong, Y.; Sun, S.; Jia, H.; Shea, P. New physical insights on power MOSFET switching losses. IEEE Trans. Power Electron. 2009, 24, 525–531. [Google Scholar] [CrossRef]
- Wang, J.; Chung, H.S.; Li, R.T. Characterization and experimental assessment of the effects of parasitic elements on the MOSFET switching performance. IEEE Trans. Power Electron. 2013, 28, 573–590. [Google Scholar] [CrossRef]
- Turzynski, M.; Kulesza, W.J. A simplified behavioral MOSFET model based on parameters extraction for circuit simulations. IEEE Trans. Power Electron. 2016, 31, 3096–3105. [Google Scholar] [CrossRef]
- Christen, D.; Biela, J. Analytical switching loss modeling based on datasheet parameters for MOSFET in a Half-bridge. IEEE Trans. Power Electron. 2019, 34, 3700–3710. [Google Scholar] [CrossRef]
- Costinett, D.; Maksimovic, D.; Zane, R. Circuit-oriented treatment of nonlinear capacitances in switched mode power supplies. IEEE Trans. Power Electron. 2015, 30, 985–995. [Google Scholar] [CrossRef]
- Sakairi, H.; Yanagi, T.; Otake, H.; Kuroda, N.; Tanigawa, H. Measurement methodology for accurate modeling of SiC MOSFET switching behavior over wide voltage and current ranges. IEEE Trans. Power Electron. 2018, 33, 7314–7325. [Google Scholar] [CrossRef]
- Roy, S.K.; Basu, K. Analytical estimation of turn ON switching loss of SiC MOSFET and schottky diode pair from datasheet parameters. IEEE Trans. Power Electron. 2019, 34, 9118–9130. [Google Scholar] [CrossRef]
- Sun, J.; Yuan, L.; Duan, R.; Lu, Z.; Zhao, Z. A semi-physical semi-behavioral analytical model for switching transient of SiC MOSFET module. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 2258–2270. [Google Scholar] [CrossRef]
- Chen, K.; Zhao, Z.; Yuan, L.; Lu, T.; He, F. The impact of nonlinear junction capacitance on switching transient and its modeling for SiC MOSFET. IEEE Trans. Electron Device 2014, 62, 333–338. [Google Scholar] [CrossRef]
- Wang, X.; Zhao, Z.; Li, K.; Zhu, Y.; Chen, K. Analytical methodology for loss calculation of SiC MOSFETs. IEEE J. Emerg. Sel. Top. Power Electron. 2018, 7, 71–83. [Google Scholar] [CrossRef]
- Xie, R.; Wang, H.; Tang, G.; Yang, X.; Chen, K.J. An analytical model for false turn-on evaluation of high-voltage enhancement-mode GaN transistor in bridge-leg configuration. IEEE Trans. Power Electron. 2017, 32, 6416–6433. [Google Scholar] [CrossRef]
- Wang, K.; Yang, X.; Li, H.; Ma, H.; Zeng, X.; Chen, W. An Analytical Switching Process Model of Low-Voltage eGaN HEMTs for Loss Calculation. IEEE Trans. Power Electron. 2016, 31, 635–647. [Google Scholar] [CrossRef]
- Chen, J.; Luo, Q.; Huang, J.; He, Q.; Du, X. A Complete Switching Analytical Model of Low-Voltage eGaN HEMTs and Its Application in Loss Analysis. IEEE Trans. Ind. Electron. 2020, 67, 1615–1625. [Google Scholar] [CrossRef]
- Kozak, J.P.; Barchowsky, A.; Hontz, M.R.; Koganti, N.B.; Stachina, W.H.; Reed, G.F.; Mao, Z.; Khana, R. An Analytical Model for Predicting Turn-ON Overshoot in Normally-OFF GaN HEMTs. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 99–110. [Google Scholar] [CrossRef]
- Xin, Y.; Chen, W.; Sun, R.; Shi, Y.; Zhang, B. Analytical Switching Loss Model for GaN-Based Control Switch and Synchronous Rectifier in Low-Voltage Buck Converters. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 7, 1485–1495. [Google Scholar] [CrossRef]
- Long, X.; Liang, W.; Jun, Z.; Chen, G. A Normalized Quantitative Method for GaN HEMT Turn-ON Overvoltage Modeling and Suppressing. IEEE Trans. Power Electron. 2019, 66, 2766–2775. [Google Scholar] [CrossRef]
- Huang, X.; Li, Q.; Liu, Z.; Lee, F.C. Analytical Loss Model of High Voltage GaN HEMT in Cascode Configuration. IEEE Trans. Power Electron. 2014, 29, 2208–2219. [Google Scholar] [CrossRef]
- Li, Q.; Liu, B.; Duan, S. Simplified analytical model for estimation of switching loss of cascode GaN HEMTs in totem-pole PFC converters. Chin. J. Electr. Eng. 2019, 5, 1–9. [Google Scholar] [CrossRef]



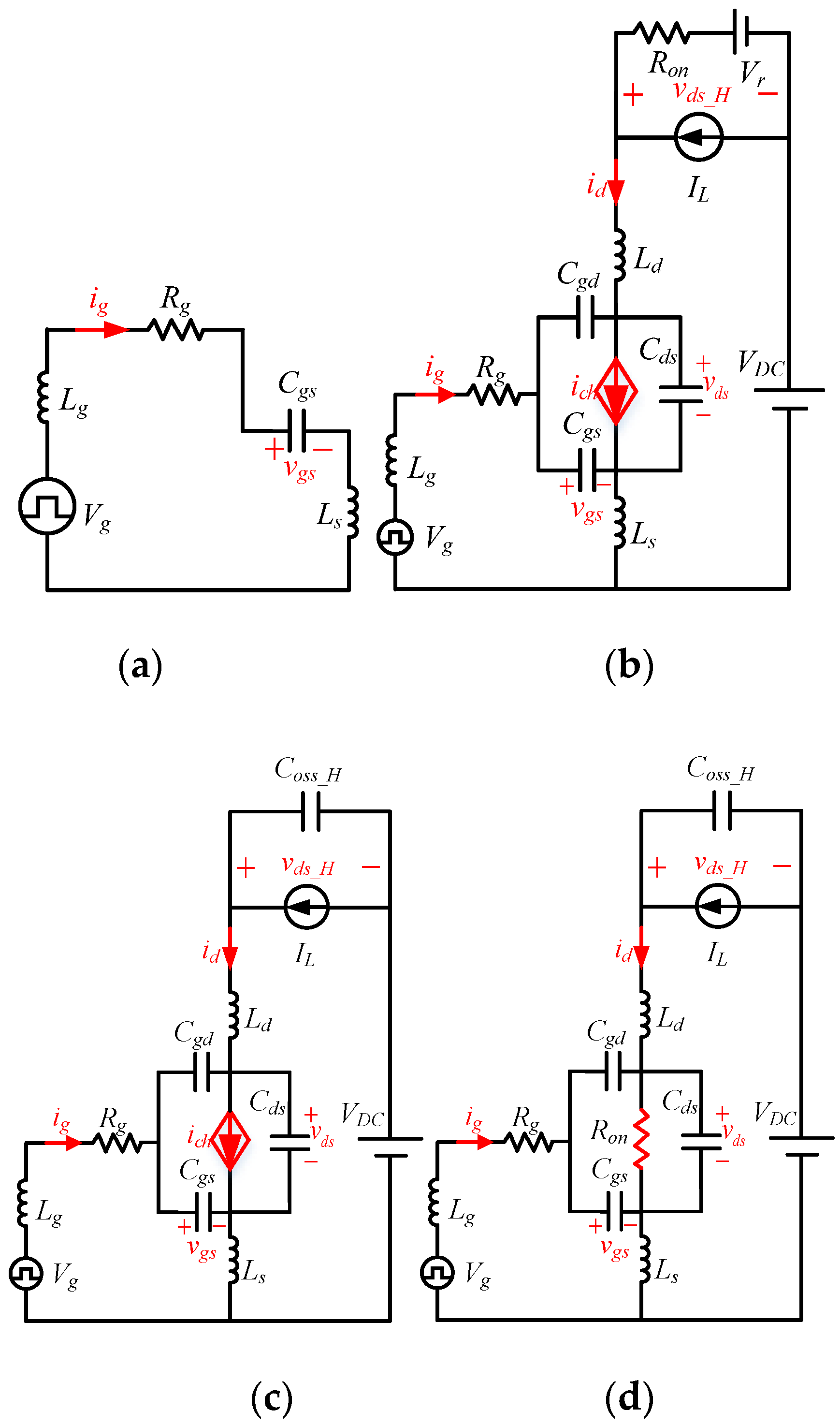


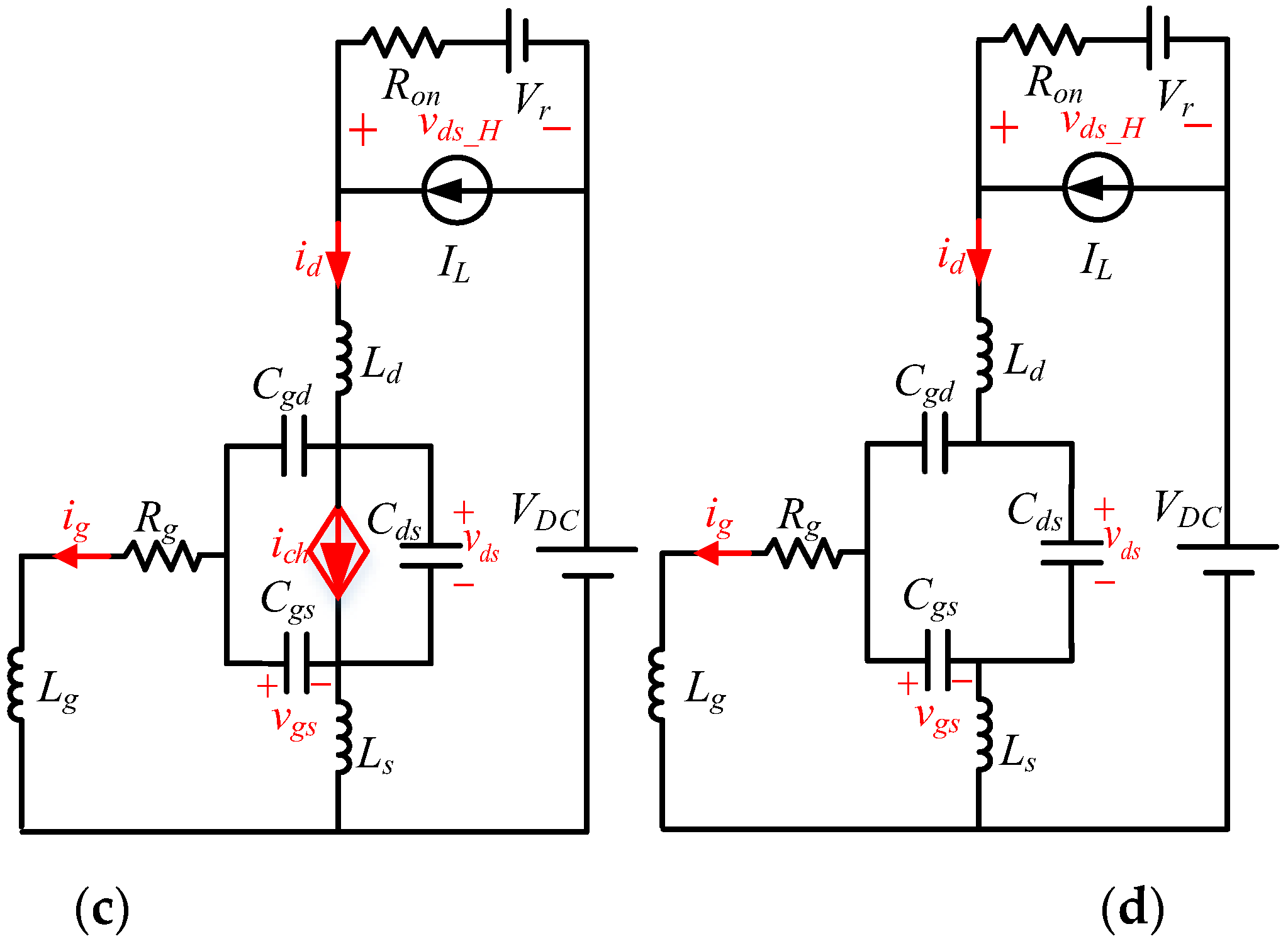





| Symbol | Value |
|---|---|
| Comax | 311 pF |
| k1 | −0.456 |
| k2 | −0.51 |
| k3 | 9.5 |
| k4 | −0.256 |
| k5 | 30 |
| k6 | 0.75 |
| Parameters | Value |
|---|---|
| VDC | 40 V |
| IL | 7 A |
| Ron | 0.57 Ω |
| Ls | 5.6 nH |
| Ld | 3.7 nH |
| Lg | 2.8 nH |
| Rg | 10 Ω |
| Vg | 6 V |
| Parameters | Turn-On Switching Speed | Turn-Off Switching Speed | Turn-On Current Overshoot | Turn-Off Voltage Overshoot | Turn-Off Current Overshoot | Turn-On Loss | Turn-Off Loss |
|---|---|---|---|---|---|---|---|
| Rg | Increase | Increase | Decrease | Decrease | Decrease | Increase | Increase |
| Lloop | Increase | Increase | Decrease | Invariant | Increase | Increase | Increase |
| Cgs | Increase | Increase | Decrease | Invariant | Decrease | Increase | Increase |
| Cgd | Increase | Increase | Increase | Invariant | Invariant | Increase | Increase |
| Cds | Invariant | Increase | Invariant | Invariant | Increase | Invariant | Increase |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, D.; Hang, L.; He, Y.; He, Z.; Zeng, P. An Accurate Switching Transient Analytical Model for GaN HEMT under the Influence of Nonlinear Parameters. Energies 2022, 15, 2966. https://doi.org/10.3390/en15082966
Yan D, Hang L, He Y, He Z, Zeng P. An Accurate Switching Transient Analytical Model for GaN HEMT under the Influence of Nonlinear Parameters. Energies. 2022; 15(8):2966. https://doi.org/10.3390/en15082966
Chicago/Turabian StyleYan, Dong, Lijun Hang, Yuanbin He, Zhen He, and Pingliang Zeng. 2022. "An Accurate Switching Transient Analytical Model for GaN HEMT under the Influence of Nonlinear Parameters" Energies 15, no. 8: 2966. https://doi.org/10.3390/en15082966
APA StyleYan, D., Hang, L., He, Y., He, Z., & Zeng, P. (2022). An Accurate Switching Transient Analytical Model for GaN HEMT under the Influence of Nonlinear Parameters. Energies, 15(8), 2966. https://doi.org/10.3390/en15082966






