The Effect of Electrospinning Parameters on Piezoelectric PVDF-TrFE Nanofibers: Experimental and Simulation Study
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Preparation of P(VDF-TrFE) Nanofibers
2.3. Fourier Transform Infrared Spectroscopy (FTIR)
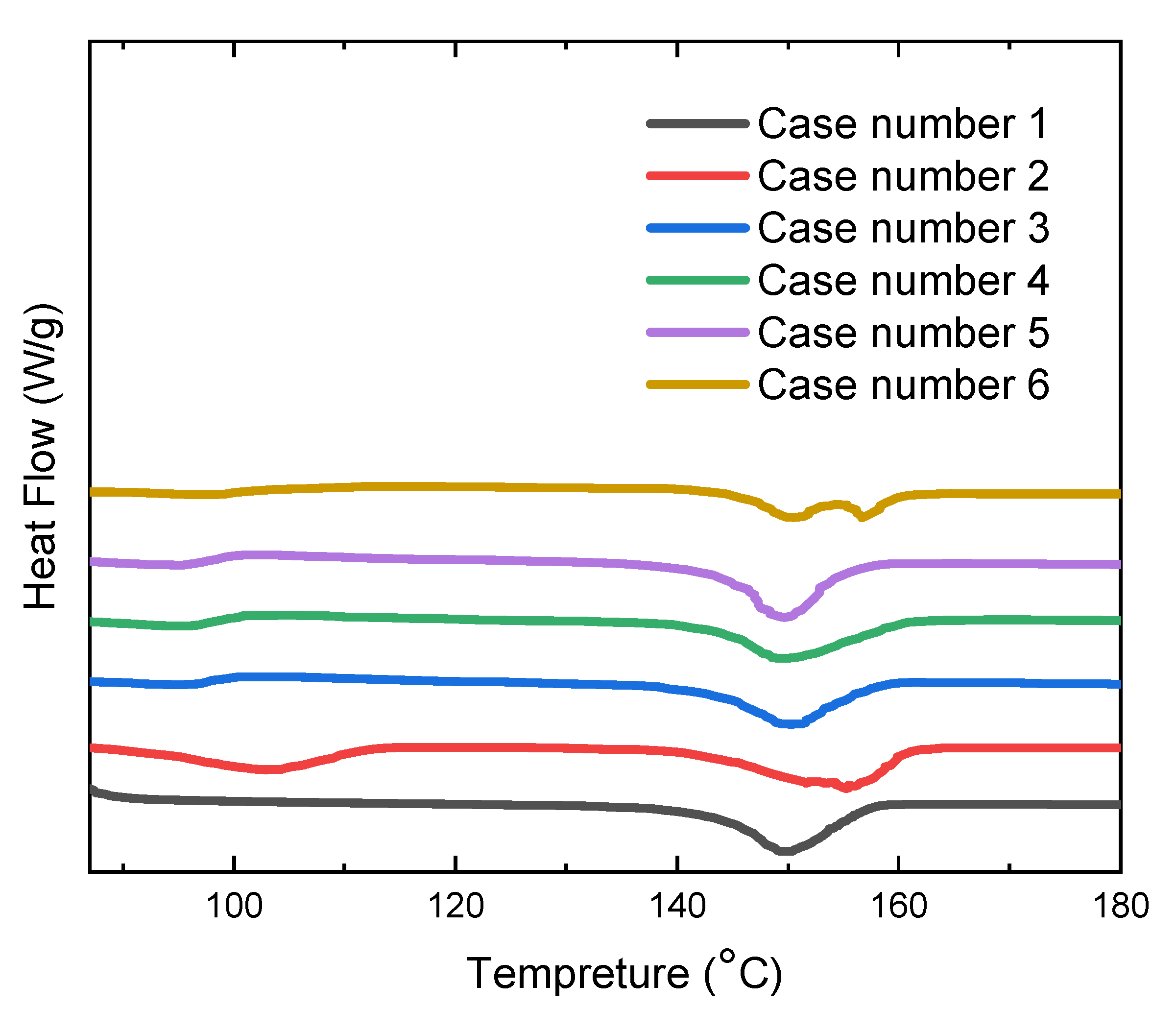
2.4. Differential Scanning Calorimetry (DSC)
2.5. Scanning Electron Microscope (SEM)
2.6. Piezoelectric Measurements
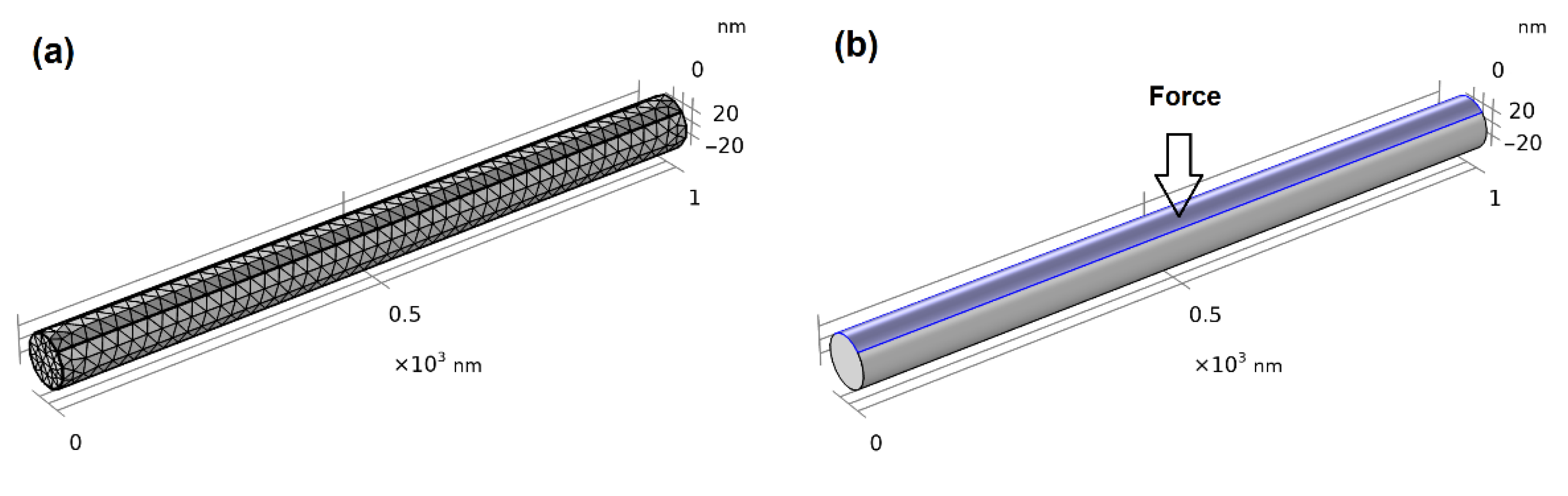
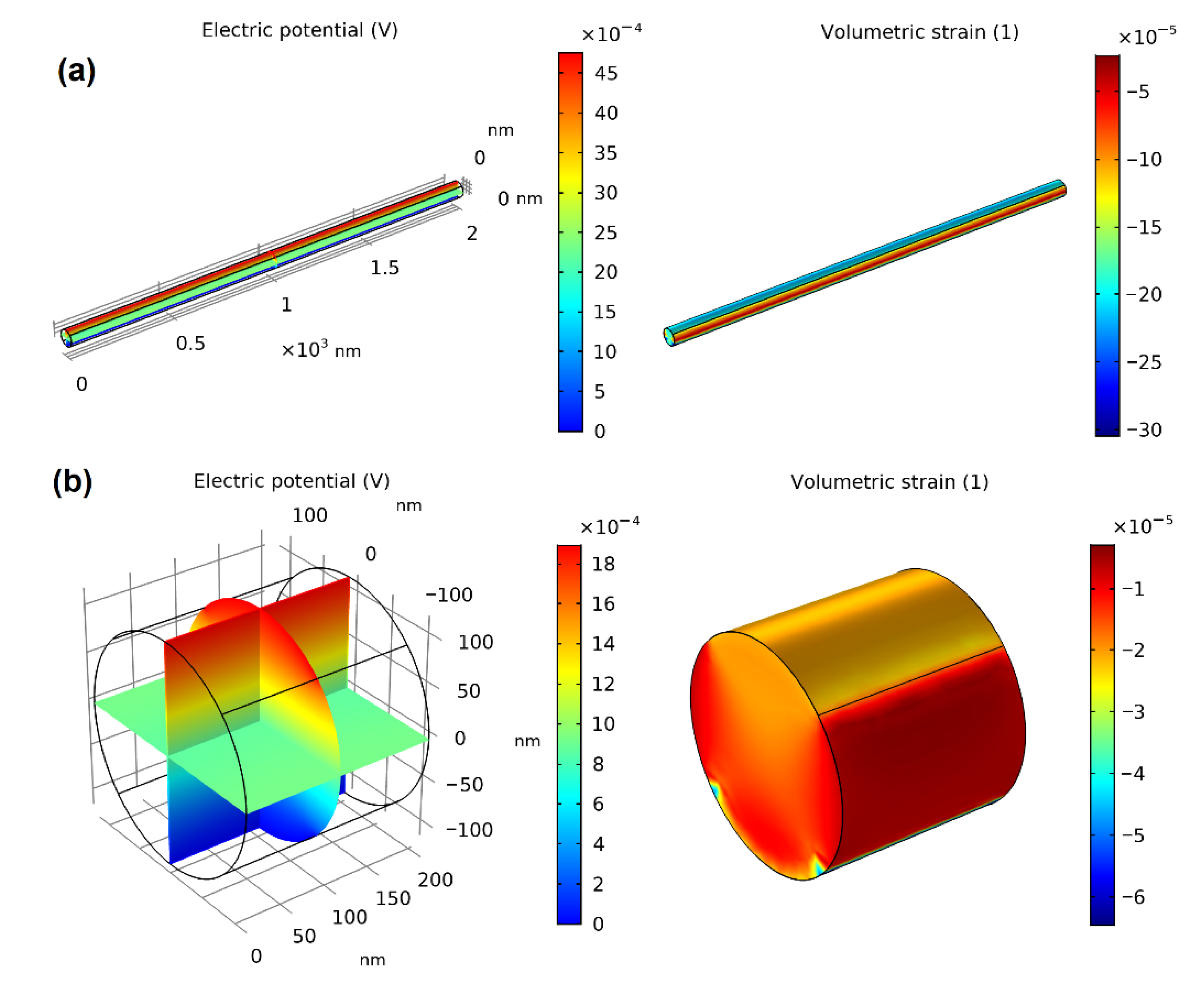
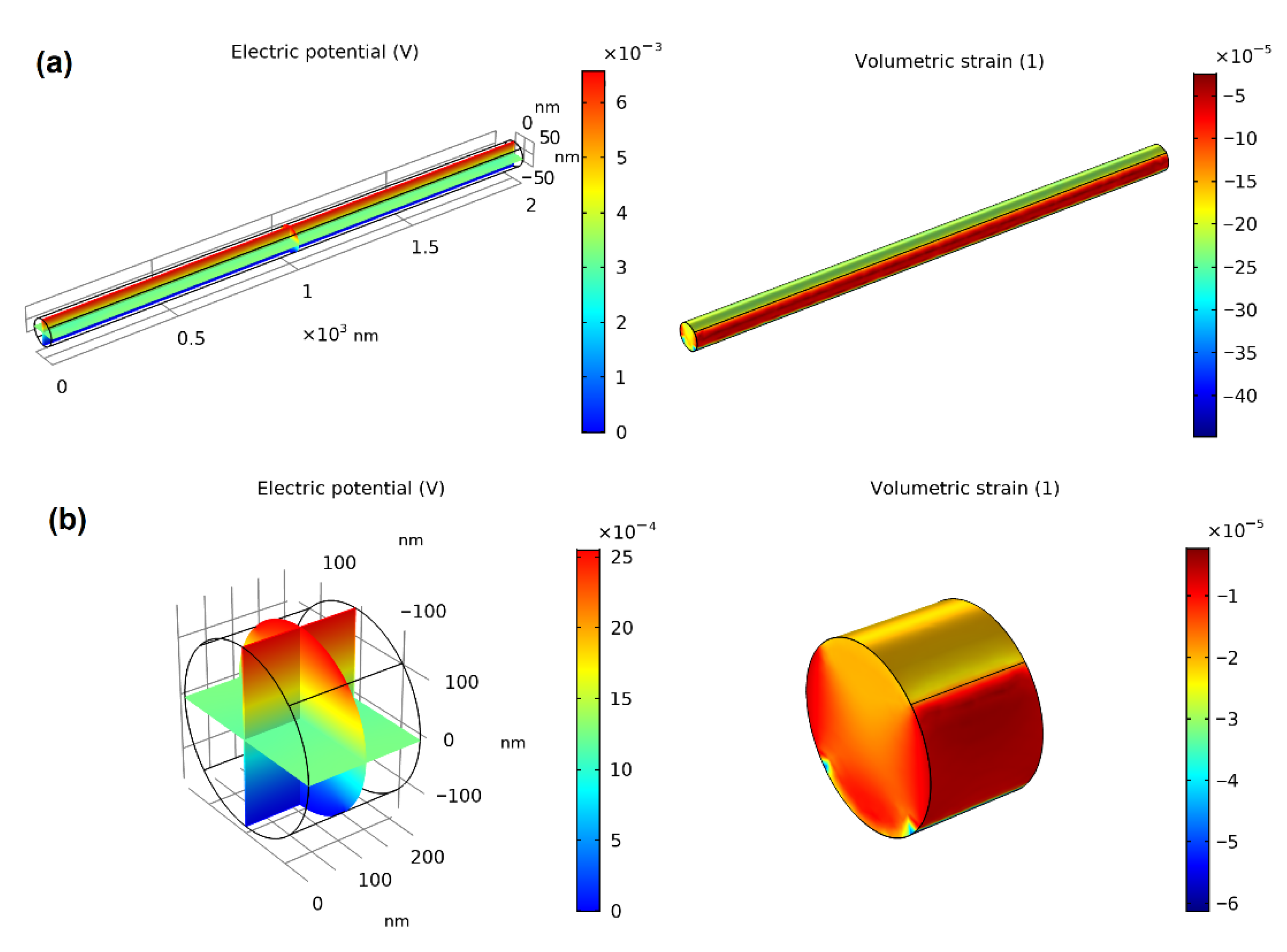
2.7. Simulation
3. Results
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ritchie, H.; Roser, M.; Rosado, P. CO2 and Greenhouse Gas Emissions; Our World in Data: Oxford, UK, 2020. [Google Scholar]
- Abolhasani, M.M.; Shirvanimoghaddam, K.; Naebe, M. PVDF/graphene composite nanofibers with enhanced piezoelectric performance for development of robust nanogenerators. Compos. Sci. Technol. 2017, 138, 49–56. [Google Scholar] [CrossRef]
- Anwar, S.; Hassanpour Amiri, M.; Jiang, S.; Abolhasani, M.M.; Rocha, P.R.; Asadi, K. Piezoelectric nylon-11 fibers for electronic textiles, energy harvesting and sensing. Adv. Funct. Mater. 2021, 31, 2004326. [Google Scholar] [CrossRef]
- Shirvanimoghaddam, M.; Abolhasani, M.M.; Farhangi, M.; Barsari, V.Z.; Liu, H.; Dohler, M.; Naebe, M. Towards a green and self-powered Internet of Things using piezoelectric energy harvesting. IEEE Access 2019, 7, 94533–94556. [Google Scholar] [CrossRef]
- Kan, J.; Fan, C.; Wang, S.; Zhang, Z.; Wen, J.; Huang, L. Study on a piezo-windmill for energy harvesting. Renew. Energy 2016, 97, 210–217. [Google Scholar] [CrossRef]
- Liu, H.; Zhong, J.; Lee, C.; Lee, S.-W.; Lin, L. A comprehensive review on piezoelectric energy harvesting technology: Materials, mechanisms, and applications. Appl. Phys. Rev. 2018, 5, 041306. [Google Scholar] [CrossRef]
- Ali, F.; Raza, W.; Li, X.; Gul, H.; Kim, K.-H. Piezoelectric energy harvesters for biomedical applications. Nano Energy 2019, 57, 879–902. [Google Scholar] [CrossRef]
- Anton, S.R.; Sodano, H.A. A review of power harvesting using piezoelectric materials (2003–2006). Smart Mater. Struct. 2007, 16, R1. [Google Scholar] [CrossRef]
- Youn Jung, P.; In-sung, B.; Seok, J.K.; Jiyoun, C.; Cheolmin, P. Control of thin ferroelectric polymer films for non-volatile memory applications. IEEE Trans. Dielectr. Electr. Insul. 2010, 17, 1135–1163. [Google Scholar]
- Lee, G.-G.; Tokumitsu, E.; Yoon, S.M.; Fujisaki, Y.; Yoon, J.W.; Ishiwara, H. The flexible non-volatile memory devices using oxide semiconductors and ferroelectric polymer poly (vinylidene fluoride-trifluoroethylene). Appl. Phys. Lett. 2011, 99, 012901. [Google Scholar] [CrossRef]
- Sharma, T.; Je, S.-S.; Gill, B.; Zhang, J.X. Patterning piezoelectric thin film PVDF–TrFE based pressure sensor for catheter application. Sens. Actuators A Phys. 2012, 177, 87–92. [Google Scholar] [CrossRef]
- Zhu, M.; Chng, S.S.; Cai, W.; Liu, C.; Du, Z. Piezoelectric polymer nanofibers for pressure sensors and their applications in human activity monitoring. RSC Adv. 2020, 10, 21887–21894. [Google Scholar] [CrossRef] [PubMed]
- Yuan, X.; Yan, A.; Lai, Z.; Liu, Z.; Yu, Z.; Li, Z.; Cao, Y.; Dong, S. A poling-free PVDF nanocomposite via mechanically directional stress field for self-powered pressure sensor application. Nano Energy 2022, 98, 107340. [Google Scholar]
- Abolhasani, M.M.; Azimi, S.; Mousavi, M.; Anwar, S.; Amiri, M.H.; Shirvanimoghaddam, K.; Naebe, M.; Michels, J.; Asadi, K. Porous graphene/poly (vinylidene fluoride) nanofibers for pressure sensing. J. Appl. Polym. Sci. 2022, 139, 51907. [Google Scholar] [CrossRef]
- Wang, Z.; Tan, L.; Pan, X.; Liu, G.; He, Y.; Jin, W.; Li, M.; Hu, Y.; Gu, H. Self-powered viscosity and pressure sensing in microfluidic systems based on the piezoelectric energy harvesting of flowing droplets. ACS Appl. Mater. Interfaces 2017, 9, 28586–28595. [Google Scholar] [CrossRef] [PubMed]
- Pérez, R.; Král, M.; Bleuler, H. Study of polyvinylidene fluoride (PVDF) based bimorph actuators for laser scanning actuation at kHz frequency range. Sens. Actuators A Phys. 2012, 183, 84–94. [Google Scholar] [CrossRef]
- Merry, R.J.; de Kleijn, N.C.T.; van de Molengraft, M.J.G.; Steinbuch, M. Using a walking piezo actuator to drive and control a high-precision stage. IEEE ASME Trans. Mechatron. 2009, 14, 21–31. [Google Scholar] [CrossRef]
- Ushijima, T.; Kumakawa, S. Active Engine Mount with Piezo-Actuator for Vibration Control; SAE Technical Paper; SAE: Warrendale, PA, USA, 1993. [Google Scholar]
- Chang, C.; Tran, V.H.; Wang, J.; Fuh, Y.-K.; Lin, L. Direct-write piezoelectric polymeric nanogenerator with high energy conversion efficiency. Nano Lett. 2010, 10, 726–731. [Google Scholar] [CrossRef]
- Abolhasani, M.M.; Naebe, M.; Amiri, M.H.; Shirvanimoghaddam, K.; Anwar, S.; Michels, J.J.; Asadi, K. Hierarchically structured porous piezoelectric polymer nanofibers for energy harvesting. Adv. Sci. 2020, 7, 2000517. [Google Scholar]
- Azimi, S.; Abolhasani, A.; Moosavi, S.M.; Vanaei, F.; Jafari, A.; Samimi-Sohrforozani, E.; Rayati, M.T.; Noori, E.; Rafiee, E.; Javadi, A.; et al. Development of a Vehicle Passage Sensor Based on a PVDF Nanogenerator. ACS Appl. Electron. Mater. 2021, 3, 4689–4698. [Google Scholar] [CrossRef]
- Chen, X.; Tian, H.; Li, X.; Shao, J.; Ding, Y.; An, N.; Zhou, Y. A high performance P (VDF-TrFE) nanogenerator with self-connected and vertically integrated fibers by patterned EHD pulling. Nanoscale 2015, 7, 11536–11544. [Google Scholar] [CrossRef]
- Azimi, S.; Golabchi, A.; Nekookar, A.; Rabbani, S.; Amiri, M.H.; Asadi, K.; Abolhasani, M.M. Self-powered cardiac pacemaker by piezoelectric polymer nanogenerator implant. Nano Energy 2021, 83, 105781. [Google Scholar] [CrossRef]
- Abolhasani, M.M.; Naebe, M.; Shirvanimoghaddam, K.; Fashandi, H.; Khayyam, H.; Joordens, M.; Pipertzis, A.; Anwar, S.; Berger, R.; Floudas, G.; et al. Thermodynamic approach to tailor porosity in piezoelectric polymer fibers for application in nanogenerators. Nano Energy 2019, 62, 594–600. [Google Scholar] [CrossRef]
- Lovinger, A.J. Poly (vinylidene fluoride). In Developments in Crystalline Polymers—1; Springer: Dordrecht, The Netherlands, 1982; pp. 195–273. [Google Scholar]
- Ribeiro, C.; Sencadas, V.; Ribelles, J.L.G.; Lanceros-Méndez, S. Influence of processing conditions on polymorphism and nanofiber morphology of electroactive poly (vinylidene fluoride) electrospun membranes. Soft Mater. 2010, 8, 274–287. [Google Scholar] [CrossRef]
- Li, D.; Xia, Y. Electrospinning of nanofibers: Reinventing the wheel? Adv. Mater. 2004, 16, 1151–1170. [Google Scholar] [CrossRef]
- Al-Dhahebi, A.M.; Gopinath, S.C.B.; Saheed, M.S.M. Graphene impregnated electrospun nanofiber sensing materials: A comprehensive overview on bridging laboratory set-up to industry. Nano Converg. 2020, 7, 27. [Google Scholar] [CrossRef]
- Oğuzcan, H.Ç.; Beyaz, M.İ. Design and experimental validation of a stress-controlled pressure sensor for wearable pulse monitoring. In Proceedings of the 2021 IEEE International Symposium on Applications of Ferroelectrics (ISAF), Sydney, Australia, 16–21 May 2021. [Google Scholar]
- Iqbal, M.; Nauman, M.M.; Khan, F.U.; Abas, P.E.; Cheok, Q.; Iqbal, A.; Aissa, B. Multimodal hybrid piezoelectric-electromagnetic insole energy harvester using PVDF generators. Electronics 2020, 9, 635. [Google Scholar] [CrossRef]
- Gu, H.; Zhao, Y.; Wang, M.L. A wireless smart PVDF sensor for structural health monitoring. Struct. Control Health Monit. Off. J. Int. Assoc. Struct. Control Monit. Eur. Assoc. Control Struct. 2005, 12, 329–343. [Google Scholar] [CrossRef]
- Sezer, N.; Koç, M. A comprehensive review on the state-of-the-art of piezoelectric energy harvesting. Nano Energy 2021, 80, 105567. [Google Scholar] [CrossRef]
- Yin, J.-Y.; Boaretti, C.; Lorenzetti, A.; Martucci, A.; Roso, M.; Modesti, M. Effects of Solvent and Electrospinning Parameters on the Morphology and Piezoelectric Properties of PVDF Nanofibrous Membrane. Nanomaterials 2022, 12, 962. [Google Scholar] [CrossRef]
- Samimi-Sohrforozani, E.; Azimi, S.; Abolhasani, A.; Malekian, S.; Arbab, S.; Zendehdel, M.; Abolhasani, M.M.; Nia, N.Y. Development of Porous Polyacrylonitrile Composite Fibers: New Precursor Fibers with High Thermal Stability. Electron. Mater. 2021, 2, 454–465. [Google Scholar] [CrossRef]
- Mitchell, G.R. Electrospinning: Principles, Practice and Possibilities; Royal Society of Chemistry: London, UK, 2015. [Google Scholar]
- Fang, J.; Niu, H.; Wang, H.; Wang, X.; Lin, T. Enhanced mechanical energy harvesting using needleless electrospun poly (vinylidene fluoride) nanofibre webs. Energy Environ. Sci. 2013, 6, 2196–2202. [Google Scholar] [CrossRef]
- Nasir, M.; Matsumoto, H.; Danno, T.; Minagawa, M.; Irisawa, T.; Shioya, M.; Tanioka, A. Control of diameter, morphology, and structure of PVDF nanofiber fabricated by electrospray deposition. J. Polym. Sci. Part B Polym. Phys. 2006, 44, 779–786. [Google Scholar] [CrossRef]
- Pise, D.D.; Ahuja, B.; Shendokar, S. Study of process parameters affecting the diameter and morphology of electrospun polyvinylidene fluoride (PVDF) nanofibers. Int. J. Sci. Res. 2015, 4, 155–160. [Google Scholar]
- Motamedi, A.S.; Mirzadeh, H.; Hajiesmaeilbaigi, F.; Bagheri-Khoulenjani, S.; Shokrgozar, M. Effect of electrospinning parameters on morphological properties of PVDF nanofibrous scaffolds. Prog. Biomater. 2017, 6, 113–123. [Google Scholar] [CrossRef]
- Zulfikar, M.; Afrianingsih, I.; Nasir, M.; Alni, A. Effect of processing parameters on the morphology of PVDF electrospun nanofiber. J. Phys. Conf. Ser. 2018, 987, 012011. [Google Scholar] [CrossRef]
- Shao, H.; Fang, J.; Wang, H.; Lin, T. Effect of electrospinning parameters and polymer concentrations on mechanical-to-electrical energy conversion of randomly-oriented electrospun poly (vinylidene fluoride) nanofiber mats. RSC Adv. 2015, 5, 14345–14350. [Google Scholar] [CrossRef]
- Andrew, J.; Clarke, D. Effect of electrospinning on the ferroelectric phase content of polyvinylidene difluoride fibers. Langmuir 2008, 24, 670–672. [Google Scholar] [CrossRef]
- Jiyong, H.; Yuanyuan, G.; Hele, Z.; Yinda, Z.; Xudong, Y. Effect of electrospinning parameters on piezoelectric properties of electrospun PVDF nanofibrous mats under cyclic compression. J. Text. Inst. 2018, 109, 843–850. [Google Scholar] [CrossRef]
- Huang, F.; Wei, Q.; Wang, J.; Cai, Y.; Huang, Y. Effect of temperature on structure, morphology and crystallinity of PVDF nanofibers via electrospinning. e-Polymers 2008, 8, 1758–1765. [Google Scholar] [CrossRef]
- Mokhtari, F.; Latifi, M.; Shamshirsaz, M. Electrospinning/electrospray of polyvinylidene fluoride (PVDF): Piezoelectric nanofibers. J. Text. Inst. 2016, 107, 1037–1055. [Google Scholar] [CrossRef]
- Wang, X.; Ding, X.; Lang, C.; Hu, J.; Yang, X.; Pan, H. Signal responses of PVDF electrospun fiber web under dynamic compression. Tech. Text. 2015, 33, 16–19. [Google Scholar]
- Damaraju, S.M.; Wu, S.; Jaffe, M.; Arinzeh, T.L. Structural changes in PVDF fibers due to electrospinning and its effect on biological function. Biomed. Mater. 2013, 8, 045007. [Google Scholar] [CrossRef]
- Wang, Y.; Zheng, J.M.; Ren, G.Y.; Zhang, P.H.; Xu, C. A flexible piezoelectric force sensor based on PVDF fabrics. Smart Mater. Struct. 2011, 20, 045009. [Google Scholar] [CrossRef]
- Magniez, K.; De Lavigne, C.; Fox, B. The effects of molecular weight and polymorphism on the fracture and thermo-mechanical properties of a carbon-fibre composite modified by electrospun poly (vinylidene fluoride) membranes. Polymer 2010, 51, 2585–2596. [Google Scholar] [CrossRef]
- Zaarour, B.; Zhu, L.; Jin, X. Controlling the surface structure, mechanical properties, crystallinity, and piezoelectric properties of electrospun PVDF nanofibers by maneuvering molecular weight. Soft Mater. 2019, 17, 181–189. [Google Scholar] [CrossRef]
- Cozza, E.S.; Monticelli, O.; Marsano, E.; Cebe, P. On the electrospinning of PVDF: Influence of the experimental conditions on the nanofiber properties. Polym. Int. 2013, 62, 41–48. [Google Scholar] [CrossRef]
- Zaarour, B.; Zhu, L.; Huang, C.; Jin, X. Fabrication of a polyvinylidene fluoride cactus-like nanofiber through one-step electrospinning. RSC Adv. 2018, 8, 42353–42360. [Google Scholar] [CrossRef]
- Slack, J.J.; Brodt, M.; Cullen, D.A.; Reeves, K.S.; More, K.L.; Pintauro, P.N. Impact of polyvinylidene fluoride on nanofiber cathode structure and durability in proton exchange membrane fuel cells. J. Electrochem. Soc. 2020, 167, 054517. [Google Scholar] [CrossRef]
- Zheng, J.; He, A.; Li, J.; Han, C.C. Polymorphism control of poly (vinylidene fluoride) through electrospinning. Macromol. Rapid Commun. 2007, 28, 2159–2162. [Google Scholar] [CrossRef]
- He, F.; Lau, S.; Chan, H.L.; Fan, J. High dielectric permittivity and low percolation threshold in nanocomposites based on poly (vinylidene fluoride) and exfoliated graphite nanoplates. Adv. Mater. 2009, 21, 710–715. [Google Scholar] [CrossRef]
- Lefki, K.; Dormans, G. Measurement of piezoelectric coefficients of ferroelectric thin films. J. Appl. Phys. 1994, 76, 1764–1767. [Google Scholar] [CrossRef]
- Murayama, N.; Nakamura, K.; Obara, H.; Segawa, M. The strong piezoelectricity in polyvinylidene fluroide (PVDF). Ultrasonics 1976, 14, 15–24. [Google Scholar] [CrossRef]
- Bera, B.; Sarkar, M.D. Piezoelectricity in PVDF and PVDF based piezoelectric nanogenerator: A concept. IOSR J. Appl. Phys. 2017, 9, 95–99. [Google Scholar] [CrossRef]
- Singh, S.; Singh, I.V. Analysis of cracked functionally graded piezoelectric material using XIGA. Eng. Fract. Mech. 2020, 230, 107015. [Google Scholar] [CrossRef]
- Kepler, R.; Anderson, R. Piezoelectricity in polymers. Crit. Rev. Solid State Mater. Sci. 1980, 9, 399–447. [Google Scholar] [CrossRef]
- Logan, D.L. A First Course in the Finite Element Method; Cengage Learning: Boston, MA, USA, 2016. [Google Scholar]
- Cai, X.; Lei, T.; Sun, D.; Lin, L. A critical analysis of the α, β and γ phases in poly (vinylidene fluoride) using FTIR. RSC Adv. 2017, 7, 15382–15389. [Google Scholar] [CrossRef]
- Baqeri, M.; Abolhasani, M.M.; Mozdianfard, M.R.; Guo, Q.; Oroumei, A.; Naebe, M. Influence of processing conditions on polymorphic behavior, crystallinity, and morphology of electrospun poly (VInylidene fluoride) nanofibers. J. Appl. Polym. Sci. 2015, 132, 42304. [Google Scholar] [CrossRef]
- Martins, P.; Lopes, A.; Lanceros-Mendez, S. Electroactive phases of poly (vinylidene fluoride): Determination, processing and applications. Prog. Polym. Sci. 2014, 39, 683–706. [Google Scholar] [CrossRef]
- He, Z.; Rault, F.; Lewandowski, M.; Mohsenzadeh, E.; Salaün, F. Electrospun PVDF nanofibers for piezoelectric applications: A review of the influence of electrospinning parameters on the β phase and crystallinity enhancement. Polymers 2021, 13, 174. [Google Scholar] [CrossRef]
- Baji, A.; Mai, Y.-W.; Li, Q.; Liu, Y. Electrospinning induced ferroelectricity in poly (vinylidene fluoride) fibers. Nanoscale 2011, 3, 3068–3071. [Google Scholar] [CrossRef]
- Li, Z.; Wang, J.; Wang, X.; Yang, Q.; Zhang, Z. Ferro-and piezo-electric properties of a poly (vinyl fluoride) film with high ferro-to para-electric phase transition temperature. RSC Adv. 2015, 5, 80950–80955. [Google Scholar] [CrossRef]
- Whiter, R.A.; Calahorra, Y.; Ou, C.; Kar-Narayan, S. Observation of confinement-induced self-poling effects in ferroelectric polymer nanowires grown by template wetting. Macromol. Mater. Eng. 2016, 301, 1016–1025. [Google Scholar] [CrossRef]
- Ma, W.; Zhang, J.; Wang, X. Effect of initial polymer concentration on the crystallization of poly (vinylidene fluoride)/poly (methyl methacrylate) blend from solution casting. J. Macromol. Sci. Part B 2007, 47, 139–149. [Google Scholar] [CrossRef]
- Jiyong, H.; Yinda, Z.; Hele, Z.; Yuanyuan, G.; Xudong, Y. Mixed effect of main electrospinning parameters on the β-phase crystallinity of electrospun PVDF nanofibers. Smart Mater. Struct. 2017, 26, 085019. [Google Scholar] [CrossRef]
- Mandal, D.; Yoon, S.; Kim, K.J. Origin of Piezoelectricity in an Electrospun Poly(vinylidene fluoride-trifluoroethylene) Nanofiber Web-Based Nanogenerator and Nano-Pressure Sensor. Macromol. Rapid Commun. 2011, 32, 831. [Google Scholar] [CrossRef]



| Case Number | Feed Rate (mL/h) | Concentration (%) | Voltage (kV) | Working Distance (cm) |
|---|---|---|---|---|
| 1 | 0.5 | 10 | 20 | 15 |
| 2 | 0.5 | 15 | 20 | 15 |
| 3 | 1 | 10 | 20 | 15 |
| 4 | 1 | 15 | 20 | 15 |
| 5 | 1.5 | 10 | 20 | 15 |
| 6 | 1.5 | 15 | 20 | 15 |
| Case Number | Concentration (%) | Feed Rate (mL/h) | Mean Diameter (nm) | β-Phase Content (%) | Output Voltage (V) | Crystallinity (%) |
|---|---|---|---|---|---|---|
| 1 | 10 | 0.5 | 75 | 0.9448 | 9.3 | 0.2152 |
| 2 | 15 | 0.5 | 235 | 0.9621 | 2.3 | 0.2393 |
| 3 | 10 | 1 | 109 | 0.9277 | 6 | 0.2097 |
| 4 | 15 | 1 | 277 | 0.9346 | 2.1 | 0.2240 |
| 5 | 10 | 1.5 | 101 | 0.8894 | 9.9 | 0.1981 |
| 6 | 15 | 1.5 | 315 | 0.9093 | 1.72 | 0.2101 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pourbafrani, M.; Azimi, S.; Yaghoobi Nia, N.; Zendehdel, M.; Abolhasani, M.M. The Effect of Electrospinning Parameters on Piezoelectric PVDF-TrFE Nanofibers: Experimental and Simulation Study. Energies 2023, 16, 37. https://doi.org/10.3390/en16010037
Pourbafrani M, Azimi S, Yaghoobi Nia N, Zendehdel M, Abolhasani MM. The Effect of Electrospinning Parameters on Piezoelectric PVDF-TrFE Nanofibers: Experimental and Simulation Study. Energies. 2023; 16(1):37. https://doi.org/10.3390/en16010037
Chicago/Turabian StylePourbafrani, Mehdi, Sara Azimi, Narges Yaghoobi Nia, Mahmoud Zendehdel, and Mohammad Mahdi Abolhasani. 2023. "The Effect of Electrospinning Parameters on Piezoelectric PVDF-TrFE Nanofibers: Experimental and Simulation Study" Energies 16, no. 1: 37. https://doi.org/10.3390/en16010037
APA StylePourbafrani, M., Azimi, S., Yaghoobi Nia, N., Zendehdel, M., & Abolhasani, M. M. (2023). The Effect of Electrospinning Parameters on Piezoelectric PVDF-TrFE Nanofibers: Experimental and Simulation Study. Energies, 16(1), 37. https://doi.org/10.3390/en16010037








