Merchant Energy Storage Investment Analysis Considering Multi-Energy Integration
Abstract
1. Introduction
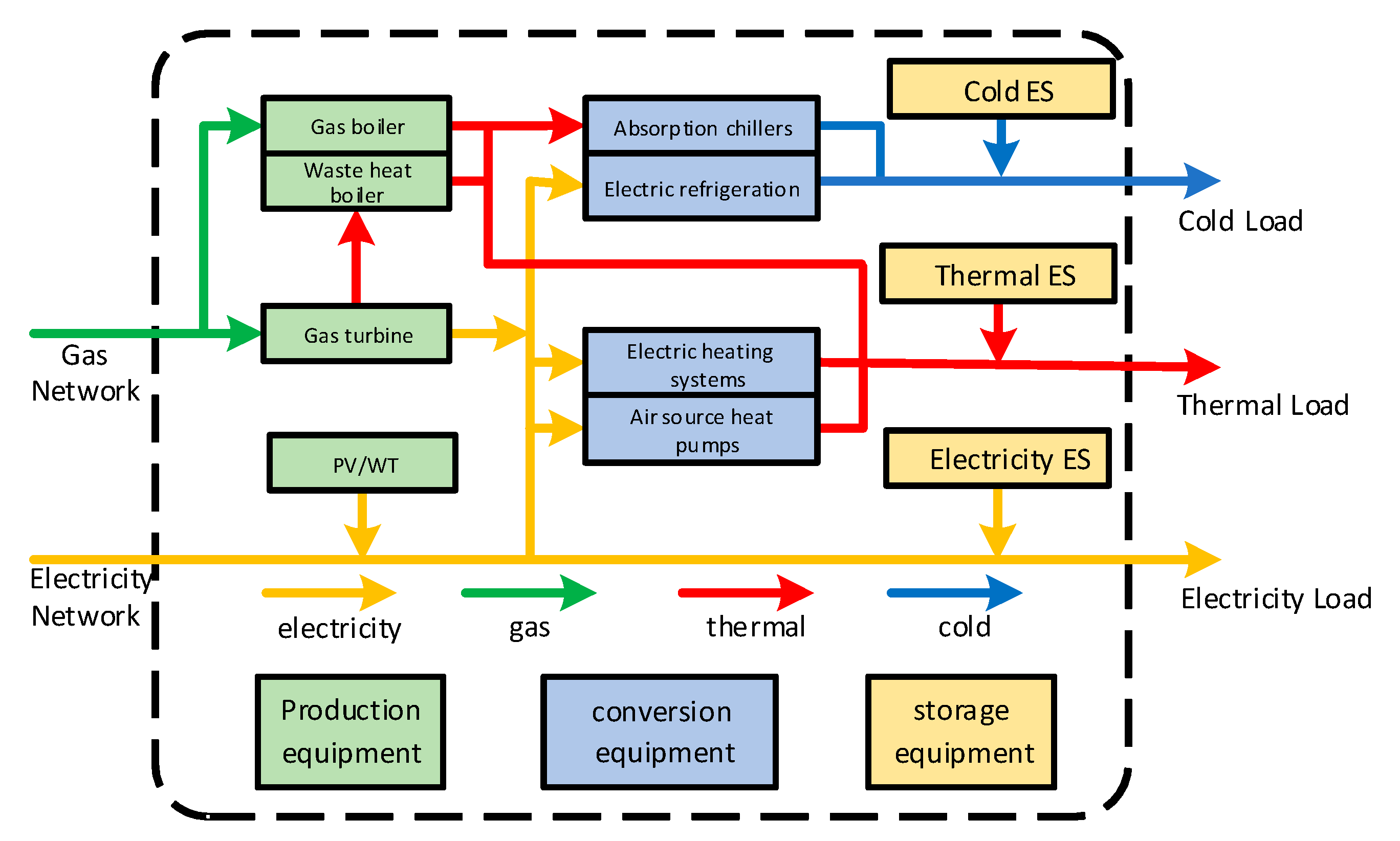
2. Integrated Energy System
3. Problem Formulation
3.1. The Upper Level: Merchant Investment
3.1.1. Objective Function
- Cost of investment and maintenance
- 2.
- Cost of electricity
- 3.
- The service fee charged by the energy storage
3.1.2. Constraints
- Energy storage investment constraints
- 2.
- Energy storage constraints
3.2. The Low Level: Optimal Operation of Multi-Energy Integration
3.2.1. Objective Function
- The cost of purchasing electricity from the grid
- 2.
- The cost of a gas turbine
3.2.2. Constraints
- Power balance constraint
- 2.
- Cooling load of CCHP constraint
- 3.
- Heating load of CCHP constraint
- 4.
- The waste heat load of the CCHP constraint
- 5.
- CCHP output constraint
- 6.
- Power output constraint
4. Solution Methodology
4.1. Reformulation
4.2. Algorithm Steps
5. Results and Discussion
5.1. Experimental Settings
5.2. Energy Storage Configuration Analysis
5.3. Configuration Results under Different Time-of-Use (TOU)
5.4. MG Power Purchase and Sale Results
5.5. MG Scheduling Results
6. Conclusions
- From the perspective of energy storage plants, the return on investment is directly related to the price of electricity. The high price of electricity and its high return does not mean high investment; on the contrary, a small investment is verified from several angles. This means that the investment is small, but that the return is high, which is related to the community having the necessary equipment. Therefore, when building an energy storage power plant, quantitative calculations need to be made based on community-specific equipment;
- The importance of resource endowment, from a community perspective, is closely related to the community’s equipment configuration. In resource-rich areas, the benefits of transmitting electricity outward and matching gas turbines with refrigeration are seen in the difference between the benefits of selling electricity and the cost of gas, further validating the profitability of investing in renewable energy. The specific return difference is quantifiable and can be calculated; in resource-poor areas, equipment such as gas turbines are particularly important, and purchasing large amounts of electricity and gas turbines to complement each other is an effective way through which to achieve affordability.
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kaldate, A.; Kanase-Patil, A.; Bewoor, A.; Kumar, R.; Lokhande, S.; Sharifpur, M.; PraveenKumar, S. Comparative feasibility analysis of an integrated renewable energy system (IRES) for an urban area. Sustain. Energy Technol. 2022, 54, 102795. [Google Scholar] [CrossRef]
- PraveenKumar, S.; Agyekum, E.B.; Kumar, A.; Velkin, V.I. Performance evaluation with low-cost aluminum reflectors and phase change material integrated to solar PV modules using natural air convection: An experimental investigation. Energy 2023, 266, 126415. [Google Scholar] [CrossRef]
- Praveenkumar, S.; Agyekum, E.B.; Kumar, A.; Velkin, V.I. Thermo-enviro-economic analysis of solar photovoltaic/thermal system incorporated with u-shaped grid copper pipe, thermal electric generators and nanofluids: An experimental investigation. J. Energy Storage 2023, 60, 106611. [Google Scholar] [CrossRef]
- Li, Z.; Wu, L.; Xu, Y.; Moazeni, S.; Tang, Z. Multi-Stage Real-Time Operation of a Multi-Energy Microgrid With Electrical and Thermal Energy Storage Assets: A Data-Driven MPC-ADP Approach. IEEE Trans. Smart Grid 2022, 13, 213–226. [Google Scholar] [CrossRef]
- Li, Z.; Wu, L.; Xu, Y.; Zheng, X. Stochastic-Weighted Robust Optimization Based Bilayer Operation of a Multi-Energy Building Microgrid Considering Practical Thermal Loads and Battery Degradation. IEEE Trans. Sustain. Energy 2022, 13, 668–682. [Google Scholar] [CrossRef]
- Lasemi, M.A.; Arabkoohsar, A.; Hajizadeh, A.; Mohammadi-ivatloo, B. A comprehensive review on optimization challenges of smart energy hubs under uncertainty factors. Renew. Sustain. Energy Rev. 2022, 160, 112320. [Google Scholar] [CrossRef]
- Mansouri, S.A.; Nematbakhsh, E.; Ahmarinejad, A.; Jordehi, A.R.; Javadi, M.S.; Matin, S.A.A. A Multi-objective dynamic framework for design of energy hub by considering energy storage system, power-to-gas technology and integrated demand response program. J. Energy Storage 2022, 50, 104206. [Google Scholar] [CrossRef]
- Mansouri, S.A.; Ahmarinejad, A.; Sheidaei, F.; Javadi, M.S.; Rezaee Jordehi, A.; Esmaeel Nezhad, A.; Catalão, J.P.S. A multi-stage joint planning and operation model for energy hubs considering integrated demand response programs. Int. J. Electr. Power 2022, 140, 108103. [Google Scholar] [CrossRef]
- Karimi, H.; Jadid, S. Multi-layer energy management of smart integrated-energy microgrid systems considering generation and demand-side flexibility. Appl. Energy 2023, 339, 120984. [Google Scholar] [CrossRef]
- Nasir, M.; Jordehi, A.R.; Tostado-Véliz, M.; Tabar, V.S.; Amir Mansouri, S.; Jurado, F. Operation of energy hubs with storage systems, solar, wind and biomass units connected to demand response aggregators. Sustain. Cities Soc. 2022, 83, 103974. [Google Scholar] [CrossRef]
- Mansouri, S.A.; Ahmarinejad, A.; Ansarian, M.; Javadi, M.S.; Catalao, J.P.S. Stochastic planning and operation of energy hubs considering demand response programs using Benders decomposition approach. Int. J. Electr. Power 2020, 120, 106030. [Google Scholar] [CrossRef]
- Nikbakht Naserabad, S.; Rafee, R.; Saedodin, S.; Ahmadi, P. Dynamic thermal analysis and 3E evaluation of a CCHP system integrated with PVT to provide dynamic loads of a typical building in a hot-dry climate. Sustain. Energy Technol. 2022, 52, 101970. [Google Scholar] [CrossRef]
- Fernandez-Blanco, R.; Dvorkin, Y.; Xu, B.; Wang, Y.; Kirschen, D.S. Optimal Energy Storage Siting and Sizing: A WECC Case Study. IEEE Trans. Sustain. Energy 2017, 8, 733–743. [Google Scholar] [CrossRef]
- Saber, H.; Heidarabadi, H.; Moeini-Aghtaie, M.; Farzin, H.; Karimi, M.R. Expansion Planning Studies of Independent-Locally Operated Battery Energy Storage Systems (BESSs): A CVaR-Based Study. IEEE Trans. Sustain. Energy 2020, 11, 2109–2118. [Google Scholar] [CrossRef]
- Dvorkin, Y.; Fernandez-Blanco, R.; Kirschen, D.S.; Pandzic, H.; Watson, J.; Silva-Monroy, C.A. Ensuring Profitability of Energy Storage. IEEE Trans. Power Syst. 2017, 32, 611–623. [Google Scholar] [CrossRef]
- Dvorkin, Y.; Fernandez-Blanco, R.; Wang, Y.; Xu, B.; Kirschen, D.S.; Pandzic, H.; Watson, J.; Silva-Monroy, C.A. Co-Planning of Investments in Transmission and Merchant Energy Storage. IEEE Trans. Power Syst. 2018, 33, 245–256. [Google Scholar] [CrossRef]
- Pandzic, K.; Pandzic, H.; Kuzle, I. Coordination of Regulated and Merchant Energy Storage Investments. IEEE Trans. Sustain. Energy 2018, 9, 1244–1254. [Google Scholar] [CrossRef]
- Kelly, J.J.; Leahy, P.G. Sizing Battery Energy Storage Systems: Using Multi-Objective Optimization to Overcome the Investment Scale Problem of Annual Worth. IEEE Trans. Sustain. Energy 2020, 11, 2305–2314. [Google Scholar] [CrossRef]
- Yan, N.; Zhang, B.; Li, W.; Ma, S. Hybrid Energy Storage Capacity Allocation Method for Active Distribution Network Considering Demand Side Response. IEEE Trans. Appl. Supercon. 2019, 29, 1–4. [Google Scholar] [CrossRef]
- Nikoobakht, A.; Aghaei, J.; Shafie-Khah, M.; Catalao, J.P.S. Assessing Increased Flexibility of Energy Storage and Demand Response to Accommodate a High Penetration of Renewable Energy Sources. IEEE Trans. Sustain. Energ 2019, 10, 659–669. [Google Scholar] [CrossRef]
- Bistline, J.E.T.; Young, D.T. Emissions impacts of future battery storage deployment on regional power systems. Appl. Energy 2020, 264, 114678. [Google Scholar] [CrossRef]
- Larsen, M.; Sauma, E. Economic and emission impacts of energy storage systems on power-system long-term expansion planning when considering multi-stage decision processes. J. Energy Storage 2021, 33, 101883. [Google Scholar] [CrossRef]
- Olsen, D.J.; Kirschen, D.S. Profitable Emissions-Reducing Energy Storage. IEEE Trans. Power Syst. 2020, 35, 1509–1519. [Google Scholar] [CrossRef]
- Olsen, D.J.; Dvorkin, Y.; Fernandez-Blanco, R.; Ortega-Vazquez, M.A. Optimal Carbon Taxes for Emissions Targets in the Electricity Sector. IEEE Trans. Power Syst. 2018, 33, 5892–5901. [Google Scholar] [CrossRef]
- Martinez Cesena, E.A.; Loukarakis, E.; Good, N.; Mancarella, P. Integrated Electricity—Heat—Gas Systems: Techno—Economic Modeling, Optimization, and Application to Multienergy Districts. Proc. IEEE 2020, 108, 1392–1410. [Google Scholar] [CrossRef]
- Kim, H.; Jung, Y.; Oh, J.; Cho, H.; Heo, J.; Lee, H. Development and evaluation of an integrated operation strategy for a poly-generation system with electrical and thermal storage systems. Energy Convers. Manag. 2022, 256, 115384. [Google Scholar] [CrossRef]
- Ghersi, D.E.; Amoura, M.; Loubar, K.; Desideri, U.; Tazerout, M. Multi-objective optimization of CCHP system with hybrid chiller under new electric load following operation strategy. Energy 2021, 219, 119574. [Google Scholar] [CrossRef]
- Tao, Y.; Qiu, J.; Lai, S.; Zhao, J. Integrated Electricity and Hydrogen Energy Sharing in Coupled Energy Systems. IEEE Trans. Smart Grid 2021, 12, 1149–1162. [Google Scholar] [CrossRef]
- Zhang, S.; Wang, S.; Zhang, Z.; Lyu, J.; Cheng, H.; Huang, M.; Zhang, Q. Probabilistic Multi-Energy Flow Calculation of Electricity–Gas Integrated Energy Systems with Hydrogen Injection. IEEE Trans. Ind. Appl. 2022, 58, 2740–2750. [Google Scholar] [CrossRef]
- Xu, D.; Zhou, B.; Wu, Q.; Chung, C.Y.; Li, C.; Huang, S.; Chen, S. Integrated Modelling and Enhanced Utilization of Power-to-Ammonia for High Renewable Penetrated Multi-Energy Systems. IEEE Trans. Power Syst. 2020, 35, 4769–4780. [Google Scholar] [CrossRef]
- Dolatabadi, A.; Mohammadi-ivatloo, B.; Abapour, M.; Tohidi, S. Optimal Stochastic Design of Wind Integrated Energy Hub. IEEE Trans. Ind. Inform. 2017, 13, 2379–2388. [Google Scholar] [CrossRef]
- Senemar, S.; Rastegar, M.; Dabbaghjamanesh, M.; Hatziargyriou, N. Dynamic Structural Sizing of Residential Energy Hubs. IEEE Trans. Sustain. Energy 2020, 11, 1236–1246. [Google Scholar] [CrossRef]
- Pazouki, S.; Haghifam, M. Optimal planning and scheduling of energy hub in presence of wind, storage and demand response under uncertainty. Int. J. Electr. Power 2016, 80, 219–239. [Google Scholar] [CrossRef]
- Shengjun, W.U.; Qun, L.I.; Jiankun, L.; Qian, Z.; Chenggen, W. Bi-level Optimal Configuration for Combined Cooling Heating and Power Multi-microgrids Based on Energy Storage Station Service. Power Syst. Technol. 2021, 45, 3822–3829. [Google Scholar]







| Time | Electricity Price (CNY/kW·h) | |||
|---|---|---|---|---|
| Buy from Grid | Buy from ES | Sell to ES | ||
| Peak | 08:00–12:00 | 1.35 | 1.15 | 0.90 |
| 17:00–21:00 | ||||
| Flat | 12:00–17:00 | 0.80 | 0.75 | 0.55 |
| 21:00–24:00 | ||||
| valley | 00:00–08:00 | 0.35 | 0.40 | 0.20 |
| ES Capacity | Max Power | Revenue | Investment | Maintenance | Payback | |
|---|---|---|---|---|---|---|
| Scheme 1 | 6946.4 | 2605.7 | 445 | 1122.3 | 15.6 | 2.6 |
| Scheme 2 | 5579.9 | 2093.1 | 470 | 901.5 | 12.6 | 2.0 |
| ES Capacity | Max Power | Revenue | Investment | Maintenance | Payback | |
|---|---|---|---|---|---|---|
| TOU 1 | 6946.4 | 2605.7 | 445.0 | 1122.3 | 15.6 | 2.6 |
| TOU 2 | 4460.1 | 1673.1 | 327.2 | 720.6 | 10.0 | 2.3 |
| TOU 3 | 5201.2 | 1951.1 | 481.4 | 840.3 | 11.7 | 1.8 |
| Time | TOU 1 | TOU 2 | TOU 3 | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Grid | ES_Sell | ES_Buy | Grid | ES_Sell | ES_Buy | Grid | ES_Sell | ES_Buy | ||
| Peak | 08:00–12:00 | 1.35 | 1.15 | 0.90 | 1.15 | 1.00 | 0.85 | 1.50 | 1.20 | 0.95 |
| 17:00–21:00 | ||||||||||
| Flat | 12:00–17:00 | 0.80 | 0.75 | 0.55 | 0.75 | 0.70 | 0.50 | 0.90 | 0.85 | 0.65 |
| 21:00–24:00 | ||||||||||
| valley | 00:00–08:00 | 0.35 | 0.40 | 0.20 | 0.3 | 0.35 | 0.15 | 0.40 | 0.50 | 0.30 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L. Merchant Energy Storage Investment Analysis Considering Multi-Energy Integration. Energies 2023, 16, 4695. https://doi.org/10.3390/en16124695
Wang L. Merchant Energy Storage Investment Analysis Considering Multi-Energy Integration. Energies. 2023; 16(12):4695. https://doi.org/10.3390/en16124695
Chicago/Turabian StyleWang, Long. 2023. "Merchant Energy Storage Investment Analysis Considering Multi-Energy Integration" Energies 16, no. 12: 4695. https://doi.org/10.3390/en16124695
APA StyleWang, L. (2023). Merchant Energy Storage Investment Analysis Considering Multi-Energy Integration. Energies, 16(12), 4695. https://doi.org/10.3390/en16124695









