1. Introduction
Various industrial policies are currently being reviewed in response to a range of pressing environmental issues, energy crises, and sluggish economic growth. Among these policies, low carbon green growth has emerged as a particularly promising solution that has generated significant attention and interest across multiple industries and sectors. The concept of low carbon green growth is predicated on the idea that economic and environmental goals need not be mutually exclusive, and that by pursuing sustainable, low carbon strategies, it is possible to achieve both simultaneously. Hydrogen has recently attracted attention as a potential clean energy source, with governments and industries around the world investing in research and development to explore its potential as a low carbon fuel. As such, this policy alternative, along with the potential of hydrogen as a clean energy source, has become a focus of intense interest and debate within the industrial community, as decision makers and stakeholders seek to identify the most effective and efficient means of addressing the pressing challenges facing society today [
1,
2].
Hydrogen can be used in its natural state or synthesized from other energy sources. Most hydrogen has been manufactured through steam reforming reactions or from other fossil fuels such as natural gas and propane. Specifically, hydrogen is generated through a process that involves water vapor and fossil fuels at high temperatures, or through the use of metal based catalysts (typically nickel), although these methods have been found to have disadvantages such as low purity and reliance on fossil fuels. To address these shortcomings, the water based hydrogen production method, which produces hydrogen and oxygen, has recently been attracting attention. While hydrogen production technology using water electrolysis have not received much attention due to its high power consumption, the outlook for hydrogen production is changing rapidly with increased energy capacity based on renewable energy sources such as solar cell and wind power generation. As such, the future of hydrogen production is rapidly evolving, with new and innovative approaches being explored in order to meet the growing demand for clean, sustainable energy [
3].
As the global interest in water electrolysis technology continues to escalate, significant research and development endeavors are actively underway. However, despite its immense potential, the technology is currently insufficient in fully replacing conventional carbon based energy sources due to the intermittent power generation characteristics of new and renewable energy sources, alongside the various stability and long-term operation challenges posed by water electrolysis systems that rely on these sources [
4,
5,
6]. Resolving these challenges is crucial for enabling the commercialization of green hydrogen production. Achieving this goal necessitates securing the stability and performance of the water electrolysis system through extensive research and technology development, aimed at augmenting the hydrogen purity rate. During the process of separating water into hydrogen within the electrolysis system, impurities such as hydrogen, oxygen, and water coexist, necessitating their elimination to increase the hydrogen purity. To remove water from the impurities, a commonly used adsorption method employing pressure swing adsorption (PSA), or thermal swing adsorption (TSA) can be utilized [
7].
In this study, we employed a dryer method using TSA to enhance the hydrogen purity rate in water electrolysis systems. Unlike pressure regeneration adsorption processes, which rely on periodic pressure changes, thermal regenerative adsorption processes maintain constant pressure and use temperature differences to control adsorption and detachment [
8,
9]. The thermal regenerative adsorption process typically comprises three stages: adsorption, removal, and cooling. During the adsorption stage, the adsorbent in the tower selectively separates the mixture into its constituents, with fewer desirable ones being concentrated and discharged from the tower. The desorption process raises the temperature in the tower, allowing for the removal of the adsorbate (water), after which the adsorbent is cooled to prepare for the next cycle. Efficient operation of the thermal regenerative adsorption process depends on the cost of thermal energy, and lower operating temperatures during adsorption can enhance adsorption performance and selectivity. However, overloading the adsorbent requires a substantial amount of thermal energy for effective removal. The adsorbent is typically removed using a flow of hot steam or gas, and effective removal can be achieved through a large dehumidification flow rate at a relatively low temperature or by utilizing a low detachment flow rate at a relatively high temperature.
Tjarks et al. [
10] investigate the energy demand of power-to-gas plants that produce hydrogen through electrolysis, gas drying, and compression. The study considers temperature swing adsorption (TSA) for gas drying, which is shown to be more efficient for low adsorbate concentrations in the feed stream. The study then determines the optimal electrolysis pressure levels for an efficient operation of the power-to-gas plant by modeling each process step to calculate the specific energy demand and combining the steps to determine the specific energy demand of the complete process chain. Bensmann et al. [
11] evaluate the energy demand of power-to-gas plants and finds that the optimal configuration and pressure levels depend on hydrogen delivery pressure and humidity requirements. The energy demand for drying is the dominant factor at low delivery pressure, and higher electrolysis pressures increase losses due to hydrogen crossover. Mechanical compression before drying can reduce energy demand, and keeping electrolysis pressure below 20 bar can decrease hydrogen crossover and enable efficient drying at high pressures. Lively et al. [
12] discuss a thermodynamic analysis of adsorption units for gas separation systems, comparing the efficiency of TSA and PSA for dilute gas separations and bulk gas separations, respectively. It is found that TSA is more efficient at low feed concentrations, while PSA is more efficient for bulk gas separations. The analysis did not consider practical process realities or energy recovery options. Tao et al. [
13] conducted to purify hydrogen from a multi-component mixture (N
2/CO/CO
2/CH
4/H
2) using a one column VPSA process with AC5-KS. A heat and mass transfer model with porous media adsorption was used to predict the performance of the VPSA process, and the simulation results closely matched experimental data. The model was utilized to analyze the effects of the P/F ratio and adsorption time in the VPSA unit. The model assessed the effects of the P/F ratio and adsorption time, revealing that increasing the P/F ratio and decreasing the feeding time led to higher hydrogen purity but lower recovery. Ye et al. [
14] investigated that a PSA cycle model was implemented on the Aspen Adsorption platform to simulate the PSA process for a ternary component gas mixture (H
2/CO
2/CO) using a Cu-BTC adsorbent bed. Increasing adsorption pressure improved hydrogen purity but reduced recovery while extending adsorption time and lowering product flow rate increased recovery but decreased purity. Sakas et al. [
15] developed a simulation model for optimizing cost and energy efficiency in an alkaline water electrolysis plant. They used MATLAB functions and Simulink to solve the energy and mass balances of the 3 MW, 16 bar plant. A TSA type dryer was used for moisture adsorption, achieving a minimum threshold moisture content through condensation drying at an ambient temperature of 25–30 °C. The thermal model accurately predicted the real-world scenario with 98.7% accuracy.
The TSA process is employed for gas purification across various systems, and a plethora of studies are being conducted in this field. Aleghafouri et al. [
16] focused on developing a comprehensive mathematical model to predict the performance of gas dehydration in natural gas and conducted a parametric study to examine the effects of various operational parameters. The study investigated the influential parameters through a parametric analysis of process efficiency. To achieve energy savings, the researchers proposed some suggestions for improving the design conditions without causing a significant impact on the purification performance. Ambrożek et al. [
17] conducted an analysis of adsorption drying for specific water-aliphatic alcohol solutions using mathematical modeling and experimental techniques. The developed isothermal model in this study incorporated various factors, such as liquid film mass transport, diffusion coefficients, variable axial diffusion, and experimentally derived Langmuir-Freundlich isotherms. The model’s calculated results demonstrated good agreement with the experimental data, and the simulations provided insights into the moisture content profile within the adsorbent bed. In previous studies, numerous models and analysis studies have been conducted to predict the performance of TSA adsorption in various systems. However, there is a lack of research on TSA dryers specifically designed for water electrolysis systems. Therefore, it is crucial to conduct research on models for TSA based dryers used in hydrogen purification processes with PEM electrolysis cells to optimize their performance. There is a scarcity of research focusing on heat transfer characteristics, whereas many studies have investigated the impact of operating conditions such as supply flow rate, duration, and pressure on adsorption characteristics. It is essential to understand these characteristics to predict the optimal temperature and arrival time of the drying tower.
This paper focuses on investigating the heat source capacity and the necessary duration to achieve and sustain the optimal temperature within the drying tower in a hydrogen purification process system. The purity of hydrogen is significantly influenced by the purification temperature and the uniformity of temperature within the drying tower. Therefore, understanding the heat source capacity and determining the duration required to reach and maintain the optimal temperature is crucial for ensuring the highest possible hydrogen purity. A numerical analysis of the heat transfer characteristics has been conducted for the dryer tower, utilizing Zeolite as an adsorbent. Based on the analysis results, a code capable of predicting transient heat transfer was meticulously developed using Visual Basic for Applications (VBA). Performing heat transfer analysis of a dryer using a commercial program can be time consuming and challenging for beginners to optimize the design of the drying tower. However, employing an in-house code enables a more efficient design process, specifically tailored to the capacity of the water electrolysis system. This approach significantly reduces the design time for the drying tower while ensuring simplicity and ease of operation.
3. Results and Discussion
Figure 6 presents the temperature history over time as the density value of Alumina changes from 780 kg/m
3 to 3850 kg/m
3. In the figure, 780_TA and 3850_TA represent the temperature histories of densities of 780 kg/m
3 and 3850 kg/m
3, respectively, over time, while 780_AL and 3850_AL signify the temperature histories of Alumina with densities of 780 kg/m
3 and 3850 kg/m
3, respectively. As observed in
Figure 6, the slope of the temperature history concerning time change increases steeply with decreasing density.
Figure 7 displays the overall layout of the in-house code alongside the input window. Upon scrutinizing the main input window, the “Time Interval of On-Off Cycle” represents the duration of On and Off cycles, following a specified heat load supplied by the heater jacket. The “Number of On-Off Cycles” specifies the repetition count of On-Off cycles, with the in-house code operating at N = 6 and N = 10 exclusively. N = 6 and N = 10 imply that the cumulative number of Off and On cycles is either 6 or 10, respectively. Furthermore, “Supplied Heat at On-Off Cycle” denotes the heat quantity provided by the heater jacket during the On-Cycle, while “Cooling Flow” is an input field for the cooling flow rate, which cools the drying tower upon completion of the On-Off Cycle. Lastly, the “Pi Factor for Convection Coefficient” serves as an input field for adjusting the convective heat transfer coefficient of the cooling flow, by inputting a coefficient value.
Figure 8 presents the regeneration process, which encompasses desorption, drying, and cooling stages. T_time denotes the total duration for which the heater jacket supplies 2000 W, whereas N×D_time signifies the cumulative time during which the heater jacket operates within an On-Off Cycle. Additionally, 3N×D_time, a period when the heat supply from the heater jacket is ‘0’, corresponds to the time allocated for drying and cooling. Thus, the comprehensive operation time applied to this program is the sum of T_time and N×D_time, and 3N×D_time, as demonstrated in the figure.
To validate the final in-house code, calculations were performed for two distinct cases.
Table 1 displays the respective input values for Case 1 and Case 2.
The objective of our in-house code was to predict the test data we gathered, as demonstrated in
Figure 9a,b. These figures present an analysis of the system’s performance in Case 1 and Case 2, respectively. In
Figure 9a, the heater jacket’s supply heat source was 1000 W, leading to a time of approximately 1400 s to reach 360 °C. Conversely, in
Figure 10b, the supplied heat source to the heater jacket was increased to 2000 W, resulting in a time of around 710 s to reach 360 °C. After the system underwent an On-Off cycle for a designated duration, the temperature of the adsorbent and H
2 increased in both Case 1 (
Figure 9a) and Case 2 (
Figure 9b). After supplying the cooling fluid, we observed a temperature jump in both the adsorbent and H
2 phases. As a result, when the cooling fluid is supplied to the dryer, the convective heat transfer coefficient rises in accordance with an increase in flow rate. The adsorbent and H
2 absorb more heat from the body than during the On-Off Cycle process, leading to a temperature increase. After that, the adsorbent and H
2 are immediately cooled. The convective heat transfer coefficient has a significant effect on the internal temperature during the dryer’s regeneration process.
Verification of In-house code was accomplished using an analogous industrial plant of the same power having the same operating systems design. The dryer’s thermal behavior of the numerical results and the water electrolysis plant were compared to ascertain the accuracy of the model.
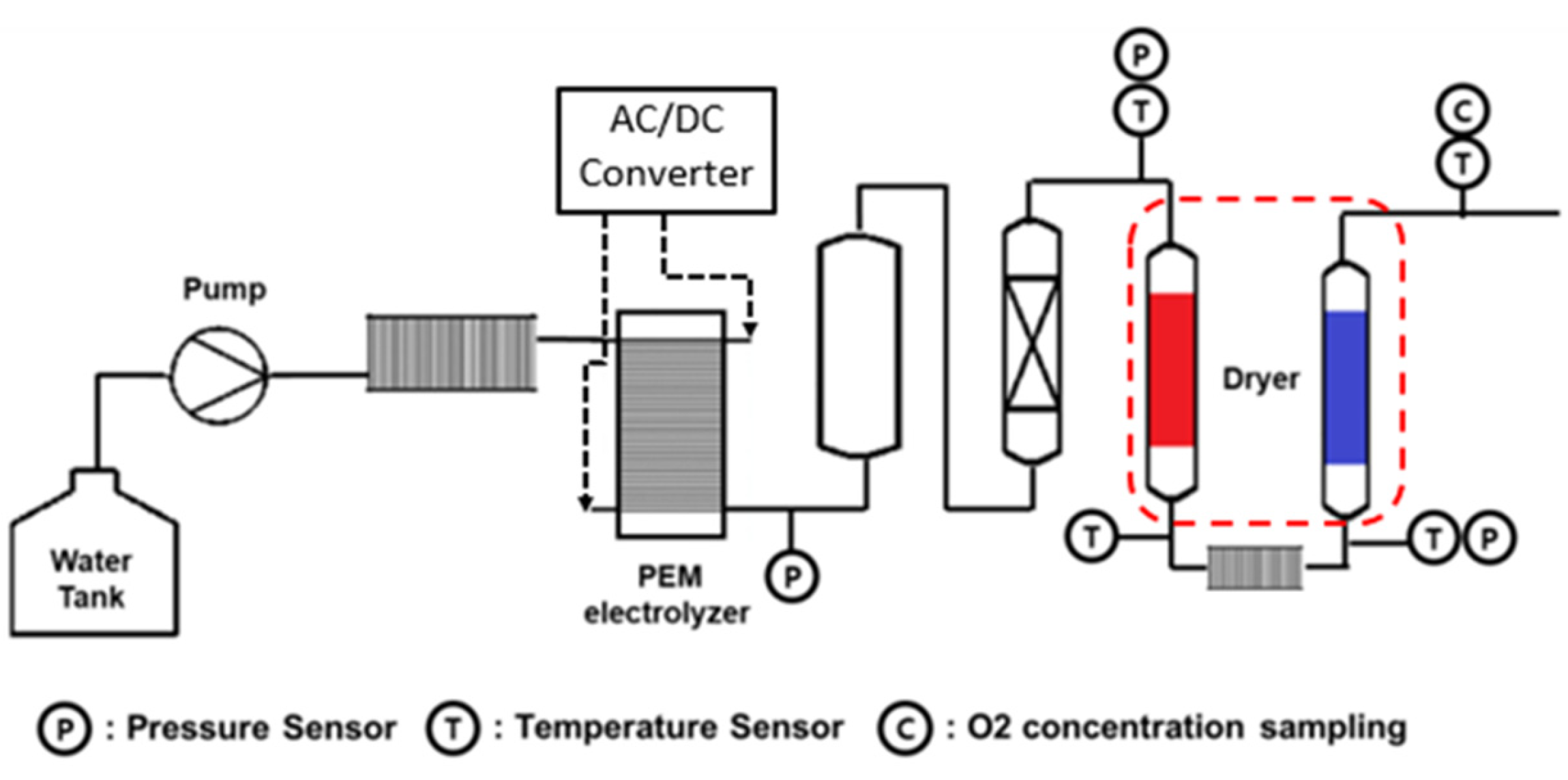
Figure 10a,b show the experimental results acquired from the water electrolysis system test, which is illustrated in
Figure 1. As demonstrated in
Figure 10a, the temperature of the heater rises to around 360 °C. As depicted in
Figure 10a, over a heating process of 701 s, the temperature of the drying tower heater rises to approximately 360 °C. When the heater reaches this temperature, it begins an on-off cycle during the regeneration process. After this cycle, the heater is switched off, while the supply of cooling fluid to the dryer continues. From the measured adsorbent temperature in
Figure 10b, the temperature discontinuity caused by the inflow of the cooling fluid, as demonstrated in the calculations in
Figure 9, also appears within the actual dryer. The actual heating time (T_time) recorded was 701 s, which deviated by approximately 9 s (1.28%) from the 710 s of Case 2. Following the heating process, the temperature measured in the dryer heater was 366.5 °C, while the analysis conducted using the in-house code yielded a result of 372.1 °C, indicating an error of approximately 6 °C (1.64%). In addition, comparing the temperatures at the upper position inside the dryer, it was observed that the actual temperatures of the adsorbent and H
2 increased rapidly from 187.1 °C to 203.7 °C when the cooling flow was introduced. In contrast, the analysis result showed a temperature range of 196.4 °C (4.97%) to 212.7 °C (4.42%), resulting in a difference of approximately 10 °C from the experimental results. During the heating process, it was observed that the temperature values measured in the experiment were consistently 6 °C to 10 °C lower than the values obtained through analysis. This discrepancy can be attributed to heat loss occurring during the transfer of heat from the actual heat jacket (heat source) to the dryer tower. Upon completion of the regeneration heating, the actual cooling time of the dryer tower was approximately 5300 s, whereas the calculated result showed approximately 4600 s. This discrepancy arises from differences between the cooling flow input values considered in the analysis and the fluctuations in temperature and flow rate of the gas supplied for cooling in the actual system.