Lowering the Temperature and Increasing the Fill Factor of Silicon Solar Cells by Filtering of Sub-Bandgap Wavelengths
Abstract
:1. Introduction
2. Experimental Setup
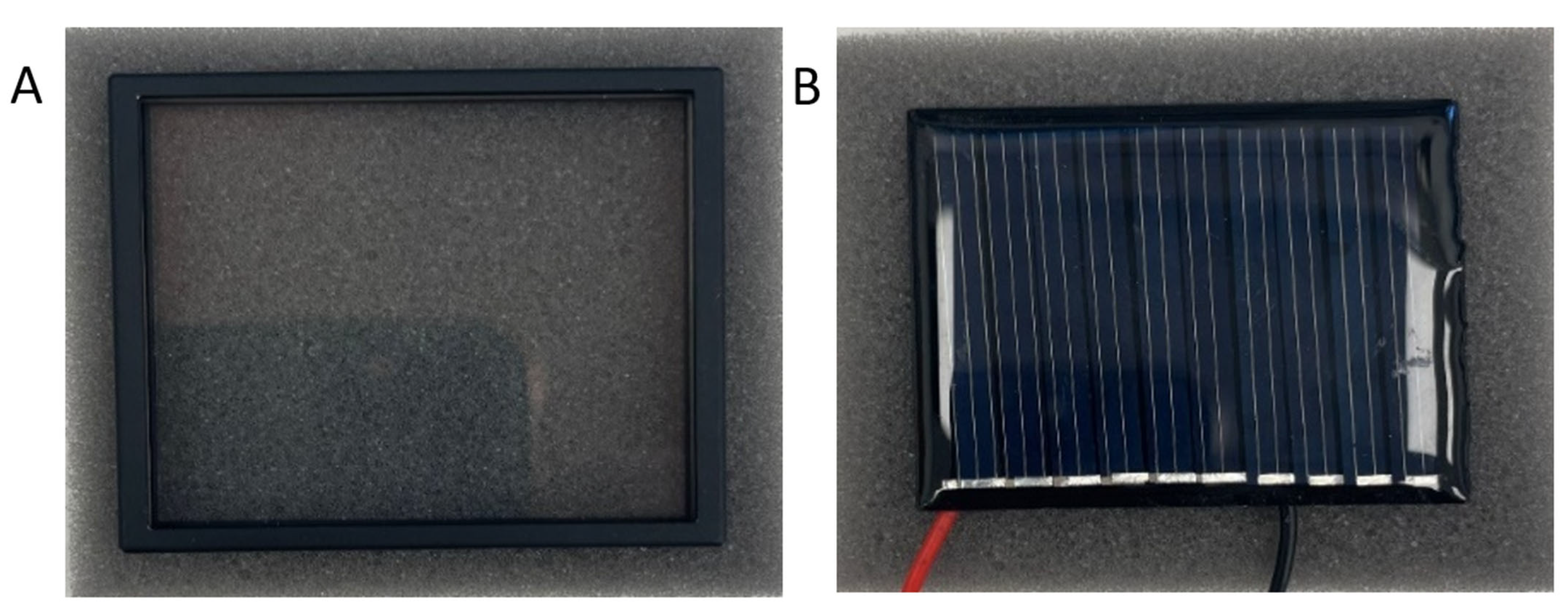
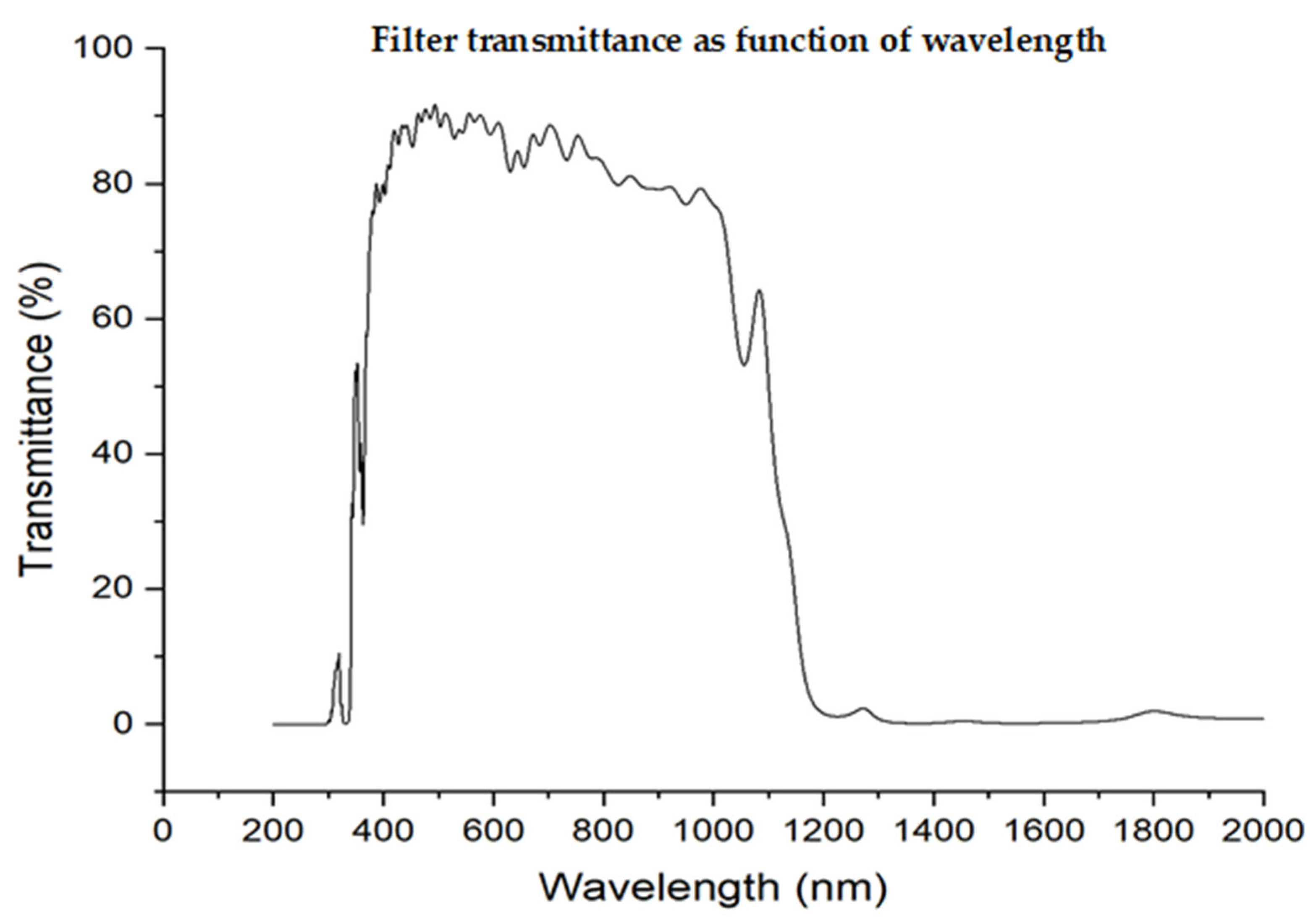
2.1. Optical Absorption Measurements
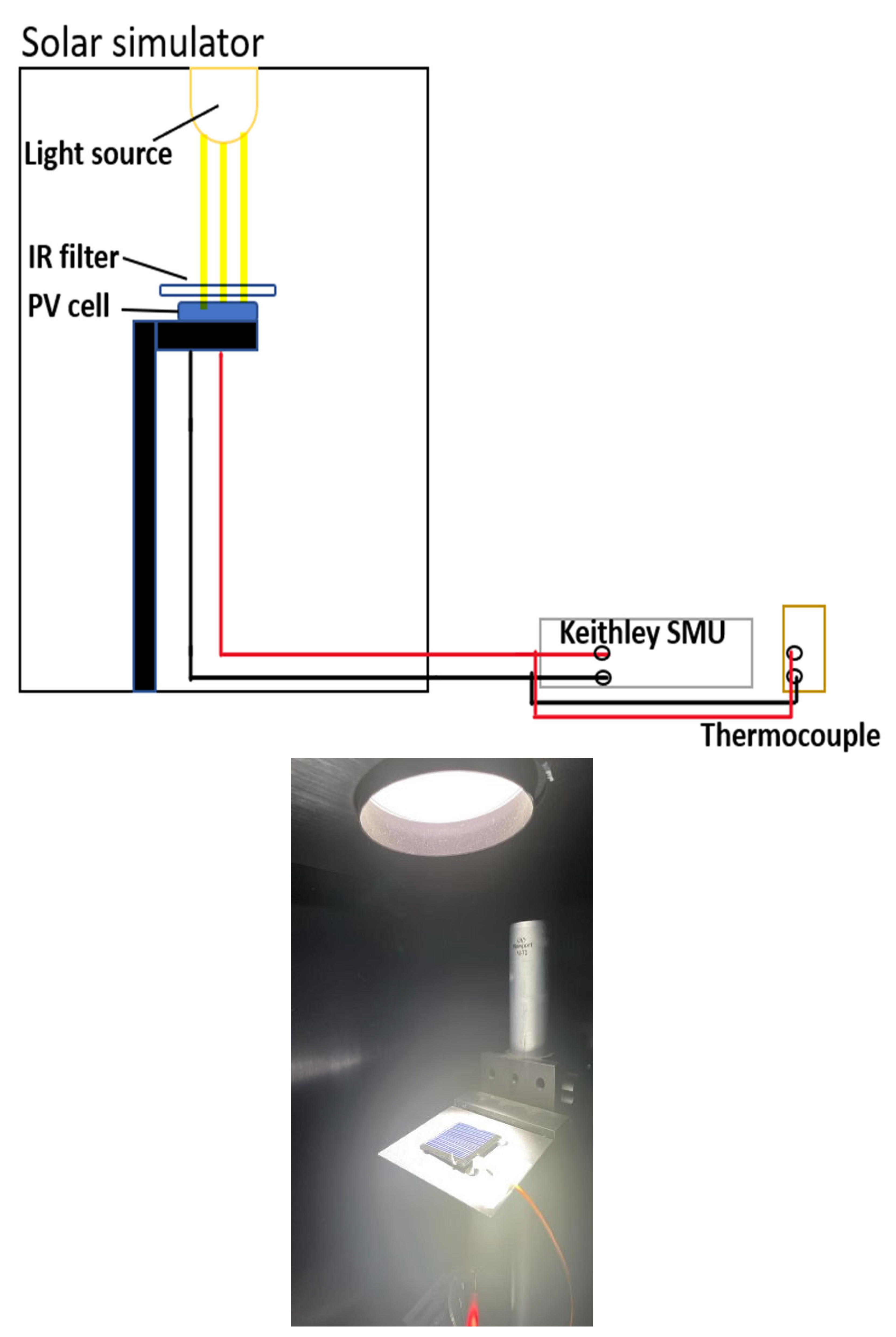
2.2. I–V Measurements
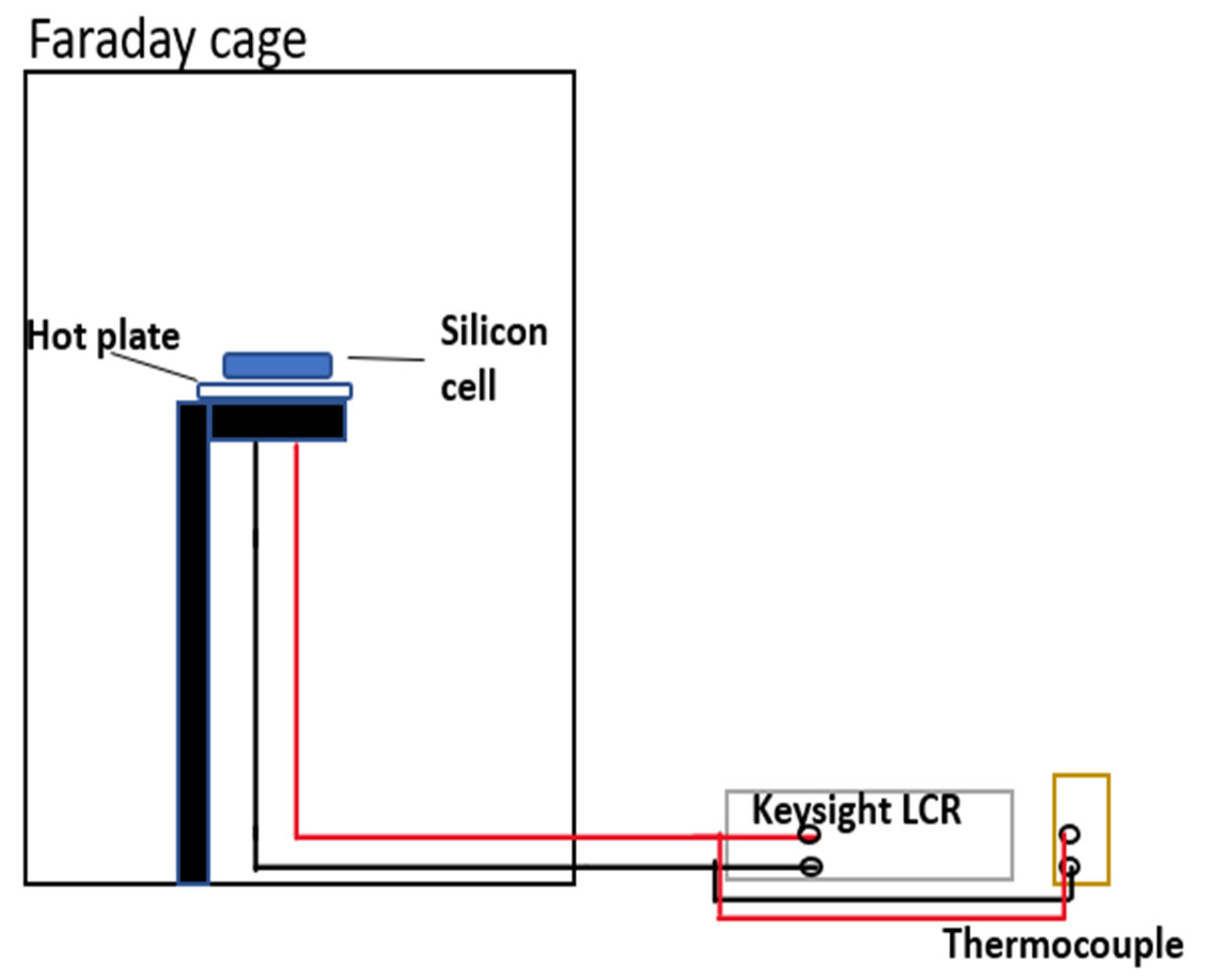
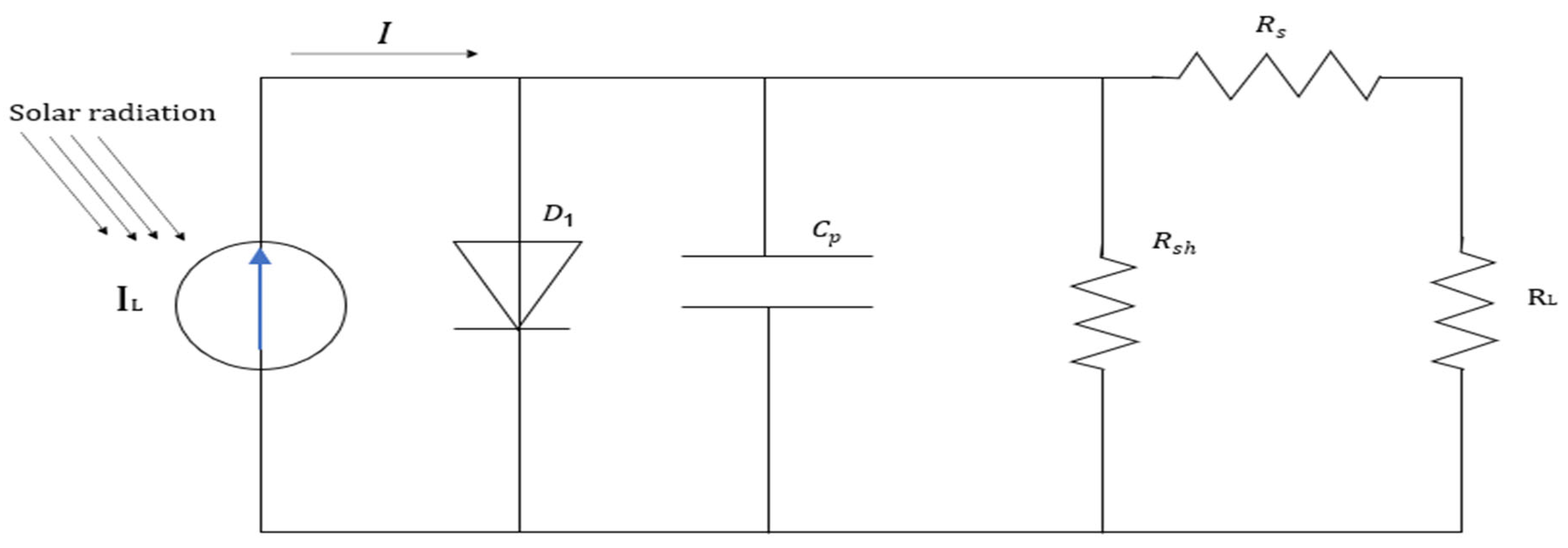
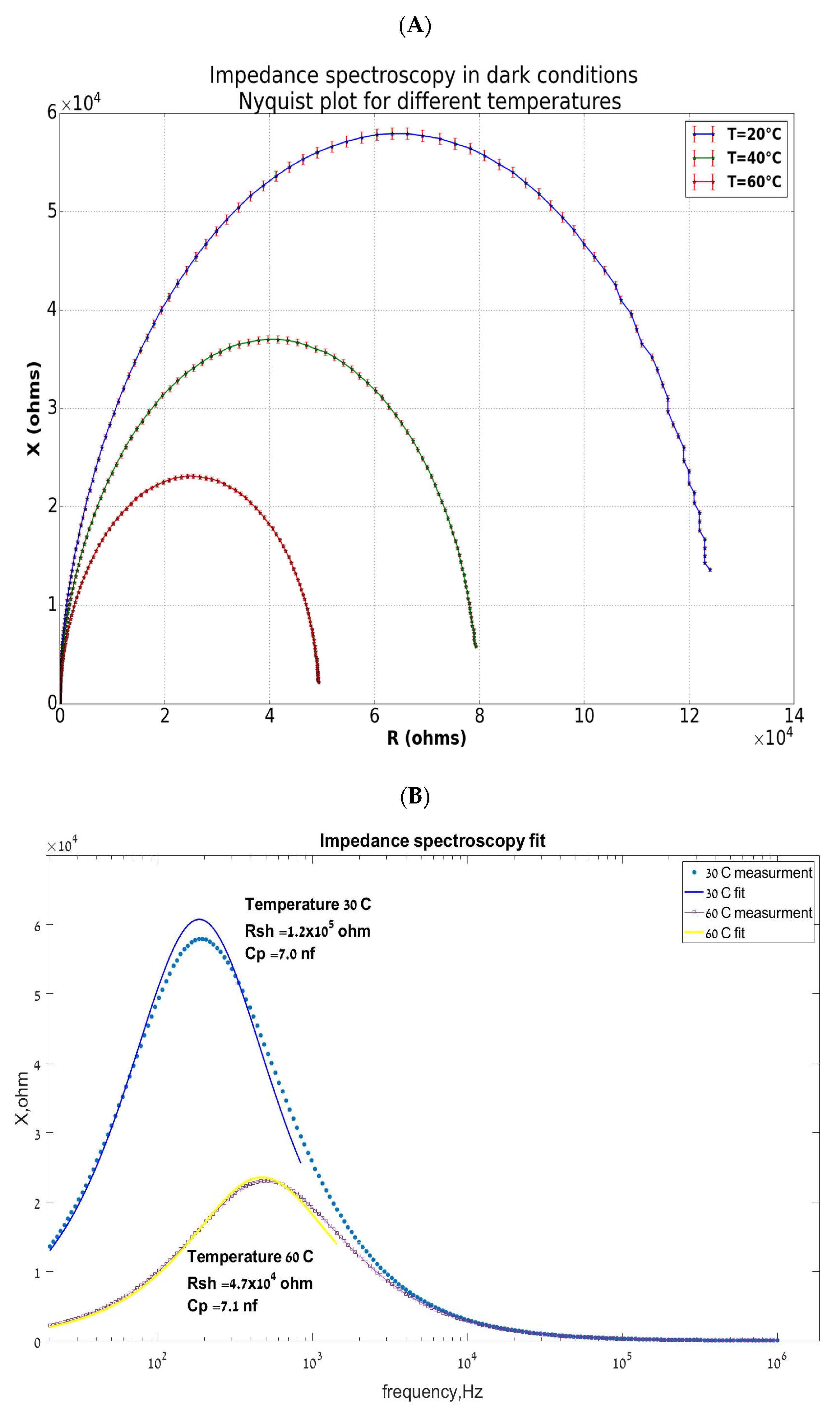
2.3. Impedance Spectroscopy
2.4. Air-SEM Microscope Measurements
3. Results and Discussion
3.1. Transmittance Results
3.2. Dark Test Results
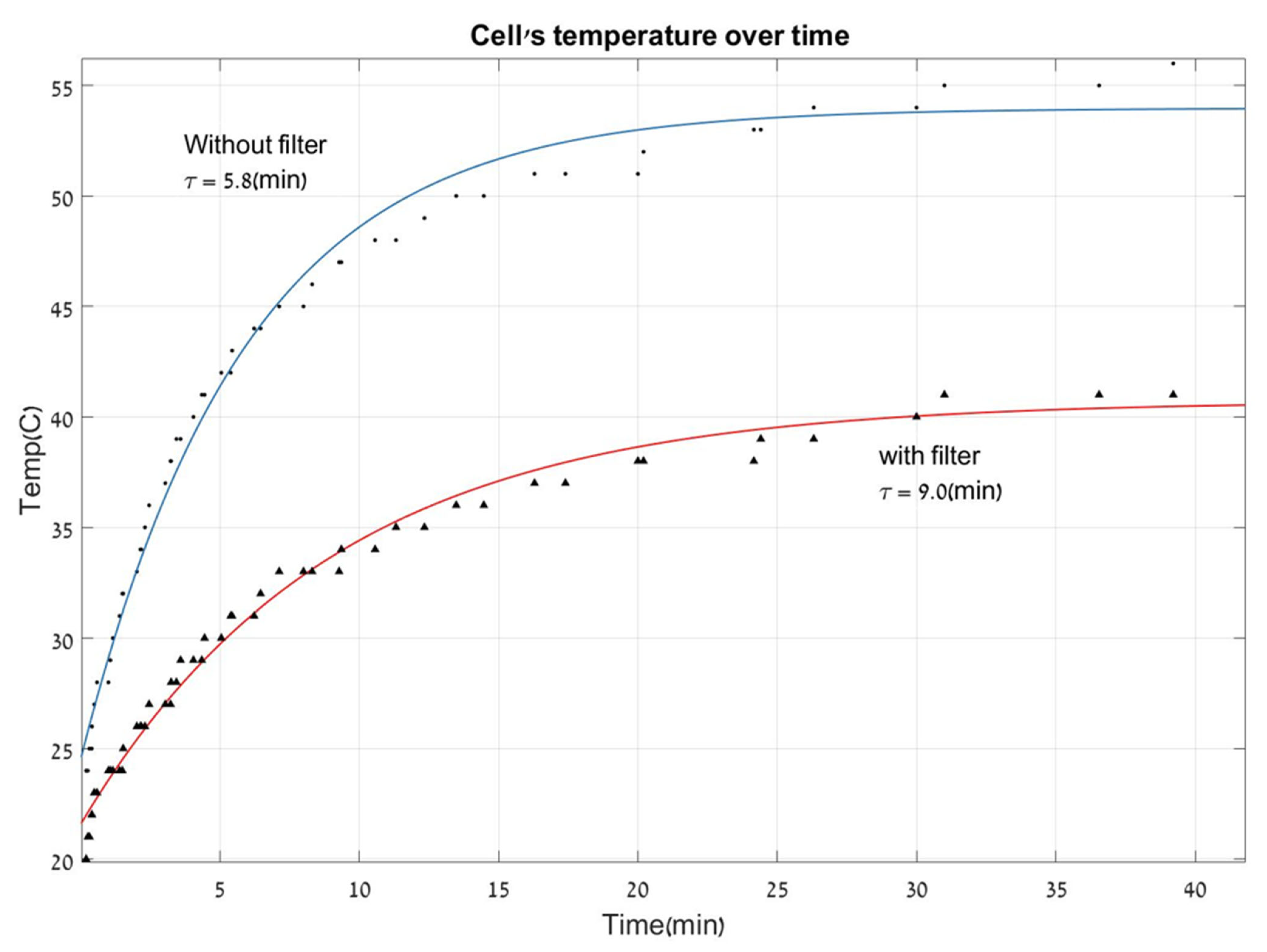
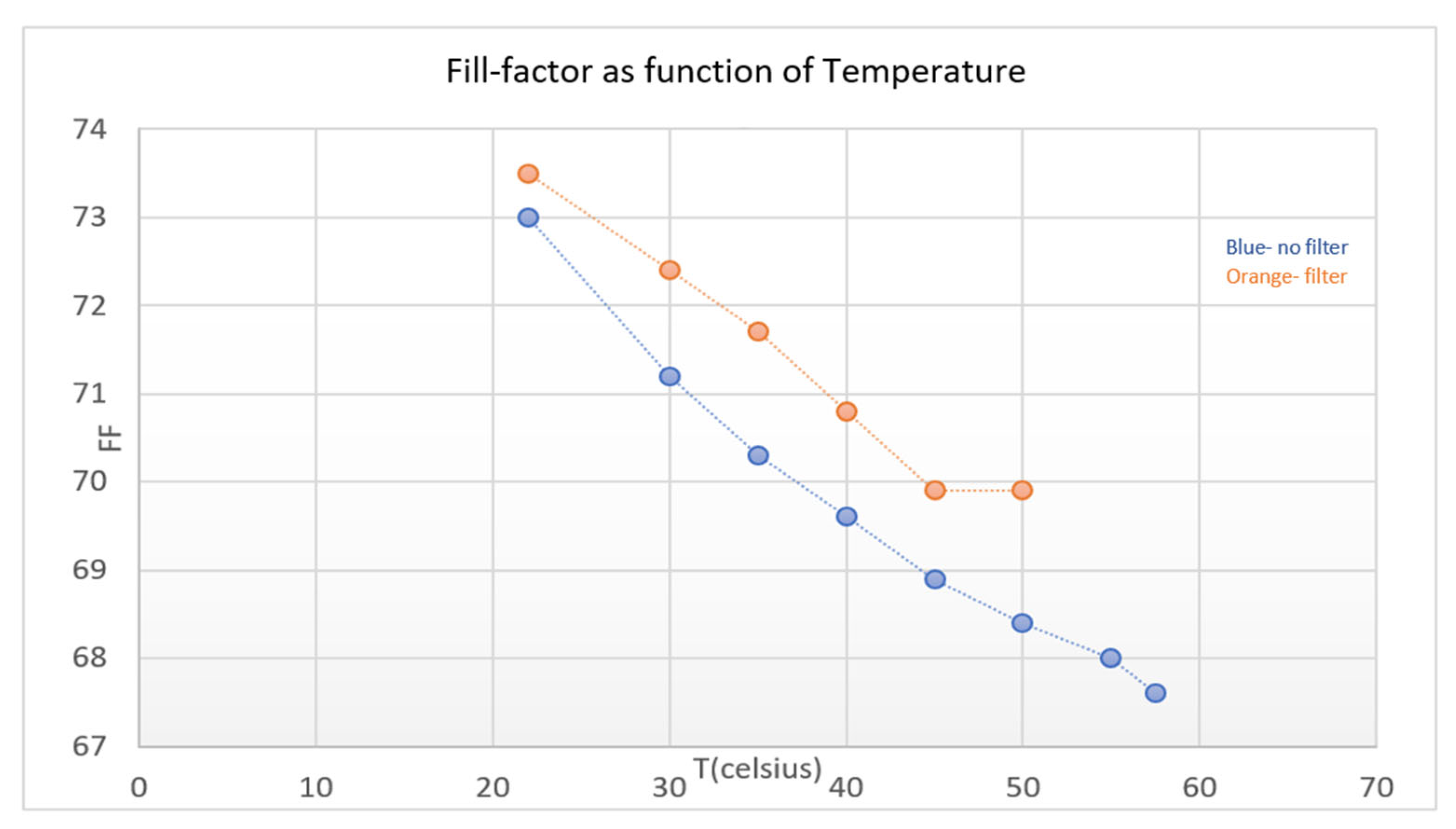
3.3. Illumination Test Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tawalbeh, M.; Al-Othman, A.; Kafiah, F.; Abdelsalam, E.; Almomani, F.; Alkasrawi, M. Environmental impacts of solar photovoltaic systems: A critical review of recent progress and future outlook. Sci. Total Environ. 2021, 759, 143528. [Google Scholar] [CrossRef] [PubMed]
- Baharwani, V.; Meena, N.; Dubey, A.; Brighu, U.; Mathur, J. Life cycle analysis of solar PV system: A review. Int. J. Environ. Res. Dev. 2014, 4, 183–190. [Google Scholar]
- Fthenakis, V.M.; Kim, H.C. Photovoltaics: Life-cycle analyses. Sol. Energy 2011, 85, 1609–1628. [Google Scholar] [CrossRef]
- Hosenuzzaman, M.; Rahim, N.A.; Selvaraj, J.; Hasanuzzaman, M.; Malek, A.B.M.A.; Nahar, A. Global Prospects, Progress, Policies, and Environmental Impact of Solar Photovoltaic Power Generation; Renewable and Sustainable Energy Reviews; Elsevier: Amsterdam, The Netherlands, 2015; Volume 41, pp. 284–297. [Google Scholar]
- Kim, J.; Rabelo, M.; Padi, S.P.; Yousuf, H.; Cho, E.-C.; Yi, J. A Review of the Degradation of Photovoltaic Modules for Life Expectancy. Energies 2021, 14, 4278. [Google Scholar] [CrossRef]
- Cuce, E.; Cuce, P.M.; Bali, T. An experimental analysis of illumination intensity and temperature dependency of photovoltaic cell parameters. Appl. Energy 2013, 111, 374–382. [Google Scholar] [CrossRef]
- Martin, L.; Poulek, V.; Kouřím, P. Temperature changes of IV characteristics of photovoltaic cells as a consequence of the Fermi energy level shift. Res. Agric. Eng. 2017, 63, 10–15. [Google Scholar]
- Kirchartz, T.; Rau, U. What Makes a Good Solar Cell? Adv. Energy Mater. 2018, 8, 1703385. [Google Scholar] [CrossRef] [Green Version]
- Klugmann-Radziemska, E. The effect of temperature on the power drop in crystalline solar cells. Renew. Energy 2003, 28, 1–12. [Google Scholar] [CrossRef]
- Romero-Fiances, I.; Livera, A.; Theristis, M.; Makrides, G.; Stein, J.S.; Nofuentes, G.; de la Casa, J.; Georghiou, G.E. Impact of duration and missing data on the long-term photovoltaic degradation rate estimation. Renew. Energy 2022, 181, 738–748. [Google Scholar] [CrossRef]
- Deceglie, M.G.; Silverman, T.J.; Johnston, S.W.; Rand, J.A.; Reed, M.J.; Flottemesch, R.; Repins, I.L. Light and Elevated Temperature Induced Degradation (LeTID) in a Utility-Scale Photovoltaic System. IEEE J. Photovolt. 2020, 10, 1084–1092. [Google Scholar] [CrossRef]
- Arora, N.D.; Hauser, J.R. Temperature dependence of silicon solar cell characteristics. Sol. Energy Mater. 1982, 6, 151–158. [Google Scholar] [CrossRef]
- Carnel, L.; Gordon, I.; Van Gestel, D.; Beaucarne, G.; Poortmans, J. Hydrogen Passivation of Thin-film Polysilicon Solar Cells. MRS Online Proc. Libr. 2006, 989, 1811. [Google Scholar] [CrossRef]
- Ahmed, A.; Alzahrani, M.; Shanks, K.; Sundaram, S.; Mallick, T.K. Effect of Using an Infrared Filter on the Performance of a Silicon Solar Cell for an Ultra-High Concentrator Photovoltaic System. Mater. Lett. 2020, 277, 128332. [Google Scholar] [CrossRef]
- Yang, W.; Xie, G. The design of UV/IR cut-off filter for silicon solar cell. Art 2006, 6149, 61492Q. [Google Scholar] [CrossRef]
- Chapter 3 Semiconductors. In Book Physics of Solar Cells by Peter Würfel Physics of Solar Cells; Wiley Online Library: Hoboken, NJ, USA, 2005; pp. 37–84. [CrossRef]
- Nguyen, K.; Holtz, M.; Richmond-Decker, J.; Muller, D. Spatial Resolution in Scanning Electron Microscopy and Scanning Transmission Electron Microscopy Without a Specimen Vacuum Chamber. Microsc. Microanal. 2016, 22, 754–767. [Google Scholar] [CrossRef]
- Newport Company Sol3A Calss AAA Solar-Simulators. Available online: https://www.newport.com/f/class-aaa-solar-simulators (accessed on 17 June 2023).
- Levi, L. (Ed.) Applied Optics: A Guide to Optical System Design; Wiley Online Library: Hoboken, NJ, USA, 1968; p. 214. [Google Scholar]
- Garland, J.E.; Crain, D.; Roy, D. Impedance spectroscopy coupled with voltammetry for quantitative evaluation of temperature and voltage dependent parameters of a silicon solar cell. Sol. Energy 2011, 85, 2912–2923. [Google Scholar] [CrossRef]
- von Hauff, E. Impedance Spectroscopy for Emerging Photovoltaics. J. Phys. Chem. C 2019, 123, 11329–11346. [Google Scholar] [CrossRef] [Green Version]
- Priyanka, S.S.N.; Singh, M.; Lal, M.H. Temperature dependence of I–V characteristics and performance parameters of silicon solar cell. Sol. Energy Mater. Sol. Cells 2008, 92, 1611–1616. [Google Scholar] [CrossRef]
- Cotfas, D.T.; Cotfas, P.A.; Machidon, O.M. Study of Temperature Coefficients for Parameters of Photovoltaic Cells. Int. J. Photoenergy 2018, 2018, 5945602. [Google Scholar] [CrossRef] [Green Version]
- Bouzidi, A.; Jilani, W.; Zahran, Y. Impedance spectroscopy of monocrystalline silicon solar cells for photosensor applications: Highly sensitive device. Phys. B Condens. Matter 2020, 596, 412375. [Google Scholar] [CrossRef]
- Mora-Sero, I.; Garcia-Belmonte, G.; Boix, P.P.; Vazquez, M.A.; Bisquert, J. Impedance spectroscopy characterisation of highly efficient silicon solar cells under different light illumination intensities Energy. Environ. Sci. 2009, 2, 678–686. [Google Scholar]
- Dhass, A.D.; Natarajan, E.; Ponnusamy, L. Influence of shunt resistance on the performance of solar photovoltaic cell. In Proceedings of the International Conference on Emerging Trends in Electrical Engineering and Energy Management (ICETEEEM), Chennai, India, 13–15 December 2012; pp. 382–386. [Google Scholar] [CrossRef]
- Chander, S.; Purohit, A.; Sharma, A.; Nehra, S.P.; Dhaka, M.S. A study on photovoltaic parameters of mono-crystalline silicon solar cell with cell temperature. Energy Rep. 2015, 1, 104–109. [Google Scholar] [CrossRef] [Green Version]
- Priyanka Singh, N.M. Ravindra, Temperature dependence of solar cell performance—An analysis. Sol. Energy Mater. Sol. Cells 2012, 101, 36–45. [Google Scholar] [CrossRef]
- Chegaar, M.; Hamzaoui, A.; Namoda, A.; Petit, P.; Aillerie, M.; Herguth, A. Effect of Illumination Intensity on Solar Cells Parameters. Energy Procedia 2013, 36, 722–729. [Google Scholar] [CrossRef]
- Yang, G.; Ren, Z.; Liu, K.; Qin, M.; Deng, W.; Zhang, H.; Wang, H.; Liang, J.; Ye, F.; Liang, Q.; et al. Stable and low-photovoltage-loss perovskite solar cells by multifunctional passivation. Nat. Photon. 2021, 15, 681–689. [Google Scholar] [CrossRef]



| No Filter | Filter | |||||
|---|---|---|---|---|---|---|
| Temperature | 20 | 40 | 60 | 20 | 40 | 50 |
| (V) | 5.88 | 5.54 | 5.33 | 5.81 | 5.60 | 5.39 |
| (A) | 0.0440 | 0.0449 | 0.0455 | 0.035 | 0.036 | 0.037 |
| FF (%) | 73 | 70 | 67 | 73 | 72 | 70 |
| MPP (W) | 0.188 | 0.175 | 0.166 | 0.153 | 0.147 | 0.141 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gindi, O.; Fradkin, Z.; Itzhak, A.; Beker, P. Lowering the Temperature and Increasing the Fill Factor of Silicon Solar Cells by Filtering of Sub-Bandgap Wavelengths. Energies 2023, 16, 5631. https://doi.org/10.3390/en16155631
Gindi O, Fradkin Z, Itzhak A, Beker P. Lowering the Temperature and Increasing the Fill Factor of Silicon Solar Cells by Filtering of Sub-Bandgap Wavelengths. Energies. 2023; 16(15):5631. https://doi.org/10.3390/en16155631
Chicago/Turabian StyleGindi, Or, Zeev Fradkin, Anat Itzhak, and Peter Beker. 2023. "Lowering the Temperature and Increasing the Fill Factor of Silicon Solar Cells by Filtering of Sub-Bandgap Wavelengths" Energies 16, no. 15: 5631. https://doi.org/10.3390/en16155631
APA StyleGindi, O., Fradkin, Z., Itzhak, A., & Beker, P. (2023). Lowering the Temperature and Increasing the Fill Factor of Silicon Solar Cells by Filtering of Sub-Bandgap Wavelengths. Energies, 16(15), 5631. https://doi.org/10.3390/en16155631






