Heating Strategies for Efficient Combined Inductive and Convective Heating of Profiles
Abstract
:1. Introduction
2. Materials and Methods
2.1. Experiments
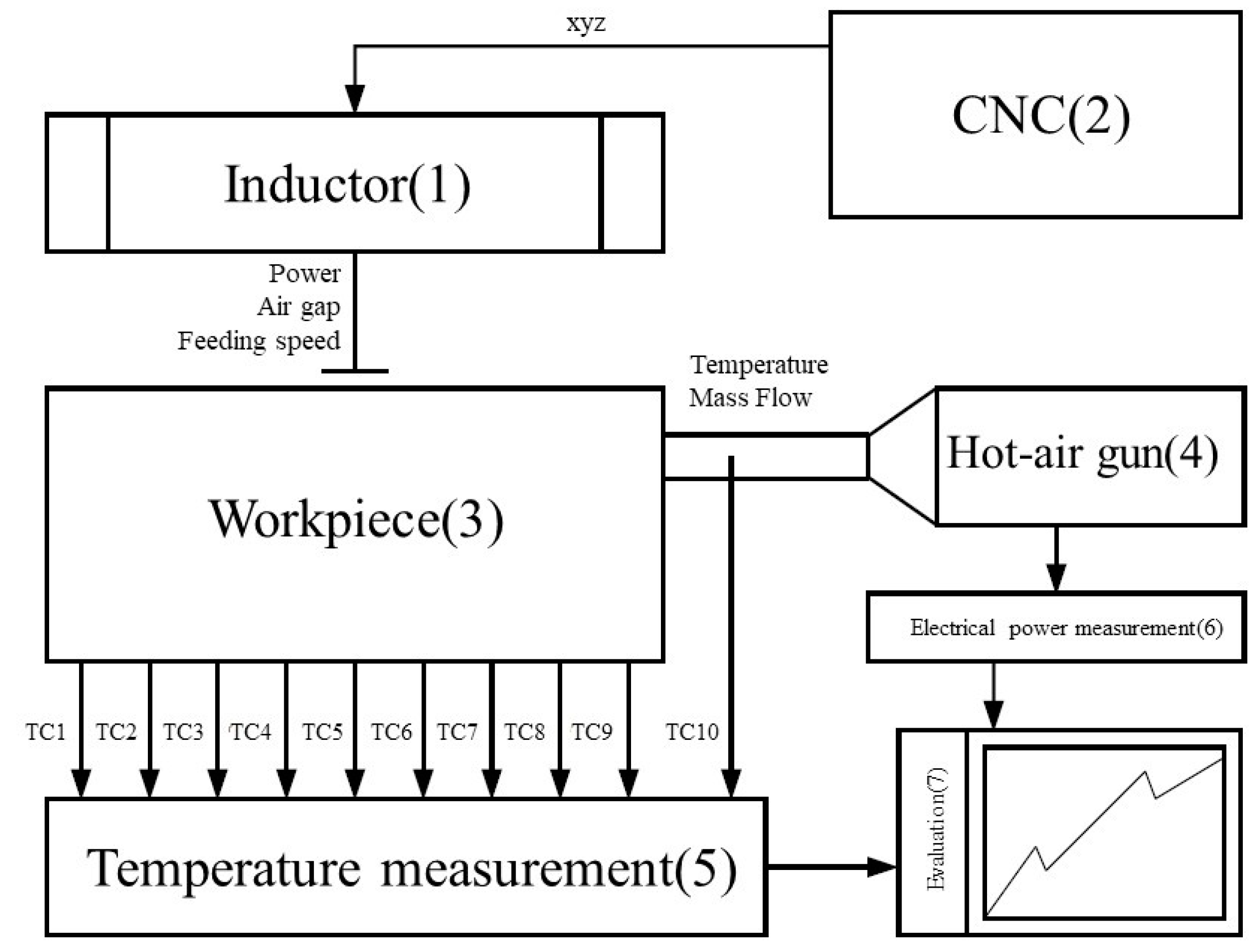
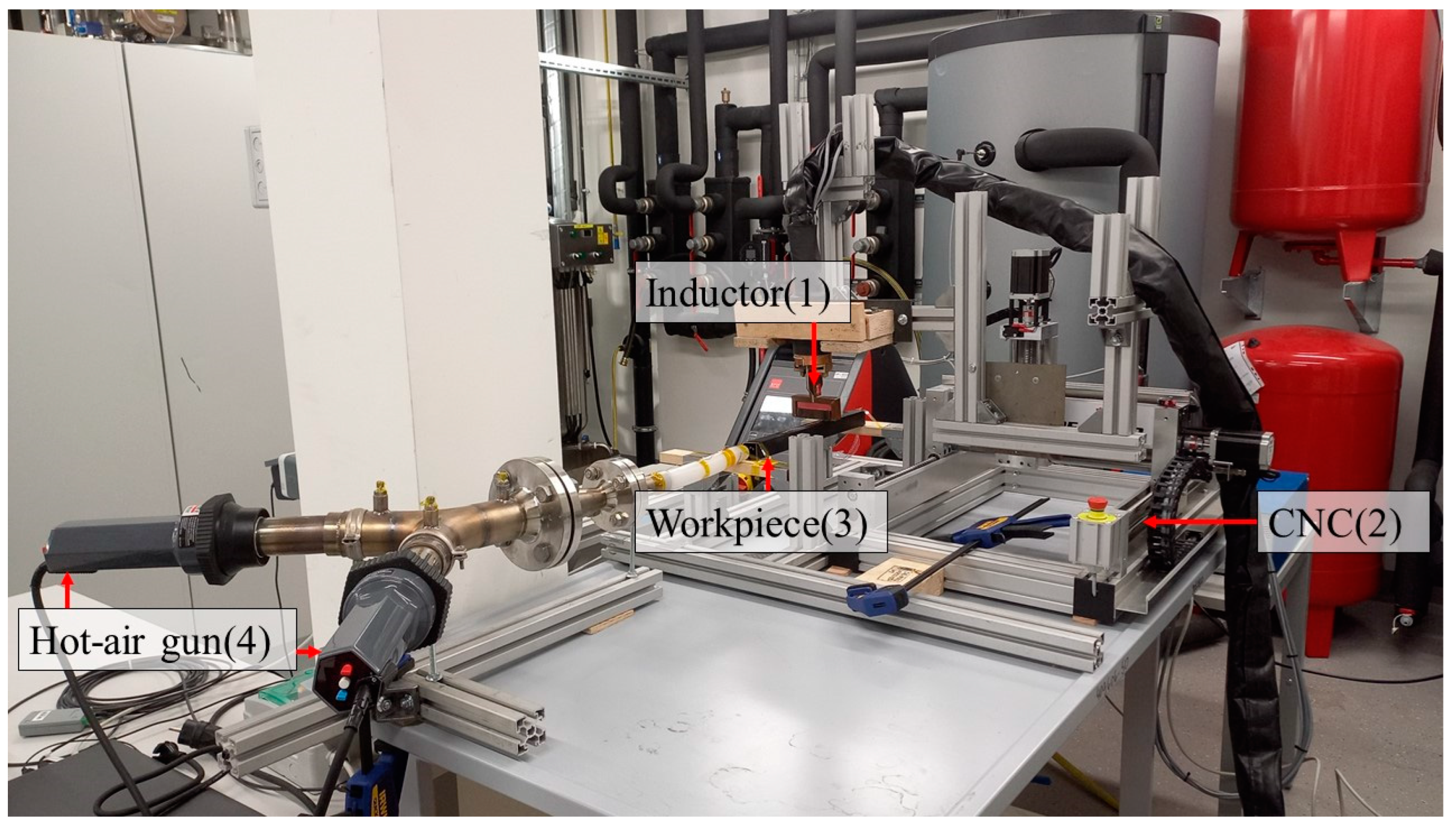
2.1.1. Structure of the Test Rig
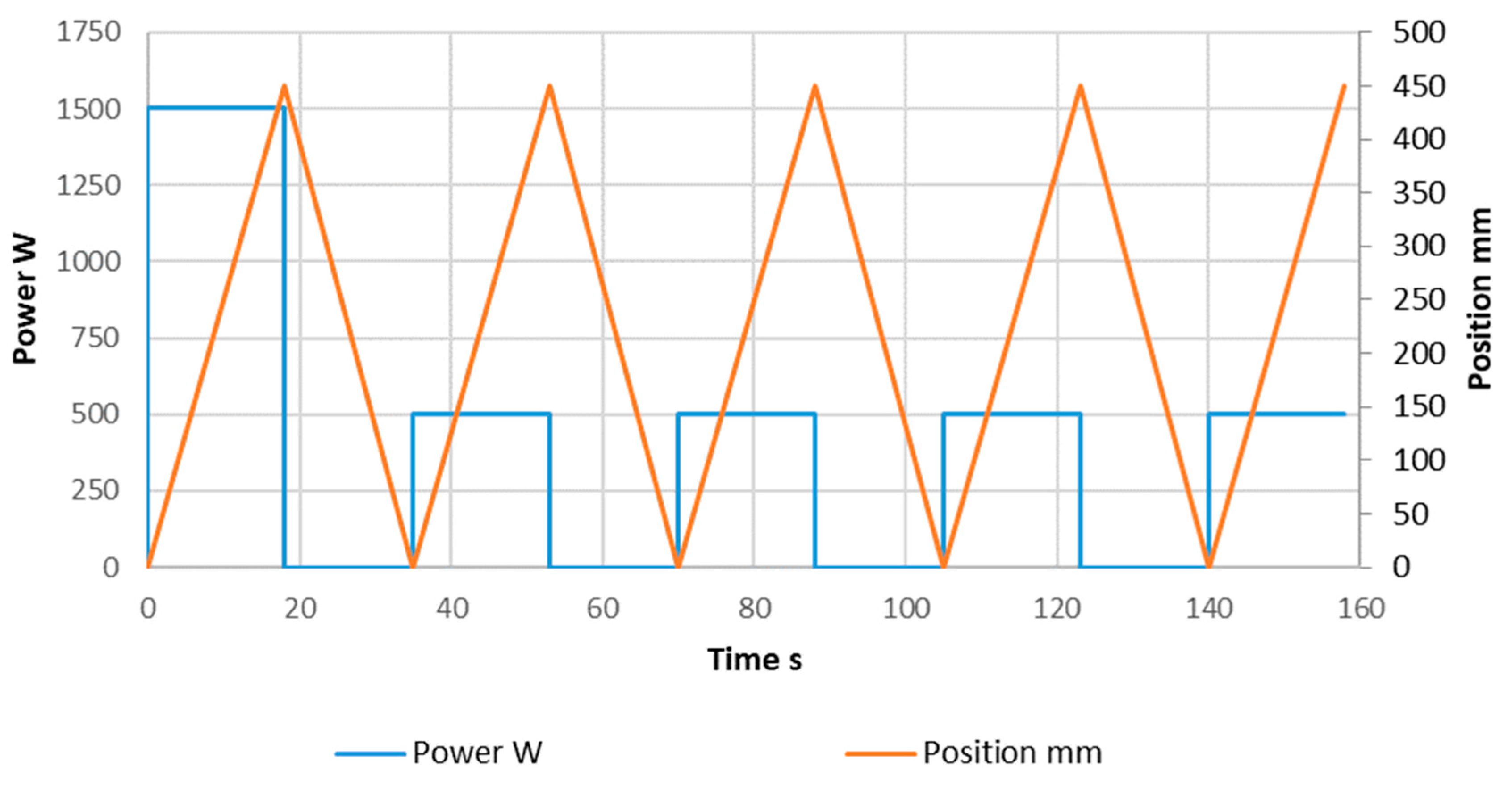
2.1.2. Execution of the Experiments
- Pure convection heating with minimal mass flow of the heat gun (~6 g/s);
- Pure convection heating with maximum mass flow of the heat gun (~12 g/s);
- Combination of induction and convection with minimal mass flow (~6 g/s);
- Combination of induction and convection with max mass flow (~12 g/s).
2.2. Simulation
2.2.1. Mathematical Description
- The current flowing through the inductor coil;
- The geometry of the coil;
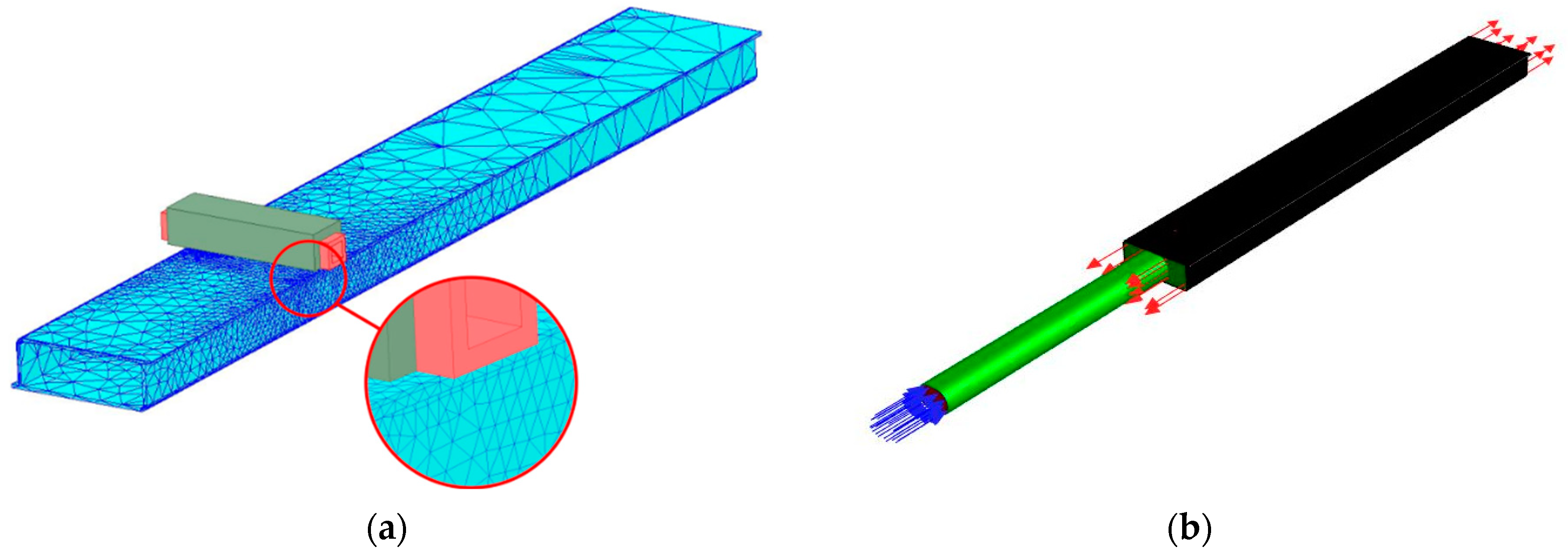
2.2.2. Execution of Simulation
3. Results
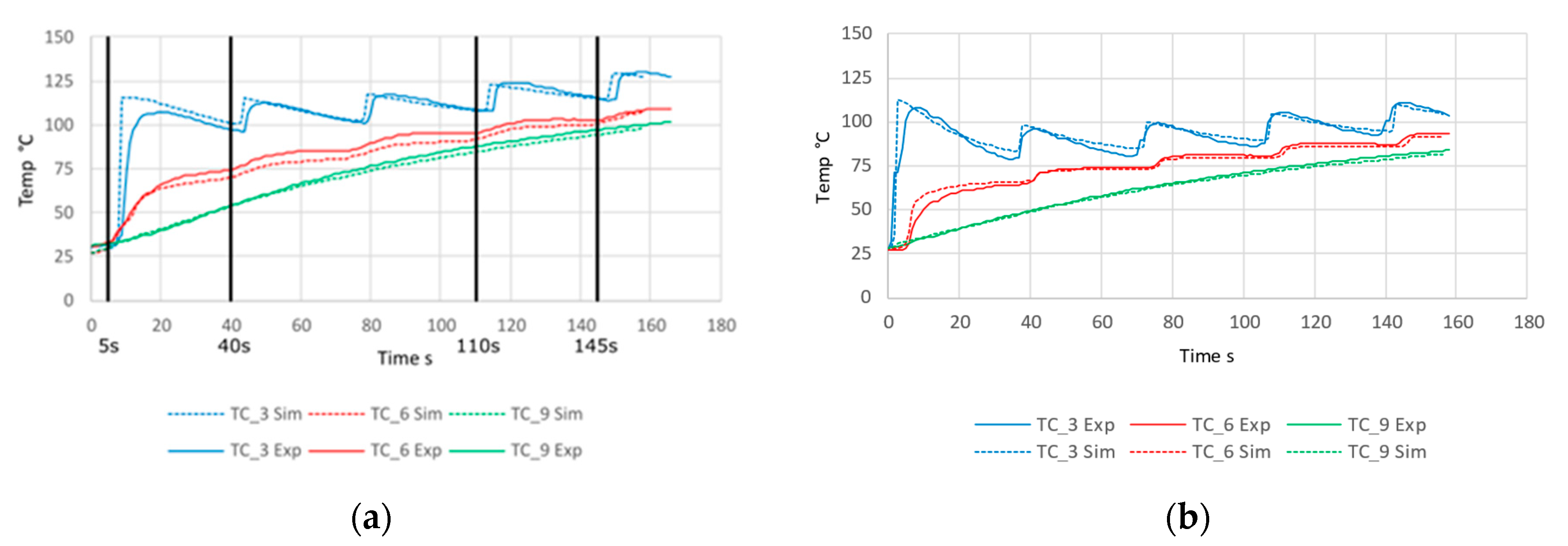
3.1. Experimental Results
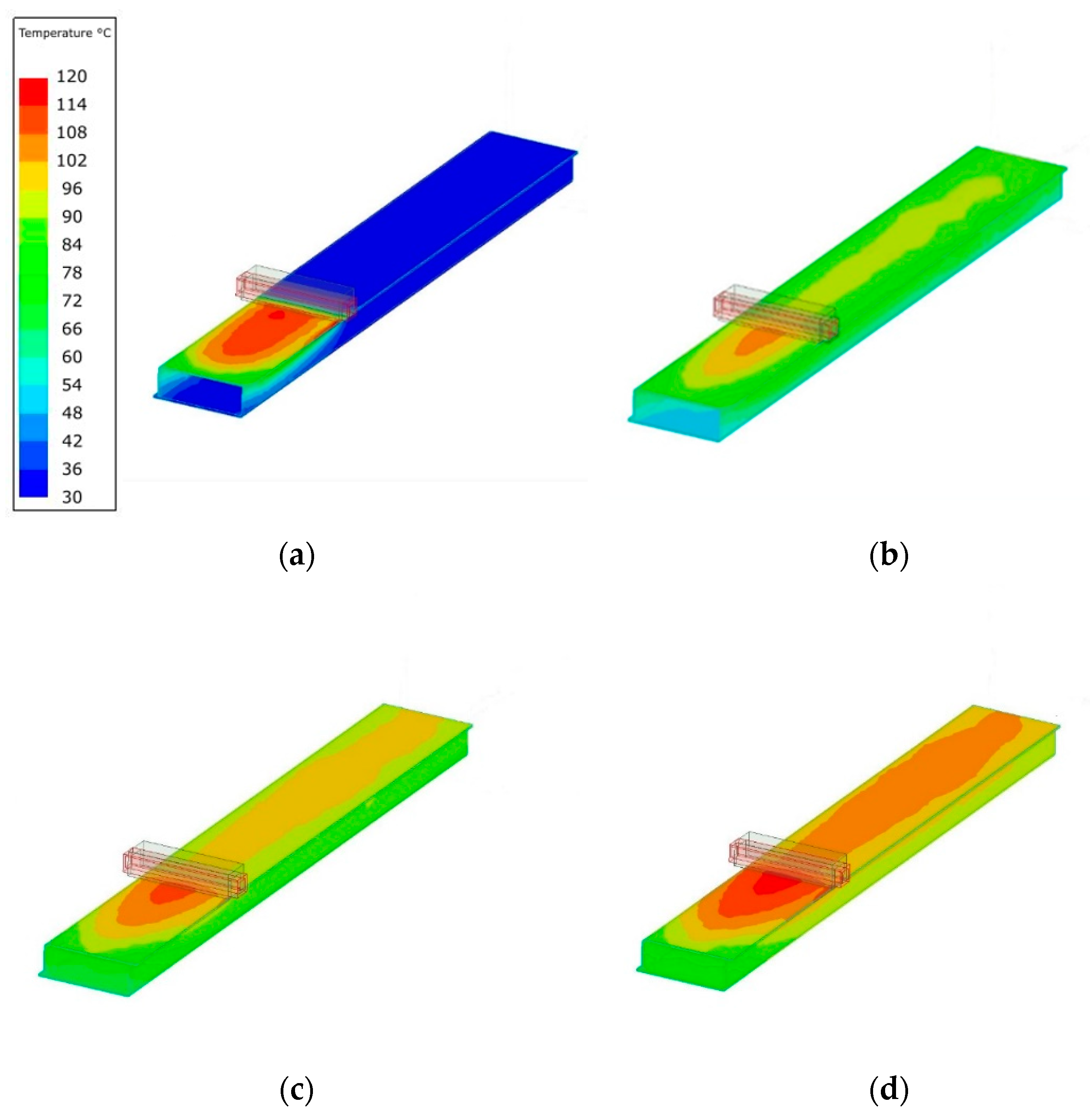
3.2. Simulation Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rudnev, V.; Loveless, D.; Raymond, L.C. Imprimerie noubelle associ-ation ouvriere. In Handbook of Induction Heating; CRC Press: Paris, France, 2017. [Google Scholar]
- Lehner, G. Elektromagnetische Feldtheorie; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Benkowsky, G.; Härten, I.; Glühen, S.; Löten, S. Grundlagen und Praktische Anleitungen für Induktionserwärmungsverfahren, Insbesondere auf dem Gebiet der Hochfrequenzerwärmung, 5th ed.; Verl. Technik: Berlin, Germany, 1990. [Google Scholar]
- Lucia, O.; Maussion, P.; Dede, E.J.; Burdio, J.M. Induction Heating Technology and Its Applications: Past Developments, Current Technology, and Future Challenges. IEEE Trans. Ind. Electron. 2014, 61, 2509–2520. [Google Scholar] [CrossRef] [Green Version]
- Davies, E.J. Conduction and Induction Heating; IET: Hertfordshire, UK, 1990. [Google Scholar]
- Rudnev, V. Metals HandBook VOL 4—Heat Treating; Amazon Publishing: Seattle, WA, USA, 1991. [Google Scholar]
- Rudnev, V. Systematic Analysis of Induction Coil Failures: Part 4: Coil Copper Electromagnetic Edge Effect; 2006; pp. 27–28. Available online: https://www.inductoheat.com/wp-content/uploads/sites/11/2019/08/133-1.pdf (accessed on 9 January 2023).
- Plumed, E.; Lope, I.; Acero, J. Induction Heating Adaptation of a Different-Sized Load With Matching Secondary Inductor to Achieve Uniform Heating and Enhance Vertical Displacement. IEEE Trans. Power Electron. 2021, 36, 6929–6942. [Google Scholar] [CrossRef]
- Huang, M.-S.; Huang, Y.-L. Effect of multi-layered induction coils on efficiency and uniformity of surface heating. Int. J. Heat Mass Transf. 2010, 53, 2414–2423. [Google Scholar] [CrossRef]
- Shih, S.-Y.; Nian, S.-C.; Huang, M.-S. Comparison between single- and multiple-zone induction heating of largely curved mold surfaces. Int. Commun. Heat Mass Transf. 2016, 75, 24–35. [Google Scholar] [CrossRef]
- Xiao, Y.; Han, Y.; Zhao, D.-F.; Wang, L.-M.; Xu, D. Temperature gradient control of frequency conversion heating for a thick-walled pipe based on energy transfer. Int. J. Heat Mass Transf. 2023, 201, 123589. [Google Scholar] [CrossRef]
- Bui, H.-T.; Hwang, S.-J. Modeling a working coil coupled with magnetic flux concentrators for barrel induction heating in an injection molding machine. Int. J. Heat Mass Transf. 2015, 86, 16–30. [Google Scholar] [CrossRef]
- Gao, K.; Qin, X.; Wang, Z.; Zhu, S.; Gan, Z. Effect of magnetizer geometry on the spot induction heating process. J. Mater. Process. Technol. 2016, 231, 125–136. [Google Scholar] [CrossRef]
- Sun, J.; Li, S.; Qiu, C.; Peng, Y. Numerical and experimental investigation of induction heating process of heavy cylinder. Appl. Therm. Eng. 2018, 134, 341–352. [Google Scholar] [CrossRef]
- Thosdeekoraphat, T.; Tanthai, K.; Lhathum, K.; Kotchapradit, S.; Santalunai, S.; Thongsopa, C. The Design of a Large-Scale Induction Heating Power Source for Organic Waste Digesters to Produce Fertilizer. Energies 2023, 16, 2123. [Google Scholar] [CrossRef]
- Fu, X.; Wang, B.; Tang, X.; Ji, H.; Zhu, X. Study on induction heating of workpiece before gear rolling process with different coil structures. Appl. Therm. Eng. 2017, 114, 1–9. [Google Scholar] [CrossRef]
- Tobias, V. (PMT/T1), Induktive Erwärmung von Formplatinen für die Warmumformung: Dissertation an der Fakultät für Maschinenbau der Technischen Universität Chemnitz; Institut für Werkzeugmaschinen und Produktionsprozesse: Chemnitz, Germany, 2016. [Google Scholar]
- Bao, L.; Liu, W.; Wang, B.; Li, H.; You, X.; Zhou, Q.; Liu, M.; Gao, S. Experimental investigation on partition controllable induction heating-hot stamping process of high-strength boron alloyed steel plates with designable temperature patterns. J. Mater. Res. Technol. 2020, 9, 13963–13976. [Google Scholar] [CrossRef]
- Wang, J.; Yi, B.; Zhang, C.; Zhou, H.; Shu, Y. Experiments of double curvature plate bending with induction heating and processing parameters investigation by computational analysis. Ocean. Eng. 2019, 192, 106596. [Google Scholar] [CrossRef]
- Zhu, B.; Meng, L.; Zeng, X.; Hu, Q.; Liu, X.; Xu, G.; Wang, D. Laser cladding high-performance maraging-steel coatings on bainitic cross wing rails assisted with in-situ induction heating: Microstructure and performance. J. Mater. Process. Technol. 2023, 313, 117886. [Google Scholar] [CrossRef]
- Li, L.; Mi, G.; Wang, C. A comparison between induction pre-heating and induction post-heating of laser-induction hybrid welding on S690QL steel. J. Manuf. Process. 2019, 43, 276–291. [Google Scholar] [CrossRef]
- Fan, W.; Tan, H.; Zhang, F.; Feng, Z.; Wang, Y.; Hu, Y.; Lin, X.; Huang, W. Effect of synchronous induction heating on residual stress for laser-based directed energy deposition of thin-walled structures. Mater. Today Commun. 2023, 35, 105702. [Google Scholar] [CrossRef]
- Kawakami, H.; Llave, Y.; Fukuoka, M.; Sakai, N. CFD analysis of the convection flow in the pan during induction heating and gas range heating. J. Food Eng. 2013, 116, 726–736. [Google Scholar] [CrossRef]
- Elsaady, W.; Moughton, C.; Nasser, A.; Lacovides, H. Coupled numerical modelling and experimental analysis of domestic induction heating systems. Appl. Therm. Eng. 2023, 227, 120170. [Google Scholar] [CrossRef]
- Park, S.M.; Jang, E.; Joo, D.; Lee, B.K. Power Curve-Fitting Control Method with Temperature Compensation and Fast-Response for All-Metal Domestic Induction Heating Systems. Energies 2019, 12, 2915. [Google Scholar] [CrossRef] [Green Version]
- Mouallem, J.; Hickey, J.-P. Induction heating of dispersed metallic particles in a turbulent flow. Int. J. Multiph. Flow 2020, 132, 103414. [Google Scholar] [CrossRef]
- Cao, L.-X.; Yin, T.; Jin, M.-X.; He, Z.-Z. Flexible circulated-cooling liquid metal coil for induction heating. Appl. Therm. Eng. 2019, 162, 114260. [Google Scholar] [CrossRef]
- Gerhardter, H.; Prieler, R.; Schluckner, C.; Knoll, M.; Hochenauer, C.; Mühlböck, M.; Tomazic, P.; Schroettner, H. Modelling convective heat transfer to non-spherical particles. Powder Technol. 2019, 343, 245–254. [Google Scholar] [CrossRef]
- Haimbaugh, R.E. Practical Induction Heat Treating; ASM International: Materials Park, OH, USA, 2015. [Google Scholar]
- Barglik, J.; Smagór, A.; Smalcerz, A.; Desisa, D.G. Induction Heating of Gear Wheels in Consecutive Contour Hardening Process. Energies 2021, 14, 3885. [Google Scholar] [CrossRef]
- Ansys Inc. Ansys Maxwell, Release 2020 R2. Available online: https://www.ansys.com/products/electronics/ansys-maxwell (accessed on 1 August 2023).
- Ansys Inc. Ansys Fluent, Release 2020 R2. Available online: https://www.ansys.com/products/fluids/ansys-fluent (accessed on 1 August 2023).
- Menter, F.R.; Kuntz, M.; Langstry, R. Ten Years of Industrial Experience with the SST Turbulance Model. Turbul. Heat Mass Transf. 2003, 4, 625–632. [Google Scholar]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. In Proceedings of the 23rd Fluid Dynamics, Plasmadynamics, and Lasers Conference, Orlando, FL, USA, 6 July 1993; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1993. [Google Scholar]
- Feurhuber, M.; Magno, M.; Miranda, M.; Hochenauer, C. CFD investigations of steam penetration, air-removal and condensation inside hollow loads and cavities. Appl. Therm. Eng. 2019, 147, 1070–1082. [Google Scholar] [CrossRef]



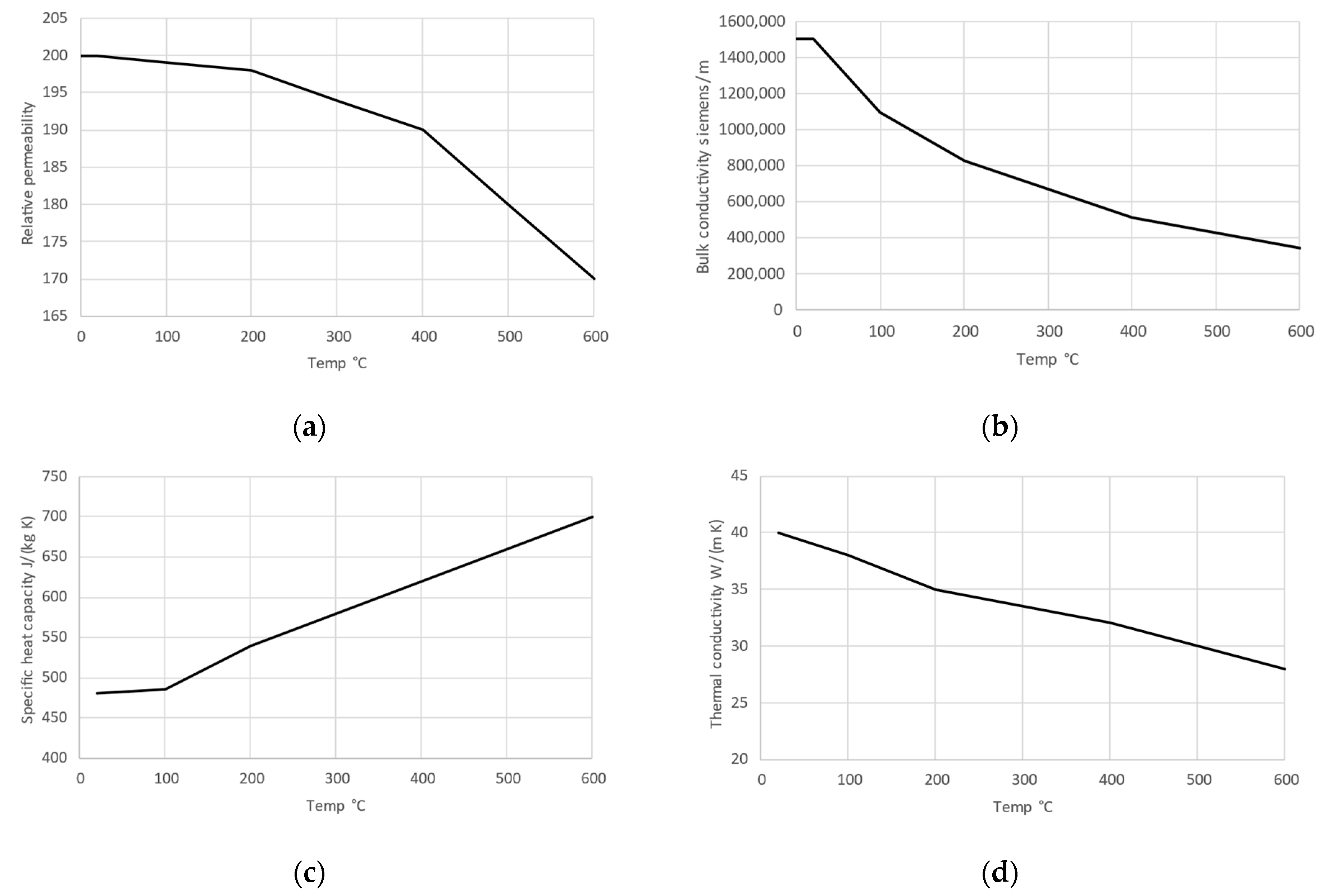




| Profile | Set Power | Current in Coil | Frequency |
|---|---|---|---|
| Rectangular profile | 1500 W | 678.4 A | 15 kHz |
| 500 W | 329.6 A | 15 kHz | |
| Angled profile | 1500 W | 646.4 A | 15 kHz |
| 500 W | 329.6 A | 15 kHz |
| Steel | Copper | Air | ||
|---|---|---|---|---|
| Relative permittivity | 1 | 1 | - | - |
| Relative permeability | Temp. dep. | 0.999991 | - | - |
| Bulk conductivity | Temp. dep. | 58,000,000 | - | S/m |
| Mass density | 7500 | 8933 | 1.225 | kg/m3 |
| Specific heat capacity | Temp. dep. | - | 1006.43 | J/(kg K) |
| Thermal conductivity | Temp. dep. | - | 0.0242 | W/(m K) |
| Viscosity | - | - | 1.79 × 10−5 | kg/(m s) |
| Experiment | Profile | Convective Power | Time Conv. Heating | Mass Flow Air | Passes Ind. after Peak | Energy Induction | Energy Convection | Total Electric Energy | Ave. End Temp |
|---|---|---|---|---|---|---|---|---|---|
| W | s | 10−3 | - | J | J | J | J | ||
| Flow min. | Rectangle | 1672.8 | 252 | 6.0 | 0 | 0 | 421.5 | 421.5 | 74.5 |
| Flow max. | Rectangle | 3073.4 | 239 | 11.1 | 0 | 0 | 734.5 | 734.5 | 96.0 |
| Comb. min. | Rectangle | 1821.7 | 153 | 6.6 | 4 | 62.2 | 278.7 | 340.9 | 97.0 |
| Comb. max. | Rectangle | 3288.2 | 185 | 11.8 | 5 | 71.1 | 608.3 | 679.4 | 121.7 |
| Flow min. | Angled | 1651.7 | 308 | 5.9 | 0 | 0 | 508.7 | 508.7 | 82.8 |
| Flow max. | Angled | 3535.3 | 191 | 11.1 | 0 | 0 | 675.5 | 675.5 | 101.0 |
| Comb. min. | Angled | 1753.5 | 210 | 6.3 | 6 | 62.2 | 368.2 | 430.4 | 103.1 |
| Comb. max. | Angled | 1430.6 | 219 | 12.4 | 6 | 80 | 751.3 | 831.3 | 117.0 |
| TC2 | TC5 | TC8 | |
|---|---|---|---|
| Fluent finer | 0.3086 K | 0.3576 K | 0.1147 K |
| Maxw. finer | 0.3765 K | 0.4864 K | 0.0966 K |
| Both finer | 0.3180 K | 0.2080 K | 0.1823 K |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gergely, R.; Hochenauer, C. Heating Strategies for Efficient Combined Inductive and Convective Heating of Profiles. Energies 2023, 16, 5895. https://doi.org/10.3390/en16165895
Gergely R, Hochenauer C. Heating Strategies for Efficient Combined Inductive and Convective Heating of Profiles. Energies. 2023; 16(16):5895. https://doi.org/10.3390/en16165895
Chicago/Turabian StyleGergely, Raphael, and Christoph Hochenauer. 2023. "Heating Strategies for Efficient Combined Inductive and Convective Heating of Profiles" Energies 16, no. 16: 5895. https://doi.org/10.3390/en16165895
APA StyleGergely, R., & Hochenauer, C. (2023). Heating Strategies for Efficient Combined Inductive and Convective Heating of Profiles. Energies, 16(16), 5895. https://doi.org/10.3390/en16165895





