Calculation of AC Losses in a 500 kJ/200 kW Multifilamentary MgB2 SMES Coil
Abstract
1. Introduction
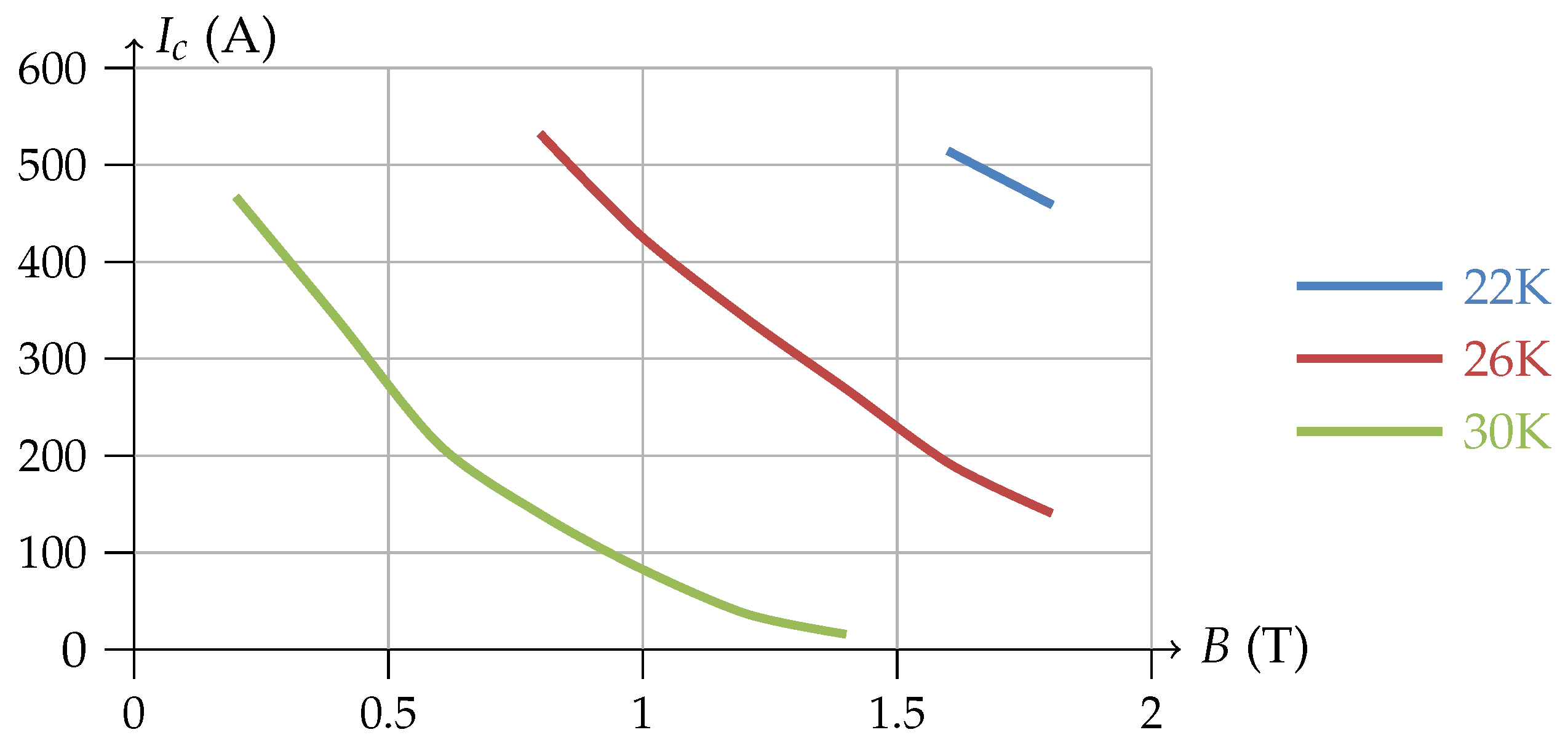
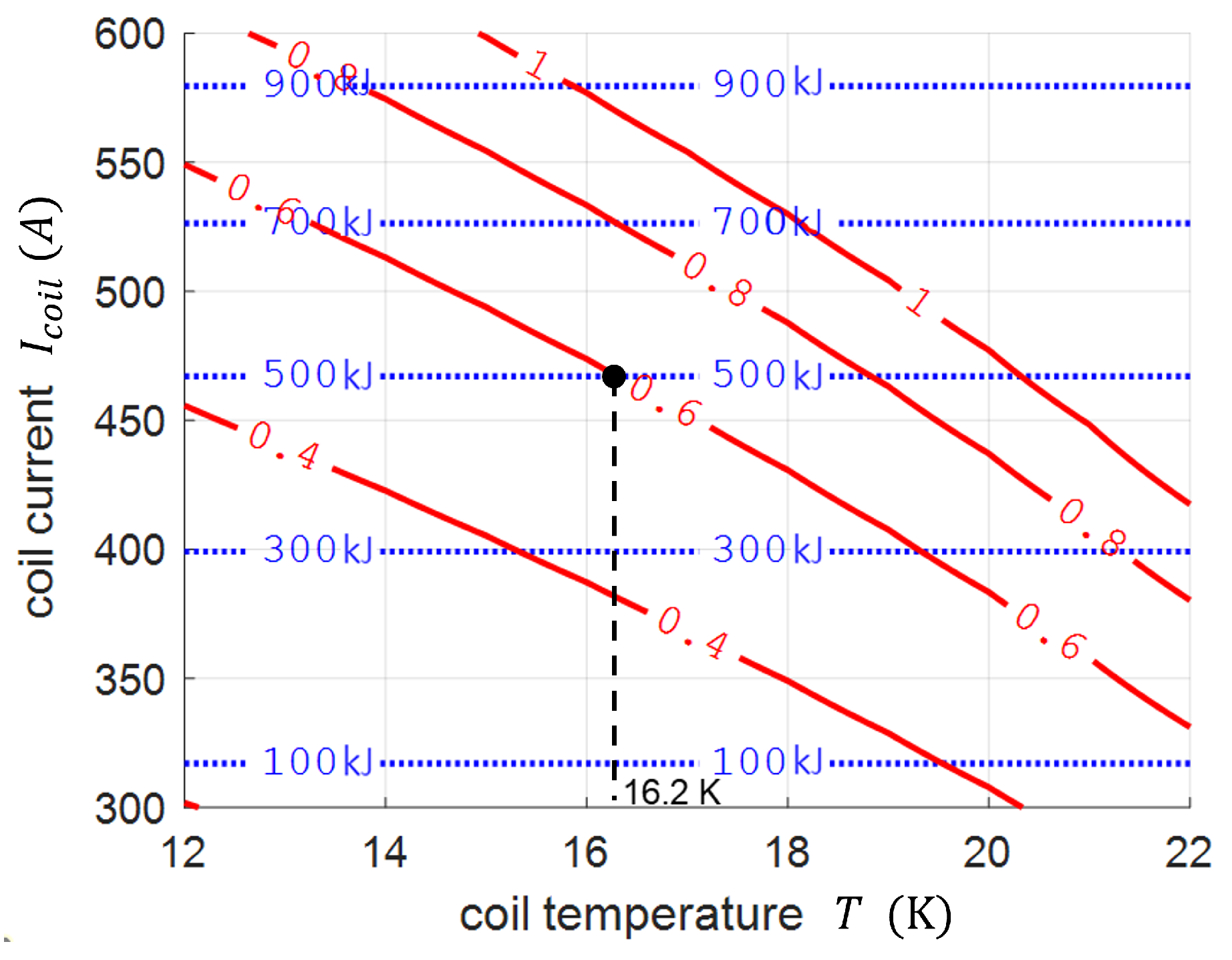
2. Reference MgB Conductor Main Characteristics and Coil Operating Conditions
3. Calculation Model and Simulated Cases
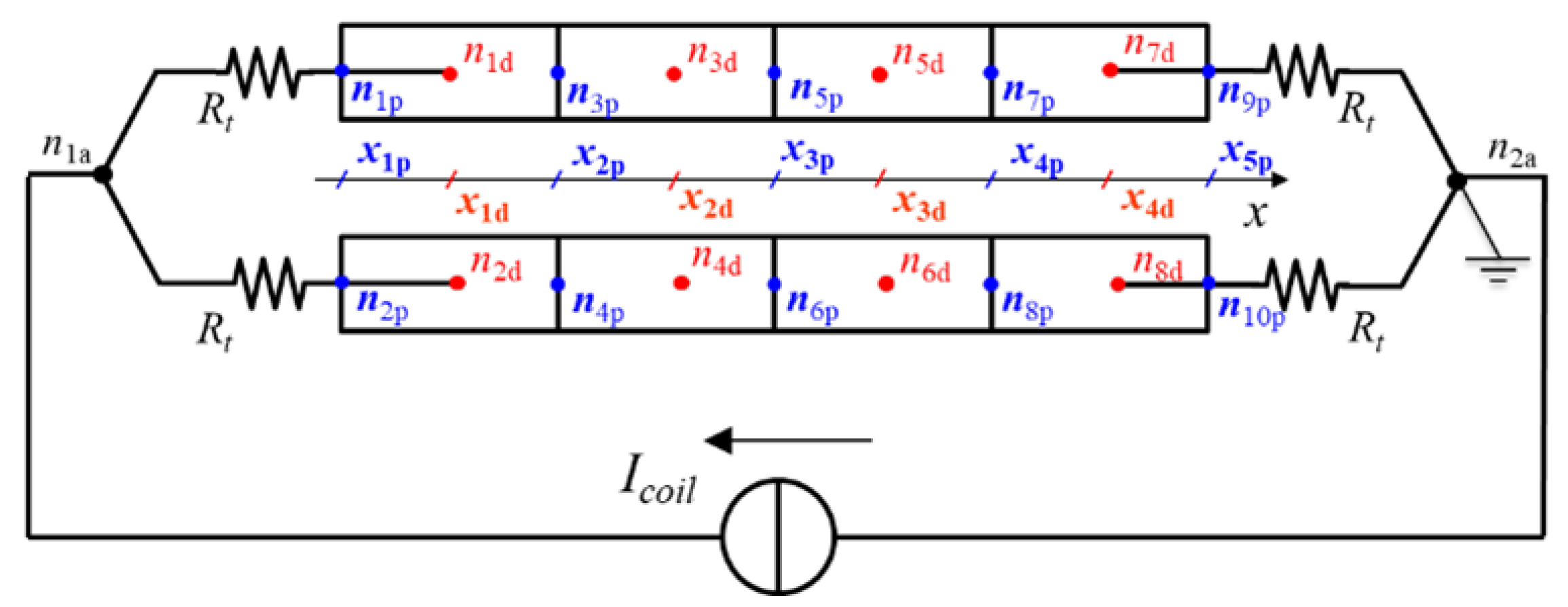
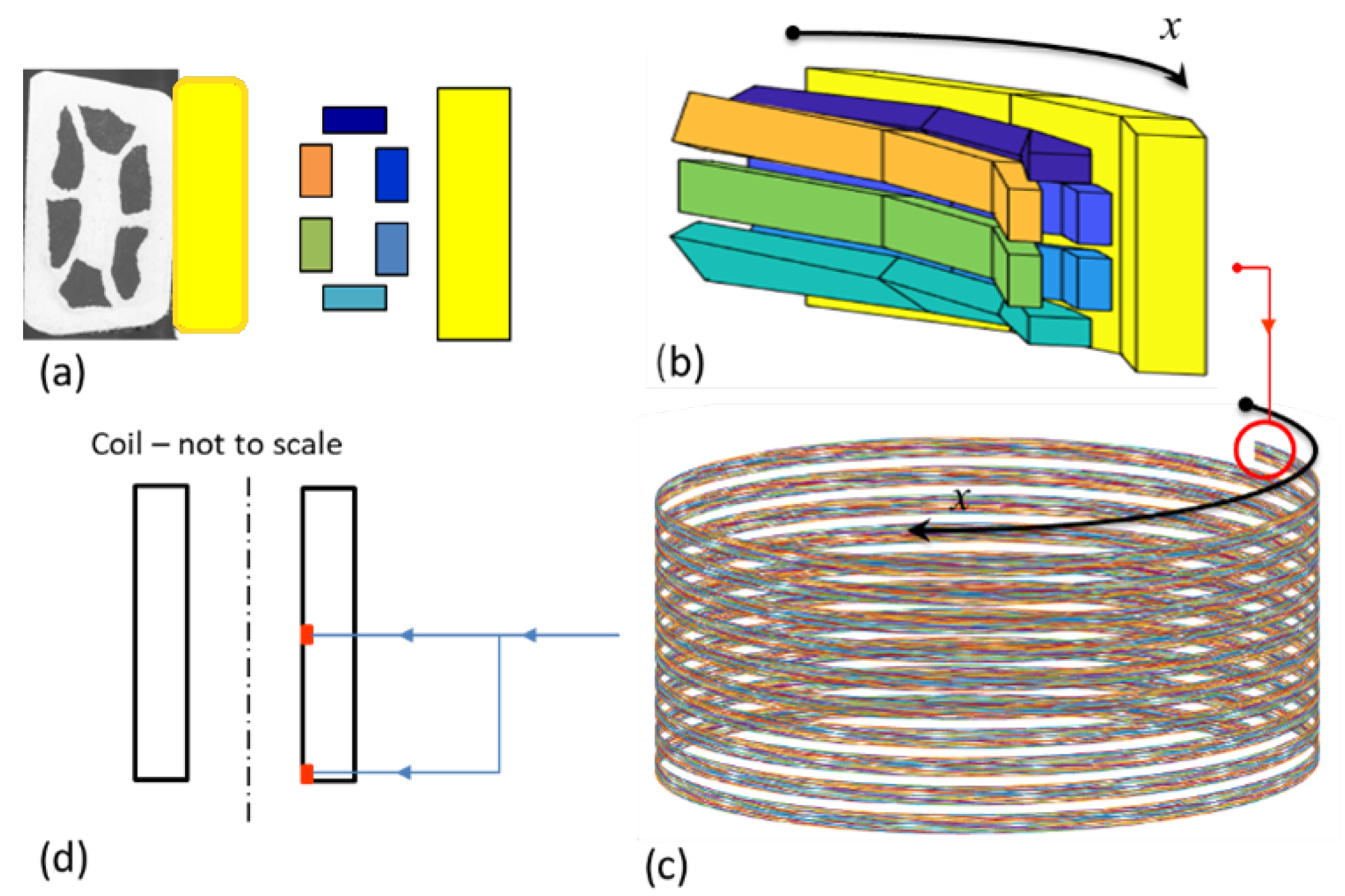
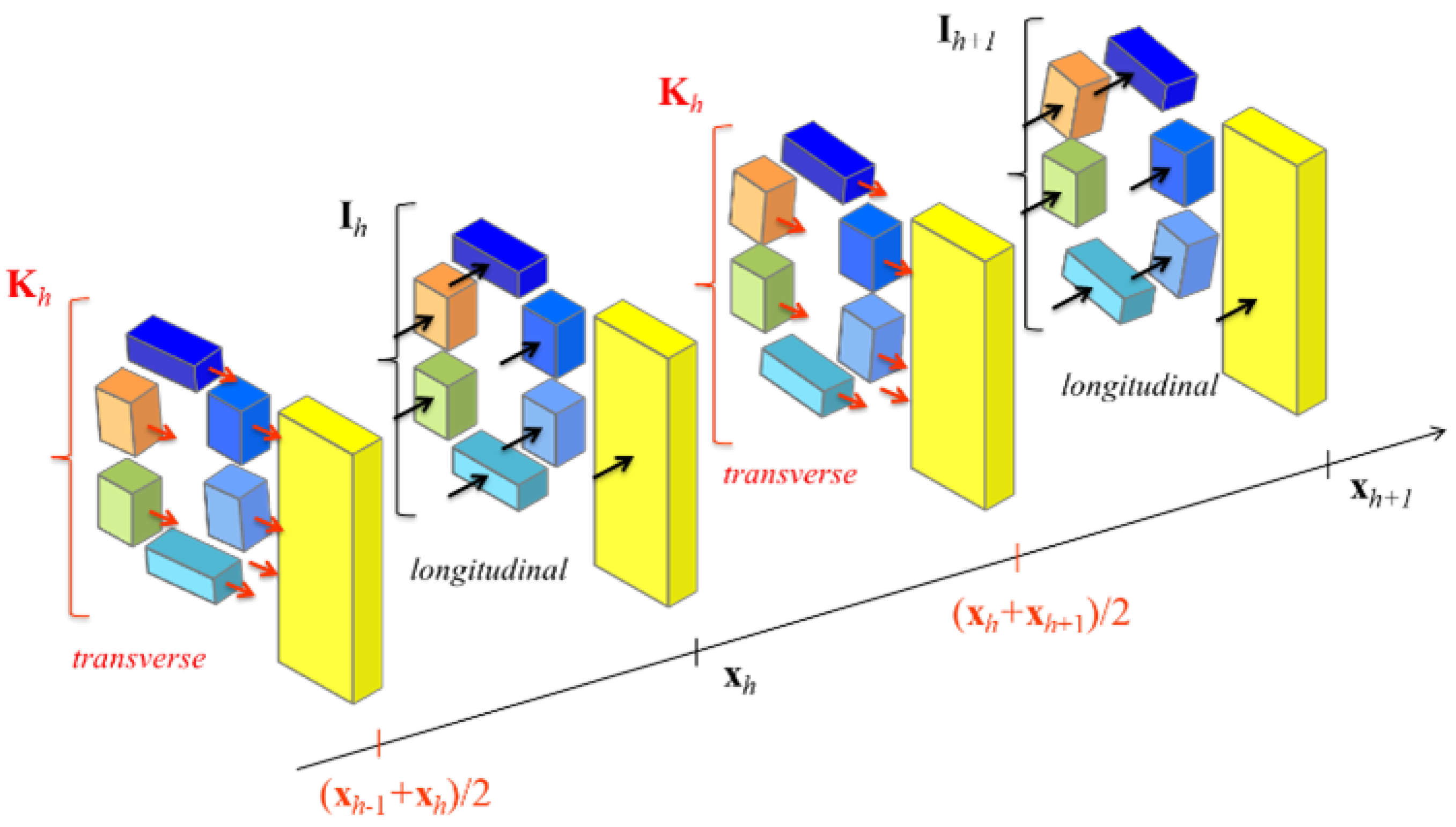
3.1. Geometrical Model and Simulated Cases
3.2. Mathematical Model
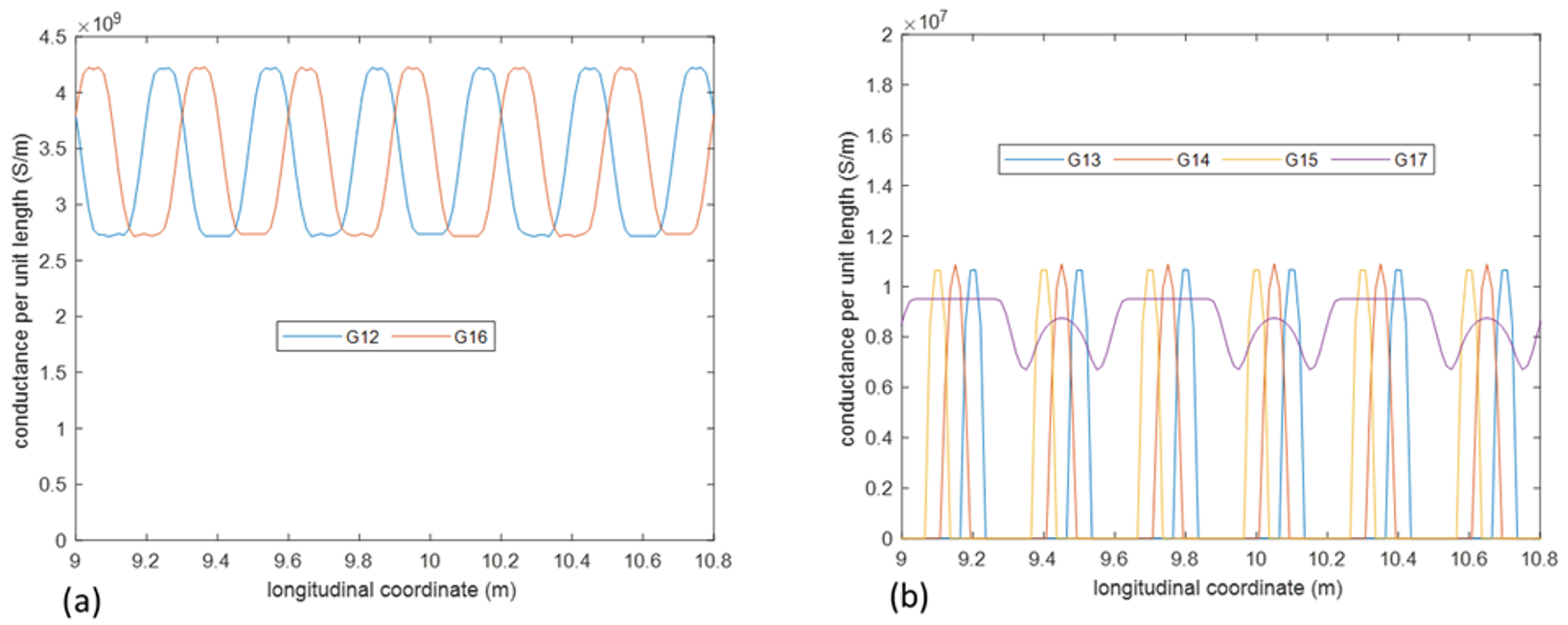
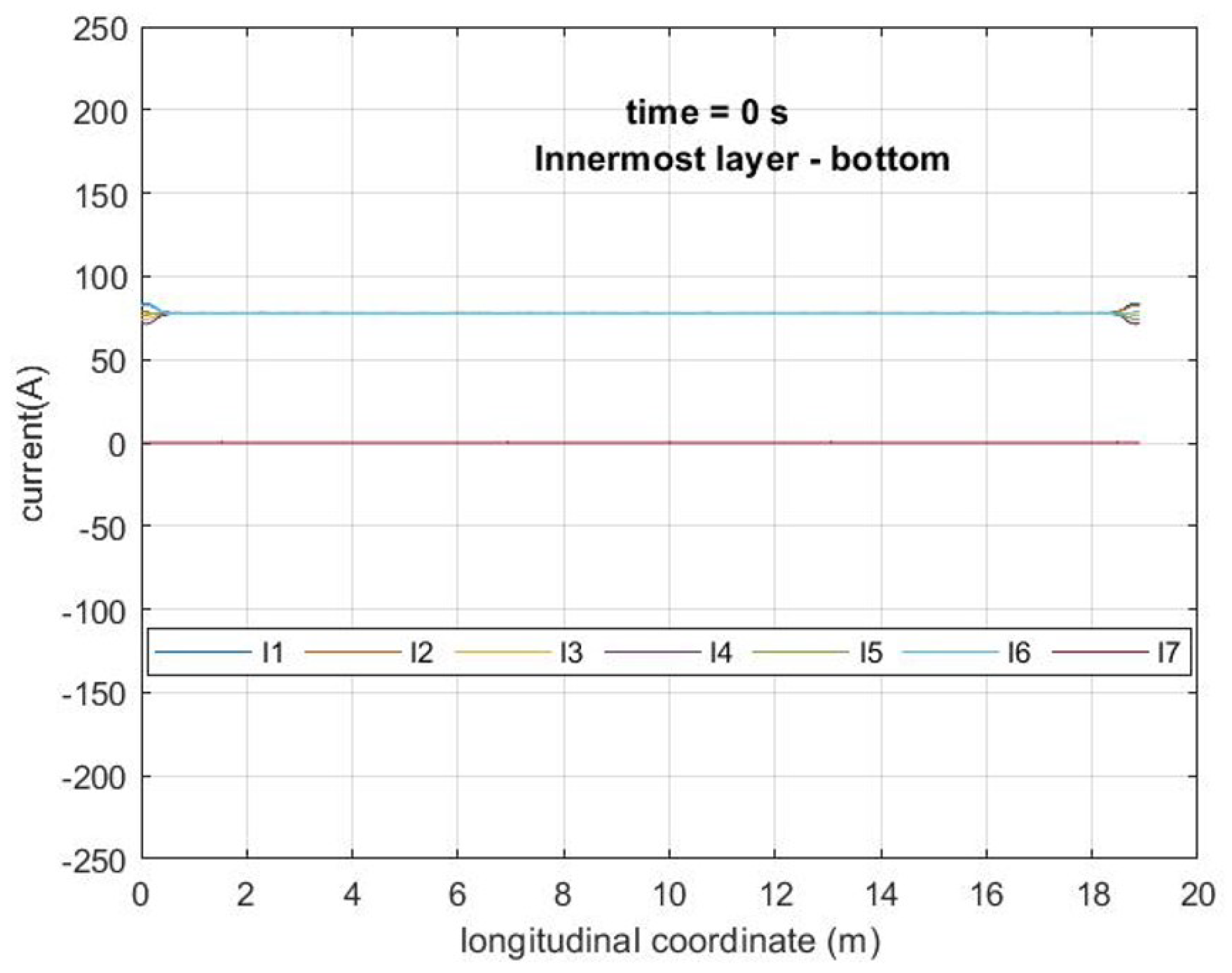
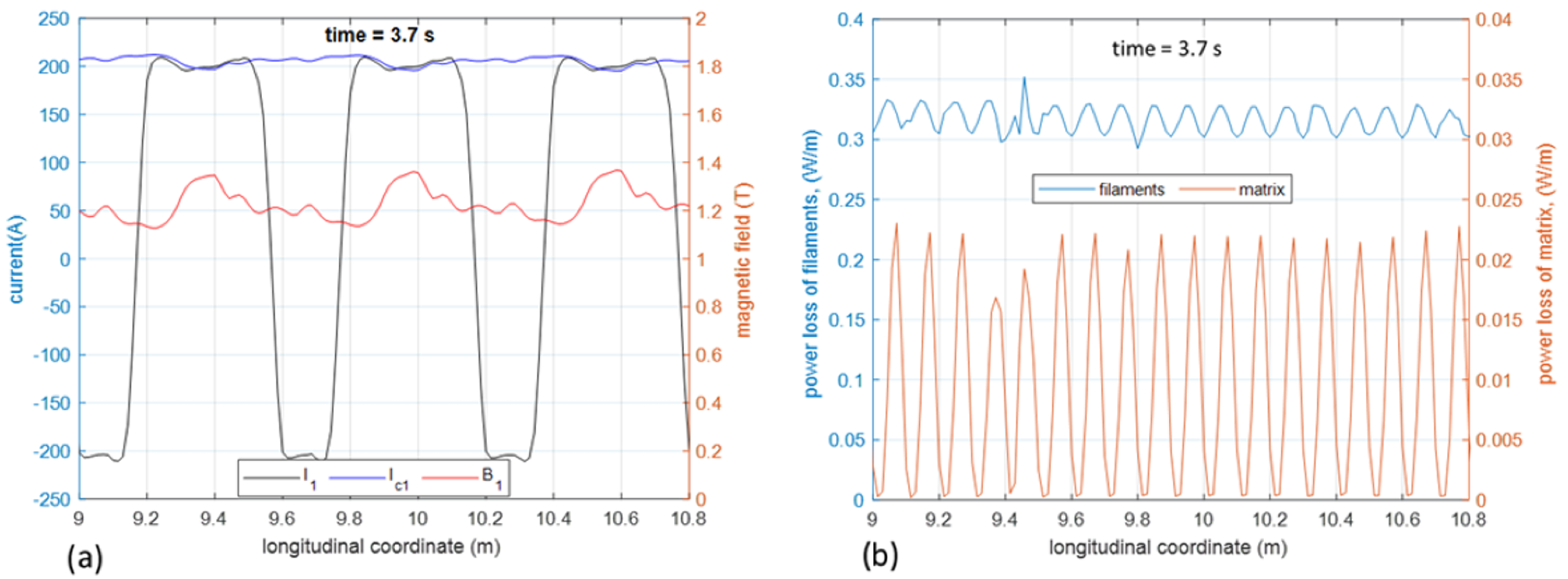
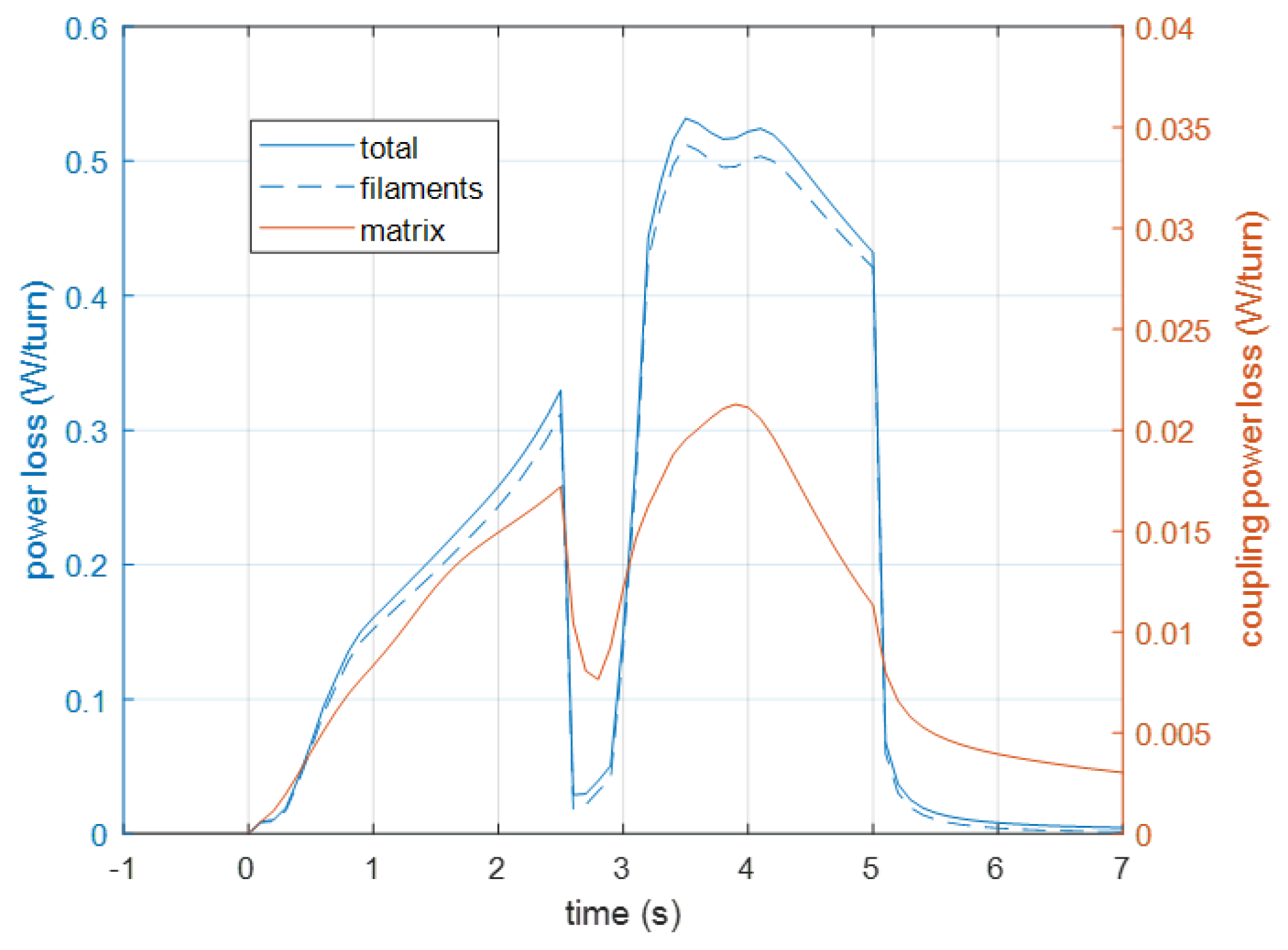
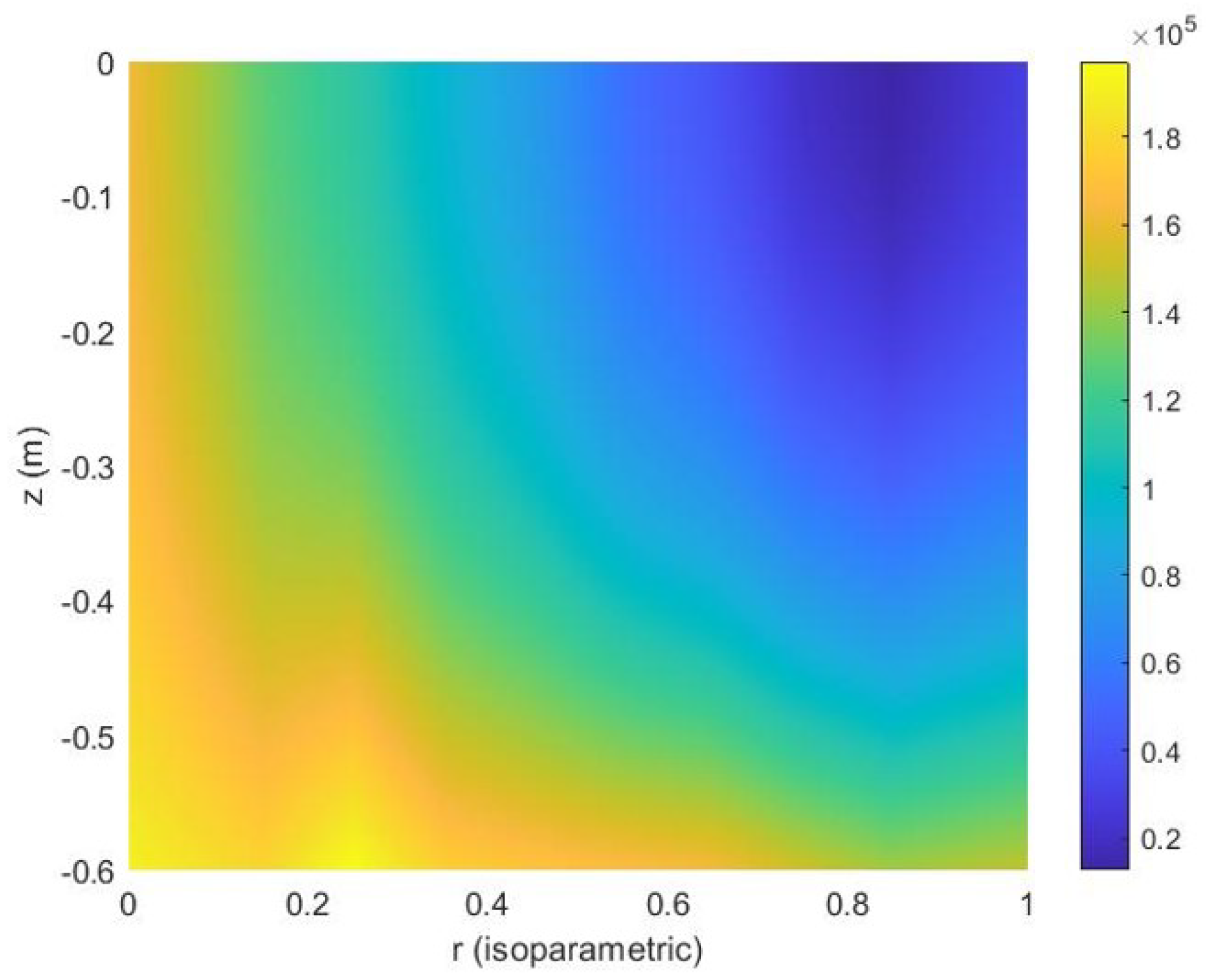
4. Numerical Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Appendix A

- Equation (A6) are applied to all the primal sections not laying on the terminals of the domain. These consist of matrix equations corresponding to scalar equations;
- Equation (A8) for all the longitudinal sections. These consist of scalar equations;
- Equation (A16) applied to all the dual sections. These consist of matrix equation corresponding to scalar equations;
- Terminal conditions in Equation (A17). These consist of 2 matrix equations corresponding to scalar equations.
- longitudinal currents at the primal sections;
- electric scalar potentials of the nodes at the dual sections;
- One electric scalar potentials of node .
References
- Boenig, H.; Hauer, J. Commissioning tests of the Bonneville Power Administration 30 MJ superconducting magnetic energy storage unit. IEEE Trans. Power Appar. Syst. 1985, 104, 302–312. [Google Scholar] [CrossRef]
- Nagaya, S.; Hirano, N.; Katagiri, T.; Tamada, T.; Shikimachi, K.; Iwatani, Y.; Saito, F.; Ishii, Y. The state of the art of the development of SMES for bridging instantaneous voltage dips in Japan. Cryogenics 2012, 52, 708–712. [Google Scholar] [CrossRef]
- Morandi, A.; Breschi, M.; Fabbri, M.; Negrini, F.; Penco, R.; Perrella, M.; Ribani, P.L.; Tassisto, M.; Trevisani, L. Design, Manufacturing and Preliminary Tests of a Conduction Cooled 200 kJ Nb-Ti μSMES. IEEE Trans. Appl. Supercond. 2008, 18, 697–700. [Google Scholar] [CrossRef]
- Ottonello, L.; Canepa, G.; Albertelli, P.; Picco, E.; Florio, A.; Masciarelli, G.; Rossi, S.; Martini, L.; Pincella, C.; Mariscotti, A.; et al. The largest italian SMES. IEEE Trans. Appl. Supercond. 2006, 16, 602–607. [Google Scholar] [CrossRef]
- Tixador, P.; Bellin, B.; Deleglise, M.; Vallier, J.C.; Bruzek, C.; Allais, A.; Saugrain, J. Design and first tests of a 800 kJ HTS SMES. IEEE Trans. Appl. Supercond. 2007, 17, 1967–1972. [Google Scholar] [CrossRef]
- Lee, S.; Yi, K.P.; Park, S.H.; Lee, J.K.; Kim, W.S.; Park, C.; Bae, J.H.; Seong, K.C.; Park, I.; Choi, K.; et al. Design of HTS toroidal magnets for a 5 MJ SMES. IEEE Trans. Appl. Supercond. 2011, 22, 5700904. [Google Scholar]
- Shikimachi, K.; Hirano, N.; Nagaya, S.; Kawashima, H.; Higashikawa, K.; Nakamura, T. System coordination of 2 GJ class YBCO SMES for power system control. IEEE Trans. Appl. Supercond. 2009, 19, 2012–2018. [Google Scholar] [CrossRef]
- Song, M.; Shi, J.; Liu, Y.; Xu, Y.; Hu, N.; Tang, Y.; Ren, L.; Li, J. 100 kJ/50 kW HTS SMES for micro-grid. IEEE Trans. Appl. Supercond. 2014, 25, 5700506. [Google Scholar] [CrossRef]
- Xu, Y.; Tang, Y.; Ren, L.; Dai, Q.; Xu, C.; Wang, Z.; Shi, J.; Li, J.; Liang, S.; Yan, S. Numerical simulation and experimental validation of a cooling process in a 150-kJ SMES magnet. IEEE Trans. Appl. Supercond. 2016, 26, 5700507. [Google Scholar] [CrossRef]
- Morandi, A.; Fabbri, M.; Gholizad, B.; Grilli, F.; Sirois, F.; Zermeño, V.M. Design and comparison of a 1-MW/5-s HTS SMES with toroidal and solenoidal geometry. IEEE Trans. Appl. Supercond. 2016, 26, 5700606. [Google Scholar] [CrossRef]
- Morandi, A.; Gholizad, B.; Fabbri, M. Design and performance of a 1 MW-5 s high temperature superconductor magnetic energy storage system. Supercond. Sci. Technol. 2015, 29, 015014. [Google Scholar] [CrossRef]
- Saichi, Y.; Miyagi, D.; Tsuda, M. A suitable design method of SMES coil for reducing superconducting wire usage considering maximum magnetic flux density. IEEE Trans. Appl. Supercond. 2013, 24, 5700505. [Google Scholar] [CrossRef]
- Sander, M.; Gehring, R.; Neumann, H. LIQHYSMES—A 48 GJ toroidal MgB2-SMES for buffering minute and second fluctuations. IEEE Trans. Appl. Supercond. 2012, 23, 5700505. [Google Scholar] [CrossRef]
- Shintomi, T.; Asami, T.; Suzuki, G.; Ota, N.; Takao, T.; Makida, Y.; Hamajima, T.; Tsuda, M.; Miyagi, D.; Kajiwara, M.; et al. Design Study of MgB2 SMES Coil for Effective Use of Renewable Energy. IEEE Trans. Appl. Supercond. 2012, 23, 5700304. [Google Scholar] [CrossRef]
- Leys, P.; Klaeser, M.; Ruf, C.; Schneider, T. Characterization of Commercial MgB 2 conductors for magnet application in SMES. IEEE Trans. Appl. Supercond. 2016, 26, 6200605. [Google Scholar] [CrossRef]
- Morandi, A.; Fiorillo, A.; Pullano, S.; Ribani, P.L. Development of a small cryogen-free MgB 2 test coil for SMES application. IEEE Trans. Appl. Supercond. 2017, 27, 5700404. [Google Scholar] [CrossRef]
- Tran, V.T.; Islam, M.R.; Muttaqi, K.M.; Sutanto, D. A novel application of magnesium di-boride superconducting energy storage to mitigate the power fluctuations of single-phase PV systems. IEEE Trans. Appl. Supercond. 2019, 29, 5700505. [Google Scholar] [CrossRef]
- Morandi, A.; Anemona, A.; Angeli, G.; Breschi, M.; Della Corte, A.; Ferdeghini, C.; Gandolfi, C.; Grandi, G.; Grasso, G.; Martini, L.; et al. The DRYSMES4GRID project: Development of a 500 kJ/200 kW cryogen-free cooled SMES demonstrator based on MgB2. IEEE Trans. Appl. Supercond. 2018, 28, 5700205. [Google Scholar] [CrossRef]
- Gandolfi, C.; Chiumeo, R.; Raggini, D.; Faranda, R.; Morandi, A.; Ferdeghini, C.; Tropeano, M.; Turtù, S. Study of a Universal Power SMES Compensator for LV Distribution Grid. In Proceedings of the 2018 AEIT International Annual Conference, Bari, Italy, 3–5 October 2018; pp. 1–6. [Google Scholar]
- Park, M.J.; Kwak, S.Y.; Kim, W.S.; Lee, S.W.; Lee, J.K.; Han, J.H.; Choi, K.D.; Jung, H.K.; Seong, K.C.; Hahn, S.y. AC loss and thermal stability of HTS model coils for a 600 kJ SMES. IEEE Trans. Appl. Supercond. 2007, 17, 2418–2421. [Google Scholar] [CrossRef]
- Wang, Z.; Tang, Y.; Ren, L.; Li, J.; Xu, Y.; Liao, Y.; Deng, X. AC loss analysis of a hybrid HTS magnet for SMES based on H-formulation. IEEE Trans. Appl. Supercond. 2016, 27, 4701005. [Google Scholar]
- Zhang, Z. AC Loss Database Built with Numerical Multi-scale Model and Status Prediction of a 150 kJ SMES. In Proceedings of the 2018 IEEE International Magnetics Conference (INTERMAG), Singapore, 23–27 April 2018; p. 1. [Google Scholar]
- Zhang, Z.; Ren, L.; Xu, Y.; Wang, Z.; Xia, Y.; Liang, S.; Yan, S.; Tang, Y. AC loss prediction model of a 150 kJ HTS SMES based on multi-scale model and artificial neural networks. IEEE Trans. Magn. 2018, 54, 7206005. [Google Scholar] [CrossRef]
- Chen, Y.; Chen, X.Y.; Zeng, L.; Xie, Q.; Lei, Y. AC loss and temperature simulations of HTS pancake coil during SMES operations. In Proceedings of the 2020 IEEE International Conference on Applied Superconductivity and Electromagnetic Devices (ASEMD), Tianjin, China, 16–18 October 2020; pp. 1–2. [Google Scholar]
- Ciotti, M.; Nijhuis, A.; Ribani, P.; Richard, L.S.; Zanino, R. THELMA code electromagnetic model of ITER superconducting cables and application to the ENEA stability experiment. Supercond. Sci. Technol. 2006, 19, 987. [Google Scholar] [CrossRef]
- Breschi, M.; Ribani, P.L. Electromagnetic modeling of the jacket in cable-in-conduit conductors. IEEE Trans. Appl. Supercond. 2008, 18, 18–28. [Google Scholar] [CrossRef]
- Fabbri, M.; Grandi, G.; Morandi, A.; Melaccio, U.; Ribani, P.L. Design of the magnet system. In Deliverable D01 of Funded Project DRYSEMS4GRID, MISE—Italian Ministry of Economic Development, Competitive Call “Research Project for Electric Power Grid”, 2017–2021; University of Bologna: Bologna, Italy, 2021. (In Italian) [Google Scholar]
- Bellina, F.; Bonicelli, T.; Breschi, M.; Ciotti, M.; Della Corte, A.; Formisano, A.; Ilyin, Y.; Marchese, V.; Martone, R.; Nijhuis, A.; et al. Superconductive cables current distribution analysis. Fusion Eng. Des. 2003, 66, 1159–1163. [Google Scholar] [CrossRef]
- Breschi, M.; Ribani, P.L.; Bellina, F. Electromagnetic analysis of the voltage-temperature characteristics of the ITER TF conductor samples. IEEE Trans. Appl. Supercond. 2009, 19, 1512–1515. [Google Scholar] [CrossRef]
- Manfreda, G.; Bellina, F.; Corato, V.; della Corte, A.; Ribani, P. Coupled thermal and electromagnetic analysis of the NAFASSY magnet. IEEE Trans. Appl. Supercond. 2014, 25, 4801406. [Google Scholar] [CrossRef]
- Breschi, M.; Cavallucci, L.; Ribani, P.; Calzolaio, C.; Sanfilippo, S. Analysis of losses in superconducting magnets based on the Nb3Sn Rutherford cable configuration for future gantries. Supercond. Sci. Technol. 2017, 31, 015005. [Google Scholar] [CrossRef]
- Breschi, M.; Ribani, P.L.; Scurti, F.; Nijhuis, A.; Bajas, H.; Devred, A. Electromechanical Modeling of Nb3Sn Superconducting Wires Subjected to Periodic Bending Strain. IEEE Trans. Appl. Supercond. 2014, 25, 1–5. [Google Scholar] [CrossRef]




| Parameter | Value |
|---|---|
| Micrography |  |
| Number of filaments | 6 |
| MgB filling factor | 29% |
| Thickness | 1.1 mm |
| Width | 2.05 mm |
| Twist pitch | 600 mm |
| Critical current at 16.2 K and 1.63 T | 772 A |
| Thickness of the tin-soldering copper strip | 500 m |
| Thickness of electrical insulation (wrapped polyester) | 125 m |
| Parameter | Value |
|---|---|
| Inner radius | 300 mm |
| Height | 1200.6 mm |
| Number of layers | 10 |
| Number of turns per layer | 522 |
| Length of cable | 10.1 km |
| Voltage of the DC-bus | 750 V |
| Min Current | 266.6 A |
| Max current | 467 A |
| Field on the conductor (at ) | 1.63 T |
| ratio (at ) | 0.6 |
| Inductance | 6.80 H |
| Total energy (at ) | 741 kJ |
| Deliverable energy | 500.4 kJ |
| Operating temperature | 16.2 K |
| Parameter | Value |
|---|---|
| Operating temperature | 16.2 K |
| Critical current density Jc of the MgB at 16.2 K and 1.63 T 1 | 1.18 × 10 A/cm |
| Power law n-exponent | 30 |
| Critical electric field of the power law | 1.0 mV/cm |
| Resistivity of Copper at 16.2 K | 0.0178 mcm |
| Resistivity of Nickel at 16.2 K | 1.1 mcm |
| Resistivity of Monel at 16.2 K | 42 mcm |
| Average AC loss per unit length of conductor at the center and at the bottom of all layers (mJ/m) | |||||
| Layers | Center | Bottom | Layers | Center | Bottom |
| layer 1 | 122.3 | 150.1 | layer 6 | 39.9 | 130.7 |
| layer 2 | 103.1 | 141.9 | layer 7 | 29.1 | 128.0 |
| layer 3 | 91.0 | 156.4 | layer 8 | 15.7 | 117.9 |
| layer 4 | 74.9 | 139.0 | layer 9 | 10.2 | 109.6 |
| layer 5 | 59.3 | 134.4 | layer 10 | 20.2 | 114.6 |
| Overall energy loss of the coil during one cycle (J) | 4319 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Morandi, A.; Fabbri, M.; Ribani, P.L.; Lo Franco, F.; Mandrioli, R.; Melaccio, U.; Magrassi, D.; Neri, M.; Capelluto, A.; Tropeano, M.; et al. Calculation of AC Losses in a 500 kJ/200 kW Multifilamentary MgB2 SMES Coil. Energies 2023, 16, 1596. https://doi.org/10.3390/en16041596
Morandi A, Fabbri M, Ribani PL, Lo Franco F, Mandrioli R, Melaccio U, Magrassi D, Neri M, Capelluto A, Tropeano M, et al. Calculation of AC Losses in a 500 kJ/200 kW Multifilamentary MgB2 SMES Coil. Energies. 2023; 16(4):1596. https://doi.org/10.3390/en16041596
Chicago/Turabian StyleMorandi, Antonio, Massimo Fabbri, Pier Luigi Ribani, Francesco Lo Franco, Riccardo Mandrioli, Umberto Melaccio, Daniele Magrassi, Martina Neri, Alessio Capelluto, Matteo Tropeano, and et al. 2023. "Calculation of AC Losses in a 500 kJ/200 kW Multifilamentary MgB2 SMES Coil" Energies 16, no. 4: 1596. https://doi.org/10.3390/en16041596
APA StyleMorandi, A., Fabbri, M., Ribani, P. L., Lo Franco, F., Mandrioli, R., Melaccio, U., Magrassi, D., Neri, M., Capelluto, A., Tropeano, M., & Gandolfi, C. (2023). Calculation of AC Losses in a 500 kJ/200 kW Multifilamentary MgB2 SMES Coil. Energies, 16(4), 1596. https://doi.org/10.3390/en16041596









