Energy and Exergy Analysis of a Geothermal Sourced Multigeneration System for Sustainable City
Abstract
:1. Introduction
2. System Description
3. Analysis
- Pressure drops across all pumps are neglected.
- The ambient state has a temperature = 30 °C and pressure 101.325 kPa.
- The turbine and pump have 80% and 50% isentropic efficiencies, respectively.
- The geothermal source temperature is 388 °C.
- The source mass flow rate is 300 kg/s.
- The electrolyzer has an efficiency of 0.56 or 56%.
3.1. Equations
3.1.1. Regenerative Rankine Cycle
3.1.2. Double Effect Vapour Absorption Cycle
3.1.3. PEM Electrolyzer
3.1.4. Space Heating
3.1.5. Double Flash Desalination Cycle
3.1.6. Overall Energy and Exergy Efficiency
3.1.7. Exergy Destruction (System)
4. Results and Discussion
4.1. State Point Values of the Subsystems
4.1.1. Geothermal Source
4.1.2. Regenerative Rankine Cycle (Power Cycle)
4.1.3. Double Effect Vapour Absorption Cycle (Cooling Cycle)
4.1.4. Double Flash Desalination Cycle
4.1.5. Hydrogen and Space Heating Production
4.2. Exergy Destruction and Energy Efficiencies Calculation
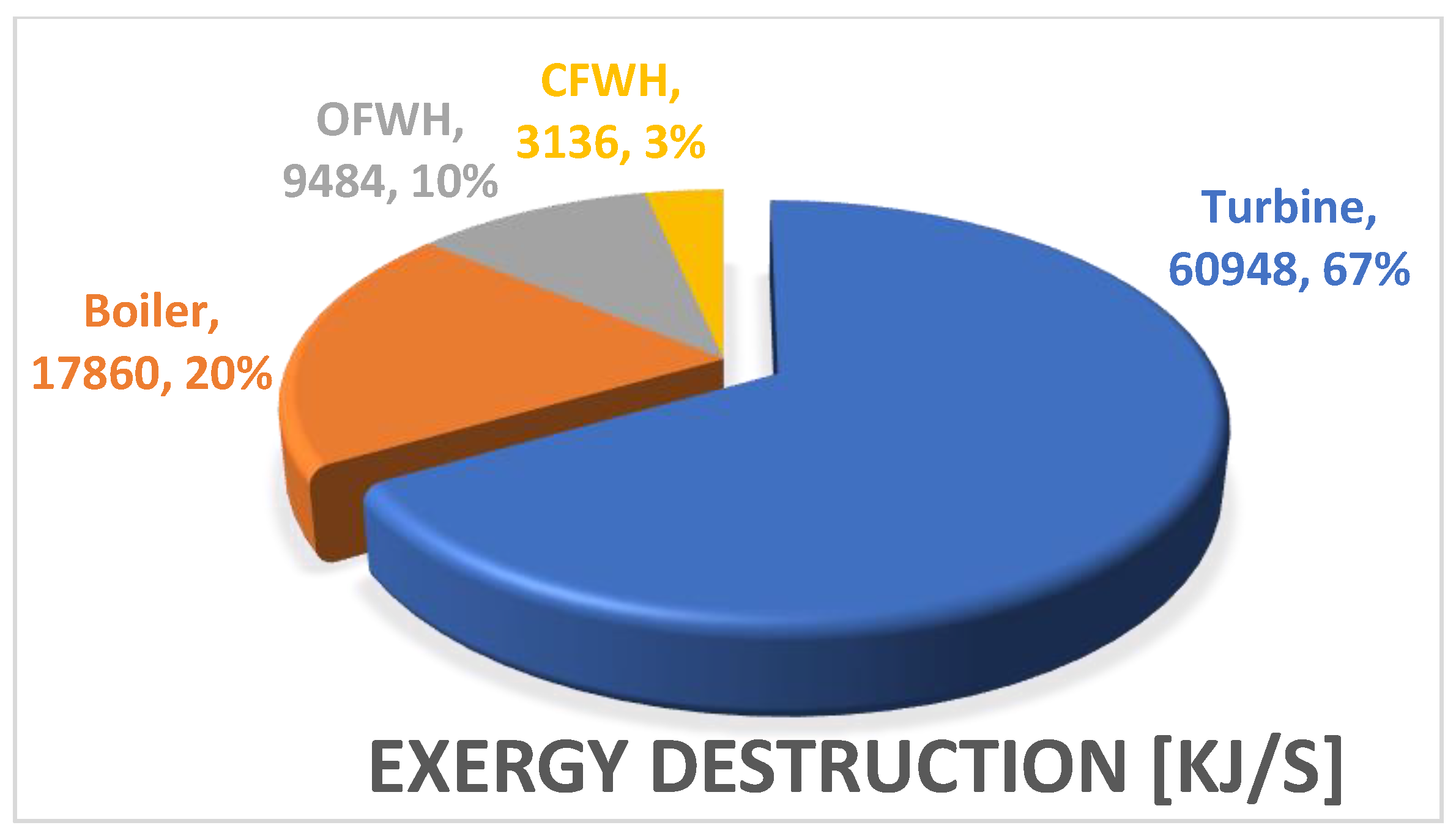
4.2.1. Major Exergy Destruction Areas
4.2.2. Energy Efficiencies at Different Production Loads
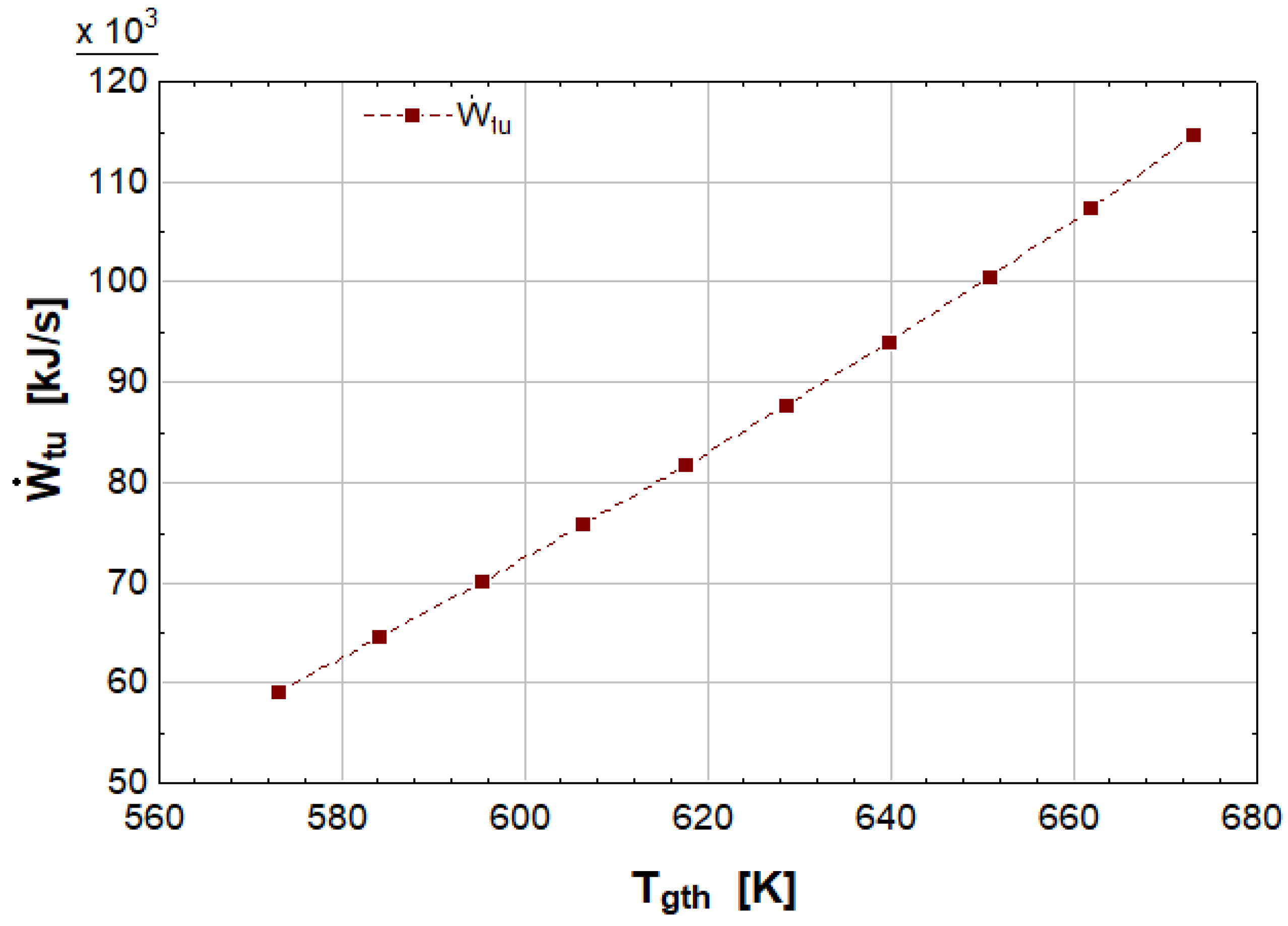
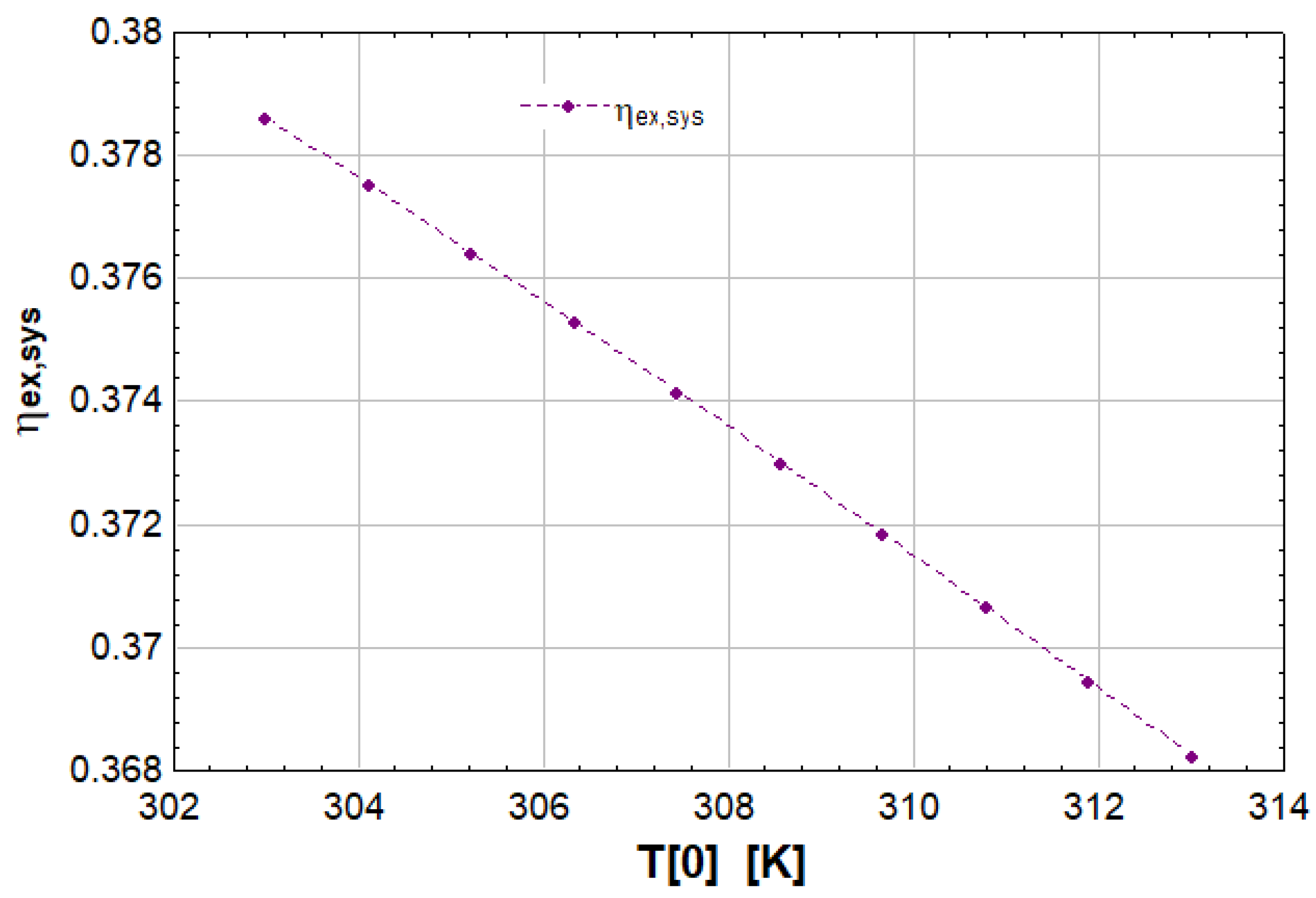
4.3. Graphical Representation of Results
5. Conclusions
- This system produces 103 MW of electricity, the production capacity of which depends on the temperature of the geothermal source. The higher the temperature, the higher would be the power generation.
- The system produces 1.35 MW and 317 MW cooling and heating capacities, respectively. These values depend on the source temperature and the output of the power cycle. Greater output power produces higher heating and cooling.
- Fresh water is produced at a rate of 37.6 kg/s. This fresh water is delivered at 40 °C; hence, a fraction of it can be used as hot water, eliminating the need to produce hot water separately.
- An amount of 0.1266 kg of hydrogen is produced per second through this system which can be transported and used to make fuel cells for automobiles or any other use.
- The system has energy and exergy efficiencies are 54.22% and 38.96%, respectively. At the same time, the coefficients of energy and exergy performance are 1.84 and 1.67, respectively. It is seen that exergy efficiency decreases with an increase in ambient temperature, which is a natural phenomenon—both COPs increase when the source temperature is increased.
- It is seen that the temperature of the geothermal source has the most significance in increasing or reducing the system’s performance. This temperature is directly responsible for power production. The higher the source temperature, the higher will be the power output. This affects power output and other products like fresh water and hydrogen production.
- Major exergy destruction areas have also been analyzed. The turbine has the highest exergy destruction rate in the whole system, indicating a significant entropy generation of 60,948 kJ/s.
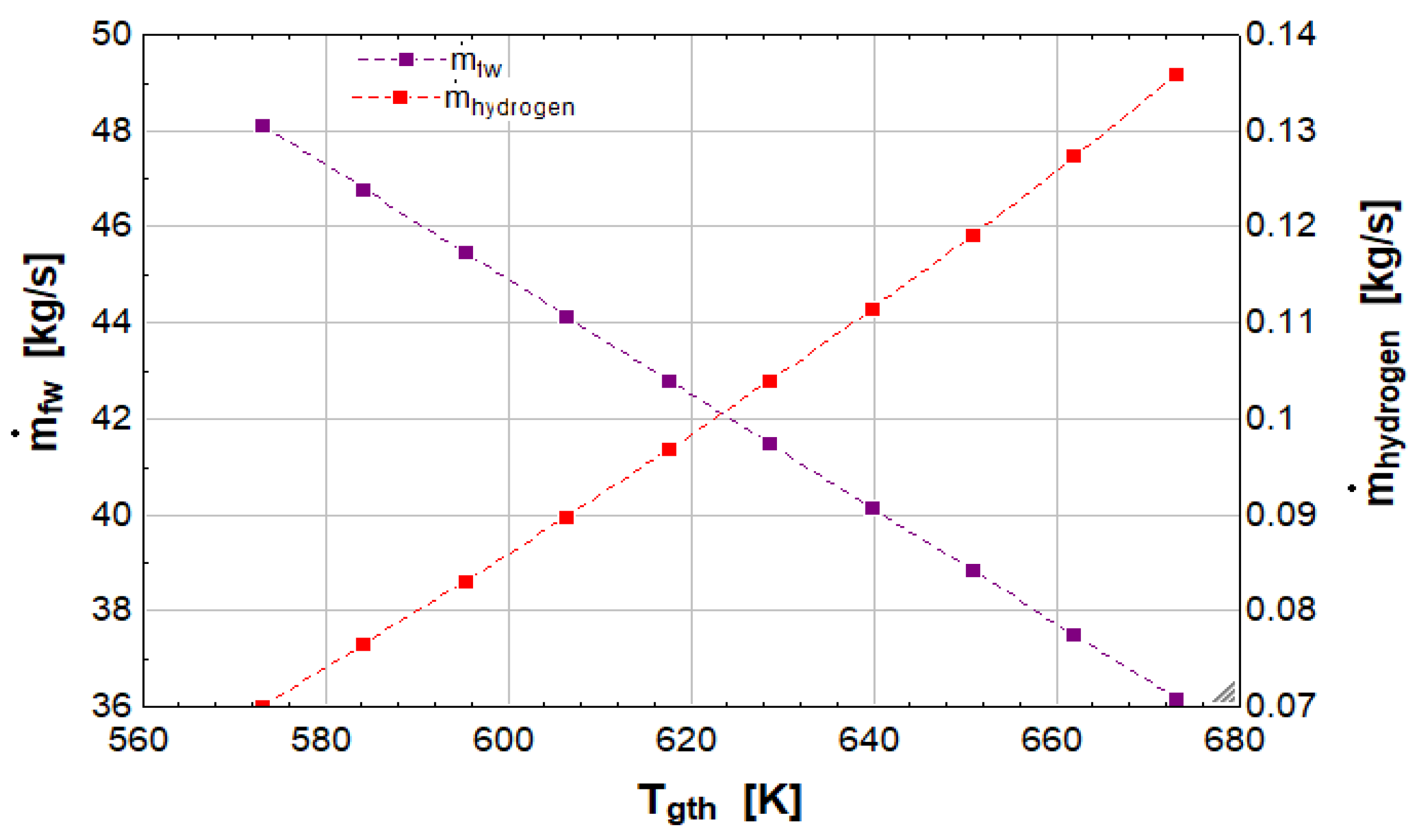
- Geothermal source temperature affects the production of fresh water and hydrogen. It increases the output of hydrogen generation and vice versa in the case of fresh water.
- Salinity has significant effects on fresh water production. An increasing variation from 20–80 (g/kg) in salinity increases fresh water production by 5 kg/s.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Symbols | |
| Efficiency | |
| Mass flow Rate | |
| Enthalpy | |
| Entropy | |
| Specific Exergy | |
| Exergy Rate | |
| Work Rate | |
| Heat Rate | |
| Temperature | |
| Thermal Efficiency | |
| Hydrogen Production Efficiency | |
| Energetic Efficiency of Fresh Water | |
| Exergy Efficiency | |
| Mass Flow Rate of Fresh Water Produced | |
| Mass Flow Rate of Hot Water Produced | |
| Heat Transfer in Condenser | |
| Heat Transfer in Boiler | |
| Turbine Work Output | |
| y | Bleed Input to Closed Feed Water Heater |
| z | Bleed Input to Open Feed Water Heater |
| Net Pump Work | |
| Net Power Output | |
| Exergy Efficiency of Power Cycle | |
| Exergetic Coefficient of Performance | |
| Energetic Coefficient of Performance | |
| Thermal Exergy of Evaporator | |
| Thermal Exergy of High-Temperature Vapour Generator | |
| Heat Transfer in Evaporator | |
| Heat Transfer through High-Temperature Generator | |
| Work Output of Electrolyzer | |
| Heat Transfer of Space Heating | |
| Exergy Destruction of the System | |
| Subscripts | |
| System | |
| Absorber | |
| Evaporator | |
| Boiler | |
| Condenser | |
| Turbine | |
| Pump | |
| Fresh Water | |
| Hot Water | |
| Sea Water | |
| Energy | |
| Exergy | |
| Electrolyzer | |
| Destruction | |
| Abbreviation | |
| Multigeneration System | |
| Heat Exchanger | |
| Expansion Valve | |
| High-Temperature Heat Exchanger | |
| Low-Temperature Heat Exchanger | |
| High-Temperature Generator | |
| Coefficient of Performance | |
| High Heating Value | |
References
- United States Census Bureau. U.S. and World Population Clock. United States Census Bureau. 2022. Available online: https://www.census.gov/popclock/ (accessed on 6 January 2023).
- Roser, M.; Ortiz-Ospina, E.; Ritchie, H.; Rodés-Guirao, L. “World Population Growth”. Our World in Data. 2013. Available online: https://ourworldindata.org/world-population-growth (accessed on 6 January 2023).
- The Intergovernmental Panel on Climate Change: 30 Years Informing Global Climate Action. 13 March 2018. Available online: https://unfoundation.org/ (accessed on 6 January 2023).
- IPCC. AR5 Climate Change 2013: The Physical Science Basis—IPCC. 2013. Available online: https://www.ipcc.ch/report/ar5/wg1/ (accessed on 6 January 2023).
- Cangel, Y.A.; Boles, M.A. Thermodynamics: An Engineering Approach 4th Edition in SI Units; McGraw-Hill: Singapore, 2002. [Google Scholar]
- International Energy Agency. IEA—The Global Energy Authority. 2022. Available online: https://www.iea.org/ (accessed on 6 January 2023).
- EPA. “US EPA”. Available online: https://www.epa.gov/ (accessed on 6 January 2023).
- Energy Information Administration. International Energy Outlook 2010. Washington, DC, USA; DOE/EIA-0484(2010). 2021. Available online: https://www.eia.gov/outlooks/ieo/ (accessed on 6 January 2023).
- Parikhani, T.; Gholizadeh, T.; Ghaebi, H.; Sadat, S.M.S.; Sarabi, M. Exergoeconomic optimization of a novel multigeneration system driven by geothermal heat source and liquefied natural gas cold energy recovery. J. Clean. Prod. 2019, 209, 550–571. [Google Scholar] [CrossRef]
- Azariyan, H.; Vajdi, M.; Takleh, H.R. Assessment of a high-performance geothermal-based multigeneration system for production of power, cooling, and hydrogen: Thermodynamic and exergoeconomic evaluation. Energy Convers. Manag. 2021, 236, 113970. [Google Scholar] [CrossRef]
- Yilmaz, F.; Ozturk, M.; Selbas, R. Modeling and design of the new combined double-flash and binary geothermal power plant for multigeneration purposes; thermodynamic analysis. Int. J. Hydrogen Energy 2021, 47, 19381–19396. [Google Scholar] [CrossRef]
- Li, K.; Ding, Y.-Z.; Ai, C.; Sun, H.; Xu, Y.-P.; Nedaei, N. Multi-objective optimization and multi-aspect analysis of an innovative geothermal-based multi-generation energy system for power, cooling, hydrogen, and freshwater production. Energy 2022, 245, 123198. [Google Scholar] [CrossRef]
- Musharavati, F.; Khanmohammadi, S.; Tariq, R. Comparative exergy, multi-objective optimization, and extended environmental assessment of geothermal combined power and refrigeration systems. Process Saf. Environ. Prot. 2021, 156, 438–456. [Google Scholar] [CrossRef]
- Habibollahzade, A.; Mehrabadi, Z.K.; Markides, C.N. Comparative thermoeconomic analyses and multi-objective particle swarm optimization of geothermal combined cooling and power systems. Energy Convers. Manag. 2021, 234, 113921. [Google Scholar] [CrossRef]
- Ansari, S.A.; Kazim, M.; Khaliq, M.A.; Ratlamwala, T.A.H. Thermal analysis of multigeneration system using geothermal energy as its main power source. Int. J. Hydrogen Energy 2021, 46, 4724–4738. [Google Scholar] [CrossRef]
- Chaiyat, N. A multigeneration system of combined cooling, heating, and power (CCHP) for low-temperature geothermal system by using air cooling. Therm. Sci. Eng. Prog. 2021, 21, 100786. [Google Scholar] [CrossRef]
- Cao, Y.; Ehyaei, M.A. Energy, exergy, exergoenvironmental, and economic assessments of the multigeneration system powered by geothermal energy. J. Clean. Prod. 2021, 313, 127823. [Google Scholar] [CrossRef]
- Ansarinasab, H.; Hajabdollahi, H. Multi-objective optimization of a geothermal-based multigeneration system for heating, power and purified water production purpose using evolutionary algorithm. Energy Convers. Manag. 2020, 223, 113476. [Google Scholar] [CrossRef]
- Ozturk, M.; Dincer, I. A new geothermally driven combined plant with energy storage option for six useful outputs in a sustainable community. Sustain. Energy Technol. Assess. 2021, 45, 101180. [Google Scholar] [CrossRef]
- Islam, S.; Dincer, I. Development, analysis and performance assessment of a combined solar and geothermal energy-based integrated system for multigeneration. Sol. Energy 2017, 147, 328–343. [Google Scholar] [CrossRef]
- Bamisile, O.; Huang, Q.; Dagbasi, M.; Adebayo, V.; Okonkwo, E.C.; Ayambire, P.; Al-Ansari, T.; Ratlamwala, T.A.H. Thermo-environ study of a concentrated photovoltaic thermal system integrated with Kalina cycle for multigeneration and hydrogen production. Int. J. Hydrogen Energy 2020, 45, 51, 26716–26732. [Google Scholar] [CrossRef]
- Ratlamwala, T.A.H.; Waseem, S.; Salman, Y.; Bham, A.A. Geothermal and solar energy–based multigeneration system for a district. Int. J. Energy Res. 2019, 43, 5230–5251. [Google Scholar] [CrossRef]
- Ebadollahi, M.; Rostamzadeh, H.; Pedram, M.Z.; Ghaebi, H.; Amidpour, M. Proposal and assessment of a new geothermal-based multigeneration system for cooling, heating, power, and hydrogen production, using LNG cold energy recovery. Renew. Energy 2019, 135, 66–87. [Google Scholar] [CrossRef]
- Alirahmi, S.M.; Assareh, E.; Pourghassab, N.N.; Delpisheh, M.; Barelli, L.; Baldinelli, A. Green hydrogen & electricity production via geothermal-driven multi-generation system: Thermodynamic modeling and optimization. Fuel 2022, 308, 122049. [Google Scholar] [CrossRef]
- Malik, M.; Dincer, I.; Rosen, M.A. Development and analysis of a new renewable energy-based multi-generation system. Energy 2015, 79, 90–99. [Google Scholar] [CrossRef]
- Bozgeyik, A.; Altay, L.; Hepbasli, A. A sub-system design comparison of renewable energy based multi-generation systems: A key review along with illustrative energetic and exergetic analyses of a geothermal energy based system. Sustain. Cities Soc. 2022, 82, 103893. [Google Scholar] [CrossRef]
- Suleman, F.; Dincer, I.; Agelin-Chaab, M. Development of an integrated renewable energy system for multigeneration. Energy 2014, 78, 196–204. [Google Scholar] [CrossRef]
- Ghaebi, H.; Namin, A.S.; Rostamzadeh, H. Performance assessment and optimization of a novel multi-generation system from thermodynamic and thermoeconomic viewpoints. Energy Convers. Manag. 2018, 165, 419–439. [Google Scholar] [CrossRef]
- Yuksel, Y.E.; Ozturk, M.; Dincer, I. Development and assessment of a novel geothermal power-based multigenerational system with hydrogen and ammonia production options. Energy Convers. Manag. 2021, 243, 114365. [Google Scholar] [CrossRef]
- Ratlamwala, T.A.H.; Dincer, I. Development of a geothermal based integrated system for building multigenerational needs. Energy Build. 2013, 62, 496–506. [Google Scholar] [CrossRef]
- Mahmoud, M.; Ramadan, M.; Naher, S.; Pullen, K.; Abdelkareem, M.A.; Olabi, A.-G. A review of geothermal energy-driven hydrogen production systems. Therm. Sci. Eng. Prog. 2021, 22, 100854. [Google Scholar] [CrossRef]
- Bicer, Y.; Dincer, I. Analysis and performance evaluation of a renewable energy based multigeneration system. Energy 2016, 94, 623–632. [Google Scholar] [CrossRef]
- Heberle, F.; Brüggemann, D. Exergy based fluid selection for a geothermal Organic Rankine Cycle for combined heat and power generation. Appl. Therm. Eng. 2010, 30, 1326–1332. [Google Scholar] [CrossRef]
- Bicer, Y.; Dincer, I. Development of a new solar and geothermal based combined system for hydrogen production. Sol. Energy 2016, 127, 269–284. [Google Scholar] [CrossRef]
- Karapekmez, A.; Dincer, I. Thermodynamic analysis of a novel solar and geothermal based combined energy system for hydrogen production. Int. J. Hydrogen Energy 2020, 45, 5608–5628. [Google Scholar] [CrossRef]
- Azhar, M.S.; Rizvi, G.; Dincer, I. Integration of renewable energy based multigeneration system with desalination. Desalination 2017, 404, 72–78. [Google Scholar] [CrossRef]
- Yuksel, Y.E.; Ozturk, M.; Dincer, I. Energetic and exergetic assessments of a novel solar power tower based multigeneration system with hydrogen production and liquefaction. Int. J. Hydrogen Energy 2019, 44, 13071–13084. [Google Scholar] [CrossRef]
- Ganjehsarabi, H. Mixed refrigerant as working fluid in Organic Rankine Cycle for hydrogen production driven by geothermal energy. Int. J. Hydrogen Energy 2019, 44, 18703–18711. [Google Scholar] [CrossRef]
- Siddiqui, O.; Ishaq, H.; Dincer, I. A novel solar and geothermal-based trigeneration system for electricity generation, hydrogen production and cooling. Energy Convers. Manag. 2019, 198, 111812. [Google Scholar] [CrossRef]
- Liu, Q.; Shang, L.; Duan, Y. Performance analyses of a hybrid geothermal–fossil power generation system using low-enthalpy geothermal resources. Appl. Energy 2016, 162, 149–162. [Google Scholar] [CrossRef]
- Gholizadeh, T.; Vajdi, M.; Rostamzadeh, H. A new trigeneration system for power, cooling, and freshwater production driven by a flash-binary geothermal heat source. Renew. Energy 2020, 148, 31–43. [Google Scholar] [CrossRef]
- Rostamzadeh, H.; Gargari, S.G.; Namin, A.S.; Ghaebi, H. A novel multigeneration system driven by a hybrid biogas-geothermal heat source, Part I: Thermodynamic modeling. Energy Convers. Manag. 2018, 177, 535–562. [Google Scholar] [CrossRef]
- Waseem, S.; Ratlamwala, T.A.H.; Salman, Y.; Bham, A.A. Geothermal and solar based mutligenerational system: A comparative analysis. Int. J. Hydrogen Energy 2020, 45, 5636–5652. [Google Scholar] [CrossRef]
- Geothermal Energy Knowledge. Available online: https://eniscuola.eni.com/en-IT/energy/geothermal/geothermal-energy-knowledge.html (accessed on 8 January 2023).
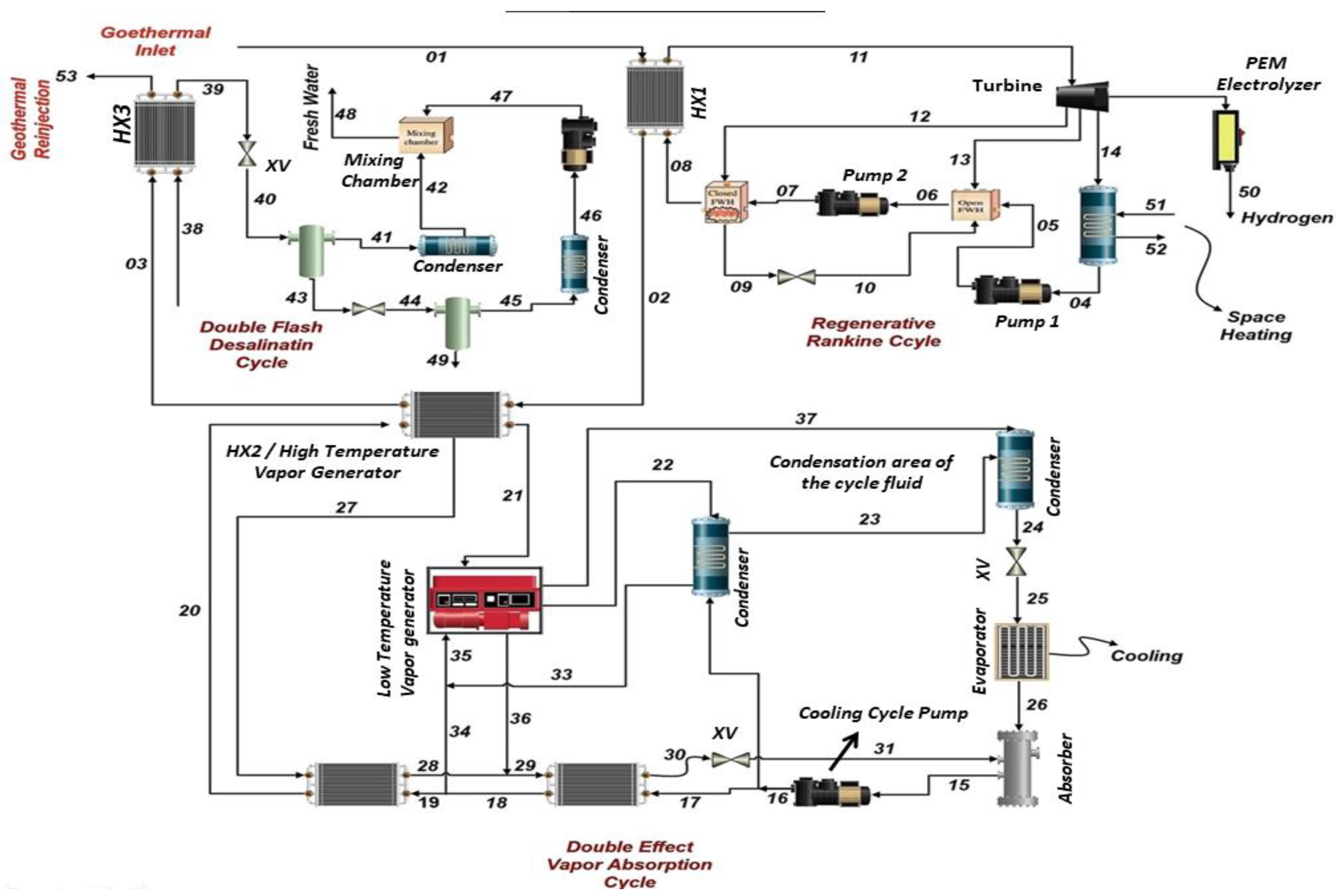



| State Point | Temperature, T [K] | Pressure, P [kPa] | Enthalpy, h [kJ/kg] | Entropy, s [kJ/kg K] | Exergy, ex [kJ/kg] | Mass Flow Rate, m [kg/s] |
|---|---|---|---|---|---|---|
| 1 | 661 | 15,000 | 2992 | 5.802 | 1171 | 300 |
| 2 | 382.4 | 15,000 | 469 | 1.398 | 51.92 | 300 |
| 3 | 381.9 | 15,000 | 467 | 1.393 | 51.51 | 300 |
| State Point | Temperature, T [K] | Pressure, P [kPa] | Enthalpy, h [kJ/kg] | Entropy, s [kJ/kg K] | Exergy, ex [kJ/kg] | Mass Flow Rate, m [kg/s] |
|---|---|---|---|---|---|---|
| 4 | 333.2 | 20 | 251.4 | 0.832 | 5.826 | 165.5 |
| 5 | 333.2 | 100 | 251.5 | 0.8321 | 5.909 | 165.5 |
| 6 | 372.8 | 100 | 417.5 | 1.303 | 29.27 | 211.8 |
| 7 | 374.7 | 15,000 | 436.9 | 1.313 | 45.52 | 211.8 |
| 8 | 438.1 | 15,000 | 705.3 | 1.975 | 113.5 | 211.8 |
| 9 | 438.1 | 700 | 697 | 1.992 | 100 | 35.34 |
| 10 | 372.8 | 100 | 697 | 2.053 | 81.58 | 35.34 |
| 11 | 623 | 15,000 | 2692 | 5.442 | 1049 | 211.8 |
| 12 | 438.1 | 700 | 2305 | 5.662 | 595.9 | 35.34 |
| 13 | 372.8 | 100 | 2029 | 5.627 | 330.9 | 10.92 |
| 14 | 333.2 | 20 | 2173 | 6.598 | 180 | 165.5 |
| State Point | Temperature, T [K] | Pressure, P [kPa] | Enthalpy, h [kJ/kg] | Entropy, s [kJ/kg K] | Exergy, ex [kJ/kg] | Mass Flow Rate, m [kg/s] |
|---|---|---|---|---|---|---|
| 15 | 273.9 | 200 | −225.9 | −0.1641 | 72.78 | 2.5 |
| 16 | 274.1 | 700 | −224.7 | −0.1619 | 73.34 | 2.5 |
| 17 | 274.1 | 700 | −224.7 | −0.1619 | 73.34 | 2 |
| 18 | 284.1 | 700 | −178.8 | 0.002663 | 69.4 | 2 |
| 19 | 284.1 | 700 | −178.8 | 0.002663 | 69.4 | 1.6 |
| 20 | 294.1 | 700 | −133.1 | 0.1606 | 67.2 | 1.6 |
| 21 | 287 | 700 | 760.5 | 2.666 | 303.6 | 0.6957 |
| 22 | 232.3 | 700 | −183.3 | −0.716 | 0.8637 | 0.6957 |
| 23 | 229.1 | 700 | −197.6 | −0.7783 | 386.1 | 0.6957 |
| 24 | 278.9 | 700 | 26.49 | 0.1063 | 342.2 | 1.087 |
| 25 | 254.3 | 200 | 26.49 | 0.1274 | 335.8 | 1.087 |
| 26 | 265.3 | 200 | 1271 | 4.906 | 104.9 | 1.087 |
| 27 | 314.1 | 700 | −9.393 | 0.5257 | 1.524 | 0.9043 |
| 28 | 295.3 | 700 | −90.18 | 0.2606 | 1.095 | 0.9043 |
| 29 | 297.4 | 700 | −81.44 | 0.29 | 0.8992 | 1.413 |
| 30 | 282.4 | 700 | −146.4 | 0.06571 | 3.864 | 1.413 |
| 31 | 282.5 | 200 | −146.4 | 0.06768 | 3.269 | 1.413 |
| 32 | 274.1 | 700 | −224.7 | −0.1619 | 73.34 | 0.5 |
| 33 | 278.5 | 700 | −204.7 | −0.08949 | 71.4 | 0.5 |
| 34 | 284.1 | 700 | −178.8 | 0.002663 | 69.4 | 0.4 |
| 35 | 281 | 700 | −193.2 | −0.0483 | 70.45 | 0.9 |
| 36 | 301 | 700 | −65.91 | 0.3419 | 0.6997 | 0.5086 |
| 37 | 301 | 700 | 1319 | 4.591 | 276.2 | 0.3914 |
| State Point | Temperature, T [K] | Pressure, P [kPa] | Enthalpy, h [kJ/kg] | Entropy, s [kJ/kg K] | Exergy, ex [kJ/kg] | Mass Flow Rate, m [kg/s] |
|---|---|---|---|---|---|---|
| 38 | 303 | 101.3 | 120.6 | 0.4204 | 2.682 | 287.5 |
| 39 | 388 | 101.3 | 464.3 | 1.42 | 44.7 | 287.5 |
| 40 | 319 | 10 | 464.3 | 1.504 | 15.24 | 287.5 |
| 41 | 319 | 10 | 183.9 | 0.623 | 4.337 | 254.7 |
| 42 | 306 | 5 | 183.9 | 0.457 | 2.738 | 254.7 |
| 43 | 306 | 5 | 2560 | 8.393 | 23.91 | 4.851 |
| 44 | 306 | 5 | 137.8 | 0.4762 | −0.03407 | 4.851 |
| 45 | 306 | 10 | 137.8 | 0.4762 | −0.03407 | 4.851 |
| 46 | 319 | 10 | 2584 | 8.148 | 121.23 | 32.75 |
| 47 | 319 | 10 | 191.8 | 0.6492 | 1.605 | 32.75 |
| 48 | 317.3 | 10 | 184.8 | 0.6237 | 1.274 | 37.6 |
| 49 | 306 | 5 | 131.9 | 0.4566 | 2.738 | 249.9 |
| State Point | Temperature, T [K] | Pressure, P [kPa] | Enthalpy, h [kJ/kg] | Entropy, s [kJ/kg K] | Exergy, ex [kJ/kg] | Mass Flow Rate, m [kg/s] |
|---|---|---|---|---|---|---|
| 50 | 303 | 101.325 | 69.59 | 65 | 117,117 | 0.1266 |
| 51 | 283 | 101.325 | 283.4 | 5.643 | 0.6994 | 15,837 |
| 52 | 303 | 101.325 | 303.4 | 5.712 | 0 | 15,837 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Haider, S.M.A.; Ratlamwala, T.A.H.; Kamal, K.; Alqahtani, F.; Alkahtani, M.; Mohammad, E.; Alatefi, M. Energy and Exergy Analysis of a Geothermal Sourced Multigeneration System for Sustainable City. Energies 2023, 16, 1616. https://doi.org/10.3390/en16041616
Haider SMA, Ratlamwala TAH, Kamal K, Alqahtani F, Alkahtani M, Mohammad E, Alatefi M. Energy and Exergy Analysis of a Geothermal Sourced Multigeneration System for Sustainable City. Energies. 2023; 16(4):1616. https://doi.org/10.3390/en16041616
Chicago/Turabian StyleHaider, Sheikh Muhammad Ali, Tahir Abdul Hussain Ratlamwala, Khurram Kamal, Fahad Alqahtani, Mohammed Alkahtani, Emad Mohammad, and Moath Alatefi. 2023. "Energy and Exergy Analysis of a Geothermal Sourced Multigeneration System for Sustainable City" Energies 16, no. 4: 1616. https://doi.org/10.3390/en16041616
APA StyleHaider, S. M. A., Ratlamwala, T. A. H., Kamal, K., Alqahtani, F., Alkahtani, M., Mohammad, E., & Alatefi, M. (2023). Energy and Exergy Analysis of a Geothermal Sourced Multigeneration System for Sustainable City. Energies, 16(4), 1616. https://doi.org/10.3390/en16041616










