Optimal SOFC-CHP Installation Planning and Operation Model Considering Geographic Characteristics of Energy Supply Infrastructure
Abstract
:1. Introduction
1.1. Motivation
1.2. Literature Survey
1.3. Contributions
1.4. Paper Organization
2. Materials and Methods
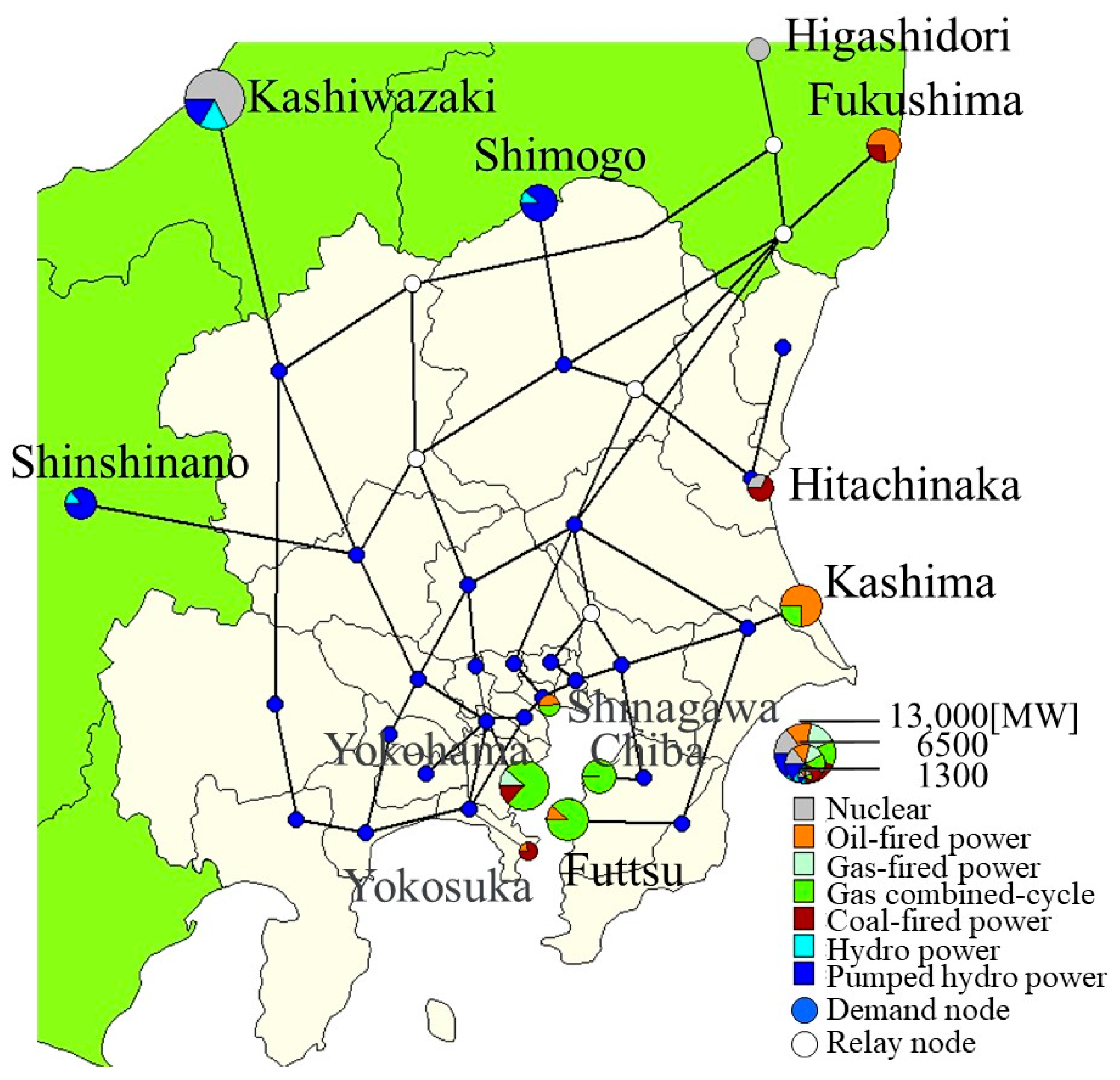
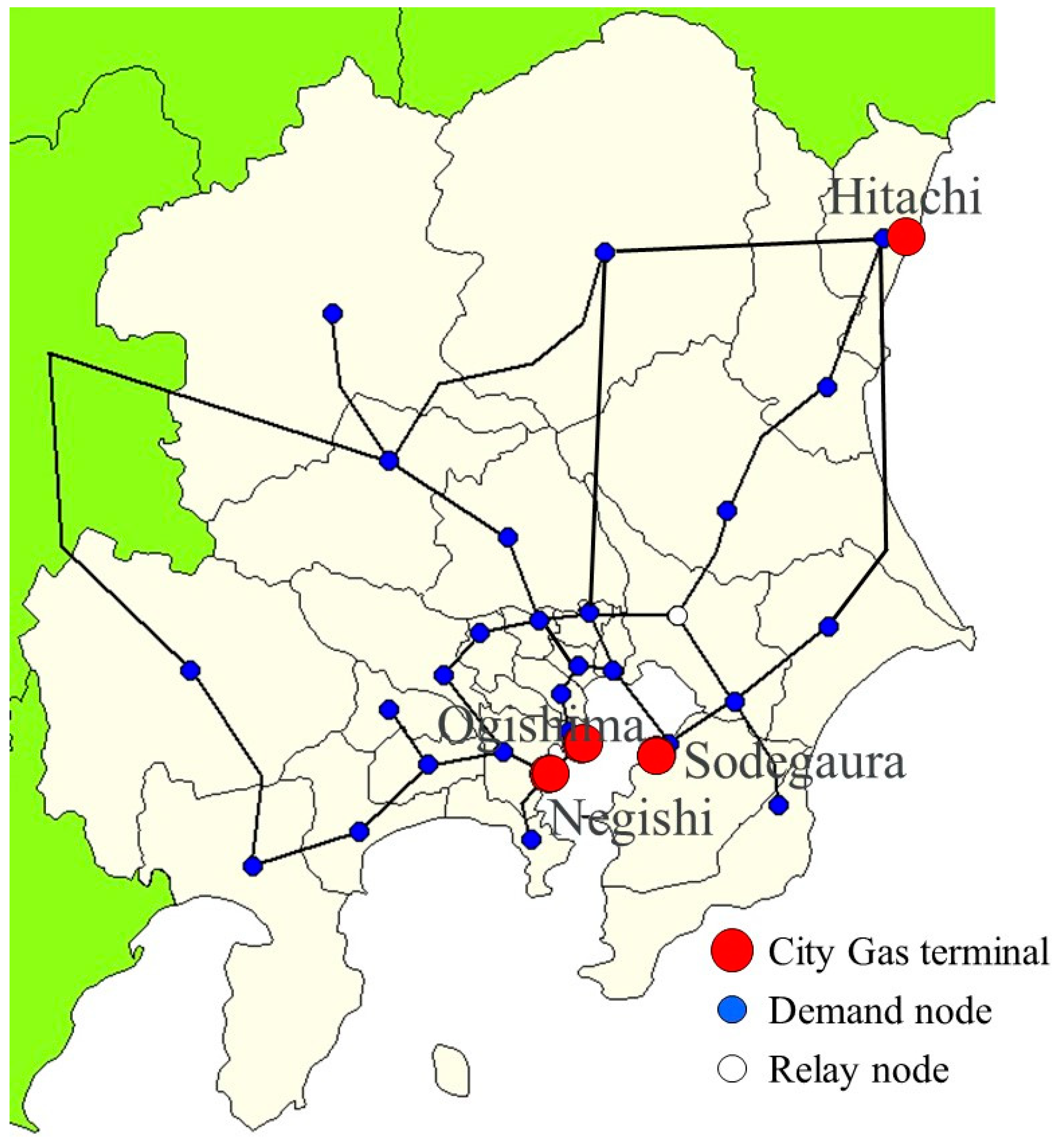
2.1. Data
2.2. Methods for the Model Development
2.2.1. Power Grid Sub-Model
2.2.2. City Gas Grid Sub-Model
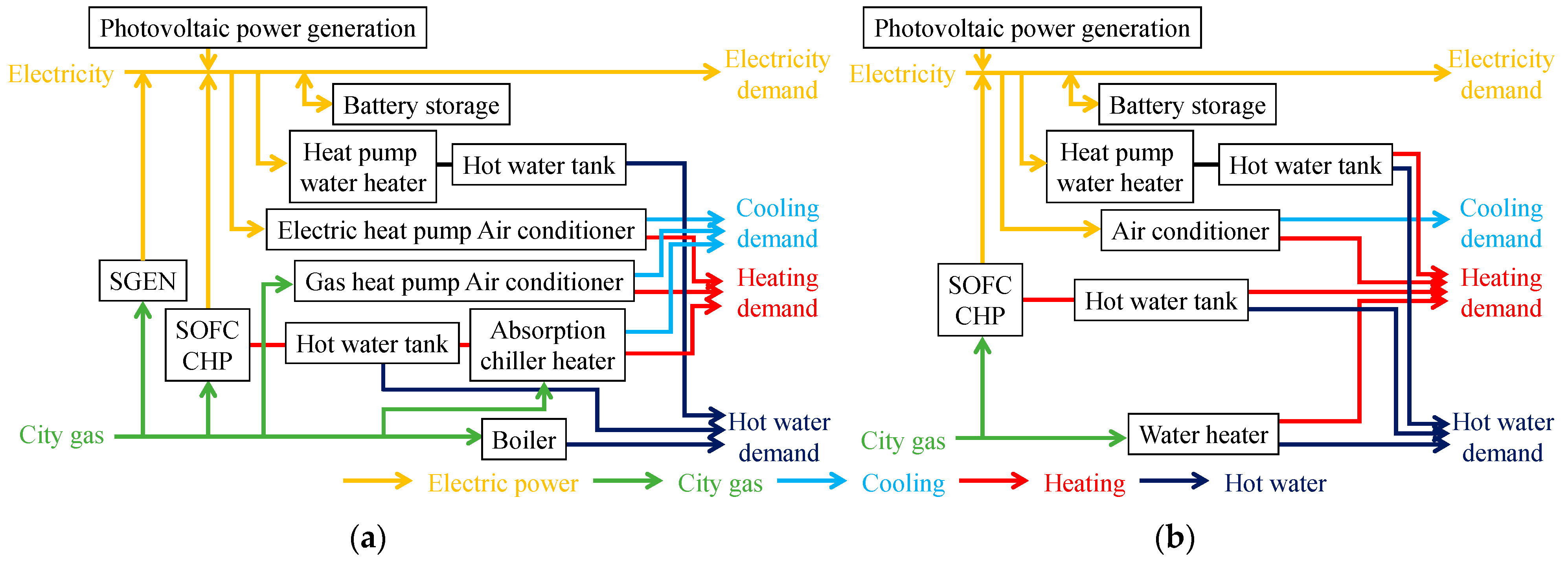
2.2.3. Consumer Facilities Sub-Model
2.2.4. Other Settings
2.2.5. Sensitivity Analysis
3. Results and Discussion
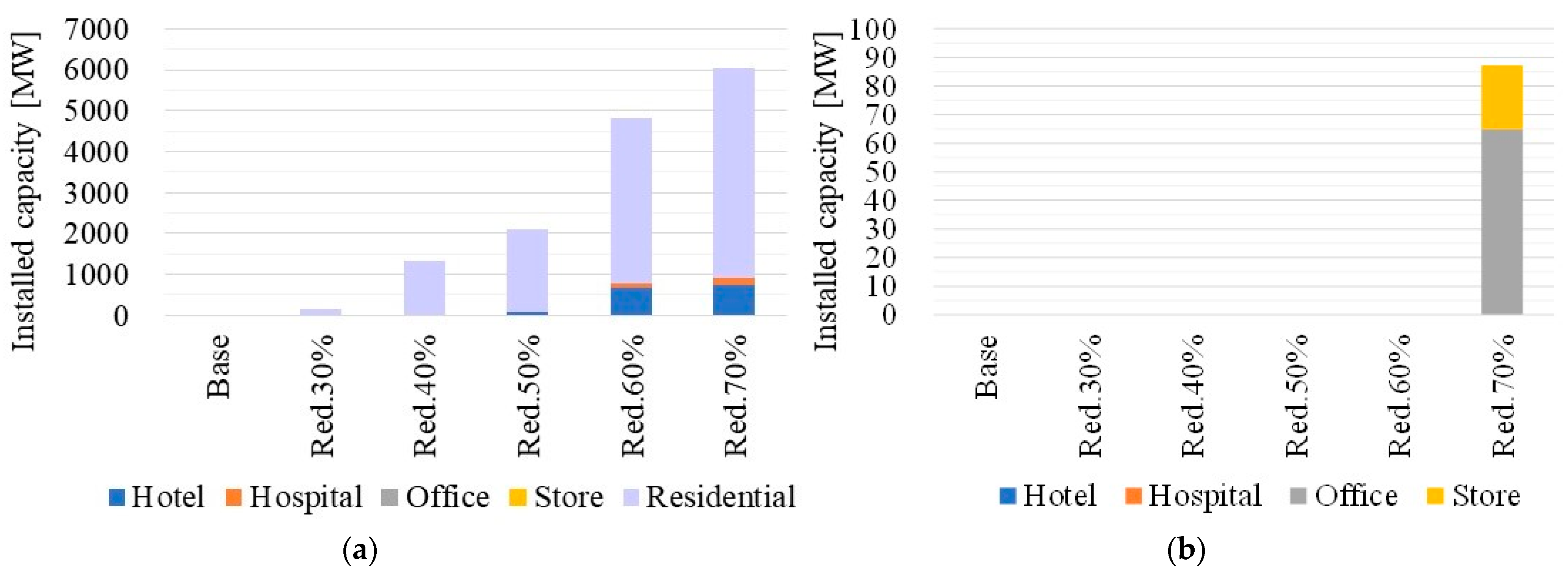
3.1. Amount of SOFC-CHP Installation
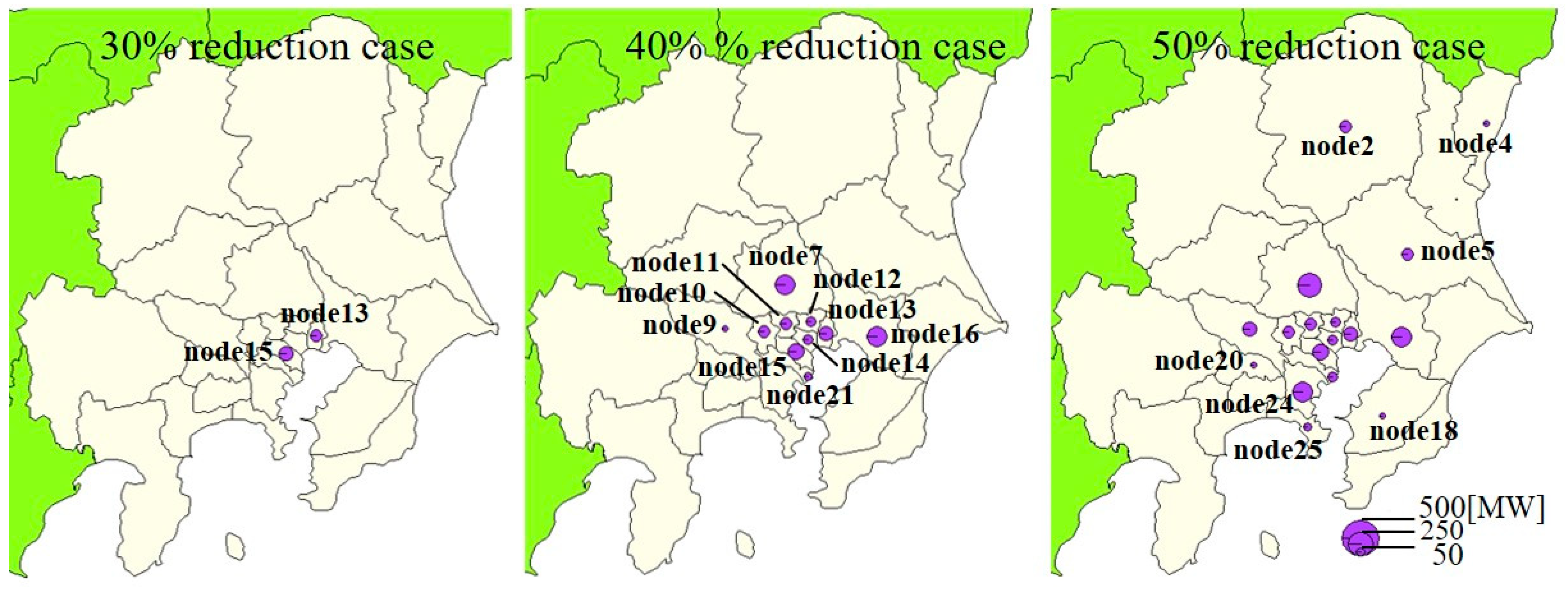
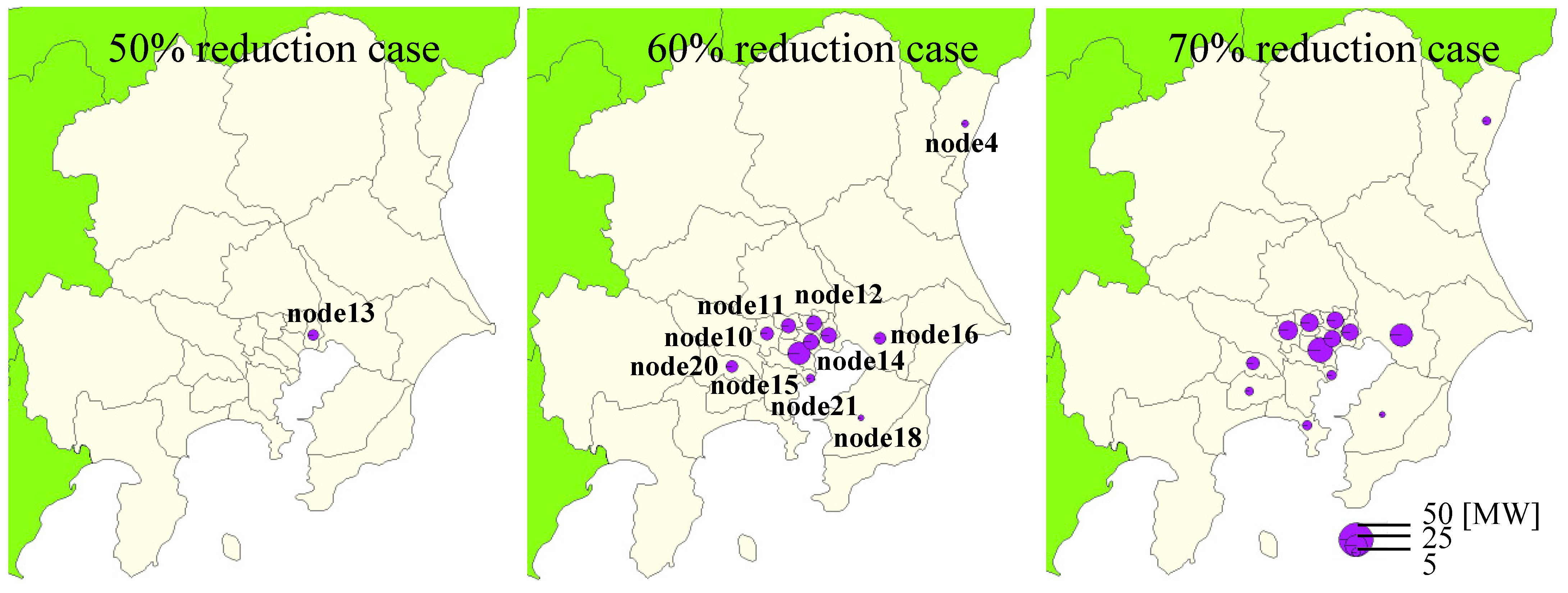
3.2. Location of SOFC-CHP Installation
3.3. Power Source Configuration
3.4. Carbon Dioxide Emission
3.5. Limitations of the Proposed Model
4. Conclusions
- Hot water demand as a percentage of demand was identified as a significant contributing factor for the introduction of SOFC-CHP. The simulation showed that the introduction of SOFC-CHP started with the residential sector, which exhibits the largest share of hot-water demand, followed by the hotel and hospital sectors. On the other hand, it was found to be difficult to introduce SOFC-CHP to offices and stores, where there is no demand for hot water supply. For SOFC-CHP to be spread over a larger area, installation cost in the residential sector ought to be reduced to 3870 USD/kW (40% lower than the base value). On the other hand, for the commercial sector, a significant cost reduction was required to spread SOFC-CHP compared to the residential sector: 1820 USD/kW for hotels (60% reduction from the base value), 1365 USD/kW for hospitals (70% reduction from the base value), and even greater reductions for offices and stores.
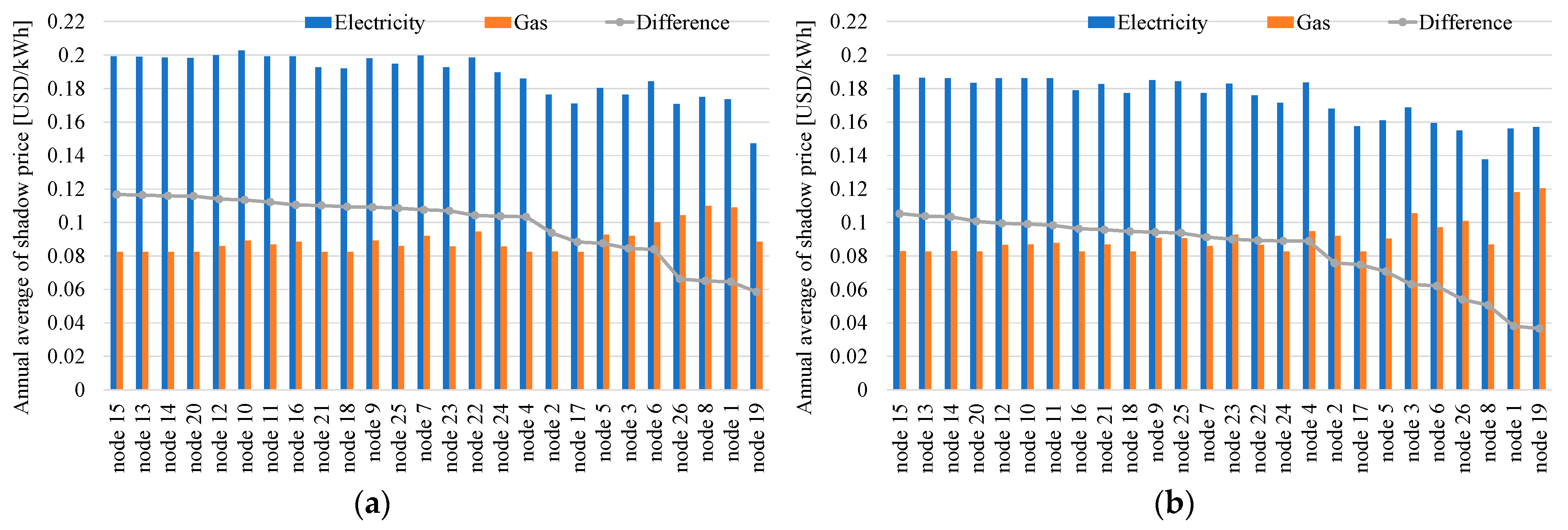
- The introduction of SOFC-CHP was additionally dependent on geographical features; the introduction was observed to proceed from areas with large shadow price differences between electricity and city gas, thus alleviating transmission congestion. The study found that the average power shadow price of all demand nodes decreased by 7.6% when approximately 6 GW of SOFC-CHP was installed.
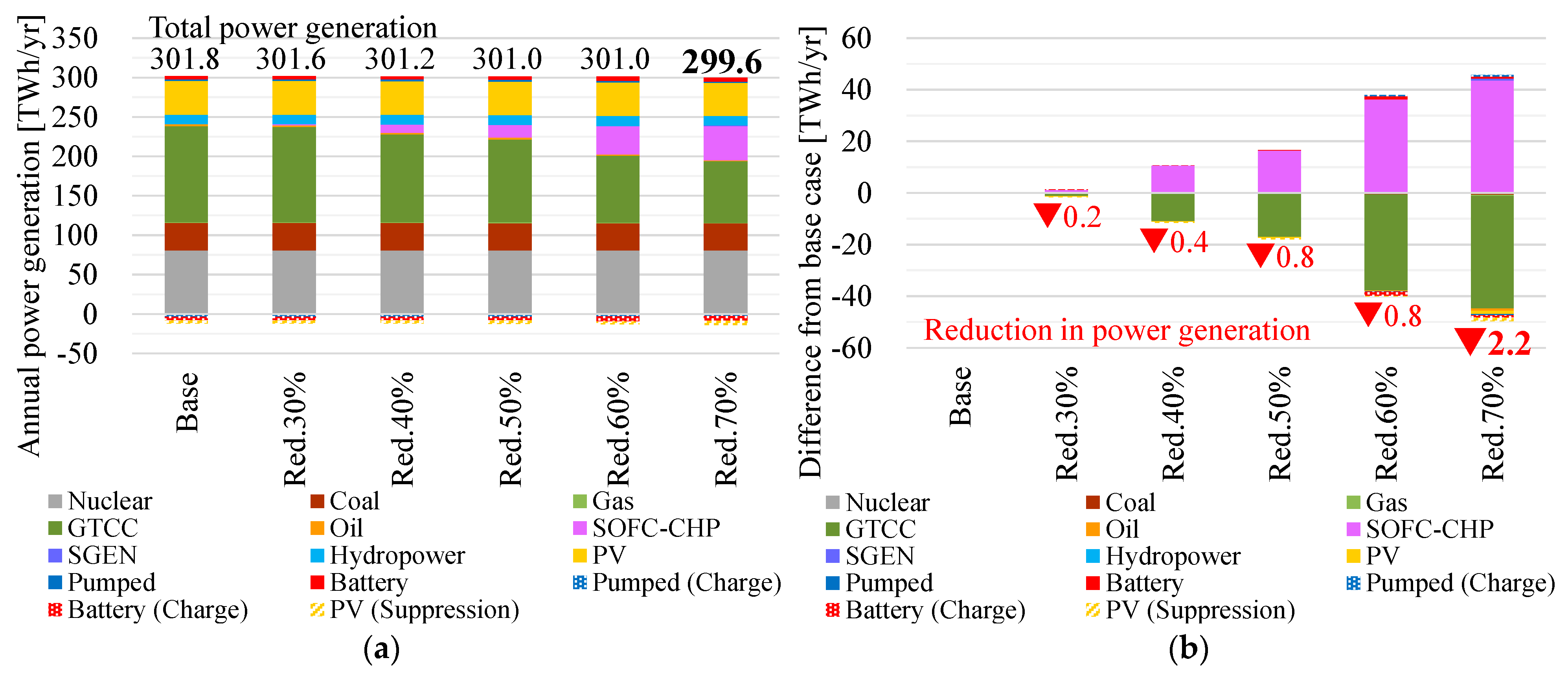
- As SOFC-CHP was installed as a substitute for GTCC, even if SOFC-CHP became widely used, its impact on the amount of PV output suppression and battery storage recharge/discharge would be marginal. On the other hand, the diffusion of SOFC-CHP decreased the amount of transmission and distribution losses, resulting in a decrease in the total power generation. In this estimation, the amount of electricity generated decreased by 2.2 TWh/y when about 6 GW of SOFC-CHP was diffused.
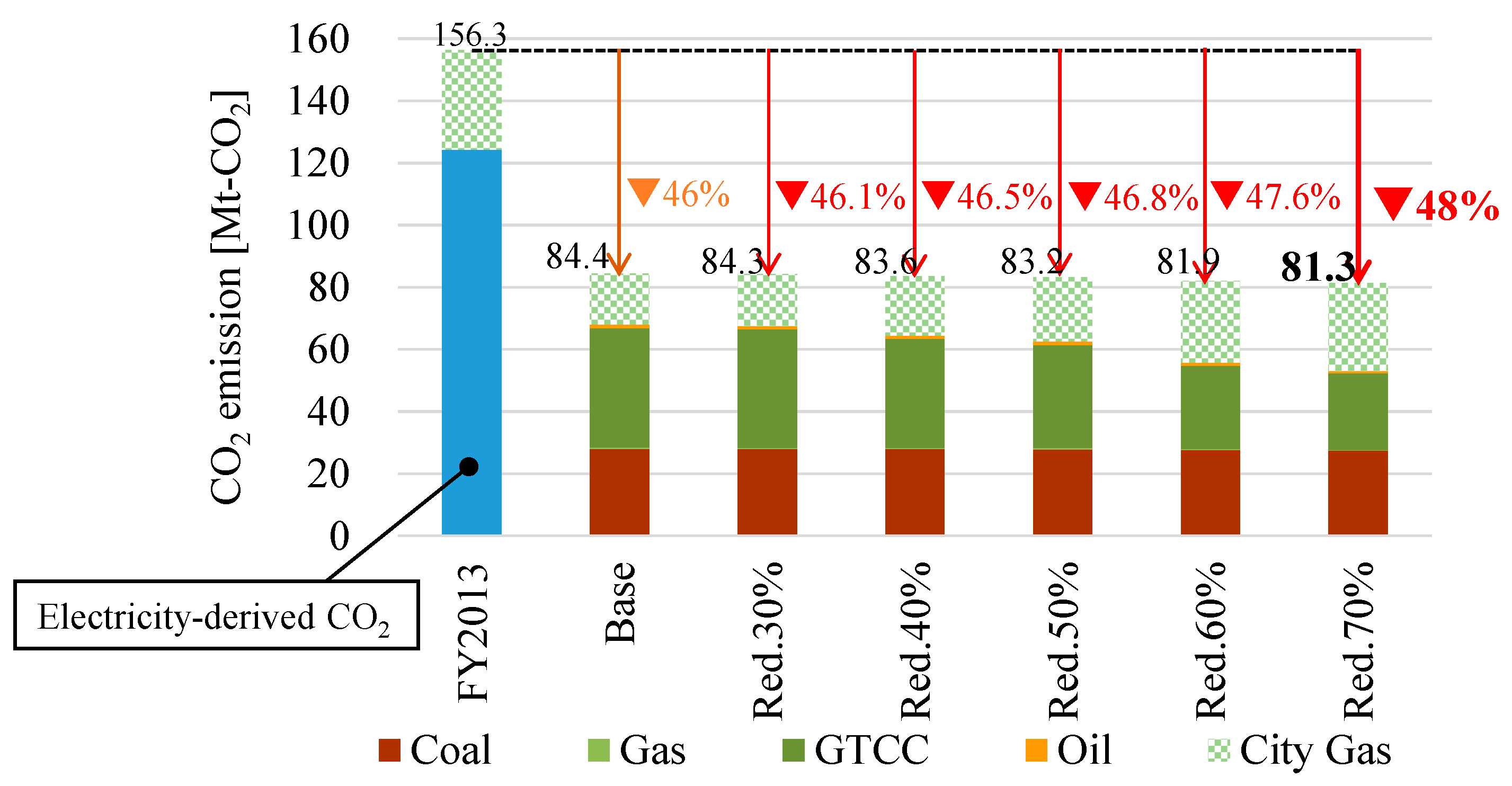
- The diffusion of SOFC-CHP will reduce CO2 emissions in an economically feasible pathway. According to this estimation, if about 6 GW of SOFC-CHP was deployed, an additional reduction of 3.1 Mt-CO2 emissions would be possible.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| AD | Matrix components to calculate load flow |
| AF | Matrix components to calculate load flow |
| AG | Matrix components to calculate city gas flow |
| AP | Matrix components to calculate city gas flow |
| CO2upper | Upper limit of CO2 emissions (84 Mt-CO2) |
| days | Total days in a seasonal category (day) |
| De | Total electricity demand (kW) |
| DFIX | Amortization cost of consumer equipment |
| Dg | Total city gas demand (kW) |
| dloss | Distribution loss (2.5%) |
| EFIX | Amortization cost of power plants |
| EFUEL | Fuel cost of power plants |
| eunit | CO2 emission intensity of power plants |
| F | Load flow of transmission lines |
| GFIX | Amortization cost of terminals and pipelines |
| GFUEL | City gas cost |
| gunit | CO2 emission intensity of city gas |
| i | Index for power plant nodes (1…12) |
| j | Index for power plant types (1…7) |
| k | Index for transmission lines (1…53) |
| l | Index for demand nodes (1…26) |
| loss | Transmission loss (2.5%) |
| m | Index for terminal nodes (1…4) |
| Make | City gas terminal output (kW) |
| minLF | minimum load factor of SOFC (50%) |
| n | Index for pipelines (1…34) |
| p | Index for demand types (1…5) |
| PF | City gas flow of pipelines |
| s | Index for seasonal categories (1…19) |
| shonai | Power plant consumption rate (%) |
| SOFC | Installed CHP capacity of SOFC (kW) |
| SOFCop | Power output of SOFC-CHP (kW) |
| SGEN | Installed generator capacity of SOFC (kW) |
| SGENop | Power output of SOFC generator (kW) |
| St | Electricity for pumping (kW) |
| t | Index for time slots of a day (1…24) |
| TC | Total annual system cost (Objective function) |
| TFIX | Amortization cost of transmission and distribution equipment |
| X | Power output (kW) |
| Yall | Power plant capacity (kW) |
| zg | Maximum operation rate of power plants (%) |
| ze | Required reserve margin for power grids (5%) |
References
- United Nations Climate Change Conference (COP26) at the Scottish Event Campus Glasgow. 2021. Available online: https://ukcop26.org/cop26-goals/ (accessed on 13 October 2022).
- Ministry of Economy, Trade and Industry. 6th Strategic Energy Plan. October 2021. Available online: https://www.meti.go.jp/english/press/2021/1022_002.html (accessed on 13 October 2022).
- Ministry of Economy, Trade and Industry. Action Plan of the Green Growth Strategy for 2050 Carbon Neutral. December 2020. Available online: https://www.meti.go.jp/english/press/2020/1225_001.html (accessed on 13 October 2022).
- United States Department of Energy. Combined Heat and Power Deployment Program Overview. 26 May 2021. Available online: https://betterbuildingssolutioncenter.energy.gov/resources/combined-heat-and-power-deployment-program-overview (accessed on 13 October 2022).
- European Steel Association. Prioritising the Use of High-Efficiency Cogeneration by Industrial Operators to Reach 2030 Climate Goals. Available online: https://www.eurofer.eu/assets/publications/position-papers/prioritising-the-use-of-high-efficiency-cogeneration-by-industrial-operators-to-reach-2030-climate-goalsnew-publication-page/FINAL_Industrial-CHP-operators_2-03-22.pdf (accessed on 13 October 2022).
- Li, X.; Wu, X.; Gui, D.; Hua, Y.; Guo, P. Power system planning based on CSP-CHP system to integrate variable renewable energy. Energy 2021, 232, 121064. [Google Scholar] [CrossRef]
- Ahn, H.; Miller, W.; Sheaffer, P.; Tutterow, V.; Rapp, V. Opportunities for installed combined heat and power (CHP) to increase grid flexibility in the U.S. Energy Policy 2021, 157, 112485. [Google Scholar] [CrossRef]
- Narayanan, M.; Mengedoht, G.; Commerell, W. Evaluation of SOFC-CHP’s ability to integrate thermal and electrical energy system decentrally in a single-family house with model predictive controller. Sustain. Energy Technol. Assess. 2021, 48, 101643. [Google Scholar] [CrossRef]
- Arinami, Y.; Sakaguchi, J.; Nakabayashi, S. Study on primary energy reduction effect by installing home-use fuel cell cogeneration system. J. Environ. Eng. (Trans. AIJ) 2019, 84, 687–697. (In Japanese) [Google Scholar] [CrossRef]
- Yuasa, K.; Yata, M.; Niino, S.; Park, S. Evaluation on SOFC-CGS installation with consideration to electricity and hot water demand variation. J. Environ. Eng. (Trans. AIJ) 2015, 80, 543–550. (In Japanese) [Google Scholar] [CrossRef] [Green Version]
- Aoki, T.; Shimoda, Y. Evaluation of residential fuel cell as the alternative to thermal power plants. JSER 2021, 42, 305–314. (In Japanese) [Google Scholar]
- Nielsen, E.R.; Prag, C.B.; Bachmann, T.M.; Carnicelli, F.; Boyd, E.; Walker, I.; Ruf, L.; Stephens, A. Status on demonstration of fuel cell based micro-CHP units in Europe. Fuel Cells 2019, 19, 340–345. [Google Scholar] [CrossRef]
- Palomba, V.; Ferraro, M.; Frazzica, A.; Vasta, S.; Sergi, F.; Antonucci, V. Experimental and numerical analysis of a SOFC-CHP system with adsorption and hybrid chillers for telecommunication applications. Appl. Energy 2018, 216, 620–633. [Google Scholar] [CrossRef]
- Jing, R.; Wang, M.; Wang, W.; Brandon, N.; Li, N.; Chen, J.; Zhao, Y. Economic and environmental multi-optimal design and dispatch of solid oxide fuel cell based CCHP system. Energy Convers. Manag. 2017, 154, 365–379. [Google Scholar] [CrossRef]
- Alns, A.; Sleiti, A.K. Combined heat and power system based on Solid Oxide Fuel Cells for low energy commercial buildings in Qatar. Sustain. Energy Technol. Assess. 2021, 48, 101615. [Google Scholar] [CrossRef]
- Oshiro, K.; Masui, T. Analysis of long-term mitigation scenarios in Japan using multi-regional end-use model. JSER 2014, 41, 31–39. (In Japanese) [Google Scholar] [CrossRef]
- Nagata, T.; Sano, F.; Akimoto, K. Analyses on the contribution of natural gas in the world and Japan as medium- and long-term global warming countermeasures. JSER 2020, 41, 196–208. (In Japanese) [Google Scholar] [CrossRef]
- Sugiyama, T.; Komiyama, R.; Fujii, Y. Optimal power generation mix model considering nationwide high-voltage power grid in Japan for the analysis of large-scale integration of PV and wind power generation. IEEJ Trans. Power Energy 2016, 136, 864–875. (In Japanese) [Google Scholar] [CrossRef]
- Johanna, B.; Lisa, G.; Fredrik, N.; Filip, J. A multiple system level modeling approach to coupled energy markets: Incentives for combined heat and power generation at the plant, city and regional energy system levels. Energy 2022, 254, 124337. [Google Scholar] [CrossRef]
- Novoa, L.; Neal, R.; Samuelsen, S.; Brouwer, J. Fuel cell transmission integrated grid energy resources to support generation-constrained power systems. Appl. Energy 2020, 276, 115485. [Google Scholar] [CrossRef]
- Shaffer, B.; Tarroja, B.; Samuelsen, S. Dispatch of fuel cells as Transmission Integrated Grid Energy Resources to support renewables and reduce emissions. Appl. Energy 2015, 148, 178–186. [Google Scholar] [CrossRef]
- Abdullah, M.A.; Muttaqi, K.M.; Agalgaonkar, A.P. Sustainable energy system design with distributed renewable resources considering economic, environmental and uncertainty aspects. Renew. Energy 2015, 78, 165–172. [Google Scholar] [CrossRef]
- Mike, B.N.; Sasa, Z.D.; Ignacio, H.G. Reliability Enhancement in Power Networks under Uncertainty from Distributed Energy Resources. Energies 2019, 12, 531. [Google Scholar] [CrossRef] [Green Version]
- Takuma, T.; Hirohisa, A.; Kotaro, K.; Masayoshi, I. Assessment of utilization of combined heat and power systems to provide grid flexibility alongside variable renewable energy systems. Energy 2021, 214, 118951. [Google Scholar] [CrossRef]
- Mathiesen, B.V.; Lund, H.; Karlsson, K. 100% Renewable energy systems, climate mitigation and economic growth. Appl. Energy 2011, 88, 488–501. [Google Scholar] [CrossRef]
- Lund, H.; Möller, B.; Mathiesen, B.V.; Dyrelund, A. The role of district heating in future renewable energy systems. Energy 2010, 35, 1381–1390. [Google Scholar] [CrossRef]
- Ministry of Economy, Trade and Industry. The Strategic Road Map for Hydrogen and Fuel Cells. March 2019. Available online: https://www.meti.go.jp/english/press/2019/0918_001.html (accessed on 13 October 2022).
- Tokyo Electric Power Company. Information on System Empty Capacity. Available online: https://www.tepco.co.jp/pg/consignment/system/pdf_new/akiyouryou_kikan.pdf (accessed on 13 October 2022). (In Japanese).
- Ministry of Economy, Trade and Industry. Report to the Subcommittee on Long-Term Energy Supply and Demand Outlook on the Verification of Power Generation Costs. May 2015. Available online: https://www.enecho.meti.go.jp/committee/council/basic_policy_subcommittee/mitoshi/cost_wg/006/pdf/006_05.pdf (accessed on 13 October 2022). (In Japanese)
- Gas Business Handbook 2021; The Japan Gas Association: Tokyo, Japan, 2022. (In Japanese)
- Ministry of Economy, Trade and Industry. Creating an Environment for Expanding Wholesale Trading Options. July 2014. Available online: https://www.meti.go.jp/shingikai/enecho/kihon_seisaku/gas_system/pdf/011_03_00.pdf (accessed on 13 October 2022). (In Japanese)
- Tokyo Gas. Completion of the Construction of the Central Trunkline Phase II. Available online: https://www.tokyo-gas.co.jp/Press/20100507-01.html (accessed on 13 October 2022). (In Japanese).
- The Society of Heating, Air-Conditioning and Sanitary Engineers of Japan. Planning, Design and Operation for City Gas Cogeneration; The Society of Heating, Air-Conditioning and Sanitary Engineers of Japan: Tokyo, Japan, 1999. (In Japanese) [Google Scholar]
- The Society of Heating, Air-Conditioning and Sanitary Engineers of Japan. Planning, Design and Operation for City Gas Cogeneration; The Society of Heating, Air-Conditioning and Sanitary Engineers of Japan: Tokyo, Japan, 2015. (In Japanese) [Google Scholar]
- Agency for Natural Resources and Energy. Energy Consumption Statistics by Prefecture. Available online: https://www.enecho.meti.go.jp/statistics/energy_consumption/ec002/results.html#headline2 (accessed on 13 October 2022). (In Japanese)
- National Hotel All Guides; Yama-kei Publishers Co., Ltd.: Tokyo, Japan, 2002. (In Japanese)
- Health Policy Study Group of the Ministry of Health, Labour and Welfare (Ed.) Hospital Handbook; Igaku-Shoin Ltd.: Tokyo, Japan, 2000. (In Japanese) [Google Scholar]
- Large-Scale Retail Stores in Japan; Toyo Keizai Inc.: Tokyo, Japan, 2002; Volume 5723, p. 6. (In Japanese)
- Ministry of Internal Affairs and Communications, Establishment and Enterprise Census. Available online: https://www.e-stat.go.jp/en/stat-search/files?page=1&toukei=00200551&tstat=000001008978&result_page=1 (accessed on 13 October 2022).
- Sumitomo, S.; Akisawa, A.; Ikegami, T.; Nakayama, M. Installation effects of solid oxide fuel cell cogeneration for commercial buildings. J. Jpn. Inst. Energy 2019, 98, 149–156. (In Japanese) [Google Scholar] [CrossRef] [Green Version]
- Tokyo Electric Power Company. Download Past Electricity Demand Data. Available online: https://www.tepco.co.jp/en/forecast/html/download-e.html (accessed on 13 October 2022).
- Tokyo District Meteorological Observatory. Daily Weather Appearance Rate in Tokyo (Statistical Period 1981–2010). Available online: http://www.data.jma.go.jp/tokyo/shosai/bocho/tenki/link.html (accessed on 13 October 2022).
- International Energy Agency. World Energy Outlook 2019. Available online: https://www.iea.org/reports/world-energy-outlook-2019 (accessed on 13 October 2022).
- Ministry of the Environment. Report of the Examination of Feasibility of Dissemination of Distributed Energy Such as Renewable Energy in 2050; Ministry of the Environment: Tokyo, Japan, 2014. (In Japanese)
- Website for Publication of Feed-In Tariff Information- the Official Web Site of Agency for Natural Resources and Energy. Available online: https://www.fit-portal.go.jp/servlet/servlet.FileDownload?file=0150K000006oQgY (accessed on 13 October 2022). (In Japanese)
- BOSCH. How Stationary Fuel Cells Are Revolutionizing the Power Supply. Available online: https://www.bosch.com/stories/sofc-system/ (accessed on 13 October 2022).
- The Japan Gas Association. Development Status and Future Prospects for Residential and Commercial Use of SOFC Systems. Available online: http://www.gas.or.jp/en/newsletter/images/22/dl/pdf01.pdf (accessed on 13 October 2022).
- Tokyo Metropolitan Center for Climate Change Actions. Project to Promote the Formation of Smart Energy Areas Using Hydrogen (Business and Industrial Sector). Available online: https://www.tokyo-co2down.jp/subsidy/hydrogen_smart_biz (accessed on 13 October 2022). (In Japanese).




| Type | Nuclear | Coal | Oil | Gas | GTCC 1 | Hydro | Pumped | |
|---|---|---|---|---|---|---|---|---|
| Unit construction cost [USD/kW] | 3180 | 2470 | 2610 | 1890 | 1490 | 3640 | 2180 | |
| Lifetime [year] | 40 | 40 | 60 | 40 | 40 | 60 | 60 | |
| Annual O&M 2 cost rate [%] | 4.0 | 4.8 | 3.9 | 3.6 | 3.6 | 2.0 | 1.0 | |
| Own consumption rate [%] | 4.0 | 6.0 | 5.0 | 4.0 | 2.0 | 0.5 | 0.5 | |
| Efficiency [%] | 100 | 42 | 39 | 40 | 57 | 100 | 65 | |
| CO2 emission intensity [kg-CO2/kWh] | 0 | 0.8 | 0.61 | 0.45 | 0.31 | 0 | 0 | |
| Maximum increase rate of output [%/hour] | 0 | 26 | 44 | 44 | 44 | 100 | 100 | |
| Maximum decrease rate of output [%/hour] | 0 | 31 | 31 | 31 | 31 | 100 | 100 | |
| Availability [%] | Seasonal peak | 100 | 85.7 | 90.1 | 91.6 | 93.1 | 85 | 85 |
| Summer (weekday) | 93.7 | 79 | 87.1 | 89.1 | 87.2 | 85 | 85 | |
| Summer (holiday) | 94.9 | 80 | 87.6 | 89.3 | 88.4 | 85 | 85 | |
| Winter (weekday) | 93.2 | 84.5 | 78.2 | 80.3 | 80.9 | 62 | 62 | |
| Winter (holiday) | 91.6 | 85.7 | 79.1 | 81.3 | 79.6 | 62 | 62 | |
| Middle (weekday) | 83.3 | 63.3 | 71.5 | 71.3 | 81.1 | 84 | 84 | |
| Middle (holiday) | 82.5 | 61.9 | 71.4 | 70.4 | 81.7 | 84 | 84 | |
| Existing capacity in 2030 [MW] | 10,697 | 5700 | 10,050 | 1000 | 19,129 | 3009 | 10,396 | |
| Type | Transmission Line | Substation | |
|---|---|---|---|
| Overhead | Underground | ||
| Unit construction cost | 1.55 [USD/kVA/km] | 9.09 [USD/kVA/km] | 145 [USD/kVA] |
| Lifetime [year] | 50 | 50 | 50 |
| Type | City Gas Terminal | Pipeline |
|---|---|---|
| Unit construction cost | 140 [USD/kW] | 2.91 [USD/kW/km] |
| Lifetime [year] | 50 | 50 |
| Annual O&M cost rate [%] | 4 | 4 |
| City gas production cost [USD/kWh] | 0.05 | |
| CO2 emission intensity [kg-CO2/kWh] | 0.18 |
| Type | Commercial (SOFC) | Commercial (SGEN) | Residential (SOFC) |
|---|---|---|---|
| Unit construction cost [USD/kW] | 4550 | 4550 | 6450 |
| Lifetime [year] | 15 | 15 | 15 |
| Hot water storage tank [L/kW] | 40 | 20 | |
| Stored hot water temperature [°C] | 60 | 70 | |
| Power generation efficiency [%] | 49.5 | 53 | 46.8 |
| Exhaust heat recover efficiency [%] | 27 | 31.5 | |
| Minimum load factor [%] | 50 | 50 | 50 |
| Type | Electric Heat Pump Air Conditioner | Gas Heat Pump Air Conditioner | Absorption Chiller Heater | Heat Pump Water Heater | Boiler |
|---|---|---|---|---|---|
| Unit construction cost [USD/kW] | 470 | 1340 | 235 | 1270 | 73.6 |
| Lifetime [year] | 15 | 15 | 15 | 15 | 15 |
| Efficiency (heating) | 4.2 | 1.45 | 0.87 | 3.1 | 0.86 |
| Efficiency (cooling) | 5.2 | 2.56 | 1.34 |
| Type | Air Conditioner | Heat Pump Water Heater | Water Heater |
|---|---|---|---|
| Unit construction cost [USD/kW] | 590 | 1360 | 43.6 |
| Lifetime [year] | 15 | 15 | 15 |
| Efficiency (heating) | 4.8 | 4.1 | 0.95 |
| Efficiency (cooling) | 5.3 |
| Type | Li-Ion |
|---|---|
| Unit construction cost [USD/kW] | 1320 |
| Lifetime [year] | 15 |
| Cycle efficiency [%] | 80 |
| Usage rate [%] | 90 |
| Electric storage capacity [kWh/kW] | 6 |
| Commercial Sector | Residential Sector | Industry Sector | ||||
|---|---|---|---|---|---|---|
| Hotel | Hospital | Office | Store | |||
| Electricity demand [TWh/year] | 12.3 | 7.2 | 38.8 | 21 | 54.3 | 110.7 |
| Cooling demand [TWh/year] | 6.8 | 4.2 | 34.9 | 14.7 | 24.3 | |
| Heating demand [TWh/year] | 3.9 | 1.6 | 5.4 | 4 | 60.2 | |
| Hot water demand [TWh/year] | 7.7 | 2.5 | 0 | 0 | 89.8 | |
| Weather [Days] | Sunny | Cloudy | Rainy |
|---|---|---|---|
| Seasonal peak | 3 | 0 | 0 |
| Summer (weekday) | 32 | 22 | 27 |
| Summer (holiday) | 15 | 10 | 13 |
| Winter (weekday) | 54 | 8 | 19 |
| Winter (holiday) | 27 | 4 | 9 |
| Middle (weekday) | 44 | 15 | 25 |
| Middle (holiday) | 20 | 7 | 11 |
| Type | Cost |
|---|---|
| Crude oil [USD/barrel] | 88 |
| Natural gas [USD/MMBtu] | 9.7 |
| Steam coal [USD/t] | 86 |
| Nuclear [USD/MWh] | 18 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Owaku, T.; Yamamoto, H.; Akisawa, A. Optimal SOFC-CHP Installation Planning and Operation Model Considering Geographic Characteristics of Energy Supply Infrastructure. Energies 2023, 16, 2236. https://doi.org/10.3390/en16052236
Owaku T, Yamamoto H, Akisawa A. Optimal SOFC-CHP Installation Planning and Operation Model Considering Geographic Characteristics of Energy Supply Infrastructure. Energies. 2023; 16(5):2236. https://doi.org/10.3390/en16052236
Chicago/Turabian StyleOwaku, Takashi, Hiromi Yamamoto, and Atsushi Akisawa. 2023. "Optimal SOFC-CHP Installation Planning and Operation Model Considering Geographic Characteristics of Energy Supply Infrastructure" Energies 16, no. 5: 2236. https://doi.org/10.3390/en16052236
APA StyleOwaku, T., Yamamoto, H., & Akisawa, A. (2023). Optimal SOFC-CHP Installation Planning and Operation Model Considering Geographic Characteristics of Energy Supply Infrastructure. Energies, 16(5), 2236. https://doi.org/10.3390/en16052236







