Heat Transfer in Cavities: Configurative Systematic Review
Abstract
1. Introduction
Aim of the Study
- Model geometry, flow domain, type, CFD modeling, parameters, and meshing.
- Different types of validations are used by researchers.
- Heat transfer behavior for different flow conditions, geometries, boundary conditions, and the presence of different obstacles inside cavities.
2. Methodology
3. Physical Domain and Mathematical Modelling
3.1. Physical Domain
3.2. Governing Equations
3.3. Meshing
| (a) | |||||
| Ref. | CFD Methods and Algorithms | Flow Domain | Dimension, Type of Flow | Parameters and Ranges | Meshing |
| Wee et al. [2] | Experimental, FDM, DADI | Rectangular cavity | 2D, unsteady, laminar | 2 105 ≤ GrT ≤ 2 106, 10 ≤ GrC ≤ 2 105, 2 104 ≤ Ra ≤ 1.47 106 | - |
| Moallemi & Jjang [11] | FVM, SIMPLIER | Lid-driven square cavity | 2D, steady, laminar, non-Newtonian | 102 ≤ Re ≤ 2200, 0.01 ≤ Pr ≤ 50, 0.01 ≤ Ri ≤ 10 | Non-uniform grid, 42 42 |
| Sasaguchi et al. [14] | Grid generation | Rectangular cavity with cylinders | 2D, unsteady, laminar | N/A | Uniform mesh, 31 101, 31 121 |
| Al-Amiri et al. [15] | FEM | Lid-driven cavity with wavy wall | 2D, steady, laminar, mixed convection | Pr = 0.71, 1, Gr = 104, 0.1 ≤ Ri ≤ 10, 0 ≤ Am ≤ 0.075, 0 ≤ ≤ 3, Re = 500 | - |
| Saha et al. [16] | FEM | Inclined rectangular enclosure | 2D, natural convection, steady, laminar | Pr = 0.71, 103 ≤ Gr ≤ 106, 0.5 ≤ A ≤ 1.0, 0 ≤ ≤ 30 | Non-uniform, six nodded 6394 elements |
| Saha et al. [17] | FVM | Triangular cavity | 2D, natural convection | Pr = 0.71, Gr = 1.33 106, 0.5 ≤ A ≤ 1.0 | 360 90, 720 160, 270 90 for A = 0.2, 0.5, 1.0 |
| Tiwari & Das [18] | FVM, SIMPLE, QUICK, TDMA | Double lid-driven square cavity | 2D, unsteady | Pr = 6.2, 0.1 ≤ Ri ≤ 10, 103 ≤ Ra ≤ 106, 0 ≤ ≤ 0.2, Cu-water nanofluid | Uniform grid, 61 61 |
| Saha et al. [19] | FEM | Inclined sinusoidal enclosure | 2D, steady, natural convection, laminar | Pr = 0.71, 103 ≤ Gr ≤ 106, 0 ≤ ≤ 45 | Non-uniform grid, 5240 elements |
| Varol et al. [20] | FDM | Triangular cavity | 2D, natural convection | 0.25 ≤ A ≤ 1.0, 102 ≤ Ra ≤ 103 | Uniform grid, 61 61 |
| Chen & Cheng [21] | FVM, SOR | Lid-driven triangular cavity | 2D, mixed convection, unsteady, laminar | Pr = 0.71, Re = 100, Gr = 5 105 | 41 41 |
| Noor et al. [22] | FDM, QUICK, RK-4, SOLA | Double lid-driven square cavity | 2D, unsteady, laminar | Pr = 0.71, 10 ≤ Re ≤ 103, 1 ≤ ≤ 5 | Clustered grid, 125 125 |
| Ouertatani et al. [23] | FVM, QUICK. CDS, RBSOR | Double lid-driven cubic cavity | 3D, mixed convection, unsteady, laminar | Pr = 0.71, 102 ≤ Re ≤ 103, 10−3 Ri ≤ 10 | 64 64 64 |
| Basak et al. [24] | FEM | Triangular porous cavities | 2D, natural convection, steady | 10−5 ≤ Da ≤ 10−1, 0.015 ≤ Pr ≤ 103, 103 ≤ Ra ≤ 5105 | 20 20–28 28 bi-quadratic elements |
| Lei & O’Neill [25] | FVM, SIMPLE, CDS, SUR | Square cavity with different corners | 2D, unsteady, natural convection | Pr = 6.62, 0 ≤ Ra ≤ 108 | 16,000 cells |
| Saha [26] | FEM | Sinusoidal corrugated enclosure | 2D, steady | Pr = 0.71, 103 ≤ Gr ≤ 106, 0 ≤ Ha ≤ 102 | Non-uniform grid, 4928 elements |
| Saha et al. [27] | FEM | Octagonal enclosure | 2D, natural convection, steady, laminar | Pr = 0.71, 7, 20, 50, 103 ≤ Ra ≤ 106 | Non-uniform, 10,466 elements |
| Saha et al. [28] | FVM, QUICK, SIMPLE | Triangular enclosure | 2D, natural convection, unsteady | Pr = 0.72, 104 ≤ Ra ≤ 7.2 106, A = 0.2, 0.5, 1.0 | 270 90, 320 80, 360 90 for A = 1, 0.5 & 0.2 |
| Saha et al. [29] | FVM, QUICK, SIMPLE | Triangular enclosure | 2D, natural convection, unsteady | Pr = 0.72, 7.2 103 ≤ Ra ≤ 1.5 106, A = 0.2, 0.5, 1.0 | 270 90, 320 80, 360 90 for A = 1, 0.5 & 0.2 |
| Saha et al. [30] | FVM, QUICK, SIMPLE | Triangular enclosure | 2D, natural convection, unsteady | Pr = 0.72, 5 ≤ Ra ≤ 1.5 107, A = 0.2, 0.5, 1.0 | 360 120, 320 90, 360 90 for A = 1, 0.5 & 0.2 |
| Sivasankaran et al. [31] | FVM, UDS, CDS, SOR | Lid-driven square cavity | 2D, unsteady, laminar, mixed convection | Pr = 0.71, 0 ≤ Am ≤ 1, 0 ≤ ≤ , 0.1 ≤ Ri ≤ 102, 102 ≤ Re ≤ 103 | Uniform grid, 81 81 |
| Sivakumar et al. [32] | FVM, SIMPLE, QUICK. CDS | Lid-driven square cavity | 2D, mixed convection, unsteady, laminar | Pr = 0.71, 102 ≤ Re ≤ 103, 102 ≤ Gr ≤ 106, 10−2 ≤ Ri ≤ 102 | Uniform grid, 81 81 |
| Al-Amiri & Khanafer [33] | ADINA, FSI, FEM, ALE | Lid-driven square cavity | 2D, steady, laminar | Pr = 0.71, 102 ≤ Re, Gr ≤ 103 | Non-uniform mesh, 120 120 |
| Cheng [34] | Compact formulation, SOR, ADI | Lid-driven square cavity | 2D, mixed convection | 10 ≤ Re ≤ 2200, 100 ≤ Gr ≤ 4.86 106, 10−2 ≤ Pr ≤ 50, 10−2 ≤ Ri ≤ 102 | 256 256 |
| Nasrin & Parvin [35] | FEM, TFM | Lid-driven square cavity with wavy wall | 2D, laminar, steady, mixed convection | 30 ≤ Re ≤ 300, 0 ≤ Ha ≤ 50, Pr = 0.71, Ra = 104 | Non-uniform, 37,123 nodes, 5604 elements |
| Saha [36] | FVM, QUICK | Triangular cavity | 2D, unsteady, natural convection | 0.2 ≤ A ≤ 1.0, 5 ≤ Pr ≤ 100, Ra = 107 | - |
| Saha [37] | FVM, SIMPLE, QUICK | Triangular cavity | 2D, unsteady, natural convection | 0.2 ≤ A ≤ 1.0, 5 ≤ Pr ≤ 100, 5 106 ≤ Ra ≤ 108 | - |
| Yu et al. [38] | Fluent, FVM, QUICK, SIMPLE | Square cavity | 2D, unsteady, natural convection | 104 ≤ Ra ≤ 106, 0 ≤ ≤ 0.04, CuO–H2O nanofluid | 100 100 |
| Al-Farhany & Turan [39] | FVM, SIMPLER | Inclined rectangular porous cavity | 2D, unsteady, natural convection | 0 ≤ ϕ ≤ 85, 0.1 ≤ Le ≤ 10, −5 ≤ N ≤ 5, Pr = 4.5, Ra = 5 × 106 | - |
| Arani et al. [40] | FVM, SIMPLIER, TDMA, CDS, UDS | Lid-driven square cavity | 2D, mixed convection, laminar, steady | 10−3 ≤ Ri ≤ 10, 0 ≤ ≤ 0.1, Gr = 102, Re = 102 Cu–H2O nanofluid | Uniform grid. 80 80 |
| Basak et al. [41] | FEM | Porous triangular cavities | 2D, natural convection, steady | Pr = 0.025, 1000, 10−5 ≤ Da ≤ 10−3, Ra = 5 105 | 28 28 bi-quadratic elements |
| Chamkha & Abu-Nada [42] | FVM, CDS, UDS, SOR, SUR | Double lid-driven square cavity | 2D, mixed convection, steady, laminar | 0.001 ≤ Ri ≤ 10, 0 ≤ ≤ 0.1, H2O-Al2O3 nanofluid | Uniform grid, 81 81 |
| Nasrin & Parvin [43] | FEM | Trapezoidal cavity | 2D, free convection, steady, laminar | Ra = 105, = 0.05, 0.65 ≤ A ≤ 2, 1.47 ≤ Pr ≤ 8.81, Cu–H2O nanofluid | 40,295 nodes, 10,936 elements |
| Raji et al. [44] | FVM, SIMPLIER | Square cavity with blocks | 2D, natural convection, laminar, steady | 103 ≤ Ra ≤ 108, Pr = 0.71 | 254 254 |
| Saha & Gu [45] | FVM | Triangular enclosure | 2D, natural convection, unsteady | Pr = 0.7, Ra = 105, A = 0.5 | Triangular grid, 8143 nodes |
| Saha & Gu [46] | FVM | Triangular enclosure | 2D, natural convection, unsteady | Pr = 0.72, 5.0 104 ≤ Ra ≤ 106, A = 0.5 | - |
| Teamah & El-Maghlany [47] | FVM, CDS, GS, TDMA | Square cavity | 2D, steady, laminar | 103 ≤ Ra ≤ 107, 0 ≤ Ha ≤ 60, 0 ≤ ≤ 0.06, −10 ≤ q ≤ 10, Pr = 6.2, H2O based Al2O, Cu, TiO2 nanofluids | 60 60 |
| Ahmed et al. [48] | Collocated FVM, UDS, CDS, TDMA | Inclined lid-driven square cavity | 2D, mixed convection, laminar, steady | 10−2 ≤ Ri ≤ 102, 0 ≤ ≤ 1.0, 0 ≤ Ha ≤ 100, 0 ≤ ≤ 90 | Uniform grid. 81 81 |
| Bhardwaj & Dalal [49] | FDM, QUICK, SOR, CDS | Porous triangular cavity | 2D, natural convection, laminar | 103 ≤ Ra ≤ 106, 10−4 ≤ Da ≤ 10−2, Am = 0.05 | Orthogonal mesh, 41 41 |
| Cho et al. [50] | FVM, SIMPLEC, TDMA | Lid-driven cavity with wavy surfaces | 2D, mixed convection, laminar, steady | 0 ≤ ≤ 0.1, 10−2 ≤ Ri ≤ 103, 101 ≤ Gr ≤ 104, 0 ≤ ≤ 0.2, Pr = 6.2, H2O based Cu, Al2O3, TiO2 nanofluids | 101 201 |
| Elsherbiny & Ragab [51] | - | Inclined rectangular cavities | 2D, laminar, natural convection | 102 ≤ Ra ≤ 106, 0.5 ≤ A ≤ 5, 0 ≤ ≤ 180 | Uniform mesh, 42 42 |
| Huelsz & Rechtman [52] | LBM | Inclined square cavity | 2D, natural convection, laminar, unsteady | Pr = 0.71, −180 ≤ ≤ 180, 103 ≤ Ra ≤ 106 | 103 103 |
| Khanafer & Aithal [53] | ADINA, FEM, NR | Lid-driven square cavity with cylinder | 2D, steady, laminar, mixed convection | Pr = 0.7, 104 ≤ Gr ≤ 106, 0.01 ≤ Ri ≤ 10, Re = 102 | Non-uniform grid, 19,520 nodes |
| Ramakrishna et al. [54] | FEM | Trapezoidal cavities | 2D, free convection, laminar | 30 ≤ ≤ 90, Pr = 0.015, 7.2, 103 ≤ Ra ≤ 105 | 28 28 bi-quadratic elements |
| Sivasankaran et al. [55] | FVM, UDS, CDS | Inclined lid-driven square cavity | 2D, unsteady, laminar, mixed convection | Pr = 0.71, 0.01 ≤ Ri ≤ 102, 0 ≤ ≤ 90 | 81 81 |
| (b) | |||||
| Ref. | CFD Methods and Algorithms | Flow Domain | Dimension, Type of Flow | Parameters and Ranges | Meshing |
| Sathitamoorthy & Chamkha [56] | FEM | Square cavity with thin partition | 2D, natural convection, steady | Pr = 0.7, 100, 103 ≤ Rah ≤ 105 | 20 20 elements, 41 41 grid points |
| Abu-Nada & Chamkha [57] | FVM, SOR, SUR, UDS | Lid-driven square cavity with wavy wall | 2D, mixed convection, laminar, steady | Pr = 6.57, 0.01 ≤ Ri ≤ 102, 0 ≤ ≤ 0.09, H2O-CuO nanofluid | Uniform grid, 81 81 |
| Ait-Taleb et al. [58] | FDM, SIMPLE, TDMA | Rectangular cavities with tiles | 2D, steady, laminar | 8.7 103 ≤ Rah ≤ 1.8 105, 4.1 105 ≤ RaH ≤ 1.23 107, Pr = 0.71 | Non-uniform mesh, 80 40 |
| Cheng & Liu [59] | Ansys Fluent, FVM, SOR, ADI | Lid-driven square and rectangular cavities | 2D, unsteady | Pr = 0.71, 10−2 ≤ Ri ≤ 102, 0.2 ≤ A ≤ 5, 0 ≤ ≤ 90, Re = 102 | Uniform grid, 640 128, 128 128, 128 640 |
| Cho [60] | FVM, SIMPLE, TDMA | Square cavity with wavy wall | 2D, natural convection, laminar, steady | 103 ≤ Ra ≤ 106, 0 ≤ ≤ 0.04, Pr = 6.2, H2O-Al2O3 nanofluid | 101 201 |
| Kalteh et al. [61] | FDM, CDS, SUR | Lid-driven square cavity with block | 2D, steady, mixed convection | Gr = 102, 0.000625 ≤ Ri ≤ 4, 0 ≤ ≤ 0.05, H2O based Al2O3, TiO2, Ag, CuO nanofluids | Regular grid, 80 80 |
| Khanafer [62] | ADINA, FEM, FSI, ALE | Lid-driven square cavity | 2D, steady, laminar, mixed convection | Pr = 0.7, 102 ≤ Gr ≤ 104, 10−4 ≤ Ri ≤ 1.0, 102 ≤ Re ≤ 103 | Non-uniform, 120 120 |
| Muthtamilselvan & Doh [63] | FVM, SIMPLE, QUICK, TDMA | Lid-driven square cavity | 2D, steady, mixed convection | 0 ≤ Rai ≤ 105, Pr = 6.2, 10−2 ≤ Ri ≤ 10, 0 ≤ ≤ 0.06, Cu-H2O nanofluid | Uniform grid, 41 41 |
| Ramakrishna et al. [64] | FEM | Porous trapezoidal cavity | 2D, natural convection, laminar, steady | 10−5 ≤ Da ≤ 10−3, 30 ≤ ≤ 90, Pr = 0.015, 103 | 28 28 bi-quadratic elements |
| Ray & Chatterjee [65] | Fluent, FVM | Lid-driven square cavity | 2D, steady, mixed convection | 0.1 ≤ Ri ≤ 10, 0 ≤ Ha ≤ 50, 0 ≤ J ≤ 5, Re = 102, Pr = 0.71 | Non-uniform, 56,126 mixed elements |
| Saha & Gu [66] | Fluent, FVM | Triangular cavity | 2D, unsteady, natural convection | Pr = 0.72, 105 ≤ Ra ≤ 108, A = 0.2, 0.5, 1.0 | Non-uniform grid, 400 100 |
| Xu & Saha [67] | FVM, SIMPLE, QUICK | Square cavity with fin | 2D, unsteady, natural convection | Pr = 0.71, 105 ≤ Ra ≤ 109 | Non-uniform grid, 198 198 |
| Cui et al. [68] | Fluent, FVM, SIMPLE, QUICK | Triangular cavity | 3D, natural convection, unsteady | A = 0.5, Pr = 6.98, Ra = 2.08 106 | Non-uniform grid, 100 66 45 |
| Elsherbiny & Ismail [69] | FDM, TDMA, CDS | Inclined rectangular cavities | 2D, laminar, steady | Pr = 0.71, 0 ≤ ≤ 180, 103 ≤ Ra ≤ 106, A = 1, 5, 10 | Uniform grid, 42 42 |
| Elsherbiny & Ragab [51] | - | Inclined rectangular cavities | 2D, laminar, natural convection | Pr = 0.71, 0 ≤ ≤ 180, 102 ≤ Ra ≤ 106 | Uniform mesh, 42 42 |
| Groşan et al. [70] | FDM, CDS | Porous square cavity | 2D, steady, natural convection | Ra = 10, 100, Le = 1, 10, H2O, aluminum foam, carbon nanotubes | 227 227 |
| Moumni et al. [71] | FVM, AB, QUICK, CDS, Euler, RBSOR | Double lid-driven square cavity | 2D, unsteady, laminar | 1 ≤ Re ≤ 102, Pr = 6.2, 10−2 ≤ Ri ≤ 20, 0 ≤ ≤ 0.2, H2O based Cu, Ag, Al2O3, TiO2 nanofluids | Uniform grid, 120 120 |
| Saha & Gu [72] | Fluent, FVM, QUICK | Triangular enclosure | 2D, natural convection, steady, unsteady | 105 ≤ Ra ≤ 106, Pr = 0.72, A = 0.2, 0.5, 1.0 | Non-uniform grid |
| Sheremet & Pop [73] | FDM, CDS, SOR | Lid-driven square cavity | 2D, unsteady, laminar, mixed convection | Re = 102, Pr = 6.26, 10 ≤ Gr ≤ 105, 10 ≤ Le ≤ 104, N, Nb, Nt = 0.1 to 0.4 | Uniform grid, 400 400 |
| Sojoudi et al. [74] | Fluent, FVM | Triangular cavity | 2D, natural convection, unsteady | 103 ≤ Ra ≤ 106, 0.2 ≤ A ≤ 1, Pr = 0.72 | 15,700 triangular elements |
| Armaghani et al. [75] | FVM, SIMPLE | L-shaped cavity with baffle | 2D, steady, natural convection | Pr = 0.71, 104 ≤ Ra ≤ 106, 0.1 ≤ A ≤ 0.7, 0 ≤ Bf ≤ 0.3, 0 ≤ ≤ 0.04, Al2O3-H2O nanofluid | Uniform grid, 100 100 |
| Jmai et al. [76] | FVM, QUICK, SOR, multigrid | Double lid-driven square cavity | 2D, unsteady, laminar, mixed convection | 10−2 ≤ Ri ≤ 102, 0 ≤ ≤ 0.1, Cu–H2O nanofluid | Non-uniform grid, 64 64 |
| Kareem et al. [77] | Fluent, URANS, LES, FVM, SIMPLE | Cubic lid-driven cavity | 3D, unsteady, mixed convection, turbulent | Re = 5000, 10,000, 15,000 and 30,000 | Structured, non-uniform, 1,699,200 grids |
| Kareem et al. [78] | FVM, UDS, SIMPLE | Lid-driven trapezoidal cavity | 2D, mixed convection | 0 ≤ ≤ 0.04, 0.1 ≤ Ri ≤ 10, 102 ≤ Re ≤ 1200, 0 ≤ ≤ 60, 0.5 ≤ A ≤ 2, H2O based Al2O3, CuO, SiO2, TiO2 nanofluids | Unstructured, non-uniform 5470 grids |
| Mamourian et al. [79] | FVM, SIMPLE | Lid-driven square cavity | 2D, steady, laminar, Mixed convection | 10−2 ≤ Ri ≤ 102, 0 ≤ ≤ 0.1, Gr = 102, Am = 0.25, Cu–H2O nanofluid | Structured non-uniform, 101 201 |
| Mejri et al. [80] | LBM | Triangular cavity | 2D, natural convection, laminar | 103 ≤ Ra ≤ 106, 0 ≤ ≤ 315, Ma = 0.1 | - |
| Rahmati et al. [81] | LBM | Double lid-driven cavity | 2D, mixed convection, laminar, steady | Gr = 100, Pr = 6.57, 10−2 ≤ Ri ≤ 102, 0 ≤ ≤ 0.06, Cu-H2O nanofluid | 100 100 |
| Rashad et al. [12] | FVM, ADI, SIMPLE, UDS, CDS | Lid-driven square cavity | 2D, mixed convection, laminar | 0 ≤ Ha ≤ 100, 10−3 ≤ Ri ≤ 10, 0 ≤ ≤ 0.01, Cu-H2O nanofluid | Uniform grid, 81 81 |
| Selimefendigil & Öztop [82] | FEM, ALE | Lid-driven inclined square cavity | 2D, steady | 10−2 ≤ Ri ≤ 102, 0 ≤ ≤ 0.05, 103 ≤ Rai ≤ 106, 0 ≤ Ha ≤ 50, 0 ≤ ≤ 90, 5 102 ≤ E ≤ 106, CuO-H2O nanofluid | Non-uniform mesh, 10,916 nodes |
| Selimefendigil & Öztop [83] | FEM, FSI, ALE | Triangular cavity | 2D, natural convection, steady | 104 ≤ Ra ≤ 106, 104 ≤ Rai ≤ 107, 0 ≤ Ha ≤ 40, 0 ≤ ≤ 90, Pr = 7.1, 5 102 ≤ E ≤ 105 | - |
| Selimefendigil et al. [84] | FEM, NR | Lid-driven square cavity | 2D, Mixed convection | 10−2 ≤ Ri ≤ 102, 0 ≤ Ha ≤ 50, 0 ≤ ≤ 90, 0 ≤ ≤ 0.05, CuO-H2O nanofluid | Non-uniform,. 17,408 elements |
| Sojoudi et al. [85] | FVM, QUICK, SIMPLE | Triangular cavity | 2D, unsteady, natural convection | 103 ≤ Ra ≤ 106, 0.2 ≤ A ≤ 1.0, Pr = 0.72 | 15,600 triangular elements |
| Sojoudi et al. [86] | FVM, QUICK, SIMPLE | Triangular cavity | 2D, unsteady, natural convection | 103 ≤ Ra ≤ 106, 0.2 ≤ A ≤ 1.0, Pr = 0.71 | 360 75, 360 90, 360 120 for A = 0.2, 0.5, 1 |
| Aparna & Seetharamu [87] | FEM | Porous trapezoidal cavity | 2D, natural convection | 100 ≤ Ra ≤ 2000 | 41 41 |
| Alsabery et al. [88] | COMSOL, FEM | Trapezoidal cavity | 2D, unsteady, non-Newtonian, laminar | 104 ≤ Ra ≤ 106, 0 ≤ ≤ 0.2, 0.1 ≤ Pr ≤ 103, 0 ≤ ≤ 21.8, H2O based TiO2, Cu, Al2O3, Ag nanofluids | Non-uniform, 4690 elements |
| Al-Weheibi et al. [89] | COMSOL, FEM | Trapezoidal enclosure | 2D, natural convection, unsteady, laminar | 103 ≤ Ra ≤ 106, 0 ≤ ≤ 0.1, 0.25 ≤ A ≤ 1.0 | 6-noded 403,388 elements, 204,436 grids |
| Balla et al. [90] | FEM | Inclined porous square cavity | 2D, free convection, laminar | 0.01 ≤ ≤ 0.3, 101 ≤ Ra ≤ 103, 0 ≤ M ≤ 10, 0 ≤ ≤ 90, H2O based TiO2, Cu, Al2O3, SiO2 nanofluids | 6-noded triangular grid, 5000 elements, 2601 nodes |
| Cui et al. [91] | FVM | Prismatic enclosure | 3D, unsteady, natural convection | 100 ≤ Ra ≤ 106, Pr = 0.71 | Non-uniform grid, 100 66 45 |
| Das et al. [92] | FEM | Square & triangular cavities | 2D, steady, laminar | Pr = 0.7, 103 ≤ Ra ≤ 105 | 34 34 biquadratic elements |
| Gangawane [93] | Fluent, FVM, SIMPLE, QUICK | Lid-driven cavity with triangular block | 2D, steady, laminar, mixed convection | 1 ≤ Re ≤ 103, 0 ≤ Gr ≤ 105, Pr = 1, 50, 102, b = 10%, 20%, 30% | Unstructured, non-uniform, 26,318 nodes, 9126 elements |
| Ghalambaz et al. [94] | FEM, FSI, ALE | Square cavity with oscillating elastic fin | 2D, natural convection, laminar, unsteady | Pr = 0.7, 104 ≤ Ra ≤ 107, 10−3 ≤ Am ≤ 0.1, 1 ≤ Tr ≤ 103, 108 ≤ E ≤ 1013 | 27,131 domain elements, 1022 boundary elements |
| Gibanov et al. [95] | FDM | Lid-driven square cavity with block | 2D, steady, laminar, mixed convection | 10−2 ≤ Ri ≤ 10, 0 ≤ ≤ 0.05, Re = 102, Pr = 6.82, Al2O3-H2O nanofluid | Uniform grid, 200 200 |
| Gibanov et al. [96] | FDM | Lid-driven inclined square cavity | 2D, unsteady, mixed convection | Ri =1, Re = 102, Pr = 6.26, Da = 10−7, 10−3, 0 ≤ Ha ≤ 102, 0 ≤ ≤ 0.05, 0 ≤ ≤ , ferrofluid | Uniform grid, 200 200 |
| Hammami et al. [97] | FVM, QUICK, RBSOR | Double lid-driven cubic cavity with cylinder | 3D, unsteady | 102 ≤ Re ≤ 1500, Pr | Staggered grid non-uniform, 80 80 80 |
| Hatami [6] | FEM, FlexPDE | Rectangular cavity with heated fins | 2D, steady | 0.03 ≤ ≤ 0.09, H2O based TiO2, Al2O3 nanofluids | - |
| Hatami et al. [98] | FEM, RSM, FlexPDE | Lid-driven T-shaped porous cavity | 2D, steady, mixed convection | Ri = 0.1, Re = 50, Da = 0.001, H2O based TiO2, Cu, Al2O3 nanofluids | - |
| Hussain et al. [99] | FEM, GE, CN | Double lid-driven square cavity | 2D, steady, mixed convection | 1 ≤ Re102, 0 ≤ ≤ 45, 0.01 ≤ Ri ≤ 10, 0 ≤ ≤ 0.04, Al2O3-H2O nanofluid | Uniform grid, 65,536 elements |
| Javed et al. [100] | FEM | Trapezoidal porous cavities | 2D, free convection, steady | Pr = 6.2, 10−5 ≤ Da ≤ 10−3, 105 ≤ Ra ≤ 107, 0 ≤ Ha ≤ 60, 0 ≤ ≤ 0.03, Cu-H2O nanofluid | 6 noded 704 elements, 1000 grids |
| Kareem & Gao [101] | Fluent, LES, URANS, SIMPLEC, QUICK | Lid-driven cubic cavity with cylinder | 3D, unsteady, mixed convection, turbulent | Pr, Re = 5000, 10,000, 15,000, 30,000, 0 ≤ ≤ 10 | Structured, non-uniform cells, 929,160 elements |
| Khanafer & Aithal [102] | ADINA, FEM | Lid-driven square cavity with cylinder | 2D, steady, laminar, mixed convection | 0.01 ≤ Ri ≤ 10, Re = 100, −10 ≤ ≤ 10 | 150 150 |
| Khatamifar et al. [103] | DNS, SIMPLE, QUICK | Rectangular cavity with partition | 2D, natural convection, unsteady | 105 ≤ Ra ≤ 109, Pr = 0.71, 0.05 ≤ Tp ≤ 0.2 | Non-uniform mesh, 300 250 |
| Mojumder et al. [104] | FEM | Lid-driven L-shaped cavity | 2D, mixed convection, laminar, steady | 1 ≤ Re ≤ 100, 103 ≤ Gr ≤ 105, 10−5 ≤ Da ≤ 10−3, Pr = 6.2 | Triangular mesh, 4452 elements |
| Selimefendigil et al. [105] | FEM, ALE, FSI | Lid-driven square cavity | 2D, steady | 0.01 ≤ Ri ≤ 5, 0 ≤ Ha ≤ 50, 102 ≤ Re ≤ 103, CuO-H2O nanofluid | Triangular elements, 17,224 grids |
| Selimefendigil et al. [106] | FEM, ALE, NR | Lid-driven Trapezoidal cavity with cylinder | 3D, steady, laminar, mixed convection | 0.5 ≤ Ri ≤ 50, 0 ≤ ≤ 20, 0 ≤ ≤ 0.04, 103 ≤ E ≤ 105, CuO-H2O nanofluid | Tetrahedral elements |
| Selimefendigil & Öztop [107] | FEM, FSI, ALE | Lid-driven triangular cavity | 2D, mixed convection, steady | 0.05 ≤ Ri ≤ 50, 0 ≤ ≤ 0.04, Pr = 6.8, 104 ≤ RaI ≤ 108, 500 ≤ E ≤ 105, H2O–CuO nanofluid | Non-uniform grid, 26,306 elements |
| Sheremet et al. [108] | FDM | Triangular cavities | 2D, natural convection | Pr = 0.7, 104 ≤ Ra ≤ 2 105, 102 ≤ Le ≤ 104, 0 ≤ N ≤ 5, Nb = Nt = 0.1 | Uniform grid, 300 150 |
| Yu et al. [3] | FDM, UCS, TVD, RK | Rectangular cavity | 2D, unsteady, laminar | Pr = 0.025, A = 2, 0 ≤ Ha ≤ 50, 0 ≤ Le ≤ 20 | Uniform grid, 41 81 |
| Aghakhani et al. [109] | FDLBM | C-shaped cavity | 2D, laminar, non-Newtonian | 103 ≤ Ra ≤ 105, 0 ≤ Ha ≤ 40, 0.2 ≤ A ≤ 0.6 | 160 160 |
| Astanina et al. [110] | FDM | Lid-driven square porous cavity | 2D, steady, mixed convection | 0.01 ≤ Ri ≤ 10, 0 ≤ ≤ 0.04, 10−7 ≤ Da ≤ 10−3, Da2 = 10−5, Re = 102, Pr = 6.82, H2O–Al2O3 nanofluid | Uniform grid, 200 200 |
| Alsabery et al. [111] | FEM, FEA, NR | Double lid-driven square cavity with block | 2D, steady, laminar, mixed convection | 1 ≤ Re ≤ 500, 10−2 ≤ Ri ≤ 102, 0 ≤ ≤ 0.04, Pr = 4.623, Le = 3.5 105, Sc = 3.55 104, H2O–Al2O3 nanofluid | Uniform triangular grid, 12,232 grids |
| Balootaki et al. [112] | LBM-BGK | Lid-driven rectangular cavity with block | 2D, mixed convection | 10−2 ≤ Ri ≤ 50, Re = 100, Pr = 0.7 | 450 150 |
| Bhowmick et al. [113] | FEM, SIMPLE, QUICK | V-shaped cavity | 2D, natural convection | 2.26 105 ≤ Ra ≤ 2.26 109, 0.1 ≤ A ≤ 1.0, Pr = 6.63 | Non-uniform, 800 150 |
| Cho [114] | FVM, TDMA, SIMPLE | Lid-driven cavity with wavy surface | 2D, steady, laminar, mixed convection | 10−2 ≤ Ri ≤ 102, 0 ≤ ≤ 0.04, 1 ≤ Re ≤ 200, Pr = 6.2, 0 ≤ Am ≤ 0.6, H2O–Cu nanofluid | 101 1001 |
| Gangawane et al. [115] | Fluent, FVM, SIMPLE, QUICK | Lid-driven square cavity with block | 2D, steady, laminar, mixed convection | Ri= 0.01, 1, 10, Pr= 1, 1 ≤ Re ≤ 103 | Unstructured, 17,874 nodes, 17,598 elements |
| Gibanov et al. [116] | FDM | Lid-driven square cavity | 2D, mixed convection, laminar, unsteady | 10−2 ≤ Ri ≤ 10, 0 ≤ ≤ 0.05, 1.0 ≤ K ≤ 20.0, Re = 100, Pr = 6.82, H2O–Al2O3 nanofluid | Uniform grid, 100 100 |
| Hussain et al. [117] | FEM, CN, GE | Double lid-driven square cavity | 2D, laminar, unsteady, mixed convection | 0 ≤ ≤ 0.04, 10−2 ≤ Ri ≤ 10, 0 ≤ Ha ≤ 102, 0 ≤ ≤ 90, H2O–Al2O3 nanofluid | Non-uniform triangular grid, 65,536 elements |
| Javed & Siddiqui [118] | FEM | Square cavity with square blockage | 2D, free convection, steady, laminar | 105 ≤ Ra ≤ 107, Pr = 0.062, 0 ≤ ≤ 0.06, 0 ≤ Ha ≤ 60, H2O–Cobalt ferrofluid | Non-uniform, 6-noded 1776 elements |
| Karbasifar et al. [119] | SIMPLEC | Lid-driven cubic cavity with cylinder | 3D, laminar, steady, mixed convection | 10−2 ≤ Ri ≤ 102, = 0, 0.1%, 0.2%, = 0, 15, 45, H2O–Al2O3 nanofluid | - |
| Kareem & Gao [120] | Fluent, URANS, SIMPLEC, QUICK | Lid-driven Cubical cavity with cylinder | 3D, mixed convection, unsteady, turbulent | 5000 ≤ Re ≤ 104, −5 ≤ Ω ≤ 5, SiO2-H2O nanofluid | 929,160 grids |
| Mikhailenko et al. [121] | FDM, CDS, Thomas, SOR | Rotating square cavity with obstacle | 2D, laminar, unsteady | Pr = 0.7, Ra = 105, 0 ≤ ≤ 180, 0 ≤ Ta ≤ 106, 0.1 ≤ Os ≤ 1.0, 0.0 ≤ ε ≤ 0.9 | 101 101 |
| Oglakkaya & Bozkaya [122] | DRBEM | Lid-driven square cavity | 2D, mixed convection, unsteady, laminar | Pr = 0.71, Re = 102, Am = 0.05, Ha = 0, 25, 50, 0 ≤ ≤ 90, J = 0, 1, 3, 5, 103 ≤ Ra ≤ 105 | 400 grids |
| Razera et al. [123] | Fluent, FVM, SIMPLEC | Lid-driven square cavity | 2D, steady, laminar, mixed convection | 101 ≤ Re ≤ 103, 103 ≤ Ra ≤ 106, Pr = 0.71 | Non-uniform, 33,248 volumes |
| Selimefendigil & Öztop [124] | FEM, COBYLA | Lid-driven trapezoidal cavity | 2D, mixed convection | 0.01 ≤ Ri ≤ 25, 0 ≤ Ha ≤ 40, 0 ≤ ≤ 90, 0 ≤ ≤ 0.03, H2O–Al2O3 nanofluid | 6282, 4685 and 3691 elements for different |
| Sheikholeslami [125] | LBM | Lid-driven square cavity with hot sphere | 3D, forced convection | 10−3 ≤ Da ≤ 102, 30 ≤ Re ≤ 180, 0 ≤ Ha ≤ 40, H2O–Al2O3 nanofluid | 81 81 81 |
| Sheremet et al. [126] | FDM | Porous square vented cavity | 2D, mixed convection, unsteady | 104 ≤ Ra ≤ 106, Nb = Nt = 10−6, 10−5 ≤ Da ≤ 10−2, 50 ≤ Re ≤ 300, Pr = 6.82, Le = 103, N = 1, H2O–Al2O3 nanofluid | Uniform grid, 201 201 |
| Taghizadeh & Asaditaheri [127] | OpenFOAM, FVM. SIMPLE | Lid-driven square cavity with cylinder | 2D, laminar, mixed convection | 0.01 ≤ Ri ≤ 10, 10−5 ≤ Da ≤ 10−2, 0 ≤ ≤ 90, Re = 100, Pr = 0.7 | Non-uniform, 11,200 |
| Zhai et al. [128] | FVM, SIMPLE, QUICK | Triangular Roof | 2D, unsteady, natural convection | 104 ≤ Ra ≤ 107, 0.1 ≤ A ≤ 1.0, Pr = 0.71 | Non-uniform mesh, 600 600 |
| Zhou et al. [129] | LBM, OpenMP | Double lid-driven cubic cavity | 3D, mixed convection, laminar, unsteady | 0.1 ≤ Ri ≤ 102, 0 ≤ ≤ 0.04, H2O–Al2O3 nanofluid | 101 101 101 |
| Alnaqi et al. [130] | FDM | Square cavity with a fin | 2D, laminar, steady | 0 ≤ Ha ≤ 60, 103 ≤ Ra ≤ 106, 0 ≤ Rd ≤ 3, 0 ≤ ≤ 0.06, H2O–Al2O3 nanofluid | Uniform grid. 120 120 |
| Al-Rashed et al. [131] | FVM, SIMPLEC | Inclined lid-driven cubical cavity | 3D, steady, mixed convection, laminar | Re, Gr, Pr, 1 ≤ Ri ≤ 102, 0 ≤ ≤ 45, H2O–Al2O3 nanofluid | - |
| Barnoon et al. [132] | FVM, SIMPLE | Lid-driven square cavity with cylinders | 2D, steady, laminar, mixed convection | 0 ≤ Ha ≤ 30, 1 ≤ Ri ≤ 102, 0 ≤ ≤ 90, 0.01 ≤ ≤ 0.03, H2O–Al2O3 nanofluid | Non-uniform grid, 19,297 elements |
| Bhowmick et al. [133] | Fluent, FVM, SIMPLE | V-shaped triangular cavity | 2D, natural convection, unsteady | 1 ≤ Ra ≤ 108, A = 0.5, Pr = 0.71 | 800 150 |
| Cho [134] | FVM, SIMPLE, TDMA | Lid-driven cavity with wavy wall | 2D, laminar, mixed convection | 1 ≤ Re ≤ 300, 0 ≤ Ha ≤ 50, 10−2 ≤ Re ≤ 102, 0 ≤ Am ≤ 0.7, 0 ≤ ≤ 0.04, 0 ≤ ≤ 360, Cu-H2O nanofluid | Non-uniform grid, 101 1001 |
| Cui et al. [135] | FVM | Prismatic enclosure | 3D, unsteady, natural convection | 100 ≤ Ra ≤ 107, Pr = 0.71, 0.1 ≤ A ≤ 1.5 | Non-uniform mesh, 138 42 51 |
| Hadavand et al. [136] | FVM, SIMPLEC | Lid-driven sim-circular cavity | 2D, mixed convection, laminar, steady | 1 ≤ Ri ≤ 10, = −90 to 90, 0 ≤ ≤ 0.06, Ag-H2O nanofluid | Unorganized triangular grid, 44,896 grids |
| Hamid et al. [137] | FEM | Trapezoidal cavity | 2D, steady, non-Newtonian, natural convection, laminar | 104 ≤ Ra ≤ 105.5, Pr = 20 | - |
| Jiang & Zhou [4] | FVM, QUICK, CDS, PISO | Rectangular cavity | 2D, steady, laminar | 0 ≤ ≤ 0.25, dp = 13, 36, 50 nm, distilled H2O-Al2O3, PGW-ZnO nanofluids | Non-uniform orthogonal grid, 200 50 |
| Karatas & Derbentli [138] | Experimental research | Rectangular cavity | 3D, unsteady | 4.5 103 ≤ Rai ≤ 1.13 108, A = 1, 2.09, 3, 4, 5, 6 | - |
| Lamarti et al. [139] | LBM | Lid-driven square cavity | 2D, mixed convection, laminar | Pr = 0.71, 102 ≤ Re ≤ 103, 102 ≤ Gr ≤ 106, 1 ≤ ≤ 5 | 100 100 |
| Louaraychi et al. [140] | FVM, SIMPLER, TDMA | Double lid-driven rectangular cavity | 2D, unsteady, mixed convection | 1 ≤ Ra ≤ 107, 0.1 ≤ Pe ≤ 500, A = 24 | Uniform grid, 381 121 |
| Mohebbi et al. [141] | LBM | Γ-shaped enclosure with obstacle | 2D, natural convection, steady, laminar | 103 ≤ Ra ≤ 106, 0 ≤ ≤ 0.05, 0.2 ≤ A ≤ 0.6, Al2O3-H2O nanofluid | 100 100 |
| Selimefendigil & Öztop [142] | FEM, ALE, NR | Lid-driven L-shaped cavity | 2D, steady, mixed convection | 0.03 ≤ Ri ≤ 30, 0 ≤ ≤ 180, 104 ≤ RaI ≤ 106, 0 ≤ Ha ≤ 50, CuO-H2O nanofluid | Non-uniform, 19,112 elements |
| (c) | |||||
| Ref. | CFD Methods and Algorithms | Flow Domain | Dimension, Type of Flow | Parameters and Ranges | Meshing |
| Abu-Hamdeh et al. [143] | FVM, SIMPLE, CDS, UDS, TDMA | Lid-driven porous square open cavity | 2D, mixed convection, steady | 102 ≤ Re ≤ 103, 10−3 ≤ Da ≤ 0.1, 103 ≤ Gr ≤ 105 | Staggered grid, 48 48 |
| Afrand et al. [144] | SIMPLE | Triangular cavity | 2D, free convection, laminar, steady | 104 ≤ Ra ≤ 106, 0 ≤ Ha ≤ 40, 0 ≤ ≤ 90, 0 ≤ ≤ 0.06, Al2O3-H2O nanofluid | Staggered grid |
| Alsabery et al. [145] | FEM | Lid-driven square cavity | 2D, mixed convection, steady, laminar | 1 ≤ Re ≤ 500, 0.01 ≤ Ri ≤ 100, 0 ≤ Ha ≤ 50, 0 ≤ ≤ 0.04, Al2O3-H2O nanofluid | Triangular grid, 6402 elements |
| Alsabery et al. [146] | FEM | Lid-driven cubic cavity with cylinder | 3D, mixed convection, steady | Re = 10, 100, Pr = 4.623, 10−2 ≤ Ri ≤ 102, 0 ≤ ≤ 0.04, Le = 3.5 105, Sc = 3.55 104, Al2O3-H2O nanofluid | Non-uniform triangular grid, 175,778 elements |
| Bilal et al. [147] | FEM | Triangular cavity with cylinder | 2D, laminar, steady, free convection | 103 ≤ Ra ≤ 106, 0.2 ≤ Pr ≤ 7 | Hybrid grid |
| Cho [148] | FVM, SIMPLE, TDMA | Square cavity with wavy walls | 2D, natural convection, steady, laminar | Pr = 6.2, 102 ≤ Ra ≤ 106, 10−6 ≤ Da ≤ 10−2, 0 ≤ ≤ 0.04, Cu-H2O nanofluid | 101 1001 |
| Ganesh et al. [149] | FEM | Square Cavity with different obstacles | 2D, steady, laminar | 103 ≤ Ra ≤ 106, 0 ≤ ≤ 0.08, 103 ≤ RaE ≤ 106, 103 ≤ RaI ≤ 105 Al2O3-H2O/Ethylene Glycol nanofluid | Circular: 6979 elements, Square: 21,916 elements, Triangular: 19,431 elements |
| Haq et al. [150] | FEM | Lid-driven hexagonal cavity with obstacle | 2D, steady | 200 ≤ Re ≤ 500, Pr = 6.2, 10−6 ≤ Ri ≤ 1, 0 ≤ Ha ≤ 20, SWCNT-H2O nanofluid | Non-uniform triangular elements |
| Khan et al. [151] | FEM | Porous trapezoidal cavity | 2D | Pr = 6.2, 102 ≤ Ha ≤ 104, 104 ≤ Ra ≤ 105, 0 ≤ ≤ 0.2%, Fe3O4-H2O ferrofluid | - |
| Li et al. [152] | FDLBM | Triangular enclosure | 2D, laminar, non-Newtonian, steady | 103 ≤ Ra ≤ 105, 0 ≤ Ha ≤ 60, 0 ≤ ≤ 90 | 160 160 or 19,600 nodes |
| Liu & Huang [153] | DNS, QUICK, SIMPLE, CDS | Rectangular cavities with or without fins | 2D, unsteady, turbulent | 1.15 108 ≤ Ra ≤ 3.68 109 | Non-uniform grid, 400 360 cells |
| Rammane et al. [154] | TS, MLS, NR, HO-MFA | Lid-driven square cavity | 2D, steady | 102 ≤ Re ≤ 2 104 | Mesh free |
| Saha et al. [155] | FVM, SIMPLE, QUICK | Triangular enclosure | 2D, unsteady, natural convection | Pr = 0.72, 105 ≤ Ra ≤ 109, 0.2 ≤ A ≤ 1.0 | 380 80, 380 100, 380 160 for A = 0.2, 0.5, 1 |
| Selimefendigil & Öztop [156] | FVM, QUICK, SIMPLE | U-shaped corrugated cavity | 2D, forced convection, laminar, steady | 102 ≤ Re ≤ 103, 0 ≤ Ha ≤ 50, 10−4 ≤ Da ≤ 5 10−2, CNT-H2O nanofluid | Non-uniform grid, 38,874 grids |
| Soomro et al. [157] | FEM | Lid-driven Triangular cavity with obstacle | 2D, mixed convection, laminar, steady | 200 ≤ Re ≤ 600, 0.01 ≤ Ri ≤ 1, 0 ≤ Ha ≤ 20, Pr = 6.2 | Around 5000 nodes |
| Thiers et al. [158] | SEM, NeK5000, GMRES | Rectangular cavity | 2D, unsteady | A = 4, Pr = 0.71, Ra = 9 107 | 183 169 |
| Aljabair et al. [159] | FDM, CDS, UDS, SOR | Sinusoidal lid-driven cavity | 2D, mixed convection, laminar | 1 ≤ Re ≤ 1000, 0 ≤ Ra ≤ 107, 0 ≤ ≤ 0.07, Cu-H2O nanofluid | 41 41 |
| Alsabery et al. [160] | FEM | Wavy lid-driven square cavity | 2D, laminar, steady, mixed convection | 0.01 ≤ Ri ≤ 10, Re = 100, 0 ≤ ≤ 0.04, H2O/Cu-Al2O3 hybrid nanofluid | - |
| Çolak et al. [161] | OpenFOAM, FVM, SIMPLE | Lid-driven cavity with porous block | 2D, mixed convection, steady | 10−1 ≤ Ri ≤ 10, Gr = 105, 10−7 ≤ Da ≤ 10−1, Pr = 6.2 | Uniform grid, 201 201 |
| Eshaghi et al. [162] | FEM | H-shaped cavity with a baffle inside | 2D, natural convection, laminar | 104 ≤ Ra ≤ 106, 2 ≤ Le ≤ 8, 1 ≤ N ≤ 3, −60 ≤ . ≤ 60, Cu-Al2O3-H2O hybrid nanofluid | - |
| Fayz-Al-Asad et al. [163] | FEM | Triangular cavity | 2D, natural convection, steady | Pr = 0.71, 104 ≤ Ra ≤ 106 | 6718 elements, 13,112 nodes |
| Hasnaoui et al. [164] | LBM, MRT, BGK | Rectangular cavity | 2D, biry mixture | Sr = −0.5, 0, 0.5, 0 ≤ Ra ≤ 80, Pr = 0.71, A = 2, Le = 2 | 120 240 |
| Hussain et al. [165] | FEM | Double lid-driven cavity with fins | 2D, laminar, steady | 0 ≤ Ha ≤ 100, 0 ≤ ≤ 90, 0.01 ≤ Ri ≤ 1, Pr = 6.2, Re = 100, = 0.02, Cu-H2O nanofluid | 33,177 grids |
| Hoston et al. [166] | IEFG–RIPM | Lid-driven square cavity | 2D, steady | Re = 10,000, 15,000, 20,000, 25,000, 30,000 and 35,000 | 150 150 (Refined at cavity walls) |
| Ibrahim & Hirpho [167] | COMSOL, FEM | Trapezoidal cavity | 2D, mixed convection, laminar | Ha = 0, 50, Ri = 0.1, 1, 10, Re = 100, Am = 0.25, 0.5, 1 | 91 91 |
| Ikram et al. [168] | FEM, ALE | Hexagonal cavity with rotating modulator | 2D, forced convection, unsteady, laminar | Pr = 0.71, 102 ≤ Re ≤ 103, 103 ≤ Bi ≤ 104, 103 ≤ Ra ≤ 107 | Non-uniform, 48,548 elements |
| Joe & Perumal [169] | OpenFOAM, FVM | Rectangular cavity containing cylinders | 2D, unsteady | Pr = 0.72, Re = 1600 | Unstructured triangular cells |
| Mondal & Mahapatra [170] | FDM, BiCGStab | Trapezoidal cavity | 2D, mixed convection, steady, laminar | Pr = 6.2, N = 5, 0.5 ≤ A ≤ 2, 10−2 ≤ Ri ≤ 102, 102 ≤ Re ≤ 103, 45 ≤ ≤ 90, 0 ≤ ≤ 0.5, 20 ≤ Ha ≤ 40, 1 ≤ Le ≤ 2, Al2O3-H2O nanofluid | 81 81 |
| Mebarek-Oudina et al. [171] | FEM | Trapezoidal porous cavity with zigzag wall | 2D, laminar, steady | 0 ≤ Ha ≤ 100, 0 ≤ ≤ 90, 103 ≤ Ra ≤ 105, 0 ≤ ≤ 0.08, Cu-Al2O3/H2O hybrid nanofluid | Triangular grid, 20,296 elements |
| Saha et al. [172] | FVM | Rectangular cavity with baffles | 2D, laminar, steady | 50 ≤ Re ≤ 250 Ri = 0, 2, 4 | 45 51 |
| Saha et al. [173] | FVM, SIMPLE, QUICK | Triangular cavity | 2D, natural convection | Pr = 0.72, 103 ≤ Ra ≤ 106, A = 0.2, 0.5, 1.0 | 360 75, 360 90, 360 120 for A = 0.2, 0.5, 1 |
| Shah et al. [174] | FEM, NR | Lid-driven trapezoidal cavity with obstacle | 2D, mixed convection, steady, laminar | 10−2 ≤ Ri ≤ 10, 0.1 ≤ Le ≤ 10, 300 ≤ Re ≤ 500, −10 ≤ N ≤ 10, Pr = 0.71 | Non-uniform, approx. 3200 elements |
| Shahid et al. [175] | MRT-LBM | Triangular lid driven cavity | 2D, mixed convection, unsteady | Pr = 0.71, 1, 7, 10−2 ≤ Ri ≤ 102, 104 ≤ Gr ≤ 107 | - |
| Shahid et al. [176] | MRT-LBM | Lid-driven rectangular cavity with obtacles | 2D, mixed convection, laminar | Pr = 0.71, 1, 7, 10−2 ≤ Ri ≤ 102, 103 ≤ Gr ≤ 106, A = 0.2, 0.5, 2, 5 | Not clearly mentioned for different A |
| Shekaramiz et al. [177] | OpenFOAM, FVM, SIMPLE | Wavy triangular cavity | 2D, free convection, steady | 5 103 ≤ Ra ≤ 2 105, Pr = 4.6, 0 ≤ Ha ≤ 50, 0 ≤ ≤ 90, = 2%, H2O-Fe3O4 nanofluid | 16,000 and 12,000 nodes |
| Tizakast et al. [178] | FVM, SIMPLER | Lid-driven rectangular cavity | 2D, laminar, unsteady, non-Newtonian | RaT ≤ 5 106, Pe ≤ 103, 10−3 ≤ Le ≤ 103, 10−3 ≤ N ≤ 103, 0.6 ≤ n ≤ 1.4 | Uniform grid, A = 24:381 121 |
| Velkennedy et al. [179] | FDM, ADI, CDS | Rectangular vented cavity | 2D, laminar, unsteady | 103 ≤ Ra ≤ 106, Pr = 0.71 | 181 121 |
| Xiong et al. [180] | FEM | Lid-driven triangular cavity | 2D, mixed convection | Pr = 6.2, 0 ≤ Ha ≤ 40, 0 ≤ Re ≤ 103, 0.01 ≤ Ri ≤ 2 | Uniform triangular mesh |
| Abbas et al. [181] | FEM | Square cavity with obstacles | 2D, steady, laminar | 0 ≤ ≤ 0.04, 10 ≤ Ha ≤ 40, 103 ≤ Ra ≤ 107, Cu-H2O nanofluid | 59,173 elements |
| Ahmed et al. [182] | Coiflet wavelet-homotopy method | Lid-driven square porous cavity | 2D, unsteady | Pr = 6.2, 10 ≤ Ri ≤ 102, 10−4 ≤ Da ≤ 10−1, 3 ≤ Le ≤ 106, Al2O3-Cu/H2O hybrid nanofluid | - |
| Alam et al. [8] | FEM | Semi-circular cavity | 2D, unsteady, semi-circular cavity, free convection | Pr = 23.0, 6.84, 1 ≤ dp ≤ 10, 0 ≤ ≤ 0.05, 0 ≤ Ha ≤ 100, 104 ≤ RaT ≤ 106, ZnO, Fe3O4, Co, Al2O3, Ag nanoparticles, H2O & kerosene as base fluids | 15,817 elements |
| Ali et al. [183] | Comsol, FEM | Lid-driven square cavity | 2D, mixed convection, steady | Re = 1, 10, 102, Ha = 0, 10, 25, = 0, 1%, 5%, Pr = 6.2, Gr = 102, Al2O3-H2O nanofluid | Non-uniform, 30,550 nodes, 60,036 elements |
| Alqaed et al. [5] | FVM, SIMPLE | Rectangular cavity with triangular blades | 2D, steady, laminar | 103 ≤ Ra ≤ 105, 0 ≤ Ha ≤ 30, = 0.3, Al2O3-H2O nanofluid | 150 450 |
| Acharya & Chamkha [184] | FEM | Hexagonal cavity with parallel fins | 2D, laminar, steady | 103 ≤ Ra ≤ 105, 0 ≤ Ha ≤ 100, 0 ≤ ≤ 0.04, Pr = 6.2, Al2O3-H2O nanofluid | Non-uniform, 34,502 elements |
| Batool et al. [185] | FVM, SIMPLE | Lid-driven square cavity | 2D, steady, laminar | 100 ≤ Re ≤ 400, micropolar nanofluids | Staggered grid |
| Charqui et al. [186] | FVM, SIMPLE, TDMA | Tall partitioned cavity | 2D, natural convection, laminar | Pr = 0.71, 7, A = 40 | Uniform mesh, 80 200 |
| Cui et al. [187] | FVM, SIMPLE | Triangular cavity | 3D, natural convection, unsteady | Pr = 0.7, 102 ≤ Ra ≤ 107, 0.1 ≤ A ≤ 1.5 | Non-uniform mesh, 141 41 51 |
| Dahani et al. [188] | LBM, MRT, BGK operator | Double lid-driven square cavity | 2D, unsteady | 0 ≤ ≤ 180, Re = 102, 10−2 ≤ Ri ≤ 102, 102 ≤ Gr ≤ 106, Pr = 0.71 | Uniform grid, 160 160 |
| Esfe et al. [9] | Fluent, FVM, SIMPLE | U-shaped porous enclosure | 2D, steady | 103 ≤ Ra ≤ 105, 0 ≤ ≤ 0.03, Al2O3-H2O nanofluid | Approx 1600 cells |
| Geridonmez & Oztop [10] | Rbf-Pum | Right Isosceles triangular cavity | 2D, natural convection, steady, laminar | 0 ≤ Ha ≤ 100, 0.25 ≤ A ≤ 1, Pr = 6.07, Ra = 105, 0 ≤ ≤ 0.02, Al2O3-Cu/H2O nanofluid | 1,842,025 & 1,741,596 nodes |
| Hirpho & Ibrahim [189] | FEM | Trapezoidal enclosure | 2D, mixed convection, non-Newtonian, steady, laminar | 10−1 ≤ Ri ≤ 102, Re = 100, Pr = 20, 0 ≤ ≤ 0.02, Al2O3-Cu/H2O hybrid nanofluid | 201 201 |
| Khalil et al. [190] | Fluent, FVM, RSM | Porous trapezoidal cavity with wavy wall | 2D, steady | 0 ≤ Ha ≤ 40, 0 ≤ Am ≤ 20, 5 102 ≤ Ra ≤ 2.4 104 | 320 320 |
| Liu [191] | CDS, SIMPLE, QUICK | Rectangular cavity with fins | 2D, free convection, unsteady | 0 ≤ ≤ 40, Ra = 1.84 109 | 360 400 |
| Noor et al. [192] | FEM | Lid-driven trapezoidal cavity with obstacle | 2D, forced convection, steady | 102 ≤ Re ≤ 700, 10−3 ≤ Ri ≤ 10, 0 ≤ Ha ≤ 102 | Trangular grid, 4622 nodes. 8929 elements |
| Nouraei et al. [193] | FVM, SIMPLE | Semi-circular vented cavity | 2D, mixed convection, laminar, steady | 10 ≤ Re ≤ 100, 0 ≤ ≤ 0.06, Cu-H2O nanofluid | 52,000 grids |
| Polasanapalli & Anupindi [194] | LBM | Concentric circular annular cavity | 2D, mixed convection, unsteady | 104 ≤ Ra ≤ 106, 0 ≤ Re ≤ 104, Pr = 0.71, 0 ≤ ≤ 360, 10−3 ≤ Ri ≤ 103 | 120 120, 180 180 |
| Prince et al. [195] | COMSOL, FEM | Trapezoidal cavity with different surface | 2D, natural convection | Pr = 0.716, 103 ≤ Ra ≤ 106, Materials: Pinewood, plexiglas, dry concrete, glass fiber | Rectangle: 6112, Triangle: 10,191, Sinusoidal: 5993 elements |
| Rahaman et al. [196] | Fluent, FVM, CDS, SIMPLE | Trapezoidal cavity | 2D, unsteady, natural convection | Pr = 0.71, 100 ≤ Ra ≤ 108, A = 0.5 | Non-uniform, 300 100 |
| Roy et al. [197] | FEM | Square enclosure with blocks | 2D, unsteady, natural convection | Pr = 6.2, 0 ≤ Rd ≤ 3, 0 ≤ ≤ 0.09, 104 ≤ Ra ≤ 106, 0 ≤ Ha ≤ 60, Cu- Al2O3/H2O hybrid nanofluid | Triangular 65,740 elements |
| Shah et al. [198] | FEM, NR | Lid-driven corrugated porous cavity | 2D, mixed convection, laminar, steady | 102 ≤ Re ≤ 400, 0 ≤ ≤ 0.05, 10−5 ≤ Da ≤ 10−1, Pr = 6.2, 10−2 ≤ Ri ≤ 100, CuO-H2O nanofluid | Approx 8000 elements |
| Tizakast et al. [199] | FVM, SIMPLE | Lid-driven Rectangular cavity | 2D, unsteady non-Newtonian, laminar | 2 ≤ A ≤ 30, 102 ≤ RaT ≤ 107, 10−3 ≤ Le ≤ 103, 0.1 ≤ Pe ≤ 104 | - |
| Xia et al. [200] | UPWIND | T-shaped lid-driven cavity | 2D, mixed convection | 0 ≤ ≤ 0.03, 0.1 ≤ Re ≤ 10, 0.2 ≤ A ≤ 0.4, Al2O3-H2O nanofluid | Uniform mesh, 250,000 cells |
| Zarei et al. [201] | FVM, SIMPLE | Square cavity with wavy walls | 2D, steady | Ra = 104, 0 ≤ Am ≤ 0.15, 0 ≤ ≤ 0.04, Cu-H2O nanofluid | 135,008 triangular cells |
| Zhang et al. [202] | FVM, SIMPLE | Semi-elliptic lid-driven cavity | 2D, mixed convection, steady, laminar | 0 ≤ ≤ 0.06, Gr = 5 104, 15 104, 25 104, 4 105, 10−1 ≤ Ri ≤ 10, Pr = 6.2, Ag-H2O nanofluid | 43,905 triangular grids |
| Akhter et al. [7] | FEM | square cavity with cylinder | 2D, steady | 104 ≤ Ra ≤ 5 106, Pr = 6.2, 0 ≤ ≤ 0.05, 0 ≤ Ha ≤ 102, Cu-Al2O3/H2O hybrid nanofluid | 24,961 nodes, 49,188 elements |
| He et al. [203] | Experimental, FVM, SIMPLE | Cubic cavity | 3D | 0 ≤ B ≤ 133, 0 ≤ ≤ 0.03, 6.9 105 ≤ Ram ≤ 11.6 105, Fe3O4-Paraffin nanofluid | 50 50 50 |
| Ikram et al. [204] | FEM, ALE | Hexagonal cavity with rotating modulator | 2D, forced convection, unsteady, laminar | Pr = 0.71, 102 ≤ Re ≤ 103, 104 ≤ Ra ≤ 106, Bi = 104 | 49,874, 50,672 and 52,601 elements |
| Ouri et al. [205] | Comsol, FEM, ANFIS | L-shaped cavity with rotating cylinder | 2D, unsteady | 200 ≤ Re ≤ 103, 102 ≤ Rew ≤ 103, 0 ≤ Ha ≤ 40, 0 ≤ ≤ 0.02, Ag-MgO/H2O hybrid nanofluid | Non-uniform triangular 65,216 elements |
| Sayed et al. [206] | FVM, LES, URANS | Cubical cavity | 3D, unsteady | Ra = 109, Pr = 0.71, Prt = 0.9 | 166,375 cells |
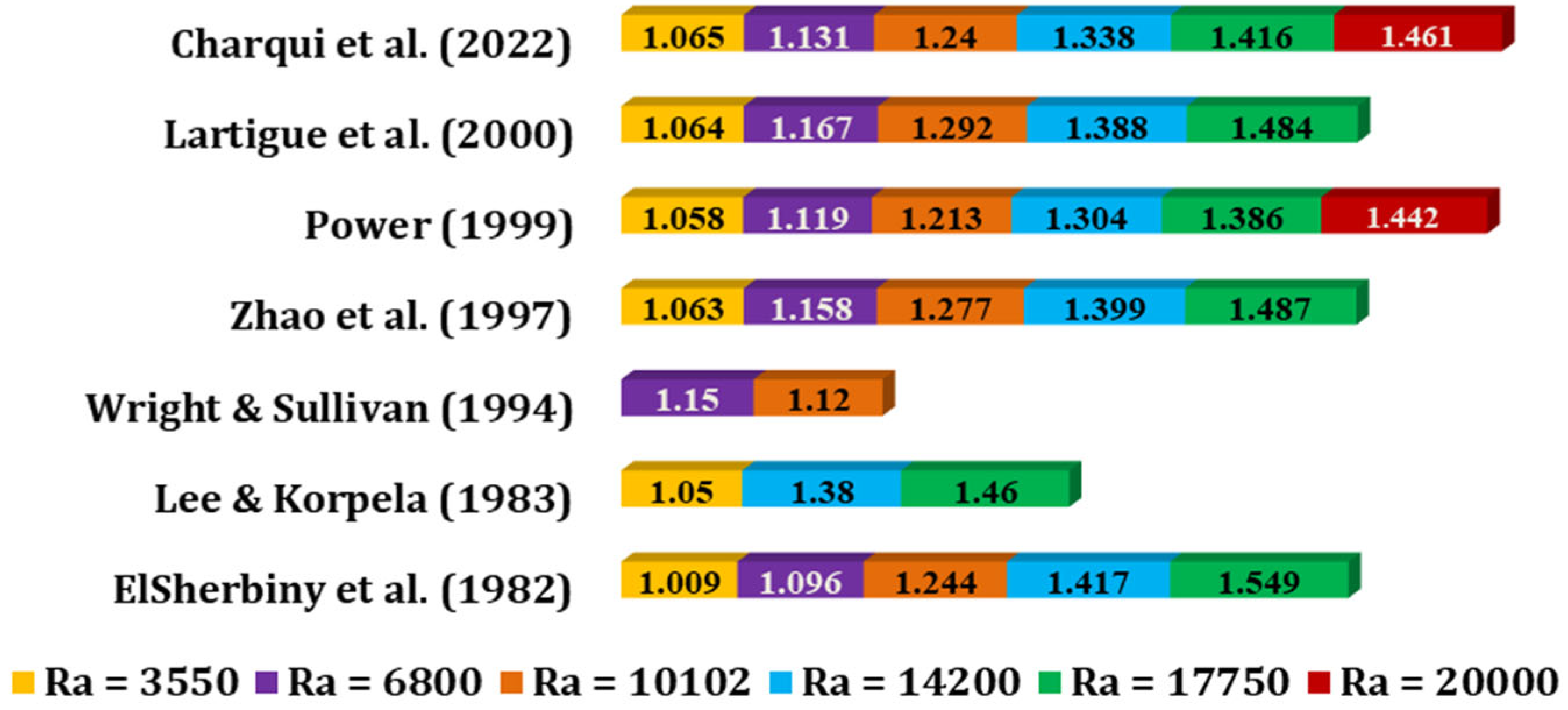
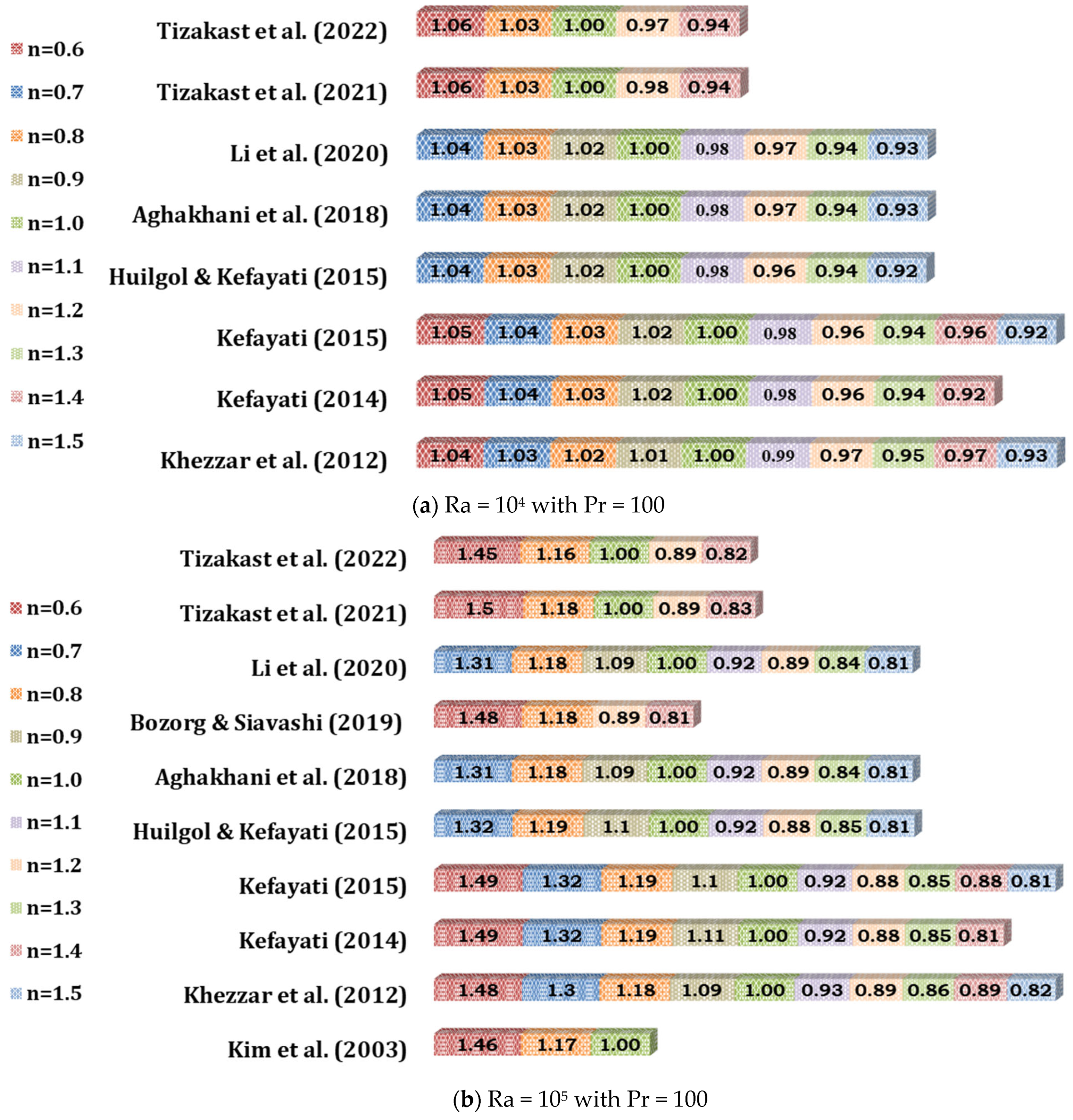
4. Validations
5. Heat Transfer Analysis
5.1. Square Cavity
5.2. Rectangular Cavity
5.3. Other Cavity Types
5.4. Lid-Driven Cavity
5.4.1. Square Cavity
5.4.2. Rectangular Cavity
5.4.3. Triangular Cavity
5.4.4. Cubical Cavity
5.4.5. Other Types of Cavities
6. Conclusions
7. Future Work
- Most of the studies focused on laminar natural/mixed/forced convection flow, but very few studies considered turbulent flow regimes.
- Most recently, rotating blades or plates inside the cavity received attention from researchers. Still, no work is conducted considering the design, shape, and aerodynamic behavior of blades.
- Will it be possible to develop a general form of correlation for the average Nusselt number?
- Very little research is found considering the LES and DNS modeling in cavities for transient/turbulent flow regimes. This area should be considered a high-interest area in the future.
- The choice of turbulence models has not been highlighted yet by researchers. It can be an important study.
- Different types of shapes are considered in the literature, but more research is suggested to find an optimum design of the physical domain that can give maximum heat transfer enhancement.
- Very little or limited research work is conducted considering the presence of nanoplastic or microplastic in fluids inside a cavity. It is highly considered research for future studies.
- Study the hydraulic and thermal behavior of two immiscible fluids (for example, air and water) inside a cavity.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | Aspect ratio |
| AB | Adams-Bashforth method |
| ADI | Alternate Direct Implicit scheme |
| ALE | Arbitrary-Lagrangian–Eulerian approach |
| Am | Amplitude |
| ANFIS | Adaptive Neuro-Fuzzy Interface System |
| b | Size of blocage (%) |
| B | Dimensionless magnetic induction |
| BGK | Bhatnagar–Gross–Krook |
| BiCGStab | Biconjugate gradient stabilized method |
| C | Concentration |
| CDS | Central differencing schemes |
| CN | Crank-Nicolson |
| COBYLA | Constrained Optimization BY Linear Approximations |
| Cp | Specific heat |
| DADI | Dynamic alternating direction implicit scheme |
| DRBEM | Dual reciprocity boundary element method |
| DNS | Direct numerical simulation |
| dp | Nanoparticles diameter |
| E | Young’s modulus |
| f | Fluid |
| FDM | Finite difference method |
| FEM | Finite element method |
| FVM | Finite volume method |
| FSI | Fluid-structure interaction |
| GE | Gaussian Elimination method |
| Gr | Grashof number |
| GrC | Mass transfer Grashof number |
| GrT | Heat transfer Grashof number |
| GS | Gauss-Seidel method |
| h | Cavity height |
| Ha | Hartmann number |
| HO-MFA | High-order mesh-free approach |
| IEFG–RIPM | Improved element-free Galerkin–reduced integration penalty method |
| J | Joul heating parameter |
| K | Thermal conductivity ratio |
| Kl | Low conductivity material |
| LB | lattice Boltzmann method |
| LES | Large Eddy Simulation |
| Le | Lewis number |
| Lh | Vertical distance between bottom of the cavity and the hot disturbance area |
| Lc | Vertical distance between bottom of the cavity and the cold disturbance area |
| M | Magnetic parameter |
| Ma | Mach number |
| MLS | Moving least squares |
| MRT | Multiple-Relaxation-Time |
| MWCNT | Multi wall carbon nanotubes |
| n | Flow behavior index for a power-law fluid |
| nf | Nanofluid |
| N | Buoyancy ratio |
| Nb | Brownian motion |
| Nt | Thermophoresis parameter |
| NR | Newton-Raphson method |
| Nu | Local Nusselt number |
| Average Nusselt number (Nuavg) | |
| NuL | Average Nusselt number based on the cavity length |
| NuymL | The mean of the local Nusselt numbers |
| Os | Ostrogradsky number |
| Pe | Peclet number |
| PCM | Phase change material |
| Pr | Prandtl number |
| Prt | Turbulent Prandtl number |
| q | Heat generation or absorption |
| QUICK | Quadratic upstream interpolation for convective kinematics |
| R | Internal to external Rayleigh numbers ratio |
| Ra | Rayleigh number |
| RaT | Generalized thermal Rayleigh number |
| RaI | Internal Rayleigh number |
| RaE | External Rayleigh number |
| Ram | Magnetic Rayleigh number |
| RaL | Average Rayleigh number based on the cavity length |
| Rayml | The mean of the local Rayleigh numbers |
| Rbf-Pum | Radial basis function-based partition of unity method |
| RBSOR | Red and black SOR method |
| Rd | Radiation parameter |
| Re | Reynolds number |
| Rew | Rotational Reynolds number |
| Ri | Richardson number |
| RK | Runge-Kutta method |
| RSM | Response surface methodology |
| s, np | Solid or nanoparticles |
| Sc | Schemidt number |
| SEM | Spectral element method |
| Sh | Sherwood number |
| SIMPLE | Semi-implicit method for pressure-linked equations |
| SOR | Successive over-relaxation |
| SUR | Successive under relaxation |
| Sr | Soret parameter |
| Ta | Taylor number |
| TDMA | Tri-diagonal matrix algorithm |
| TFM | Triangular factorization method |
| Tp | Partition thickness |
| Tr | Thermal conductivity ratio, |
| TS | Taylor series |
| UCS | Upwind compact Scheme |
| UDS | Upwind difference Scheme |
| URANS | Averaged Navier–Stokes |
| x | Horizontal coordinate |
| y | Vertical coordinate |
| Inclination angle | |
| Nanoparticles volume fraction | |
| Emissivity | |
| Number of undulation | |
| Thermal conductivity | |
| Phase deviation | |
| Density | |
| Rotational speed | |
| Oscillation frequency | |
| Angular frequency | |
| Dynamic viscosity |
References
- Holman, J.P. Heat Transfer, 10th ed.; Mcgraw-Hill Higher Education: Boston, MA, USA, 2010; ISBN 0073529362. [Google Scholar]
- Wee, H.K.; Keey, R.B.; Cunningam, M.J. Heat and moisture transfer by natural convection in a rectangular cavity. Int. J. Heat Mass Transf. 1989, 32, 1765–1778. [Google Scholar] [CrossRef]
- Yu, P.X.; Xiao, Z.; Wub, S.; Tian, Z.F.; Cheng, X. High accuracy numerical investigation of double-diffusive convection in a rectangular cavity under a uniform horizontal magnetic field and heat source. Int. J. Heat Mass Transf. 2017, 110, 613–628. [Google Scholar] [CrossRef]
- Jiang, Y.; Zhou, X. Analysis of flow and heat transfer characteristics of nanofluids surface tension driven convection in a rectangular cavity. Int. J. Mech. Sci. 2019, 153–154, 154–163. [Google Scholar] [CrossRef]
- Alqaed, S.; Mustafa, J.; Sharifpur, M. Numerical investigation and optimization of natural convection and entropy generation of alumina/H2O nanofluid in a rectangular cavity in the presence of a magnetic field with artificial neural networks. Eng. Anal. Bound. Elem. 2022, 140, 507–518. [Google Scholar] [CrossRef]
- Hatami, M. Numerical study of nanofluids natural convection in a rectangular cavity including heated fins. J. Mol. Liq. 2017, 233, 1–8. [Google Scholar] [CrossRef]
- Akhter, R.; Ali, M.M.; Alim, M.A. Entropy generation due to hydromagnetic buoyancy-driven hybrid-nanofluid flow in partially heated porous cavity containing heat conductive obstacle. Alex. Eng. J. 2023, 62, 17–45. [Google Scholar] [CrossRef]
- Alam, M.S.; Billah, M.M.; Hossain, S.M.C.; Keya, S.S.; Haque, M.M. MHD influence on convective heat transfer in a semi-circular cavity using nonhomogeneous nanofluid model. Int. J. 2022, 16, 100197. [Google Scholar]
- Esfe, M.H.; Rostamian, H.; Toghraie, D.; Hekmatifar, M.; Abad, A.T.K. Numerical study of heat transfer of U-shaped enclosure containing nanofluids in a porous medium using two-phase mixture method. Case Stud. Therm. Eng. 2022, 38, 102150. [Google Scholar] [CrossRef]
- Geridonmez, B.P.; Oztop, H.F. The effect of inclined periodic magnetic field on natural convection flow of Al2O3-Cu/water nanofluid inside right isosceles triangular closed spaces. Eng. Anal. Bound. Elem. 2022, 141, 222–234. [Google Scholar] [CrossRef]
- Moallemi, M.K.; Jjang, K.S. Prandtl number effects on laminar mixed convection heat transfer in a lid-driven cavity. Int. J. Heat Mass Transf. 1992, 35, 1881–1892. [Google Scholar] [CrossRef]
- Rashad, A.M.; Ismael, M.A.; Chamkha, A.J.; Mansour, M.A. MHD mixed convection of localized heat source/sink in a nanofluid-filled lid-driven square cavity with partial slip. J. Taiwan Inst. Chem. Eng. 2016, 68, 173–186. [Google Scholar] [CrossRef]
- Versteeg, H.K. An Introduction to Computational Fluid Dynamics: The Finite Volume Method, 2nd ed.; Henk, K., Malalasekera, W., Malalasekera, W., Eds.; Pearson Education Ltd.: Harlow, UK, 2007. [Google Scholar]
- Sasaguchi, K.; Kusano, K.; Viskanta, R. A numerical analysis of solid-liquid phase change heat transfer around a single and two horizontal. vertically spaced cylinders in a rectangular cavity. Int. J. Heat Mass Transf. 1997, 40, 1349–1354. [Google Scholar] [CrossRef]
- Al-Amiri, A.; Khanafer, K.; Bull, J.; Pop, I. Effect of sinusoidal wavy bottom surface on mixed convection heat transfer in a lid-driven cavity. Int. J. Heat Mass Transf. 2007, 50, 1771–1780. [Google Scholar] [CrossRef]
- Saha, G.; Saha, S.; Islam, M.Q.; Akhanda, M.A.R. Natural convection in enclosure with discrete isothermal heating from below. J. Nav. Archit. Mar. Eng. 2007, 4, 1–13. [Google Scholar] [CrossRef]
- Saha, S.C.; Lei, C.; Patterson, J.C. Effect of aspect ratio on natural convection in attics subject to periodic thermal forcing. ANZIAM J. 2007, 48, C677–C691. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Saha, S.; Sultana, T.; Saha, G.; Rahman, M.M. Effects of discrete isoflux heat source size and angle of inclination on natural convection heat transfer flow inside a sinusoidal corrugated enclosure. Int. Commun. Heat Mass Transf. 2008, 35, 1288–1296. [Google Scholar] [CrossRef]
- Varol, Y.; Oztop, H.F.; Mobedi, M.; Pop, I. Visualization of natural convection heat transport using heatline method in porous non-isothermally heated triangular cavity. Int. J. Heat Mass Transf. 2008, 51, 5040–5051. [Google Scholar] [CrossRef]
- Chen, C.-L.; Cheng, C.-H. Numerical study of the effects of lid oscillation on the periodic flow pattern and convection heat transfer in a triangular cavity. Int. Commun. Heat Mass Transf. 2009, 36, 590–596. [Google Scholar] [CrossRef]
- Noor, D.Z.; Kanna, P.R.; Chern, M.-J. Flow and heat transfer in a driven square cavity with double-sided oscillating lids in anti-phase. Int. J. Heat Mass Transf. 2009, 52, 3009–3023. [Google Scholar] [CrossRef]
- Ouertatani, N.; Cheikh, N.B.; Beya, B.B.; Lili, T.; Campo, A. Mixed convection in a double lid-driven cubic cavity. Int. J. Therm. Sci. 2009, 48, 1265–1272. [Google Scholar] [CrossRef]
- Basak, T.; Roy, S.; Ramakrishna, D.; Pandey, B.D. Analysis of heat recovery and heat transfer within entrapped porous triangular cavities via heatline approach. Int. J. Heat Mass Transf. 2010, 53, 3655–3669. [Google Scholar] [CrossRef]
- Lei, C.; O’Neill, A. Effects of adiabatic extensions on heat transfer through a differentially heated square cavity. Int. Commun. Heat Mass Transf. 2010, 37, 1221–1225. [Google Scholar] [CrossRef]
- Saha, G. Finite element simulation of magnetoconvection inside a sinusoidal corrugated enclosure with discrete isoflux heating from below. Int. Commun. Heat Mass Transf. 2010, 37, 393–400. [Google Scholar] [CrossRef]
- Saha, G.; Saha, S.; Hasan, M.N.; Islam, M.Q. Natural convection heat transfer within octagonal enclosure. IJE Trans. A Basics 2010, 23, 1–10. [Google Scholar]
- Saha, S.C.; Patterson, J.C.; Lei, C. Natural convection in attics subject to instantaneous and ramp cooling boundary conditions. Energy Build. 2010, 42, 1192–1204. [Google Scholar] [CrossRef]
- Saha, S.C.; Patterson, J.C.; Lei, C. Natural convection and heat transfer in attics subject to periodic thermal forcing. Int. J. Therm. Sci. 2010, 49, 1899–1910. [Google Scholar] [CrossRef]
- Saha, S.C.; Patterson, J.C.; Lei, C. Natural convection in attic-shaped spaces subject to sudden and ramp heating boundary conditions. Heat Mass Transf. 2010, 46, 621–638. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Sivakumar, V.; Prakash, P. Numerical study on mixed convection in a lid-driven cavity with non-uniform heating on both sidewalls. Int. J. Heat Mass Transf. 2010, 53, 4304–4315. [Google Scholar] [CrossRef]
- Sivakumar, V.; Sivasankaran, S.; Prakash, P.; Lee, J. Effect of heating location and size on mixed convection in lid-driven cavities. Comput. Math. Appl. 2010, 59, 3053–3065. [Google Scholar] [CrossRef]
- Al-Amiri, A.; Khanafer, K. Fluid–structure interaction analysis of mixed convection heat transfer in a lid-driven cavity with a flexible bottom wall. Int. J. Heat Mass Transf. 2011, 54, 3826–3836. [Google Scholar] [CrossRef]
- Cheng, T.S. Characteristics of mixed convection heat transfer in a lid-driven square cavity with various Richardson and Prandtl numbers. Int. J. Therm. Sci. 2011, 50, 197–205. [Google Scholar] [CrossRef]
- Nasrin, R.; Parvin, S. Hydromagnetic effect on mixed convection in a lid-driven cavity with sinusoidal corrugated bottom surface. Int. Commun. Heat Mass Transf. 2011, 38, 781–789. [Google Scholar] [CrossRef]
- Saha, S.C. Unsteady natural convection in a triangular enclosure under isothermal heating. Energy Build. 2011, 43, 695–703. [Google Scholar] [CrossRef]
- Saha, S.C. Scaling of free convection heat transfer in a triangular cavity for Pr > 1. Energy Build. 2011, 43, 2908–2917. [Google Scholar] [CrossRef]
- Yu, Z.-T.; Wang, W.; Xu, X.; Fan, L.-W.; Hu, Y.-C.; Cen, K.-F. A numerical investigation of transient natural convection heat transfer of aqueous nanofluids in a differentially heated square cavity. Int. Commun. Heat Mass Transf. 2011, 38, 585–589. [Google Scholar] [CrossRef]
- Al-Farhany, K.; Turan, A. Numerical study of double-diffusive natural convective heat and mass transfer in an inclined rectangular cavity filled with porous medium. Int. Commun. Heat Mass Transf. 2012, 39, 174–181. [Google Scholar] [CrossRef]
- Arani, A.A.A.; Sebdani, S.M.; Mahmoodi, M.; Ardeshiri, A.; Aliakbari, M. Numerical study of mixed convection flow in a lid-driven cavity with sinusoidal heating on sidewalls using nanofluid. Superlattices Microstruct. 2012, 51, 893–911. [Google Scholar] [CrossRef]
- Basak, T.; Gunda, P.; Anandalakshmi, R. Analysis of entropy generation during natural convection in porous right-angled triangular cavities with various thermal boundary conditions. Int. J. Heat Mass Transf. 2012, 55, 4521–4535. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Abu-Nada, E. Mixed convection flow in single- and double-lid driven square cavities filled with water–Al2O3 nanofluid: Effect of viscosity models. Eur. J. Mech. B Fluid. 2012, 36, 82–96. [Google Scholar] [CrossRef]
- Nasrin, R.; Parvin, S. Investigation of buoyancy-driven flow and heat transfer in a trapezoidal cavity filled with water–Cu nanofluid. Int. Commun. Heat Mass Transf. 2012, 39, 270–274. [Google Scholar] [CrossRef]
- Raji, A.; Hasnaoui, M.; Naïmi, M.; Slimani, K.; Ouazzani, M.T. Effect of the subdivision of an obstacle on the natural convection heat transfer in a square cavity. Comput. Fluid. 2012, 68, 1–15. [Google Scholar] [CrossRef]
- Saha, S.C.; Gu, Y.T. Free convection in a triangular enclosure with fluid-saturated porous medium and internal heat generation. ANZIAM J. 2012, 53, C127–C141. [Google Scholar] [CrossRef]
- Saha, S.C.; Gu, Y.T. Natural convection in a triangular enclosure due to non-uniform cooling on top. ANZIAM J. 2012, 53, C53–C68. [Google Scholar] [CrossRef]
- Teamah, M.A.; El-Maghlany, W.M. Augmentation of natural convective heat transfer in square cavity by utilizing nanofluids in the presence of magnetic field and uniform heat generation/absorption. Int. J. Therm. Sci. 2012, 58, 130–142. [Google Scholar] [CrossRef]
- Ahmed, S.E.; Mansour, M.A.; Mahdy, A. MHD mixed convection in an inclined lid-driven cavity with opposing thermal buoyancy force: Effect of non-uniform heating on both side walls. Nucl. Eng. Des. 2013, 265, 938–948. [Google Scholar] [CrossRef]
- Bhardwaj, S.; Dalal, A. Analysis of natural convection heat transfer and entropy generation inside porous right-angled triangular enclosure. Int. J. Heat Mass Transf. 2013, 65, 500–513. [Google Scholar] [CrossRef]
- Cho, C.-C.; Chen, C.-L.; Chen, C.-K. Mixed convection heat transfer performance of water-based nanofluids in lid-driven cavity with wavy surfaces. Int. J. Therm. Sci. 2013, 68, 181–190. [Google Scholar] [CrossRef]
- Elsherbiny, S.M.; Ragab, E.H. Laminar natural convection in inclined rectangular cavities with a localized heat source. Alex. Eng. J. 2013, 52, 249–257. [Google Scholar] [CrossRef]
- Huelsz, G.; Rechtman, R. Heat transfer due to natural convection in an inclined square cavity using the lattice Boltzmann equation method. Int. J. Therm. Sci. 2013, 65, 111–119. [Google Scholar] [CrossRef]
- Khanafer, K.; Aithal, S.M. Laminar mixed convection flow and heat transfer characteristics in a lid-driven cavity with a circular cylinder. Int. J. Heat Mass Transf. 2013, 66, 200–209. [Google Scholar] [CrossRef]
- Ramakrishna, D.; Basak, T.; Roy, S. Analysis of heatlines and entropy generation during free convection within trapezoidal cavities. Int. Commun. Heat Mass Transf. 2013, 45, 32–40. [Google Scholar] [CrossRef]
- Sivasankaran, S.; Sivakumar, V.; Hussein, A.K. Numerical study on mixed convection in an inclined lid-driven cavity with discrete heating. Int. Commun. Heat Mass Transf. 2013, 46, 112–125. [Google Scholar] [CrossRef]
- Sathiyamoorthy, M.; Chamkha, J. Analysis of natural convection in a square cavity with a thin partition for linearly heated side walls. Int. J. Numer. Method. Heat Fluid Flow 2014, 24, 1057–1072. [Google Scholar] [CrossRef]
- Abu-Nada, E.; Chamkha, A.J. Mixed convection flow of a nanofluid in a lid-driven cavity with a wavy wall. Int. Commun. Heat Mass Transf. 2014, 57, 36–47. [Google Scholar] [CrossRef]
- Ait-Taleb, T.; Abdelbaki, A.; Zrikem, Z. Simulation of coupled heat transfers in a hollow tile with two vertical and three horizontal uniform rectangular cavities heated from below or above. Energy Build. 2014, 84, 628–632. [Google Scholar] [CrossRef]
- Cheng, T.S.; Liu, W.-H. Effects of cavity inclination on mixed convection heat transfer in lid-driven cavity flows. Comput. Fluid. 2014, 100, 108–122. [Google Scholar] [CrossRef]
- Cho, C.-C. Heat transfer and entropy generation of natural convection in nanofluid-filled square cavity with partially-heated wavy surface. Int. J. Heat Mass Transf. 2014, 77, 818–827. [Google Scholar] [CrossRef]
- Kalteh, M.; Javaherdeh, K.; Toraj Azarbarzin, T. Numerical solution of nanofluid mixed convection heat transfer in a lid-driven square cavity with a triangular heat source. Powder Technol. 2014, 253, 780–788. [Google Scholar] [CrossRef]
- Khanafer, K. Comparison of flow and heat transfer characteristics in a lid-driven cavity between flexible and modified geometry of a heated bottom wall. Int. J. Heat Mass Transf. 2014, 78, 1032–1041. [Google Scholar] [CrossRef]
- Muthtamilselvan, M.; Doh, D.H. Mixed convection of heat generating nanofluid in a lid-driven cavity with uniform and non-uniform heating of bottom wall. Appl. Math. Model. 2014, 38, 3164–3174. [Google Scholar] [CrossRef]
- Ramakrishna, D.; Basak, T.; Roy, S.; Momoniat, E. Analysis of thermal efficiency via analysis of heat flow and entropy generation during natural convection within porous trapezoidal cavities. Int. J. Heat Mass Transf. 2014, 77, 98–113. [Google Scholar] [CrossRef]
- Ray, S.; Chatterjee, D. MHD mixed convection in a lid-driven cavity including heat conducting circular solid object and corner heaters with Joule heating. Int. Commun. Heat Mass Transf. 2014, 57, 200–207. [Google Scholar] [CrossRef]
- Saha, S.C.; Gu, Y.T. Transient air flow and heat transfer in a triangular enclosure with a conducting partition. Appl. Math. Model. 2014, 38, 3879–3887. [Google Scholar] [CrossRef]
- Xu, F.; Saha, S.C. Transition to an unsteady flow induced by a fin on the sidewall of a differentially heated air-filled square cavity and heat transfer. Int. J. Heat Mass Transf. 2014, 71, 236–244. [Google Scholar] [CrossRef]
- Cui, H.; Xu, F.; Saha, S.C. A three-dimensional simulation of transient natural convection in a triangular cavity. Int. J. Heat Mass Transf. 2015, 85, 1012–1022. [Google Scholar] [CrossRef]
- Elsherbiny, S.M.; Ismail, O.I. Heat transfer in inclined air rectangular cavities with two localized heat sources. Alex. Eng. J. 2015, 54, 917–927. [Google Scholar] [CrossRef]
- Groşan, T.; Revnic, C.; Pop, I.; Ingham, D.B. Free convection heat transfer in a square cavity filled with a porous medium saturated by a nanofluid. Int. J. Heat Mass Transf. 2015, 87, 36–41. [Google Scholar] [CrossRef]
- Moumni, H.; Welhezi, H.; Djebali, R.; Sediki, E. Accurate finite volume investigation of nanofluid mixed convection in two-sided lid-driven cavity including discrete heat sources. Appl. Math. Model. 2015, 39, 4164–4179. [Google Scholar] [CrossRef]
- Saha, S.C.; Gu, Y.T. Natural convection in a triangular enclosure heated from below and non-uniformly cooled from top. Int. J. Heat Mass Transf. 2015, 80, 529–538. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Pop, I. Mixed convection in a lid-driven square cavity filled by a nanofluid: Buongiorno’s mathematical model. Appl. Math. Comput. 2015, 266, 792–808. [Google Scholar] [CrossRef]
- Sojoudi, A.; Saha, S.C.; Gu, Y.T. Natural convection due to differential heating of inclined walls and heat source placed on bottom wall of an attic shaped space. Energy Build. 2015, 89, 153–162. [Google Scholar] [CrossRef]
- Armaghani, T.; Kasaeipoor, A.; Alavi, N.; Rashidi, M.M. Numerical investigation of water-alumina nanofluid natural convection heat transfer and entropy generation in a baffled L-shaped cavity. J. Mol. Liq. 2016, 223, 243–251. [Google Scholar] [CrossRef]
- Jmai, R.; Ben-Beya, B.; Lili, T. Numerical analysis of mixed convection at various walls speed ratios in two-sided lid-driven cavity partially heated and filled with nanofluid. J. Mol. Liq. 2016, 221, 691–713. [Google Scholar] [CrossRef]
- Kareem, A.K.; Gao, S.; Ahmed, A.Q. Unsteady simulations of mixed convection heat transfer in a 3D closed lid-driven cavity. Int. J. Heat Mass Transf. 2016, 100, 121–130. [Google Scholar] [CrossRef]
- Kareem, A.K.; Mohammed, H.A.; Hussein, A.K.; Gao, S. Numerical investigation of mixed convection heat transfer of nanofluids in a lid-driven trapezoidal cavity. Int. Commun. Heat Mass Transf. 2016, 77, 195–205. [Google Scholar] [CrossRef]
- Mamourian, M.; Shirvan, K.M.; Ellahi, R.; Rahimi, A.B. Optimization of mixed convection heat transfer with entropy generation in a wavy surface square lid-driven cavity by means of Taguchi approach. Int. J. Heat Mass Transf. 2016, 102, 544–554. [Google Scholar] [CrossRef]
- Mejri, I.; Mahmoudi, A.; Abbassi, M.A.; Omri, A. LBM simulation of natural convection in an inclined triangular cavity filled with water. Alex. Eng. J. 2016, 55, 1385–1394. [Google Scholar] [CrossRef]
- Rahmati, A.R.; Roknabadi, A.R.; Abbaszadeh, M. Numerical simulation of mixed convection heat transfer of nanofluid in a double lid-driven cavity using lattice Boltzmann method. Alex. Eng. J. 2016, 55, 3101–3114. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Analysis of MHD mixed convection in a flexible walled and nanofluids filled lid-driven cavity with volumetric heat generation. Int. J. Mech. Sci. 2016, 118, 113–124. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Natural convection in a flexible sided triangular cavity with internal heat generation under the effect of inclined magnetic field. J. Magn. Magn. Mater. 2016, 417, 327–337. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F.; Chamkha, A.J. MHD mixed convection and entropy generation of nanofluid filled lid driven cavity under the influence of inclined magnetic fields imposed to its upper and lower diagonal triangular domains. J. Magn. Magn. Mater. 2016, 406, 266–281. [Google Scholar] [CrossRef]
- Sojoudi, A.; Saha, S.C.; Sefidan, A.M.; Gu, Y.T. Natural convection subject to sinusoidal thermal forcing on inclined walls and heat source located on bottom wall of an attic-shaped space. Energy Build. 2016, 128, 845–866. [Google Scholar] [CrossRef]
- Sojoudi, A.; Saha, S.C.; Xu, F.; Gu, Y.T. Transient air flow and heat transfer due to differential heating on inclined walls and heat source placed on the bottom wall in a partitioned attic shaped space. Energy Build. 2016, 113, 39–50. [Google Scholar] [CrossRef]
- Aparna, K.; Seetharamu, K.N. Investigations on the effect of non-uniform temperature on fluid flow and heat transfer in a trapezoidal cavity filled with porous media. Int. J. Heat Mass Transf. 2017, 108, 63–78. [Google Scholar] [CrossRef]
- Alsabery, A.I.; Chamkha, A.J.; Saleh, H.; Hashim, I. Transient natural convective heat transfer in a trapezoidal cavity filled with non-Newtonian nanofluid with sinusoidal boundary conditions on both sidewalls. Powder Technol. 2017, 308, 214–234. [Google Scholar] [CrossRef]
- Al-Weheibi, S.M.; Rahman, M.M.; Alam, M.S.; Vajravelu, K. Numerical simulation of natural convection heat transfer in a trapezoidal enclosure filled with nanoparticles. Int. J. Mech. Sci. 2017, 131–132, 599–612. [Google Scholar] [CrossRef]
- Balla, C.S.; Kishan, N.; Gorla, R.S.R.; Gireesha, B.J. MHD boundary layer flow and heat transfer in an inclined porous square cavity filled with nanofluids. Ain Shams Eng. J. 2017, 8, 237–254. [Google Scholar] [CrossRef]
- Cui, H.; Xu, F.; Saha, S.C. Transition to unsteady natural convection flow in a prismatic enclosure of triangular section. Int. J. Therm. Sci. 2017, 111, 330–339. [Google Scholar] [CrossRef]
- Das, D.; Lukose, L.; Basak, T. Role of finite element based grids and simulations on evaluation of Nusselt numbers for heatfunctions within square and triangular cavities involving multiple discrete heaters. Int. Commun. Heat Mass Transf. 2017, 89, 39–46. [Google Scholar] [CrossRef]
- Gangawane, K.M. Computational analysis of mixed convection heat transfer characteristics in lid-driven cavity containing triangular block with constant heat flux: Effect of Prandtl and Grashof numbers. Int. J. Heat Mass Transf. 2017, 105, 34–57. [Google Scholar] [CrossRef]
- Ghalambaz, M.; Jamesahar, E.; Ismael, M.A.; Chamkha, A.J. Fluid-structure interaction study of natural convection heat transfer over a flexible oscillating fin in a square cavity. Int. J. Therm. Sci. 2017, 111, 256–273. [Google Scholar] [CrossRef]
- Gibanov, N.S.; Sheremet, M.A.; Oztop, H.F.; Al-Salem, K. Convective heat transfer in a lid-driven cavity with a heat-conducting solid backward step under the effect of buoyancy force. Int. J. Heat Mass Transf. 2017, 112, 158–168. [Google Scholar] [CrossRef]
- Gibanov, N.S.; Sheremet, M.A.; Oztop, H.F.; Abu-Hamdeh, N. Effect of uniform inclined magnetic field on mixed convection in a lid-driven cavity having a horizontal porous layer saturated with a ferrofluid. Int. J. Heat Mass Transf. 2017, 114, 1086–1097. [Google Scholar] [CrossRef]
- Hammami, F.; Souayeh, B.; Ben-Cheikh, N.; Ben-Beya, B. Computational analysis of fluid flow due to a two-sided lid driven cavity with a circular cylinder. Comput. Fluids 2017, 156, 317–328. [Google Scholar] [CrossRef]
- Hatami, M.; Zhou, J.; Geng, J.; Song, D.; Jing, D. Optimization of a lid-driven T-shaped porous cavity to improve the nanofluids mixed convection heat transfer. J. Mol. Liq. 2017, 231, 620–631. [Google Scholar] [CrossRef]
- Hussain, S.; Ahmad, S.; Mehmood, K.; Sagheer, M. Effects of inclination angle on mixed convective nanofluid flow in a double lid-driven cavity with discrete heat sources. Int. J. Heat Mass Transf. 2017, 106, 847–860. [Google Scholar] [CrossRef]
- Javed, T.; Mehmood, Z.; Siddiqui, M.A.; Pop, I. Study of heat transfer in water-Cu nanofluid saturated porous medium through two entrapped trapezoidal cavities under the influence of magnetic field. J. Mol. Liq. 2017, 240, 402–411. [Google Scholar] [CrossRef]
- Kareem, A.K.; Gao, S. Mixed convection heat transfer of turbulent flow in a three-dimensional lid-driven cavity with a rotating cylinder. Int. J. Heat Mass Transf. 2017, 112, 185–200. [Google Scholar] [CrossRef]
- Khanafer, K.; Aithal, S.M. Mixed convection heat transfer in a lid-driven cavity with a rotating circular cylinder. Int. Commun. Heat Mass Transf. 2017, 86, 131–142. [Google Scholar] [CrossRef]
- Khatamifar, M.; Lin, W.; Armfield, S.W.; Holmes, D.; Kirkpatrick, M.P. Conjugate natural convection heat transfer in a partitioned differentially-heated square cavity. Int. Commun. Heat Mass Transf. 2017, 81, 92–103. [Google Scholar] [CrossRef]
- Mojumder, S.; Saha, S.; Rahman, M.R.; Rahman, M.M.; Rabbi, K.M.; Ibrahim, T.A. Numerical study on mixed convection heat transfer in a porous L-shaped cavity. Eng. Sci. Technol. Int. J. 2017, 20, 272–282. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F.; Chamkha, A.J. Fluid–structure-magnetic field interaction in a nanofluid filled lid-driven cavity with flexible side wall. Eur. J. Mech. B Fluid. 2017, 61, 77–85. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F.; Chamkha, A.J. Analysis of mixed convection of nanofluid in a 3D lid-driven trapezoidal cavity with flexible side surfaces and inner cylinder. Int. Commun. Heat Mass Transf. 2017, 87, 40–51. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Mixed convection in a partially heated triangular cavity filled with nanofluid having a partially flexible wall and internal heat generation. J. Taiwan Inst. Chem. Eng. 2017, 70, 168–178. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Revnic, C.; Pop, I. Natural convective heat transfer through two entrapped triangular cavities filled with a nanofluid: Buongiorno’s mathematical model. Int. J. Mech. Sci. 2017, 133, 484–494. [Google Scholar] [CrossRef]
- Aghakhani, S.; Pordanjani, A.H.; Karimipour, A.; Abdollahi, A.; Afrand, M. Numerical investigation of heat transfer in a power-law non-Newtonian fluid in a C-Shaped cavity with magnetic field effect using finite difference lattice Boltzmann method. Comput. Fluid. 2018, 176, 51–67. [Google Scholar] [CrossRef]
- Astanina, M.S.; Sheremet, M.A.; Oztop, H.F. MHD natural convection and entropy generation of ferrofluid in an open trapezoidal cavity partially filled with a porous medium. Int. J. Mech. Sci. 2018, 48, 326–337. [Google Scholar] [CrossRef]
- Alsabery, A.I.; Ismael, M.A.; Chamkha, A.J.; Hashim, I. Mixed convection of Al2O3-water nanofluid in a double lid-driven square cavity with a solid inner insert using Buongiorno’s two-phase model. Int. J. Heat Mass Transf. 2018, 119, 939–961. [Google Scholar] [CrossRef]
- Balootaki, A.; Karimipour, A.; Toghraie, D. Nano scale lattice Boltzmann method to simulate the mixed convection heat transfer of air in a lid-driven cavity with an endothermic obstacle inside. Phys. A Stat. Mech. Its Appl. 2018, 508, 681–701. [Google Scholar]
- Bhowmick, S.; Xu, F.; Zhang, X.; Saha, S.C. Natural convection and heat transfer in a valley shaped cavity filled with initially stratified water. Int. J. Therm. Sci. 2018, 128, 59–69. [Google Scholar] [CrossRef]
- Cho, C.-C. Heat transfer and entropy generation of mixed convection flow in Cu-water nanofluid-filled lid-driven cavity with wavy surface. Int. J. Heat Mass Transf. 2018, 119, 163–174. [Google Scholar] [CrossRef]
- Gangawane, K.M.; Oztop, H.F.; Abu-Hamdeh, N. Mixed convection characteristic in a lid-driven cavity containing heated triangular block: Effect of location and size of block. Int. J. Heat Mass Transf. 2018, 124, 860–875. [Google Scholar] [CrossRef]
- Gibanov, N.S.; Sheremet, M.A.; Oztop, H.F.; Abu-Hamdeh, N. Mixed convection with entropy generation of nanofluid in a lid-driven cavity under the effects of a heat-conducting solid wall and vertical temperature gradient. Eur. J. Mech. B Fluid. 2018, 70, 148–159. [Google Scholar] [CrossRef]
- Hussain, S.; Öztop, H.F.; Mehmood, K.; Abu-Hamdeh, N. Effects of inclined magnetic field on mixed convection in a nanofluid filled double lid-driven cavity with volumetric heat generation or absorption using finite element method. Chin. J. Phys. 2018, 56, 484–501. [Google Scholar] [CrossRef]
- Javed, T.; Siddiqui, M.A. Effect of MHD on heat transfer through ferrofluid inside a square cavity containing obstacle/heat source. Int. J. Therm. Sci. 2018, 125, 419–427. [Google Scholar] [CrossRef]
- Karbasifar, B.; Akbari, M.; Toghraie, D. Mixed convection of Water-Aluminum oxide nanofluid in an inclined lid-driven cavity containing a hot elliptical centric cylinder. Int. J. Heat Mass Transf. 2018, 116, 1237–1249. [Google Scholar] [CrossRef]
- Kareem, A.K.; Gao, S. A comparison study of mixed convection heat transfer of turbulent nanofluid flow in a three-dimensional lid-driven enclosure with a clockwise versus an anticlockwise rotating cylinder. Int. Commun. Heat Mass Transf. 2018, 90, 44–55. [Google Scholar] [CrossRef]
- Mikhailenko, S.A.; Sheremet, M.A.; Mohamad, A.A. Convective-radiative heat transfer in a rotating square cavity with a local heat-generating source. Int. J. Mech. Sci. 2018, 142–143, 530–540. [Google Scholar] [CrossRef]
- Oglakkaya, F.S.; Bozkaya, C. Unsteady MHD mixed convection flow in a lid-driven cavity with a heated wavy wall. Int. J. Mech. Sci. 2018, 148, 231–245. [Google Scholar] [CrossRef]
- Razera, A.L.; da Fonseca, R.J.C.; Isoldi, L.A.; dos Santos, E.D.; Rocha, L.A.O.; Biserni, C. Constructal design of a semi-elliptical fin inserted in a lid-driven square cavity with mixed convection. Int. J. Heat Mass Transf. 2018, 126, 81–94. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Modeling and optimization of MHD mixed convection in a lid-driven trapezoidal cavity filled with alumina–water nanofluid: Effects of electrical conductivity models. Int. J. Mech. Sci. 2018, 136, 264–278. [Google Scholar] [CrossRef]
- Sheikholeslami, M. Influence of magnetic field on Al2O3-H2O nanofluid forced convection heat transfer in a porous lid-driven cavity with hot sphere obstacle by means of LBM. J. Mol. Liq. 2018, 263, 472–488. [Google Scholar] [CrossRef]
- Sheremet, M.A.; Roşca, N.C.; Roşca, A.V.; Pop, I. Mixed convection heat transfer in a square porous cavity filled with a nanofluid with suction/injection effect. Comput. Math. Appl. 2018, 76, 2665–2677. [Google Scholar] [CrossRef]
- Taghizadeh, S.; Asaditaheri, A. Heat transfer and entropy generation of laminar mixed convection in an inclined lid-driven enclosure with a circular porous cylinder. Int. J. Therm. Sci. 2018, 134, 242–257. [Google Scholar] [CrossRef]
- Zhai, H.; Xu, F.; Hou, Y.; Saha, S.C. Natural Convection and Heat Transfer on a Section-Triangular Roof. Int. Commun. Heat Mass Transf. 2018, 92, 23–30. [Google Scholar] [CrossRef]
- Zhou, W.; Yan, Y.; Liu, X.; Chen, H.; Liu, B. Lattice Boltzmann simulation of mixed convection of nanofluid with different heat sources in a double lid-driven cavity. Int. Commun. Heat Mass Transf. 2018, 97, 39–46. [Google Scholar] [CrossRef]
- Alnaqi, A.A.; Aghakhani, S.; Pordanjani, A.H.; Bakhtiari, R.; Asadi, A.; Minh-Duc Tran, M.-D. Effects of magnetic field on the convective heat transfer rate and entropy generation of a nanofluid in an inclined square cavity equipped with a conductor fin: Considering the radiation effect. Int. J. Heat Mass Transf. 2019, 133, 256–267. [Google Scholar] [CrossRef]
- Al-Rashed, A.A.A.A.; Shahsavar, A.; Akbari, M.; Toghraie, D.; Akbari, M.; Afrand, M. Finite Volume Simulation of mixed convection in an inclined lid-driven cavity filled with nanofluids: Effects of a hot elliptical centric cylinder cavity angle and volume fraction of nanoparticles. Phys. A Stat. Mech. Its Appl. 2019, 527, 121122. [Google Scholar]
- Barnoon, P.; Toghraie, D.; Dehkordi, R.B.; Abed, H. MHD mixed convection and entropy generation in a lid-driven cavity with rotating cylinders filled by a nanofluid using two phase mixture model. J. Magn. Magn. Mater. 2019, 483, 224–248. [Google Scholar] [CrossRef]
- Bhowmick, S.; Saha, S.C.; Qiao, M.; Xu, F. Transition to a chaotic flow in a V-shaped triangular cavity heated from below. Int. J. Heat Mass Transf. 2019, 128, 76–86. [Google Scholar] [CrossRef]
- Cho, C.-C. Mixed convection heat transfer and entropy generation of Cu-water nanofluid in wavy-wall lid-driven cavity in presence of inclined magnetic field. Int. J. Mech. Sci. 2019, 151, 703–714. [Google Scholar] [CrossRef]
- Cui, H.; Xu, F.; Saha, S.C.; Liu, Q. Transient free convection heat transfer in a section-triangular prismatic enclosure with different aspect ratios. Int. J. Therm. Sci. 2019, 139, 282–291. [Google Scholar] [CrossRef]
- Hadavand, M.; Yousefzadeh, S.; Akbari, O.A.; Pourfattah, F.; Nguyen, H.M.; Asadi, A. A numerical investigation on the effects of mixed convection of Ag-water nanofluid inside a sim-circular lid-driven cavity on the temperature of an electronic silicon chip. Appl. Therm. Eng. 2019, 162, 114298. [Google Scholar] [CrossRef]
- Hamid, M.; Usman, M.; Khan, Z.H.; Haq, R.U.; Wang, W. Heat transfer and flow analysis of Casson fluid enclosed in a partially heated trapezoidal cavity. Int. Commun. Heat Mass Transf. 2019, 108, 104284. [Google Scholar] [CrossRef]
- Karatas, H.; Derbentli, T. Natural convection in differentially heated rectangular cavities with time-periodic boundary condition on one side. Int. J. Heat Mass Transf. 2019, 129, 224–237. [Google Scholar] [CrossRef]
- Lamarti, H.; Mahdaoui, M.; Bennacer, R.; Chahboun, A. Numerical simulation of mixed convection heat transfer of fluid in a cavity driven by an oscillating lid using lattice Boltzmann method. Int. J. Heat Mass Transf. 2019, 137, 615–629. [Google Scholar] [CrossRef]
- Louaraychi, A.; Lamsaadi, M.; Naïmi, M.; Harfi, H.E.; Kaddiri, M.; Raji, A.; Hasnaoui, M. Mixed convection heat transfer correlations in shallow rectangular cavities with single and double-lid driven boundaries. Int. J. Heat Mass Transf. 2019, 132, 394–406. [Google Scholar] [CrossRef]
- Mohebbi, R.; Izadi, M.; Sajjadi, H.; Delouei, A.A.; Sheremet, M.A. Examining of nanofluid natural convection heat transfer in a Γ-shaped enclosure including a rectangular hot obstacle using the lattice Boltzmann method. Phys. A Stat. Mech. Its Appl. 2019, 526, 120831. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. MHD mixed convection of nanofluid in a flexible walled inclined lid-driven L-shaped cavity under the effect of internal heat generation. Phys. A Stat. Mech. Its Appl. 2019, 534, 122144. [Google Scholar] [CrossRef]
- Abu-Hamdeh, N.H.; Oztop, H.F.; Alnefaie, K.A. A computational study on mixed convection in a porous media filled and partially heated lid-driven cavity with an open side. Alex. Eng. J. 2020, 59, 1735–1750. [Google Scholar] [CrossRef]
- Afrand, M.; Pordanjani, A.H.; Aghakhani, S.; Oztop, H.F.; Abu-Hamdeh, N. Free convection and entropy generation of a nanofluid in a tilted triangular cavity exposed to a magnetic field with sinusoidal wall temperature distribution considering radiation effects. Int. Commun. Heat Mass Transf. 2020, 112, 104507. [Google Scholar] [CrossRef]
- Alsabery, A.I.; Armaghani, T.; Chamkha, A.J.; Hashim, I. Two-phase nanofluid model and magnetic field effects on mixed convection in a lid-driven cavity containing heated triangular wall. Alex. Eng. J. 2020, 59, 129–148. [Google Scholar] [CrossRef]
- Alsabery, A.I.; Sheremet, M.A.; Chamkha, A.J.; Hashim, I. Energy transport of two-phase nanofluid approach inside a three-dimensional lid-driven cubic cavity containing solid cylinder and heat source. Chem. Eng. Process. Process Intensif. 2020, 154, 108010. [Google Scholar] [CrossRef]
- Bilal, S.; Mahmood, R.; Majeed, A.H.; Khan, I.; Nisar, K.S. Finite element method visualization about heat transfer analysis of Newtonian material in triangular cavity with square cylinder. J. Mater. Res. Technol. 2020, 9, 4904–4918. [Google Scholar] [CrossRef]
- Cho, C.-C. Effects of porous medium and wavy surface on heat transfer and entropy generation of Cu-water nanofluid natural convection in square cavity containing partially-heated surface. Int. Commun. Heat Mass Transf. 2020, 119, 104925. [Google Scholar] [CrossRef]
- Ganesh, N.V.; Javed, S.; Al-Mdallal, Q.M.; Kalaivanan, R.; Chamkha, A.J. Numerical study of heat generating γ Al2O3–H2O nanofluid inside a square cavity with multiple obstacles of different shapes. Heliyon 2020, 6, e05752. [Google Scholar] [CrossRef]
- Haq, R.U.; Soomro, F.A.; Wang, X.; Tlili, I. Partially heated lid-driven flow in a hexagonal cavity with inner circular obstacle via FEM. Int. Commun. Heat Mass Transf. 2020, 117, 104732. [Google Scholar] [CrossRef]
- Khan, Z.H.; Makinde, O.D.; Hamid, M.; Haq, R.U.; Khan, W.A. Hydromagnetic flow of ferrofluid in an enclosed partially heated trapezoidal cavity filled with a porous medium. J. Magn. Magn. Mater. 2020, 499, 166241. [Google Scholar] [CrossRef]
- Li, Z.; Shahsavar, A.; Niazi, K.; Al-Rashed, A.A.A.A.; Talebizadehsardari, P. The effects of vertical and horizontal sources on heat transfer and entropy generation in an inclined triangular enclosure filled with non-Newtonian fluid and subjected to magnetic field. Powder Technol. 2020, 364, 924–942. [Google Scholar] [CrossRef]
- Liu, Y.; Huang, H. Effect of three modes of linear thermal forcing on convective flow and heat transfer in rectangular cavities. Int. J. Heat Mass Transf. 2020, 147, 118951. [Google Scholar] [CrossRef]
- Rammane, M.; Mesmoudi, S.; Tri, A.; Braikat, B.; Damil, N. Solving the incompressible fluid flows by a high-order mesh-free approach. Int. J. Numer. Method. Fluid. 2020, 92, 422–435. [Google Scholar] [CrossRef]
- Saha, S.C.; Sefidan, A.M.; Sojoudi, A. Unsteady natural convection within an attic-shaped space subject to sinusoidal heat flux on inclined walls. Energy Eng. 2020, 117, 1–17. [Google Scholar]
- Selimefendigil, F.; Öztop, H.F. Magnetohydrodynamics forced convection of nanofluid in multi-layered U-shaped vented cavity with a porous region considering wall corrugation effects. Int. Commun. Heat Mass Transf. 2020, 113, 104551. [Google Scholar] [CrossRef]
- Soomro, F.A.; Haq, R.U.; Algehyne, E.A.; Tlili, I. Thermal performance due to magnetohydrodynamics mixed convection flow in a triangular cavity with circular obstacle. J. Energy Storage 2020, 31, 101702. [Google Scholar] [CrossRef]
- Thiers, N.; Gers, R.; Skurtys, O. Heat transfer enhancement by localised time varying thermal perturbations at hot and cold walls in a rectangular differentially heated cavity. Int. J. Therm. Sci. 2020, 151, 106245. [Google Scholar] [CrossRef]
- Aljabair, S.; Ekaid, A.L.; Ibrahim, S.H.; Alesbe, I. Mixed convection in sinusoidal lid-driven cavity with non-uniform temperature distribution on the wall utilizing nanofluid. Heliyon 2021, 7, e06907. [Google Scholar] [CrossRef] [PubMed]
- Alsabery, A.I.; Tayebi, T.; Kadhim, H.T.; Ghalambaz, M.; Hashim, I.; Chamkha, A.J. Impact of two-phase hybrid nanofluid approach on mixed convection inside wavy lid-driven cavity having localized solid block. J. Adv. Res. 2021, 30, 63–74. [Google Scholar] [CrossRef] [PubMed]
- Çolak, E.; Ekici, O.; Öztop, H.F. Mixed convection in a lid-driven cavity with partially heated porous block. Int. Commun. Heat Mass Transf. 2021, 126, 105450. [Google Scholar] [CrossRef]
- Eshaghi, S.; Izadpanah, F.; Dogonchi, A.S.; Chamkha, A.J.; Hamida, M.B.B.; Alhumade, H. The optimum double-diffusive natural convection heat transfer in H-Shaped cavity with a baffle inside and a corrugated wall. Case Stud. Therm. Eng. 2021, 28, 101541. [Google Scholar] [CrossRef]
- Fayz-Al-Asad, M.; Alam, M.N.; Ahmad, H.; Sarker, M.M.A.; Alsulami, M.D.; Gepreel, K.A. Impact of a closed space rectangular heat source on natural convective flow through the triangular cavity. Results Phys. 2021, 23, 104011. [Google Scholar] [CrossRef]
- Hasnaoui, S.; Amahmid, A.; Raji, A.; Beji, H.; Mansouri, A.E.; Hasnaoui, M. LBM simulation of stabilizing/destabilizing effects of thermodiffusion and heat generation in a rectangular cavity filled with a binary mixture. Int. Commun. Heat Mass Transf. 2021, 126, 105417. [Google Scholar] [CrossRef]
- Hussain, S.; Jamal, M.; Geridonmez, B.P. Impact of fins and inclined magnetic field in double lid-driven cavity with Cu–water nanofluid. Int. J. Therm. Sci. 2021, 61, 106707. [Google Scholar] [CrossRef]
- Hostos, J.C.A.; Bove, J.C.S.; Cruchaga, M.A.; Fachinotti, V.D.; Agelvis, R.A.M. Solving steady-state lid-driven square cavity flows at high Reynolds numbers via a coupled improved element-free Galerkin–reduced integration penalty method. Comput. Math. Appl. 2021, 99, 211–228. [Google Scholar] [CrossRef]
- Ibrahim, W.; Hirpho, M. Finite element analysis of mixed convection flow in a trapezoidal cavity with non-uniform temperature. Heliyon 2021, 7, e05933. [Google Scholar] [CrossRef]
- Ikram, M.M.; Saha, G.; Saha, S.C. Conjugate forced convection transient flow and heat transfer analysis in a hexagonal partitioned, air filled cavity with dynamic modulator. Int. J. Heat Mass Transf. 2021, 167, 120786. [Google Scholar] [CrossRef]
- Joe, E.S.; Perumal, D.A. Computational analysis of nonhomogeneous fluid flow in a two-cylinder-driven rectangular cavity. Appl. Eng. Sci. 2021, 7, 100064. [Google Scholar] [CrossRef]
- Mondal, P.; Mahapatra, T.R. MHD double-diffusive mixed convection and entropy generation of nanofluid in a trapezoidal cavity. Int. J. Mech. Sci. 2021, 208, 106665. [Google Scholar] [CrossRef]
- Mebarek-Oudina, F.; Fares, R.; Aissa, A.; Lewis, R.W.; Abu-Hamdeh, N.H. Entropy and convection effect on magnetized hybrid nano-liquid flow inside a trapezoidal cavity with zigzagged wall. Int. Commun. Heat Mass Transf. 2021, 125, 105279. [Google Scholar] [CrossRef]
- Saha, S.; Biswas, P.; Das, A.N.; Raut, S. Analysis of heat transfer characteristics through an rectangular enclosure. Mater. Today Proc. 2021, 47, 2905–2911. [Google Scholar] [CrossRef]
- Saha, S.C.; Sefidan, A.M.; Sojoudi, A.; Molla, M.M. Transient free convection and heat transfer in a partitioned attic-shaped space under diurnal thermal forcing. Energy Eng. 2021, 118, 487–506. [Google Scholar] [CrossRef]
- Shah, S.S.; Haq, R.U.; Al-Kouz, W. Mixed convection analysis in a split lid-driven trapezoidal cavity having elliptic shaped obstacle. Int. Commun. Heat Mass Transf. 2021, 126, 105448. [Google Scholar] [CrossRef]
- Shahid, H.; Yaqoob, I.; Khan, W.A.; Rafique, A. Mixed convection in an isosceles right triangular lid driven cavity using multi relaxation time lattice Boltzmann method. Int. Commun. Heat Mass Transf. 2021, 128, 105552. [Google Scholar] [CrossRef]
- Shahid, H.; Yaqoob, I.; Khan, W.A.; Aslam, M. Multi relaxation time Lattice Boltzmann analysis of lid-driven rectangular cavity subject to various obstacle configurations. Int. Commun. Heat Mass Transf. 2021, 129, 105658. [Google Scholar] [CrossRef]
- Shekaramiz, M.; Fathi, S.; Ataabadi, H.A.; Kazemi-Varnamkhasti, H.; Toghraie, D. MHD nanofluid free convection inside the wavy triangular cavity considering periodic temperature boundary condition and velocity slip mechanisms. Int. J. Therm. Sci. 2021, 170, 107179. [Google Scholar] [CrossRef]
- Tizakast, Y.; Kaddiri, M.; Lamsaadi, M. Double-diffusive mixed convection in rectangular cavities filled with non-Newtonian fluids. Int. J. Mech. Sci. 2021, 208, 106667. [Google Scholar] [CrossRef]
- Velkennedy, R.; Nisrin, J.J.; Kalidasan, K.; Rajeshkanna, P. Numerical investigation of convective heat transfer in a rectangular vented cavity with two outlets and cold partitions. Int. Commun. Heat Mass Transf. 2021, 129, 105659. [Google Scholar] [CrossRef]
- Xiong, P.-Y.; Hamid, A.; Iqbal, K.; Irfan, M.; Khan, M. Numerical simulation of mixed convection flow and heat transfer in the lid-driven triangular cavity with different obstacle configurations. Int. Commun. Heat Mass Transf. 2021, 123, 105202. [Google Scholar] [CrossRef]
- Abbas, S.Z.; Wang, X.; Khan, W.A.; Hobiny, A.; Iqbal, K. Finite element analysis of nanofluid flow and heat transfer in a square cavity with two circular obstacles at different positions in the presence of magnetic field. J. Energy Storage 2022, 51, 104462. [Google Scholar] [CrossRef]
- Ahmed, S.; Xu, H.; Zhou, Y.; Qiang Yu, Q. Modelling convective transport of hybrid nanofluid in a lid-driven square cavity with consideration of Brownian diffusion and thermophoresis. Int. Commun. Heat Mass Transf. 2022, 137, 106226. [Google Scholar] [CrossRef]
- Ali, M.M.; Akhter, R.; Alim, M.A. Magneto-mixed convection in a lid driven partially heated cavity equipped with nanofluid and rotating flat plate. Alex. Eng. J. 2022, 61, 257–278. [Google Scholar] [CrossRef]
- Acharya, N.; Chamkha, A.J. On the magnetohydrodynamic Al2O3-water nanofluid flow through parallel fins enclosed inside a partially heated hexagonal cavity. Int. Commun. Heat Mass Transf. 2022, 132, 105885. [Google Scholar] [CrossRef]
- Batool, S.; Rasool, G.; Alshammari, N.; Khan, I.; Kaneez, H.; Hamadneh, N. Numerical analysis of heat and mass transfer in micropolar nanofluids flow through lid-driven cavity: Finite volume approach. Case Stud. Therm. Eng. 2022, 37, 102233. [Google Scholar] [CrossRef]
- Charqui, Z.; Moutaouakil, L.E.; Boukendil, M.; Hidki, R. Numerical study of heat transfer in a tall, partitioned cavity confining two different fluids: Application to the water Trombe wall. Int. J. Therm. Sci. 2022, 171, 107266. [Google Scholar] [CrossRef]
- Cui, H.; Wang, W.; Xu, F.; Saha, S.C.; Liu, Q. Transitional free convection flow and heat transfer within attics in cold climate. Therm. Sci. 2022, 26, 4699–4709. [Google Scholar] [CrossRef]
- Dahani, Y.; Hasnaoui, M.; Amahmid, A.; Hasnaoui, S. A Multiple-Relaxation-Time lattice Boltzmann analysis of coupled mixed convection and radiation effect in a tilted two-sided lid-driven enclosure. Chem. Phys. Lett. 2022, 791, 139386. [Google Scholar] [CrossRef]
- Hirpho, M.; Ibrahim, W. Modeling and simulation of hybrid Casson nanofluid mixed convection in a partly heated trapezoidal enclosure. Int. J. 2022, 15, 100166. [Google Scholar] [CrossRef]
- Khalil, W.H.; Azzawi, I.D.J.; Al-damook, A. The optimisation of MHD free convection inside porous trapezoidal cavity with the wavy bottom wall using response surface method. Int. Commun. Heat Mass Transf. 2022, 134, 106035. [Google Scholar] [CrossRef]
- Liu, Y. Free convection and heat transfer characteristics in differentially heated finned cavities with inclination. Int. J. Therm. Sci. 2022, 182, 107729. [Google Scholar] [CrossRef]
- Noor, N.F.M.; Haq, R.U.; Wong, H.F.; Alzahrani, A.K.; Ullah, M.Z. Flow and heat transfer due to partially heated moving lid in a trapezoidal cavity with different constraints at inner circular obstacle. Int. Commun. Heat Mass Transf. 2022, 135, 106111. [Google Scholar] [CrossRef]
- Nouraei, S.; Anvari, A.; Abed, A.M.; Akbari, O.A.; Montazerifar, F.; Baghaei, S.; Fatholahi, M. Heat transfer and entropy analysis for nanofluid flow in a semi-circular open cavity under mixed convection. Alex. Eng. J. 2022, 64, 309–334. [Google Scholar] [CrossRef]
- Polasanapalli, S.R.G.; Anupindi, K. Mixed convection heat transfer in a two-dimensional annular cavity using an off-lattice Boltzmann method. Int. J. Therm. Sci. 2022, 179, 107677. [Google Scholar] [CrossRef]
- Prince, H.A.; Rozin, E.H.; Sagor, M.J.H.; Saha, S. Evaluation of overall thermal performance for conjugate natural convection in a trapezoidal cavity with different surface corrugations. Int. Commun. Heat Mass Transf. 2022, 130, 105772. [Google Scholar] [CrossRef]
- Rahaman, M.M.; Bhowmick, S.; Mondal, R.N.; Saha, S.C. Unsteady natural convection in an initially stratified air-filled trapezoidal enclosure heated from below. Processes 2022, 10, 1383. [Google Scholar] [CrossRef]
- Roy, N.C.; Amin, R.; Ishak, A. Magnetohydrodynamic Natural Convective Hybrid Nanofluid Flow in a Square Enclosure with Different Blocks. Iran. J. Sci. Technol. Trans. Mech. Eng. 2022. [Google Scholar] [CrossRef]
- Shah, S.S.; Haq, R.U.; McCash, L.B.; Bahaidarah, H.M.S.; Aziz, T. Numerical simulation of lid driven flow in a curved corrugated porous cavity filled with CuO-water in the presence of heat generation/absorption. Alex. Eng. J. 2022, 61, 2749–2767. [Google Scholar] [CrossRef]
- Tizakast, Y.; Kaddiri, M.; Lamsaadi, M. Rayleigh-Bénard double-diffusive mixed convection in two-dimensional rectangular cavities filled with non-Newtonian fluids. Int. J. Mech. Sci. 2022, 227, 107448. [Google Scholar] [CrossRef]
- Xia, S.; Mostafavi, M.; Alghazali, T.; Sadi, S.; Guerrero, J.W.G.; Suksatan, W.; Toghraie, D.; Khan, A. Numerical investigation of nanofluid mixed convection in a T-shaped cavity by considering a thermal barrier. Alex. Eng. J. 2022, 61, 7393–7415. [Google Scholar] [CrossRef]
- Zarei, M.S.; Abad, A.T.K.; Hekmatifar, M.; Toghraie, D. Heat transfer in a square cavity filled by nanofluid with sinusoidal wavy walls at different wavelengths and amplitudes. Case Stud. Therm. Eng. 2022, 34, 101970. [Google Scholar] [CrossRef]
- Zhang, H.; Nie, X.; Bokov, D.O.; Toghraie, D.; Akbari, O.A.; Montazerifar, F.; Pourfattah, F.; Esmaeili, Y.; Khodaparast, R. Numerical study of mixed convection and entropy generation of Water-Ag nanofluid filled semi-elliptic lid-driven cavity. Alex. Eng. J. 2022, 61, 8875–8896. [Google Scholar] [CrossRef]
- He, W.; Zhuang, Y.; Chen, Y.; Wang, C. Experimental and numerical investigations on the melting behavior of Fe3O4 nanoparticles composited paraffin wax in a cubic cavity under a magnetic-field. Int. J. Therm. Sci. 2023, 184, 107961. [Google Scholar] [CrossRef]
- Ikram, M.M.; Saha, G.; Saha, S.C. Unsteady Conjugate Heat Transfer Characteristics in Hexagonal Cavity Equipped with a Multi-Blade Dynamic Modulator. Int. J. Heat Mass Transf. 2023, 200C, 123527. [Google Scholar] [CrossRef]
- Ouri, H.; Selimefendigil, F.; Bouterra, M.; Omri, M.; Alshammari, B.M.; Kolsi, L. MHD hybrid nanofluid convection and phase change process in an L-shaped vented cavity equipped with an inner rotating cylinder and PCM-packed bed system. Alex. Eng. J. 2023, 63, 563–582. [Google Scholar] [CrossRef]
- Sayed, M.A.; Hadžiabdić, M.; Dehbi, A.; Ničeno, B.; Mikityuk, K. Simulation of flow and heat transfer in a differentially heated cubical cavity using coarse Large Eddy Simulation. Int. J. Therm. Sci. 2023, 183, 107892. [Google Scholar] [CrossRef]
- Iwatsu, R.; Hyun, J.M.; Kuwahara, K. Mixed convection in a driven cavity with a stable vertical temperature gradient. Int. J. Heat Mass Transf. 1993, 36, 1601–1608. [Google Scholar] [CrossRef]
- Khanafer, K.M.; Chamkha, A.J. Mixed convection flow in a lid-driven enclosure filled with a fluid-saturated porous medium. Int. J. Heat Mass Transf. 1999, 42, 2465–2481. [Google Scholar] [CrossRef]
- Pekmen, B.; Tezer-Sezgin, M. MHD flow and heat transfer in a lid-driven porous enclosure. Comput. Fluid. 2014, 89, 191–199. [Google Scholar] [CrossRef]
- Selimefendigil, F.; Öztop, H.F. Mixed convection of ferrofluids in a lid driven cavity with two rotating cylinders. Eng. Sci. Technol. Int. J. 2015, 18, 439–451. [Google Scholar] [CrossRef]
- Sharif, M.A.R. Laminar mixed convection in shallow inclined driven cavities with hot moving lid on top and cooled from bottom. Appl. Therm. Eng. 2007, 27, 1036–1042. [Google Scholar] [CrossRef]
- Al-Balushi, L.M.; Uddin, M.J.; Rahman, M.M. Natural convective heat transfer in a square enclosure utilizing magnetic nanoparticles. Propuls. Power Res. 2019, 8, 194–209. [Google Scholar] [CrossRef]
- Anandalakshmi, R.; Basak, T. Heat flow visualization for natural convection in rhombic enclosures due to isothermal and non-isothermal heating at the bottom wall. Int. J. Heat Mass Transf. 2012, 55, 1325–1342. [Google Scholar] [CrossRef]
- Ashrafizadeh, A.; Nikfar, M. On the numerical solution of generalized convection heat transfer problems via the method of proper closure equations–Part II: Application to test problems. Numerical Heat Transfer. Part B 2016, 70, 204–222. [Google Scholar] [CrossRef]
- Barakos, G.; Mitsoulis, E.; Assimacopoulos, D. Natural convection flow in a square cavity revisited: Laminar and turbulent models with wall functions. Numer. Method. Fluid. 1994, 18, 695–719. [Google Scholar] [CrossRef]
- Biswas, N.; Chamkha, A.J.; Manna, N.K. Energy-saving method of heat transfer enhancement during magneto-thermal convection in typical thermal cavities adopting aspiration. SN Appl. Sci. 2020, 2, 1911. [Google Scholar] [CrossRef]
- Bouabid, M.; Hidouri, N.; Magherbi, M.; Brahim, A.B. Analysis of the magnetic field effect on entropy generation at thermosolutal convection in a square cavity. Entropy 2011, 13, 1034–1054. [Google Scholar] [CrossRef]
- Choi, Y.J. A Numerical Study on a Lumped-Parameter Model and a CFD Code Coupling for the Analysis of the Loss of Coolant Accident in a Reactor Containment. Ph.D. Thesis, Universit’e de Marne-la-Vall´ee, Paris, France, 2005. [Google Scholar]
- Chenoweth, D.R.; Paolucci, S. Natural convection in an enclosed vertical air layer with large horizontal temperature differences. J. Fluid Mech. 1986, 169, 173–210. [Google Scholar] [CrossRef]
- Corzo, S.F.; Damian, S.M.; Ramajo, D.; Nigro, N.M. Numerical simulation of natural convection phenomena. Mec. Comput. 2011, 30, 277–296. [Google Scholar]
- Deng, Q.H.; Tang, G.F. Numerical visualization of mass and heat transport for conjugate natural convection/heat conduction by streamline and heatline. Int. J. Heat Mass Transf. 2002, 45, 2373–2385. [Google Scholar] [CrossRef]
- de Vahl Davis, G. Natural convection of air in a square cavity: A benchmark numerical solutions. Int. J. Numer. Method. Fluid. 1983, 3, 249–264. [Google Scholar] [CrossRef]
- D’Orazio, A.; Corcione, M.; Celata, G.P. Application to natural convection enclosed flows of a lattice Boltzmann BGK model coupled with a general purpose thermal boundary condition. Int. J. Therm. Sci. 2004, 43, 575–586. [Google Scholar] [CrossRef]
- Dixit, H.N.; Babu, V. Simulation of high Rayleigh number natural convection in a square cavity using the lattice Boltzmann method. Int. J. Heat Mass Transf. 2006, 49, 727–739. [Google Scholar] [CrossRef]
- Djebali, R.; Ganaoui, M.E.l.; Sammouda, H.; Bennacer, R. Some benchmarks of a side wall heated cavity using Lattice Boltzmann approach. Fluid Dyn. Mater. Process. 2009, 5, 261–282. [Google Scholar]
- Elatar, A.; Teamah, M.A.; Hassab, M.A. Numerical study of laminar natural convection inside square enclosure with single horizontal fin. Int. J. Therm. Sci. 2016, 99, 41–51. [Google Scholar] [CrossRef]
- El-Gendi, M.M. Numerical simulation of unsteady natural convection flow inside a pattern of connected open square cavities. Int. J. Therm. Sci. 2018, 127, 373–383. [Google Scholar] [CrossRef]
- Fu, C.; Rahmani, A.; Suksatan, W.; Alizadeh, S.M.; Zarringhalam, M.; Chupradit, S.; Toghraie, D. Comprehensive investigations of mixed convection of Fe–ethylene-glycol nanofluid inside an enclosure with different obstacles using lattice Boltzmann method. Sci. Rep. 2021, 11, 20710. [Google Scholar] [CrossRef] [PubMed]
- Fusegi, T.; Kuwahara, K.; Farouk, B. A numerical study of three-dimensional natural convection in a differentially heated cubic enclosure. Int. J. Heat Mass Transf. 1991, 34, 1543–1557. [Google Scholar] [CrossRef]
- Ghasemi, B.; Aminossadati, S.M.; Raisi, A. Magnetic field effect on natural convection in a nanofluid-filled square enclosure. Int. J. Therm. Sci. 2011, 50, 1748–1756. [Google Scholar] [CrossRef]
- Ha, M.Y.; Jung, M.J. A numerical study of three-dimensional conjugate heat transfer of natural convection and conduction in a differentially heated cubic enclosure with a heat-generating cubic conducting body. Int. J. Heat Mass Transf. 2000, 43, 4229–4248. [Google Scholar] [CrossRef]
- Hadjisophocleous, G.V.; Sousa, A.C.M.; Venart, J.E.S. Prediction of the transient natural convection in enclosures of arbitrary geometry using a nonorthogonal numerical model. Numer. Heat Transf. Part A 1988, 13, 373–392. [Google Scholar]
- Ho, C.J.; Lin, F.H. Simulation of natural convection in a vertical enclosure by using a new incompressible flow formulation-pseudovorticity-velocity formulation. Numer. Heat Transf. Part A Appl. 1997, 31, 881–896. [Google Scholar] [CrossRef]
- Islam, T.; Alam, M.N.; Asjad, M.I.; Parveen, N.; Chu, Y.-M. Heatline visualization of MHD natural convection heat transfer of nanofluid in a prismatic enclosure. Sci. Rep. 2021, 11, 10972. [Google Scholar] [CrossRef] [PubMed]
- Kalita, J.C.; Dalal, D.C.; Dass, A.K. Fully compact higher-order computation of steady-state natural convection in a square cavity. Phys. Rev. E 2001, 64, 066703. [Google Scholar] [CrossRef]
- Karki, P.; Yadav, A.K.; Perumal, D.A. Study of adiabatic obstacles on natural convection in a square cavity using Lattice Boltzmann method. J. Therm. Sci. Eng. Appl. 2019, 11, 034502. [Google Scholar] [CrossRef]
- Kahveci, K. A differential quadrature solution of natural convection in an enclosure with a finite-thickness partition. Numer. Heat Transf. Part A Appl. 2007, 51, 979–1002. [Google Scholar] [CrossRef]
- Kahveci, K. Buoyancy driven heat transfer of nanofluids in a tilted enclosure. ASME J. Heat Transf. 2010, 132, 062501. [Google Scholar] [CrossRef]
- Khanafer, K.; Vafai, K.; Lightstone, M. Buoyancy-driven heat transfer enhancement in a two-dimensional enclosure utilizing nanofluids. Int. J. Heat Mass Transf. 2003, 46, 3639–3653. [Google Scholar] [CrossRef]
- Khatamifar, M.; Lin, W.W.; Dong, L. Transient conjugate natural convection heat transfer in a differentially-heated square cavity with a partition of finite thickness and thermal conductivity. Case Stud. Therm. Eng. 2021, 25, 100952. [Google Scholar] [CrossRef]
- Kocutar, P.; Skerget, L.; Ravnik, J. Hybrid les/urans simulation of turbulent natural convection by bem. Eng. Anal. Bound. Elem. 2015, 61, 16–26. [Google Scholar] [CrossRef]
- Kuznik, F.; Vareilles, J.; Rusaouen, G.; Krauss, G. A double-population lattice Boltzmann method with non-uniform mesh for the simulation of natural convection in a square cavity. Int. J. Heat Fluid Flow 2007, 28, 862–870. [Google Scholar] [CrossRef]
- Leal, M.A.; Machado, H.A.; Cotta, R.M. Integral transform solutions of transient natural convection in enclosures with variable fluid properties. Int. J. Heat Mass Transf. 2007, 43, 3977–3990. [Google Scholar] [CrossRef]
- Leong, W.H.; Hollands, K.G.T.; Brunger, A.P. Experimental Nusselt numbers for a cubical-cavity benchmark problem in natural convection. Int. J. Heat Mass Transf. 1999, 42, 1979–1989. [Google Scholar] [CrossRef]
- Lo, D.C.; Young, D.L.; Murugesan, K.; Tsai, C.C.; Gou, M.H. Velocity-vorticity Formulation for 3-D Natural Convection in an Inclined Cavity by DQ Method. Int. J. Heat Mass Transf. 2007, 50, 479–491. [Google Scholar] [CrossRef]
- Ma, Y.; Mohebbi, R.; Rashidi, M.M.; Yang, Z. Simulation of nanofluid natural convection in a U-shaped cavity equipped by a heating obstacle: Effect of cavity’s aspect ratio. J. Taiwan Inst. Chem. Eng. 2018, 93, 263–276. [Google Scholar] [CrossRef]
- Mamourian, M.; Shirvan, K.M.; Pop, I. Sensitivity analysis for MHD effects and inclination angles on natural convection heat transfer and entropy generation of Al2O3-water nanofluid in square cavity by Response Surface Methodology. Int. Commun. Heat Mass Transf. 2016, 79, 46–57. [Google Scholar] [CrossRef]
- Manzari, M.T. An explicit finite element algorithm for convection heat transfer problems. Int. J. Numer. Method. Heat Fluid Flow 1999, 9, 860–877. [Google Scholar] [CrossRef]
- Markatos, N.C.; Pericleous, K.A. Laminar and turbulent natural convection in an enclosed cavity. Int. J. Heat Mass Transf. 1984, 27, 755–772. [Google Scholar] [CrossRef]
- Mayeli, P.; Sheard, G.J. Natural convection and entropy generation in square and skew cavities due to large temperature differences: A Gay–Lussac-type vorticity stream-function approach. Int. J. Numer. Method. Fluid. 2021, 93, 2396–2420. [Google Scholar] [CrossRef]
- Nag, A.; Sarkar, A.; Sastri, V.M.K. Natural convection in a differentially heated square cavity with a horizontal partition plate on the hot wall. Comput. Methods Appl. Mech. Eng. 1993, 110, 143–156. [Google Scholar] [CrossRef]
- Nithyadevi, N.; Sivasankaran, S.; Kandaswamy, P. Buoyancy-driven convection of water near its density maximum with time-periodic partially active vertical walls. Meccanica 2007, 42, 503–510. [Google Scholar] [CrossRef]
- Olayemi, O.A.; Khaled, A.-F.; Temitope, O.J.; Victor, O.O.; Odetunde, C.B.; Adegun, I.K. Parametric study of natural convection heat transfer from an inclined rectangular cylinder embedded in a square enclosure. Aust. J. Mech. Eng. 2021, 1–14. [Google Scholar] [CrossRef]
- Oueslati, F.S.; Bennacer, R. Heterogeneous nanofluids: Natural convection heat transfer enhancement. Nanoscale Res. Lett. 2011, 6, 222. [Google Scholar] [CrossRef]
- Oztop, H.; Abu-Nada, E. Numerical study of natural convection in partially heated rectangular enclosures filled with nanofluids. Int. J. Heat Fluid Flow 2008, 29, 1326–1336. [Google Scholar] [CrossRef]
- Qi, C.; He, Y.; Yan, S.; Tian, F.; Hu, Y. Numerical simulation of natural convection in a square enclosure filled with nanofluid using the two-phase Lattice Boltzmann method. Nanoscale Res. Lett. 2013, 8, 1–16. [Google Scholar] [CrossRef] [PubMed]
- Qu´er´e, P.L.; Roquefortt, T.A.D. Computation of natural convection in two- dimensional cavities with Chebyshev polynomials. J. Comput. Phys. 1985, 57, 210–228. [Google Scholar] [CrossRef]
- Sai, B.V.K.S.; Seetharamu, K.N.; Narayana, P.A.A. Solution of transient laminar natural convection in a square cavity by an explicit finite element scheme. Numer. Heat Transf. Part A Appl. 1994, 25, 412–422. [Google Scholar]
- Shi, X.; Khodadadi, J.M. Laminar natural convection heat transfer in a differentially heated square cavity due to a thin fin on the hot wall. J. Heat Transf. 2003, 125, 624–634. [Google Scholar] [CrossRef]
- Son, J.H.; Park, I.S. Numerical study of MHD natural convection in a rectangular enclosure with an insulated block. Numer. Heat Transf. Part A Appl. 2017, 71, 1004–1022. [Google Scholar] [CrossRef]
- Thangavelu, M.; Nagarajan, N.; Yang, R.-J. Magnetohydrodynamic effect on thermal transport by silver nanofluid flow in enclosure with central and lower heat sources. Heat Transf. Eng. 2022, 43, 1755–1768. [Google Scholar] [CrossRef]
- Tric, E.; Labrosse, G.; Betrouni, M. A First Incursion into the 3-D Structure of Natural Convection of Air in a Differentially Heated Cubic Cavity from Accurate Numerical Solutions. Int. J. Heat Mass Transf. 2000, 43, 4043–4056. [Google Scholar] [CrossRef]
- Trodi, A.; Benhamza, M.E.H.B. Particle shape and aspect ratio effect of Al2O3–water nanofluid on natural convective heat transfer enhancement in differentially heated square enclosures. Chem. Eng. Commun. 2017, 204, 158–167. [Google Scholar] [CrossRef]
- Wan, C.; Patnaik, B.S.V.; Wei, D.G.W. A new benchmark quality solution for the buoyancy-driven cavity by discrete singular convolution. Numer. Heat Transf. Part B Fundam. 2001, 40, 199–228. [Google Scholar]
- Yıldız, C.; Arıcı, M.; Karabay, H. Comparison of a theoretical and experimental thermal conductivity model on the heat transfer performance of Al2O3-SiO2/water hybrid-nanofluid. Int. J. Heat Mass Transf. 2019, 140, 598–605. [Google Scholar] [CrossRef]
- Zhang, W.; Huang, Z.; Zhang, C.; Xi, G. Conjugate wall conduction-fluid natural convection in a three-dimensional inclined enclosure. Numer. Heat Transf. Part A Appl. 2012, 61, 122–141. [Google Scholar] [CrossRef]
- Zhang, X.H.; Saeed, T.; Algehyne, E.A.; El-Shorbagy, M.A.; El-Refaey, A.M.; Ibrahim, M. Effect of L-shaped heat source and magnetic field on heat transfer and irreversibilities in nanofluid-filled oblique complex enclosure. Sci. Rep. 2021, 11, 16458. [Google Scholar] [CrossRef]
- Abdelkhalek, M.M. Mixed convection in a square cavity by a perturbation technique. Comput. Mater. Sci. 2008, 42, 212–219. [Google Scholar] [CrossRef]
- Abu-Nada, E.; Chamkha, A.J. Mixed convection flow in a lid-driven inclined square enclosure filled with a nanofluid. Eur. J. Mech. B Fluid. 2010, 29, 472–482. [Google Scholar] [CrossRef]
- Al-Amiri, A.M. Analysis of momentum and energy transfer in a lid-driven cavity filled with a porous medium. Int. J. Heat Mass Transf. 2000, 43, 3513–3527. [Google Scholar] [CrossRef]
- Biswas, N.; Manna, N.K.; Mahapatra, P.S. Enhanced thermal energy transport using adiabatic block inside lid-driven cavity. Int. J. Heat Mass Transf. 2016, 100, 407–427. [Google Scholar] [CrossRef]
- Gresho, P.M.; Lee, R.L.; Sani, R.L. On the time-dependent solution of the incompressible Navier–Stokes equations in two and three dimensions. In Recent Adv. Numerical Methods in Fluids; Pineridge: Swansea, UK, 1980. [Google Scholar]
- Kefayati, G.R. Double-diffusive mixed convection of pseudoplastic fluids in a two-sided lid-driven cavity using FDLBM. J. Taiwan Inst. Chem. Eng. 2014, 45, 2122–2139. [Google Scholar] [CrossRef]
- Khanafer, K.M.; Al-Amiri, A.M.; Pop, I. Numerical simulation of unsteady mixed convection in a driven cavity. Using an externally excited sliding lid. Eur. J. Mech. B Fluid. 2007, 26, 669–687. [Google Scholar] [CrossRef]
- Mehmood, K.; Hussain, S.; Sagheer, M. Mixed convection in alumina-water nanofluid filled lid driven square cavity with an isothermally heated square blockage inside with magnetic field effect. Int. J. Heat Mass Transf. 2017, 109, 397–409. [Google Scholar] [CrossRef]
- Rashad, A.M.; Mansour, M.A.; Armaghani, T.; Chamkha, A.J. MHD mixed convection and entropy generation of nanofluid in a lid-driven U-shaped cavity with internal heat and partial slip. Phys. Fluid. 2019, 31, 042006. [Google Scholar] [CrossRef]
- Roy, M.; Roy, S.; Basak, T. Role of various moving walls on energy transfer rates via heat flow visualization during mixed convection in square cavities. Energy 2015, 82, 1–22. [Google Scholar] [CrossRef]
- Waheed, M.A. Mixed convective heat transfer in rectangular enclosures driven by a continuously moving horizontal plate. Int. J. Heat Mass Transf. 2009, 52, 5055–5063. [Google Scholar] [CrossRef]
- ElSherbiny, S.M.; Raithby, G.D.; Hollands, K.G.T. Heat transfer by natural convection across vertical and inclined air layers. J. Heat Transf. Trans. ASME 1982, 104, 96–102. [Google Scholar] [CrossRef]
- Lartigue, B.; Lorente, S.; Bourret, B. Multicellular natural convection in a high aspect ratio cavity: Experimental and numerical results. Int. J. Heat Mass Transf. 2000, 43, 3157–3170. [Google Scholar] [CrossRef]
- Lee, Y.; Korpela, S.A. Multicellular natural convection in a vertical slot. J. Fluid Mech. 1983, 126, 91–121. [Google Scholar] [CrossRef]
- Power, J.P. Finite Element Model of Turbulent Flow and Heat Transfer in a Fenestration System. Ph.D. Thesis, The University of Massachusetts Amherst, Amherst, MA, USA, 1999. [Google Scholar]
- Wright, J.L.; Sullivan, H.F. A two-dimensional numerical model for natural convection in a vertical. rectangular window cavity. ASHRAE Trans. 1994, 100, 1193–1206. [Google Scholar]
- Zhao, Y.; Goss, W.P.; Curcija, D. Prediction of the multicellular flow regime of natural convection in fenestration glazing cavities. ASHRAE Trans. 1997, 10, 1–12. [Google Scholar]
- Baytas, A.C.; Pop, I. Free convection in a square porous cavity using a thermal non-equilibrium model. Int. J. Therm. Sci. 2002, 41, 861–870. [Google Scholar] [CrossRef]
- Bejan, A. On the boundary layer regime in a vertical enclosure filled with a porous medium. Lett. Heat Mass Transf. 1979, 6, 93–102. [Google Scholar] [CrossRef]
- Goyeau, B.; Songbe, J.P.; Gobin, D. Numerical study of double-diffusive natural convection in a porous cavity using the Darcy-Brinkman formulation. Int. J. Heat Mass Transf. 1996, 39, 1363–1378. [Google Scholar] [CrossRef]
- Saeid, N.H.; Pop, I. Natural convection from a discrete heater in a square cavity filled with a porous medium. J. Porous Media 2005, 8, 55–63. [Google Scholar] [CrossRef]
- Ho, C.J.; Liu, W.K.; Chang, Y.S.; Lin, C.C. Natural convection heat transfer of alumina-water nanofluid in vertical square enclosures: An experimental study. Int. J. Therm. Sci. 2010, 49, 1345–1353. [Google Scholar] [CrossRef]
- Omri, M.; Jamal, M.; Hussain, S.; Kolsi, L.; Maatki, C. Conjugate natural convection of a hybrid nanofluid in a cavity filled with porous and non-newtonian layers: The impact of the power law index. Mathematics 2022, 10, 2044. [Google Scholar] [CrossRef]
- Saghir, M.Z.; Ahadi, A.; Mohamad, A.; Srinivasan, S. Water aluminum oxide nanofluid benchmark model. Int. J. Therm. Sci. 2016, 109, 148–158. [Google Scholar] [CrossRef]
- Sheremet, M.; Chinnasamy, S. Convective–radiative heat transfer in a cavity filled with a nanofluid under the effect of a nonuniformly heated plate. Int. J. Numer. Method. Heat Fluid Flow 2018, 28, 1392–1409. [Google Scholar] [CrossRef]
- Shulepova, E.V.; Sheremet, M.A.; Oztop, H.F.; Abu-Hamdeh, N. Mixed convection of Al2O3–H2O nanoliquid in a square chamber with complicated fin. Int. J. Mech. Sci. 2020, 165, 105192. [Google Scholar] [CrossRef]
- Huilgol, R.R.; Kefayati, G.H.R. Natural convection problem in a Bingham fluid using the operator-splitting method. J. Non Newton. Fluid Mech. 2015, 220, 22–32. [Google Scholar] [CrossRef]
- Kefayati, G.R. Mesoscopic simulation of magnetic field effect on natural convection of power-law fluids in a partially heated cavity. Chem. Eng. Res. Des. 2015, 94, 337–354. [Google Scholar] [CrossRef]
- Khezzar, L.; Siginer, D.; Vinogradov, I. Natural convection of power law fluids in inclined cavities. Int. J. Therm. Sci. 2012, 53, 8–17. [Google Scholar] [CrossRef]
- Kim, G.B.; Hyun, J.M.; Kwak, H.S. Transient buoyant convection of a power-law non-Newtonian fluid in an enclosure. Int. J. Heat Mass Transf. 2003, 46, 3605–3617. [Google Scholar]


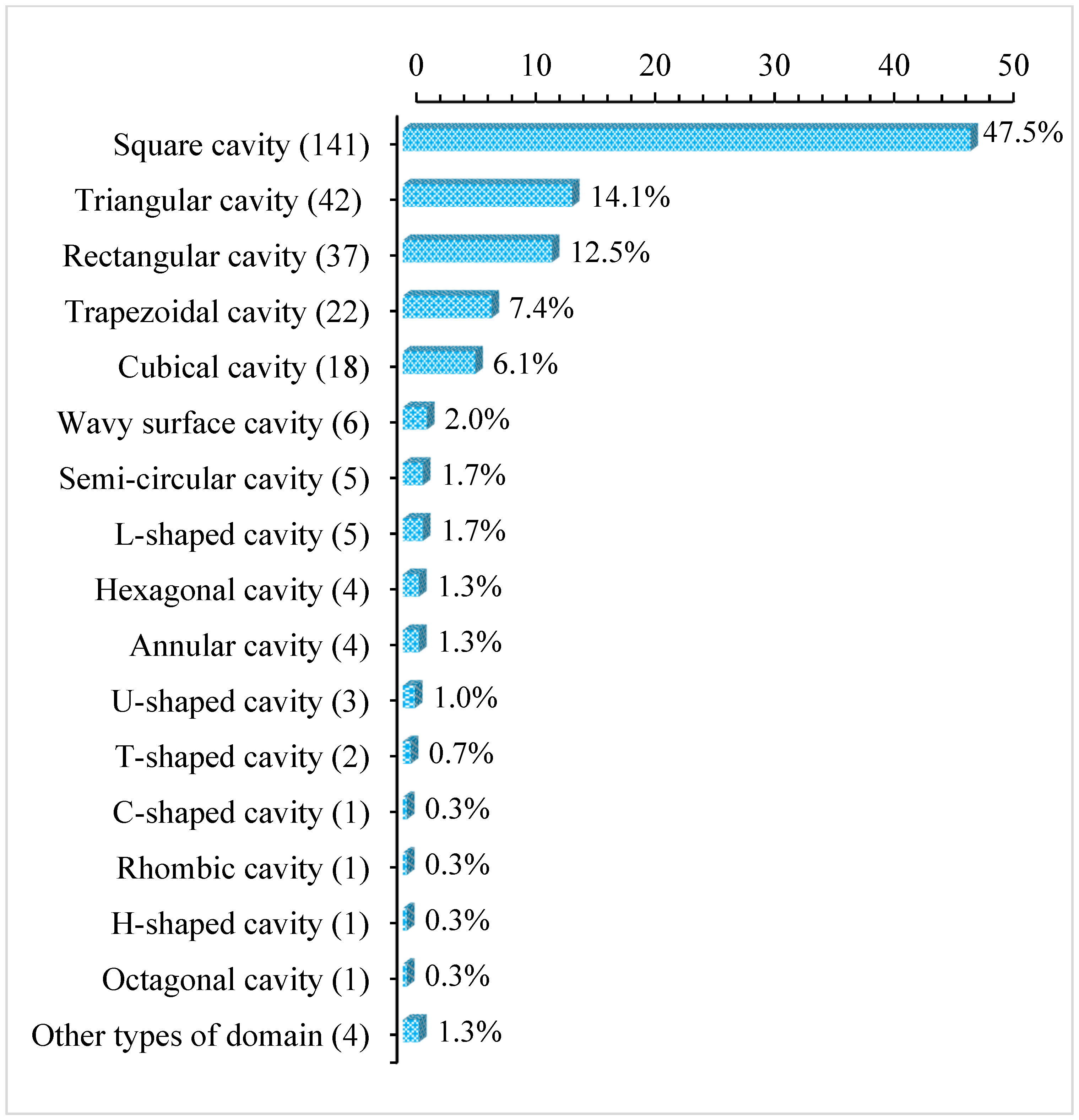
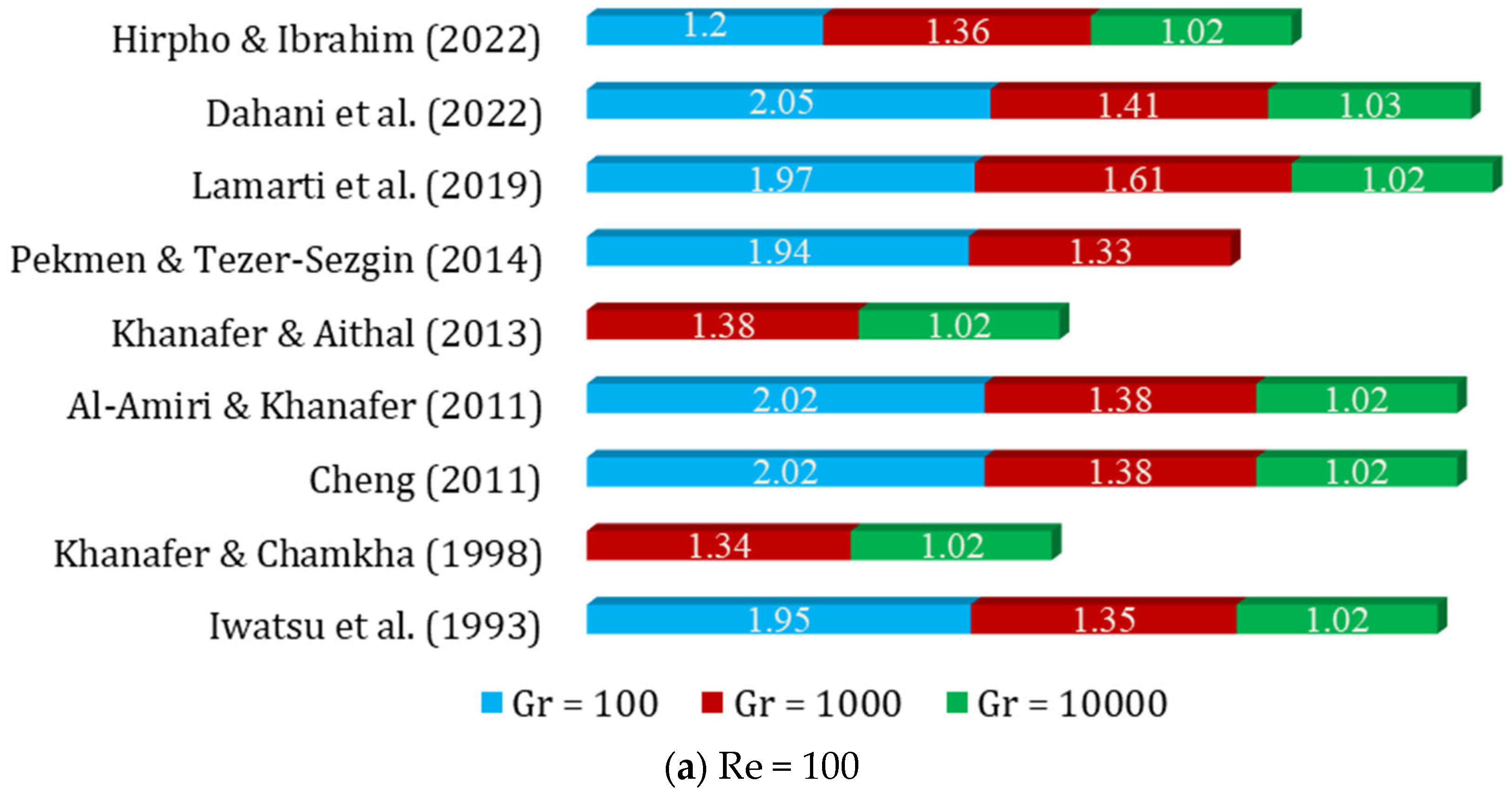
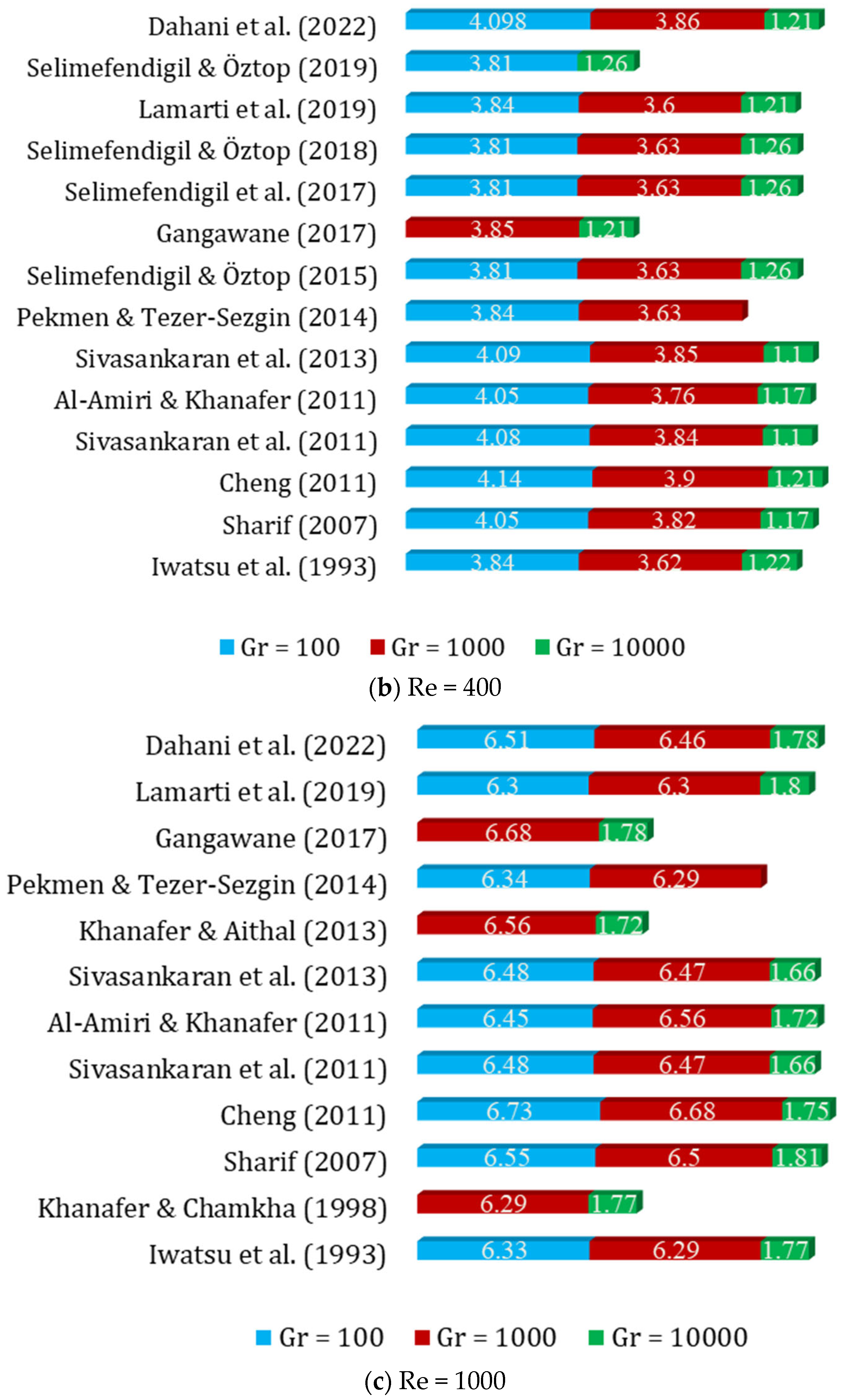


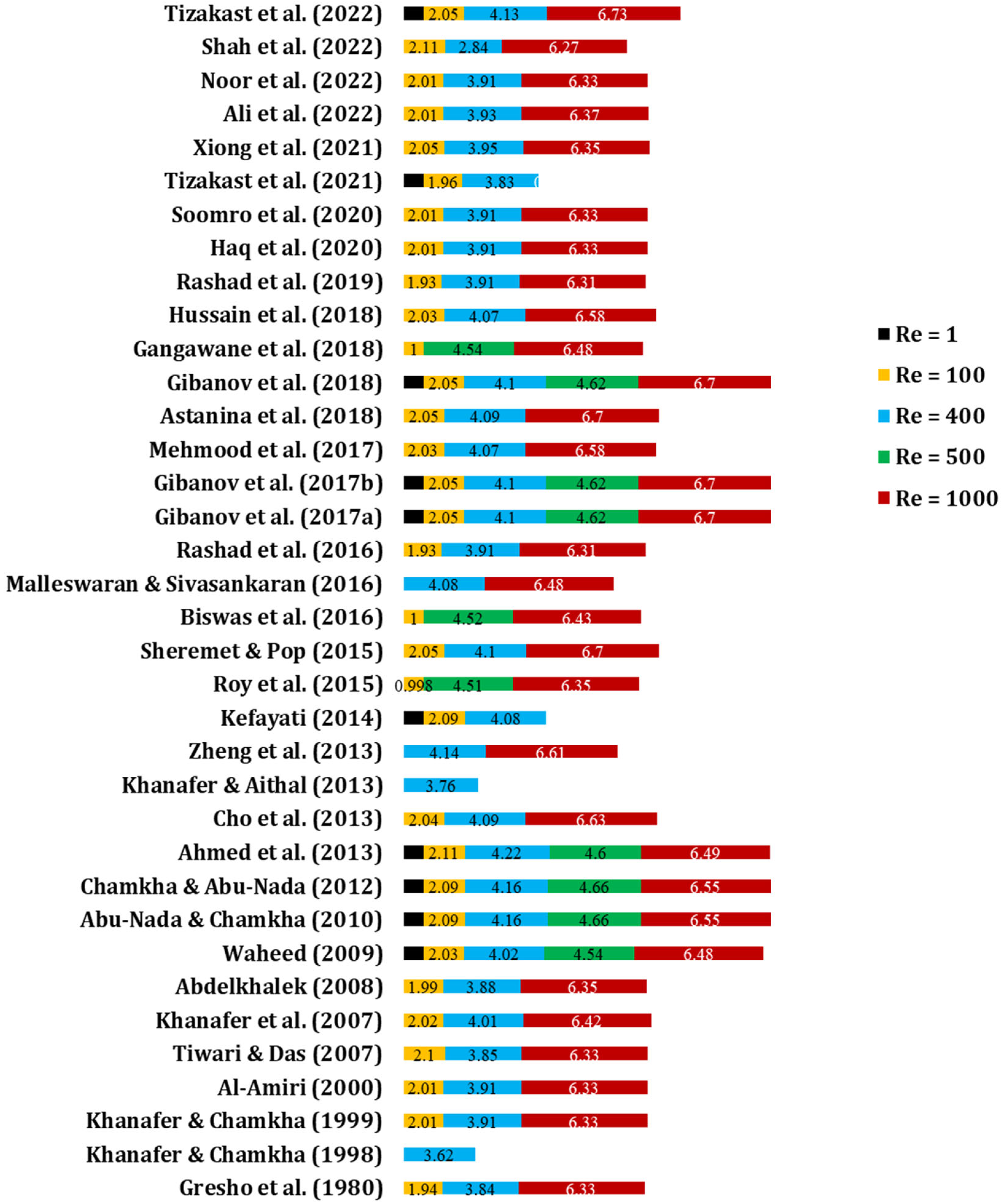
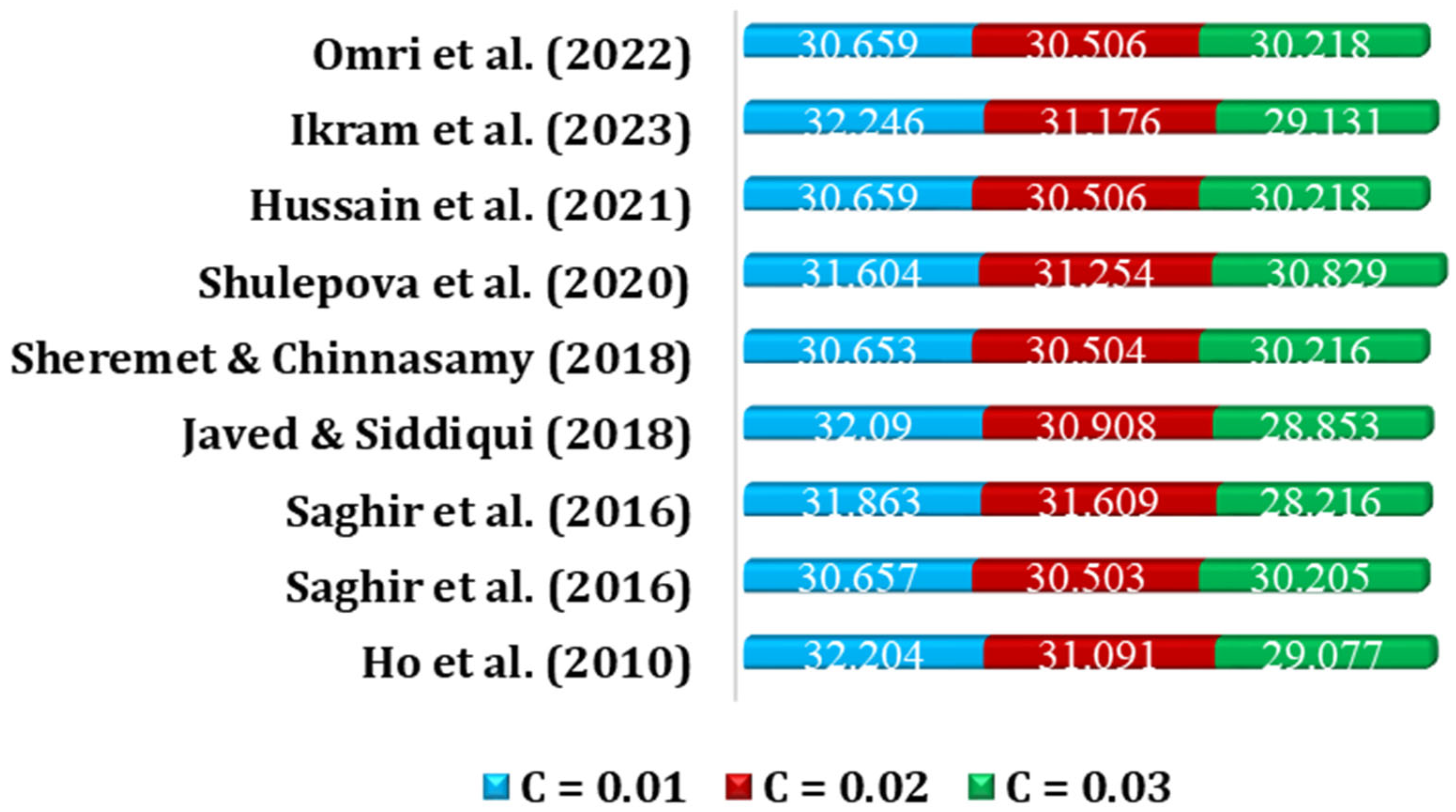
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saha, G.; Al-Waaly, A.A.Y.; Paul, M.C.; Saha, S.C. Heat Transfer in Cavities: Configurative Systematic Review. Energies 2023, 16, 2338. https://doi.org/10.3390/en16052338
Saha G, Al-Waaly AAY, Paul MC, Saha SC. Heat Transfer in Cavities: Configurative Systematic Review. Energies. 2023; 16(5):2338. https://doi.org/10.3390/en16052338
Chicago/Turabian StyleSaha, Goutam, Ahmed A.Y. Al-Waaly, Manosh C. Paul, and Suvash C. Saha. 2023. "Heat Transfer in Cavities: Configurative Systematic Review" Energies 16, no. 5: 2338. https://doi.org/10.3390/en16052338
APA StyleSaha, G., Al-Waaly, A. A. Y., Paul, M. C., & Saha, S. C. (2023). Heat Transfer in Cavities: Configurative Systematic Review. Energies, 16(5), 2338. https://doi.org/10.3390/en16052338