Study on Erosion Model Optimization and Damage Law of Coiled Tubing
Abstract
1. Introduction
2. Experiment
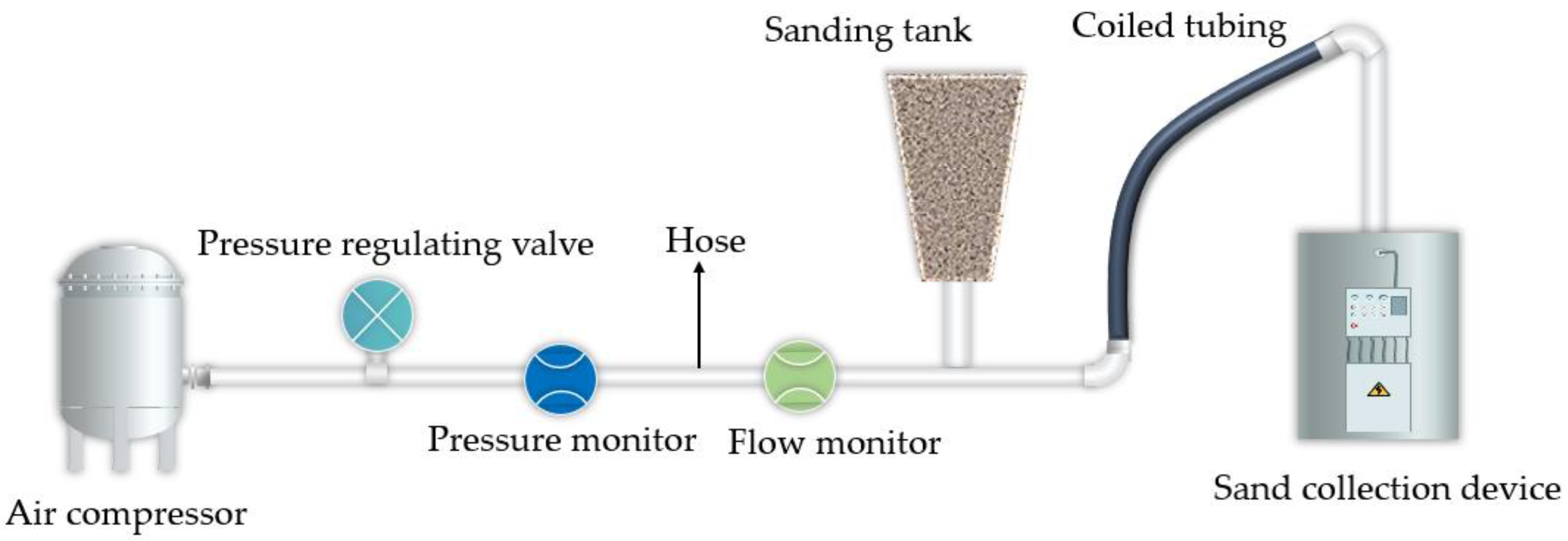
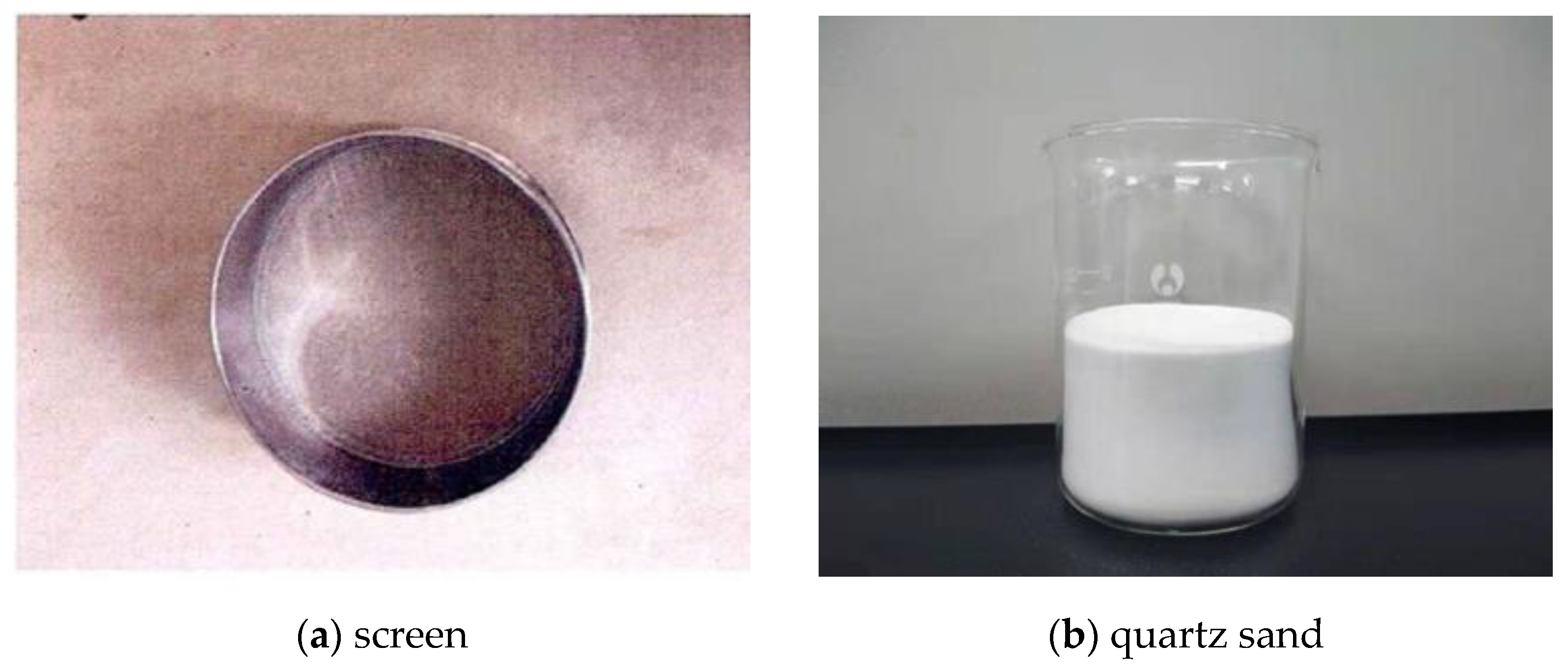
2.1. Experimental Device and Materials
2.2. Experimental Results
2.2.1. Macroscopic Damage Analysis
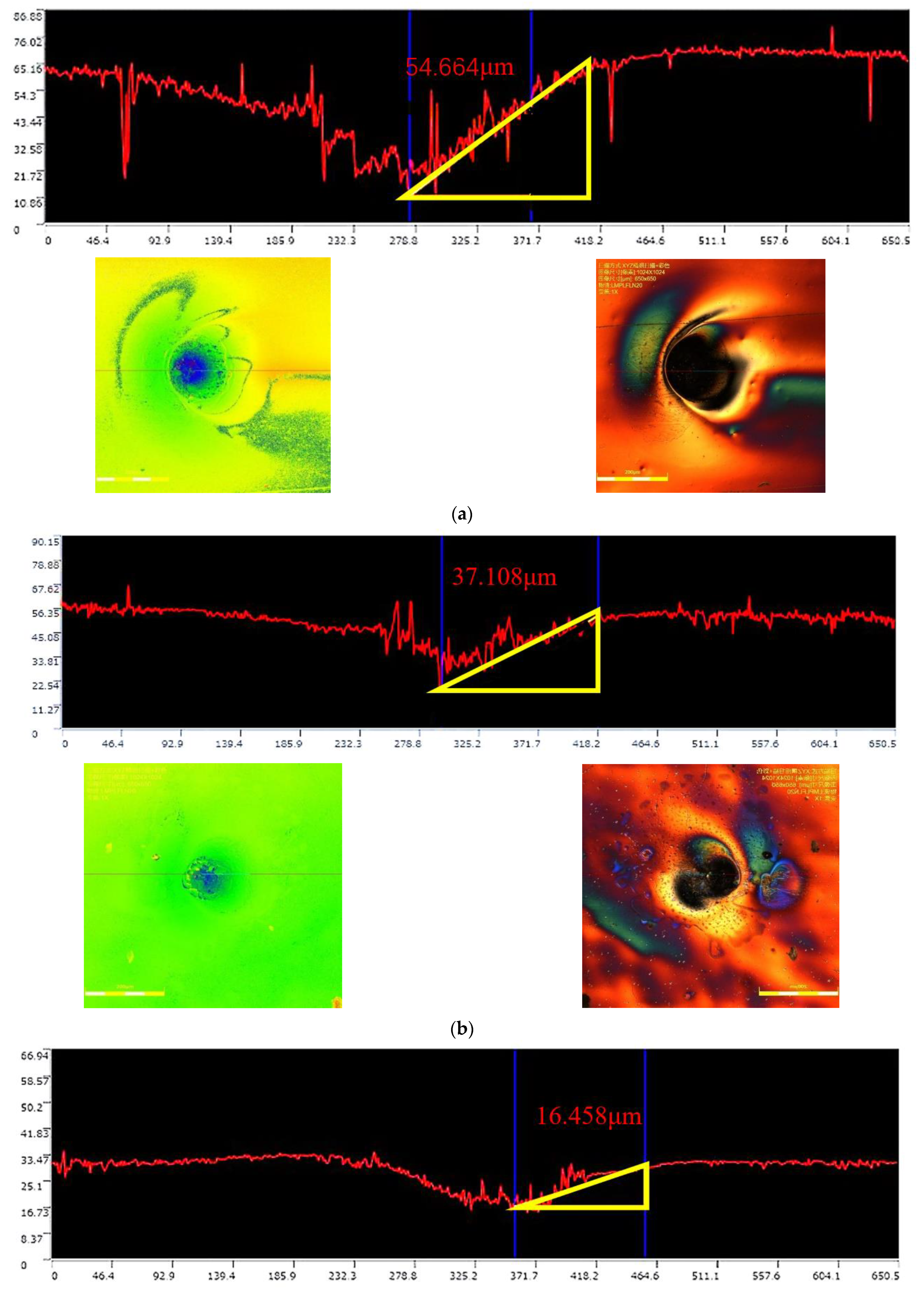
2.2.2. Microscopic Damage Analysis
3. Numerical Simulation
3.1. Theoretical Models and Control Equations
3.1.1. Continuous-Phase Equation
3.1.2. Turbulence Model
3.1.3. Discrete-Phase Model
3.1.4. Particle Rebound Model
3.1.5. Erosion Model
3.2. Model Optimization
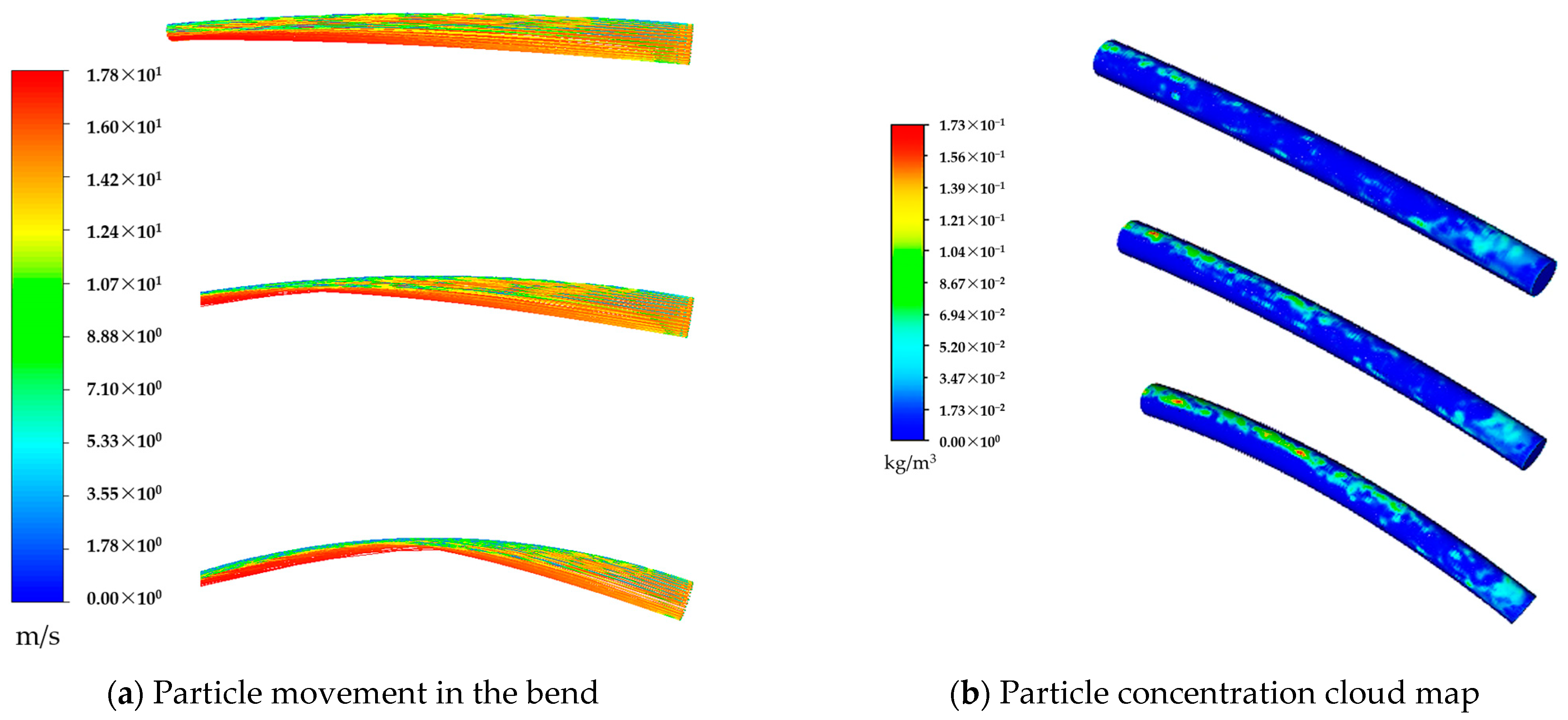
3.3. Sensitivity Analysis
4. Conclusions and Recommendations
- (1)
- For a pipe with small diameter and low bending degree, the Finnie model shows high accuracy in predicting the maximum erosion rate of the pipe by gas–solid two-phase flow, and the average error of the maximum erosion rate is 8.3%.
- (2)
- The inner wall of the outer bend of the pipe is the most serious area for erosion and wear. As the radius of curvature of the pipe decreases, the location of the maximum erosion rate moves to the inlet, and the maximum erosion rate increases.
- (3)
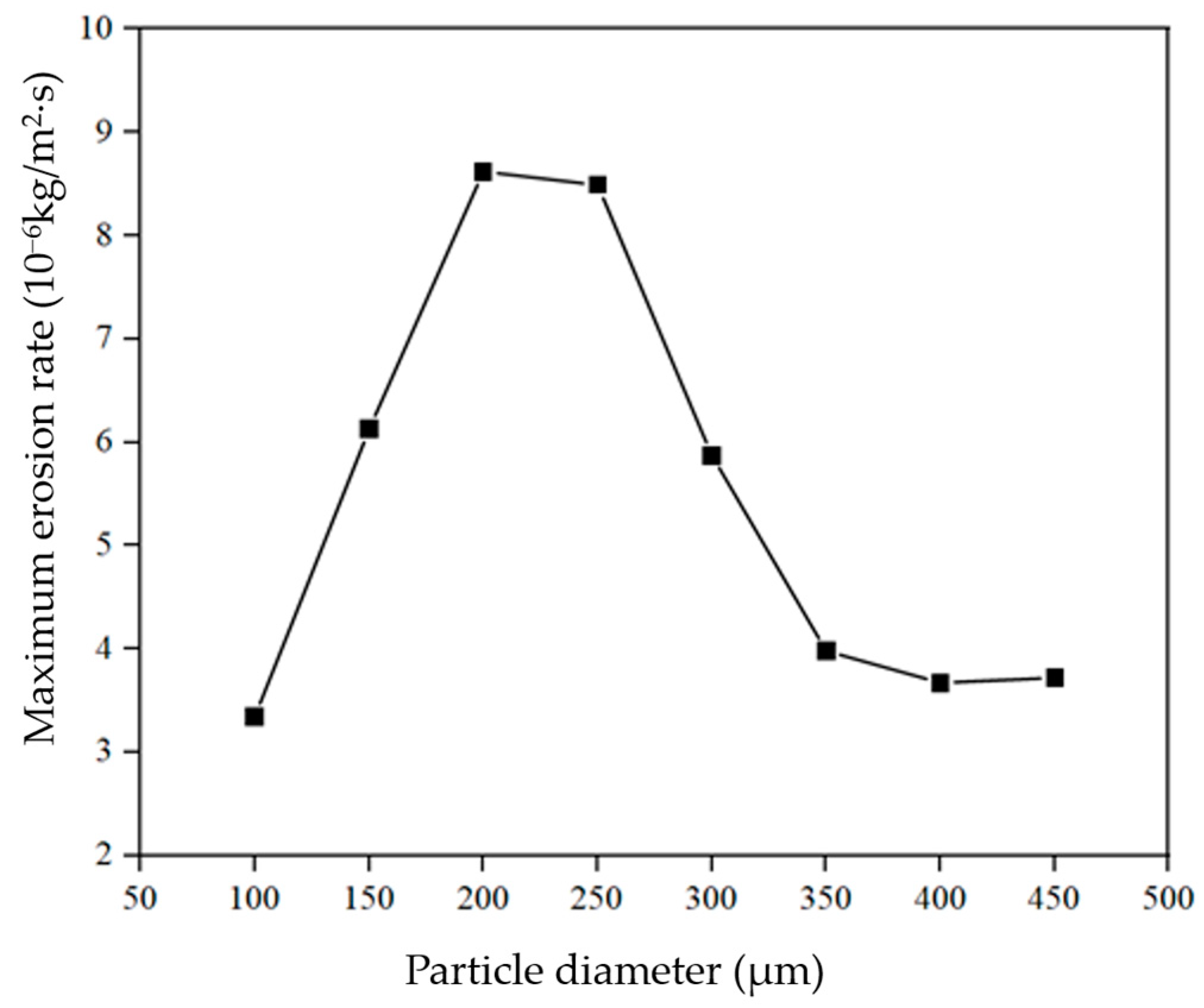
- The gas flow rate and the maximum erosion rate are positively correlated. The particle size and the maximum erosion rate show a complex relationship; with an increase in the particle size, the maximum erosion rate first increases, then decreases before stabilizing, with a critical particle size.
- (4)
- In order to extend the effective life of coiled tubing, the maximum erosion rate can be reduced by controlling the production rate to reduce the gas flow rate in the tubing column and by adopting reasonable sand control methods for old wells. The maximum erosion rate can be reduced by adjusting the borehole trajectory to reduce the bending of the tubing column for new wells.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Velocity vector, m/s | |
| Gas density, kg/m3 | |
| t | Time, s |
| p | Pressure, Pa |
| Stress tensor | |
| Gravitational acceleration, m/s2 | |
| Viscosity, Pa∙s | |
| Source term | |
| k | Turbulent kinetic energy, J |
| ε | Turbulent kinetic energy dissipation rate, J/s |
| ui | Velocity of the fluid in direction i, m/s |
| xi, xj | Spatial coordinate component, mm |
| μt | Eddy viscosity, Pa·s |
| ρp | Particle density, kg/m3 |
| up | Particle velocity, m/s |
| ug | Airflow velocity, m/s |
| ρg | Gas density, kg/m3 |
| Drag force on the particle, N | |
| Fother | Other forces on the particle, N |
| CD | Drag coefficient |
| Erosion rate, kg/(m2·s) | |
| Particle mass flow rate, kg/s | |
| Function of particle size | |
| Impact angle function | |
| Relative velocity function of particles | |
| Wall area, m2 | |
| Particle velocity, m/s | |
| α | Impact angle of particles |
| n | Velocity index |
| Bh | Brinell hardness, Mpa |
References
- Jiang, G.C.; Li, Y.Z.; He, Y.B.; Dong, T.F.; Sheng, K.M.; Sun, Z. Subsection and superposition method for reservoir formation damage evaluation of complex-structure wells. Petrol. Sci. 2023, in press. [Google Scholar] [CrossRef]
- Wang, Z.; Xu, Y.; Khan, N.; Zhu, C.; Gao, Y. Effects of the Surfactant, Polymer, and Crude Oil Properties on the Formation and Stabilization of Oil-Based Foam Liquid Films: Insights from the Microscale. J. Mol. Liq. 2023, 373, 121194. [Google Scholar] [CrossRef]
- Reynolds, O. On the action of a blast of sand in cutting hard material. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1873, 46, 337–343. [Google Scholar] [CrossRef]
- Rayleigh, L. The sand blast. Nature 1914, 93, 188. [Google Scholar] [CrossRef]
- Finnie, I. The mechanism of erosion of ductile metals. In Proceedings of the 3rd U.S. National Congress of Applied Mechanics, Providence, RI, USA, 11–14 June 1958; pp. 527–532. [Google Scholar]
- Tilly, G.P. A two stage mechanism of ductile erosion. Wear 1973, 23, 87–96. [Google Scholar] [CrossRef]
- Evans, A.G.; Gulden, M.E.; Rosenblatt, M. Impact damage in brittle materials in the elastic-plastic response regime. Proc. R. Soc. Lond. A Math. Phys. Sci. 1978, 361, 343–365. [Google Scholar]
- Tabakoff, W.; Kotwal, R.; Hamed, A. Erosion study of different materials affected by coal ash particles. Wear 1979, 52, 161–173. [Google Scholar] [CrossRef]
- Hutchings, I.M. A model for the erosion of metals by spherical particles at normal incidence. Wear 1981, 70, 269–281. [Google Scholar] [CrossRef]
- Zeng, L.; Zhang, G.A.; Guo, X.P. Erosion–corrosion at different locations of X65 carbon steel elbow. Corros. Sci. 2014, 85, 318–330. [Google Scholar] [CrossRef]
- Arabnejad, H.; Mansouri, A.; Shirazi, S.A.; McLaury, B.S. Abrasion erosion modeling in particulate flow. Wear 2017, 376, 1194–1199. [Google Scholar] [CrossRef]
- Shahali, H.; Ghasemi, H.M.; Abedini, M. Contributions of corrosion and erosion in the erosion-corrosion of Sanicro28. Mater. Chem. Phys. 2019, 233, 366–377. [Google Scholar] [CrossRef]
- Zhao, L.; Yan, Y.; Yan, X. A semi-empirical model for CO2 erosion-corrosion of carbon steel pipelines in wet gas-solid flow. J. Petrol. Sci. Eng. 2021, 196, 107992. [Google Scholar] [CrossRef]
- Edwards, J.K.; McLaury, B.S.; Shirazi, S.A. Modeling solid particle erosion in elbows and plugged tees. J. Energy Resour. Technol. 2001, 123, 277–284. [Google Scholar] [CrossRef]
- Shah, S.N.; Jain, S. Coiled tubing erosion during hydraulic fracturing slurry flow. Wear 2008, 264, 279–290. [Google Scholar] [CrossRef]
- Pandya, D.; Dennis, B.; Russell, R. An Improved Computational Fluid Dynamics (CFD) Model for Erosion Prediction in Oil and Gas Industry Applications. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering, San Francisco, CA, USA, 8–13 June 2014. [Google Scholar]
- Lin, N.; Lan, H.; Xu, Y.; Dong, S.; Barber, G. Effect of the gas–solid two-phase flow velocity on elbow erosion. J. Nat. Gas Sci. Eng. 2015, 26, 581–586. [Google Scholar] [CrossRef]
- Hong, B.; Li, Y.; Li, X.; Ji, S.; Yu, Y.; Fan, D.; Qian, Y.; Gong, J. Numerical simulation of gas-solid two-phase erosion for elbow and tee pipe in gas field. Energies 2021, 14, 6609. [Google Scholar] [CrossRef]
- Zhao, X.; Cao, X.; Xie, Z.; Cao, H.; Wu, C.; Bian, J. Numerical study on the particle erosion of elbows mounted in series in the gas-solid flow. J. Nat. Gas Sci. Eng. 2022, 99, 104423. [Google Scholar] [CrossRef]
- Li, B.; Zeng, M.; Wang, Q. Numerical simulation of erosion wear for continuous elbows in different directions. Energies 2022, 15, 1901. [Google Scholar] [CrossRef]
- Bilal, F.S.; Sedrez, T.A.; Shirazi, S.A. Experimental and CFD investigations of 45 and 90 degrees bends and various elbow curvature radii effects on solid particle erosion. Wear 2021, 476, 203646. [Google Scholar] [CrossRef]
- Zhu, X.; Peng, P.; Jing, J.; Song, W. Research on casing erosion in reservoir section of gas storage wells sased on Gas-solid Two-phase flow. Eng. Fail. Anal. 2022, 142, 106688. [Google Scholar] [CrossRef]
- Wang, K.; Li, X.; Wang, Y.; He, R. Numerical investigation of the erosion behavior in elbows of petroleum pipelines. Powder Technol. 2017, 314, 490–499. [Google Scholar] [CrossRef]
- Forder, A.; Thew, M.; Harrison, D. A numerical investigation of solid particle erosion experienced within oilfield control valves. Wear 1998, 216, 184–193. [Google Scholar] [CrossRef]
- Dennis, S.C.R.; Singh, S.N.; Ingham, D.B. The steady flow due to a rotating sphere at low and moderate Reynolds numbers. J. Fluid Mech. 1980, 101, 257–279. [Google Scholar] [CrossRef]
- Finnie, I. Erosion of surfaces by solid particles. Wear 1960, 3, 87–103. [Google Scholar] [CrossRef]
- Haugen, K.; Kvernvold, O.; Ronold, A.; Sandberg, R. Sand erosion of wear-resistant materials: Erosion in choke valves. Wear 1995, 186, 179–188. [Google Scholar] [CrossRef]
- McLaury, B.S.; Shirazi, S.A. Generalization of API RP 14E for erosive service in multiphase production. In Proceedings of the SPE Annual Technical Conference and Exhibition, Houston, TX, USA, 3–6 October 1999. [Google Scholar]
- McLaury, B.S.; Shirazi, S.A. An alternate method to API RP 14E for predicting solids erosion in multiphase flow. J. Energy Resour. Technol. 2000, 122, 115–122. [Google Scholar] [CrossRef]









| Author | Method | Summary |
|---|---|---|
| Finnie | Experimental | Various erosion models based on different influencing factors were established |
| Tilly | ||
| Evans | ||
| Tabakoff | ||
| Hutchings | ||
| Arabnejad | ||
| Edwards | Numerical simulation | The selection of erosion models varies widely |
| Shah | ||
| Pandya | ||
| Lin | ||
| Hong | ||
| Zhao | ||
| Bilal | ||
| Wang |
| Mass Flow Rate (kg/s) | Head (m) |
|---|---|
| 6.25 | 30 |
| Flow Rate (m3/s) | Head (m) | Rotational Speed (r/min) | Inlet and Outlet Diameter (m) | Equipped Motor (kW) | Shaft Power (kW) |
|---|---|---|---|---|---|
| 15 | 32 | 2900 | 50 × 40 | 3.5 | 5.5 |
| Radius of Curvature (m) | Measured Value (μm) | Simulated Value (μm) | Error % |
|---|---|---|---|
| 1.5 | 54.664 | 58.403 | 6.84 |
| 3 | 37.108 | 39.347 | 6.03 |
| 6 | 16.458 | 18.435 | 12.02 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, B.; Deng, J.; Lin, H.; Xu, J.; Wang, G.; Yan, W.; Wang, K.; Li, F. Study on Erosion Model Optimization and Damage Law of Coiled Tubing. Energies 2023, 16, 2775. https://doi.org/10.3390/en16062775
Zhang B, Deng J, Lin H, Xu J, Wang G, Yan W, Wang K, Li F. Study on Erosion Model Optimization and Damage Law of Coiled Tubing. Energies. 2023; 16(6):2775. https://doi.org/10.3390/en16062775
Chicago/Turabian StyleZhang, Binqi, Jingen Deng, Hai Lin, Jie Xu, Guiping Wang, Wei Yan, Kongyang Wang, and Fuli Li. 2023. "Study on Erosion Model Optimization and Damage Law of Coiled Tubing" Energies 16, no. 6: 2775. https://doi.org/10.3390/en16062775
APA StyleZhang, B., Deng, J., Lin, H., Xu, J., Wang, G., Yan, W., Wang, K., & Li, F. (2023). Study on Erosion Model Optimization and Damage Law of Coiled Tubing. Energies, 16(6), 2775. https://doi.org/10.3390/en16062775





