A Transient Analysis of Latent Thermal Energy Storage Using Phase Change Materials in a Refrigerated Truck
Abstract
1. Introduction
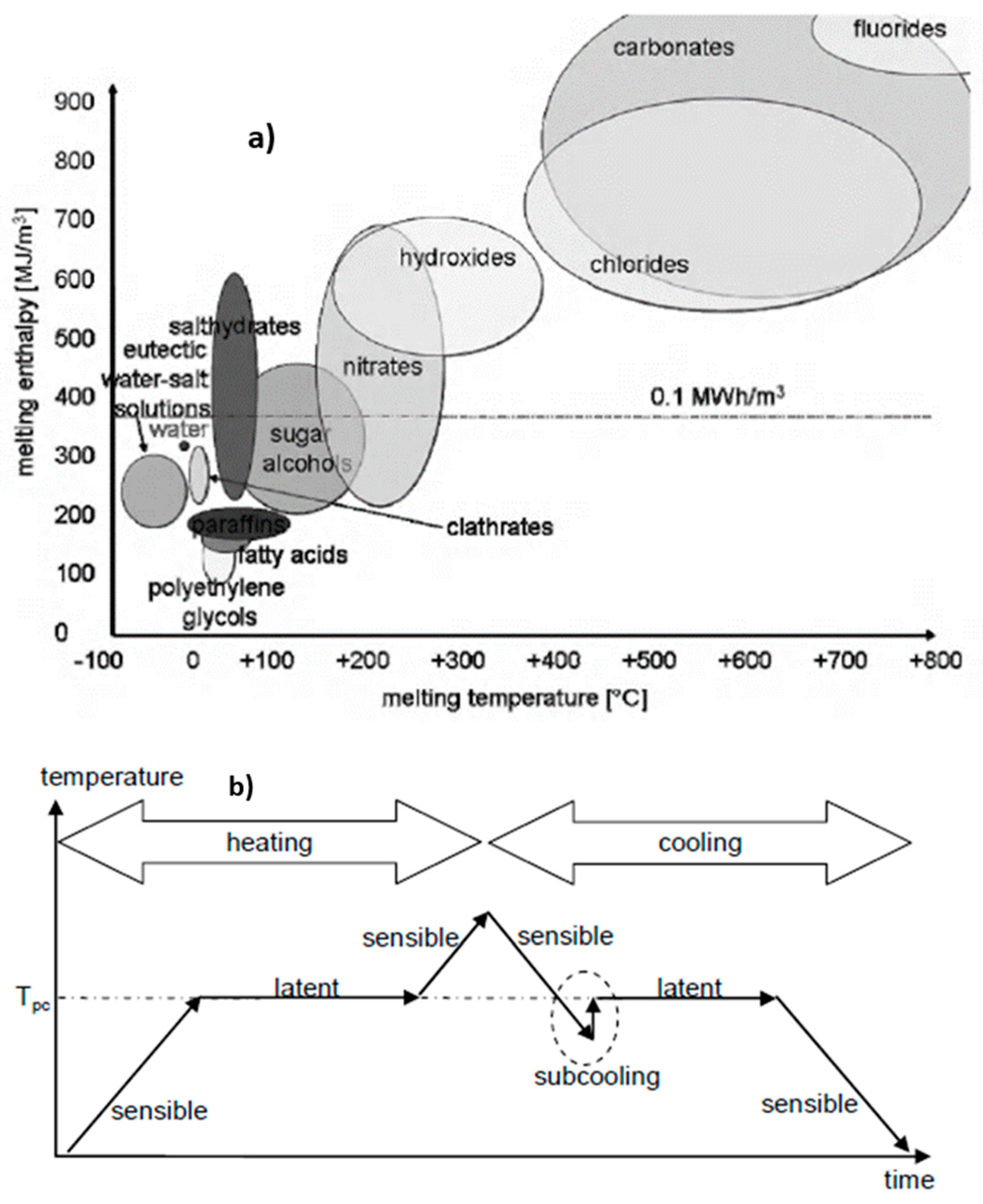
2. Physical and Technical Requirements of the PCMs
- low-temperature PCM, in which phase change occurs below 15 °C. Typical applications are air conditioning and cooling.
- PCM at average temperature (phase change temperature between 15 °C and 90 °C). They are the most widespread because they are used for the storage of hot water in solar plants, as passive systems in buildings for the maintenance of comfort and environmental air conditioning, and in the cooling of electronic components.
- High-temperature PCM (more than 90 °C) is used in industrial applications, in solar power generation plants, and in aerospace applications.
3. Theoretical Approach for Temperature Control in Transport Containers with PCM
4. Case Study and TRNSYS Model
- the reference climatic conditions are those of Naples (Italy) and the external temperatures considered in this study are those that are the warmest during the year, as the hottest day of the year was chosen for sizing the PCM in order to meet the cooling demand on all days of the year;
- the van makes 10 stops during which the operator opens the door for 1 min. It was considered that during the journey which lasts 10 h (from 8.00 a.m. to 6.00 p.m.), the truck makes one stop per hour to load or unload the food products;
- food products that are stored inside the van are already at the storage temperature set at −18 °C, therefore, the thermal load due to the cooling of food products is neglected;
- Two different velocities of the van (30 and 80 km h−1) have been considered to study two different scenarios: the first refers to a van transporting food products in an urban area where the speed limit is 50 km h−1 (so the average speed of a van is 30 km h−1); the second considers transport on a highway where the speed limit is 90 km h−1 (with an average speed of a van at 80 km h−1).
Transient Model and Governing Equations
- the specific heat of the PCM is constant and equal in both liquid and solid state;
- the thermal contact resistance between the PCM and adjacent layers is negligible;
- the solidification and melting processes take place at a fixed temperature.
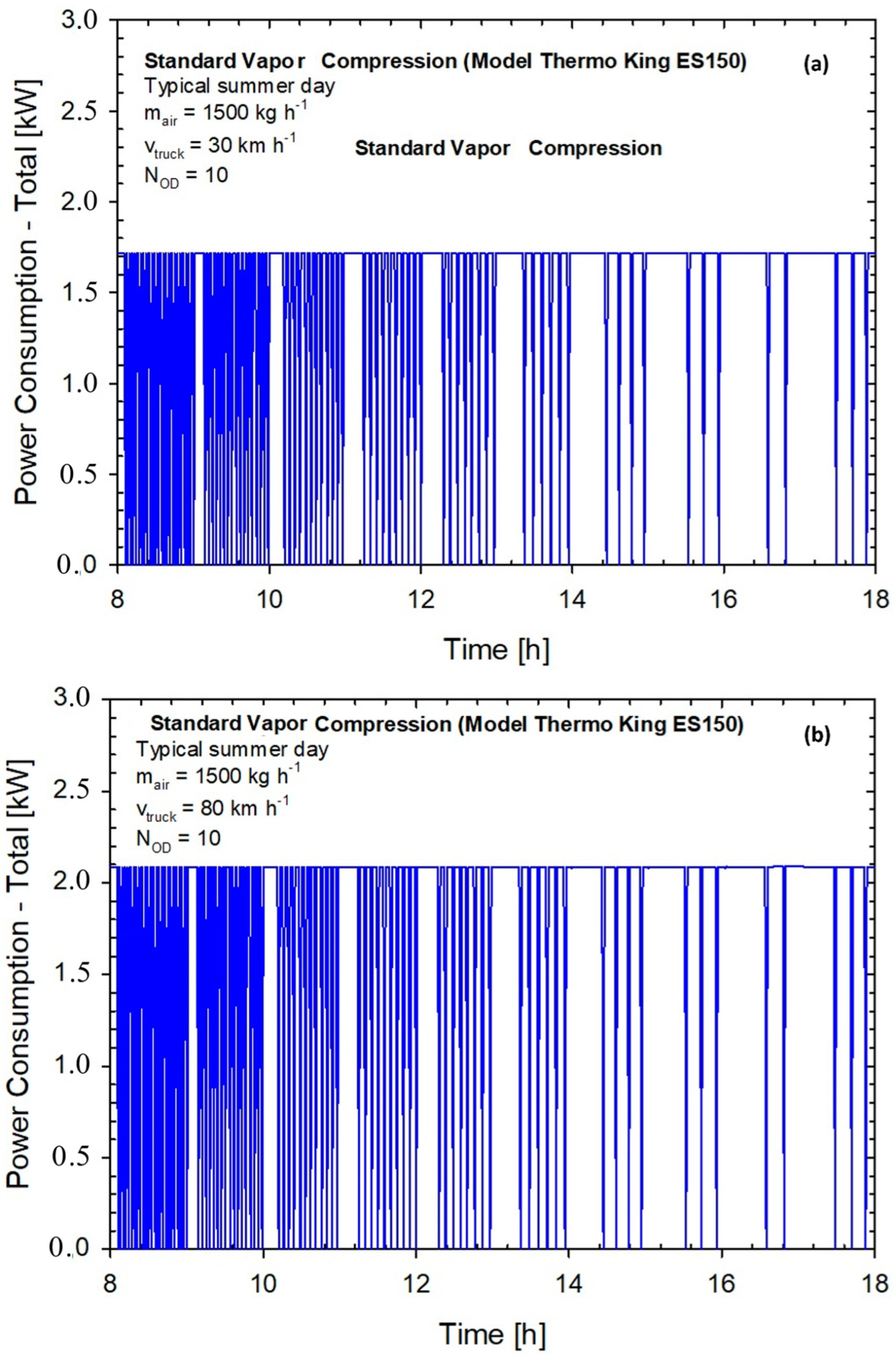
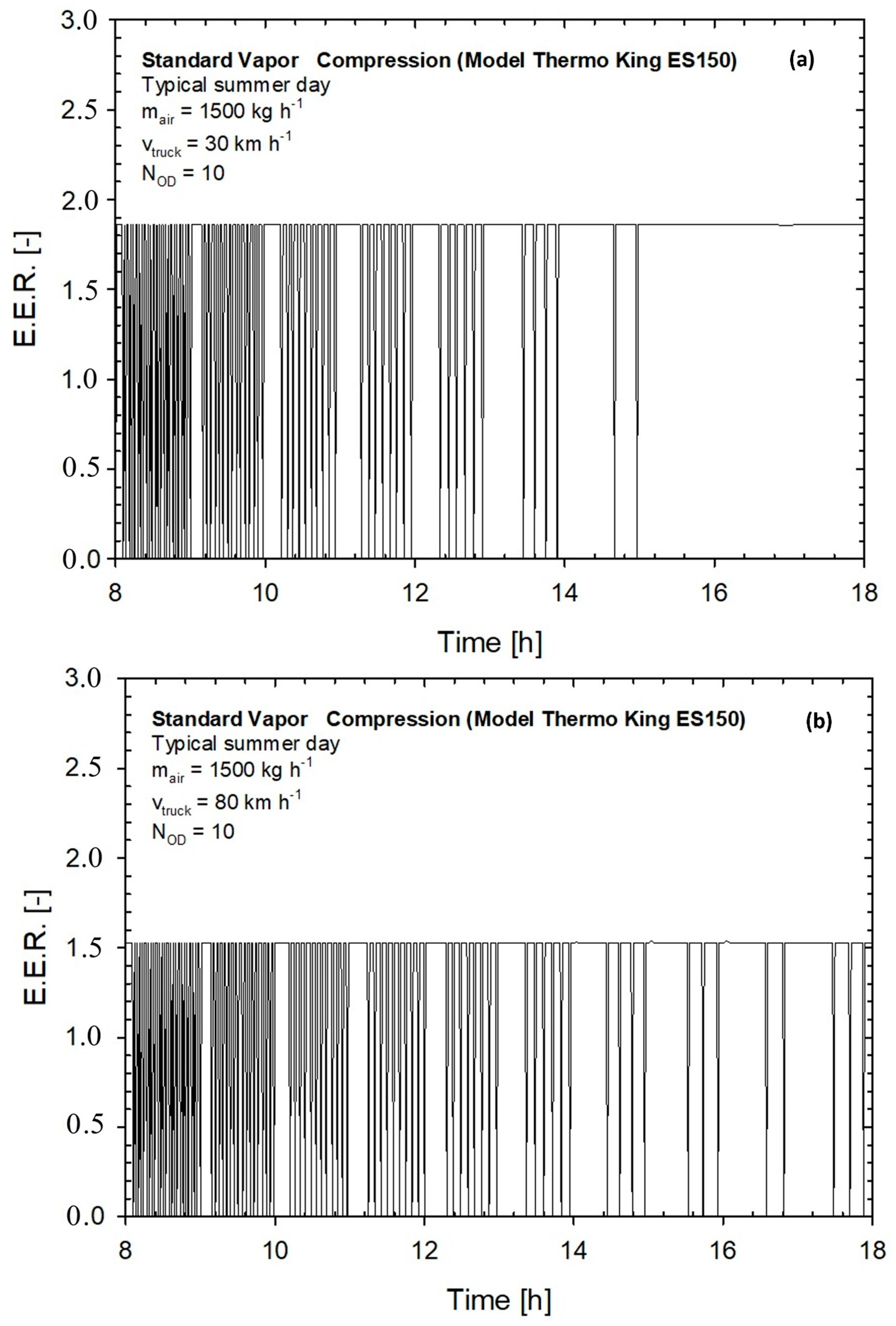
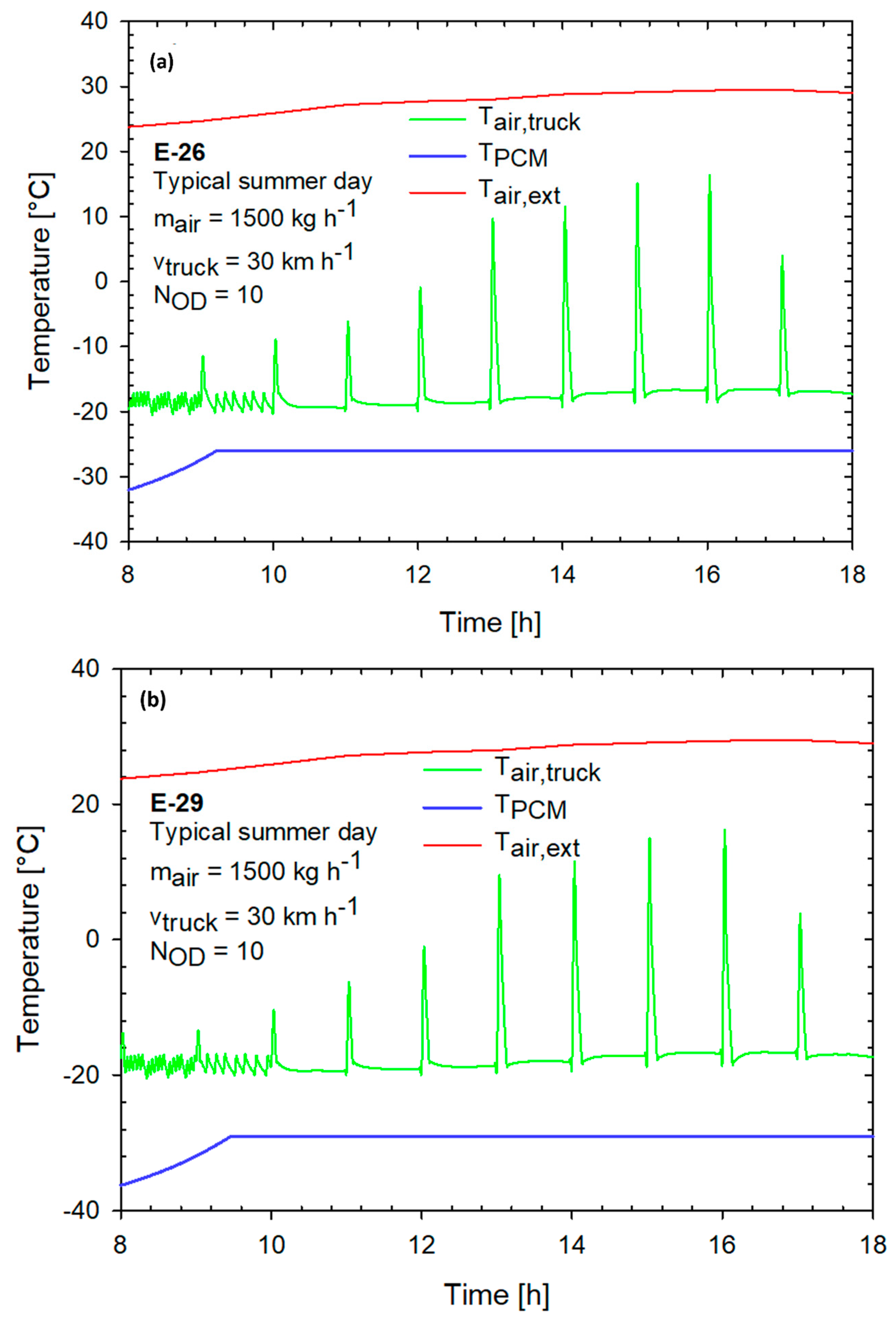
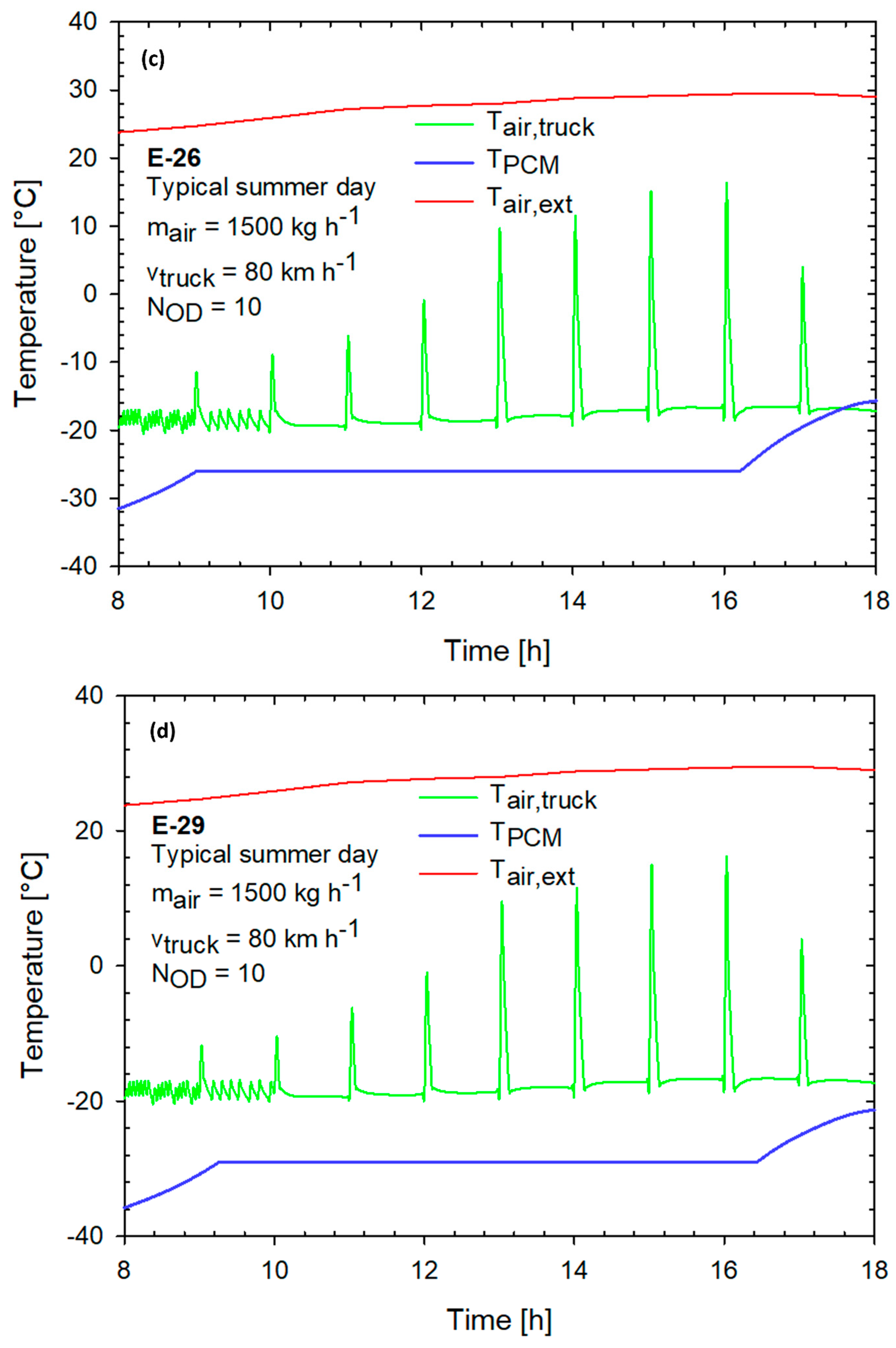
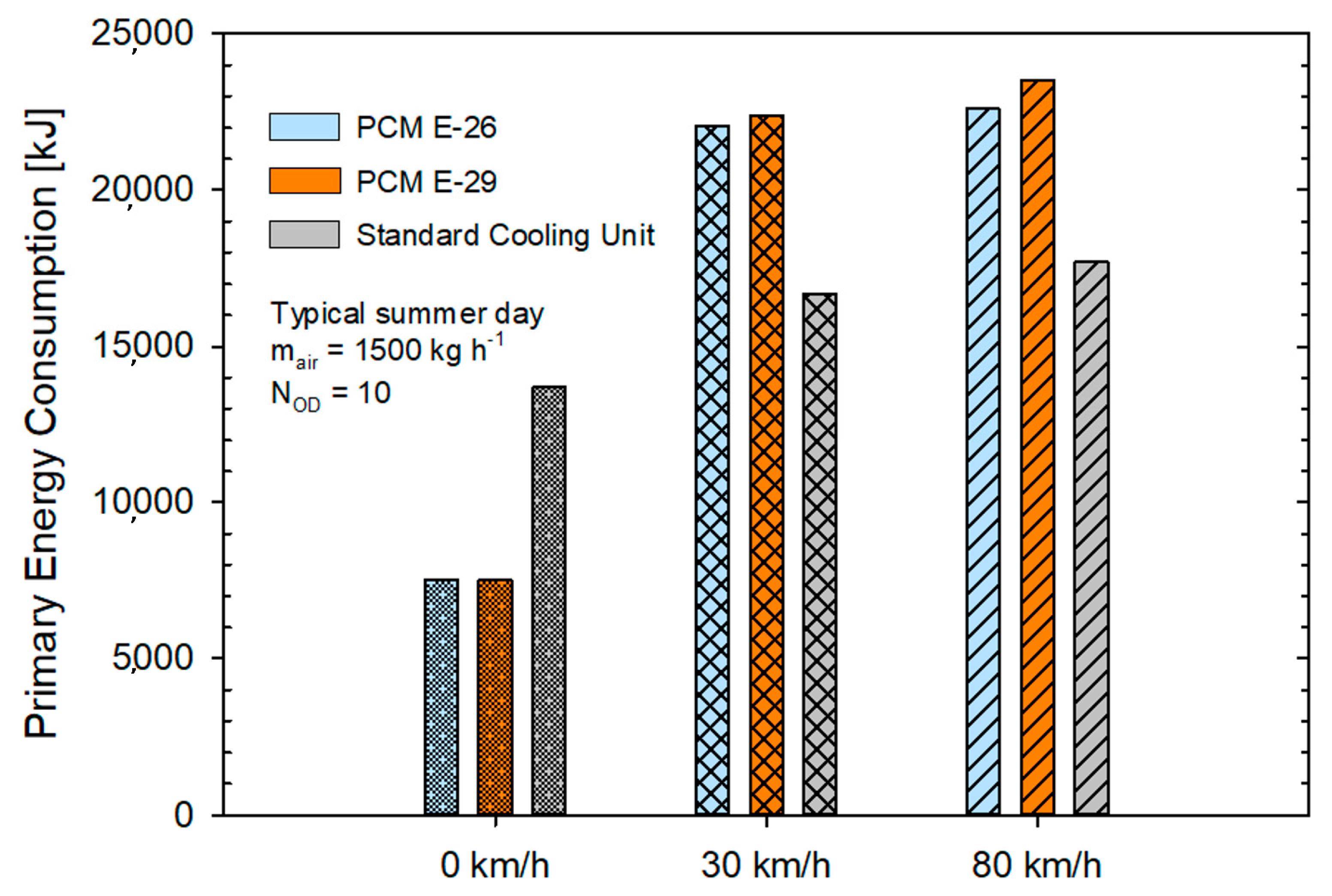
5. Results
6. Conclusions
- -
- the use of PCMs E-26 and E-29 can guarantee the cold chain, considering the average speed of the van at both 30 and 80 km h−1 for the entire working day (8.00 a.m.–6 p.m.) and a stationary situation at 0 km h−1;
- -
- the PCM E-26 exhausts its latent energy approximately 20 min before the E-29 PCM at an average van speed of 80 km h−1; while in the case of steady-state conditions, the PCM never reaches a fully melted configuration;
- -
- the average daily cost of the PCM E-26 is equal to EUR 0.96 and EUR 0.98, considering average speed of the van of 30 and 80 km h−1, respectively; in the case of 0 km h−1, the average daily cost is approximately EUR 0.58;
- -
- the average daily cost of the PCM E-29 is equal to EUR 0.99 and EUR 1.03, considering average speed of the van at 30 and 80 km h−1, respectively; in the case of 0 km h−1, the average daily cost is approximately EUR 0.59;
- -
- the daily economic saving due to the use of PCMs is always greater than 70%, up to a maximum of 78.84%, considering the PCM E-26 and the average van speed of 80 km h−1; in the case of 0 km h−1, the daily economic saving is around 83% for both PCMs;
- -
- for the primary energy consumptions, the worst case is connected with the recharging energy of PCM E-29 with a van speed of 80 km h−1; meanwhile, the 0 km h−1 configuration is more cost-effective and energy-efficient, but it represents a small portion of the entire workday for a van transporting food products.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Roman Symbols | |
| m | Mass, kg |
| L | Latent heat, kJ |
| h | Specific enthalpy, kJ/kg |
| H | Door height, m |
| t | Time |
| Power, kJ/h | |
| g | Gravity constant, m/s2 |
| A | Surface, m2 |
| Fm | Density factor, - |
| U | Overall heat transfer coefficient, W/(m2K) |
| C | Conductance surface, (W/ m2K) |
| x | Thickness, m |
| k | Thermal conductivity, W/(mK) |
| cp | Specific heat, kJ/(kgK) |
| E.E.E. | Energy efficiency ratio, - |
| PCM | Phase change material |
| N | Number |
| Greek symbols | |
| ρ | Density, kg/m3 |
| ∆ | Differential |
| λ | Heat transfer coefficient, (W/ m2K) |
| Subscripts | |
| i | Internal |
| r | Refrigerated |
| sen | Sensible |
| lat | Latent |
| tras | Transmission |
| PCM | Phase change material |
| inf | Infiltration |
| mech | Mechanical |
| M | Melting |
| OD | Open door |
| ext | External |
References
- Richards, G. Warehouse Management: A Complete Guide to Improving Efficiency and Minimizing Costs in the Modern Warehouse, 2nd ed.; Kogan Page: London, UK, 2014. [Google Scholar]
- Zhou, G.H.; Xu, X.L.; Liu, Y. Preservation technologies for fresh meat—A review. Meat Sci. 2010, 86, 119–128. [Google Scholar] [CrossRef]
- Meneghetti, A.; Monti, L. Greening the food supply chain: An optimisation model for sustainable design of refrigerated automated warehouses. Int. J. Prod. Res. 2015, 53, 6567–6587. [Google Scholar] [CrossRef]
- Mania, T.; Kawa, J. Heat and Cold Energy Storage Technologies as a Practical Complement to Renewable Energy Installations. Eco-Energetics Technol. Environ. Law Econ. 2018, 1, 41–50. [Google Scholar] [CrossRef]
- Vadhera, J.; Sura, A.; Nandan, G.; Dwivedi, G. Study of Phase Change materials and its domestic application. Mater. Today Proc. 2018, 5, 3411–3417. [Google Scholar] [CrossRef]
- Rosen, M.A.; Ibrahim, D. Thermal Energy Storage: Systems and Applications, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Gielen, D.; Boshell, F.; Saygin, D.; Bazilian, M.D.; Wagner, N.; Gorini, R. The role of renewable energy in the global energy transformation. Energy Strategy Rev. 2019, 24, 38–50. [Google Scholar] [CrossRef]
- Dávila, P.; Bourouis, M.; Francisco Nicolalde, J.; Martínez-Gómez, J. CO2/acetone mixture desorption process in a plate heat exchanger for compression/resorption heat pumps. Appl. Therm. Eng. 2024, 244, 122704. [Google Scholar] [CrossRef]
- Liu, M.; Saman, W.; Bruno, F. Development of a novel refrigeration system for refrigerated trucks incorporating phase change material. Appl. Energy 2012, 92, 336–342. [Google Scholar] [CrossRef]
- Liu, M.; Saman, W.; Bruno, F. Computer simulation with TRNSYS for a mobile refrigeration system incorporating a phase change thermal storage unit. Appl. Energy 2014, 132, 226–235. [Google Scholar] [CrossRef]
- Mousazade, A.; Rafee, R.; Valipour, M.S. Thermal performance of cold panels with phase change materials in a refrigerated truck. Int. J. Refrig. 2020, 120, 119–126. [Google Scholar] [CrossRef]
- Ben Taher, M.A.; Kousksou, T.; Zeraouli, Y.; Ahachad, M.; Mahdaoui, M. Thermal performance investigation of door opening and closing processes in a refrigerated truck equipped with different phase change materials. J. Energy Storage 2021, 42, 103097. [Google Scholar] [CrossRef]
- Liu, G.; Li, Q.; Wu, J.; Xie, R.; Zou, Y.; Marson, A.; Scipioni, A.; Manzardo, A. Improving system performance of the refrigeration unit using phase change material (PCM) for transport refrigerated vehicles: An experimental investigation in South China. J. Energy Storage 2022, 51, 104435. [Google Scholar] [CrossRef]
- TUmate, B.; Sawarkar, P.D. A review on thermal energy storage using phase change materials for refrigerated trucks: Active and passive approaches. J. Energy Storage 2024, 75, 109704. [Google Scholar] [CrossRef]
- De Gracia, A.; Cabeza, L.F. Phase change materials and thermal energy storage for buildings. Energy Build. 2015, 103, 414–419. [Google Scholar] [CrossRef]
- Zhou, Z.; Zhang, Z.; Zuo, J.; Huang, K.; Zhang, L. Phase change materials for solar thermal energy storage in residential buildings in cold climate. Renew. Sustain. Energy Rev. 2015, 48, 692–703. [Google Scholar] [CrossRef]
- Zhang, N.; Yuan, Y.; Cao, X.; Du, Y.; Zhang, Z.; Gui, Y. Latent Heat Thermal Energy Storage Systems with Solid–Liquid Phase Change Materials: A Review. Adv. Eng. Mater. 2018, 20, 1700753. [Google Scholar] [CrossRef]
- Mehling, H. Use of Phase Change Materials for Food Applications—State of the Art in 2022. Appl. Sci. 2023, 13, 3354. [Google Scholar] [CrossRef]
- EAssis; Katsman, L.; Ziskind, G.; Letan, R. Numerical and experimental study of melting in a spherical shell. Int. J. Heat Mass Transf. 2007, 50, 1790–1804. [Google Scholar] [CrossRef]
- Teng, T.P.; Cheng, C.M.; Cheng, C.P. Performance assessment of heat storage by phase change materials containing MWCNTs and graphite. Appl. Therm. Eng. 2013, 50, 637–644. [Google Scholar] [CrossRef]
- Omara, Z.M.; Ahmed, M.M.Z.; Alawee, W.H.; Shanmugan, S.; Elashmawy, M. A comprehensive review of nano-enhanced phase change materials on solar stills with scientometric analysis. Results Eng. 2024, 22, 102088. [Google Scholar] [CrossRef]
- Lane, G.A. Solar Heat Storage: Latent Heat Material—Volume I: Background and Scientific Principles, 1st ed.; CRC Press: Boca Raton, FL, USA, 1983. [Google Scholar]
- Khattari, Y.; El-Otmany, H.; El Rhafiki, T.; Kousksou, T.; Ahmed, A.; Ghoulam, E.B. Physical models to evaluate the performance of impure phase change material dispersed in building materials. J. Energy Storage 2020, 31, 101661. [Google Scholar] [CrossRef]
- Zeneli, M.; Nikolopoulos, A.; Karellas, S.; Nikolopoulos, N. Numerical methods for solid-liquid phase-change problems. In Ultra-High Temperature Thermal Energy Storage, Transfer and Conversion; Elsevier: Amsterdam, The Netherlands, 2020; pp. 165–199. [Google Scholar] [CrossRef]
- Gonçalves, J.C.; Costa, J.J.; Lopes, A.M.G. Analysis of the air infiltration through the doorway of a refrigerated room using different approaches. Appl. Therm. Eng. 2019, 159, 113927. [Google Scholar] [CrossRef]
- Chandran, R.; Hasanuzzaman, M.; Arıcı, M.; Kumar, L. Energy, economic and environmental impact analysis of phase change materials for cold chain transportation in Malaysia. J. Energy Storage 2022, 55, 105481. [Google Scholar] [CrossRef]




| Description | k [W/(mk)] | cp [kJ/(kgK)] | ρ [kg/m3] | x [mm] |
|---|---|---|---|---|
| Polyurethane | 0.025 | 1.5 | 45 | 50 |
| Galvanized Steel | 52 | 3.69 | 7860 | 1 |
| PCM | TM [°C] | ρ [kg/m3] | L [kJ/kg] | cp [kJ/(kgK)] | k [W/(mK)] |
|---|---|---|---|---|---|
| E-26 | −26 | 1250 | 265 | 3.67 | 0.58 |
| E-29 | −29 | 1420 | 250 | 3.69 | 0.64 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cirillo, L.; Greco, A.; Masselli, C. A Transient Analysis of Latent Thermal Energy Storage Using Phase Change Materials in a Refrigerated Truck. Energies 2024, 17, 2665. https://doi.org/10.3390/en17112665
Cirillo L, Greco A, Masselli C. A Transient Analysis of Latent Thermal Energy Storage Using Phase Change Materials in a Refrigerated Truck. Energies. 2024; 17(11):2665. https://doi.org/10.3390/en17112665
Chicago/Turabian StyleCirillo, Luca, Adriana Greco, and Claudia Masselli. 2024. "A Transient Analysis of Latent Thermal Energy Storage Using Phase Change Materials in a Refrigerated Truck" Energies 17, no. 11: 2665. https://doi.org/10.3390/en17112665
APA StyleCirillo, L., Greco, A., & Masselli, C. (2024). A Transient Analysis of Latent Thermal Energy Storage Using Phase Change Materials in a Refrigerated Truck. Energies, 17(11), 2665. https://doi.org/10.3390/en17112665








