Second-Order Sliding-Mode Control Applied to Microgrids: DC & AC Buck Converters Powering Constant Power Loads
Abstract
1. Introduction
- SOSMC offers a fast and stable response to disturbances for AC and DC buses.
- A single SOSMC can provide robustness to a microgrid that experiences disturbances in three stages (the Main Bus and A.C. and D.C. Buses), given that the appropriate manifold and parameters are selected.
- The selection of the constant value in the SOSMC manifold is of utmost importance, as an improper choice of this value may lead to chattering and/or the overshoot phenomena in the output signal.
2. Materials and Methods
2.1. General Methodology
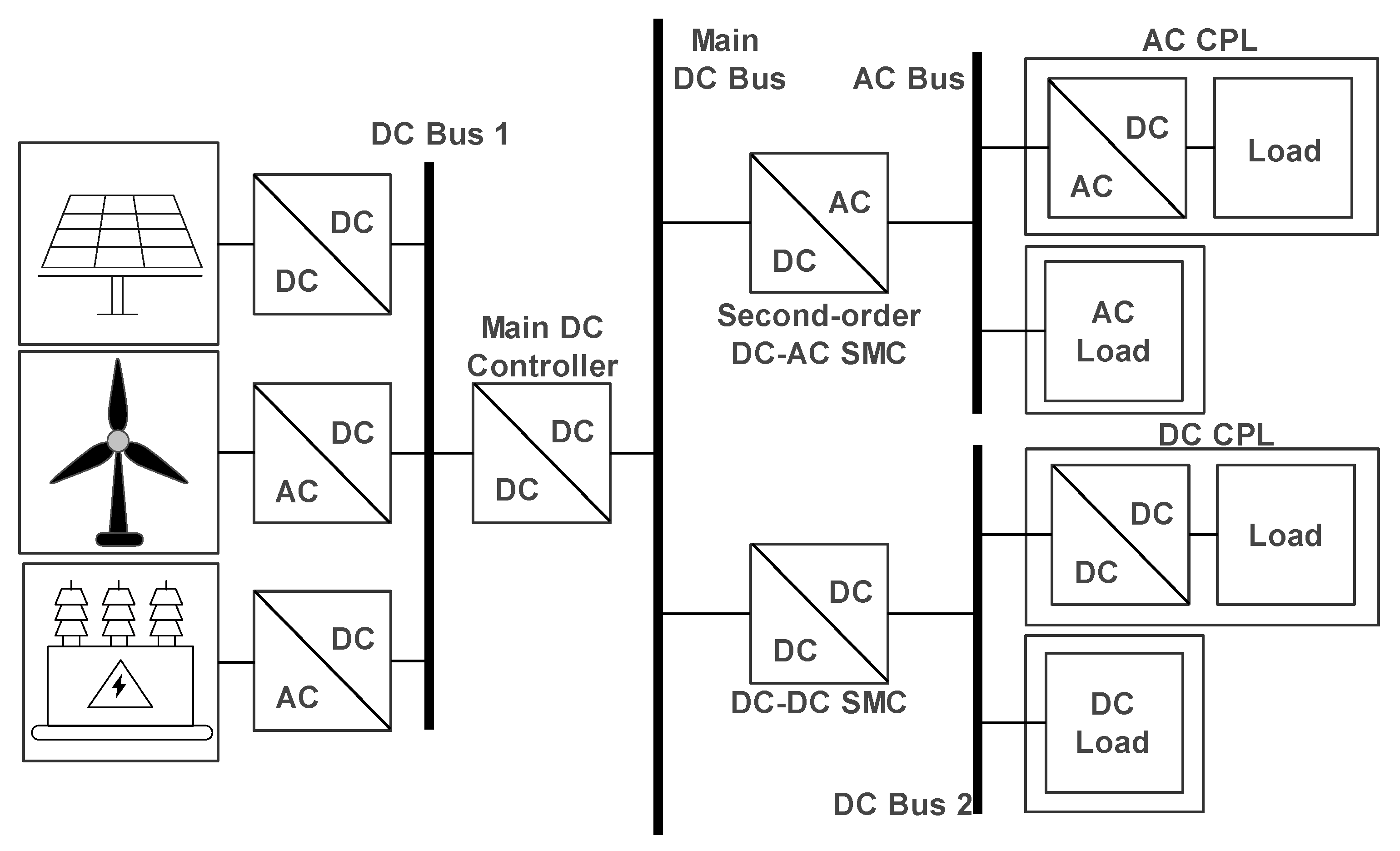
2.2. Architecture
2.3. Power Controller
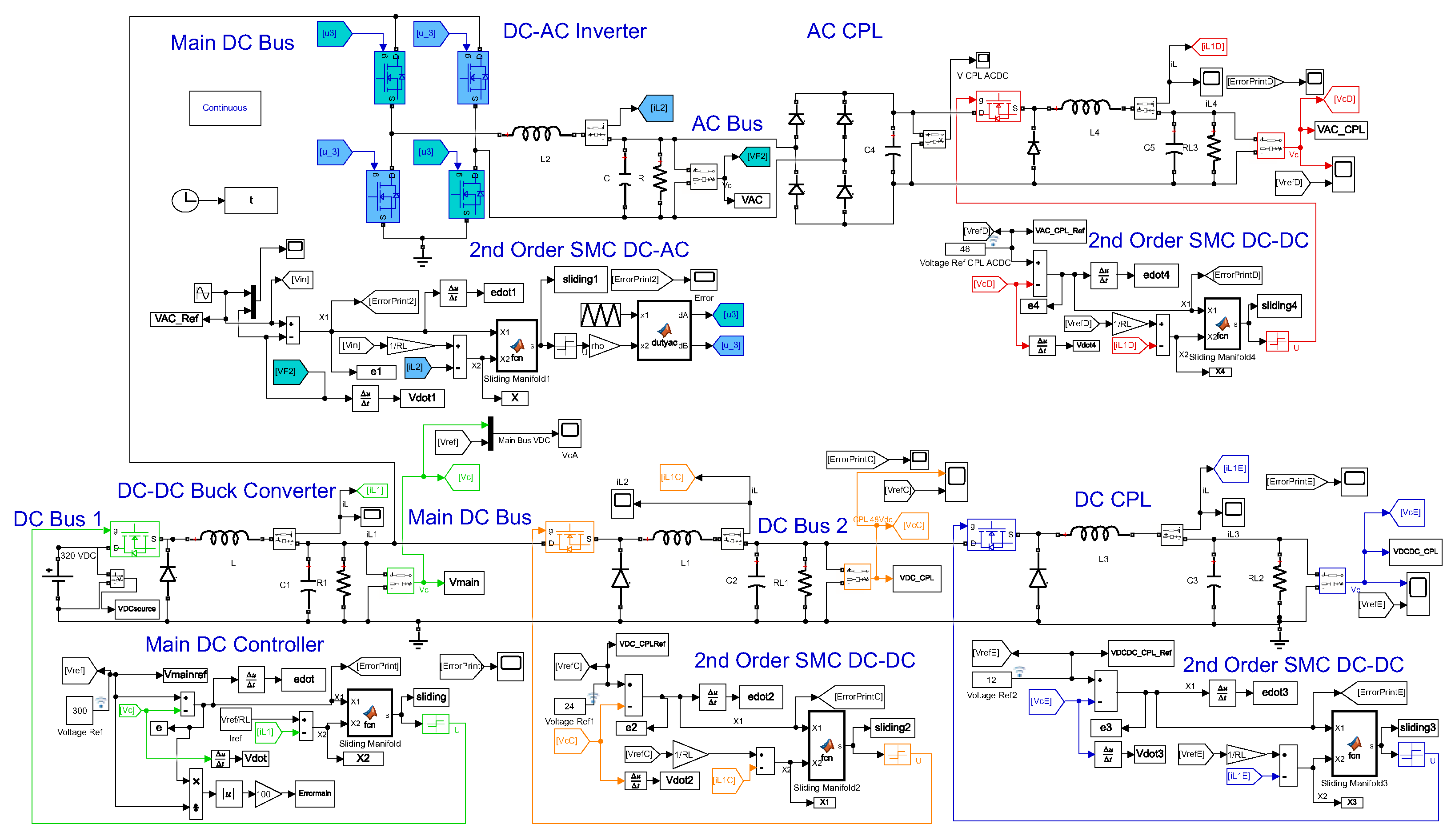
2.4. Mathematical Model of the Controller
2.5. Dynamics of Buck Converter (PC SMC)
2.6. Response of Controller
3. Results and Analysis
3.1. SOSMC in a DC–AC Buck Converter
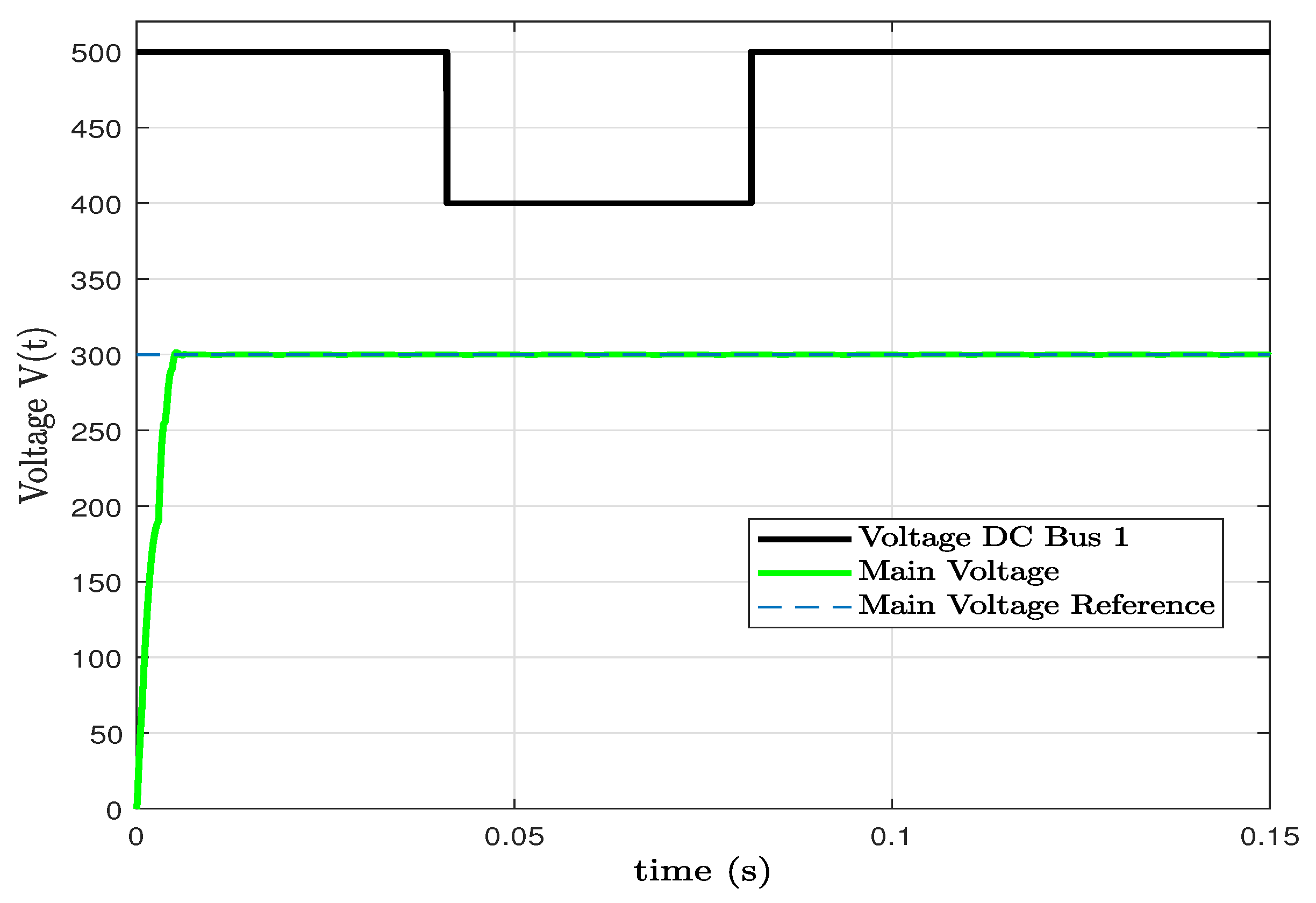
3.2. Variations in Voltage Reference of the Main DC Bus
3.3. Voltage Reference Changes in DC Bus 1
3.4. Change in the Voltage of DC Bus 1
3.5. Frequency Reference Variation in the AC Bus
4. Conclusions
- The SOSMC demonstrated robustness in the microgrid, with a stable response in terms of voltage and frequency for commercial applications. The average error during the simulations was less than 0.2% for disturbances of 28% in the main voltage feeder.
- The constant on the design surface, which must be >0, represents substantial consequences in the controller response. The correlation showed that a higher value of k produces a faster response. However, it will be overcome with a higher chattering effect at a determined value (greater than 25 for this particular application). Furthermore, a higher value of k will have a higher capacity to compensate for disturbances.
- SMC is not a novel control. However, the demonstrations presented in this work show evidence that, for modern applications, it is considered to have robust behavior. With a simple mathematical model, applied from a practical standpoint in an application of high relevance and importance, it was shown to provide the required stability for industrial and residential microgrid applications.
- In the case of alternating-current controllers, it was observed that the second-order controller presents outstanding stability, even for changes in the frequency of up to 60 Hz concerning its original set point. The SOSMC demonstrated stable performance against frequency variations: after a substantial variation of 60 Hz (from 60 to 120 Hz) in the input signal set point, the SOSMC maintained stability in all stages of the MG.
- In DC–DC converters, simple and SOSMC controllers showed a much higher response speed and less overshoot than the conventional PID-type control.
- There are still restrictions from the methodological standpoint, such as the dependence on the sampling frequency, since different results are obtained depending on the selection of this frequency value. It is suggested to continue the investigation into a completely real model with real microgrid loads that allows for the validation of the findings. In this way, the functionality of the SMC controllers would be empirically demonstrated.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ahmad, S.; Shafiullah, M.; Ahmed, C.B.; Alowaifeer, M. A Review of Microgrid Energy Management and Control Strategies. IEEE Access 2023, 11, 21729–21757. [Google Scholar] [CrossRef]
- Gursoy, M.; Zhuo, G.; Lozowski, A.G.; Wang, X. Photovoltaic Energy Conversion Systems with Sliding Mode Control. Energies 2021, 14, 6071. [Google Scholar] [CrossRef]
- Ding, S.; Zheng, W.X.; Sun, J.; Wang, J. Second-Order Sliding-Mode Controller Design and Its Implementation for Buck Converters. IEEE Trans. Ind. Inf. 2018, 14, 1990–2000. [Google Scholar] [CrossRef]
- RakhtAla, S.M.; Yasoubi, M.; HosseinNia, H. Design of second order sliding mode and sliding mode algorithms: A practical insight to DC-DC buck converter. IEEE/CAA J. Autom. Sin. 2017, 4, 483–497. [Google Scholar] [CrossRef]
- Cucuzzella, M.; Incremona, G.P.; Ferrara, A. Design of Robust Higher Order Sliding Mode Control for Microgrids. IEEE J. Emerg. Sel. Top. Circuits Syst. 2015, 5, 393–401. [Google Scholar] [CrossRef]
- Le Nhu Ngoc Thanh, H.; Hong, S.K. Quadcopter Robust Adaptive Second Order Sliding Mode Control Based on PID Sliding Surface. IEEE Access 2018, 6, 66850–66860. [Google Scholar] [CrossRef]
- Khan, M.R.; Haider, Z.M.; Malik, F.H.; Almasoudi, F.M.; Alatawi, K.S.S.; Bhutta, M.S. A Comprehensive Review of Microgrid Energy Management Strategies Considering Electric Vehicles, Energy Storage Systems, and AI Techniques. Processes 2024, 12, 270. [Google Scholar] [CrossRef]
- Cabana-Jiménez, K.; Candelo-Becerra, J.E.; Sousa Santos, V. Comprehensive Analysis of Microgrids Configurations and Topologies. Sustain. Sci. Pract. Policy 2022, 14, 1056. [Google Scholar] [CrossRef]
- Khodabakhsh, J.; Moschopoulos, G. Simplified Hybrid AC–DC Microgrid with a Novel Interlinking Converter. IEEE Trans. Ind. Appl. 2020, 56, 5023–5034. [Google Scholar] [CrossRef]
- Gong, X.; Dong, F.; Mohamed, M.A.; Abdalla, O.M.; Ali, Z.M. A Secured Energy Management Architecture for Smart Hybrid Microgrids Considering PEM-Fuel Cell and Electric Vehicles. IEEE Access 2020, 8, 47807–47823. [Google Scholar] [CrossRef]
- Espina, E.; Cárdenas-Dobson, R.; Simpson-Porco, J.W.; Sáez, D.; Kazerani, M. A Consensus-Based Secondary Control Strategy for Hybrid AC/DC Microgrids with Experimental Validation. IEEE Trans. Power Electron. 2021, 36, 5971–5984. [Google Scholar] [CrossRef]
- Sahoo, S.; Mishra, S. A Distributed Finite-Time Secondary Average Voltage Regulation and Current Sharing Controller for DC Microgrids. IEEE Trans. Smart Grid 2019, 10, 282–292. [Google Scholar] [CrossRef]
- Yan, H.; Zhou, X.; Zhang, H.; Yang, F.; Wu, Z.G. A Novel Sliding Mode Estimation for Microgrid Control with Communication Time Delays. IEEE Trans. Smart Grid 2019, 10, 1509–1520. [Google Scholar] [CrossRef]
- Shi, S.; Wang, X.; Li, S.; Yang, J. Prescribed-Time Second-Order Sliding Mode Controller Design Subject to Mismatched Term. IEEE Trans. Circuits Syst. Express Briefs 2023, 70, 1976–1980. [Google Scholar] [CrossRef]
- Guo, J. The Load Frequency Control by Adaptive High Order Sliding Mode Control Strategy. IEEE Access 2022, 10, 25392–25399. [Google Scholar] [CrossRef]
- Jiang, H.; Wei, M.; Zhao, Y.; Han, J. Design of Decentralized Adaptive Sliding Mode Controller for the Islanded AC Microgrid with Ring Topology. Front. Energy Res. 2021, 9, 732997. [Google Scholar] [CrossRef]
- Huangfu, Y.; Ma, R.; Miraoui, A. Avoidance High-Frequency Chattering Second-Order Sliding Mode Controller Design: Buck Converter in Wind Power System. Int. J. Antennas Propag. 2012, 2012, 176830. [Google Scholar] [CrossRef]
- Guerrero, J.M.; Chandorkar, M.; Lee, T.L.; Loh, P.C. Advanced Control Architectures for Intelligent Microgrids—Part I: Decentralized and Hierarchical Control. IEEE Trans. Ind. Electron. 2013, 60, 1254–1262. [Google Scholar] [CrossRef]
- Guerrero, J.M.; Loh, P.C.; Lee, T.L.; Chandorkar, M. Advanced Control Architectures for Intelligent Microgrids—Part II: Power Quality, Energy Storage, and AC/DC Microgrids. IEEE Trans. Ind. Electron. 2013, 60, 1263–1270. [Google Scholar] [CrossRef]
- Katiraei, F.; Iravani, R.; Hatziargyriou, N.; Dimeas, A. Microgrids management. IEEE Power Energ. Mag. 2008, 6, 54–65. [Google Scholar] [CrossRef]
- Rakhtala, S.M.; Casavola, A. Real-Time Voltage Control Based on a Cascaded Super Twisting Algorithm Structure for DC–DC Converters. IEEE Trans. Ind. Electron. 2022, 69, 633–641. [Google Scholar] [CrossRef]
- Du, W.; Zhang, J.; Zhang, Y.; Qian, Z. Stability Criterion for Cascaded System with Constant Power Load. IEEE Trans. Power Electron. 2013, 28, 1843–1851. [Google Scholar] [CrossRef]
- Utkin, V. Variable structure systems with sliding modes. IEEE Trans. Autom. Control. 1977, 22, 212–222. [Google Scholar] [CrossRef]
- Songbin, L.; Zhiyuan, F.; Yang, G.; Hai, K.L.; Peng, W. Second-order sliding-mode control of synchronous buck converter based on sub-optimal algorithm. In Proceedings of the 2017 Asian Conference on Energy, Power and Transportation Electrification (ACEPT), Singapore, 24–26 October 2017; pp. 1–6. [Google Scholar]
- Kaplan, O.; Bodur, F. Second-order sliding mode controller design of buck converter with constant power load. Int. J. Control 2023, 96, 1210–1226. [Google Scholar] [CrossRef]
- Cucuzzella, M.; Lazzari, R.; Trip, S.; Sandroni, C.; Ferrara, A. Robust voltage regulation of boost converters in DC microgrids. In Proceedings of the 2018 European Control Conference (ECC), Limassol, Cyprus, 12–15 June 2018; pp. 2350–2355. [Google Scholar]
- Incremona, G.P.; Cucuzzella, M.; Ferrara, A. Adaptive suboptimal second-order sliding mode control for microgrids. Int. J. Control 2016, 89, 1849–1867. [Google Scholar] [CrossRef]
- Han, Y.; Ma, R.; Cui, J. Adaptive Higher-Order Sliding Mode Control for Islanding and Grid-Connected Operation of a Microgrid. Energies 2018, 11, 1459. [Google Scholar] [CrossRef]
- Baghaee, H.R.; Mirsalim, M.; Gharehpetian, G.B.; Talebi, H.A. Decentralized Sliding Mode Control of WG/PV/FC Microgrids under Unbalanced and Nonlinear Load Conditions for On- and Off-Grid Modes. IEEE Syst. J. 2018, 12, 3108–3119. [Google Scholar] [CrossRef]
- Yoo, H.J.; Nguyen, T.T.; Kim, H.M. Consensus-Based Distributed Coordination Control of Hybrid AC/DC Microgrids. IEEE Trans. Sustain. Energy 2020, 11, 629–639. [Google Scholar] [CrossRef]
- Shen, X.; Liu, J.; Alcaide, A.M.; Yin, Y.; Leon, J.I.; Vazquez, S.; Wu, L.; Franquelo, L.G. Adaptive Second-Order Sliding Mode Control for Grid-Connected NPC Converters with Enhanced Disturbance Rejection. IEEE Trans. Power Electron. 2022, 37, 206–220. [Google Scholar] [CrossRef]
- Lai, J.; Lu, X.; Dong, Z.; Cheng, S. Resilient Distributed Multiagent Control for AC Microgrid Networks Subject to Disturbances. IEEE Trans. Syst. Man Cybern. Syst. 2022, 52, 43–53. [Google Scholar] [CrossRef]
- Xu, Q.; Vafamand, N.; Chen, L.; Dragičević, T.; Xie, L.; Blaabjerg, F. Review on Advanced Control Technologies for Bidirectional DC/DC Converters in DC Microgrids. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 1205–1221. [Google Scholar] [CrossRef]
- Gheisarnejad, M.; Farsizadeh, H.; Khooban, M.H. A Novel Nonlinear Deep Reinforcement Learning Controller for DC–DC Power Buck Converters. IEEE Trans. Ind. Electron. 2021, 68, 6849–6858. [Google Scholar] [CrossRef]
- Hassan, M.A.; Su, C.L.; Pou, J.; Sulligoi, G.; Almakhles, D.; Bosich, D.; Guerrero, J.M. DC Shipboard Microgrids with Constant Power Loads: A Review of Advanced Nonlinear Control Strategies and Stabilization Techniques. IEEE Trans. Smart Grid 2022, 13, 3422–3438. [Google Scholar] [CrossRef]
- Wu, J.; Yang, L.; Lu, Z.; Wang, Q. Robust adaptive composite control of DC–DC boost converter with constant power load in DC microgrid. Energy Rep. 2023, 9, 855–865. [Google Scholar] [CrossRef]
- Yi, S.; Zhai, J. Adaptive second-order fast nonsingular terminal sliding mode control for robotic manipulators. ISA Trans. 2019, 90, 41–51. [Google Scholar] [CrossRef] [PubMed]
- Chen, S.Y.; Chiang, H.H.; Liu, T.S.; Chang, C.H. Precision Motion Control of Permanent Magnet Linear Synchronous Motors Using Adaptive Fuzzy Fractional-Order Sliding-Mode Control. IEEE/ASME Trans. Mechatron. 2019, 24, 741–752. [Google Scholar] [CrossRef]
- Khooban, M.H.; Gheisarnejad, M.; Farsizadeh, H.; Masoudian, A.; Boudjadar, J. A New Intelligent Hybrid Control Approach for DC–DC Converters in Zero-Emission Ferry Ships. IEEE Trans. Power Electron. 2020, 35, 5832–5841. [Google Scholar] [CrossRef]
- Monsalve-Rueda, M.; Candelo-Becerra, J.E.; Hoyos, F.E. Dynamic Behavior of a Sliding-Mode Control Based on a Washout Filter with Constant Impedance and Nonlinear Constant Power Loads. Appl. Sci. 2019, 9, 4548. [Google Scholar] [CrossRef]
- Shtessel, Y.; Edwards, C.; Fridman, L.; Levant, A. Sliding Mode Control and Observation; Springer: New York, NY, USA, 2014. [Google Scholar]
- Levaggi, L. Sliding modes in Banach spaces. Differ. Integral Equ. 2002, 15, 167–189. [Google Scholar] [CrossRef]
- Triggiani, R. On the stabilizability problem in Banach space. J. Math. Anal. Appl. 1975, 52, 383–403. [Google Scholar] [CrossRef]

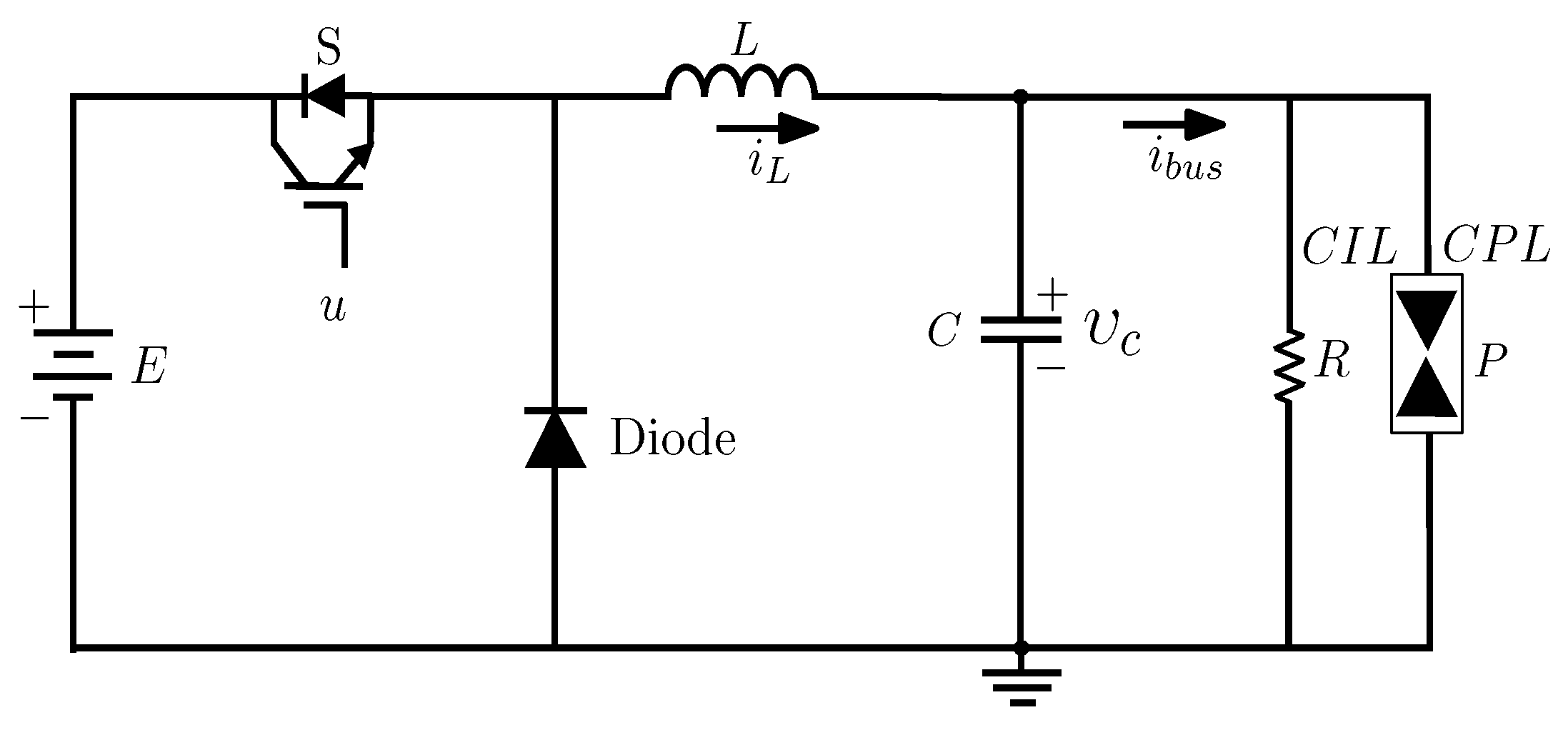



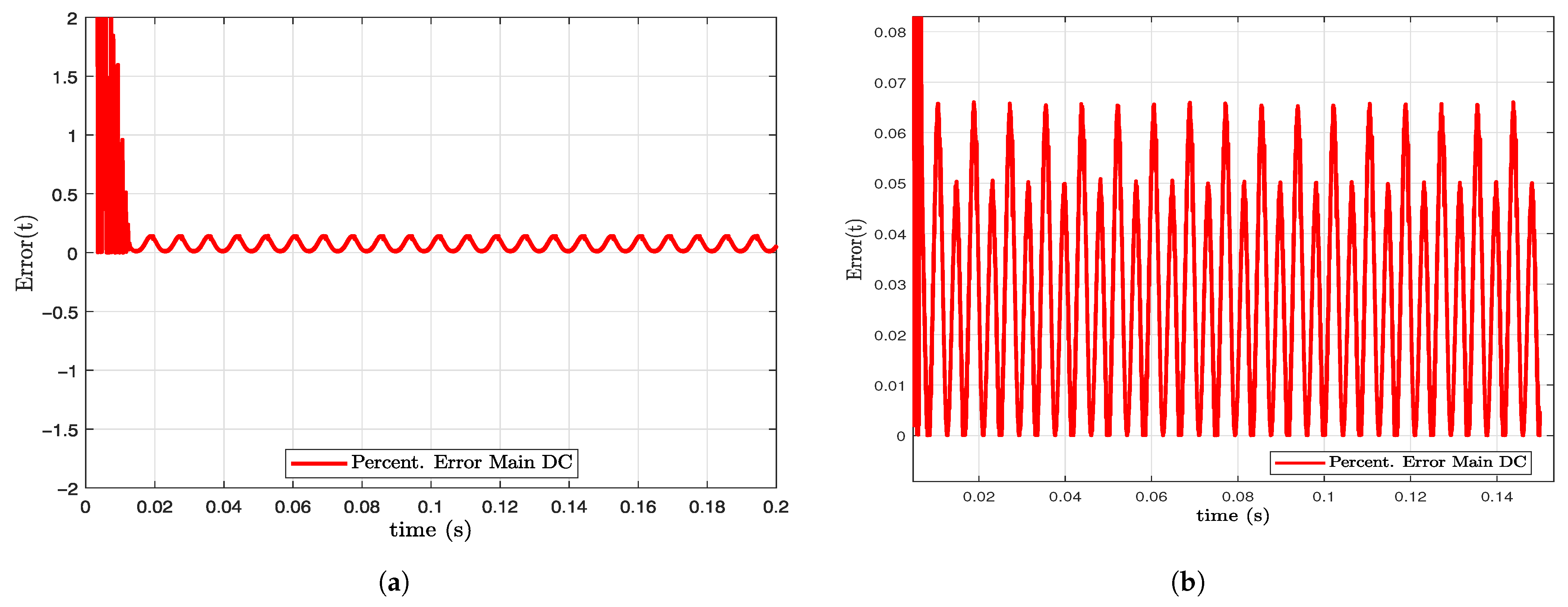





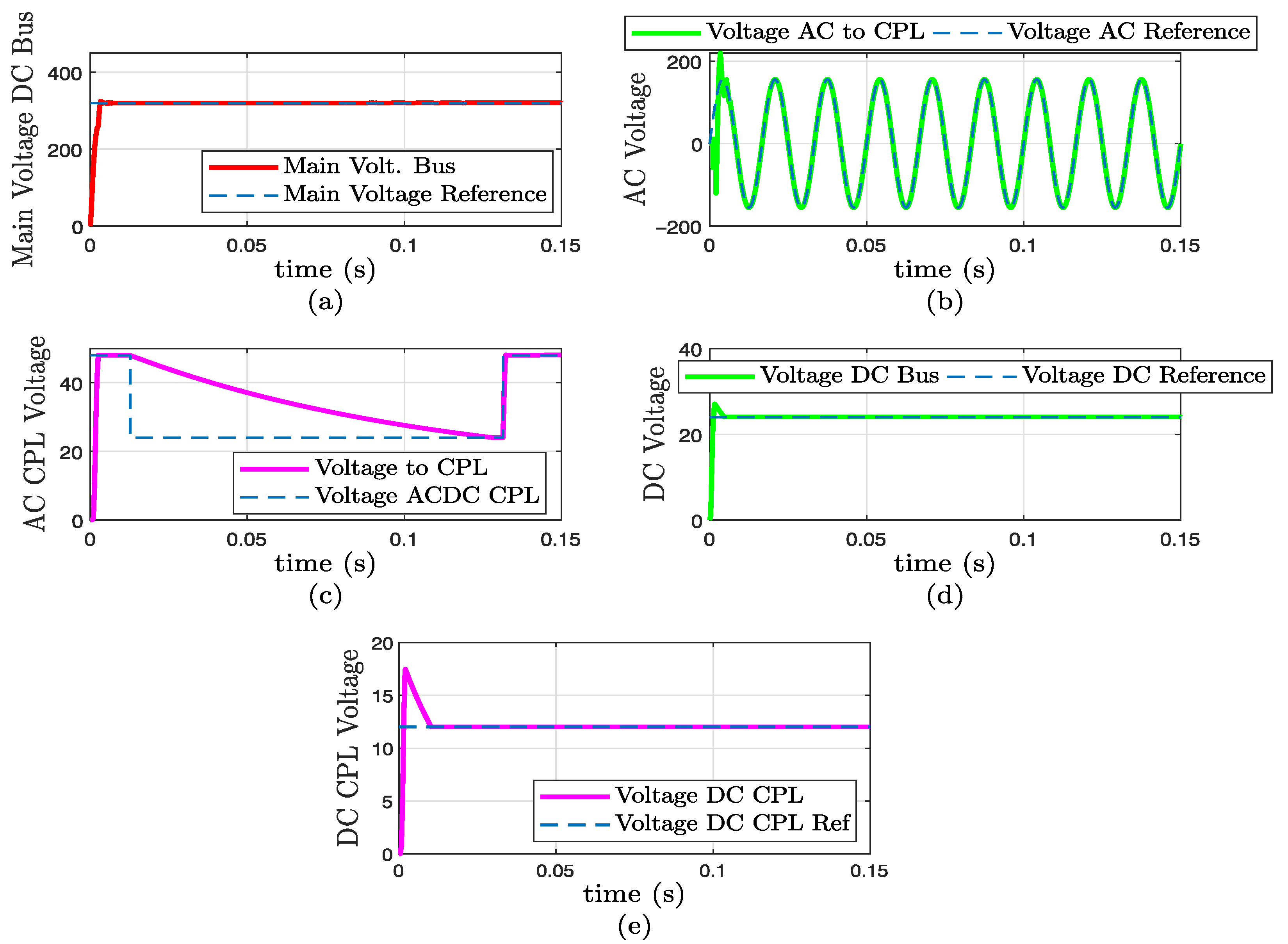

| Item | Element | Value |
|---|---|---|
| 1 | Load Resistor | 10 |
| 2 | Inductance | 2.47 mH |
| 3 | Capacitor | 46 F |
| 4 | Source | 320 V |
| 5 | Vreference | 120 VRMS |
| 6 | Frequency | 60 Hz |
| 7 | Disturbance Resistor | 2 |
| Time (s) | Main DC Bus | AC Bus | CPL |
|---|---|---|---|
| 0–0.02 | Initial response, reaching a set point of 300 Vdc. Settling time: 0.14 ms. Overshoot: 1.33%. | AC signal stable after 7 ms | AC CPL reaches the reference after 7 ms, and DC CPL reaches the reference after 7 ms |
| 0.02–0.04 | Reaching a set point of 400 Vdc. Settling time: 0.12 ms. Overshoot: 5.53%. | AC signal stable | Both CPLs keep stable |
| 0.04–0.06 | Reaching a set point of 450 Vdc. Settling time: 6 ms. Overshoot: 5.53%. | AC signal stable | Both CPLs keep stable |
| 0.06–0.08 | Down to a set point of 400 Vdc. Settling time: 6 ms. Overshoot: 5.53%. | AC signal stable | Both CPLs keep stable |
| 0.08–0.1 | Down to a set point of 300 Vdc. Settling time: 6 ms. Overshoot: 5.53%. | AC signal stable | Both CPLs keep stable |
| 0.1–0.14 | Down to a set point of 100 Vdc. Settling time: 6 ms. Overshoot: 5.53%. | AC signal unstable after 0.12 s | Both CPLs keep stable |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Monsalve-Rueda, M.; Candelo-Becerra, J.E.; Hoyos, F.E. Second-Order Sliding-Mode Control Applied to Microgrids: DC & AC Buck Converters Powering Constant Power Loads. Energies 2024, 17, 2701. https://doi.org/10.3390/en17112701
Monsalve-Rueda M, Candelo-Becerra JE, Hoyos FE. Second-Order Sliding-Mode Control Applied to Microgrids: DC & AC Buck Converters Powering Constant Power Loads. Energies. 2024; 17(11):2701. https://doi.org/10.3390/en17112701
Chicago/Turabian StyleMonsalve-Rueda, Miguel, John E. Candelo-Becerra, and Fredy E. Hoyos. 2024. "Second-Order Sliding-Mode Control Applied to Microgrids: DC & AC Buck Converters Powering Constant Power Loads" Energies 17, no. 11: 2701. https://doi.org/10.3390/en17112701
APA StyleMonsalve-Rueda, M., Candelo-Becerra, J. E., & Hoyos, F. E. (2024). Second-Order Sliding-Mode Control Applied to Microgrids: DC & AC Buck Converters Powering Constant Power Loads. Energies, 17(11), 2701. https://doi.org/10.3390/en17112701








